Solvent Effects on Catechol Crystal Habits and Aspect Ratios: A Combination of Experiments and Molecular Dynamics Simulation Study
Abstract
1. Introduction
2. Calculation Methodology
2.1. Theory
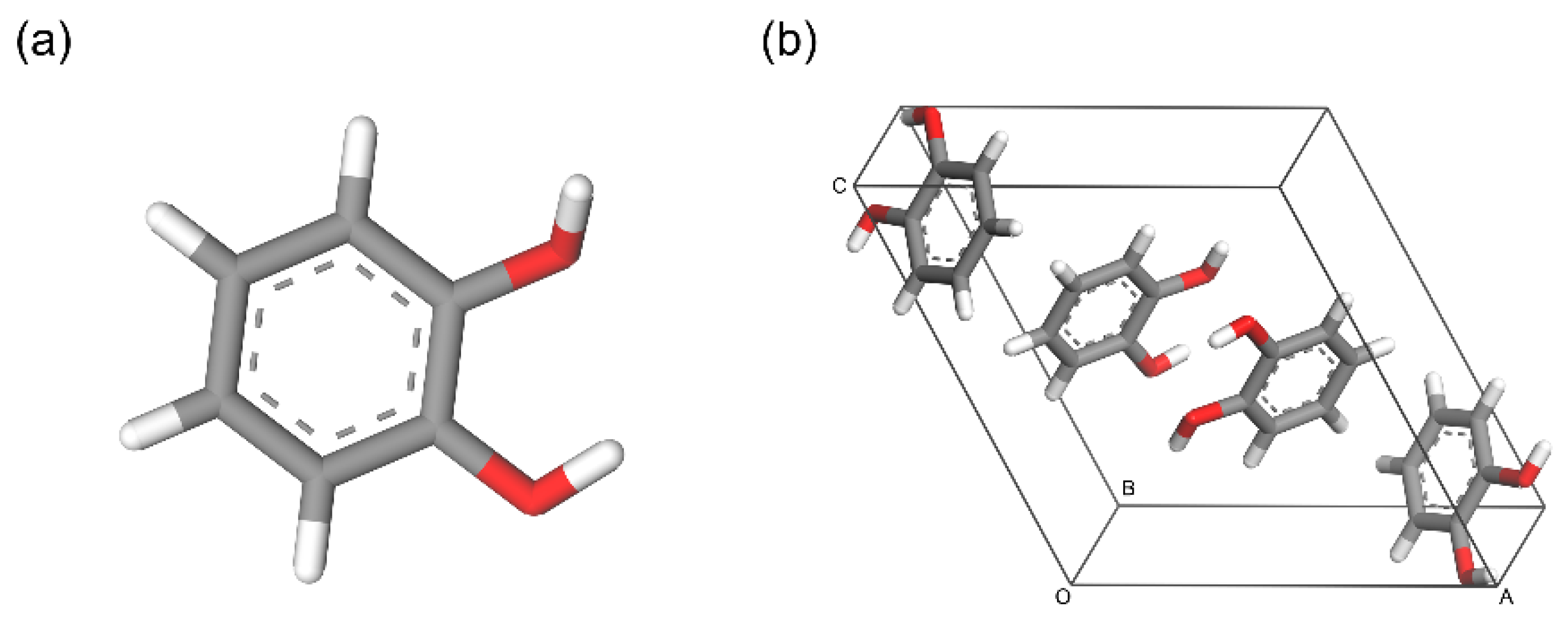
2.2. Simulation Details
3. Experiment and Characterization
3.1. Materials
3.2. Cooling Crystallization Experiments
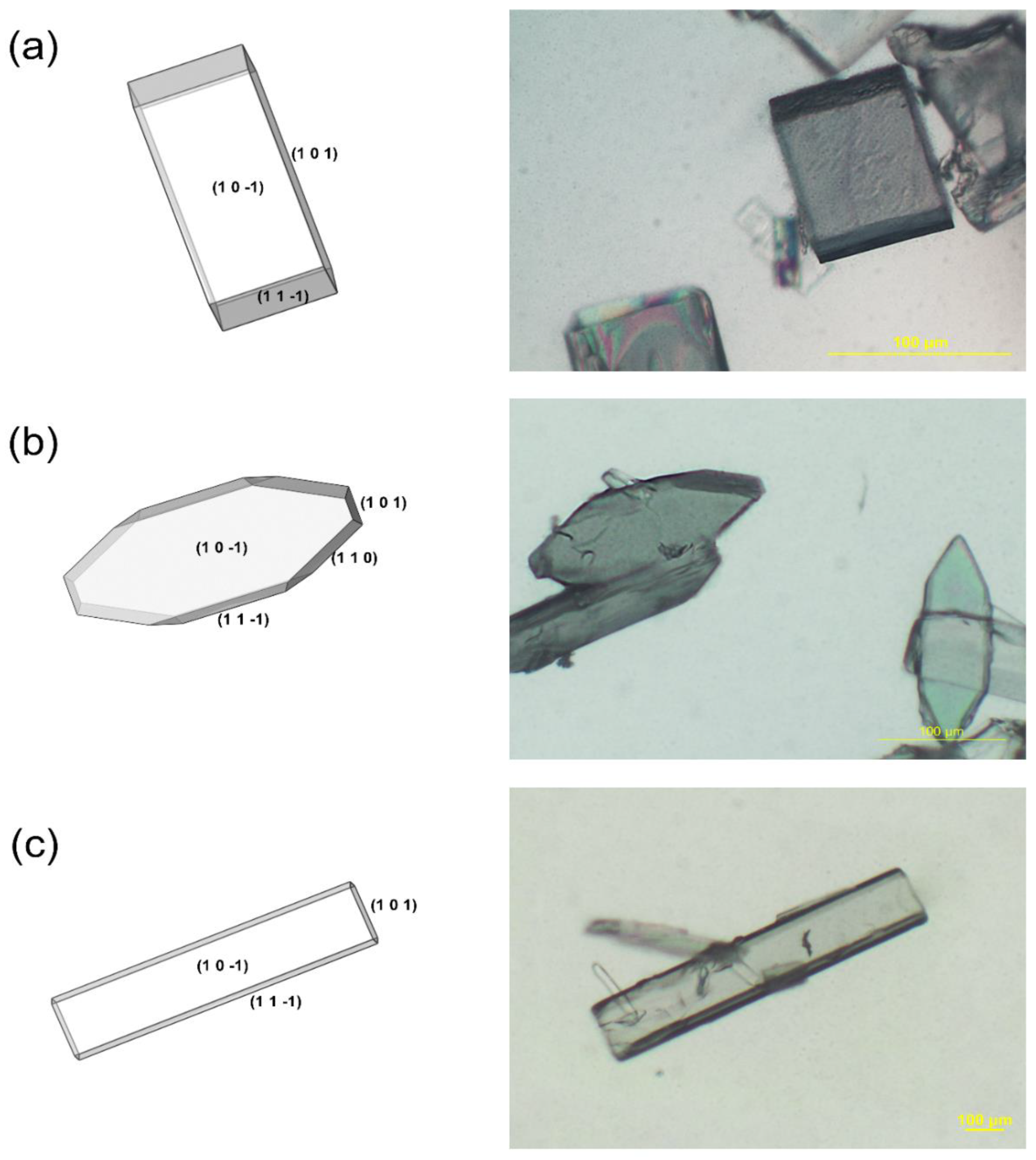
3.3. Crystal Characterization
4. Results and Discussion
4.1. Polymorph Identification
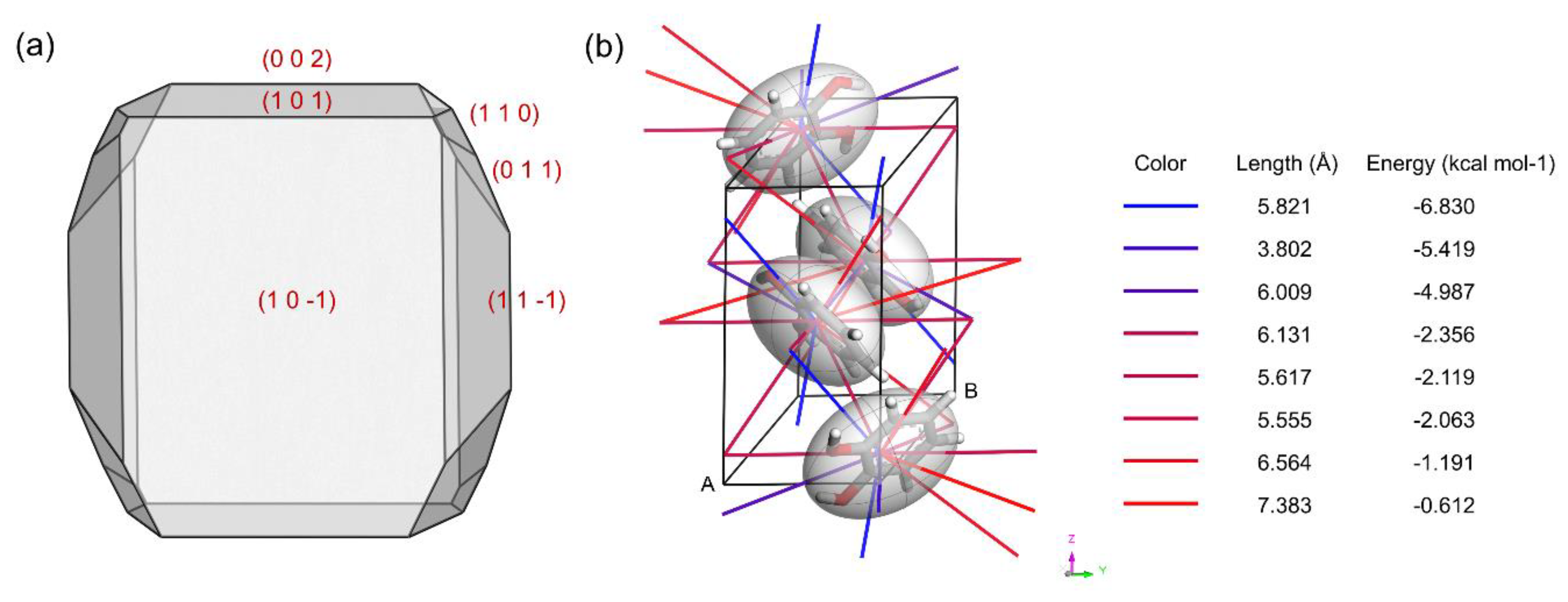
4.2. Prediction of Catechol Crystal Morphology in Vacuum
4.3. Modified Results of Catechol Crystal Morphology in Solvent Systems
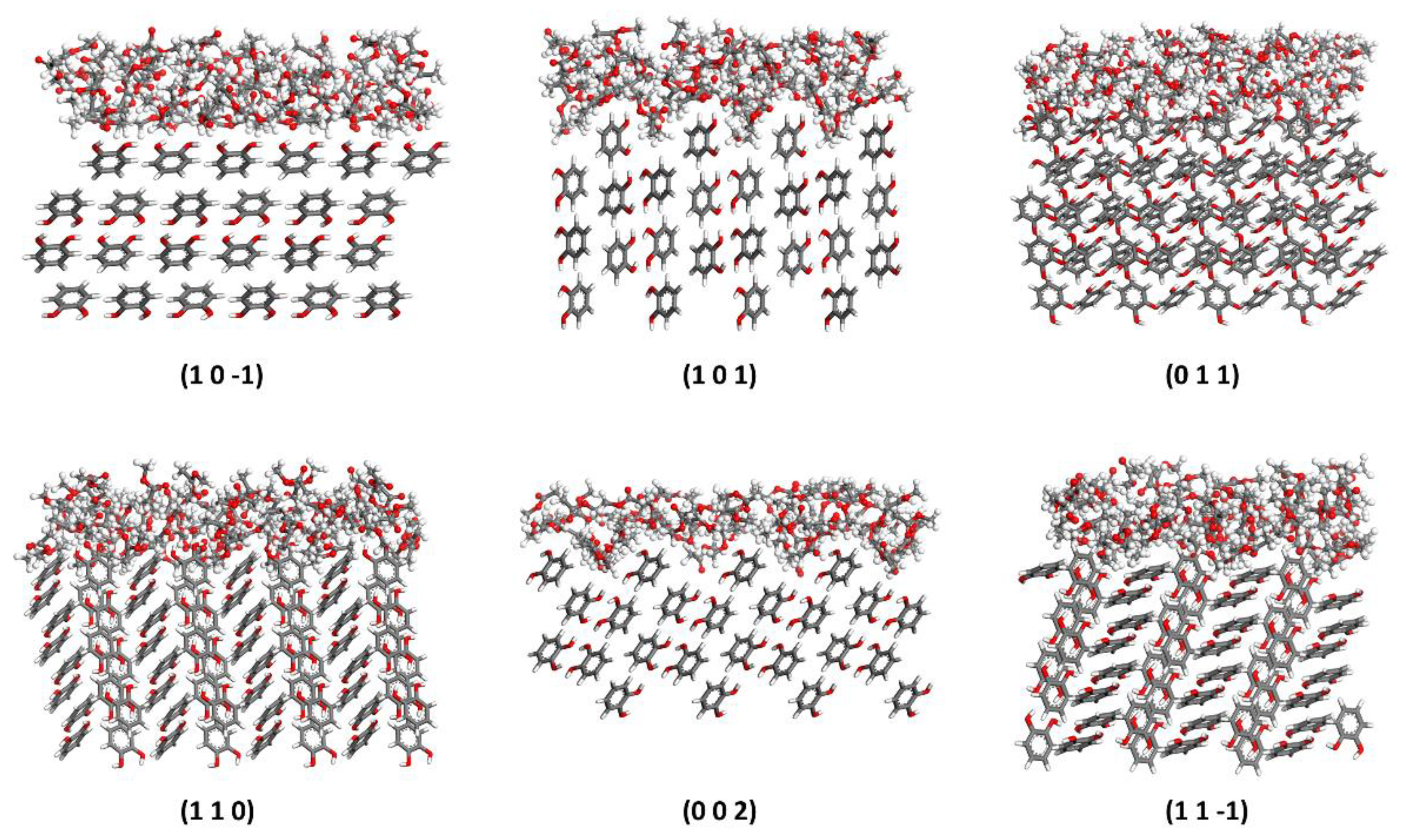
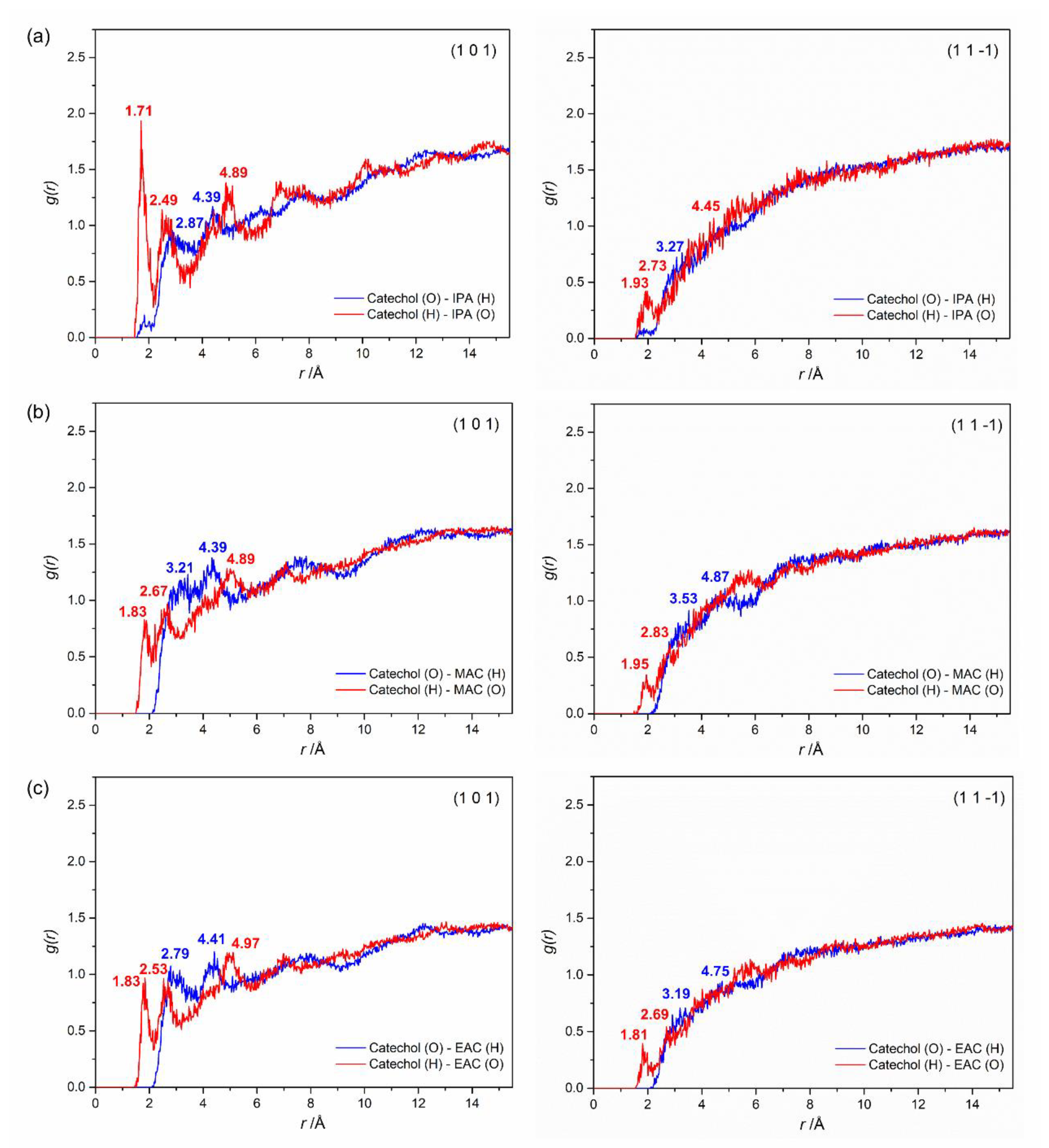
4.4. Solvent–Crystal Interactions on the Interface
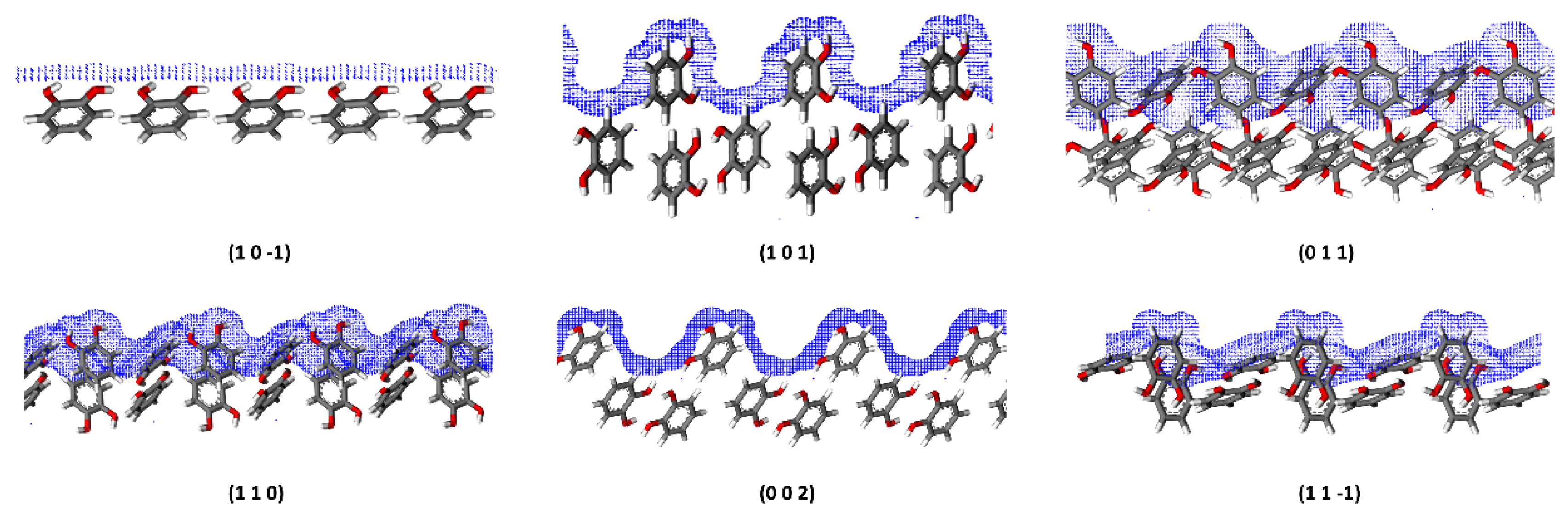
4.4.1. Molecular Alignment on Crystal Surfaces
4.4.2. Roughness of Surfaces
4.4.3. Diffusion Coefficient
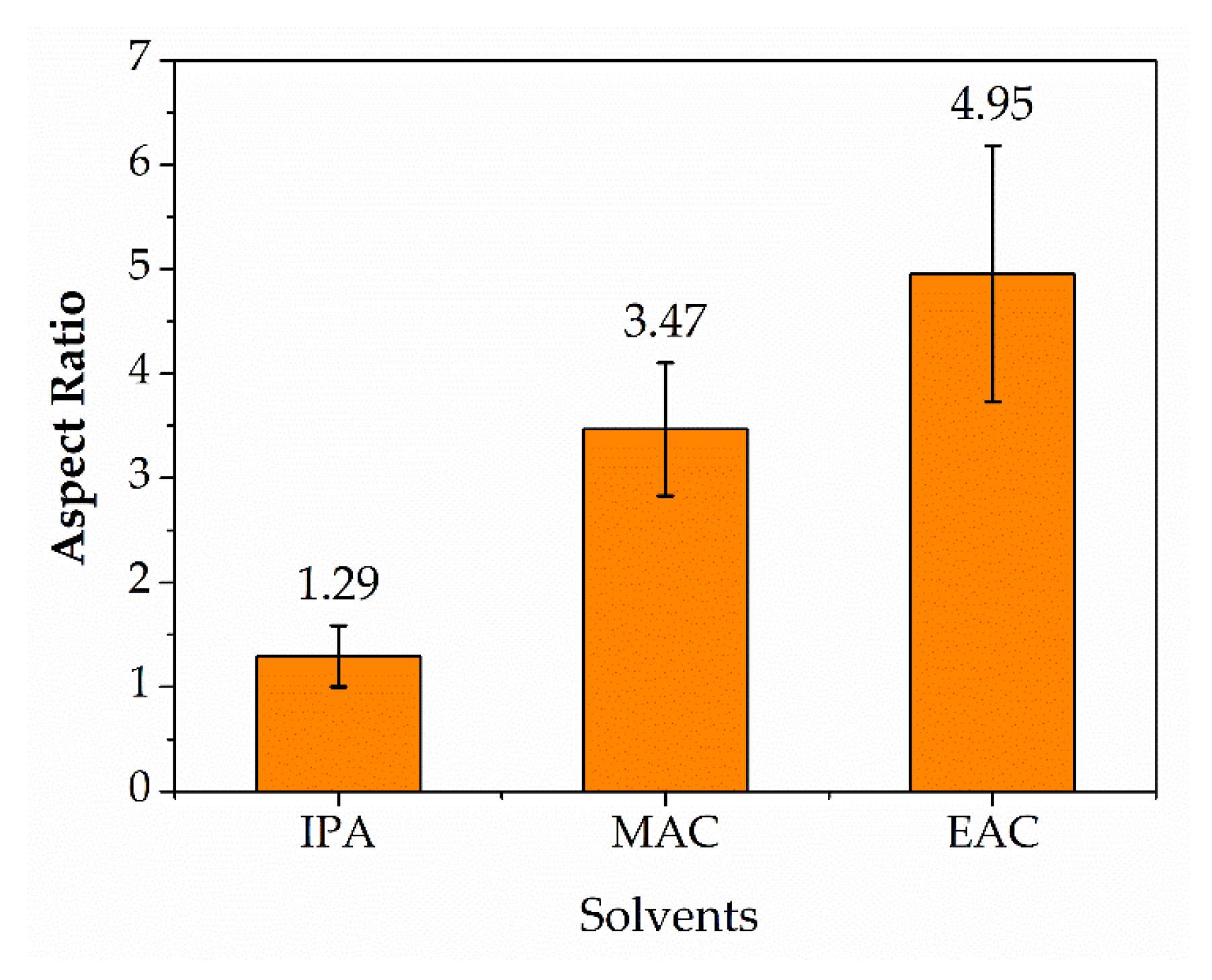
4.4.4. Solvent Effects on Crystal Aspect Ratios
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Beckmann, W. Crystallization: Basic Concepts and Industrial Applications; Wiley-VCH: Weinheim, Germany, 2013. [Google Scholar]
- MacLeod, C.S.; Muller, F.L. On the Fracture of Pharmaceutical Needle-Shaped Crystals during Pressure Filtration: Case Studies and Mechanistic Understanding. Org. Process Res. Dev. 2012, 16, 425–434. [Google Scholar] [CrossRef]
- Kim, S.; Lotz, B.; Lindrud, M.; Girard, K.; Moore, T.; Nagarajan, K.; Alvarez, M.; Lee, T.; Nikfar, F.; Davidovich, M.; et al. Control of the particle properties of a drug substance by crystallization engineering and the effect on drug product formulation. Org. Process Res. Dev. 2005, 9, 894–901. [Google Scholar] [CrossRef]
- Rasenack, N.; Müller, B.W. Ibuprofen crystals with optimized properties. Int. J. Pharm. 2002, 245, 9–24. [Google Scholar] [CrossRef]
- Širola, I.; Lukšić, J.; Šimunić, B.; Kujundžić, N. Effect of crystal size and shape on bulk density of pharmaceutical powders. J. Cryst. Growth 1997, 181, 403–409. [Google Scholar] [CrossRef]
- Stoica, C.; Verwer, P.; Meekes, H.; van Hoof, P.J.C.M.; Kaspersen, F.M.; Vlieg, E. Understanding the effect of a solvent on the crystal habit. Cryst. Growth Des. 2004, 4, 765–768. [Google Scholar] [CrossRef]
- Cashell, C.; Corcoran, D.; Hodnett, B.K. Effect of amino acid additives on the crystallization of L-glutamic acid. Cryst. Growth Des. 2005, 5, 593–597. [Google Scholar] [CrossRef]
- Yang, D.; Hrymak, A.N. Crystal Morphology of Hydrogenated Castor Oil in the Crystallization of Oil-in-Water Emulsions: Part I. Effect of Temperature. Ind. Eng. Chem. Res. 2011, 50, 11585–11593. [Google Scholar] [CrossRef]
- Boerrigter, S.X.M.; Cuppen, H.M.; Ristic, R.I.; Sherwood, J.N.; Bennema, P.; Meekes, H. Explanation for the supersaturation-dependent morphology of monoclinic paracetamol. Cryst. Growth Des. 2002, 2, 357–361. [Google Scholar] [CrossRef]
- Di Renzo, F. Zeolites as tailor-made catalysts: Control of the crystal size. Catal. Today 1998, 41, 37–40. [Google Scholar] [CrossRef]
- Blagden, N.; Davey, R.J.; Lieberman, H.F.; Williams, L.; Payne, R.; Roberts, R.; Rowe, R.; Docherty, R. Crystal chemistry and solvent effects in polymorphic systems—Sulfathiazole. J. Chem. Soc. Faraday T 1998, 94, 1035–1044. [Google Scholar] [CrossRef]
- Urbelis, J.H.; Swift, J.A. Solvent Effects on the Growth Morphology and Phase Purity of CL-20. Cryst. Growth Des. 2014, 14, 1642–1649. [Google Scholar] [CrossRef]
- Liu, J.; Chang, Z.; He, Y.; Yang, M.; Dang, J. Solvent effects on the crystallization of avermectin B1a. J. Cryst. Growth 2007, 307, 131–136. [Google Scholar] [CrossRef]
- Khoshkhoo, S.; Anwar, J. Study of the effect of solvent on the morphology of crystals using molecular simulation: Application to α-resorcinol and N-n-octyl-D-gluconamide. J. Chem. Soc. Faraday Trans. 1996, 92, 1023–1025. [Google Scholar] [CrossRef]
- de Graaf, J.; Filion, L.; Marechal, M.; van Roij, R.; Dijkstra, M. Crystal-structure prediction via the floppy-box Monte Carlo algorithm: Method and application to hard (non)convex particles. J. Chem. Phys. 2012, 137, 214101. [Google Scholar] [CrossRef]
- Zhang, W.-B.; Chen, C.; Zhang, S.-Y. Equilibrium Crystal Shape of Ni from First Principles. J. Phys. Chem. C 2013, 117, 21274–21280. [Google Scholar] [CrossRef]
- Dzade, N.Y.; Roldan, A.; de Leeuw, N.H. Surface and shape modification of mackinawite (FeS) nanocrystals by cysteine adsorption: A first-principles DFT-D2 study. Phys. Chem. Chem. Phys. 2016, 18, 32007–32020. [Google Scholar] [CrossRef]
- Lynch, A.; Verma, V.; Zeglinski, J.; Bannigan, P.; Rasmuson, A. Face indexing and shape analysis of salicylamide crystals grown in different solvents. CrystEngComm 2019, 21, 2648–2659. [Google Scholar] [CrossRef]
- Shi, W.; Chu, Y.; Xia, M.; Lei, W.; Wang, F. Crystal morphology prediction of 1,3,3-trinitroazetidine in ethanol solvent by molecular dynamics simulation. J. Mol. Graph. Model. 2016, 64, 94–100. [Google Scholar] [CrossRef]
- Song, L.; Chen, L.; Wang, J.; Chen, F.; Lan, G. Prediction of crystal morphology of 3,4-Dinitro-1H-pyrazole (DNP) in different solvents. J. Mol. Graph. Model. 2017, 75, 62–70. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, X.; Du, W.; Huang, Y.-H.; Guo, M.-X.; Li, Y.; Zhang, Z.-X.; Hou, B.-H.; Yin, Q.-X. Effects of solvent and supersaturation on crystal morphology of cefaclor dihydrate: A combined experimental and computer simulation study. CrystEngComm 2016, 18, 9085–9094. [Google Scholar] [CrossRef]
- Zhang, M.; Liang, Z.; Wu, F.; Chen, J.-F.; Xue, C.; Zhao, H. Crystal engineering of ibuprofen compounds: From molecule to crystal structure to morphology prediction by computational simulation and experimental study. J. Cryst. Growth 2017, 467, 47–53. [Google Scholar] [CrossRef]
- Poornachary, S.K.; Chia, V.D.; Yani, Y.; Han, G.; Chow, P.S.; Tan, R.B.H. Anisotropic Crystal Growth Inhibition by Polymeric Additives: Impact on Modulation of Naproxen Crystal Shape and Size. Cryst. Growth Des. 2017, 17, 4844–4854. [Google Scholar] [CrossRef]
- Fiege, H.; Voges, H.W.; Hamamoto, T.; Umemura, S.; Iwata, T.; Miki, H.; Fujita, Y.; Buysch, H.J.; Garbe, D.; Paulus, W. Phenol Derivatives. In Ullmann’s Encyclopedia of Industrial Chemistry; Wiley-VCH: Weinheim, Germany, 2000; pp. 552–555. [Google Scholar]
- Krab-Hüsken, L. Production of Catechols: Microbiology and Technology; Wageningen University: Wageningen, The Netherlands, 2002. [Google Scholar]
- Mabrouk, A.; Erdocia, X.; Alriols, M.G.; Labidi, J. Economic analysis of a biorefinery process for catechol production from lignin. J. Clean. Prod. 2018, 198, 133–142. [Google Scholar] [CrossRef]
- Docherty, R.; Clydesdale, G.; Roberts, K.J.; Bennema, P. Application of Bravais-Friedel-Donnay-Harker, attachment energy and Ising models to predicting and understanding the morphology of molecular crystals. J. Phys. D: Appl. Phys. 1991, 24, 89–99. [Google Scholar] [CrossRef]
- Hartman, P.; Bennema, P. The attachment energy as a habit controlling factor: I. Theoretical considerations. J. Cryst. Growth 1980, 49, 145–156. [Google Scholar] [CrossRef]
- Hartman, P. The attachment energy as a habit controlling factor II. Application to anthracene, tin tetraiodide and orthorhombic sulphur. J. Cryst. Growth 1980, 49, 157–165. [Google Scholar] [CrossRef]
- Hartman, P. The attachment energy as a habit controlling factor: III. Application to corundum. J. Cryst. Growth 1980, 49, 166–170. [Google Scholar] [CrossRef]
- Hartman, P.; Perdok, W.G. On the relations between structure and morphology of crystals. I. Acta Crystallogr. 1955, 8, 49–52. [Google Scholar] [CrossRef]
- Weissbuch, I.; Addadi, L.; Lahav, M.; Leiserowitz, L. Molecular Recognition at Crystal Interfaces. Science 1991, 253, 637–645. [Google Scholar] [CrossRef]
- Connolly, M. Analytical molecular surface calculation. J. Appl. Crystallogr. 1983, 16, 548–558. [Google Scholar] [CrossRef]
- Clegg, W.; Scott, A.J. CCDC 1575175: Experimental Crystal Structure Determination. CSD Commun. 2017. [Google Scholar] [CrossRef]
- Materials Studio, 6.0; Acceryls Inc.: San Diego, CA, USA, 2012.
- Sun, H. COMPASS: An ab Initio Force-Field Optimized for Condensed-Phase ApplicationsOverview with Details on Alkane and Benzene Compounds. J. Phys. Chem. B 1998, 102, 7338–7364. [Google Scholar] [CrossRef]
- Lan, G.; Jin, S.; Li, J.; Wang, J.; Li, J.; Chen, S.; Li, L. The study of external growth environments on the crystal morphology of ε-HNIW by molecular dynamics simulation. J. Mater. Sci. 2018, 53, 12921–12936. [Google Scholar] [CrossRef]
- Li, J.; Jin, S.; Lan, G.; Xu, Z.; Wu, N.; Chen, S.; Li, L. The effect of solution conditions on the crystal morphology of β-HMX by molecular dynamics simulations. J. Cryst. Growth 2019, 507, 38–45. [Google Scholar] [CrossRef]
- Xia, X.; Jiang, D. Determination and correlation of solubilities of catechol. CIESC J. 2007, 58, 1082–1085. [Google Scholar] [CrossRef]
- Parmar, M.M.; Khan, O.; Seton, L.; Ford, J.L. Polymorph selection with morphology control using solvents. Cryst. Growth Des. 2007, 7, 1635–1642. [Google Scholar] [CrossRef]
- Kitamura, M.; Ishizu, T. Growth kinetics and morphological change of polymorphs of L-glutamic acid. J. Cryst. Growth 2000, 209, 138–145. [Google Scholar] [CrossRef]
- Ter Horst, J.H.; Kramer, H.J.M.; van Rosmalen, G.M.; Jansens, P.J. Molecular modelling of the crystallization of polymorphs. Part I: The morphology of HMX polymorphs. J. Cryst. Growth 2002, 237, 2215–2220. [Google Scholar] [CrossRef]
- Wunderlich, H.; Mootz, D. Die Kristallstruktur von Brenzcatechin: Eine Neubestimmung. Acta Crystallogr. B 1971, 27, 1684–1686. [Google Scholar] [CrossRef]
- Zhao, Q.; Liu, N.; Wang, B.; Wang, W. A study of solvent selectivity on the crystal morphology of FOX-7 via a modified attachment energy model. RSC Adv. 2016, 6, 59784–59793. [Google Scholar] [CrossRef]
- Liang, Z.; Chen, J.-F.; Ma, Y.; Wang, W.; Han, X.; Xue, C.; Zhao, H. Qualitative rationalization of the crystal growth morphology of benzoic acid controlled using solvents. CrystEngComm 2014, 16, 5997–6002. [Google Scholar] [CrossRef]
- Einstein, A. Über einen die Erzeugung und Verwandlung des Lichtes betreffenden heuristischen Gesichtspunkt [AdP 17, 132 (1905)]. Annalen der Physik 2005, 14, 164–181. [Google Scholar] [CrossRef]
- Frenkel, D.; Smit, B. Understanding Molecular Simulation: From Algorithms to Applications; Academic Press: Cornwall, UK, 2001; Volume 1, pp. 84–105. [Google Scholar]
- Shi, W.; Xia, M.; Lei, W.; Wang, F. Solvent effect on the crystal morphology of 2,6-diamino-3,5-dinitropyridine-1-oxide: A molecular dynamics simulation study. J. Mol. Graph. Model. 2014, 50, 71–77. [Google Scholar] [CrossRef] [PubMed]
- Gupta, K.M.; Yani, Y.; Poornachary, S.K.; Chow, P.S. Atomistic Simulation To Understand Anisotropic Growth Behavior of Naproxen Crystal in the Presence of Polymeric Additives. Cryst. Growth Des. 2019, 19, 3768–3776. [Google Scholar] [CrossRef]
- Li, J.; Jin, S.; Lan, G.; Ma, X.; Ruan, J.; Zhang, B.; Chen, S.; Li, L. Morphology control of 3-nitro-1,2,4-triazole-5-one (NTO) by molecular dynamics simulation. CrystEngComm 2018, 20, 6252–6260. [Google Scholar] [CrossRef]
- Sun, T.; Liu, Q.; Xiao, J.; Zhao, F.; Xiao, H. Molecular Dynamics Simulation of Interface Interactions and Mechanical Properties of CL-20/HMX Cocrystal and Its Based PBXs. Acta Chim. Sinica 2014, 72, 1036. [Google Scholar] [CrossRef]

| Lattice Parameter | a (Å) | b (Å) | c (Å) | α (°) | β (°) | γ (°) |
|---|---|---|---|---|---|---|
| Initial | 10.941 | 5.509 | 10.069 | 90.000 | 119.000 | 90.000 |
| COMPASS optimized | 10.969 | 5.555 | 9.588 | 90.000 | 121.403 | 90.000 |
| Relative error/% | 0.26 | 0.83 | 4.78 | 0 | 2.02 | 0 |
| Faces (h k l) | Multiplicity | dhkl (Å) | Eatt (kcal mol−1) | Area (%) |
|---|---|---|---|---|
| (1 0 −1) | 2 | 8.19 | −18.09 | 48.55 |
| (1 0 1) | 2 | 5.47 | −43.45 | 19.10 |
| (0 1 1) | 4 | 4.78 | −46.15 | 10.97 |
| (1 1 0) | 4 | 4.71 | −50.50 | 1.25 |
| (0 0 2) | 2 | 4.68 | −47.41 | 0.10 |
| (1 1 −1) | 4 | 4.60 | −37.64 | 20.03 |
| Solvent | Faces | Etot | Esur | Esol | Eint | Es | E’att | R’hkl | Area/% |
|---|---|---|---|---|---|---|---|---|---|
| Isopropanol | (1 0 −1) | −11,206.93 | −3093.73 | −7916.14 | −197.05 | −13.48 | −4.61 | 1.00 | 43.87 |
| (1 0 1) | −10,774.85 | −2646.98 | −7913.94 | −213.93 | −37.01 | −6.44 | 1.40 | 35.57 | |
| (0 1 1) | −12,056.38 | −3950.79 | −7947.46 | −158.13 | −26.70 | −19.45 | 4.21 | - | |
| (1 1 0) | −12,186.14 | −4017.98 | −7954.85 | −213.31 | −30.16 | −20.35 | 4.41 | - | |
| (0 0 2) | −10,824.04 | −2703.56 | −7877.07 | −243.41 | −17.44 | −29.97 | 6.50 | - | |
| (1 1 −1) | −11,326.17 | −3180.69 | −7990.31 | −155.17 | −25.20 | −12.44 | 2.70 | 20.56 | |
| Methyl acetate | (1 0 −1) | −6423.31 | −3093.60 | −3046.64 | −283.06 | −19.36 | 1.27 | 1.00 | 70.81 |
| (1 0 1) | −6027.75 | −2646.91 | −3052.75 | −328.10 | −56.76 | 13.31 | 10.49 | 2.50 | |
| (0 1 1) | −7330.46 | −3950.59 | −2990.34 | −389.53 | −65.77 | 19.63 | 15.47 | - | |
| (1 1 0) | −7395.82 | −4017.81 | −2971.41 | −406.60 | −57.48 | 6.98 | 5.50 | 14.97 | |
| (0 0 2) | −6059.46 | −2703.51 | −3024.78 | −331.17 | −23.72 | −23.68 | 18.67 | - | |
| (1 1 −1) | −6504.15 | −3180.63 | −3062.49 | −261.03 | −42.39 | 4.75 | 3.75 | 11.72 | |
| Ethyl acetate | (1 0 −1) | −8963.16 | −3093.20 | −5609.78 | −260.18 | −17.80 | −0.30 | 1.00 | 71.14 |
| (1 0 1) | −8479.98 | −2646.54 | −5548.65 | −284.79 | −49.27 | 5.82 | 19.65 | 3.98 | |
| (0 1 1) | −9801.90 | −3950.16 | −5555.32 | −296.41 | −50.05 | 3.90 | 13.17 | - | |
| (1 1 0) | −9927.83 | −4017.27 | −5527.68 | −382.88 | −54.13 | 3.63 | 12.24 | - | |
| (0 0 2) | −8487.97 | −2703.17 | −5532.21 | −252.59 | −18.10 | −29.31 | 98.96 | - | |
| (1 1 −1) | −9038.79 | −3180.23 | −5633.03 | −225.53 | −36.62 | −1.01 | 3.42 | 24.88 |
| Faces | Ahkl (Å2) | Aacc (Å2) | S |
|---|---|---|---|
| (1 0 −1) | 60.93 | 75.02 | 1.23 |
| (1 0 1) | 91.11 | 189.13 | 2.08 |
| (0 1 1) | 104.40 | 211.54 | 2.03 |
| (1 1 0) | 105.99 | 179.81 | 1.70 |
| (0 0 2) | 53.25 | 91.56 | 1.72 |
| (1 1 −1) | 108.51 | 158.59 | 1.46 |
| Faces | Isopropanol | Methyl Acetate | Ethyl Acetate |
|---|---|---|---|
| (1 0 −1) | 1.09 | 3.00 | 2.53 |
| (1 0 1) | 0.75 | 2.25 | 2.28 |
| (0 1 1) | 0.69 | 2.57 | 1.94 |
| (1 1 0) | 0.67 | 2.52 | 2.11 |
| (0 0 2) | 0.91 | 2.88 | 2.21 |
| (1 1 −1) | 0.74 | 2.82 | 2.18 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, D.; Zhang, S.; Cui, P.; Wang, C.; Dai, J.; Zhou, L.; Huang, Y.; Hou, B.; Hao, H.; Zhou, L.; et al. Solvent Effects on Catechol Crystal Habits and Aspect Ratios: A Combination of Experiments and Molecular Dynamics Simulation Study. Crystals 2020, 10, 316. https://doi.org/10.3390/cryst10040316
Zhu D, Zhang S, Cui P, Wang C, Dai J, Zhou L, Huang Y, Hou B, Hao H, Zhou L, et al. Solvent Effects on Catechol Crystal Habits and Aspect Ratios: A Combination of Experiments and Molecular Dynamics Simulation Study. Crystals. 2020; 10(4):316. https://doi.org/10.3390/cryst10040316
Chicago/Turabian StyleZhu, Dan, Shihao Zhang, Pingping Cui, Chang Wang, Jiayu Dai, Ling Zhou, Yaohui Huang, Baohong Hou, Hongxun Hao, Lina Zhou, and et al. 2020. "Solvent Effects on Catechol Crystal Habits and Aspect Ratios: A Combination of Experiments and Molecular Dynamics Simulation Study" Crystals 10, no. 4: 316. https://doi.org/10.3390/cryst10040316
APA StyleZhu, D., Zhang, S., Cui, P., Wang, C., Dai, J., Zhou, L., Huang, Y., Hou, B., Hao, H., Zhou, L., & Yin, Q. (2020). Solvent Effects on Catechol Crystal Habits and Aspect Ratios: A Combination of Experiments and Molecular Dynamics Simulation Study. Crystals, 10(4), 316. https://doi.org/10.3390/cryst10040316








