Matching with Nonexclusive Contracts †
Abstract
1. Introduction
- Is it possible to obtain contract coexistence in a continuously differentiable setting?
- If contracts can coexist, which productivity types sign which contract, and under what conditions?
2. Related Literature and Contribution
2.1. Nonexclusive Contracting
2.2. Two-Sided Matching
3. Model
3.1. Matching and Output
- (i)
- and if .
- (ii)
- , , and if .
- (iii)
- iff .
3.2. Contracts and Timing
3.3. Principal–Agent Pair
4. Equilibrium Matching and Stability
4.1. Principal–Agent Matching
4.2. Stability
- 1.
- Individual Rationality:
- (i)
- Principals: .
- (ii)
- Agents: and .
- 2.
- No Blocking: If
- (i)
- Then .
- (ii)
- And if , then .
4.3. Decentralized Principal–Agent Market
- 1.
- The menu of contracts is feasible and Constrained Pareto Optimal.
- 2.
- The assignment object is pairwise stable.
5. Analysis
5.1. Isolation vs. Market
- Case 1:
- 1.
- If the lowest type of market exhibits exclusive contracts, then all other markets will also exhibit exclusive contracts.
- 2.
- If the lowest type of market exhibits nonexclusive contracts, then markets with high enough productivity will sign exclusive contracts.
- Case 2:
- 1.
- If the lowest type of market exhibits exclusive contracts, then markets with high enough productivity will sign nonexclusive contracts.
- 2.
- If the lowest type of market exhibits nonexclusive contracts, then all markets will sign nonexclusive contracts.
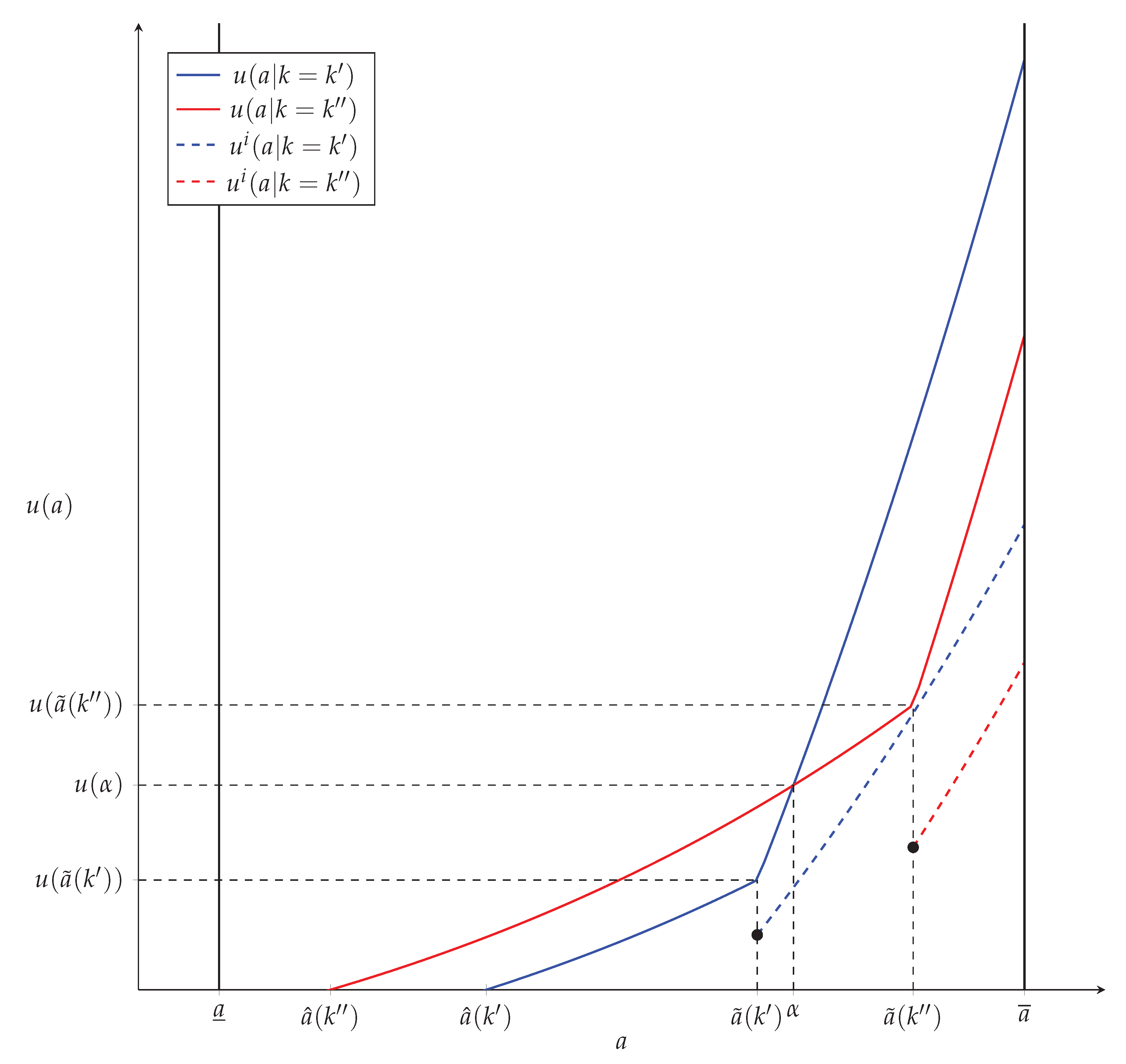
5.2. Equilibrium Contract Patterns
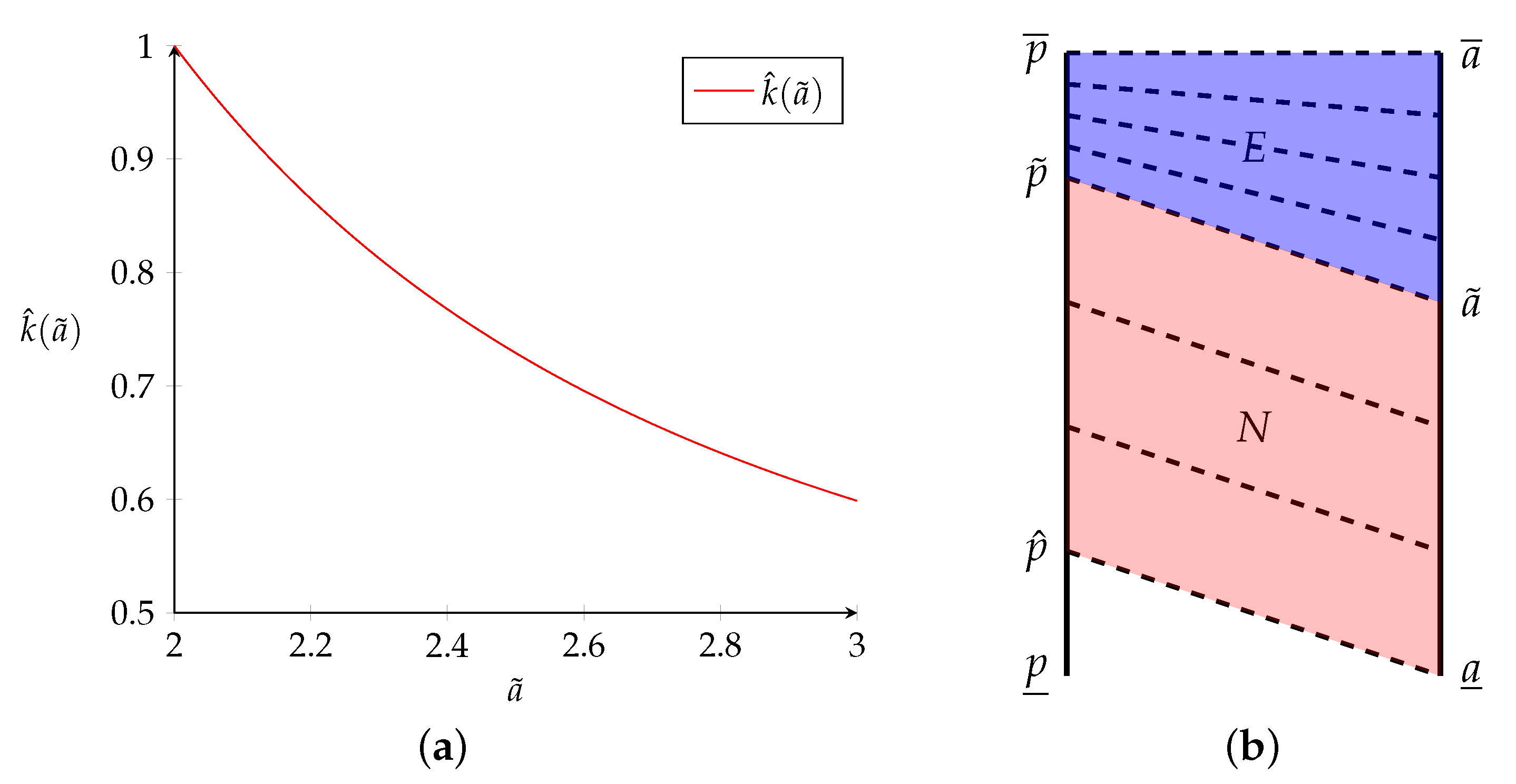
5.2.1. Threshold Function
5.2.2. More Agents than Principals
5.2.3. More Principals than Agents
5.3. Utility Functions
5.3.1. Agent Unemployment
5.3.2. Principal Unemployment
6. Implications
7. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Proofs
Appendix A.1. Second-Order Conditions
Appendix A.2
Appendix A.3
- Above the Marginal Coalition:
- Below the Marginal Coalition:
- Across the Marginal Coalition:
Appendix A.4
Appendix A.5
- First, I show when condition 2 will hold.
- Then, I express the requirements for condition 2 to hold as a restriction on the exclusive matching function.
- Finally, I derive conditions for the set K to be unique.
- Step One:
- Step One:
- Step Three (Interval Uniqueness):
Appendix A.6
Appendix B. Numerical Example
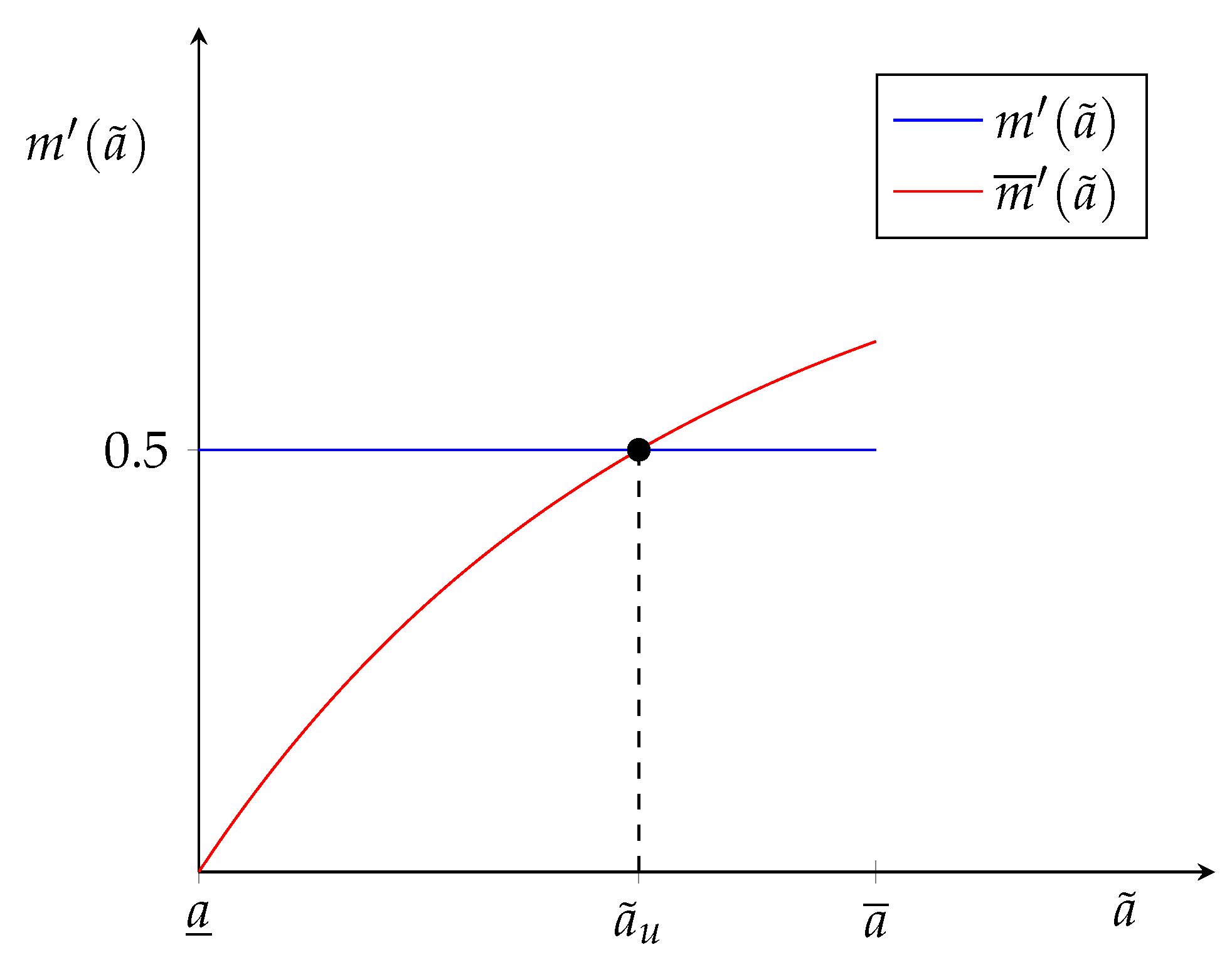
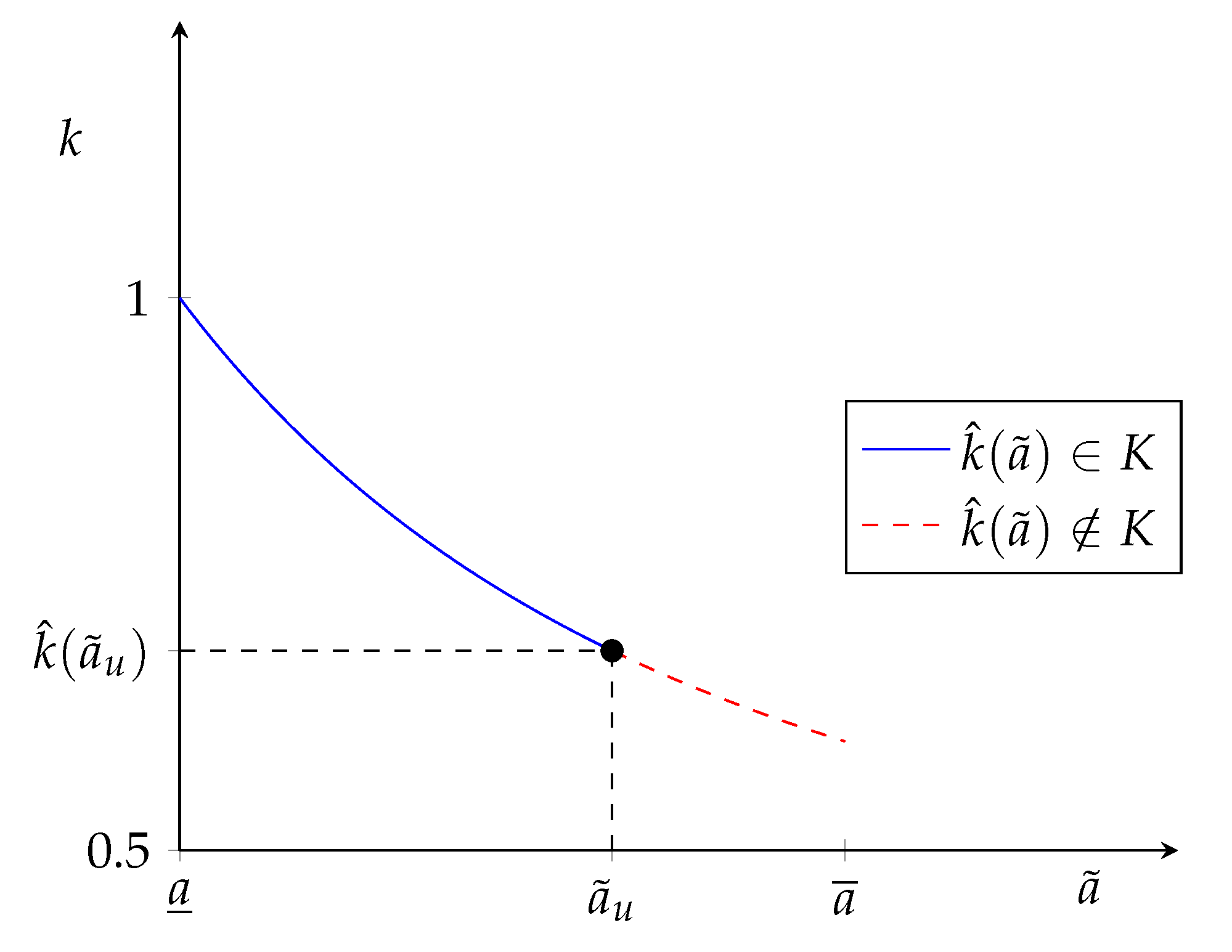
Appendix C. General Matching Functions
Appendix C.1. Matching Functions
Appendix C.2. Contract Patterns
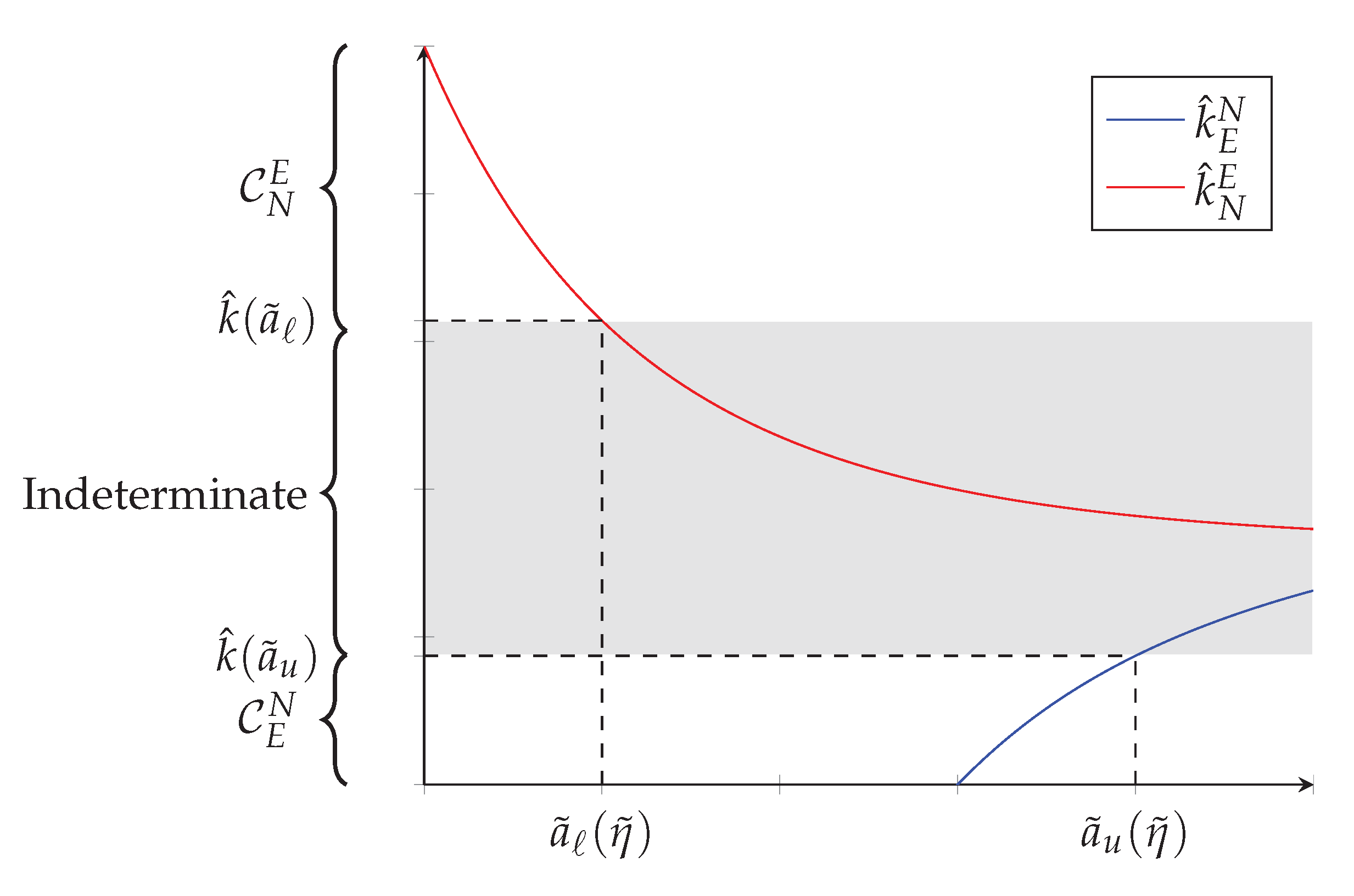
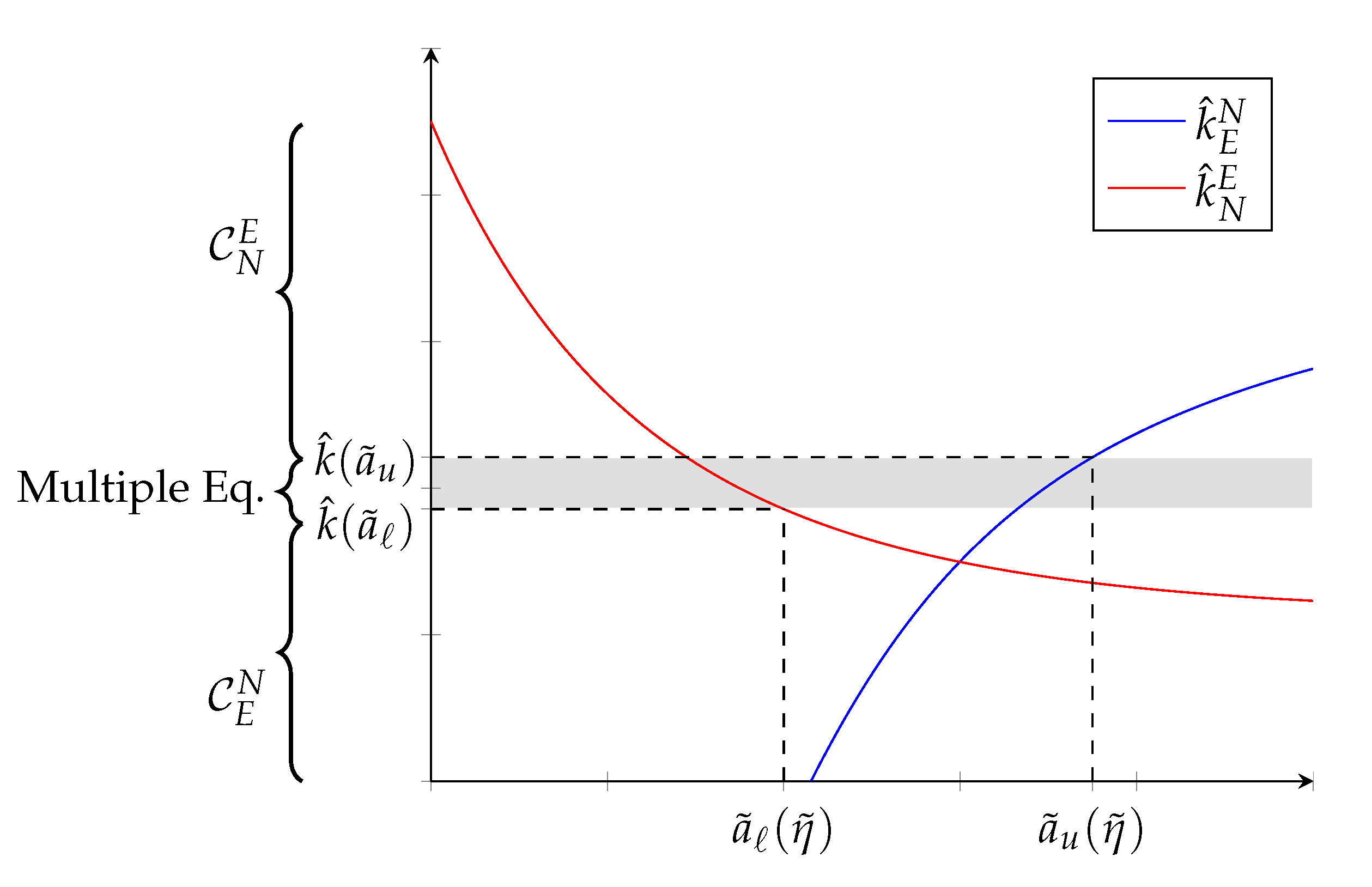
| 1 | A contract pattern is monotonic if there is no more than one point in the type distributions of the principals and agents where the type of contract signed changes. |
| 2 | Here, I use the subscripts i and j to distinguish between two principals of the same productivity type. As will become clear after the analysis of the contracting problem, I only need to examine a principal-agent pair, because principal j’s type does not enter into the problem for principal i. |
| 3 | One could imagine a cost function of the form , where effort exerted for each principal is impacted by effort exerted for the other. The effort externality causes stability issues in the matching market when there are a continuum of types. |
| 4 | In Section 3.3 I will solve the contracting problem for a principal-agent pair. |
| 5 | Initial work on a model with moral hazard found that the result regarding the monotonicity of contract patterns was not affected by incentive contracts alone. In order to have nonmonotonicity, the model must have a limited liability constraint that binds for some principal-agent pairs and not for others. |
| 6 | I will discuss the bargaining frontier in greater detail in Section 4. |
| 7 | I leave an in depth discussion of the utility functions for Section 5. |
| 8 | This is just the sum of the utilities received from the principals entering nonexclusive contracts with the agent, so that . |
| 9 | Definitions 3 and 4 are borrowed from [11], and altered to fit my model. |
| 10 | In their setting with colleges and students, a college’s preferences over a set of students is responsive to its preferences over individual students, if there are two matchings that differ only by two students, and the college prefers the set containing the more preferred student. Consider a preference ranking for an agent in my model, where . The agent’s preferences are responsive if . |
| 11 | It is important to note that in the model , because no more than two principals can match with an agent. |
| 12 | Recall, the total surplus generated by a principal-agent coalition under a nonexclusive contract is just the sum of the surpluses generated by the two principals matched with a given agent. For example, . |
| 13 | Since it is a local condition, satisfying the (26) does not ensure the equilibrium is pairwise stable. Thus I say I evaluate at a proposed equilibrium assignment and not a stable equilibrium assignment . |
| 14 | If we do not make this change, then differentiating both sides of the inequality with respect to agent productivity type incorrectly readjusts the relevant principal type in the nonexclusive surplus function. |
| 15 | Note that these are potential contracts, and they may or may not be stable. |
| 16 | Note that these are potential contracts, and they may or may not be stable. |
| 17 | This is a slight abuse of notation, since it is technically , but is suppressed for exposition. |
| 18 | The underlying assumption is that the increased complexity does not lead to increased revenue. This could be due to all firms adopting a new innovation, and an intensely competitive market structure preventing firms from gaining an advantage. |
| 19 | The matching market can be thought of as a short-term problem that is solved each period. Eventually it may be optimal for the firm to build more plants, which would change the assignment problem. |
References
- Marvel, H.P. Exclusive Dealing. J. Law Econ. 1982, 25, 64–103. [Google Scholar] [CrossRef]
- Sass, T.R.; Gisser, M. Agency Cost, Firm Size, and Exclusive Dealing. J. Law Econ. 1989, 32, 381–400. [Google Scholar] [CrossRef]
- von Lilienfeld-Toal, U.; Mookherjee, D. A General Equilibrium Analysis of Personal Bankruptcy Law. Economica 2016, 83, 31–58. [Google Scholar] [CrossRef]
- Parlour, C.A.; Rajan, U. Competition in Loan Contracts. Am. Econ. Rev. 2001, 91, 1311–1328. [Google Scholar] [CrossRef]
- Matouschek, N.; Ramezzana, P. The Role Of Exclusive Contracts In Facilitating Market Transactions. J. Ind. Econ. 2007, 55, 347–371. [Google Scholar] [CrossRef]
- Sass, T.R. The competitive effects of exclusive dealing: Evidence from the U.S. beer industry. Int. J. Ind. Organ. 2005, 23, 203–225. [Google Scholar] [CrossRef]
- Besley, T.; Ghatak, M. Competition and Incentives with Motivated Agents. Am. Econ. Rev. 2005, 95, 616–636. [Google Scholar] [CrossRef]
- Dam, K.; Pérez-Castrillo, D. The Principal-Agent Matching Market. Front. Theor. Econ. 2006, 2, 20021001. [Google Scholar] [CrossRef]
- Serfes, K. Endogenous Matching in a Market with Heterogeneous Principals and Agents. Int. J. Game Theory 2008, 36, 587–619. [Google Scholar] [CrossRef]
- Dam, K.; Ripperger-Suhler, D.; Serfes, K. Two-sided productivity heterogeneity, firm boundaries, and assortative matching. J. Econ. Manag. Strategy, 2023; in press. [Google Scholar]
- Alonso-Paulí, E.; Pérez-Castrillo, D. Codes of Best Practice in Competitive Markets for Managers. Econ. Theory 2012, 49, 113–141. [Google Scholar] [CrossRef][Green Version]
- Macho-Stadler, I.; Pérez-Castrillo, D.; Porteiro, N. Coexistence of Long-term and Short-term Contracts. Games Econ. Behav. 2014, 86, 145–164. [Google Scholar] [CrossRef][Green Version]
- Hatfield, J.; Milgrom, P. Matching with Contracts. Am. Econ. Rev. 2005, 95, 913–935. [Google Scholar] [CrossRef]
- Kelso, A.S.; Crawford, V.P. Job Matching, Coalition Formation, and Gross Substitutes. Econometrica 1982, 50, 1483–1504. [Google Scholar] [CrossRef]
- Hatfield, J.W.; Kojima, F. Substitutes and Stability for Matching with Contracts. J. Econ. Theory 2010, 145, 1704–1723. [Google Scholar] [CrossRef]
- Azevedo, E.M. Imperfect Competition in Two-Sided Matching Markets. Games Econ. Behav. 2014, 83, 207–223. [Google Scholar] [CrossRef]
- Eeckhout, J.; Kircher, P. Assortative Matching with Large Firms. Econometrica 2018, 86, 85–132. [Google Scholar] [CrossRef]
- Maier, N.; Ottaviani, M. Information Sharing in Common Agency: When is Transparency Good? J. Eur. Econ. Assoc. 2009, 7, 162–187. [Google Scholar] [CrossRef][Green Version]
- Holmstrom, B.; Milgrom, P. Common Agency and Exclusive Dealing; Working Paper; Yale University, School of Management: New Haven, CT, USA, 1988. [Google Scholar]
- Holmstrom, B.; Milgrom, P. Multitask Principal-Agent Analyses: Incentive Contracts, Asset Ownership, and Job Design. J. Law Econ. Organ. 1991, 7, 24–52. [Google Scholar] [CrossRef]
- Dutta, B.; Masso, J. Stability of Matchings When Individuals Have Preferences over Colleagues. J. Econ. Theory 1997, 75, 464–475. [Google Scholar] [CrossRef]
- Roth, A.E.; Sotomayor, M. Two-Sided Matching: A Study in Game-Theoretic Modeling and Analysis; Wiley: Hoboken, NJ, USA, 1990. [Google Scholar]
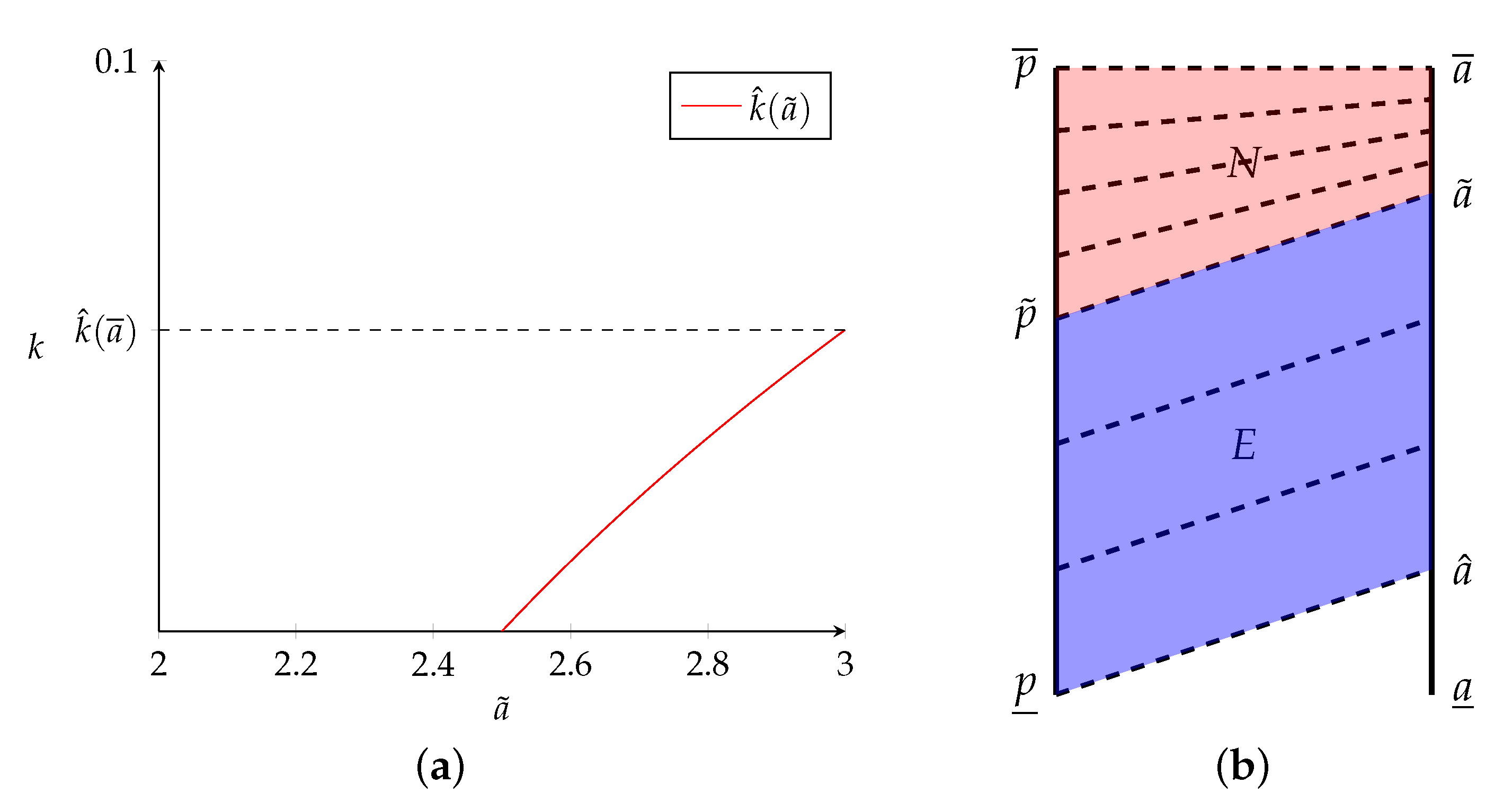

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ripperger-Suhler, D. Matching with Nonexclusive Contracts. Games 2024, 15, 11. https://doi.org/10.3390/g15020011
Ripperger-Suhler D. Matching with Nonexclusive Contracts. Games. 2024; 15(2):11. https://doi.org/10.3390/g15020011
Chicago/Turabian StyleRipperger-Suhler, Daniel. 2024. "Matching with Nonexclusive Contracts" Games 15, no. 2: 11. https://doi.org/10.3390/g15020011
APA StyleRipperger-Suhler, D. (2024). Matching with Nonexclusive Contracts. Games, 15(2), 11. https://doi.org/10.3390/g15020011





