Design and Assessment of a Novel Biconical Human-Sized Alternating Magnetic Field Coil for MNP Hyperthermia Treatment of Deep-Seated Cancer
Abstract
Simple Summary
Abstract
1. Introduction
2. Materials and Methods
2.1. Virtual Human Model
2.2. Magnetic Nanoparticles
2.3. Alternating Electromagnetic Fields Calculations
2.4. AMF Coil Design
2.5. Bio-Heat Equation
3. Results
3.1. Analysis of the Limitations of the Standard Approach
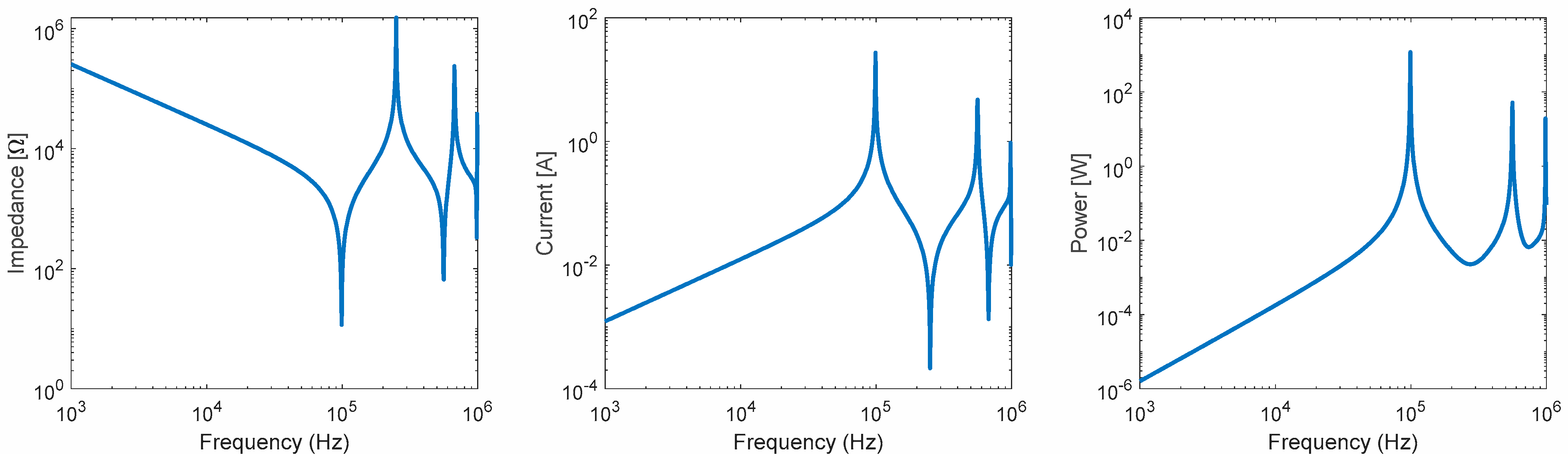
3.2. Human-Sized Biconical Coil: SARJoule and Temperature Distributions
3.3. Human-Sized Coil: Assessing MNPH Efficacy and Temperature Distributions
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Stauffer, P.R.; Cetas, T.C.; Jones, R.C. Magnetic Induction Heating of Ferromagnetic Implants for Inducing Localized Hyperthermia in Deep-Seated Tumors. IEEE Trans. Biomed. Eng. 1984, BME-31, 235–251. [Google Scholar] [CrossRef] [PubMed]
- Siegel, R.L.; Miller, K.D.; Fuchs, H.E.; Jemal, A. Cancer statistics, 2022. CA Cancer J. Clin. 2022, 72, 7–33. [Google Scholar] [CrossRef] [PubMed]
- Ruarus, A.; Vroomen, L.; Puijk, R.; Scheffer, H.; Meijerink, M. Locally Advanced Pancreatic Cancer: A Review of Local Ablative Therapies. Cancers 2018, 10, 16. [Google Scholar] [CrossRef] [PubMed]
- Kamisawa, T.; Wood, L.D.; Itoi, T.; Takaori, K. Pancreatic cancer. Lancet 2016, 388, 73–85. [Google Scholar] [CrossRef]
- Conroy, T.; Desseigne, F.; Ychou, M.; Bouche, O.; Guimbaud, R.; Becouarn, Y.; Adenis, A.; Raoul, J.L.; Gourgou-Bourgade, S.; de la Fouchardiere, C.; et al. FOLFIRINOX versus gemcitabine for metastatic pancreatic cancer. N. Engl. J. Med. 2011, 364, 1817–1825. [Google Scholar] [CrossRef]
- Ducreux, M.; Cuhna, A.S.; Caramella, C.; Hollebecque, A.; Burtin, P.; Goere, D.; Seufferlein, T.; Haustermans, K.; Van Laethem, J.L.; Conroy, T.; et al. Cancer of the pancreas: ESMO Clinical Practice Guidelines for diagnosis, treatment and follow-up. Ann. Oncol. 2015, 26 (Suppl. S5), 56–68. [Google Scholar] [CrossRef]
- Khorana, A.A.; Mangu, P.B.; Berlin, J.; Engebretson, A.; Hong, T.S.; Maitra, A.; Mohile, S.G.; Mumber, M.; Schulick, R.; Shapiro, M.; et al. Potentially Curable Pancreatic Cancer: American Society of Clinical Oncology Clinical Practice Guideline Update. J. Clin. Oncol. 2017, 35, 2324–2328. [Google Scholar] [CrossRef]
- Vogl, T.J.; Panahi, B.; Albrecht, M.H.; Naguib, N.N.N.; Nour-Eldin, N.A.; Gruber-Rouh, T.; Thompson, Z.M.; Basten, L.M. Microwave ablation of pancreatic tumors. Minim. Invasive Ther. Allied Technol. 2018, 27, 33–40. [Google Scholar] [CrossRef] [PubMed]
- Lafond, M.; Lambin, T.; Drainville, R.A.; Dupré, A.; Pioche, M.; Melodelima, D.; Lafon, C. Pancreatic Ductal Adenocarcinoma: Current and Emerging Therapeutic Uses of Focused Ultrasound. Cancers 2022, 14, 2577. [Google Scholar] [CrossRef]
- Huggett, M.T.; Jermyn, M.; Gillams, A.; Illing, R.; Mosse, S.; Novelli, M.; Kent, E.; Bown, S.G.; Hasan, T.; Pogue, B.W.; et al. Phase I/II Study of Verteporfin Photodynamic Therapy in Locally Advanced Pancreatic Cancer. Br. J. Cancer 2014, 110, 1698–1704. [Google Scholar] [CrossRef]
- Attaluri, A.; Kandala, S.K.; Zhou, H.; Wabler, M.; DeWeese, T.L.; Ivkov, R. Magnetic nanoparticle hyperthermia for treating locally advanced unresectable and borderline resectable pancreatic cancers: The role of tumor size and eddy-current heating. Int. J. Hyperth. 2020, 37, 108–119. [Google Scholar] [CrossRef] [PubMed]
- Kucharczyk, K.; Kaczmarek, K.; Jozefczak, A.; Slachcinski, M.; Mackiewicz, A.; Dams-Kozlowska, H. Hyperthermia treatment of cancer cells by the application of targeted silk/iron oxide composite spheres. Mater. Sci. Eng. C 2021, 120, 111654. [Google Scholar] [CrossRef] [PubMed]
- Ivkov, R.; Attaluri, A.; Guiriba, T.; Liu, Y.; Zhou, H.; Hedayati, M.; DeWeese, T.; Liapi, E.; Herman, J. Magnetic Nanoparticle Hyperthermia and Radiation for Locally Advanced Pancreas Cancer: An In Vitro and In Vivo Study. Int. J. Radiat. Oncol. Biol. Phys. 2014, 90, S172–S173. [Google Scholar] [CrossRef]
- Deng, X.; Liang, H.; Yang, W.; Shao, Z. Polarization and function of tumor-associated macrophages mediate graphene oxide-induced photothermal cancer therapy. J. Photochem. Photobiol. B Biol. 2020, 208, 111913. [Google Scholar] [CrossRef] [PubMed]
- Wust, P.; Gneveckow, U.; Johannsen, M.; Nohmer, D.; Henkel, T.; Kahmann, F.; Sehouli, J.; Felix, R.; Ricke, J.; Jordan, A. Magnetic nanoparticles for interstitial thermotherapy—Feasibility, tolerance and achieved temperatures. Int. J. Hyperth. 2006, 22, 673–685. [Google Scholar] [CrossRef]
- Mendez, M.H.; Joh, D.Y.; Gupta, R.; Polascik, T.J. Current Trends and New Frontiers in Focal Therapy for Localized Prostate Cancer. Curr. Urol. Rep. 2015, 16, 35. [Google Scholar] [CrossRef]
- Maier-Hauff, K.; Rothe, R.; Scholz, R.; Gneveckow, U.; Wust, P.; Thiesen, B.; Feussner, A.; Von Deimling, A.; Waldoefner, N.; Felix, R.; et al. Intracranial thermotherapy using magnetic nanoparticles combined with external beam radiothera-py: Results of a feasibility study on patients with glioblastoma multiforme. J. Neurooncol. 2007, 81, 53–60. [Google Scholar] [CrossRef]
- Espinosa, A.; Kolosnjaj-Tabi, J.; Abou-Hassan, A.; Sangnier, A.P.; Curcio, A.; Silva, A.K.A.; Di Corato, R.; Neveu, S.; Pellegrino, T.; Liz-Marzán, L.M.; et al. Magnetic (Hyper)Thermia or Photothermia? Progressive Comparison of Iron Oxide and Gold Nanoparticles Heating in Water, in Cells, and In Vivo. Adv. Funct. Mater. 2018, 28, 1803660. [Google Scholar] [CrossRef]
- Johannsen, M.; Gneveckow, U.; Taymoorian, K.; Thiesen, B.; Waldöfner, N.; Scholz, R.; Jung, K.; Jordan, A.; Wust, P.; Loening, S.A. Morbidity and quality of life during thermotherapy using magnetic nanoparticles in locally recurrent prostate cancer: Results of a prospective phase I trial. Int. J. Hyperth. 2007, 23, 315–323. [Google Scholar] [CrossRef]
- Clinical Trials: NCT02033447 (Prostate), NCT00848042 (Head and Neck Tumors). Available online: https://clinicaltrials.gov/ct2/show/NCT02033447;https://clinicaltrials.gov/ct2/show/NCT00848042 (accessed on 16 November 2022).
- Iacovita, C.; Florea, A.; Scorus, L.; Pall, E.; Dudric, R.; Moldovan, A.I.; Stiufiuc, R.; Tetean, R.; Lucaciu, C.M. Hyperthermia, Cytotoxicity, and Cellular Uptake Properties of Manganese and Zinc Ferrite Magnetic Nanoparticles Synthesized by a Polyol-Mediated Process. Nanomaterials 2019, 9, 1489. [Google Scholar] [CrossRef]
- Ito, A.; Kuga, Y.; Honda, H.; Kikkawa, H.; Horiuchi, A.; Watanabe, Y.; Kobayashi, T. Magnetite nanoparticle-loaded anti-HER2 immunoliposomes for combination of antibody therapy with hyperthermia. Cancer Lett. 2004, 212, 167–175. [Google Scholar] [CrossRef] [PubMed]
- Kossatz, S.; Ludwig, R.; Dähring, H.; Ettelt, V.; Rimkus, G.; Marciello, M.; Salas, G.; Patel, V.; Teran, F.J.; Hilger, I. High Therapeutic Efficiency of Magnetic Hyperthermia in Xenograft Models Achieved with Moderate Temperature Dosages in the Tumor Area. Pharm. Res. 2014, 31, 3274–3288. [Google Scholar] [CrossRef] [PubMed]
- Basel, M.; Balivada, S.; Wang, H.; Shrestha, T.B.; Seo, G.M.; Pyle, M.; Ayabaweera, G.; Dani, R.K.; Koper, O.B.; Tamura, M.; et al. Cell-delivered magnetic nanoparticles caused hyperthermia-mediated increased survival in a murine pancreatic cancer model. Int. J. Nanomed. 2012, 7, 297–306. [Google Scholar] [CrossRef] [PubMed]
- Brezovich, A.I.; Atkinson, W.J.; Lilly, M.B. Local hyperthermia with interstitial techniques. Cancer Res 1984, 44, 4752s–4756s. [Google Scholar] [PubMed]
- Atkinson, W.J.; Brezovich, I.A.; Chakraborty, D.P. Useable frequencies in hyperthermia with thermal seeds. IEEE (Inst. Electr. Electron Eng.) Trans. Biomed. Eng. 1984, 31, 70–75. [Google Scholar]
- Etheridge, M.L.; Bischof, J.C. Optimizing Magnetic Nanoparticle Based Thermal Therapies within the Physical Limits of Heating. Ann. Biomed. Eng. 2012, 41, 78–88. [Google Scholar] [CrossRef]
- Wust, P.; Nadobny, J.; Szimtenings, M.; Stetter, E.; Gellermann, J. IMPLICATIONS OF CLINICAL RF HYPERTHERMIA ON PROTECTION LIMITS IN THE RF RANGE. Health Phys. 2007, 92, 565–573. [Google Scholar] [CrossRef]
- Stigliano, R.V.; Shubitidze, F.; Petryk, J.D.; Shoshiashvili, L.; Petryk, A.A.; Hoopes, P.J. Mitigation of eddy current heating during magnetic nanoparticle hyperthermia therapy. Int. J. Hyperth. 2016, 32, 735–748. [Google Scholar] [CrossRef]
- Hergt, R.; Dutz, S. Magnetic particle hyperthermia—Biophysical limitations of a visionary tumour therapy. J. Magn. Magn. Mater. 2007, 311, 187–192. [Google Scholar] [CrossRef]
- Shubitidze, F.; Kekalo, K.; Stigliano, R.; Baker, I. Magnetic nanoparticles with high specific absorp-tion rate of electromagnetic energy at low field strength for hyperthermia therapy. J. Appl. Phys. 2015, 117, 094302. [Google Scholar] [CrossRef]
- Bakker, F.; Paulides, M.M.; Neufeld, E.; Christ, A.; Kuster, N.; van Rhoon, G.C. Children and adults exposed to electromag-netic fields at the ICNIRP reference levels: Theoretical assessment of the induced peak temperature increase. Phys. Med. Biol. 2011, 56, 4967. [Google Scholar] [CrossRef]
- Christ, A.; Kainz, W.; Hahn, E.G.; Honegger, K.; Zefferer, M.; Neufeld, E.; Rascher, W.; Janka, R.; Bautz, W.; Chen, J.; et al. The Virtual Family—Development of surface-based anatomical models of two adults and two children for dosimetric simulations. Phys. Med. Biol. 2009, 55, N23–N38. [Google Scholar] [CrossRef]
- Available online: https://itis.swiss/virtual-population/tissue-properties/database/ (accessed on 12 January 2023).
- Kekalo, K.; Baker, I. Magnetic Nanoparticles, Composites, Suspensions and Collids with High Specific Absorption Rate (SAR). Patent 089464A1, 6 December 2013. [Google Scholar]
- Kekalo, K.; Baker, I.; Meyers, R.; Shyong, J. Magnetic Nanoparticles with High Specific Absorption Rate at Low Alternating Magnetic Field. Nano LIFE 2015, 5, 1550002. [Google Scholar] [CrossRef] [PubMed]
- Griffith, J.M.; Pan, G.W. Time Harmonic Fields Produced by Circular Current Loops. IEEE Trans. Magn. 2011, 47, 2029–2033. [Google Scholar] [CrossRef]
- Attaluri, A.; Jackowski, J.; Sharma, A.; Kandala, S.K.; Nemkov, V.; Yakey, C.; Deweese, T.L.; Kumar, A.; Goldstein, R.C.; Ivkov, R. Design and construction of a Maxwell-type induction coil for magnetic nanoparticle hyperthermia. Int. J. Hyperth. 2020, 37, 1–14. [Google Scholar] [CrossRef] [PubMed]
- Gneveckow, U.; Jordan, A.; Scholz, R.; Brüß, V.; Waldöfner, N.; Ricke, J.; Feussner, A.; Hildebrandt, B.; Rau, B.; Wust, P. Description and charac-terization of the novel hyperthermia- and thermoablation-systemMFHVR300F for clinical magnetic fluid hyperthermia. Med. Phys. 2004, 31, 1444–1451. [Google Scholar] [CrossRef]
- Pennes, H.H. Analysis of tissue and arterial blood temperatures in the resting human forearm. J. Appl. Physiol. 1948, 1, 93–122. [Google Scholar] [CrossRef]
- Razmadze, A.; Shoshiashvili, L.; Kakulia, D.; Zaridze, R.; Bit-Babik, G.; Faraone, A. Influence of Specific Absorption Rate Averaging Schemes on Correlation between Mass-Averaged Specific Absorption Rate and Temperature Rise. Electromagnetics 2009, 29, 77–90. [Google Scholar] [CrossRef]
- Ga¨hwiler, B.; Mamoon, A.; Schlapfer, W.; Tobias, C. Effects of temperature on spontaneous bioelectric activity of cultured nerve cells. Brain Res. 1972, 40, 527–533. [Google Scholar] [CrossRef]
- Emcos Studio 2022. Available online: https://www.emcos.com/ (accessed on 25 February 2023).
- Prijic, S.; Sersa, G. Magnetic nanoparticles as targeted delivery systems in oncology. Radiol. Oncol. 2011, 45, 1–16. [Google Scholar] [CrossRef]
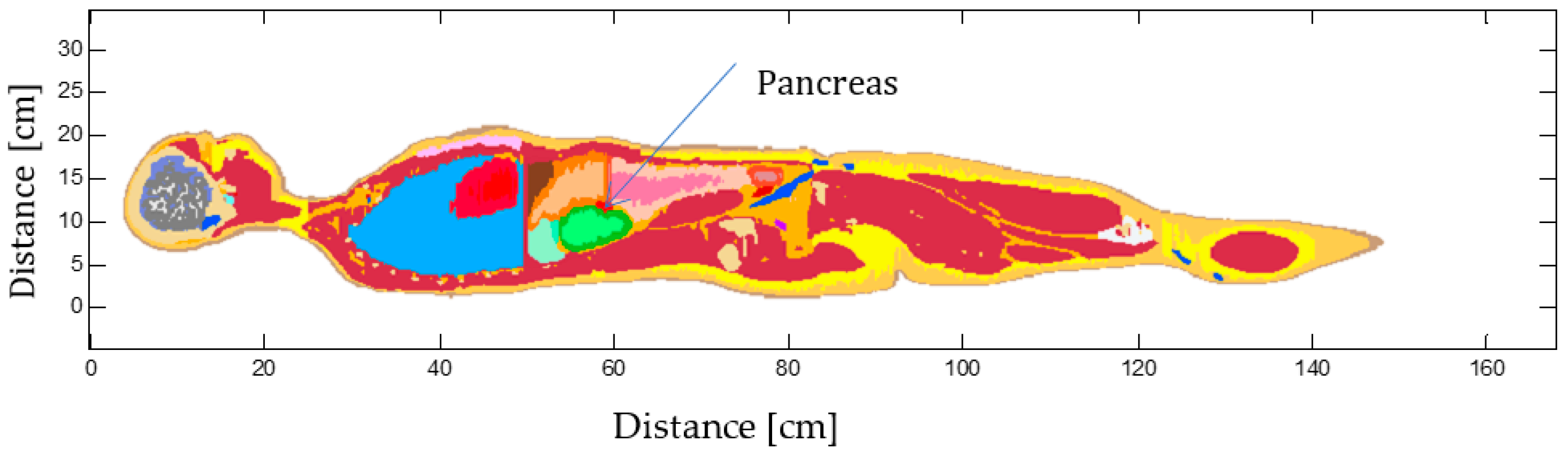

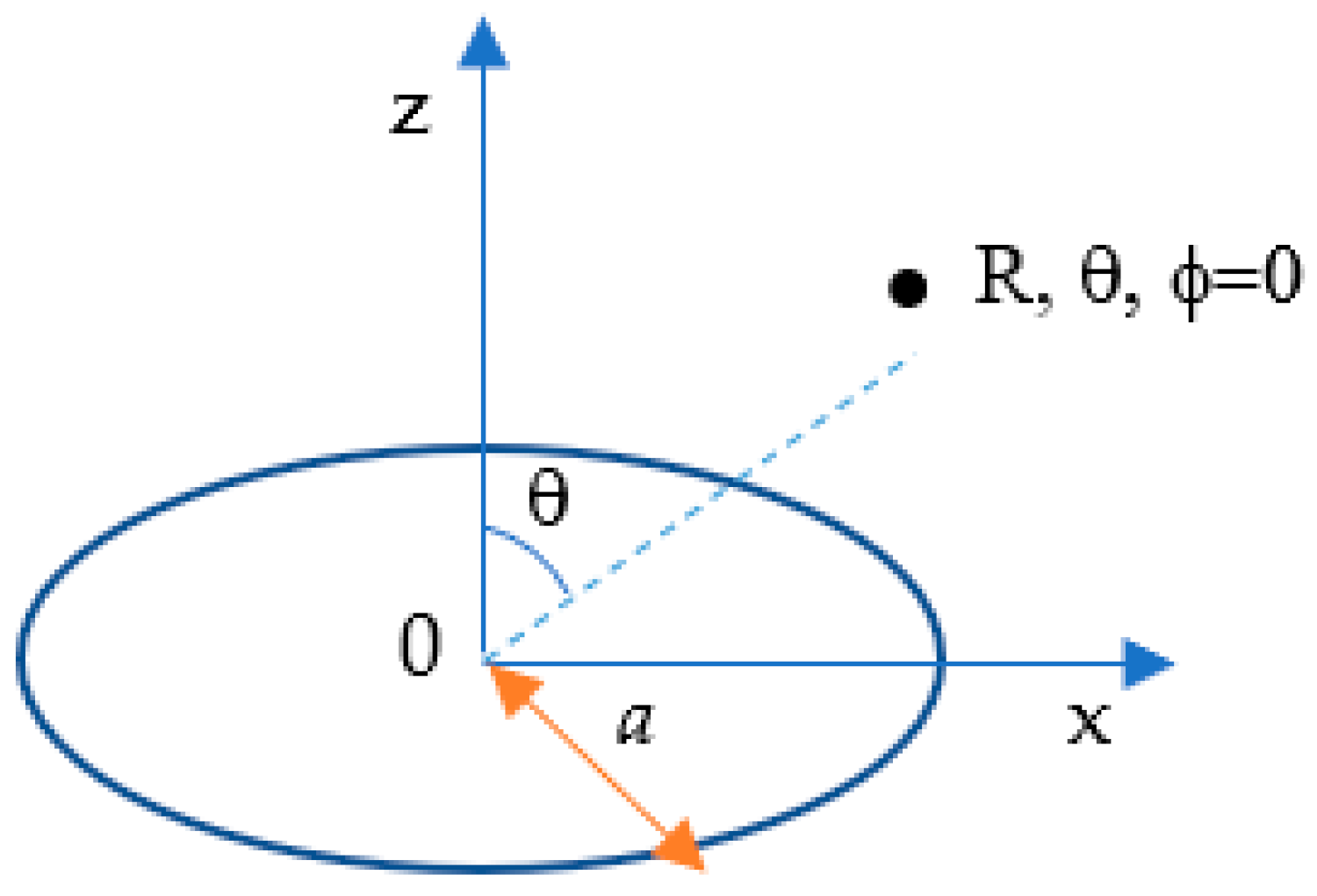
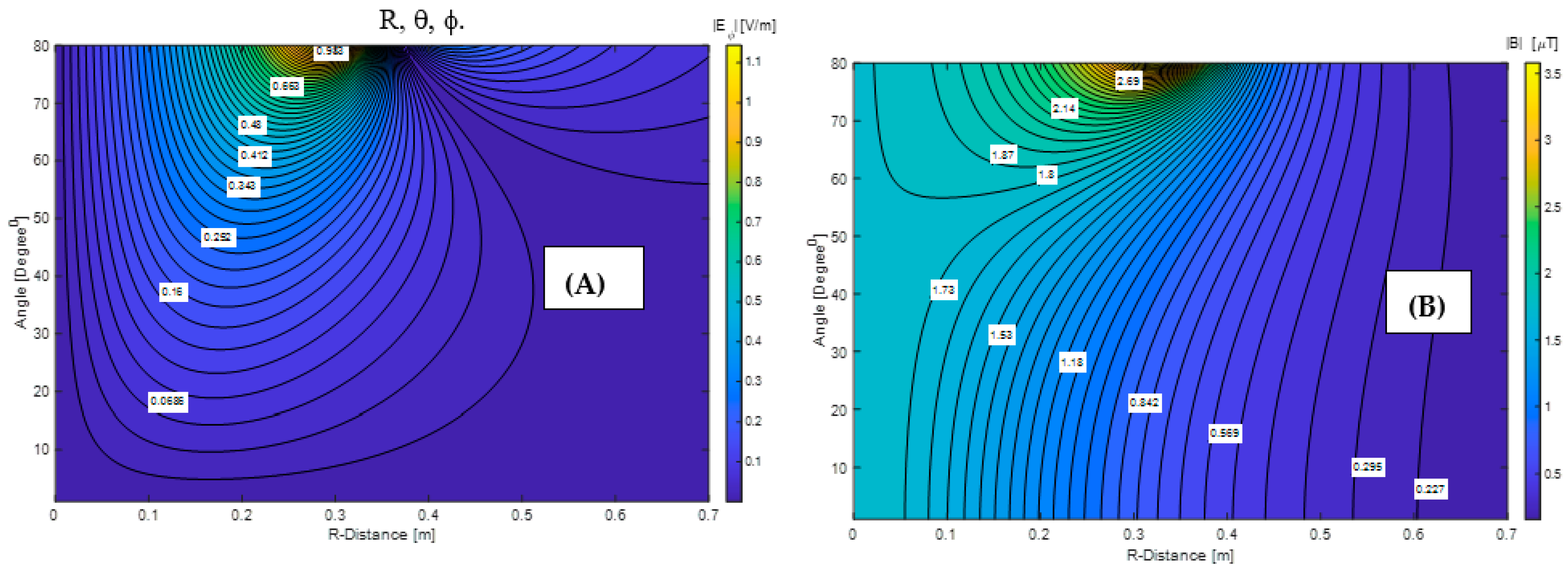







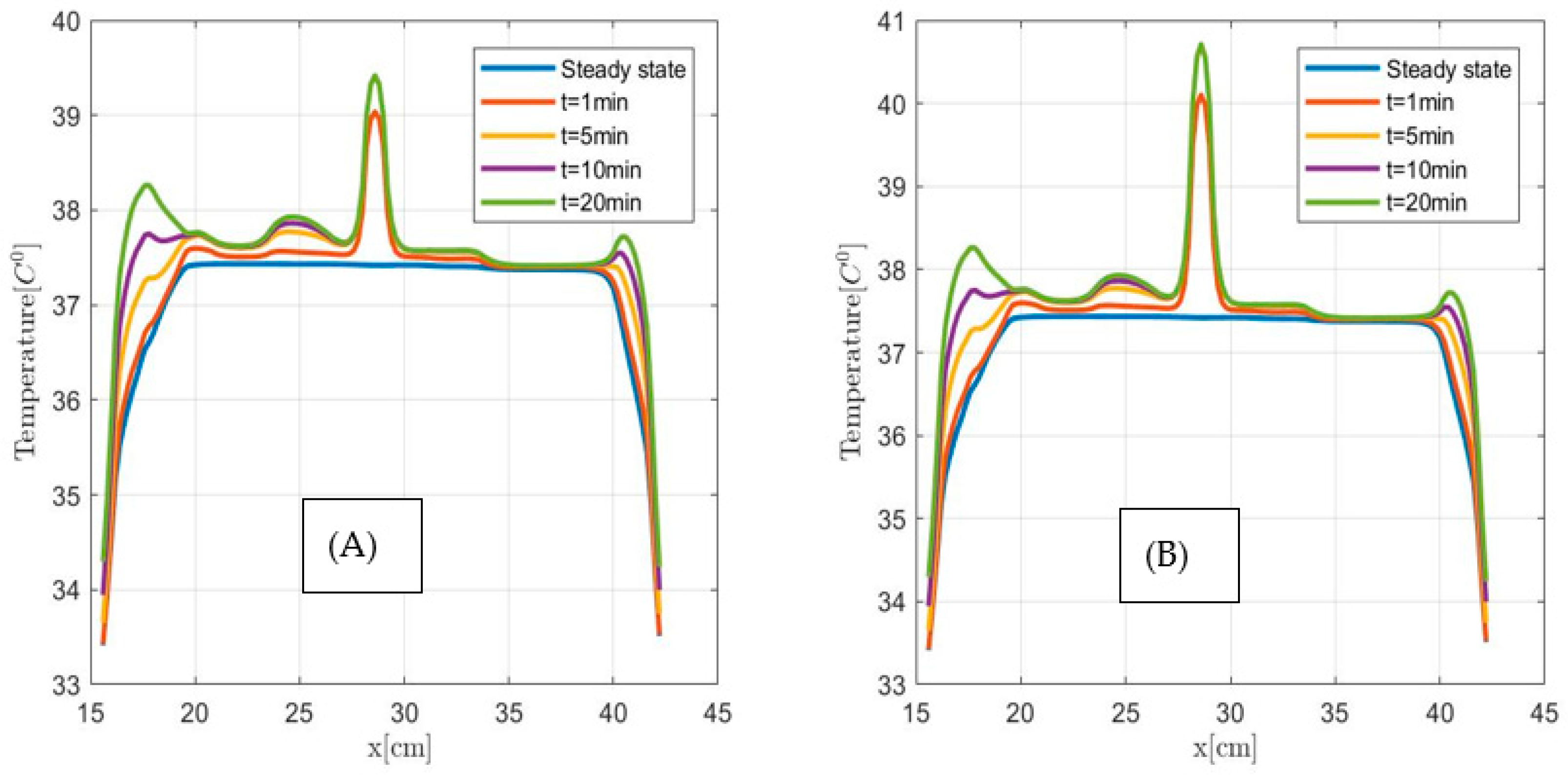

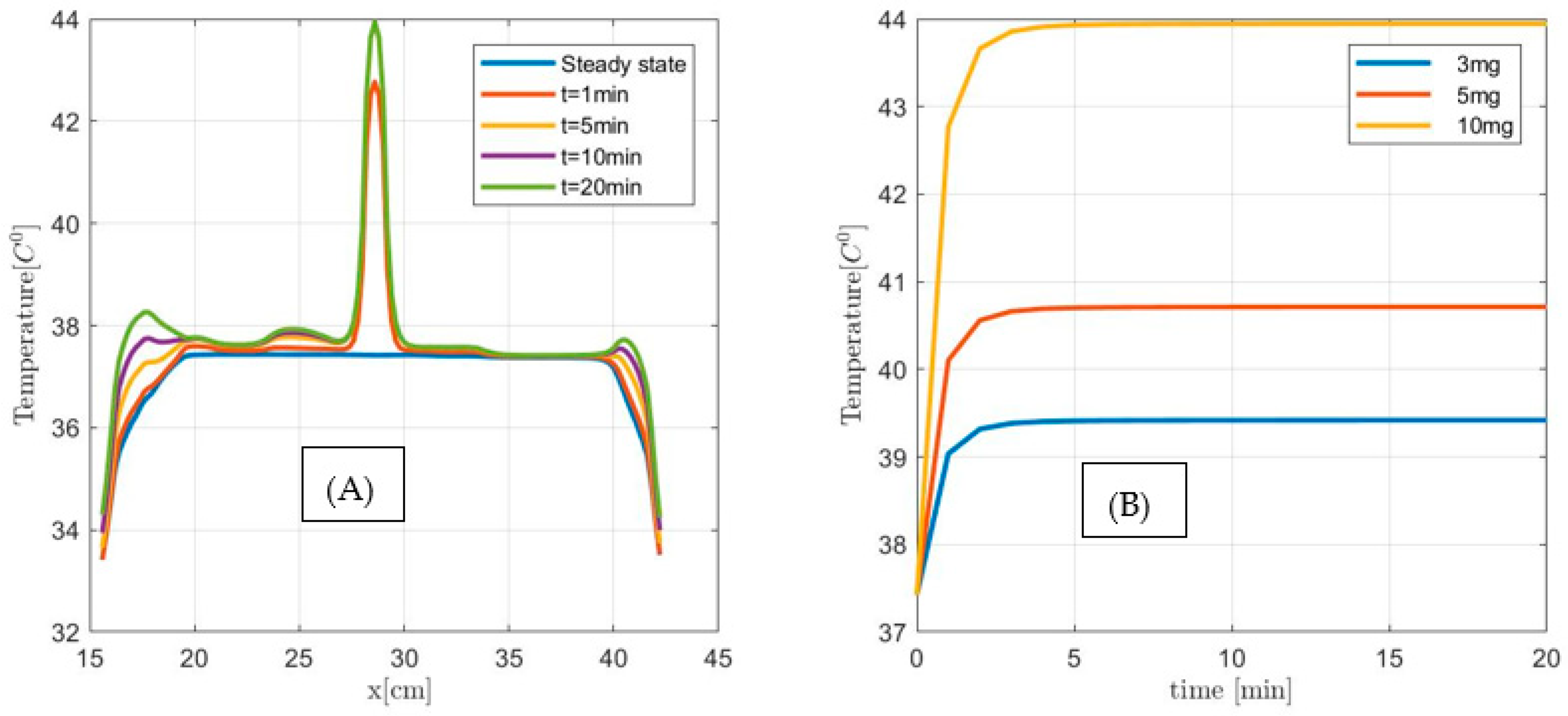
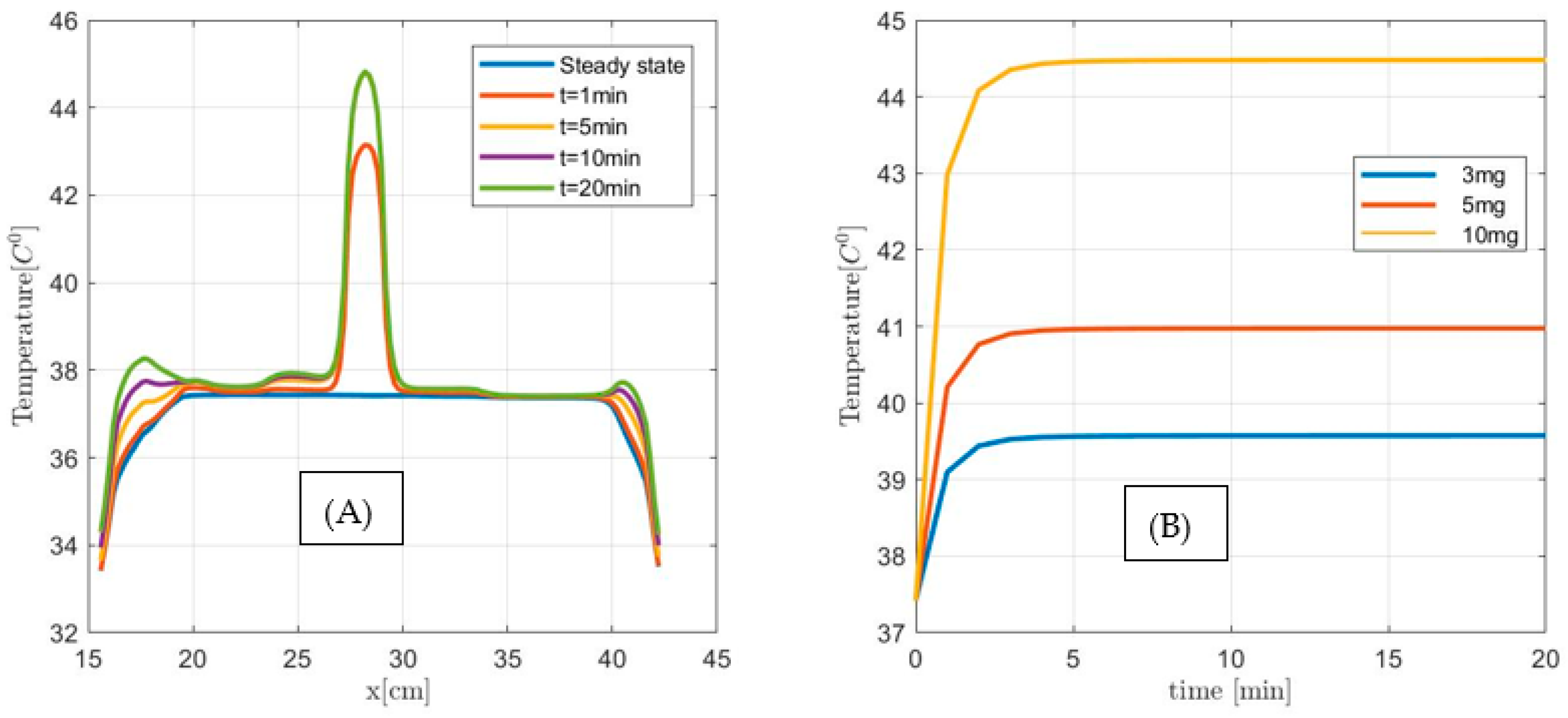
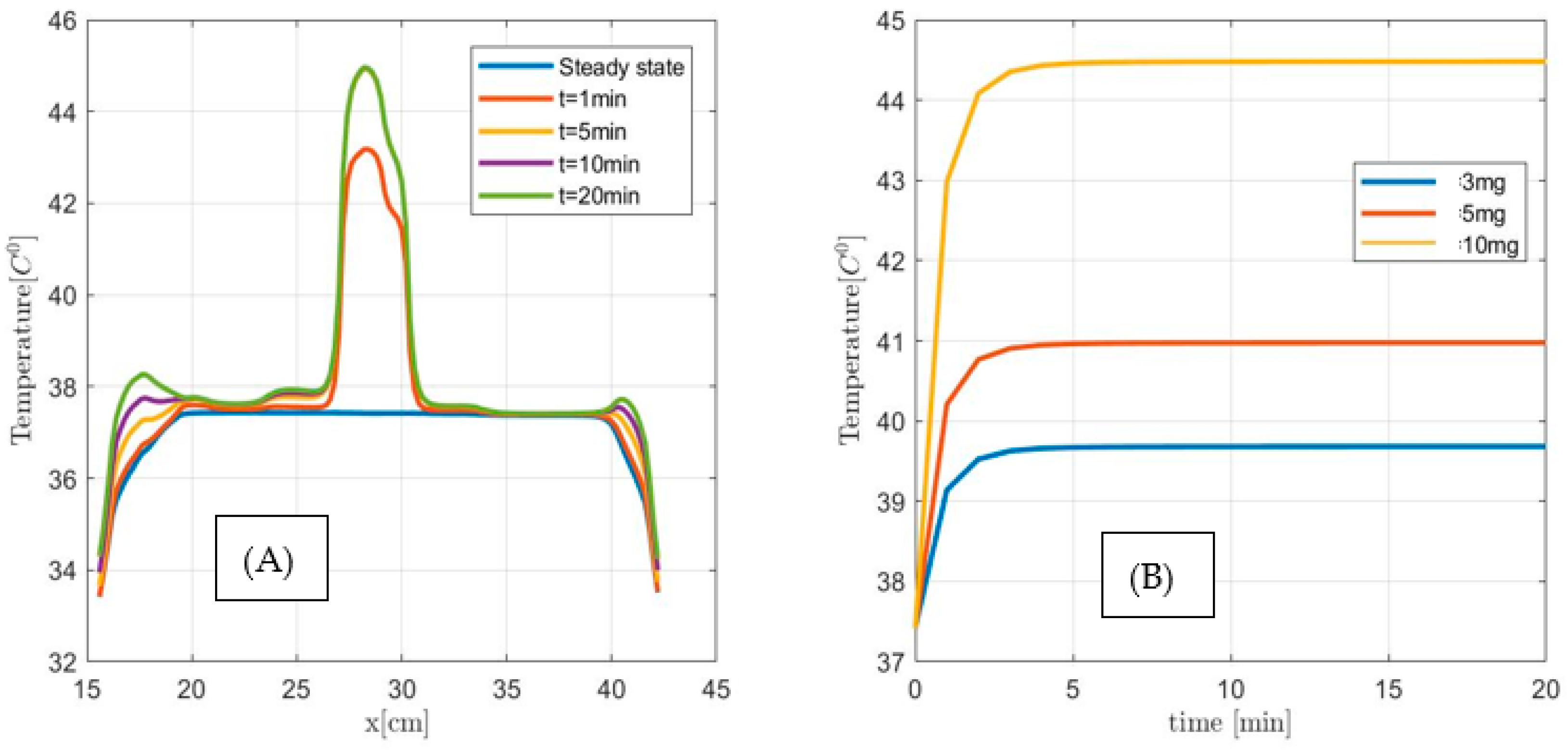
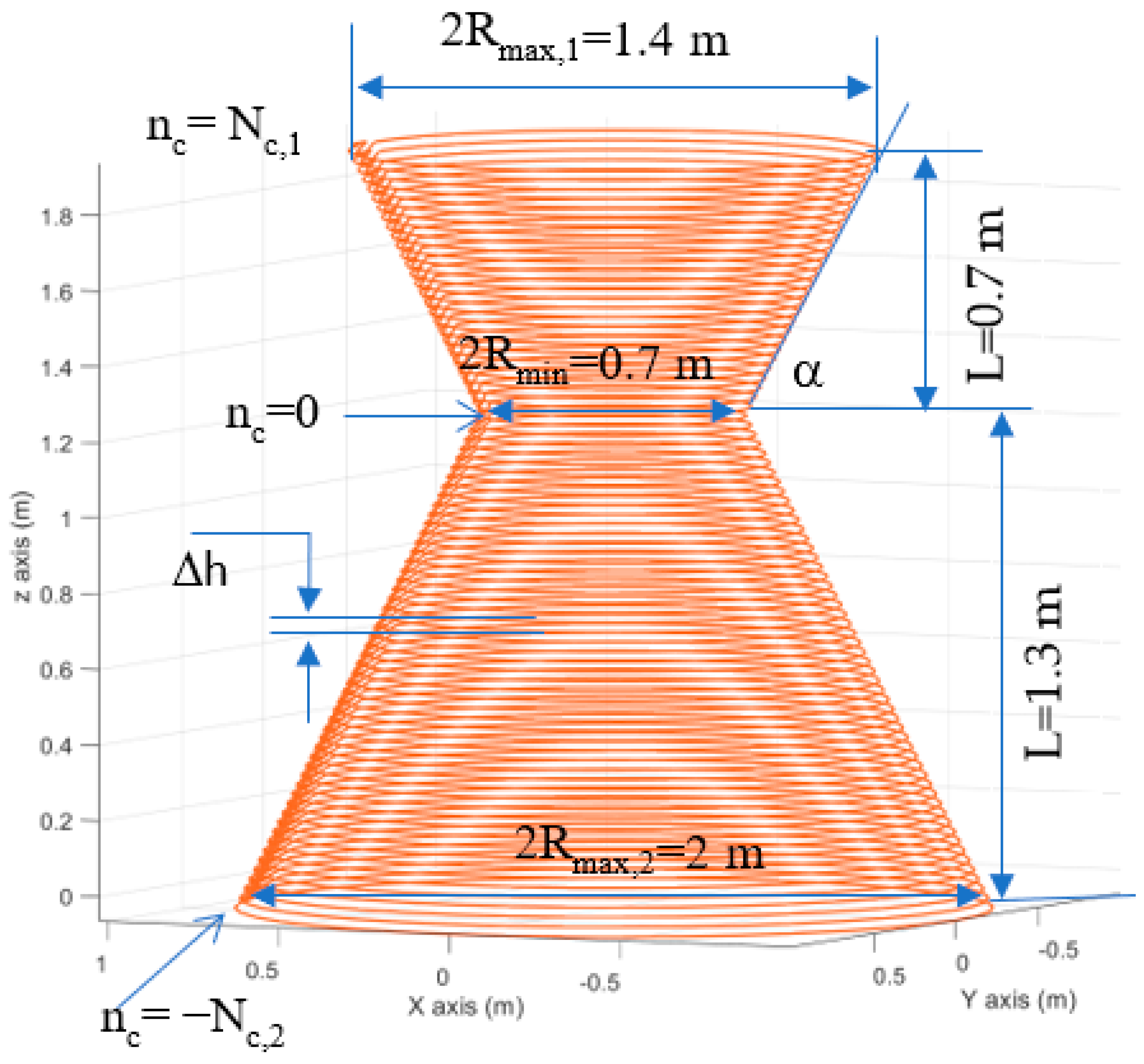
| Material | Electric Conductivity (S/m) | Density (kg/m3) | Heat Capacity (J/kg/°C) | Thermal Conductivity (W/m/°C) | Heat Generation Rate (W/kg) | Heat Transfer Rate 1.667 × 10−8 (m3/s/kg) |
|---|---|---|---|---|---|---|
| Air | 0 | 1 | 1000 | 0 | 0 | 0 |
| Adrenal gland | 0.620009 | 1028 | 3513 | 0.44 | 22.58 | 1458 |
| Air internal | 0 | 1 | 1004 | 0 | 0 | 0 |
| Artery | 0.705077 | 1102 | 3617 | 0.52 | 0 | 10,000 |
| Bladder | 0.219954 | 1086 | 3581 | 0.52 | 0 | 78 |
| Blood vessel | 0.705077 | 1060 | 3306 | 0.52 | 0 | 10,000 |
| Bone | 0.02089 | 1908 | 1313 | 0.32 | 0.15 | 10 |
| Brain grey matter | 0.136564 | 1045 | 3696 | 0.55 | 15.54 | 764 |
| Brain white matter | 0.083885 | 1041 | 3583 | 0.48 | 4.32 | 212 |
| Bronchi | 0.341515 | 1102 | 3306 | 0.46 | 3.69 | 238 |
| Bronchi_lumen | 0 | 1 | 1004 | 0 | 0 | 0 |
| Cartilage | 0.179826 | 1100 | 3568 | 0.49 | 0.54 | 35 |
| Cerebellum | 0.15662 | 1045 | 3653 | 0.51 | 15.67 | 770 |
| Cerebrospinal fluid | 2 | 1007 | 4096 | 0.57 | 0 | 0 |
| Commissura anterior | 0.083885 | 1041 | 3583 | 0.48 | 4.32 | 212 |
| Commissura posterior | 0.083885 | 1041 | 3583 | 0.48 | 4.32 | 212 |
| Connective tissue | 0.388886 | 1027 | 2372 | 0.39 | 0.58 | 37 |
| Cornea | 0.508027 | 1062 | 3615 | 0.54 | 0 | 38 |
| Diaphragm | 0.369328 | 1090 | 3421 | 0.49 | 2.44 | 99 |
| Ear cartilage | 0.179826 | 1100 | 3568 | 0.49 | 0.54 | 35 |
| Ear skin | 0.000628 | 1109 | 3391 | 0.37 | 1.65 | 106 |
| Epididymis | 0.441861 | 1082 | 3778 | 0.52 | 3.09 | 200 |
| Esophagus | 0.537457 | 1040 | 3500 | 0.53 | 2.94 | 190 |
| Esophagus lumen | 0 | 1 | 1004 | 0 | 0 | 0 |
| Eye lens | 0.200834 | 1076 | 3133 | 0.43 | 0 | 0 |
| Eye Sclera | 0.521255 | 1032 | 4200 | 0.58 | 5.89 | 380 |
| Eye vitreous humor | 1.50003 | 1005 | 4047 | 0.59 | 0 | 0 |
| Fat | 0.043458 | 911 | 2348 | 0.21 | 0.51 | 33 |
| Gallbladder | 0.900145 | 1071 | 3716 | 0.52 | 0.46 | 30 |
| Heart lumen | 0.705077 | 1050 | 3617 | 0.52 | 0 | 10,000 |
| Heart muscle | 0.224545 | 1081 | 3686 | 0.56 | 39.45 | 1026 |
| Hippocampus | 0.136564 | 1045 | 3696 | 0.55 | 15.54 | 764 |
| Hypophysis | 0.539026 | 1053 | 3687 | 0.51 | 13.71 | 885 |
| Hypothalamus | 0.136564 | 1045 | 3696 | 0.55 | 15.54 | 764 |
| Intervertebral disc | 0.830007 | 1100 | 3568 | 0.49 | 0.54 | 35 |
| Kidney cortex | 0.177821 | 1049 | 3587 | 0.53 | 18.43 | 3874 |
| Kidney medulla | 0.177821 | 1044 | 3745 | 0.54 | 2.85 | 599 |
| Large intestine | 0.25011 | 1088 | 3655 | 0.54 | 11.85 | 765 |
| Large intestine lumen | 0.369328 | 1045 | 3801 | 0.56 | 0 | 0 |
| Larynx | 0.179826 | 1100 | 3568 | 0.49 | 0.54 | 35 |
| Liver | 0.092243 | 1079 | 3540 | 0.52 | 9.93 | 860 |
| Lung | 0.109608 | 394 | 3886 | 0.39 | 6.21 | 401 |
| Mandible | 0.02089 | 1908 | 1313 | 0.32 | 0.15 | 10 |
| Marrow red | 0.10297 | 1029 | 2666 | 0.28 | 2.09 | 135 |
| Medulla oblongata | 0.15662 | 1046 | 3630 | 0.51 | 11.37 | 559 |
| Meniscus | 0.179826 | 1100 | 3568 | 0.49 | 0 | 35 |
| Midbrain | 0.15662 | 1046 | 3630 | 0.51 | 11.37 | 559 |
| Mucosa | 0.369328 | 1102 | 3150 | 0.34 | 9.19 | 594 |
| Muscle | 0.369328 | 1090 | 3421 | 0.49 | 0.91 | 37 |
| Nerve | 0.085742 | 1075 | 3613 | 0.49 | 2.48 | 160 |
| Pancreas | 0.539026 | 1087 | 3164 | 0.51 | 11.89 | 767 |
| Patella | 0.02089 | 1908 | 1313 | 0.32 | 0.15 | 10 |
| Penis | 0.319555 | 1102 | 3306 | 0.46 | 0.19 | 12 |
| Pharynx | 0 | 1 | 1004 | 0 | 0 | 0 |
| Pineal body | 0.539026 | 1053 | 3687 | 0.51 | 13.71 | 885 |
| Pons | 0.15662 | 1046 | 3630 | 0.51 | 11.37 | 559 |
| Prostate | 0.441861 | 1045 | 3760 | 0.51 | 6.1 | 394 |
| SAT | 0.043458 | 911 | 2348 | 0.21 | 0.51 | 33 |
| Skin | 0.000628 | 1109 | 3391 | 0.37 | 1.65 | 106 |
| Skull | 0.02089 | 1908 | 1313 | 0.32 | 0.15 | 10 |
| Small intestine | 0.603279 | 1030 | 3595 | 0.49 | 15.89 | 1026 |
| Small intestine lumen | 0.369328 | 1045 | 3801 | 0.56 | 0 | 0 |
| Spinal cord | 0.085742 | 1075 | 3630 | 0.51 | 2.48 | 160 |
| Spleen | 0.124573 | 1089 | 3596 | 0.53 | 24.11 | 1557 |
| Stomach | 0.537457 | 1088 | 3690 | 0.53 | 7.13 | 460 |
| Stomach lumen | 0.369328 | 1045 | 3801 | 0.56 | 0 | 0 |
| Teeth | 0.02089 | 2180 | 1255 | 0.59 | 0 | 0 |
| Tendon ligament | 0.388886 | 1142 | 3432 | 0.47 | 0.45 | 29 |
| Testis | 0.441861 | 1082 | 3778 | 0.52 | 3.09 | 200 |
| Thalamus | 0.136564 | 1045 | 3696 | 0.55 | 13.93 | 685 |
| Thymus | 0.630011 | 1023 | 3043 | 0.34 | 3.83 | 247 |
| Thyroid gland | 0.539026 | 1050 | 3609 | 0.52 | 87.1 | 5634 |
| Tongue | 0.290727 | 1090 | 3421 | 0.49 | 1.21 | 78 |
| Trachea | 0.341515 | 1080 | 3568 | 0.49 | 0.54 | 35 |
| Trachea lumen | 0 | 1 | 1004 | 0 | 0 | 0 |
| Ureter urethra | 0.319555 | 1102 | 3306 | 0.46 | 2.91 | 188 |
| Vein | 0.705077 | 1050 | 3617 | 0.52 | 0 | 10,000 |
| Vertebrae | 0.02089 | 1908 | 1313 | 0.32 | 0.15 | 10 |
| Tumor | 0.539026 | 1087 | 3164 | 0.51 | 11.89 | 767 |
| Coil Type | a Radius [cm] | N (Number of Turns) | SARJouleMax [W/kg] | I [A] | |||
|---|---|---|---|---|---|---|---|
| Helmholtz symmetric respect x-axis | 60 | 1 | 22,582 | 10,000 | |||
| Helmholtz symmetric respect z-axis | 60 | 1 | 5773 | 9900 | |||
| Inner layer | Outer layer | ||||||
| Double layer solenoid | amin = 35 | amax = 37.5 | 164 | 70 | 156 | ||
| Double layer biconical | amin = 35 | amax = 70 | amin = 37.5 | amax = 72.5 | 164 | 42 | 133 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shoshiashvili, L.; Shamatava, I.; Kakulia, D.; Shubitidze, F. Design and Assessment of a Novel Biconical Human-Sized Alternating Magnetic Field Coil for MNP Hyperthermia Treatment of Deep-Seated Cancer. Cancers 2023, 15, 1672. https://doi.org/10.3390/cancers15061672
Shoshiashvili L, Shamatava I, Kakulia D, Shubitidze F. Design and Assessment of a Novel Biconical Human-Sized Alternating Magnetic Field Coil for MNP Hyperthermia Treatment of Deep-Seated Cancer. Cancers. 2023; 15(6):1672. https://doi.org/10.3390/cancers15061672
Chicago/Turabian StyleShoshiashvili, Levan, Irma Shamatava, David Kakulia, and Fridon Shubitidze. 2023. "Design and Assessment of a Novel Biconical Human-Sized Alternating Magnetic Field Coil for MNP Hyperthermia Treatment of Deep-Seated Cancer" Cancers 15, no. 6: 1672. https://doi.org/10.3390/cancers15061672
APA StyleShoshiashvili, L., Shamatava, I., Kakulia, D., & Shubitidze, F. (2023). Design and Assessment of a Novel Biconical Human-Sized Alternating Magnetic Field Coil for MNP Hyperthermia Treatment of Deep-Seated Cancer. Cancers, 15(6), 1672. https://doi.org/10.3390/cancers15061672






