Radiofrequency Electromagnetic Fields Cause Non-Temperature-Induced Physical and Biological Effects in Cancer Cells
Abstract
Simple Summary
Abstract
1. Introduction
- So far, no reproducible measurement method has been established that can clearly detect differences in the power deposition in cells exposed either to RF or AMRF.
- There is a lack of irrefutable in vitro and in vivo experiments that compare WB heating with RF or AMRF and document additional RF and/or AMRF-induced anticancer effects. A typical knockout argument is the assertion of hidden thermal effects (local temperature rises, so-called hot spots). Therefore, such confounders must be methodically excluded using a thorough analysis of the experimental setup.
2. Materials and Methods
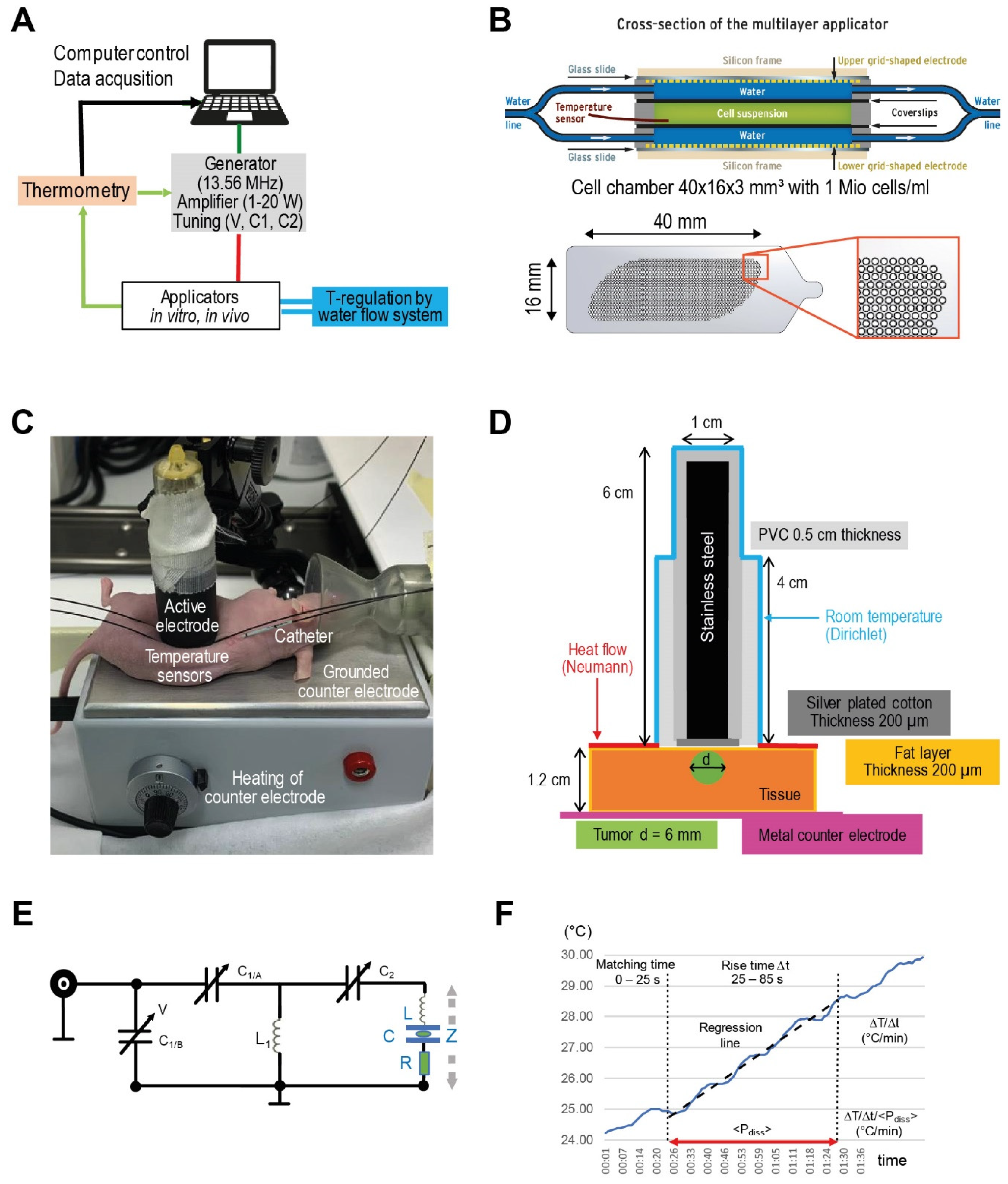
2.1. Experimental System, Applicators, and Physical Parameters
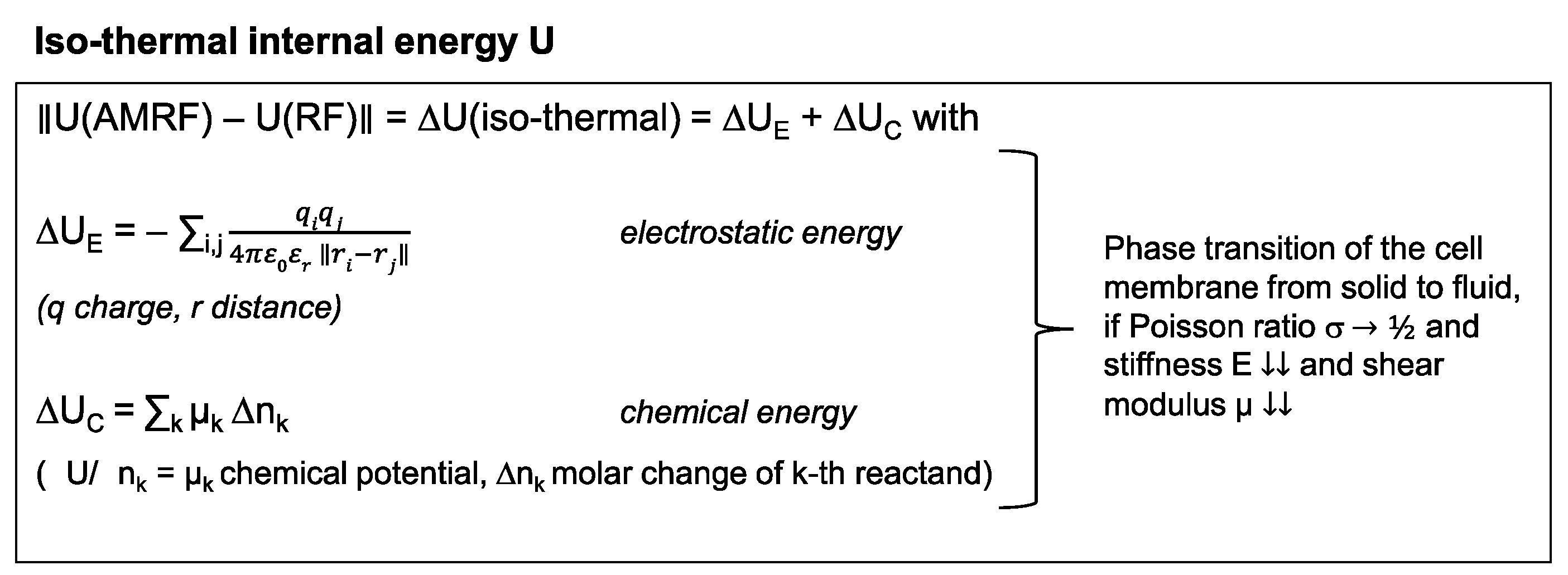
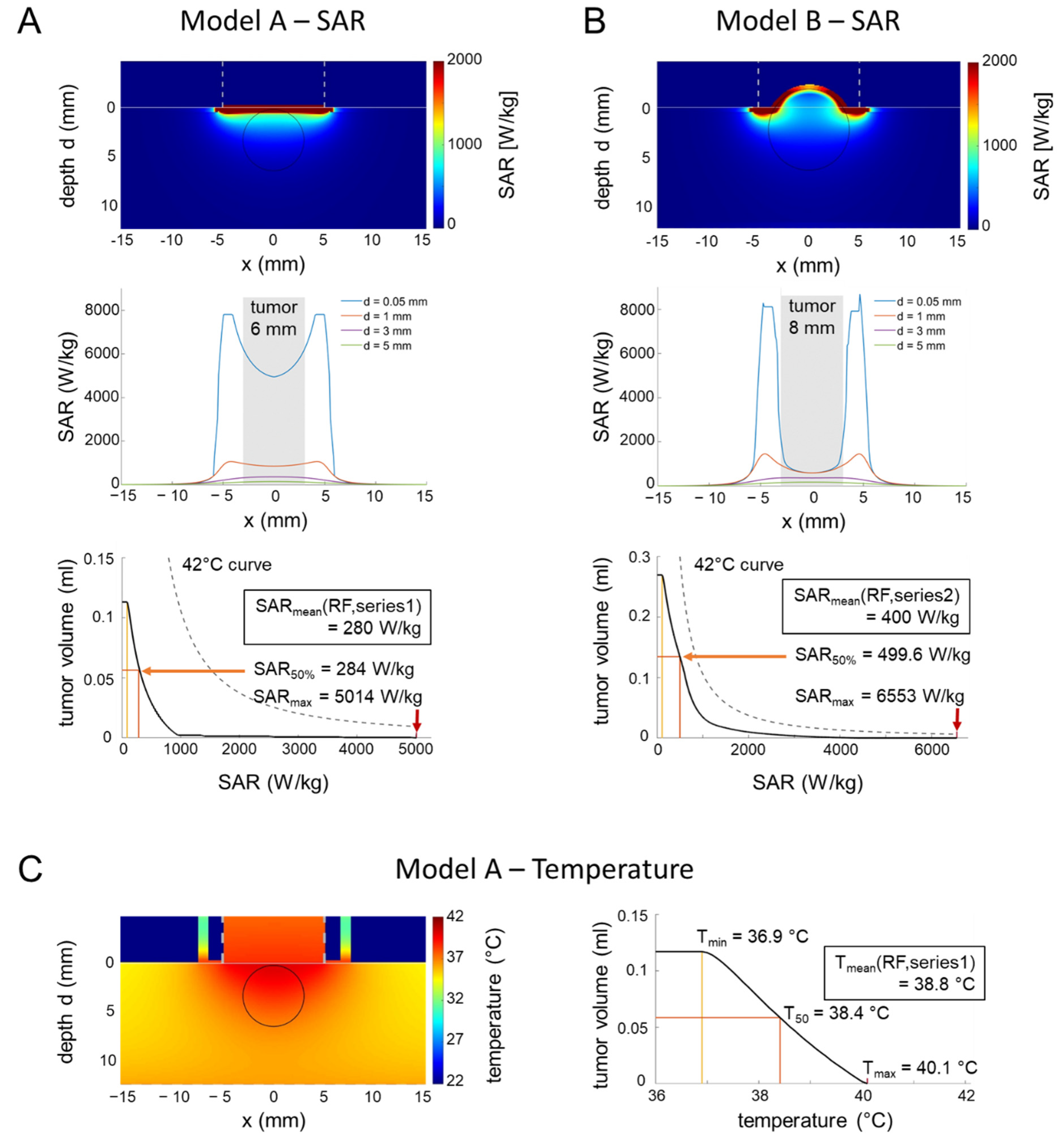
2.2. Applicator Models, Simulation Studies, and Isothermal Energy
2.3. In Vitro Studies
2.4. In Vivo Study
2.5. Statistics
3. Results
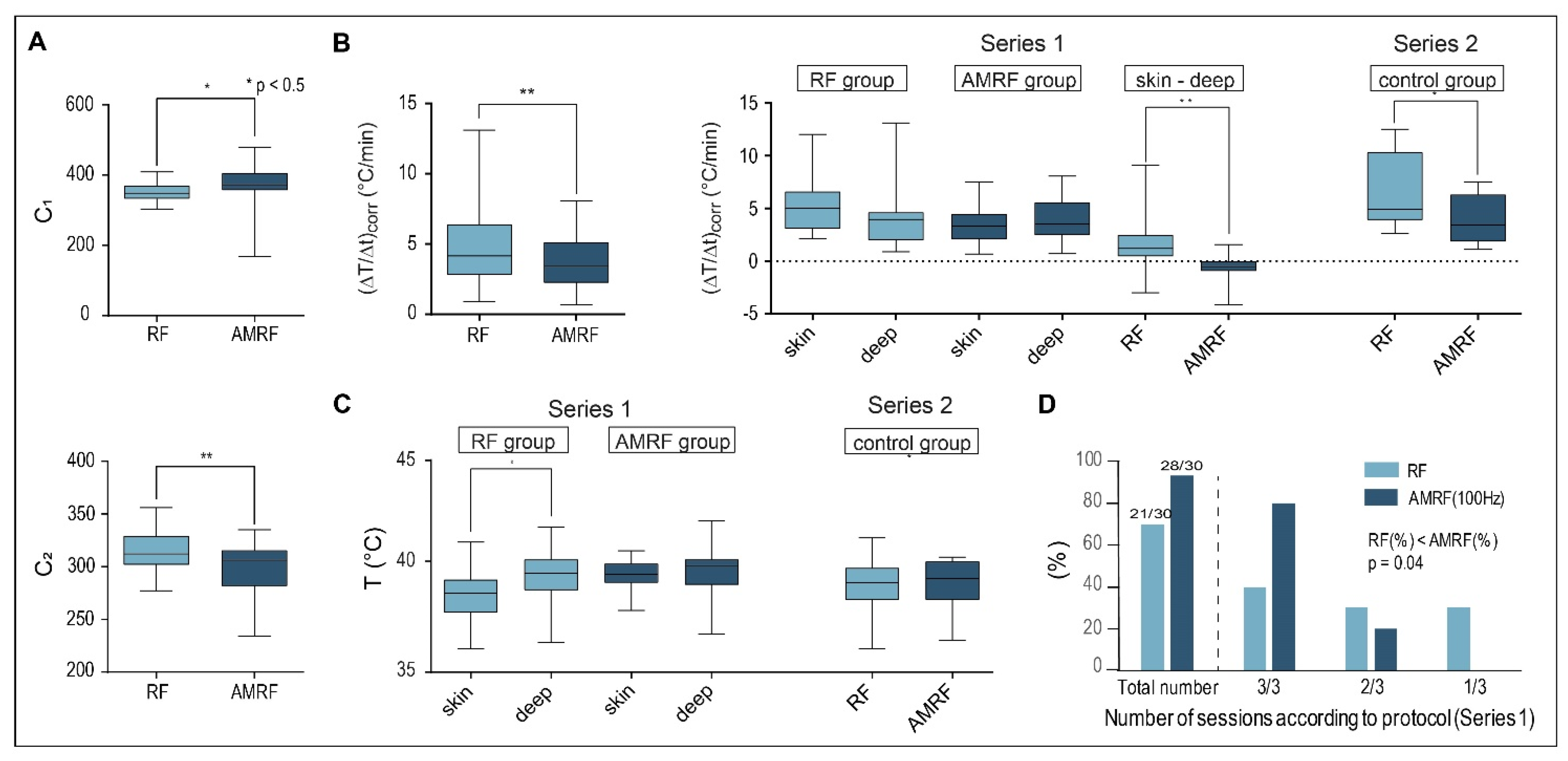
3.1. Physical Parameters (In Vitro Applicator)
3.2. Biological Effectiveness of RF and AMRF Versus WB In Vitro
3.3. Biological Effectiveness of RF and AMRF Versus WB In Vivo
3.4. Physical Parameters (In Vivo Applicator)
3.5. Measured and Simulated Temperatures for the Applicators
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wust, P.; Hildebrandt, B.; Sreenivasa, G.; Rau, B.; Gellermann, J.; Riess, H.; Felix, R.; Schlag, P.M. Hyperthermia in combined treatment of cancer. Lancet. Oncol. 2002, 3, 487–497. [Google Scholar] [CrossRef]
- Issels, R.D.; Lindner, L.H.; Verweij, J.; Wessalowski, R.; Reichardt, P.; Wust, P.; Ghadjar, P.; Hohenberger, P.; Angele, M.; Salat, C.; et al. European Organization for the Research and Treatment of Cancer-Soft Tissue and Bone Sarcoma Group and the European Society for Hyperthermic Oncology Effect of neoadjuvant chemotherapy plus regional hyperthermia on long-term outcomes among patients with localized high-risk soft tissue sarcoma: The EORTC 62961-ESHO 95 randomized clinical trial. JAMA Oncology 2018, 4, 483–492. [Google Scholar] [PubMed]
- Van Der Zee, J.; González González, D.; Van Rhoon, G.C.; van Dijk, J.D.; van Putten, W.L.; Hart, A.A. Comparison of radiotherapy alone with radiotherapy plus hyperthermia in locally advanced pelvic tumours: A prospective, randomised, multicentre trial. Dutch Deep Hyperthermia Group. Lancet 2000, 355, 1119–1125. [Google Scholar] [CrossRef]
- Harima, Y.; Nagata, K.; Harima, K.; Ostapenko, V.V.; Tanaka, Y.; Sawada, S. A randomized clinical trial of radiation therapy versus thermoradiotherapy in stage IIIB cervical carcinoma. Int. J. Hyperth. 2001, 17, 97–105. [Google Scholar] [CrossRef]
- Ademaj, A.; Veltsista, D.P.; Ghadjar, P.; Marder, D.; Oberacker, E.; Ott, O.J.; Wust, P.; Puric, E.; Hälg, R.A.; Rogers, S.; et al. Clinical evidence for thermometric parameters to guide hyperthermia treatment. Cancers 2022, 14, 625. [Google Scholar] [CrossRef]
- Mei, X.; Ten Cate, R.; van Leeuwen, C.M.; Rodermond, H.M.; de Leeuw, L.; Dimitrakopoulou, D.; Stalpers, L.J.A.; Crezee, J.; Kok, H.P.; Franken, N.A.P.; et al. Radiosensitization by hyperthermia: The effects of temperature, sequence, and time interval in cervical cell lines. Cancers 2020, 12, 582. [Google Scholar] [CrossRef]
- Kim, S.; Lee, J.H.; Cha, J.; You, S.H. Beneficial effects of modulated electro-hyperthermia during neoadjuvant treatment for locally advanced rectal cancer. Int. J. Hyperth. 2021, 38, 144–151. [Google Scholar] [CrossRef]
- Minnaar, C.A.; Kotzen, J.A.; Ayeni, O.A.; Naidoo, T.; Tunmer, M.; Sharma, V.; Vangu, M.D.; Baeyens, A. The effect of modulated electro-hyperthermia on local disease control in HIV-positive and -negative cervical cancer women in South Africa: Early results from a phase III randomised controlled trial. PLoS ONE 2019, 14, e0217894. [Google Scholar] [CrossRef]
- Minnaar, C.A.; Maposa, I.; Kotzen, J.A.; Baeyens, A. Effects of modulated electro-hyperthermia (mEHT) on two and three year survival of locally advanced cervical cancer patients. Cancers 2022, 14, 656. [Google Scholar] [CrossRef]
- Barbault, A.; Costa, F.P.; Bottger, B.; Munden, R.F.; Bomholt, F.; Kuster, N.; Pasche, B. Amplitude-modulated electromagnetic fields for the treatment of cancer: Discovery of tumor-specific frequencies and assessment of a novel therapeutic approach. J. of Exp. Clin. Cancer Res. 2009, 28, 51. [Google Scholar] [CrossRef]
- Costa, F.P.; De Oliveira, A.C.; Meirelles, R.; Machado, M.C.; Zanesco, T.; Surjan, R.; Chammas, M.C.; de Souza Rocha, M.; Morgan, D.; Cantor, A.; et al. Treatment of advanced hepatocellular carcinoma with very low levels of amplitude-modulated electromagnetic fields. Br. J. Cancer 2011, 105, 640–648. [Google Scholar] [CrossRef] [PubMed]
- Wust, P.; Stein, U.; Ghadjar, P. Non-thermal membrane effects of electromagnetic fields and therapeutic applications in oncology. Int. J. Hyperth. 2021, 38, 715–731. [Google Scholar] [CrossRef] [PubMed]
- Wust, P.; Kortüm, B.; Strauss, U.; Nadobny, J.; Zschaeck, S.; Beck, M.; Stein, U.; Ghadjar, P. Non-thermal effects of radiofrequency electromagnetic fields. Sci. Rep. Sci. Rep. 2020, 10, 13488. [Google Scholar] [CrossRef] [PubMed]
- Bawin, S.M.; Kaczmarek, L.K.; Adey, W.R. Effects of modulated VHF fields on the central nervous system. Ann. N. Y. Acad. Sci. 1975, 247, 74–81. [Google Scholar] [CrossRef] [PubMed]
- Blackman, C.F.; Elder, J.A.; Weil, C.M.; Benane, S.G.; Eichinger, D.C.; House, D.E. Induction of calcium-ion efflux from brain tissue by radio-frequency radiation: Effects of modulation frequency and field strength. Radio Sci. 1979, 14, 93–98. [Google Scholar] [CrossRef]
- Blackman, C.F.; Benane, S.G.; Elder, J.A.; House, D.E.; Lampe, J.A.; Faulk, J.M. Induction of calcium-ion efflux from brain tissue by radiofrequency radiation: Effect of sample number and modulation frequency on the power-density window. Bioelectromagnetics 1980, 1, 35–43. [Google Scholar] [CrossRef]
- Diem, E.; Schwarz, C.; Adlkofer, F.; Jahn, O.; Rüdiger, H. Non-thermal DNA breakage by mobile-phone radiation (1800 MHz) in human fibroblasts and in transformed GFSH-R17 rat granulosa cells in vitro. Mutat. Res. 2005, 583, 178–183. [Google Scholar] [CrossRef]
- Panagopoulos, D.J.; Chavdoula, E.D.; Karabarbounis, A.; Margaritis, L.H. Comparison of bioactivity between GSM 900 MHz and DCS 1800 MHz mobile telephony radiation. Electromagn. Biol. Med. 2007, 26, 33–44. [Google Scholar] [CrossRef]
- Franzellitti, S.; Valbonesi, P.; Ciancaglini, N.; Biondi, C.; Contin, A.; Bersani, F.; Fabbri, E. Transient DNA damage induced by high-frequency electromagnetic fields (GSM 1.8GHz) in the human trophoblast HTR-8/SVneo cell line evaluated with the alkaline comet assay. Mutat. Res. 2010, 683, 35–42. [Google Scholar] [CrossRef]
- Szasz, A.; Szasz, O.; Iluri, N. Radiofrequency hyperthermia device with targeted feedback signal modulation. United States Patent US 9(320), 911 B2 (2016).
- Yang, K.L.; Huang, C.C.; Chi, M.S.; Chiang, H.C.; Wang, Y.S.; Hsia, C.C.; Andocs, G.; Wang, H.E.; Chi, K.H. In vitro comparison of conventional hyperthermia and modulated electro-hyperthermia. Oncotarget 2016, 7, 84082–84092. [Google Scholar] [CrossRef]
- Danics, L.; Schvarcz, C.A.; Viana, P.; Vancsik, T.; Krenács, T.; Benyó, Z.; Kaucsár, T.; Hamar, P. Exhaustion of protective heat shock response 2 induces significant tumor damage by apoptosis after modulated electro-hyperthermia treatment of triple negative breast cancer isografts in mice. Cancers 2020, 12, 2581. [Google Scholar] [CrossRef] [PubMed]
- Andocs, G.; Renner, H.; Balogh, L.; Fonyad, L.; Jakab, C.; Szasz, A. Strong synergy of heat and modulated electromagnetic field in tumor cell killing. Strahlenther. Onkol. Organ Der Deutsch. Rontgenges. 2009, 185, 120–126. [Google Scholar] [CrossRef]
- Wust, P.; Stahl, H.; Löffel, J.; Seebass, M.; Riess, H.; Felix, R. Clinical, physiological and anatomical determinants for radiofrequency hyperthermia. Int. J. Hyperther. 1995, 11, 151–167. [Google Scholar] [CrossRef] [PubMed]
- Wust, P.; Ghadjar, P.; Nadobny, J.; Beck, M.; Kaul, D.; Winter, L.; Zschaeck, S. Physical analysis of temperature-dependent effects of amplitude-modulated electromagnetic hyperthermia. Int. J. Hyperther. 2019, 36, 1246–1254. [Google Scholar] [CrossRef] [PubMed]
- Wust, P.; Veltsista, P.D.; Oberacker, E.; Ghadjar, P. (Department of Radiation Oncology, Charité—Universitätsmedizin Berlin, Augustenburger Platz 1, 13353 Berlin, Germany). Personal Observation, 2021.
- Wust, P.; Cho, C.H.; Hildebrandt, B.; Gellermann, J. Thermal monitoring: Invasive, minimal-invasive and non-invasive approaches. Int. J. Hyperther. 2006, 22, 255–262. [Google Scholar] [CrossRef] [PubMed]
- Gordon, C.J. The mouse thermoregulatory system: Its impact on translating biomedical data to humans. Physiol. Behav. 2017, 179, 55–66. [Google Scholar] [CrossRef]
- Streif, J.U.; Hiller, K.H.; Waller, C.; Nahrendorf, M.; Wiesmann, F.; Bauer, W.R.; Rommel, E.; Haase, A. In vivo assessment of absolute perfusion in the murine skeletal muscle with spin labeling MRI. Magnetic resonance imaging. J. Magn. Resonance Imaging 2003, 17, 147–152. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Theory of Elasticity; Pergamon Press: Oxford, UK, 1986. [Google Scholar]
- Schwan, H.P. Electrical properties of tissue and cell suspensions. Adv. Biol. Med. Phys. 1957, 5, 147–209. [Google Scholar]
- Martinsen, O.G.; Grimnes, S.; Schwan, H.P. Interface phenomena and dielectric properties of biological tissue. Encycl. Surf. Colloid Sci. 2002, 20, 2643–2653. [Google Scholar]
- Stoy, R.D.; Foster, K.R.; Schwan, H.P. Dielectric properties of mammalian tissues from 0.1 to 100 MHz: A summary of recent data. Phys. Med. Biol. 1982, 27, 501–513. [Google Scholar] [CrossRef]
- Weibel, E.R.; Stäubli, W.; Gnägi, H.R.; Hess, F.A. Correlated morphometric and biochemical studies on the liver cell. I. Morphometric model, stereologic methods, and normal morphometric data for rat liver. J. Cell Biol. 1969, 42, 68–91. [Google Scholar] [CrossRef] [PubMed]
- Beck, M.; Wust, P.; Oberacker, E.; Rattunde, A.; Päßler, T.; Chrzon, B.; Veltsista, P.D.; Nadobny, J.; Pellicer, R.; Herz, E.; et al. Experimental and computational evaluation of capacitive hyperthermia. Int. J. Hyperther. 2022, 39, 504–516. [Google Scholar] [CrossRef] [PubMed]
- Jimenez, H.; Wang, M.; Zimmerman, J.W.; Pennison, M.J.; Sharma, S.; Surratt, T.; Xu, Z.X.; Brezovich, I.; Absher, D.; Myers, R.M.; et al. Tumour-specific amplitude-modulated radiofrequency electromagnetic fields induce differentiation of hepatocellular carcinoma via targeting Cav3.2 T-type voltage-gated calcium channels and Ca2+ influx. EBiomedicine 2019, 44, 209–224. [Google Scholar] [CrossRef] [PubMed]
- Sharma, S.; Wu, S.Y.; Jimenez, H.; Xing, F.; Zhu, D.; Liu, Y.; Wu, K.; Tyagi, A.; Zhao, D.; Lo, H.; et al. Ca2+ and CACNA1H mediate targeted suppression of breast cancer brain metastasis by AM RF EMF. EBioMedicine 2019, 44, 194–208. [Google Scholar] [CrossRef]
- Zimmerman, J.W.; Pennison, M.J.; Brezovich, I.; Yi, N.; Yang, C.T.; Ramaker, R.; Absher, D.; Myers, R.M.; Kuster, N.; Costa, F.P.; et al. Cancer cell proliferation is inhibited by specific modulation frequencies. Br. J. Cancer 2012, 106, 307–313. [Google Scholar] [CrossRef]



| (A) | |||
| Electrical Parameters | In Vitro Applicator | In Vivo Applicator | |
| electrodes upper electrode counter electrode | grid-shaped electrodes (steel) 4 × 1.6 × 0.75 cm2 electrode area reduced by 0.75 | stainless steel ø 1 = 1 cm, h = 6 cm ø 2 = (ø 12 + d2)1/2 | |
| distance d (cm) between electrodes | 1.3 | 1.2 (mouse) | |
| RPMI-Medium between electrodes | εr = 72.5, σ = 1.2 S/m | εr = 100 (2/3 medium) εr = 79 (phantom) | |
| additional interfaces | coverslips, polyolefin 2 × 0.2 mm, εr = 2 | silver plated cotton 0.2 mm, εr = 2 | |
| (B) | |||
| Thermal Parameters | In Vivo Applicator | ||
| Thermal conductivity λ (W/m/°C) | Electrode (steel): 20 Cover (silver plated cotton): 90 Tissue (mixture muscle/fat): 0.5 | ||
| Heat capacitance c (Ws/kg/°C) | Electrode (steel): 420 Tissue: 3600 | ||
| Heat transfer HTC (W/m2/°C) | Mouse body: 6 | ||
| Perfusion (mL/100 g/min) | Tissue: 100 | ||
| Heat generation (W/kg) | Tissue: 10 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wust, P.; Veltsista, P.D.; Oberacker, E.; Yavvari, P.; Walther, W.; Bengtsson, O.; Sterner-Kock, A.; Weinhart, M.; Heyd, F.; Grabowski, P.; et al. Radiofrequency Electromagnetic Fields Cause Non-Temperature-Induced Physical and Biological Effects in Cancer Cells. Cancers 2022, 14, 5349. https://doi.org/10.3390/cancers14215349
Wust P, Veltsista PD, Oberacker E, Yavvari P, Walther W, Bengtsson O, Sterner-Kock A, Weinhart M, Heyd F, Grabowski P, et al. Radiofrequency Electromagnetic Fields Cause Non-Temperature-Induced Physical and Biological Effects in Cancer Cells. Cancers. 2022; 14(21):5349. https://doi.org/10.3390/cancers14215349
Chicago/Turabian StyleWust, Peter, Paraskevi D. Veltsista, Eva Oberacker, Prabhusrinivas Yavvari, Wolfgang Walther, Olof Bengtsson, Anja Sterner-Kock, Marie Weinhart, Florian Heyd, Patricia Grabowski, and et al. 2022. "Radiofrequency Electromagnetic Fields Cause Non-Temperature-Induced Physical and Biological Effects in Cancer Cells" Cancers 14, no. 21: 5349. https://doi.org/10.3390/cancers14215349
APA StyleWust, P., Veltsista, P. D., Oberacker, E., Yavvari, P., Walther, W., Bengtsson, O., Sterner-Kock, A., Weinhart, M., Heyd, F., Grabowski, P., Stintzing, S., Heinrich, W., Stein, U., & Ghadjar, P. (2022). Radiofrequency Electromagnetic Fields Cause Non-Temperature-Induced Physical and Biological Effects in Cancer Cells. Cancers, 14(21), 5349. https://doi.org/10.3390/cancers14215349








