Directional Fluidity of Dense Emulsion Activated by Transverse Wedge-Shaped Microroughness
Abstract
1. Introduction
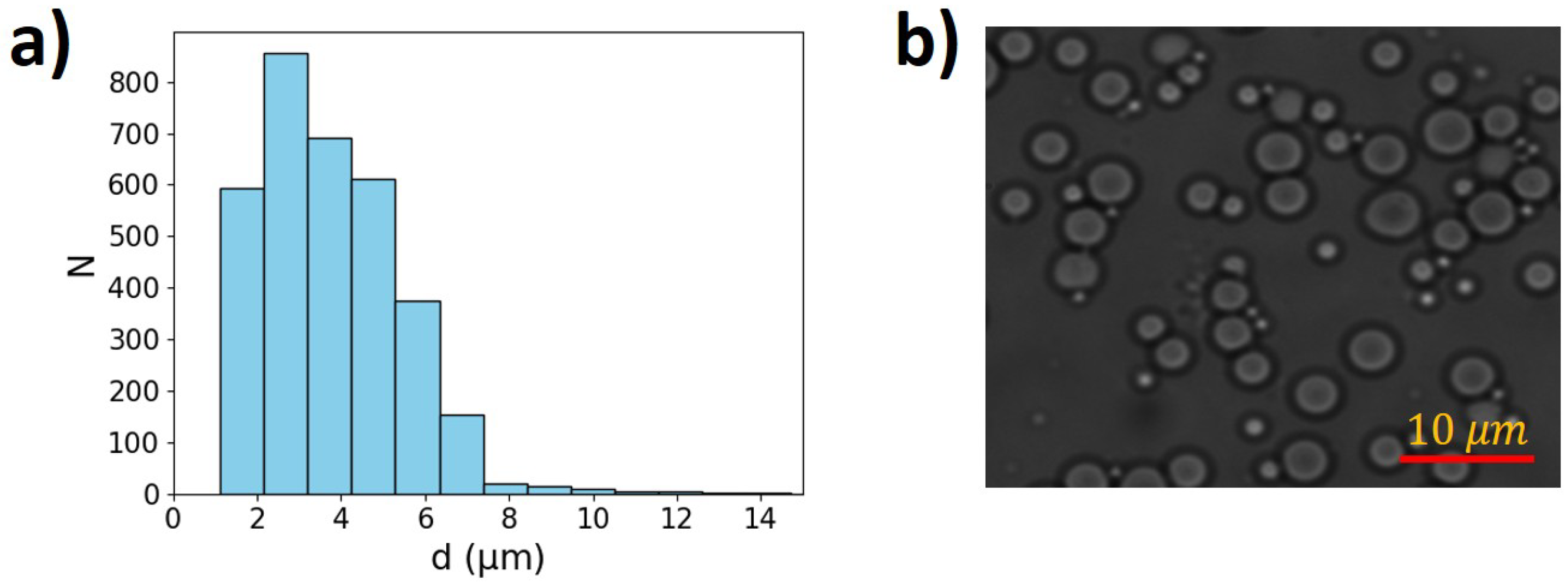
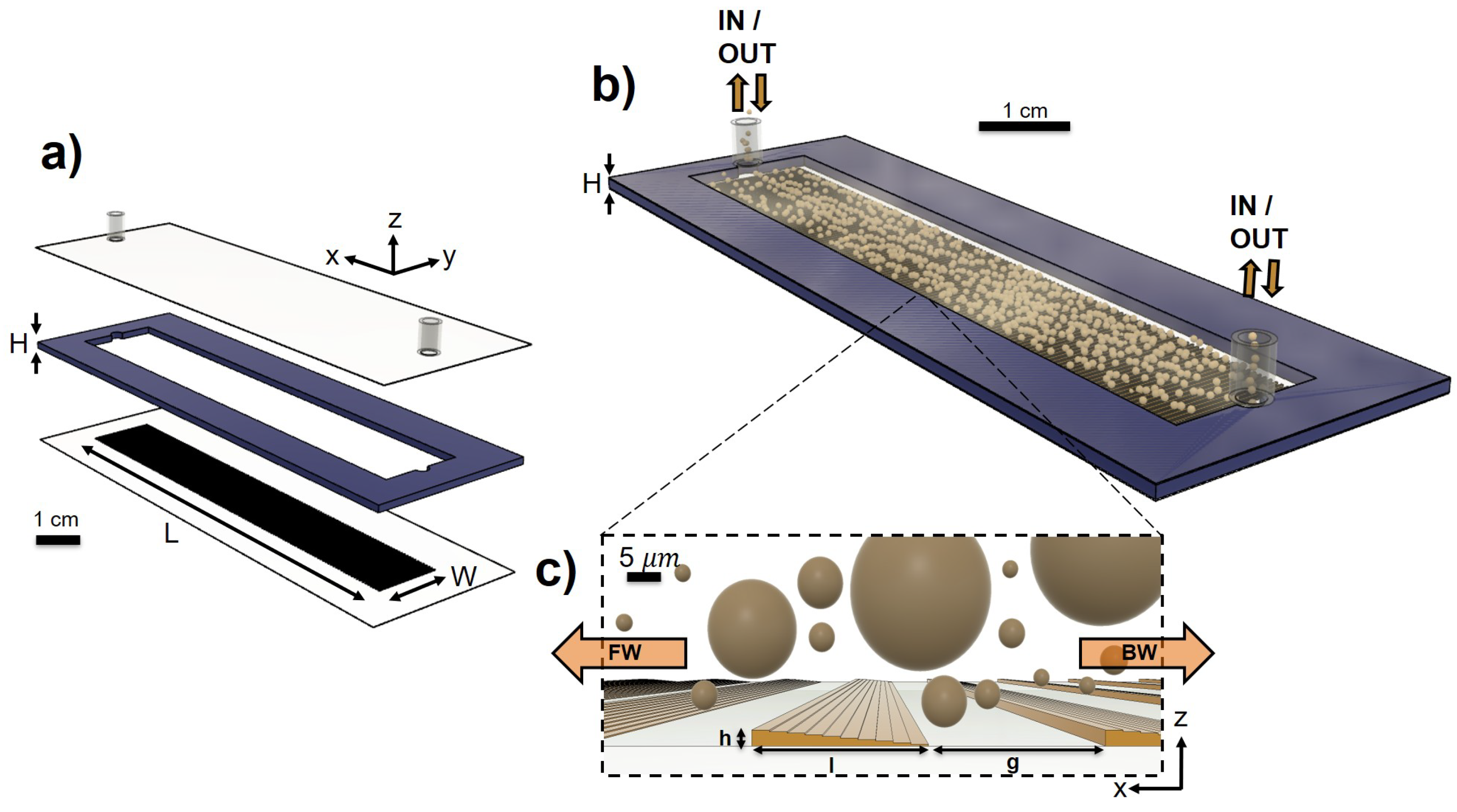
2. Materials and Methods
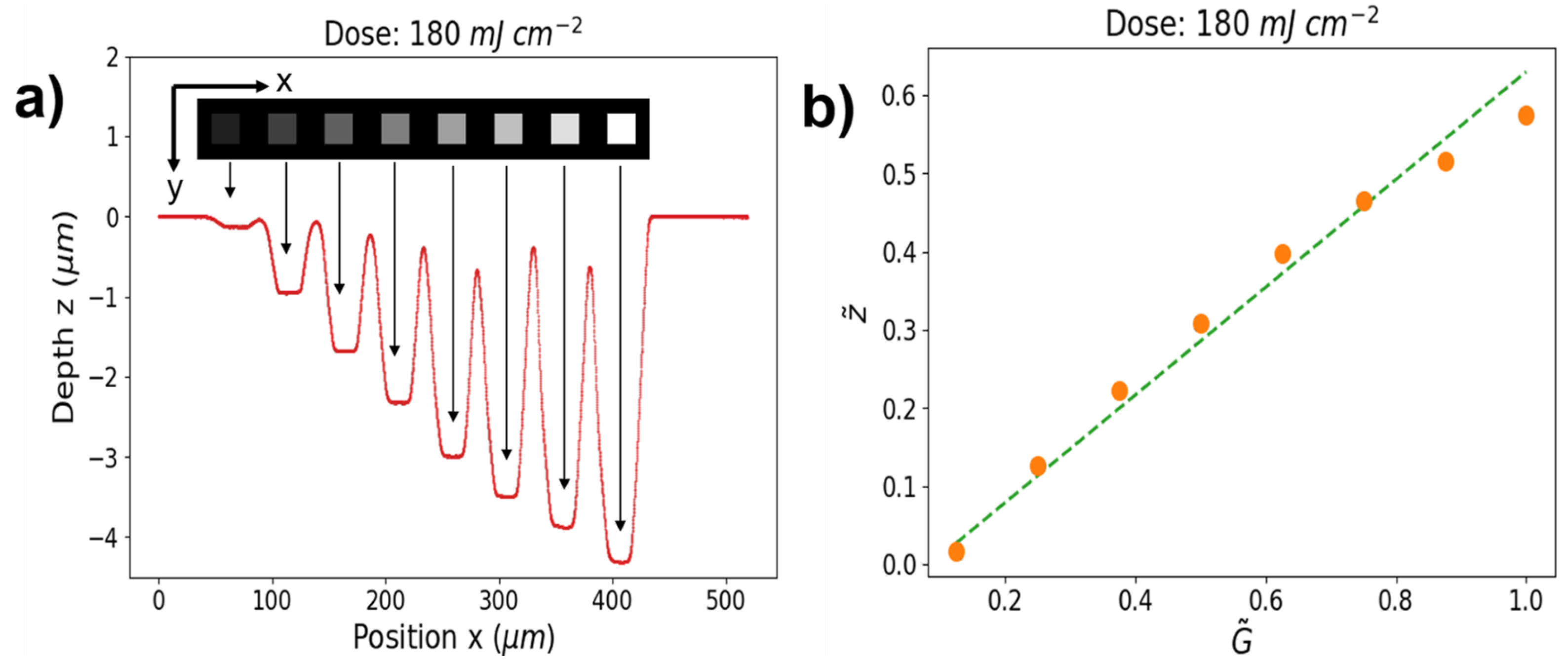
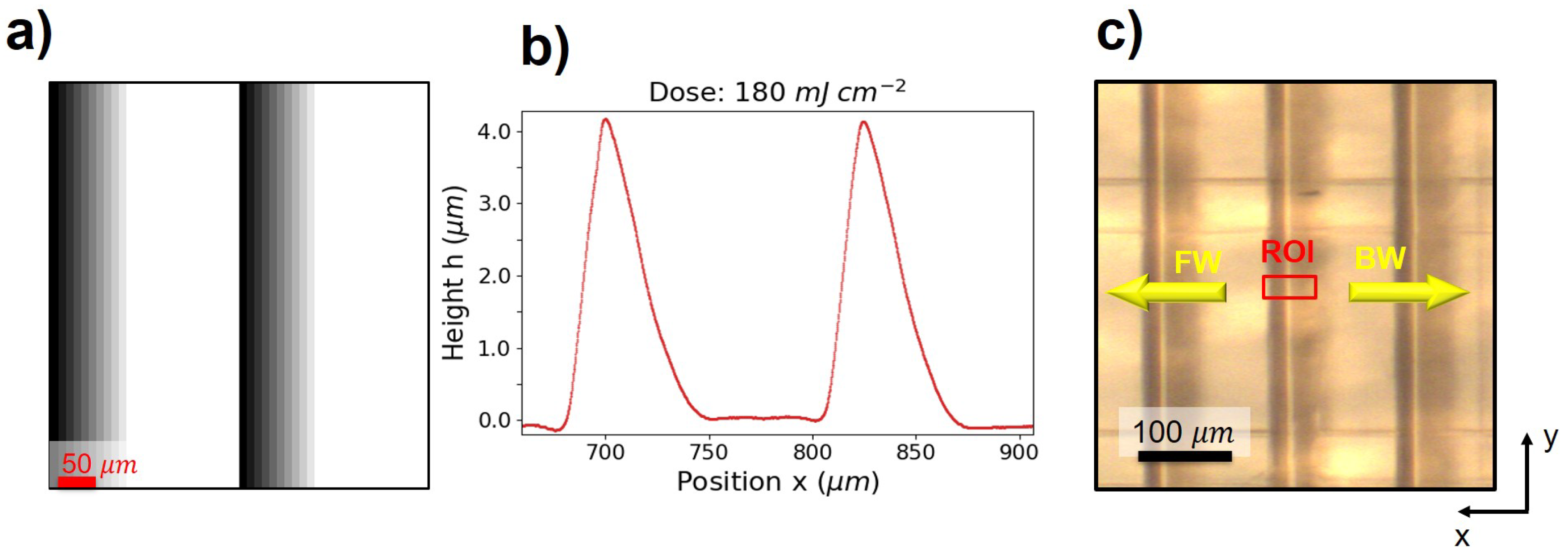
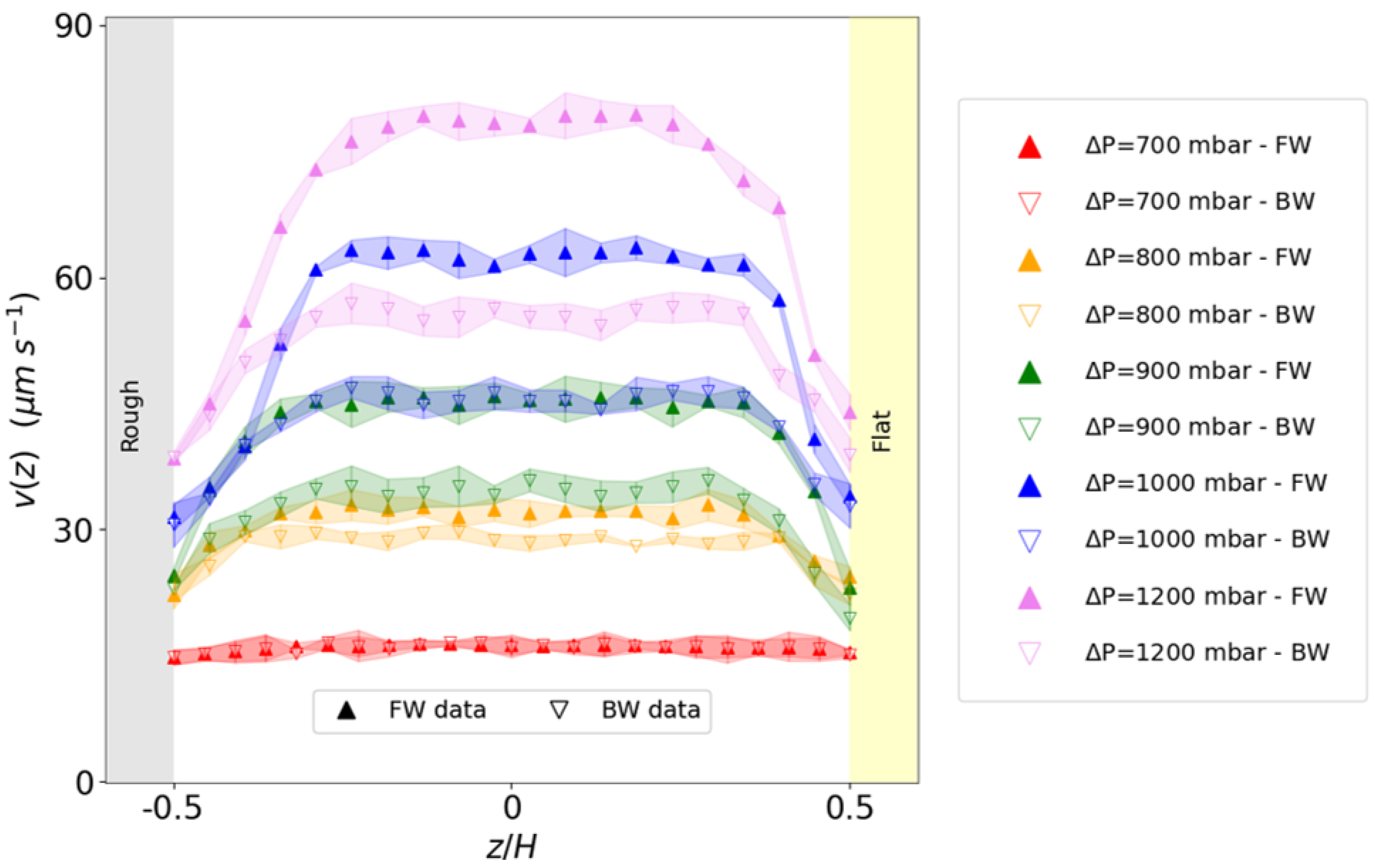
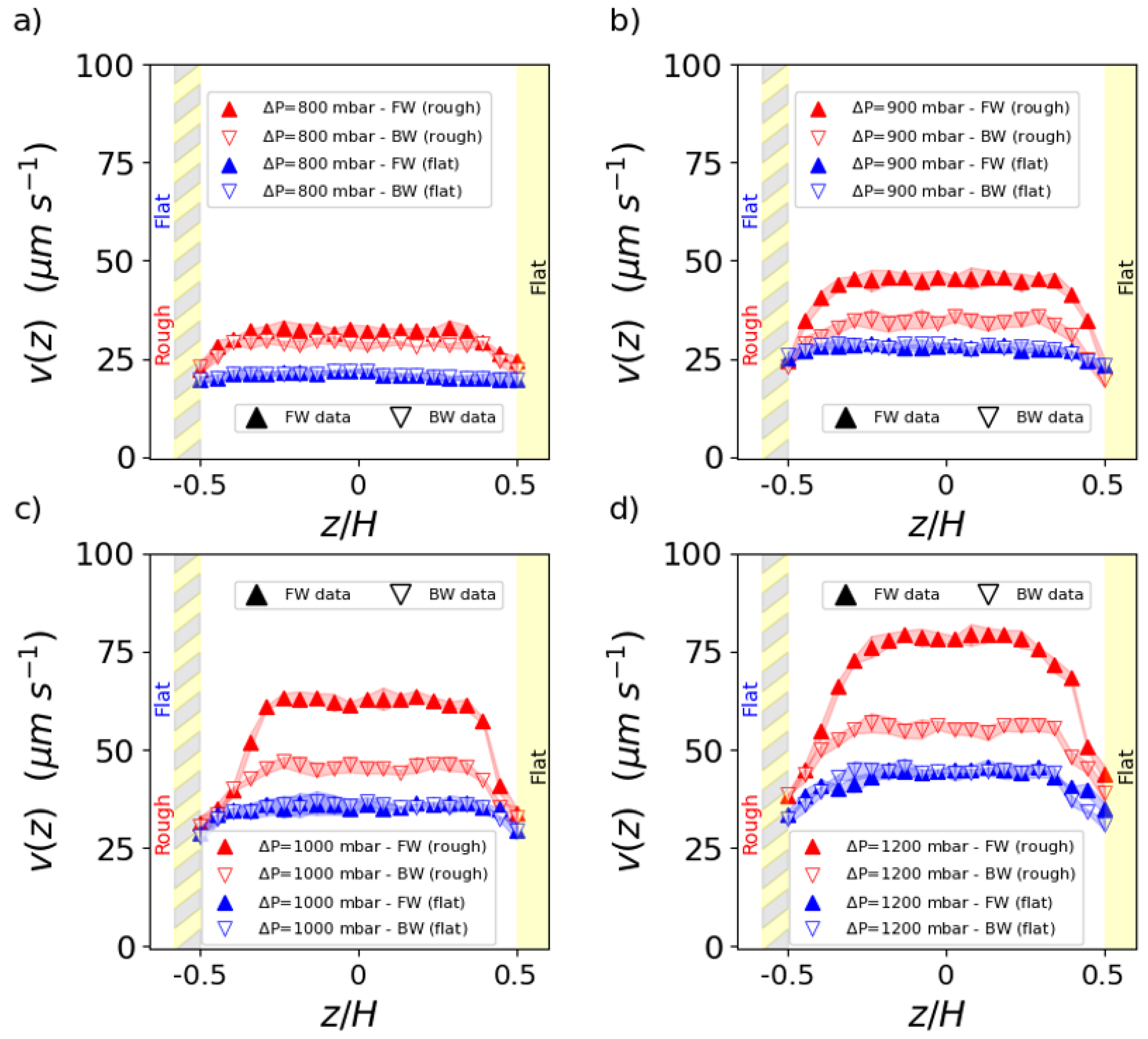
3. Results
4. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ROI | Region of Interest |
| -MLA | Micro Maskless Aligner |
| SLM | Spatial Light Modulator |
| FW | Forward |
| BW | Backward |
| MEMSs | Microelectromechanical Systems |
| CMOS | Complementary Metal-Oxide Semiconductor |
| TTAB | Tetradecyl Trymethyl Ammonium Bromide |
| PVP | Poly Vinyl Pyrrolidone |
| MFCS | Microfluidic Flow Control System |
Appendix A. Microfabrication
| Sample N. | (μm) | (μm) | (μm) | |
|---|---|---|---|---|
| 1 | 4.2 ± 0.2 | 51 ± 4 | 4.7 ± 0.4 | 75 ± 1 |
| 2 | 3.9 ± 0.3 | 50 ± 5 | 4.9 ± 0.6 | 78 ± 1 |
| 3 | 4.3 ± 0.2 | 55 ± 3 | 4.4 ± 0.4 | 74 ± 1 |
Appendix B. Experimental Setup for Measuring the Velocity Profiles

References
- Kröger, M.; Vermant, J. The Structure and Rheology of Complex Fluids. Appl. Rheol. 2000, 10, 110–111. [Google Scholar] [CrossRef]
- Barnes, H.A. The yield stress—A review or ‘παντα ρϵι’—Everything flows? J. -Non-Newton. Fluid Mech. 1999, 81, 133–178. [Google Scholar] [CrossRef]
- Russel, W.B.; Saville, D.A.; Schowalter, W.R. Colloidal Dispersions; Cambridge University Press: Cambridge, UK, 1989. [Google Scholar] [CrossRef]
- Barnes, H.A. Rheology of emulsions—A review. Colloids Surfaces Physicochem. Eng. Asp. 1994, 91, 89–95. [Google Scholar] [CrossRef]
- Yu, L.; Ding, B.; Dong, M.; Jiang, Q. A new model of emulsion flow in porous media for conformance control. Fuel 2019, 241, 53–64. [Google Scholar] [CrossRef]
- Bonn, D.; Denn, M.M.; Berthier, L.; Divoux, T.; Manneville, S. Yield stress materials in soft condensed matter. Rev. Mod. Phys. 2017, 89, 35005. [Google Scholar] [CrossRef]
- Divoux, T.; Fardin, M.A.; Manneville, S.; Lerouge, S. Shear Banding of Complex Fluids. Annu. Rev. Fluid Mech. 2016, 48, 81–103. [Google Scholar] [CrossRef]
- Divoux, T.; Agoritsas, E.; Aime, S.; Barentin, C.; Barrat, J.L.; Benzi, R.; Berthier, L.; Bi, D.; Biroli, G.; Bonn, D.; et al. Ductile-to-brittle transition and yielding in soft amorphous materials: Perspectives and open questions. Soft Matter 2024, 20, 6868–6888. [Google Scholar] [CrossRef]
- Benzi, R.; Divoux, T.; Barentin, C.; Manneville, S.; Sbragaglia, M.; Toschi, F. Unified Theoretical and Experimental View on Transient Shear Banding. Phys. Rev. Lett. 2019, 123, 248001. [Google Scholar] [CrossRef]
- Goyon, J.; Colin, A.; Ovarlez, G.; Ajdari, A.; Bocquet, L. Spatial cooperativity in soft glassy flows. Nature 2008, 454, 84–87. [Google Scholar] [CrossRef]
- Dunne, P.; Adachi, T.; Dev, A.A.; Sorrenti, A.; Giacchetti, L.; Bonnin, A.; Bourdon, C.; Mangin, P.H.; Coey, J.M.; Doudin, B.; et al. Liquid flow and control without solid walls. Nature 2020, 581, 58–62. [Google Scholar] [CrossRef]
- Paredes, J.; Shahidzadeh, N.; Bonn, D. Wall slip and fluidity in emulsion flow. Phys. Rev. E 2015, 92, 042313. [Google Scholar] [CrossRef] [PubMed]
- Mansard, V.; Bocquet, L.; Colin, A. Boundary conditions for soft glassy flows: Slippage and surface fluidization. Soft Matter 2014, 10, 6984–6989. [Google Scholar] [CrossRef] [PubMed]
- Derzsi, L.; Filippi, D.; Lulli, M.; Mistura, G.; Bernaschi, M.; Garstecki, P.; Sbragaglia, M.; Pierno, M. Wall fluidization in two acts: From stiff to soft roughness. Soft Matter 2018, 14, 1088–1093. [Google Scholar] [CrossRef]
- Seth, J.R.; Locatelli-Champagne, C.; Monti, F.; Bonnecaze, R.T.; Cloitre, M. How do soft particle glasses yield and flow near solid surfaces? Soft Matter 2011, 8, 140–148. [Google Scholar] [CrossRef]
- Goyon, J.; Colin, A.; Bocquet, L. How does a soft glassy material flow: Finite size effects, non local rheology, and flow cooperativity. Soft Matter 2010, 6, 2668–2678. [Google Scholar] [CrossRef]
- Pelusi, F.; Sbragaglia, M.; Scagliarini, A.; Lulli, M.; Bernaschi, M.; Succi, S. On the impact of controlled wall roughness shape on the flow of a soft material. Europhys. Lett. 2019, 127, 34005. [Google Scholar] [CrossRef]
- Filippi, D.; Derzsi, L.; Nalin, F.; Vezzani, A.; Ferraro, D.; Zaltron, A.; Mistura, G.; Pierno, M. Boost and Contraction of Flow by Herringbone Surface Design on the Microfluidic Channel Wall. Adv. Mater. Technol. 2023, 8, 2201748. [Google Scholar] [CrossRef]
- Pelusi, F.; Filippi, D.; Derzsi, L.; Pierno, M.; Sbragaglia, M. Emulsions in microfluidic channels with asymmetric boundary conditions and directional surface roughness: Stress and rheology. Soft Matter 2024, 20, 5203–5211. [Google Scholar] [CrossRef]
- Tabeling, P. Introduction to Microfluidics; Oxford University PressOxford: Oxford, UK, 2023. [Google Scholar] [CrossRef]
- Convery, N.; Gadegaard, N. 30 years of microfluidics. Micro Nano Eng. 2019, 2, 76–91. [Google Scholar] [CrossRef]
- Levinson, H.J. Principles of Lithography, 4th ed.; SPIE Press: Washington, DC, USA, 2019. [Google Scholar] [CrossRef]
- Watson, G.P.; Aksyuk, V.; Simon, M.E.; Tennant, D.M.; Cirelli, R.A.; Mansfield, W.M.; Pardo, F.; Lopez, D.O.; Bolle, C.A.; Papazian, A.R.; et al. Spatial Light Modulator for maskless optical projection lithography. J. Vac. Sci. Technol. Microelectron. Nanometer Struct. Process. Meas. Phenom. 2006, 24, 2852–2856. [Google Scholar] [CrossRef]
- Judy, J.W. Microelectromechanical systems (MEMS):fabrication, design and applications. Smart Mater. Struct. 2001, 10, 1115. [Google Scholar] [CrossRef]
- Loomis, J.; Ratnayake, D.; McKenna, C.; Walsh, K.M. Grayscale lithography—Automated mask generation for complex three-dimensional topography. J. Micro/Nanolithography MEMS MOEMS 2016, 15, 013511. [Google Scholar] [CrossRef]
- Bekker, C.; Arshad, M.J.; Cilibrizzi, P.; Nikolatos, C.; Lomax, P.; Wood, G.S.; Cheung, R.; Knolle, W.; Ross, N.; Gerardot, B.; et al. Scalable fabrication of hemispherical solid immersion lenses in silicon carbide through grayscale hard-mask lithography. Appl. Phys. Lett. 2023, 122, 173507. [Google Scholar] [CrossRef]
- Grushina, A. Direct-write grayscale lithography. Adv. Opt. Technol. 2019, 8, 163–169. [Google Scholar] [CrossRef]
- Ito, T.; Okazaki, S. Pushing the limits of lithography. Nature 2000, 406, 1027–1031. [Google Scholar] [CrossRef] [PubMed]
- Rammohan, A.; Dwivedi, P.K.; Martinez-Duarte, R.; Katepalli, H.; Madou, M.J.; Sharma, A. One-step maskless grayscale lithography for the fabrication of 3-dimensional structures in SU-8. Sens. Actuators B Chem. 2011, 153, 125–134. [Google Scholar] [CrossRef]
- Totsu, K.; Esashi, M. Gray-scale photolithography using maskless exposure system. J. Vac. Sci. Technol. Microelectron. Nanometer Struct. Process. Meas. Phenom. 2005, 23, 1487–1490. [Google Scholar] [CrossRef]
- Waldbaur, A.; Waterkotte, B.; Schmitz, K.; Rapp, B.E. Maskless Projection Lithography for the Fast and Flexible Generation of Grayscale Protein Patterns. Small 2012, 8, 1570–1578. [Google Scholar] [CrossRef]
- Mansoor, M.; Haneef, I.; De Luca, A.; Coull, J.; Udrea, F. A maskless etching technique for fabrication of 3D MEMS structures in SOI CMOS devices. J. Micromech. Microeng. 2018, 28, 085013. [Google Scholar] [CrossRef]
- Micro Resist Technology GmbH Germany. MA-P 1200G Series Photoresist Datasheet; Micro Resist Technology GmbH Germany: Berlin, Germany, 2024. [Google Scholar]
- Heidelberg Instrument GmbH. Tabletop Maskless Alliger; Heidelberg Instrument GmbH: Heidelberg, Germany, 2020. [Google Scholar]
- Kim, K.R.; Yi, J.; Cho, S.H.; Kang, N.H.; Cho, M.W.; Shin, B.S.; Choi, B. SLM-based maskless lithography for TFT-LCD. Appl. Surf. Sci. 2009, 255, 7835–7840. [Google Scholar] [CrossRef]
- Khazi, I.; Muthiah, U.; Mescheder, U. 3D free forms in c-Si via grayscale lithography and RIE. Microelectron. Eng. 2018, 193, 34–40. [Google Scholar] [CrossRef]
- Kanikella, P. Process Development and Applications of a Dry Film Photoresist. Master’s Thesis, University of Missouri, Rolla, MO, USA, 2007. [Google Scholar]
- Saleem, M.; Singh, U.; Haque, A.; Wang, A.J.; Reverberi, A.P.; Cardone, A.; Hassaan, M.; Saleem, U.; Singh, A.; Haque, J.; et al. Recent Advances in Positive Photoresists: Mechanisms and Fabrication. Materials 2024, 17, 2552. [Google Scholar] [CrossRef]
- Boateng, L.K.; Mirshahghassemi, S.; Wu, H.; Flora, J.R.V.; Grassian, V.H.; Lead, J.R. Mechanistic study of oil adsorption onto PVP-coated magnetic nanoparticles: An integrated experimental and molecular dynamics study to inform remediation. Environ. Sci. Nano 2021, 8, 485–492. [Google Scholar] [CrossRef]
- Derzsi, L.; Filippi, D.; Mistura, G.; Pierno, M.; Lulli, M.; Sbragaglia, M.; Bernaschi, M.; Garstecki, P. Fluidization and wall slip of soft glassy materials by controlled surface roughness. Phys. Rev. E 2017, 95, 052602. [Google Scholar] [CrossRef]
- Adrian, R.J. Particle-imaging techniques for experimental fluid mechanics. Annu. Rev. Fluid Mech. 1991, 23, 261–304. [Google Scholar] [CrossRef]
- Schindelin, J.; Arganda-Carreras, I.; Frise, E.; Kaynig, V.; Longair, M.; Pietzsch, T.; Preibisch, S.; Rueden, C.; Saalfeld, S.; Schmid, B.; et al. Fiji: An open-source platform for biological-image analysis. Nat. Methods 2012, 9, 676–682. [Google Scholar] [CrossRef]
- Ershov, D.; Phan, M.S.; Pylvänäinen, J.W.; Rigaud, S.U.; Le Blanc, L.; Charles-Orszag, A.; Conway, J.R.; Laine, R.F.; Roy, N.H.; Bonazzi, D.; et al. TrackMate 7: Integrating state-of-the-art segmentation algorithms into tracking pipelines. Nat. Methods 2022, 19, 829–832. [Google Scholar] [CrossRef] [PubMed]
- Tinevez, J.Y.; Perry, N.; Schindelin, J.; Hoopes, G.M.; Reynolds, G.D.; Laplantine, E.; Bednarek, S.Y.; Shorte, S.L.; Eliceiri, K.W. TrackMate: An open and extensible platform for single-particle tracking. Methods 2017, 115, 80–90. [Google Scholar] [CrossRef]
- Nicolas, A.; Barrat, J.L. Spatial Cooperativity in Microchannel Flows of Soft Jammed Materials: A Mesoscopic Approach. Phys. Rev. Lett. 2013, 110, 138304. [Google Scholar] [CrossRef]
- Hull, S.M.; Lindsay, C.D.; Brunel, L.G.; Shiwarski, D.J.; Tashman, J.W.; Roth, J.G.; Myung, D.; Feinberg, A.W.; Heilshorn, S.C. 3D Bioprinting using UNIversal Orthogonal Network (UNION) Bioinks. Adv. Funct. Mater. 2021, 31, 2007983. [Google Scholar] [CrossRef]
- Fang, Y.; Guo, Y.; Ji, M.; Li, B.; Guo, Y.; Zhu, J.; Zhang, T.; Xiong, Z. 3D Printing of Cell-Laden Microgel-Based Biphasic Bioink with Heterogeneous Microenvironment for Biomedical Applications. Adv. Funct. Mater. 2022, 32, 2109810. [Google Scholar] [CrossRef]
- Gungor-Ozkerim, P.S.; Inci, I.; Zhang, Y.S.; Khademhosseini, A.; Dokmeci, M.R. Bioinks for 3D bioprinting: An overview. Biomater. Sci. 2018, 6, 915–946. [Google Scholar] [CrossRef] [PubMed]
- Xu, H.Q.; Liu, J.C.; Zhang, Z.Y.; Xu, C.X. A review on cell damage, viability, and functionality during 3D bioprinting. Mil. Med. Res. 2022, 9, 70. [Google Scholar] [CrossRef] [PubMed]



| H (μm) | L (cm) | W (cm) | H (μm) | L (μm) | g (μm) | |
|---|---|---|---|---|---|---|
| 120 ± 5 | 5.00 ± 0.01 | 1.00 ± 0.01 | 4.2 ± 0.2 | 51 ± 4 | 75 ± 1 | 4.7 ± 0.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guastella, G.; Filippi, D.; Ferraro, D.; Mistura, G.; Pierno, M. Directional Fluidity of Dense Emulsion Activated by Transverse Wedge-Shaped Microroughness. Micromachines 2025, 16, 335. https://doi.org/10.3390/mi16030335
Guastella G, Filippi D, Ferraro D, Mistura G, Pierno M. Directional Fluidity of Dense Emulsion Activated by Transverse Wedge-Shaped Microroughness. Micromachines. 2025; 16(3):335. https://doi.org/10.3390/mi16030335
Chicago/Turabian StyleGuastella, Giacomo, Daniele Filippi, Davide Ferraro, Giampaolo Mistura, and Matteo Pierno. 2025. "Directional Fluidity of Dense Emulsion Activated by Transverse Wedge-Shaped Microroughness" Micromachines 16, no. 3: 335. https://doi.org/10.3390/mi16030335
APA StyleGuastella, G., Filippi, D., Ferraro, D., Mistura, G., & Pierno, M. (2025). Directional Fluidity of Dense Emulsion Activated by Transverse Wedge-Shaped Microroughness. Micromachines, 16(3), 335. https://doi.org/10.3390/mi16030335







