Periodic Arrays of Plasmonic Ag-Coated Multiscale 3D-Structures with SERS Activity: Fabrication, Modelling and Characterisation
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Computational Simulations
2.3. Fabrication of Ordered Arrays of Ag-Coated Multiscale 3D-Structures
2.4. Imaging
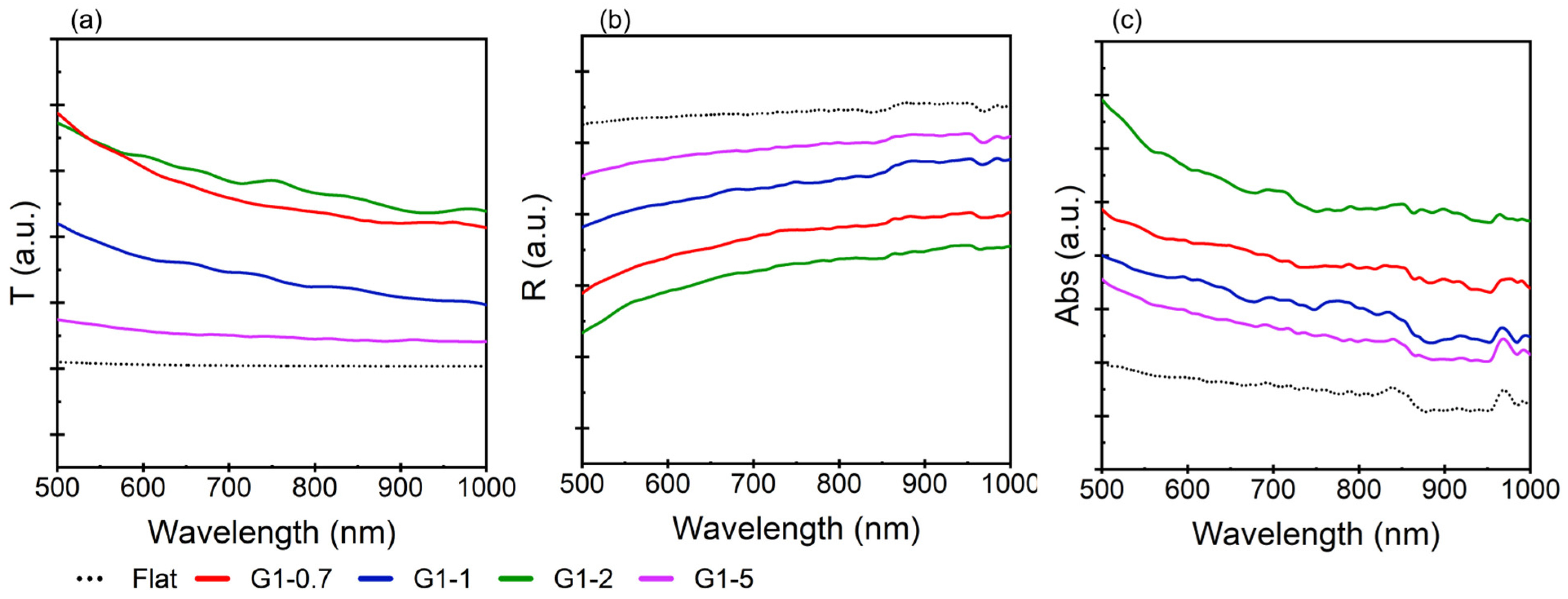
2.5. Optical Characterization
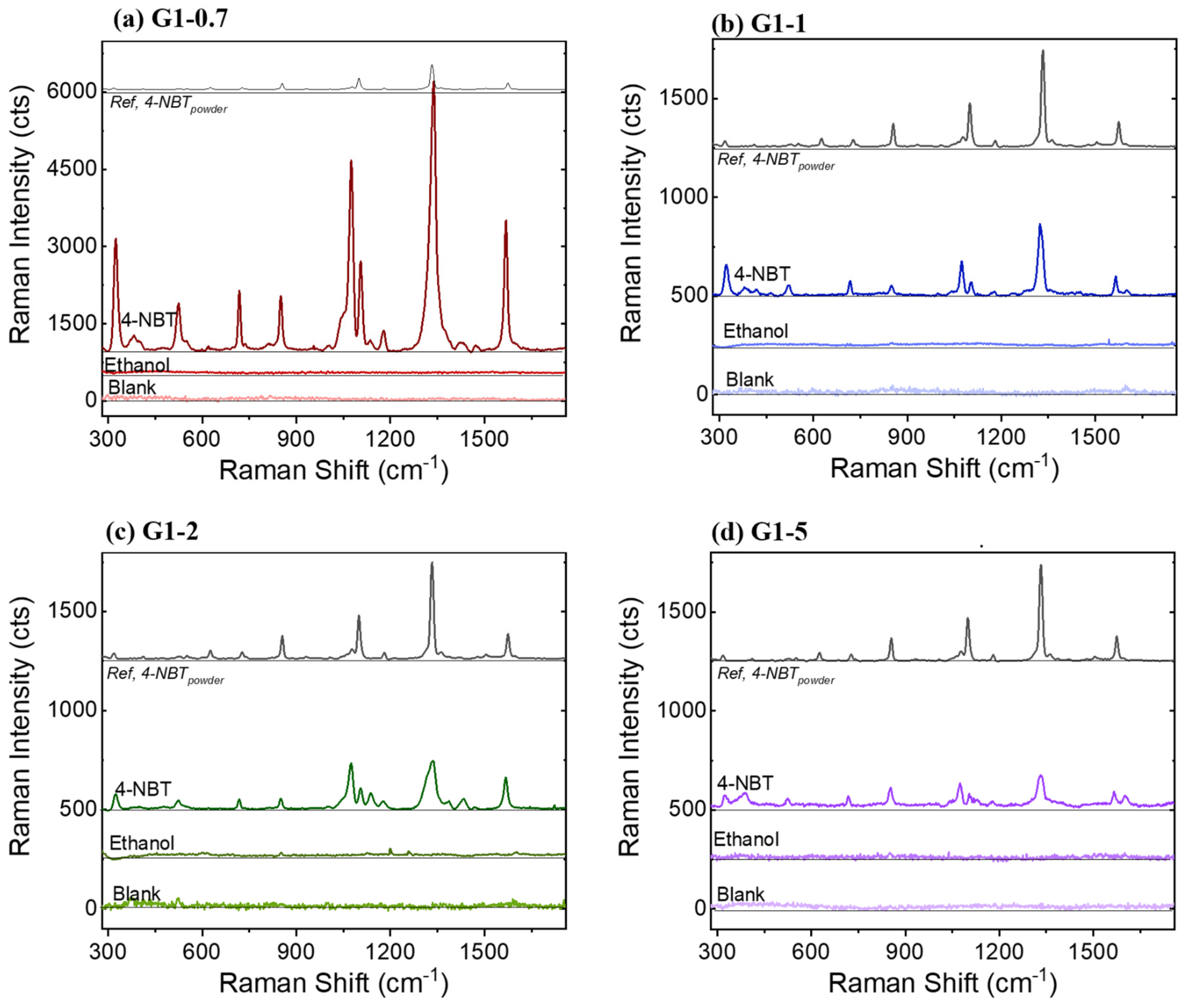
2.6. Raman and SERS Measurements
2.7. Calculation of Analytical Enhancement Factor (AEF)
2.8. Calculation of Limit of Detection (LOD)
2.8.1. Blank Measurements
2.8.2. 4-NBT Solutions
3. Results and Discussion
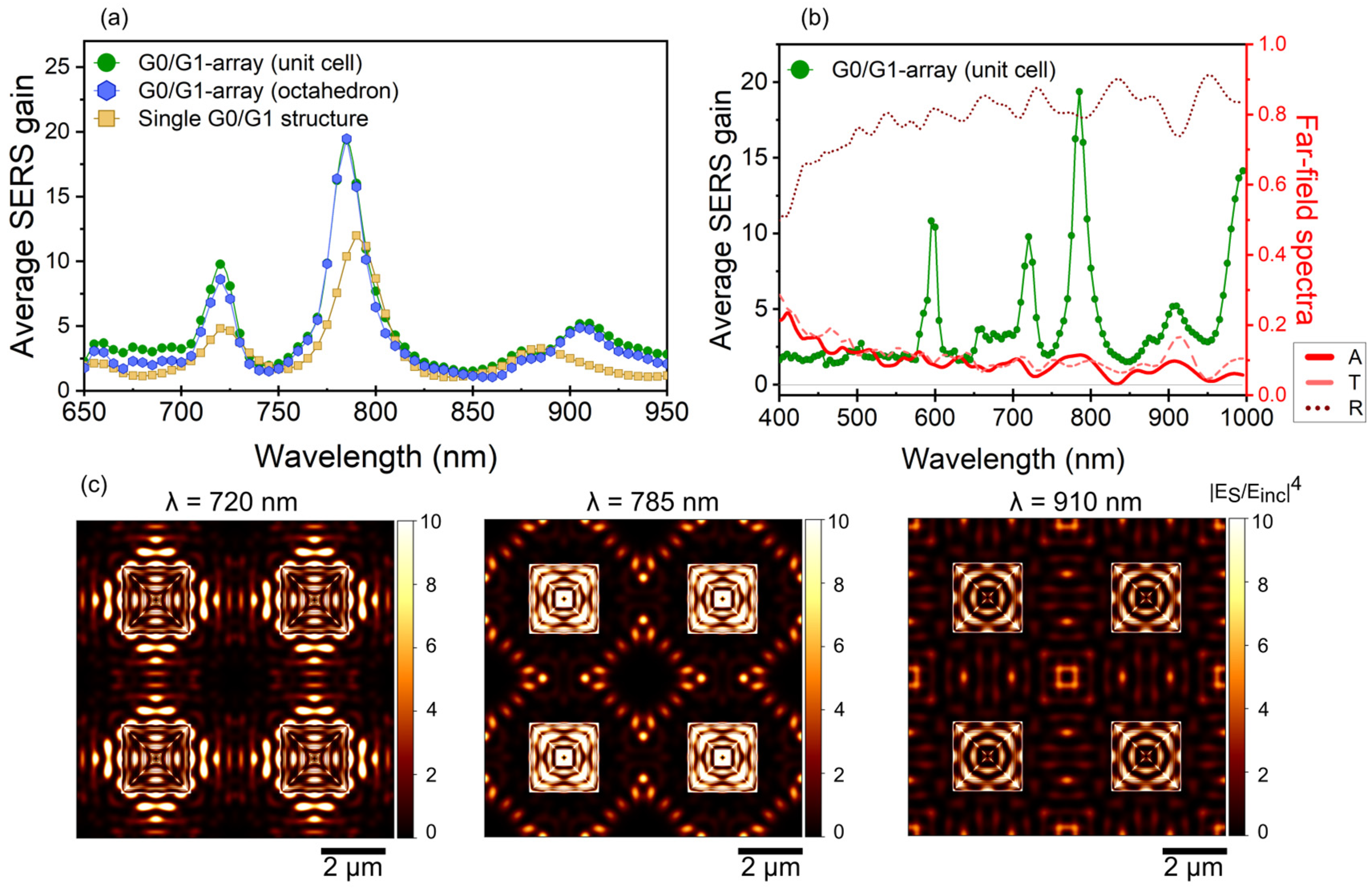
3.1. Modelling of Ordered Arrays of Ag-Coated Multiscale 3D-Structures
3.2. Fabrication of Ordered Arrays of Ag-Coated Multiscale 3D-Structures
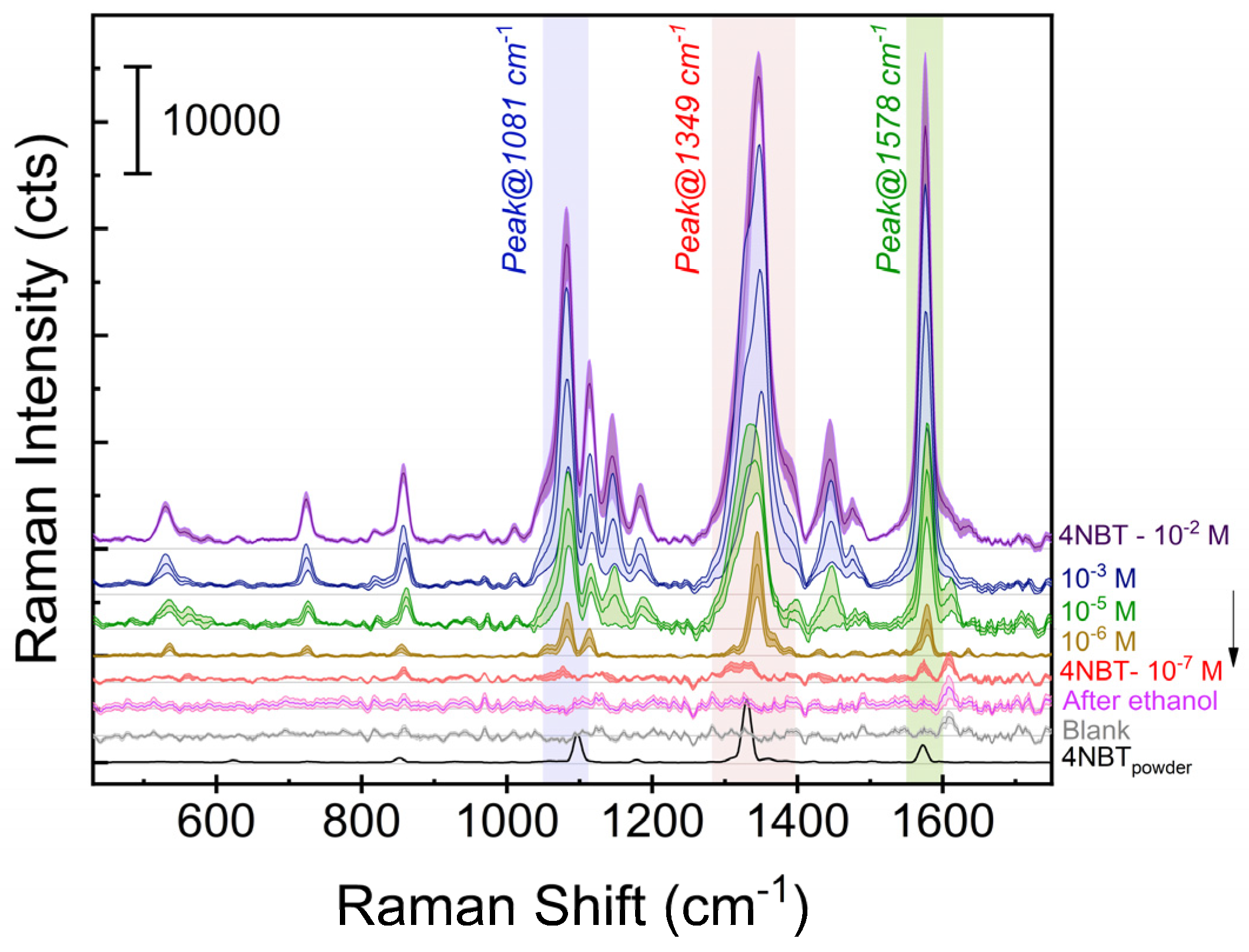
3.3. SERS Activity of Ag-Coated Multiscale 3D-Structures
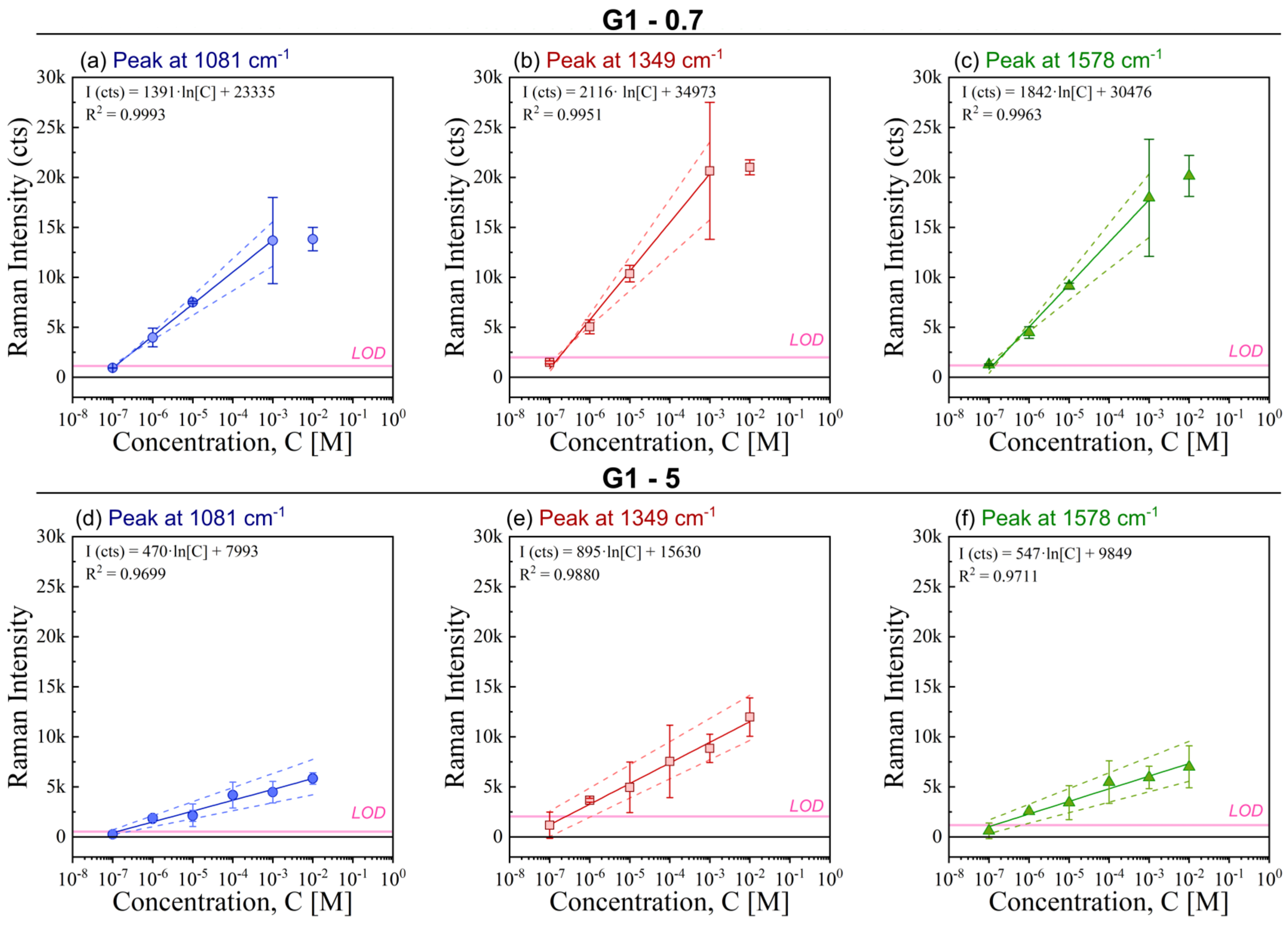
3.4. Sensitivity of Ag-Coated Multiscale 3D-Structures
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Specimen I.D. | Widths G1/G0 [µm] | Pattern of Circular Openings (Diameter [µm]); Pitch [µm]; Packing | KOH Etch Time [min] | Wet Oxidation Time [min] | 1% HF Etch Time [min] | TMAH Etch Time [min] |
|---|---|---|---|---|---|---|
| G1-0.7 | ~0.7/2 | 1.5; 5; hexagonal | 9.5 | 81 | 10 | 16 |
| G1-1 | ~1/2 | 21 | ||||
| G1-2 | ~2/2 | 43 | ||||
| G1-5 | ~5/5 | 5; 20; square | 7.5 | 164 | 20 | 140 |
Appendix B
| Monitoring Peak Centered at [cm−1] | Coloured-Band in Figure | Lower Limit [cm−1] | Upper Limit [cm−1] | Spectral Width [cm−1] | Number of Points Averaged |
|---|---|---|---|---|---|
| 1081 | Blue | 1027 | 1096 | 69 | 36 |
| 1351 | Red | 1292 | 1416 | 124 | 62 |
| 1575 | Green | 1506 | 1588 | 82 | 24 |
| Monitoring Peak Centered at [cm−1] | Coloured-Band in Figure | Lower Limit [cm−1] | Upper Limit [cm−1] | Spectral Width [cm−1] | Number of Points Averaged |
|---|---|---|---|---|---|
| 1081 | Blue | 1026 | 1144 | 118 | 60 |
| 1351 | Red | 1268 | 1416 | 148 | 75 |
| 1575 | Green | 1506 | 1614 | 108 | 55 |
Appendix C
Appendix D

References
- Langer, J.; Jimenez de Aberasturi, D.; Aizpurua, J.; Alvarez-Puebla, R.A.; Auguié, B.; Baumberg, J.J.; Bazan, G.C.; Bell, S.E.J.; Boisen, A.; Brolo, A.G.; et al. Present and Future of surface-enhanced Raman scattering. ACS Nano 2019, 14, 28–117. [Google Scholar] [CrossRef] [PubMed]
- Vendamani, V.S.; Rao, S.V.S.N.; Pathak, A.P.; Soma, V.R. Silicon nanostructures for molecular sensing: A review. ACS Appl. Nano Mater. 2022, 5, 4550–4582. [Google Scholar] [CrossRef]
- Le Ru, E.C.; Auguié, B. Enhancement factors: A central concept during 50 years of surface-enhanced Raman spectroscopy. ACS Nano 2024, 18, 9773–9783. [Google Scholar] [CrossRef] [PubMed]
- Valley, N.; Greeneltch, N.; Van Duyne, R.P.; Schatz, G.C. A look at the origin and magnitude of the chemical contribution to the enhancement mechanism of surface-enhanced Raman spectroscopy (SERS): Theory and experiment. J. Phys. Chem. Lett. 2013, 4, 2599–2604. [Google Scholar] [CrossRef]
- Stiles, P.L.; Dieringer, J.A.; Shah, N.C.; Van Duyne, R.P. Surface-enhanced Raman spectroscopy. Annu. Rev. Anal. Chem. 2008, 1, 601–626. [Google Scholar] [CrossRef]
- Shiohara, A.; Wang, Y.; Liz-Marzán, L.M. Recent Approaches Toward Creation of Hot Spots for SERS Detection. In Colloidal Synthesis of Plasmonic Nanometals, 1st ed.; Liz-Marzán, L., Ed.; Jenny Stanford Publishing: New York, NY, USA, 2020; pp. 563–622. [Google Scholar] [CrossRef]
- Shiohara, A.; Wang, Y.; Liz-Marzán, L.M. Recent approaches toward creation of hot spots for SERS detection. J. Photochem. Photobiol. C Photochem. Rev. 2014, 21, 2–25. [Google Scholar] [CrossRef]
- Fan, M.; Andrade, G.F.S.; Brolo, A.G. A review on the fabrication of substrates for surface enhanced Raman spectroscopy and their applications in analytical chemistry. Anal. Chim. Acta 2011, 693, 7–25. [Google Scholar] [CrossRef]
- Caridad, J.M.; Winters, S.; McCloskey, D.; Duesberg, G.S.; Donegan, J.F.; Krstić, V. Hot-volumes as uniform and reproducible SERS-detection enhancers in weakly-coupled metallic nanohelices. Sci. Rep. 2017, 7, 45548. [Google Scholar] [CrossRef]
- Fan, M.; Andrade, G.F.S.; Brolo, A.G. A review on recent advances in the applications of surface-enhanced Raman scattering in analytical chemistry. Anal. Chim. Acta 2020, 1097, 1–29. [Google Scholar] [CrossRef]
- Freeman, R.G.; Grabar, K.C.; Allison, K.J.; Bright, R.M.; Davis, J.A.; Guthrie, A.P.; Hommer, M.B.; Jackson, M.A.; Smith, P.C.; Walter, D.G.; et al. Self-assembled metal colloid monolayers: An approach to SERS substrates. Science 1995, 267, 1629–1632. [Google Scholar] [CrossRef]
- Rodríguez-Lorenzo, L.; Álvarez-Puebla, R.A.; Pastoriza-Santos, I.; Mazzucco, S.; Stéphan, O.; Kociak, M.; Liz-Marzán, L.M.; De Abajo, F.J.G. Zeptomol detection through controlled ultrasensitive surface-enhanced Raman scattering. J. Am. Chem. Soc. 2009, 131, 4616–4618. [Google Scholar] [CrossRef]
- Pieczonka, N.P.W.; Goulet, P.J.G.; Aroca, R.F. Chemically selective sensing through layer-by-layer incorporation of biorecognition into thin film substrates for surface-enhanced resonance Raman scattering. J. Am. Chem. Soc. 2006, 128, 12626–12627. [Google Scholar] [CrossRef]
- Lee, J.; Hua, B.; Park, S.; Ha, M.; Lee, Y.; Fan, Z.; Ko, H. Tailoring surface plasmons of high-density gold nanostar assemblies on metal films for surface-enhanced Raman spectroscopy. Nanoscale 2014, 6, 616–623. [Google Scholar] [CrossRef] [PubMed]
- Martin, M.N.; Basham, J.I.; Chando, P.; Eah, S.-K. Charged gold nanoparticles in non-polar solvents: 10-min synthesis and 2D self-assembly. Langmuir 2010, 26, 7410–7417. [Google Scholar] [CrossRef] [PubMed]
- Lafuente, M.; Almazán, F.; Bernad, E.; Florea, I.; Arenal, R.; Urbiztondo, M.A.; Mallada, R.; Pina, M.P. On-chip monitoring of toxic gases: Capture and label-free SERS detection with plasmonic mesoporous sorbents. Lab Chip 2023, 23, 3160–3171. [Google Scholar] [CrossRef] [PubMed]
- Lafuente, M.; Ruiz-Rincón, S.; Mallada, R.; Cea, P.; Pilar Pina, M. Towards the reproducible fabrication of homogeneous SERS substrates by Langmuir-Schaefer technique: A low cost and scalable approach for practical SERS based sensing applications. Appl. Surf. Sci. 2020, 506, 144663. [Google Scholar] [CrossRef]
- Rajapandiyan, P.; Yang, J. Photochemical method for decoration of silver nanoparticles on filter paper substrate for SERS application. J. Raman Spectrosc. 2014, 45, 574–580. [Google Scholar] [CrossRef]
- Zhang, X.; Salcedo, W.J.; Rahman, M.M.; Brolo, A.G. Surface-enhanced Raman scattering from bowtie nanoaperture arrays. Surf. Sci. 2018, 676, 39–45. [Google Scholar] [CrossRef]
- Yue, W.; Gong, T.; Long, X.; Kravets, V.; Gao, P.; Pu, M.; Wang, C. Sensitive and reproducible surface-enhanced Raman spectroscopy (SERS) with arrays of dimer-nanopillars. Sens. Actuators B Chem. 2020, 322, 128563. [Google Scholar] [CrossRef]
- Zhao, Q.; Yang, H.; Nie, B.; Luo, Y.; Shao, J.; Li, G. Wafer-scale and cost-effective manufacturing of controllable nanogap arrays for highly sensitive SERS sensing. ACS Appl. Mater. Interfaces 2022, 14, 3580–3590. [Google Scholar] [CrossRef]
- Cai, J.; Wang, Z.; Wang, M.; Zhang, D. Au nanoparticle-grafted hierarchical pillars array replicated from diatom as reliable SERS substrates. Appl. Surf. Sci. 2021, 541, 148374. [Google Scholar] [CrossRef]
- Jonker, D.; Srivastava, K.; Lafuente, M.; Susarrey-Arce, A.; Van der Stam, W.; Van den Berg, A.; Odijk, M.; Gardeniers, H.J.G.E. Low-variance surface-enhanced Raman spectroscopy using confined gold nanoparticles over silicon nanocones. ACS Appl. Nano Mater. 2023, 6, 9657–9669. [Google Scholar] [CrossRef] [PubMed]
- Lafuente, M.; Muñoz, P.; Berenschot, E.J.W.; Tiggelaar, R.M.; Susarrey-Arce, A.; Rodrigo, S.G.; Kooijman, L.J.; García-Blanco, S.M.; Mallada, R.; Pina, M.P.; et al. Exploring the surface-enhanced Raman scattering (SERS) activity of gold nanostructures embedded around nanogaps at wafer scale: Simulations and experiments. Appl. Mater. Today 2023, 35, 101929. [Google Scholar] [CrossRef]
- Im, H.; Bantz, K.C.; Lindquist, N.C.; Haynes, C.L.; Oh, S.H. Vertically oriented sub-10-nm plasmonic nanogap arrays. Nano Lett. 2010, 10, 2231–2236. [Google Scholar] [CrossRef] [PubMed]
- Wu, K.; Li, T.; Schmidt, M.S.; Rindzevicius, T.; Boisen, A.; Ndoni, S. Gold nanoparticles sliding on recyclable nanohoodoos—Engineered for surface-enhanced Raman spectroscopy. Adv. Funct. Mater. 2018, 28, 1704818. [Google Scholar] [CrossRef]
- Schmidt, M.S.; Hübner, J.; Boisen, A. Large area fabrication of leaning silicon nanopillars for surface enhanced Raman spectroscopy. Adv. Mater. 2012, 24, 11–18. [Google Scholar] [CrossRef]
- Sim, J.H.; Lee, S.H.; Yang, J.Y.; Lee, W.C.; Mun, C.W.; Lee, S.; Park, S.G.; Cho, Y.R. Plasmonic hotspot engineering of Ag-coated polymer substrates with high reproducibility and photothermal stability. Sens. Actuators B Chem. 2022, 354, 13110. [Google Scholar] [CrossRef]
- Liu, B.; Yao, X.; Chen, S.; Lin, H.; Yang, Z.; Liu, S.; Ren, B. Large-area hybrid plasmonic optical cavity (HPOC) substrates for surface-enhanced Raman spectroscopy. Adv. Funct. Mater. 2018, 28, 1802263. [Google Scholar] [CrossRef]
- Zhang, C.; Jiang, S.Z.; Yang, C.; Li, C.H.; Huo, Y.Y.; Liu, X.Y.; Liu, A.H.; Wei, Q.; Gao, S.S.; Gao, X.G.; et al. Gold@silver bimetal nanoparticles/pyramidal silicon 3D substrate with high reproducibility for high-performance SERS. Sci. Rep. 2016, 6, 25243. [Google Scholar] [CrossRef]
- Thuy, N.T.N.; Luan, H.N.T.; Hieu, V.V.K.; Ngan, M.T.T.; Trung, N.T.; Hung, L.V.T.; Van, T.T.T. Optimum fabrication parameters for preparing high performance SERS substrates based on Si pyramid structure and silver nanoparticles. RSC Adv. 2021, 11, 31189–31196. [Google Scholar] [CrossRef]
- Zhang, C.; Jiang, S.Z.; Huo, Y.Y.; Liu, A.H.; Xu, S.C.; Liu, X.Y.; Sun, Z.C.; Xu, Y.Y.; Li, Z.; Man, B.Y. SERS detection of R6G based on a novel graphene oxide/silver nanoparticles/silicon pyramid arrays structure. Opt. Express 2015, 23, 24811. [Google Scholar] [CrossRef] [PubMed]
- de Sousa Junior, J.F.; Columbus, S.; Hammouche, J.; Ramachandran, K.; Daoudi, K.; Gaidi, M. Engineered micro-pyramids functionalized with silver nanoarrays as excellent cost-effective SERS chemosensors for multi-hazardous pollutants detection. Appl. Surf. Sci. 2023, 613, 156092. [Google Scholar] [CrossRef]
- Das, A.; Pant, U.; Cao, C.; Moirangthem, R.S.; Kamble, H.B. Fabrication of plasmonic nanopyramidal array as flexible SERS substrate for biosensing application. Nano Res. 2023, 16, 1132–1140. [Google Scholar] [CrossRef]
- Zhang, J.; Wu, J.; Wang, B.; Geng, Y.; Wang, Z. A new strategy for constructing 3D hybrid graphene/Au/rectangular pyramids PMMA on a flexible SERS substrate for trace molecule detection. Sens. Actuators B Chem. 2024, 410, 135711. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Z.; Chen, C.; Liu, J.; Lu, J.; Lu, N. Fabrication of flexible pyramid array as SERS substrate for direct sampling and reproducible detection. Anal. Chem. 2023, 95, 14184–14191. [Google Scholar] [CrossRef]
- Zhang, J.; Yan, Y.; Miao, P.; Cai, J. Fabrication of gold-coated PDMS surfaces with arrayed triangular micro/nanopyramids for use as SERS substrates. Beilstein J. Nanotechnol. 2017, 8, 2271–2282. [Google Scholar] [CrossRef]
- Das, A.; Pant, U.; Cao, C.; Moirangthem, R.S.; Kamble, H.B. Wearable surface-enhanced Raman spectroscopy sensor using inverted bimetallic nanopyramids for biosensing and sweat monitoring. ACS Appl. Opt. Mater. 2023, 1, 1938–1951. [Google Scholar] [CrossRef]
- Jin, M.; Pully, V.; Otto, C.; Van den Berg, A.; Carlen, E.T. High-density periodic arrays of self-aligned subwavelength nanopyramids for surface-enhanced Raman spectroscopy. J. Phys. Chem. C 2010, 114, 21953–21959. [Google Scholar] [CrossRef]
- Wu, H.; Niu, G.; Ren, W.; Jiang, L.; Zhao, J.; Quan, Y.; Ren, M.X.; Yu, W.; Zhang, Y.; Cao, X.; et al. Highly sensitive label-free detection of analytes at different scales using uniform graphene-nanopyramids hybrid SERS system. Sens. Actuators B Chem. 2022, 354, 131205. [Google Scholar] [CrossRef]
- Lafuente, M.; Berenschot, E.J.W.; Tiggelaar, R.M.; Mallada, R.; Tas, N.R.; Pina, M.P. 3D fractals as SERS active platforms: Preparation and evaluation for gas phase detection of G-nerve agents. Micromachines 2018, 9, 60. [Google Scholar] [CrossRef]
- Rodrigo, S.G. Optical Properties of Nanostructured Metallic Systems, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar] [CrossRef]
- Palik, E.D. Handbook of Optical Constants and Solids, 1st ed.; Academic Press Handbook Series; Academic Press: New York, NY, USA, 1985. [Google Scholar]
- Rodrigo, S.G.; García-Vidal, F.J.; Martín-Moreno, L. Influence of material properties on extraordinary optical transmission through hole arrays. Phys. Rev. B 2008, 77, 075401. [Google Scholar] [CrossRef]
- Skadtchenko, B.O.; Aroca, R. Surface-enhanced Raman scattering of p-nitrothiophenol: Molecular vibrations of its silver salt and the surface complex formed on silver islands and colloids. Spectrochim. Acta A 2001, 57, 1009–1016. [Google Scholar] [CrossRef] [PubMed]
- Le Ru, E.C.; Blackie, E.; Meyer, M.; Etchegoint, P.G. Surface enhanced Raman scattering enhancement factors: A comprehensive study. J. Phys. Chem. C 2007, 111, 13794–13803. [Google Scholar] [CrossRef]
- Armbruster, D.A.; Pry, T. Limit of Blank, Limit of Detection and Limit of Quantitation. Clin. Biochem. Rev. 2008, 29, S49–S52. [Google Scholar] [PubMed]
- Barnes, W.L.; Dereux, A.; Ebbesen, T.W. Surface plasmon subwavelength optics. Nature 2003, 424, 824–830. [Google Scholar] [CrossRef]
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer Science and Business Media: New York, NY, USA, 2007. [Google Scholar]
- Campos, A.; Arbouet, A.; Martin, J.; Gérard, D.; Proust, J.; Plain, J.; Kociak, M. Plasmonic breathing and edge modes in aluminum nanotriangles. ACS Photonics 2017, 4, 1257–1263. [Google Scholar] [CrossRef]
- Moreno, E.; Rodrigo, S.G.; Bozhevolnyi, S.I.; Martín-Moreno, L.; García-Vidal, F.J. Guiding and focusing of electromagnetic fields with wedge plasmon polaritones. Phys. Rev. Lett. 2008, 100, 023901. [Google Scholar] [CrossRef]
- Berenschot, E.; Tiggelaar, R.M.; Borgelink, B.; Van Kampen, C.; Deenen, C.S.; Pordeli, Y.; Witteveen, H.; Gardeniers, H.J.G.E.; Tas, N.R. Self-aligned crystallographic multiplication of nanoscale silicon wedges for high-density fabrication of 3D nanodevices. ACS Appl. Nano Mater. 2022, 5, 15847–15854. [Google Scholar] [CrossRef]
- Kooijman, L.J.; Pordeli, Y.; Berenschot, J.W.; Tas, N.R. Lateral fractal formation by crystallographic silicon micromachining. Fractal Fract. 2023, 7, 202. [Google Scholar] [CrossRef]
- Berenschot, J.W.; Tiggelaar, R.M.; Geerlings, J.; Gardeniers, J.G.E.; Tas, N.R.; Malankowska, M.; Pina, M.P.; Mallada, R. 3D-Fractal Engineering Based on Oxide-Only Corner Lithography. In Proceedings of the Symposium on Design, Test, Integration and Packaging of MEMS/MOEMS (DTIP), Budapest, Hungary, 30 May–2 June 2016. [Google Scholar] [CrossRef]
- Bell, S.E.J.; Charron, G.; Cortés, E.; Kneipp, J.; Lamy de la Chapelle, M.; Langer, J.; Procházka, M.; Tran, V.; Schlücker, S. Towards reliable and quantitative surface-enhanced Raman scattering (SERS): From key parameters to good analytical practice. Angew. Chem. 2020, 132, 5496–5505. [Google Scholar] [CrossRef]


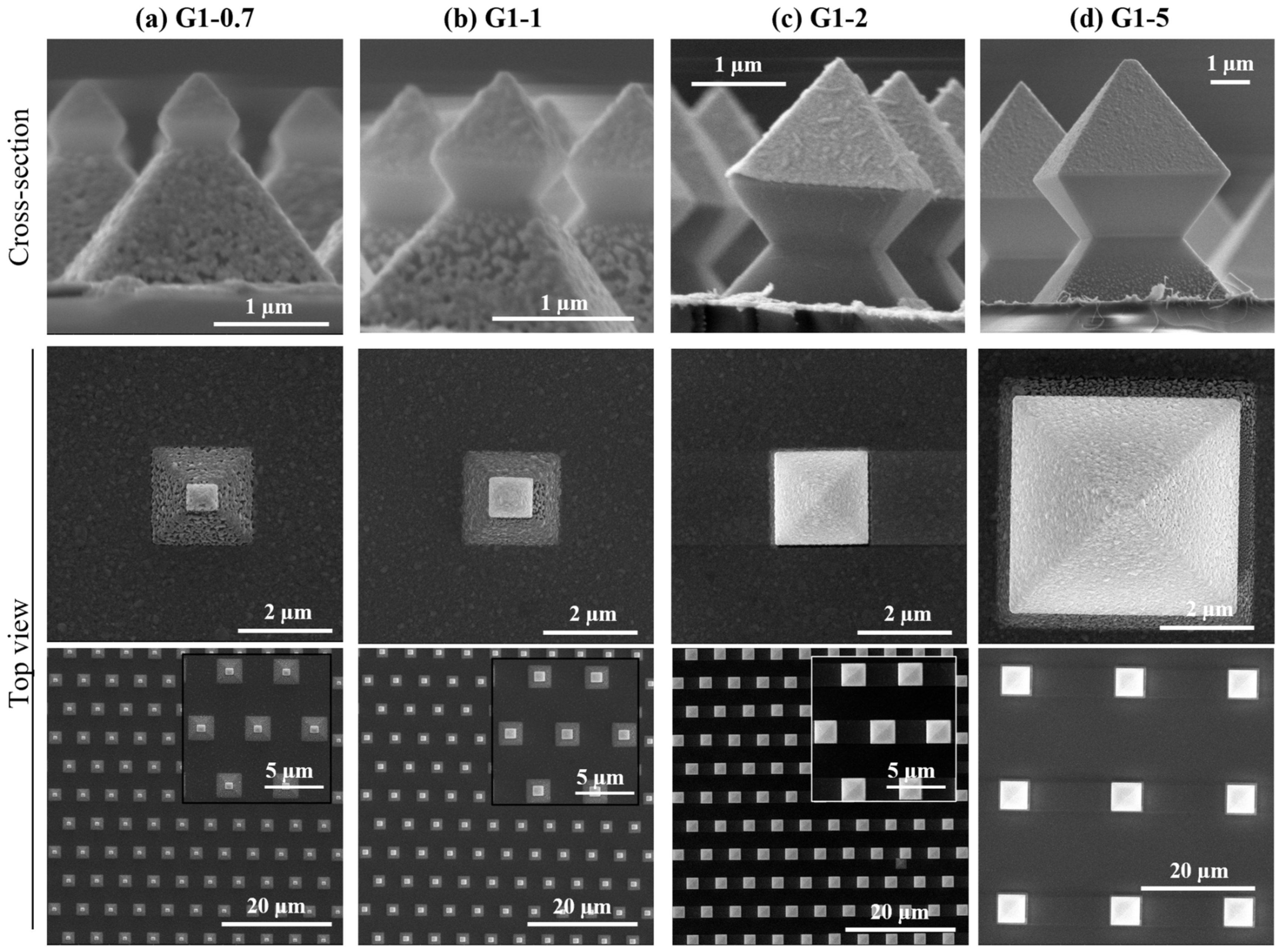

| Specimen I.D. | Widths G1 [µm] | Widths G0 [µm] | Pitch [µm] | Array Packing |
|---|---|---|---|---|
| G1-0.7 | 0.68 ± 0.04 | 2.20 ± 0.04 | 4.95 ± 0.04 | Hexagonal |
| 0.58 ± 0.01 | 2.15 ± 0.04 | |||
| G1-1 | 0.95 ± 0.03 | 2.08 ± 0.04 | 4.93 ± 0.03 | Hexagonal |
| 0.88 ± 0.03 | 2.03 ± 0.04 | |||
| G1-2 | 2.10 ± 0.06 | 2.28 ± 0.07 | 4.94 ± 0.04 | Hexagonal |
| 2.02 ± 0.01 | 2.19 ± 0.08 | |||
| G1-5 | 4.85 ± 0.14 | 5.58 ± 0.13 | 19.87 ± 0.11 | Square |
| 4.83 ± 0.08 | 5.52 ± 0.08 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lafuente, M.; Kooijman, L.J.; Rodrigo, S.G.; Berenschot, E.; Mallada, R.; Pina, M.P.; Tas, N.R.; Tiggelaar, R.M. Periodic Arrays of Plasmonic Ag-Coated Multiscale 3D-Structures with SERS Activity: Fabrication, Modelling and Characterisation. Micromachines 2024, 15, 1129. https://doi.org/10.3390/mi15091129
Lafuente M, Kooijman LJ, Rodrigo SG, Berenschot E, Mallada R, Pina MP, Tas NR, Tiggelaar RM. Periodic Arrays of Plasmonic Ag-Coated Multiscale 3D-Structures with SERS Activity: Fabrication, Modelling and Characterisation. Micromachines. 2024; 15(9):1129. https://doi.org/10.3390/mi15091129
Chicago/Turabian StyleLafuente, Marta, Lucas J. Kooijman, Sergio G. Rodrigo, Erwin Berenschot, Reyes Mallada, María P. Pina, Niels R. Tas, and Roald M. Tiggelaar. 2024. "Periodic Arrays of Plasmonic Ag-Coated Multiscale 3D-Structures with SERS Activity: Fabrication, Modelling and Characterisation" Micromachines 15, no. 9: 1129. https://doi.org/10.3390/mi15091129
APA StyleLafuente, M., Kooijman, L. J., Rodrigo, S. G., Berenschot, E., Mallada, R., Pina, M. P., Tas, N. R., & Tiggelaar, R. M. (2024). Periodic Arrays of Plasmonic Ag-Coated Multiscale 3D-Structures with SERS Activity: Fabrication, Modelling and Characterisation. Micromachines, 15(9), 1129. https://doi.org/10.3390/mi15091129











