Active, Reactive, and Apparent Power in Dielectrophoresis: Force Corrections from the Capacitive Charging Work on Suspensions Described by Maxwell-Wagner’s Mixing Equation
Abstract
:1. Introduction
2. Theory
2.1. General Remarks
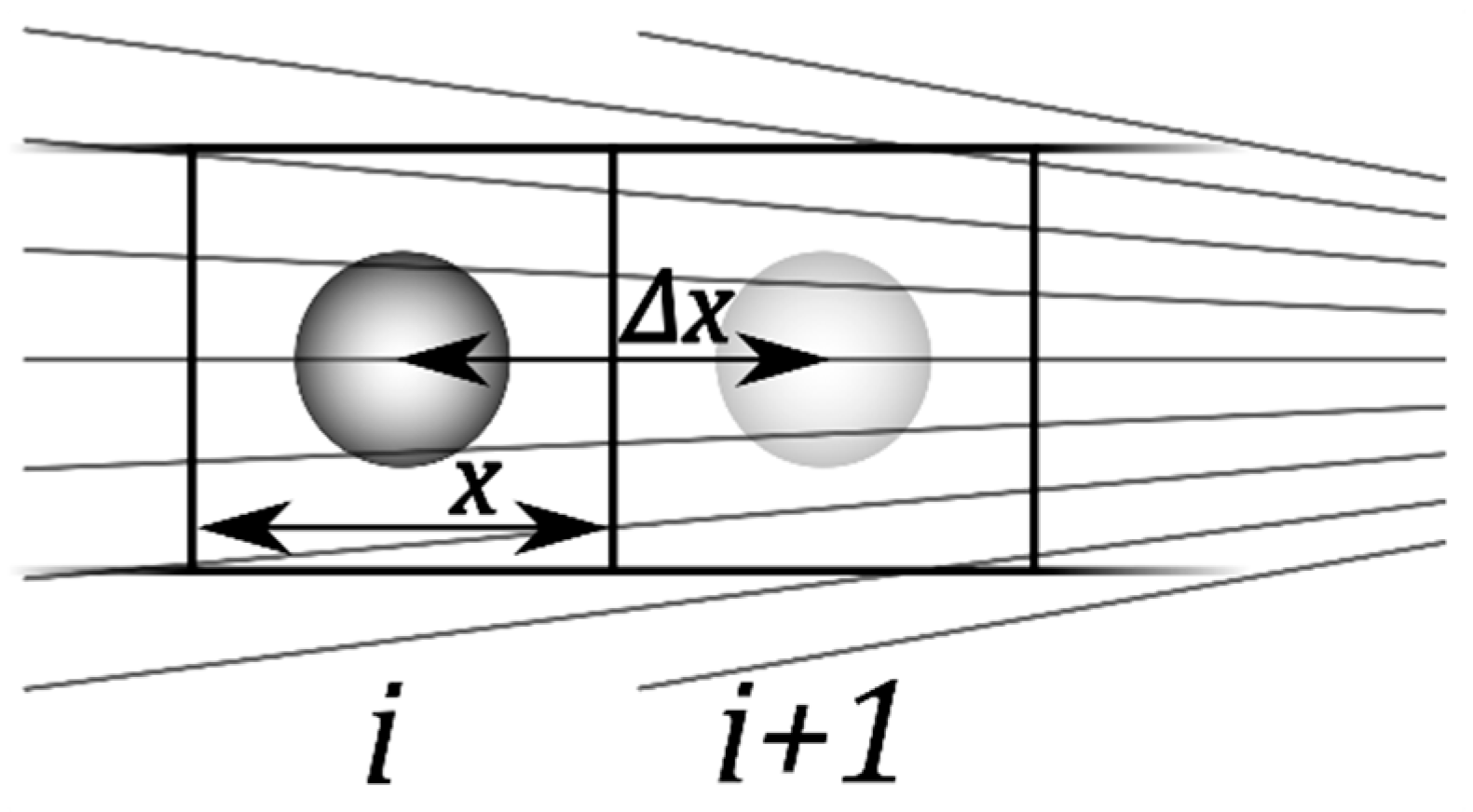
2.2. Approximation of the Field Gradient
2.3. DEP Force in the Classical Dipole Approximation
2.4. Charging Work for External and Suspension Media Boxes
2.5. DEP Force Approximation by a Capacitor-Charging Cycle
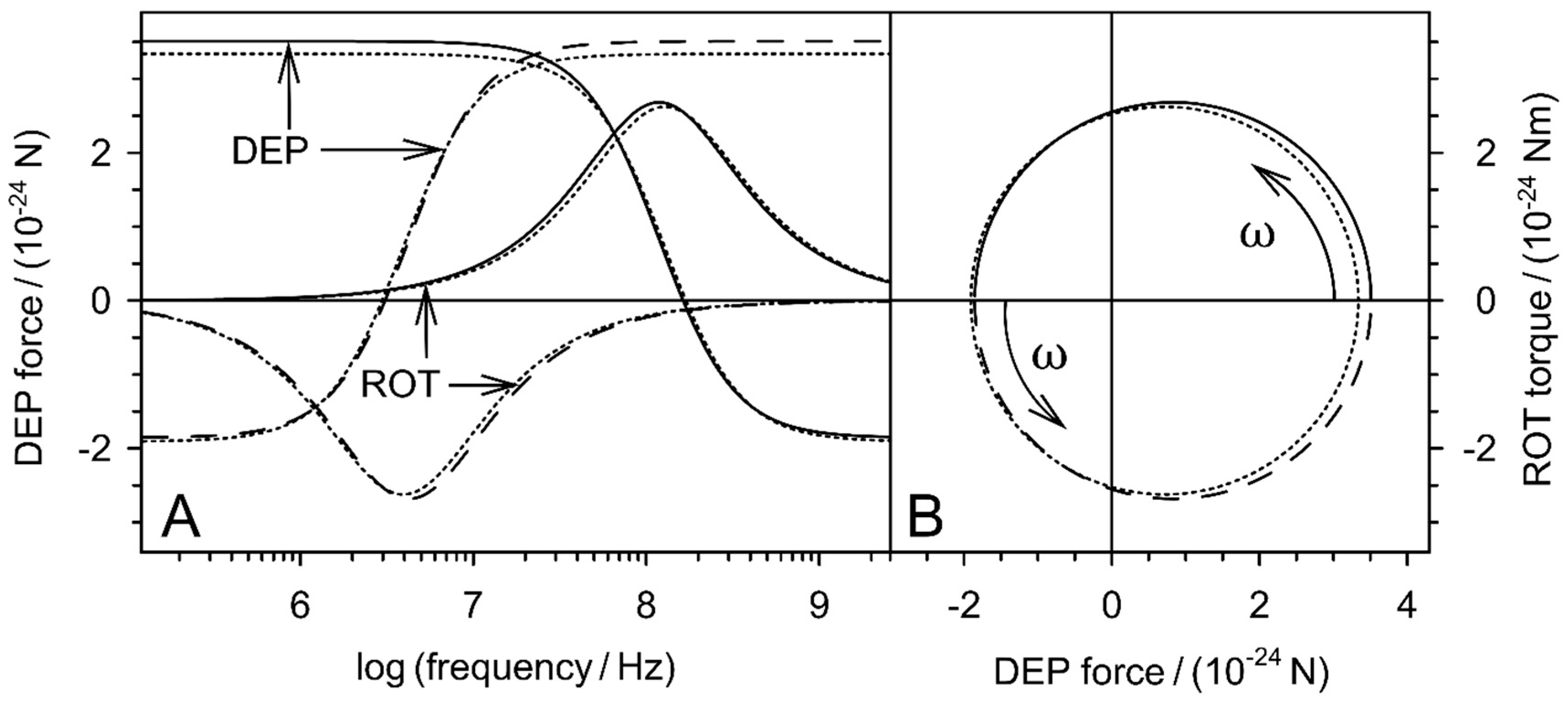
2.6. Electrorotation (ROT) Torque
3. Modelling Results and Discussion
3.1. Model Parameters
- I
- = 0.01 S/m, with = 800, and
- II
- = 1 S/m with = 8.
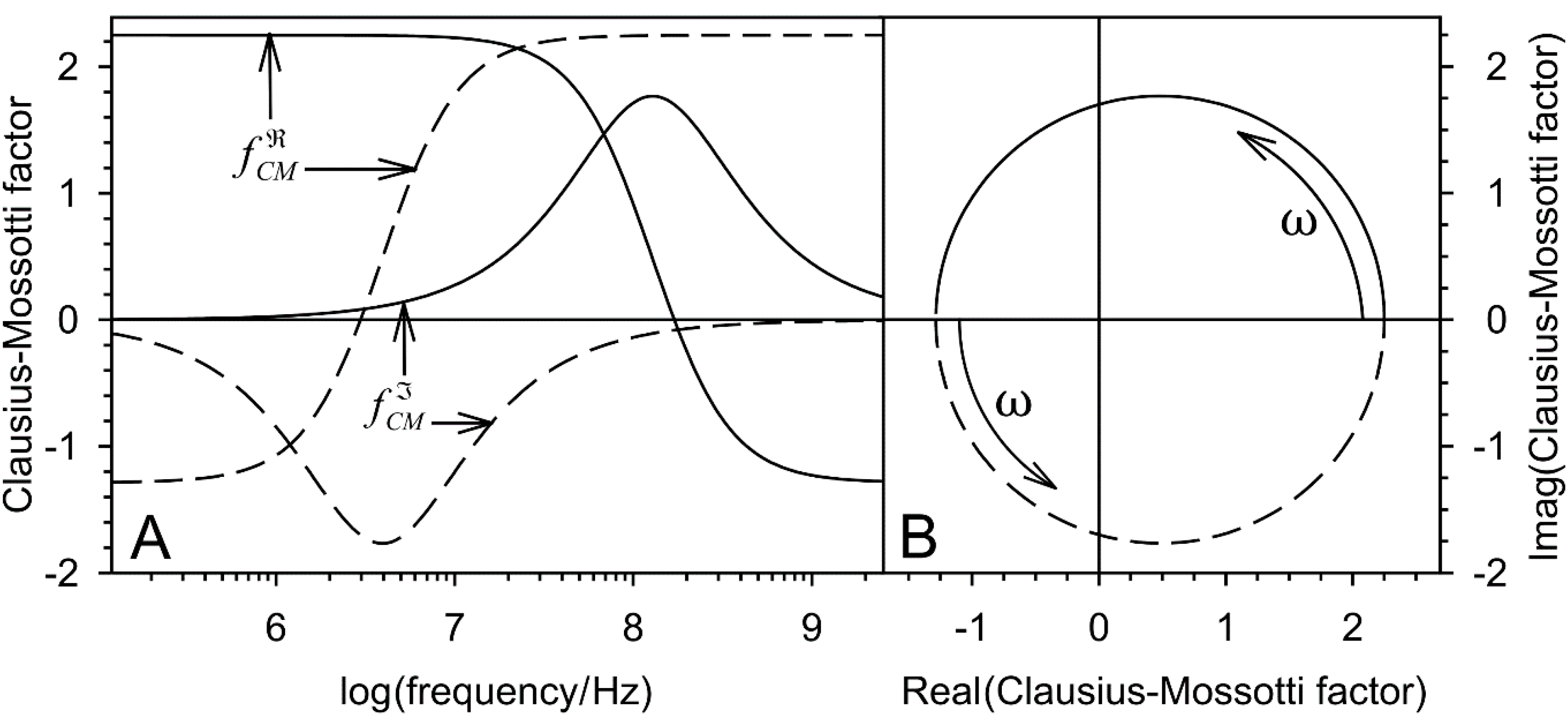
3.2. Clausius-Mossotti Factor
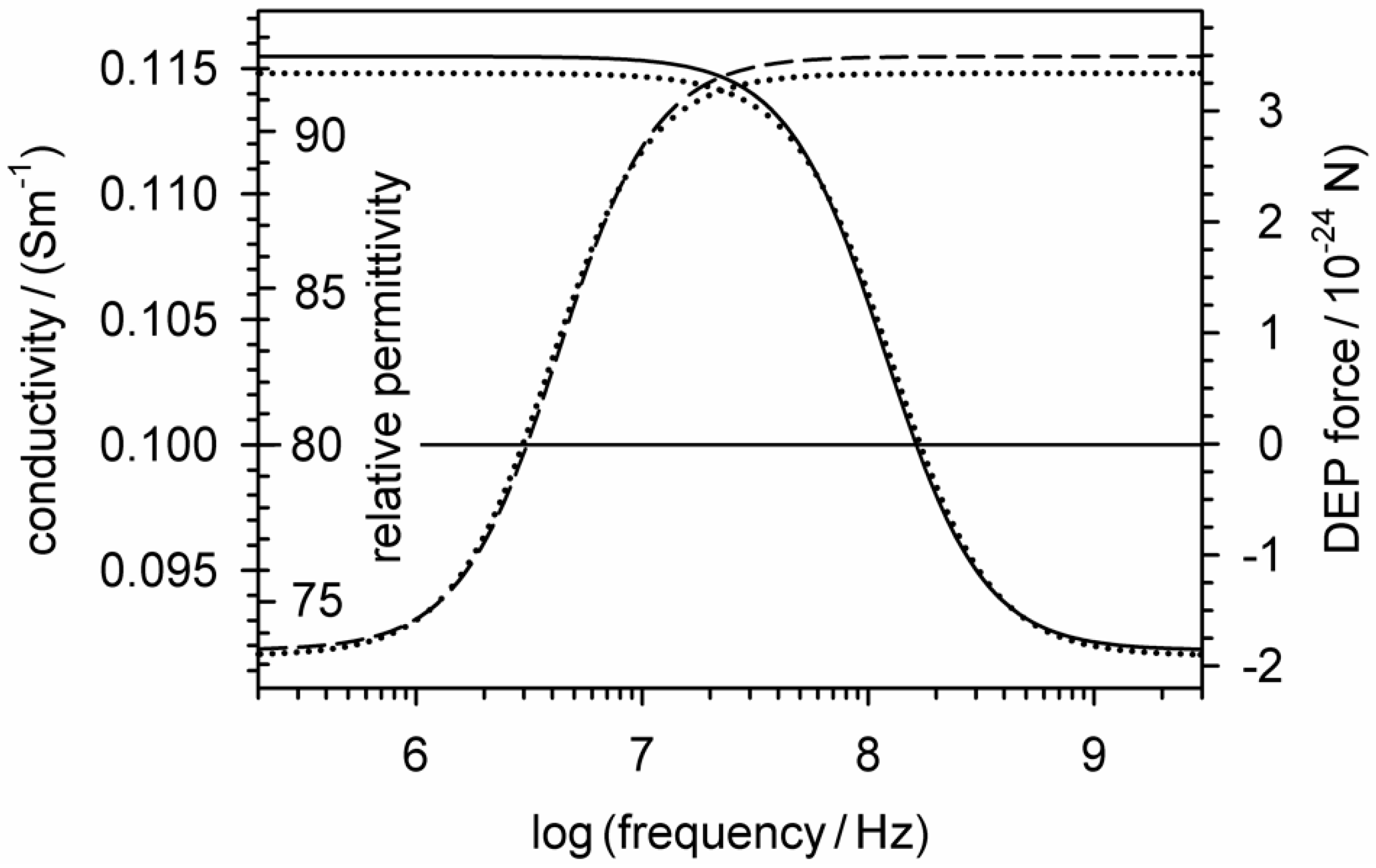
3.3. Conductivity and Dissipation
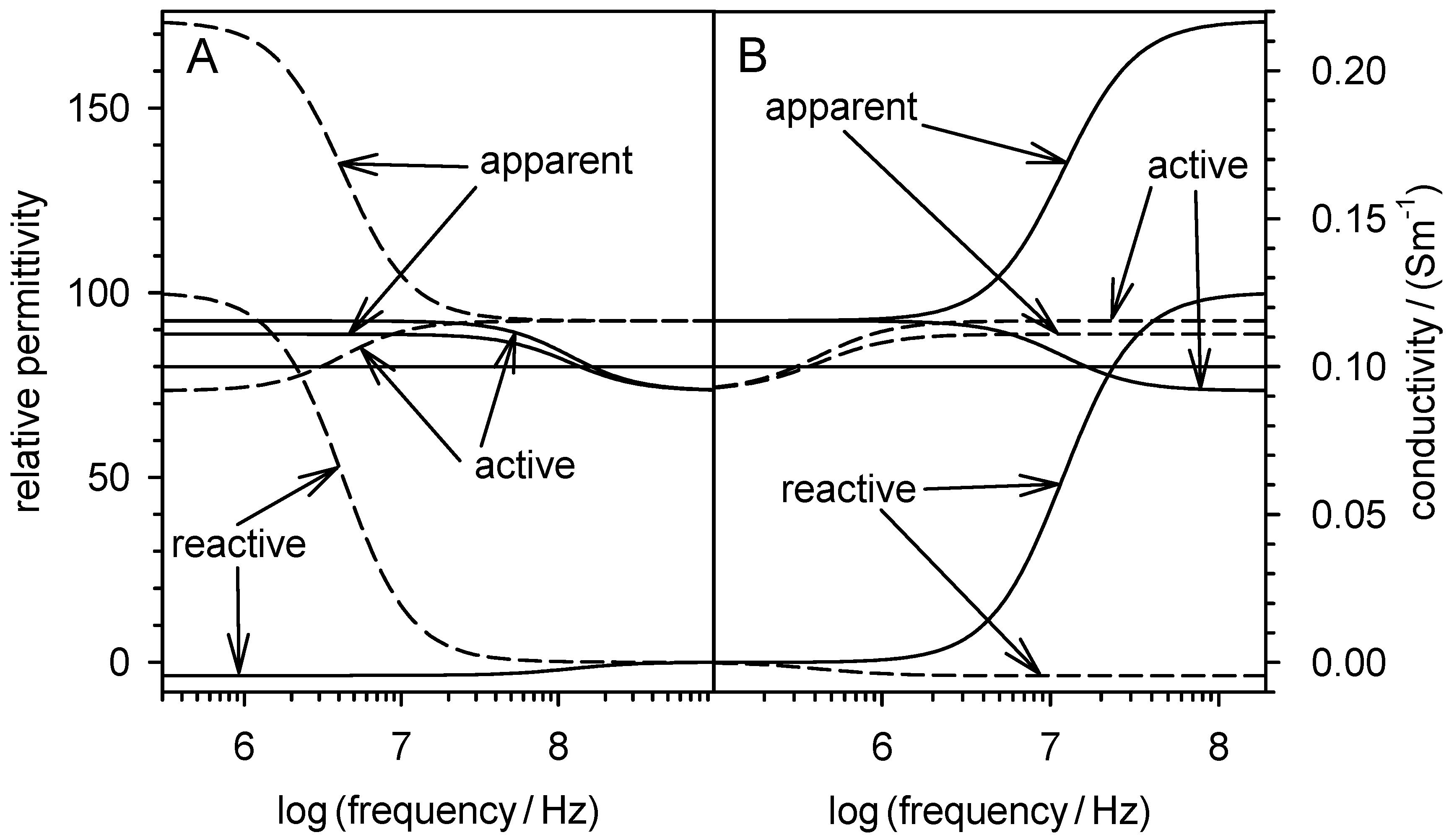
3.4. Dispersion Relation, Active, Reactive, and Apparent Power
3.5. Dissipation and Charging Work in the Box Chain
3.6. DEP Force in the Box Chain
4. General Discussion
4.1. Higher Precision for the DEP Force?
4.2. DEP as Conditioned Polarization Process
4.3. Relations to the Law of Maximum Entropy Production (LMEP)
5. Conclusions and Outlook
Supplementary Materials
Funding
Acknowledgments
Conflicts of Interest
References
- Gimsa, J.; Wachner, D. A Unified Resistor-Capacitor Model for Impedance, Dielectrophoresis, Electrorotation, and Induced Transmembrane Potential. Biophys. J. 1998, 75, 1107–1116. [Google Scholar] [CrossRef] [Green Version]
- Foster, K.R.; Schwan, H.P. Dielectric Properties of Tissues. In Handbook of Biological Effects of Electromagnetic Fields, 2nd ed.; Polk, C., Pastow, E., Eds.; CRC Press: Boca Raton, FL, USA, 1996; pp. 25–102. [Google Scholar]
- Jones, T.B. Electromechanics of Particles; Cambridge University Press: Cambridge, UK; New York, NY, USA, 1995; ISBN 9780521431965. [Google Scholar]
- Gimsa, J. Can the law of maximum entropy production describe the field-induced orientation of ellipsoids of rotation? J. Phys. Commun. 2020, 4, 085017. [Google Scholar] [CrossRef]
- Fuhr, G.R.; Gimsa, J.; Glaser, R. Interpretation of electrorotation of protoplasts. 1. Theoretical considerations. Studia Biophys. 1985, 108, 149–164. [Google Scholar]
- Gimsa, J. A comprehensive approach to electro-orientation, electrodeformation, dielectrophoresis, and electrorotation of ellipsoidal particles and biological cells. B1101. Bioelectrochemistry 2001, 54, 23–31. [Google Scholar] [CrossRef]
- Huang, J.P.; Karttunen, M.; Yu, K.W.; Dong, L.; Gu, G.Q. Electrokinetic behavior of two touching inhomogeneous biological cells and colloidal particles: Effects of multipolar interactions. Phys. Rev. E 2004, 69, 51402. [Google Scholar] [CrossRef] [Green Version]
- Barat, D.; Spencer, D.; Benazzi, G.; Mowlem, M.C.; Morgan, H. Simultaneous high speed optical and impedance analysis of single particles with a microfluidic cytometer. Lab. Chip 2012, 12, 118–126. [Google Scholar] [CrossRef]
- Chen, Q.; Yuan, Y.J. A review of polystyrene bead manipulation by dielectrophoresis. RSC Adv. 2019, 9, 4963–4981. [Google Scholar] [CrossRef] [Green Version]
- Jiang, T.; Jia, Y.; Sun, H.; Deng, X.; Tang, D.; Ren, Y. Dielectrophoresis Response of Water-in-Oil-in-Water Double Emulsion Droplets with Singular or Dual Cores. Micromachines 2020, 11, 1121. [Google Scholar] [CrossRef] [PubMed]
- Morgan, H.; Holmes, D.; Green, N.G. High speed simultaneous single particle impedance and fluorescence analysis on a chip. Curr. Appl. Phys. 2006, 6, 367–370. [Google Scholar] [CrossRef] [Green Version]
- Ramos, A.; Morgan, H.; Green, N.G.; Castellanos, A. AC electrokinetics: A review of forces in microelectrode structures. J. Phys. D Appl. Phys. 1998, 31, 2338–2353. [Google Scholar] [CrossRef] [Green Version]
- Landau, L.D.; Lifšic, E.M.; Pitaevskij, L.P. Electrodynamics of Continuous Media, 2nd ed.; Pergamon Press: Oxford, UK, 1984; ISBN 0080302750. [Google Scholar]
- Gimsa, J.; Wachner, D. A Polarization Model Overcoming the Geometric Restrictions of the Laplace Solution for Spheroidal Cells: Obtaining New Equations for Field-Induced Forces and Transmembrane Potential. B1115. Biophys. J. 1999, 77, 1316–1326. [Google Scholar] [CrossRef] [Green Version]
- Maxwell, J.C. A Treatise on Electricity and Magnetism; Clarendon Press: Oxford, UK, 1873; ISBN 0198503733. [Google Scholar]
- Wagner, K.W. Erklärung der dielektrischen Nachwirkungsvorgänge auf Grund Maxwellscher Vorstellungen. Archiv. F. Elektrotechnik 1914, 2, 371–387. [Google Scholar] [CrossRef] [Green Version]
- Pastushenko, V.P.; Kuzmin, P.I.; Chizmadshev, Y.A. Dielectrophoresis and electrorotation: A unified theory of spherically symmetrical cells. Studia Biophys. 1985, 110, 51–57. [Google Scholar]
- Asami, K.; Hanai, T.; Koizumi, N. Dielectric Approach to Suspensions of Ellipsoidal Particles Covered with a Shell in Particular Reference to Biological Cells. Jpn. J. Appl. Phys. 1980, 19, 359–365. [Google Scholar] [CrossRef]
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering of Light by Small Particles; Wiley: New York, NY, USA, 1998; ISBN 9780471293408. [Google Scholar]
- Kakutani, T.; Shibatani, S.; Sugai, M. Electrorotation of non-spherical cells: Theory for ellipsoidal cells with an arbitrary number of shells. Bioelectrochem. Bioenerg. 1993, 31, 131–145. [Google Scholar] [CrossRef]
- Sokirko, A.V. The electrorotation of axisymmetrical cell. Biol. Mem. 1992, 6, 587–600. [Google Scholar]
- Stubbe, M.; Gimsa, J. Maxwell’s Mixing Equation Revisited: Characteristic Impedance Equations for Ellipsoidal Cells. Biophys. J. 2015, 109, 194–208. [Google Scholar] [CrossRef] [Green Version]
- Gimsa, J.; Gimsa, U. The influence of insulating and conductive ellipsoidal objects on the impedance and permittivity of media. J. Electrostat. 2017, 90, 131–138. [Google Scholar] [CrossRef]
- Gimsa, J.; Stubbe, M.; Gimsa, U. A short tutorial contribution to impedance and AC-electrokinetic characterization and manipulation of cells and media: Are electric methods more versatile than acoustic and laser methods? J. Electr. Bioimpedance 2014, 5, 74–91. [Google Scholar] [CrossRef] [Green Version]
- Hanai, T. Electrical properties of emulsions. In Emulsion Science; Sherman, P., Ed.; Academic Press: London, UK; New York, NY, USA, 1968; pp. 354–477. [Google Scholar]
- Tuncer, E.; Gubański, S.M.; Nettelblad, B. Dielectric relaxation in dielectric mixtures: Application of the finite element method and its comparison with dielectric mixture formulas. J. Appl. Phys. 2001, 89, 8092–8100. [Google Scholar] [CrossRef]
- Swenson, R. The fourth law of thermodynamics or the law of maximum entropy production (LMEP). Chemistry 2009, 18, 333–339. [Google Scholar]
- Stenholm, S. On entropy production. Ann. Phys. 2008, 323, 2892–2904. [Google Scholar] [CrossRef]
- Niven, R.K. Steady state of a dissipative flow-controlled system and the maximum entropy production principle. Phys. Rev. E 2009, 80, 21113. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Grinstein, G.; Linsker, R. Comments on a derivation and application of the ‘maximum entropy production’ principle. J. Phys. A Math. Theor. 2007, 40, 9717–9720. [Google Scholar] [CrossRef]
- Atkins, P.W. Physical Chemistry, 5th ed.; Oxford University Press: Oxford, UK, 1994. [Google Scholar]
- Glaser, R. Biophysics: An Introduction, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2012; ISBN 9783642252129. [Google Scholar]
- Scaife, B. On the Rayleigh dissipation function for dielectric media. J. Mol. Liq. 1989, 43, 101–107. [Google Scholar] [CrossRef]
- Gimsa, J. New Light-Scattering and Field-Trapping Methods Access the Internal Electric Structure of Submicron Particles, like Influenza Viruses. In Electrical Bioimpedance Methods: Applications to Medicine and Biotechnology; Riu, P.J., Ed.; New York Academy of Sciences: New York, NY, USA, 1999; pp. 287–298. ISBN 1-57331-191-X. [Google Scholar]
- Hölzel, R.; Pethig, R. Protein Dielectrophoresis: I. Status of Experiments and an Empirical Theory. Micromachines 2020, 11, 533. [Google Scholar] [CrossRef] [PubMed]
- Green, N.G.; Ramos, A.; Morgan, H. AC electrokinetics: A survey of sub-micrometre particle dynamics. J. Phys. D Appl. Phys. 2000, 33, 632–641. [Google Scholar] [CrossRef] [Green Version]
- Zheng, X.; Palffy-Muhoray, P. Electrical energy storage and dissipation in materials. Phys. Lett. A 2015, 379, 1853–1856. [Google Scholar] [CrossRef] [Green Version]

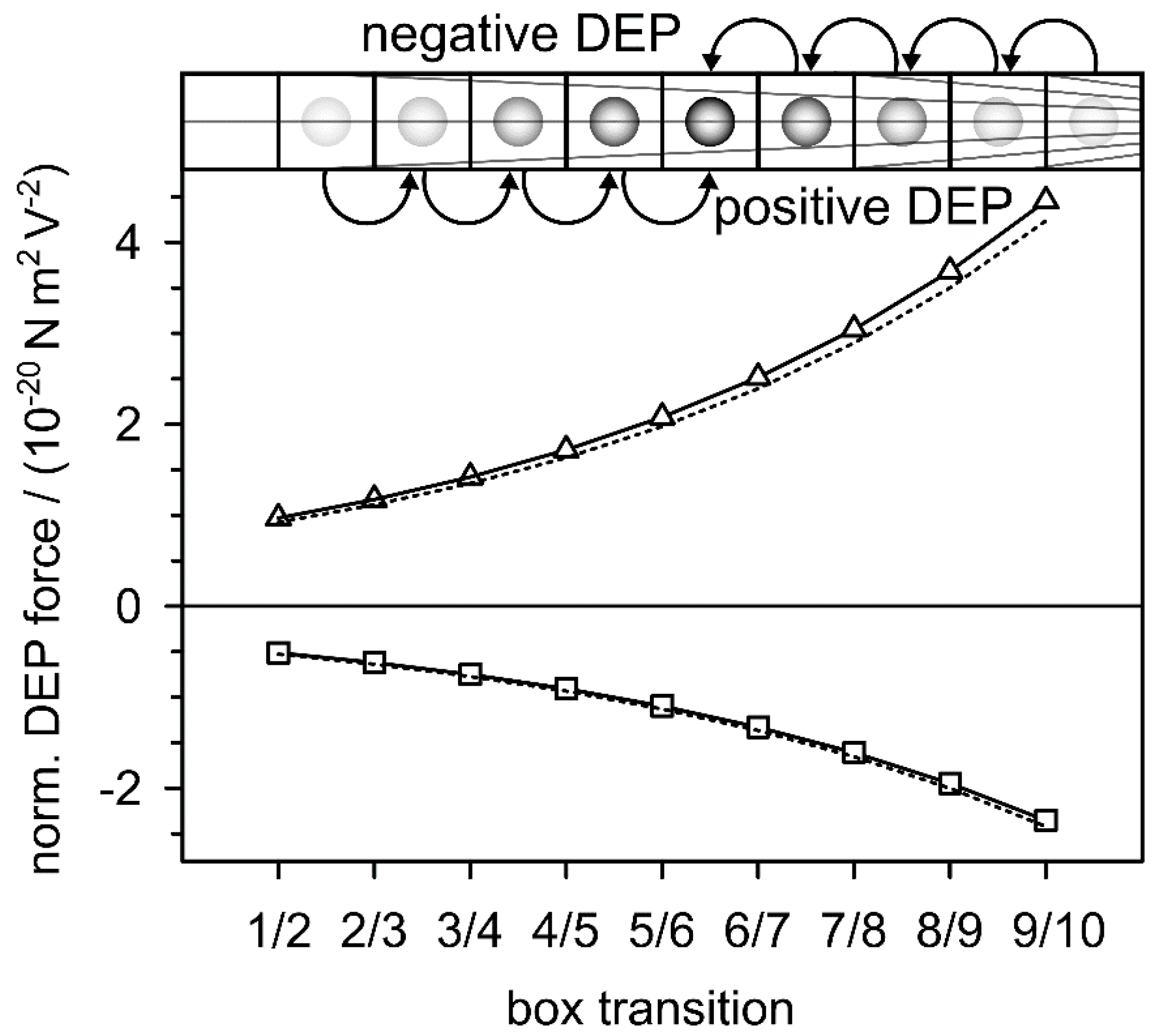
| Box | Normalized Field | Normalized Field Squared | Transition from Box | Squared Normalized Mean Field at Box Interfaces |
| 1 | 1 | 1 | ||
| 2 | 1.1 | 1.21 | 1.1025 | |
| 3 | 1.21 | 1.4641 | 1.3340 | |
| 4 | 1.331 | 1.7716 | 1.6142 | |
| 5 | 1.4641 | 2.1436 | 1.9531 | |
| 6 | 1.6105 | 2.5937 | 2.3633 | |
| 7 | 1.7716 | 3.1384 | 2.8596 | |
| 8 | 1.9487 | 3.7975 | 3.4601 | |
| 9 | 2.1436 | 4.5950 | 4.1867 | |
| 10 | 2.3579 | 5.5599 | 5.0660 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gimsa, J. Active, Reactive, and Apparent Power in Dielectrophoresis: Force Corrections from the Capacitive Charging Work on Suspensions Described by Maxwell-Wagner’s Mixing Equation. Micromachines 2021, 12, 738. https://doi.org/10.3390/mi12070738
Gimsa J. Active, Reactive, and Apparent Power in Dielectrophoresis: Force Corrections from the Capacitive Charging Work on Suspensions Described by Maxwell-Wagner’s Mixing Equation. Micromachines. 2021; 12(7):738. https://doi.org/10.3390/mi12070738
Chicago/Turabian StyleGimsa, Jan. 2021. "Active, Reactive, and Apparent Power in Dielectrophoresis: Force Corrections from the Capacitive Charging Work on Suspensions Described by Maxwell-Wagner’s Mixing Equation" Micromachines 12, no. 7: 738. https://doi.org/10.3390/mi12070738
APA StyleGimsa, J. (2021). Active, Reactive, and Apparent Power in Dielectrophoresis: Force Corrections from the Capacitive Charging Work on Suspensions Described by Maxwell-Wagner’s Mixing Equation. Micromachines, 12(7), 738. https://doi.org/10.3390/mi12070738







