Prediction on Flow and Thermal Characteristics of Ultrathin Lubricant Film of Hydrodynamic Journal Bearing
Abstract
:1. Introduction
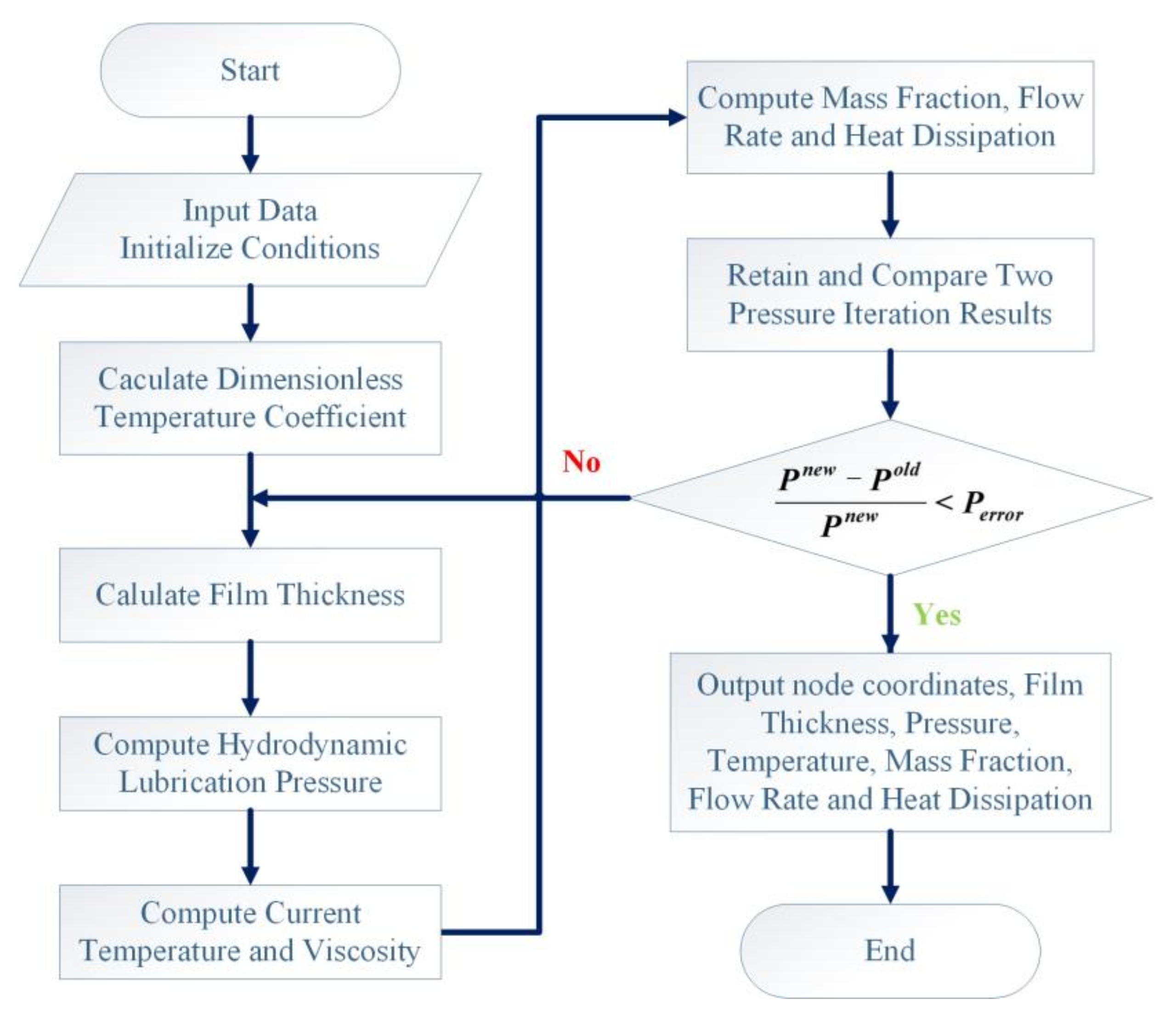
2. Numerical Method
2.1. Physical Model
2.2. Governing Equations
2.2.1. Reynolds Equation
2.2.2. Energy Equation
2.2.3. Viscosity-Temperature Equation
2.2.4. Cavitation
2.2.5. Sommerfeld Number
2.3. Boundary Conditions
2.3.1. No-Slip Boundary Conditions
2.3.2. Slip Boundary Conditions
2.4. Mesh Independence Verification
2.5. Validation of Model
3. Results and Discussion
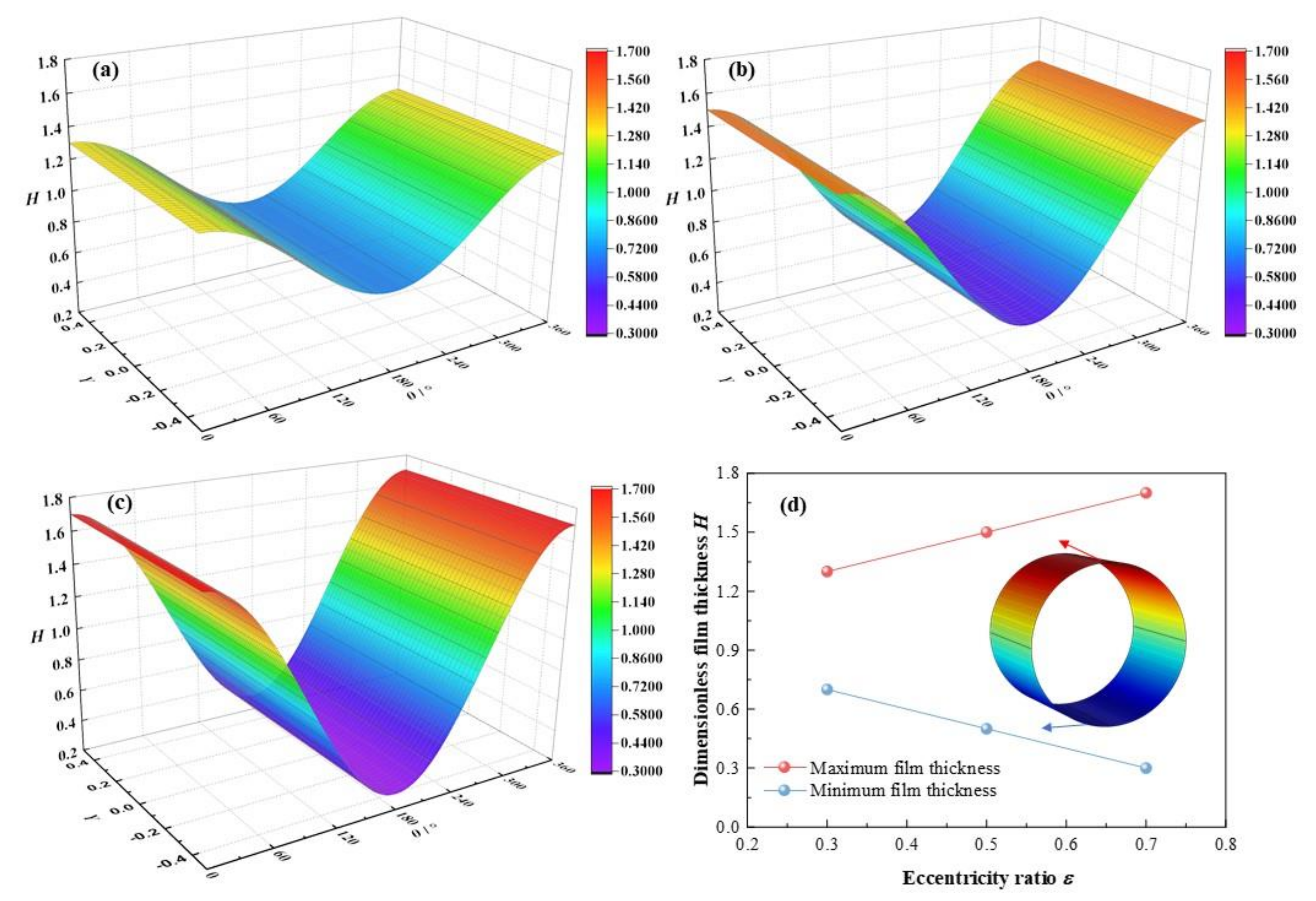
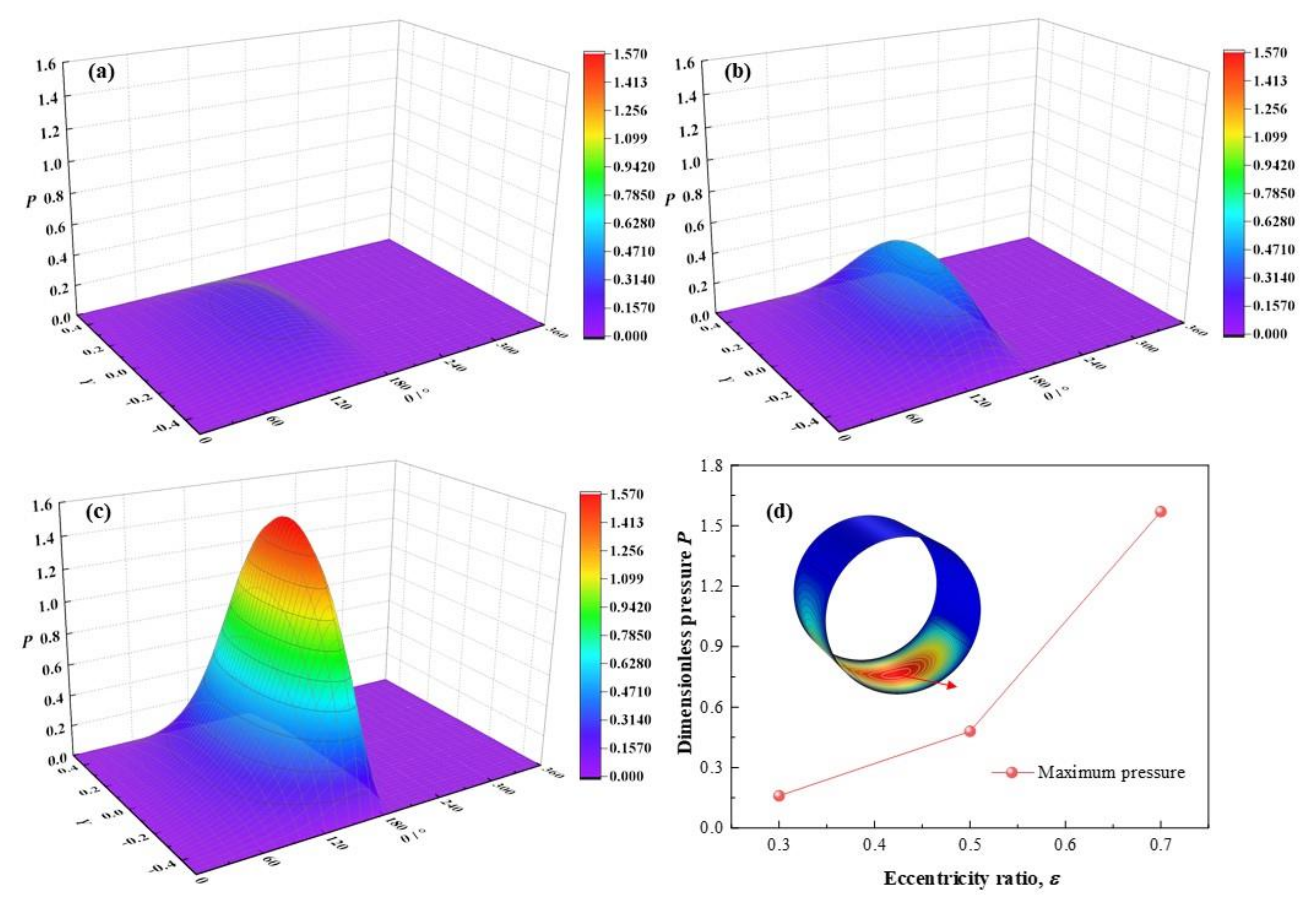
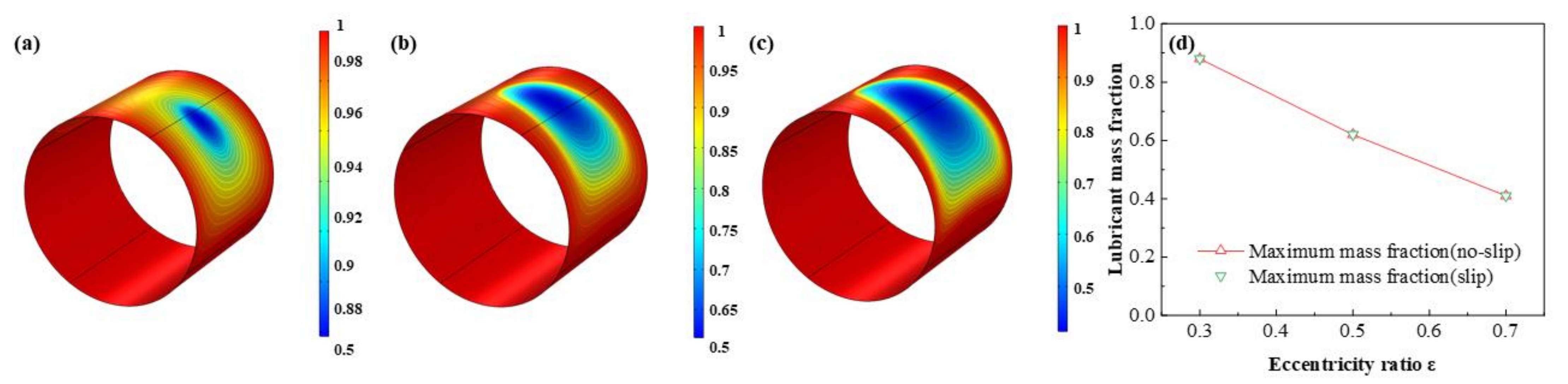
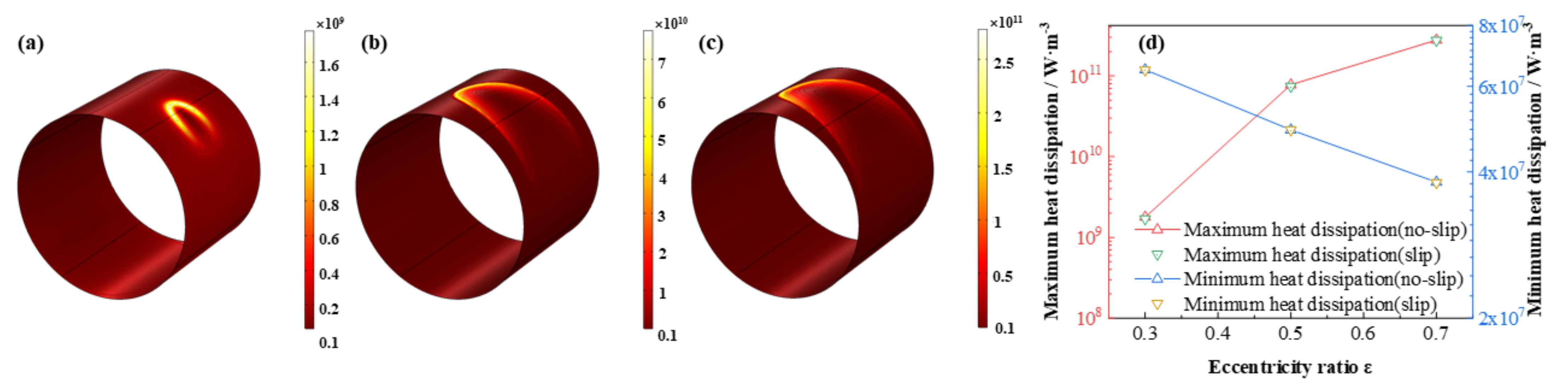
3.1. Effects of Eccentricity Ratios
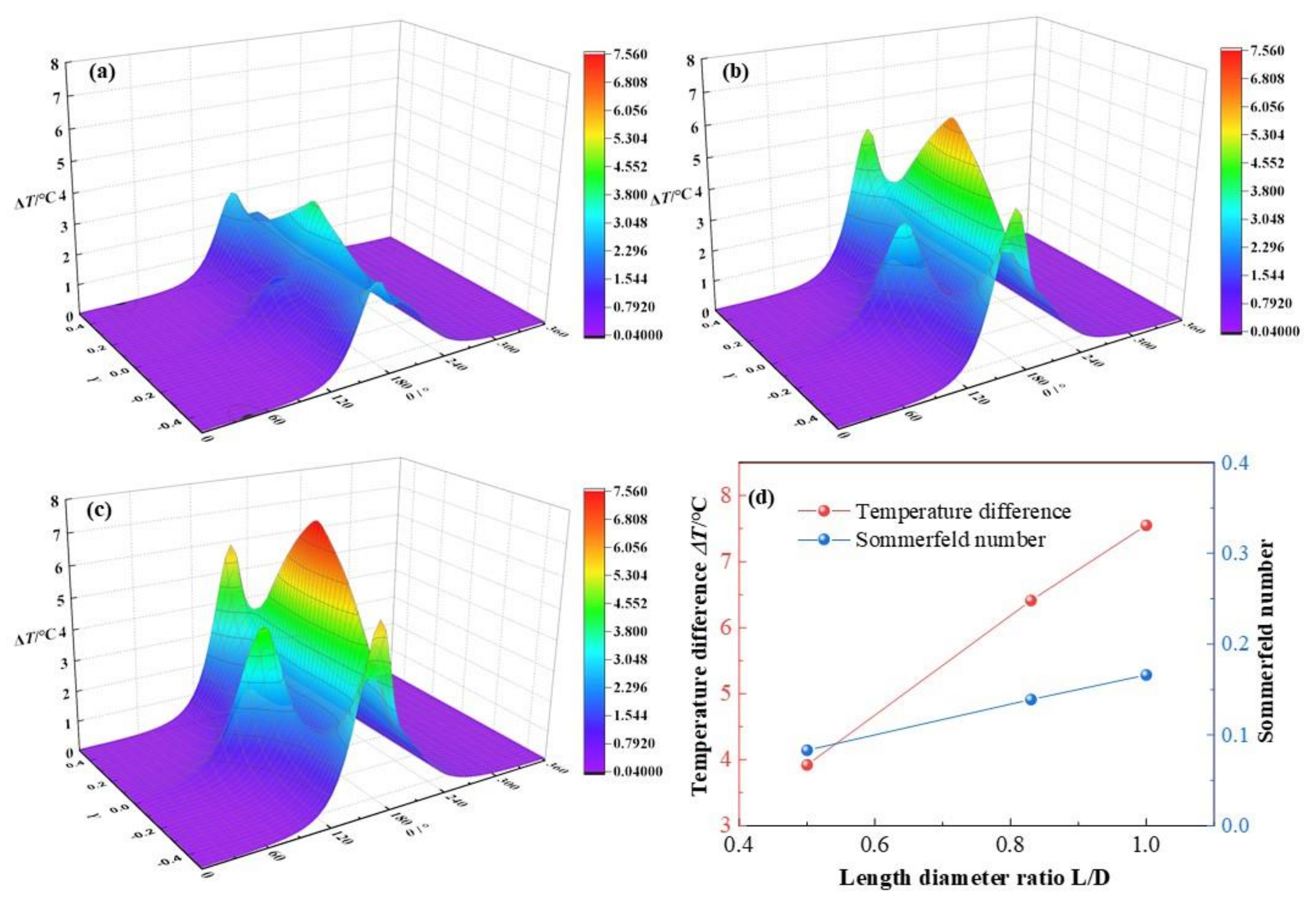
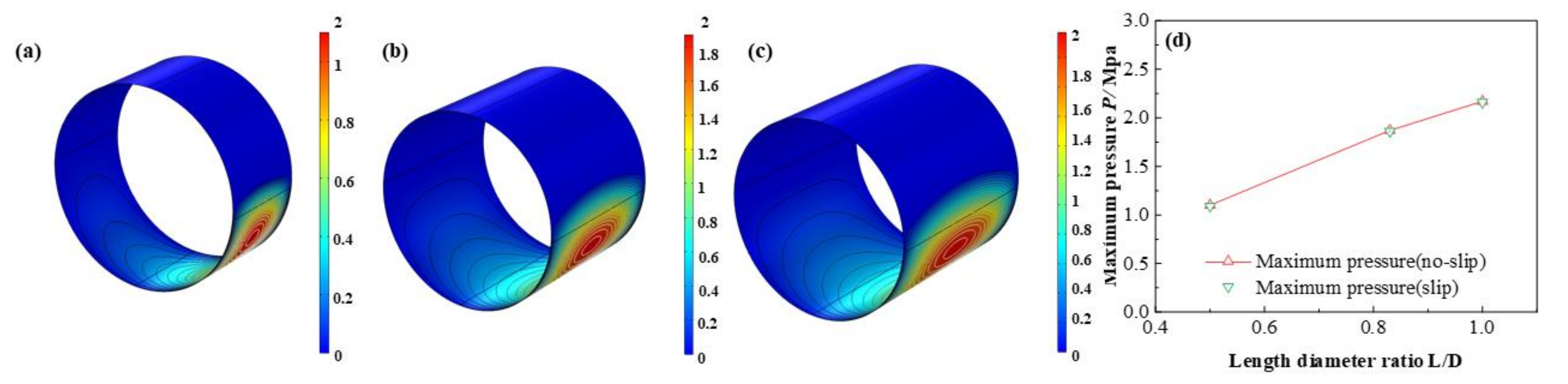
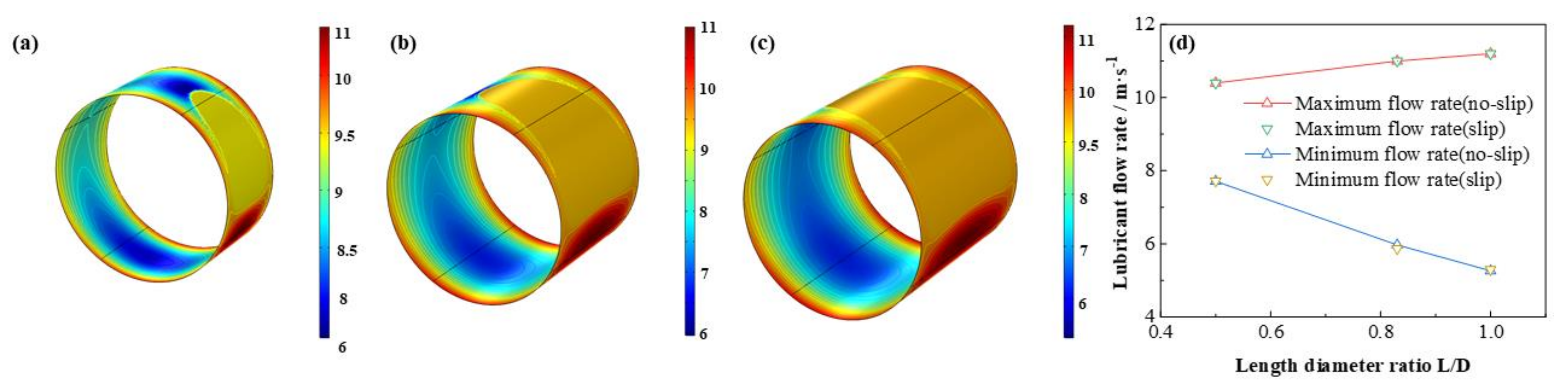
3.2. Effects of Length Diameter Ratios
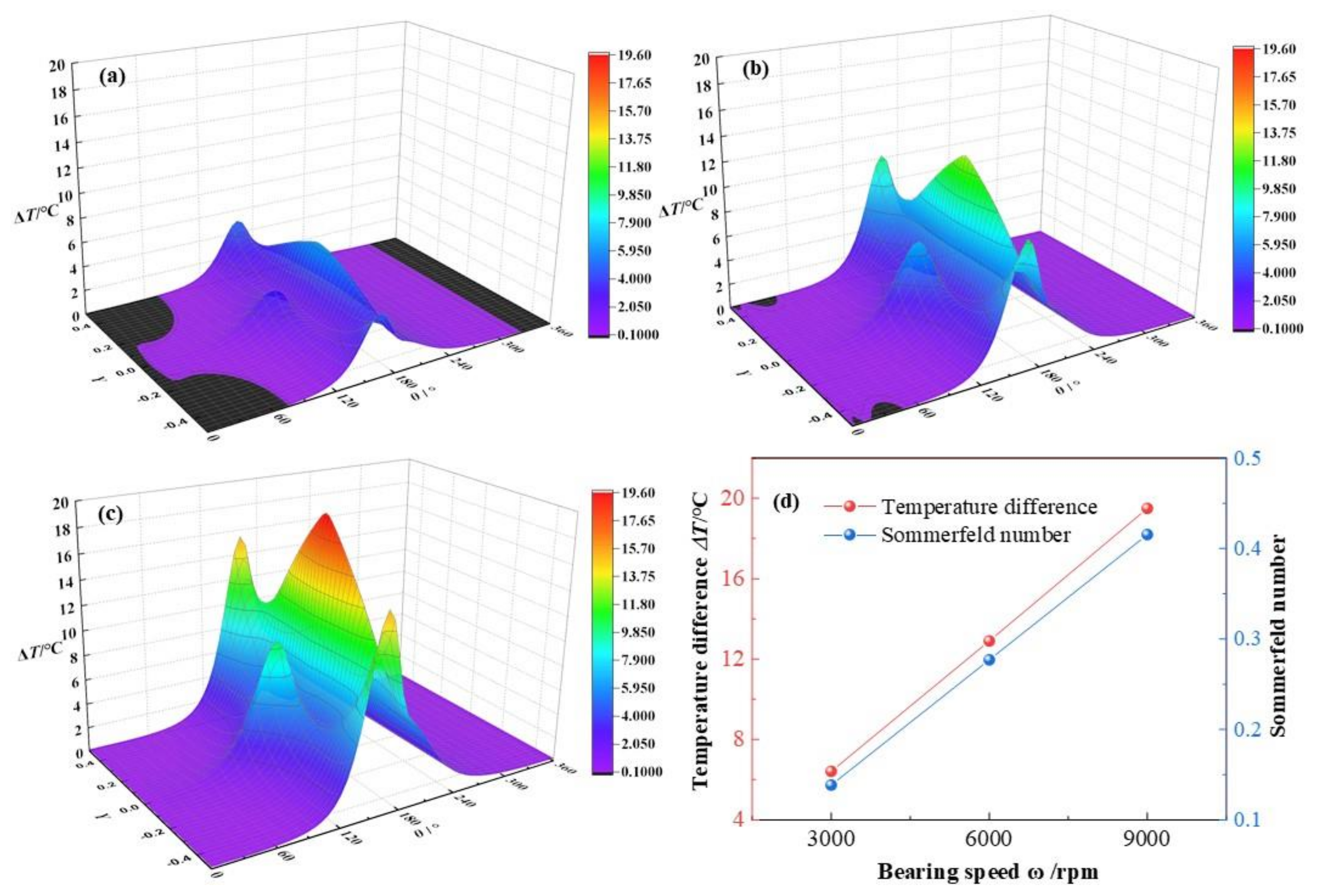
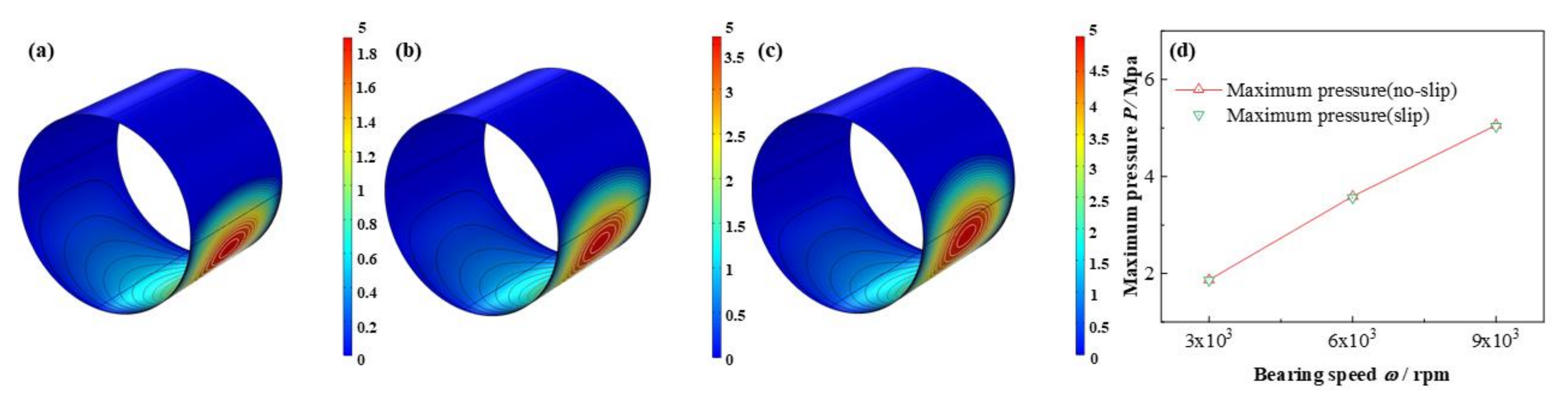
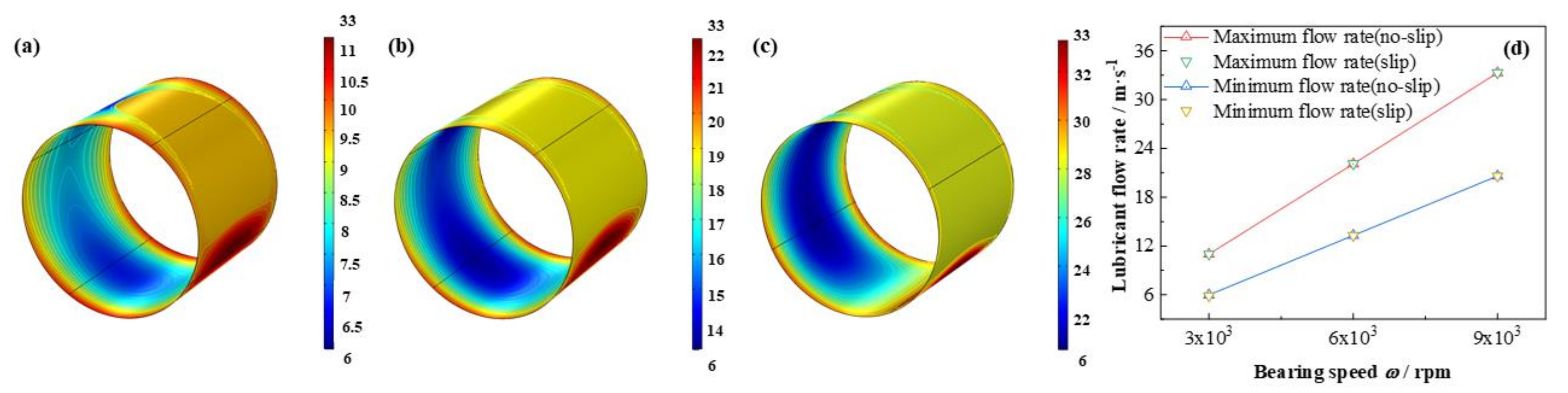
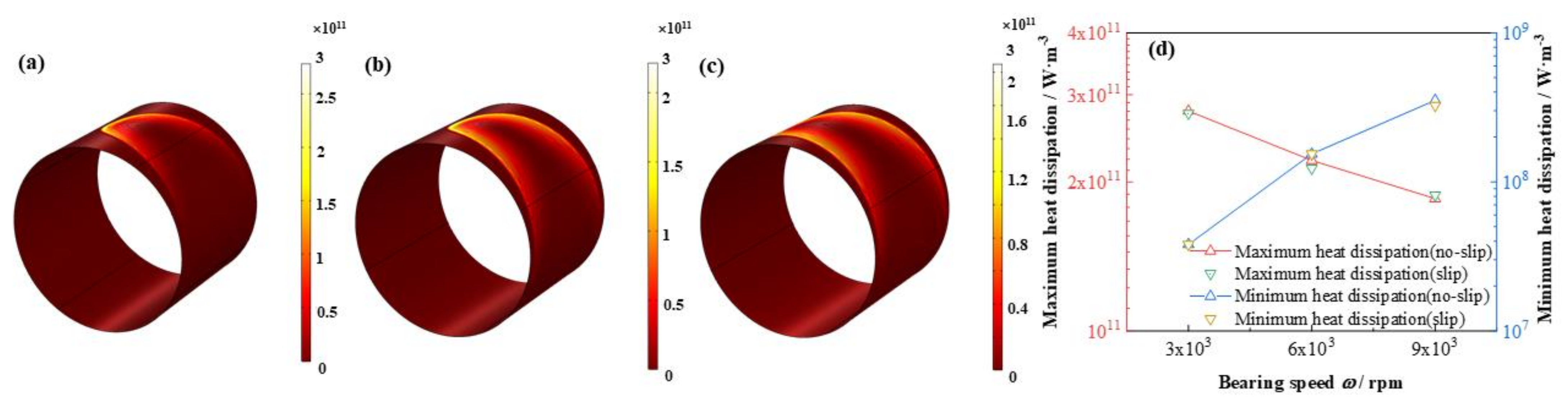
3.3. Effects of Bearing Speeds
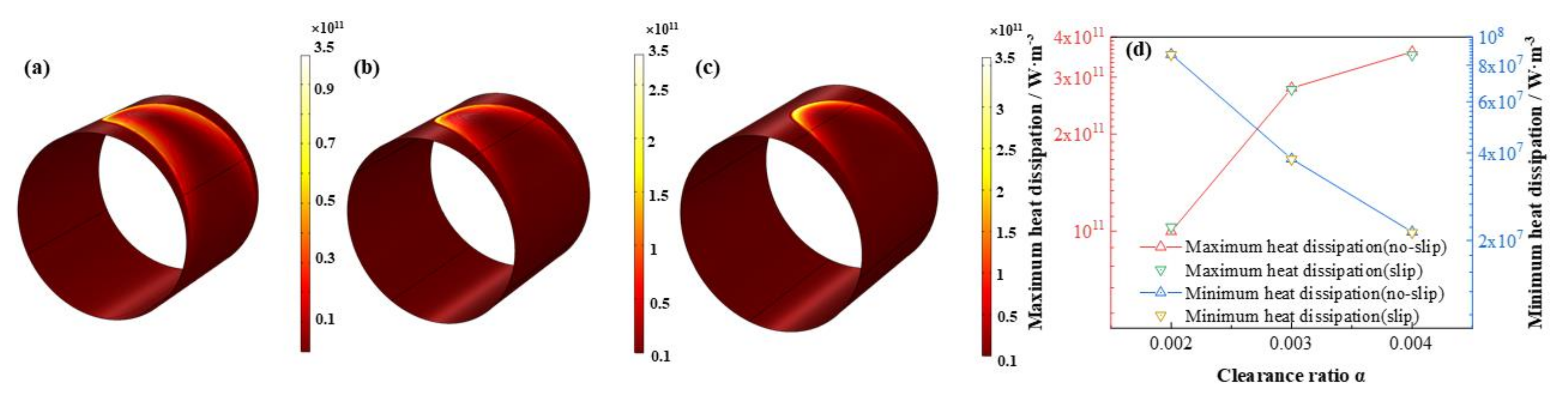
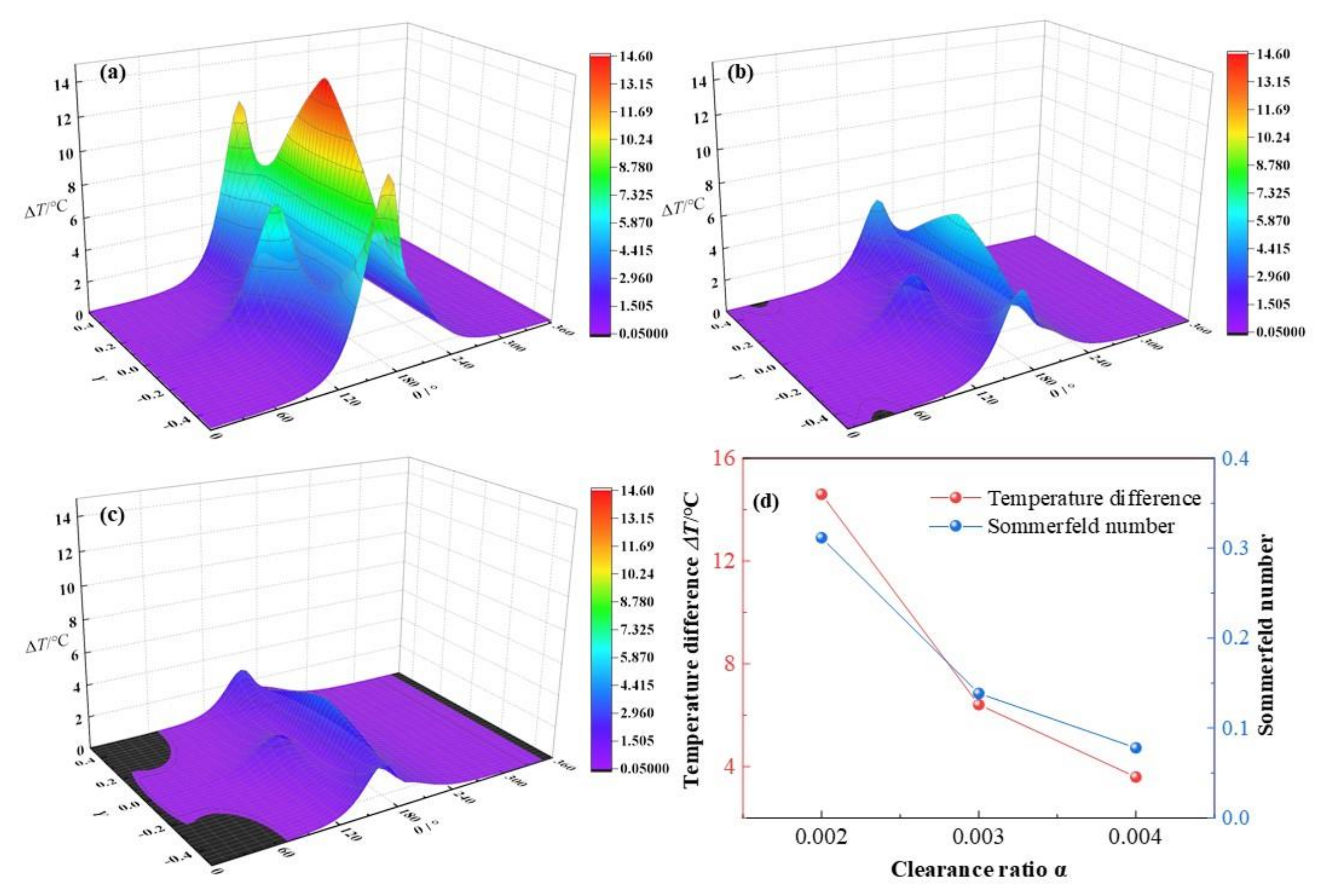
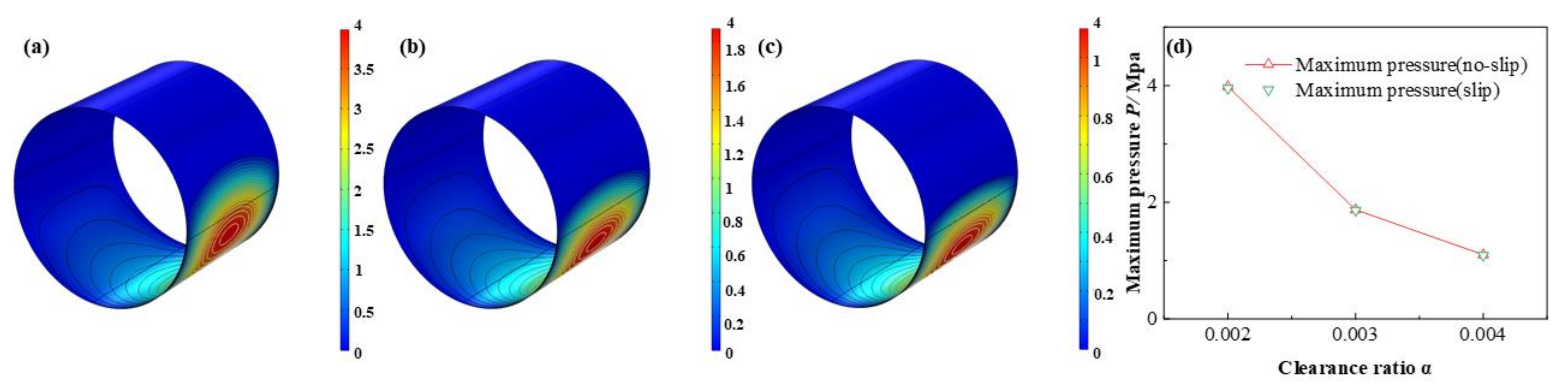
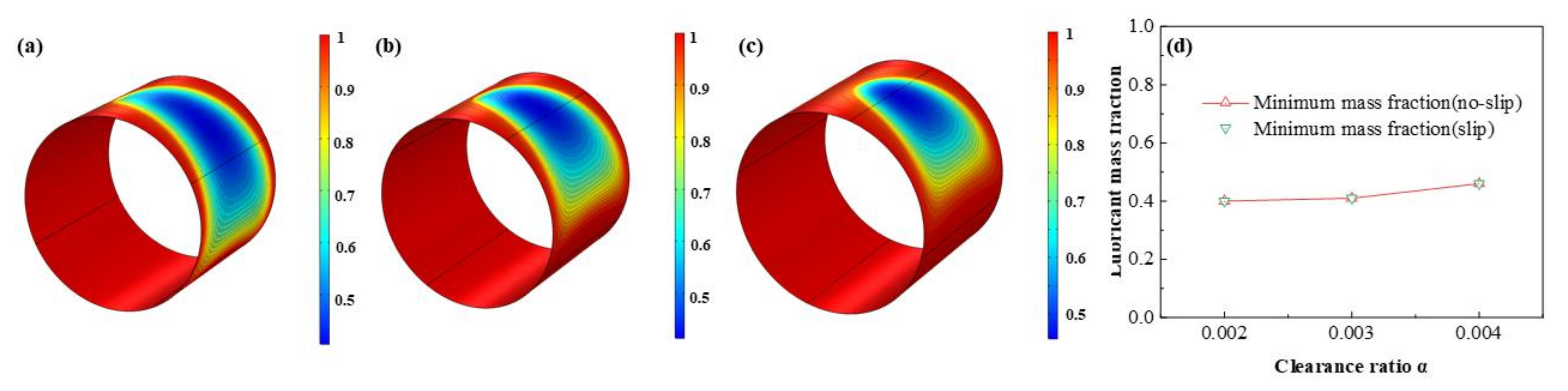
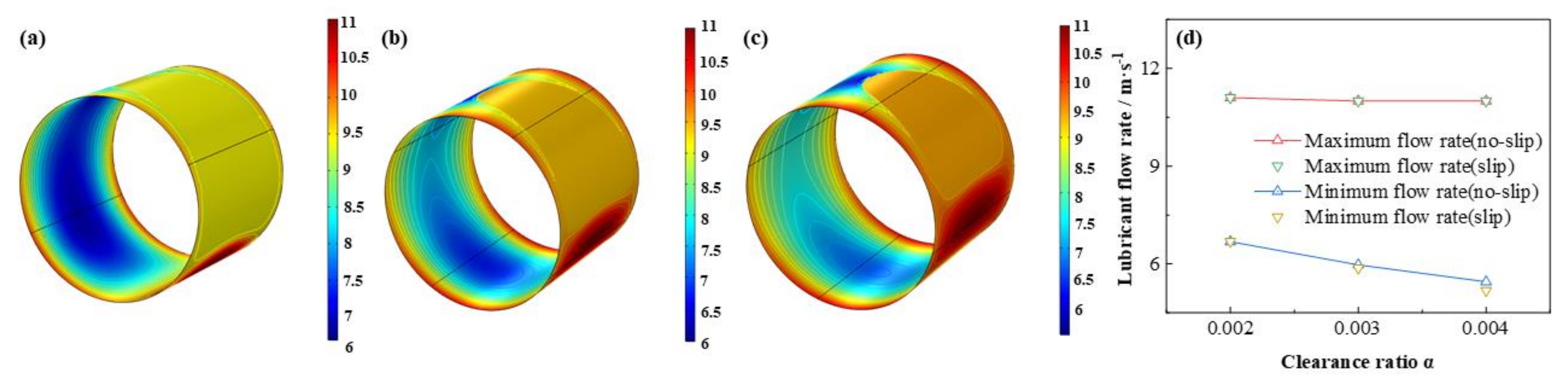
3.4. Effects of Clearance Ratios
4. Conclusions
- An increase in eccentricity ratio enlarges the differences between maximum and minimum peaks of thickness, flow rate and heat dissipation, severely strengthens the maximum peaks of pressure and temperature, and aggravates the degree of cavitation.
- A growth in length diameter ratio leads to the rise of the maximum peaks in pressure and temperature distributions, and promotes the degree of cavitation, meanwhile, intensify the differences between the maximum and minimum peaks of flow rate and heat dissipation.
- A rise in the bearing speed strongly increases the maximum peak of temperature, magnifies the distinctions between maximum and minimum peaks of flow rate, nevertheless, shrinks those of heat dissipation, and has slightly influence of cavitation.
- A lift in clearance ratios result in a sharply decline in maximum peak of temperature, exaggerating the differences between maximum and minimum peaks of heat dissipation, a mild decrease in the minimum flow rate, and slight effect on the maximum flow rate and cavitation.
- It is suggested that the optimization conditions for HJB can be a relative high eccentricity, medium length diameter ratio, relative high bearing speed, as well as medium clearance ratio, and in this research the combination could be ε = 0.6, L/D = 0.7, ω = 6 × 103 rpm and α = 0.003.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Symbols | Z | Dimensionless bearing length | |
| C | Radial clearance, m | z | Axial direction |
| C | Specific heat capacity, J·(kg·K)−1 | ||
| D | Journal diameter, m | Greek | |
| e | Eccentricity | α | Clearance ratio |
| H | Dimensionless film thickness | ε | Eccentricity ratio |
| h | Film thickness, m | μ | Dynamic viscosity, Pa·s−1 |
| k | heat transfer coefficient, W·(m2·K)−1 | μ0 | Initial dynamic viscosity, Pa·s−1 |
| L | Bearing length, m | θ | Angular coordinate, rad |
| P | Dimensionless pressure | η* | Dimensionless viscosity |
| p | Lubricant pressure, Pa | viscosity dissipation, W·m−3 | |
| R | Journal radius, m | ρ | Lubricant density, kg·m−3 |
| T | Lubricant temperature, K | ρ0 | Initial lubricant density, kg·m−3 |
| U | Tangential velocity of bearing, m/s | ω | Bearing speed, rpm |
| u | velocity component in x direction | ||
| v | velocity component in y, z direction | Subscripts | |
| W | Bearing load, N | b | bearing |
| w | velocity component in z direction | c | cavitation |
| x | Circumference direction | j | journal |
| y | Radial direction | o | original |
References
- Luo, J.B. Investigation on the origin of friction and superlubricity. Chin. Sci. Bull. 2020, 65, 2966–2978. [Google Scholar] [CrossRef]
- Cubillo, A.; Perinpanayagam, S.; Miguez, M.E. A review of physics-based models in prognostics: Application to gears and bearings of rotating machinery. Adv. Mech. Eng. 2016, 8, 1–21. [Google Scholar] [CrossRef] [Green Version]
- Shin, D.; Yang, J.; Tong, X.M.; Suh, J.H.; Palazzolo, A. A review of journal bearing thermal effects on rotordynamic response. J. Tribol. 2021, 143, 031803. [Google Scholar] [CrossRef]
- Koutsovasilis, P. Automotive turbocharger rotordynamics: Interaction of thrust and radial bearings in shaft motion simulation. J. Sound. Vib. 2019, 455, 413–429. [Google Scholar] [CrossRef]
- Takabi, J.; Khonsari, M.M. On the thermally-induced seizure in bearings: A review. Tribol. Int. 2015, 91, 118–130. [Google Scholar] [CrossRef]
- Delprete, C.; Razavykia, A. Piston dynamics, lubrication and tribological performance evaluation: A review. Int. J. Engine Res. 2020, 21, 725–741. [Google Scholar] [CrossRef]
- Solghar, A.A.; Brito, F.P.; Abdolzadeh, M.; Farajpour, A. Numerical study of twin groove journal bearings performance under steady--state condition. Lubr. Sci. 2015, 27, 83–102. [Google Scholar] [CrossRef]
- Zhang, Z.S.; Yang, Y.M.; Dai, X.D.; Xie, Y.B. Effects of thermal boundary conditions on plain journal bearing thermohydrodynamic lubrication. Tribol. Int. 2013, 56, 759–770. [Google Scholar] [CrossRef]
- Feng, H.H.; Jiang, S.Y.; Ji, A.M. Investigations of the static and dynamic characteristics of water-lubricated hydrodynamic journal bearing considering turbulent, thermohydrodynamic and misaligned effects. Tribol. Int. 2019, 130, 245–260. [Google Scholar] [CrossRef]
- Mo, J.T.; Gu, C.H.; Pan, X.H.; Zheng, S.Y.; Ying, G.Y. A Thermohydrodynamic Analysis of the Self-Lubricating Bearings Applied in Gear Pumps Using Computational Fluid Dynamics Method. J. Tribol. 2018, 140, 011102. [Google Scholar] [CrossRef]
- Chen, Y.; Feng, J.; Sun, Y.; Peng, X.; Dai, Q.; Yu, C.T. Effect of groove shape on the hydrodynamic lubrication of journal bearing considering cavitation. Eng. Computation 2020, 37, 1557–1576. [Google Scholar] [CrossRef]
- Zhang, X.L.; Yin, Z.W.; Gao, G.Y.; Li, Z. Determination of stiffness coefficients of hydrodynamic water-lubricated plain journal bearings. Tribol. Int. 2015, 85, 37–47. [Google Scholar] [CrossRef]
- Song, Y.; Gu, C.W. Development and validation of a three-dimensional computational fluid dynamics analysis for journal bearings considering cavitation and conjugate heat transfer. J. Eng. Gas. Turbines Power 2015, 12, 122502. [Google Scholar] [CrossRef]
- Xiang, G.; Han, Y.F.; He, T.; Wang, J.X.; Xiao, K.; Li, J.Y. Wear and fluid-solid-thermal transient coupled model for journal bearings. Appl. Math. Model 2021, 85, 19–45. [Google Scholar] [CrossRef]
- Throat, M.R.; Pettinato, B.C.; Choudhury, P.D. Metal temperature correlations in tilting pad journal bearings. J. Eng. Gas. Turbines Power 2014, 136, 112503. [Google Scholar] [CrossRef]
- Nichols, B.R.; Fittro, R.L.; Goyne, C.P. Steady-state tilting-pad bearing performance under reduced oil supply flow rates. J. Tribol. 2018, 140, 051701. [Google Scholar] [CrossRef]
- Ding, A.S.; Ren, X.D.; Li, X.S.; Gu, C.W. Friction power analysis and improvement for a tilting-pad journal bearing considering air entrainment. Appl. Therm. Eng. 2018, 145, 763–771. [Google Scholar] [CrossRef]
- Armentrout, R.W.; He, M.H.; Haykin, T.; Reed, A.E. Analysis of turbulence and convective inertia in a water-lubricated tilting-pad journal bearing using conventional and CFD approaches. Tribol. Int. 2017, 60, 1129–1147. [Google Scholar] [CrossRef]
- Hagemann, T.; Schwarze, H. Theoretical and experimental analyses of directly lubricated tilting-pad journal bearings with leading edge groove. ASME. J. Eng. Gas. Turbines Power 2019, 141, 051010. [Google Scholar] [CrossRef]
- Li, M.X.; Gu, C.H.; Pan, X.H.; Zheng, S.Y.; Li, Q. A new dynamic mesh algorithm for studying the 3D transient flow field of tilting pad journal bearings. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2016, 230, 1470–1482. [Google Scholar] [CrossRef]
- Liu, G.; Li, M. Experimental study on the lubrication characteristics of water-lubricated rubber bearings at high rotating speeds. Tribol. Int. 2021, 157, 106868. [Google Scholar] [CrossRef]
- Zhang, X.H.; Yu, T.; Guo, F.; Liang, P. Analysis of the influence of small quantity secondary lubricant on water lubrication. Tribol. Int. 2021, 159, 106998. [Google Scholar] [CrossRef]
- Li, L.L.; Liu, Z.F.; Wang, C.Y.; Xie, Y.H. Influence of Temperature Effect on the Static and Dynamic Performance of Gas-Lubricated Microbearings. Micromachines. 2020, 11, 716. [Google Scholar] [CrossRef]
- Wu, Y.; Yang, L.H.; Xu, T.F.; Xu, H.L. Combined Effect of Rarefaction and Effective Viscosity on Micro-Elasto-Aerodynamic Lubrication Performance of Gas Microbearings. Micromachines. 2019, 10, 657. [Google Scholar] [CrossRef] [Green Version]
- Chen, G.D.; Chen, Y.J.; Lu, Q.; Wu, Q.H.; Wang, M.H. Multi-Physics Fields Based Nonlinear Dynamic Behavior Analysis of Air Bearing Motorized Spindle. Micromachines 2020, 11, 723. [Google Scholar] [CrossRef]
- Yu, M.X.; Lv, P.; Xu, T.T.; Tan, X.; Li, H.W. Manufacturing of a Compact Micro Air Bearing Device for Power Micro Electro Mechanical System (MEMS) Applications Using Silica Film Assisted Processing. Micromachines 2018, 9, 166. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dowson, D. A generalized Reynolds Equation for fluid-film lubrication. Int. J. Mech. Sci. 1962, 4, 159–170. [Google Scholar] [CrossRef]
- Khonsari, M.M.; Jang, J.Y.; Fillon, M. On the generalization of thermohydrodynamic analyses for journal bearings. J. Tribol. 1996, 118, 571–579. [Google Scholar] [CrossRef]
- Elrod, H.G. A cavitation algorithm. J. Lubr. Tech. 1981, 103, 350–354. [Google Scholar] [CrossRef]
- Roelands, C.J.A.; Vulger, J.C.; Waterman, H.I. The viscosity temperature-pressure relationship of lubricating oils and its correlation with chemical constitution. ASME. J. Basic. Eng. 1963, 85, 601–610. [Google Scholar] [CrossRef]
- Elrod, H.G.; Adams, M.L. A computer program for cavitation and starvation problems. In Proceedings of the 1st Leeds-Lyon Symposium on Tribology, Leeds, UK, 1 December 1975. [Google Scholar]
- Jeung, S.H.; Suh, J.; Yoon, H.S. Performance of tilting pad journal bearings with the same Sommerfeld number. Appl. Sci.-Basel. 2020, 10, 3529. [Google Scholar] [CrossRef]
- Ferron, J.; Frene, J.; Boncompain, R. A study of the thermalhydrodynamic performance of a plain journal bearing comparison between theory and experiments. J. Lubr. Tech. ASME. 1983, 105, 422–428. [Google Scholar] [CrossRef]
- Li, Q.; Zhang, S.; Wang, Y.J.; Wang, W.W. Investigations of the three-dimensional temperature field of journal bearings considering conjugate heat transfer and cavitation. Ind. Lubr. Tribol. 2019, 71, 109–118. [Google Scholar] [CrossRef]
- Fitzgerald, M.K.; Neal, P.B. Temperature distributions and heat transfer in journal bearings. J. Tribol. 1992, 114, 112–130. [Google Scholar] [CrossRef]
- Poddar, S.; Tandon, N. Detection of journal bearing vapour cavitation using vibration and acoustic emission techniques with the aid of oil film photography. Tribol. Int. 2016, 103, 95–101. [Google Scholar] [CrossRef]
- Dhande, D.Y.; Pnade, D.W. Multiphase flow analysis of hydrodynamic journal bearing using CFD coupled Fluid Structure Interaction considering cavitation. King Saud. Univ. Sci. 2018, 1, 345–354. [Google Scholar] [CrossRef] [Green Version]
- Solghar, A.A.; Nassab, S.A.G. Thermohydrodynamic Behaviors of Finite Journal Bearings with Cavitation. Mec. Ind. 2011, 12, 5–15. [Google Scholar] [CrossRef]









| Parameter Name | Value |
|---|---|
| Bearing length (L), m | 6 × 10−2~1.2 × 10−1 |
| Shaft radius (R), m | 6 × 10−2 |
| Initial Radial clearance (C0), m | 1.2 × 10−2~2.4 × 10−2 |
| Initial temperature (T0), K | 313 |
| Initial dynamic viscosity of lubricant oil at 313 K (η0), Pa·s | 2.77 × 10−2 |
| Initial density of lubricant at 313 K (ρ0), kg·m−3 | 860 |
| Specific heat capacity of lubricant (C), J·(kg·K)−1 | 2000 |
| Heat transfer coefficient of lubricant (h), W·(m2·K)−1 | 80 |
| Eccentricity ratio (ε) | 0.3~0.7 |
| Bearing speed (ω), rpm | 3 × 103~9 × 103 |
| Bearing Length (L), m | Shaft Radius (R), m | Initial Radial Clearance (C0), m | Eccentricity Ratio (ε) | Bearing Speed (ω), rpm |
|---|---|---|---|---|
| 0.1 × 10−1 | 0.6 × 10−1 | 0.18 × 10−3 | 0.6 | 6 × 103 |
| Bearing Length (L), m | Shaft Radius (R), m | Initial Radial Clearance (C0), m | Lubricant Viscosity at 313 K (μ), Pa·s | Bearing Speed (ω), rpm |
|---|---|---|---|---|
| 0.8 × 10−1 | 0.5 × 10−1 | 0.145 × 10−3 | 0.0277 | 6 × 103 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, Y.; Liang, B.; Huang, Z.; Chen, Z.; Xu, B. Prediction on Flow and Thermal Characteristics of Ultrathin Lubricant Film of Hydrodynamic Journal Bearing. Micromachines 2021, 12, 1208. https://doi.org/10.3390/mi12101208
Jiang Y, Liang B, Huang Z, Chen Z, Xu B. Prediction on Flow and Thermal Characteristics of Ultrathin Lubricant Film of Hydrodynamic Journal Bearing. Micromachines. 2021; 12(10):1208. https://doi.org/10.3390/mi12101208
Chicago/Turabian StyleJiang, Yulong, Bo Liang, Zhongwen Huang, Zhenqian Chen, and Bo Xu. 2021. "Prediction on Flow and Thermal Characteristics of Ultrathin Lubricant Film of Hydrodynamic Journal Bearing" Micromachines 12, no. 10: 1208. https://doi.org/10.3390/mi12101208
APA StyleJiang, Y., Liang, B., Huang, Z., Chen, Z., & Xu, B. (2021). Prediction on Flow and Thermal Characteristics of Ultrathin Lubricant Film of Hydrodynamic Journal Bearing. Micromachines, 12(10), 1208. https://doi.org/10.3390/mi12101208







