Characterization of Modal Frequencies and Orientation of Axisymmetric Resonators in Coriolis Vibratory Gyroscopes
Abstract
:1. Introduction
2. Dynamics and 2D Transfer Function of Axisymmetric Resonators in CVGs
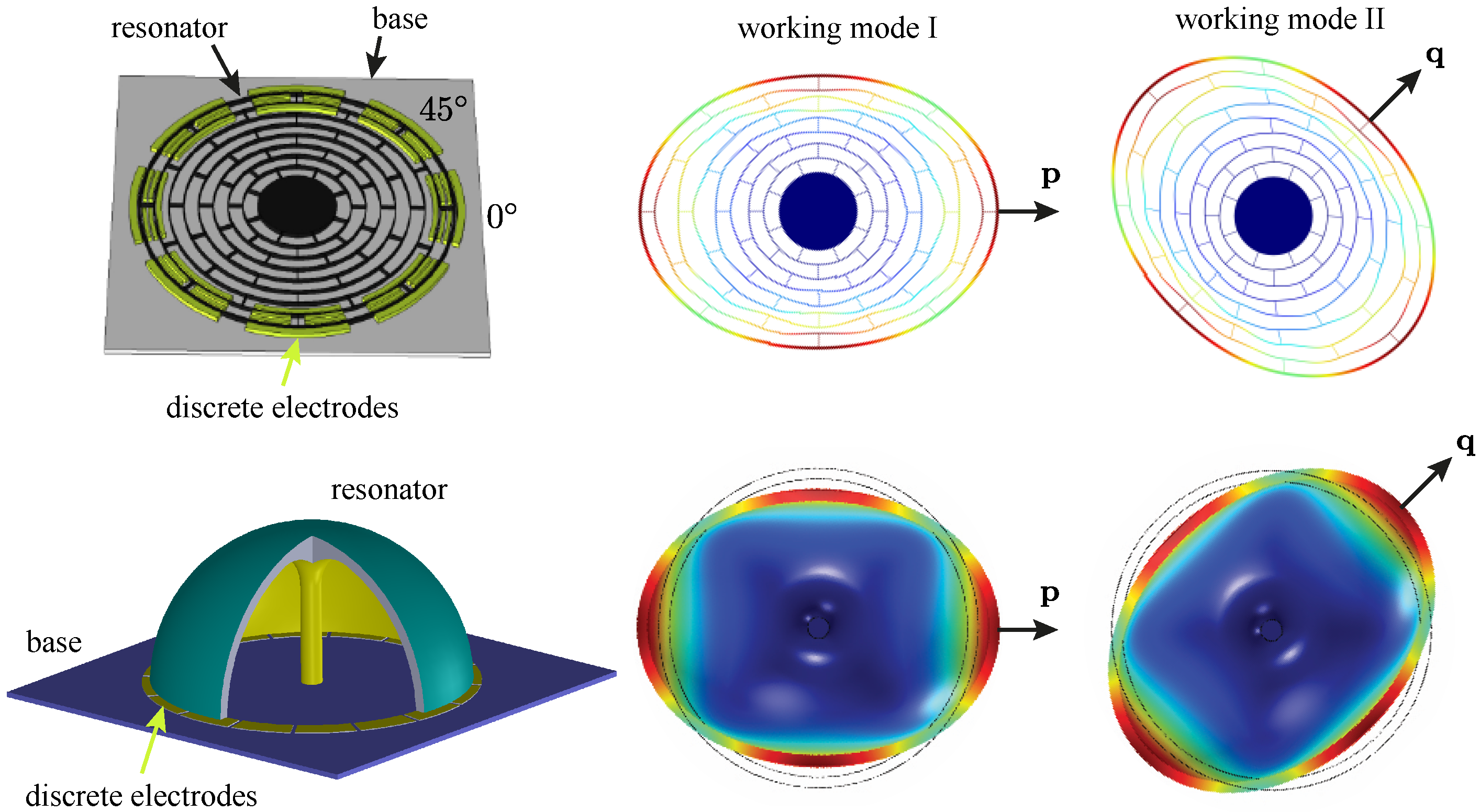
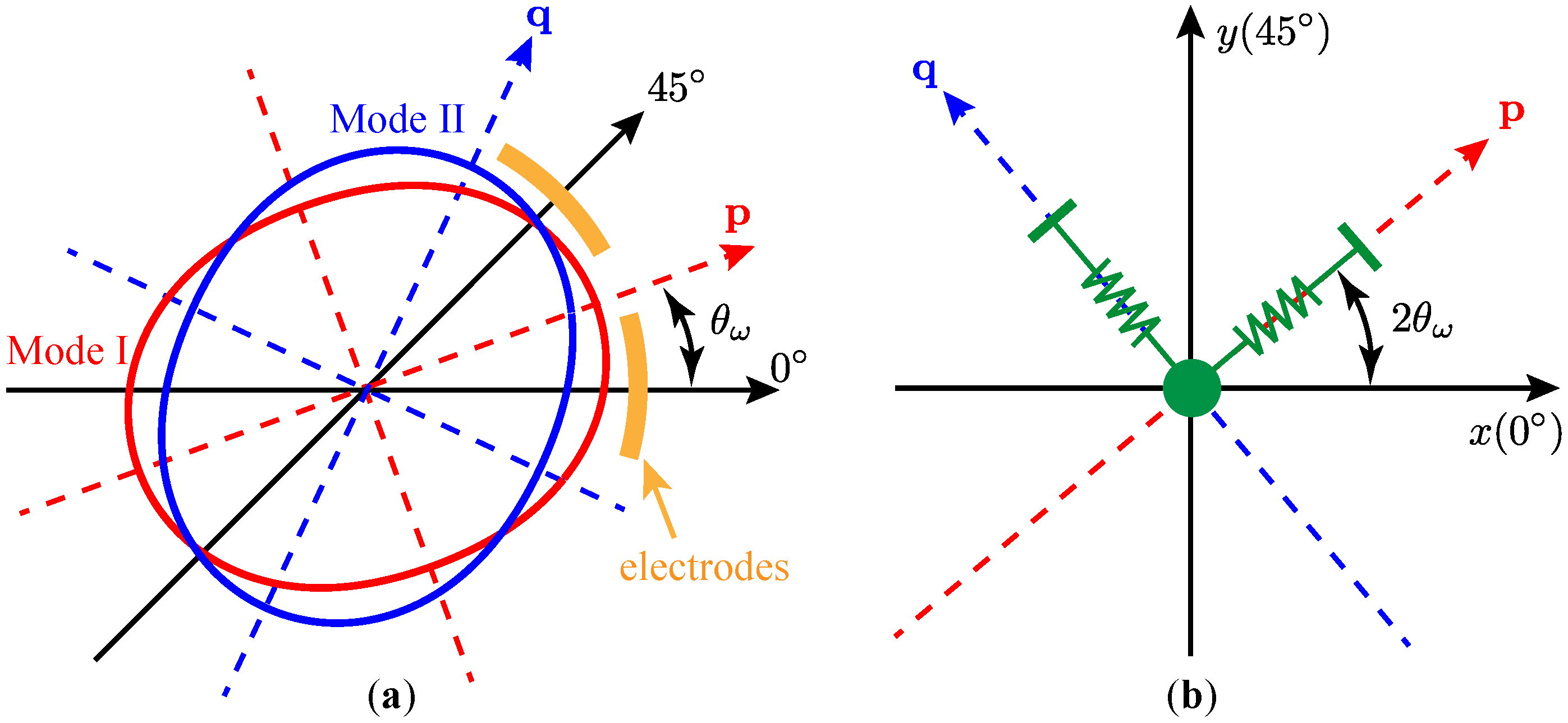
2.1. Equations of Motion of Axisymmetric Resonators’ Working Modes
2.2. Two-Dimensional Transfer Function
3. Modal Frequencies and Orientation of Stiffness Axes
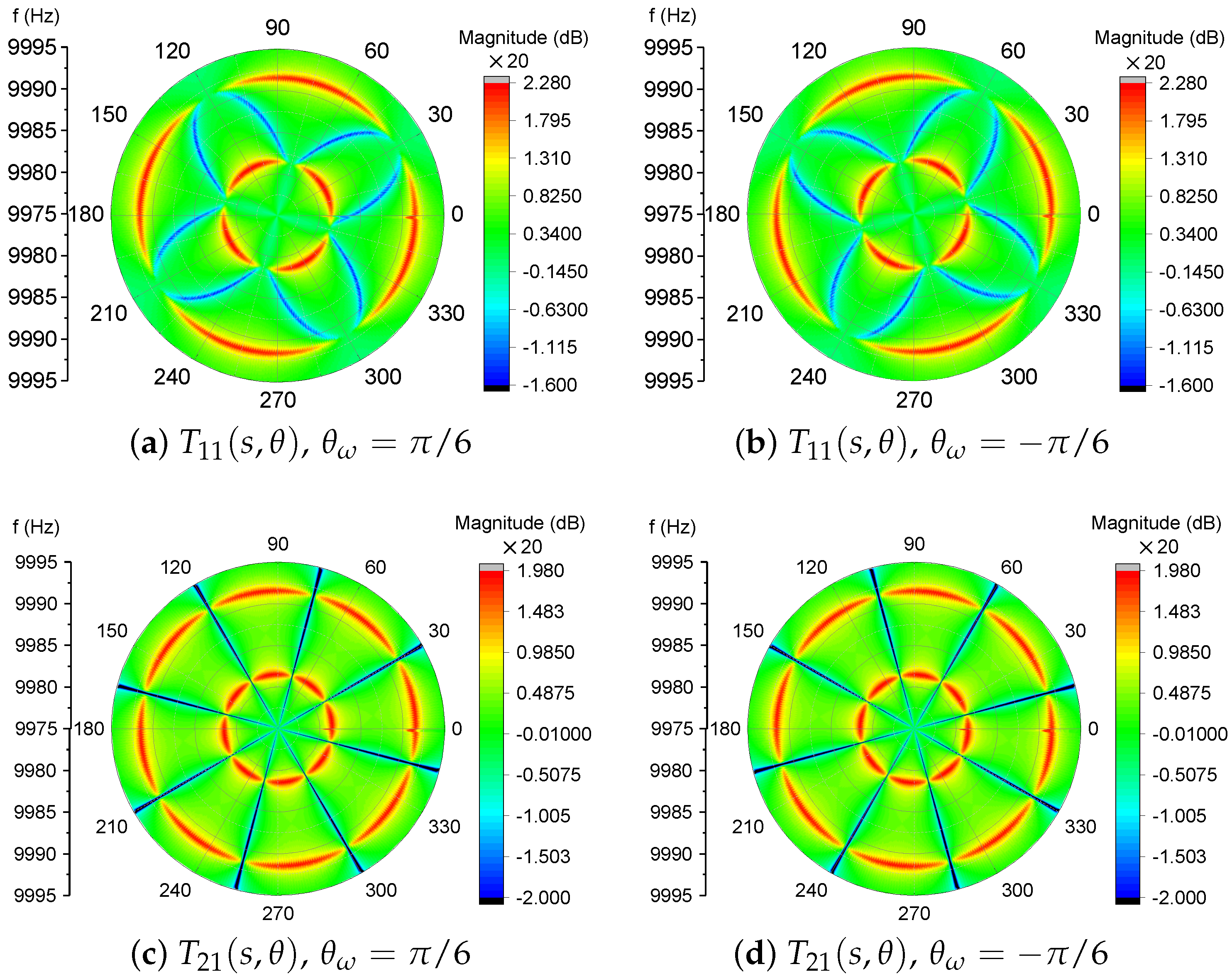
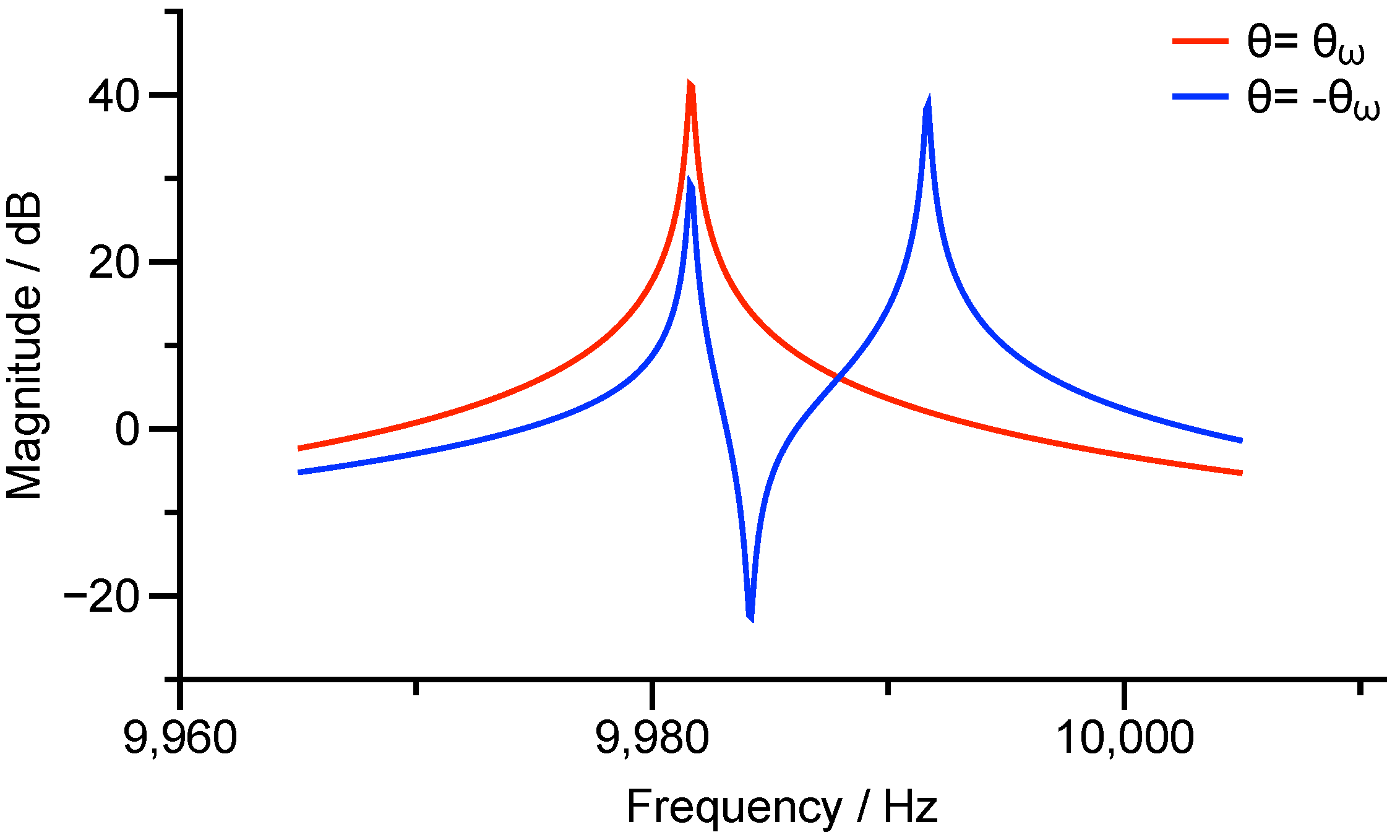
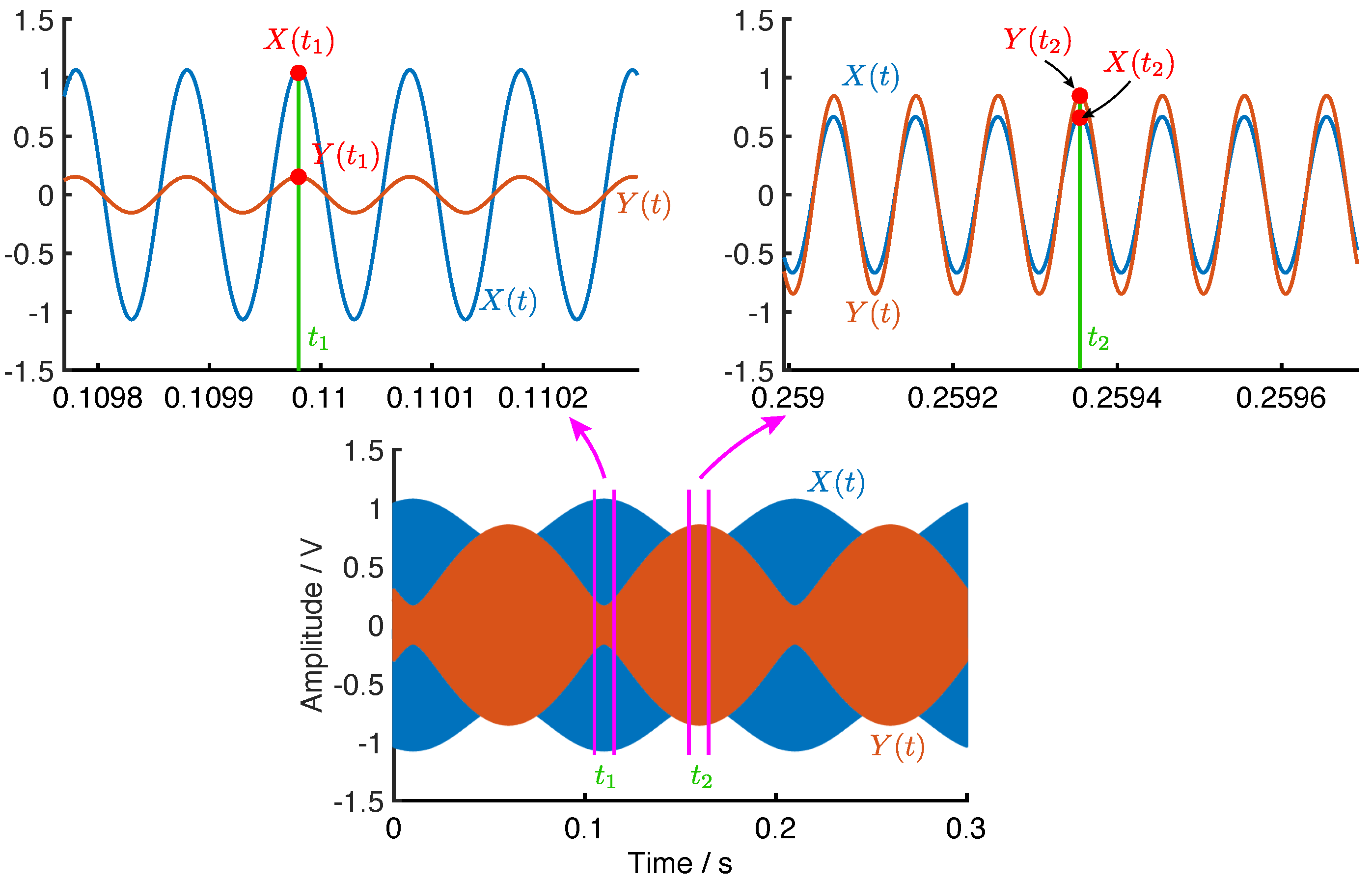
3.1. Poles and Zeros in Magnitude-Frequency Response of the Transfer Function
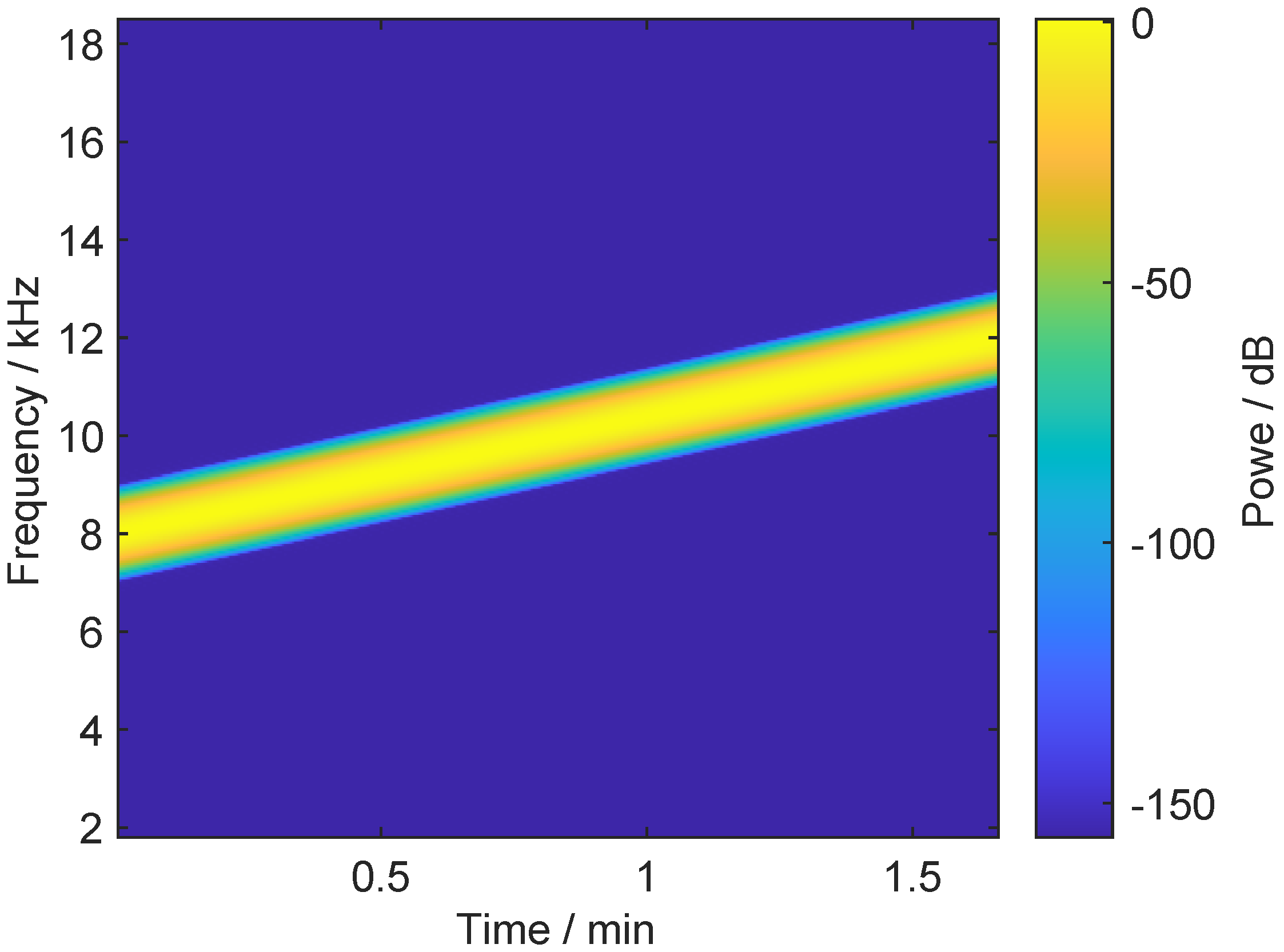
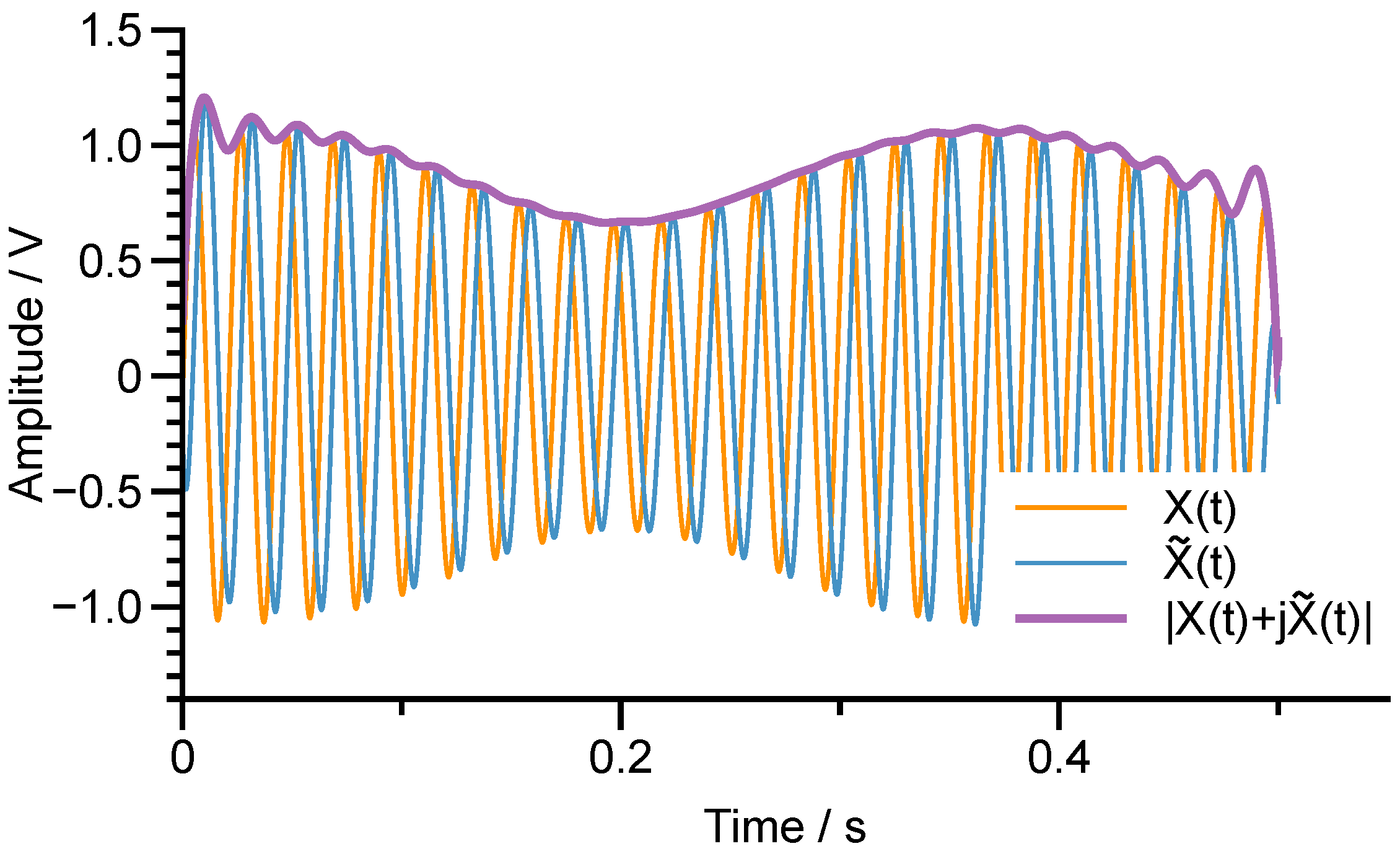
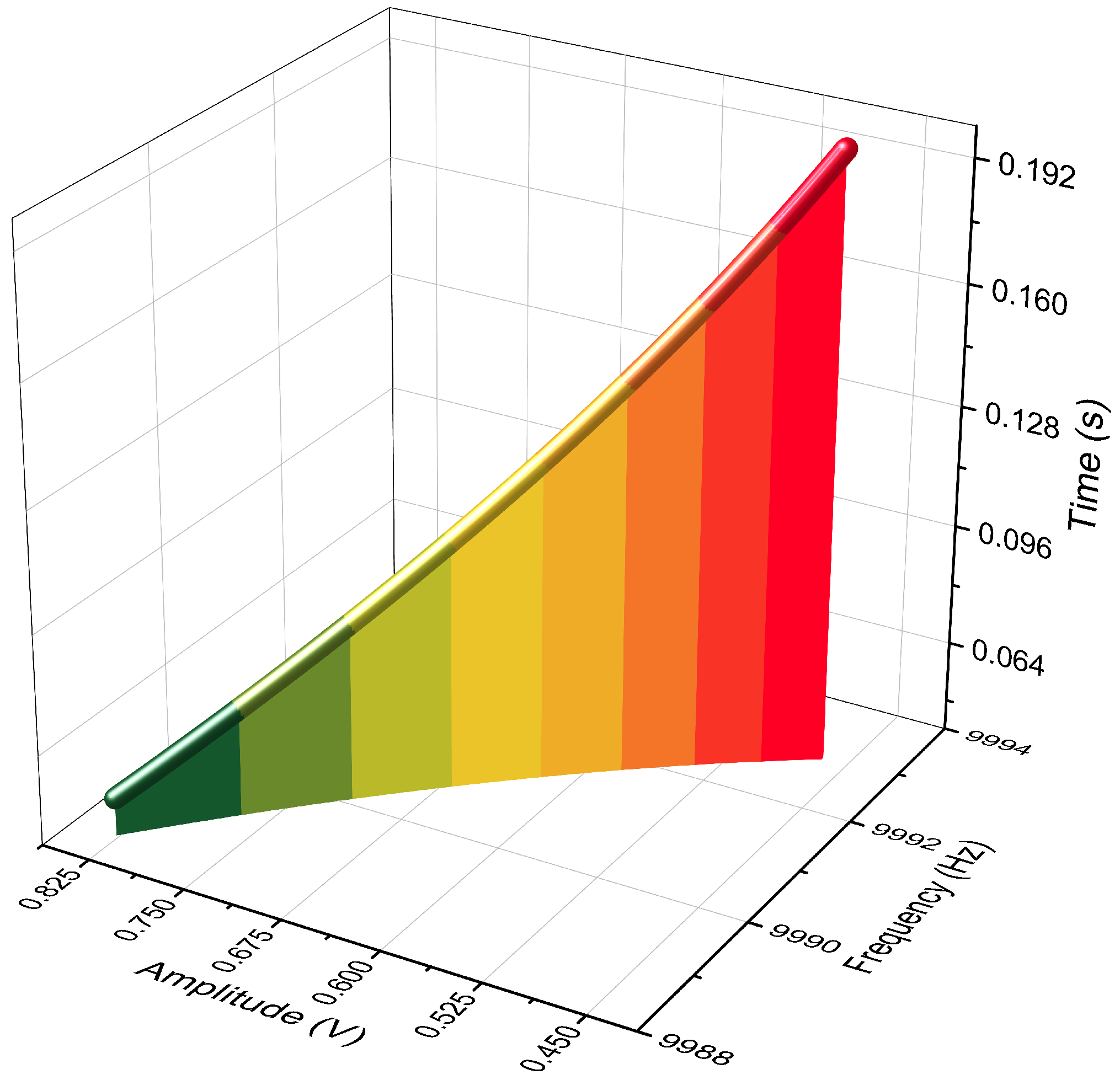
3.2. Continuous Linear Frequency Sweep
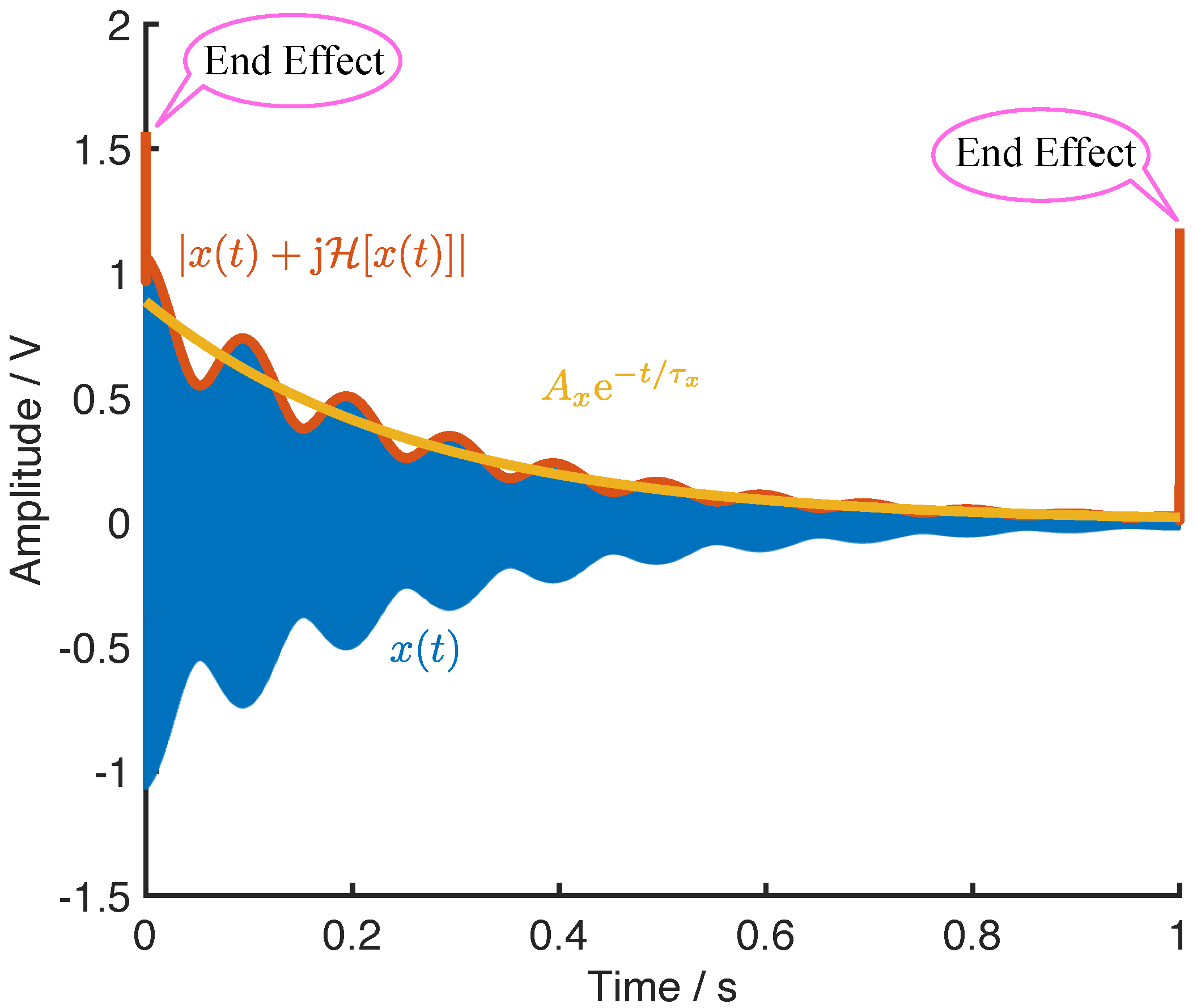
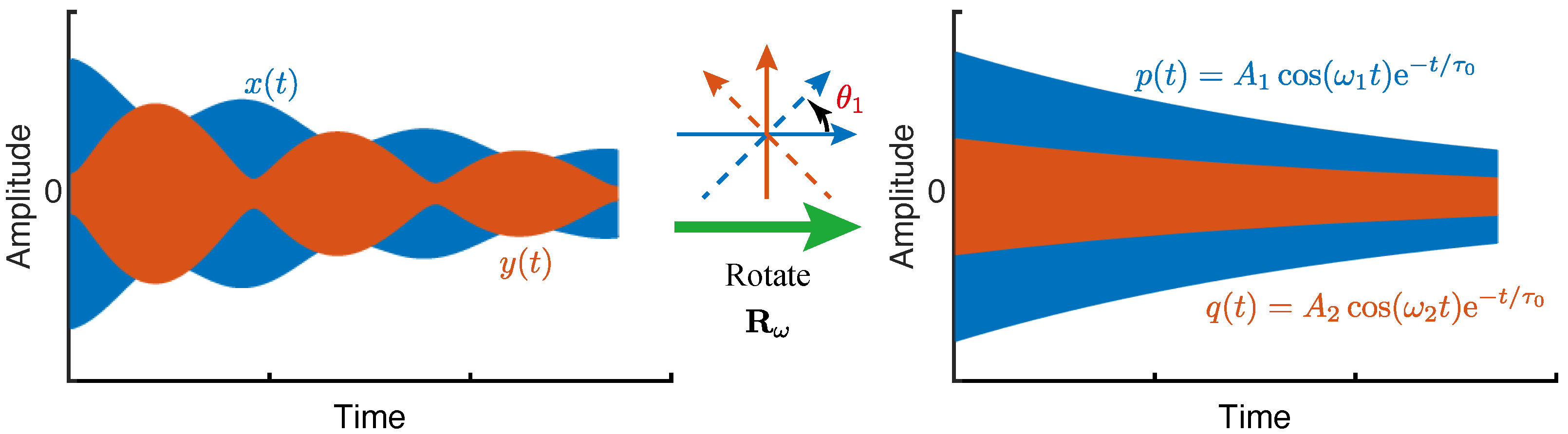
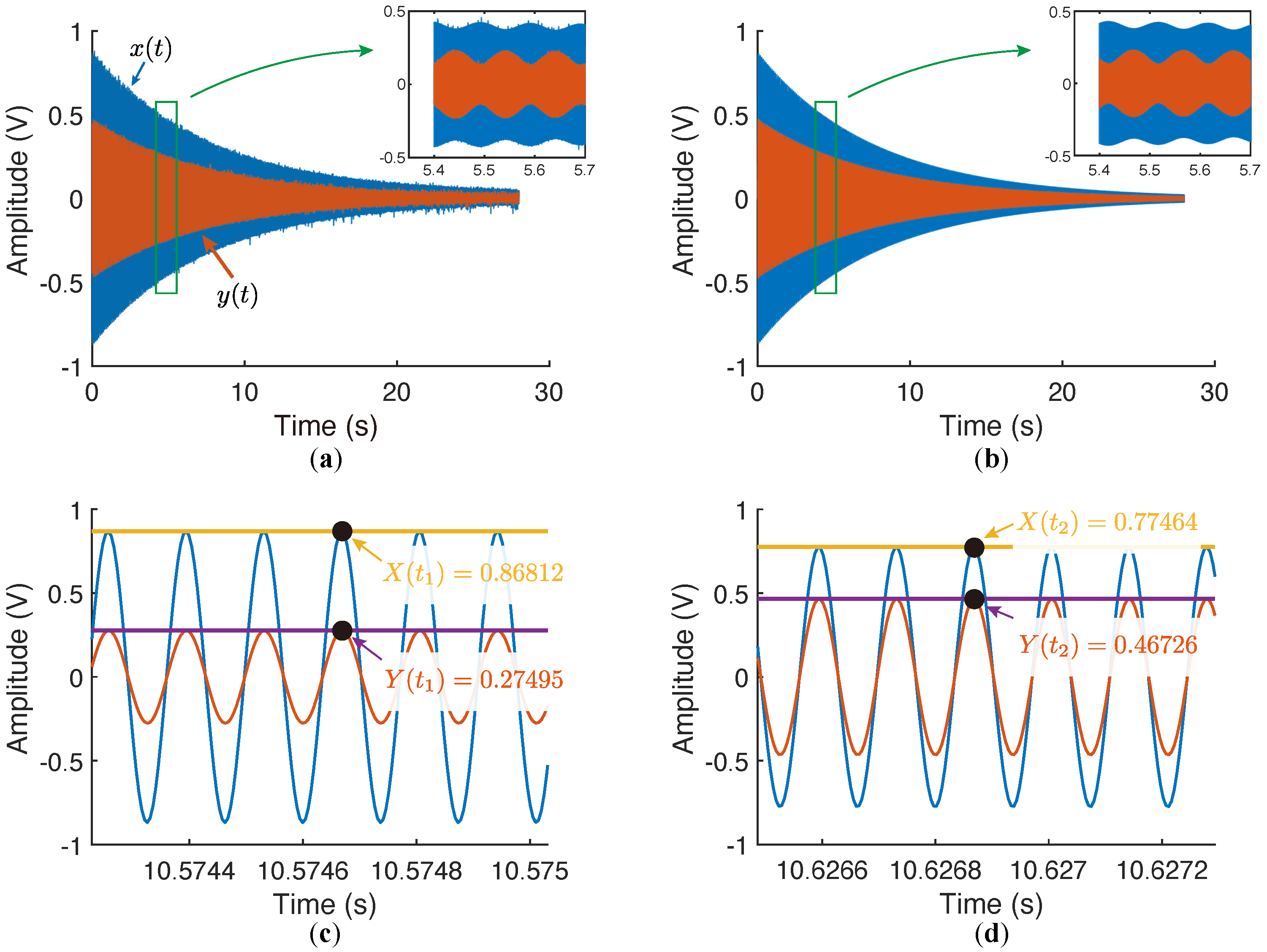
4. Ring-Down and Nonlinear Stiffness Coefficient
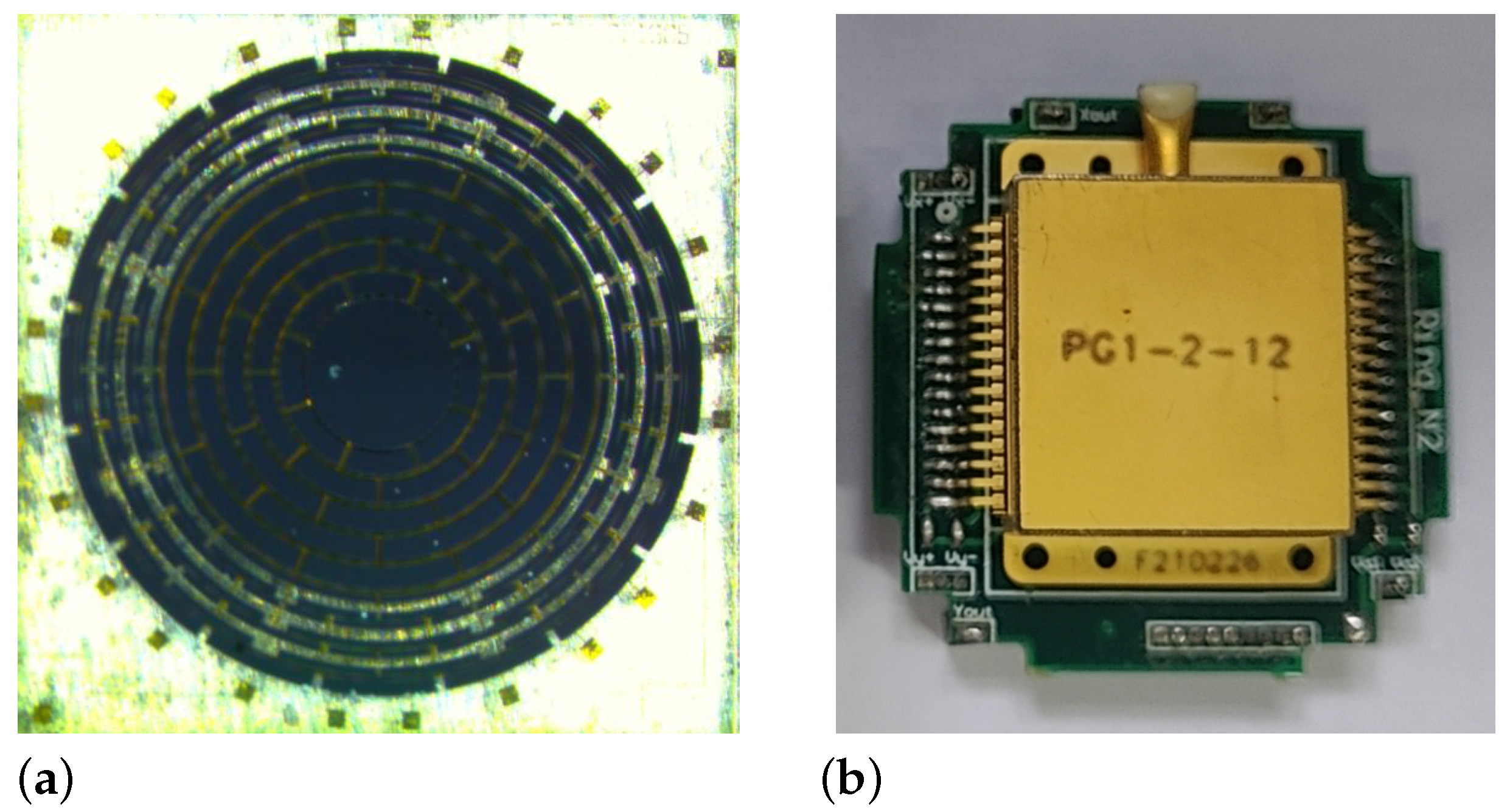
5. Experiments
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Gadola, M.; Buffoli, A.; Sansa, M.; Berthelot, A.; Robert, P.; Langfelder, G. 1.3 mm2 Nav-Grade NEMS-Based Gyroscope. J. Microelectromech. Syst. 2021, 30, 513–520. [Google Scholar] [CrossRef]
- Xu, Y.; Li, Q.; Wang, P.; Zhang, Y.; Zhou, X.; Yu, L.; Wu, X.; Xiao, D. 0.015 Degree-Per-Hour Honeycomb Disk Resonator Gyroscope. IEEE Sens. J. 2020, 21, 7326–7338. [Google Scholar] [CrossRef]
- Endean, D.; Christ, K.; Duffy, P.; Freeman, E.; Glenn, M.; Gnerlich, M.; Johnson, B.; Weinmann, J. Near-Navigation Grade Tuning Fork MEMS Gyroscope. In Proceedings of the 2019 IEEE International Symposium on Inertial Sensors and Systems (INERTIAL), Naples, FL, USA, 1–5 April 2019; pp. 1–4. [Google Scholar] [CrossRef]
- Koenig, S.; Rombach, S.; Gutmann, W.; Jaeckle, A.; Weber, C.; Ruf, M.; Grolle, D.; Rende, J. Towards a navigation grade Si-MEMS gyroscope. In Proceedings of the 2019 DGON Inertial Sensors and Systems (ISS), Braunschweig, Germany, 10–11 September 2019; pp. 1–18. [Google Scholar]
- Challoner, A.D.; Howard, H.G.; Liu, J.Y. Boeing disc resonator gyroscope. In Proceedings of the 2014 IEEE/ION Position, Location and Navigation Symposium-PLANS 2014, Monterey, CA, USA, 5–8 May 2014; pp. 504–514. [Google Scholar]
- Jia, J.; Ding, X.; Qin, Z.; Ruan, Z.; Li, W.; Liu, X.; Li, H. Overview and analysis of MEMS Coriolis vibratory ring gyroscope. Measurement 2021, 182, 109704. [Google Scholar] [CrossRef]
- Parajuli, M.; Sobreviela, G.; Seshia, A.A. Electrostatic Frequency Tuning of Bulk Acoustic Wave Disk Gyroscopes. In Proceedings of the 2020 Joint Conference of the IEEE International Frequency Control Symposium and International Symposium on Applications of Ferroelectrics (IFCS-ISAF), Keystone, CO, USA, 19–23 July 2020; pp. 1–4. [Google Scholar]
- Qin, Z.; Gao, Y.; Jia, J.; Ding, X.; Huang, L.; Li, H. The effect of the anisotropy of single crystal silicon on the frequency split of vibrating ring gyroscopes. Micromachines 2019, 10, 126. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shen, X.; Zhao, L.; Xia, D. Research on the Disc Sensitive Structure of a Micro Optoelectromechanical System (MOEMS) Resonator Gyroscope. Micromachines 2019, 10, 264. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shi, Y.; Xi, X.; Li, B.; Chen, Y.; Wu, Y.; Xiao, D.; Wua, X.; Lu, K. Micro Hemispherical Resonator Gyroscope with Teeth-Like Tines. IEEE Sens. J. 2021, 21, 13098–13106. [Google Scholar] [CrossRef]
- Singh, S.; Darvishian, A.; Cho, J.Y.; Shiari, B.; Najafi, K. Resonant characteristics of birdbath shell resonator in N = 3 wine-glass mode. In Proceedings of the 2018 IEEE SENSORS, New Delhi, India, 28–31 October 2018; pp. 1–4. [Google Scholar]
- Xu, Z.; Xi, B.; Yi, G.; Wang, D. A novel model for fully closed-loop system of hemispherical resonator gyroscope under force-to-rebalance mode. IEEE Trans. Instrum. Meas. 2020, 69, 9918–9930. [Google Scholar] [CrossRef]
- Askari, S.; Asadian, M.H.; Shkel, A.M. Performance of Quad Mass Gyroscope in the Angular Rate Mode. Micromachines 2021, 12, 266. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Tsukamoto, T.; Tanaka, S. Quad mass gyroscope with 16 ppm frequency mismatch trimmed by focus ion beam. In Proceedings of the 2019 IEEE International Symposium on Inertial Sensors and Systems (INERTIAL), Naples, FL, USA, 1–5 April 2019; pp. 1–4. [Google Scholar]
- Askari, S.; Asadian, M.; Kakavand, K.; Shkel, A. Near-navigation grade quad mass gyroscope with Q-factor limited by thermoelastic damping. ENERGY 2016, 44, 124–127. [Google Scholar]
- Zhao, W.; Yang, H.; Liu, F.; Su, Y.; Li, C. High sensitivity rate-integrating hemispherical resonator gyroscope with dead area compensation for damping asymmetry. Sci. Rep. 2021, 11, 1–12. [Google Scholar]
- Guo, K.; Wu, Y.; Zhang, Y.; Xiao, D.; Wu, X. Adaptive compensation of damping asymmetry in whole-angle hemispherical resonator gyroscope. AIP Adv. 2020, 10, 105109. [Google Scholar] [CrossRef]
- Trusov, A.A.; Phillips, M.R.; Mccammon, G.H.; Rozelle, D.M.; Meyer, A.D. Continuously self-calibrating CVG system using hemispherical resonator gyroscopes. In Proceedings of the 2015 IEEE International Symposium on Inertial Sensors and Systems (ISISS), Hapuna Beach, HI, USA, 23–26 March 2015; pp. 1–4. [Google Scholar]
- Norouzpour-Shirazi, A.; Serrano, D.; Zaman, M.; Casinovi, G.; Ayazi, F. A dual-mode gyroscope architecture with in-run mode-matching capability and inherent bias cancellation. In Proceedings of the 2015 Transducers-2015 18th International Conference on Solid-State Sensors, Actuators and Microsystems (TRANSDUCERS), Anchorage, AL, USA, 21–25 June 2015; pp. 23–26. [Google Scholar]
- Schwartz, D.M.; Kim, D.; Stupar, P.; DeNatale, J.; M’Closkey, R.T. Modal parameter tuning of an axisymmetric resonator via mass perturbation. J. Microelectromech. Syst. 2015, 24, 545–555. [Google Scholar] [CrossRef] [Green Version]
- Courcimault, C.G.; Allen, M.G. High-Q mechanical tuning of MEMS resonators using a metal deposition-annealing technique. In Proceedings of the 13th International Conference on Solid-State Sensors, Actuators and Microsystems (Digest of Technical Papers. TRANSDUCERS’05), Seoul, Korea, 5–9 June 2005; Volume 1, pp. 875–878. [Google Scholar]
- Joachim, D.; Lin, L. Selective polysilicon deposition for frequency tuning of MEMS resonators. In Proceedings of the Technical Digest. MEMS 2002 IEEE International Conference. Fifteenth IEEE International Conference on Micro Electro Mechanical Systems (Cat. No. 02CH37266), Las Vegas, NV, USA, 24 January 2002; pp. 727–730. [Google Scholar]
- Liu, Z.; Daruwalla, A.; Hamelin, B.; Ayazi, F. A Study Of Mode-Matching And Alignment In Piezoelectric Disk Resonator Gyros Via Femtosecond Laser Ablation. In Proceedings of the 2021 IEEE 34th International Conference on Micro Electro Mechanical Systems (MEMS), Gainesville, FL, USA, 25–29 January 2021; pp. 342–345. [Google Scholar]
- Lu, K.; Xi, X.; Li, W.; Shi, Y.; Hou, Z.; Zhuo, M.; Wu, X.; Wu, Y.; Xiao, D. Research on precise mechanical trimming of a micro shell resonator with T-shape masses using femtosecond laser ablation. Sens. Actuators A Phys. 2019, 290, 228–238. [Google Scholar] [CrossRef]
- Gallacher, B.; Hedley, J.; Burdess, J.; Harris, A.; McNie, M. Multimodal tuning of a vibrating ring using laser ablation. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2003, 217, 557–576. [Google Scholar] [CrossRef]
- Basarab, M.; Lunin, B.; Matveev, V.; Chumankin, E. Balancing of hemispherical resonator gyros by chemical etching. Gyroscopy Navig. 2015, 6, 218–223. [Google Scholar] [CrossRef]
- M’Closkey, R.T.; Gibson, S.; Hui, J. System identification of a MEMS gyroscope. J. Dyn. Syst. Meas. Control. 2001, 123, 201–210. [Google Scholar] [CrossRef]
- Ding, X.; Jia, J.; Qin, Z.; Ruan, Z.; Zhao, L.; Li, H. A Lumped Mass Model for Circular Micro-Resonators in Coriolis Vibratory Gyroscopes. Micromachines 2019, 10, 378. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Aldridge, D.F. Mathematics of Linear Sweeps. Can. J. Explor. Geophys. 1992, 28, 62–68. [Google Scholar]
- Ding, X.; Zhu, K.; Li, H. A switch-bridge-based readout circuit for differential capacitance measurement in MEMS resonators. IEEE Sens. J. 2017, 17, 6978–6985. [Google Scholar] [CrossRef]






| Parameters | Values | Units |
|---|---|---|
| 9995 | Hz | |
| 10,005 | Hz | |
| Hz | ||
| s | ||
| m | kg | |
| N/m/V | ||
| V |
| Parameters | Values | Units |
|---|---|---|
| 8000 | Hz | |
| 12,000 | Hz | |
| T | 100 | s |
| A | V |
| Parameters | Frequency Sweep | Ring-Down | Units |
|---|---|---|---|
| rad/s | |||
| rad/s | |||
| – | |||
| – |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, X.; Zhang, H.; Huang, L.; Zhao, L.; Li, H. Characterization of Modal Frequencies and Orientation of Axisymmetric Resonators in Coriolis Vibratory Gyroscopes. Micromachines 2021, 12, 1206. https://doi.org/10.3390/mi12101206
Ding X, Zhang H, Huang L, Zhao L, Li H. Characterization of Modal Frequencies and Orientation of Axisymmetric Resonators in Coriolis Vibratory Gyroscopes. Micromachines. 2021; 12(10):1206. https://doi.org/10.3390/mi12101206
Chicago/Turabian StyleDing, Xukai, Han Zhang, Libin Huang, Liye Zhao, and Hongsheng Li. 2021. "Characterization of Modal Frequencies and Orientation of Axisymmetric Resonators in Coriolis Vibratory Gyroscopes" Micromachines 12, no. 10: 1206. https://doi.org/10.3390/mi12101206
APA StyleDing, X., Zhang, H., Huang, L., Zhao, L., & Li, H. (2021). Characterization of Modal Frequencies and Orientation of Axisymmetric Resonators in Coriolis Vibratory Gyroscopes. Micromachines, 12(10), 1206. https://doi.org/10.3390/mi12101206






