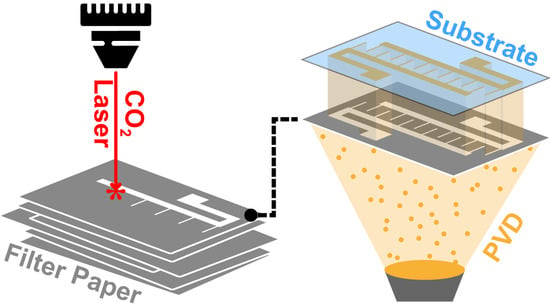
Low-Cost PVD Shadow Masks with Submillimeter Resolution from Laser-Cut Paper
Abstract
1. Introduction
2. Materials and Methods
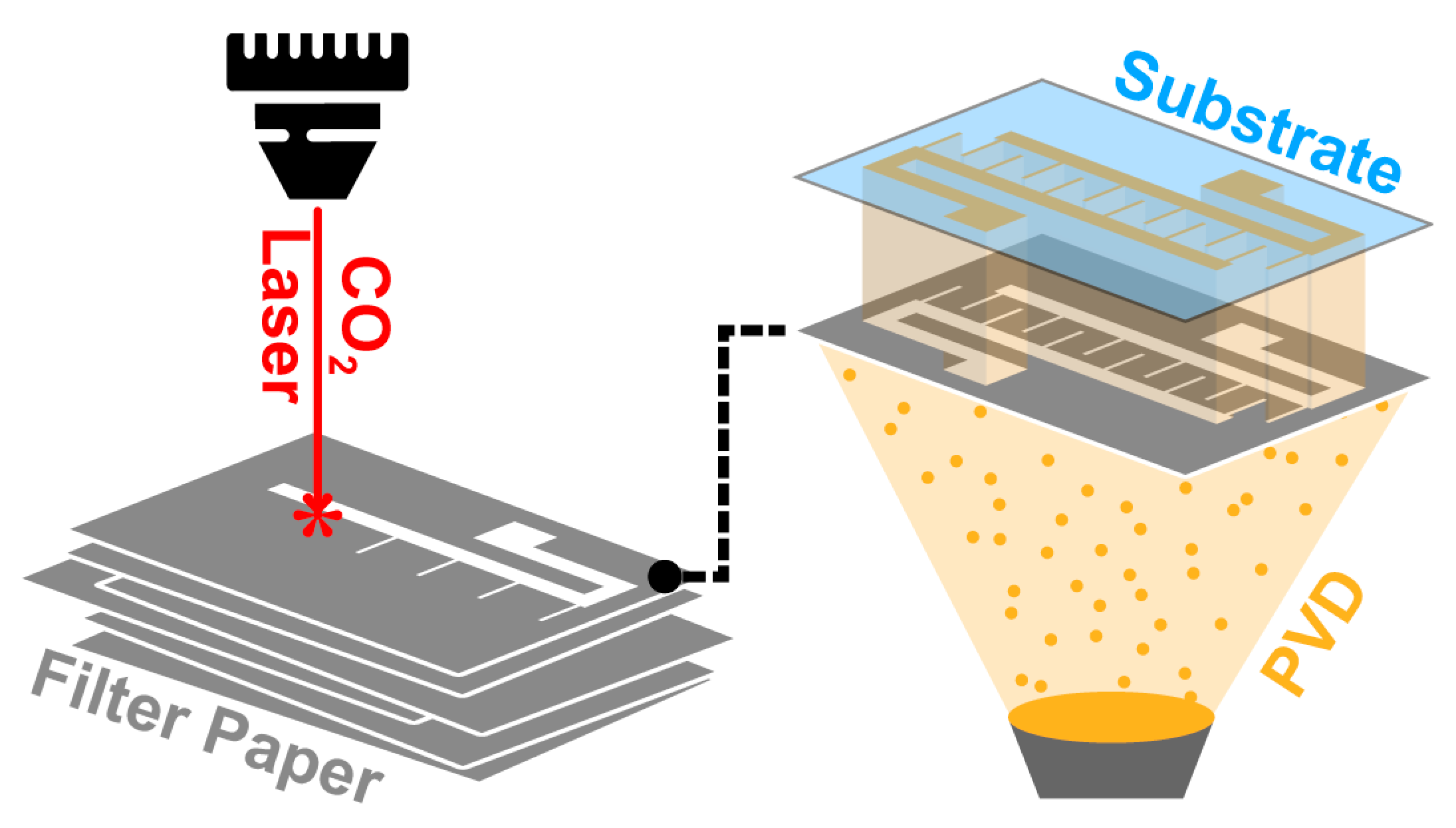
2.1. Design
2.2. Materials
2.3. Laser Cutting
2.4. PVD
2.5. Lateral Characterization
2.6. Vertical Characterization
2.7. Statistics and Data Availability
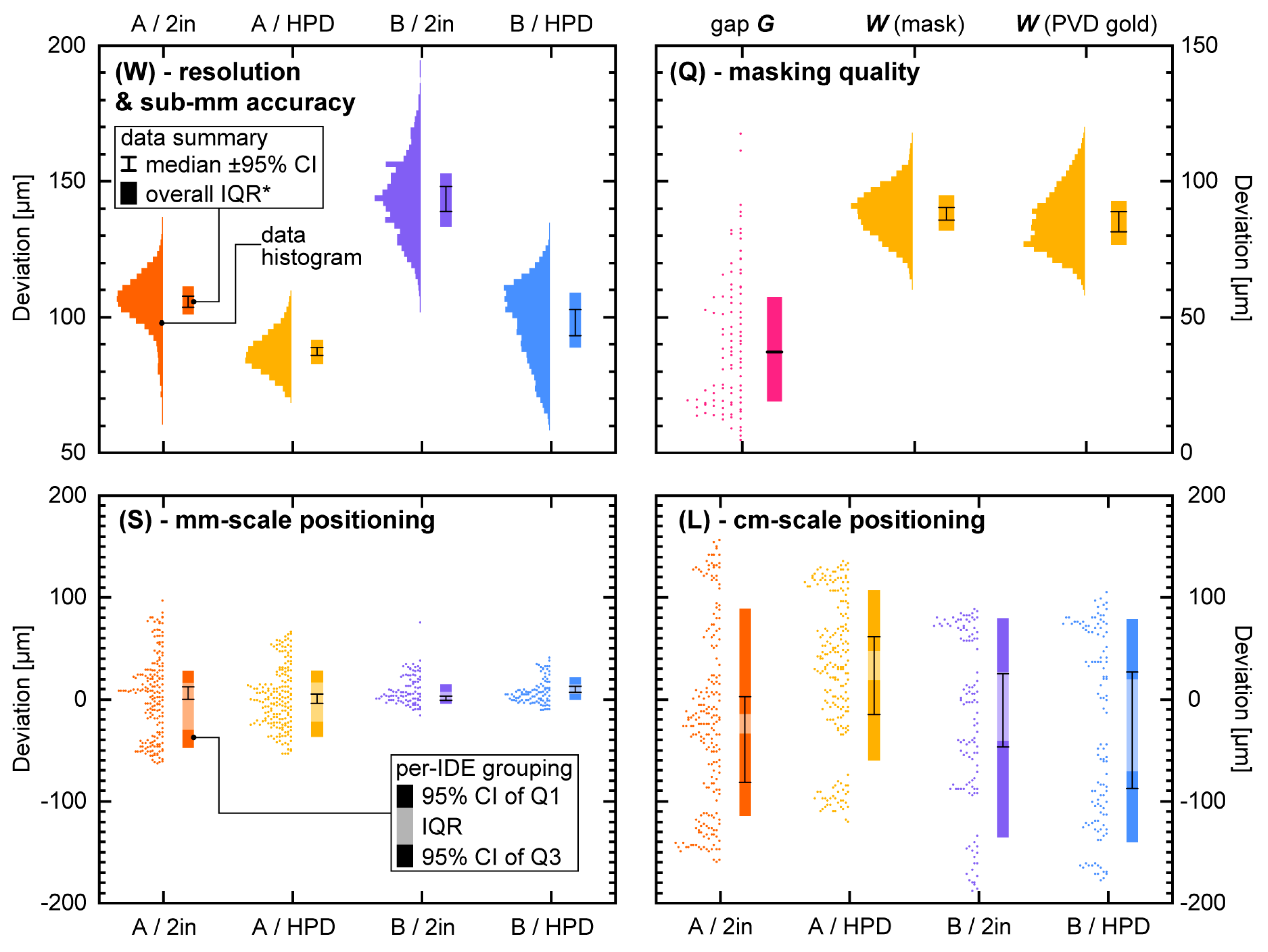
3. Results and Discussion
3.1. Materials Selection
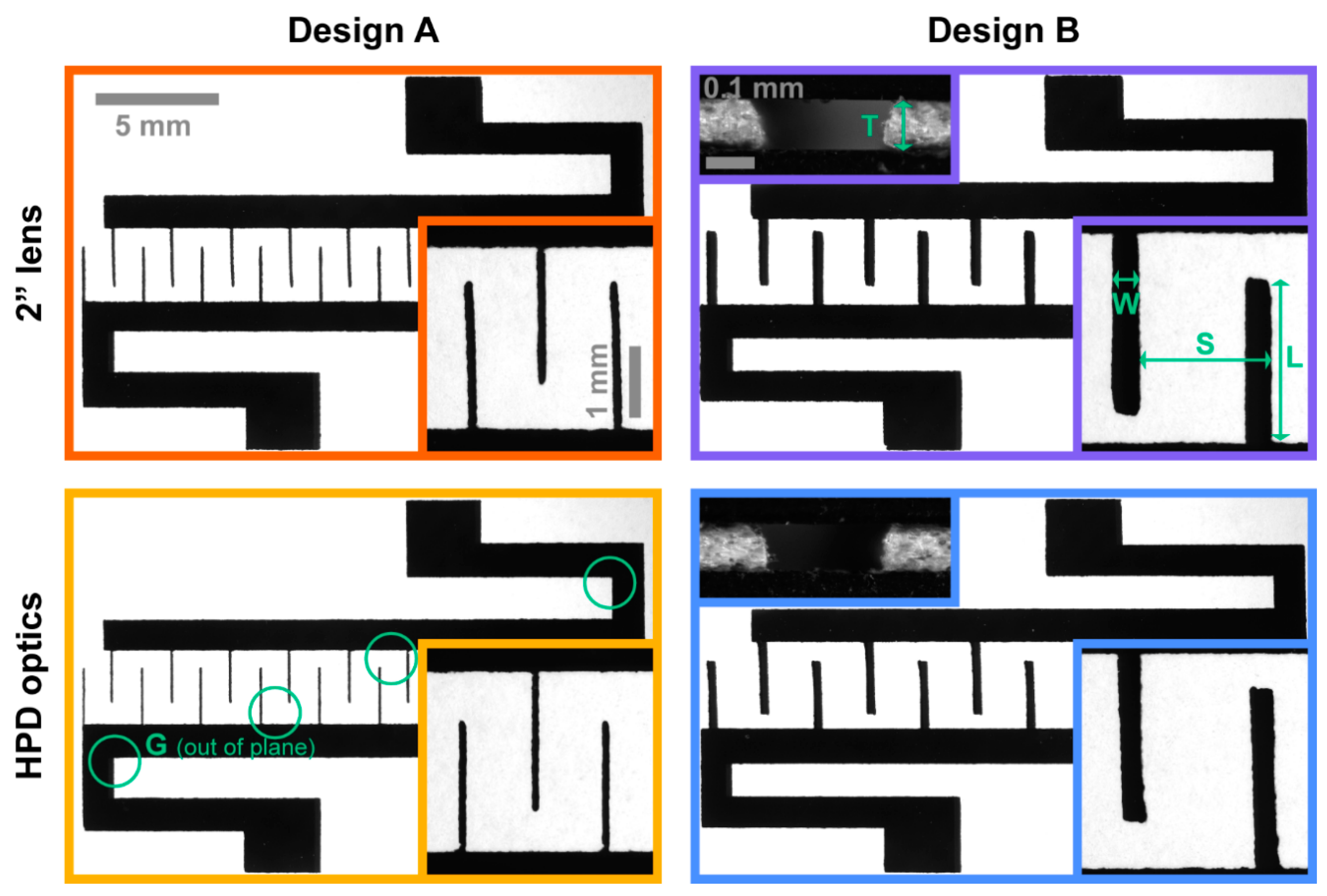
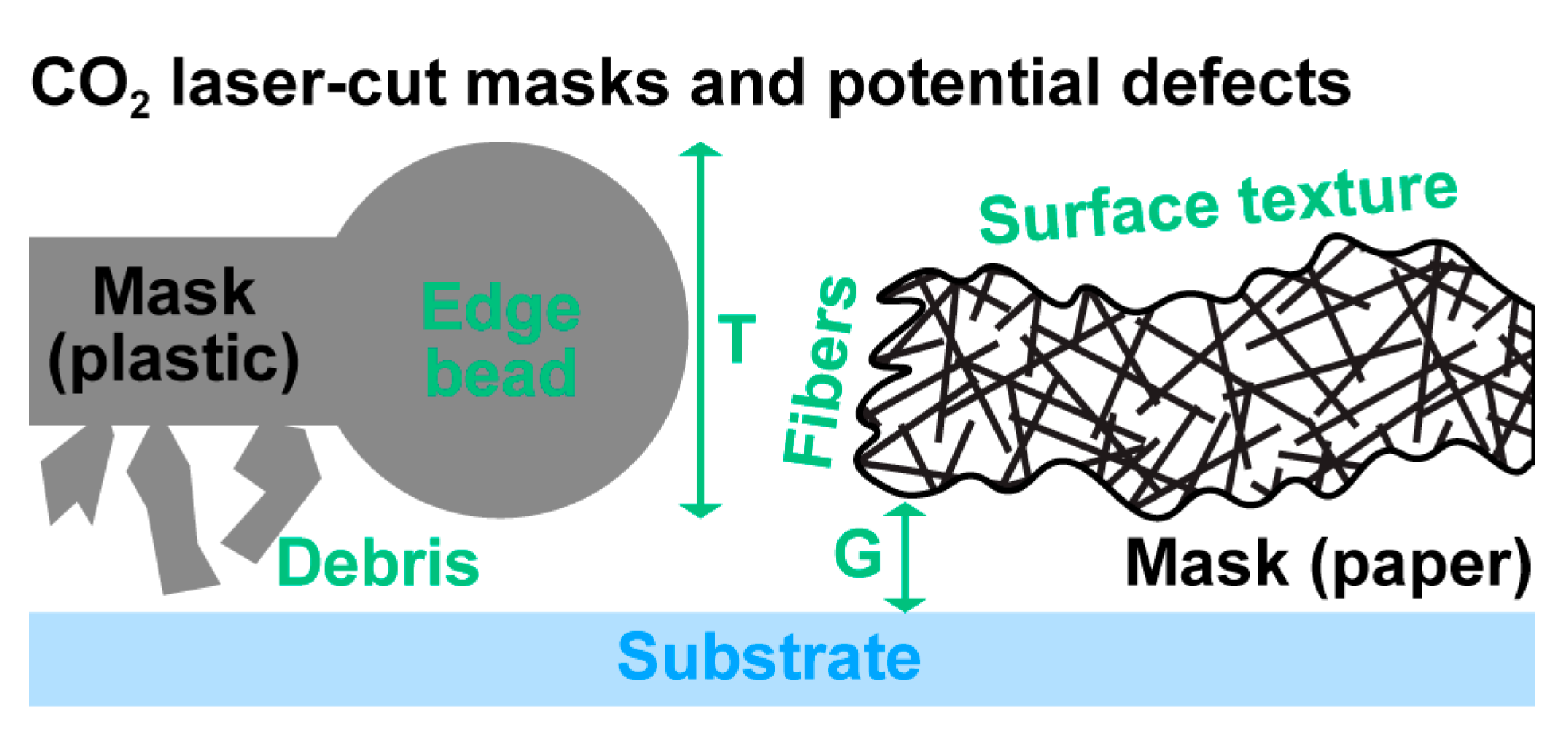
3.2. Cutting Quality
3.3. Masking Quality
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lammel, G. The future of MEMS sensors in our connected world. In Proceedings of the 2015 28th IEEE International Conference on Micro Electro Mechanical Systems (MEMS), Estoril, Portugal, 18–22 January 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 61–64. [Google Scholar]
- Khan, S.; Lorenzelli, L.; Dahiya, R.S. Technologies for Printing Sensors and Electronics Over Large Flexible Substrates: A Review. IEEE Sens. J. 2015, 15, 3164–3185. [Google Scholar] [CrossRef]
- Du, K.; Ding, J.; Liu, Y.; Wathuthanthri, I.; Choi, C.-H. Stencil Lithography for Scalable Micro- and Nanomanufacturing. Micromachines 2017, 8, 131. [Google Scholar] [CrossRef]
- Vazquez-Mena, O.; Gross, L.; Xie, S.; Villanueva, L.G.; Brugger, J. Resistless nanofabrication by stencil lithography: A review. Microelectron. Eng. 2015, 132, 236–254. [Google Scholar] [CrossRef]
- Kay, R.; Desmulliez, M. A review of stencil printing for microelectronic packaging. Solder. Surf. Mt. Technol. 2012, 24, 38–50. [Google Scholar] [CrossRef]
- Tao, H.; Chieffo, L.R.; Brenckle, M.A.; Siebert, S.M.; Liu, M.; Strikwerda, A.C.; Fan, K.; Kaplan, D.L.; Zhang, X.; Averitt, R.D.; et al. Metamaterials on Paper as a Sensing Platform. Adv. Mater. 2011, 23, 3197–3201. [Google Scholar] [CrossRef] [PubMed]
- Tao, H.; Brenckle, M.A.; Yang, M.; Zhang, J.; Liu, M.; Siebert, S.M.; Averitt, R.D.; Mannoor, M.S.; McAlpine, M.C.; Rogers, J.A.; et al. Silk-Based Conformal, Adhesive, Edible Food Sensors. Adv. Mater. 2012, 24, 1067–1072. [Google Scholar] [CrossRef]
- Kim, H.; Horwitz, J.S.; Kushto, G.P.; Kafafi, Z.H.; Chrisey, D.B. Indium tin oxide thin films grown on flexible plastic substrates by pulsed-laser deposition for organic light-emitting diodes. Appl. Phys. Lett. 2001, 79, 284–286. [Google Scholar] [CrossRef]
- Elmi, I.; Zampolli, S.; Cardinali, G.C. Optimization of a wafer-level process for the fabrication of highly reproducible thin-film MOX sensors. Sens. Actuators B Chem. 2008, 131, 548–555. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, B.; Hojaiji, H.; Wang, Z.; Lin, S.; Yeung, C.; Lin, H.; Nguyen, P.; Chiu, K.; Salahi, K.; et al. A wearable freestanding electrochemical sensing system. Sci. Adv. 2020, 6, eaaz0007. [Google Scholar] [CrossRef]
- Alatraktchi, F.; Bakmand, T.; Dimaki, M.; Svendsen, W. Novel Membrane-Based Electrochemical Sensor for Real-Time Bio-Applications. Sensors 2014, 14, 22128–22139. [Google Scholar] [CrossRef]
- Shiroma, L.Y.; Santhiago, M.; Gobbi, A.L.; Kubota, L.T. Separation and electrochemical detection of paracetamol and 4-aminophenol in a paper-based microfluidic device. Anal. Chim. Acta 2012, 725, 44–50. [Google Scholar] [CrossRef] [PubMed]
- Ishikawa, F.N.; Stauffer, B.; Caron, D.A.; Zhou, C. Rapid and label-free cell detection by metal-cluster-decorated carbon nanotube biosensors. Biosens. Bioelectron. 2009, 24, 2967–2972. [Google Scholar] [CrossRef] [PubMed]
- Moreno, S.; Baniasadi, M.; Mohammed, S.; Mejia, I.; Chen, Y.; Quevedo-Lopez, M.A.; Kumar, N.; Dimitrijevich, S.; Minary-Jolandan, M. Biocompatible Collagen Films as Substrates for Flexible Implantable Electronics. Adv. Electron. Mater. 2015, 1, 1500154. [Google Scholar] [CrossRef]
- Cheng, M.-Y.; Lin, C.-L.; Lai, Y.-T.; Yang, Y.-J. A Polymer-Based Capacitive Sensing Array for Normal and Shear Force Measurement. Sensors 2010, 10, 10211–10225. [Google Scholar] [CrossRef] [PubMed]
- Tönshoff, H.K.; Ostendorf, A.; Körber, K.; Kulik, C.; Kamlage, G. Micro Material Processing Using UV Laser and Femtosecond Laser. In Initiatives of Precision Engineering at the Beginning of a Millennium, Proceedings of the 10th International Conference on Precision Engineering (ICPE), Yokohama, Japan, 18–20 July 2001; Springer US: Boston, MA, USA, 2001; pp. 62–66. [Google Scholar]
- Li, L. Lasers in Technology. In Physical Methods, Instruments and Measurements; Tsipenyuk, Y.M., Ed.; EOLSS Publications: Oxford, UK, 2009; Volume 4, p. 390. ISBN 978-1-905839-57-5. [Google Scholar]
- Meng, L.; Mahpeykar, S.M.; Xiong, Q.; Ahvazi, B.; Wang, X. Strain sensors on water-soluble cellulose nanofibril paper by polydimethylsiloxane (PDMS) stencil lithography. RSC Adv. 2016, 6, 85427–85433. [Google Scholar] [CrossRef]
- Hwang, S.-W.; Tao, H.; Kim, D.-H.; Cheng, H.; Song, J.-K.; Rill, E.; Brenckle, M.A.; Panilaitis, B.; Won, S.M.; Kim, Y.-S.; et al. A Physically Transient Form of Silicon Electronics. Science 2012, 337, 1640–1644. [Google Scholar] [CrossRef]
- MatWeb Material Property Database. Available online: http://www.matweb.com/ (accessed on 1 July 2020).
- Fan, Y.; Li, H.; Yi, Y.; Foulds, I.G. Laser micromachined wax-covered plastic paper as both sputter deposition shadow masks and deep-ultraviolet patterning masks for polymethylmethacrylate-based microfluidic systems. J. Micro/Nanolithography MEMS MOEMS 2013, 12, 049701. [Google Scholar] [CrossRef][Green Version]
- Liu, X.; Mwangi, M.; Li, X.; O’Brien, M.; Whitesides, G.M. Paper-based piezoresistive MEMS sensors. Lab. Chip 2011, 11, 2189–2196. [Google Scholar] [CrossRef]
- Mahmud, M.A.; Blondeel, E.J.M.; Kaddoura, M.; MacDonald, B.D. Features in Microfluidic Paper-Based Devices Made by Laser Cutting: How Small Can They Be? Micromachines 2018, 9, 220. [Google Scholar] [CrossRef]
- Duchemin, B.J.C.; Mathew, A.P.; Oksman, K. All-cellulose composites by partial dissolution in the ionic liquid 1-butyl-3-methylimidazolium chloride. Compos. Part Appl. Sci. Manuf. 2009, 40, 2031–2037. [Google Scholar] [CrossRef]
- Manevich, A.I.; Ponomarenko, E.A.; Prokopalo, E.F. Stability of Orthotropic Cylindrical Shells under Bending by a Transverse Force. Part 2. Experiment. Strength Mater. 2013, 45, 205–209. [Google Scholar] [CrossRef]
- Soares, S.; Camino, G.; Levchik, S. Comparative study of the thermal decomposition of pure cellulose and pulp paper. Polym. Degrad. Stab. 1995, 49, 275–283. [Google Scholar] [CrossRef]
- Stine, J.M.; Beardslee, L.A.; Sathyam, R.M.; Bentley, W.E.; Ghodssi, R. Electrochemical Dissolved Oxygen Sensor-Integrated Platform for Wireless In Situ Bioprocess Monitoring. Sens. Actuators B Chem. 2020, 128381. [Google Scholar] [CrossRef]
- Pang, S.; Gao, Y.; Choi, S. Flexible and stretchable microbial fuel cells with modified conductive and hydrophilic textile. Biosens. Bioelectron. 2018, 100, 504–511. [Google Scholar] [CrossRef] [PubMed]
- Wissman, J.; Lu, T.; Majidi, C. Soft-matter electronics with stencil lithography. In Proceedings of the 2013 IEEE SENSORS, Baltimore, MD, USA, 3–6 January 2013; IEEE: Piscataway, NJ, USA, 2013; pp. 1–4. [Google Scholar]
- Schindelin, J.; Arganda-Carreras, I.; Frise, E.; Kaynig, V.; Longair, M.; Pietzsch, T.; Preibisch, S.; Rueden, C.; Saalfeld, S.; Schmid, B.; et al. Fiji: An open-source platform for biological-image analysis. Nat. Methods 2012, 9, 676–682. [Google Scholar] [CrossRef] [PubMed]
- Meijering, E.H.W.; Niessen, W.J.; Viergever, M.A. Quantitative evaluation of convolution-based methods for medical image interpolation. Med. Image Anal. 2001, 5, 111–126. [Google Scholar] [CrossRef]
- Li, C.H.; Tam, P.K.S. An iterative algorithm for minimum cross entropy thresholding. Pattern Recognit. Lett. 1998, 19, 771–776. [Google Scholar] [CrossRef]
- Sahoo, P. Surface topography. In Tribology for Engineers; Davim, J.P., Ed.; Woodhead Publishing: Cambridge, UK, 2011; pp. 1–32. ISBN 978-0-85709-114-7. [Google Scholar]
- Elhami Nik, F.; Matthiesen, I.; Herland, A.; Winkler, T.E. Raw Data Repository. Available online: https://doi.org/10.6084/m9.figshare.12595616 (accessed on 1 July 2020).
- Vazquez-Mena, O.; Villanueva, L.G.; Savu, V.; Sidler, K.; Langlet, P.; Brugger, J. Analysis of the blurring in stencil lithography. Nanotechnology 2009, 20, 415303. [Google Scholar] [CrossRef]
- Davim, J.P.; Barricas, N.; Conceição, M.; Oliveira, C. Some experimental studies on CO2 laser cutting quality of polymeric materials. J. Mater. Process. Technol. 2008, 198, 99–104. [Google Scholar] [CrossRef]
- GE Life Sciences. Laboratory Filtration Product Guide; GE Healthcare Bio-Sciences Corp.: Marlborough, MA, USA, 2019. [Google Scholar]
- Sartorius. Laboratory Filtration Products; Sartorius Lab Instruments GmbH & Co. KG: Göttingen, Germany, 2020. [Google Scholar]
| Photolithography | “Cold” Laser Machining | CO2 Laser Cutting | ||
|---|---|---|---|---|
| Tools | Full cleanroom | >€10,000 laser | >€100 laser | |
| Typical materials | Si, SiN, metal | Metal, plastics | Plastics | Filter paper (our work) |
| Thickness | <1 µm | <10 µm | ~100 µm | ~100 µm |
| Process cost $ | >€1000 | >€10–100 | <€1 | <€1 |
| Process complexity | Complex | Complex mounting | Simple, single-step | |
| Process artifacts | None | None | Particle ejecta, edge deformation | None |
| Mask handling | Fragile (brittle) | Fragile (thin) | Potential x/y/z distortion * | Resilient |
| Resolution | <1 µm | <10 µm | ~100 µm | 87 µm line width, ±58 µm accuracy (wafer-scale) |
| Design | Width W | Length L | Spacing S |
|---|---|---|---|
| A | 0 µm * | 1875 µm | 1000 µm |
| B | 200 µm | 2375 µm | 1800 µm |
| Type | Density 1 | Nominal T 1 | T2 | Roughness 2 | Waviness 2 |
|---|---|---|---|---|---|
| Whatman 50 | 0.83 g/cm³ | 115 µm | 98 ± 5 µm | 3.6 ± 0.4 µm | 1.1 ± 0.2 µm |
| Whatman 540 | 0.53 g/cm³ | 160 µm | 128 ± 8 µm | 7.0 ± 1.5 µm | 4.1 ± 2.9 µm |
| Sartorius 393 | 0.59 g/cm³ | 170 µm | 135 ± 3 µm | 4.5 ± 0.5 µm | 2.1 ± 0.3 µm |
| Sartorius 392 | 0.49 g/cm³ | 170 µm | 126 ± 3 µm | 11.4 ± 1.7 µm | 7.2 ± 2.2 µm |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elhami Nik, F.; Matthiesen, I.; Herland, A.; Winkler, T.E. Low-Cost PVD Shadow Masks with Submillimeter Resolution from Laser-Cut Paper. Micromachines 2020, 11, 676. https://doi.org/10.3390/mi11070676
Elhami Nik F, Matthiesen I, Herland A, Winkler TE. Low-Cost PVD Shadow Masks with Submillimeter Resolution from Laser-Cut Paper. Micromachines. 2020; 11(7):676. https://doi.org/10.3390/mi11070676
Chicago/Turabian StyleElhami Nik, Farzad, Isabelle Matthiesen, Anna Herland, and Thomas E. Winkler. 2020. "Low-Cost PVD Shadow Masks with Submillimeter Resolution from Laser-Cut Paper" Micromachines 11, no. 7: 676. https://doi.org/10.3390/mi11070676
APA StyleElhami Nik, F., Matthiesen, I., Herland, A., & Winkler, T. E. (2020). Low-Cost PVD Shadow Masks with Submillimeter Resolution from Laser-Cut Paper. Micromachines, 11(7), 676. https://doi.org/10.3390/mi11070676