Intermittency of Gravity Wave Potential Energy Generated by Mountains Revealed from COSMIC-2 Observations
Abstract
1. Introduction
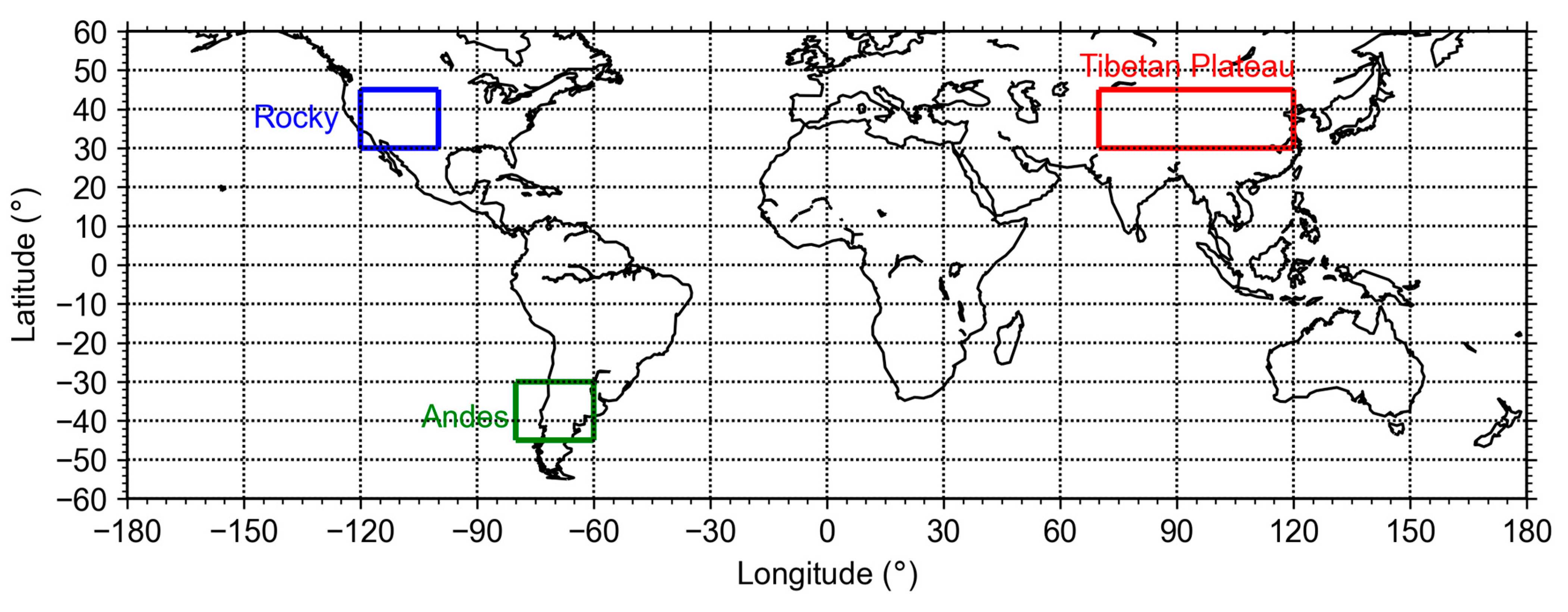
2. Data and Methods
2.1. Data
2.2. Calculating Gravity Wave Potential Energy
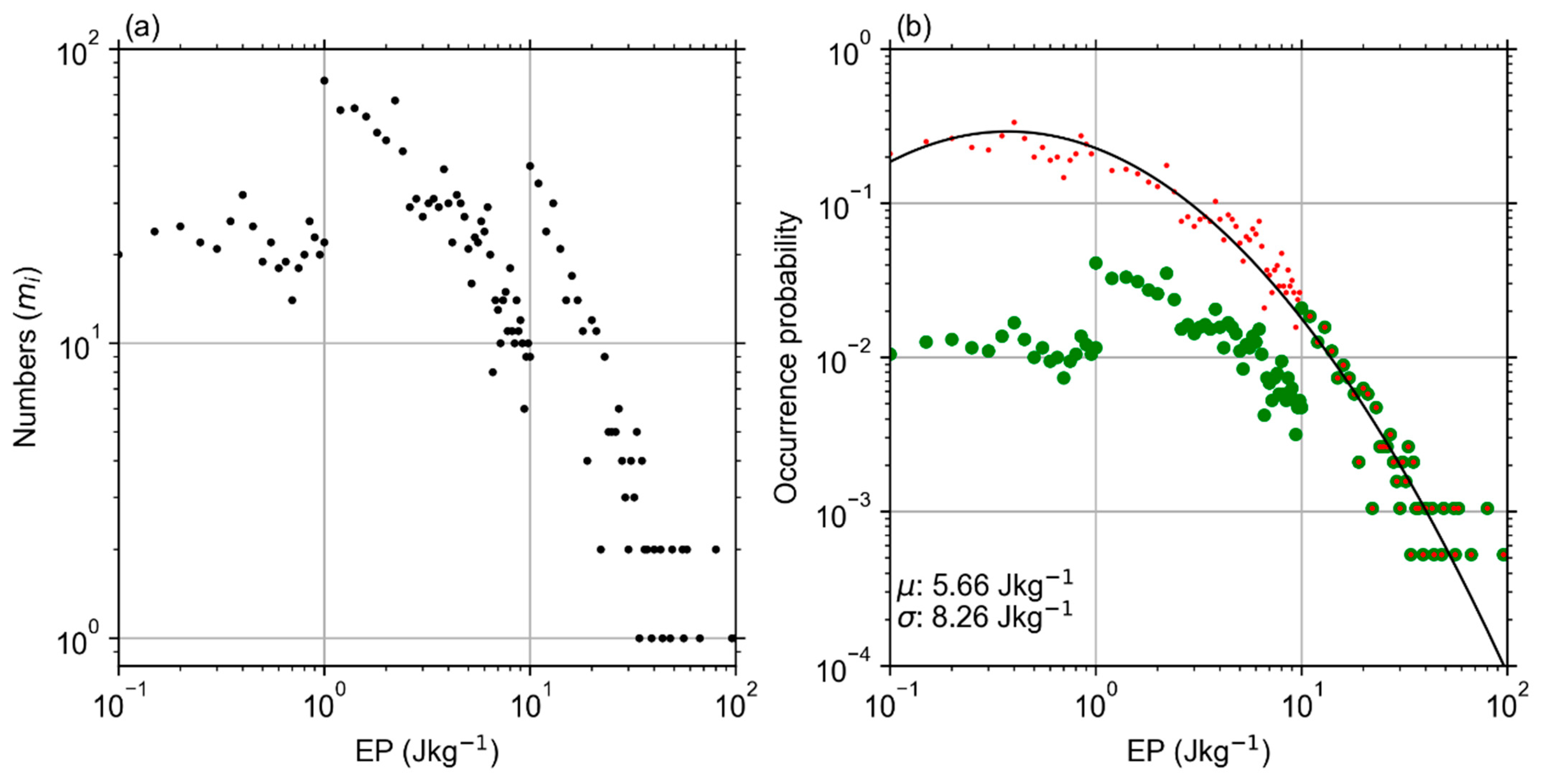
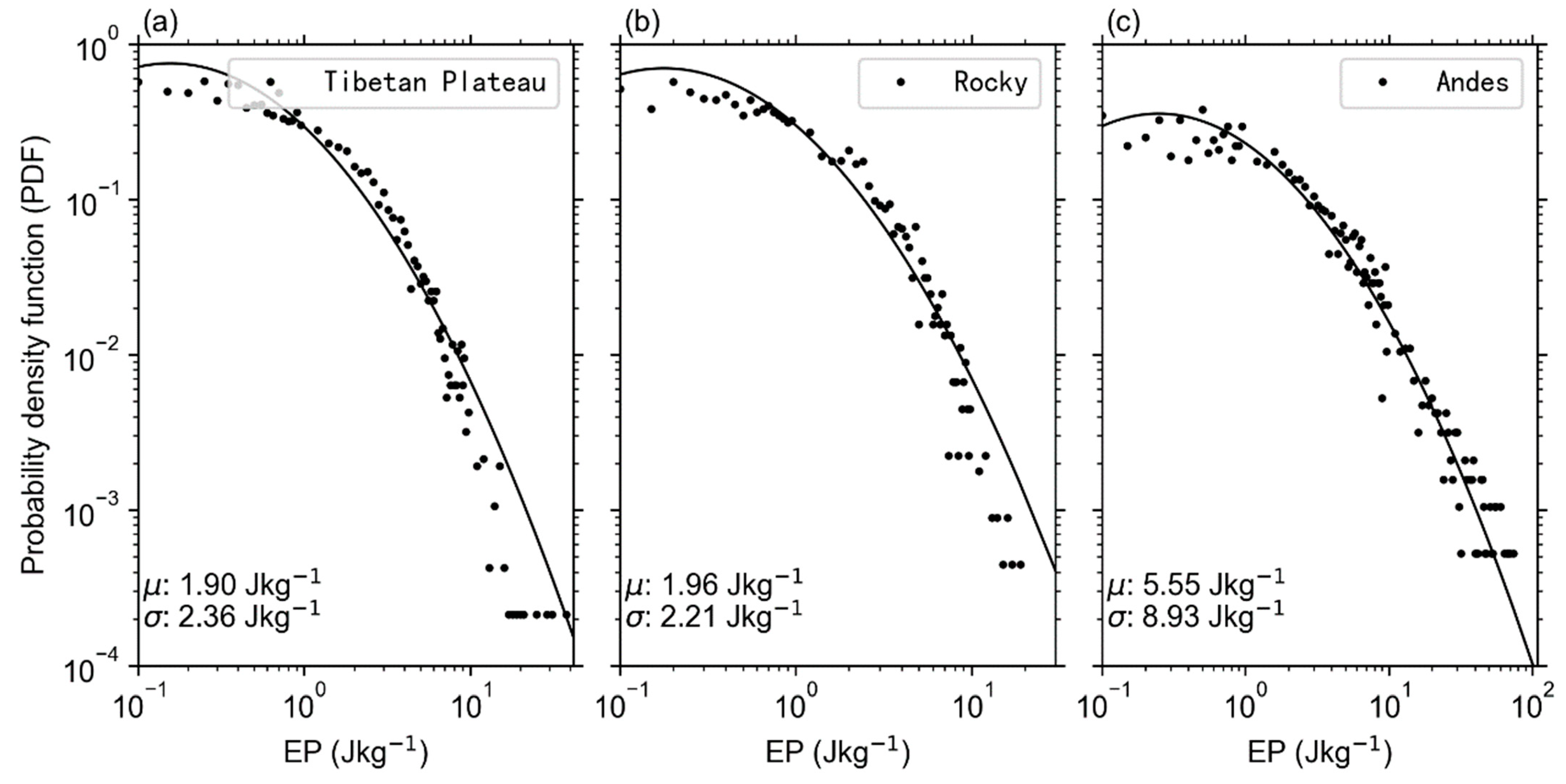
2.3. Calculating the PDF of GWPE
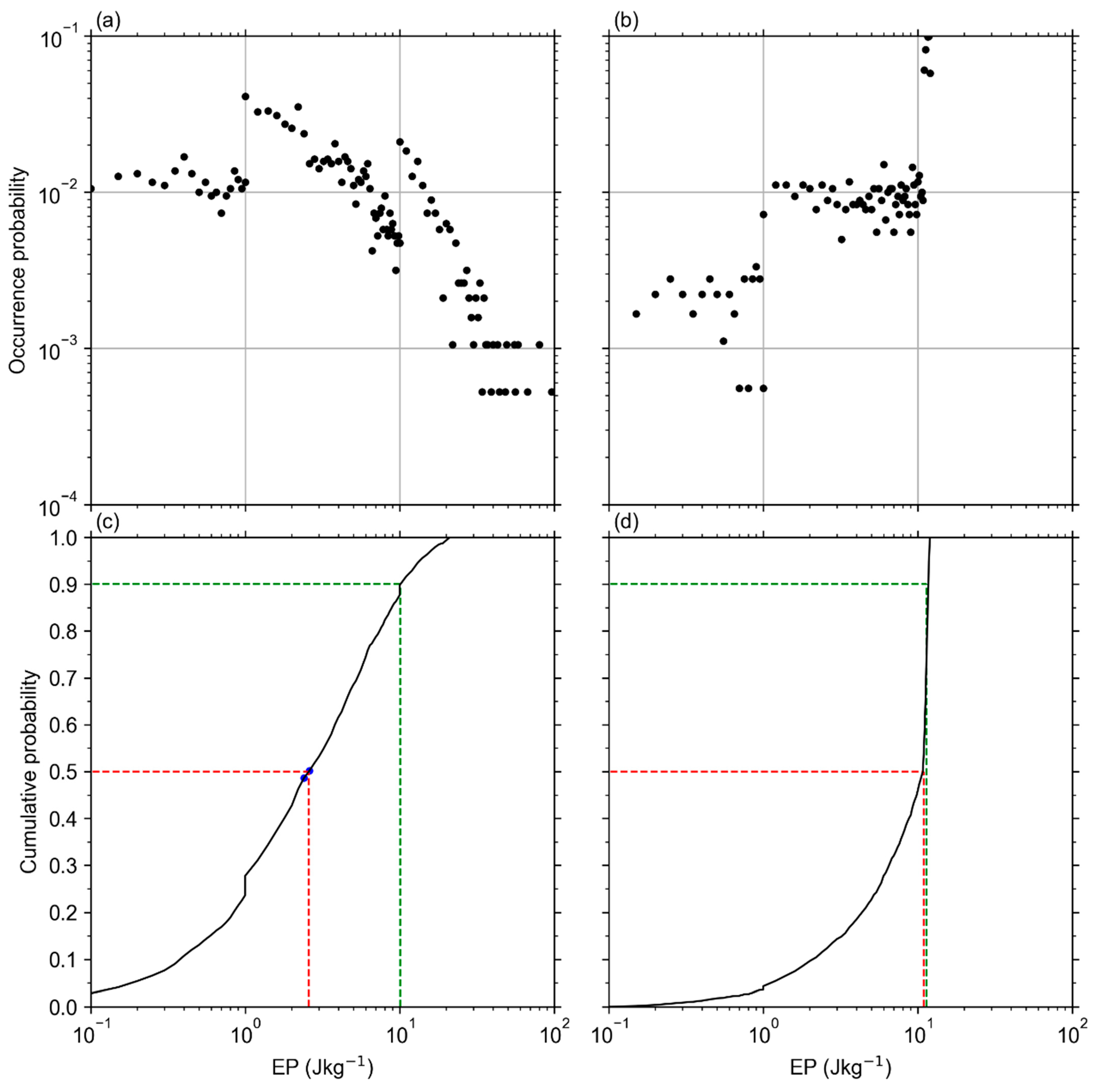
2.4. Calculating the GWPE Intermittency
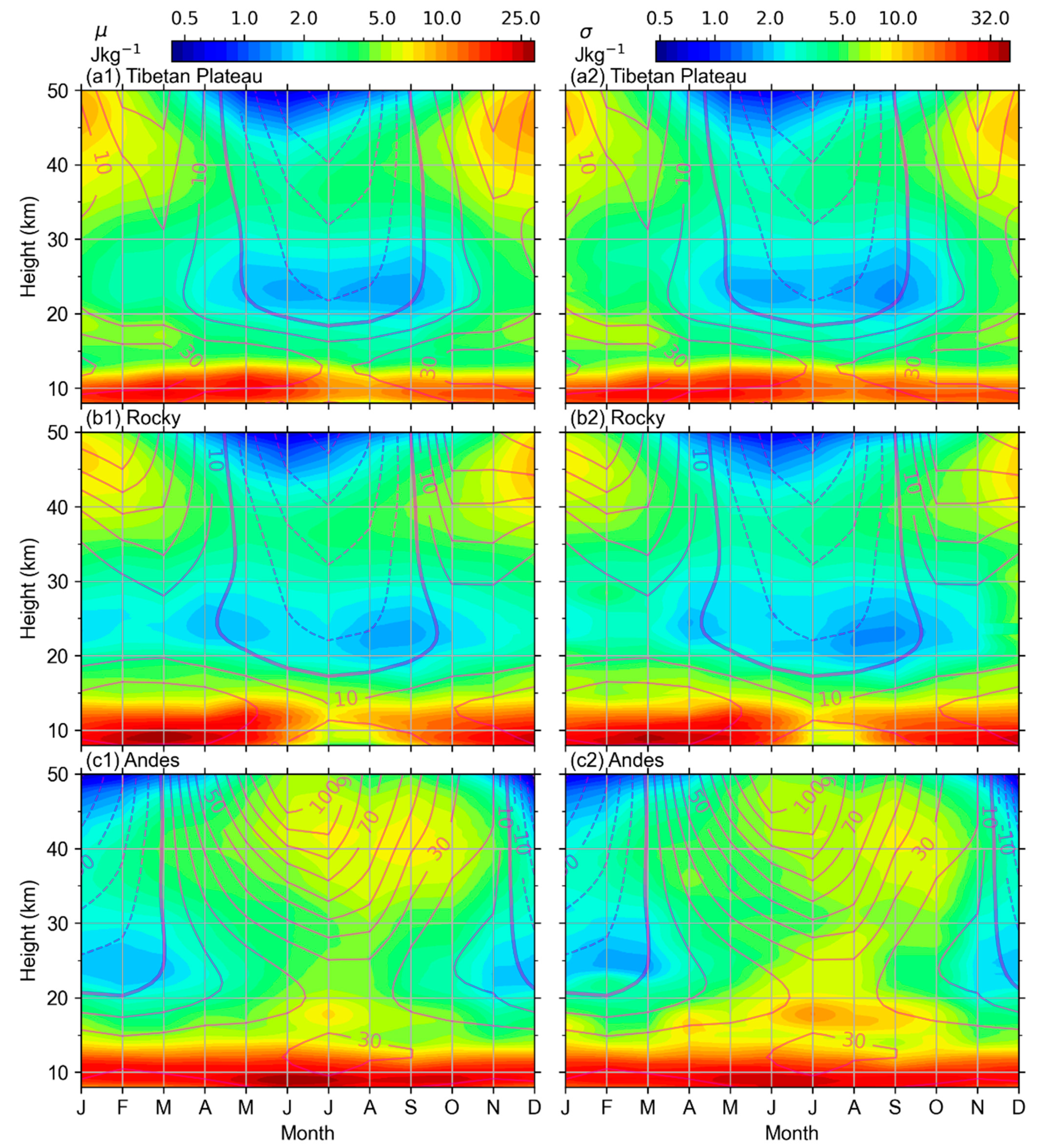
3. PDFs of GWPE over Mountains
4. Intermittency of GWPE over Mountains
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fritts, D.C.; Alexander, M.J. Gravity wave dynamics and effects in the middle atmosphere. Rev. Geophys. 2003, 41, 1–64. [Google Scholar] [CrossRef]
- Bacmeister, J.T.; Schoeberl, M.R. Breakdown of vertically propagating two-dimensional gravity waves forced by orography. J. Atmos. Sci. 1989, 46, 2109–2134. [Google Scholar] [CrossRef]
- Vadas, S.L.; Fritts, D.C. The importance of spatial variability in the generation of secondary gravity waves from local body forces. Geophys. Res. Lett. 2002, 29, 45-1–45-4. [Google Scholar] [CrossRef]
- Vadas, S.L.; Fritts, D.C.; Alexander, M.J. Mechanism for the generation of secondary waves in wave breaking regions. J. Atmos. Sci. 2003, 60, 194–214. [Google Scholar] [CrossRef]
- Vadas, S.L.; Becker, E. Numerical modeling of the generation of tertiary gravity waves in the mesosphere and thermosphere during strong mountain wave events over the Southern Andes. J. Geophys. Res. Space Phys. 2019, 124, 7687–7718. [Google Scholar] [CrossRef]
- Vadas, S.L.; Xu, S.; Yue, J.; Bossert, K.; Becker, E.; Baumgarten, G. Characteristics of the quiet-time hot spot gravity waves observed by GOCEE over the Southern Andes on 5 July 2010. J. Geophys. Res. Space Phys. 2019, 124, 7034–7061. [Google Scholar] [CrossRef]
- Hertzog, A.; Alexander, M.J.; Plougonven, R. On the intermittency of gravity wave momentum flux in the stratosphere. J. Atmos. Sci. 2012, 69, 3433–3448. [Google Scholar] [CrossRef]
- Kuchar, A.; Sacha, P.; Eichinger, R.; Jacobi, C.; Pisoft, P.; Rieder, H.E. On the intermittency of orographic gravity wave hotspots and its importance for middle atmosphere dynamics. Weather. Clim. Dyn. 2020, 1, 481–495. [Google Scholar] [CrossRef]
- Hertzog, A.; Boccara, G.; Vincent, R.A.; Vial, F.; Cocquerez, P. Estimation of gravity wave momentum flux and phase speeds from quasi-lagrangian stratospheric balloon flights. Part II: Results from the vorcore campaign in antarctica. J. Atmos. Sci. 2008, 65, 3056–3070. [Google Scholar] [CrossRef]
- Cao, B.; Liu, A.Z. Intermittency of gravity wave momentum flux in the mesopause region observed with an all-sky airglow imager. J. Geophys. Res. Atmos. 2016, 121, 650–663. [Google Scholar] [CrossRef]
- Ern, M.; Preusse, P.; Riese, M. Intermittency of gravity wave potential energies and absolute momentum fluxes derived from infrared limb sounding satellite observations. Atmos. Chem. Phys. 2022, 22, 15093–15133. [Google Scholar] [CrossRef]
- Wright, C.J.; Osprey, S.M.; Gille, J.C. Global observations of gravity wave intermittency and its impact on the observed momentum flux morphology. J. Geophys. Res. Atmos. 2013, 118, 10980–10993. [Google Scholar] [CrossRef]
- Jewtoukoff, V.; Hertzog, A.; Plougonven, R.; de la Cámara, A.; Lott, F. Comparison of gravity waves in the southern hemisphere derived from balloon observations and the ECMWF analyses. J. Atmos. Sci. 2015, 72, 3449–3468. [Google Scholar] [CrossRef]
- Alexander, S.P.; Sato, K.; Watanabe, S.; Kawatani, Y.; Murphy, D.J. Southern hemisphere extratropical gravity wave sources and intermittency revealed by a middle-atmosphere general circulation model. J. Atmos. Sci. 2016, 73, 1335–1349. [Google Scholar] [CrossRef]
- Love, P.T.; Murphy, D.J. Gravity wave momentum flux in the mesosphere measured by VHF radar at Davis, Antarctica. J. Geophys. Res. Atmos. 2016, 121, 12723–12736. [Google Scholar] [CrossRef]
- Minamihara, Y.; Sato, K.; Tsutsumi, M. Intermittency of gravity waves in the Antarctic troposphere and lower stratosphere revealed by the PANSY radar observation. J. Geophys. Res. Atmos. 2020, 125, e2020JD032543. [Google Scholar] [CrossRef]
- Bühler, O. Equatorward propagation of inertia-gravity waves due to steady and intermittent wave sources. J. Atmos. Sci. 2003, 60, 1410–1419. [Google Scholar] [CrossRef]
- Plougonven, R.; Hertzog, A.; Guez, L. Gravity waves over antarctica and the southern ocean: Consistent momentum fluxes in mesoscale simulations and stratospheric balloon observations. Q. J. R. Meteor. Soc. 2013, 139, 101–118. [Google Scholar] [CrossRef]
- de la Cámara, A.; Lott, F. A parameterization of gravity waves emitted by fronts and jets. Geophys. Res. Lett. 2015, 42, 2071–2078. [Google Scholar] [CrossRef]
- Jewtoukoff, V.; Plougonven, R.; Hertzog, A. Gravity waves generated by deep tropical convection: Estimates from balloon observations and mesoscale simulations. J. Geophys. Res. Atmos. 2013, 118, 9690–9707. [Google Scholar] [CrossRef]
- Ding, Y.H. Effects of the Qinghai-Xizang (Tibetan) plateau on the circulation features over the plateau and its surrounding areas. Adv. Atmos. Sci. 1992, 9, 112–130. [Google Scholar]
- Lin, Y.H.; Zhang, L.J. Characteristics of gravity waves over the Tibetan Plateau during the PRC-Japan Cooperative JICA Project in 2008. J. Meteorol. Soc. Jpn. 2012, 90C, 215–223. [Google Scholar] [CrossRef]
- Anthes, R.; Schreiner, W. Six new satellites watch the atmosphere over Earth’s equator. Eos 2019, 100. [Google Scholar] [CrossRef]
- Schreiner, W.S.; Weiss, J.P.; Anthes, R.A.; Braun, J.; Chu, V.; Fong, J. COSMIC-2 radio occultation constellation: First results. Geophys. Res. Lett. 2020, 47, e2019GL086841. [Google Scholar] [CrossRef]
- Ho, S.P.; Zhou, X.; Shao, X.; Zhang, B.; Adhikari, L.; Kireev, S. Initial assessment of the COSMIC-2/FORMOSAT-7 neutral atmosphere data quality in nesdis/star using in situ and satellite data. Remote Sens. 2020, 12, 4099. [Google Scholar] [CrossRef]
- Hindley, N.P.; Wright, C.J.; Smith, N.D.; Mitchell, N.J. The southern stratospheric gravity-wave hot spot: Individual waves and their momentum fluxes measured by COSMIC GPS-RO. Atmos. Chem. Phys. 2015, 15, 7797–7818. [Google Scholar] [CrossRef]
- Gelaro, R.; McCarty, W.; Suárez, M.J.; Todling, R.; Molod, A.; Takacs, L. The Modern-Era Retrospective Analysis for Research and Applications, Version 2 (MERRA-2). J. Clim. 2017, 30, 5419–5454. [Google Scholar] [CrossRef]
- Coy, L.; Wargan, K.; Molod, A.M.; McCarty, W.R.; Pawson, S. Structure and dynamics of the quasi-biennial oscillation in merra-2. J. Clim. 2016, 29, 5339–5354. [Google Scholar] [CrossRef]
- Manney, G.L.; Hegglin, M.I. Seasonal and regional variations of long-term changes on upper-tropospheric jets from reanalyses. J. Clim. 2018, 31, 423–448. [Google Scholar] [CrossRef]
- Liu, X.; Yue, J.; Xu, J.; Garcia, R.R.; Russell, J.M.; Mlynczak, M. Variations of global gravity waves derived from 14 years of saber temperature observations. J. Geophys. Res. Atmos. 2017, 122, 6231–6249. [Google Scholar] [CrossRef]
- Liu, X.; Xu, J.; Yue, J.; Vadas, S.L.; Becker, E. Orographic primary and secondary gravity waves in the middle atmosphere from 16-year saber observations. Geophys. Res. Lett. 2019, 46, 4512–4522. [Google Scholar] [CrossRef]
- Liu, X.; Xu, J.; Yue, J.; Liu, H. Gravity-wave-perturbed wind shears derived from saber temperature observations. Atmos. Chem. Phys. 2020, 20, 14437–14456. [Google Scholar] [CrossRef]
- Torrence, C.; Compo, G.P. A practical guide to wavelet analysis. Bull. Am. Meteor. Soc. 1998, 79, 61–78. [Google Scholar] [CrossRef]
- Tsuda, T.; Nishida, M.; Rocken, C.; Ware, R.H. A global morphology of gravity wave activity in the stratosphere revealed by the GPS occultation data (GPS/MET). J. Geophys. Res. Atmos. 2000, 105, 7257–7273. [Google Scholar] [CrossRef]
- Wang, L.; Geller, M.A. Morphology of gravity-wave energy as observed from 4 years (1998–2001) of high vertical resolution U.S. radiosonde data. J. Geophys. Res. Atmos. 2003, 108. [Google Scholar] [CrossRef]
- Liu, X.; Yue, J.; Xu, J.; Wang, L.; Yuan, W.; Russell, J.M., III; Hervig, M.E. Gravity wave variations in the polar stratosphere and mesosphere from SOFIE/AIM temperature observations. J. Geophys. Res. Atmos. 2014, 119, 7368–7381. [Google Scholar] [CrossRef]
- Schoon, L.; Zülicke, C. A novel method for the extraction of local gravity wave parameters from gridded three-dimensional data: Description, validation, and application. Atmos. Chem. Phys. 2018, 18, 6971–6983. [Google Scholar] [CrossRef]
- Baumgaertner, A.J.G.; McDonald, A.J. A gravity wave climatology for Antarctica compiled from Challenging Minisatellite Payload/Global Positioning System (CHAMP/GPS) radio occultations. J. Geophys. Res. Atmos. 2007, 112, D5. [Google Scholar] [CrossRef]
- Liu, X.; Xu, J.; Liu, H.L.; Ma, R. Nonlinear interactions between gravity waves with different wavelengths and diurnal tide. J. Geophys. Res. 2008, 113, D08112. [Google Scholar] [CrossRef]
- Li, T.; Leblanc, T.; McDermid, I.S.; Wu, D.L.; Dou, X.; Wang, S. Seasonal and interannual variability of gravity wave activity revealed by long-term lidar observations over Mauna Loa Observatory, Hawaii. J. Geophys. Res. 2010, 115, D13103. [Google Scholar] [CrossRef]
- Hei, H.; Tsuda, T.; Hirooka, T. Characteristics of atmospheric gravity wave activity in the polar regions revealed by GPS radio occultation data with CHAMP. J. Geophys. Res. 2008, 113, D04107. [Google Scholar] [CrossRef]
- Yan, X.; Arnold, N.; Remedios, J. Global observations of gravity waves from High Resolution Dynamics Limb Sounder temperature measurements: A yearlong record of temperature amplitude and vertical wavelength. J. Geophys. Res. Atmos. 2010, 115, D10113. [Google Scholar] [CrossRef]
- Faber, A.; Llamedo, P.; Schmit, T.; de la Torre, A.; Wickert, J. On the determination of gravity wave momentum flux from GPS radio occultation data. Atmos. Meas. Technol. 2013, 6, 3169–3180. [Google Scholar] [CrossRef]
- Ern, M.; Trinh, Q.T.; Preusse, P.; Gille, J.C.; Mlynczak, M.G.; Iii, J.M.R.; Riese, M. GRACILE: A comprehensive climatology of atmospheric gravity wave parameters based on satellite limb soundings. Earth Syst. Sci. Data 2018, 10, 857–892. [Google Scholar] [CrossRef]
- Alexander, S.P.; Tsuda, T.; Kawatani, Y. COSMIC GPS observations of Northern Hemisphere winter stratospheric gravity waves and comparisons with an atmospheric general circultation model. Geophys. Res. Lett. 2008, 35, L10808. [Google Scholar] [CrossRef]
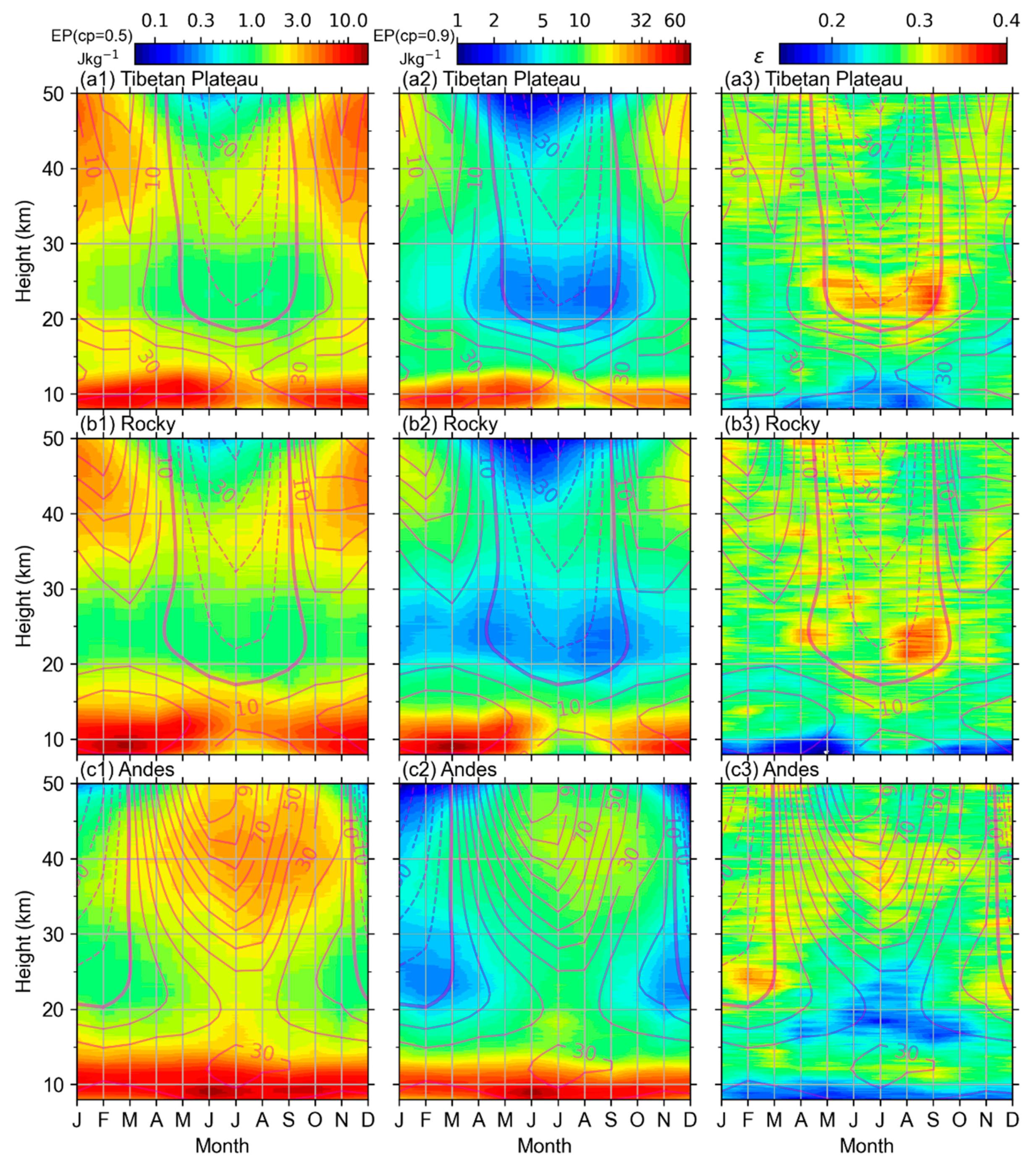
| Boreal Spring | Boreal Summer | Boreal Autumn | Boreal Winter | ||
|---|---|---|---|---|---|
| 8–10 km | Tibetan Plateau | ~0.2 (weaker) | 0.18–0.2 (stronger) | 0.2–0.3 (weaker) | 0.18–0.2 (stronger) |
| Rocky | 0.14–0.18 (strongest) | 0.2–0.3 (weaker) | 0.14–0.18 (strongest) | 0.14–0.18 (strongest) | |
| Andes | 0.18–0.2 (stronger) | 0.18–0.2 (stronger) | 0.18–0.2 (stronger) | 0.18–0.2 (stronger) | |
| 10–13 km | Tibetan Plateau | 0.2–0.3 (stronger) | 0.14–0.2 (strongest) | 0.2–0.3 (stronger) | 0.2–0.3 (stronger) |
| Rocky | 0.2–0.3 (stronger) | 0.25–0.35 (weaker) | 0.2–0.3 (stronger) | 0.2–0.3 (stronger) | |
| Andes | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | |
| 13–20 km | Tibetan Plateau | 0.2–0.3 (stronger) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.2–0.3 (stronger) |
| Rocky | 0.2–0.3 (stronger) | 0.25–0.35 (weaker) | 0.2–0.3 (stronger) | 0.2–0.3 (stronger) | |
| Andes | 0.14–0.2 (strongest) | 0.14–0.2 (strongest) | 0.14–0.2 (strongest) | 0.2–0.3 (stronger) | |
| 20–25 km | Tibetan Plateau | 0.2–0.3 (stronger) | 0.3–0.4 (weakest) | 0.3–0.4 (weakest) | 0.2–0.3 (stronger) |
| Rocky | 0.25–0.35 (weaker) | 0.3–0.4 (weakest) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | |
| Andes | 0.2–0.3 (stronger) | 0.14–0.2 (strongest) | 0.2–0.3 (stronger) | 0.3–0.4 (weakest) | |
| 25–30 km | Tibetan Plateau | 0.2–0.3 (stronger) | 0.3–0.4 (weakest) | 0.3–0.4 (weakest) | 0.2–0.3 (stronger) |
| Rocky | 0.25–0.35 (weaker) | 0.3–0.4 (weakest) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | |
| Andes | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.3–0.4 (weakest) | |
| 30–50 km | Tibetan Plateau | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) |
| Rocky | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | |
| Andes | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) | 0.25–0.35 (weaker) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, J.; Xu, J.; Liu, X. Intermittency of Gravity Wave Potential Energy Generated by Mountains Revealed from COSMIC-2 Observations. Remote Sens. 2024, 16, 1577. https://doi.org/10.3390/rs16091577
Wei J, Xu J, Liu X. Intermittency of Gravity Wave Potential Energy Generated by Mountains Revealed from COSMIC-2 Observations. Remote Sensing. 2024; 16(9):1577. https://doi.org/10.3390/rs16091577
Chicago/Turabian StyleWei, Jiarui, Jiyao Xu, and Xiao Liu. 2024. "Intermittency of Gravity Wave Potential Energy Generated by Mountains Revealed from COSMIC-2 Observations" Remote Sensing 16, no. 9: 1577. https://doi.org/10.3390/rs16091577
APA StyleWei, J., Xu, J., & Liu, X. (2024). Intermittency of Gravity Wave Potential Energy Generated by Mountains Revealed from COSMIC-2 Observations. Remote Sensing, 16(9), 1577. https://doi.org/10.3390/rs16091577




