Joint Radar Jamming and Communication System Design Based on Universal Filtered Multicarrier Chirp Waveform
Abstract
1. Introduction
2. Contributions of Our Work
- Generated UFMC chirp waveforms for the joint function of radar jamming and communication.
- Analyzed the advantages of UFMC in radar jamming and communication joint systems, discussed the constraints of joint waveforms, provided the auxiliary process of joint waveforms for communication subsystems, and provided a design trade-off analysis of radar jamming and communication.
- Conducted simulation experiments; the results indicate that the joint waveform based on UFMC chirp can effectively enable jamming with radar and exhibit excellent communication error rate performance.
- Implemented the proposed method on software defined radio (SDR) devices and demonstrated its feasibility.
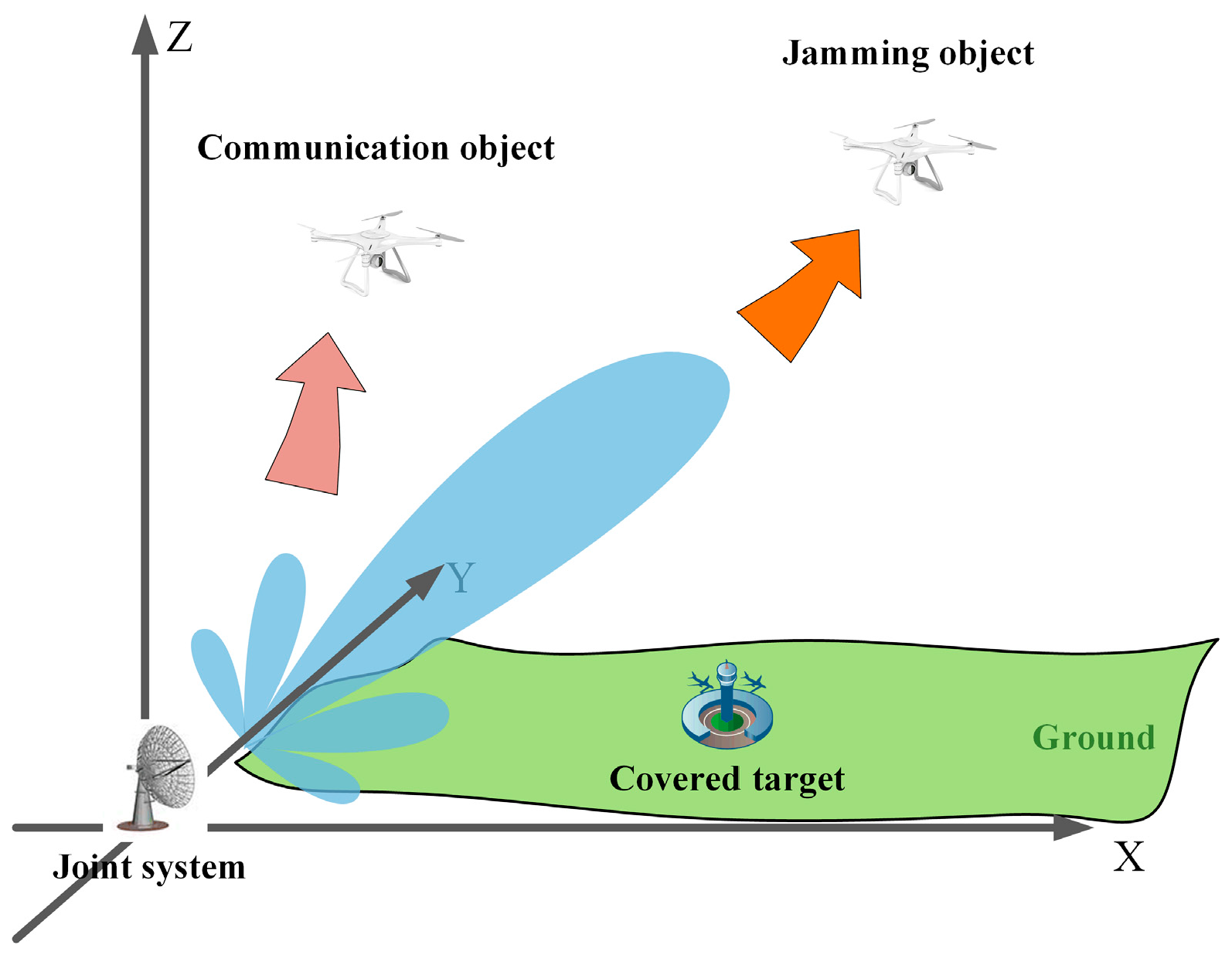
3. Model Construction and Application Scenarios
4. Implementation of Joint Plans
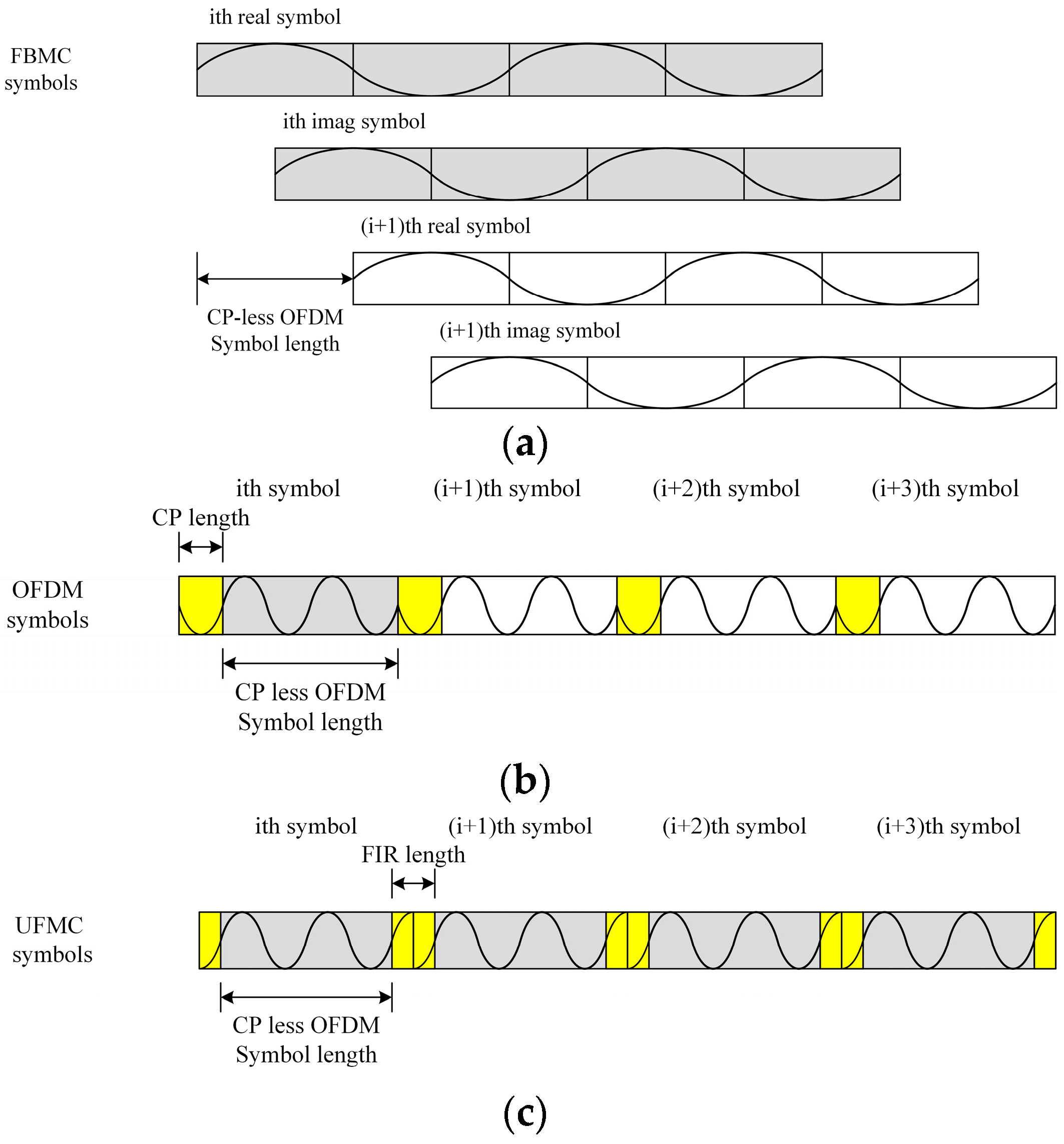
4.1. UFMC Modulation Principle
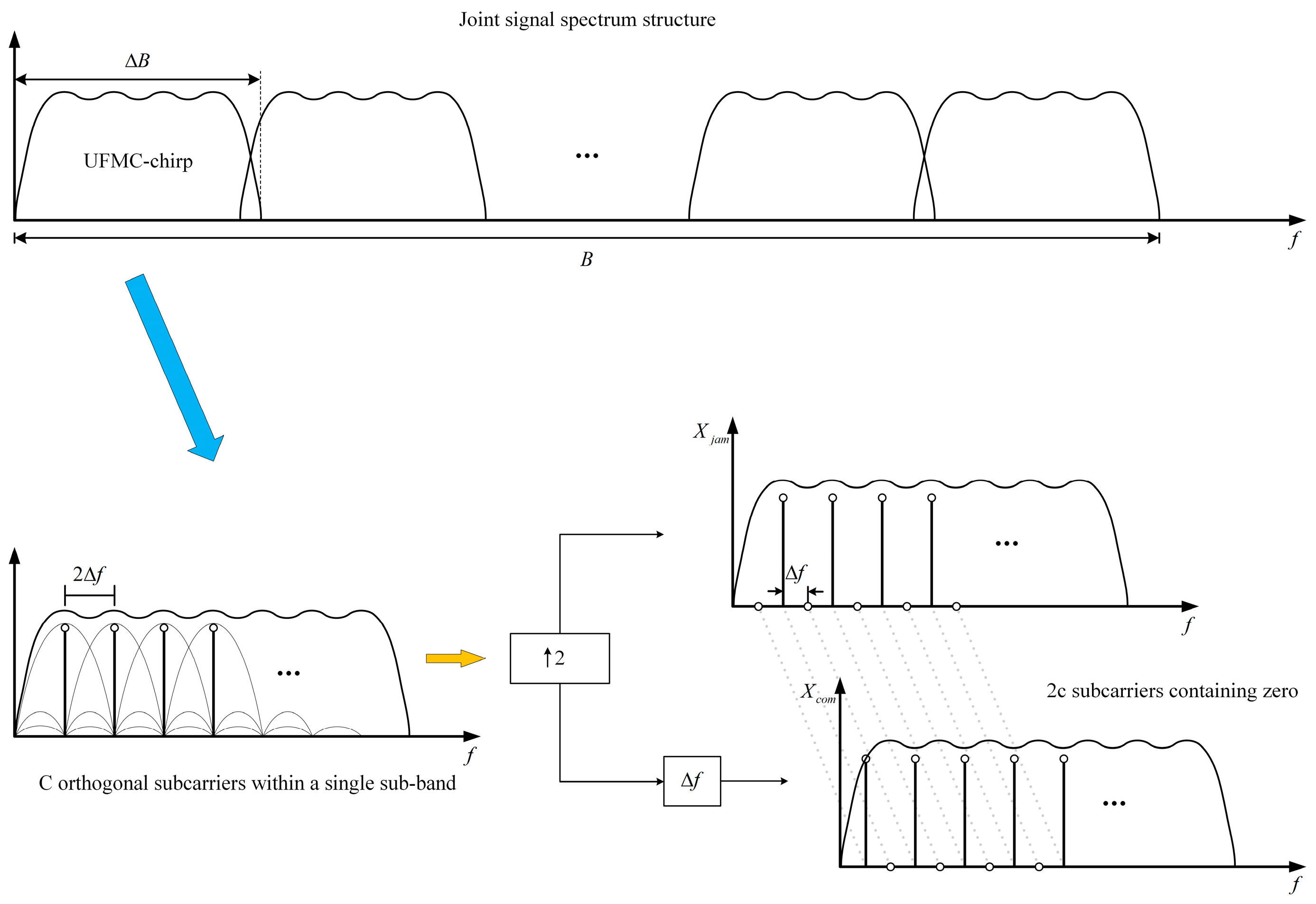
4.2. Joint Signal Design
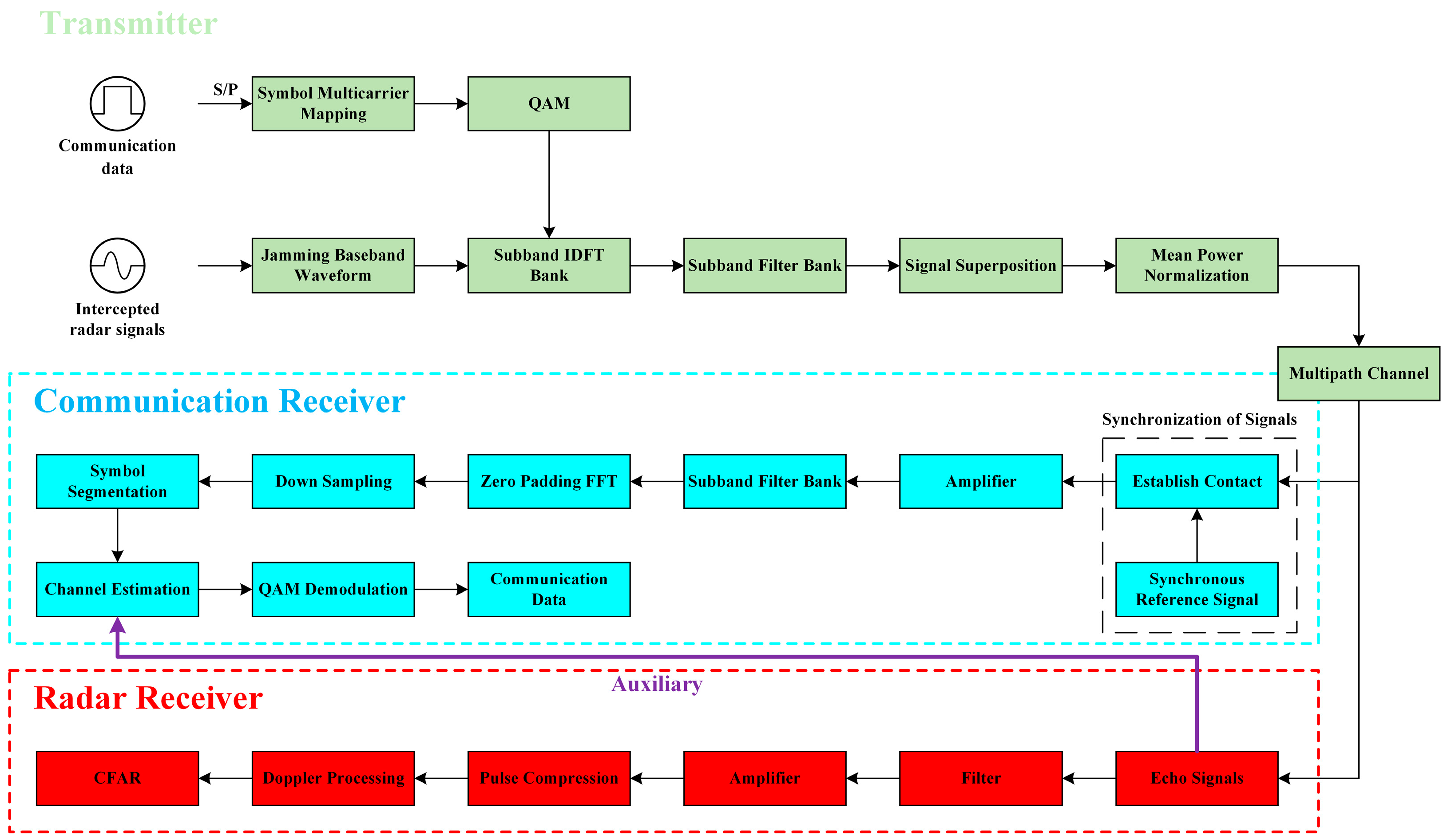
4.3. Joint Signal Processing Flow
5. System Constraints
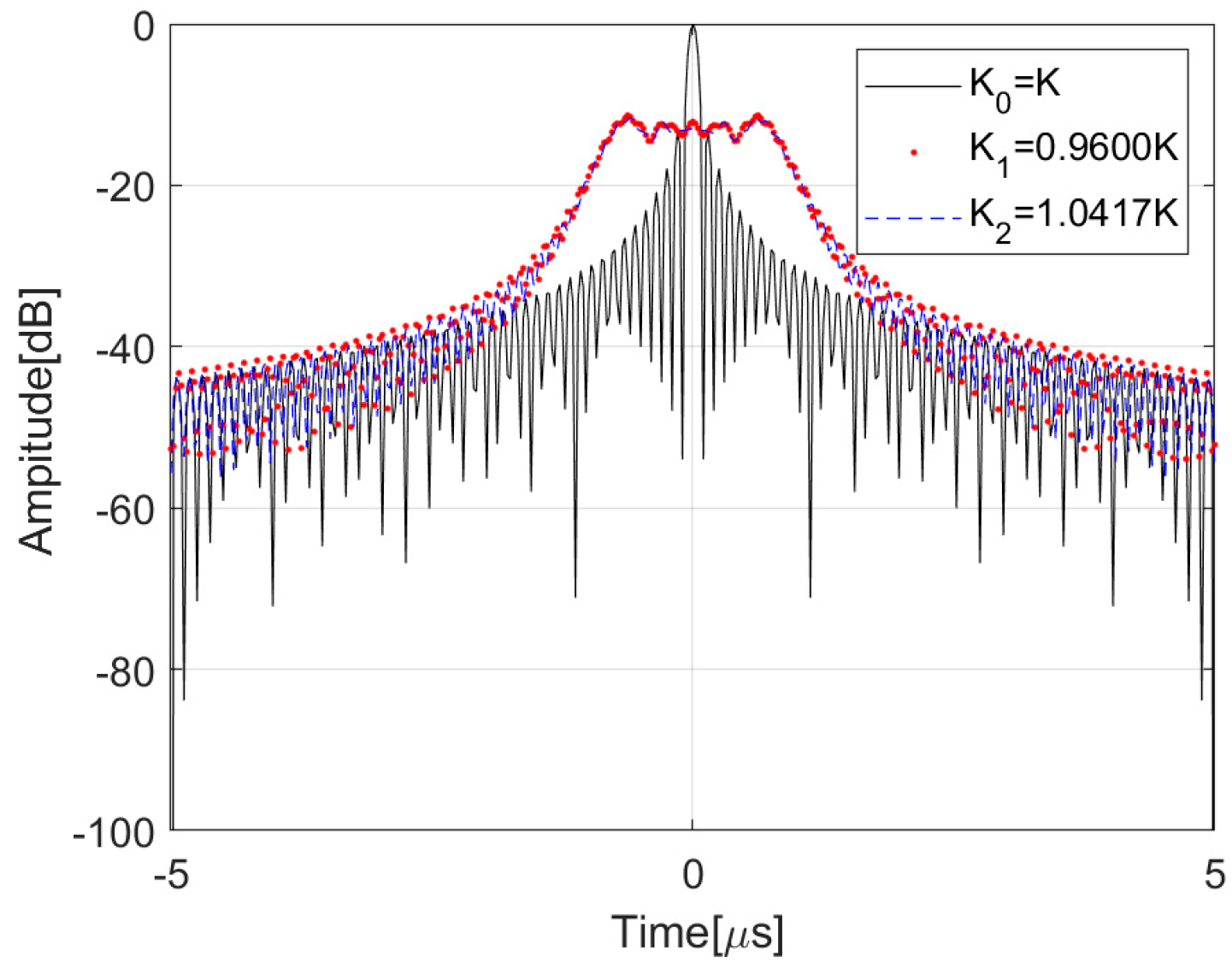
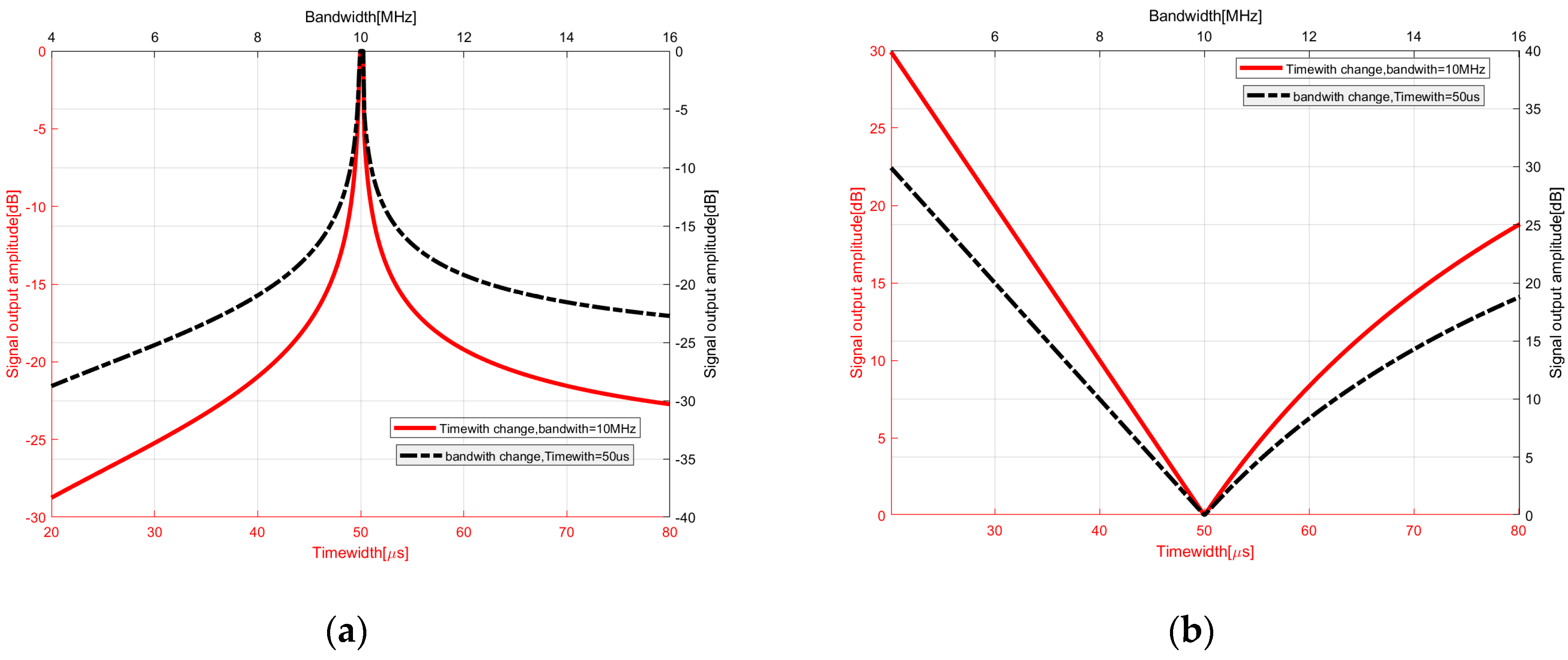
5.1. Design of Frequency Modulation Slope
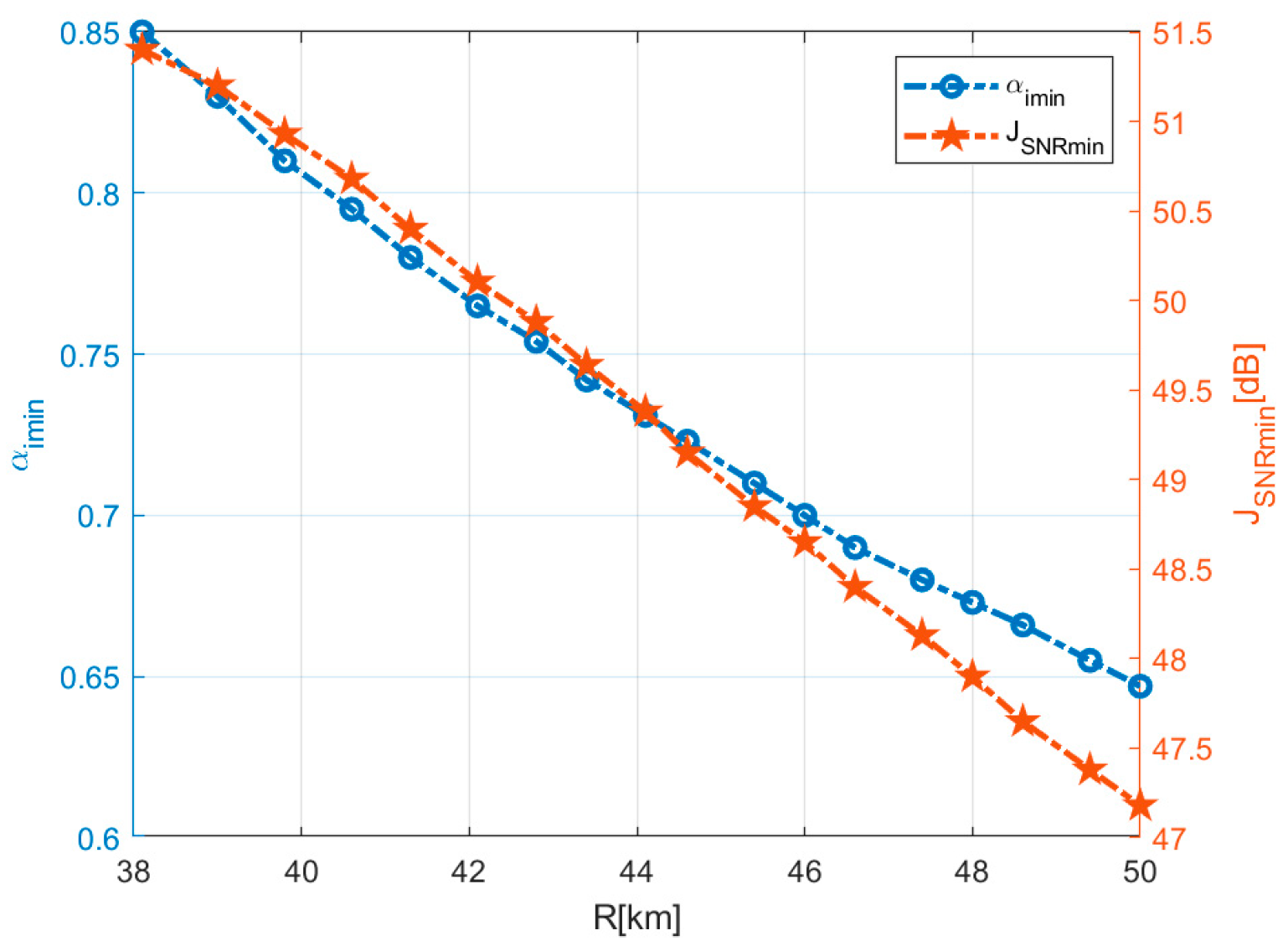
5.2. Minimum Detectable Signal-to-Noise Ratio and Matched Gain Ratio
5.3. Design of False Target Delay Interval
5.4. Design of the Number of False Targets
6. Simulation Experiments and Performance Analysis
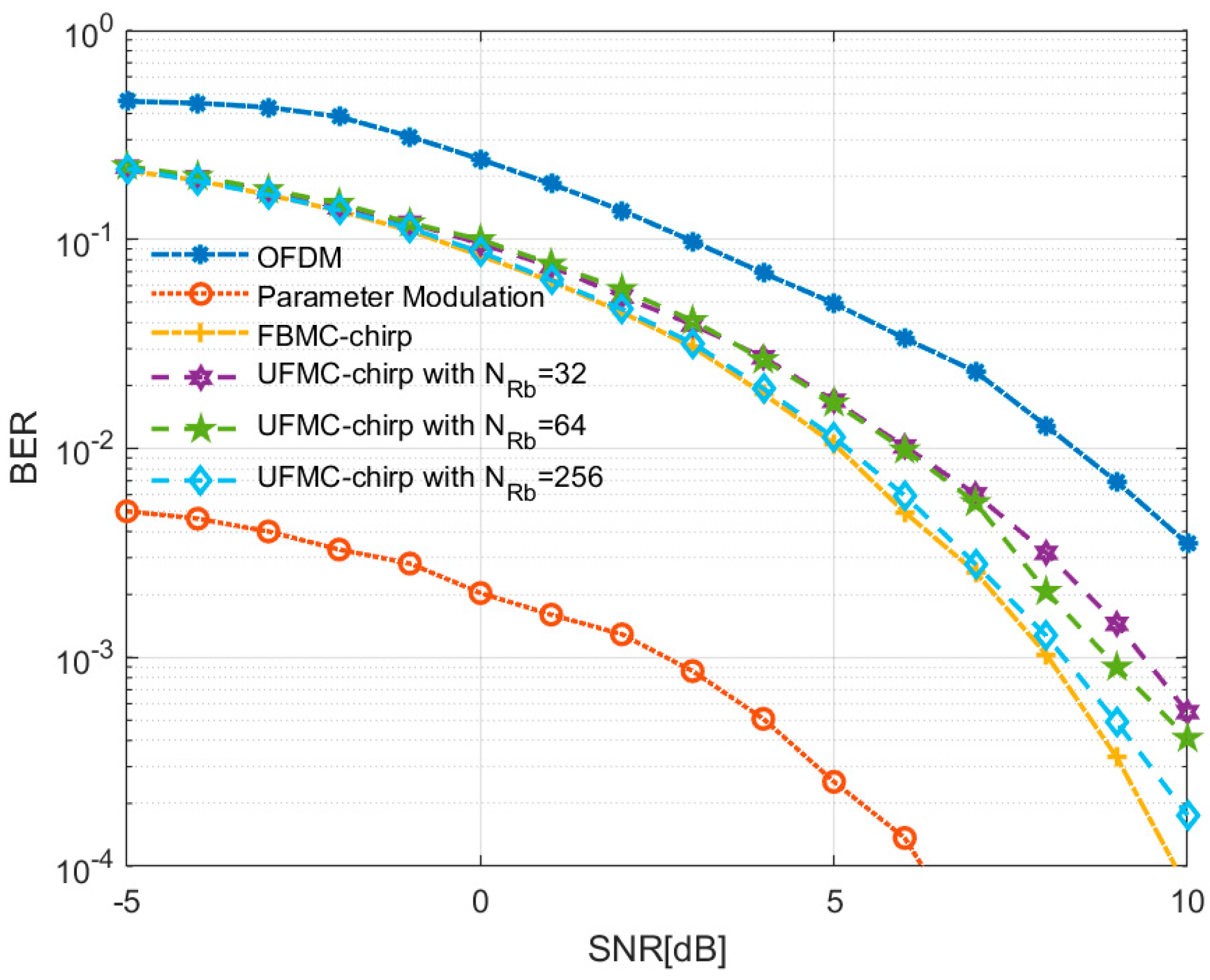
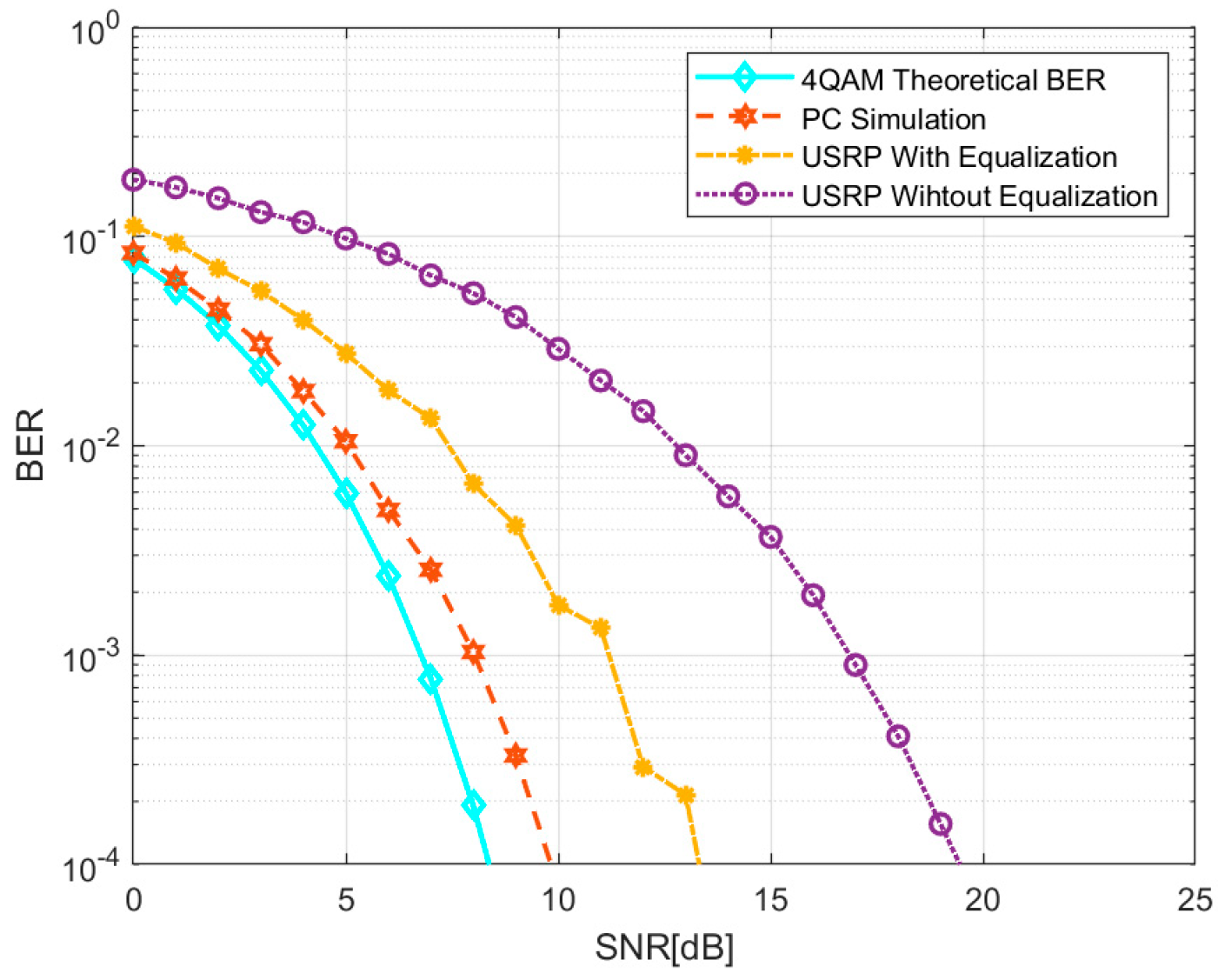
6.1. Communication Performance Analysis
6.2. Jamming Performance Analysis
6.3. The Trade-Off between Radar Jamming and Communication Performance
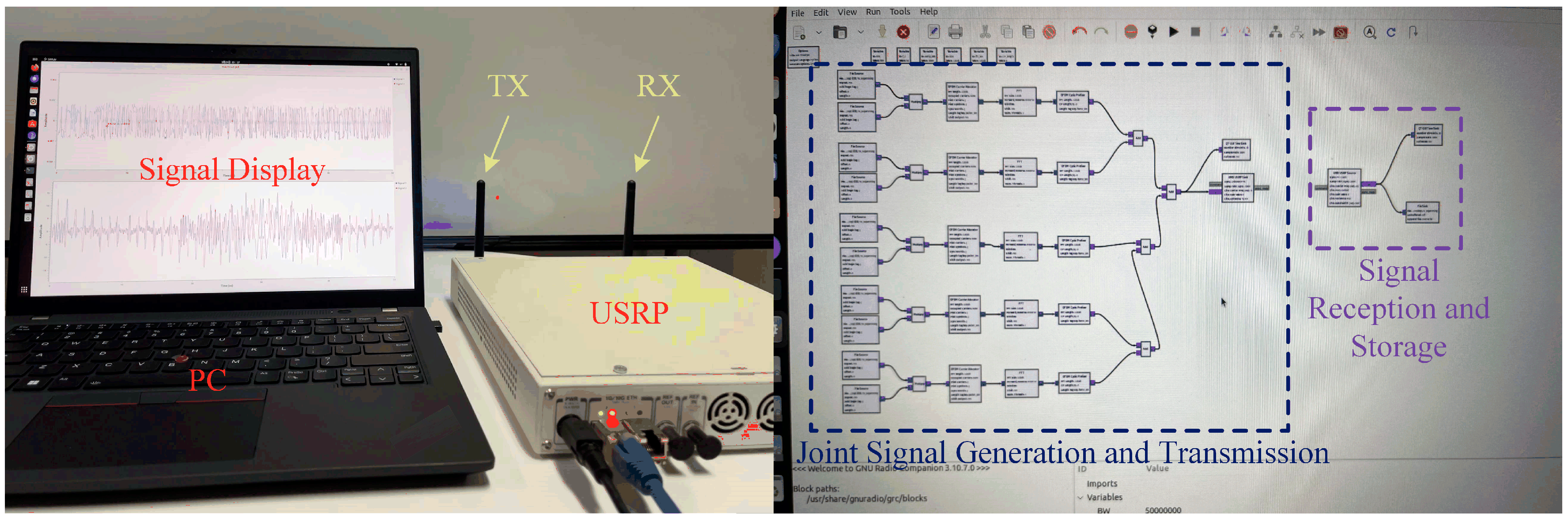
7. Hardware Implementation
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liu, L.; Liang, X.; Li, Y.; Liu, Y.; Bu, X.; Wang, M. A Spatial–Temporal Joint Radar-Communication Waveform Design Method with Low Sidelobe Level of Beampattern. Remote Sens. 2023, 15, 1167. [Google Scholar] [CrossRef]
- Sturm, C.; Wiesbeck, W. Waveform Design and Signal Processing Aspects for Fusion of Wireless Communications and Radar Sensing. Proc. IEEE 2011, 99, 1236–1259. [Google Scholar] [CrossRef]
- Mealey, R.M. A Method for Calculating Error Probabilities in a Radar Communication System. IEEE Trans. Space Electron. Telem. 1963, 9, 37–42. [Google Scholar] [CrossRef]
- Zhang, J.A.; Liu, F.; Masouros, C.; Heath, R.W.; Feng, Z.; Zheng, L.; Petropulu, A. An Overview of Signal Processing Techniques for Joint Communication and Radar Sensing. IEEE J. Sel. Top. Signal Process. 2021, 15, 1295–1315. [Google Scholar] [CrossRef]
- Han, L.; Wu, K. Joint Wireless Communication and Radar Sensing Systems—State of the Art and Future Prospects. IET Microw. Antennas Propag. 2013, 7, 876–885. [Google Scholar] [CrossRef]
- Alaee-Kerahroodi, M.; Raei, E.; Kumar, S.; Bhavani Shankar, M.R. Cognitive Radar Waveform Design and Prototype for Coexistence with Communications. IEEE Sens. J. 2022, 22, 9787–9802. [Google Scholar] [CrossRef]
- Sen, P.; Harutyunyan, A.; Umar, M.; Kamal, S. Joint Communication and Radar Sensing: RF Hardware Opportunities and Challenges—A Circuits and Systems Perspective. Sensors 2023, 23, 7673. [Google Scholar] [CrossRef] [PubMed]
- Cohen, D.; Mishra, K.V.; Eldar, Y.C. Spectrum Sharing Radar: Coexistence via Xampling. IEEE Trans. Aerosp. Electron. Syst. 2018, 54, 1279–1296. [Google Scholar] [CrossRef]
- Blunt, S.D.; Yatham, P.; Stiles, J. Intrapulse Radar-Embedded Communications. IEEE Trans. Aerosp. Electron. Syst. 2010, 46, 1185–1200. [Google Scholar] [CrossRef]
- Ahmed, A.; Zhang, Y.D.; Hassanien, A. Joint Radar-Communications Exploiting Optimized OFDM Waveforms. Remote Sens. 2021, 13, 4376. [Google Scholar] [CrossRef]
- Kumar, S.; Mishra, K.V.; Gautam, S.; Shankar, M.R.B.; Ottersten, B. Interference Mitigation Methods for Coexistence of Radar and Communication. In Proceedings of the 2021 15th European Conference on Antennas and Propagation (EuCAP), Dusseldorf, Germany, 22–26 March 2021; pp. 1–4. [Google Scholar]
- Deng, H.; Himed, B. Interference Mitigation Processing for Spectrum-Sharing Between Radar and Wireless Communications Systems. IEEE Trans. Aerosp. Electron. Syst. 2013, 49, 1911–1919. [Google Scholar] [CrossRef]
- Pappu, C.S.; Beal, A.N.; Flores, B.C. Chaos Based Frequency Modulation for Joint Monostatic and Bistatic Radar-Communication Systems. Remote Sens. 2021, 13, 4113. [Google Scholar] [CrossRef]
- Wang, J.; Liang, X.-D.; Chen, L.-Y.; Li, Y.-L. Waveform Designs for Joint Wireless Communication and Radar Sensing: Pitfalls and Opportunities. IEEE Internet Things J. 2023, 10, 15252–15265. [Google Scholar] [CrossRef]
- Wu, W.; Tang, B.; Wang, X. Constant-Modulus Waveform Design for Dual-Function Radar-Communication Systems in the Presence of Clutter. IEEE Trans. Aerosp. Electron. Syst. 2023, 59, 4005–4017. [Google Scholar] [CrossRef]
- Xu, Z.; Petropulu, A. A Bandwidth Efficient Dual-Function Radar Communication System Based on a MIMO Radar Using OFDM Waveforms. IEEE Trans. Signal Process. 2023, 71, 401–416. [Google Scholar] [CrossRef]
- Wen, C.; Huang, Y.; Davidson, T.N. Efficient Transceiver Design for MIMO Dual-Function Radar-Communication Systems. IEEE Trans. Signal Process. 2023, 71, 1786–1801. [Google Scholar] [CrossRef]
- Liu, X.; Huang, T.; Shlezinger, N.; Liu, Y.; Zhou, J.; Eldar, Y.C. Joint Transmit Beamforming for Multiuser MIMO Communications and MIMO Radar. IEEE Trans. Signal Process. 2020, 68, 3929–3944. [Google Scholar] [CrossRef]
- Chen, L.; Liu, F.; Wang, W.; Masouros, C. Joint Radar-Communication Transmission: A Generalized Pareto Optimization Framework. IEEE Trans. Signal Process. 2021, 69, 2752–2765. [Google Scholar] [CrossRef]
- Liu, F.; Masouros, C.; Li, A.; Sun, H.; Hanzo, L. MU-MIMO Communications With MIMO Radar: From Co-Existence to Joint Transmission. IEEE Trans. Wirel. Commun. 2018, 17, 2755–2770. [Google Scholar] [CrossRef]
- Dokhanchi, S.H.; Shankar, M.R.B.; Stifter, T.; Ottersten, B. OFDM-Based Automotive Joint Radar-Communication System. In Proceedings of the 2018 IEEE Radar Conference (RadarConf18), Oklahoma City, OK, USA, 23–27 April 2018; pp. 0902–0907. [Google Scholar]
- Liu, Y.; Liao, G.; Xu, J.; Yang, Z.; Zhang, Y. Adaptive OFDM Integrated Radar and Communications Waveform Design Based on Information Theory. IEEE Commun. Lett. 2017, 21, 2174–2177. [Google Scholar] [CrossRef]
- Keskin, M.F.; Wymeersch, H.; Koivunen, V. MIMO-OFDM Joint Radar-Communications: Is ICI Friend or Foe? IEEE J. Sel. Top. Signal Process. 2021, 15, 1393–1408. [Google Scholar] [CrossRef]
- Wen, C.; Huang, Y.; Zheng, L.; Liu, W.; Davidson, T.N. Transmit Waveform Design for Dual-Function Radar-Communication Systems via Hybrid Linear-Nonlinear Precoding. IEEE Trans. Signal Process. 2023, 71, 2130–2145. [Google Scholar] [CrossRef]
- Wang, Y.; Jin, G.; Song, C.; Wang, Y.; Lu, P.; Han, S.; Lv, J.; Zhang, Y.; Wu, D.; Zhu, D. Parameterized and Large-Dynamic-Range 2-D Precise Controllable SAR Jamming: Characterization, Modeling, and Analysis. IEEE Trans. Geosci. Remote Sens. 2023, 61, 3279461. [Google Scholar] [CrossRef]
- Goj, W.W. Synthetic-Aperture Radar and Electronic Warfare; Artech House: Boston, MA, USA, 1993. [Google Scholar]
- Neng-Jing, L.; Yi-Ting, Z. A Survey of Radar ECM and ECCM. IEEE Trans. Aerosp. Electron. Syst. 1995, 31, 1110–1120. [Google Scholar] [CrossRef]
- Feng, D.; Xu, L.; Pan, X.; Wang, X. Jamming Wideband Radar Using Interrupted-Sampling Repeater. IEEE Trans. Aerosp. Electron. Syst. 2017, 53, 1341–1354. [Google Scholar] [CrossRef]
- Dumper, K.; Cooper, P.S.; Wons, A.F.; Condley, C.J.; Tully, P. Spaceborne Synthetic Aperture Radar and Noise Jamming. In Proceedings of the Radar 97 (Conf. Publ. No. 449), Edinburgh, UK, 14–16 October 1997; pp. 411–414. [Google Scholar]
- Ye, W.; Ruan, H.; Zhang, S.; Yan, L. Study of Noise Jamming Based on Convolution Modulation to SAR. In Proceedings of the 2010 International Conference on Computer, Mechatronics, Control and Electronic Engineering, Changchun, China, 24–26 August 2010; Volume 6, pp. 169–172. [Google Scholar]
- Garmatyuk, D.S.; Narayanan, R.M. ECCM Capabilities of an Ultrawideband Bandlimited Random Noise Imaging Radar. IEEE Trans. Aerosp. Electron. Syst. 2002, 38, 1243–1255. [Google Scholar] [CrossRef]
- Schuerger, J.; Garmatyuk, D. Deception Jamming Modeling in Radar Sensor Networks. In Proceedings of the MILCOM 2008—2008 IEEE Military Communications Conference, San Diego, CA, USA, 16–19 November 2008; pp. 1–7. [Google Scholar]
- Zhou, F.; Zhao, B.; Tao, M.; Bai, X.; Chen, B.; Sun, G. A Large Scene Deceptive Jamming Method for Space-Borne SAR. IEEE Trans. Geosci. Remote Sens. 2013, 51, 4486–4495. [Google Scholar] [CrossRef]
- Sun, Q.; Shu, T.; Yu, K.-B.; Yu, W. Efficient Deceptive Jamming Method of Static and Moving Targets Against SAR. IEEE Sens. J. 2018, 18, 3610–3618. [Google Scholar] [CrossRef]
- Yang, K.; Ye, W.; Ma, F.; Li, G.; Tong, Q. A Large-Scene Deceptive Jamming Method for Space-Borne SAR Based on Time-Delay and Frequency-Shift with Template Segmentation. Remote Sens. 2020, 12, 53. [Google Scholar] [CrossRef]
- Wang, Y.; Jin, G.; Shi, T.; Ma, R.; Zhang, Y.; Wu, D.; Zhu, D. Knowledge-Aided Precise Jamming Technique Against Spaceborne SAR System. IEEE Trans. Aerosp. Electron. Syst. 2024, 1–15. [Google Scholar] [CrossRef]
- Liu, G.; Yang, W.; Wang, Y.; Wang, Z.; Huang, D.; Li, P.; Bao, D.; Man, X.; Wu, B. Joint Communication Interference System Design Based on Parameter Modulation. Appl. Opt. 2022, 61, 1057. [Google Scholar] [CrossRef] [PubMed]
- Sharma, G.K.; Arya, R.K.; Nigam, V.S. Performance Analysis of UFMC for 5G Technologies with Different Channel Coding Techniques. In Proceedings of the 2023 International Conference on Signal Processing, Computation, Electronics, Power and Telecommunication (IConSCEPT), Karaikal, India, 25–26 May 2023; pp. 1–6. [Google Scholar]
- Heil, M.M.; Hammoodi, A.T.; Rasool, J.M. Multi-Windowing Technique for 5G and Beyond. J. Commun. 2023, 18, 714–721. [Google Scholar] [CrossRef]
- Liu, G.; Yang, W.; Bao, Y.; Wang, Y.; Li, P. Joint Communication and Jamming System Design Based on Filter Bank Multicarrier Chirp Waveform: Using for Curvilinear Flight Scenario. Remote Sens. 2023, 15, 1239. [Google Scholar] [CrossRef]
- Khelouani, I.; Elbahhar, F.; Elassali, R.; Idboufker, N. Performance Evaluation of 5G Waveforms for Joint Radar Communication over 77 GHz and 24 GHz ISM Bands. Energies 2022, 15, 2049. [Google Scholar] [CrossRef]
- Kim, J.H.; Younis, M.; Moreira, A.; Wiesbeck, W. A Novel OFDM Chirp Waveform Scheme for Use of Multiple Transmitters in SAR. IEEE Geosci. Remote Sens. Lett. 2013, 10, 568–572. [Google Scholar] [CrossRef]


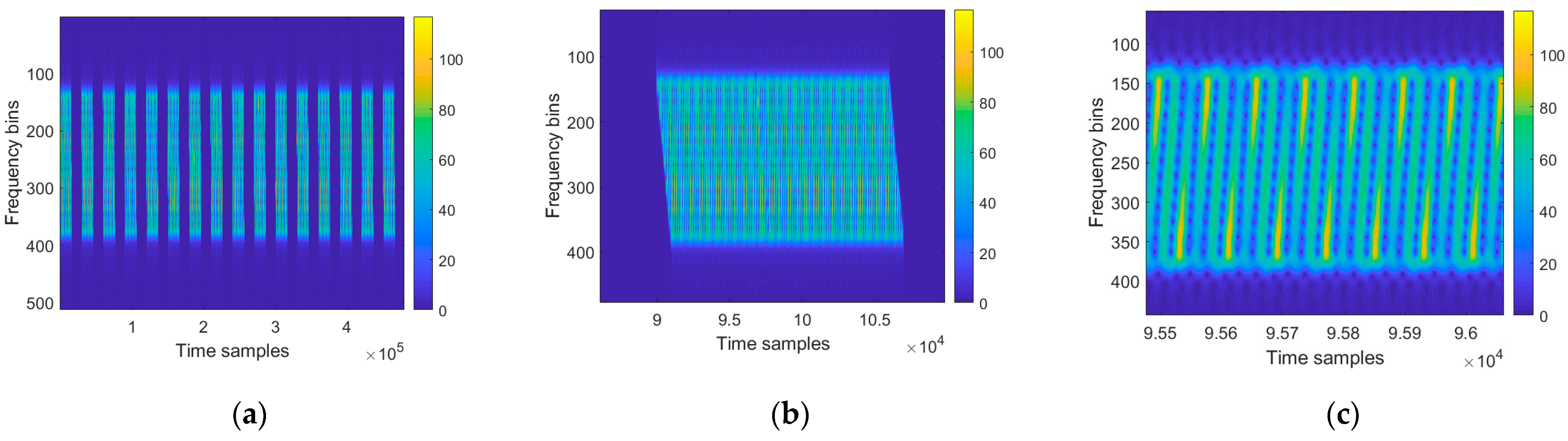
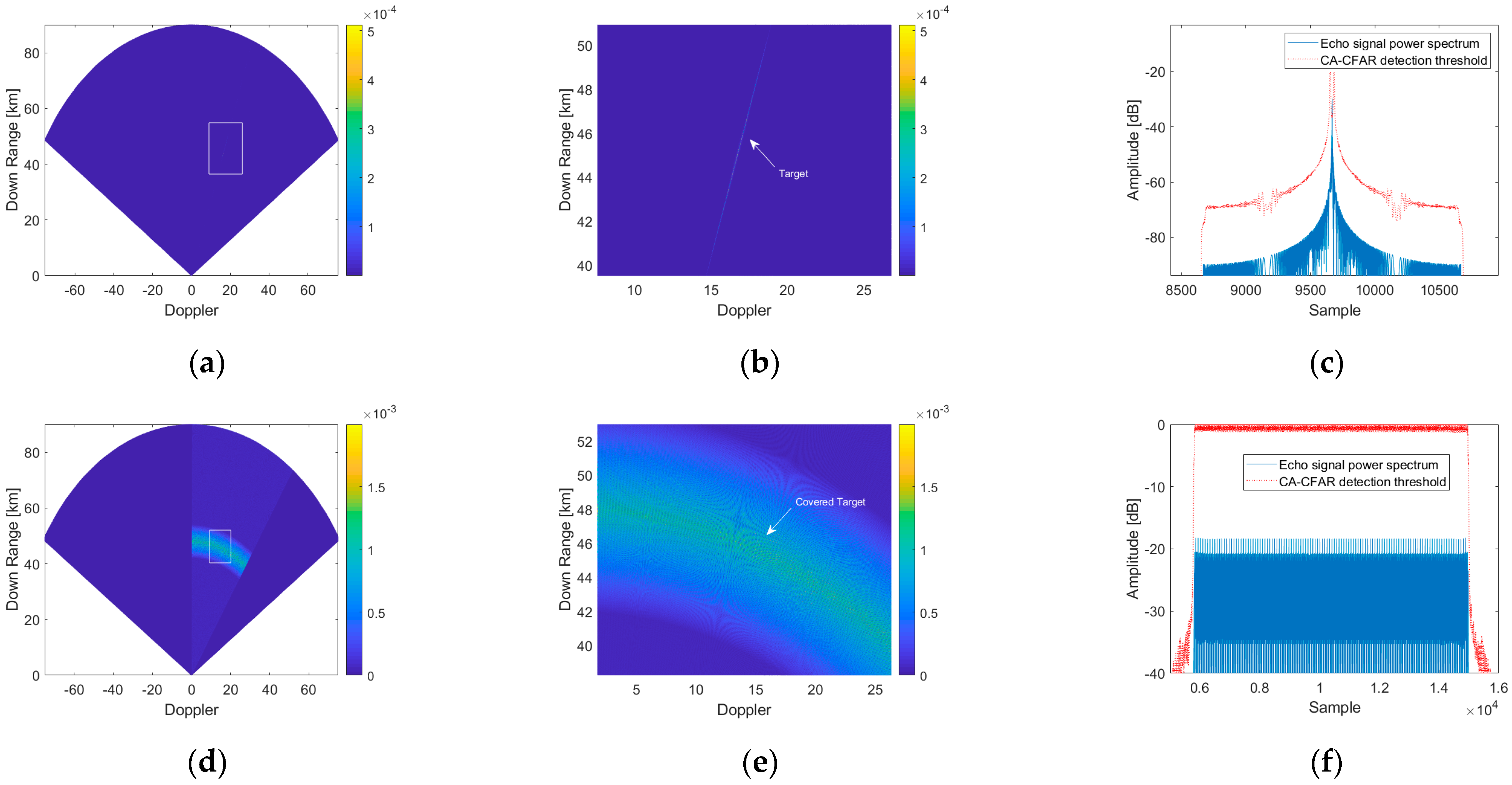
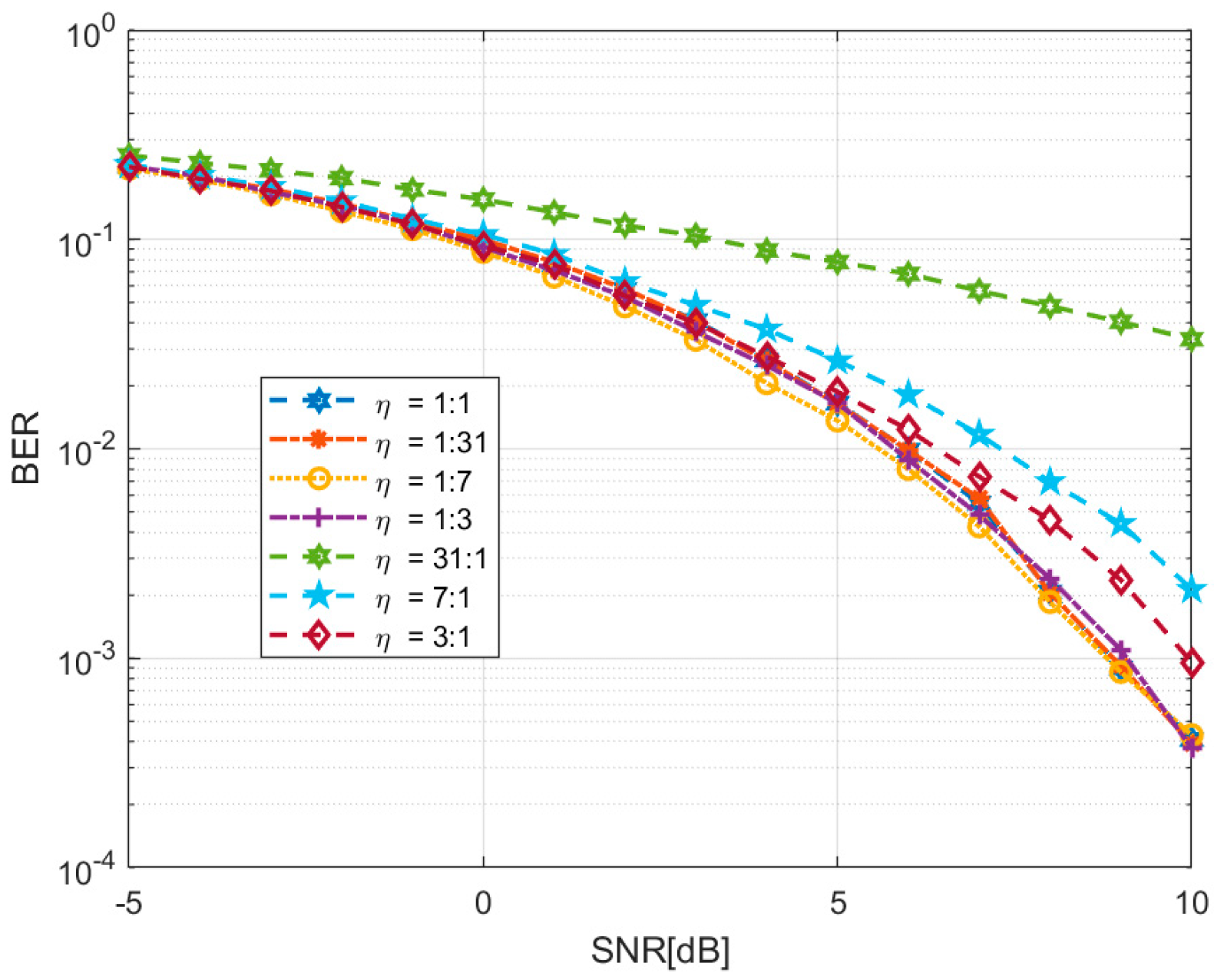
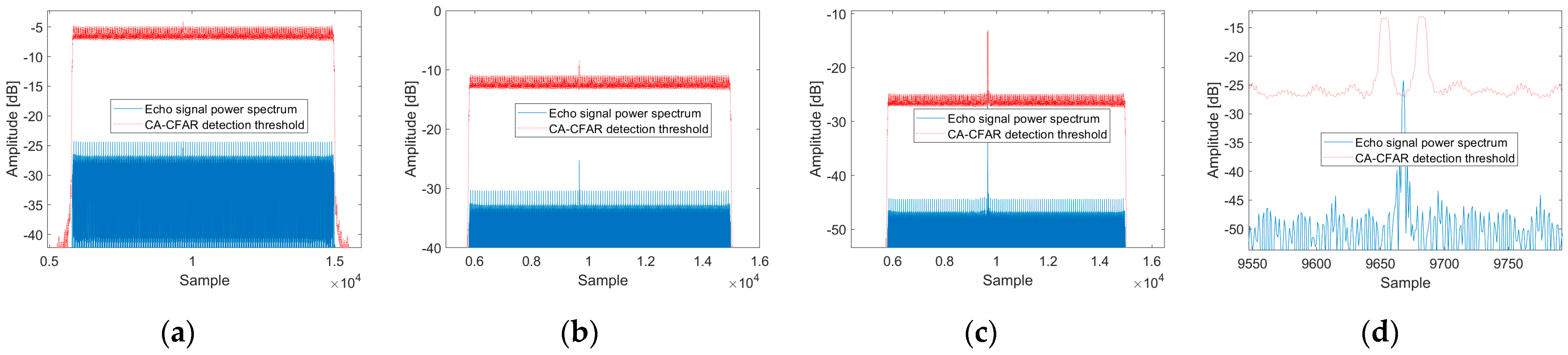

| Parameter | Value |
|---|---|
| Modulation mode | 4QAM |
| Sub-band number | 32, 64, 256 |
| Number of multipath channels | 5 |
| Parameter | Value |
|---|---|
| PRT | 1.5 ms |
| Bandwidth | 10 MHz |
| Number of pulses | 16 |
| Pulse width | 50 us |
| Carrier frequency | 4 GHz |
| Number of symbols | 100 |
| Number of false targets | 600 |
| Modulation mode | 4QAM |
| SNR | 10 dB |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, G.; Huang, Z.; Zhang, Q.; Mu, B.; Guo, H. Joint Radar Jamming and Communication System Design Based on Universal Filtered Multicarrier Chirp Waveform. Remote Sens. 2024, 16, 1383. https://doi.org/10.3390/rs16081383
Liu G, Huang Z, Zhang Q, Mu B, Guo H. Joint Radar Jamming and Communication System Design Based on Universal Filtered Multicarrier Chirp Waveform. Remote Sensing. 2024; 16(8):1383. https://doi.org/10.3390/rs16081383
Chicago/Turabian StyleLiu, Gaogao, Ziyu Huang, Qidong Zhang, Beibei Mu, and Hongfu Guo. 2024. "Joint Radar Jamming and Communication System Design Based on Universal Filtered Multicarrier Chirp Waveform" Remote Sensing 16, no. 8: 1383. https://doi.org/10.3390/rs16081383
APA StyleLiu, G., Huang, Z., Zhang, Q., Mu, B., & Guo, H. (2024). Joint Radar Jamming and Communication System Design Based on Universal Filtered Multicarrier Chirp Waveform. Remote Sensing, 16(8), 1383. https://doi.org/10.3390/rs16081383







