Thermal Deformation Analysis of a Star Camera to Ensure Its High Attitude Measurement Accuracy in Orbit

Abstract

With the continuous advancement of high-resolution satellite technology, the impact of thermal deformation on the performance of star cameras is becoming more significant, particularly in relation to installation conditions and orbital environments. To address this challenge, an in-depth investigation of the thermal design of a star camera is conducted in this study. The thermal deformation of this camera is evaluated systematically through simulation analysis, thermal balance tests, and on-orbit temperature measurements. In addition, a simulation analysis is used to identify and quantitatively evaluate the thermal deformation error sources that affect the spatial attitude measurement accuracy of the star camera. The results indicate that thermal deformations of the optical system, the mounting surface of the star camera, and the support significantly impact on-orbit measurement accuracy. Ultimately, the limit error attributable to on-orbit thermal deformation is determined to be 0.62″. In the thermal balance experiments, the maximum absolute difference between the test results and the thermal simulation analysis results is under 1.8 °C. Additionally, analysis of the orbital temperature data reveals that the maximum absolute difference between the orbital results and the thermal simulation results is 1.18 °C, while the attitude accuracy of the star sensor is better than 0.54″. These findings validate the effectiveness of the thermal design and the accuracy of the thermal simulation analysis. The analysis of error sources presented in this paper offers crucial insights for effectively controlling the thermal deformation errors of star cameras and lays the groundwork for optimizing overall thermal design.

1. Introduction

As attitude sensors, star cameras have the advantages of high accuracy, light mass, low power consumption, no drift, and multiple operating modes [1,2]. Due to their small field of view and long focal length, these cameras have a higher measurement accuracy than other types of star sensors. They are widely used in aerospace technology fields such as Earth remote sensing, Earth measurement, planetary exploration, planetary mapping, interstellar communication, and intercontinental missiles [3,4,5]. In view of the high precision requirements, they are very sensitive to temperature changes [6]. The external heat flux of the camera, particularly due to periodic variations in solar radiation, can lead to temperature fluctuations that ultimately affect the measurement accuracy of the optical system [7].

These fluctuations can cause the expansion or contraction of various components within the optical system, resulting in changes in focal length and principal point position [8,9]. This not only affects the imaging quality of the optical system but also impacts the overall structural stability [10,11]. Through the combination of finite element analysis and optical tracking methods, Liu Haibo and Tan Wei et al. [12,13] determined the relationship between the temperature distribution of the star camera and the attitude measurement error, which provided theoretical support for the thermal design of a star camera optical system. Hu Xiongchao et al. [14] discussed and studied the influence of temperature on the lens distortion of star cameras, and they proposed a method to measure the effect of temperature on the measurement error of star points, in addition to a method to compensate for this effect. Podbreznik et al. [15] found that when the camera temperature increases, the images and parameters used in the imaging model change, and a model to eliminate the measurement error caused by certain temperature conditions was thus proposed. Jiang Guangwen [16] studied the temperature effect and a method to eliminate this effect in camera measurements by combining semi-physical simulations and finite element analysis. In summary, the influence of the thermal deformation of star cameras cannot be ignored. However, current research mainly focuses on the effect of the deformation of star sensors’ optical systems on imaging. Therefore, to ensure the high precision of star sensors, it is necessary to carry out comprehensive on-orbit thermal stability and thermal control analyses. These analyses should not only consider the components of the optical system but should also consider the installation, actual operations in the on-orbit environment, and the interaction with other devices. Guan Zhichao [17,18] and Tang Yujie [19] also mentioned that the precise installation relationship between the star camera and the star sensor is a key factor in the geometric positioning accuracy of optical remote sensing satellites.

In this study, thermal design and thermal deformation analyses are performed for a star camera that is about to be launched on a satellite platform. The attitude accuracy requirement for the camera is less than 1″. Through simulation analysis and experimental research, the thermal deformation factors affecting the on-orbit attitude measurement error of the star camera are analyzed. The main error sources during installation of the camera are identified and quantitatively analyzed. In addition, the thermal balance test and on-orbit temperature measurement are completed and compared with the thermal simulation analysis results, aiming to guide the thermal stability analysis of the star camera.

2. Structure of the Star Camera

2.1. The Overall Structure of the Star Camera

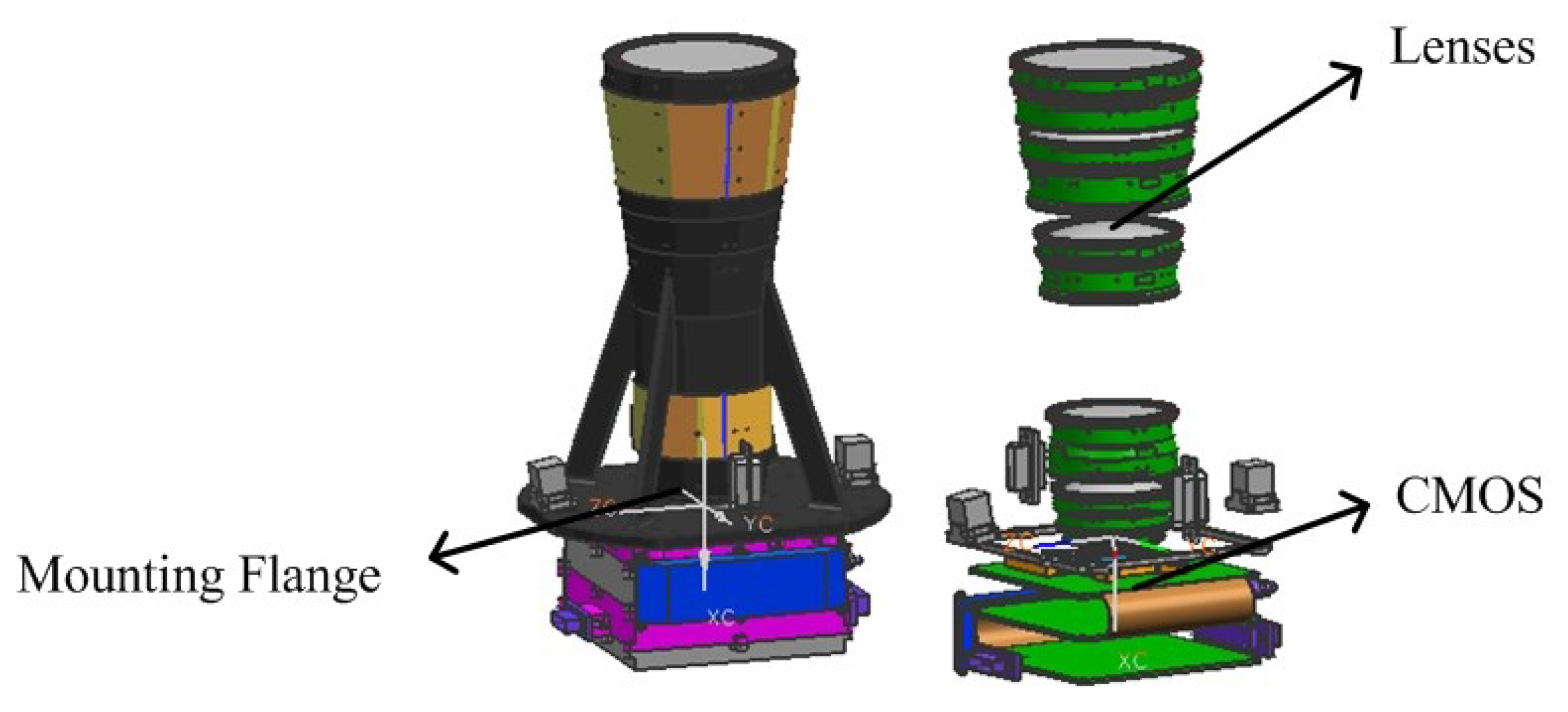
The star camera consists of a baffle, a lens tube, a mounting flange, CMOS components, and an electronics assembly. The lens tube and the mounting flange have integrated casting. The lens group and the electronics assembly are located in the upper and lower parts of the mounting flange, respectively. The main structure of the star camera without the baffle is shown in Figure 1.

2.2. Optical System of the Star Camera

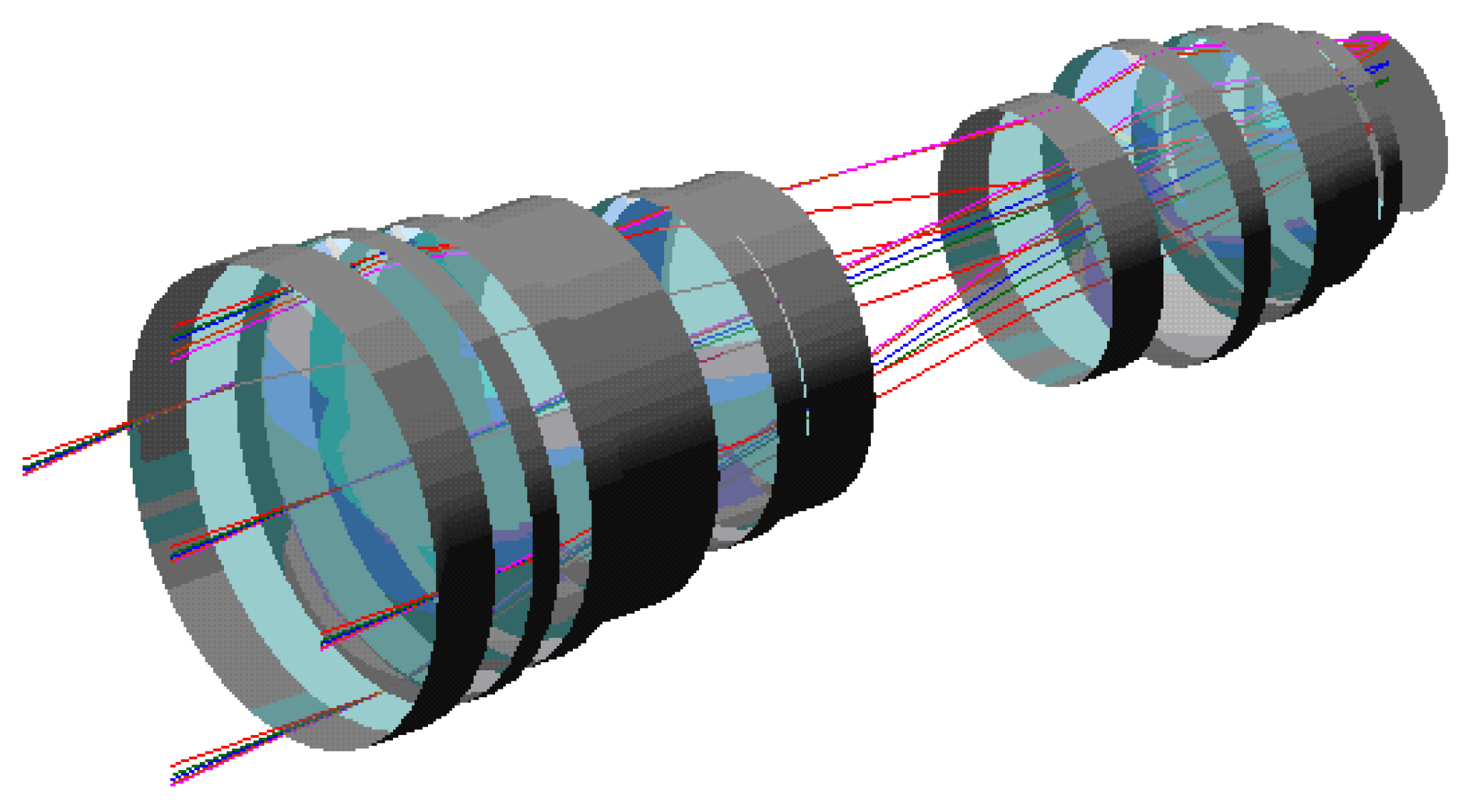
The optical system of the camera is shown in Figure 2. It is a refractive optical system composed of 10 lenses, including window glass and filters. The aperture is 78 mm and the focal length is 170 mm. Star cameras usually feature a telecentric optical path design to effectively correct distortion. This design can greatly reduce the influence of aberrations on system performance.

2.3. Installation of the Star Camera on the Satellite

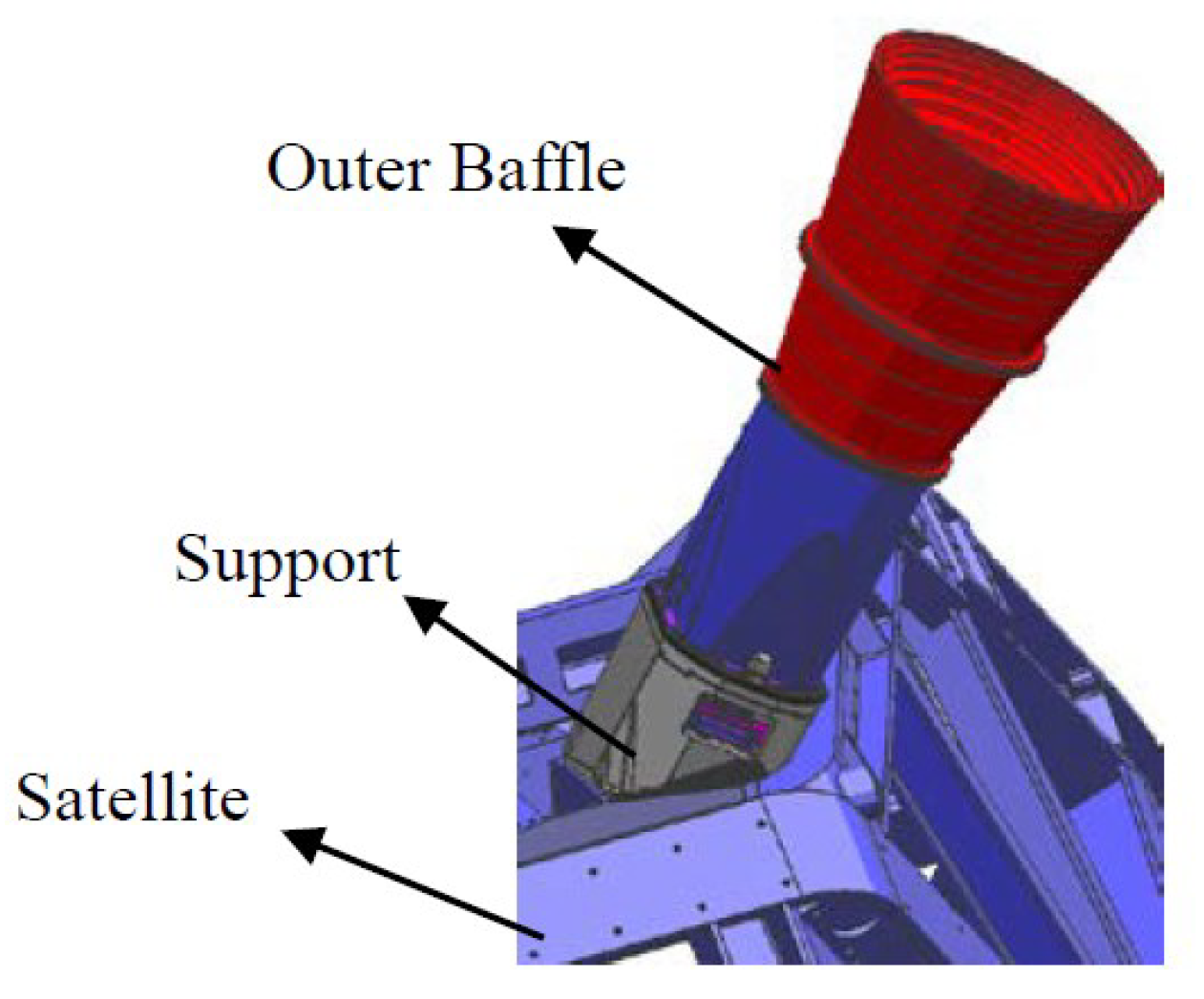
A support is designed to adjust the line of sight of the camera to avoid it being directly affected by solar radiation. According to Figure 3, the camera tilts from the front of the satellite to the shadow side through the support.

3. Measurement Errors Caused by Thermal Deformations

Studies have shown that when the root mean square wave difference (RMS) overall error is allocated, the thermal deformation error accounts for about half of the total error in numerical terms [20]. Temperature has the following effects on the star camera: First, a change in temperature field causes the thermal deformation of its optical system, mainly the eccentricity, surface inclination, change in surface shape, and change in spherical radius of each lens. These effects can be quantitatively assessed by evaluating the changes in the principal point and the focal length of the optical system. Secondly, temperature changes can lead to thermal deformation of the mounting surface, including deformations in the star camera flange and the reference mounting surface. Temperature changes cause expansion or contraction of the mounting structure, which in turn changes the geometry and may lead to optical axis deviation and coordinate system misalignment.

Thermal deformation of an optical system in a star camera can be analyzed by tracking the light as it passes through the lens group. Assessing changes in focal length across different fields of view and variations in light height allows us to determine the angle change, which represents the thermal deformation error, and the thermal deformation of the mounting surface of the star camera can be combined with thermal and structural analyses. First, the temperature distribution under various conditions is obtained through thermal analysis. Subsequently, the structural analysis provides the changes in the spatial coordinates of nodes on each mounting surface based on these temperature distributions. The least-squares method is employed to fit the plane equation of the deformed installation surface. Finally, the normal angle of the installation surface is determined, representing the thermal deformation error.

It is necessary to conduct sufficient thermal design and thermal simulation analysis for the star camera to predict the on-orbit temperature and reduce the influence of temperature on the camera’s measurement accuracy.

4. Thermal Deformation Simulation Analysis of the Star Camera

4.1. Simulation Model

To analyze the thermal deformation of the star camera, a finite element model is created, as shown in Figure 4. The structural analysis is performed using MSC NASTRAN2012, while THERMAL DESKTOP 5.3 is utilized for thermal analysis.

According to the orbit parameters and the thermal-physics parameters of multi-layer insulations (MLIs) coated on the outer side of the star camera, the worst cases are set and calculated by the software. The worst case with regard to heat is maximum external heat flow in orbit.

The boundary conditions of the worst heat case in the simulation analysis of the software are defined as follows. When the sun’s orbit angle β is at a maximum, the solar constant is 1412 W/m², the Earth’s albedo coefficient is 0.35, and the Earth’s infrared radiation is 237 W/m². The solar absorptance and the infrared emissivity of the external surfaces of MLIs are ${\alpha }_{\mathrm{S}}/{\epsilon }_{\mathrm{H}}=0.5/0.62$ (the material properties at the end of life are taken). The heat generated by the star camera during on-orbit operation is 4.5 W. The mounting plate temperature of the star camera support is 21 °C. The material parameters used in the thermal simulation analysis are shown in

Table 1. Material parameters used in the thermal simulation analysis.

Component	Material	Specific Heat Capacity (J/kg/°C)	Thermal Conductivity (W/m/k)	Solar Absorptivity	Infrared Emissivity
Spacer ring, pressure ring, top wire, lens tube	Cast titanium alloy ZTC4	557	8.80	0.85	0.85
Baffle, support cylinder	Aluminum alloy 2A12	921	121.00	0.85	0.85
Lens 1	Fused quartz	830	1.38	0.02	0.80
Lens 2–10	Optical glass	830	0.85	0.02	0.80
Star camera mount	Titanium alloy	557	6.80	0.85	0.85

.

4.2. Thermal Analysis of the Optical System

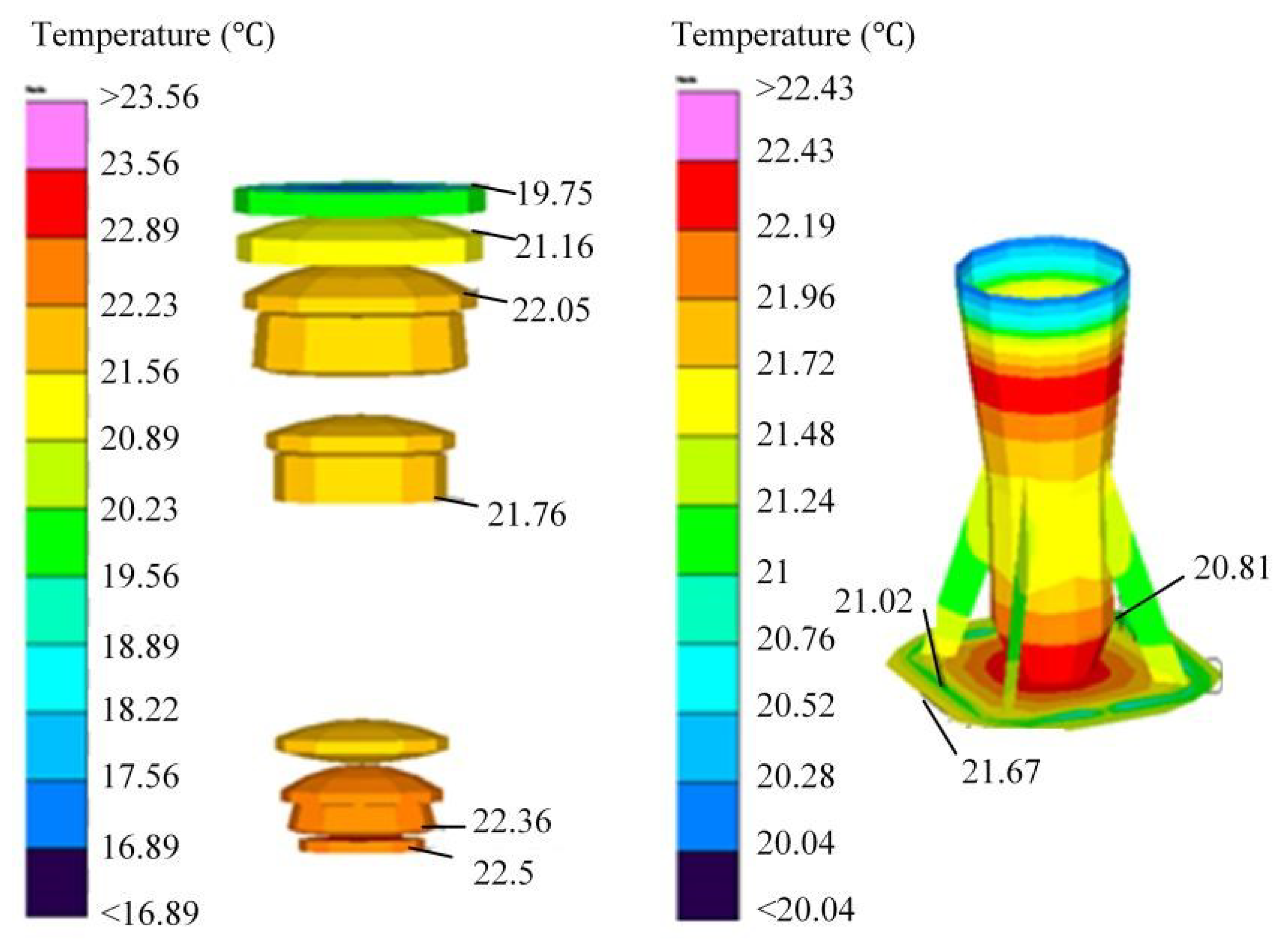
Thermal simulation of the optical system is carried out for the worst heat case. The temperature of the lens group and the lens tube in this case is shown in Figure 5. It can be seen that the temperature range of the lens group is 19.75~22.50 °C. The temperature gradient of the lens tube is 2.39 °C, as shown on the right side of Figure 5. This result shows that the temperature difference in the lens group mainly occurs axially, and that of the single lens mainly occurs radially.

The temperature data of the lens group are imported into the structural model for thermal deformation analysis to obtain lens surface inclinations, surface shape changes, and radius changes of each lens.

The result in the worst heat case is shown in

Table 2. Parameter varieties of the lens in the worst heat case.

Surface	Obliquity (″)		Shape Error (nm)		Curvature Radius (mm)
	${\mathit{\theta }}_{\mathbf{x}}$	${\mathit{\theta }}_{\mathbf{y}}$	PV	RMS	Original	After Deformation
1	0.7	−0.8	2.2	0.4	∞	∞
2	0.7	−0.8	2.4	0.6	∞	∞
3	0.7	−0.8	1.3	0.3	111.48	111.48
4	0.7	−0.8	1.0	0.3	348.2976	348.2975
5	0.7	−0.8	1.2	0.3	73.27994	73.27992
6	0.7	−0.8	1.1	0.3	209.3989	209.3989
7	0.7	−0.8	1.1	0.3	170.0006	170.0005
8	0.7	−0.8	1.4	0.3	46.64595	46.64594
9	0.7	−0.8	1.1	0.3	83.18591	83.1859
10	0.7	−0.8	2.7	0.7	95.95015	95.95071
11	0.7	−0.9	1.3	0.3	875.0009	875.1084
12	0.7	−0.8	21.3	5.7	60.00001	60.00110
13	0.7	−0.9	9.7	1.6	70.79996	70.80127
14	0.7	−0.9	11.1	1.8	92.49995	92.50045
15	0.7	−1.0	37.2	7.5	46.41500	46.41777
16	0.7	−0.7	34.4	7.1	132.6200	132.6435
17	0.7	−1.0	67.0	1.7	863.9994	865.0906
18	1.0	−1.1	71.4	1.8	40.64095	40.63950
19	0.7	−0.8	127.7	38.5	∞	∞
20	0.7	−0.8	127.5	38.6	∞	∞

. Each lens has an upper and lower surface. These surfaces on the first lens are named 1 and 2, those of the second lens are named 3 and 4, and so on, respectively. In Table 2,${\theta }_{\mathrm{x}}$ and ${\theta }_{\mathrm{y}}$ represent the title angle of the lens surface in two directions perpendicular to the optical axis. The surface shape errors are represented by the peak-to-valley (PV) and root mean square (RMS) values of the wave front error and adjustment error after removal, respectively. The last two columns correspond to the curvature radius changes before and after the calculation. The changes in ${\theta }_{\mathrm{x}}$, ${\theta }_{\mathrm{y}}$, PV, and RMS indicate how thermal deformation impacts the lens, including the extent of surface tilt and deformation. These factors can significantly influence the curvature radius of the optical system, which ultimately affects its focusing and imaging abilities.

Both the first and last lenses are planar, so there is no change in the radius of curvature. Through optical analysis, the focal length variation and the deviation in the chief ray in the full field of view can be obtained from the data in Table 2. The focal length of the optical system is 167.9827 mm, and this design is considered to have a relatively large depth of field, which helps to optimize the accuracy performance of the star camera. The height difference of the chief ray in the full field of view before and after thermal deformation was calculated and converted to the angle change (the attitude error), as shown in

Table 3. Chief ray deviation by thermal deformation.

Relative Field of View	Initial Value (mm)	Height of the Chief Ray (mm)	Transformation (mm)	$\mathbf{Angle} \mathbf{Change} {\mathsf{\theta }}_{\mathbf{o}\mathbf{p}\mathbf{t}\mathbf{i}\mathbf{c}\mathbf{a}\mathbf{l}}$ (″)
0	0	0	0	0
0.5	7.923246	7.923086	0.000160	0.196
1	15.581017	15.580824	0.000193	0.237

. The attitude error ${\theta }_{\mathrm{o}\mathrm{p}\mathrm{t}\mathrm{i}\mathrm{c}\mathrm{a}\mathrm{l}}$ of a single star caused by thermal deformation of the optical system does not exceed 0.24″ in the full field of view.

4.3. Thermal Analysis of the Mounting Surface

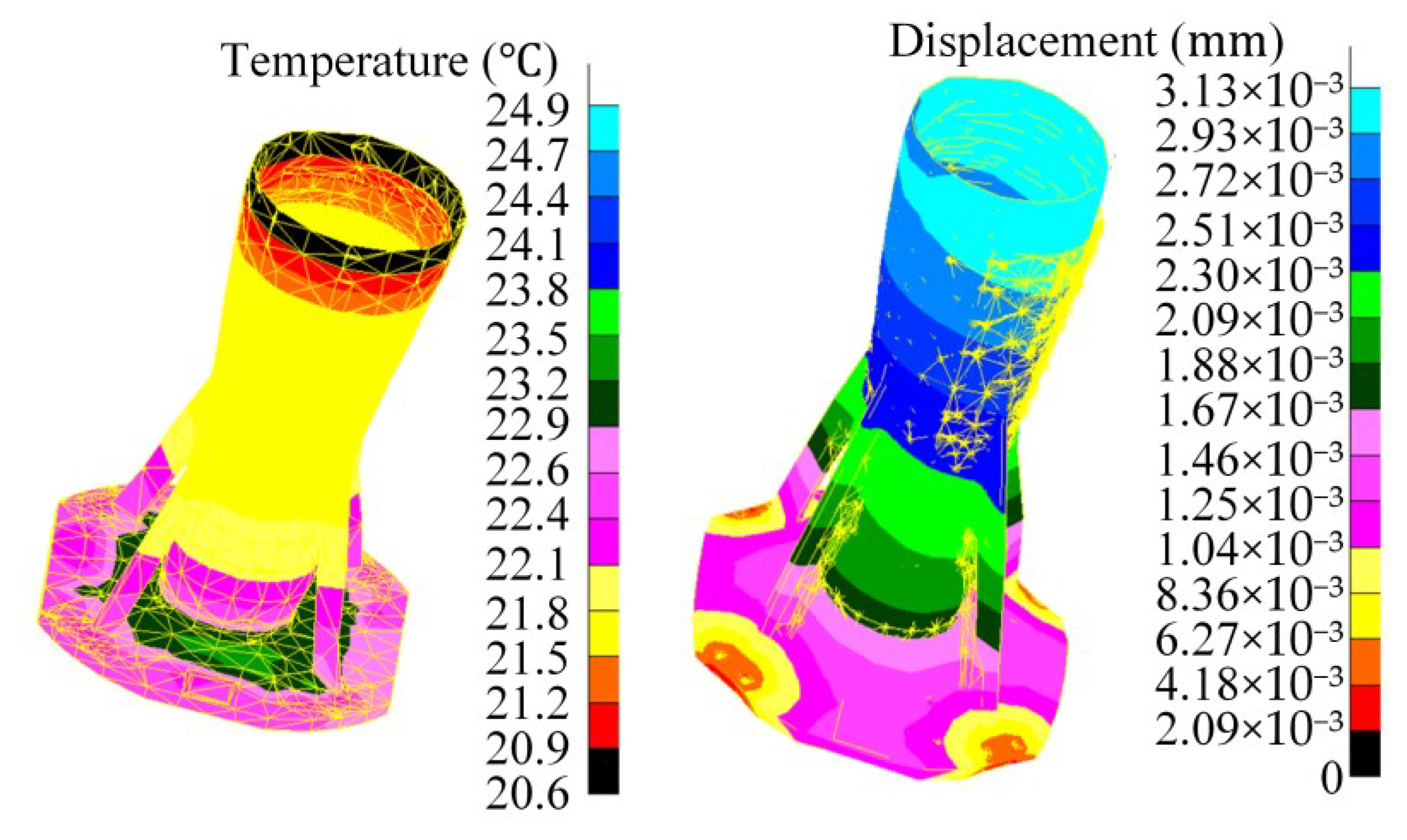
The variation in external heat flux and the degradation of MLI cause changes in the temperature of the star camera’s mounting surface, resulting in thermal stress and deformation. As shown in Figure 6, the temperature range of the mounting surface in the worst heat case is 22.10~23.20 °C, revealing a maximum temperature gradient of 2.0 °C on the mounting surface.

By mapping the temperature data onto the structure model, the thermal deformation can be calculated through structural analysis. The total displacements of all finite element nodes are recorded, as illustrated in Figure 6. The surface equation after deformation is fitted to determine the angle change relative to the original surface.

The thermal deformation data of the mounting surface of the star camera are counted. The spatial coordinate variations of the nodes on each installation surface under different temperature distributions are obtained through structural analysis. The deformed installation surface is then fitted using the least-squares method to derive the plane equation. Finally, the change in the normal angle of the installation surface is calculated. The angles between the normal direction of the mounting surface and the x and y axes of the coordinate system change by 0.25 and 0.18″, respectively. According to calculations, the change in the normal direction of the mounting surface ${\theta }_{\mathrm{m}\mathrm{o}\mathrm{u}\mathrm{n}\mathrm{t}\mathrm{i}\mathrm{n}\mathrm{g}}$ by thermal deformation is 0.31″.

4.4. Thermal Analysis of the Support

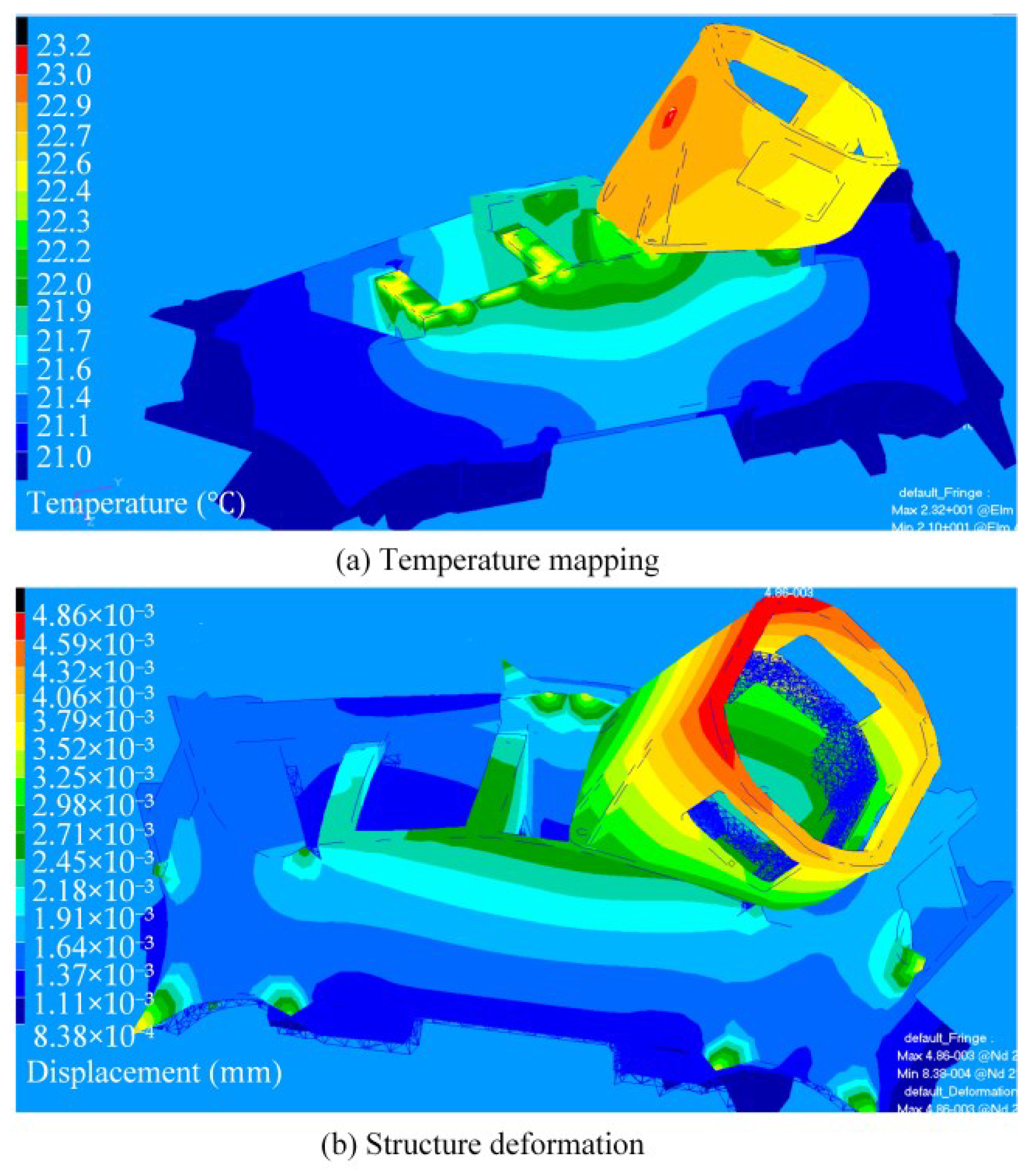
The thermal deformation analysis of the star camera support follows the same methodology as that used for the same analysis of the mounting surface. The temperature data are mapped onto the structural model to determine the displacement of each node, as illustrated in Figure 7. The initial temperature is 21 °C. The temperature range of the support is 22.40~23.26 °C. It can be observed that the maximum temperature gradient of the star camera support is 0.86 °C. Relative to the initial temperature, the maximum temperature rises by 2.26 °C.

The angles between the normal direction of the mounting surface and the x- and y-axes of the coordinate system change by 0.25″ and 0.19″, respectively. The angle change ${\theta }_{\mathrm{s}\mathrm{u}\mathrm{p}\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}}$ can be calculated by rotating the coordinate system, which is 0.31″.

4.5. Results Analysis

4.5.1. Temperature Results Analysis

Thermal simulation analysis shows that the temperature gradient of the lens tube is 2.39 °C under extreme temperature conditions. The temperature range of the mounting surface in the worst heat case is 22.10~23.20 °C, revealing a maximum temperature gradient of 2.0 °C. The temperature of the lens tube meets the temperature requirement of 21 ± 3 °C. The temperature difference between the top and bottom of the lens tube does not exceed 3 °C, which verifies the thermal design.

4.5.2. Error Analysis

The three thermal deformation errors obtained from the simulation analysis are the maximum errors under the extreme temperature conditions in orbit, which belong to the limit errors. ${\theta }_{\mathrm{t}\mathrm{h}\mathrm{e}\mathrm{r}\mathrm{m}\mathrm{a}\mathrm{l}}=\sqrt{{\theta }_{\mathrm{o}\mathrm{p}\mathrm{t}\mathrm{i}\mathrm{c}\mathrm{a}\mathrm{l}}^2+{\theta }_{\mathrm{m}\mathrm{o}\mathrm{u}\mathrm{n}\mathrm{t}\mathrm{i}\mathrm{n}\mathrm{g}}^2+{\theta }_{\mathrm{s}\mathrm{u}\mathrm{p}\mathrm{p}\mathrm{o}\mathrm{r}\mathrm{t}}^2}$. According to error synthesis theory, the composite limit error of on-orbit thermal deformation is 0.50″, as determined by the square sum root method.

The star camera uses a quadratic 2000 × 2000 pixel CMOS as the focal plane. The size of a single pixel is 11 μm × 11 μm. The star centroid noise is 1/16 of a pixel, and there are 52 stars on average in the FOV. Thus, the noise-equivalent angle is 0.12″ (pitch or yaw). Combined with the thermal deformation error and the noise-equivalent angle, the limit error prediction value in orbit ${\theta }_{\mathrm{t}\mathrm{o}\mathrm{t}\mathrm{a}\mathrm{l}}=\sqrt{{{\theta }_{\mathrm{t}\mathrm{h}\mathrm{e}\mathrm{r}\mathrm{m}\mathrm{a}\mathrm{l}}}^2+{\left(3{\sigma }_{\mathrm{x},\mathrm{y}}\right)}^2}$ is 0.62″, which is less than 1″.

The results of our research indicate that thermal deformation significantly affects the attitude measurement accuracy of star cameras. Consequently, it is crucial to identify and analyze the sources of thermal deformation errors. By quantitatively assessing these error sources, effective preventive measures can be developed for thermal design. Moreover, robust thermal design plays a vital role in minimizing thermal deformation errors.

5. Experimental Study

The correctness of the thermal and thermal simulation temperature results was verified by the thermal balance test, and that of the predicted value of on-orbit thermal deformation error was also verified.

The test was carried out in a ZM4300 vacuum tank (Changchun Institute of Optics, Fine Mechanics and Physics, Chinese Academy of Sciences, Changchun, China). The temperature of the heat sink was 100 ± 5 K, and the vacuum degree was better than 1.33 × 10⁻³ Pa. In the test, a light source, an integrating sphere, and a collimator were used to simulate stars, as shown in Figure 8.

5.1. Simulation of Thermal Boundary Conditions

The star camera was installed on the rear frame of the Earth observation camera through the star camera support. In the test, a device composed of several thin plates was designed as the satellite cabin, as shown in Figure 9. The installation interface temperature was controlled at 21 °C in the worst heat case. The inner and outer surfaces of the satellite cabin plates and the upper and lower surfaces of the simulated mounting panels were covered with 20 layers of insulation components.

5.2. External Heat Flux

The star camera was divided into three parts according to the condition of receiving external heat flux: the light entrance, the baffle, and the lens tube. The heat fluxes absorbed by the baffle and the lens tube were both set as the average value of one orbital period. The parameters of the orbital heat flux are shown in

Table 4. Parameters of external heat flux.

Component	Power Dissipation (W)
The light entrance	0~3785 s	3785~5677 s
	1.8	27.5
The MLI covered on the baffle	18.7
The MLI covered on the lens tube	4.1

.

5.3. Temperature Results and Analysis

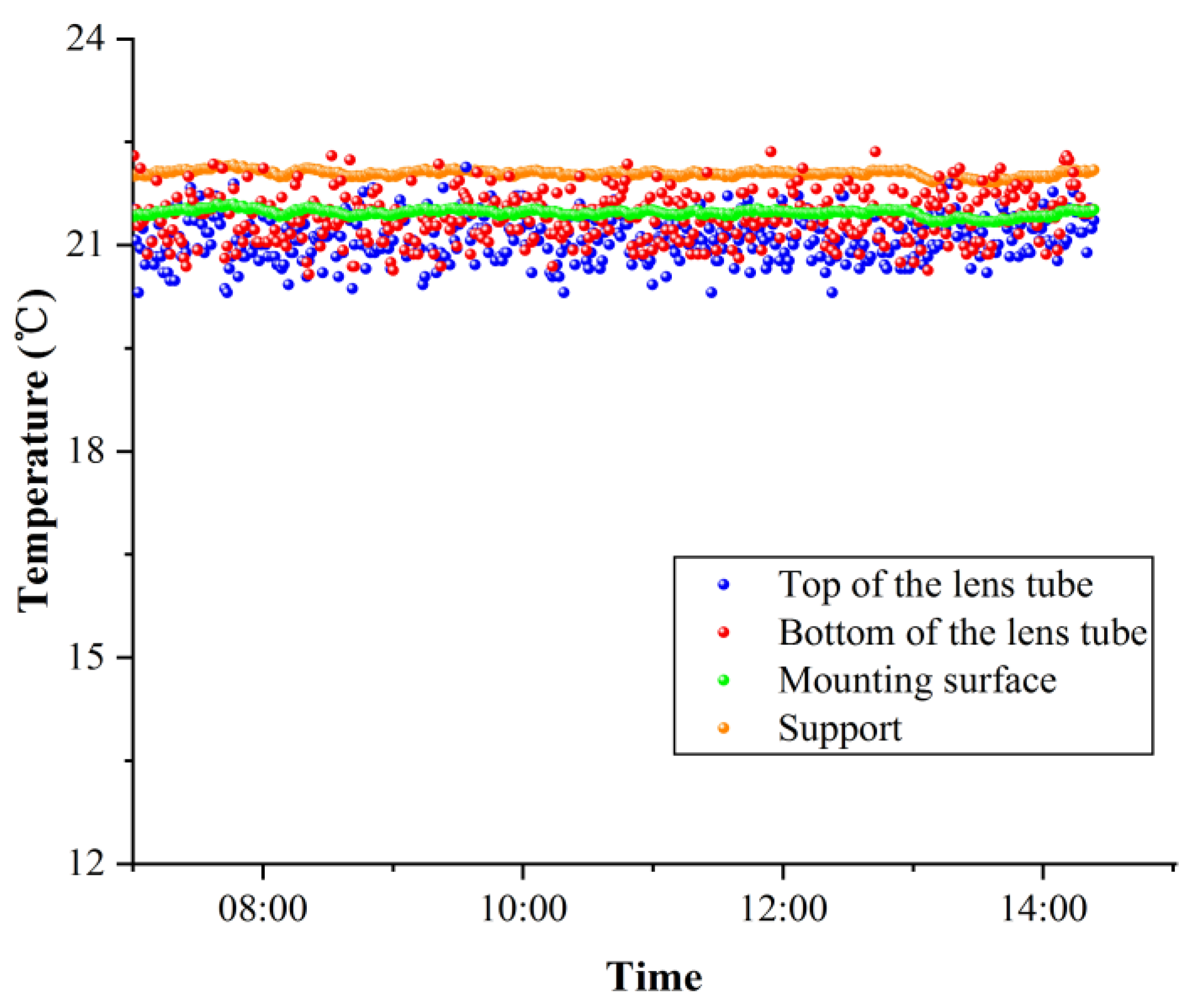
At 07:45 on the third day of testing, the worst heat case reached equilibrium, and the temperatures of the star camera are shown in Figure 10 and

Table 5. Temperatures of the star camera in the worst heat case.

	Results of Thermal Test (°C)	Results of Thermal Simulation Analysis (°C)	Absolute Difference Value
The top of the lens tube	20.54~21.72	20.18~22.10	<0.38
The bottom of the lens tube	20.75~22.00	20.38~22.24	<0.37
The mounting surface	21.40~21.50	20.93~23.30	<1.80
The star camera support	22.00~22.10	21.50~23.28	<1.18

. The temperature difference between the top and bottom of the lens tube did not exceed 3 °C. The maximum absolute difference between the test results and the thermal simulation was 1.8 °C. Discrepancies between the test results and the simulation analysis primarily arise from the simplifications made in the simulation model, errors related to the vacuum environment, and data acquisition inaccuracies.

6. On-Orbit Temperature Results and Analysis

Since its launch into orbit in 2018, the star camera has been operating well so far. The retrieved telemetry temperature data in comparison to thermal simulation data are shown in

Table 6. Temperatures of the star camera in orbit.

	Temperature in Orbit (°C)	Result of Thermal Simulation Analysis (°C)	Absolute Difference (°C)
Top of the lens tube	20.57~20.92	20.18~22.10	<1.18
Bottom of the lens tube	20.73~21.19	20.38~22.24	<1.05
Mounting surface	21.00~22.67	20.93~23.30	<0.63
Star camera support	21.62~23.00	21.50~23.28	<0.28

. The maximum absolute difference between the telemetry data and the thermal simulation analysis is only 1.18 °C, which proves the correctness of the thermal design.

The image of a star detected by the star camera in orbit is shown in Figure 11. Some attitude accuracy tests have been carried out in orbit, and the results show that the attitude accuracy of the camera is better than 0.54″, which is below our predicted maximum error of 0.62″.

Therefore, the correctness of the thermal design scheme and the thermal simulation analysis is verified, and the prediction value of the maximum thermal deformation error of the orbiting camera is also significant and correct.

7. Conclusions

In this study, the thermal design of a star camera and the thermal deformation of this camera in orbit are studied using simulation analysis. Then, the thermal balance test and on-orbit temperature analysis are carried out to verify the effectiveness of the thermal design scheme and the accuracy of the thermal simulation analysis. The major conclusions are listed as follows:

(1) The thermal deformation error significantly impacts the on-orbit attitude accuracy of the star camera. Specifically, the thermal deformation of the optical system is 0.24″, while that of the mounting surface and the support surface is 0.31″. The comprehensive limit error due to on-orbit thermal deformation is determined to be 0.5″. Combined with the thermal deformation error and the noise-equivalent angle, the limit error prediction value in orbit is 0.62″.

(2) During the thermal balance test, the temperature difference between the top and bottom of the lens tube does not exceed 3 °C. The temperature range of the mounting surface is 21.40 to 21.50 °C, while that of the star camera support is 22.00 to 22.10 °C. Notably, the maximum absolute difference between the test results and the thermal simulation analysis results is only 1.80 °C.

(3) Temperature data during orbit show that the maximum absolute difference between real-life conditions and the thermal simulation analysis is only 1.18 °C, and the attitude accuracy of the star camera is better than 0.54″. These results fully demonstrate the accuracy of the thermal design and the necessity of predicting thermal deformation errors in orbit.