1. Introduction
Magnetic storms, also known as solar storms, are phenomena where energetic particles and magnetic fields in the solar wind interact with the Earth’s magnetic field. These interactions can lead to violent disturbances in the Earth’s magnetosphere, causing sharp changes in the Earth’s magnetic field [
1,
2]. Geomagnetic storms are typically caused by disturbances in solar wind and are accompanied by a prolonged southward interplanetary magnetic field. These events release substantial amounts of energy and charged particles, which are carried through space by the solar wind [
3,
4,
5]. When these charged particles interact with the Earth’s magnetic field, they can trigger a complex array of phenomena. In extreme cases, magnetic storms can severely impact the Earth’s communication and navigation systems [
6,
7,
8,
9]. Identifying the specific frequencies affected by magnetic storms will not only aid in the in-depth study of these phenomena, but also be crucial for the anti-jamming design of spacecraft and communication equipment.
The primary data used to study magnetic storms include interplanetary magnetic field and solar wind data, ionospheric and magnetospheric data, and geomagnetic disturbance indices. However, interplanetary signature data or geomagnetic disturbance indices do not identify specific frequencies and bands affected by magnetic storms. It is the use of electric and magnetic field data that allows for in-depth study of this topic. With advances in space technology, mankind began to utilize satellites and other space probes to observe solar activity and magnetic storms. Observation of the Earth’s magnetic field from space began more than 60 years ago. However, continuous monitoring of the geomagnetic field using low-Earth-orbit (LEO) satellites did not begin until 1999 [
10]. Since the launch of DEMETER in 2004, the satellite has provided technical support for direct observation of the ionospheric electric field [
11]. In 2018, the CSES-1 satellite was launched and became the second LEO satellite capable of directly observing changes in the electric field in space in the F-region of the ionosphere [
12].
The use of satellite electric and magnetic field data to study frequencies with significant perturbations caused by anomalous space phenomena has been partially investigated by previous researchers. In 2002, Trautner [
13] analyzed electric field data in the frequency range of 10 Hz to 300 Hz during magnetic storms, including the Schumann resonance frequency, and concluded that the peaks of the perturbation signals were concentrated in the range of 10 to 200 Hz. Parkhomov [
14] observed short bursts of geomagnetic pulsations in the frequency range of 0.2 to 5 Hz during magnetic storms, with a global maximum at a frequency of 2.78 ± 0.38 Hz. Although a preliminary range of anomalous frequencies has been identified in the ULF band, the most significant specific frequencies affected by magnetic storms have not yet been determined.
In the ULF band, the study of Pc waves constitutes a significant aspect of ULF wave research. As early as 1993, Fraser-Smith [
15] conducted electromagnetic monitoring of ULF waves and found that ELF/VLF waves are much less affected than ULF waves when a magnetic storm is approaching. It has been demonstrated that the response of ULF waves during magnetic storms is closely related to geomagnetic activity and solar parameters [
16]. ULF wave power is linearly correlated with the absolute value of the SYM-H index during the main phase of the storm and exponentially correlated with the absolute value of the SYM-H index during the recovery phase [
17]. The intensity of geomagnetic pulsations with a frequency of 27 mHz during the initial phase of the magnetic storm reaches a maximum in the morning and night segments at polar and auroral latitudes, respectively. Daytime Pc5 wave pulsations are strongest during the main phase of the magnetic storm, not during the recovery phase as previously thought [
18,
19]. Different interplanetary sources cause varying pulsation strengths. The higher latitude position of the Pc5 pulsation intensity maximum in CIR storms suggests larger dimensions of the daytime magnetosphere during CIR storms compared to CME storms [
20].
In the ELF and VLF bands, Tatsuta [
21] analyzed two years of nightly data from the VLF/LF observation network in Japan and found that high latitudes are more affected by geomagnetic activity compared to mid-latitude and low-mid-latitude paths. During the strong magnetic storm from 8 to 10 November 2004, intense electromagnetic harmonic emissions between 500 and 2000 Hz were detected at mid-latitudes. Similar emissions were also observed on 21–22 January 2005 and on 15 May 2005 during two magnetic storms of lower intensity [
22]. Pinto and Gonzalez [
23] suggest that the enhancement of these waves during geomagnetic storms and substorms is characterized by a peak at 550 Hz and that their intensity is highly dependent on magnetic activity. Zhima [
24,
25] found that very low-frequency waves below 3 kHz were significantly enhanced throughout the magnetic storm, whereas high-frequency waves above 3 kHz were significantly enhanced in the later part of the main phase and the earlier part of the recovery phase.
In the study of the HF band, Blagoveshchenskii [
26] analyzed the manifestation of the so-called main ionospheric effect on the propagation properties of ten-meter waves during geomagnetic storms. Specifically, these parameters increase before the active phase of the disturbance, decrease during the active phase, and increase again after this phase. Since the response of magnetic storms in the HF band is not as pronounced as in the ULF/ELF/VLF bands, fewer studies have been conducted on magnetic storms using the HF band.
Although it is widely accepted that magnetic storms affect different frequencies and bands differently, detailed conclusions regarding specific frequencies and bands are lacking. In this paper, we utilize EFD spectrum data from CSES-1 to investigate the spectral characteristics of frequencies and bands exhibiting strong disturbances in different regions during the magnetic storm activity (Dst < −100 nT) on 4 November 2021. This paper fills a gap in the study of the frequency and band of strong ionospheric disturbances during magnetic storms.
2. Materials and Methods
The main scientific objective of the CSES-1 [
12] is to monitor ionospheric perturbations associated with natural hazards in the quest for possible anomaly forecasting. The CSES-1 completes 15.2 orbits around Earth per day, with an orbital period of ~94.6 min and a five-day recursive period over the same geographic area with the ascending/descending node local time of 02 a.m./02 p.m., respectively. Orbit altitude is 507 km. Eight payloads are assembled on CSES-1, that is, a high-precision magnetometer (HPM), search coil magnetometer (SCM), electric field detector (EFD), Langmuir probe (LA), plasma analyzer (PAP), high-energy particle detector (HEPD), GNSS occultation receiver (GOR), and tri-band beacon (TBB). The entire satellite is capable of acquiring 17.6 h of scientific exploration data per day and has the capability of continuous exploration within the latitude of 65° north and south at all hours of the day [
27].
The electric field is detected by an electric field detector (EFD). The EFD is an advanced detector for space-based measurements of electric fields in a wide frequency band, specifically conceived for operation in the ionosphere–magnetosphere transition zone. The instrument, designed to be installed on the 3-axis stabilized CSES-1 satellite, includes: 4 probes exposed to the ionospheric plasma, accommodated on the tips of 4 booms (each of them four meters long, deployed far from the spacecraft body in order to reduce the electromagnetic disturbances induced by the satellite equipment) and a data acquisition unit (for signal conditioning, sampling of input signals and spectral analysis). The electric field detector (EFD) data of the CSES-1 satellite has waveform data (mV/m) and PSD (Hz) data. The PSD data are obtained by Fourier transform of the waveform data. EFD retrieves the electric field components measured between pairs of probes in the following bands: ULF (Ultra-Low-Frequency, DC to 16 Hz), ELF (Extremely Low-Frequency, from 6 Hz to 2.2 kHz), VLF (Very Low-Frequency, 1.8 to 20 kHz), and HF (High-Frequency, from 18 kHz to 3.5 MHz), with sampling rates of 128 Hz, 5 kHz, 50 kHz, and 10 MHz, respectively.
2.1. Satellite Data Process
We use the average degree of difference between electric field data and the background field data during magnetic storms, which we call the D value, as a criterion for determining the magnitude of the effect of magnetic storms. The background field data are set to be a revisited orbit in a quiet period (with Dst values ranging from −30 to 30 nT) within one month before the current orbit. Since the revisit period of the CSES-1 satellite is 5 days, a date with an interval of multiple of 5 can be used as the background field. Because the Dst values from 28 October 2021 to 1 November 2021 are within the range above, it is a quiet period. Therefore, the electric field power spectrum data of these 5 days are selected as the background field data. The power spectrum of the satellite electric field with background noise removed for the selected latitude width is
The average degree of difference of frequency j is
The variation growth rate is
is the selected latitude width. is the satellite electric field power spectrum for the selected latitude width. is the value of the quiet period.
2.2. Wavelet Coherence (WTC) Analysis
This work uses wavelet coherence (WTC) to analyze the correlation between electric field power sequences and Dst values at different frequencies. Continuous Wavelet Transform (CWT) and Discrete Wavelet Transform (DWT) are the two types of WT. Because of its appropriate time and frequency localization, the Morlet wavelet (dimensionless frequency,
) is an ideal option for extracting features [
28]. The WTC spectrum quantifies the degree to which two-time series co-vary as functions of time and frequency. The XWT spectrum exposes large shared power areas and relative phases between two-time series in time frequency space [
29,
30]. The XWT of two-time series X(t) and Y(t) is defined as
The CWT coefficients of sequences and at frequency scale s are denoted by , and respectively, and denotes the complex conjugate.
The square of the wavelet coherence factor is defined as
is the smoothing operator and is the scale. WTC values around 1 indicate a higher degree of resemblance across time series, whilst coherence values near 0 indicate no correlation.
This paper focuses on the analysis of the 4 January 2021 magnetic storm by examining data from two days before and two days after the storm, covering the initial, main, and recovery phases. After removing background noise through satellite data processing, we conducted a quantitative analysis of the magnetic storm’s impact on different hemispheres and frequency bands using D value analysis, variation growth rate analysis, and WTC analysis. This analysis identified the strong disturbance frequencies and frequency bands of the magnetic storm, as well as its spatial and temporal characteristics. The technical route of this paper is shown in
Figure 1.
3. Results
3.1. Single-Frequency PSD Analysis
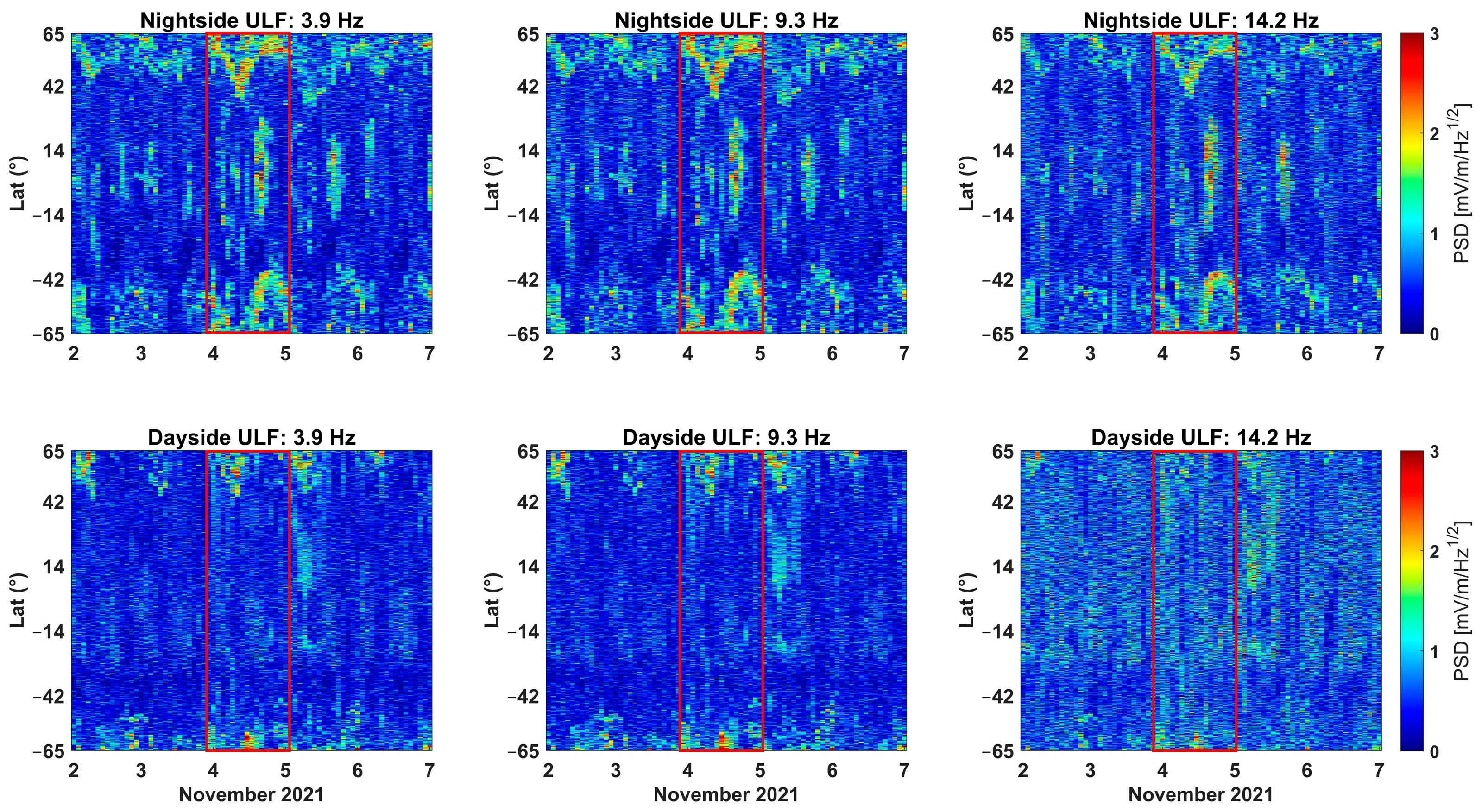
To understand the responses of different frequency bands to magnetic storms, we first denoised the satellite electric field data and then obtained the power spectrum data (PSD) of four frequency bands (
Figure 2 and
Figure 3).
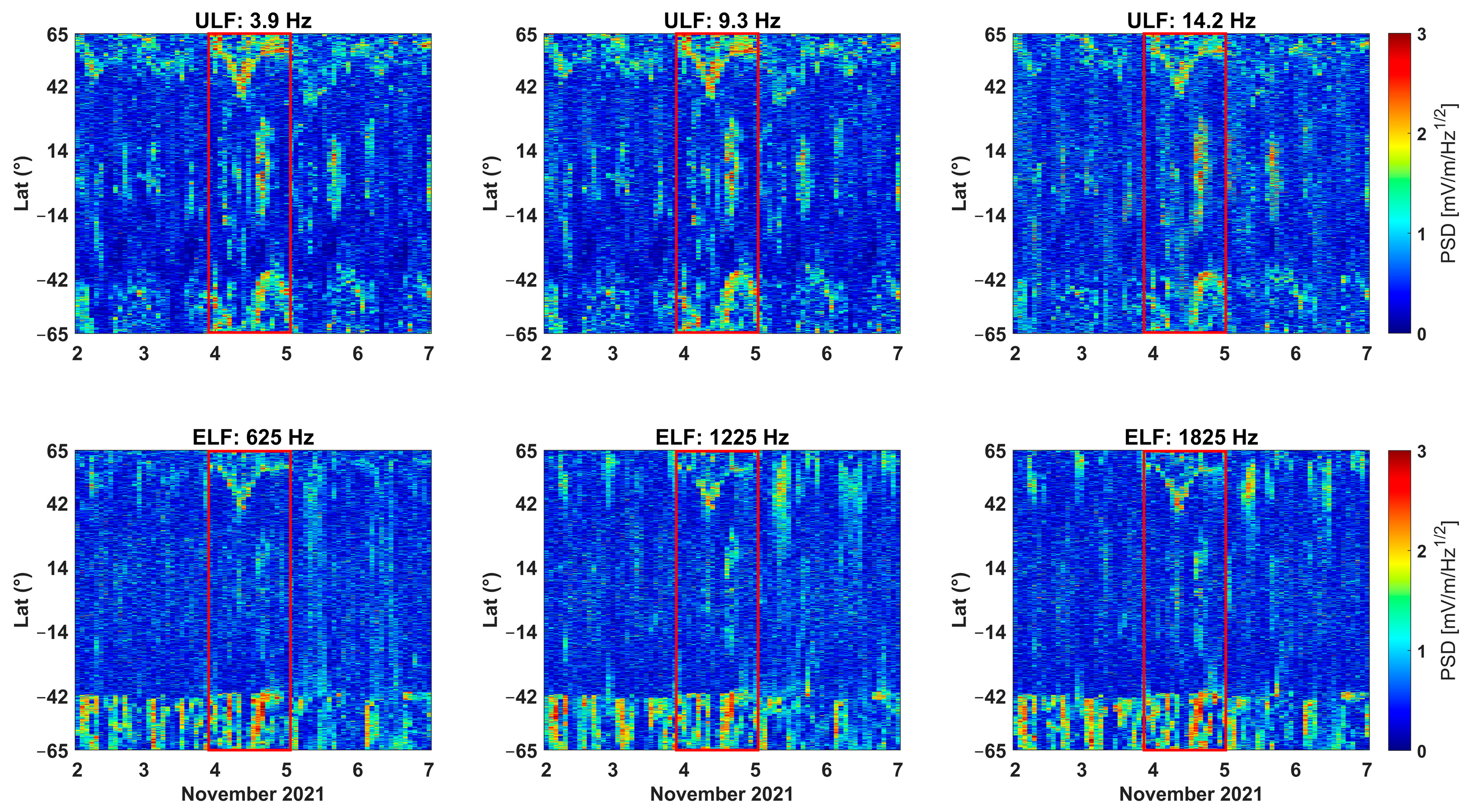
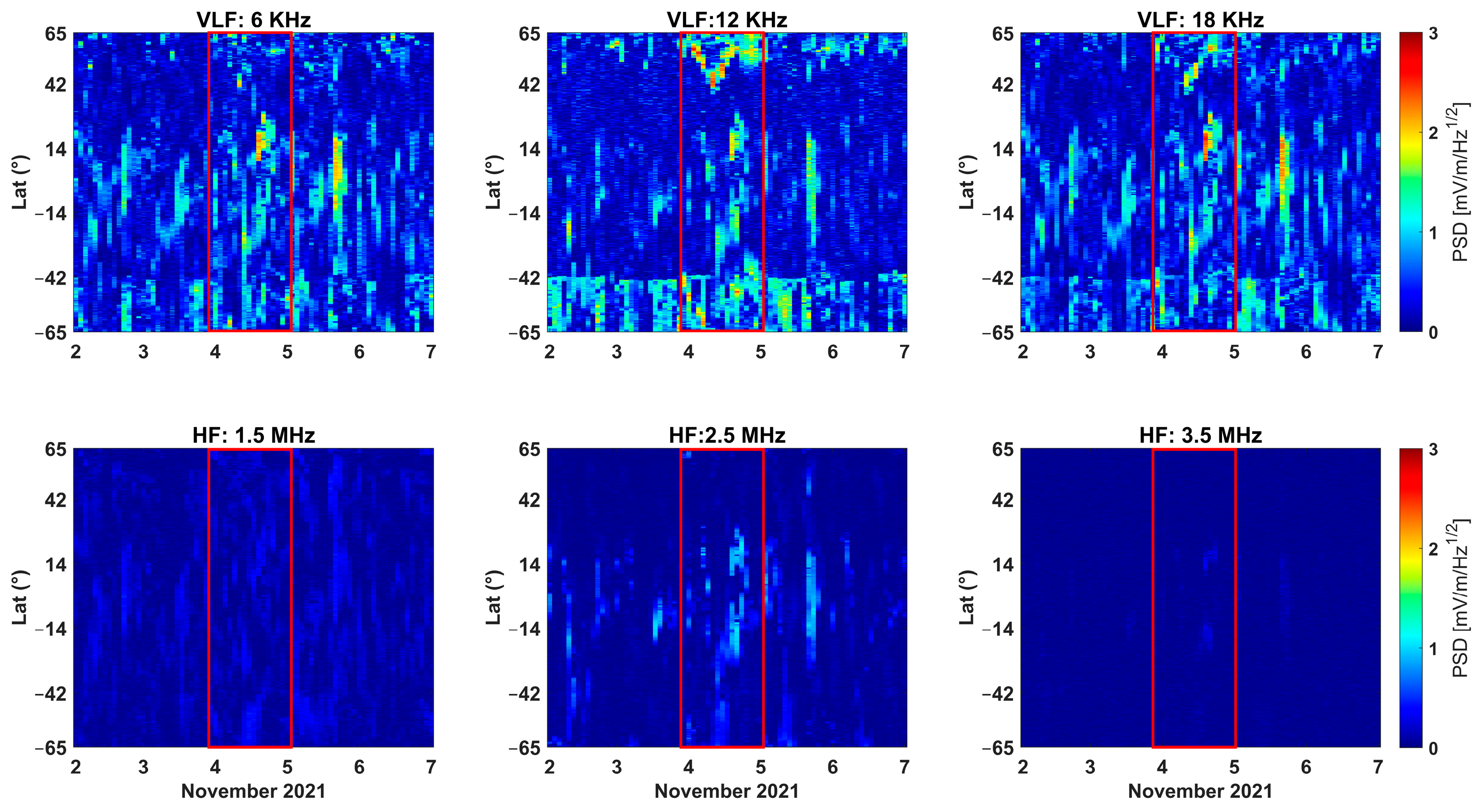
Figure 2 and
Figure 3 illustrate three frequencies from the ULF, ELF, VLF, and HF bands, respectively, to observe the changes during the initial, main, and recovery phases of the magnetic storms. The main phase is highlighted by red rectangles.
Three frequencies, 3.9 Hz, 9.3 Hz, and 14.2 Hz, were selected for the ULF band. These three frequencies showed significant disturbance enhancement on 4 November 2021 (blue indicates quiet, red indicates disturbance). The main disturbance area is above 22°, and the latitudinal extent of the disturbance varies over time. In the southern hemisphere, the latitudinal extent of the disturbance gradually widens. In the northern hemisphere, the latitudinal range of the disturbance first widens and then narrows. An electric field disturbance with a duration of about 1 h (two orbits have color anomalies and one orbit’s observation time is 0.5 h) occurs in the middle and low latitudes. The red anomalies at 3.9 Hz and 9.3 Hz are darker than those at 14.2 Hz, indicating that the disturbance strength at these two frequencies is greater than that at 14.2 Hz.
In the ELF band, significant electric field disturbances were observed in the northern hemisphere during the main phase of the magnetic storm. The disturbance pattern is similar to that of the ULF band, with the red anomalous region being smaller in color intensity and density. This indicates that the disturbance intensity and extent in the ELF band are smaller than those in the ULF band. In the southern hemisphere, there is a constant electric field disturbance in the range of 42°N to 65°N. A deepening of the red color during the main phase indicates a strengthening of the constant electric field perturbation, although the exact perturbation requires quantitative analysis later. Among the three frequencies in the ELF band, the electric field perturbation is stronger at 1825 Hz than at 625 Hz and 1225 Hz.
There are significant differences in the perturbations at different frequencies in the VLF band. The 12 kHz frequency point shows obvious disturbances in the northern hemisphere, the 18 kHz frequency point shows slightly weaker disturbances, and the 6 kHz frequency point shows almost no disturbances. In the southern hemisphere, there is a constant disturbance in the VLF band in the range of 42°N to 65°N, which is weaker than that in the ELF band. No significant electric field perturbations are observed at any of the three frequencies in the HF band.
Comparing the four frequency bands, the frequencies in the ULF, ELF, and VLF bands all show electric field perturbations during magnetic storms, whereas the frequencies in the HF band show almost no perturbations. From the several frequencies illustrated in the figure, the ULF band shows obvious electric field disturbances in both the northern and southern hemispheres, while the ELF and VLF bands show obvious electric field disturbances only in the northern hemisphere. The range of electric field disturbances in the ULF band is larger than that in the ELF and VLF bands. Magnetic storms do not significantly affect the HF band.
3.2. ULF Band’ D Value Analysis
As discussed above, the responses of magnetic storms observed at different frequencies are different. The prevalent view of the storm–substorm relationship has been that substorms are the main building blocks of storms [
13]. Although this view was questioned, both magnetic storms and substorms have an effect on the ionosphere [
1], and magnetic storms and substorms generally occur in tandem. A substorm event was also present during the 4 November 2021 magnetic storm event. The disturbances recorded in the satellite electric field were the result of a combination of magnetic storms and substorms.
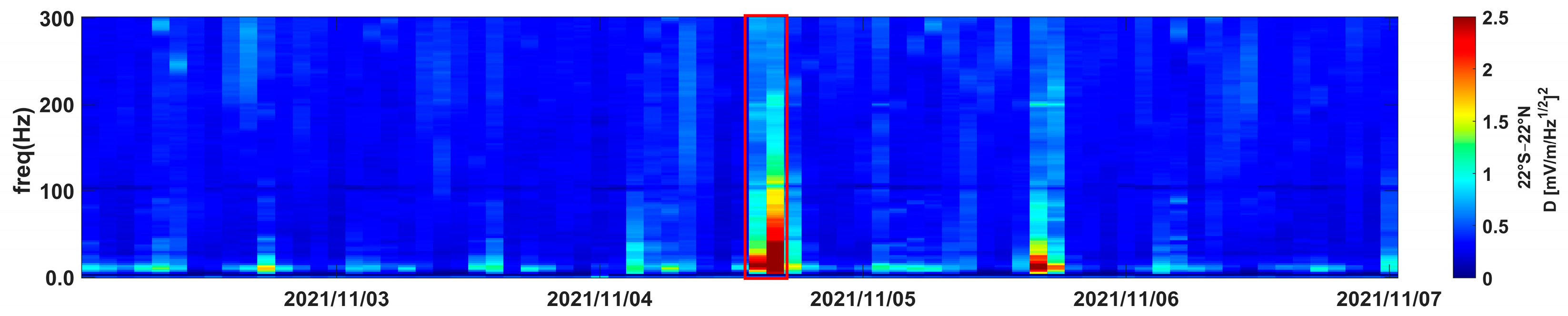
Each frequency band contains many frequencies, and it is insufficient to analyze them using the PSD plots of a single frequency point. Therefore, it is necessary to analyze all frequencies collectively to identify the frequencies and bands most disturbed by magnetic storms. From the above analysis, the disturbance caused by magnetic storms varies across different regions. Therefore, the world is divided into three regions for analysis: 65°S to 65°N, 22°S to 65°S, 22°S to 22°N, and 22°N to 65°N.
Table 1 lists the maximum D values of the different bands, the corresponding frequencies, and the frequency bands that are most disturbed.
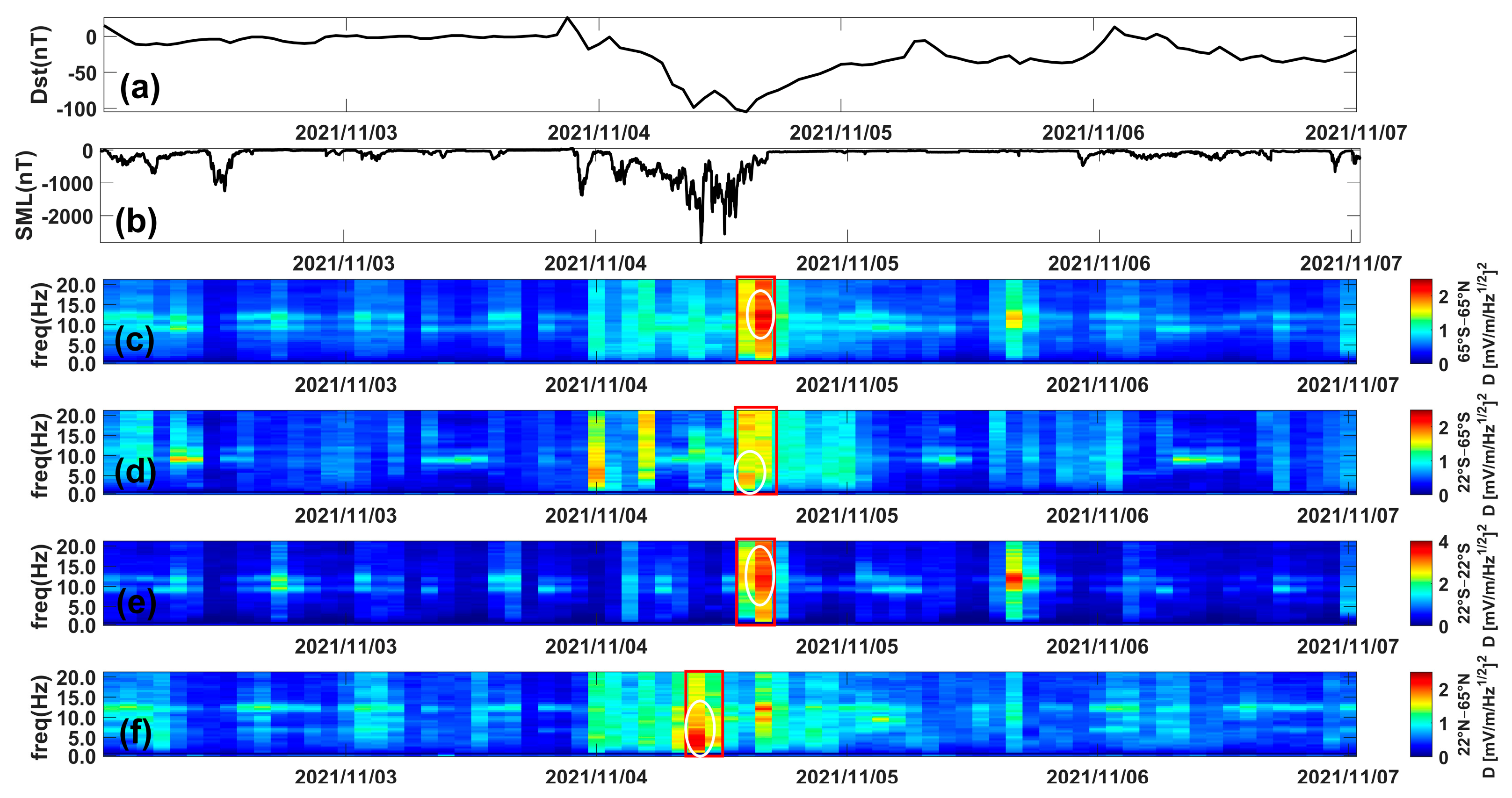
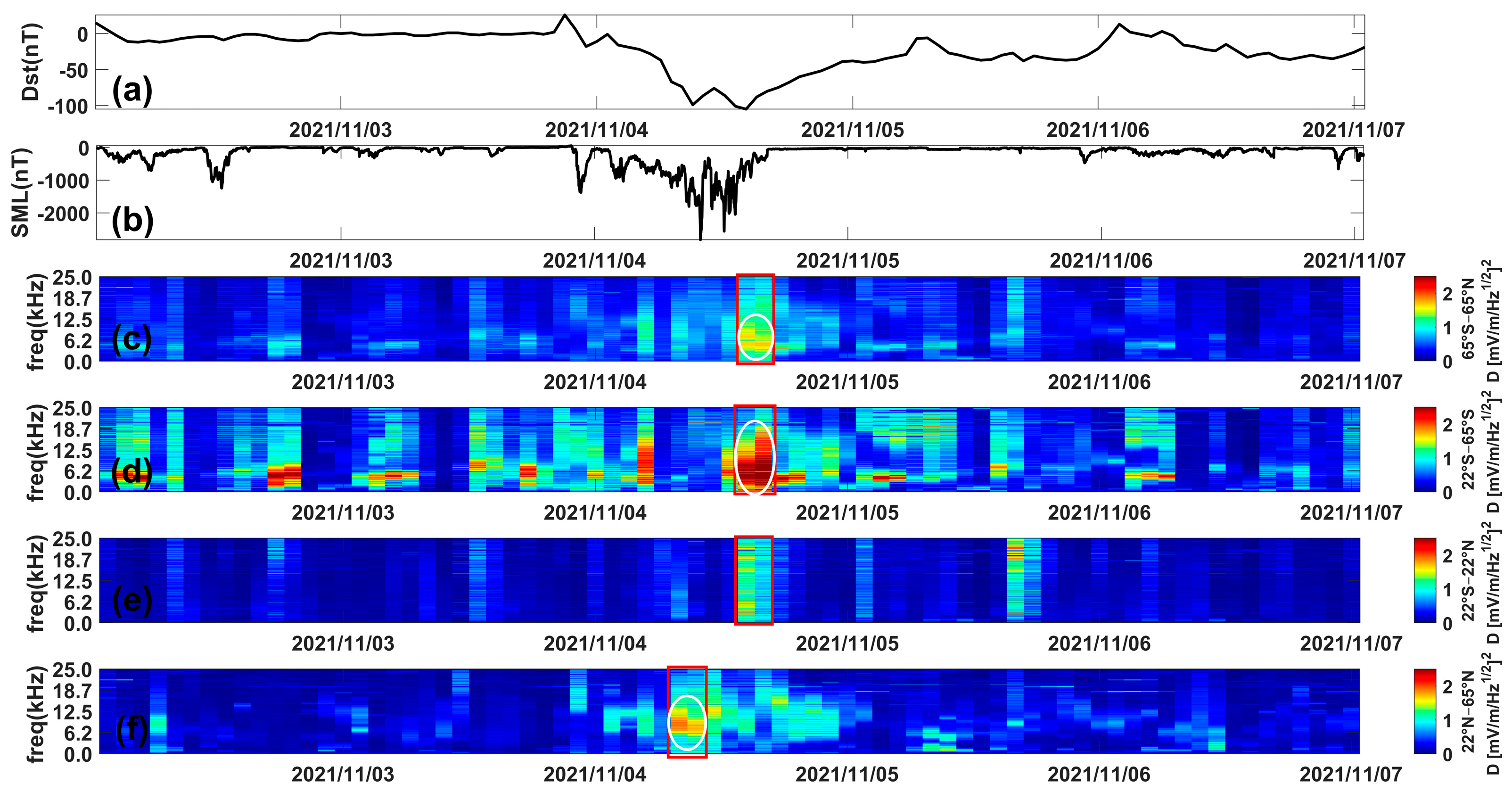
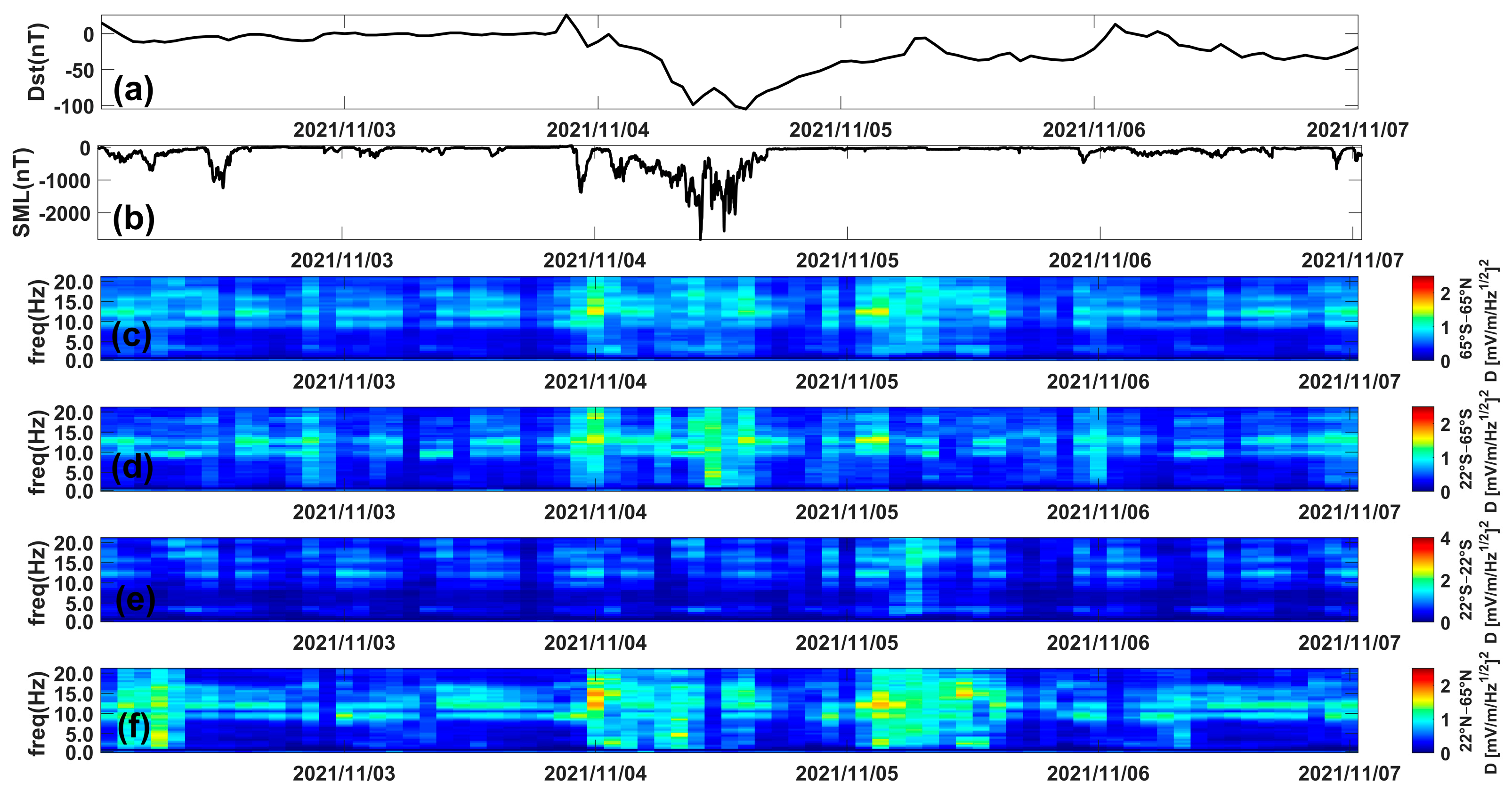
Figure 4 shows the Dst index (a), SML index (b), and the D values from 1 November 2021 to 7 November 2021. The Dst index represents the intensity of the magnetic storm and the SML index represents the intensity of the substorm. The Dst index decreases sharply during magnetic storms, and the D values also show significant changes. The D values in all four regions increase from approximately 0–1 to about 2 during the quiet period. Comparing the high and middle-to-low latitudes in the northern and southern hemispheres reveals a clear difference in the anomalous enhancement of the electric field in the ULF band. In the ranges 65°S to 65°N (c), 22°S to 65°S (d), and 22°S to 22°N (e), the maximum perturbation orbits correspond to the second Dst minimum. The maximum perturbation orbits in the range 22°N to 65°N (f) correspond to the first minimum. A clear hemispheric asymmetry is observed. The maximum D value in the range of 65°S to 65°N (c) occurs near 13 Hz, and the D value is essentially above 1.8 throughout the band. This is consistent with the perturbation in the range 22°S to 22°N (e), where the maximum frequency point of the perturbation is 13.2 Hz, corresponding to a maximum D value of 2.83. In contrast, the maximum frequency point of the perturbation in the ranges 22°S to 65°S and 22°N to 65°N is not around 13 Hz, but is in the frequency band of 0–5 Hz. The maximum D value in the range of 22°S to 65°S (d) is 1.81, corresponding to a frequency point of 4.4 Hz. The maximum D value in the range of 22°N to 65°N (f) is 2.30, corresponding to a frequency point of 2.9 Hz. In the different regions, the orbits with large disturbances are generally two orbits (each with an observation time of about 0.5 h), indicating that the time from strong disturbance to recovery is about 1 h.
3.3. ELF Band’ D Value Analysis
In the ELF band, disturbance is intensified by a change in the full-band D value from 0–1 to 1–2 at the second Dst minimum across the range of 65°S to 65°N. As analyzed in
Section 3.1, there is a constant electric field perturbation in the ELF band in the southern hemisphere, making it impossible to directly observe the electric field perturbation induced by magnetic storms in this region. According to
Figure 5, there is a disturbance corresponding to the second minimum value of Dst in the range of 65°S to 65°N (c), with a disturbance strength of approximately 1. However, the D values in the ranges of 22°N to 22°S (e) and 22°N to 65°N (f) are generally less than 1. Therefore, the disturbance of D values in the range of 65°S to 65°N (c) originates from the range of 22°S to 65°S (d). There is a perturbed response to magnetic storms in the range of 22°S to 65°S (d) with a perturbation intensity greater than 1. The maximum D value in the range of 22°S to 65°S (d) is 3.25 (
Table 1), corresponding to a frequency point of 2441 Hz. Due to the presence of a constant perturbation, the value of 3.25 cannot be considered as the magnitude of the perturbation caused by the magnetic storm. Besides the frequency 2500 Hz, there is also a strong disturbance band between 300 Hz and 900 Hz. Zhang et al. (2022) used satellite electric field data at 225, 725, 1125, 5000, 7500, and 13,500 Hz in their study of electric field disturbances induced by magnetic storms. Their results show that the electric field anomalies are most pronounced at 725 Hz, which agrees with the conclusions of our analysis.
The maximum D value in the range of 22°N to 65°N (f) does not exceed 2, and no distinct strong perturbation frequency point or band is observed. In the range of 22°S to 22°N (e), there is a strong perturbation band below 300 Hz. Due to the broad range of the ELF band, specific strong perturbation frequency bands cannot be directly identified. Consequently, we analyze the frequency range from 0 to 300 Hz by segmenting the ELF band (
Figure 6). It can be observed that electric field disturbances in the range of 22°S to 22°N (e) are primarily below 100 Hz. During this magnetic storm event, the frequency band of equatorial ionospheric anomalies, induced by penetrating electric fields from high to low latitudes, ranges from 0 to 100 Hz.
3.4. VLF Band’ D Value Analysis
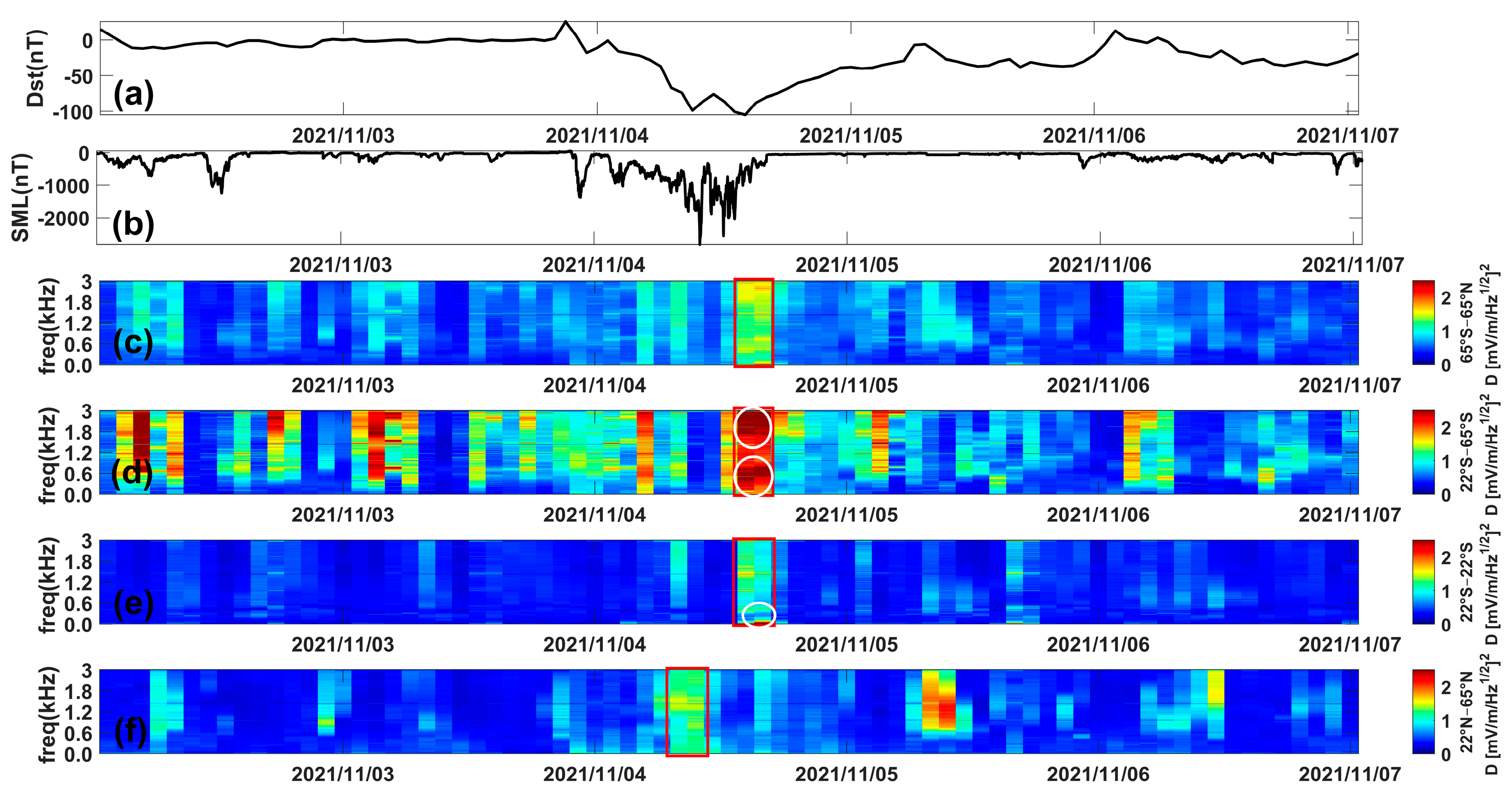
In the VLF band, magnetic storm-induced electric field anomalies are concentrated in the frequency range of 2.5 to 10 kHz across the latitude range of 65°S to 65°N (
Figure 7). A constant electric field disturbance is observed around 4–5 kHz, primarily originating from the southern hemisphere. Magnetic storm-induced anomalies at high latitudes in the southern hemisphere are primarily concentrated between 3 and 10 kHz, with the strongest anomaly occurring at 5396 Hz, corresponding to a D value of 3.82. Since this frequency falls within the range of constant disturbances, the D value cannot be used to quantify the strength of magnetic storm disturbances. However, aside from the constant interference band, the 3–10 kHz range shows significant enhancement, with D values exceeding 2. Electric field disturbances in the 22°N to 22°S (d) range are also slightly enhanced. However, disturbances at middle and low latitudes are weak and insignificant compared to those at high latitudes in both hemispheres. In the northern hemisphere, electric field anomalies are concentrated between 6 and 15 kHz, with a peak anomaly frequency around 8887 Hz and a maximum D value of 1.93. As there is no constant electric field perturbation in the northern hemisphere, the D value of 1.93 can be considered indicative of the strength of magnetic storm-induced electric field perturbations. The anomalous bands in the northern and southern hemispheres roughly overlap, with VLF band anomalies caused by strong magnetic storms predominantly occurring in the 3 to 15 kHz range. Electric field disturbances in the VLF band above 18 kHz are relatively minor.
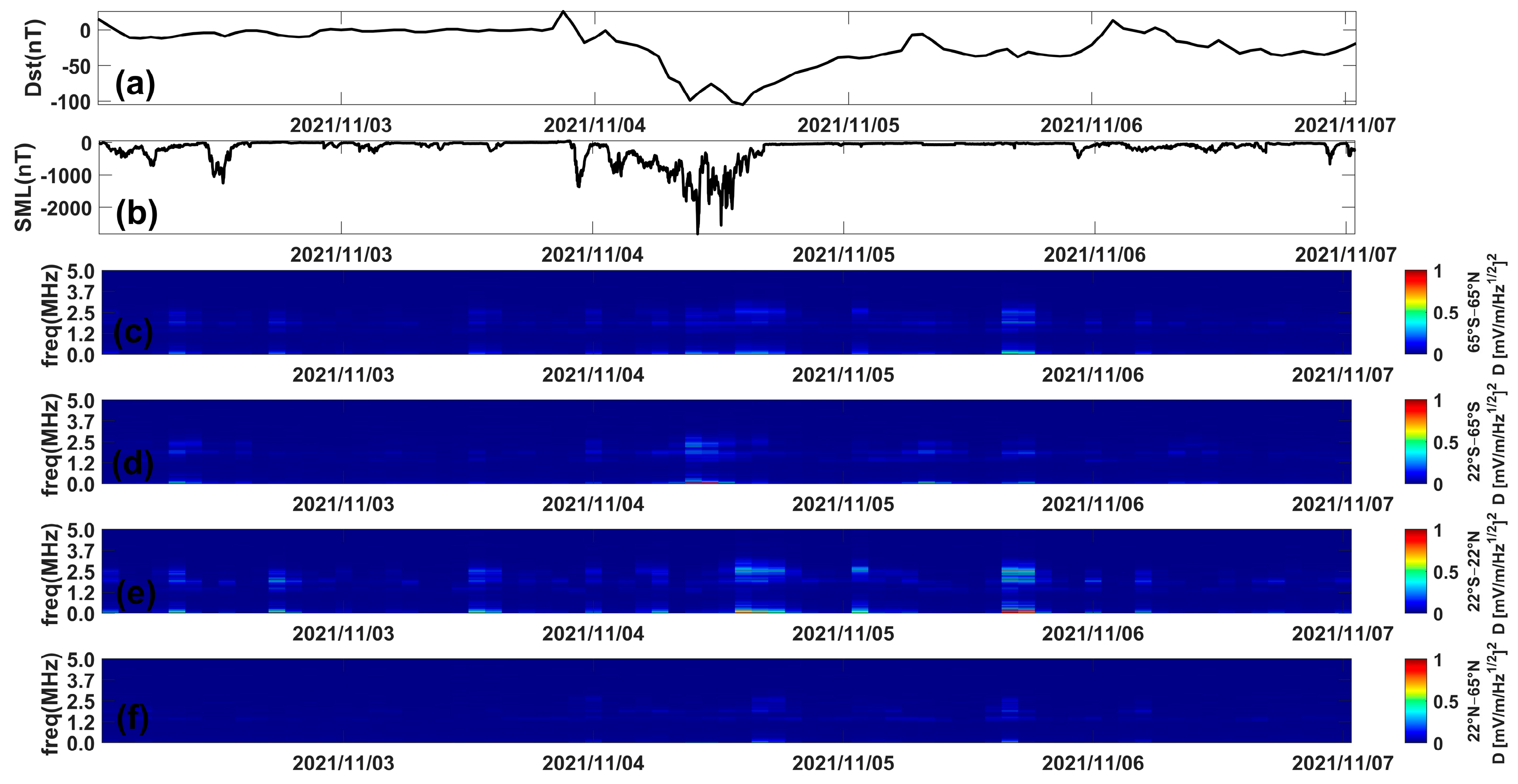
3.5. HF Band’ D Value Analysis
In the HF band, the impact of magnetic storms is nearly negligible compared to the electric field anomalies observed in the ULF, ELF, and VLF bands, with maximum D values in different regions being less than 1 (
Figure 8). Consistent with the findings in the VLF band, the impact of magnetic storms diminishes above 18 kHz and is virtually negligible in the HF band.
In conclusion, the frequency bands affected by magnetic storms are the ULF, ELF, and VLF bands. All three bands exhibit more pronounced perturbations at high latitudes, with only the ULF band showing significant enhancement of electric field perturbations near the Schumann wave frequency point at middle and low latitudes. Overall, the ULF band shows a greater response to magnetic storms compared to the ELF and VLF bands. However, the D value only indicates the absolute increment. To obtain a more accurate assessment, the growth rate of perturbations across different frequency bands needs to be calculated.
3.6. Comparison of Ex, Ey and Ez
Since the satellite electric field has three components, and the Ex component was used in the previous study, to ensure the reliability of the conclusions, the trends of all three components need to be consistent. This section discusses the consistency of the three components based on the disturbance maximal orbit. Since the electric field components Ex, Ey, and Ez, after background noise removal, exhibit more outliers, we employ the median absolute deviation (MAD) method to eliminate these anomaly outliers. Subsequently, the sliding averages method [
31] is applied to smooth the signal, which reduces random noise while preserving the original trend.
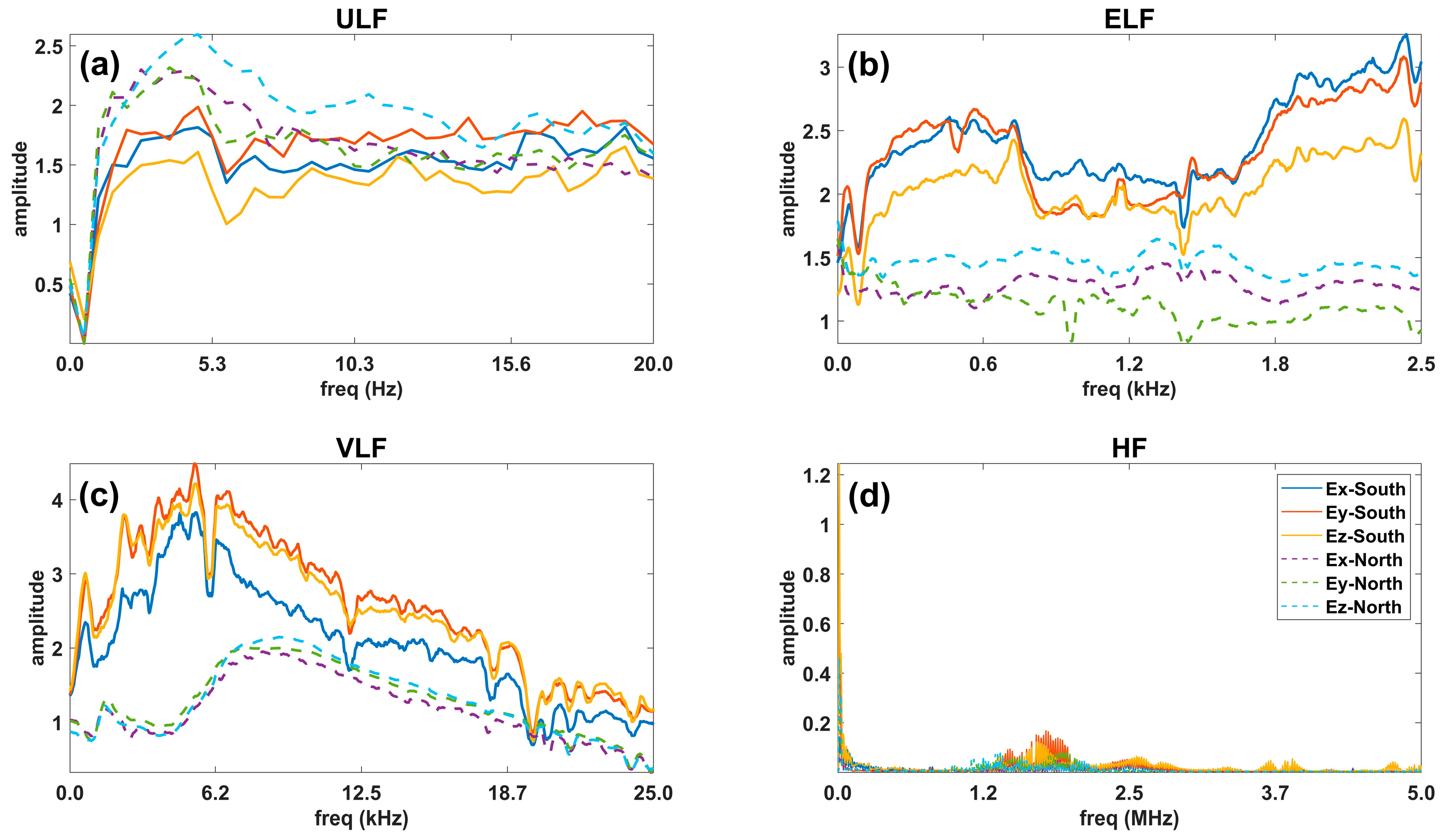
Figure 9 illustrates the three-component electric field plots for the 22°S to 65°S and 22°N to 65°N ranges across the four bands of the perturbed maximum orbit. The figure shows that the trends of the curves for the different electric field components Ex, Ey, and Ez are essentially consistent, with only slight variations in the magnitudes of the energy amplitudes.
In the ULF band, the trend is consistent between the northern and southern hemispheres, characterized by an initial increase followed by a decrease, with an extreme value observed in the 0–5 Hz range. This value decreases after reaching its peak and subsequently stabilizes. In contrast, trends in the ELF and VLF bands are not consistent between the hemispheres. In the ELF band, the southern hemisphere shows a maximum near 2441 Hz, whereas the northern hemisphere does not exhibit any significant extreme values. In the VLF band, the southern hemisphere exhibits an extreme value at 5.40 kHz, while the northern hemisphere shows an extreme value at 8.89 kHz. The three-component perturbations in the HF band remain below 0.2.
3.7. Analysis of Variation Growth Rate
The analysis of D values in
Section 3.2,
Section 3.3,
Section 3.4 and
Section 3.5 only reflects the absolute changes in disturbances. To verify conclusions such as the ULF band being the most affected by magnetic storms, this section will calculate the growth rate of perturbations, or the relative change, across different frequency bands.
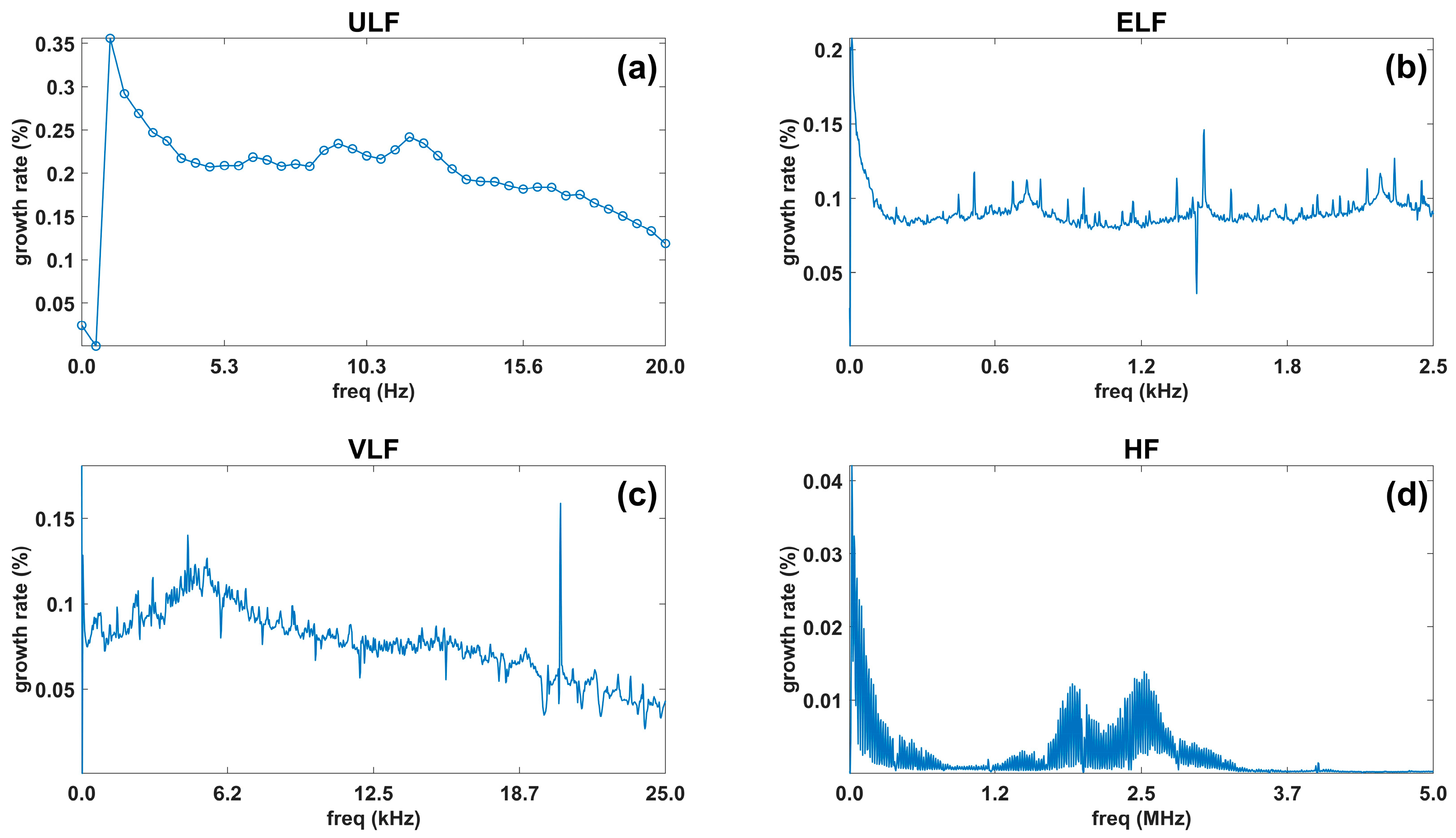
Figure 10 illustrates the perturbation growth rates across the four frequency bands for the period of magnetic storms in November 2021. In the ULF band, the most significant relative changes occur at frequencies below 5 Hz, with notable relative changes also observed around 8 Hz and 13 Hz. The relative changes in the ELF and VLF bands are considerably weaker compared to those in the ULF band. The high-frequency band exhibits the smallest relative change, which is not discernible as a color difference in the figure. Therefore, magnetic storms have the most significant effect on the ULF band and a negligible impact on the HF band.
In order to quantitatively analyze the disturbance growth rate in the four frequency bands, we draw the curve of the maximum orbit of the disturbance growth rate (
Figure 11). The figure shows that the disturbance growth rate in the ULF band tends to decrease. It decays sharply at 0–5 Hz and gradually stabilizes after 5 Hz. In the 0–5 Hz band, the disturbance growth rate is greater than 20%. Above 5 Hz, the disturbance growth rate remains around 20% and gradually decreases. Around 8 Hz and 13 Hz, there is a slight increase in the perturbation growth rate. The disturbance growth rate in the ELF band remains around 8%. The disturbance growth rate in the VLF band reaches an extreme value (greater than 10%) near 5 KHz, and then gradually decreases, basically not exceeding 10%. The disturbance growth rate in the HF band is in the neighborhood of 2%.
3.8. Wavelet Coherence (WTC) Analysis
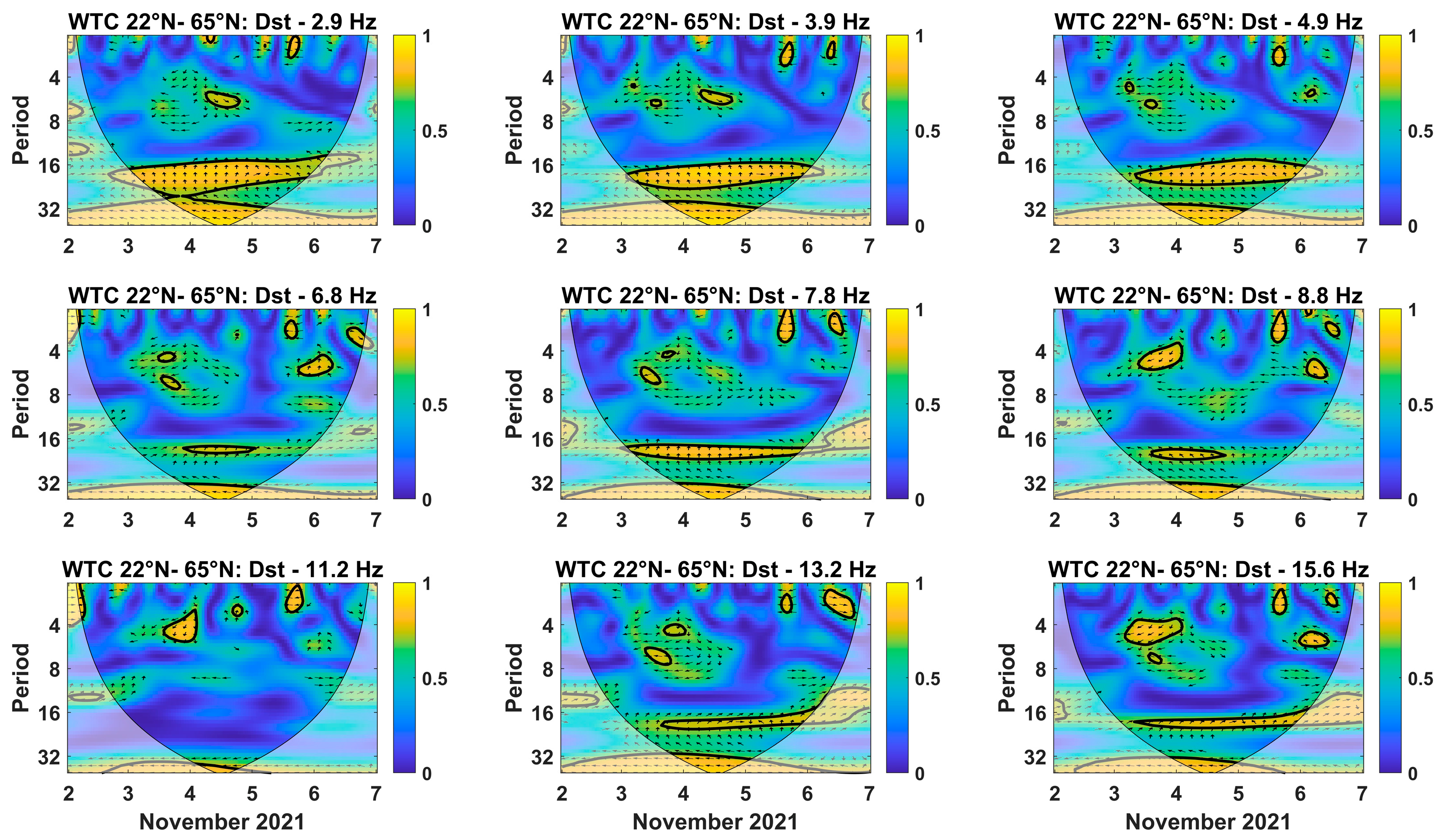
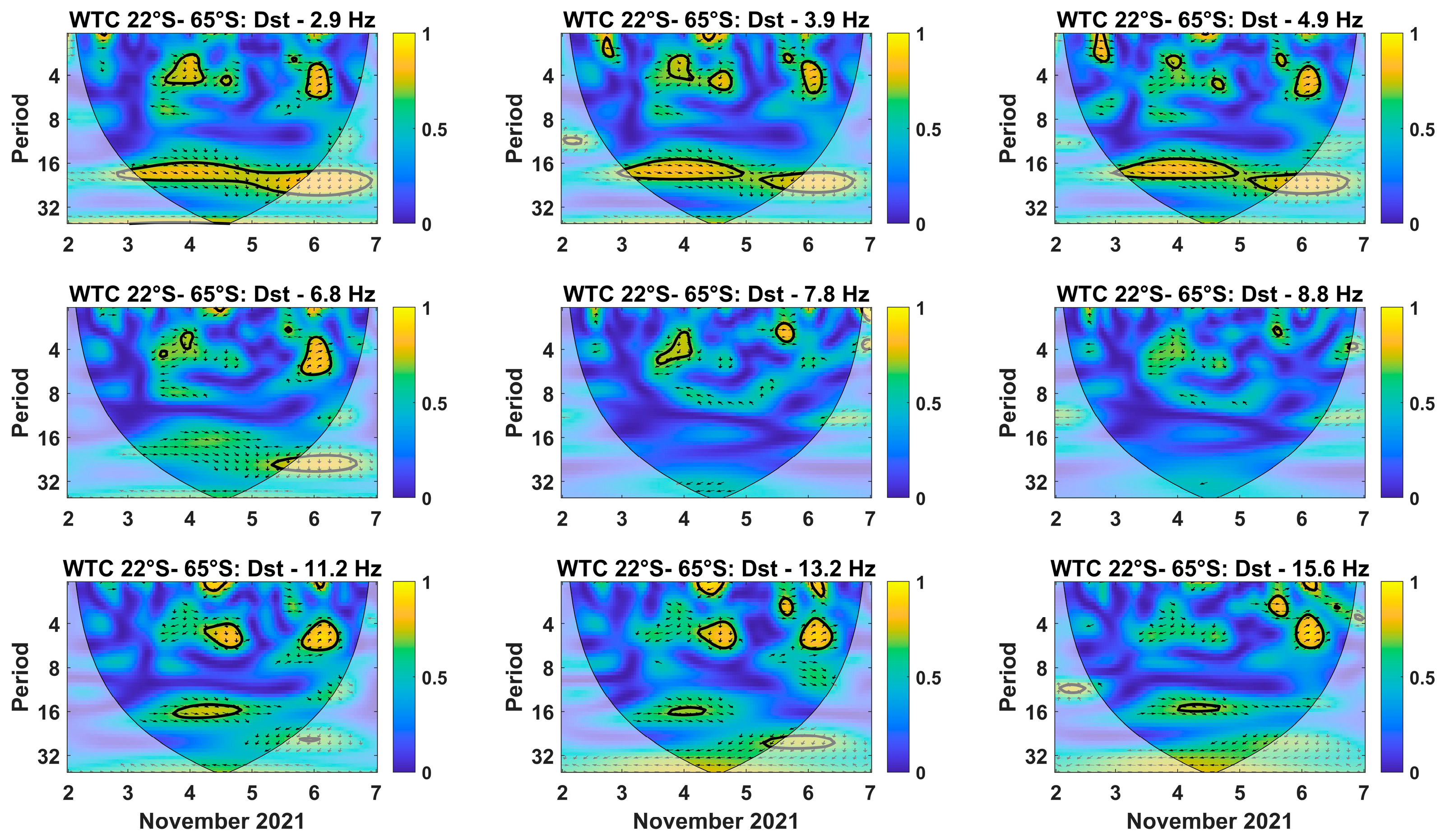
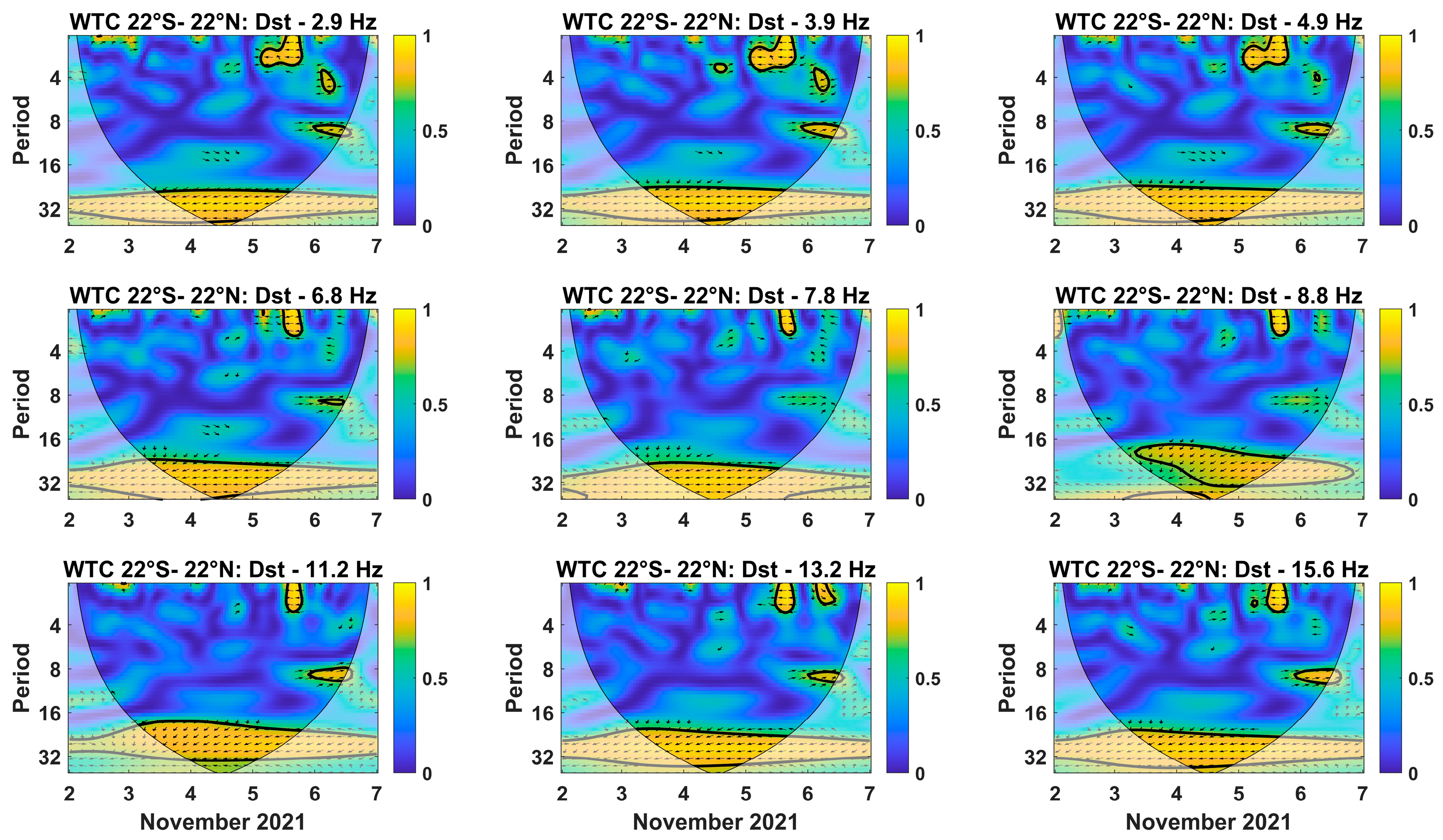
From the analysis above, it is clear that the ULF frequency band exhibits the strongest response to magnetic storms. To determine the correlation between different frequencies in the ULF band and magnetic storms, wavelet coherence analysis was performed on the Dst curves and D value curves at various frequencies. We obtained wavelet coherence spectrograms for the 22°S–65°S, 22°S–22°N, and 22°N–65°N regions. Spectrogram values approaching 1 indicate high-energy regions (yellow areas), which represent strong correlations.
In the wavelet coherence spectrograms of Dst versus D value curves for the 22°S–65°S range (
Figure 12), there are significantly more high-energy zones at 2.9 Hz, 3.9 Hz, and 4.9 Hz compared to other frequencies. High-energy regions are clearly observed near periods 4 and 16. Small, high-energy regions are noted at 11.2 Hz and 13.2 Hz (Schumann wave frequency point) near periods 4 and 16, while 8.8 Hz shows almost no high-energy regions. The frequencies 2.9 Hz, 3.9 Hz, and 4.9 Hz exhibit the strongest correlations with magnetic storms and are significantly perturbed by their effects. In contrast, at 8.8 Hz, the correlation with magnetic storms is very weak, indicating minimal impact from the storms.
In the 22°N–65°N range, high-energy regions remain more prominent at 2.9 Hz, 3.9 Hz, and 4.9 Hz compared to other frequencies (
Figure 13). The periods of these high-energy regions are approximately 4 and 16. In contrast to 22°S–65°S, 7.8 Hz and 13.2 Hz also exhibit distinct and prolonged high-energy regions at period 16. Since these two frequencies correspond to Schumann wave frequencies, Schumann waves are more affected by magnetic storm perturbations in the northern hemisphere compared to the southern hemisphere.
In the 22°S–22°N range, no high-energy regions are observed during the main phase of the magnetic storm, either at the 0–5 Hz frequency range or at the Schumann wave frequency (
Figure 14). The D value curves in this latitude range show a weak correlation with the Dst curves. The correlation between electric field disturbances and magnetic storms at mid and low latitudes is not significant, indicating that magnetic storms do not directly or significantly induce electric field disturbances in the ionosphere at these latitudes. In contrast, electric field disturbances at high latitudes are strongly correlated with magnetic storm activity.