Classical and Atomic Gravimetry
Abstract
1. Introduction
2. Classical Gravimetry
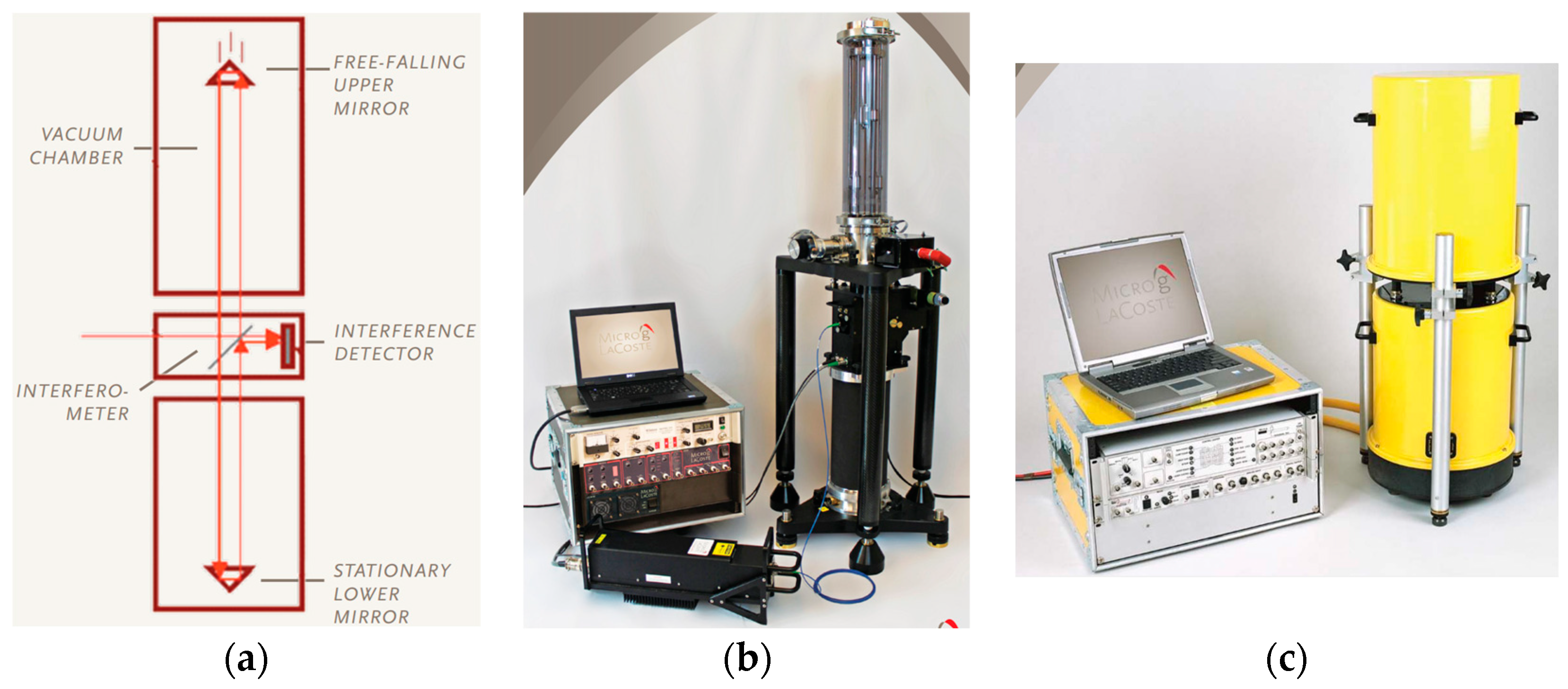
2.1. LI-Based Absolute Gravimeters
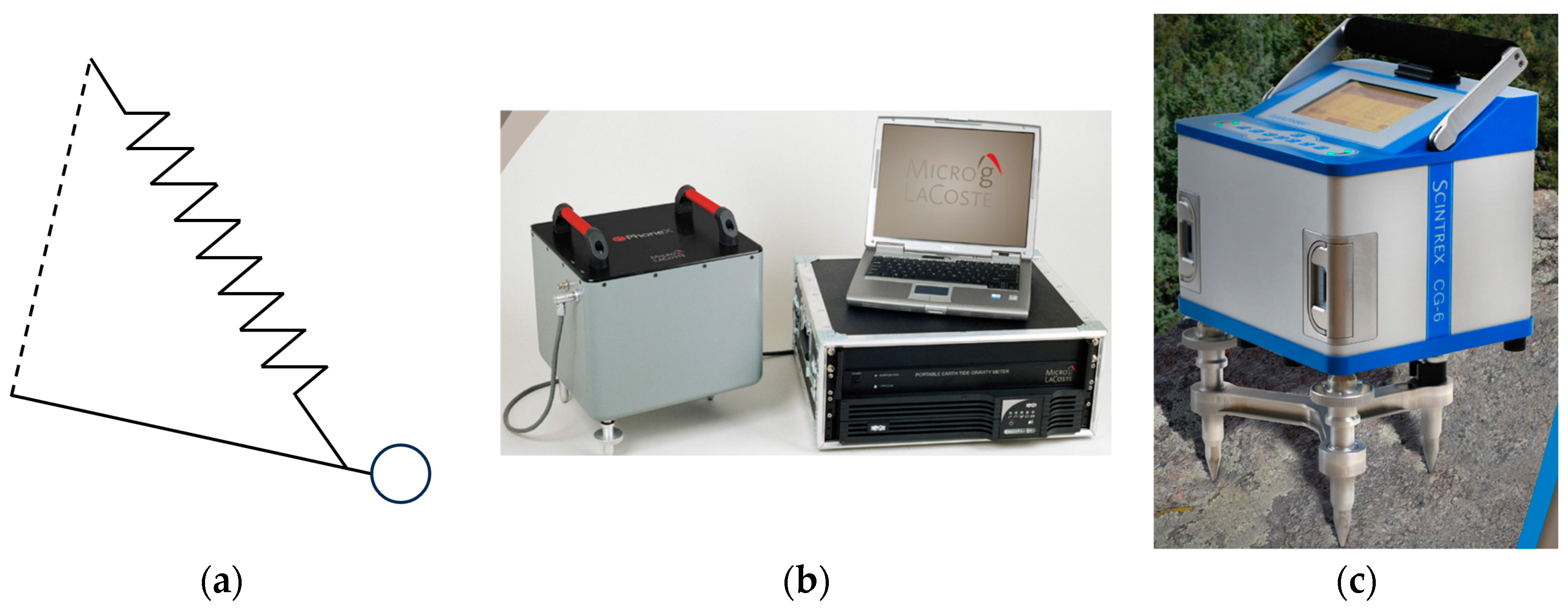
2.2. Spring Relative Gravimeters
2.3. Superconducting Gravimeters
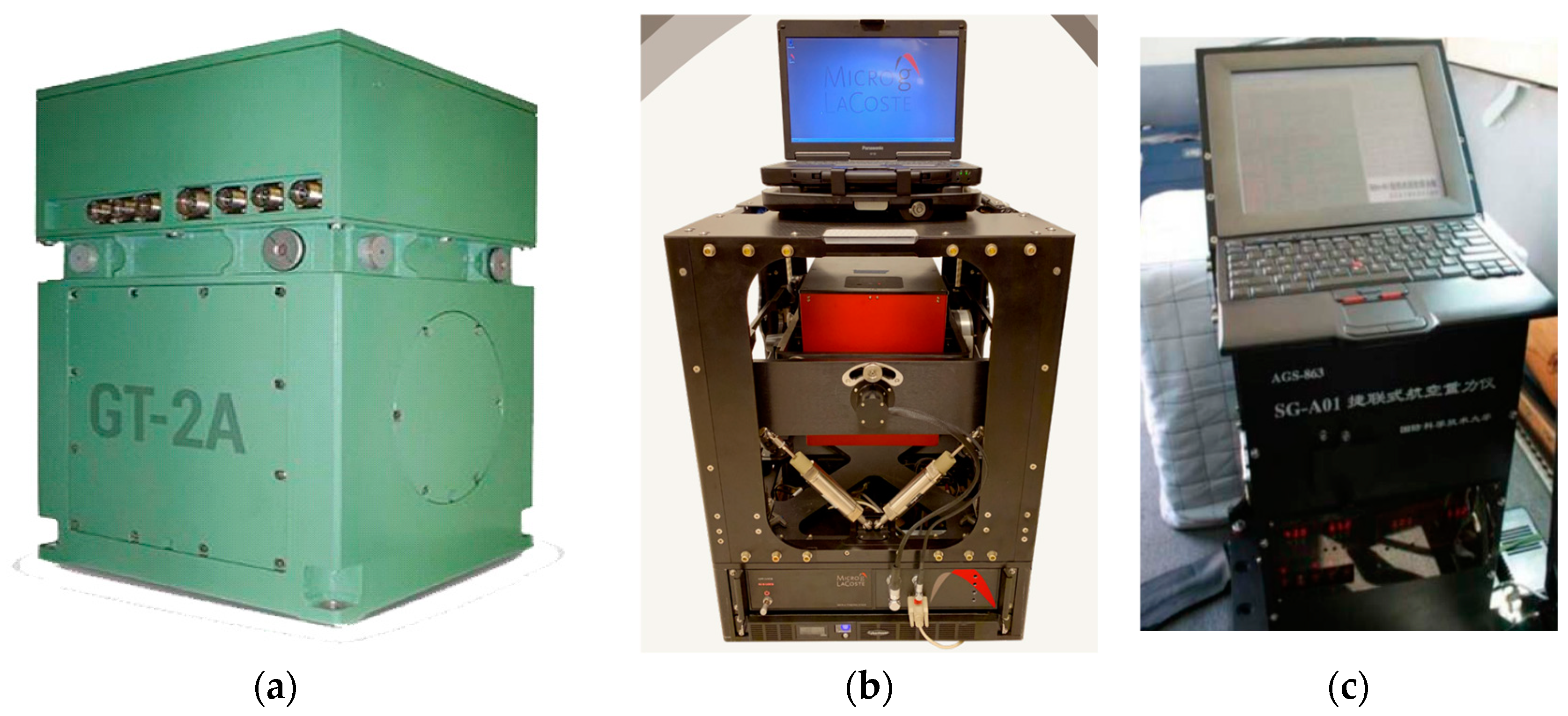
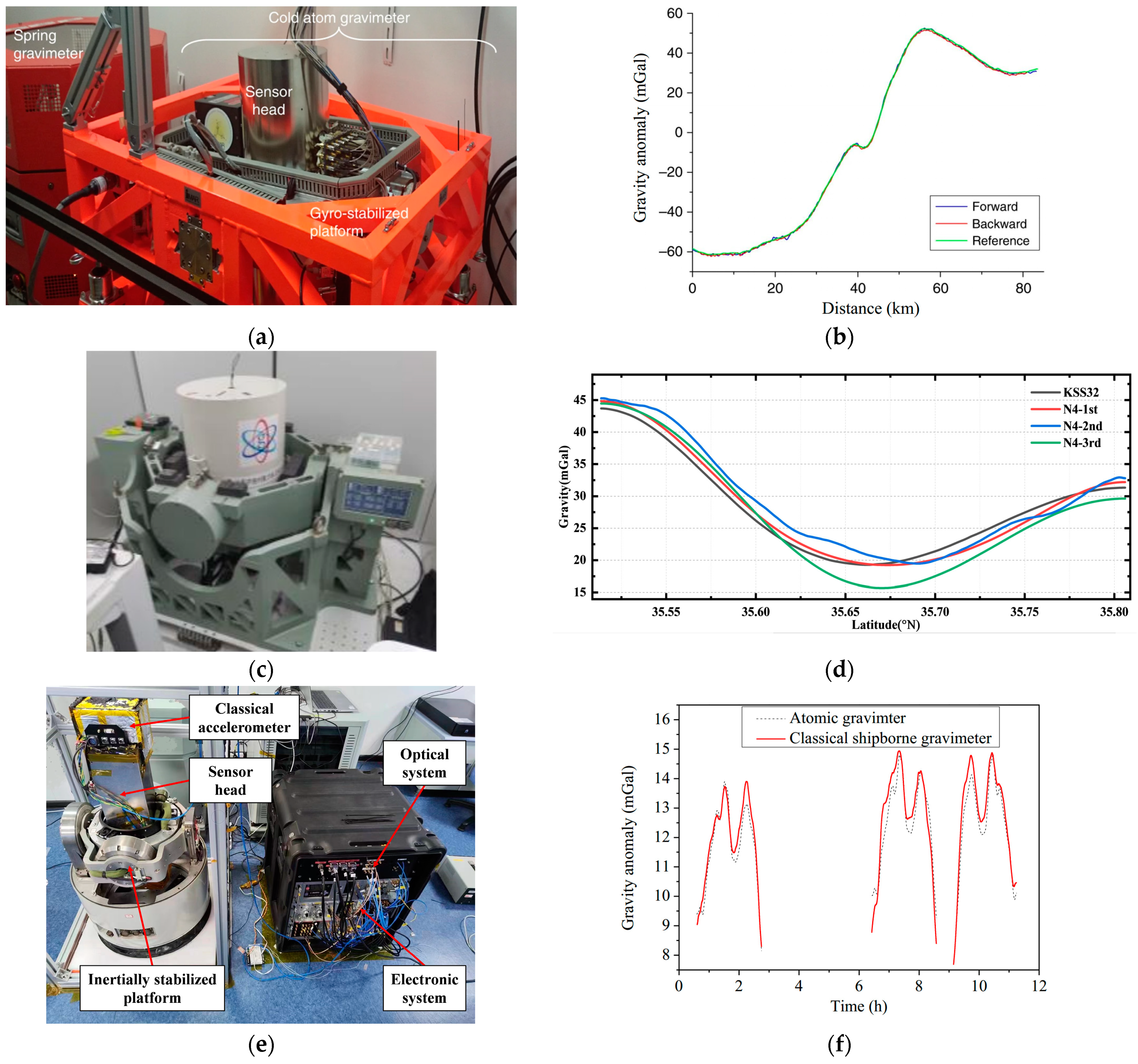
2.4. Airborne/Marine Gravimeters
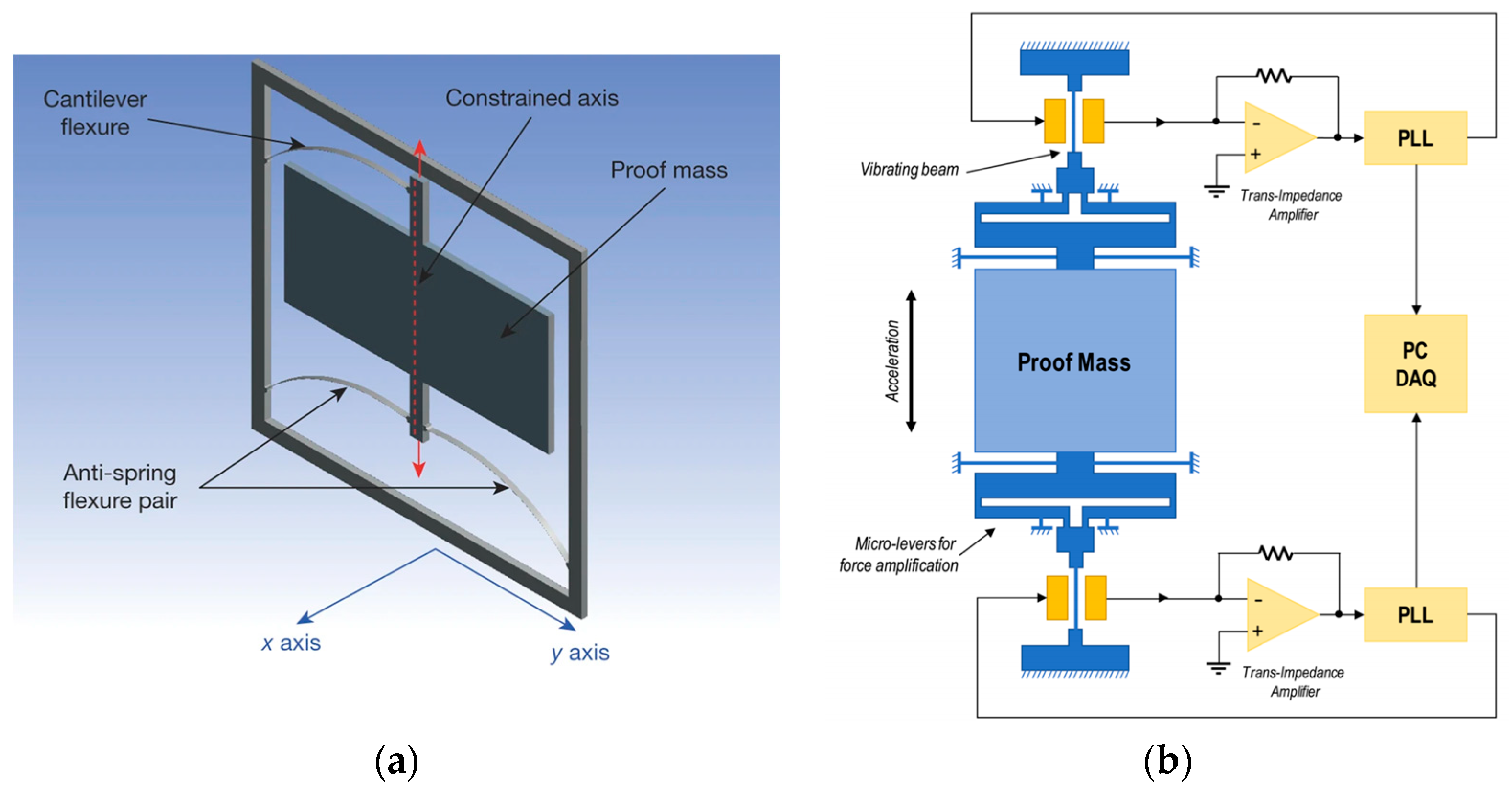
2.5. MEMS Gravimeters
2.6. Dedicated Gravity Satellite Missions
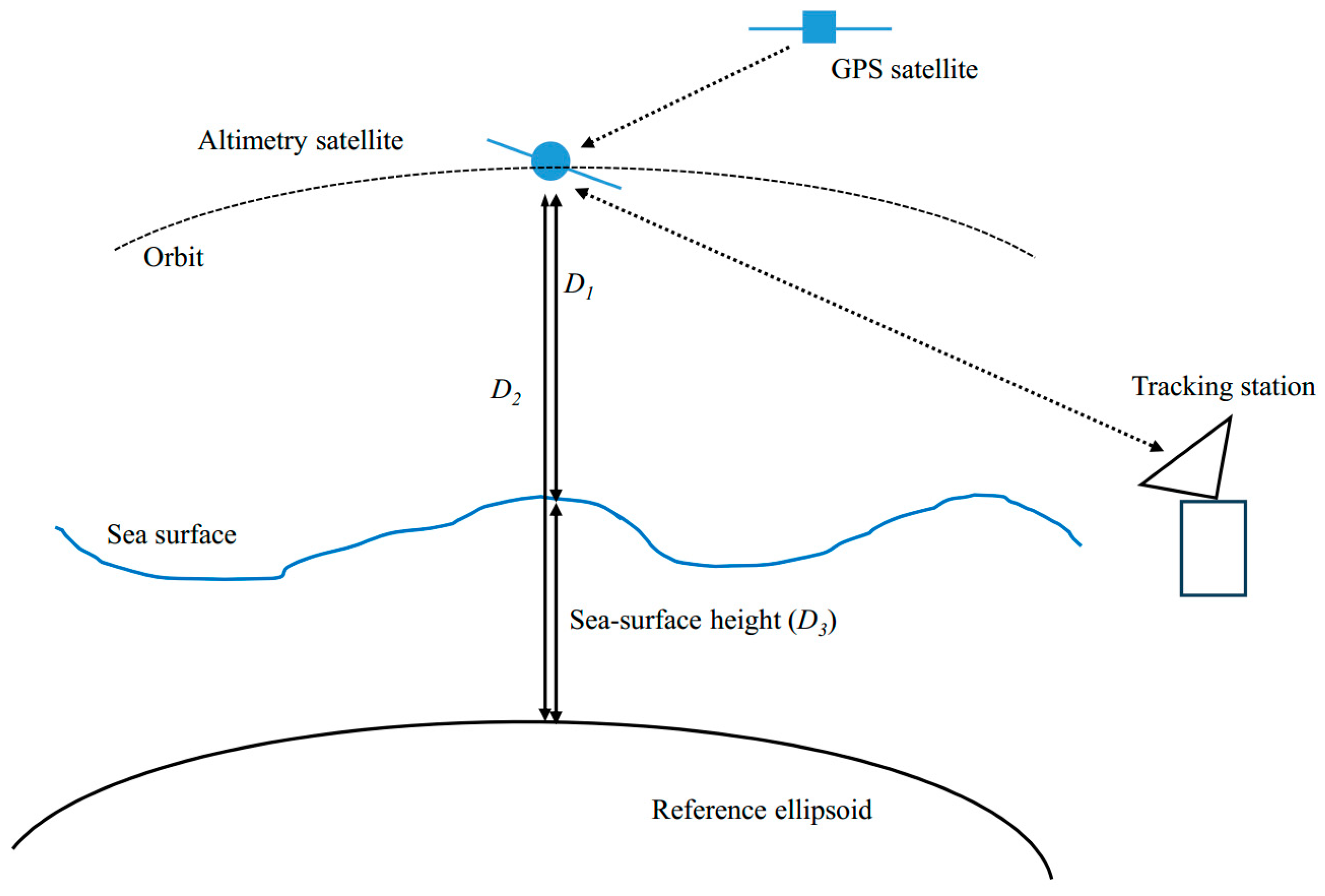
2.7. Satellite Altimetry
3. Atomic Gravimetry
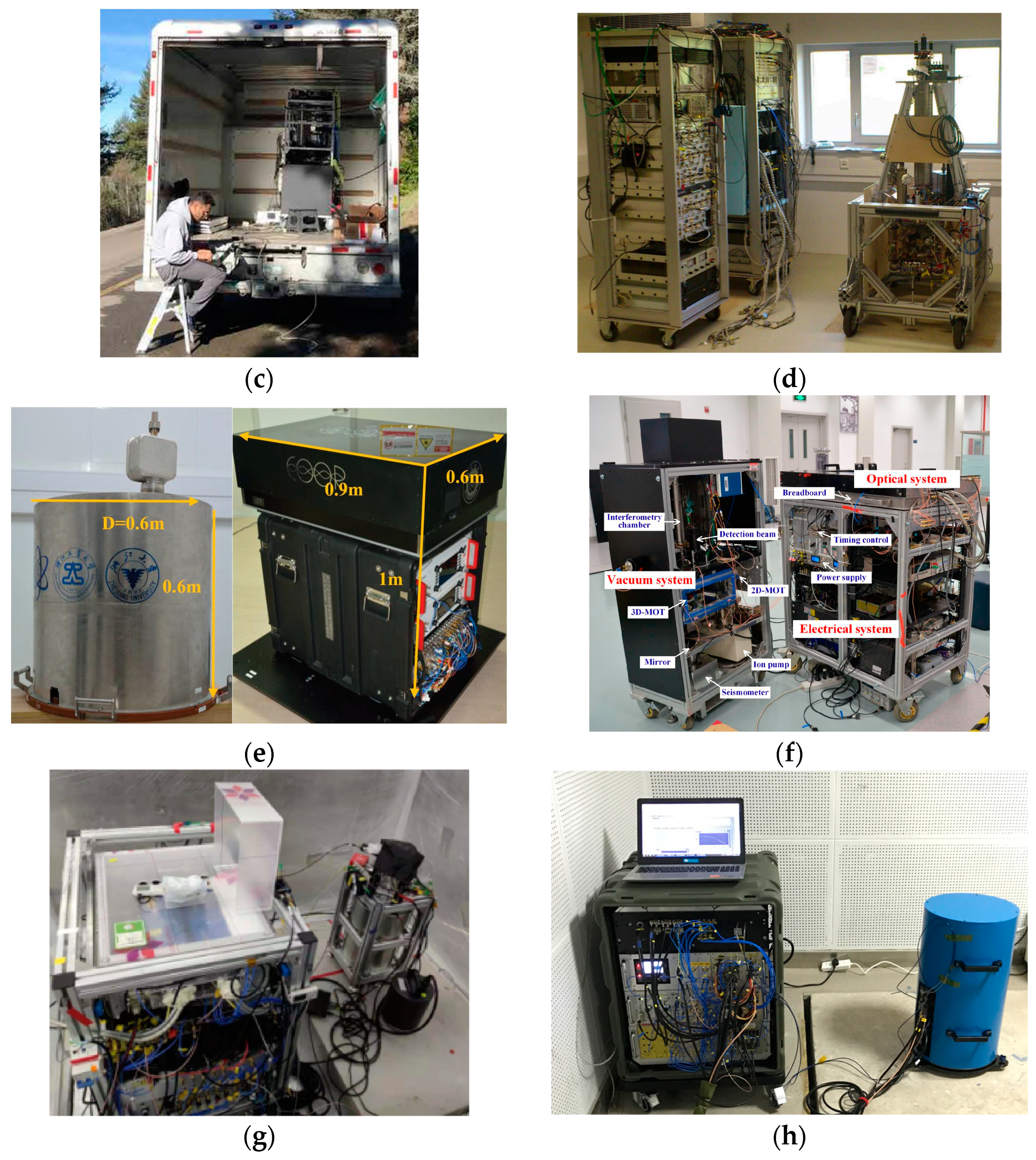
3.1. Static Atomic Gravimeters
3.2. Dynamic Atomic Gravimeters
3.3. Space Atom Interferometers
3.4. Atom Gravity Gradiometer
4. Comparisons between Classical and Atomic Gravimetry
5. Conclusions and Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sandwell, D.T.; Müller, R.D.; Smith, W.H.; Garcia, E.; Francis, R. New global marine gravity model from CryoSat-2 and Jason-1 reveals buried tectonic structure. Science 2014, 346, 65–67. [Google Scholar] [CrossRef]
- Davis, K.; Li, Y.G.; Batzle, M. Time-lapse gravity monitoring A systematic 4D approach with application to aquifer storage and recovery. Geophysics 2008, 73, WA61–WA69. [Google Scholar] [CrossRef]
- Wang, H.B.; Wu, L.; Chai, H.; Hsu, H.; Wang, Y. Technology of gravity aided inertial navigation system and its trial in South China Sea. IET Radar Sonar Navig. 2016, 10, 862–869. [Google Scholar] [CrossRef]
- Welker, T.C.; Pachter, M.; Huffman, R.E. Gravity gradiometer integrated inertial navigation. In Proceedings of the 2013 European Control Conference (ECC), Zurich, Switzerland, 17–19 July 2013. [Google Scholar]
- Abd El Gelil, M.; Pagiatakis, S.; El-Barrany, A. On the potential of least squares response method for the calibration of superconducting gravimeters. Int. J. Geophys. 2012, 11, 741729. [Google Scholar] [CrossRef]
- Fukuda, Y.; Okuno, J.; Doi, K.; Lee, C. Gravity observations at Jang Bogo Station, Antarctica, and scale factor calibrations of different relative gravimeters. Polar Sci. 2021, 29, 100702. [Google Scholar] [CrossRef]
- Pavlis, N.; Holmes, S.; Kenyon, S.; Factor, J. The development and evaluation of the Earth Gravitational Model 2008 (EGM2008). J. Geophys. Res. Solid Earth 2012, 117, B04406. [Google Scholar] [CrossRef]
- Kasevich, M.; CHU, S. Atomic interferometry using stimulated Raman transitions. Phys. Rev. Lett. 1991, 67, 181–184. [Google Scholar] [CrossRef] [PubMed]
- Peters, A.; Chung, K.Y.; Chu, S. Measurement of gravitational acceleration by dropping atoms. Nature 1999, 400, 849–852. [Google Scholar] [CrossRef]
- McGuirk, J.M.; Foster, G.T.; Fixler, J.B.; Snadden, M.J.; Kasevich, M.A. Sensitive absolute-gravity gradiometry using atom interferometry. Phys. Rev. A 2002, 65, 033608. [Google Scholar] [CrossRef]
- Gustavson, T.L.; Bouyer, P.; Kasevich, M.A. Precision rotation measurements with an atom interferometer gyroscope. Phys. Rev. Lett. 1997, 78, 2046–2049. [Google Scholar] [CrossRef]
- Weiss, D.; Young, B.; Chu, S. Precision measurement of ℏ/m Cs based on photon recoil using laser-cooled atoms and atomic interferometry. Appl. Phys. B 1994, 59, 217–256. [Google Scholar] [CrossRef]
- Yu, C.H.; Zhong, W.C.; Estey, B.; Kwan, J.; Parker, R.H.; Müller, H. Atom-interferometry measurement of the fine structure constant. Ann. Phys. 2019, 531, 1800346. [Google Scholar] [CrossRef]
- Fixler, J.B.; Foster, G.T.; McGuirk, J.M.; Kasevich, M.A. Atom interferometer measurement of the Newtonian constant of gravity. Science 2007, 315, 74–77. [Google Scholar] [CrossRef] [PubMed]
- Asenbaum, P.; Overstreet, C.; Kim, M.; Curti, J.; Kasevich, M.A. Atom-interferometric test of the equivalence principle at the 10−12 level. Phys. Rev. Lett. 2020, 125, 191101. [Google Scholar] [CrossRef] [PubMed]
- Zhou, L.; He, C.A.; Yan, S.T.; Chen, X.; Gao, D.F.; Duan, W.T.; Ji, Y.H.; Xu, R.D.; Tang, B.; Zhou, C.; et al. Joint mass-and-energy test of the equivalence principle at the 10−10 level using atoms with specified mass and internal energy. Phys. Rev. A 2021, 104, 022822. [Google Scholar] [CrossRef]
- Yu, N.; Tinto, M. Gravitational wave detection with single-laser atom interferometers. Gen. Relat. Gravit. 2011, 43, 1943–1952. [Google Scholar] [CrossRef]
- Dimopoulos, S.; Graham, P.W.; Hogan, J.M.; Kasevich, M.A.; Rajendran, S. Gravitational wave detection with atom interferometry. Phys. Lett. B 2009, 678, 37–40. [Google Scholar] [CrossRef]
- Gao, D.F.; Ju, P.; Zhang, B.; Zhan, M.S. Gravitational-wave detection with matter-wave interferometers based on standing light waves. Gen. Relativ. Grav. 2011, 43, 2027–2036. [Google Scholar] [CrossRef]
- Peters, A.; Chung, K.Y.; Chu, S. High-precision gravity measurements using atom interferometry. Metrologia 2001, 38, 25–61. [Google Scholar] [CrossRef]
- Freier, C.; Hauth, M.; Schkolnik, V.; Leykauf, B.; Schilling, M.; Wziontek, H.; Scherneck, H.G.; Müller, J.; Peters, A. Mobile quantum gravity sensor with unprecedented stability. J. Phys. Conf. Ser. 2016, 723, 012050. [Google Scholar] [CrossRef]
- Fang, B.; Dutta, I.; Gillot, P.; Savoie, D.; Lautier, J.; Cheng, B.; Garrido Alzar, C.L.; Geiger, R.; Merlet, S.; Dos Santos, F.P.; et al. Metrology with atom interferometry: Inertial sensors from laboratory to field applications. J. Phys. Conf. Ser. 2016, 723, 012049. [Google Scholar] [CrossRef]
- Zhang, T.; Chen, L.L.; Shu, Y.B.; Xu, W.J.; Cheng, Y.; Luo, Q.; Hu, Z.K.; Zhou, M.K. Ultrahigh-sensitivity Bragg atom gravimeter and its application in testing Lorentz violation. Phys. Rev. Appl. 2023, 20, 014067. [Google Scholar] [CrossRef]
- Guo, M.Y.; Bai, J.H.; Hu, D.; Tang, Z.M.; You, J.Q.; Chen, R.N.; Wang, Y. A vibration correction system for cold atom gravimeter. Meas. Sci. Technol. 2024, 35, 035011. [Google Scholar] [CrossRef]
- Xu, Y.Y.; Cui, J.F.; Qi, K.; Chen, L.L.; Deng, X.B.; Luo, Q.; Zhang, H.; Tan, Y.J.; Shao, C.G.; Zhou, M.K.; et al. Evaluation of the transportable atom gravimeter HUST-QG. Metrologia 2022, 59, 055001. [Google Scholar] [CrossRef]
- Li, C.Y.; Long, J.B.; Huang, M.Q.; Chen, B.; Yang, Y.M.; Jiang, X.; Xiang, C.F.; Ma, Z.L.; He, D.Q.; Chen, L.K.; et al. Continuous gravity measurement with a portable atom gravimeter. Phys. Rev. A 2023, 108, 032811. [Google Scholar] [CrossRef]
- Wang, S.K.; Zhao, Y.; Zhuang, W.; Li, T.C.; Wu, S.Q.; Feng, J.Y.; Li, C.J. Shift evaluation of the atomic gravimeter NIM-AGRb-1 and its comparison with FG5X. Metrologia 2018, 55, 360–365. [Google Scholar] [CrossRef]
- Bidel, Y.; Zahzam, N.; Blanchard, C.; Bonnin, A.; Cadoret, M.; Bresson, A.; Rouxel, D.; Lequentrec-Lalancette, M.F. Absolute marine gravimetry with matter-wave interferometry. Nat. Commun. 2018, 9, 627. [Google Scholar] [CrossRef]
- Bidel, Y.; Zahzam, N.; Bresson, A.; Blanchard, C.; Bonnin, A.; Bernard, J.; Cadoret, M.; Jensen, T.E.; Forsberg, R.; Salaun, C.; et al. Airborne absolute gravimetry with a quantum sensor comparison with classical technologies. JGR Solid Earth 2023, 128, e2022JB025921. [Google Scholar] [CrossRef]
- Janvier, C.; Ménoret, V.; Desruelle, B.; Merlet, S.; Landragin, A.; Dos Santos, F.P. Compact differential gravimeter at the quantum projection-noise limit. Phys. Rev. A 2022, 105, 022801. [Google Scholar] [CrossRef]
- Lyu, W.; Zhong, J.Q.; Zhang, X.W.; Liu, W.; Zhu, L.; Xu, W.H.; Chen, X.; Tang, B.; Wang, J.; Zhan, M.S. Compact high-resolution absolute-gravity gradiometer Based on atom interferometers. Phys. Rev. Appl. 2022, 18, 054091. [Google Scholar] [CrossRef]
- Biedermann, G.W.; Wu, X.; Deslauriers, L.; Roy, S.; Mahadeswaraswamy, C.; Kasevich, M.A. Testing gravity with cold-atom interferometers. Phys. Rev. A 2015, 91, 033629. [Google Scholar] [CrossRef]
- Becker, D.; Lachmann, M.D.; Seidel, S.T.; Ahlers, H.; Dinkelaker, A.N.; Grosse, J.; Hellmig, O.; Müntinga, H.; Schkolnik, V.; Wendrich, T.; et al. Space-borne Bose-Einstein condensation for precision interferometry. Nature 2018, 562, 391–395. [Google Scholar] [CrossRef] [PubMed]
- Lachmann, M.D.; Ahlers, H.; Becker, D.; Dinkelaker, A.N.; Grosse, J.; Hellmig, O.; Müntinga, H.; Schkolnik, V.; Seidel, S.T.; Wendrich, T.; et al. Ultracold atom interferometry in space. Nat. Commun. 2021, 12, 1317. [Google Scholar] [CrossRef] [PubMed]
- Elliott, E.R.; Krutzik, M.C.; Williams, J.R.; Thompson, R.J.; Aveline, D.C. NASA’s Cold Atom Lab (CAL): System development and ground test status. NPJ Microgravity 2018, 4, 16. [Google Scholar] [CrossRef]
- Elliott, E.R.; Aveline, D.C.; Bigelow, N.P.; Boegel, P.; Botsi, S.; Charron, E.; D’Incao, J.P.; Engels, P.; Estrampes, T.; Gaaloul, N.; et al. Quantum gas mixtures and dual-species atom interferometry in space. Nature 2023, 23, 502–508. [Google Scholar] [CrossRef] [PubMed]
- Williams, J.R.; Sackett, C.A.; Ahlers, H.; Aveline, D.C.; Boegel, P.; Botsi, S.; Charron, E.; Elliott, E.R.; Gaaloul, N.; Giese, E.; et al. Interferometry of atomic matter waves in the Cold Atom Lab onboard the International Space Station. arXiv 2024, arXiv:2402.14685v1. [Google Scholar]
- He, M.; Chen, X.; Fang, J.; Chen, Q.F.; Sun, H.Y.; Wang, Y.B.; Zhong, J.Q.; Zhou, L.; He, C.; Li, J.T.; et al. The space cold atom interferometer for testing the equivalence principle in the China Space Station. NPJ Microgravity 2023, 9, 58. [Google Scholar] [CrossRef] [PubMed]
- Li, J.T.; Chen, X.; Zhang, D.F.; Wang, W.Z.; Zhou, Y.; He, M.; Fang, J.; Zhou, L.; He, C.; Jiang, J.J.; et al. Realization of a cold atom gyroscope in space. arXiv 2024, arXiv:2405.20659v1. [Google Scholar]
- Milsom, J. The Hunt for Earth Gravity, 1st ed.; Springer: Cham, Switzerland, 2018; pp. 1–2. [Google Scholar]
- Faller, J.E. Precision Measurement of the Acceleration of Gravity: Measurements of g have always made maximum use of the available technology in measurement of length and time. Science 1967, 158, 60–67. [Google Scholar] [CrossRef]
- Faller, J.E.; Marson, I. Ballistic methods of measuring g-the direct free-fall and symmetrical rise-and-fall methods compared. Metrologia 1988, 25, 49–55. [Google Scholar] [CrossRef]
- Marson, I.; Faller, J.E. g -the acceleration of gravity: Its measurement and its importance. J. Phys. E Sci. Instrum. 1986, 19, 1–12. [Google Scholar] [CrossRef]
- Niebauer, T.M.; Sasagawa, G.S.; Faller, J.E.; Hilt, R.; Klopping, F. A new generation of absolute gravimeters. Metrologia 1995, 32, 159–180. [Google Scholar] [CrossRef]
- Niebauer, T.M. Treatise on Geophysics, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2015; pp. 1525–1546. [Google Scholar]
- FG5-X-Brochure. Available online: https://microglacoste.com/wp-content/uploads/2018/02/FG5-X-Brochure.pdf (accessed on 21 May 2024).
- Crossley, D.; Hinderer, J.; Riccardi, U. The measurement of surface gravity. Rep. Prog. Phys. 2013, 76, 046101. [Google Scholar] [CrossRef] [PubMed]
- Brochure-A10. Available online: https://microglacoste.com/wp-content/uploads/2017/03/Brochure-A10.pdf (accessed on 21 May 2024).
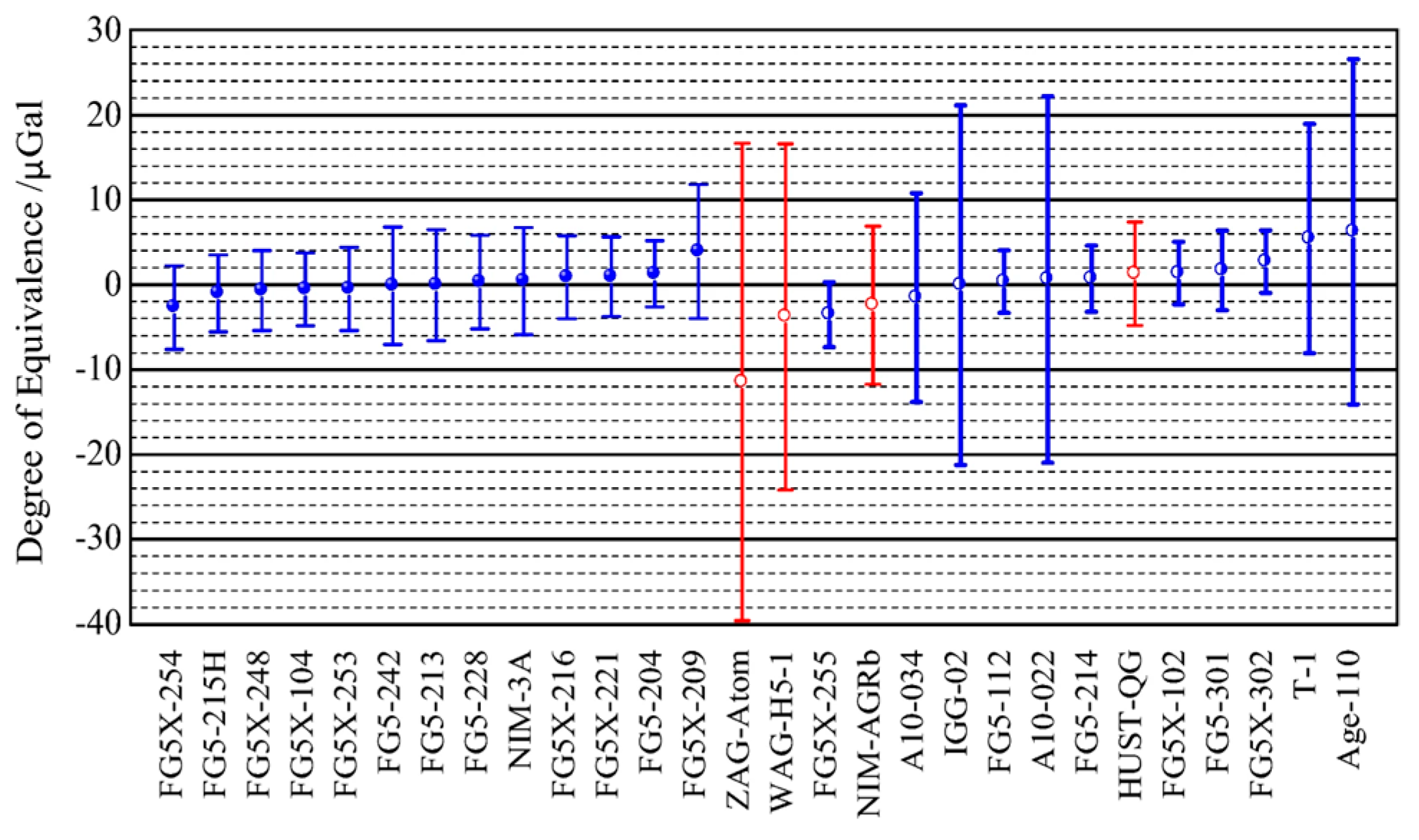
- Wu, S.Q.; Feng, J.Y.; Li, C.J.; Su, D.W.; Wang, Q.Y.; Hu, R.; Mou, L.S. The results of 10th International Comparison of Absolute Gravimeters (ICAG-2017). J. Geod. 2021, 95, 63. [Google Scholar] [CrossRef]
- gPhoneX-Brochure. Available online: https://microglacoste.com/wp-content/uploads/2017/03/MgL_gPhoneX-Brochure.pdf (accessed on 21 May 2024).
- CG-6-Brochure_R5. Available online: https://microglacoste.com/wp-content/uploads/2019/01/CG-6-Brochure_R5.pdf (accessed on 21 May 2024).
- Hugill, A. Scintrex CG-3 automated gravity meter: Description and field results. In Proceedings of the SEG Annual Meeting, San Francisco, CA, USA, 23–27 September 1990. [Google Scholar]
- Operating Principles of the Superconducting Gravity Meter. Available online: http://www.gwrinstruments.com/pdf/principles-of-operation.pdf (accessed on 21 May 2024).
- Goodkind, J.M. The superconducting gravimeter. Rev. Sci. Instrum. 1999, 70, 4131–4152. [Google Scholar] [CrossRef]
- Rosat, S.; Hinderer, J. Noise levels of superconducting gravimeters: Updated comparison and time stability. B Seismol. Soc. Am. 2011, 101, 1233–1241. [Google Scholar] [CrossRef]
- iGrav Brochure_Dec 2014 Rev 3.00. Available online: http://www.gwrinstruments.com/pdf/igrav-brochure.pdf (accessed on 21 May 2024).
- iGRAV® Gravity Sensors. Available online: http://www.gwrinstruments.com/igrav-gravity-sensors.html (accessed on 21 May 2024).
- Bidel, Y.; Zahzam, N.; Bresson, A.; Blanchard, C.; Cadoret, M.; Olesen, A.V.; Forsberg, R. Absolute airborne gravimetry with a cold atom sensor. J. Geod. 2020, 94, 20. [Google Scholar] [CrossRef]
- Torge, W.; Müller, J. Geodesy, 4th ed.; De Gruyter: Berlin, Germany, 2012; pp. 190–195. [Google Scholar]
- Xu, G.C. Sciences of Geodesy-I, 1st ed.; Springer: Berlin, Germany, 2010; pp. 83–85. [Google Scholar]
- Studinger, M.; Bell, R.; Frearson, N. Comparison of AIRGrav and GT-1A airborne gravimeters for research applications. Geophysics 2008, 73, I51–I61. [Google Scholar] [CrossRef]
- Yuan, Y.; Gao, J.Y.; Wu, Z.C.; Shen, Z.Y.; Wu, G.C. Performance estimate of some prototypes of inertial platform and strapdown marine gravimeters. Earth Planets Space 2020, 72, 89. [Google Scholar] [CrossRef]
- Jensen, T.E.; Olesen, A.V.; Forsberg, R.; Olsson, P.A.; Josefsson, Ö. New results from strapdown airborne gravimetry using temperature stabilisation. Remote Sens. 2019, 11, 2682. [Google Scholar] [CrossRef]
- GT-1A and GT-2A Specifications. Available online: http://canadianmicrogravity.com/pdf/GT_1A_and_GT_2A_Specifications_2012_06_01.pdf (accessed on 21 May 2024).
- SEA-III-Brochure-R12. Available online: https://microglacoste.com/wp-content/uploads/2020/07/SEA-III-Brochure-R12.pdf (accessed on 21 May 2024).
- Tu, H.B.; Liu, K.; Sun, H.; Cui, Q.; Yuan, Y.; Liu, S.J.; He, J.A.; Liu, L.T. Study on the CHZ-II Gravimeter and its calibrations along forward and reverse overlapping survey lines. Micromachines 2022, 13, 2124. [Google Scholar] [CrossRef] [PubMed]
- Huang, Y.M.; Olesen, A.V.; Wu, M.P.; Zhang, K.D. SGA-WZ: A new strapdown airborne gravimeter. Sensors 2012, 12, 9336–9348. [Google Scholar] [CrossRef] [PubMed]
- Middlemiss, R.; Samarelli, A.; Paul, D.; Rowan, S.; Hammond, G.D. Measurement of the Earth tides with a MEMS gravimeter. Nature 2016, 531, 614–617. [Google Scholar] [CrossRef] [PubMed]
- Tang, S.H.; Liu, H.F.; Yan, S.T.; Xu, X.C.; Wu, W.J.; Fan, J.; Liu, J.Q.; Hu, C.Y.; Tu, L.C. A high-sensitivity MEMS gravimeter with a large dynamic range. Microsyst. Nanoeng. 2019, 5, 45. [Google Scholar] [CrossRef] [PubMed]
- Xu, X.C.; Wang, Q.; Tian, J.A.; Yang, L.J.; Fang, Y.Y.; Wang, Q.; Zhao, C.; Hu, F.J.; Tu, L.C. On the air buoyancy effect in MEMS-based gravity sensors for high resolution gravity measurements. IEEE Sens. J. 2021, 21, 22480–22488. [Google Scholar] [CrossRef]
- Carbone, D.; Antoni-Micollier, L.; Hammond, G.; de Zeeuw–van Dalfsen, E.; Rivalta, E.; Bonadonna, C.; Messina, A.; Lautier-Gaud, J.; Toland, K.; Koymans, M.; et al. The Newton-g gravity imager: Towards new paradigms for terrain gravimetry. Front. Earth Sci. 2020, 8, 452. [Google Scholar] [CrossRef]
- Prasad, A.; Bramsiepc, S.G.; Middlemiss, R.P.; Hough, J.; Rowan, S.; Hammond, G.D.; Paul, D.J. A Portable MEMS Gravimeter for the Detection of the Earth Tides. In Proceedings of the IEEE Sensors, New Delhi, India, 28–31 October 2018. [Google Scholar]
- Pike, W.T.; Standley, I.M.; Calcutt, S.B.; Mukherjee, A.G. A broad-band silicon microseismometer with 0.25 NG/rtHz performance. In Proceedings of the 2018 IEEE Micro Electro Mechanical Systems (MEMS), Belfast, UK, 21–25 January 2018. [Google Scholar]
- Mustafazade, A.; Pandit, M.; Zhao, C.; Sobreviela, G.; Du, Z.; Steinmann, P.; Zou, X.; Howe, R.T.; Seshia, A.A. Vibrating beam MEMS accelerometer for gravity and seismic measurements. Sci. Rep. 2020, 10, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Fang, Z.; Yin, Y.; Chen, C.; Zhang, S.J.; Liu, Y.F.; Han, F.T. A sensitive micromachined resonant accelerometer for moving-base gravimetry. Sens. Actuators A 2020, 325, 112694. [Google Scholar] [CrossRef]
- GRAV10–High-Precision Gravimeter. Available online: https://silicong.com/images/Surface-Gravimeter.pdf (accessed on 22 June 2024).
- Liu, H.F.; Luo, Z.C.; Hu, Z.K.; Yang, S.Q.; Tu, L.C.; Zhou, Z.B.; Kraft, M. A review of high-performance MEMS sensors for resource exploration and geophysical applications. Petrol. Sci. 2022, 19, 2631e2648. [Google Scholar] [CrossRef]
- Reigber, C.; Balmino, G.; Schwintzer, P.; Biancale, R.; Bode, A.; Lemoine, J.-M.; König, R.; Loyer, S.; Neumayer, H.; Marty, J.-C.; et al. Global gravity field recovery using solely GPS tracking and accelerometer data from CHAMP. Space Sci. Rev. 2003, 108, 55–66. [Google Scholar] [CrossRef]
- Reigber, C. Gravity field recovery from satellite tracking data. In Proceedings of the International Summer School of Theoretical Geodes, Assisi, Italy, 23 May–3 June 1989. [Google Scholar]
- Tapley, B.D.; Bettadpur, S.; Watkins, M.; Reigber, C. The Gravity Recovery and Climate Experiment: Mission overview and early results. Geophys. Res. Lett. 2004, 31, 4. [Google Scholar] [CrossRef]
- Chen, J.; Cazenave, A.; Dahle, C.; Llovel, W.; Panet, I.; Pfeffer, J.; Moreira, L. Applications and challenges of GRACE and GRACE Follow-On satellite gravimetry. Surv. Geophys. 2022, 43, 305–345. [Google Scholar] [CrossRef] [PubMed]
- Pail, R.; Bruinsma, S.; Migliaccio, F.; Förste, C.; Goiginger, H.; Schuh, W.D.; Höck, E.; Reguzzoni, M.; Brockmann, J.M.; Abrikosov, O.; et al. First GOCE gravity field models derived by three different approaches. J. Geod. 2011, 85, 819–843. [Google Scholar] [CrossRef]
- Flechtner, F.; Reigber, C.; Rummel, R.; Balmino, G. Satellite gravimetry: A review of its realization. Surv. Geophys. 2021, 42, 1029–1074. [Google Scholar] [CrossRef] [PubMed]
- Van der Meijde, M.; Pail, R.; Bingham, R.; Floberghagen, R. GOCE data, models, and applications: A review. Int. J. Appl. Earth. Obs. 2015, 35, 4–15. [Google Scholar] [CrossRef]
- Sandwell, D.T.; Smith, W.H.F. Marine gravity anomaly from Geosat and ERS-1 satellite altimetry. J. Geophys. Res. Solid Earth 1997, 102, 10039–10054. [Google Scholar] [CrossRef]
- Vignudelli, S.; Kostianoy, A.G.; Cipollini, P.; Benveniste, J. Coastal Altimetry, 1st ed.; Springer: Heidelberg, Germany, 2011; pp. 104–108. [Google Scholar]
- Sandwell, D.T.; Smith, W.H.F. Global marine gravity from retracked Geosat and ERS-1 altimetry: Ridge segmentation versus spreading rate. J. Geophys. Res. Solid Earth 2009, 114, B01411. [Google Scholar] [CrossRef]
- Andersen, O.B.; Knudsen, P.; Berry, P.A.M. The DNSC08GRA global marine gravity field from double retracked satellite altimetry. J. Geod. 2010, 84, 191–199. [Google Scholar] [CrossRef]
- Sandwell, D.T.; Harper, H.; Tozer, B.; Smith, W.H.F. Gravity field recovery from geodetic altimeter missions. Adv. Space Res. 2021, 68, 1059–1072. [Google Scholar] [CrossRef]
- Sandwell, D.T.; Garcia, E.M.; Smith, W.H.; Soofi, K.; Wessel, P.; Francis, R. Toward 1-mGal accuracy in global marine gravity from CryoSat-2, Envisat, and Jason-1. Lead. Edge 2013, 32, 892–899. [Google Scholar] [CrossRef]
- Garcia, E.S.; Sandwell, D.T.; Smith, W.H.F. Retracking CryoSat-2, Envisat and Jason-1 radar altimetry wave forms for improve d gravity field recovery. Geophys. J. Int. 2014, 196, 1402–1422. [Google Scholar] [CrossRef]
- Kasevich, M.; Weiss, D.S.; Riis, E.K.; Kasapi, S.; Chu, S. Atomic velocity selection using stimulated Raman transitions. Phys. Rev. Lett. 1991, 66, 2297–2300. [Google Scholar] [CrossRef] [PubMed]
- Müller, H.; Chiow, S.W.; Long, Q.; Herrmann, S.; Chu, S. Atom interferometry with up to 24-photon-momentum-transfer beam splitters. Phys. Rev. Lett. 2008, 100, 180405. [Google Scholar] [CrossRef] [PubMed]
- Mazzoni, T.; Zhang, X.; Del Aguila, R.; Salvi, L.; Poli, N.; Tino, G.M. Large-momentum-transfer Bragg interferometer with strontium atoms. Phys. Rev. A 2015, 92, 053619. [Google Scholar] [CrossRef]
- Carnal, O.; Mlynek, J. Young’s double-slit experiment with atoms: A simple atom interferometer. Phys. Rev. Lett. 1991, 66, 2689–2692. [Google Scholar] [CrossRef] [PubMed]
- Rasel, E.M.; Oberthaler, M.K.; Batelaan, H.; Schmiedmayer, J.; Zeilinger, A. Atom wave interferometry with diffraction gratings of light. Phys. Rev. Lett. 1995, 75, 2633–2637. [Google Scholar] [CrossRef] [PubMed]
- Keith, D.W.; Ekstrom, C.R.; Turchette, Q.A.; Pritchard, D.E. An interferometer for atoms. Phys. Rev. Lett. 1991, 66, 2693–2696. [Google Scholar] [CrossRef] [PubMed]
- Berman Paul, R. Atom Interferometry, 1st ed.; Academic Press: San Diego, CA, USA, 1997; pp. 363–394. [Google Scholar]
- Clauser, J.F. Ultra-high sensitivity accelerometers and gyroscopes using neutral atom matter-wave interferometry. Physica B 1988, 151, 262–272. [Google Scholar] [CrossRef]
- Bodart, Q.; Merlet, S.; Malossi, N.; Dos Santos, F.P.; Bouyer, P.; Landragin, A. A cold atom pyramidal gravimeter with a single laser beam. Appl. Phys. Lett. 2010, 96, 134101. [Google Scholar] [CrossRef]
- Ménoret, V.; Vermeulen, P.; Le Moigne, N.; Bonvalot, S.; Bouyer, P.; Landragin, A.; Desruelle, B. Gravity measurements below 10(-9) g with a transportable absolute quantum gravimeter. Sci. Rep. 2018, 8, 12300. [Google Scholar] [CrossRef]
- Wu, X.J.; Pagel, Z.; Malek, B.S.; Nguyen, T.H.; Zi, F.; Scheirer, D.S.; Müller, H. Gravity surveys using a mobile atom interferometer. Sci. Adv. 2019, 5, eaax0800. [Google Scholar] [CrossRef] [PubMed]
- Merlet, S.; Volodimer, L.; Lours, M.; Dos Santos, F.P. A simple laser system for atom interferometry. Appl. Phys. B 2014, 117, 749–754. [Google Scholar] [CrossRef]
- Zhang, X.W.; Zhong, J.Q.; Tang, B.; Chen, X.; Zhu, L.; Huang, P.W.; Wang, J.; Zhan, M.S. Compact portable laser system for mobile cold atom gravimeters. Appl. Opt. 2018, 57, 6545–6551. [Google Scholar] [CrossRef]
- Schmidt, M.; Prevedelli, M.; Giorgini, A.; Tino, G.M.; Peters, A. A portable laser system for high-precision atom interferometry experiments. Appl. Phys. B 2011, 102, 11–18. [Google Scholar] [CrossRef]
- Schkolnik, V.; Hellmig, O.; Wenzlawski, A.; Grosse, J.; Kohfeldt, A.; Doeringshoff, K.; Wicht, A.; Windpassinger, P.; Sengstock, K.; Braxmaier, C.; et al. A compact and robust diode laser system for atom interferometry on a sounding rocket. Appl. Phys. B 2016, 122, 217. [Google Scholar] [CrossRef]
- Carraz, O.; Lienhart, F.; Charriere, R.; Cadoret, M.; Zahzam, N.; Bidel, Y.; Bresson, A. Compact and robust laser system for onboard atom interferometry. Appl. Phys. B 2009, 97, 405–411. [Google Scholar] [CrossRef]
- Wang, Q.Y.; Wang, Z.Y.; Fu, Z.J.; Liu, W.Y.; Lin, Q. A compact laser system for the cold atom gravimeter. Opt. Commun. 2016, 358, 82–87. [Google Scholar] [CrossRef]
- Thorpe, J.I.; Numata, K.; Livas, J. Laser frequency stabilization and control through offset sideband locking to optical cavities. Opt. Express 2008, 16, 15980–15990. [Google Scholar] [CrossRef]
- Theron, F.; Carraz, O.; Renon, G.; Zahzam, N.; Bidel, Y.; Cadoret, M.; Bresson, A. Narrow linewidth single laser source system for onboard atom interferometry. Appl. Phys. B 2015, 118, 1–5. [Google Scholar] [CrossRef]
- Chiow, S.W.; Yu, N. Compact atom interferometer using single laser. Appl. Phys. B 2018, 124, 96. [Google Scholar] [CrossRef]
- Fang, J.; Hu, J.G.; Chen, X.; Zhu, H.R.; Zhou, L.; Zhong, J.Q.; Wang, J.; Zhan, M.S. Realization of a compact one-seed laser system for atom interferometer-based gravimeters. Opt. Express 2018, 26, 1586–1596. [Google Scholar] [CrossRef]
- Malek, B.S.; Pagel, Z.; Wu, X.J.; Müller, H. Embedded control system for mobile atom interferometers. Rev. Sci. Instrum. 2019, 90, 073103. [Google Scholar] [CrossRef]
- Farah, T.; Guerlin, C.; Landragin, A.; Bouyer, P.; Gaffet, S.; Dos Santos, F.P.; Merlet, S. Underground operation at best sensitivity of the mobile LNE-SYRTE cold atom gravimeter. Gyrosc. Navig. 2014, 5, 266–274. [Google Scholar] [CrossRef]
- Hauth, M.; Freier, C.; Schkolnik, V.; Peters, A.; Wziontek, H.; Schilling, M. Atom interferometry for absolute measurements of local gravity. In Proceedings of the International School of Physics “Enrico Fermi” Course 188 on Atom Interferometry, Varenna, Italy, 15–20 July 2013. [Google Scholar]
- Fu, Z.J.; Wu, B.; Cheng, B.; Zhou, Y.; Weng, K.X.; Zhu, D.; Wang, Z.Y.; Lin, Q. A new type of compact gravimeter for long-term absolute gravity monitoring. Metrologia 2019, 56, 025001. [Google Scholar] [CrossRef]
- Ge, G.G.; Chen, X.; Li, J.T.; Zhang, D.F.; He, M.; Wang, W.Z.; Zhou, Y.; Zhong, J.Q.; Tang, B.; Fang, J.; et al. Accuracy improvement of a compact 85Rb atom gravimeter by suppressing laser crosstalk and light Shift. Sensors 2023, 23, 6115. [Google Scholar] [CrossRef] [PubMed]
- Biedermann, G.W.; Wu, X.; Deslauriers, L.; Takase, K.; Kasevich, M.A. Low-noise simultaneous fluorescence detection of two atomic states. Opt. Lett. 2009, 34, 347–349. [Google Scholar] [CrossRef] [PubMed]
- Song, H.W.; Zhong, J.Q.; Chen, X.; Zhu, L.; Wang, Y.P.; Wang, J.; Zhan, M.S. Normalized detection by using the blow-away signal in cold atom interferometry. Opt. Express 2017, 24, 28392–28399. [Google Scholar] [CrossRef]
- Le Gouet, J.; Mehlstaeubler, T.E.; Kim, J.; Merlet, S.; Clairon, A.; Landragin, A.; Dos Santos, F.P. Limits to the sensitivity of a low noise compact atomic gravimeter. Appl. Phys. B 2008, 92, 133–144. [Google Scholar] [CrossRef]
- Hu, Z.K.; Sun, B.L.; Duan, X.C.; Zhou, M.K.; Chen, L.L.; Zhan, S.; Zhang, Q.Z.; Luo, J. Demonstration of an ultrahigh-sensitivity atom-interferometry absolute gravimeter. Phys. Rev. A 2013, 88, 043610. [Google Scholar] [CrossRef]
- Zhou, L.; Xiong, Z.Y.; Yang, W.; Tang, B.; Peng, W.C.; Wang, Y.B.; Xu, P.; Wang, J.; Zhan, M.S. Measurement of Local Gravity via a Cold Atom Interferometer. Chin. Phys. Lett. 2011, 28, 013701. [Google Scholar] [CrossRef]
- Zhu, L.X.; Lien, Y.H.; Hinton, A.; Niggebaum, A.; Rammeloo, C.; Bongs, K.; Holynski, M. Application of optical single-sideband laser in Raman atom interferometry. Opt. Express 2018, 26, 6542–6553. [Google Scholar] [CrossRef] [PubMed]
- Merlet, S.; Le Goueet, J.; Bodart, Q.; Clairon, A.; Landragin, A.; Dos Santos, F.P.; Rouchon, P. Operating an atom interferometer beyond its linear range. Metrologia 2009, 46, 87–94. [Google Scholar] [CrossRef]
- Wu, K.; Li, G.; Hu, H.; Wang, L.J. Active low-frequency vertical vibration isolation system for precision measurements. Chin. J. Mech. Eng. 2017, 30, 164–169. [Google Scholar] [CrossRef]
- Tang, B.; Zhou, L.; Xiong, Z.Y.; Wang, J.; Zhan, M.S. A programmable broadband low frequency active vibration isolation system for atom interferometry. Rev. Sci. Instrum. 2014, 85, 093109. [Google Scholar] [CrossRef] [PubMed]
- Freier, C. Measurement of Local Gravity Using Atom Interferometry. Ph.D. Thesis, University of Humboldt, Berlin, Germany, 2010. [Google Scholar]
- Ruan, C.J.; Zhuang, W.; Yao, J.M.; Zhao, Y.; Ma, Z.H.; Yi, C.; Tian, Q.; Wu, S.Q.; Fang, F.; Wen, Y.H. A transportable atomic gravimeter with constraint-structured active vibration isolation. Sensors 2024, 24, 2395. [Google Scholar] [CrossRef] [PubMed]
- Gong, W.B.; Li, A.; Ma, J.X.; Wu, P.F.; Qin, F.J. An Ultralow-Frequency Vertical Isolation System Based on Composite Feedforward and Feedback Control. IEEE Sens. J. 2023, 23, 29109–29118. [Google Scholar] [CrossRef]
- Zhou, Y.; Luo, D.Y.; Wu, B.; Cheng, B.; Lin, Q. Active vibration isolation system based on the LADRC algorithm for atom interferometry. Appl. Opt. 2020, 59, 3487–3493. [Google Scholar] [CrossRef] [PubMed]
- Chen, B.; Long, J.B.; Xie, H.T.; Chen, L.K.; Chen, S. A mobile three-dimensional active vibration isolator and its application to cold atom interferometry. Acta Phys. Sin. 2019, 68, 183301. [Google Scholar] [CrossRef]
- Louchet-Chauvet, A.; Farah, T.; Bodart, Q.; Clairon, A.; Landragin, A.; Merlet, S.; Dos Santos, F.P. The influence of transverse motion within an atomic gravimeter. New J. Phys. 2011, 13, 065025. [Google Scholar] [CrossRef]
- Gauguet, A.; Mehlstaubler, T.E.; Leveque, T.; Le Gouet, J.; Chaibi, W.; Canuel, B.; Clairon, A.; Dos Santos, F.P.; Landragin, A. Off-resonant Raman transition impact in an atom interferometer. Phys. Rev. A 2008, 78, 043615. [Google Scholar] [CrossRef]
- Lan, S.Y.; Kuan, P.C.; Estey, B.; Haslinger, P.; Müller, H. Influence of the Coriolis force in atom interferometry. Phys. Rev. Lett. 2012, 108, 090402. [Google Scholar] [CrossRef]
- Xie, H.T.; Chen, B.; Long, J.B.; Xue, C.; Chen, L.K.; Chen, S. Calibration of a compact absolute atomic gravimeter. Chin. Phys. B 2020, 29, 093701. [Google Scholar] [CrossRef]
- Xu, Y.Y.; Cui, J.F.; Qi, K.; Deng, X.B.; Zhou, M.K.; Duan, X.C.; Hu, Z.K. On-site calibration of the Raman laser absolute frequency for atom gravimeters. Phys. Rev. A 2018, 97, 063626. [Google Scholar] [CrossRef]
- Carraz, O.; Charrière, R.; Cadoret, M.; Zahzam, N.; Bidel, Y.; Bresson, A. Phase shift in an atom interferometer induced by the additional laser lines of a Raman laser generated by modulation. Phys. Rev. A 2012, 86, 033605. [Google Scholar] [CrossRef]
- Luo, Q.; Zhou, H.; Chen, L.L.; Duan, X.C.; Zhou, M.K.; Hu, Z.K. Eliminating the phase shifts arising from additional sidebands in an atom gravimeter with a phase-modulated Raman laser. Opt. Lett. 2022, 47, 114–117. [Google Scholar] [CrossRef]
- Jiang, Z.; Palinkas, V.; Arias, F.E.; Liard, J.; Merlet, S.; Wilmes, H.; Vitushkin, L.; Robertsson, L.; Tisserand, L.; Dos Santos, F.P.; et al. The 8th International Comparison of Absolute Gravimeters 2009: The first Key Comparison (CCM.G-K1) in the field of absolute gravimetry. Metrologia 2012, 49, 666–684. [Google Scholar] [CrossRef]
- Cooke, A.; Champollion, C.; Le Moigne, N. First evaluation of an absolute quantum gravimeter (AQG#B01) for future field experiments. Geosci. Instrum. Method. Data Syst. 2021, 10, 65–79. [Google Scholar]
- Shettell, N.; Lee, K.S.; Oon, F.E.; Maksimova, E.; Hufnagel, C.; Wei, S.J.; Dumke, R. Geophysical survey based on hybrid gravimetry using relative measurements and an atomic gravimeter as an absolute reference. Sci. Rep. 2024, 14, 6511. [Google Scholar] [CrossRef] [PubMed]
- Bidel, Y.; Crraz, O.; Charrière, R.; Cadoret, M.; Zahzam, N.; Bresson, A. Compact cold atom gravimeter for field applications. Appl. Phys. Lett. 2013, 102, 144107. [Google Scholar] [CrossRef]
- Huang, P.W.; Tang, B.; Chen, X.; Zhong, J.Q.; Xiong, Z.Y.; Zhou, L.; Wang, J.; Zhan, M.S. Accuracy and stability evaluation of the 85Rb atom gravimeter WAG-H5-1 at the 2017 International Comparison of Absolute Gravimeters. Metrologia 2019, 56, 045012. [Google Scholar] [CrossRef]
- Geiger, R.; Ménoret, V.; Stern, G.; Zahzam, N.; Cheinet, P.; Battelier, B.; Villing, A.; Moron, F.; Lours, M.; Bidel, Y.; et al. Detecting inertial effects with airborne matter-wave interferometry. Nat. Commun. 2011, 2, 474. [Google Scholar] [CrossRef] [PubMed]
- Lautier, J.; Volodimer, L.; Hardin, T.; Merlet, S.; Lours, M.; Dos Santos, F.P.; Landragin, A. Hybridizing matter-wave and classical accelerometers. Appl. Phys. Lett. 2014, 105, 144102. [Google Scholar] [CrossRef]
- Qiao, Z.K.; Yuan, P.; Zhang, J.J.; Zhang, Z.Y.; Li, L.L.; Zhu, D.; Jiang, M.R.; Shi, H.Y.; Hu, R.; Zhou, F.; et al. Error analysis and filtering methods for absolute ocean gravity data. IEEE Sens. J. 2023, 23, 14346–14355. [Google Scholar] [CrossRef]
- Wu, B.; Zhang, C.; Wang, K.N.; Cheng, B.; Zhu, D.; Li, R.; Wang, X.L.; Lin, Q.; Qiao, Z.K.; Zhou, Y. Marine absolute gravity field surveys based on cold atomic gravimeter. IEEE Sens. J. 2023, 23, 24292–24299. [Google Scholar] [CrossRef]
- Zhou, Y.; Wang, W.Z.; Ge, G.G.; Li, J.T.; Zhang, D.F.; He, M.; Tang, B.; Zhong, J.Q.; Zhou, L.; Li, R.B.; et al. High-precision atom interferometer-based dynamic gravimeter measurement by eliminating the cross-coupling effect. Sensors 2024, 24, 1016. [Google Scholar] [CrossRef]
- Cheiney, P.; Fouché, L.; Templier, S.; Napolitano, F.; Battelier, B.; Bouyer, P.; Barrett, B. Navigation-compatible hybrid quantum accelerometer using a Kalman filter. Phys. Rev. Appl. 2018, 10, 034030. [Google Scholar] [CrossRef]
- Huang, C.F.; Li, A.; Qin, F.J.; Fang, J.; Chen, X. An atomic gravimeter dynamic measurement method based on Kalman filter. Meas. Sci. Technol. 2023, 34, 015013. [Google Scholar] [CrossRef]
- Zhu, D.; Xu, H.; Zhou, Y.; Wu, B.; Cheng, B.; Wang, K.N.; Chen, P.J.; Gao, S.T.; Weng, K.X.; Wang, H.L.; et al. Data processing of shipborne absolute gravity measurement based on the extended Kalman fiter algorithm. Acta Phys. Sin. 2022, 71, 133702. [Google Scholar] [CrossRef]
- Yankelev, D.; Avinadav, C.; Davidson, N.; Firstenberg, O. Atom interferometry with thousand-fold increase in dynamic range. Sci. Adv. 2020, 6, eabd0650. [Google Scholar] [CrossRef]
- Vu, D.T.; Bonvalot, S.; Seoane, L.; Gabalda, G.; Remy, D.; Bruinsma, S.; Bidel, Y.; Bresson, A.; Zahzam, N.; Rouxel, D.; et al. Potential of cold-atom airborne gravimetry to improve coastal gravity field and quasigeoid modelling. J. Geod. 2024, 98, 28. [Google Scholar] [CrossRef]
- Guo, J.; Ma, S.Q.; Zhou, C.; Liu, J.X.; Wang, B.; Pan, D.B.; Mao, H.C. Vibration compensation for a vehicle-mounted atom gravimeter. IEEE Sens. J. 2022, 22, 12939–12946. [Google Scholar] [CrossRef]
- Struckmann, C.; Corgier, R.; Loriani, S.; Kleinsteinberg, G.; Gox, N.; Giese, E.; Métris, G.; Gaaloul, N.; Wolf, P. Platform and environment requirements of a satellite quantum test of the Weak Equivalence Principle at the 10−17 level. Phys. Rev. D 2023, 109, 064010. [Google Scholar] [CrossRef]
- Hu, J.G.; Chen, X.; Fang, J.; Zhou, L.; Zhong, J.Q.; Wang, J.; Zhan, M.S. Analysis and suppression of wave-front-aberration phase noise in weak-equivalence-principle tests using dual-species atom interferometers. Phys. Rev. A 2017, 96, 023618. [Google Scholar] [CrossRef]
- Hu, J.G.; Chen, X.; Wang, L.Y.; Liao, Q.H.; Wang, Q.N. Systematic error suppression scheme of the weak equivalence principle test by dual atom interferometers in space based on spectral correlation. Chin. Phys. B 2020, 29, 110305. [Google Scholar] [CrossRef]
- Snadden, M.J.; McGuirk, J.M.; Bouyer, P.; Haritos, K.G.; Kasevich, M.A. Measurement of the Earth’s gravity gradient with an atom interferometer-based gravity gradiometer. Phys. Rev. Lett. 1998, 81, 971–974. [Google Scholar] [CrossRef]
- Mahadeswaraswamy, C. Atom Interferometric Gravity Gradiometer: Disturbance Compensation and Mobile Gradiometry. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 2009. [Google Scholar]
- Sorrentino, F.; Bodart, Q.; Cacciapuoti, L.; Lien, Y.H.; Prevedelli, M.; Rosi, G.; Salvi, L.; Tino, G.M. Sensitivity limits of a Raman atom interferometer as a gravity gradiometer. Phys. Rev. A 2014, 89, 023607. [Google Scholar] [CrossRef]
- Rosi, G.; Sorrentino, F.; Cacciapuoti, L.; Prevedelli, M.; Tino, G.M. Precision measurement of the Newtonian gravitational constant using cold atoms. Nature 2014, 510, 518–521. [Google Scholar] [CrossRef] [PubMed]
- D’Amico, G.; Borselli, F.; Cacciapuoti, L.; Prevedelli, M.; Rosi, G.; Sorrentino, F.; Tino, G.M. Bragg interferometer for gravity gradient measurements. Phys. Rev. A 2016, 93, 063628. [Google Scholar] [CrossRef]
- Mao, D.K.; Deng, X.B.; Luo, H.Q.; Xu, Y.Y.; Zhou, M.K.; Duan, X.C.; Hu, Z.K. A dual-magneto-optical-trap atom gravity gradiometer for determining the Newtonian gravitational constant. Rev. Sci. Instrum. 2021, 92, 053202. [Google Scholar] [CrossRef]
- Stray, B.; Lamb, A.; Kaushik, A.; Vovrosh, J.; Rodgers, A.; Winch, J.; Hayati, F.; Boddice, D.; Stabrawa, A.; Niggebaum, A.; et al. Quantum sensing for gravity cartography. Nature 2022, 602, 590–594. [Google Scholar] [CrossRef]
- Perrin, I.; Bidel, Y.; Zahzam, N.; Blanchard, C.; Bresson, A.; Cadoret, M. Proof-of-principle demonstration of vertical-gravity-gradient measurement using a single-proof-mass double-loop atom interferometer. Phys. Rev. A 2019, 99, 013601. [Google Scholar] [CrossRef]
- Yang, G.D.; Weng, K.X.; Wu, B.; Cheng, B.; Lin, Q. Research progress of quantum gravity gradiometer. Navig. Position. Timing 2021, 8, 20–29. [Google Scholar]
- Carraz, O.; Siemes, C.; Massotti, L.; Haagmans, R.; Silvestrin, P. A spaceborne gravity gradiometer concept based on cold atom interferometers for measuring Earth’s gravity field. Meas. Sci. Technol. 2014, 26, 139–145. [Google Scholar] [CrossRef]
- Trimeche, A.; Battelier, B.; Becker, D.; Bertoldi, A.; Bouyer, P.; Braxmaier, C.; Charron, E.; Corgier, R.; Cornelius, M.; Douch, K.; et al. Concept study and preliminary design of a cold atom interferometer for space gravity gradiometry. Class. Quantum Gravity 2019, 36, 215004. [Google Scholar] [CrossRef]
- Rakholia, A.; Sugarbaker, A.; Black, A.; Kasevich, M.; Saif, B.; Luthcke, S.; Callahan, L.; Seery, B.; Feinberg, L.; Mather, J.; et al. Development of an atom interferometer gravity gradiometer for Earth sciences. In Proceedings of the Annual DAMOP Meeting, Sacramento, CA, USA, 5–9 June 2017. [Google Scholar]
- Zhu, Z.; Liao, H.; Tu, H.B.; Duan, X.C.; Zhao, Y.B. Spaceborne atom-interferometry gravity gradiometry design towards future satellite gradiometric missions. Aerospace 2022, 9, 253. [Google Scholar] [CrossRef]
- Van Camp, M.; de Viron, O.; Watlet, A.; Meurers, B.; Francis, O.; Caudron, C. Geophysics from terrestrial time-variable gravity measurements. Rev. Geophys. 2017, 55, 938–992. [Google Scholar] [CrossRef]





| Institution | Sensitivity (μGal/) | Accuracy (μGal) | Reference |
|---|---|---|---|
| Stanford University | 20 | 3.4 | [20] |
| University of California, Berkeley | 37 | 20 | [102] |
| Humboldt University of Berlin | 9.6 | 3.9 | [21] |
| LNE-SYRTE, France | 13 | 4.3 | [22] |
| ONERA, France | 42 | 25 | [142] |
| Exail, France | 50 | -- | [101] |
| HUST | 24 | 3 | [25] |
| Zhejiang University/Zhejiang University of Technology | 300 * | 15.8 | [116] |
| University of Science and Technology of China | 38 | 2 | [26] |
| National Institute of Metrology, China | 44 | 5.2 | [27] |
| Changcheng Institute of Metrology and Measurement | 18 | -- | [24] |
| APM (WAG-H5-1) | 30 | <10 | [143] |
| APM (WAG-C5-1) | 109 | -- | [117] |
| Technology | Characteristic | Example | Applications |
|---|---|---|---|
| LI-based absolute gravimeters | Sensitivity: 15 μGal/Hz Accuracy: 2 μGal | FG5 | Absolute gravity acceleration measurements in a quiet site |
| Spring gravimeters | Precision: <5 μGal Drift: 1.5 mGal/month Fast setup | gPhoneX CG6 | Time-varying gravity monitoring (gPhoneX) and regional gravity mapping (CG6) |
| Superconductive gravimeters | Resolution: <0.3 μGal @ 2 min Drift: <0.5 μGal/month | iGrav SG | Long-term continuous measurements |
| Classical airborne gravimeters | Precision: ~1 mGal Spatial resolution: 4–6 km Drift: <3 mGal/month | GT-2A | Airborne gravity measurements |
| Classical marine gravimeters | Precision: ~1 mGal, Spatial resolution: <2 km Drift: ~3 mGal/month | SEA III | Marine gravity measurements |
| MEMS gravimeters | Resolution: <10 μGal Drift (compensated): 50 μGal/day Low cost and small | GRAV10 | Borehole measurements and creating gravity sensor networks |
| Gravity satellites | Spatial resolution: a few hundred km Desired accuracy of GOCE: 1 mGal | GOCE, GRACE, CHAMP | Globally mapping the Earth’s gravity field |
| Satellite altimetry | Precision: ~2 mGal | CryoSat-2 Jason-1 | Marine gravity measurements |
| Static atomic gravimeters | Sensitivity: ~10 μGal/Hz Accuracy: 2–5.2 μGal | GAIN CAG | Continuous absolute gravity acceleration measurements |
| Marine atomic gravimeters | Precision: <1 mGal Zero drift | ONERA in France | Marine gravity measurements without drift |
| Airborne atomic gravimeters | Precision: ~1 mGal Zero drift | GIRAFE | Airborne gravity measurements without drift |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fang, J.; Wang, W.; Zhou, Y.; Li, J.; Zhang, D.; Tang, B.; Zhong, J.; Hu, J.; Zhou, F.; Chen, X.; et al. Classical and Atomic Gravimetry. Remote Sens. 2024, 16, 2634. https://doi.org/10.3390/rs16142634
Fang J, Wang W, Zhou Y, Li J, Zhang D, Tang B, Zhong J, Hu J, Zhou F, Chen X, et al. Classical and Atomic Gravimetry. Remote Sensing. 2024; 16(14):2634. https://doi.org/10.3390/rs16142634
Chicago/Turabian StyleFang, Jie, Wenzhang Wang, Yang Zhou, Jinting Li, Danfang Zhang, Biao Tang, Jiaqi Zhong, Jiangong Hu, Feng Zhou, Xi Chen, and et al. 2024. "Classical and Atomic Gravimetry" Remote Sensing 16, no. 14: 2634. https://doi.org/10.3390/rs16142634
APA StyleFang, J., Wang, W., Zhou, Y., Li, J., Zhang, D., Tang, B., Zhong, J., Hu, J., Zhou, F., Chen, X., Wang, J., & Zhan, M. (2024). Classical and Atomic Gravimetry. Remote Sensing, 16(14), 2634. https://doi.org/10.3390/rs16142634









