Polarization Characteristics Distortion for L-Band Fully Polarimetric Radar Subject to Magnetized Plasma Sheath
Abstract
1. Introduction
2. Basics of Magnetized Plasma Sheath
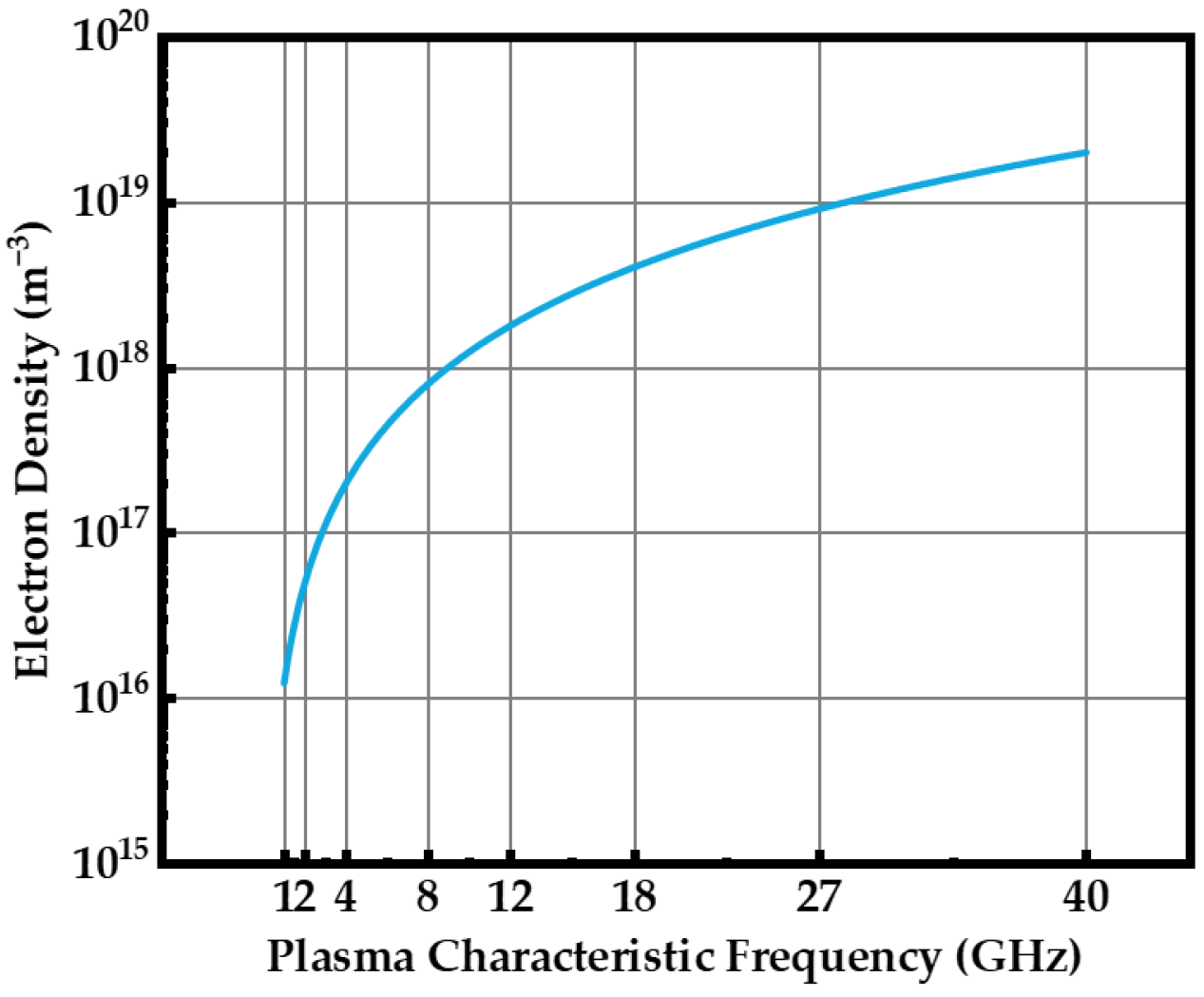
2.1. Geomagnetic Environment of Plasma Sheath
2.2. Typical Parameters of Plasma Sheath
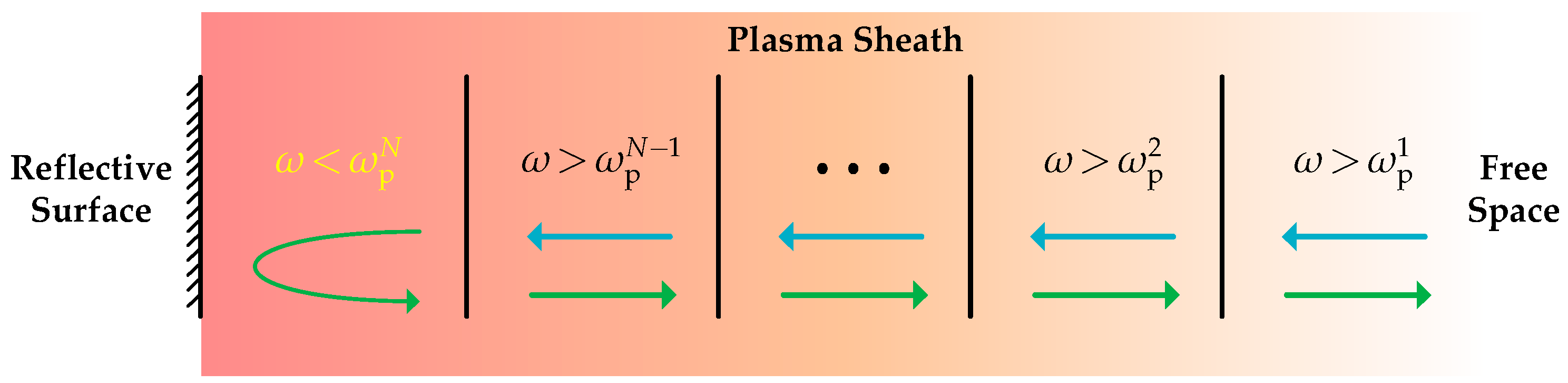
2.3. Phase Screen Model in Non-Uniform Plasma Sheath
3. Polarimetric Error Model of Fully Polarimetric Radar in Magnetized Plasma Sheath
3.1. Characteristic of EM Wave in Magnetized Plasma Sheath
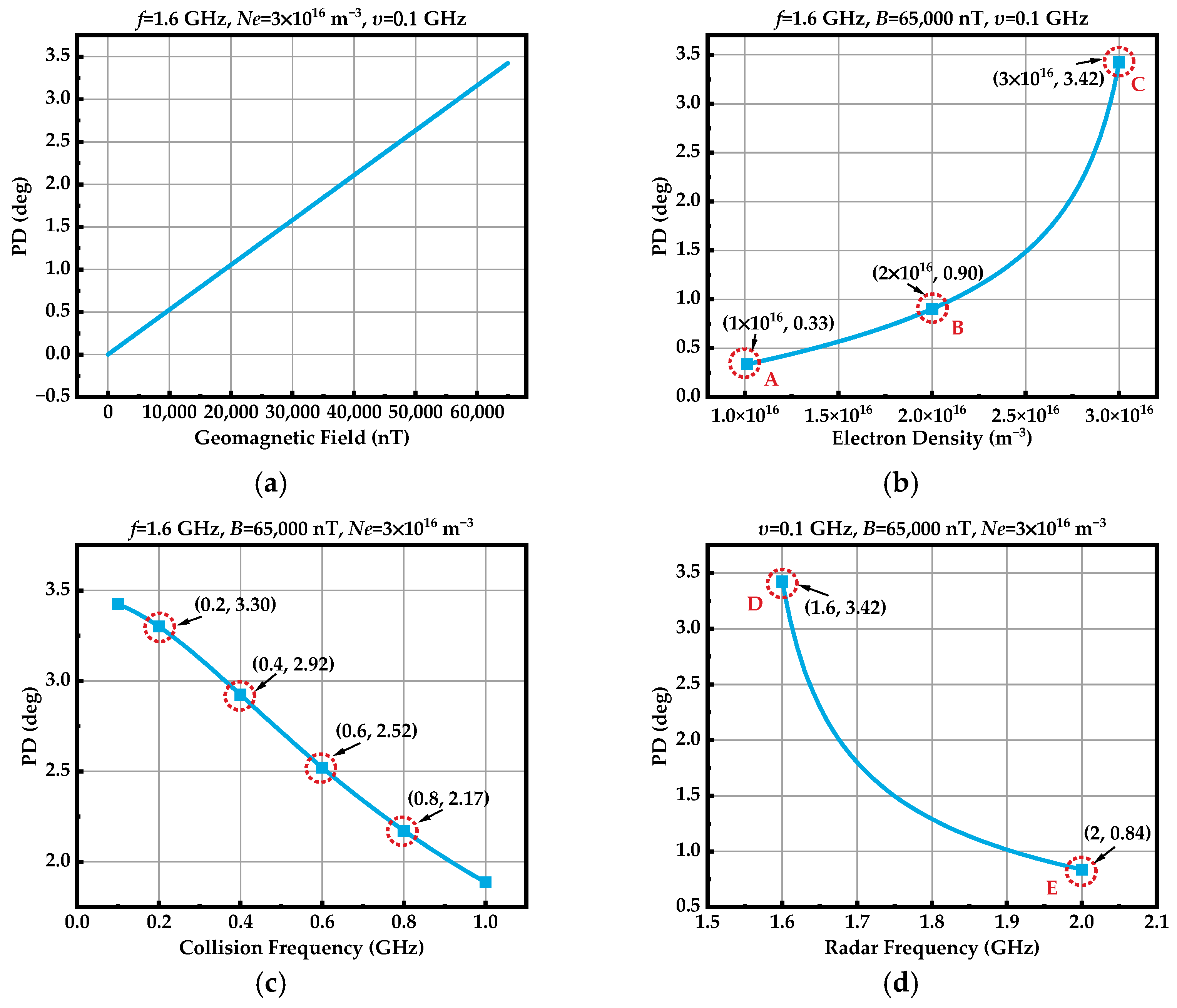
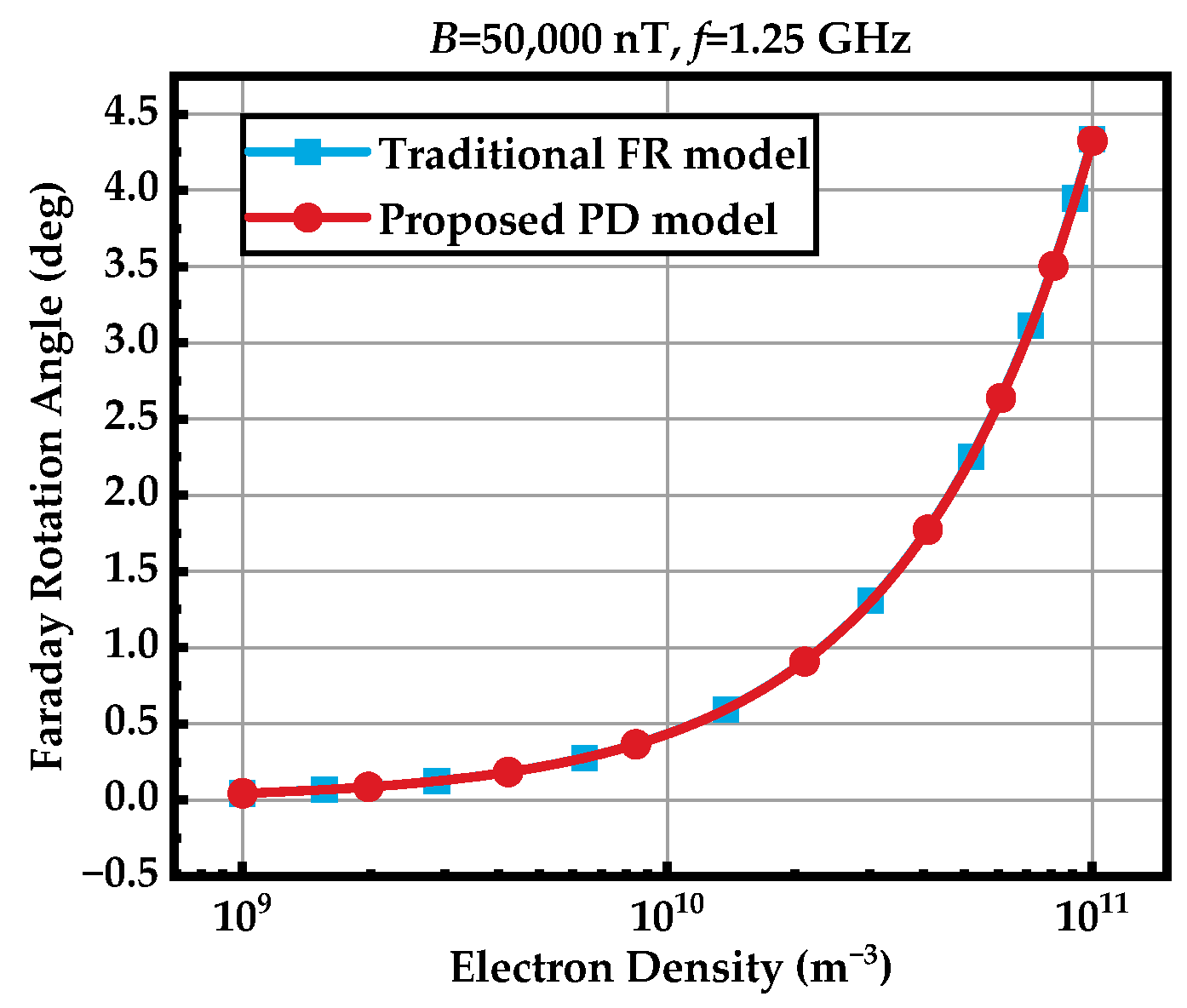
3.2. Polarization Deflection
- The necessary condition for the PD is the existence of the geomagnetic field, and the PD value is approximately linearly proportional to the geomagnetic field intensity;
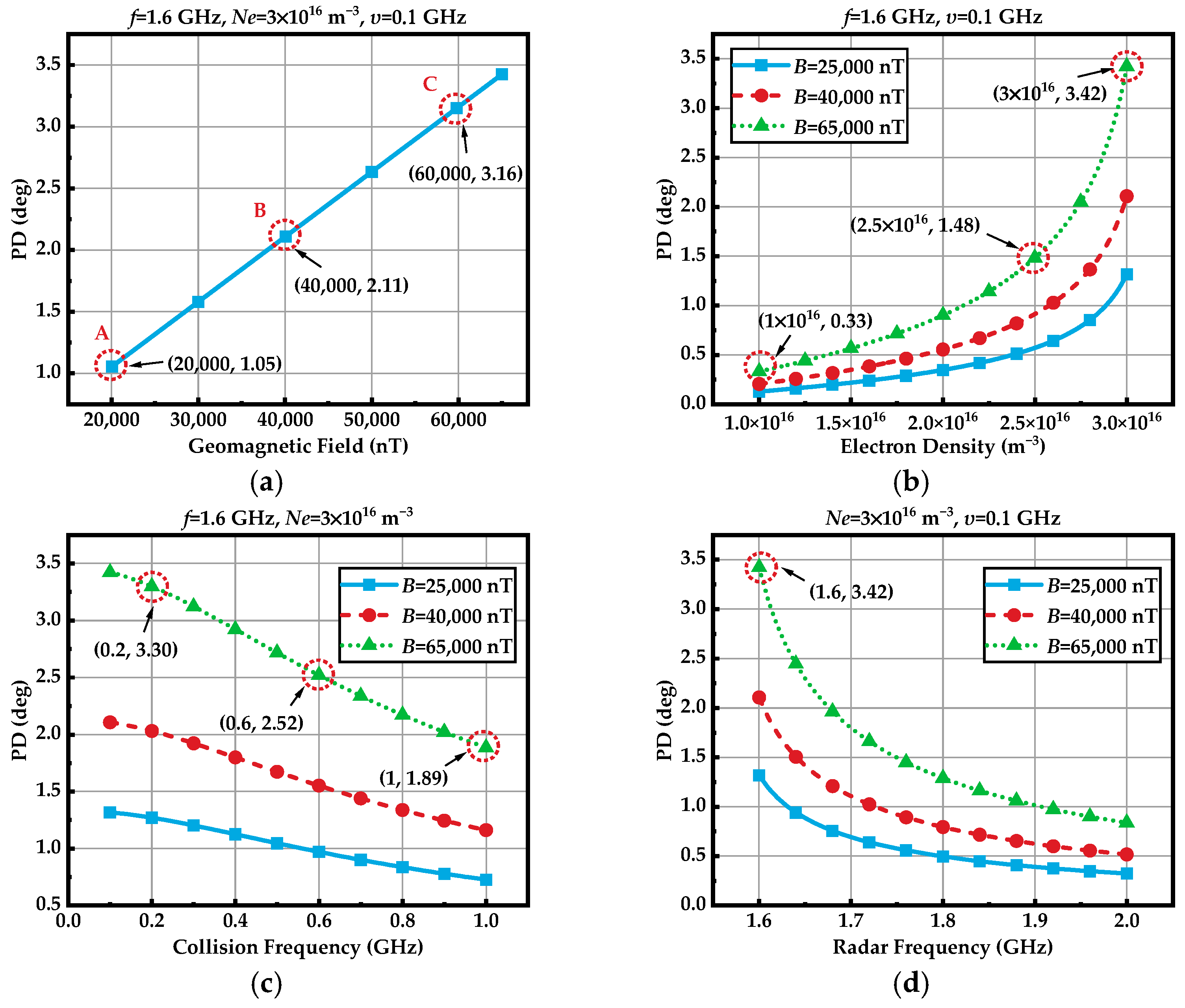
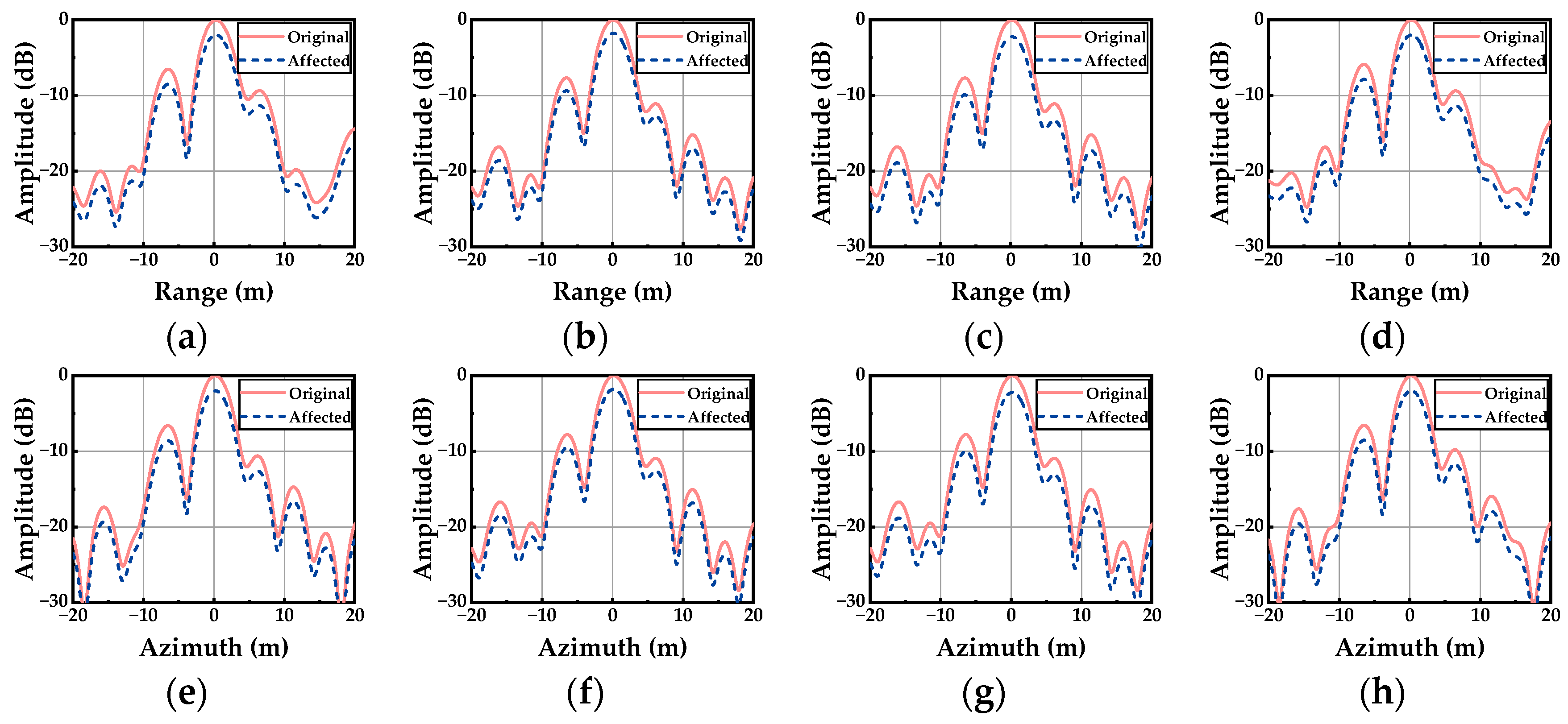
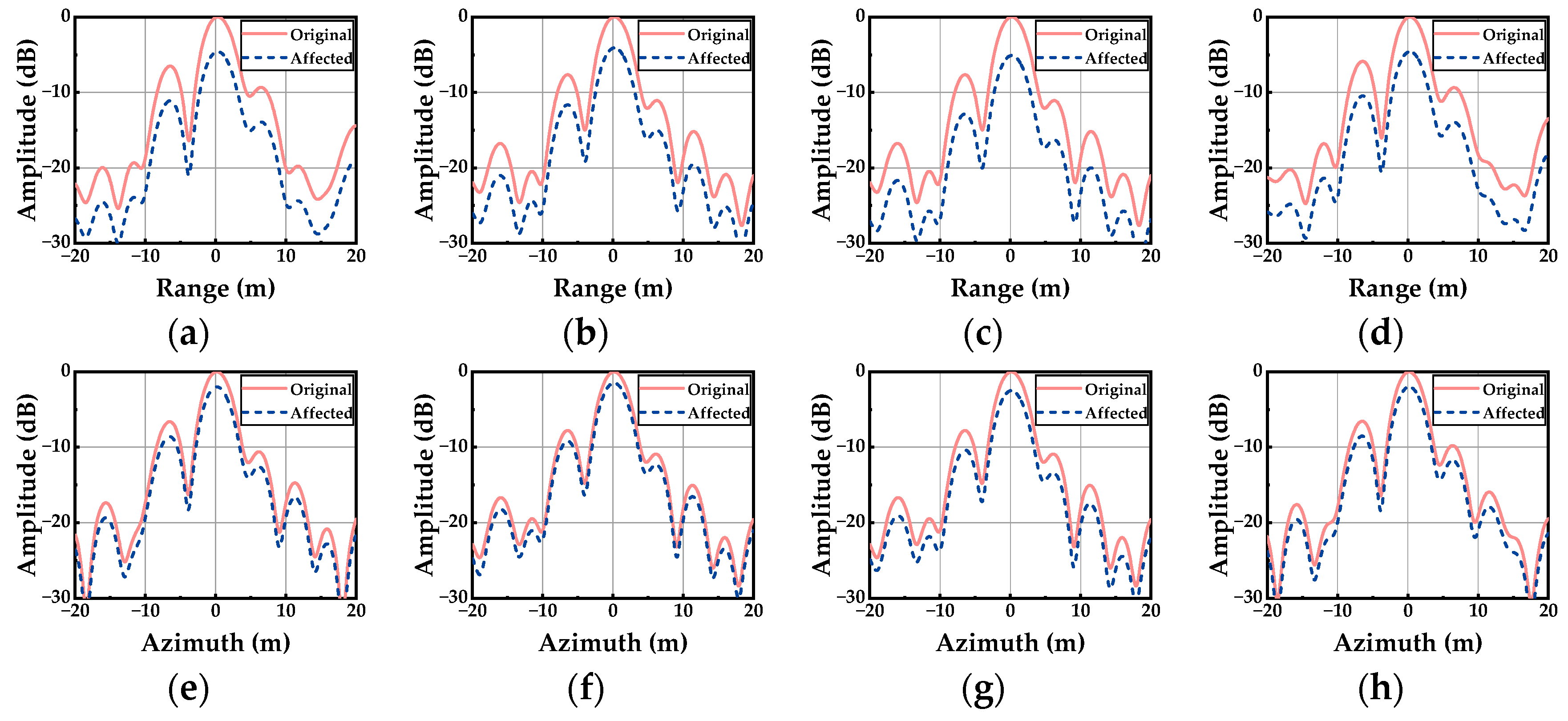
- The electron density, collision frequency, and radar frequency will affect the PD. As the electron density increases, the rate of change for PD increases, indicating that electron density gradually becomes the dominant factor influencing PD. In the “A–B” section of the curve (in Figure 6b), the electron density increases by 1 × 1016 m−3, and the PD increases by 0.57°. In the “B–C” section (in Figure 6b), the electron density increases by 1 × 1016 m−3, and the PD increases by 2.52°, which is 4.4 times that of the “A–B” section. The collision frequency plays the opposite role. When the collision frequency increases from 0.2 GHz to 0.4 GHz, PD decreases by 0.38° (in Figure 6c). However, when it increases from 0.6 GHz to 0.8 GHz, PD only decreases by 0.35° (in Figure 6c). Obviously, PD is also very sensitive to radar frequency. When the radar frequency is 1.6 GHz, the PD is approximately 4 times that when the radar frequency is 2 GHz, as illustrated at points D and E in Figure 6d.
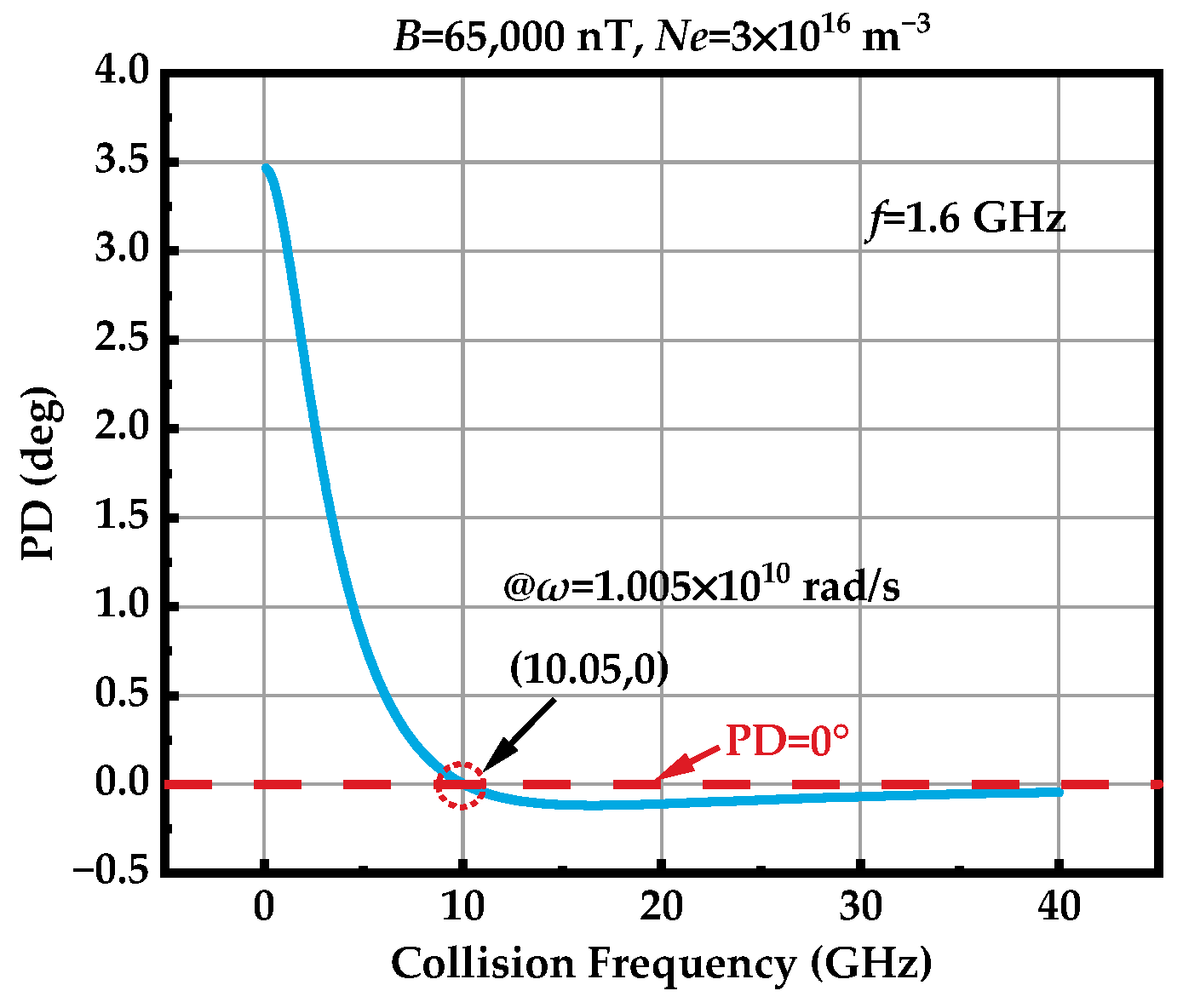
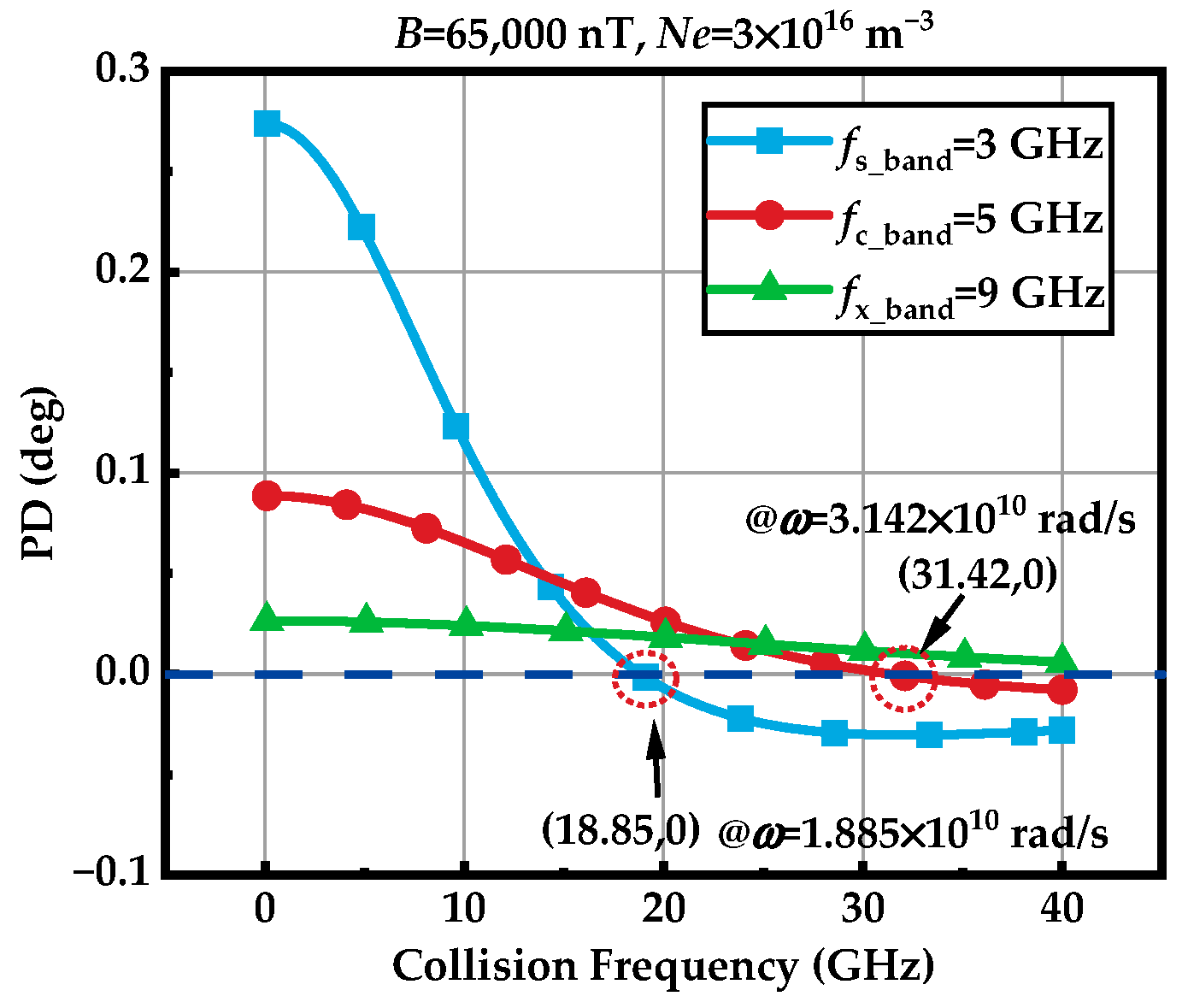
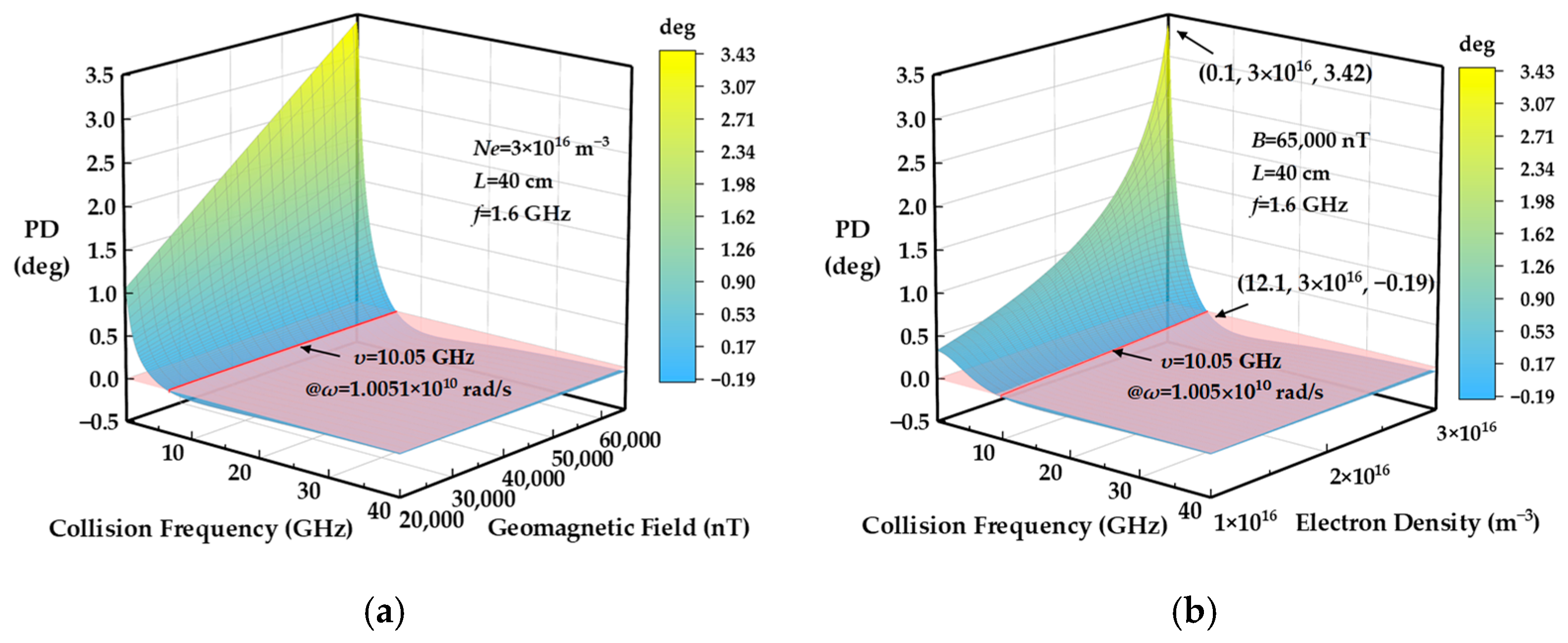
3.3. PD Anomaly Phenomenon
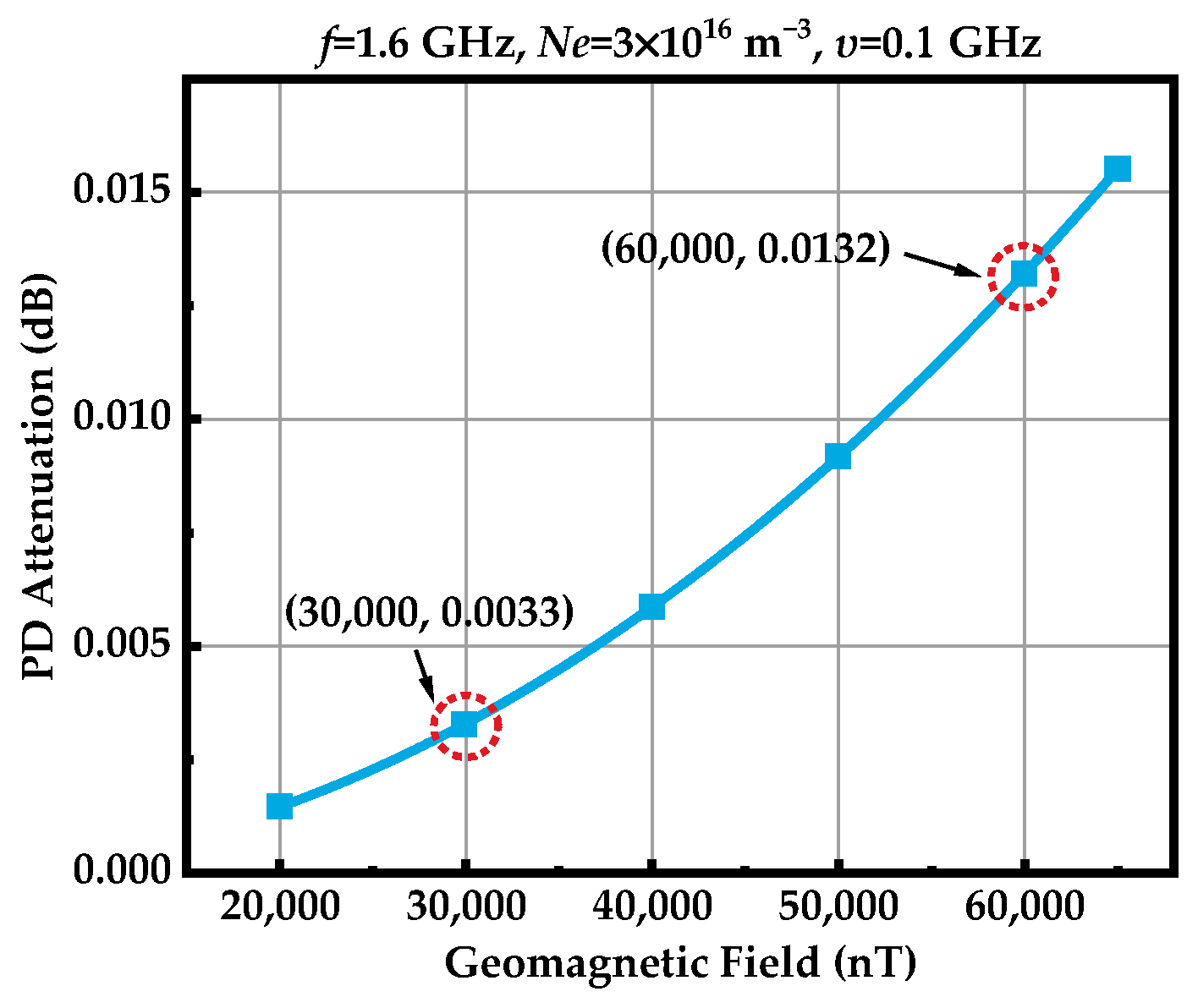
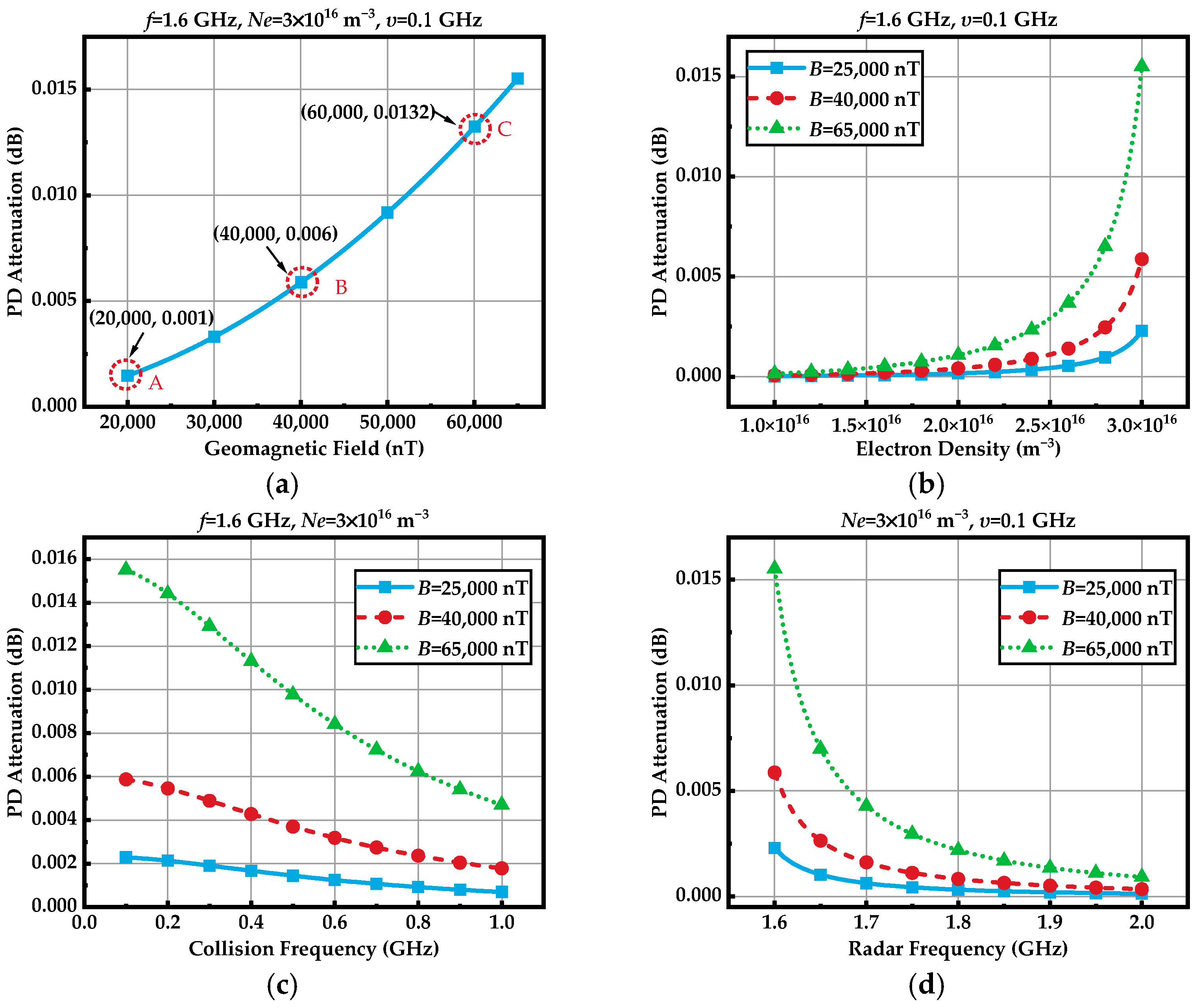
3.4. PD Attenuation
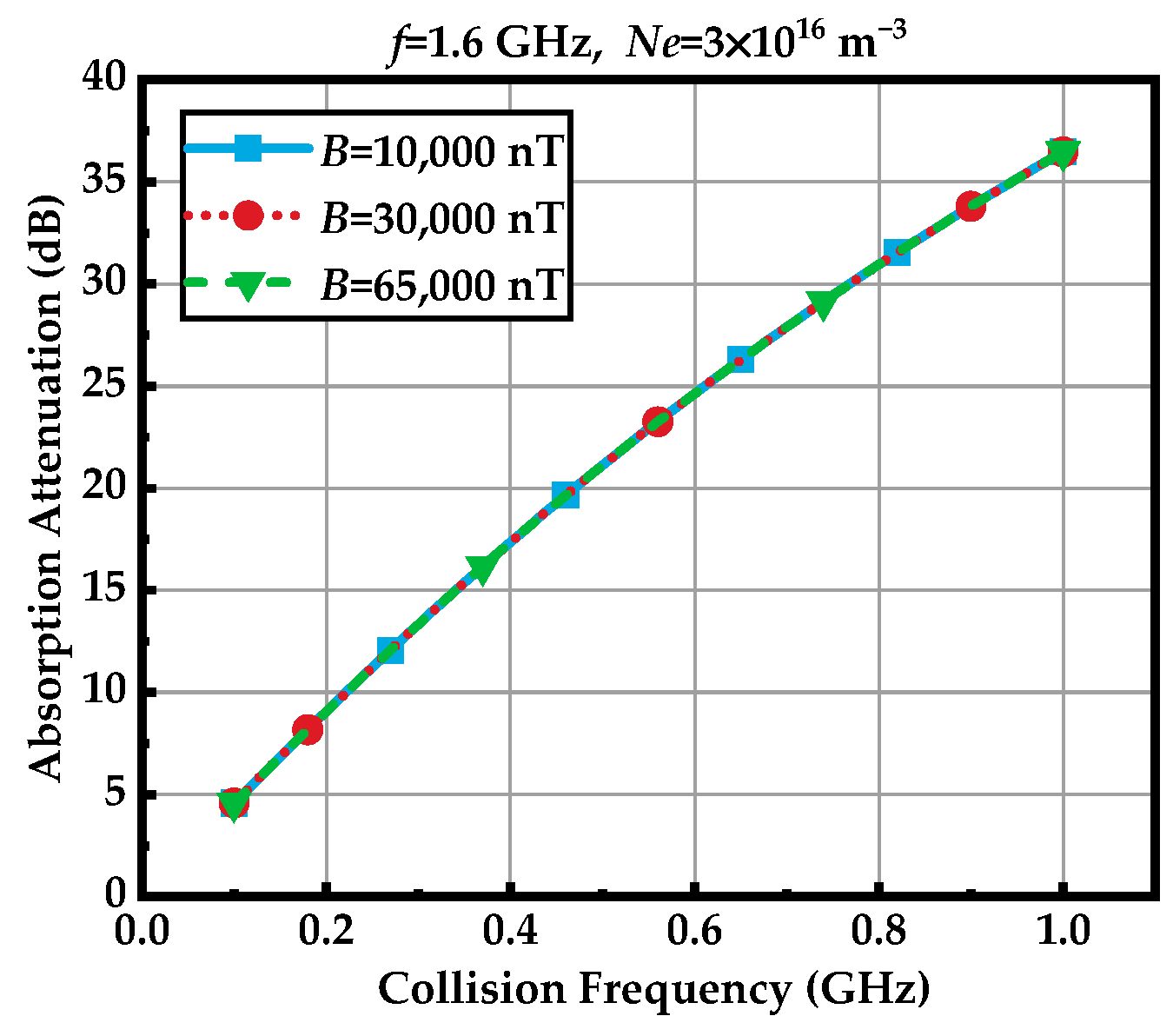
3.5. Absorption Attenuation
3.6. PD Error Model for Fully Polarimetric Radar
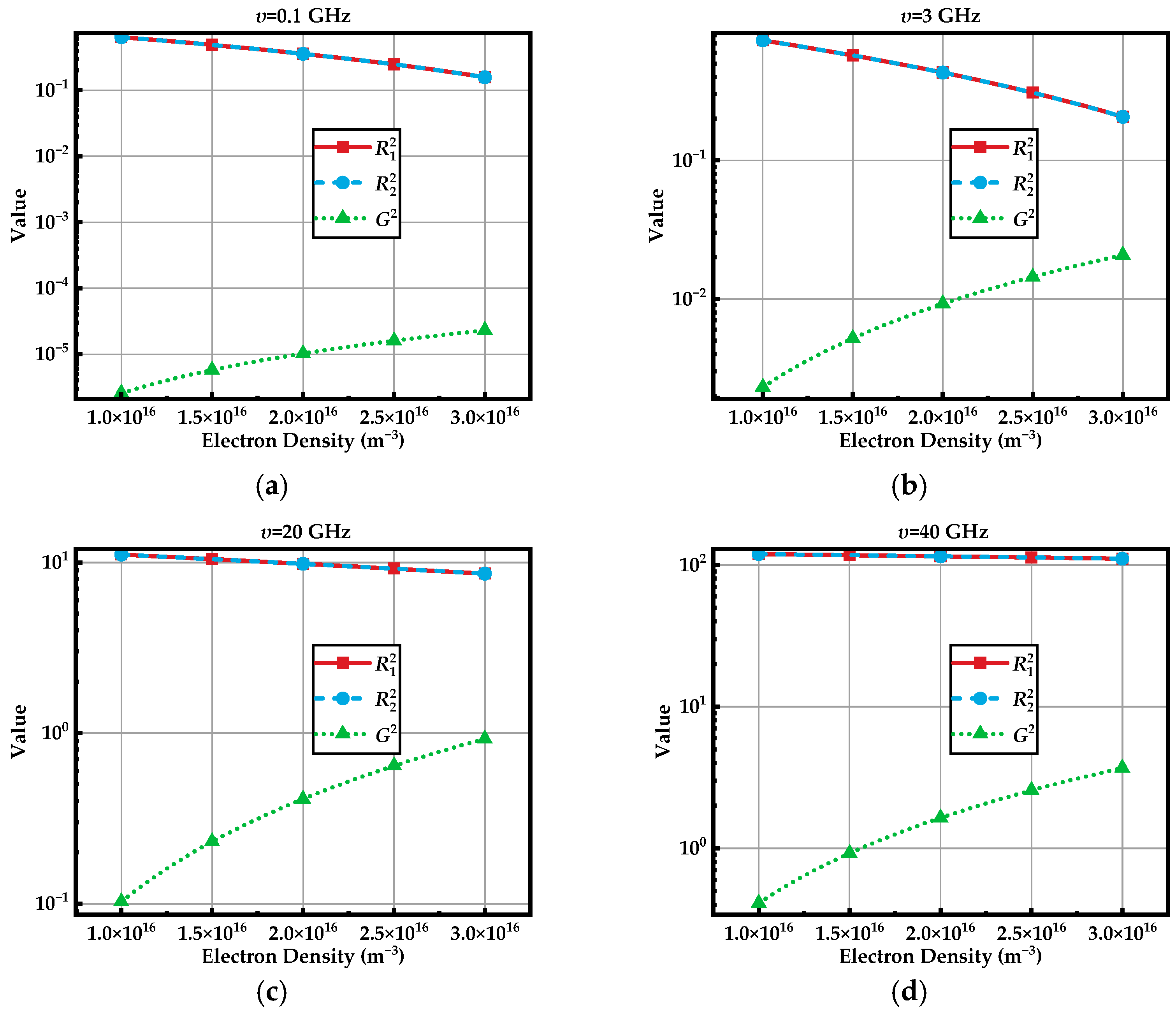
4. Simulations and Analyses
4.1. PD
4.2. PD Anomaly Phenomenon
4.3. PD Attenuation
4.4. Absorption Attenuation
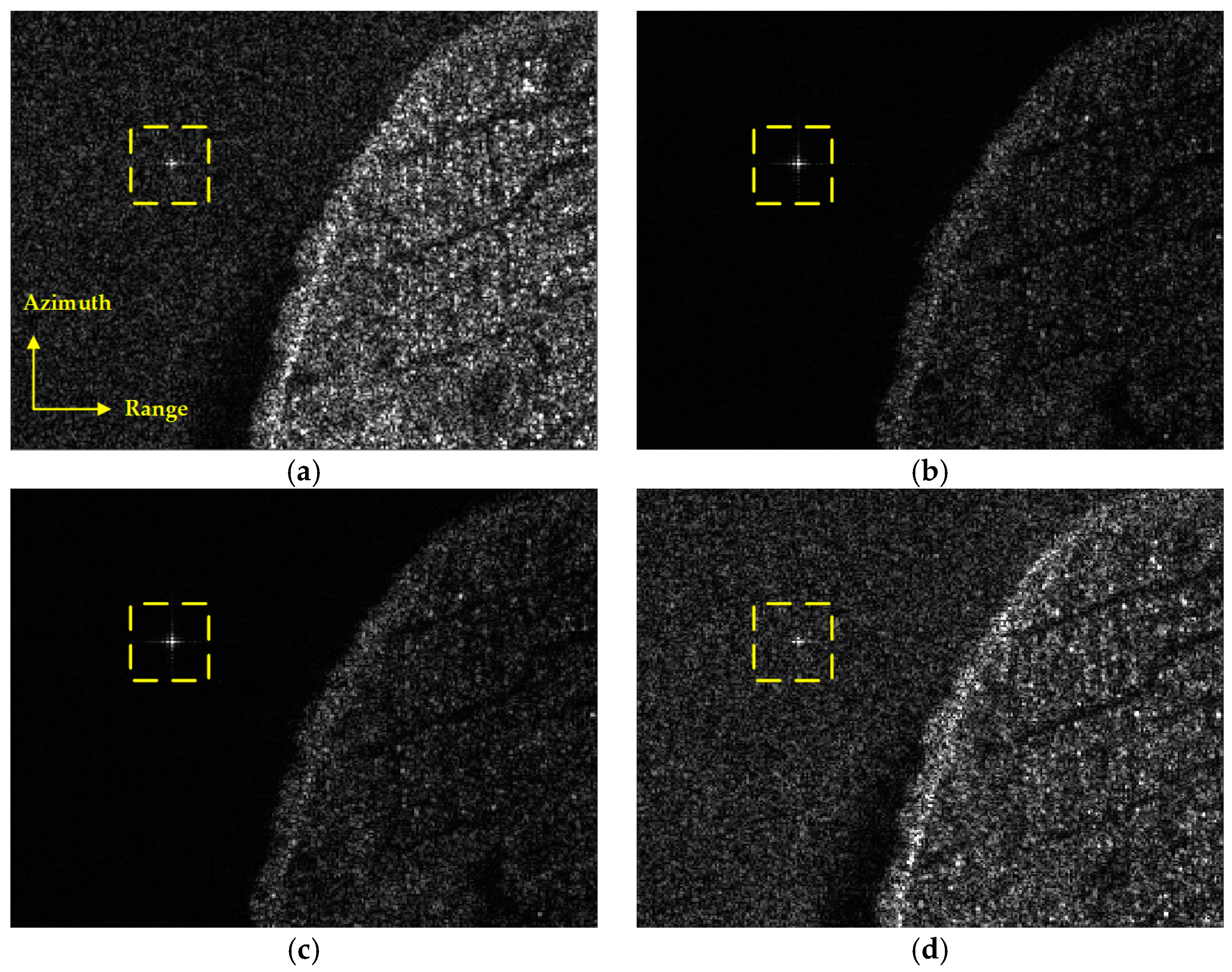
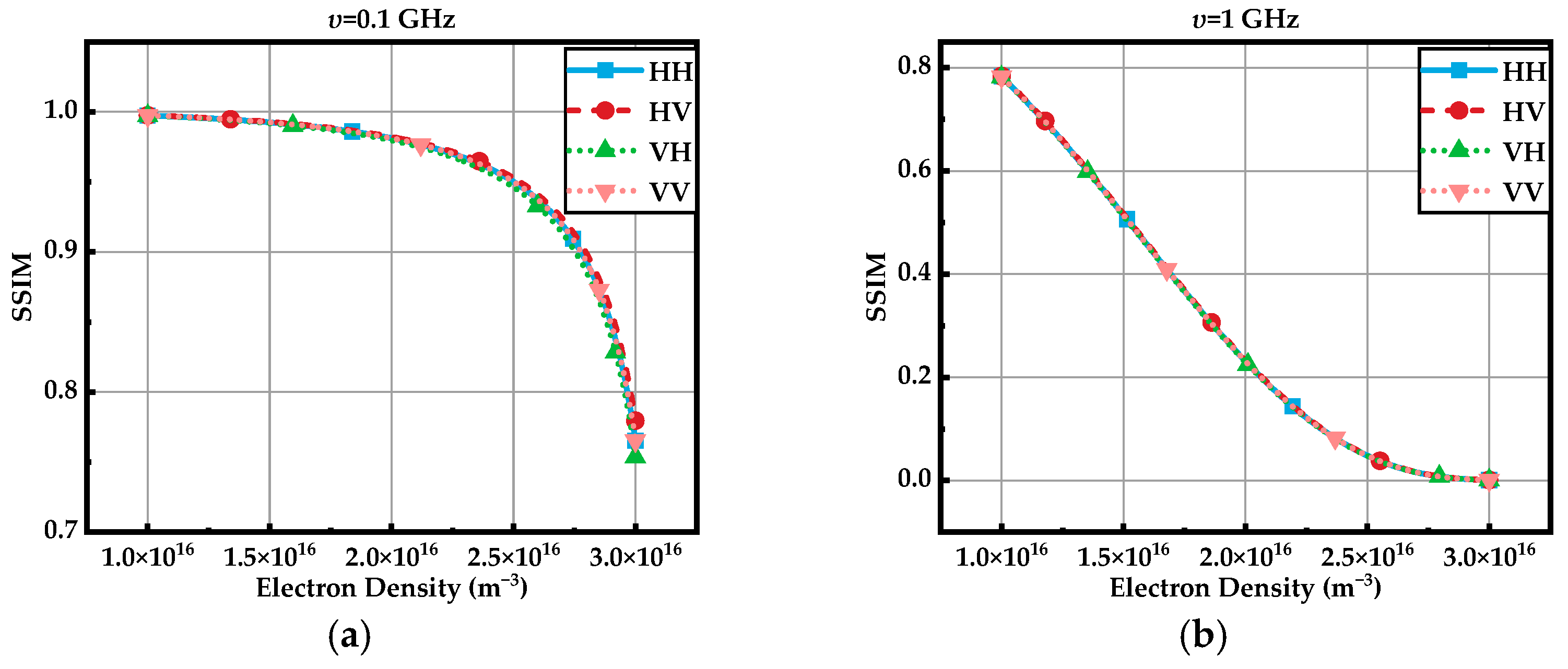
4.5. Polarization Distortions for Fully Polarimetric Radar
5. Discussion
- (1)
- The primary issue is that this article only considers the polarization distortions for an L-band fully polarimetric radar system. For spaceborne or missile-borne SAR platforms, various frequency bands are utilized, such as S-band, C-band, X-band, etc., and the variable states of the vehicle could also broaden the range of parameters for the plasma sheath, including the electron density, the collision frequency, the thickness, etc. To provide a more comprehensive and precise analysis of the impact of a magnetized plasma sheath on fully polarimetric radars, we are now conducting additional experiments considering various radar bands and a wider range of plasma sheath parameters.
- (2)
- The other problem concerns the error correction methods used for a fully polarimetric SAR subject to a magnetized plasma sheath. The error model established in this article and the simulation experiment provide a good reference for the follow-up compensation. For now, the decoupling processing is the key point for us, which aims to better separate the errors from the plasma sheath and the ionosphere. Based on this, the development of a comprehensive compensation process benefited by autofocus and TEC inversion is in progress.
- (3)
- Although the L-band fully polarimetric radar data are specifically discussed here, the derived error models of polarization distortions (including the PD, PD attenuation, and absorption attenuation) are suitable for any frequency band. In order to better illustrate the influence of the magnetized plasma sheath more intuitively, the L-band is chosen here, and the ALOS-2 data are used. This result can be compared directly with the situation in the ionosphere (another typical magnetized plasma environment, or a specific case of our proposed analyses). In future, the multiband data of a fully polarimetric radar system will be considered, such as the GaoFen-3, the latest spaceborne C-band SAR launched in 2016, with rich working modes and high resolution. According to the typical parameters of the plasma sheath, PD and absorption attenuation are both approximately less than 0.1° and 0.1 dB, respectively (lower than the L-band system), when considering the parameters of the GaoFen-3 satellite. This conforms to the analyses presented in Section 3, which highlight an inverse relationship between the polarization distortions and the radar frequency. For now, we are applying for access to the fully polarimetric SAR data of the GaoFen-3 from the Land Satellite Remote Sensing Application Center, and experiments and analyses are currently in progress.
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Li, Z.; Zhang, X.; Yang, Q.; Xiao, Y.; An, H.; Yang, H.; Wu, J. Hybrid SAR-ISAR Image Formation via Joint FrFT-WVD Processing for BFSAR Ship Target High-Resolution Imaging. IEEE Trans. Geosci. Remote Sens. 2021, 60, 5215713. [Google Scholar] [CrossRef]
- Ding, Y.; Bai, B.; Niu, G.; Li, X.; Liu, Y. A Radar Detection Method of Reentry Target Based on Frequency-Domain Processing. IEEE Trans. Plasma Sci. 2023, 51, 649–659. [Google Scholar] [CrossRef]
- Rawhouser, R. Overview of the AF Avionics Laboratory Reentry Electromagnetics Program. In Proceedings of the Entry Plasma Sheath and Its Effects on Space Vehicle Electromagnetic Systems, Hampton, VA, USA, 13–15 October 1970; pp. 3–17. [Google Scholar]
- Kong, J.A. Electromagnetic Wave Theory; Wiley: New York, NY, USA, 1986. [Google Scholar]
- Liu, Y.; Zhang, X.; Bai, B.; Ding, Y.; Li, X. A Calculation Method of Electromagnetic Wave Reflection in Plasma Sheath Environment. IEEE Trans. Plasma Sci. 2022, 50, 2030–2038. [Google Scholar] [CrossRef]
- Shen, F.; Zhang, Z.; Bai, B.; Li, X.; Chen, X.; Liu, Y. Research on the Reflection Characteristics of the Broadband Electromagnetic Wave in Nonuniform Plasma. IEEE Trans. Plasma Sci. 2024, 52, 657–665. [Google Scholar] [CrossRef]
- Song, L.; Tan, E.L.; Heh, D.Y.; Bai, B.; Li, X.; Liu, Y. Newton-ADE FDTD Method for Time-Varying Plasma. IEEE T. Microw. Theory 2023, 71, 1447–1457. [Google Scholar] [CrossRef]
- Huang, Z.; Feng, M.; Gao, P.; Jiang, X. FDTD Solution for Signal Transmission in Plasma Sheath. In Proceedings of the 2023 International Applied Computational Electromagnetics Society Symposium (ACES-China), Hangzhou, China, 15–18 August 2023; pp. 1–3. [Google Scholar]
- Laroussi, M.; Roth, J.R. Numerical Calculation of the Reflection, Absorption, and Transmission of Microwaves by a Nonuniform Plasma Slab. IEEE Trans. Plasma Sci. 1993, 21, 366–372. [Google Scholar] [CrossRef]
- Song, L.; Li, X.; Liu, Y. Effect of Time-Varying Plasma Sheath on Hypersonic Vehicle-Borne Radar Target Detection. IEEE Sens. J. 2021, 21, 16880–16893. [Google Scholar] [CrossRef]
- Bai, B.; Li, X.; Liu, Y.; Xu, J.; Shi, L.; Xie, K. Effects of Reentry Plasma Sheath on the Polarization Properties of Obliquely Incident EM Waves. IEEE Trans. Plasma Sci. 2014, 42, 3365–3372. [Google Scholar] [CrossRef]
- Bai, B.; Zhao, Z.; Yuan, K.; Tang, R.; Deng, X.; Mao, M.; Xiong, J.; Wang, K. Characteristics of EHF Wave Propagation in Hypersonic Plasma Sheaths Magnetized by Dipole Magnetic Fields. Appl. Sci. 2022, 12, 3105. [Google Scholar] [CrossRef]
- Lawrence, R.S.; Little, G.C.; Chivers, H.J.A. A Survey of Ionospheric Effects upon Earth-Space Radio Propagation. Proc. IEEE. 1964, 52, 4–27. [Google Scholar] [CrossRef]
- Bickel, S.H.; Bates, R.H.T. Effects of Magneto-ionic Propagation on the Polarization Scattering Matrix. Proc. IEEE. 1965, 53, 1089–1091. [Google Scholar] [CrossRef]
- Gail, W.B. Effect of Faraday Rotation on Polarimetric SAR. IEEE Trans. Aerosp. Electron. Syst. 1998, 34, 301–307. [Google Scholar] [CrossRef]
- Freeman, A.; Saatchi, S.S. On the Detection of Faraday Rotation in Linearly Polarized L-Band SAR Backscatter Signatures. IEEE Trans. Geo Remote Sens. 2004, 42, 1607–1616. [Google Scholar] [CrossRef]
- Belcher, D.P. Theoretical Limits on SAR Imposed by the Ionosphere. IET Radar Sonar Navig. 2008, 2, 435–448. [Google Scholar] [CrossRef]
- Belcher, D.P.; Rogers, N.C. Theory and Simulation of Ionospheric Effects on Synthetic Aperture Radar. IET Radar Sonar Navig. 2009, 3, 541–551. [Google Scholar] [CrossRef]
- Jehle, M.; Frey, O.; Small, D.; Meier, E. Measurement of Ionospheric TEC in Spaceborne SAR data. IEEE Trans. Geosci. Electron. 2010, 48, 2460–2468. [Google Scholar] [CrossRef]
- Bittencourt, J.A. Fundamentals of Plasma Physics; Springer: New York, NY, USA, 2004. [Google Scholar]
- Ouyang, W.; Jin, T.; Wu, Z.; Deng, W. Study of Terahertz Wave Propagation in Realistic Plasma Sheath for the Whole Reentry Process. IEEE Trans. Plasma Sci. 2021, 49, 460–465. [Google Scholar] [CrossRef]
- Grill, A. Cold Plasma Materials Fabrication: From Fundamentals to Applications; Wiley-IEEE Press: Hoboken, NJ, USA, 1994. [Google Scholar]
- Dubois, D.F.; Gilinsky, V.; Kivelson, M.G. Propagation of Electromagnetic Waves in Plasmas. Phys. Rev. 1963, 129, 6. [Google Scholar] [CrossRef]
- Beck, F.B.; Castellow, S.L.; Swift, C.T.; Thomson, J. RAM C-3 S-band Diagnostic Experiment. NASA Spec. Publ. 1970, 1, 1. [Google Scholar]
- Zhou, H.; Li, X.; Liu, Y.; Bai, B.; Xie, K. Effects of Nonuniform Magnetic Fields on the “Magnetic Window” in Blackout Mitigation. IEEE Trans. Plasma Sci. 2017, 45, 15–23. [Google Scholar] [CrossRef]
- Ding, Y.; Bai, B.; Gao, H.; Liu, Y.; Li, X.; Mao, Z. Method of Detecting a Target Enveloped by a Plasma Sheath Based on Doppler Frequency Compensation. IEEE Trans. Plasma Sci. 2020, 48, 4103–4111. [Google Scholar] [CrossRef]
- Ding, M.; Tong, P.; Wei, Y.; Yu, L. Multiple Phase Screen Modeling of HF Wave Field Scintillations Caused by the Irregularities in Inhomogeneous Media. Radio Sci. 2021, 56, e2020RS007239. [Google Scholar] [CrossRef]
- Li, J.; Yang, S.; Guo, L. Electromagnetic Waves Propagation in Hypersonic Turbulence using Fractal Phase Screen Method. J. Electromagn. Wave 2017, 31, 250–262. [Google Scholar]
- Chen, X.; Shen, F.; Liu, Y.; Ai, W.; Li, X. Study of Plasma-Based Stable and Ultra-Wideband Electromagnetic Wave Absorption for Stealth Application. Plasma Sci. Technol. 2018, 20, 065503. [Google Scholar] [CrossRef]
- Guru, B.S. Electromagnetic Field Theory Fundamentals; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Taylor, M. The Appleton-Hartree Formula and Dispersion Curves for the Propagation of Electromagnetic Waves through an Ionized Medium in the Presence of an External Magnetic Field. Proc. Phys. Soc. 1934, 46, 245–265. [Google Scholar] [CrossRef]
- Pederick, L.; Harris, T.; MacKinnon, A.; Reid, I. Response of a Polarimetric Antenna to Ionospherically Propagated Signals. IEEE Trans. Antennas Propag. 2021, 69, 7846–7854. [Google Scholar] [CrossRef]
- Smith, D.R.; Kroll, N. Negative Refractive Index in Left-Handed Materials. Phys. Rev. Lett. 2000, 85, 2933–2936. [Google Scholar] [CrossRef] [PubMed]
- Xu, Z.; Wu, J.; Wu, Z. A Survey of Ionospheric Effects on Space-Based Radar. Waves Random Media 2004, 14, 189–273. [Google Scholar] [CrossRef]
- Ishimaru, A. Electromagnetic Wave Propagation, Radiation, and Scattering: From Fundamentals to Applications; Wiley: Hoboken, NJ, USA, 2017. [Google Scholar]
- Wang, Y.; Zhang, X.; Zhan, X.; Zhang, T.; Zhou, L.; Shi, J.; Wei, S. An RCS Measurement Method Using Sparse Imaging Based 3-D SAR Complex Image. IEEE Antenn. Wirel. Propag. Lett. 2021, 21, 24–28. [Google Scholar] [CrossRef]
- Horé, A.; Ziou, D. Image Quality Metrics: PSNR vs. SSIM. In Proceedings of the 2010 20th International Conference on Pattern Recognition, Istanbul, Turkey, 23–26 August 2010; pp. 2366–2369. [Google Scholar]
- Niu, G.; Liu, Y.; Bai, B.; Ding, Y.; Song, L.; Li, X. Polarization Scattering Properties of Inhomogeneous Plasma-Covered Metal Plate. IEEE Trans. Plasma Sci. 2023, 51, 1232–1238. [Google Scholar] [CrossRef]
- Mohanty, S.; Singh, G.; Yamaguchi, Y. Faraday rotation Correction and Total Electron Content Estimation using ALOS-2/PALSAR-2 Full Polarimetric SAR Data. In Proceedings of the 2016 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Beijing, China, 10–15 July 2016; pp. 4754–4756. [Google Scholar]
- Zawdie, K.A.; Drob, D.P.; Siskind, D.E.; Coker, C. Calculating the Absorption of HF Radio Waves in the Ionosphere. Radio Sci. 2017, 52, 767–783. [Google Scholar] [CrossRef]





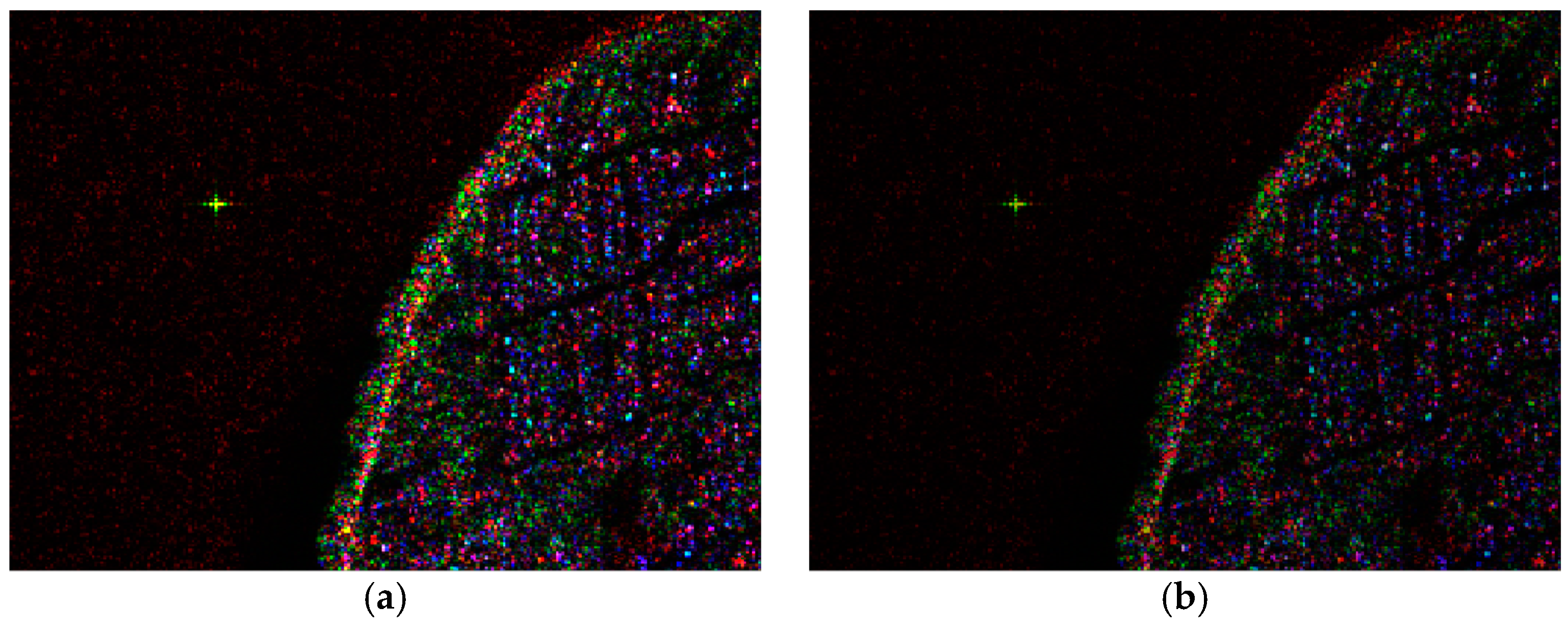
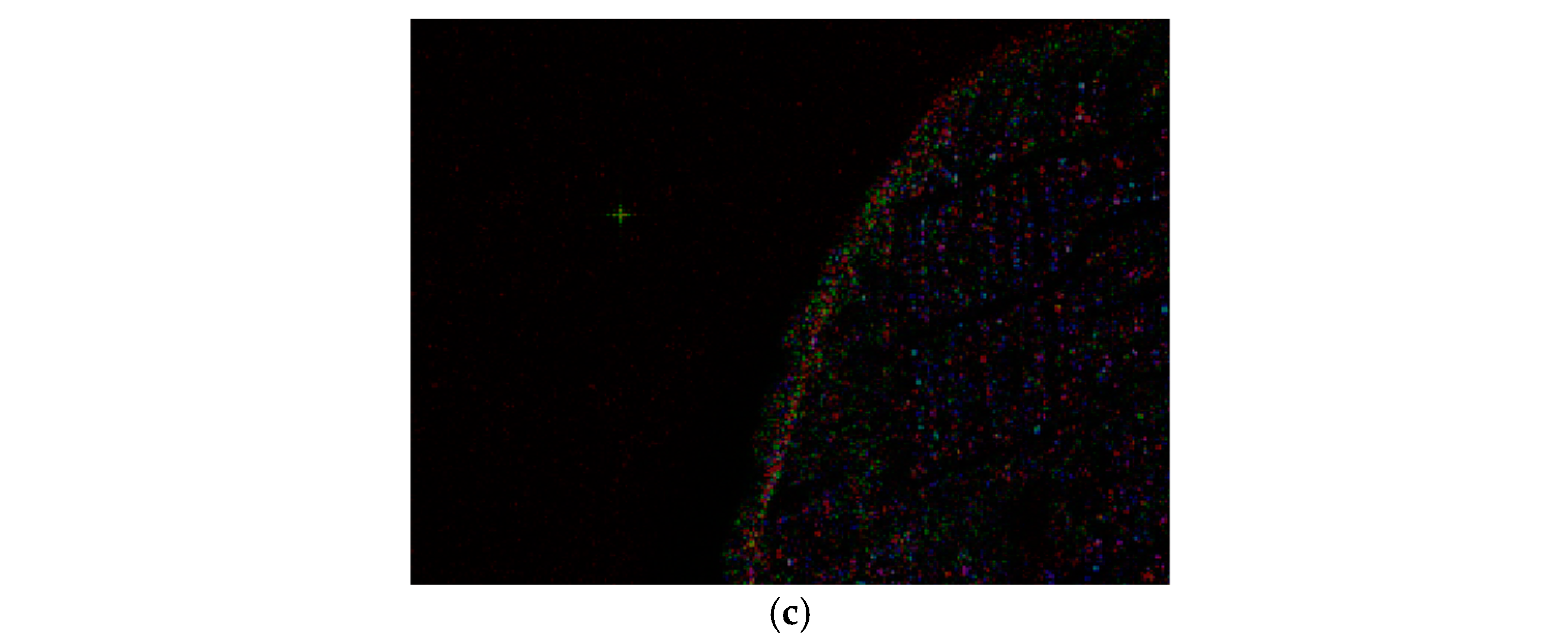
| Parameter | Value |
|---|---|
| electron density | 1 × 1016–3 × 1016 m−3 |
| geomagnetic field intensity | 20,000–65,000 nT |
| collision frequency | 0.1–40 GHz |
| radar frequency | 1.6–2 GHz |
| penetration depth | 40 cm |
| Parameter | Value |
|---|---|
| geomagnetic field intensity | 65,000 nT |
| electron density | 2.5 × 1016 m−3, 3 × 1016 m−3 |
| collision frequency | 0.1 GHz |
| radar frequency | 1.6 GHz |
| penetration depth | 40 cm |
| Channel | HH | HV | VH | VV | |
|---|---|---|---|---|---|
| Situation | |||||
| situation 1 with Ne = 2.5 × 1016 m−3 | 0.9502 | 0.9528 | 0.9463 | 0.9502 | |
| situation 2 with Ne = 3 × 1016 m−3 | 0.7655 | 0.7797 | 0.7539 | 0.7654 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, W.; Hu, Y.; Shen, F.; Xiao, P. Polarization Characteristics Distortion for L-Band Fully Polarimetric Radar Subject to Magnetized Plasma Sheath. Remote Sens. 2024, 16, 2061. https://doi.org/10.3390/rs16122061
Guo W, Hu Y, Shen F, Xiao P. Polarization Characteristics Distortion for L-Band Fully Polarimetric Radar Subject to Magnetized Plasma Sheath. Remote Sensing. 2024; 16(12):2061. https://doi.org/10.3390/rs16122061
Chicago/Turabian StyleGuo, Wei, Yanpeng Hu, Fangfang Shen, and Peng Xiao. 2024. "Polarization Characteristics Distortion for L-Band Fully Polarimetric Radar Subject to Magnetized Plasma Sheath" Remote Sensing 16, no. 12: 2061. https://doi.org/10.3390/rs16122061
APA StyleGuo, W., Hu, Y., Shen, F., & Xiao, P. (2024). Polarization Characteristics Distortion for L-Band Fully Polarimetric Radar Subject to Magnetized Plasma Sheath. Remote Sensing, 16(12), 2061. https://doi.org/10.3390/rs16122061








