Figure 1.
converges to the when , with .
Figure 1.
converges to the when , with .
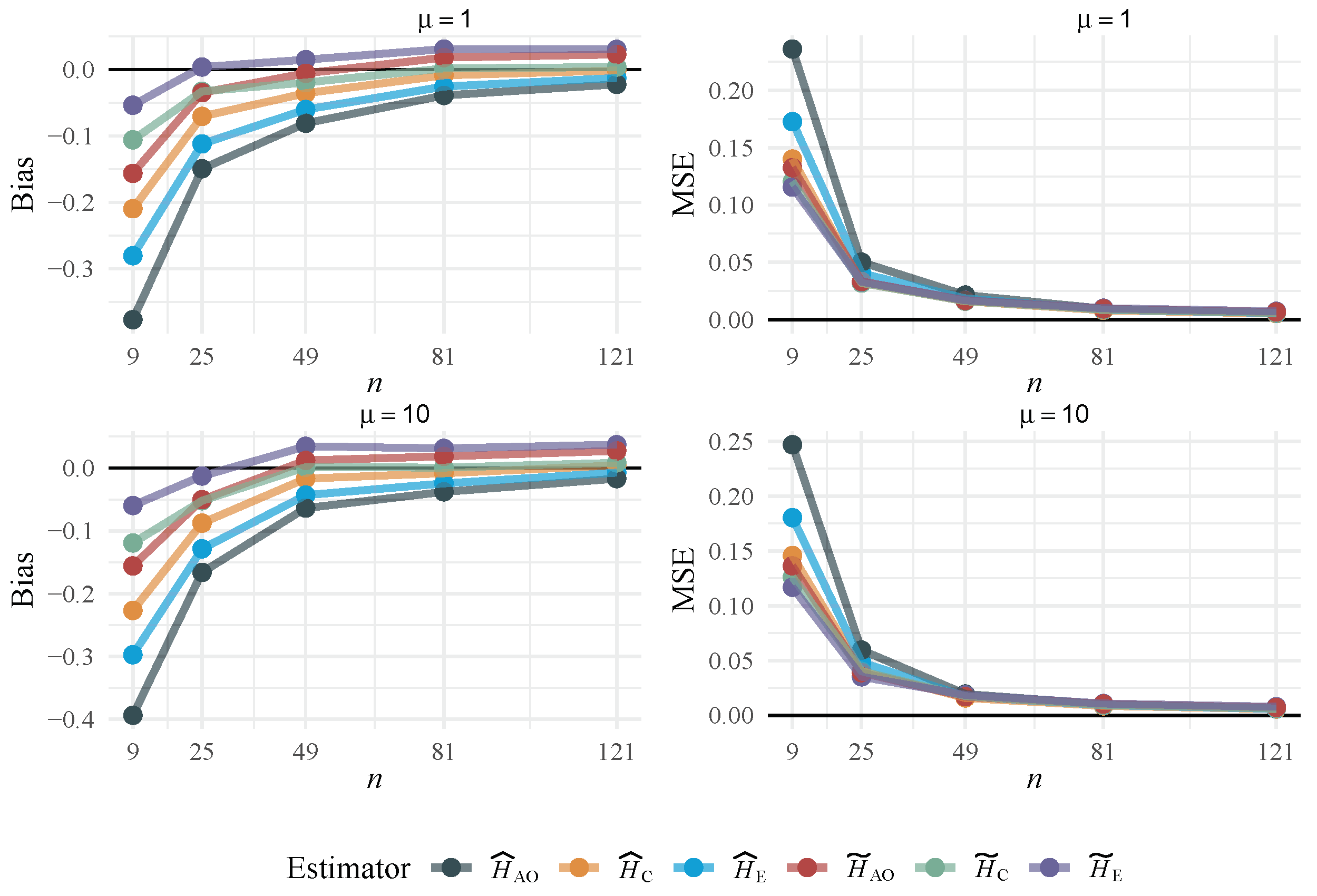
Figure 2.
Bias and MSE of the entropy estimators for the with .
Figure 2.
Bias and MSE of the entropy estimators for the with .
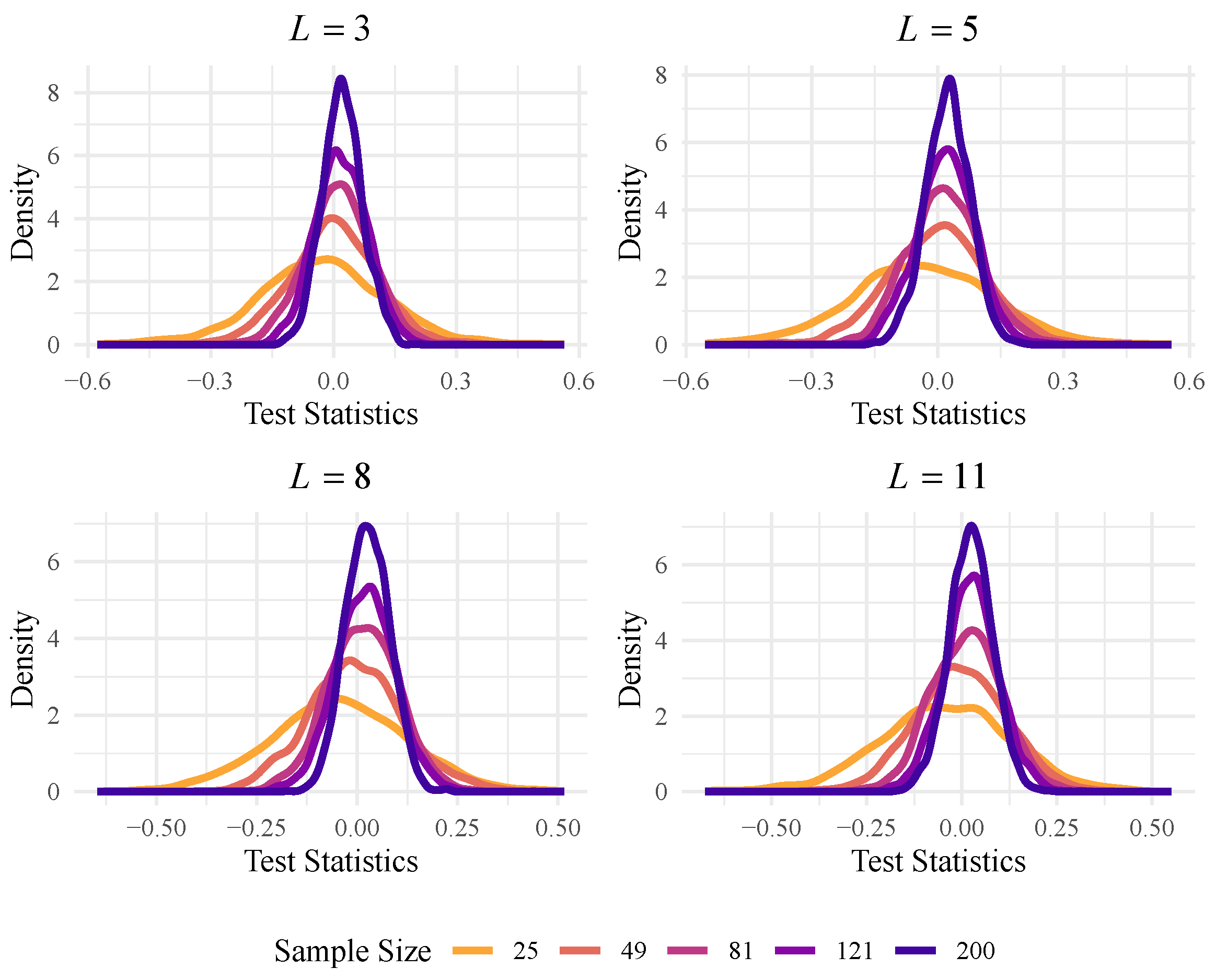
Figure 3.
Empirical densities obtained from test under the null hypothesis.
Figure 3.
Empirical densities obtained from test under the null hypothesis.
Figure 4.
Normal Q–Q plots for . The dashed green lines in the Q–Q plots represent theoretical expected quantiles for a normal distribution. Variations in the scales of the axes reflect differences in the range of values across datasets.
Figure 4.
Normal Q–Q plots for . The dashed green lines in the Q–Q plots represent theoretical expected quantiles for a normal distribution. Variations in the scales of the axes reflect differences in the range of values across datasets.
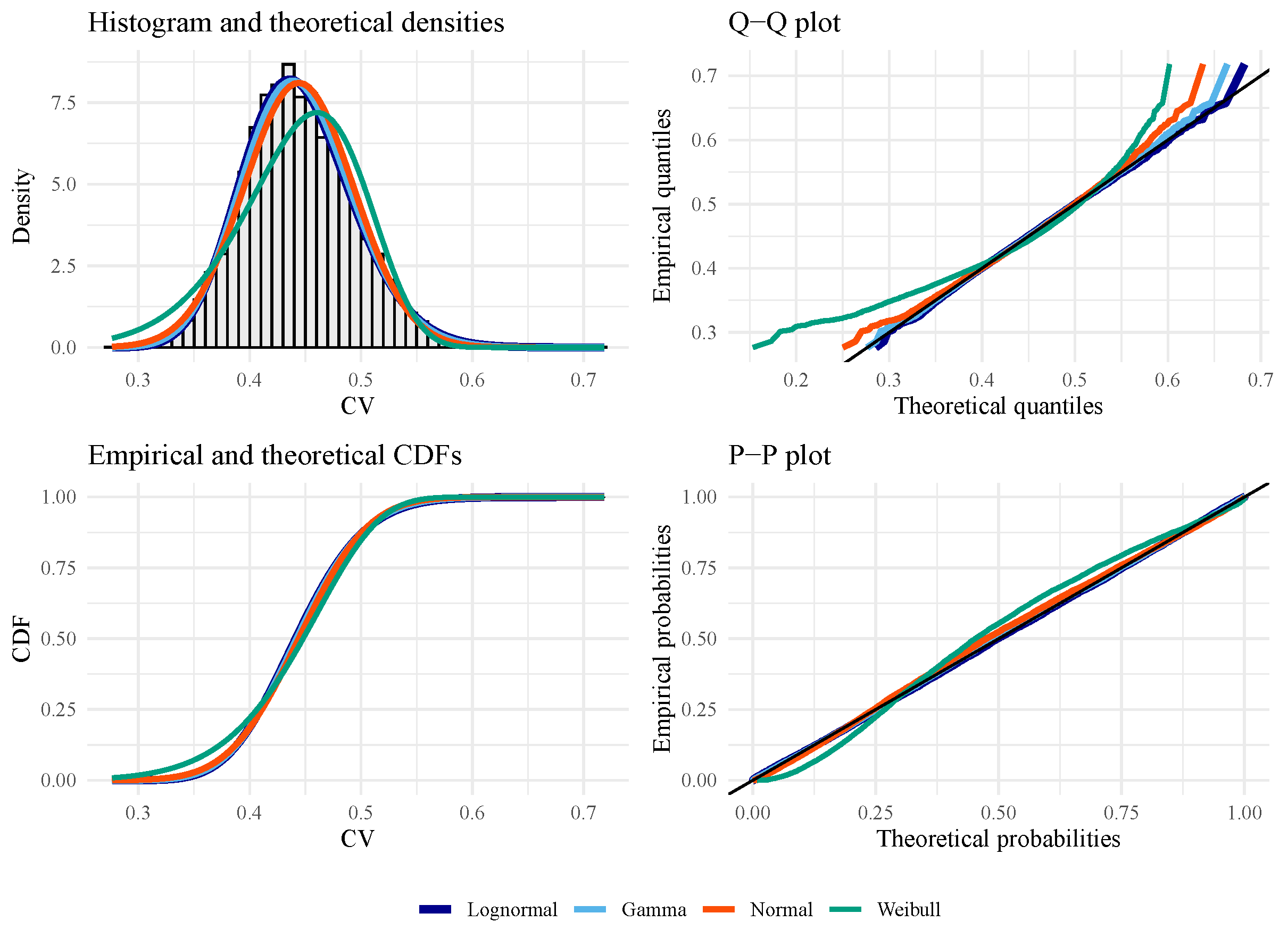
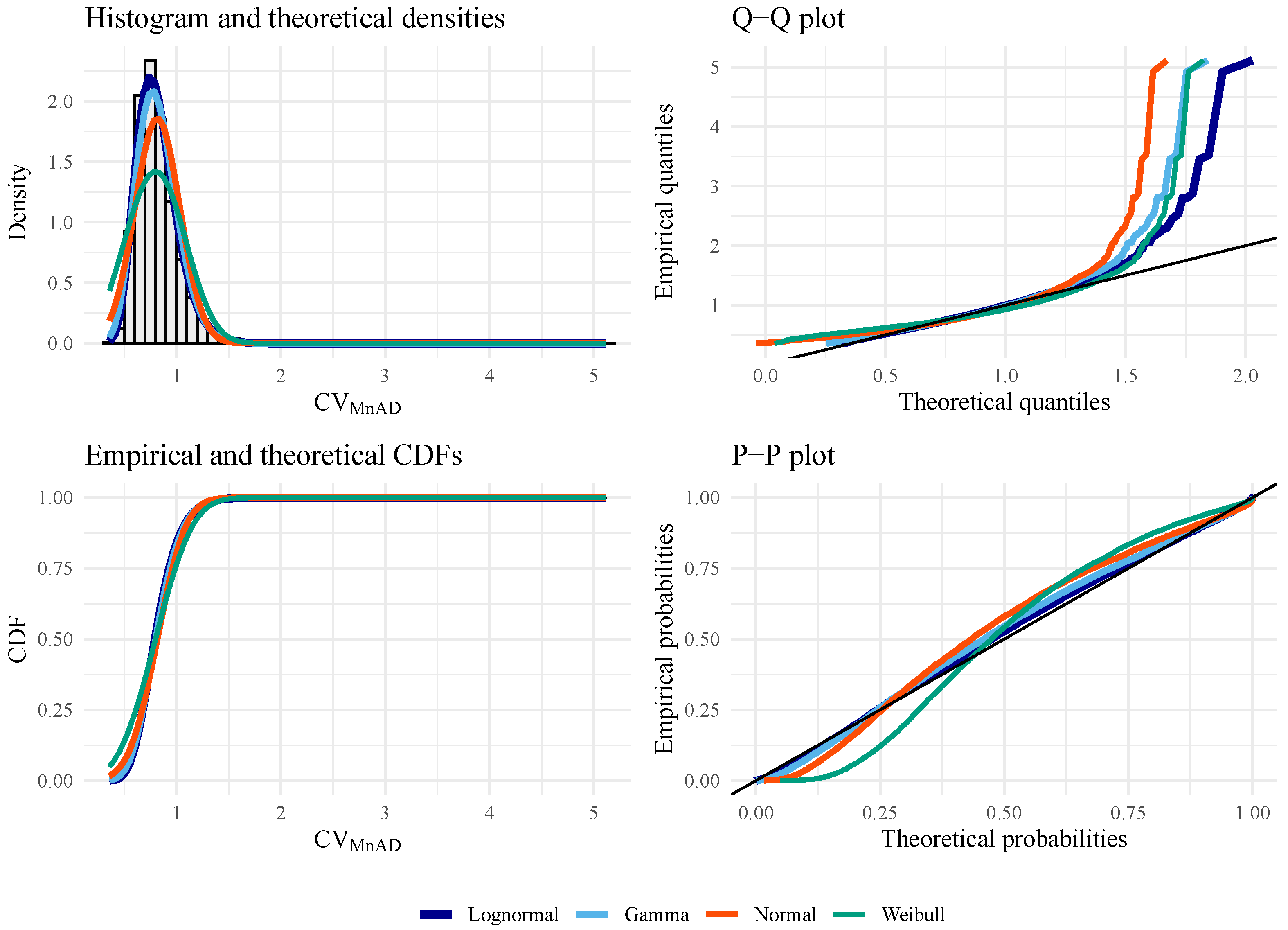
Figure 5.
Goodness-of-fit plots for evaluating the best distribution with CV data from (under the null hypothesis) with , , and .
Figure 5.
Goodness-of-fit plots for evaluating the best distribution with CV data from (under the null hypothesis) with , , and .
Figure 6.
Goodness-of-fit plots for evaluating the best distribution with CV data from (under the alternative hypothesis) with , , , and .
Figure 6.
Goodness-of-fit plots for evaluating the best distribution with CV data from (under the alternative hypothesis) with , , , and .
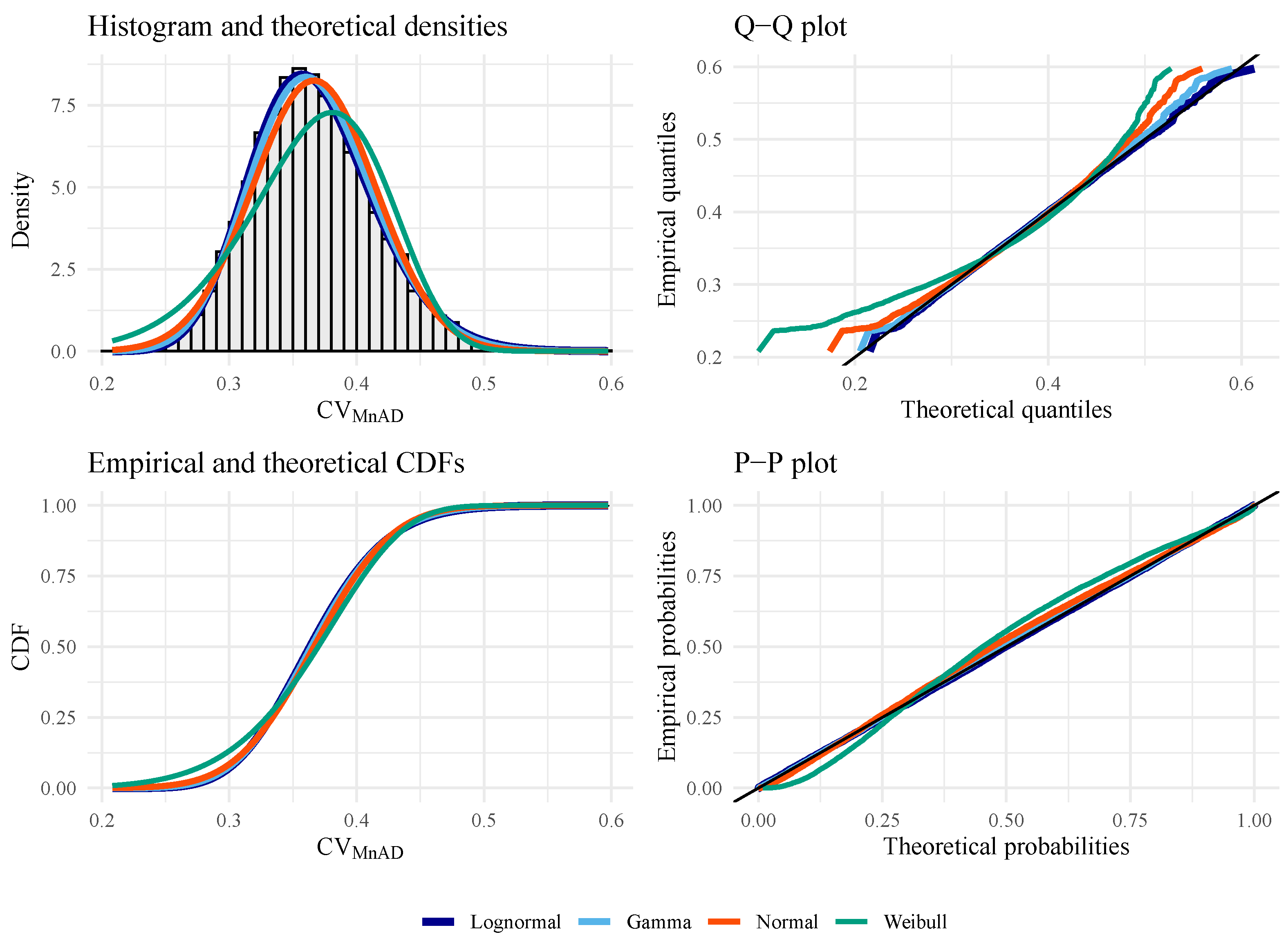
Figure 7.
Goodness-of-fit plots for evaluating the best distribution with data from (under the null hypothesis) with , , and .
Figure 7.
Goodness-of-fit plots for evaluating the best distribution with data from (under the null hypothesis) with , , and .
Figure 8.
Goodness-of-fit plots for evaluating the best distribution with data from (under the alternative hypothesis) with , , , and .
Figure 8.
Goodness-of-fit plots for evaluating the best distribution with data from (under the alternative hypothesis) with , , , and .
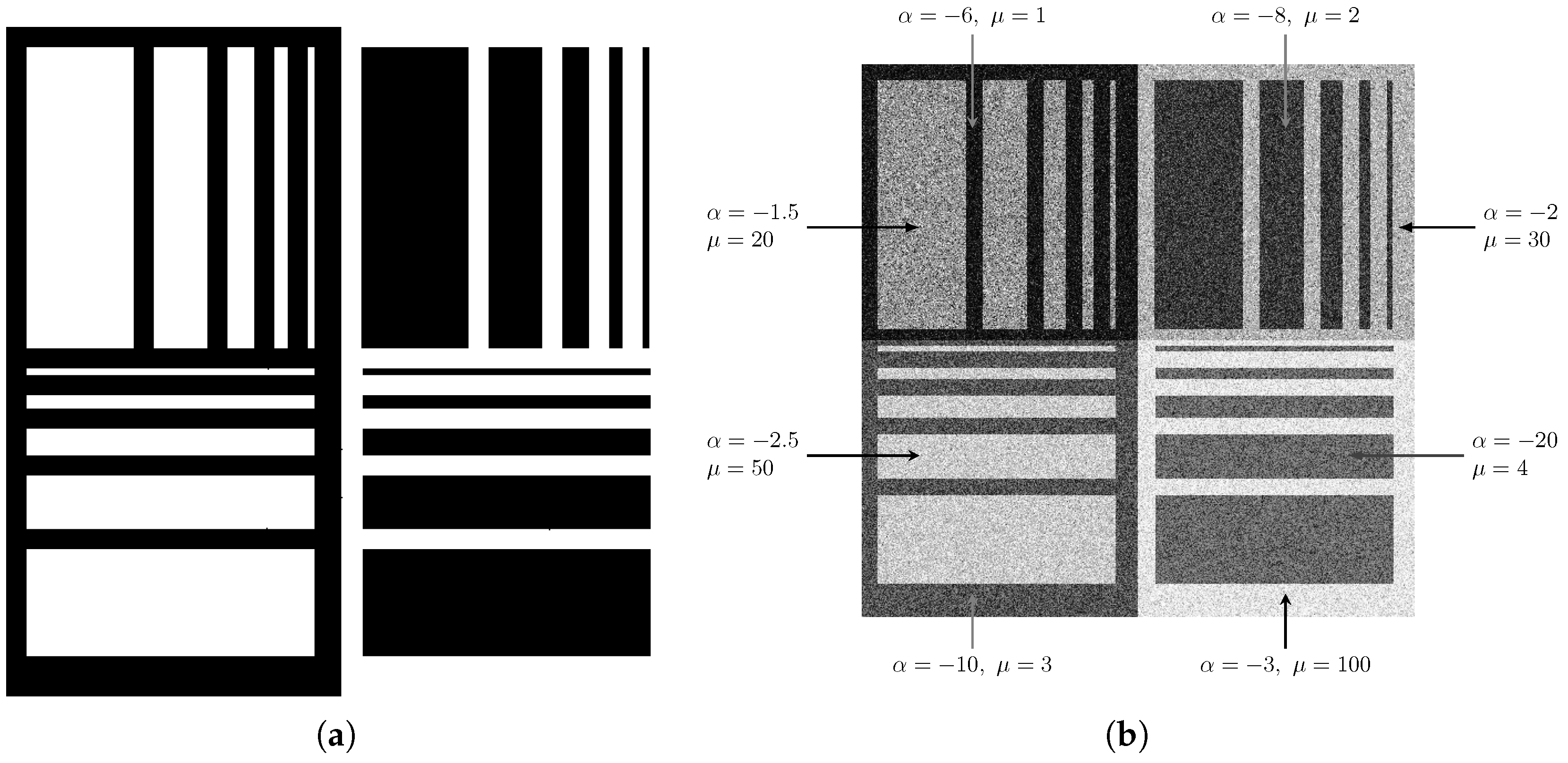
Figure 9.
Synthetic dataset: (a) Phantom. (b) Simulated image, varying and , with .
Figure 9.
Synthetic dataset: (a) Phantom. (b) Simulated image, varying and , with .
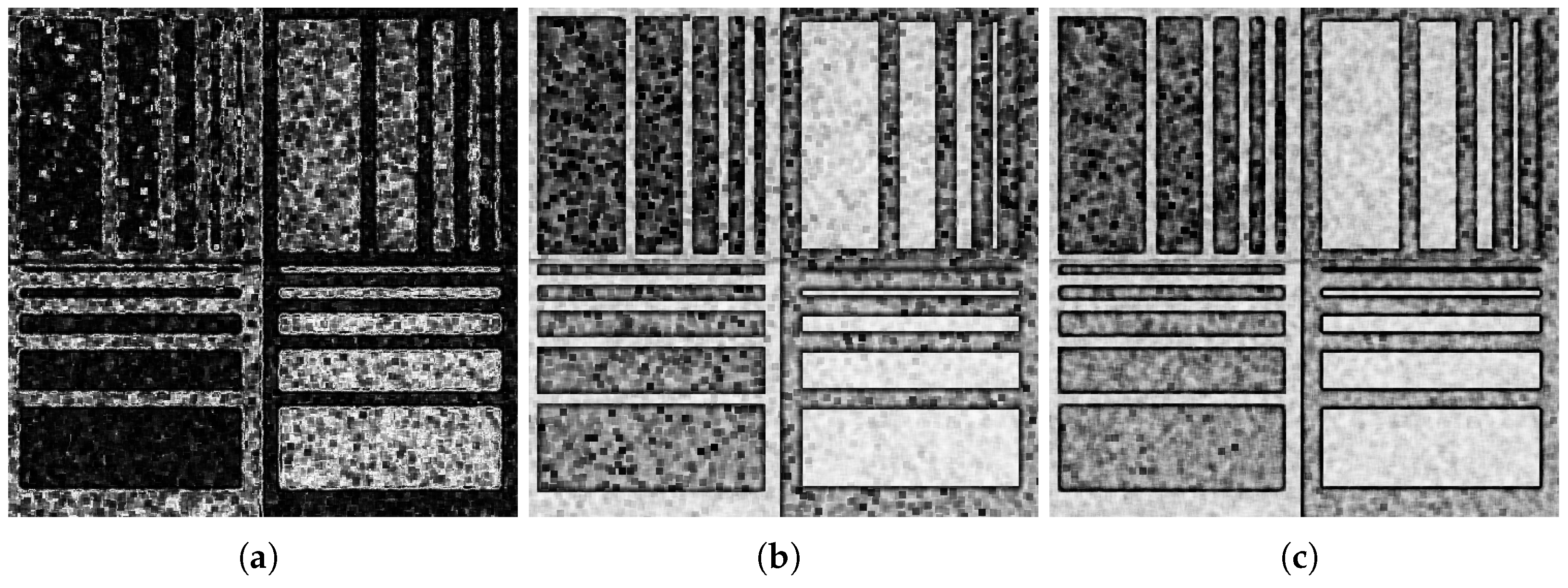
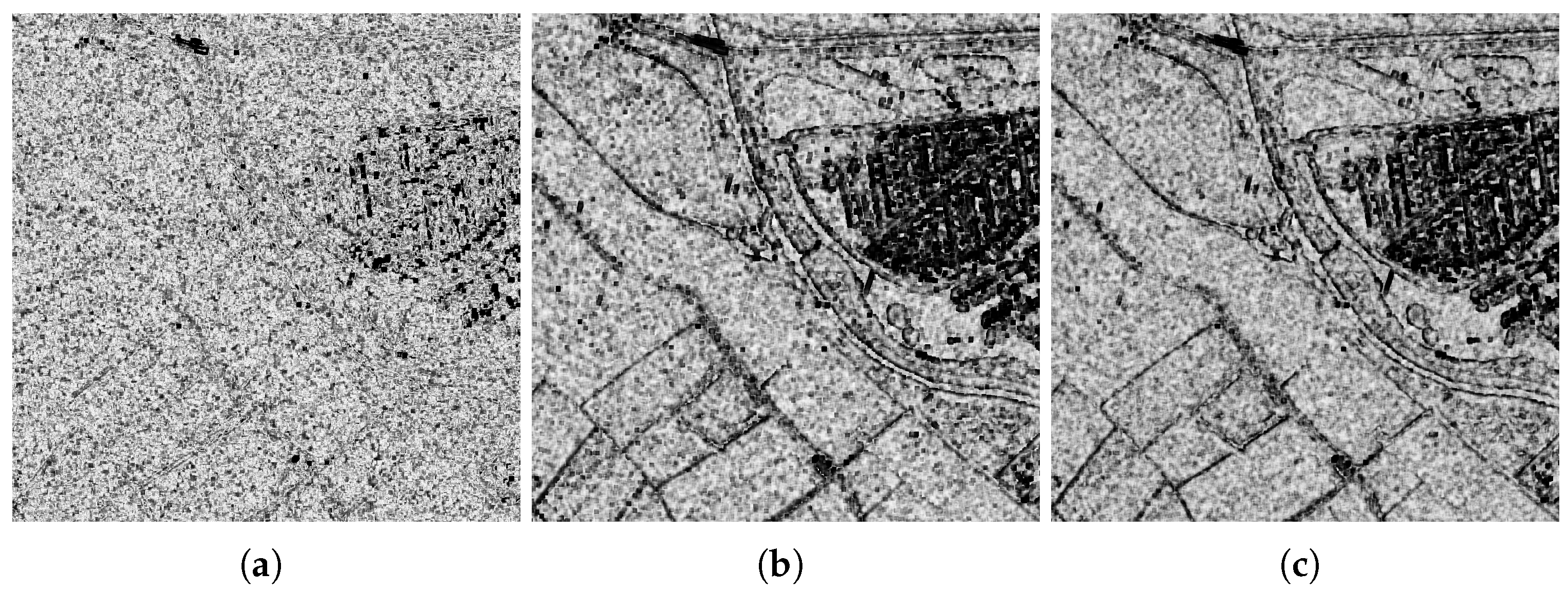
Figure 10.
Results of applying the test statistics: (a) , (b) , and (c) .
Figure 10.
Results of applying the test statistics: (a) , (b) , and (c) .
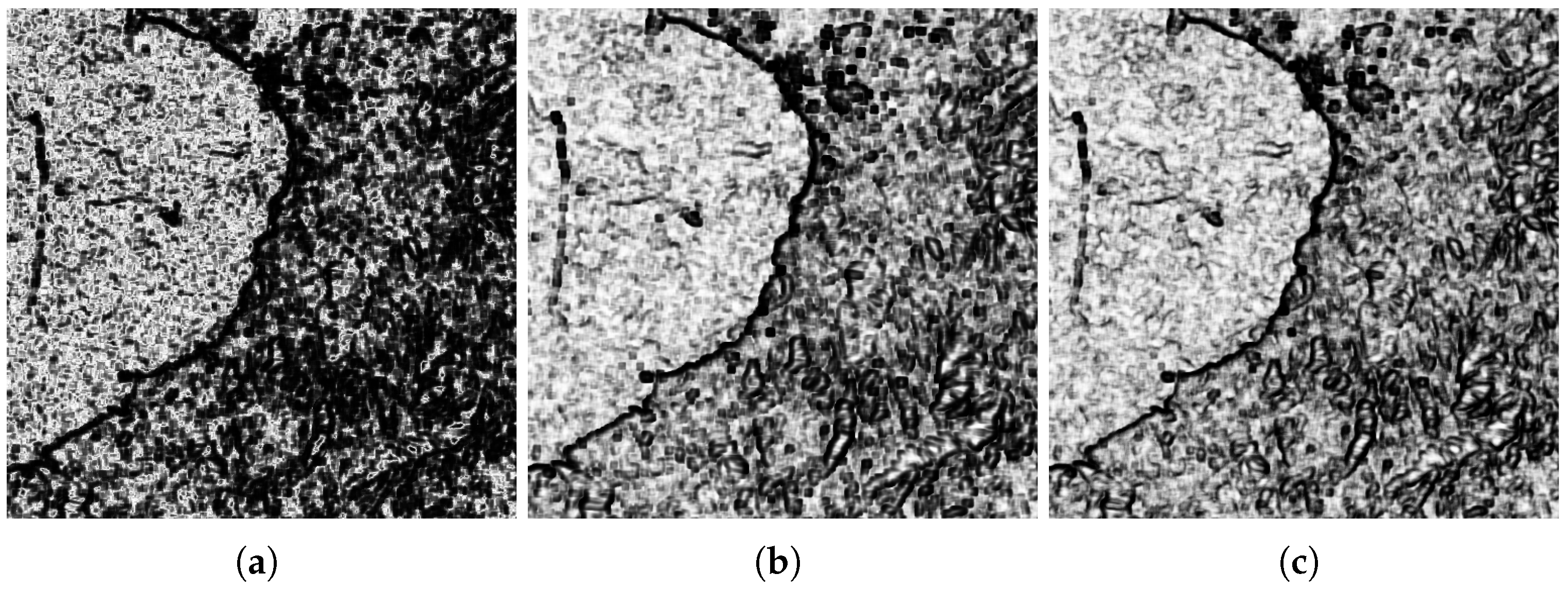
Figure 11.
Map of p-values: (a) , (b) , and (c) .
Figure 11.
Map of p-values: (a) , (b) , and (c) .
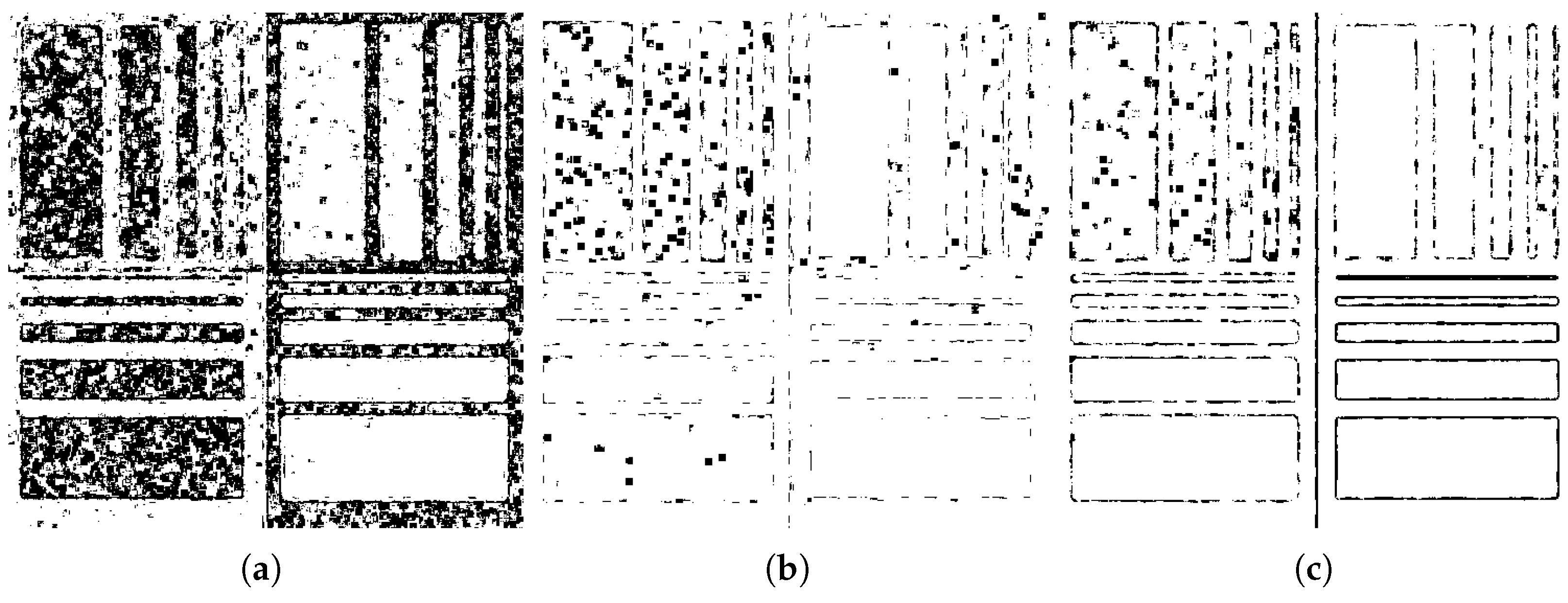
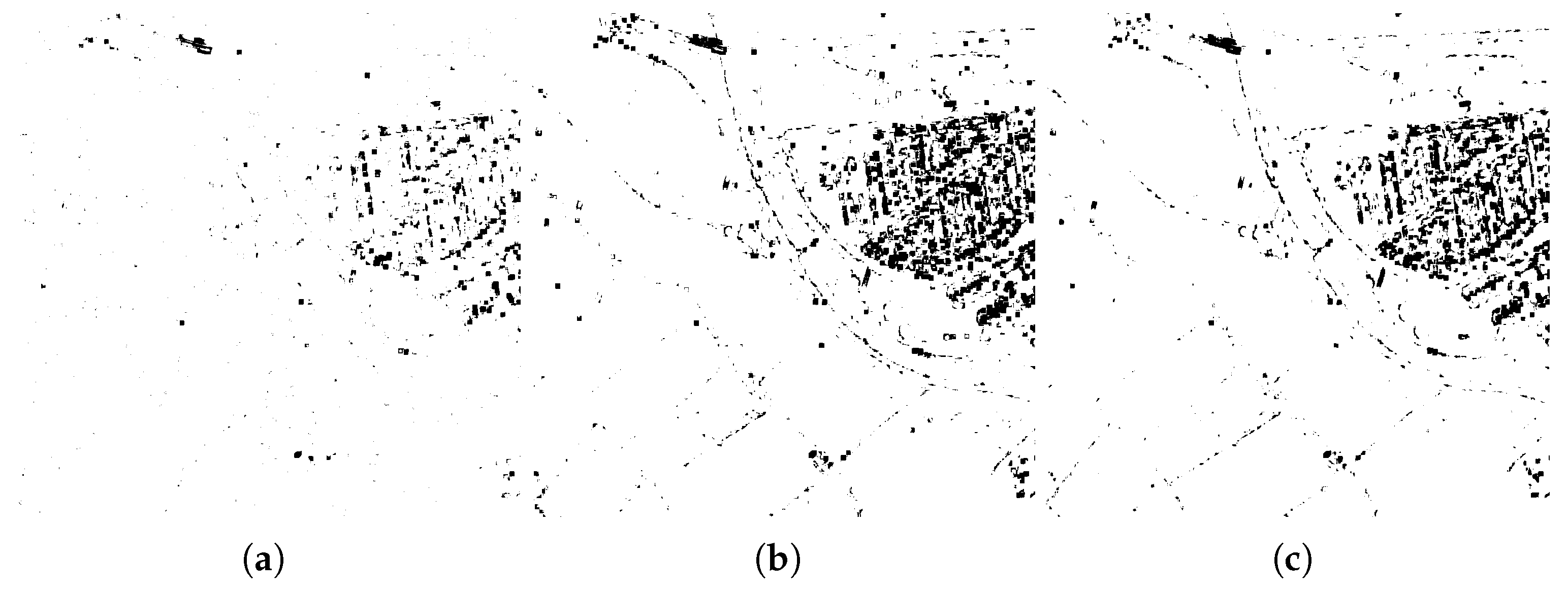
Figure 12.
Results for a threshold of of the p-value: (a) , (b) , and (c) .
Figure 12.
Results for a threshold of of the p-value: (a) , (b) , and (c) .
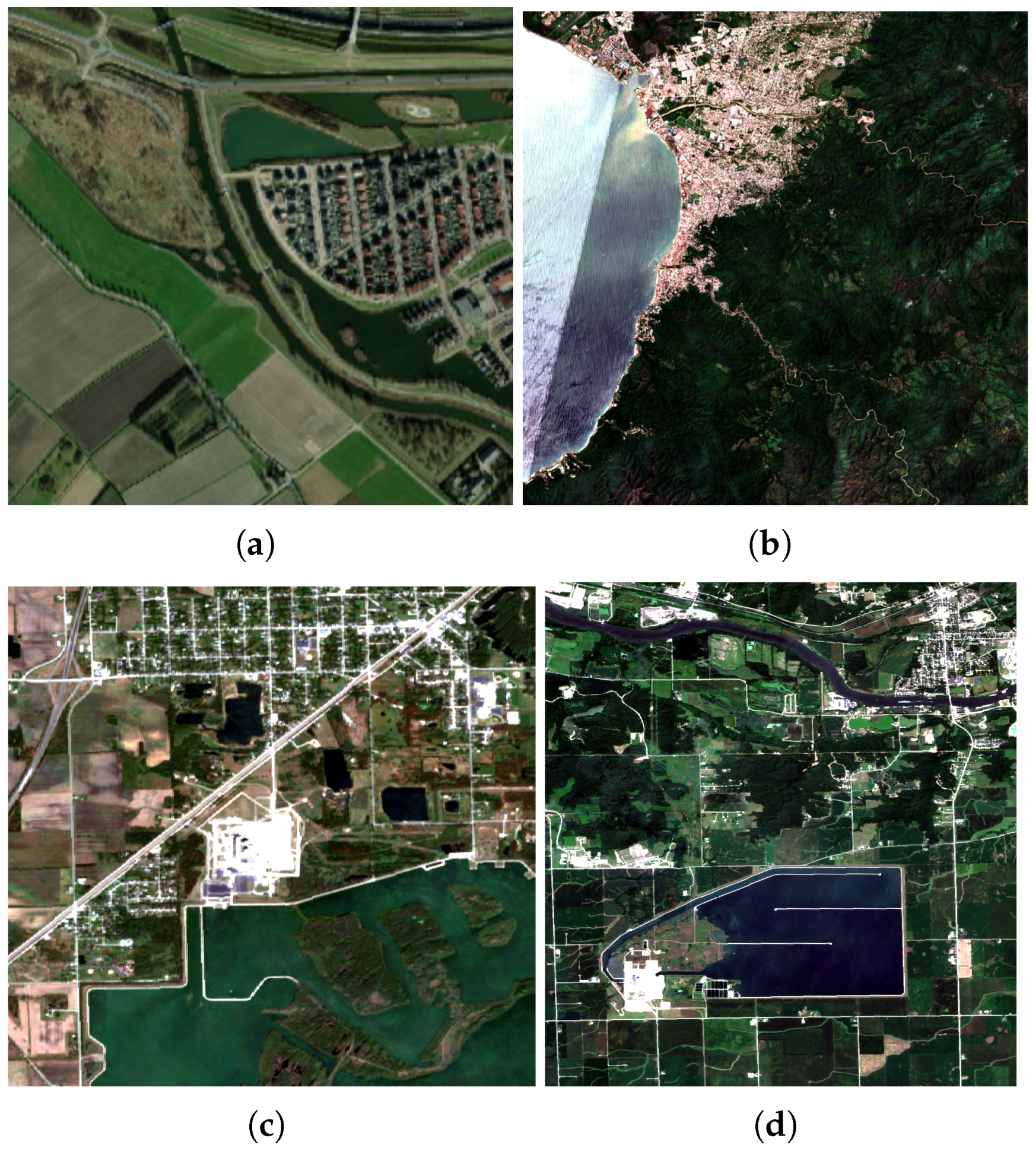
Figure 13.
SAR images: (a) Rotterdam, (b) Coast of Jalisco, (c) Illinois-Region 1, (d) Illinois-Region 2.
Figure 13.
SAR images: (a) Rotterdam, (b) Coast of Jalisco, (c) Illinois-Region 1, (d) Illinois-Region 2.
Figure 14.
Optical images: (a) Rotterdam, (b) Coast Jalisco, (c) Illinois-Region 1, (d) Illinois-Region 2.
Figure 14.
Optical images: (a) Rotterdam, (b) Coast Jalisco, (c) Illinois-Region 1, (d) Illinois-Region 2.
Figure 15.
Results of applying the test statistics, Rotterdam: (a) , (b) , and (c) .
Figure 15.
Results of applying the test statistics, Rotterdam: (a) , (b) , and (c) .
Figure 16.
Results of applying the test statistics, Coast of Jalisco: (a) , (b) , and (c) .
Figure 16.
Results of applying the test statistics, Coast of Jalisco: (a) , (b) , and (c) .
Figure 17.
Results of applying the test statistics, Illinois-Region 1: (a) , (b) , and (c) .
Figure 17.
Results of applying the test statistics, Illinois-Region 1: (a) , (b) , and (c) .
Figure 18.
Results of applying the test statistics, Illinois-Region 2: (a) , (b) , and (c) .
Figure 18.
Results of applying the test statistics, Illinois-Region 2: (a) , (b) , and (c) .
Figure 19.
Map of p-values, Rotterdam: (a) . (b) . (c) .
Figure 19.
Map of p-values, Rotterdam: (a) . (b) . (c) .
Figure 20.
Map of p-values, Coast of Jalisco: (a) . (b) . (c) .
Figure 20.
Map of p-values, Coast of Jalisco: (a) . (b) . (c) .
Figure 21.
Map of p-values, Illinois-Region 1: (a) , (b) , and (c) .
Figure 21.
Map of p-values, Illinois-Region 1: (a) , (b) , and (c) .
Figure 22.
Map of p-values, Illinois-Region 2: (a) , (b) , and (c) .
Figure 22.
Map of p-values, Illinois-Region 2: (a) , (b) , and (c) .
Figure 23.
Results for a threshold of of the p-value, Rotterdam. (a) , (b) , and (c) .
Figure 23.
Results for a threshold of of the p-value, Rotterdam. (a) , (b) , and (c) .
Figure 24.
Results for a threshold of of the p-value, Coast of Jalisco. (a) , (b) , and (c) .
Figure 24.
Results for a threshold of of the p-value, Coast of Jalisco. (a) , (b) , and (c) .
Figure 25.
Results for a threshold of of the p-value, Illinois-Region 1. (a) , (b) , and (c) .
Figure 25.
Results for a threshold of of the p-value, Illinois-Region 1. (a) , (b) , and (c) .
Figure 26.
Results for a threshold of of the p-value, Illinois-Region 2. (a) , (b) , and (c) .
Figure 26.
Results for a threshold of of the p-value, Illinois-Region 2. (a) , (b) , and (c) .
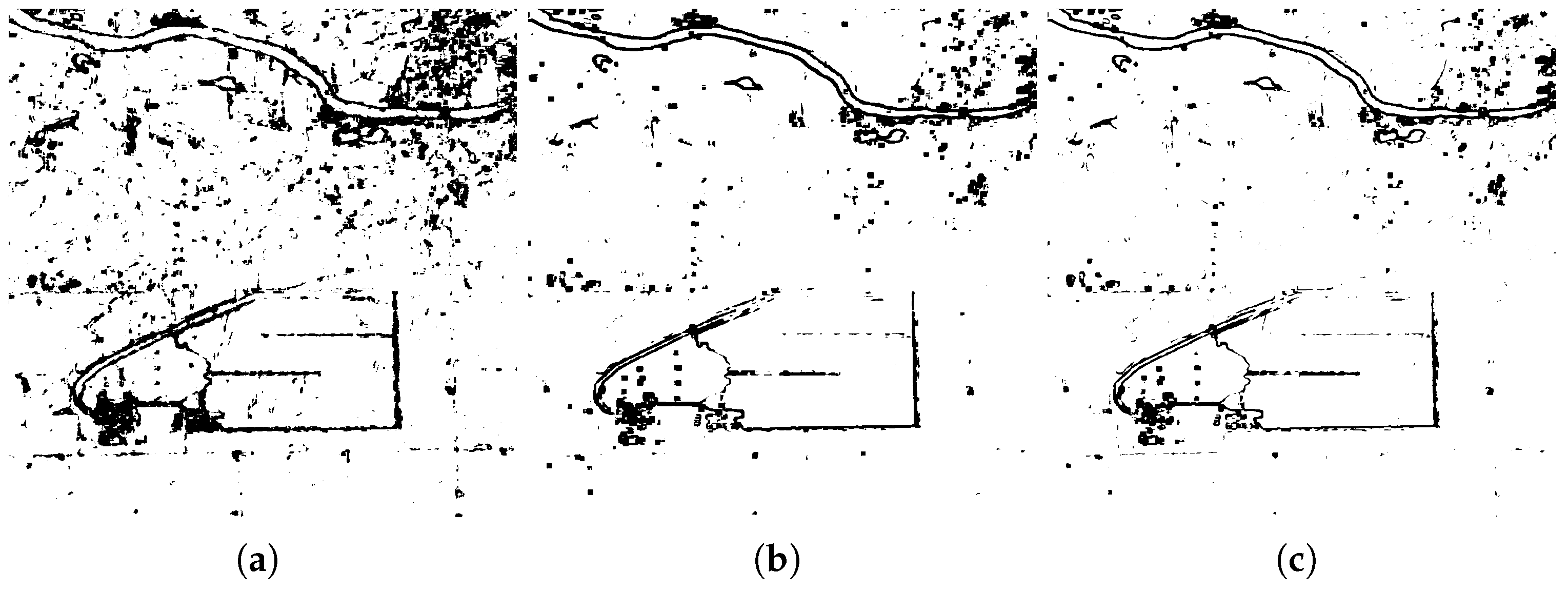
Figure 27.
Image of Illinois-Region 2 with reference samples.
Figure 27.
Image of Illinois-Region 2 with reference samples.
Table 1.
Bias and MSE of the entropy estimators for the with .
Table 1.
Bias and MSE of the entropy estimators for the with .
| | | Bias | MSE |
|---|
| | | | | | | | | | | | | |
| 1 | 9 | | | | | | | | | | | | |
| 25 | | | | | | | | | | | | |
| 49 | | | | | | | | | | | | |
| 81 | | | | | | | | | | | | |
| 121 | | | | | | | | | | | | |
| 10 | 9 | | | | | | | | | | | | |
| 25 | | | | | | | | | | | | |
| 49 | | | | | | | | | | | | |
| 81 | | | | | | | | | | | | |
| 121 | | | | | | | | | | | | |
Table 2.
Tests accuracy and processing time for each bootstrap-improved estimator.
Table 2.
Tests accuracy and processing time for each bootstrap-improved estimator.
| Estimator | L | n | | Time (s) |
|---|
| 2 | 25 | | 22.53 |
| 49 | | 40.35 |
| 81 | | 63.93 |
| 121 | | 97.06 |
| 8 | 25 | | 22.25 |
| 49 | | 33.42 |
| 81 | | 50.94 |
| 121 | | 97.35 |
| 2 | 25 | | 4.66 |
| 49 | | 5.55 |
| 81 | | 6.89 |
| 121 | | 7.90 |
| 8 | 25 | | 4.81 |
| 49 | | 5.43 |
| 81 | | 6.38 |
| 121 | | 7.46 |
| 2 | 25 | | 4.61 |
| 49 | | 5.19 |
| 81 | | 6.70 |
| 121 | | 7.41 |
| 8 | 25 | | 4.74 |
| 49 | | 5.35 |
| 81 | | 6.21 |
| 121 | | 7.48 |
Table 3.
Descriptive analysis of , with and .
Table 3.
Descriptive analysis of , with and .
| L | n | Mean | SD | Var | SK | EK | p-Value |
|---|
| 3 | 25 | | | | | | |
| 49 | | | | | | |
| 81 | | | | | | |
| 121 | | | | | | |
| 200 | | | | | | |
| 5 | 25 | | | | | | |
| 49 | | | | | | |
| 81 | | | | | | |
| 121 | | | | | | |
| 200 | | | | | | |
| 8 | 25 | | | | | | |
| 49 | | | | | | |
| 81 | | | | | | |
| 121 | | | | | | |
| 200 | | | | | | |
| 11 | 25 | | | | | | |
| 49 | | | | | | |
| 81 | | | | | | |
| 121 | | | | | | |
| 200 | | | | | | |
Table 4.
Size and power of the test statistic.
Table 4.
Size and power of the test statistic.
| | | Size | Power |
|---|
| | | | | | | |
|---|
| 3 | 25 | | | | | | |
| 49 | | | | | | |
| 81 | | | | | | |
| 121 | | | | | | |
| 5 | 25 | | | | | | |
| 49 | | | | | | |
| 81 | | | | | | |
| 121 | | | | | | |
| 8 | 25 | | | | | | |
| 49 | | | | | | |
| 81 | | | | | | |
| 121 | | | | | | |
| 11 | 25 | | | | | | |
| 49 | | | | | | |
| 81 | | | | | | |
| 121 | | | | | | |
Table 5.
AIC and BIC values for evaluating the best distribution with CV data from .
Table 5.
AIC and BIC values for evaluating the best distribution with CV data from .
| Criterion | n | Normal | Lognormal | Gamma | Weibull | Inverse Gaussian |
|---|
| AIC | 25 | | | | | |
| 49 | | | | | |
| 81 | | | | | |
| 121 | | | | | |
| BIC | 25 | | | | | |
| 49 | | | | | |
| 81 | | | | | |
| 121 | | | | | |
Table 6.
AIC and BIC values for evaluating the best distribution with CV data from .
Table 6.
AIC and BIC values for evaluating the best distribution with CV data from .
| Criterion | n | Normal | Lognormal | Gamma | Weibull | Inverse Gaussian |
|---|
| AIC | 25 | | | | | |
| 49 | | | | | |
| 81 | | | | | |
| 121 | | | | | |
| BIC | 25 | | | | | |
| 49 | | | | | |
| 81 | | | | | |
| 121 | | | | | |
Table 7.
AIC and BIC values for evaluating the best distribution with data from .
Table 7.
AIC and BIC values for evaluating the best distribution with data from .
| Criterion | n | Normal | Lognormal | Gamma | Weibull | Inverse Gaussian |
|---|
| AIC | 25 | | | | | |
| 49 | | | | | |
| 81 | | | | | |
| 121 | | | | | |
| BIC | 25 | | | | | |
| 49 | | | | | |
| 81 | | | | | |
| 121 | | | | | |
Table 8.
AIC and BIC values for evaluating the best distribution with data from .
Table 8.
AIC and BIC values for evaluating the best distribution with data from .
| Criterion | n | Normal | Lognormal | Gamma | Weibull | Inverse Gaussian |
|---|
| AIC | 25 | | | | | |
| 49 | | | | | |
| 81 | | | | | |
| 121 | | | | | |
| BIC | 25 | | | | | |
| 49 | | | | | |
| 81 | | | | | |
| 121 | | | | | |
Table 9.
Parameters of selected SAR images.
Table 9.
Parameters of selected SAR images.
| Site | Mission | Mode | Band | Polarization | Size | L | Resolution Rg/Az [m] | Acquisition Date |
|---|
| Rotterdam | TerraSAR-X | HS | X | HH | | 1 | | 06-12-2018 |
| Coast of Jalisco | Sentinel-1B | GRD EW | C | VV | | 18 | | 29-08-2021 |
| Illinois-Region 1 | Sentinel-1B | GRD SM | C | VV | | 36 | | 30-06-2020 |
| Illinois-Region 2 | Sentinel-1B | GRD SM | C | VV | | 36 | | 28-08-2021 |
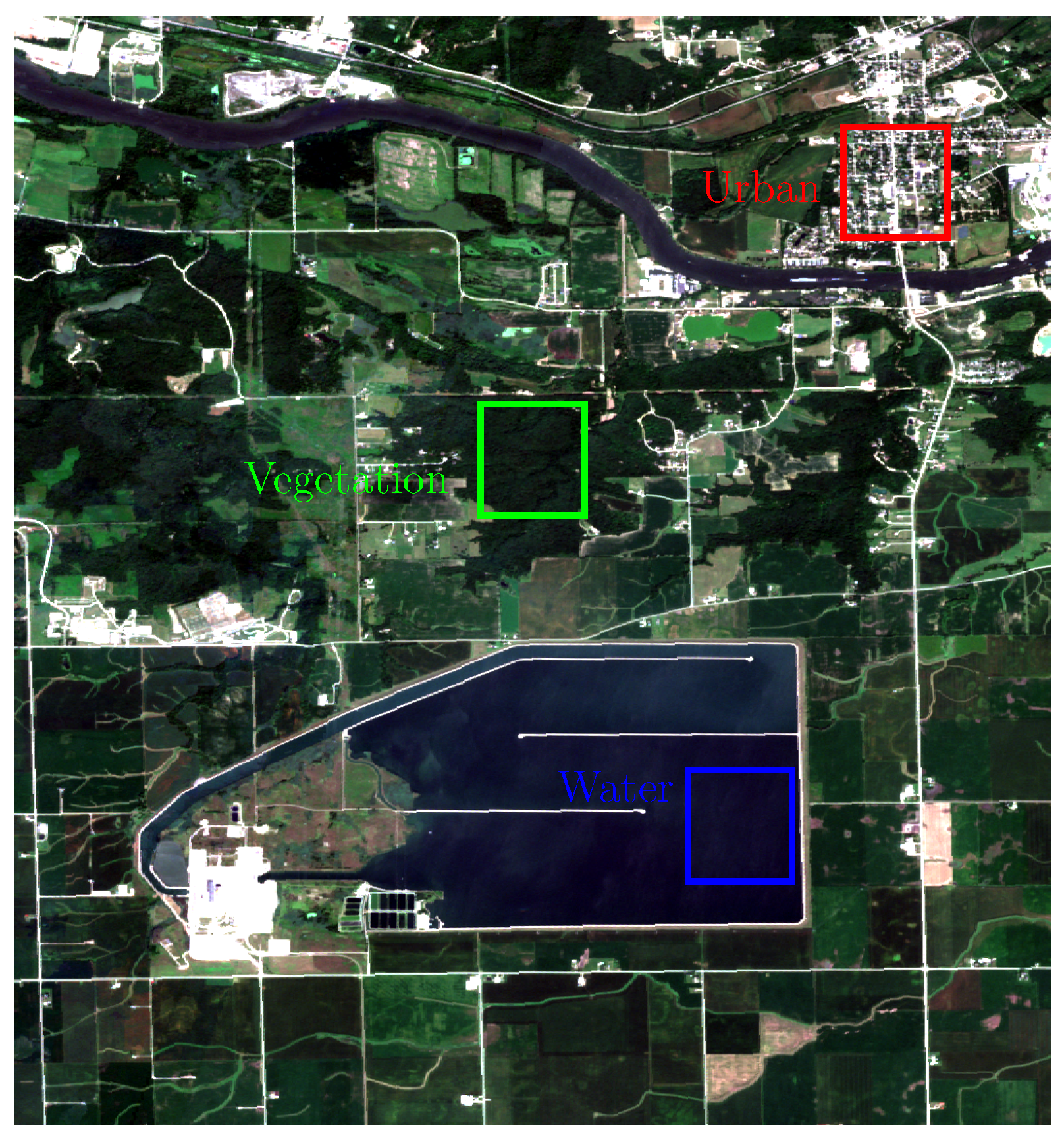
Table 10.
Analysis of test statistics for different areas of reference.
Table 10.
Analysis of test statistics for different areas of reference.
| Area of Reference | Test Statistic | Mean | SD |
|---|
| Water | | 0.05029 | 0.15415 |
| 0.15827 | 0.02584 |
| 0.13013 | 0.02183 |
| Vegetation | | 0.36482 | 0.28489 |
| 0.29421 | 0.10957 |
| 0.24501 | 0.09829 |
| Urban | | 0.82970 | 0.21910 |
| 0.56846 | 0.33938 |
| 0.47176 | 0.29486 |