The Atmospheric Heating Mechanism over the Tharsis Bulge of Mars and the Impact of Global Dust Storms
Abstract
1. Introduction
2. Materials and Methods
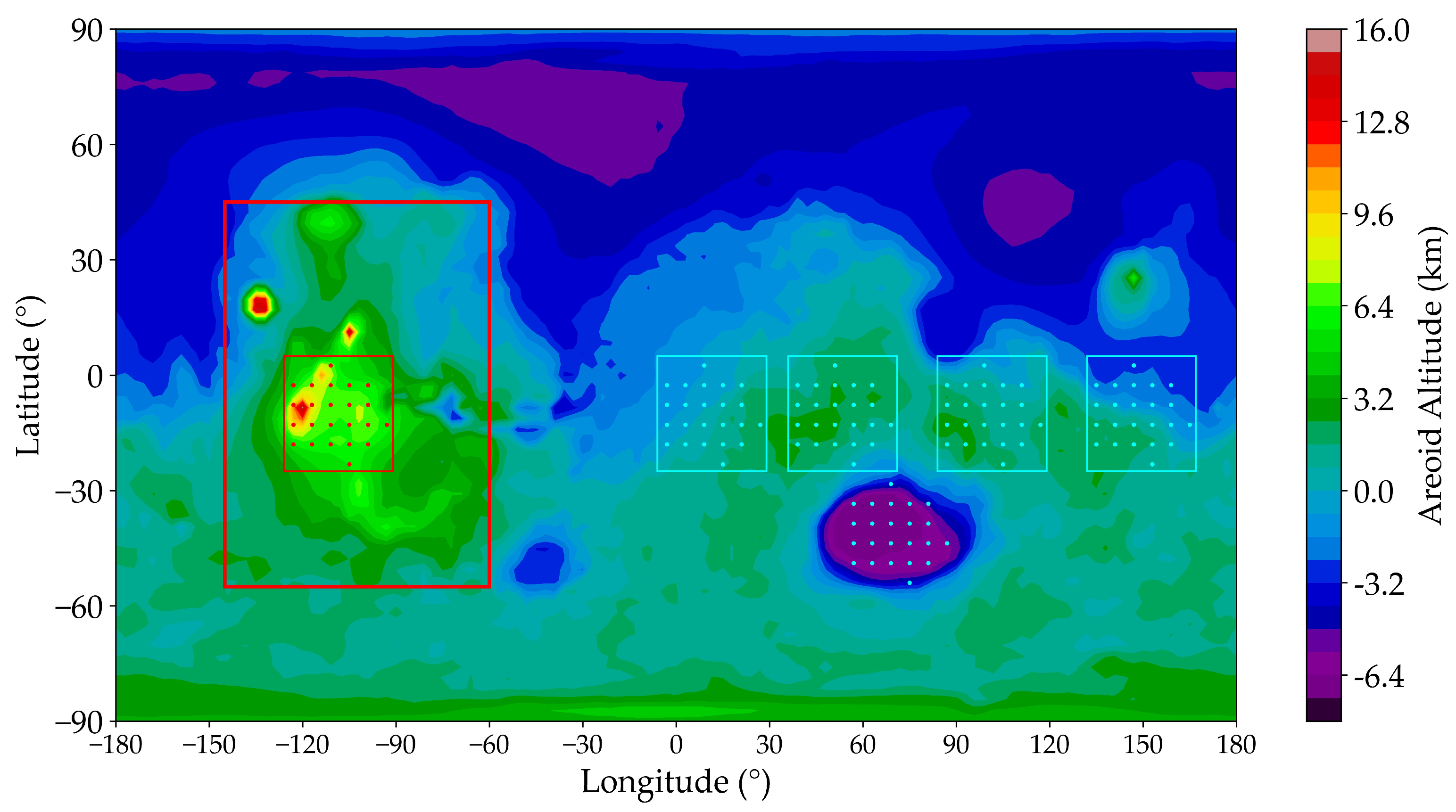
2.1. Data
2.2. Methods
3. Results
3.1. Characteristics of the Planetary Boundary Layer
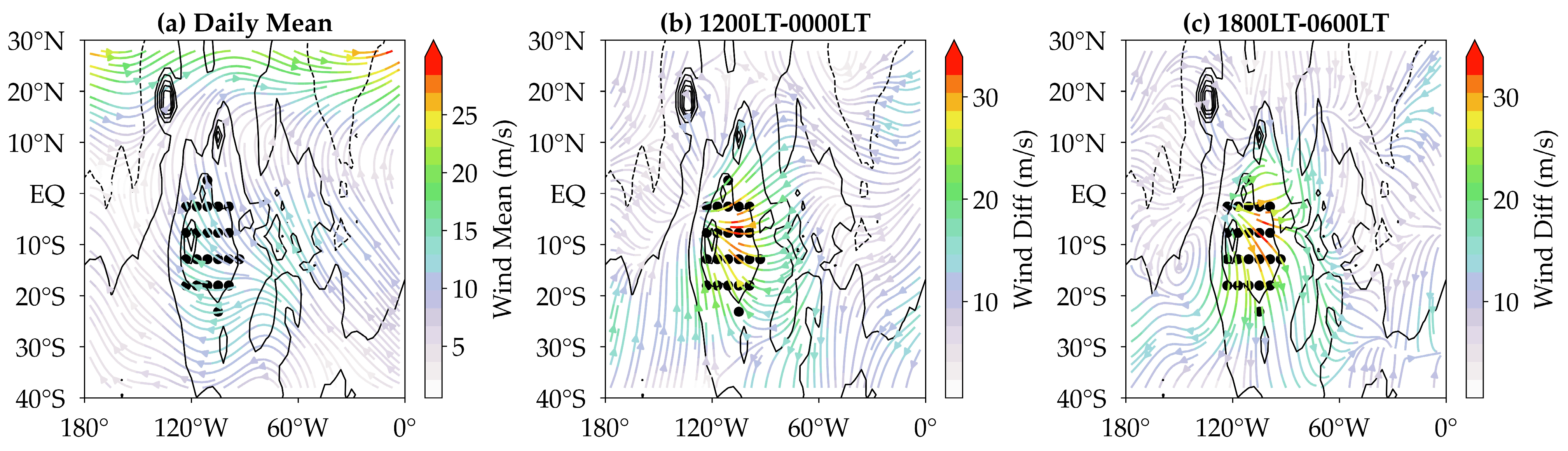
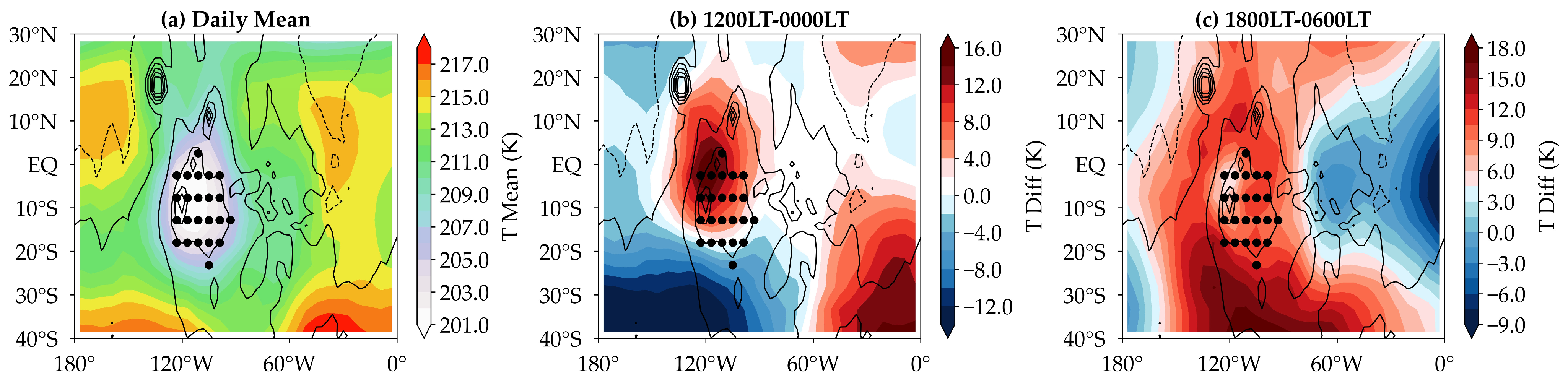
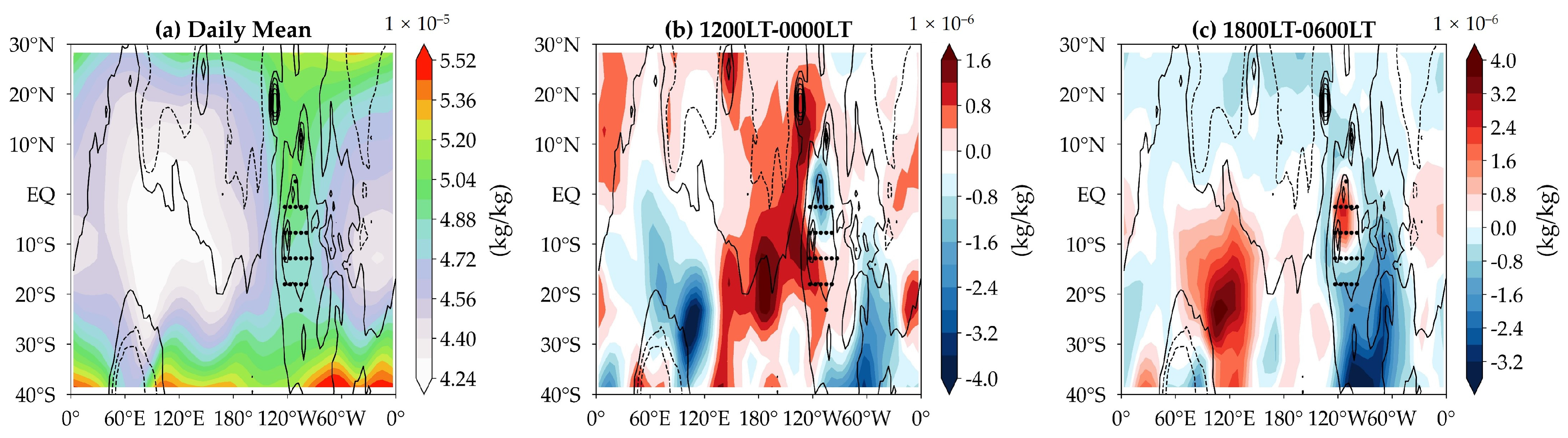
3.2. Basic Analysis of Large-Scale Wind, Pressure, Temperature Fields, and Dust Distribution Characteristics
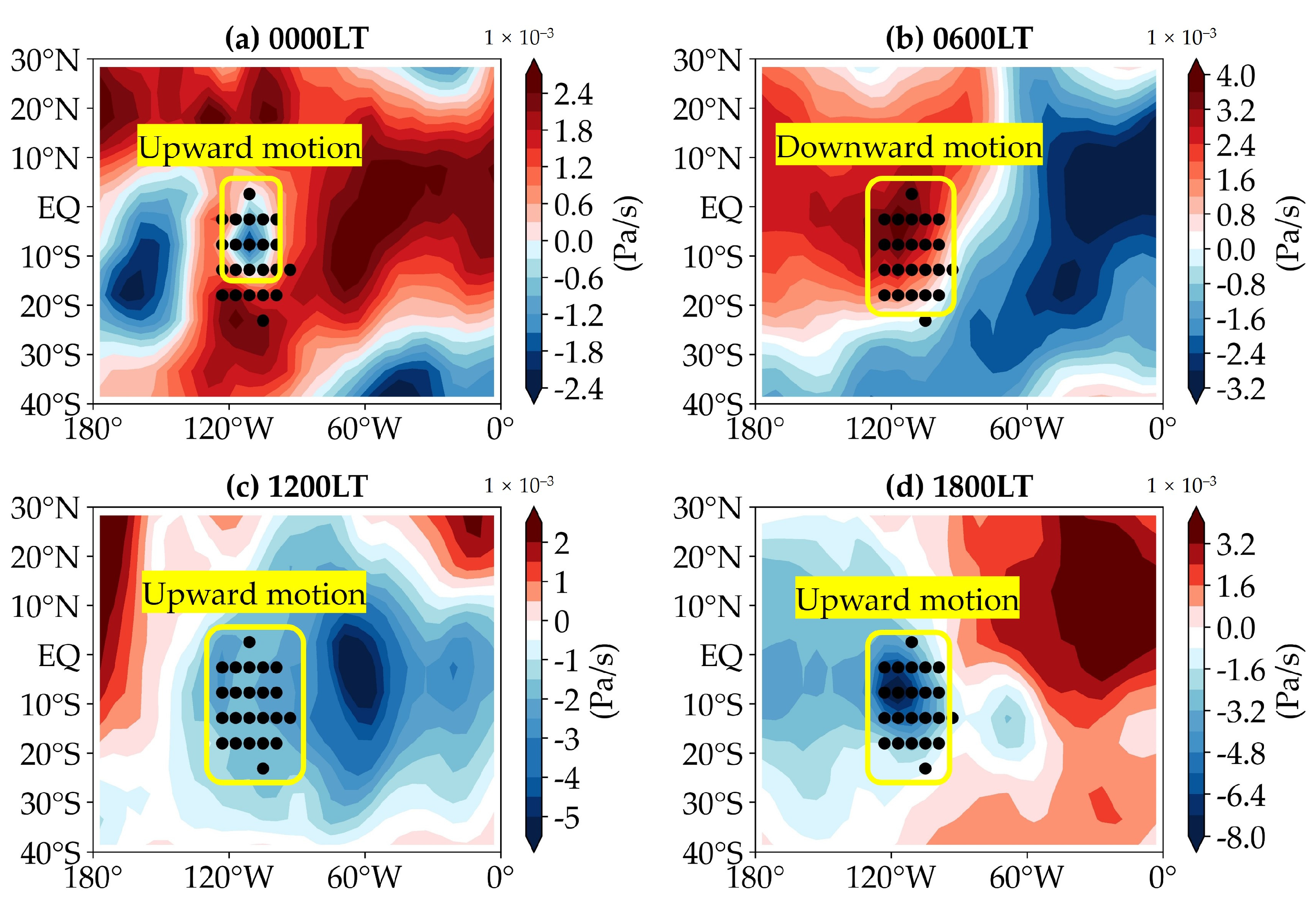
3.3. Plateau Heating Mechanism on Tharsis
3.4. The Impact of GDSs
4. Conclusions
- Our work embarked on an in-depth investigation of the atmospheric characteristics, boundary layer structure, and heating mechanisms over the CH region of Tharsis on Mars. We also discussed the significant GDS impacts on the atmospheric heating mechanisms. Through a series of combination of diurnal and seasonal analyses of dynamics and thermodynamics, we characterized the planetary boundary layer properties, large-scale wind patterns, pressure and temperature fields, and dust distribution.
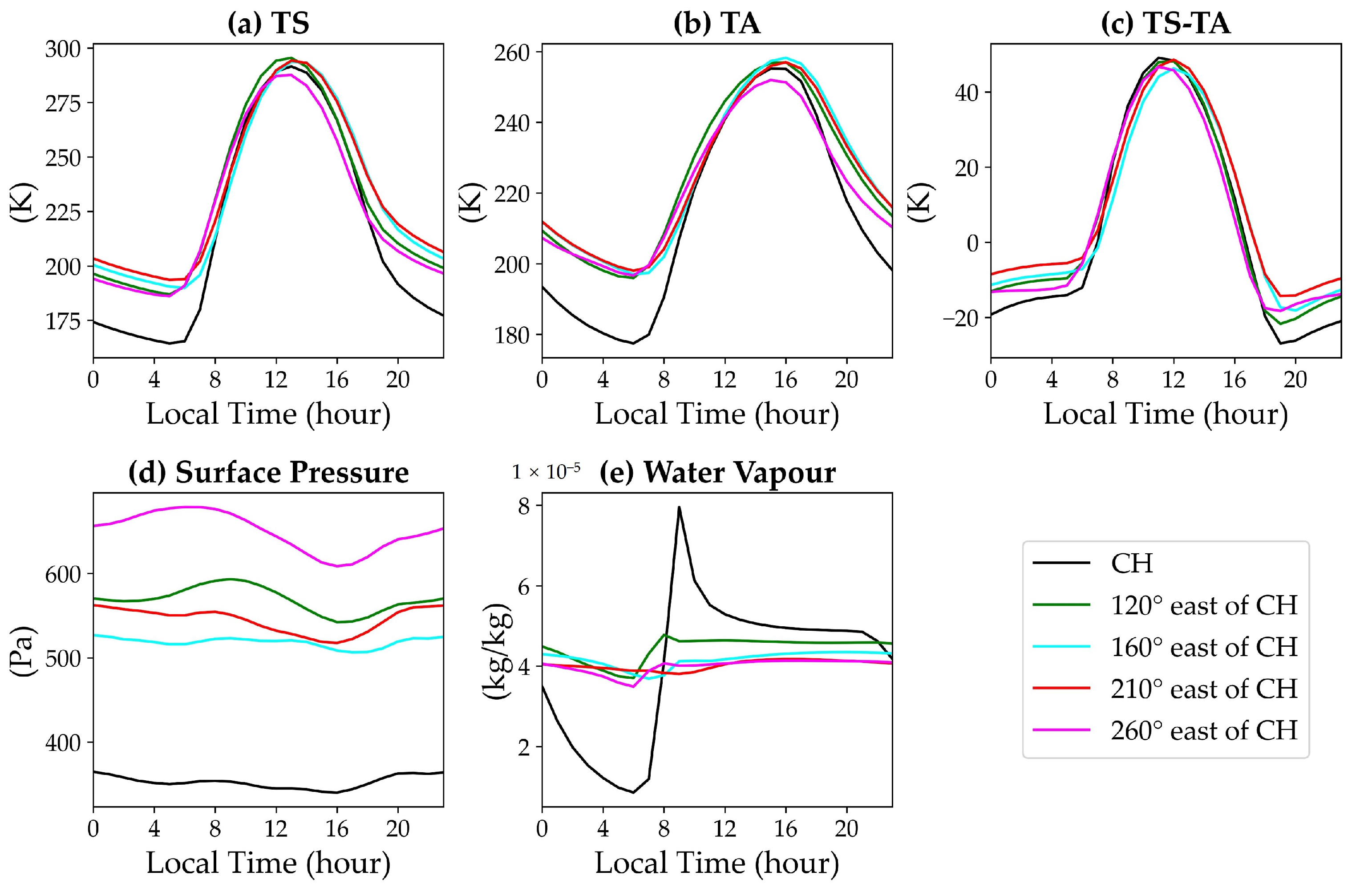
- Extreme Diurnal Temperature Variability: Our research quantified extreme diurnal surface temperature fluctuations, which reached up to 130 K, and the diurnal cycle showed surface pressure swings of approximately 12.5 Pa, dwarfing the Earth’s Tibetan Plateau’s range by a factor of 3 to 4. We also showed that the Tharsis Plateau experiences diurnal surface temperature variations ~25 K higher than the areas at the same latitude, along with diurnal near-surface air temperature fluctuations of ~20 K higher. The water vapor mixing ratio reached its lowest value in the early morning, then rapidly peaked at the local time of 9, showing a different diurnal pattern compared to the Tibetan Plateau on Earth. The boundary layer showed strong convective activity during the day, leading to well-mixed conditions, with a super-adiabatic lapse rate layer indicating strong instability during midday. These characteristics highlight the Tharsis region’s susceptibility to significant thermal extremes compared to previously studied Martian plains or Earth’s high-altitude regions like the Tibetan Plateau, where diurnal temperature variations are considerably less.
- Convective Dynamics and Atmospheric Stability: The pronounced convective activity during daylight, characterized by a super-adiabatic lapse rate indicating strong thermal instability around noon, transitions to a more stabilized atmospheric state by dusk. At the 150 Pa level, there is a massive cold high-pressure system above the plateau, while the CH region’s northwest corner exhibits an average diurnal temperature difference of up to 16 K at nearly the same altitude. This is the first quantification of such dynamic atmospheric behavior over Tharsis, detailing transitions from a noontime instability with a super-adiabatic lapse rate to homogenized conditions by dusk.
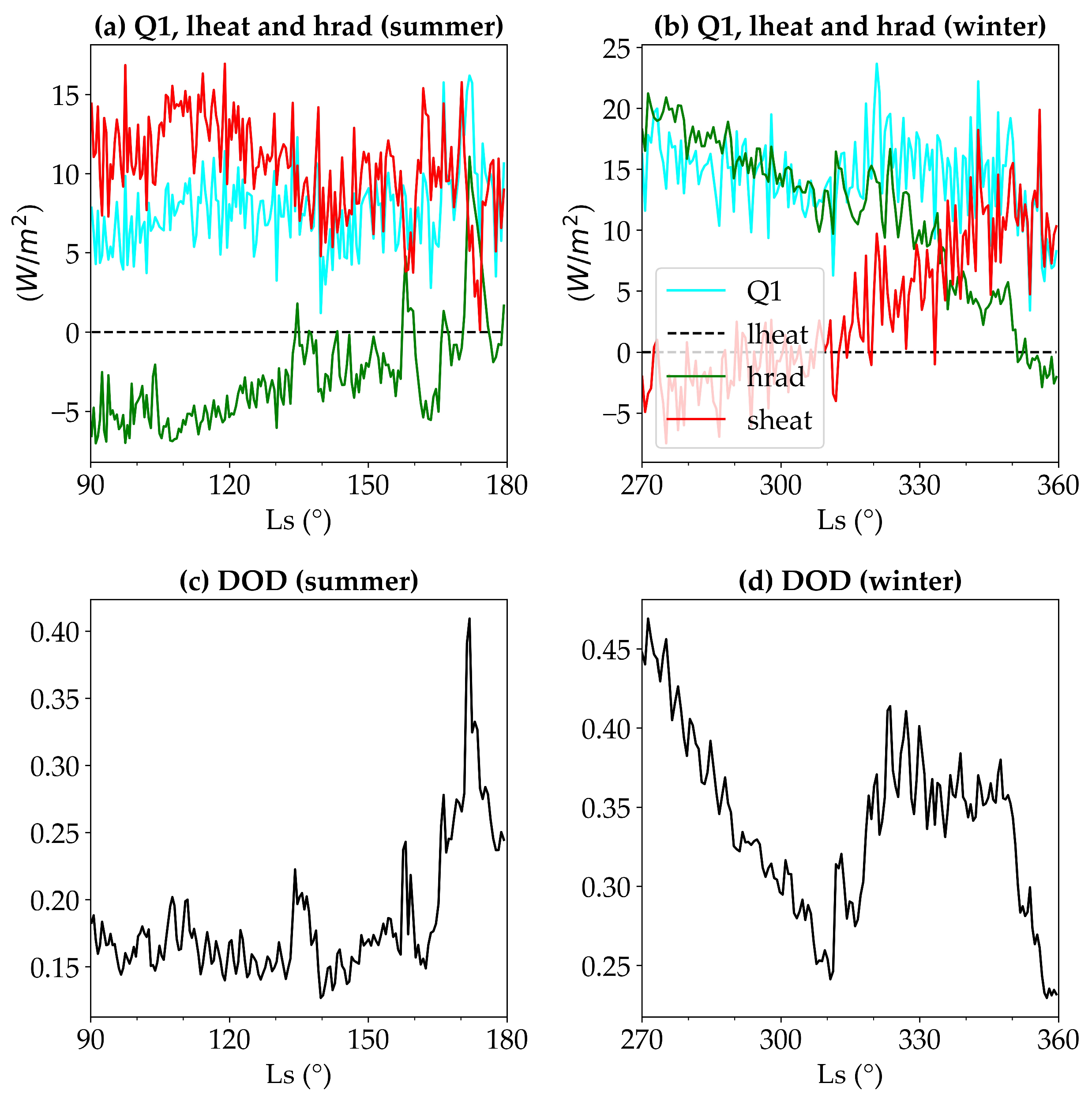
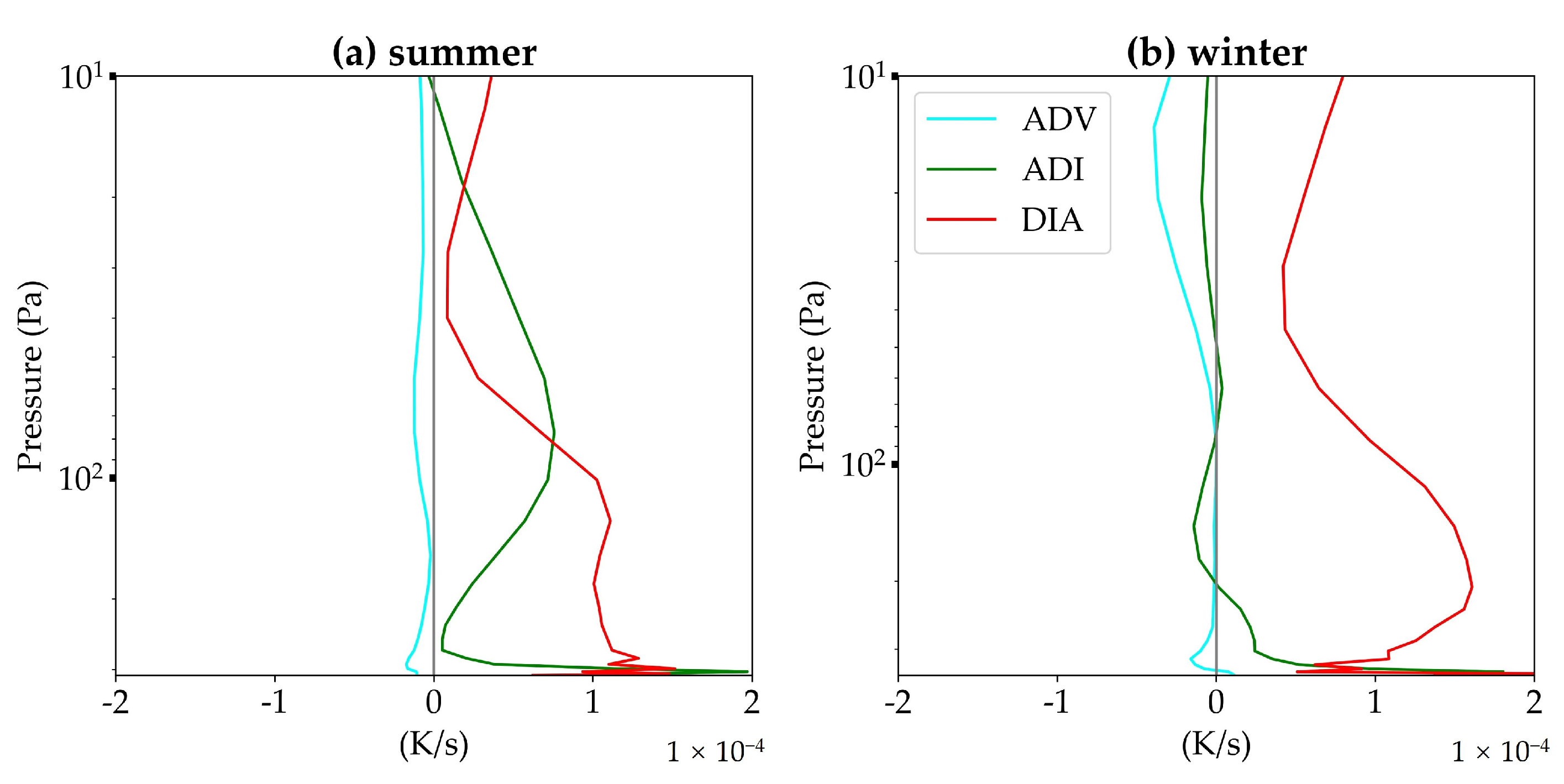
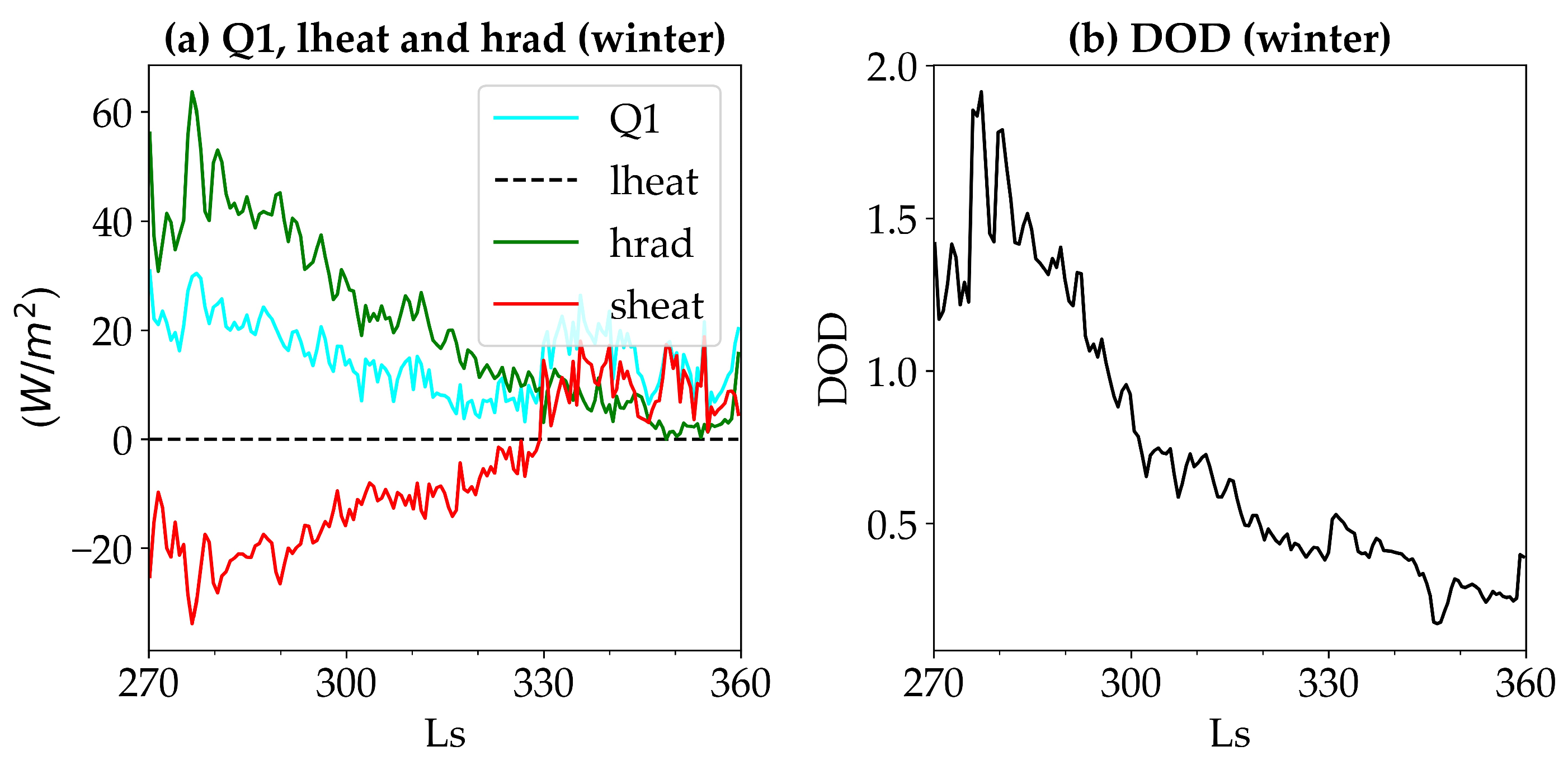
- Seasonal Heating Mechanisms: We delineate the seasonal dominance of sensible and radiative heating. Sensible heat predominantly controls the atmospheric heating in the local summer (10 W/m2), whereas radiative heating prevails during the local winter, which contrasts with earlier assumptions that did not account for such distinct seasonal shifts. The Martian dry state and dusty conditions significantly affected the heating dynamics, with latent heat being negligible, a stark contrast to Earth’s atmospheric dynamics.
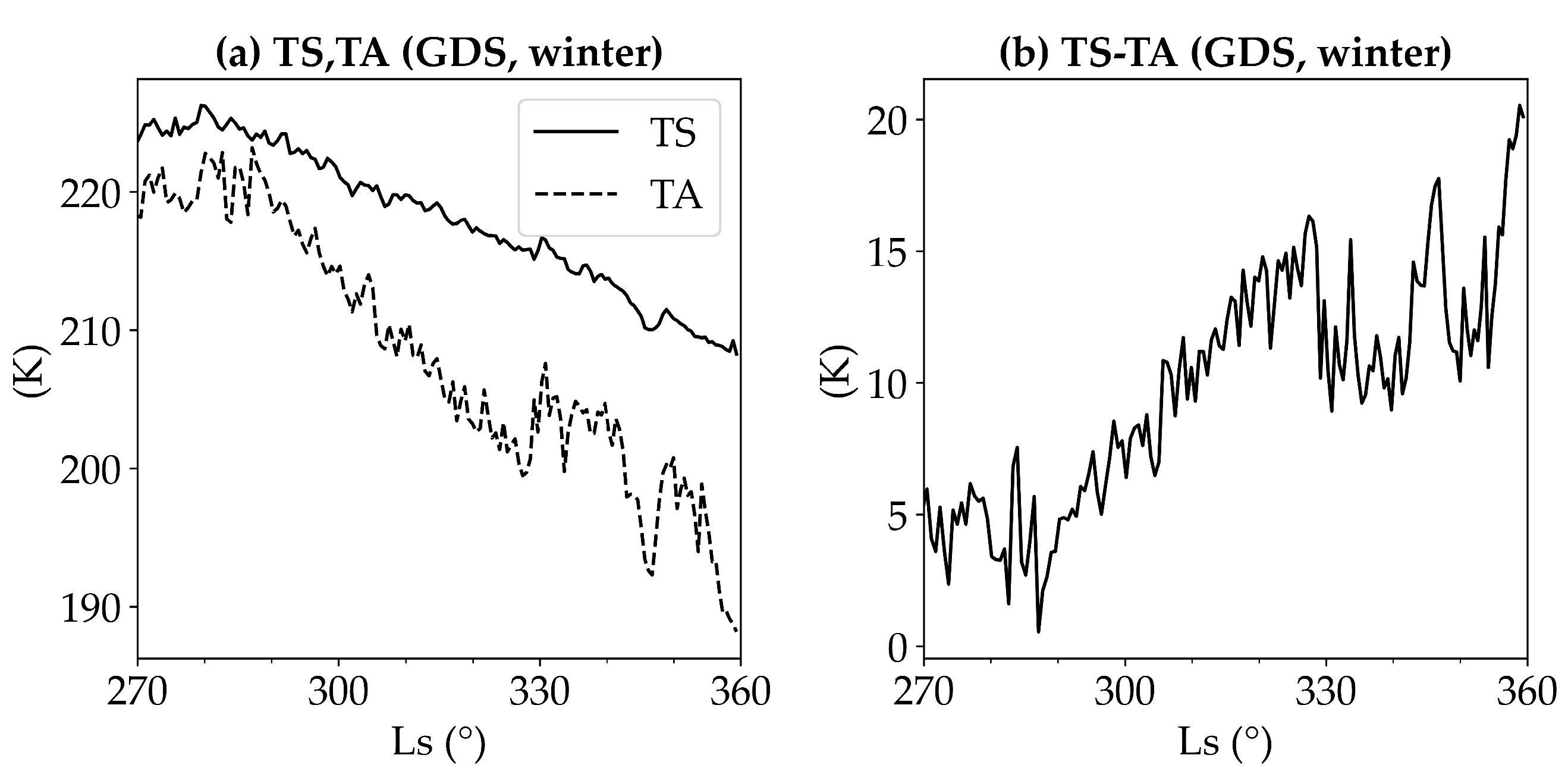
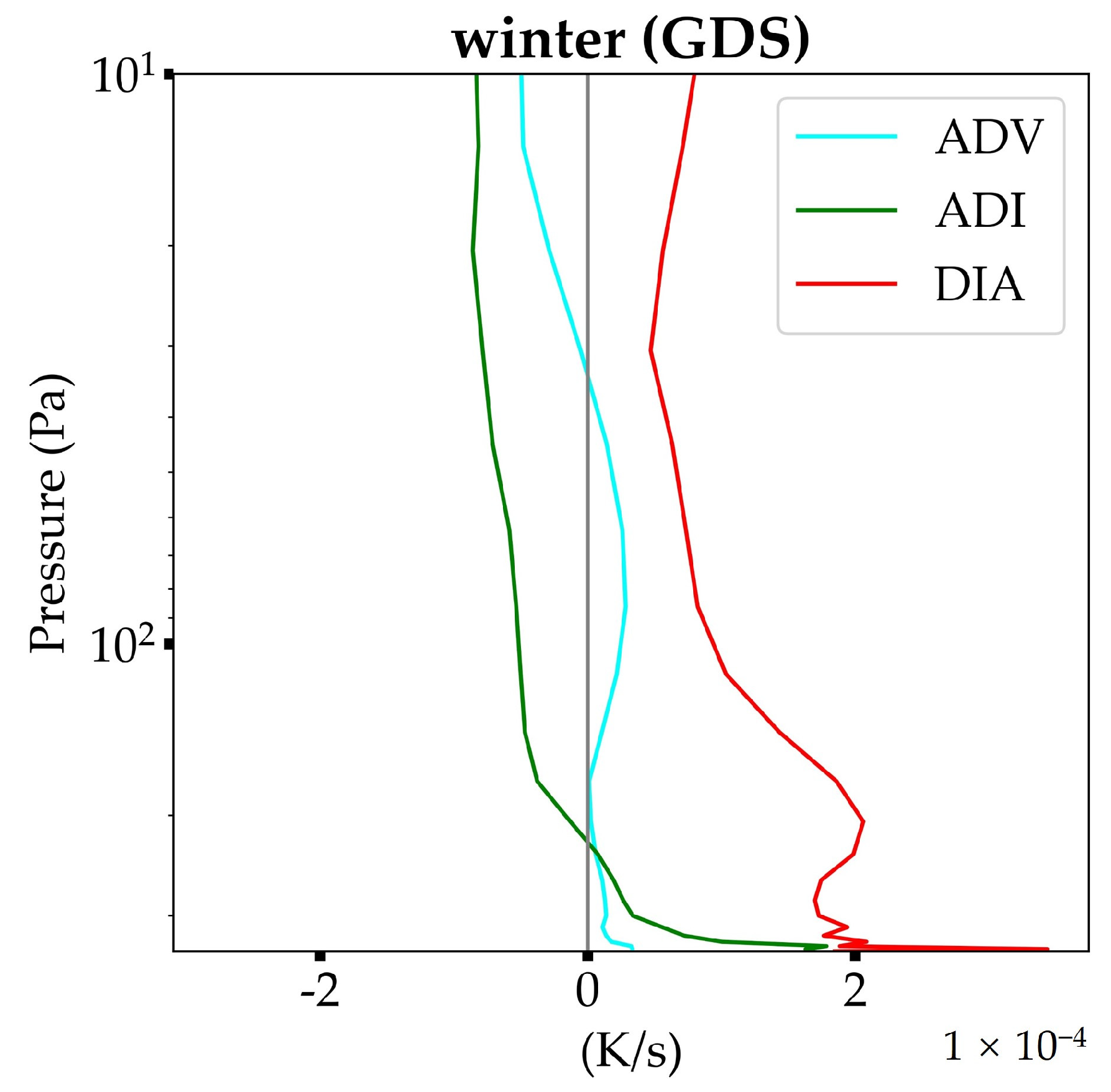
- Impact of Global Dust Storms (GDS): The influence of GDSs significantly alters the local atmospheric dynamics, reducing the temperature differential between the ground and air from an average of 17 K to about 5 K during storm events. The dust-driven radiative heating dominated, altering the typical heating pattern and revealing the critical role of dust in local processes. The overall diabatic heating below 10 Pa increased by and by at least above 200 Pa. This change in temperature disparity underscores the profound impact of dust aerosols on radiative heating processes.
- Implications for Martian General Circulation Mode Ls: These findings are pivotal for revising Martian climatological models to better incorporate localized topographical effects and extreme diurnal thermal variations. The evidence suggests that Martian atmospheric models, especially those used in simulations and planning for missions, must account for these localized and seasonal dynamics to enhance prediction accuracy and operational reliability during Martian explorations.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Huang, J.; Zhou, X.; Wu, G.; Xu, X.; Zhao, Q.; Liu, Y.; Duan, A.; Xie, Y.; Ma, Y.; Zhao, P.; et al. Global Climate Impacts of Land-Surface and Atmospheric Processes Over the Tibetan Plateau. Rev. Geophys. 2023, 61, e2022RG000771. [Google Scholar] [CrossRef]
- Wu, G.; Duan, A.; Liu, Y.; Mao, J.; Ren, R.; Bao, Q.; He, B.; Liu, B.; Hu, W. Tibetan Plateau climate dynamics: Recent research progress and outlook. Natl. Sci. Rev. 2015, 2, 100–116. [Google Scholar] [CrossRef]
- Wu, G.; Liu, Y. Impacts of the Tibetan Plateau on Asian Climate. Meteorol. Monogr. 2016, 56, 7.1–7.29. [Google Scholar] [CrossRef]
- Yang, H.; Shen, X.; Yao, J.; Wen, Q. Portraying the Impact of the Tibetan Plateau on Global Climate. J. Clim. 2020, 33, 3565–3583. [Google Scholar] [CrossRef]
- Yao, X.; Liu, Q.; Zhang, S.; Ma, J. Mechanism of Atmospheric Diabatic Heating Effect on the Intensity of Zonal Shear Line Over the Tibetan Plateau in Boreal Summer. J. Geophys. Res. Atmos. 2021, 126, e2021JD034840. [Google Scholar] [CrossRef]
- Zhang, C.; Duan, A.; Jia, X.; Wang, Z.; Pan, Z. A dynamic link between spring Arctic sea ice and the Tibetan Plateau snow increment indicator. npj Clim. Atmos. Sci. 2023, 6, 191. [Google Scholar] [CrossRef]
- Dong, Y.; Li, G.; Xie, X.; Yang, L.; Zhang, P.; Zeng, B. Mechanism of Diabatic Heating on Precipitation and the Track of a Tibetan Plateau Vortex over the Eastern Slope of the Tibetan Plateau. Adv. Atmos. Sci. 2023, 41, 155–172. [Google Scholar] [CrossRef]
- Duan, A.M.; Wu, G.X. Role of the Tibetan Plateau thermal forcing in the summer climate patterns over subtropical Asia. Clim. Dyn. 2005, 24, 793–807. [Google Scholar] [CrossRef]
- Wu, G.; Liu, Y.; Wang, T.; Wan, R.; Liu, X.; Li, W.; Wang, Z.; Zhang, Q.; Duan, A.; Liang, X. The Influence of Mechanical and Thermal Forcing by the Tibetan Plateau on Asian Climate. J. Hydrometeorol. 2007, 8, 770–789. [Google Scholar] [CrossRef]
- Yanai, M.; Li, C. Mechanism of Heating and the Boundary Layer over the Tibetan Plateau. Mon. Weather Rev. 1994, 122, 305–323. [Google Scholar] [CrossRef]
- Miyamoto, A.; Nakagawa, H.; Kuroda, T.; Takami, K.; Murata, I.; Medvedev, A.S.; Yoshida, N.; Aoki, S.; Sagawa, H.; Kasaba, Y.; et al. Intense Zonal Wind in the Martian Mesosphere During the 2018 Planet-Encircling Dust Event Observed by Ground-Based Infrared Heterodyne Spectroscopy. Geophys. Res. Lett. 2021, 48, e2021GL092413. [Google Scholar] [CrossRef]
- Shaposhnikov, D.S.; Medvedev, A.S.; Rodin, A.V.; Yiğit, E.; Hartogh, P. Martian Dust Storms and Gravity Waves: Disentangling Water Transport to the Upper Atmosphere. J. Geophys. Res. Planets 2022, 127, e2021JE007102. [Google Scholar] [CrossRef]
- Yiğit, E.; Medvedev, A.S.; Benna, M.; Jakosky, B.M. Dust Storm-Enhanced Gravity Wave Activity in the Martian Thermosphere Observed by MAVEN and Implication for Atmospheric Escape. Geophys. Res. Lett. 2021, 48, e2020GL092095. [Google Scholar] [CrossRef]
- Wu, Z.; Li, T.; Heavens, N.; Newman, C.; Richardson, M.; Yang, C.; Li, J.; Cui, J. Earth-like thermal and dynamical coupling processes in the Martian climate system. Earth-Sci. Rev. 2022, 229, 104023. [Google Scholar] [CrossRef]
- Kahn, R.; Gierasch, P. Long cloud observations on Mars and implications for boundary layer characteristics over slopes. J. Geophys. Res. 1982, 87, 867–880. [Google Scholar] [CrossRef]
- Hinson, D.; Wilson, R. Temperature inversions, thermal tides, and water ice clouds in the Martian tropics. J. Geophys. Res 2004, 109, E01002. [Google Scholar] [CrossRef]
- Wilson, R.J.; Neumann, G.A.; Smith, M.D. Diurnal variation and radiative influence of Martian water ice clouds. Geophys. Res. Lett. 2007, 34, L02710. [Google Scholar] [CrossRef]
- Benson, J.L.; Bonev, B.P.; James, P.B.; Shan, K.J.; Cantor, B.A.; Caplinger, M.A. The seasonal behavior of water ice clouds in the Tharsis and Valles Marineris regions of Mars: Mars Orbiter Camera Observations. Icarus 2003, 165, 34–52. [Google Scholar] [CrossRef]
- Montmessin, F.; Forget, F.; Rannou, P.; Cabane, M.; Haberle, R.M. Origin and role of water ice clouds in the Martian water cycle as inferred from a general circulation model. J. Geophys. Res. Planets 2004, 109, E10004. [Google Scholar] [CrossRef]
- Wordsworth, R.; Forget, F.; Millour, E.; Head, J.W.; Madeleine, J.B.; Charnay, B. Global modelling of the early martian climate under a denser CO2 atmosphere: Water cycle and ice evolution. Icarus 2013, 222, 1–19. [Google Scholar] [CrossRef]
- Streeter, P.; Lewis, S.; Patel, M.; Holmes, J.; Kass, D. Surface Warming During the 2018/Mars Year 34 Global Dust Storm. Geophys. Res. Lett. 2020, 47, e2019GL083936. [Google Scholar] [CrossRef]
- Savijärvi, H. Mechanisms of the diurnal cycle in the atmospheric boundary layer of Mars. Q. J. R. Meteorol. Soc. 2012, 138, 552–560. [Google Scholar] [CrossRef]
- Savijärvi, H. Radiative and turbulent heating rates in the clear-air boundary layer. Q. J. R. Meteorol. Soc. 2006, 132, 147–161. [Google Scholar] [CrossRef]
- Savijärvi, H.; Crisp, D.; Harri, A.-M. Effects of CO2 and dust on present-day solar radiation and climate on Mars. Q. J. R. Meteorol. Soc. 2005, 131, 2907–2922. [Google Scholar] [CrossRef]
- Medvedev, A.S.; Kuroda, T.; Hartogh, P. Influence of dust on the dynamics of the martian atmosphere above the first scale height. Aeolian Res. 2011, 3, 145–156. [Google Scholar] [CrossRef]
- Toon, O.B.; Pollack, J.B.; Sagan, C. Physical properties of the particles composing the Martian dust storm of 1971–1972. Icarus 1977, 30, 663–696. [Google Scholar] [CrossRef]
- Clancy, R.T.; Wolff, M.J.; Christensen, P.R. Mars aerosol studies with the MGS TES emission phase function observations: Optical depths, particle sizes, and ice cloud types versus latitude and solar longitude. J. Geophys. Res. Planets 2003, 108, E95098. [Google Scholar] [CrossRef]
- Clancy, R.T.; Wolff, M.J.; Whitney, B.A.; Cantor, B.A.; Smith, M.D.; McConnochie, T.H. Extension of atmospheric dust loading to high altitudes during the 2001 Mars dust storm: MGS TES limb observations. Icarus 2010, 207, 98–109. [Google Scholar] [CrossRef]
- Vasilyev, A.V.; Mayorov, B.S.; Bibring, J.P. The retrieval of altitude profiles of the Martian aerosol microphysical characteristics from the limb measurements of the Mars Express OMEGA spectrometer. Sol. Syst. Res. 2009, 43, 392–404. [Google Scholar] [CrossRef]
- Määttänen, A.; Listowski, C.; Montmessin, F.; Maltagliati, L.; Reberac, A.; Joly, L.; Bertaux, J.-L. A complete climatology of the aerosol vertical distribution on Mars from MEx/SPICAM UV solar occultations. Icarus 2013, 223, 892–941. [Google Scholar] [CrossRef]
- Guzewich, S.D.; Smith, M.D.; Wolff, M.J. The vertical distribution of Martian aerosol particle size. J. Geophys. Res. Planets 2014, 119, 2694–2708. [Google Scholar] [CrossRef]
- Smith, M.D.; Martínez, G.M.; Sebastián, E.; Lemmon, M.T.; Wolff, M.J.; Apéstigue, V.; Arruego, I.; Toledo, D.; Viúdez-Moreiras, D.; Rodriguez-Manfredi, J.A.; et al. Diurnal and Seasonal Variations of Aerosol Optical Depth Observed by MEDA/TIRS at Jezero Crater, Mars. J. Geophys. Res. Planets 2023, 128, e2022JE007560. [Google Scholar] [CrossRef]
- Cozzolino, F.; Franzese, G.; Cortecchia, F.; Molfese, C.; Esposito, F.; Mongelluzzo, G.; Ruggeri, A.C.; Porto, C.; Silvestro, S.; Popa, C.I.; et al. Development and testing of the MicroMED sensor: From BreadBoard model to flight model. Adv. Space Res. 2024, 73, 5335–5348. [Google Scholar] [CrossRef]
- Kuroda, T.; Medvedev, A.S.; Yiğit, E. Gravity Wave Activity in the Atmosphere of Mars During the 2018 Global Dust Storm: Simulations with a High-Resolution Model. J. Geophys. Res. Planets 2020, 125, e2020JE006556. [Google Scholar] [CrossRef]
- Medvedev, A.S.; Yiğit, E.; Kuroda, T.; Hartogh, P. General circulation modeling of the Martian upper atmosphere during global dust storms. J. Geophys. Res. Planets 2013, 118, 2234–2246. [Google Scholar] [CrossRef]
- Sánchez-Lavega, A.; del Río-Gaztelurrutia, T.; Hernández-Bernal, J.; Delcroix, M. The Onset and Growth of the 2018 Martian Global Dust Storm. Geophys. Res. Lett. 2019, 46, 6101–6108. [Google Scholar] [CrossRef]
- Streeter, P.M.; Lewis, S.R.; Patel, M.R.; Holmes, J.A.; Fedorova, A.A.; Kass, D.M.; Kleinböhl, A. Asymmetric Impacts on Mars’ Polar Vortices from an Equinoctial Global Dust Storm. J. Geophys. Res. Planets 2021, 126, e2020JE006774. [Google Scholar] [CrossRef]
- Sun, M.; Hao, G.; Cui, J.; Wu, X.; Huang, X.; Ni, Y.; Wu, Z.; Li, L. Enhanced Hydrogen Escape on Mars during the 2018 Global Dust Storm: Impact of Horizontal Wind Field. Astrophys. J. 2023, 953, 71. [Google Scholar] [CrossRef]
- Boutle, I.A.; Joshi, M.; Lambert, F.H.; Mayne, N.J.; Lyster, D.; Manners, J.; Ridgway, R.; Kohary, K. Mineral dust increases the habitability of terrestrial planets but confounds biomarker detection. Nat. Commun. 2020, 11, 2731. [Google Scholar] [CrossRef]
- Bonev, B.P.; Hansen, G.B.; Glenar, D.A.; James, P.B.; Bjorkman, J.E.J.P.; Science, S. Albedo models for the residual south polar cap on Mars: Implications for the stability of the cap under near-perihelion global dust storm conditions. Planet. Space Sci. 2008, 56, 181–193. [Google Scholar] [CrossRef]
- Chen, F.; Man, W.; Wang, S.; Esper, J.; Meko, D.; Büntgen, U.; Yuan, Y.; Hadad, M.; Hu, M.; Zhao, X.; et al. Southeast Asian ecological dependency on Tibetan Plateau streamflow over the last millennium. Nat. Geosci. 2023, 16, 1151–1158. [Google Scholar] [CrossRef]
- Xie, Y.; Huang, J.; Wu, G.; Liu, Y.; Dong, W.; Lu, M.; He, B.; Su, Z.; Bao, Q.; Zhao, Q.; et al. Oceanic repeaters boost the global climatic impact of the Tibetan Plateau. Sci. Bull. 2023, 68, 2225–2235. [Google Scholar] [CrossRef]
- Zhu, Z.; Wang, M.; Wang, J.; Ma, X.; Luo, J.; Yao, X. Diurnal Variation Characteristics of the Surface Sensible Heat Flux over the Tibetan Plateau. Atmosphere 2023, 14, 128. [Google Scholar] [CrossRef]
- Greybush, S.J.; Kalnay, E.; Wilson, R.J.; Hoffman, R.N.; Nehrkorn, T.; Leidner, M.; Eluszkiewicz, J.; Gillespie, H.E.; Wespetal, M.; Zhao, Y.; et al. The Ensemble Mars Atmosphere Reanalysis System (EMARS) Version 1.0. Geosci. Data J. 2019, 6, 137–150. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Ji, Q.; Sheng, Z.; He, M.; He, Y.; Zuo, X.; He, Z.; Qin, Z.; Wu, G. Observation based climatology Martian atmospheric waves perturbation Datasets. Sci. Data 2023, 10, 4. [Google Scholar] [CrossRef] [PubMed]
- Montabone, L.; Marsh, K.; Lewis, S.R.; Read, P.L.; Smith, M.D.; Holmes, J.; Spiga, A.; Lowe, D.; Pamment, A. The Mars Analysis Correction Data Assimilation (MACDA) Dataset V1.0. Geosci. Data J. 2014, 1, 129–139. [Google Scholar] [CrossRef]
- Holmes, J.; Lewis, S.; Patel, M. OpenMARS: A global record of martian weather from 1999–2015. Planet. Space Sci. 2020, 188, 104962. [Google Scholar] [CrossRef]
- Battalio, J.M.; Lora, J.M. Annular modes of variability in the atmospheres of Mars and Titan. Nat. Astron. 2021, 5, 1139–1147. [Google Scholar] [CrossRef]
- Bouche, J.; Coheur, P.-F.; Giuranna, M.; Wolkenberg, P.; Nardi, L.; Amoroso, M.; Vandaele, A.C.; Daerden, F.; Neary, L.; Bauduin, S. Seasonal and Spatial Variability of Carbon Monoxide (CO) in the Martian Atmosphere from PFS/MEX Observations. J. Geophys. Res. Planets 2021, 126, e2020JE006480. [Google Scholar] [CrossRef]
- Shaheen, F.; Nee Lala, M.G.; Krishna, A.P.; Payra, S. Seasonal variation in atmospheric optical depth (AOD) and thermal inertia (TI) inter-relationship over Martian Gale crater. Planet. Space Sci. 2024, 242, 105865. [Google Scholar] [CrossRef]
- Forbes, J.M.; Zhang, X.; Forget, F.; Millour, E.; Kleinböhl, A. Solar tides in the middle and upper atmosphere of Mars. J. Geophys. Res. Space Phys. 2020, 125, 8140–8145. [Google Scholar] [CrossRef]
- Lee, C.; Lawson, W.G.; Richardson, M.I.; Heavens, N.G.; Kleinbohl, A.; Banfield, D.; McCleese, D.J.; Zurek, R.; Kass, D.; Schofield, J.T.; et al. Thermal tides in the Martian middle atmosphere as seen by the Mars Climate Sounder. J. Geophys. Res. 2009, 114, 1–6. [Google Scholar] [CrossRef]
- Wu, Z.; Li, T.; Dou, X. Seasonal variation of Martian middle atmosphere tides observed by the Mars Climate Sounder. J. Geophys. Res. Planets 2015, 120, 2206–2223. [Google Scholar] [CrossRef]
- Wu, Z.; Li, T.; Zhang, X.; Li, J.; Cui, J. Dust tides and rapid meridional motions in the Martian atmosphere during major dust storms. Nat. Commun. 2020, 11, 614. [Google Scholar] [CrossRef]
- Williams, D.R. Mars Fact Sheet. Available online: https://nssdc.gsfc.nasa.gov/planetary/factsheet/marsfact.html (accessed on 10 January 2024).
- Chang, S.; Li, Y.; Shi, C.; Guo, D. Combined Effects of the ENSO and the QBO on the Ozone Valley over the Tibetan Plateau. Remote Sens. 2022, 14, 4935. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Sheng, Z.; He, M. The Atmospheric Heating Mechanism over the Tharsis Bulge of Mars and the Impact of Global Dust Storms. Remote Sens. 2024, 16, 1950. https://doi.org/10.3390/rs16111950
Zhang J, Sheng Z, He M. The Atmospheric Heating Mechanism over the Tharsis Bulge of Mars and the Impact of Global Dust Storms. Remote Sensing. 2024; 16(11):1950. https://doi.org/10.3390/rs16111950
Chicago/Turabian StyleZhang, Jie, Zheng Sheng, and Mingyuan He. 2024. "The Atmospheric Heating Mechanism over the Tharsis Bulge of Mars and the Impact of Global Dust Storms" Remote Sensing 16, no. 11: 1950. https://doi.org/10.3390/rs16111950
APA StyleZhang, J., Sheng, Z., & He, M. (2024). The Atmospheric Heating Mechanism over the Tharsis Bulge of Mars and the Impact of Global Dust Storms. Remote Sensing, 16(11), 1950. https://doi.org/10.3390/rs16111950








