Estimation of Antarctic Ice Sheet Thickness Based on 3D Density Interface Inversion Considering Terrain and Undulating Observation Surface Simultaneously
Abstract
1. Introduction
2. Methodology
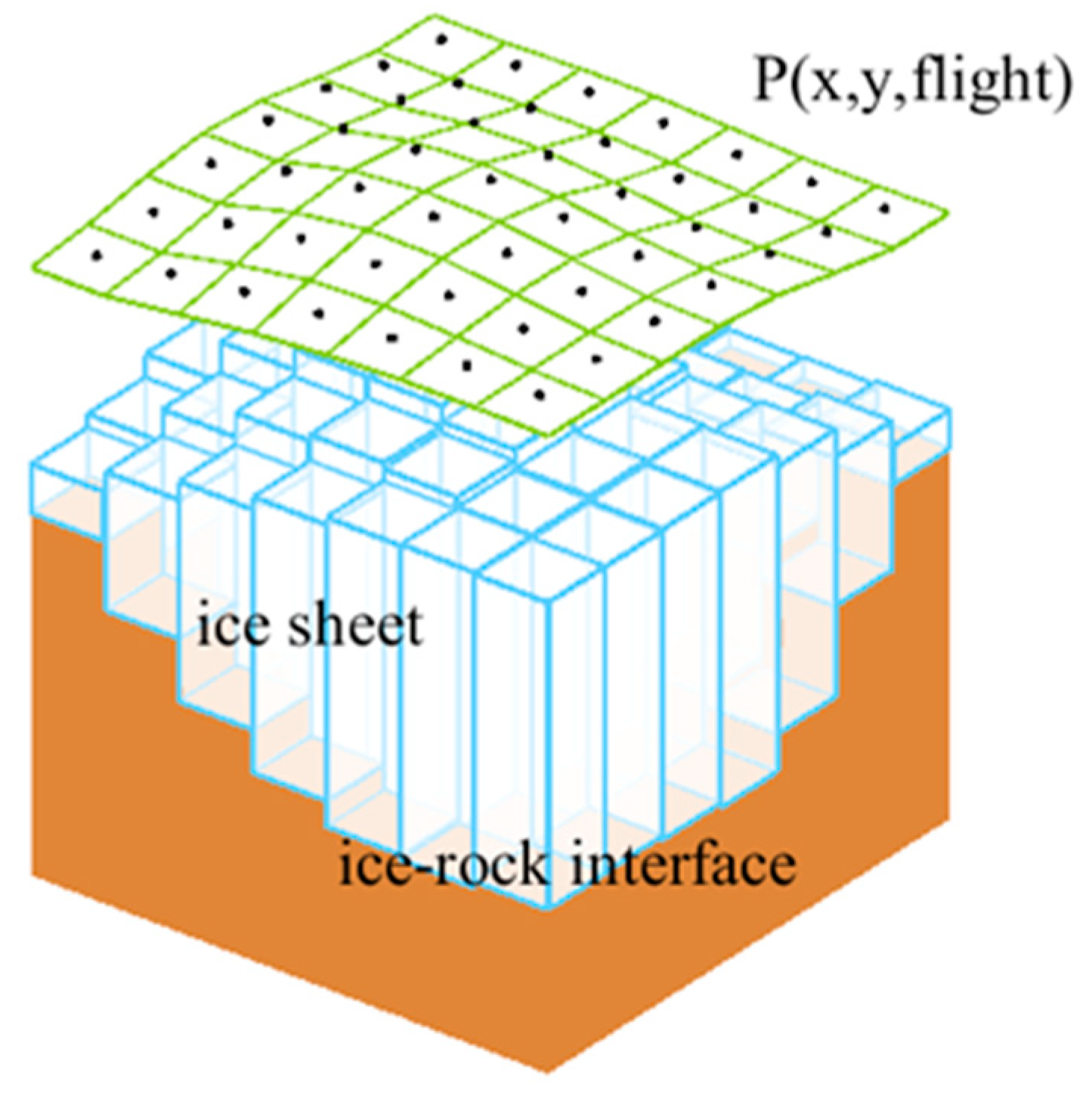
2.1. Forward Modeling Considering Terrain and UOS
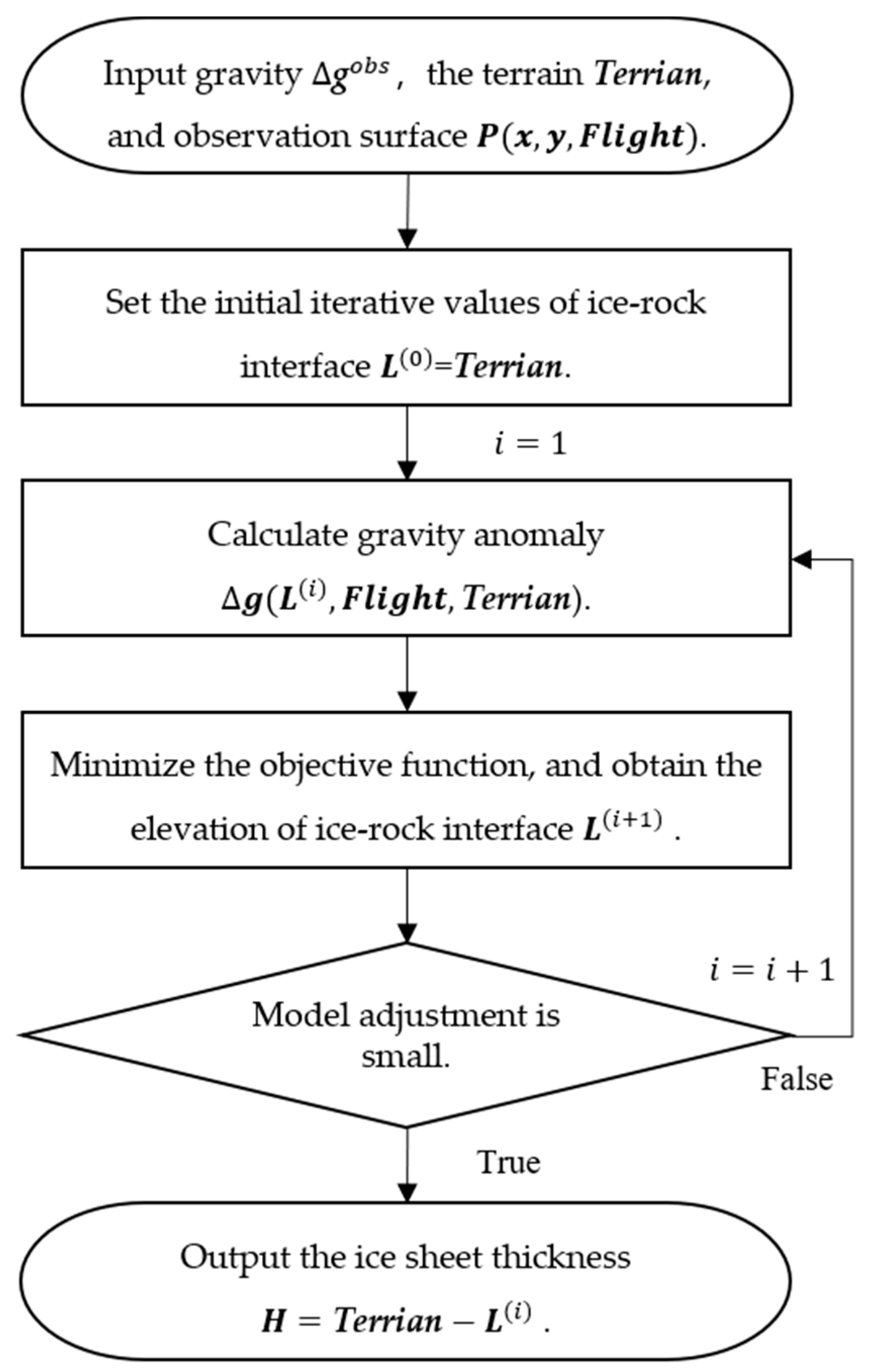
2.2. Inverse Ice–Rock Interface under Terrain and UOS
3. Synthetic Examples
3.1. Simple Synthetic Example
3.2. Complex Synthetic Example
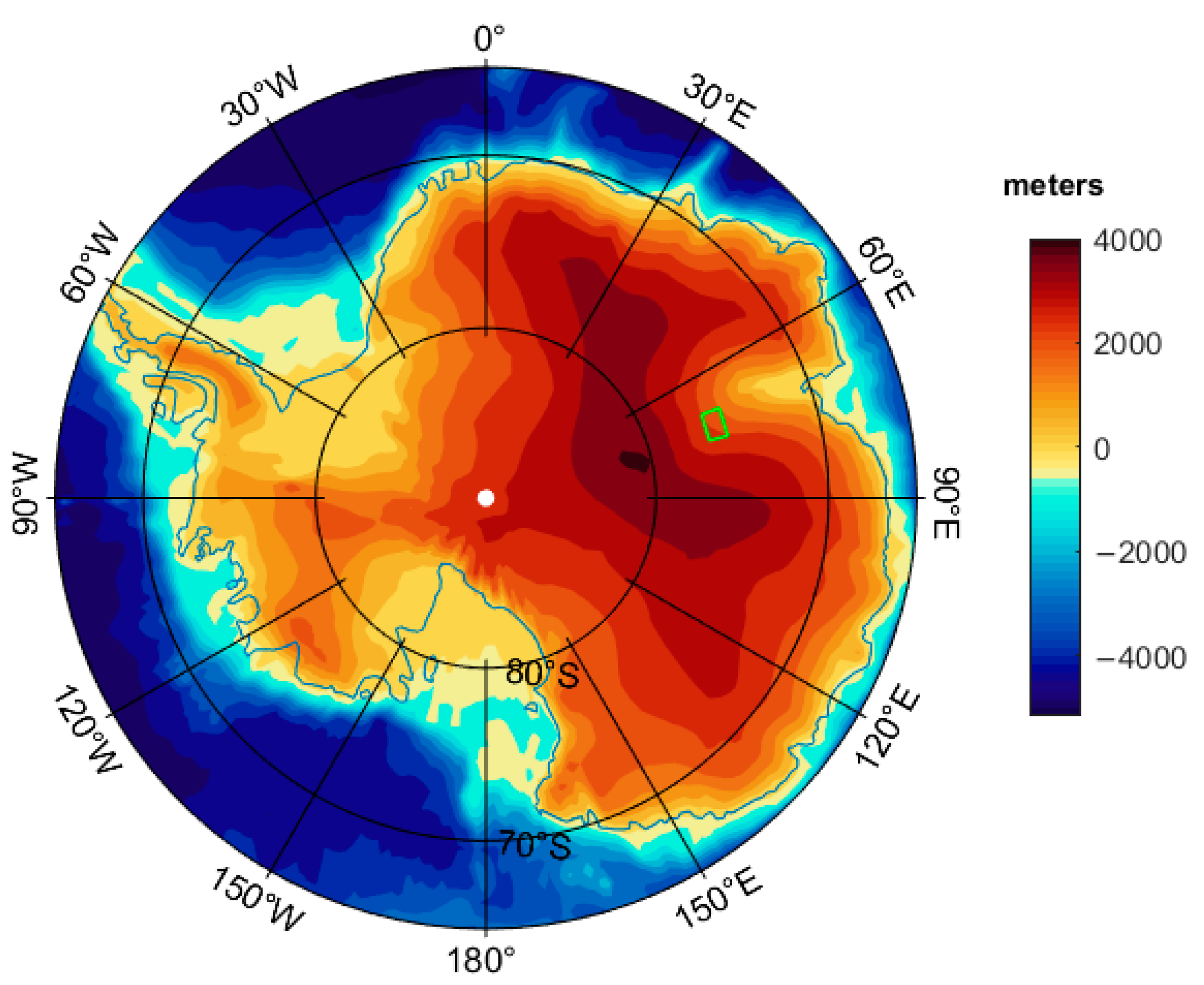
4. Real Data Application
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bamber, J.L.; Layberry, R.L.; Gogineni, S.P. A new ice thickness and bed data set for the Greenland ice sheet: 1. Measurement, data reduction, and errors. J. Geophys. Res. Atmos. 2001, 106, 33773–33780. [Google Scholar] [CrossRef]
- Yang, T.; Liang, Q.; Zheng, L.; Li, T.; Chen, Z.; Hui, E.; Cheng, X. Mass Balance of the Antarctic Ice Sheet in the Early 2lst Century. Remote Sens 2023, 15, 1677. [Google Scholar] [CrossRef]
- Corbató, C.E. Thickness and Basal Configuration of Lower Blue Glacier, Washington, Determined by Gravimetry. J. Glaciol. 1965, 5, 637–650. [Google Scholar] [CrossRef]
- Millan, R.; Rignot, E.; Rivera, A.; Martineau, V.; Mouginot, J.; Zamora, R.; Uribe, J.; Lenzano, G.; De Fleurian, B.; Li, X.; et al. Ice thickness and bed elevation of the Northern and Southern Patagonian Icefields. Geophys. Res. Lett. 2019, 46, 6626–6635. [Google Scholar] [CrossRef]
- MacGregor, J.A.; Colgan, W.T.; Paxman, G.J.G.; Tinto, K.J.; Csathó, B.; Darbyshire, F.A.; Fahnestock, M.A.; Kokfelt, T.F.; MacKie, E.J.; Morlighem, M.; et al. Geologic provinces beneath the Greenland Ice Sheet constrained by geophysical data synthesis. Geophys. Res. Lett. 2024, 51, e2023GL107357. [Google Scholar] [CrossRef]
- Stevens, C.W.; Moorman, B.J.; Solomon, S.M.; Hugenholtz, C.H. Mapping subsurface conditions within the near-shore zone of an Arctic delta using ground penetrating radar. Cold Reg. Sci. Technol. 2009, 56, 30–38. [Google Scholar]
- Fretwell, P.; Pritchard, H.D.; Vaughan, D.G.; Bamber, J.L.; Barrand, N.E.; Bell, R.; Bianchi, C.; Bingham, R.G.; Blankenship, D.D.; Casassa, G.; et al. Bedmap2: Improved ice bed, surface and thickness datasets for Antarctica. Cryosphere 2013, 7, 375–393. [Google Scholar] [CrossRef]
- Liu, J.; Wang, S.; He, Y.; Li, Y.; Wang, Y.; Wei, Y.; Che, Y. Estimation of ice thickness and the features of subglacial media detected by ground penetrating radar at the Baishui River Glacier No. 1 in Mt, Yulong, China. Remote Sens 2020, 12, 4105. [Google Scholar] [CrossRef]
- Jin, H.; Yao, X.; Wei, Q.; Zhou, S.; Zhang, Y.; Chen, J.; Yu, Z. Ice Thickness Assessment of Non-Freshwater Lakes in the Qinghai-Tibetan Plateau Based on Unmanned Aerial Vehicle-Bornelce-Penetrating Radar: A Case Study of Qinghai Lake and Gahai Lake. Remote Sens 2024, 16, 959. [Google Scholar] [CrossRef]
- Yan, J.; Lü, Q.; Luo, F.; Cheng, S.; Zhang, K.; Zhang, Y.; Xu, Y.; Zhang, C.; Liu, Z.; Ruan, S.; et al. A gravity and magnetic study of lithospheric architecture and structures of South China with implications for the distribution of plutons and mineral systems of the main metallogenic belts. J. Asian Earth Sci. 2021, 221, 104938. [Google Scholar] [CrossRef]
- Liu, Y.; Huang, Y.; Lü, Q.; Liu, S. Adaptive mesh-free approach for gravity inversion using modified radial basis function. IEEE Trans. Geosci. Remote Sens. 2023, 61, 5907612. [Google Scholar] [CrossRef]
- Bull, C.; Hardy, J.R. The Determination of the Thickness of a Glacier from Measurements of the Value of Gravity. J. Glaciol. 1956, 2, 755–763. [Google Scholar] [CrossRef]
- Thiel, E.; Lachapelle, E.; Behrendt, J. The Thickness of Lemon Creek Glacier, Alaska, As Determined by Gravity Measurements. Eos Trans. Am. Geophys. Union 1957, 38, 745. [Google Scholar]
- Parker, R.L. The rapid calculation of potential anomalies. Geophys. J. Int. 1973, 31, 447–455. [Google Scholar] [CrossRef]
- Gourlet, P.; Rignot, E.; Rivera, A.; Casassa, G. Ice thickness of the northern half of the Patagonia Icefields of South America from high-resolution airborne gravity surveys. Geophys. Res. Lett. 2016, 43, 241–249. [Google Scholar] [CrossRef]
- An, L.; Rignot, E.; Milan, R.; Tinto, K.; Willis, J. Bathymetry of northwest Greenland using ocean melting Greenland (OMG) high-resolution airborne gravity and other data. Remote Sens. 2019, 11, 131. [Google Scholar] [CrossRef]
- Eisermann, H.; Eagles, G.; Jokat, W. Coastal bathymetry in central Dronning Maud Land controls ice shelf stability. Sci. Rep. 2024, 14, 1367. [Google Scholar] [CrossRef] [PubMed]
- Yao, C.; Huang, W.; Guan, Z. Fast splines conversion of curved-surface potential field and vertical gradient data into horizontal-plane data. Oil Geophys. Prospect. 1997, 32, 229–236. [Google Scholar]
- Pilkington, M.; Boulanger, O. Potential field continuation between arbitrary surfaces—Comparing methods. Geophysics 2017, 82, J9–J25. [Google Scholar] [CrossRef]
- Jekeli, C. The downward continuation of aerial gravimetric data without density hypothesis. Bull. Geod. 1987, 61, 319–329. [Google Scholar] [CrossRef]
- Yang, J.; Jekeli, C.; Liu, L. Seafloor topography estimation from gravity gradients using simulated annealing. J. Geophys. Res. Solid Earth 2018, 123, 6958–6975. [Google Scholar] [CrossRef]
- Tikhonov, A.N.; Arsenin, V.Y. Solutions of Ill-Posed Problems; V. H. Winston & Sons: Washington, DC, USA, 1977. [Google Scholar]
- Ferraccioli, F.; Finn, C.; Jordan, T.A.; Bell, R.E.; Anderson, L.M.; Damaske, D. East Antarctic rifting triggers uplift of the Gamburtsev Mountains. Nature 2011, 479, 388–392. [Google Scholar] [CrossRef] [PubMed]
- Bell, R.E.; Ferraccioli, F.; Creyts, T.T.; Braaten, D.; Corr, H.; Das, I.; Damaske, D.; Frearson, N.; Jordan, T.; Rose, K.; et al. Widespread Persistent Thickening of the East Antarctic Ice Sheet by Freezing from the Base. Science 2011, 331, 1592–1595. [Google Scholar] [CrossRef] [PubMed]
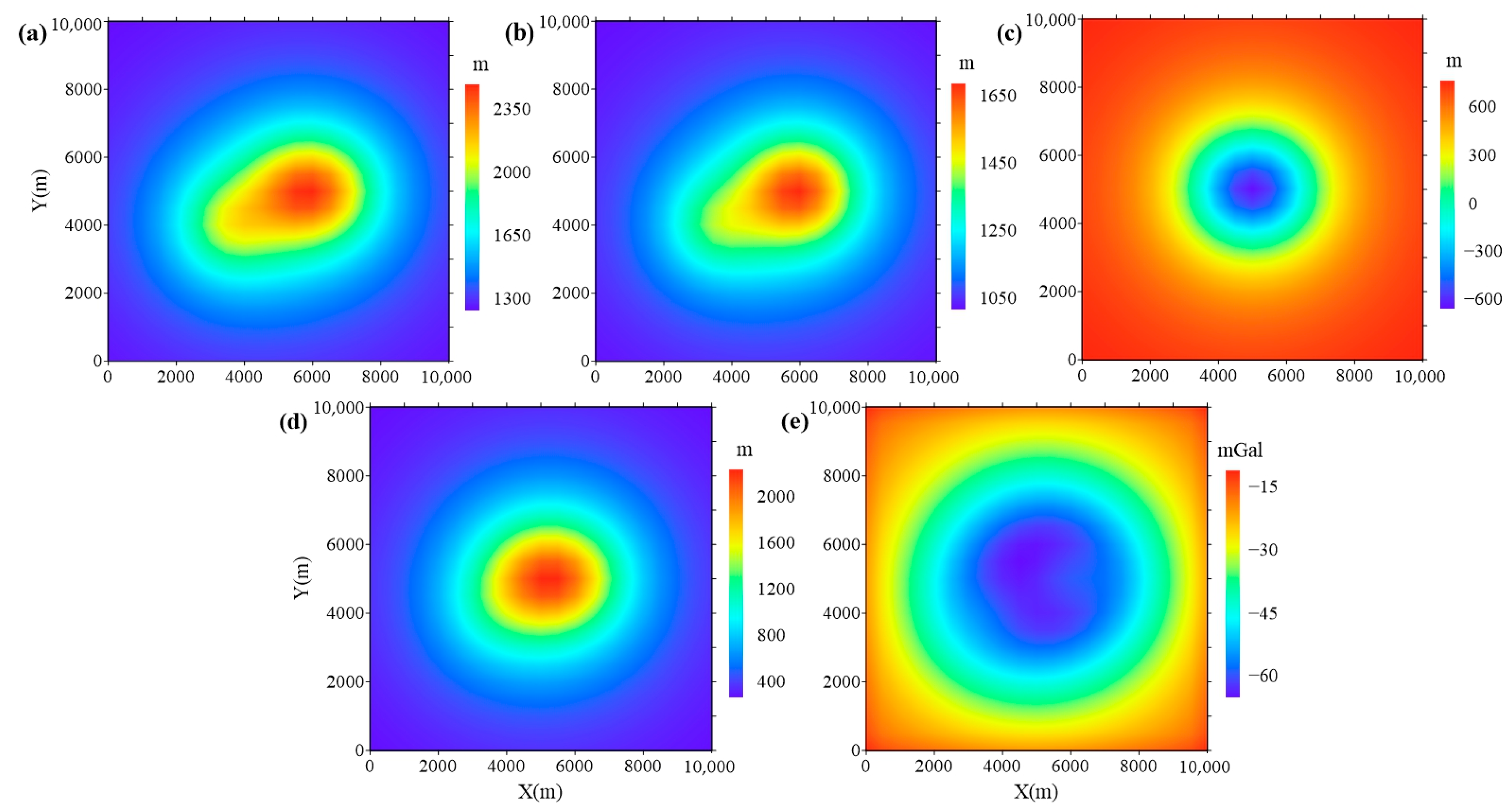

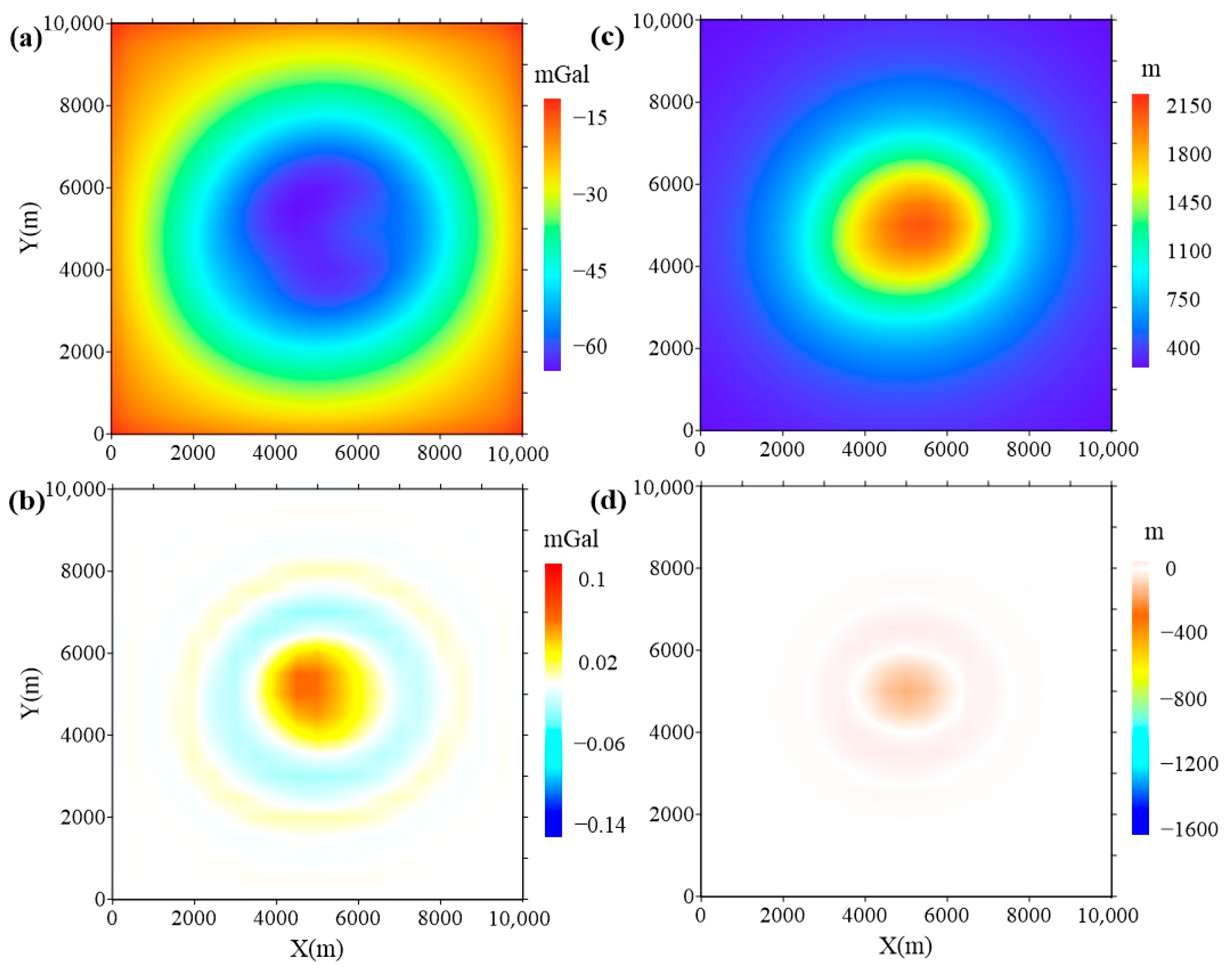
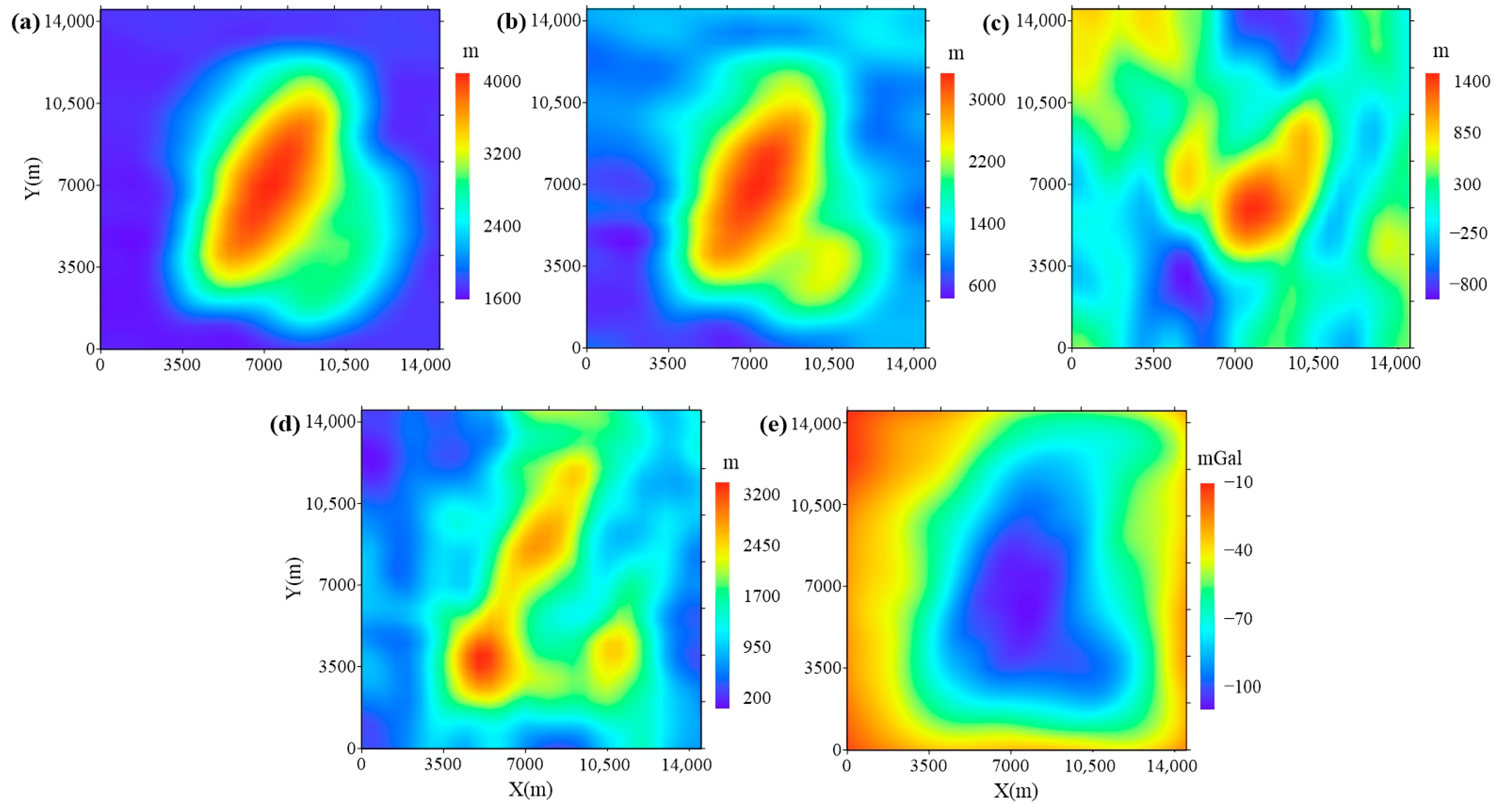
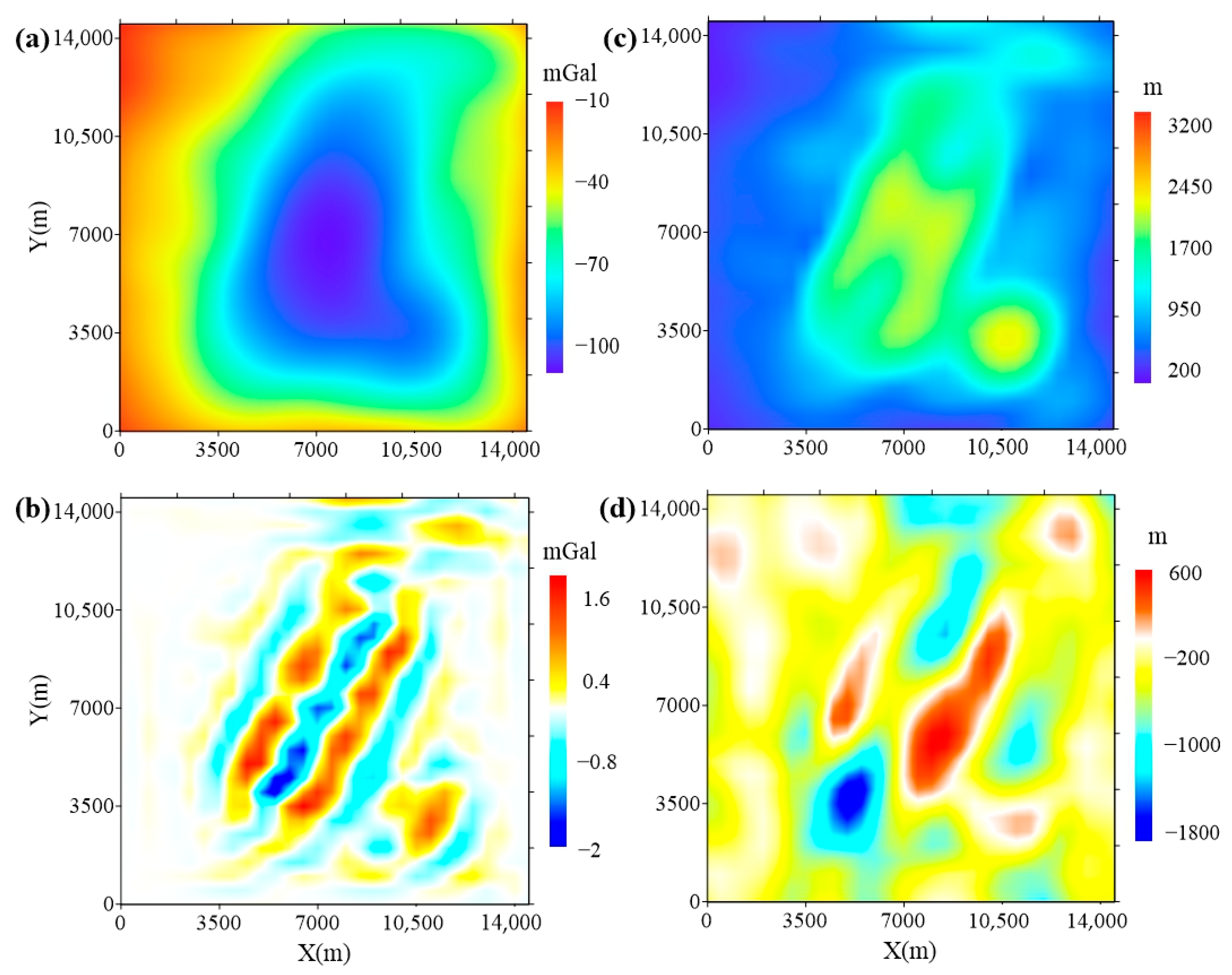
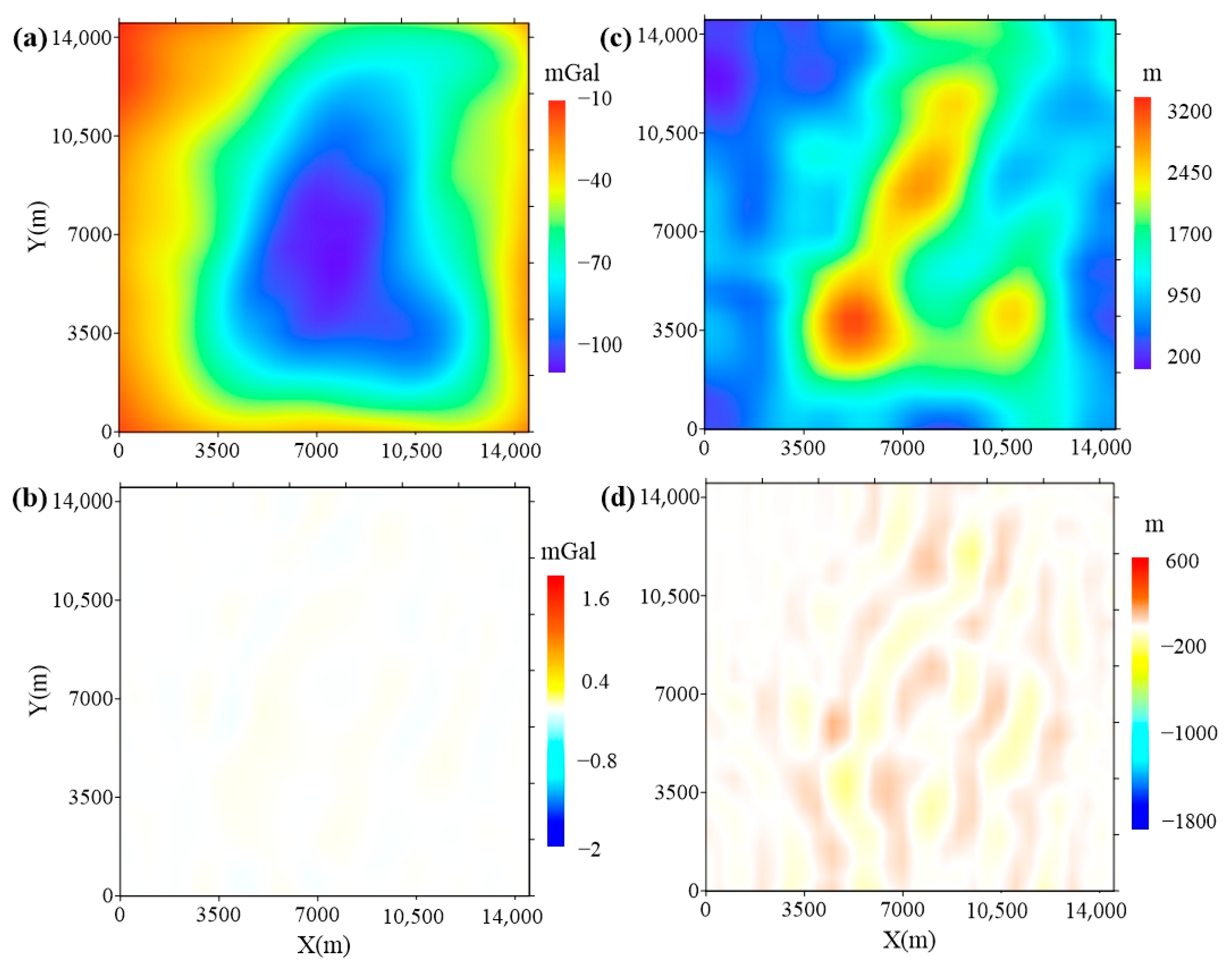
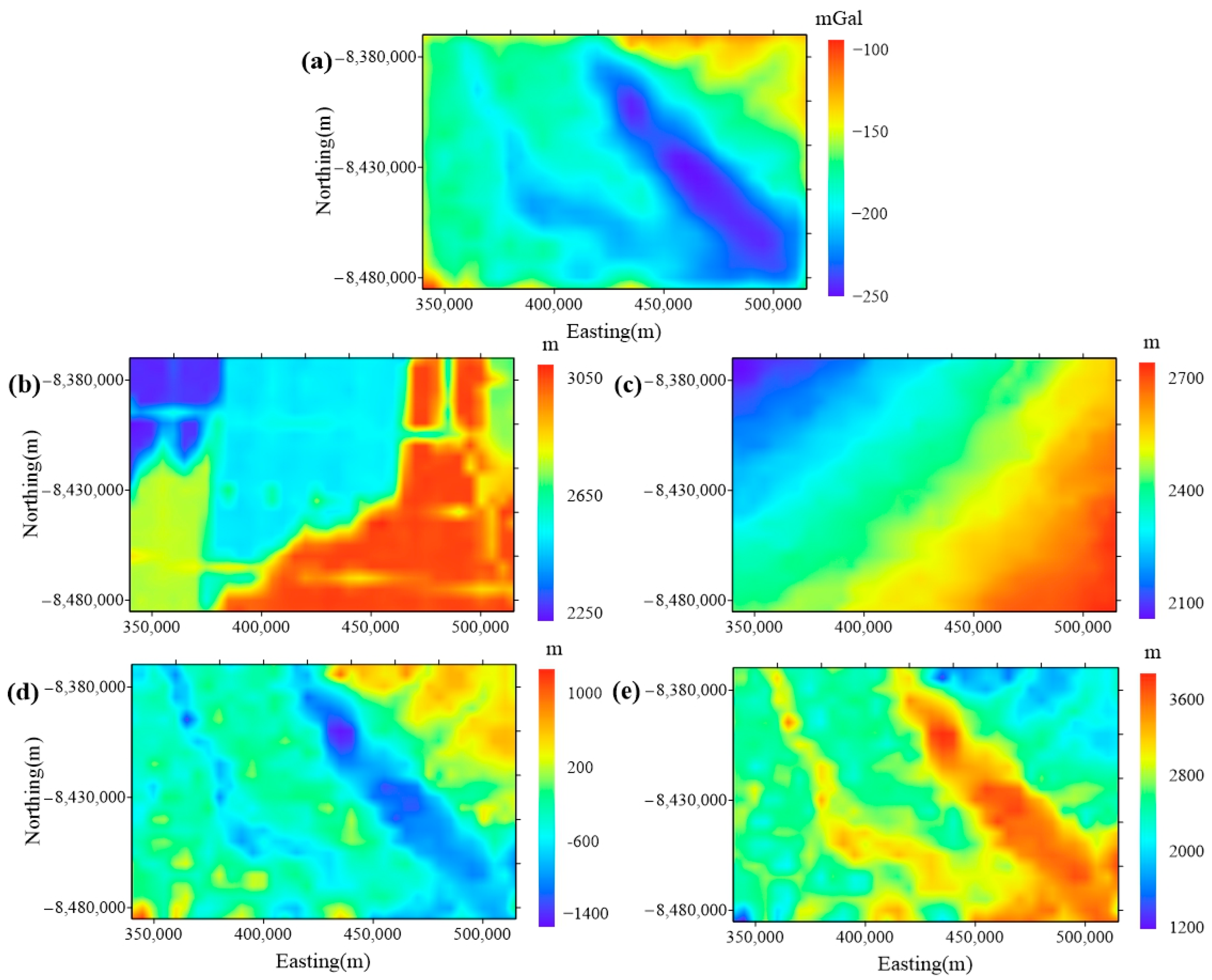
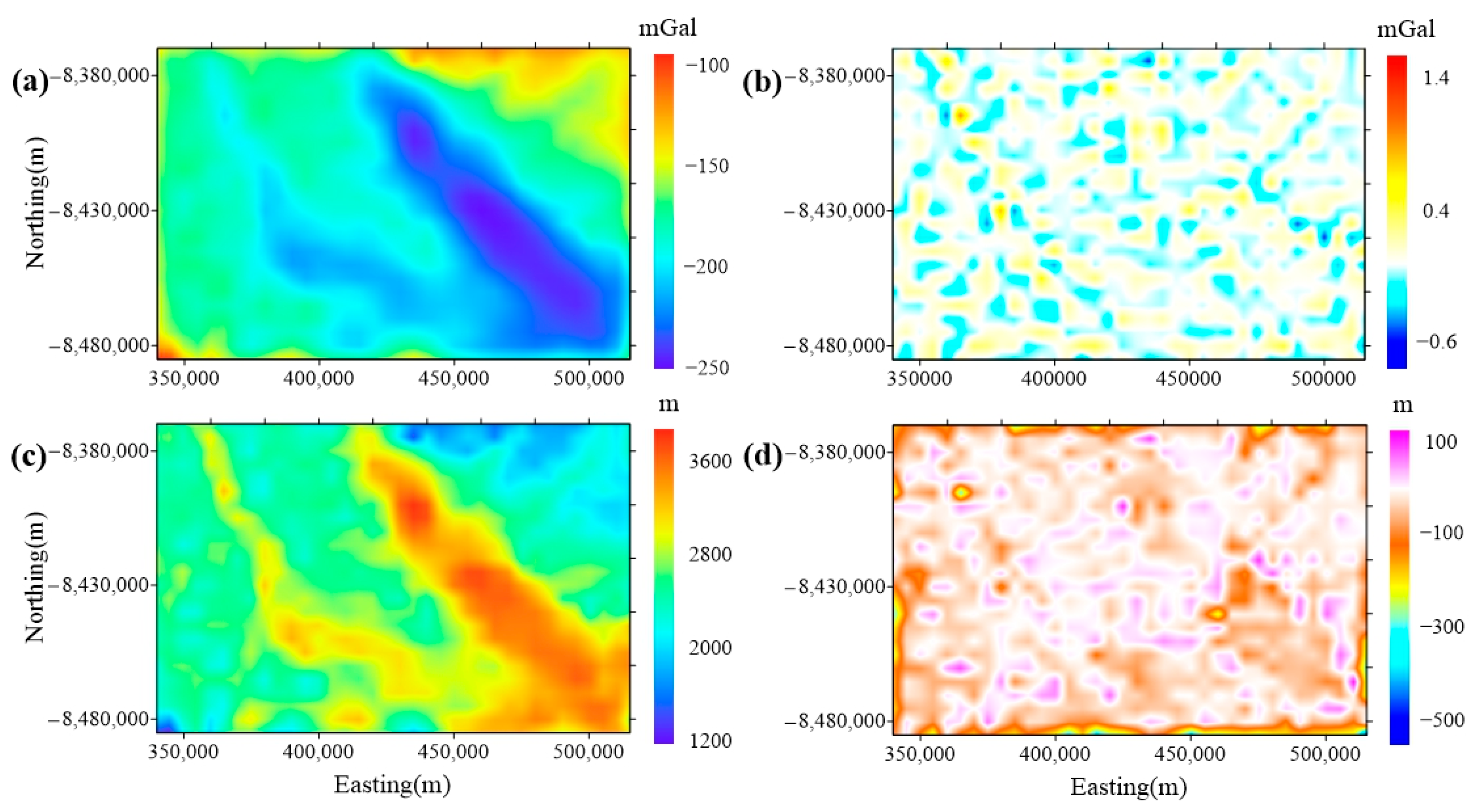

| Type | Minimum Value (m/mGal) | Maximum Value (m/mGal) | Mean Value (m/mGal) |
|---|---|---|---|
| observation surface | 1233.0 | 2487.6 | 1487.9 |
| terrain | 1016.1 | 1686.8 | 1142.9 |
| ice–rock interface | −646.0 | 758.6 | 526.1 |
| ice sheet thickness | 257.5 | 2230.5 | 616.8 |
| gravity anomaly | −64.65 | −10.80 | −34.37 |
| Result | RMSE (m/mGal) | Minimum Value (m/mGal) | Maximum Value (m/mGal) | Minimum Error (m/mGal) | Maximum Error (m/mGal) |
|---|---|---|---|---|---|
| Without: gravity anomaly | 0.03 | −64.55 | −10.80 | −0.15 | 0.12 |
| Without: ice thickness | 232.2 | 147.1 | 1357.5 | −1622.5 | 15.5 |
| With: gravity anomaly | 0.01 | −64.59 | −10.80 | −0.03 | 0.06 |
| With: ice thickness | 17.2 | 257.7 | 2109.0 | −151.3 | 44.60 |
| Type | Minimum Value (m/mGal) | Maximum Value (m/mGal) | Mean Value (m/mGal) |
|---|---|---|---|
| observation surface | 1586.0 | 4088.0 | 2245.7 |
| terrain | 428.0 | 3338.0 | 1426.7 |
| ice–rock interface | −929.7 | 1470.0 | 149.6 |
| ice sheet thickness | 46.2 | 3373.0 | 1277.1 |
| gravity anomaly | −108.73 | −9.87 | −60.30 |
| Result | RMSE (m/mGal) | Minimum Value (m/mGal) | Maximum Value (m/mGal) | Minimum Error (m/mGal) | Maximum Error (m/mGal) |
|---|---|---|---|---|---|
| Without: gravity anomaly | 0.44 | −108.56 | −9.88 | −2.01 | 1.93 |
| Without: ice thickness | 491.4 | 118.1 | 2299.8 | −1858.2 | 627.9 |
| With: gravity anomaly | 0.01 | −108.73 | −9.88 | −0.03 | 0.03 |
| With: ice thickness | 38.9 | 45.8 | 3221.8 | −171.4 | 138.7 |
| Type | Minimum Value (m/mGal) | Maximum Value (m/mGal) | Mean Value (m/mGal) |
|---|---|---|---|
| residual gravity anomaly | −250.11 | −93.60 | −187.87 |
| aircraft altitude | 2221.2 | 3095.0 | 2727.0 |
| ice surface elevation | 2056.5 | 2741.1 | 2433.9 |
| radar-derived ice bed elevation | −1484.7 | 1249.7 | −292.5 |
| radar-derived ice thickness | 1178.1 | 3853.7 | 2726.4 |
| Result | RMSE (m/mGal) | Minimum Value (m/mGal) | Maximum Value (m/mGal) | Minimum Error (m/mGal) | Maximum Error (m/mGal) |
|---|---|---|---|---|---|
| Without: gravity anomaly | 0.18 | −250.03 | −93.66 | −0.59 | 1.05 |
| Without: ice thickness | 79.2 | 1197.0 | 3832.6 | −548.7 | 123.5 |
| With: gravity anomaly | 0.21 | −250.23 | −93.65 | −0.79 | 1.57 |
| With: ice thickness | 19.8 | 1187.3 | 3876.1 | −172.6 | 86.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Wang, J.; Li, F.; Meng, X. Estimation of Antarctic Ice Sheet Thickness Based on 3D Density Interface Inversion Considering Terrain and Undulating Observation Surface Simultaneously. Remote Sens. 2024, 16, 1905. https://doi.org/10.3390/rs16111905
Liu Y, Wang J, Li F, Meng X. Estimation of Antarctic Ice Sheet Thickness Based on 3D Density Interface Inversion Considering Terrain and Undulating Observation Surface Simultaneously. Remote Sensing. 2024; 16(11):1905. https://doi.org/10.3390/rs16111905
Chicago/Turabian StyleLiu, Yandong, Jun Wang, Fang Li, and Xiaohong Meng. 2024. "Estimation of Antarctic Ice Sheet Thickness Based on 3D Density Interface Inversion Considering Terrain and Undulating Observation Surface Simultaneously" Remote Sensing 16, no. 11: 1905. https://doi.org/10.3390/rs16111905
APA StyleLiu, Y., Wang, J., Li, F., & Meng, X. (2024). Estimation of Antarctic Ice Sheet Thickness Based on 3D Density Interface Inversion Considering Terrain and Undulating Observation Surface Simultaneously. Remote Sensing, 16(11), 1905. https://doi.org/10.3390/rs16111905





