Influence of Inter-System Biases on Combined Single-Frequency BDS-2 and BDS-3 Pseudorange Positioning of Different Types of Receivers
Abstract
1. Introduction
2. Mathematical Models and Methods
2.1. Pseudorange SPP Model Considering Code ISBs
2.2. Pseudorange Differential Positioning Model Considering Code ISBs
2.3. The Principle of Carrier-Phase Smoothing
3. Experiment and Result Analysis
3.1. Experimental Data and Data Processing Strategies
3.1.1. Geodetic GNSS Receiver Data Preparation
3.1.2. Low-Cost Receiver u-Blox M8T Preparation
3.1.3. Pseudorange Positioning Experiment Processing Strategies
3.2. Pseudorange Positioning Analysis of Geodetic GNSS Receiver
3.2.1. Data Availability Analysis of Geodetic GNSS Receiver
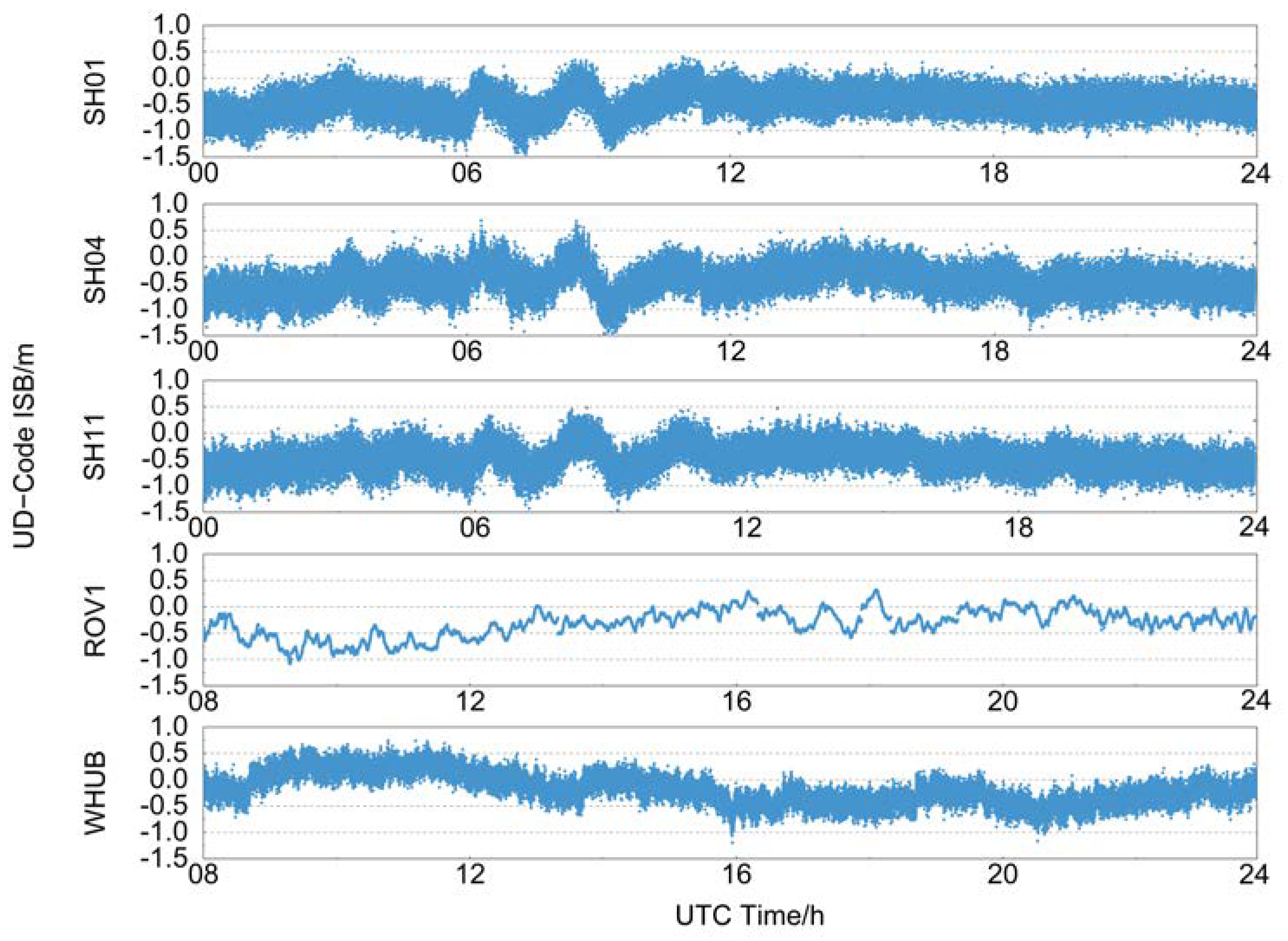
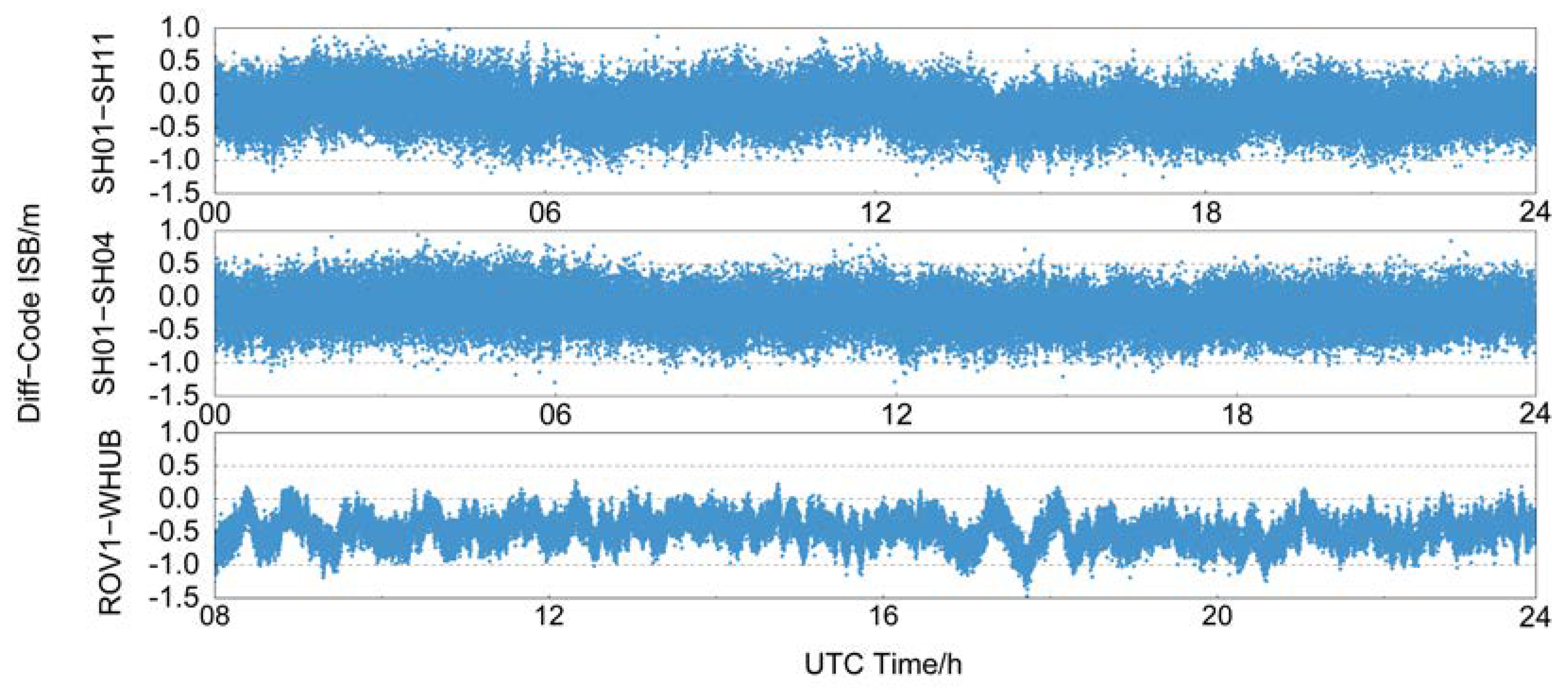
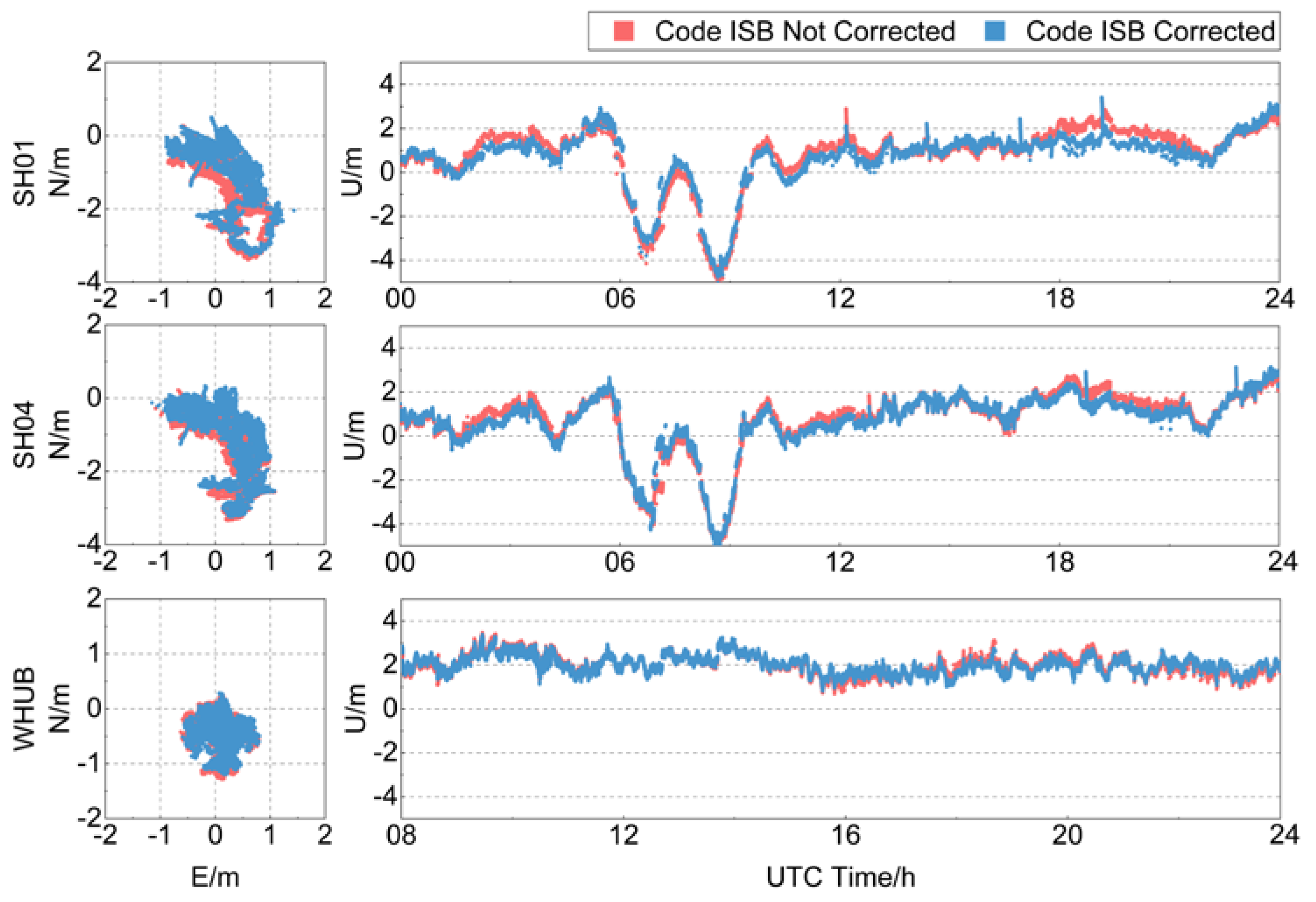
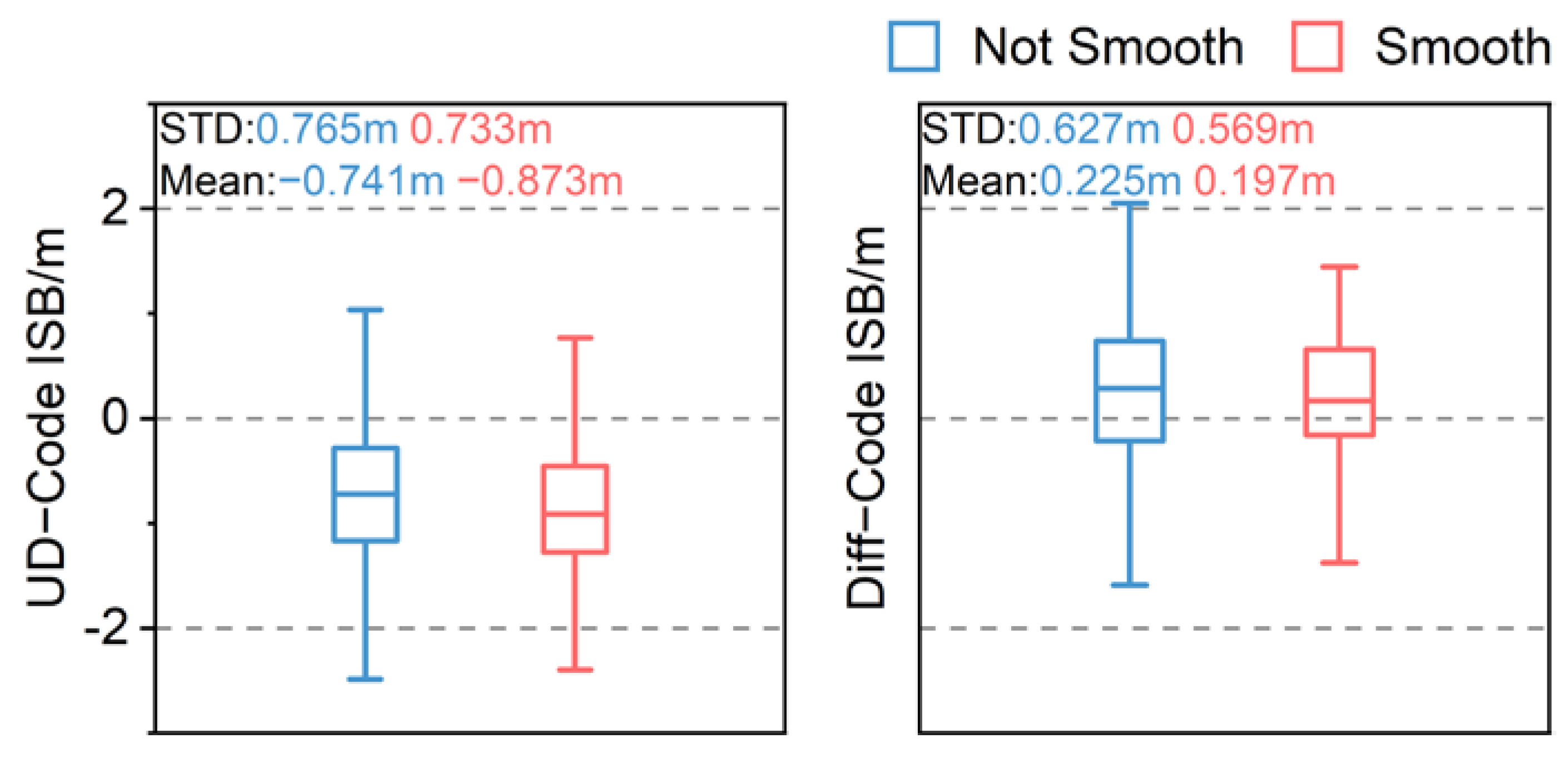
3.2.2. Analysis of Code ISB Characterization
- (1)
- Un-differential code ISBs
- (2)
- Differential code ISBs
- (3)
- Code ISBs before and after carrier-phase smoothing
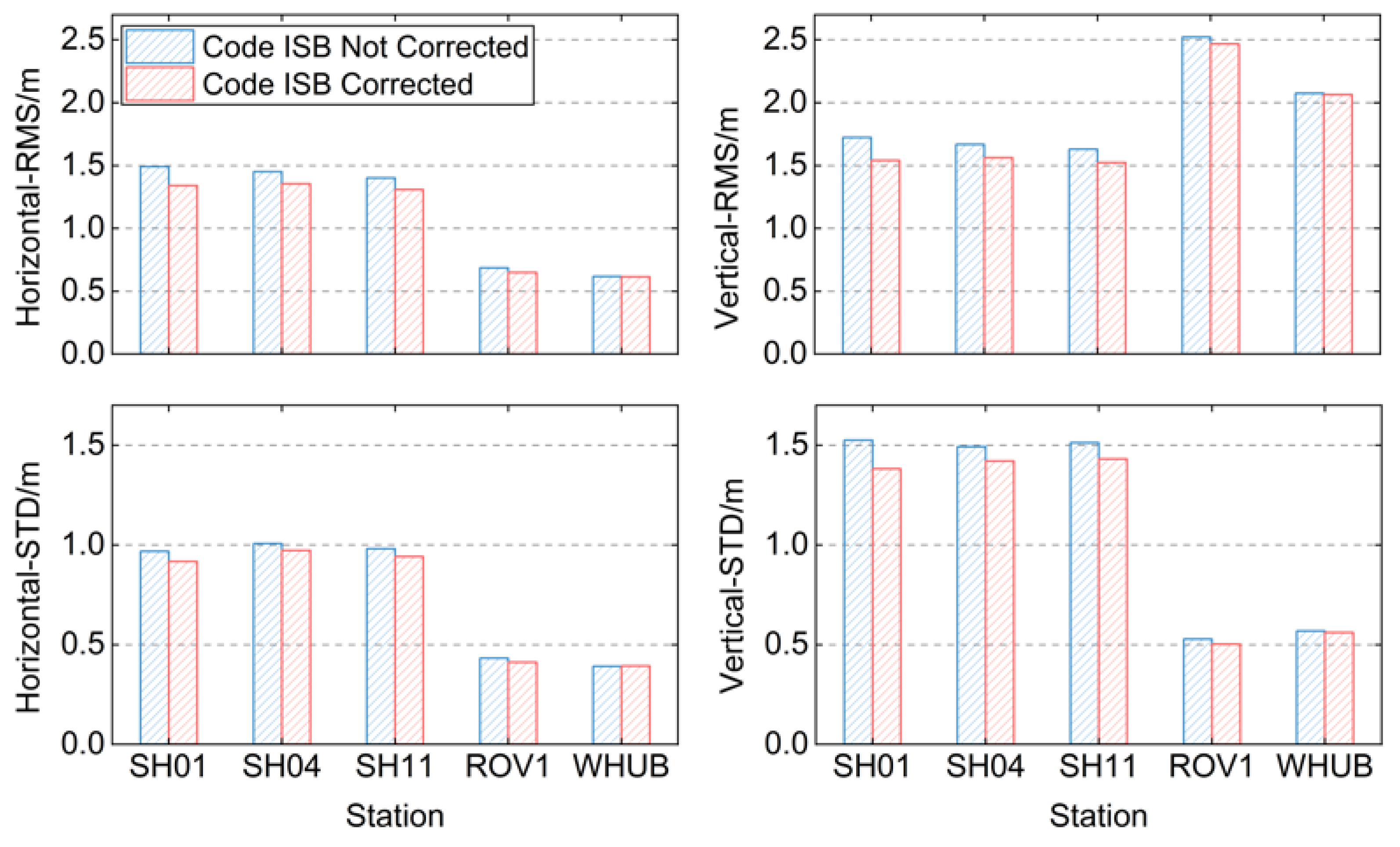
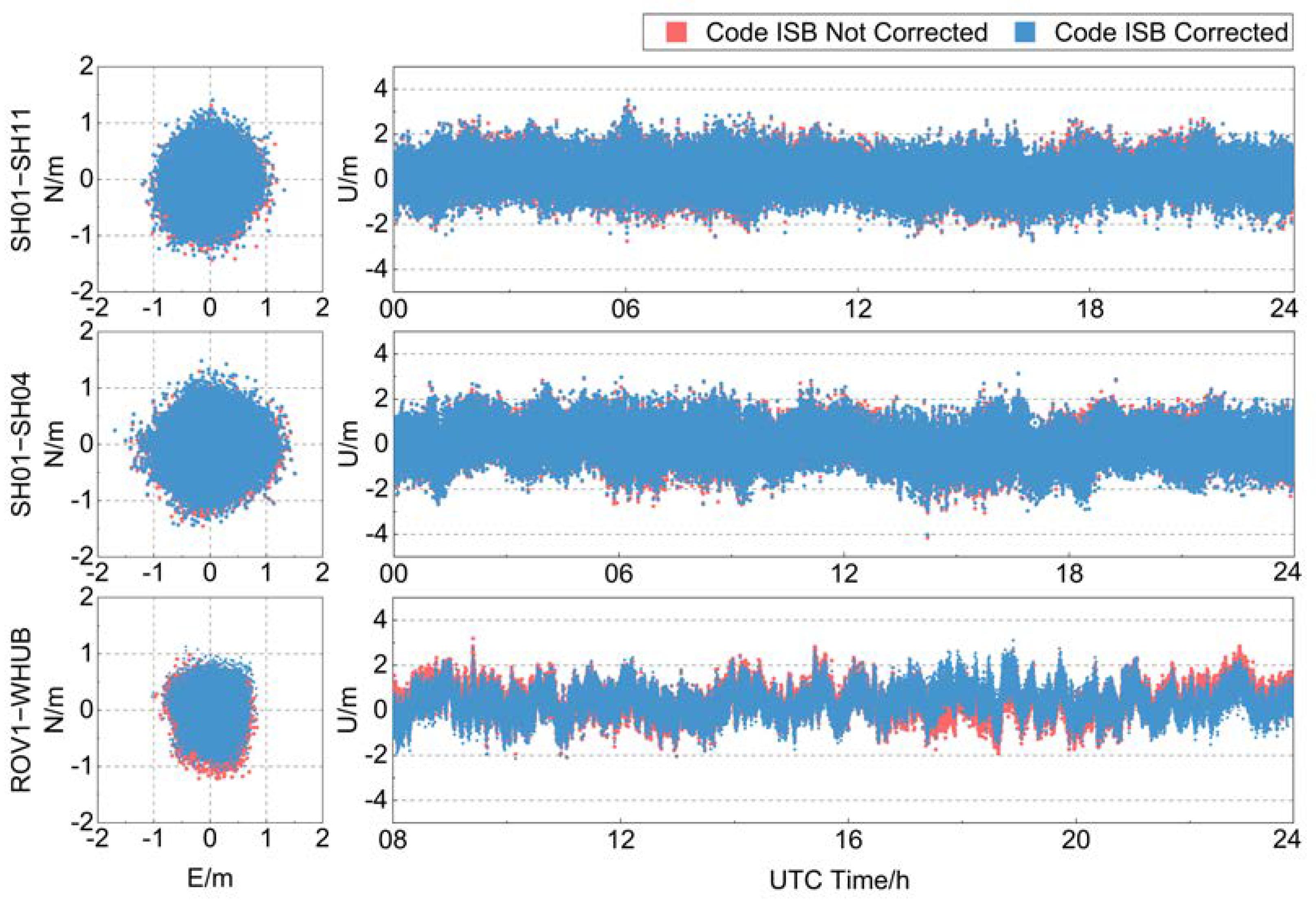
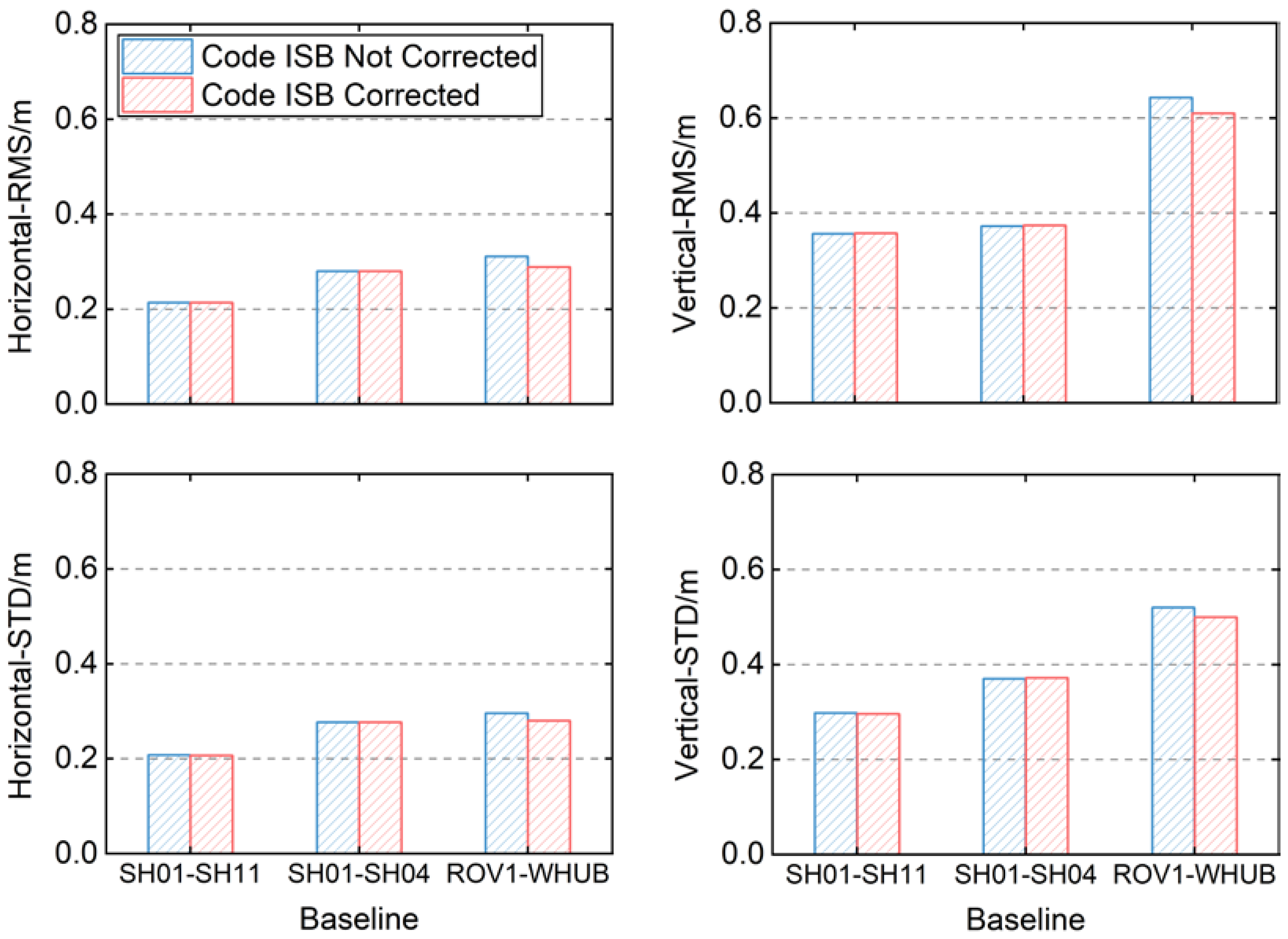
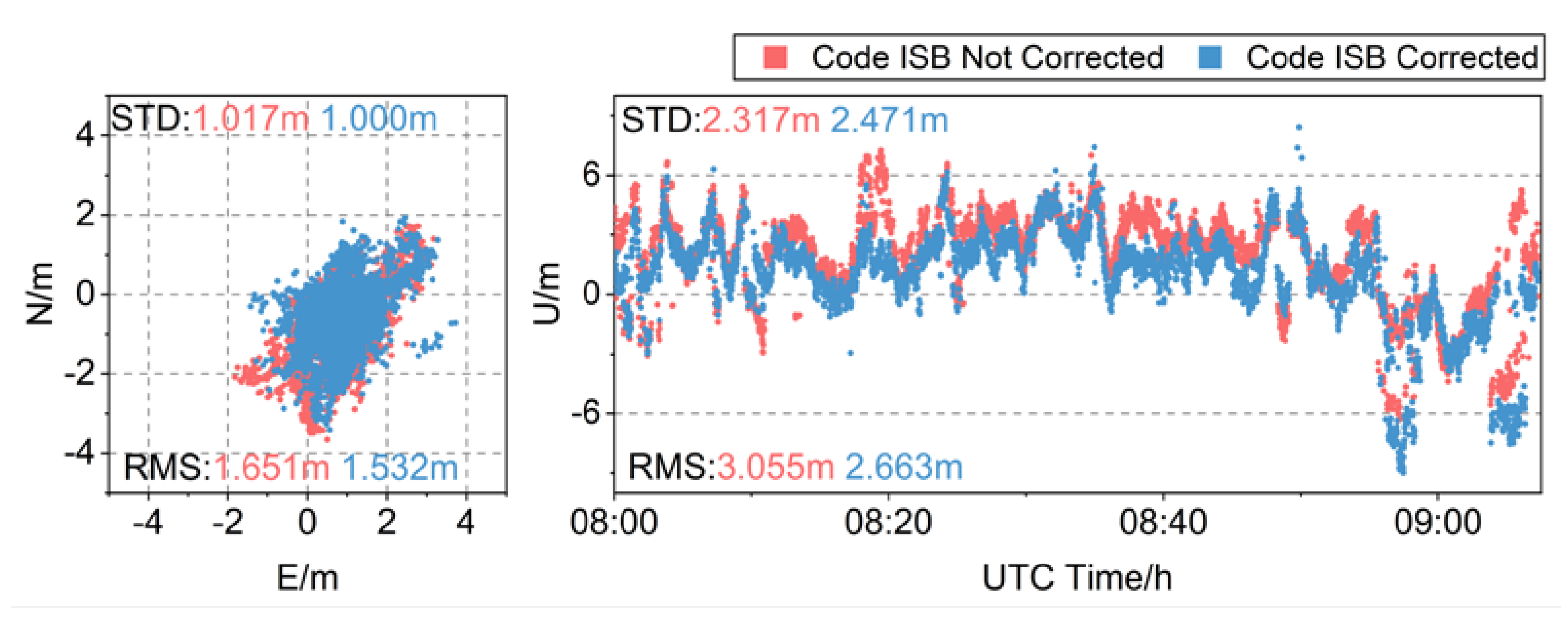
3.2.3. Impact of Code ISBs on Pseudorange Positioning
- (1)
- Pseudorange SPP
- (2)
- Pseudorange Differential Positioning
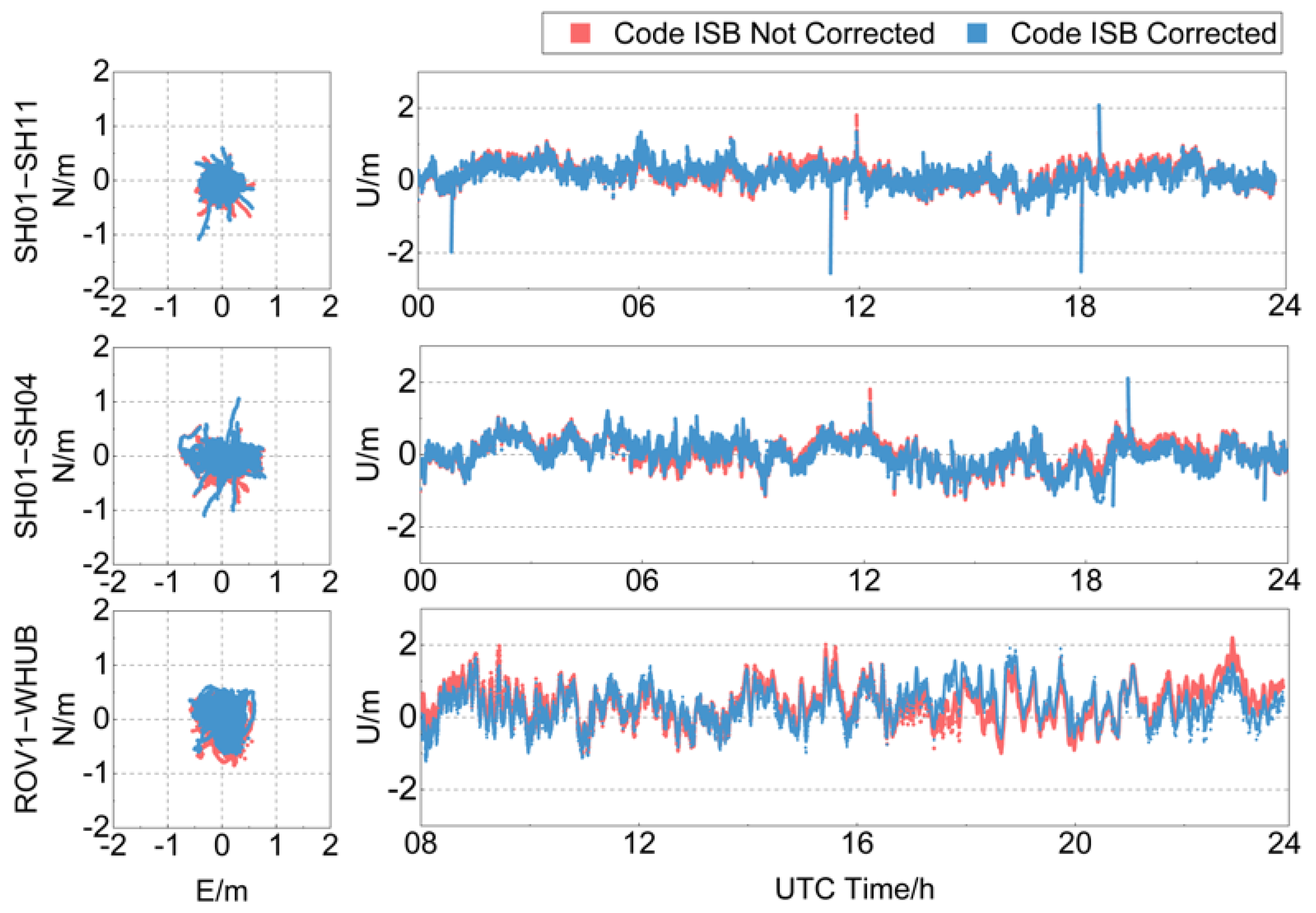
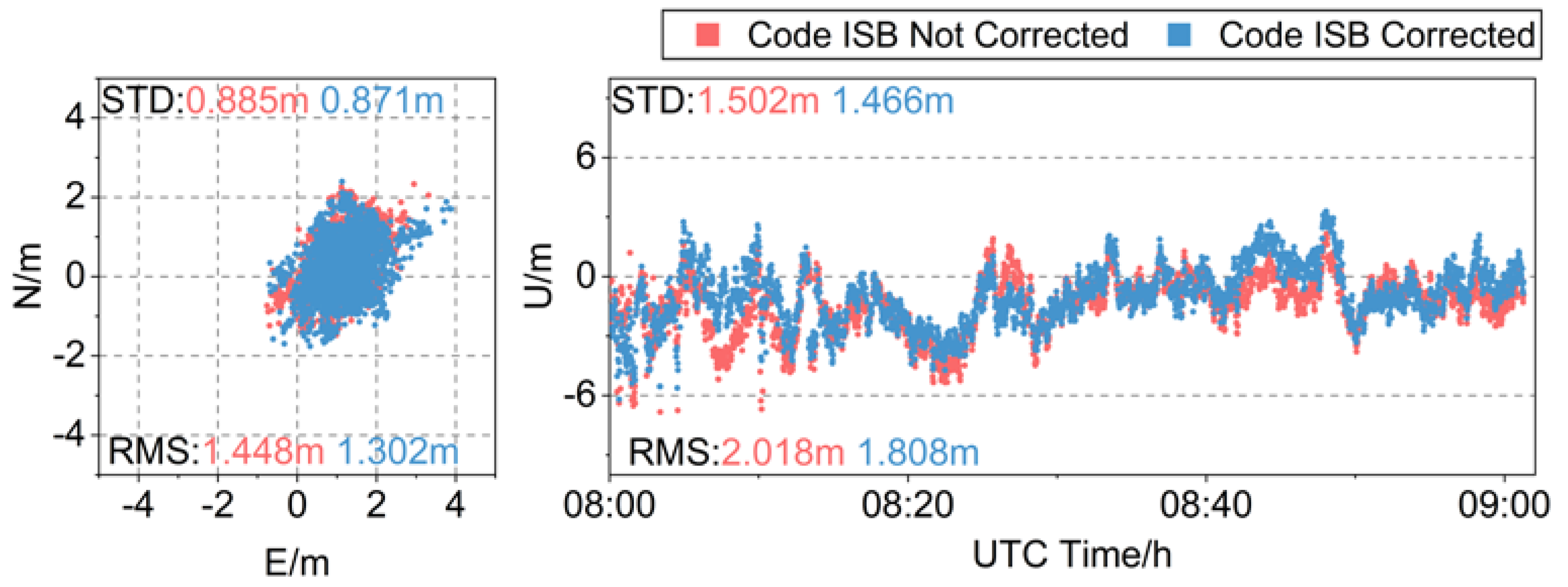
3.2.4. Impact of Code ISBs on Carrier-Phase-Smoothed Pseudorange Positioning
- (1)
- Carrier-Phase-Smoothed Pseudorange SPP
- (2)
- Carrier-phase-smoothed pseudorange differential positioning
3.3. Pseudorange Positioning Analysis of Low-Cost Receiver
3.3.1. Data Availability Analysis of Low-Cost Receiver
3.3.2. Analysis of Code ISB Characterization in Low-Cost Receiver
- (1)
- Un-Differential Code ISBs
- (2)
- Differential code ISBs
- (3)
- Code ISBs before and after carrier-phase smoothing
3.3.3. Impact of Code ISBs on Pseudorange Positioning
- (1)
- Pseudorange SPP
- (2)
- Pseudorange Differential Positioning
3.3.4. Impact of Code ISBs on Carrier-Phase-Smoothed Pseudorange Positioning
- (1)
- Carrier-phase-smoothed pseudorange SPP
- (2)
- Carrier-phase-smoothed pseudorange differential positioning
4. Discussion
5. Conclusions
- (1)
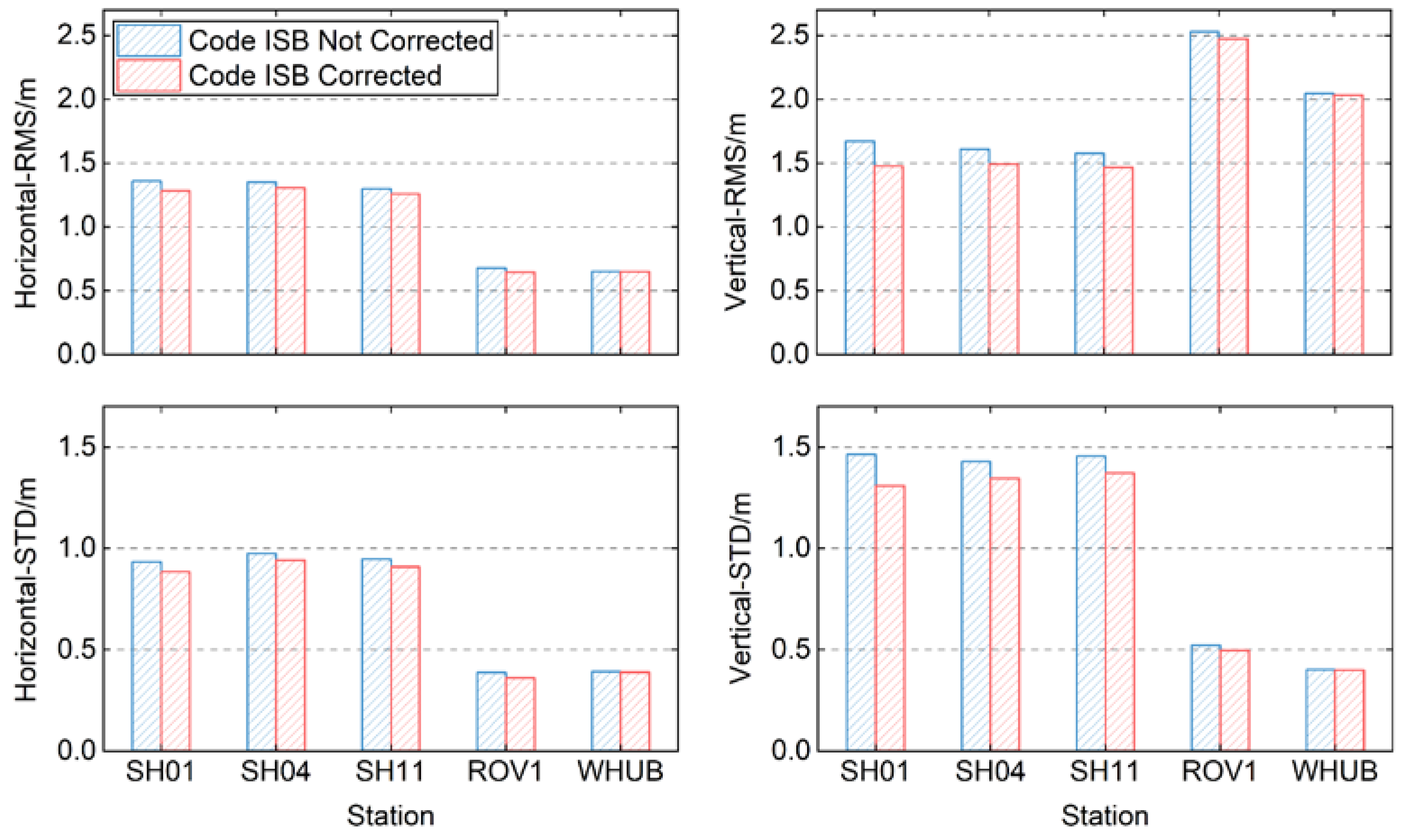
- Code ISBs vary among different receivers, but the STDs are close, which shows the code ISB distribution in the homogeneous receivers has strong consistency. The code ISBs in each type of geodetic GNSS receiver are about −0.3 m to −0.8 m. The code ISB in the baseline with homogeneous receivers is reduced to about −0.2 m, while the code ISB in the baseline with heterogeneous receivers is still large, about −0.5 m.
- (2)
- The STD values of code ISBs in the low-cost receiver u-blox M8T are larger than that in geodetic GNSS receivers, which are unstable in a short time. For low-cost receivers, correcting the code ISBs can improve the availability of positioning data at different satellite elevation angles, which can effectively improve the performance of low-cost navigation and positioning.
- (3)
- After correcting the code ISBs, the SPP position deviations of each geodetic GNSS receiver can be reduced by about 7%. For the baseline with homogeneous receivers, the influence of the code ISBs of the B1I frequency on positioning accuracy is less than 1%, which is negligible. For the baselines with heterogeneous receivers, the differential positioning position deviations can be reduced by 4% after correcting the code ISBs.
- (4)
- After correcting the code ISBs, the SPP position deviations of the low-cost receiver u-blox M8T can be reduced by about 11%, and the pseudorange differential positioning position deviations can be reduced by about 12%. Correcting the code ISBs in the low-cost receiver can effectively improve the pseudorange positioning accuracy.
- (5)
- The distribution dispersion of the estimated code ISBs can be reduced after carrier-phase smoothing. For geodetic GNSS receivers, the STD values of undifferential and differential code ISBs are reduced by 30% and 50%, respectively. For low-cost receivers, due to the unstable data quality, the STD values of code ISBs are reduced by 4% to 7%.
- (6)
- For geodetic GNSS receivers, the position deviations of the carrier-phase-smoothed pseudorange SPP and differential positioning with heterogeneous receivers can be reduced by about 9% and 6%, respectively, when correcting the code ISBs. For the low-cost receiver u-blox M8T, the position deviations of the carrier-phase-smoothed pseudorange SPP can be reduced by about 14% after ISB correction, and the differential positioning reduced by about 5%.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hegarty, C.; Powers, E.; Foville, B. Accounting for timing biases between GPS, modernized GPS, and Galileo signals. In Proceedings of the 36th Annual Precise Time and Time Interval Meeting, Washington, DC, USA, 7–9 December 2004; pp. 307–317. [Google Scholar]
- Montenbruck, O.; Hauschild, A.; Hessels, U. Characterization of GPS/GIOVE sensor stations in the CONGO network. Gps Solut. 2011, 15, 193–205. [Google Scholar] [CrossRef]
- Odijk, D.; Teunissen, P.J.G. Characterization of between-receiver GPS-Galileo inter-system biases and their effect on mixed ambiguity resolution. Gps Solut. 2013, 17, 521–533. [Google Scholar] [CrossRef]
- Paziewski, J.; Sieradzki, R.; Wielgosz, P. Selected properties of GPS and Galileo-IOV receiver intersystem biases in multi-GNSS data processing. Meas. Sci. Technol. 2015, 26, 95008–95009. [Google Scholar] [CrossRef]
- Deng, C.; Liu, Q.; Zou, X.; Tang, W.; Cui, J.; Wang, Y.; Guo, C. Investigation of Tightly Combined Single-Frequency and Single-Epoch Precise Positioning Using Multi-GNSS Data. Remote Sens. 2020, 12, 285. [Google Scholar] [CrossRef]
- Li, M.; Rovira-Garcia, A.; Nie, W.; Xu, T.; Xu, G. Inter-system biases solution strategies in multi-GNSS kinematic precise point positioning. Gps Solut. 2023, 27, 100. [Google Scholar] [CrossRef]
- Liu, X.; Jiang, W.; Chen, H.; Zhao, W.; Huo, L.; Huang, L.; Chen, Q. An analysis of inter-system biases in BDS/GPS precise point positioning. Gps Solut. 2019, 23, 116. [Google Scholar] [CrossRef]
- Mi, X.; Zhang, B.; Yuan, Y. Multi-GNSS inter-system biases: Estimability analysis and impact on RTK positioning. Gps Solut. 2019, 23, 81. [Google Scholar] [CrossRef]
- Mi, X.; Zhang, B.; Yuan, Y.; Luo, X. Characteristics of GPS, BDS2, BDS3 and Galileo inter-system biases and their influence on RTK positioning. Meas. Sci. Technol. 2020, 31, 15009. [Google Scholar] [CrossRef]
- Jiang, N.; Xu, Y.; Xu, T.; Xu, G.; Sun, C.; Schuh, H. GPS/BDS short-term ISB modelling and prediction. Gps Solut. 2017, 21, 163–175. [Google Scholar] [CrossRef]
- Tang, W.; Liu, Q.; Shen, M.; Deng, C.; Cui, J. BDS/GPS inter-system deviation estimation algorithm and performance analysis. Geod Geodyn. 2019, 39, 1–6. [Google Scholar]
- Zeng, A.; Yang, Y.; Ming, F.; Jing, Y. BDS–GPS inter-system bias of code observation and its preliminary analysis. Gps Solut. 2017, 21, 1573–1581. [Google Scholar] [CrossRef]
- Angrisano, A.; Gaglione, S.; Gioia, C. Performance assessment of GPS/GLONASS single point positioning in an urban environment. Acta Geod. Geophys. 2013, 48, 149–161. [Google Scholar] [CrossRef]
- Pan, L.; Zhang, Z.; Yu, W.; Dai, W. Intersystem Bias in GPS, GLONASS, Galileo, BDS-3, and BDS-2 Integrated SPP: Characteristics and Performance Enhancement as a Priori Constraints. Remote Sens. 2021, 13, 4650. [Google Scholar] [CrossRef]
- Pan, J.; Hu, X.; Zhou, S.; Tang, C.; Guo, R.; Zhu, L.; Tang, G.; Hu, G. Time synchronization of new-generation BDS satellites using inter-satellite link measurements. Adv. Space Res. 2018, 61, 145–153. [Google Scholar] [CrossRef]
- Jiao, G.; Song, S.; Jiao, W. Improving BDS-2 and BDS-3 joint precise point positioning with time delay bias estimation. Meas. Sci. Technol. 2020, 31, 25001. [Google Scholar] [CrossRef]
- Deng, C.; Qi, S.; Tang, W.; Hui, M.; Zou, X.; Wang, Y.; Li, Y.; Guo, C. Model comparison and performance analysis of multi-frequency precise positioning with the joint BDS-2 and BDS-3 system. Adv. Space Res. 2022, 69, 3044–3058. [Google Scholar] [CrossRef]
- Mi, X.; Sheng, C.; El-Mowafy, A.; Zhang, B. Characteristics of receiver-related biases between BDS-3 and BDS-2 for five frequencies including inter-system biases, differential code biases, and differential phase biases. Gps Solut. 2021, 25, 113. [Google Scholar] [CrossRef]
- Liu, W.; Wu, M.; Zhang, X.; Wang, W.; Ke, W.; Zhu, Z. Single-epoch RTK performance assessment of tightly combined BDS-2 and newly complete BDS-3. Satell. Navig. 2021, 2, 6. [Google Scholar] [CrossRef]
- Zhang, Z.; Pan, L. Current performance of open position service with almost fully deployed multi-GNSS constellations: GPS, GLONASS, Galileo, BDS-2, and BDS-3. Adv. Space Res. 2022, 69, 1994–2019. [Google Scholar] [CrossRef]
- Liu, H.; Shu, B.; Xu, L.; Qian, C.; Zhang, R.; Zhang, M. Accounting for Inter-System Bias in DGNSS Positioning with GPS/GLONASS/BDS/Galileo. J. Navig. 2017, 70, 686–698. [Google Scholar] [CrossRef]
- Kang, C. A Differential Dynamic Positioning Algorithm Based on GPS/Beidou. Procedia Eng. 2016, 137, 590–598. [Google Scholar] [CrossRef]
- Meng, F.; Li, S. BDS triple-frequency carrier phase combination smoothing pseudo-range algorithm. IOP conference series. Mater. Sci. Eng. 2020, 780, 32044. [Google Scholar]
- Cui, J.; Yan, R.; Deng, C.; Tang, W.; Zou, X.; Shen, M.; Liu, Q.; Wang, Y.; Li, Y. GPS + BDS Network Real-Time Differential Positioning Using a Position Domain Estimation Method. Remote Sens. 2019, 11, 1480. [Google Scholar] [CrossRef]
- Tang, W.; Cui, J.; Hui, M.; Deng, C. Performance Analysis for BDS Phase-smoothed Pseudorange Differential Positioning. J. Navig. 2016, 69, 1011–1023. [Google Scholar] [CrossRef]
- Teunissen, P.J.G.; Amiri-Simkooei, A.R. Least-squares variance component estimation. J. Geodesy. 2008, 82, 65–82. [Google Scholar] [CrossRef]
- King, R.W.; Bock, Y. Documentation for the GAMIT GPS Analysis Software; Massachusetts Institute of Technology: Cambridge, MA, USA, 1999. [Google Scholar]
- Hatch, R.R. The Synergism of GPS Code and Carrier Measurements. Int. Geod. Symp. Satell. Doppler Position. 1982, 2, 1213–1231. [Google Scholar]
- Swider, R.; Kaser KBra, R. Recent Developments in the LAAS Program. In Proceedings of the Position Location and Navigation Symposium IEEE 1998, Palm Springs, CA, USA, 20–23 April 1998; pp. 441–470. [Google Scholar]
- Bona, P. Precision, Cross Correlation, and Time Correlation of GPS Phase and Code Ob-servations. Gps Solut. 2000, 4, 3–13. [Google Scholar] [CrossRef]
- Geng, J.; Chen, X.; Pan, Y.; Mao, S.; Li, C.; Zhou, J.; Zhang, K. PRIDE PPP-AR: An open-source software for GPS PPP ambiguity resolution. Gps Solut. 2019, 23, 91. [Google Scholar] [CrossRef]
- Klobuchar, J.A. Ionospheric time delay algorithm for single frequency GPS users. IEEE Trans. Aerosp. Electron. Syst. 1987, 23, 325–331. [Google Scholar] [CrossRef]
- Saastamoinen, J. Atmospheric Correction for the Troposphere and Stratosphere in Radio Ranging Satellites. In Geophysical Monograph Series; American Geophysical Union: Washington, DC, USA, 1972; Volume 15, pp. 247–251. [Google Scholar]
- Zhang, B.; Teunissen, P.J.G. Characterization of multi-GNSS between-receiver differential code biases using zero and short baselines. Sci. Bull. 2015, 60, 1840–1849. [Google Scholar] [CrossRef]
- Pan, L.; Zhang, X.; Liu, J.; Li, X.; Li, X. Performance Evaluation of Single-frequency Precise Point Positioning with GPS, GLONASS, BeiDou and Galileo. J. Navig. 2017, 70, 465–482. [Google Scholar] [CrossRef]
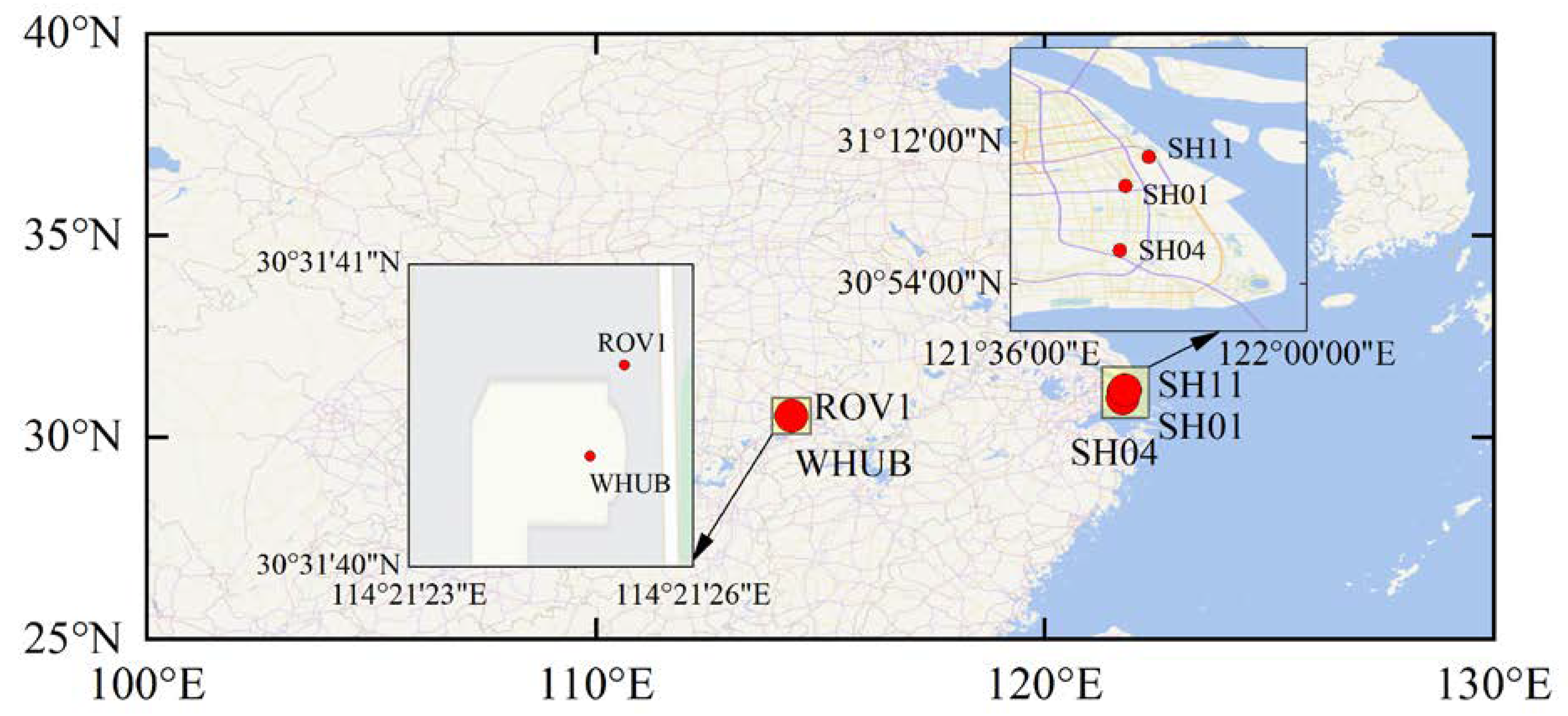










| Station Name | Receiver Type | Firmware Version | Elevation Mask | Start Time (UTC) | End Time (UTC) | Sampling Interval | Location |
|---|---|---|---|---|---|---|---|
| SH01 | NOV OEM4-G2 | 2.10 | 10° | 29 June 2021 00:05:23 | 30 June 2021 00:05:23 | 1 s | Shanghai, China |
| SH04 | NOV OEM4-G2 | 2.10 | 10° | 29 June 2021 00:05:23 | 30 June 2021 00:05:23 | 1 s | Shanghai, China |
| SH11 | NOV OEM4-G2 | 2.10 | 10° | 29 June 2021 00:05:23 | 30 June 2021 00:05:23 | 1 s | Shanghai, China |
| ROV1 | PANDA PD51A | 5.0.0 | 10° | 14 January 2021 08:10:42 | 14 January 2021 23:59:37 | 1 s | Wuhan, China |
| WHUB | TRIMBLE ALLOY | 6.05 | 10° | 14 January 2021 07:48:03 | 14 January 2021 23:59:41 | 1 s | Wuhan, China |
| Items | Methods |
|---|---|
| Satellite systems | BDS-2/BDS-3 |
| Estimator | Least Square method |
| Observations | Pseudorange observableCarrier-phase-smoothed pseudorange observable |
| Signal selection | BDS-2: B1I; BDS-3: B1I |
| Elevation mask | 10° |
| Observation weight | Elevation dependent weight |
| Ionospheric delay | Undifferenced: Klobuchar model [32] |
| Differenced: Not estimated | |
| Tropospheric delay | Undifferenced: Saastamoinen model [33] |
| Differenced: Not estimated |
| Station | Availability Epoch when the ISB Is Not Corrected | Availability Epoch when the ISB Is Corrected | Total Epoch | Data Availability Rate |
|---|---|---|---|---|
| SH01 | 86,400 | 86,400 | 86,400 | 100% |
| SH04 | 86,400 | 86,400 | 86,400 | 100% |
| SH11 | 86,400 | 86,400 | 86,400 | 100% |
| ROV1 | 56,936 | 56,936 | 56,936 | 100% |
| WHUB | 56,936 | 56,936 | 56,936 | 100% |
| Baseline Name | Length | Roving Station Receiver Type | Base Station Receiver Type |
|---|---|---|---|
| SH01-SH11 | 7.52 km | NOV OEM4-G2 | NOV OEM4-G2 |
| SH01-SH04 | 15.14 km | NOV OEM4-G2 | NOV OEM4-G2 |
| ROV1-WHUB | 0.02 km | PANDA PD51A | TRIMBLE ALLOY |
| Elevation Mask | Availability Epoch when the ISB Is Not Corrected | Availability Epoch when the ISB Is Corrected | Total Epoch | Data Availability Rate |
|---|---|---|---|---|
| 10° | 1857 | 2045 | 4150 | 45.18–49.28% |
| 20° | 4035 | 4046 | 4150 | 97.23–97.49% |
| 30° | 3065 | 3141 | 4150 | 73.86–75.52% |
| 40° | 475 | 479 | 4150 | 11.45–11.52% |
| 50° | 0 | 0 | 4150 | 0% |
| Station/Baseline | Horizontal Components RMS/m | Change/% | Vertical Components RMS/m | Change/% | ||
|---|---|---|---|---|---|---|
| Before Smoothing | After Smoothing | Before Smoothing | After Smoothing | |||
| SH01-SH11 | 0.421 | 0.215 | 48.93 | 0.704 | 0.358 | 49.15 |
| ROV1-WHUB | 0.359 | 0.289 | 19.50 | 0.723 | 0.610 | 15.63 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, Z.; Cui, J.; Liu, Z.; Su, X.; Xiang, Y.; Xu, Y.; Deng, C.; Hui, M.; Li, Q. Influence of Inter-System Biases on Combined Single-Frequency BDS-2 and BDS-3 Pseudorange Positioning of Different Types of Receivers. Remote Sens. 2024, 16, 1710. https://doi.org/10.3390/rs16101710
Ma Z, Cui J, Liu Z, Su X, Xiang Y, Xu Y, Deng C, Hui M, Li Q. Influence of Inter-System Biases on Combined Single-Frequency BDS-2 and BDS-3 Pseudorange Positioning of Different Types of Receivers. Remote Sensing. 2024; 16(10):1710. https://doi.org/10.3390/rs16101710
Chicago/Turabian StyleMa, Zeyu, Jianhui Cui, Zhimin Liu, Xing Su, Yan Xiang, Yan Xu, Chenlong Deng, Mengtang Hui, and Qing Li. 2024. "Influence of Inter-System Biases on Combined Single-Frequency BDS-2 and BDS-3 Pseudorange Positioning of Different Types of Receivers" Remote Sensing 16, no. 10: 1710. https://doi.org/10.3390/rs16101710
APA StyleMa, Z., Cui, J., Liu, Z., Su, X., Xiang, Y., Xu, Y., Deng, C., Hui, M., & Li, Q. (2024). Influence of Inter-System Biases on Combined Single-Frequency BDS-2 and BDS-3 Pseudorange Positioning of Different Types of Receivers. Remote Sensing, 16(10), 1710. https://doi.org/10.3390/rs16101710







