Pre-Launch Multi-Energy Radiance Calibration of the OMS-N

Abstract

This paper presents the prelaunch radiometric calibration of the Ozone Monitor Suite-Nadir (OMS-N) instrument, a vital payload on the FY-3F satellite. FY-3F achieved a successful launch on 3 August 2023. The radiance calibration of the OMS-N instrument was achieved using an integrating sphere, with known exit radiance ascertained through a transferring radiometer. The calibration model incorporates six energy levels. The Solar Simulator Standard System was employed to validate the calibration results, selecting specific rows to represent varying spatial dimensions. Considering the influence of xenon lamp characteristic peaks and transmission errors during the calibration process, the average deviation remained within 2.3% for the VIS channel, 3% for the UV1 channel, and 2.2% for the UV2 channel. Furthermore, the uncertainty of the radiometric calibration was analyzed. The results indicated an absolute uncertainty of 2.33% for both the UV1 and UV2 channels and 1.69% for the VIS channel. The relative uncertainty was 1.84% for both the UV1 and UV2 channels and 1.45% for the VIS channel. The obtained calibration coefficients are accurate and reliable and can be used for the inversion of product parameters, which is of great significance to the quantitative application of satellite data and the advancement of scientific research on quantitative remote sensing.

1. Introduction

In recent years, China’s space optical remote sensing detection has witnessed rapid development and growth. After years of continuous progress and accumulation, the functionality of China’s on-board space probes has matured significantly. This evolution is attributed to the critical role of optical radiation as the fundamental carrier of optical remote sensing information. Consequently, the key application of remote sensing instruments is to achieve the inversion of atmospheric trace component gases through the analysis of atmospheric backscattered spectral radiation, establishing corresponding relationship models in the process. The achievement of high-precision trace gas detection hinges not solely on the performance of the instrument itself but also on the quality of its detection data. In other words, it depends on the extent to which the original data products enable the inversion of target gas content. Within the quantitative inversion of payload detection data, ground radiance calibration [1,2] stands as an irreplaceable and pivotal technology. To ensure the practical value of the quantitative detection results, the radiance calibration accuracy of satellite-based spaceborne optical remote sensing detection instruments has escalated significantly. The primary objective of radiance calibration for on-board high-resolution imaging spectrometers is to calibrate the Digit Number (DN) output of the payload’s charge-coupled device (CCD) with the incident DN of the CCD. One such instrument, the Ozone Monitor Suite-Nadir (OMS-N), is a prominent component of the FY-3(06) satellite payload. The FY-3F satellite comprises 10 remote sensing instruments that offer advanced quantitative remote sensing capabilities, which can effectively improve the accuracy of detection. Based on global imaging and vertical atmospheric detection techniques, FY-3F enables Earth surface imaging, revealing global ozone distribution and monitoring global snow and ice cover, sea surface temperature, natural disasters, and ecological environments. It also provides remote sensing information required for short-term climate prediction and climate change prediction, improving the ability to respond to emergencies and upgrading the overall efficacy of meteorological disaster prevention and mitigation. FY-3F will be grouped with other FY-3 satellites in orbit for all-weather meteorological monitoring, improving the accuracy and timeliness of weather forecasting. The OMS-N in FY-3 is expected to provide daily global information and dynamic monitoring of critical atmospheric trace gases that influence air quality and climate change. These gases include total ozone (O₃) and profiles at Nadir, sulfur dioxide (SO₂), nitrogen dioxide (NO₂), BrO, CHOLC, aerosols, and clouds, among others.

OMS-N is a spectrometer designed to measure atmospheric backscattered radiance and solar irradiance within the ultraviolet and visible range, spanning from 250 to 495 nm, with a spectral resolution ranging between 0.5 to 1.0 nm. It employs a wide-angle telescope to achieve global coverage on a daily basis.

Ground-based calibrations serve two primary purposes: establishing radiometric sensitivity [3,4,5] and providing measurement uncertainty data for the payload. On-orbit calibrations [6,7] are subsequently performed to continuously monitor the degradation of payload sensitivity. Regardless of the method or equipment employed for calibration, it is crucial to recognize that calibration results may not be absolutely accurate. Therefore, there is an inherent systematic error in radiation calibration that cannot be entirely eliminated. To meet the stringent radiation calibration accuracy requirements of OMS-N, it is imperative to construct an instrument radiometric response model using a multi-energy approach, involving stable and uniform light sources [8,9]. This method plays a pivotal role in correcting the effects of radiometric nonlinearities in OMS-N.

The radiometric calibration of OMS-N is crucial to the quantification of remote sensing products because it enables the conversion of the electronic information received by OMS-N into real and reliable radiometric values. Moreover, acquiring high-precision quantitative data from OMS-N highly depends on the accuracy of ground-based radiometric calibration. For more accurate data for the inversion of global atmospheric ozone content, this study focused on the pre-launch radiometric calibration of the OMS-N instrument, conducted under vacuum conditions. The factors affecting the OMS-N radiative transfer link were comprehensively analyzed, and multi-energy-level radiometric calibration methods were investigated through laboratory validation tests. Subsequently, a radiance response model and coefficients for in-flight conditions were derived. Additionally, we established a straightforward validation approach to test and refine the calibration results. This study makes scientific and application-oriented contributions, achieving remote sensing data of replacement products that conform to the expected quality standards.

2. Instrument Description

The OMS-N is a wide field-of-view (FOV) imaging spectrograph, employing two CCDs as detectors to facilitate simultaneous spectral and spatial imaging. One dimension on the CCD corresponds to the expansive FOV across the track in the flight direction, while the other dimension registers the spectrum.

Table 1. Specifications of OMS-N.

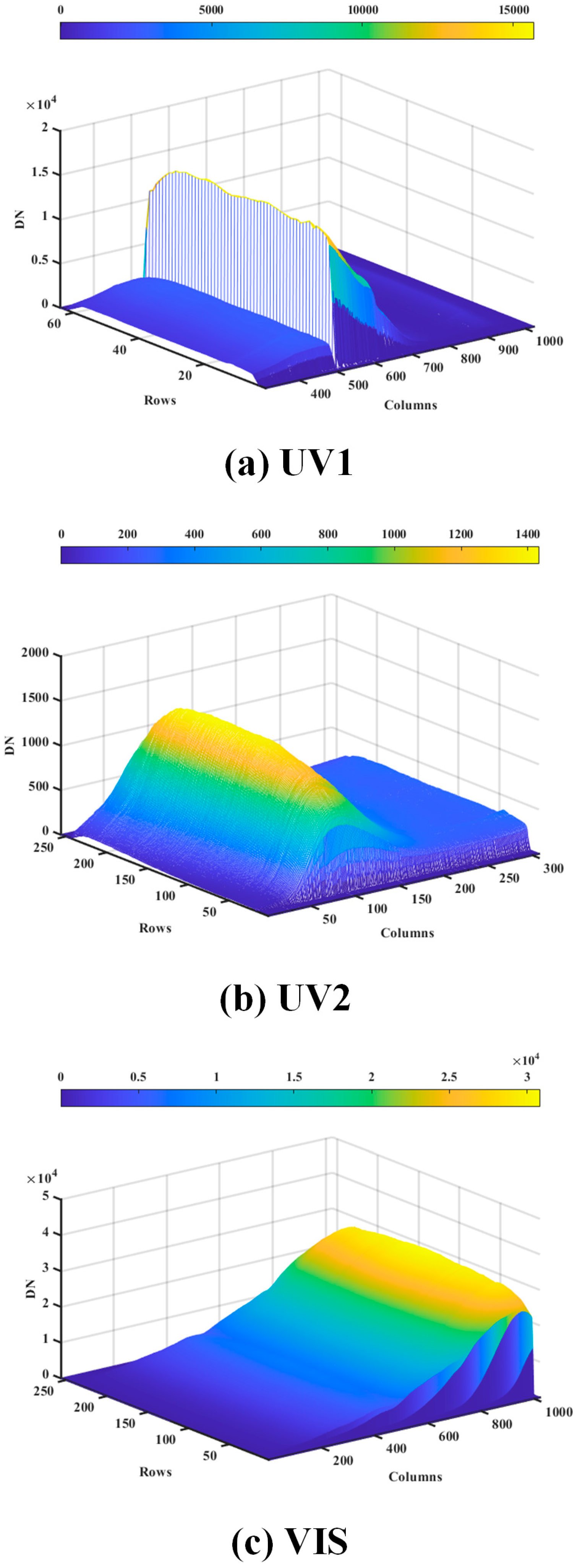
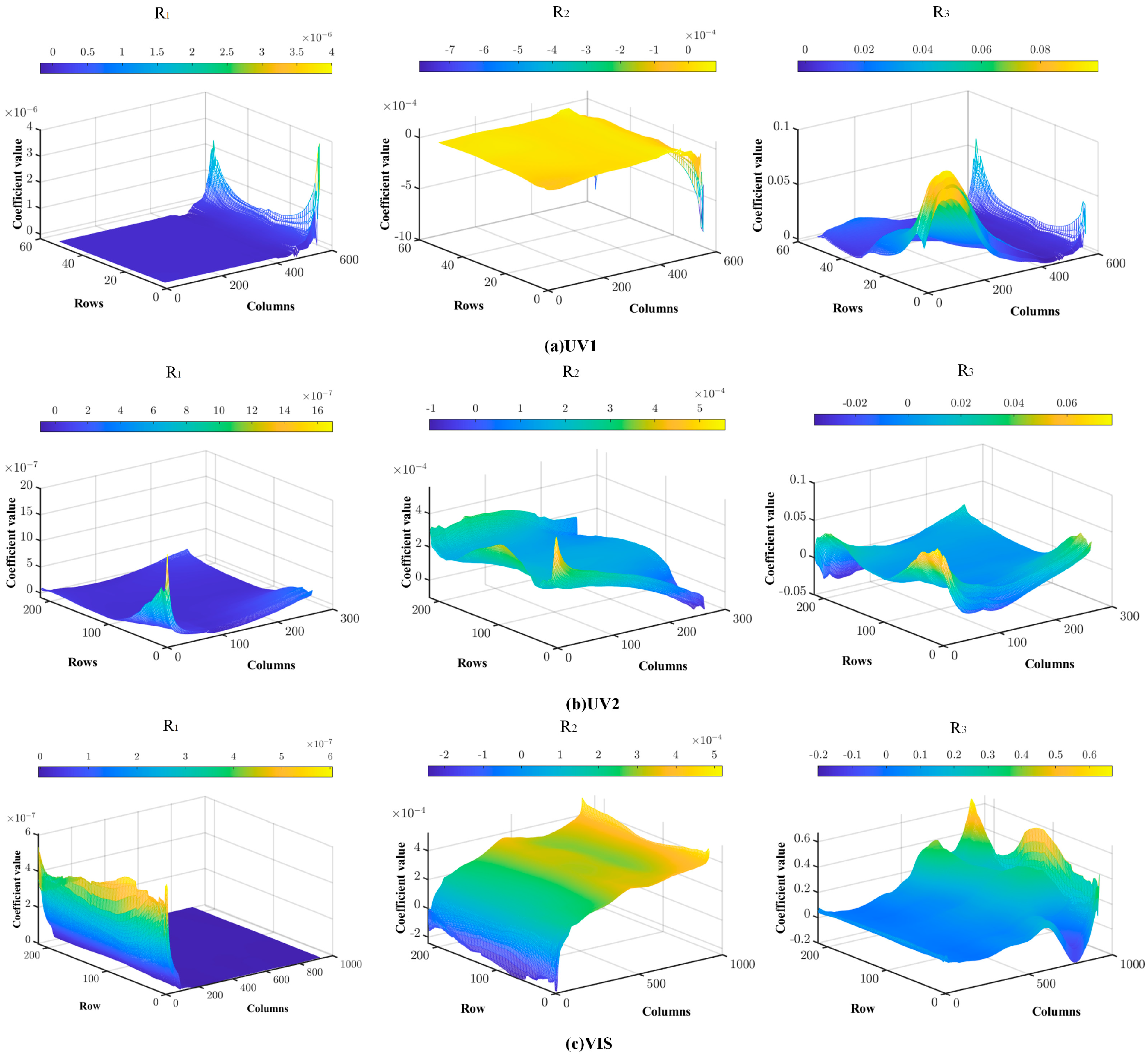
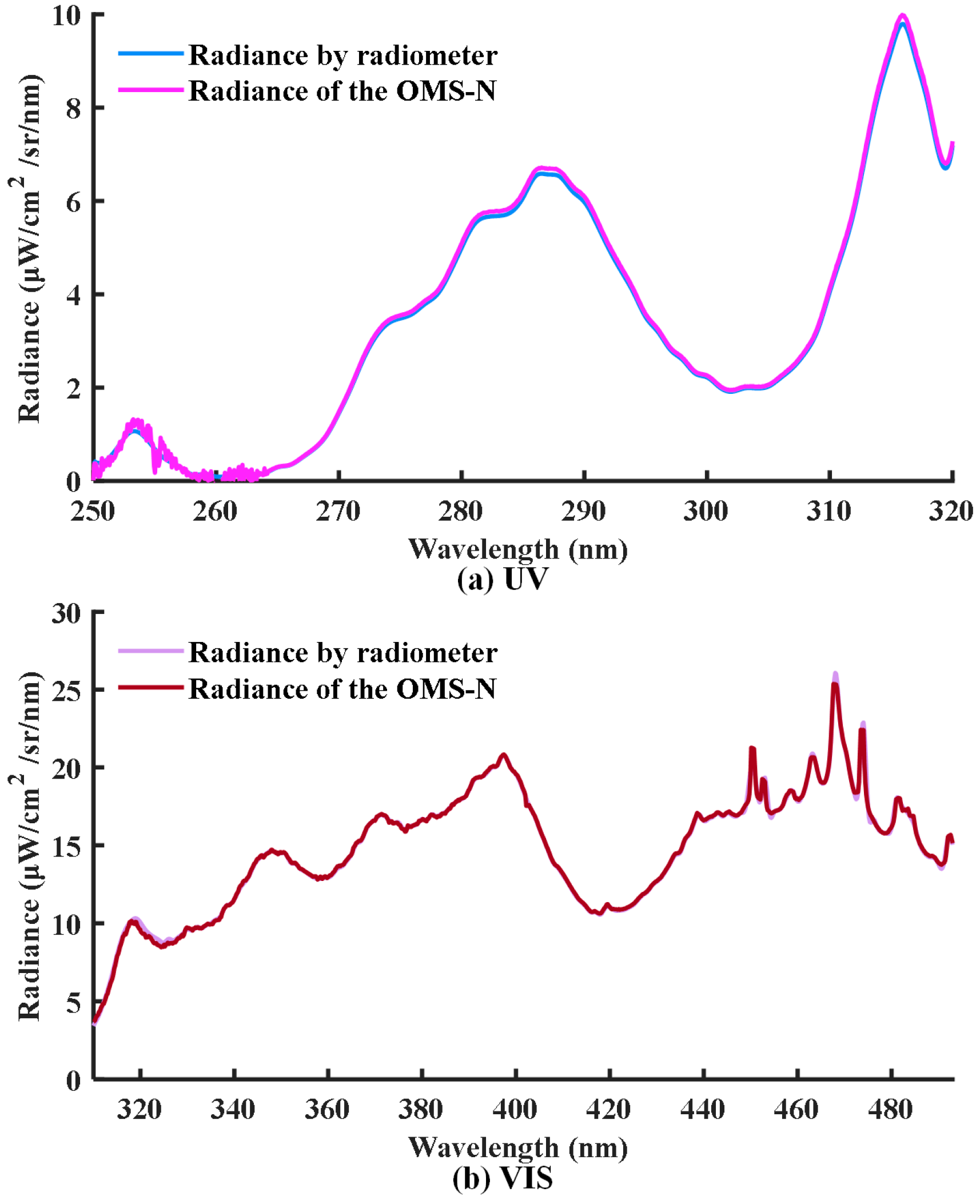
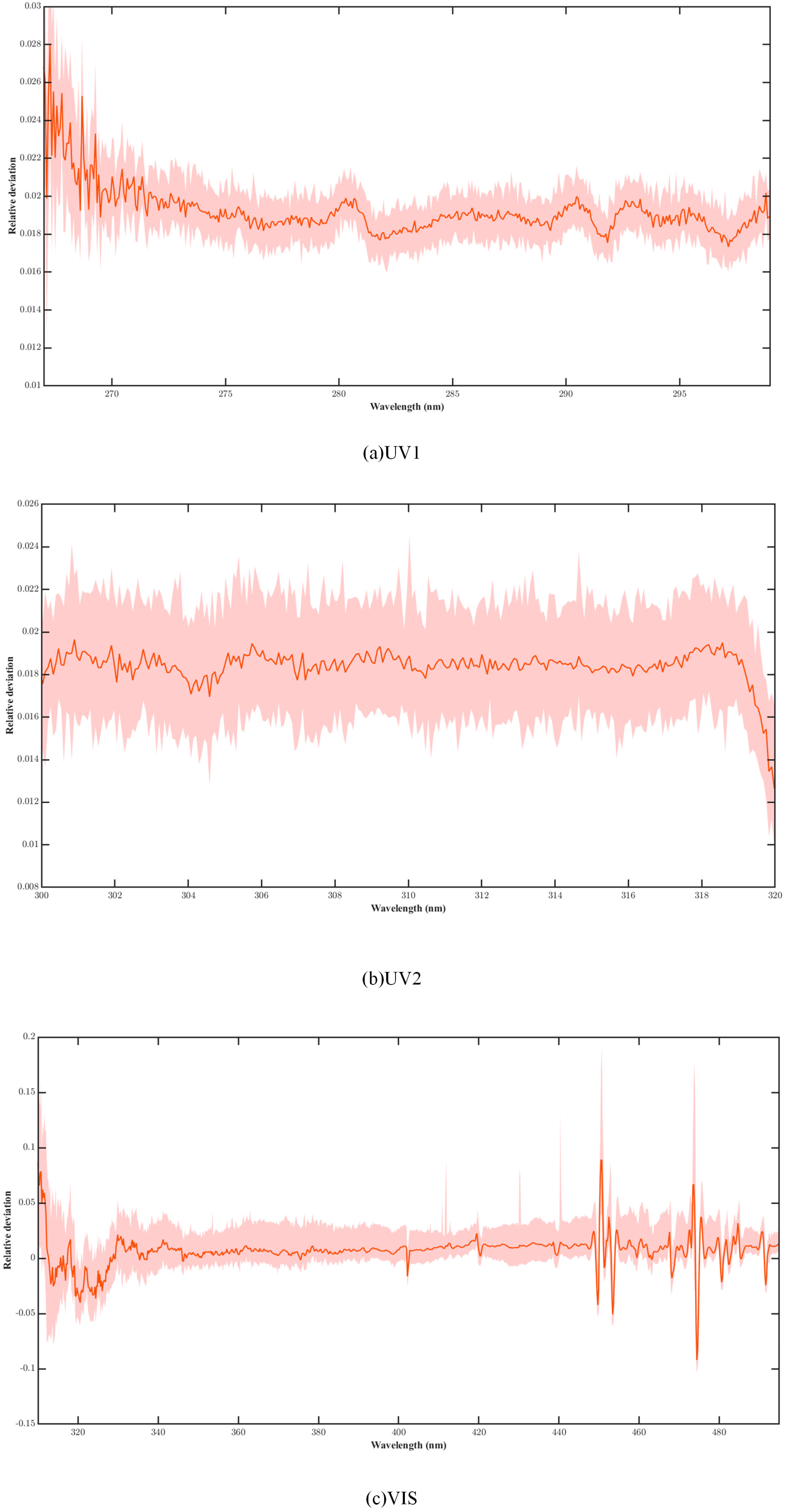
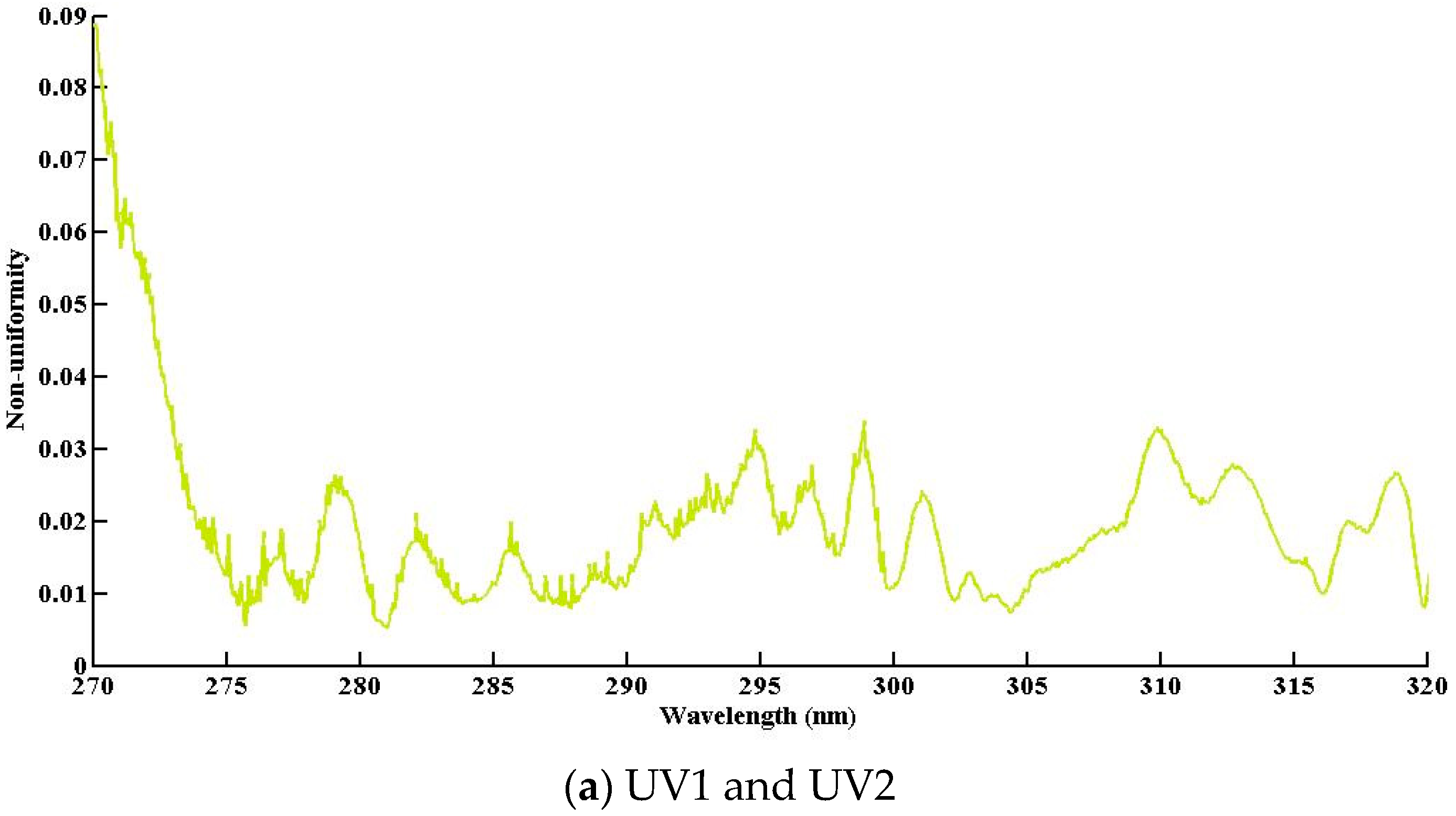
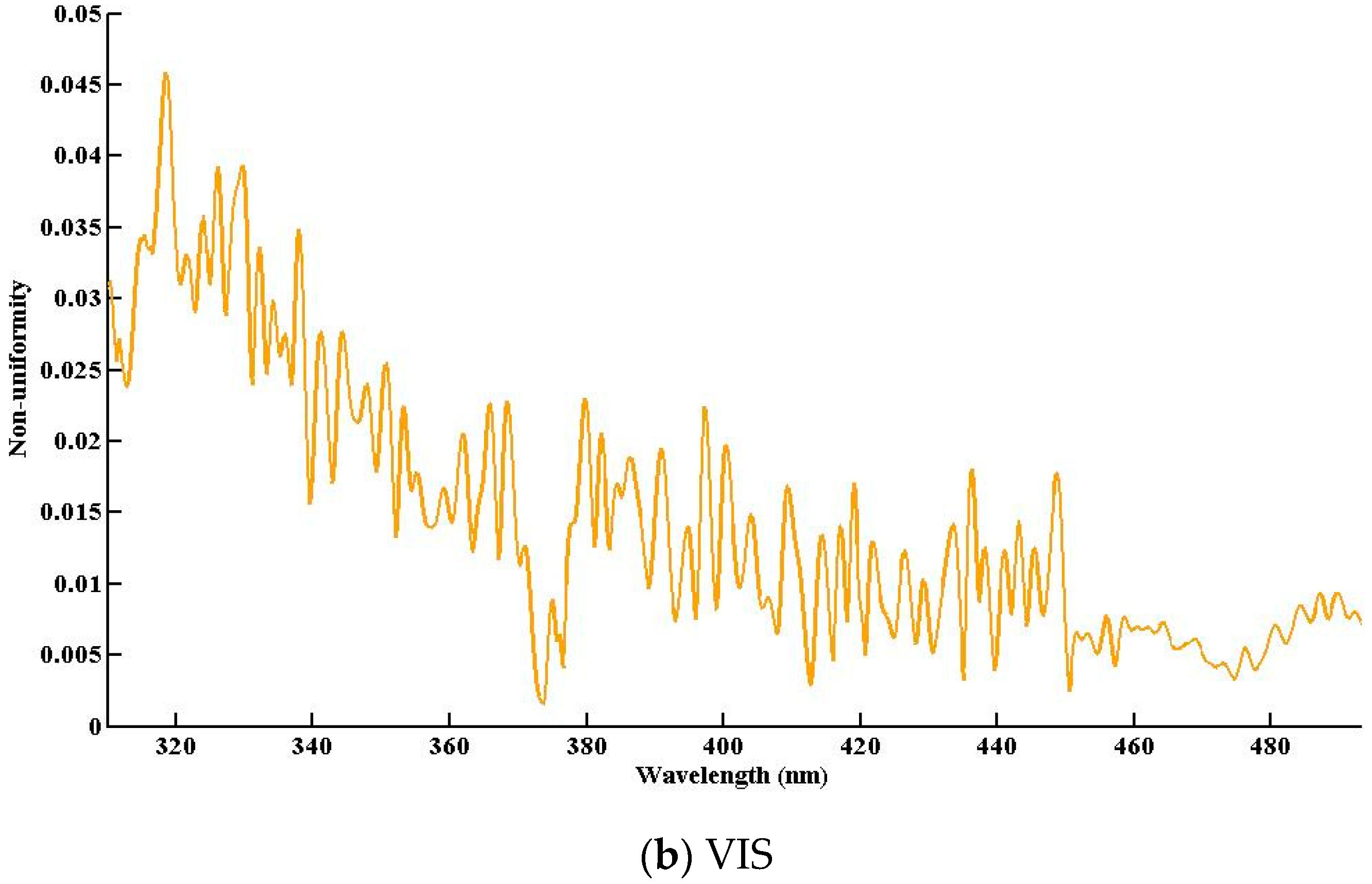
Index	Characteristics
Scientific Objectives	O3, SO2, NO2, BrO, O3 profile, Aerosol, Cloud, etc.
Channel	UV1	UV2	VIS
Spectral range/nm	250–300 nm	300–320 nm	310–495 nm
Spectral resolution/nm	~1	0.5	~0.6
Field of View	112°
Spatial resolution	28 km × 21 km (at nadir)	7 km × 7 km (at nadir)	7 km × 7 km (at nadir)
SNR	>50	>200	>200@ UV >1000@ VIS
Wavelength calibration accuracy	0.05 nm	0.05 nm	0.05 nm
Relative radiometric calibration accuracy	2%	2%	2%
Absolute radiometric calibration accuracy	3%	3%	3%

provides an overview of critical OMS-N specifications.

OMS-N features three spectral channels with two CCDs, one with spatial dimension merger numbers varying with wavelength, therefore divided into the UV1 channel (wavelength range: 250–300 nm) and the UV2 channel (wavelength range: 300–320 nm), and the other CCD operates on the visible channel (wavelength range: 310–495 nm). The primary objective of radiance calibration is to establish a mathematical relationship between the DN output of the OMS-N and the radiance of the incident light. However, due to the presence of ambient background radiation and the spectral, temporal, and polarization variability of radiance, achieving high-precision radiance calibration is a notably challenging and complex task.

Figure 1 illustrates the block diagram depicting the operational principle of OMS-N. Atmospheric backscattered radiation enters the instrument through the primary mirror of the wide-angle telescope system, where it is reflected and subsequently deflected by the depolarizer. The second mirror within the super wide-angle telescope system converges the light onto the incident slit of the spectrometer. A dichroic mirror divides the light into two components: one directed to the UV1 and UV2 channel through a plane grating, passing through the imaging objective lens, and eventually forming an image on the FPA of the CCD detector. The other component is routed to the VIS channel, similarly coupled by a plane grating and imaging objective lens, before reaching the CCD detector.

To ensure long-term stability and a lifespan exceeding 8 years, on-orbit calibration plays a pivotal role. Two diffusers are utilized for measuring solar irradiance, and wavelength shift monitoring is achieved by exploiting solar Fraunhofer lines during solar calibration. Additionally, a white-light source serves as an inter-calibration reference for detector performance.

Temperature control for the optical bench and detector module is accomplished through a passive radiator plate. The operational temperature of the optical bench is maintained at 293.15 ± 2 K, while the detector operates at a lower temperature of −243.15 K.

3. Radiance Calibration

3.1. Principle of Radiance Calibration

Radiance calibration for spaceborne optical imaging spectrometers typically employs one of two methods: [6,7] the first involves a standard diffuser coupled with a 1000 W NIST lamp [10], while the second method employs an integrating sphere [11,12,13]. In the diffuser method [11,14,15], a standard lamp with a known radiance illuminates a standard diffuse reflector, creating a large-area light source of known radiance that then irradiates the target under measurement. The alternative approach utilizes a large-aperture integrating sphere for radiance calibration [16,17]. Due to its uniform exit light distribution and substantial aperture, this method ensures improved measurement accuracy of spatial response characteristics. After calibration by a Metrological Institute, the radiance value at the integrating sphere’s exit surface can be directly employed for ground-based radiance calibration. The OMS-N is a wide FOV imaging spectrometer with a 112° FOV. Regardless of the chosen radiance calibration method, a single calibration cannot cover the entire field of view. Consequently, the radiance calibration process necessitates rotating the instrument through a rotary table in the direction of the Earth port, conducting multiple measurements to complete radiance response calibration.

For the calibration of OMS-N, an integrating sphere is used as a standard light source. The radiance emitted from the integrating sphere is adjustable, and the radiance at each energy level is calibrated using a radiometer that has been directly calibrated by a black body. Different light sources are selected for radiometric calibration based on different spectral channels of OMS-N. The integrating sphere system comprises a xenon lamp for calibrating the UV1 and UV2 channel and a tungsten lamp for calibrating the VIS channel. Xenon lamps yield high output energy at 250–320 nm and characteristic long-wave peaks near 460 nm, which can enhance the accuracy of radiometric calibration at 460 nm, and tungsten halogen lamps are exceedingly low in energy at 250–300 nm but exhibit smooth spectral curves at 310–493 nm. Given the OMS-N’s 112° FOV, a single illumination can only cover a 2° portion of the field of view. Consequently, it is necessary to adjust the turntable 57 times during calibration to cover the full OMS-N field of view. To account for the OMS-N’s non-linearity, the integrating sphere is controlled to output different spectral radiances at six levels (L(i), i = 1, 2, 3, 4, 5, 6). The distribution of the six energy levels of the light source is illustrated in Figure 2.

Radiance calibration is conducted at each energy level, and finally, radiance sensitivity is fitted using Formula (1):

$$L\left(row,col\right)=R_1\left(row,col\right)\times D{N}^2(row,col)+R_2\left(row,col\right)\times DN(row,col)+R_3\left(row,col\right)$$

(1)

where row and col represent the pixel position, $L\left(row,col\right)$ represents the radiance of the pixel (μW/(cm²/sr/nm)), $R_n\left(row,col\right)$ (n = 1, 2, 3) represents the radiance responsivity of the pixel, and $DN(row,col)$ represents the signal counts after subtracting the dark background.

During calibration, 50 frames of signal and dark images are captured each time, from which the pure signal is obtained using Equation (2):

$$DN(row,col)=\frac{1}{50}{\displaystyle \sum _{j=1}^{50}D{N}_{sphere}(row,col,j)}-\frac{1}{50}{\displaystyle \sum _{j=1}^{50}D{N}_{dark}(row,col,j)}$$

(2)

where row and col represent the pixel position, j represents the number of captures, $D{N}_{sphere}$ represents the light signal, $D{N}_{\mathrm{dark}}$ represents the dark signal, and $DN(row,col)$ represents the signal counts after subtracting the dark background.

3.2. Calibration Setup

An integrating sphere serves as the standard radiance source, and a transfer radiometer is employed to measure the primary radiance source. A NIST standard diffuser is used for verification of the calibration results. The calibration vacuum tank comprises customized UV high transmittance glass. Standard light intensity measured using the radiometer is multiplied by the transmittance to ensure that the standard irradiance reaches the OMS-N. The calibration schematic is depicted in Figure 3.

• Five-dimensional rotating station

The target load is mounted on a five-dimensional rotating platform capable of translation in three directions and rotation along two axes [7]. This platform enables field scanning to calibrate each pixel on the image plane.

b.

Integrating Sphere

A 200 cm diameter integrating sphere with a 20 cm exit port diameter was utilized. Its inner surface was coated with polytetrafluoroethylene. Eight external 1000 W halogen tungsten lamps and two internal 300 W xenon lamps served as light sources within the sphere. A Labsphere interior integrated silicon unit detector (5-mm) was connected to a Labsphere electrostatic meter via a Bayonet Neill–Concelman interface to monitor sphere radiance stability. A 10-level adjustable diaphragm was positioned between each external tungsten lamp source and the sphere entrance. During calibration, the sphere was placed outside the thermal-vacuum chamber, fully illuminating the instrument entrance slit through a window.

c.

Transfer Radiometer

To calibrate the radiometric characterization of the calibration system, the intensity field of the integrating sphere was measured. The radiometer’s original calibration certificate was traceable to the National Institute of Metrology (NIM). We calibrated the radiometer using a blackbody, with a spectral radiance scale uncertainty of 2% (k = 2) for the radiometer. Similarly, as a monitoring standard, radiometer linearity was calibrated for radiometer traceability and corrected for radiometer linearity to prevent a confounding effect on the uncertainty of OMS-N radiometric measurements.

d.

Solar Simulator Standard System (SSSS)

Designed primarily to verify OMS-N’s radiance calibration results, this system consists of a solar simulator and a diffuser. The simulator employs reflective off-axis optical design and utilizes a 3000 W lamp as a source with superior UV intensity. The simulator’s spectral radiance scale uncertainties (k = 2) are 2.1%. A Labsphere diffuser was employed for spectral radiance standard transfer from the lamp to OMS-N and the radiometer via an apparatus. This apparatus, positioned at 700 mm from the diffuser’s center, held the simulator normal to the diffuser’s surface. Calibration coefficients were verified by comparing radiance values obtained from the radiometer with those calculated by OMS-N.

4. Results and Discussion

4.1. Radiance Calibration

The OMS-N boasts a 112° field of view, with each illumination covering only 2° of this expansive expanse. Consequently, the spatial dimension of the CCD encompasses just five rows at a time. By skillfully adjusting the turntable multiple times, we assemble a comprehensive focal plane signal map from data collected at various angles, as illustrated in Figure 4. The division shown in Figure 4a can be attributed to varying gain settings on either side of the detector. During the radiometric calibration process, the dark background is extracted, and the background noise and dark background gray scale changes are monitored to ensure the stability of the electronic performance during the calibration process. Figure 4 illustrates the trend of DN distribution when illuminated by uniform light. For DN, the optical system is inhomogeneous, that is, the transmittance of uniform light varies with the field of view angles, thereby necessitating full-pixel calibration using the calibration coefficients to convert each pixel to a specified brightness and then by calculating the spatial dimension uniformity.

Applying Equations (1) and (2), the signal maps acquired at the six energy levels were fitted to the radiance of the integrating sphere, a value derived through radiometer calibration. The objective was to calculate the fitting coefficients. The distribution of these coefficients is visually represented in Figure 5.

To validate the multi-energy level fitting coefficients, we employed data obtained from the Solar Simulator Standard System (SSSS). After subtracting the dark background from images captured with the SSSS and substituting the calibration coefficients, we calculated radiance values. We then compared these calculated values with standard values to ascertain the accuracy of the fitting results. Figure 6 shows the fitted residuals for one pixel. Figure 7 displays the radiance values of the OMS-N in the central field of view alongside the standard radiance values from the transfer radiometer. The discrepancy between these two sets of values is elucidated in Figure 8. For validation purposes, we selected various rows indicating different spatial dimensions. In the case of the UVIS channel, distinct colors represent different spatial rows—specifically, rows 15, 30, 45, 60, 75, 90, 105, 120, 135, 150, 165, …, 243. Rows 5, 8, 11, 14, 17, 20, 23, 26, 29, 32, 35, …, 61 were selected for UV1, considering the wavelengths from 270 to 300 nm. For the UV2 channel, rows 18, 33, 48, 63, 78, 93, 108, 123, 138, 153, 168, …, 239 were selected. Radiance comparison was not performed in the 250–270 nm range to prevent lower signal-to-noise ratios and increased errors. Considering xenon lamp characteristic peaks, the average deviation remained within 2.8% for VIS, 3% for UV1, and 2.2% for UV2. This deviation can be attributed to transmission errors encountered during the calibration process. As Figure 8a demonstrates, signal fluctuations were more pronounced in the shortwave UV, stemming from the lower signal-to-noise ratio in the UV1 band between 250 and 270 nm. Consequently, subsequent results in this text exclusively pertain to the 270–493 nm range.

4.2. Uncertainty of Radiance Calibration

The absolute accuracy of the OMS-N encompasses factors such as the black body traceability of transfer radiometers at the National Institute of Metrology (NIM), OMS-N instability, source non-uniformity, source exit angle uniformity, and source stability. The error can be calculated using Formula (3).

Table 2. Absolute accuracy of OMS-N.

Channel	Transfer Radiometer Uncertainty	Source Exit Angle Uniformity	Source Stability	Source Non-Uniformity	OMS-N Instability	Total Uncertainty
UV1 + UV2	2.1%	0.2%	0.1%@5 h	0.9%	0.42%	2.33%
VIS	1.33%	0.2%	0.1%@5 h	0.9%	0.47%	1.69%

summarizes these results.

$$\delta =\sqrt{x_1{}^2+x_2{}^2+x_3{}^2+x_4{}^2\cdots +x_n{}^2}\times 100\%$$

(3)

where $\delta$ denotes the total uncertainty, and x_n denotes the uncertainty of each sub-component.

Source non-uniformity is tested before calibration, with the integrating sphere aperture range for the meter-shaped point detection of light intensity and light intensity distribution of the non-uniformity of 0.9%. The method for calibrating OMS-N instability in Table 2 was used to extract multiple images to calculate the optical noise under the same light intensity condition, and the ratio of the noise to the average value of DN indicates the stability of the OMS-N, representing the absolute calibration accuracy.

Relative radiometric accuracy serves as an indicator of instrument response homogeneity. To assess the relative accuracy of the calibration, we utilized the calibration system to record the output at a given radiance input. For instance, for a specific spectral column, the relative uncertainty for all spatial data under uniform illumination can be expressed by Formula (4):

$${\delta }_{\lambda }=\frac{\sqrt{\frac{{\displaystyle \sum _{i=1}^n{\left(L_i-\overline{L}\right)}^2}}{n-1}}}{\overline{L}}\times 100\%$$

(4)

L_i represents the DN value of the the row, $\overline{L}$ represents the average value of all the rows, and δ_λ represents the spatial column response non-uniformity. The variation of relative accuracy with wavelength is depicted in Figure 9. The average relative uncertainties for UV and VIS are 1.84% and 1.45%, respectively.

5. Conclusions

The Ozone Monitor Suite-Nadir (OMS-N) is a crucial payload aboard the FY-3F satellite, primarily tasked with dynamically monitoring the total global ozone budget and the column density of various trace gases. It accomplishes this by detecting solar backscatter radiation within the ultraviolet and visible wavelength ranges. OMS-N operates through three spectral channels utilizing two CCDs: the UV1 channel spans wavelengths from 250 to 300 nm, the UV2 channel ranges from 300 to 320 nm, and the visible channel covers 310 to 495 nm. Additionally, OMS-N boasts an impressive 112° field of view. As highlighted earlier, the accuracy and stability of radiometric calibration play pivotal roles in achieving high-precision retrievals of global atmospheric concentrations. The successful launch of OMS-N on 3 August 2023, marked a significant milestone, and the pre-launch calibration results have been seamlessly integrated into the data preprocessing procedures. This paper has elucidated the pre-launch radiometric calibration method and radiometric model, both constructed through a multi-level approach to derive the necessary calibration coefficients. These calibration results underwent rigorous verification using the Solar Simulator Standard System. An in-depth analysis of the radiometric calibration’s uncertainty revealed that the absolute uncertainty for the UV calibration stood at 2.33%, while for VIS, it was 1.69%. Furthermore, the relative uncertainty for the UV was determined to be 1.84%, with the VIS exhibiting an uncertainty of 1.45%. The OMS-N pre-launch radiometric calibration is the working basis for evaluating the instrument’s post-launch radiometric performance. The relevant performance indicators will be analyzed and assessed through post-launch performance tests to obtain high-quality hyperspectral data and excellent radiometric performance, thus contributing to the advancement of quantitative meteorological applications.