Stratospheric Water Vapor from the Hunga Tonga–Hunga Ha’apai Volcanic Eruption Deduced from COSMIC-2 Radio Occultation

Abstract

The eruption of the Hunga Tonga–Hunga Ha’apai (HTHH) volcano on 15 January 2022 injected large amounts of water vapor (H₂O) directly into the stratosphere. While normal background levels of stratospheric H₂O are not detectable in radio occultation (RO) measurements, effects of the HTHH eruption are clearly observed as anomalous refractivity profiles from COSMIC-2, suggesting the possibility of detecting the HTHH H₂O signal. To separate temperature and H₂O effects on refractivity, we use co-located temperature observations from the Microwave Limb Sounder (MLS) to constrain a simplified H₂O retrieval. Our results show enhancements of H₂O up to ~2500–3500 ppmv in the stratosphere (~29–33 km) in the days following the HTHH eruption, with propagating patterns that follow the dispersing volcanic plume. The stratospheric H₂O profiles derived from RO are in reasonable agreement with limited radiosonde observations over Australia. The H₂O profiles during the first few days after the eruption show descent of the plume at a rate of ~−1 km/day, likely due to strong radiative cooling (~−10 K/day) induced by high H₂O concentrations; slower descent (~−200 m/day) is observed over the following week as the plume disperses. The total mass of H₂O injected by HTHH is estimated as 110 ± 14 Tg from measurements in the early plumes during 16–18 January, which equates to approximately 8% of the background global mass of stratospheric H₂O. These RO measurements provide novel quantification of the unprecedented H₂O amounts and the plume evolution during the first week after the HTHH eruption.

1. Introduction

The January 2022 volcanic eruption of Hunga Tonga–Hunga Ha’apai (~20°S, 175°W; hereafter HTHH) was the most explosive eruption in the satellite era, spanning the last four decades. Because HTHH was a submarine eruption, it injected large amounts of water vapor (H₂O) directly into the stratosphere. This behavior is distinctive from other large eruptions from land-locked volcanoes, such as El Chichon in 1982 and Pinatubo in 1991, where enhanced stratospheric H₂O was not observed. Measurements from operational radiosonde balloons over Australia in the first several days after the HTHH eruption show local H₂O mixing ratios greater than 1000 ppmv over altitudes ~25–30 km (the top of the balloon measurements) [1,2,3], compared with typical stratospheric background H₂O values of 5 ppmv. The isolated balloon measurements showed that the H₂O enhancements occurred in relatively thin ~1–2 km layers. Measurements from the Microwave Limb Sounder (MLS) instrument on the NASA Aura satellite show H₂O perturbations from HTHH that are unprecedented in the satellite data record, in terms of both altitude and magnitude [4]. The MLS data show enhanced H₂O at altitudes up to 53 km immediately after the eruption (15 January), and maxima between ~25–35 km during the following few days (16–18 January). Maximum H₂O values in these MLS retrievals, which represent vertical averages greater than ~3 km, were ~200–400 ppmv. However, the standard MLS retrievals are not designed for the anomalously high stratospheric H₂O values from HTHH, and many of these early retrievals did not pass the MLS quality screening criteria [4]. The HTHH plume traveled westward and dispersed in the stratosphere, and MLS data show that local H₂O maxima decreased to ~50 ppmv by early February and to ~10–20 ppmv by late March. The MLS retrievals are much better characterized by the lower H₂O values after late January. Anomalously high H₂O from HTHH persists in the stratosphere through boreal winter 2023 and has spread over much of the globe [3,5,6,7].

The objective of this paper is to quantify the extreme stratospheric H₂O amounts from HTHH in the first week after the eruption using COSMIC-2 (C2) GNSS radio occultation (RO) data. RO measures the bending angle of radio waves propagating through the atmosphere, which is closely related to atmospheric refractivity (N), which, in turn, is dependent on temperature and moisture. Under normal conditions, H₂O makes virtually no contribution to N in the stratosphere and, thus, is not detectable by RO measurements. However, effects from HTHH are clearly observed as anomalous stratospheric bending angle and N profiles from C2 that follow the HTHH plume during the first week after the eruption [3,8,9]. These anomalous N profiles can be associated potentially with both temperature and H₂O effects from the HTHH eruption, and we separate these influences by using independent temperatures from nearby MLS measurements. Reference [3] performed similar calculations using reanalysis temperatures to estimate extreme H₂O amounts in the 15 January HTHH eruption plume. Our analyses focus on the evolution of stratospheric H₂O during the first week after the eruption, as the RO measurements are sensitive only to extreme H₂O amounts. We include comparisons with the limited radiosonde H₂O measurements over Australia (up to ~30 km) to help evaluate the H₂O profiles derived from C2 measurements.

2. Materials and Methods

(a)

C2 GNSS-RO observations

By measuring the phase delay of radio waves from GNSS satellites as they slow and bend in Earth’s atmosphere, profiles of bending angles and refractivity can be obtained, e.g., [10]. At microwave frequencies in the troposphere and stratosphere, the refractivity varies due to contributions from the dry air and water vapor. Specifically, the atmospheric refractivity N can be related to atmospheric pressure P, temperature T, and water vapor partial pressure e [11]:

$$N=77.6\left(\frac{P}{T}\right)+3.73\times {10}^5\left(\frac{e}{T^2}\right)$$

(1)

In this study, we obtain level-2 C2 GNSS-RO profiles for January 2022 processed by the COSMIC Data Analysis and Archive Center (CDAAC) at the University Corporation for Atmospheric Research (UCAR). C2 is providing ~6000 profiles/day between ~40°N–40°S. We use the ‘atmPrf’ product, which provides refractivity and dry temperature (retrieved under the assumption of dry air) from near the surface up to ~60 km; data above 40 km are strongly influenced by climatology; thus, we focus on altitudes 25–35 km in this study. The profiles are quality controlled at CDACC by assigning ‘bad’ flags on the basis of several metrics, including deviation from the climatology by a specific threshold. A few C2 measurements intersect the early HTHH plume on 15 January and indicate large bending angle or N anomalies [3,6,8,9], but these are flagged as ‘bad’ retrievals, and most of our analyses focus on observations for 16 January and beyond (we also include a few profiles later in the day on 15 January that passed quality control). The effective vertical resolution of RO soundings varies from ~200 m in the lower troposphere to ~1–2 km in the upper stratosphere (more details below), while the horizontal footprint (horizontal scale represented by a single observation) is ~200 km, e.g., [10].

At CDAAC, the neutral atmosphere profiles are retrieved in the geometric optics approximation above 20 km. In this approximation, the resolution is physically limited by the 1st Fresnel zone $F_o$ [12], which depends on altitude and is approximately 1.4 km at 30 km. At CDAAC, data smoothing is performed using the Savitzky–Golay filter; details can be found in [13]. The half-width of the filter response function used for the standard processing is $1.1F_0$~1.5 km at 30 km. Specifically for this study, we investigated the possibility of modifying the filtering in order to better resolve those $N$ structures in the stratosphere induced by HTHH eruption. By modeling and testing we found that reducing the half-width of the response function by one-half, to 0.75 km at 30 km, results in improvement of the vertical resolution at 30 km, without substantial increase of the effect of observational noise (mainly from the ionosphere), while further reduction increases the noise and creates artifacts in the profiles. Thus, C2 RO data used in this study were processed with twice increased vertical resolution compared with the standard retrieval.

(b)

MLS temperatures

MLS temperatures are retrieved using measured limb emission from atmospheric oxygen (O₂), and these data are not strongly influenced by the HTHH eruption. We use MLS temperature profiles based on the v4.2 retrieval [14]. MLS provides global sampling with approximately 3500 measurements per day. Temperature retrievals are provided on standard pressure levels; data extend from 261 hPa (~9 km) to greater than 1 hPa (~48 km), with an effective vertical resolution of 3–4 km over the main region of interest here (~30 km).

(c)

Radiosondes

We include comparisons with radiosonde measurements of stratospheric H₂O during the first few days after the HTHH eruption. These data are discussed in detail in [2] and are based on measurements from the operational upper air network using the Vaisala RS41 radiosonde. These data can detect the large stratospheric H₂O from HTHH, and provide measurements up to ~30 km (depending on the balloon burst altitude of individual flights) with vertical resolution of ~100 m.

3. Results

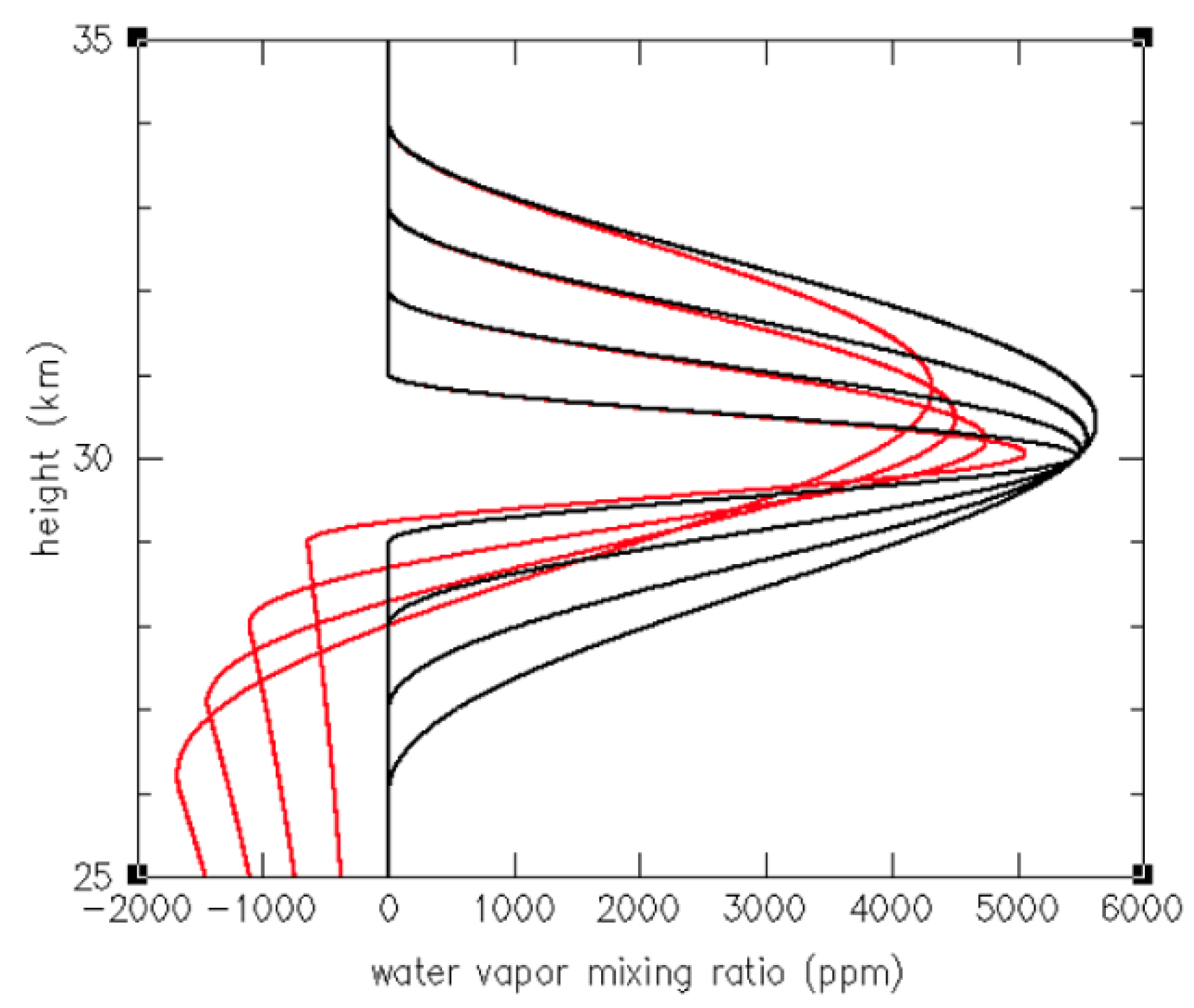
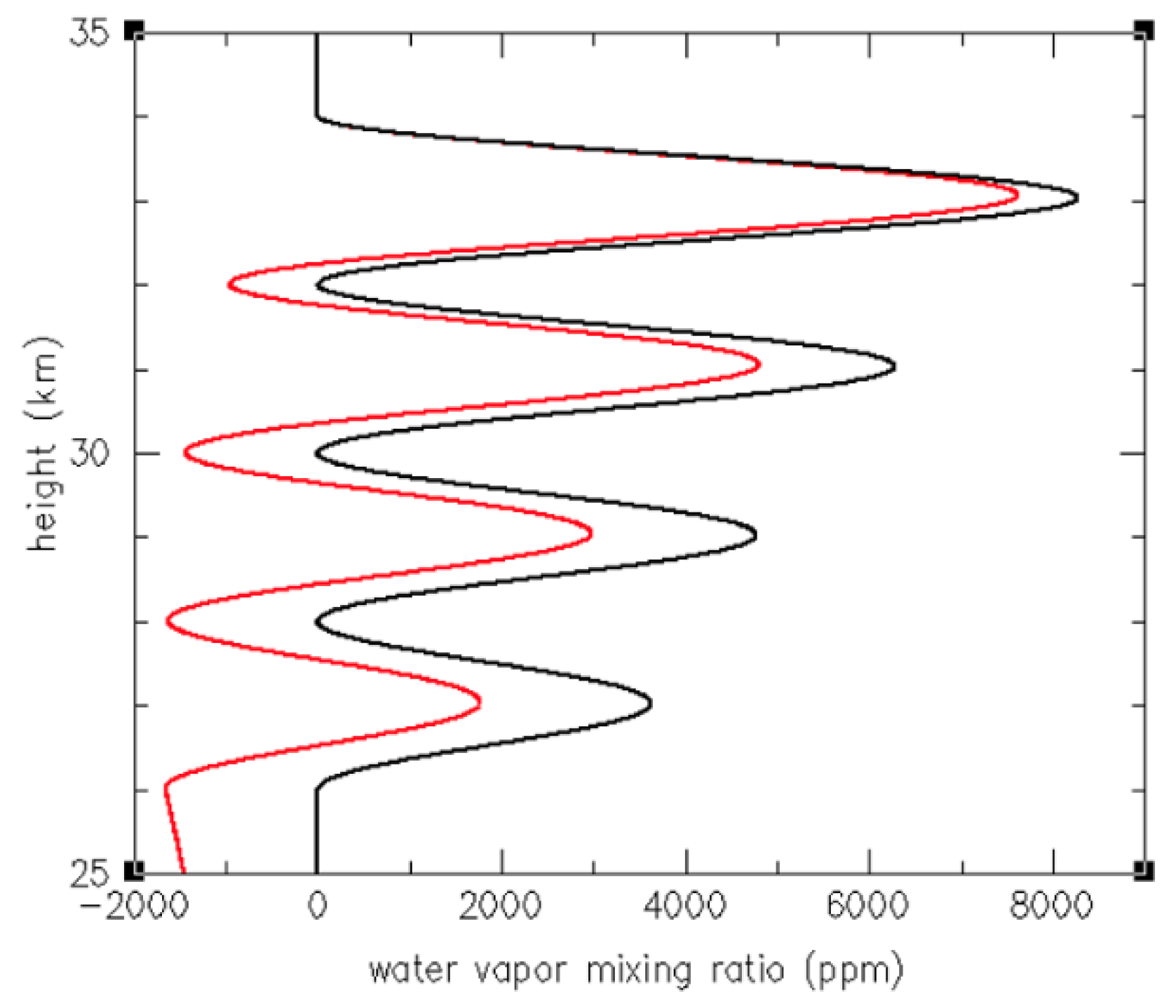
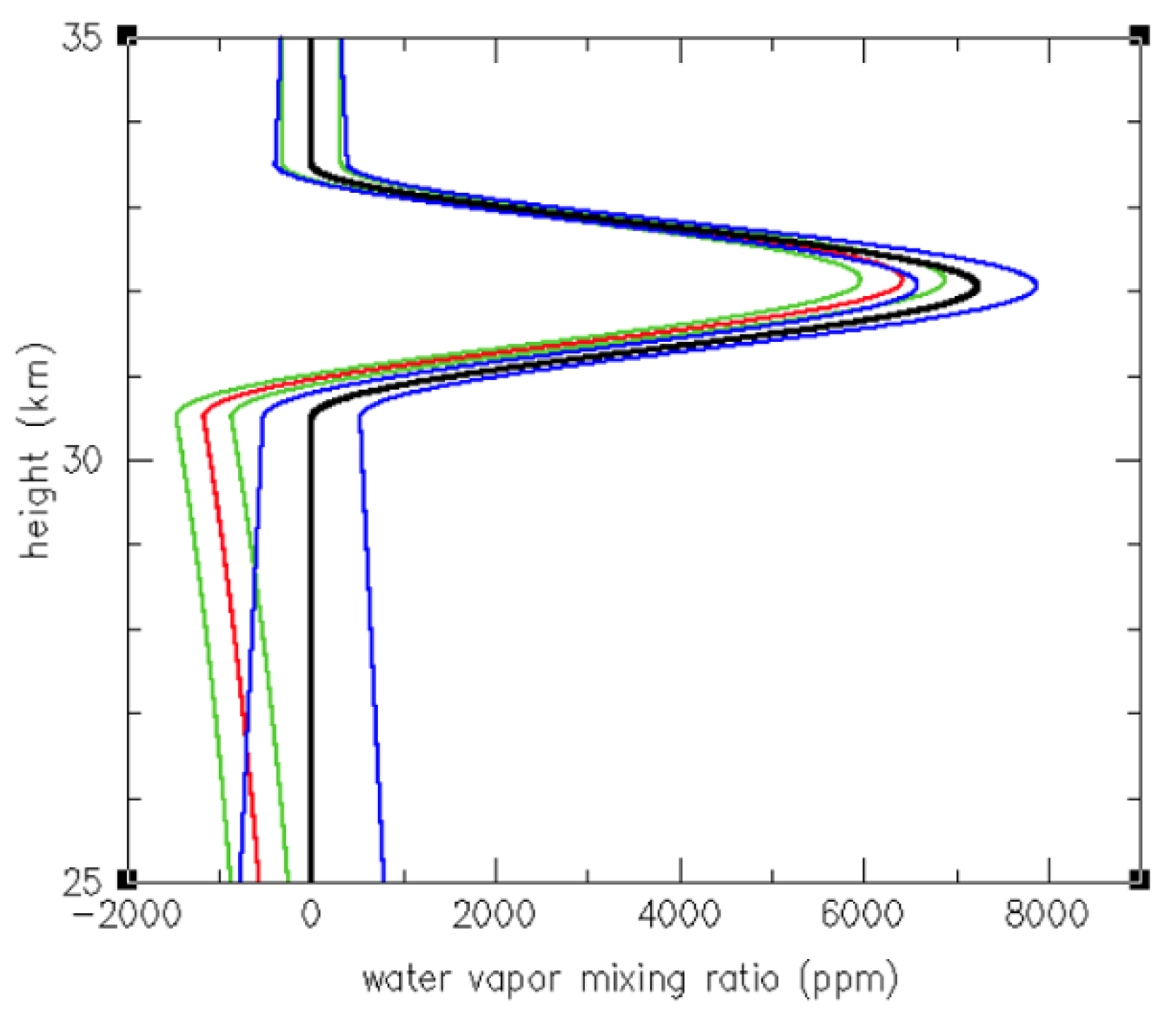
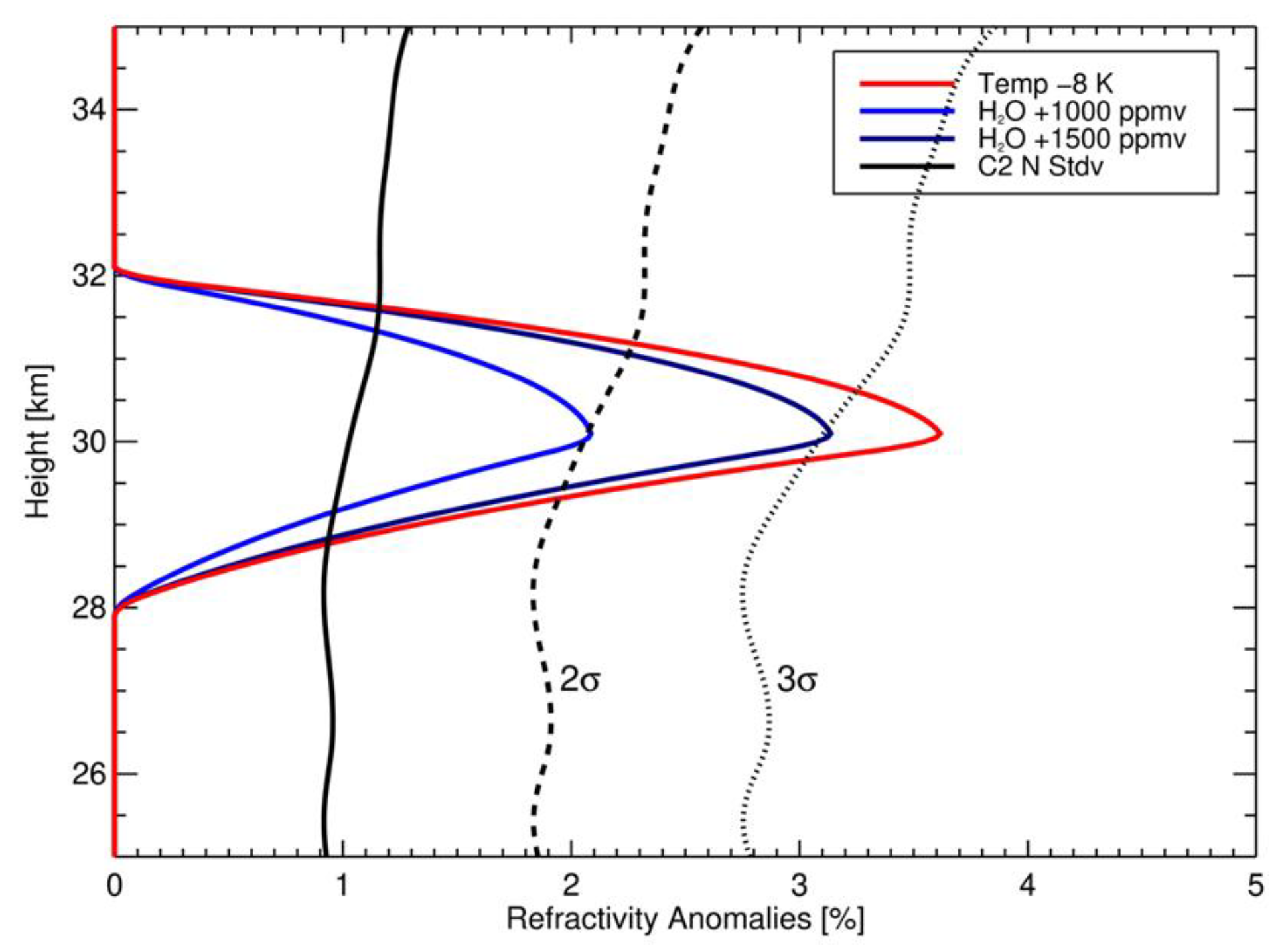
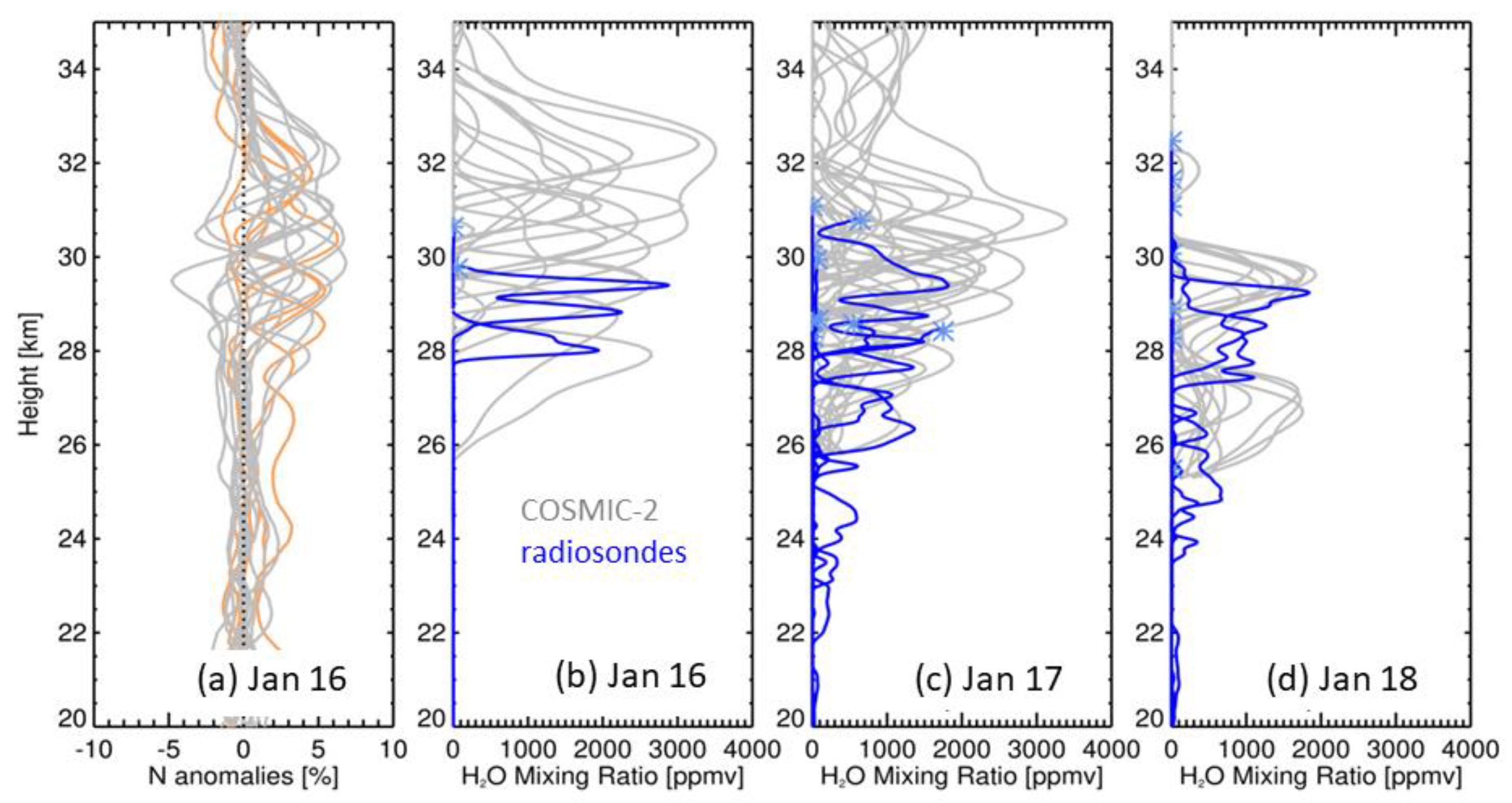
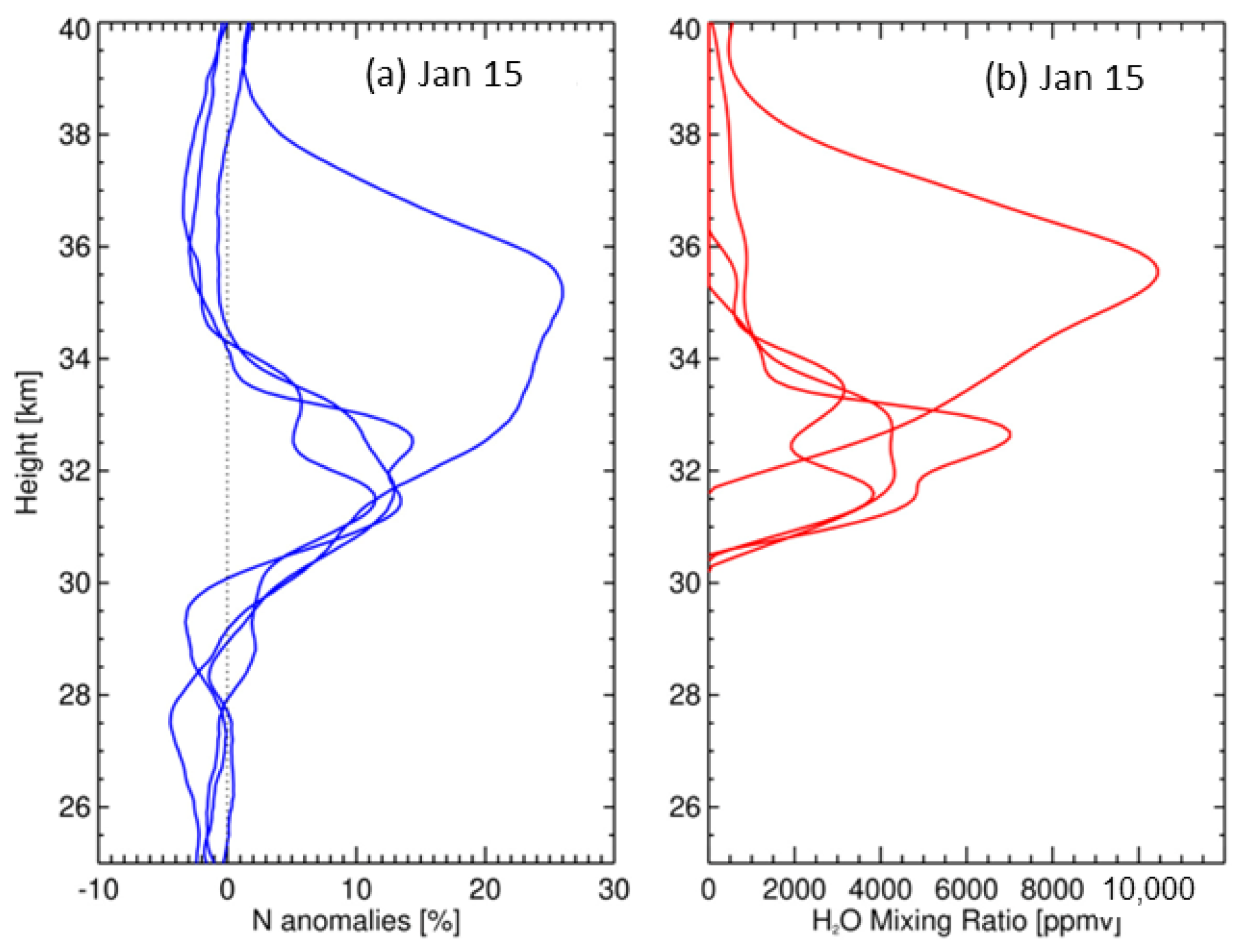
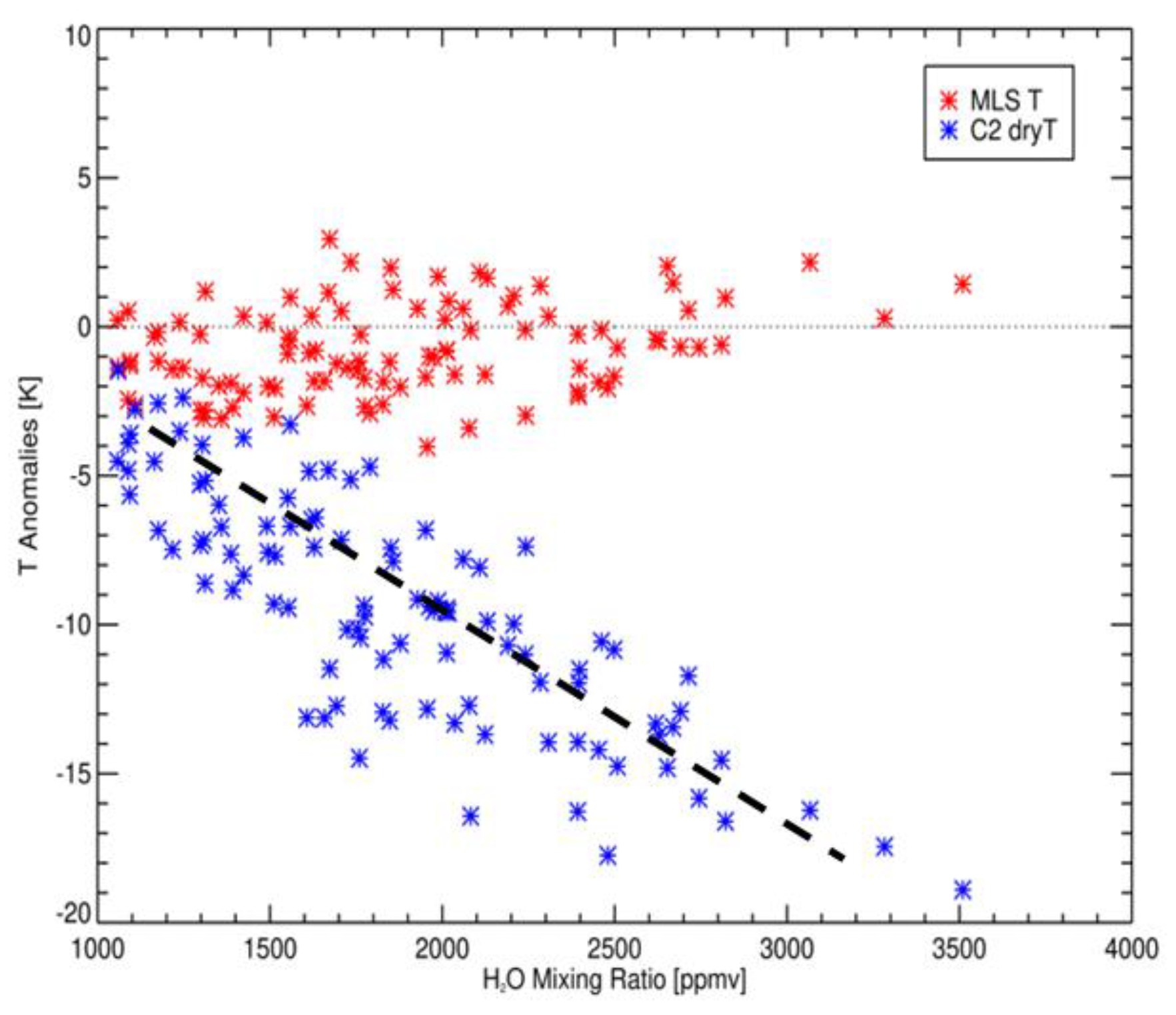
3.1. RO Sensitivity to Stratospheric H₂O

N decreases with altitude in the troposphere and stratosphere, and the H₂O term in (1) contributes little to N above the middle troposphere, where H₂O < 100 ppmv (e.g., [15]). Sensitivity tests based on (1) can determine the magnitude of GNSS-RO refractivity variations expected from isolated H₂O and temperature anomalies in the stratosphere. The sensitivity of N to isolated large stratospheric H₂O perturbations (1000 and 1500 ppmv anomalies at 30 km) is illustrated in Figure 1, showing positive N anomalies of ~2% and 3%, respectively, compared with an unperturbed background. While these are relatively small fractional N anomalies, they are not small compared with the observed N standard deviation in the stratosphere measured by C2, with typical values of 1% background levels (as shown in Figure 1; these were calculated from the pre-volcanic C2 measurements during 1–14 January in the vicinity of HTHH). Hence, an N anomaly due to a 1500 ppmv increase in H₂O is comparable to a (rare) positive 3-sigma C2 noise event. Figure 1 also shows the N anomaly associated with a local −8 K temperature perturbation at 30 km, which is similar (~3.5%) to the effect of 1500 ppmv H₂O. These calculations suggest that C2 RO measurements may be able to detect a large stratospheric H₂O perturbation on the order of 1000 ppmv or larger but that similar N signals can arise from large negative temperature anomalies.

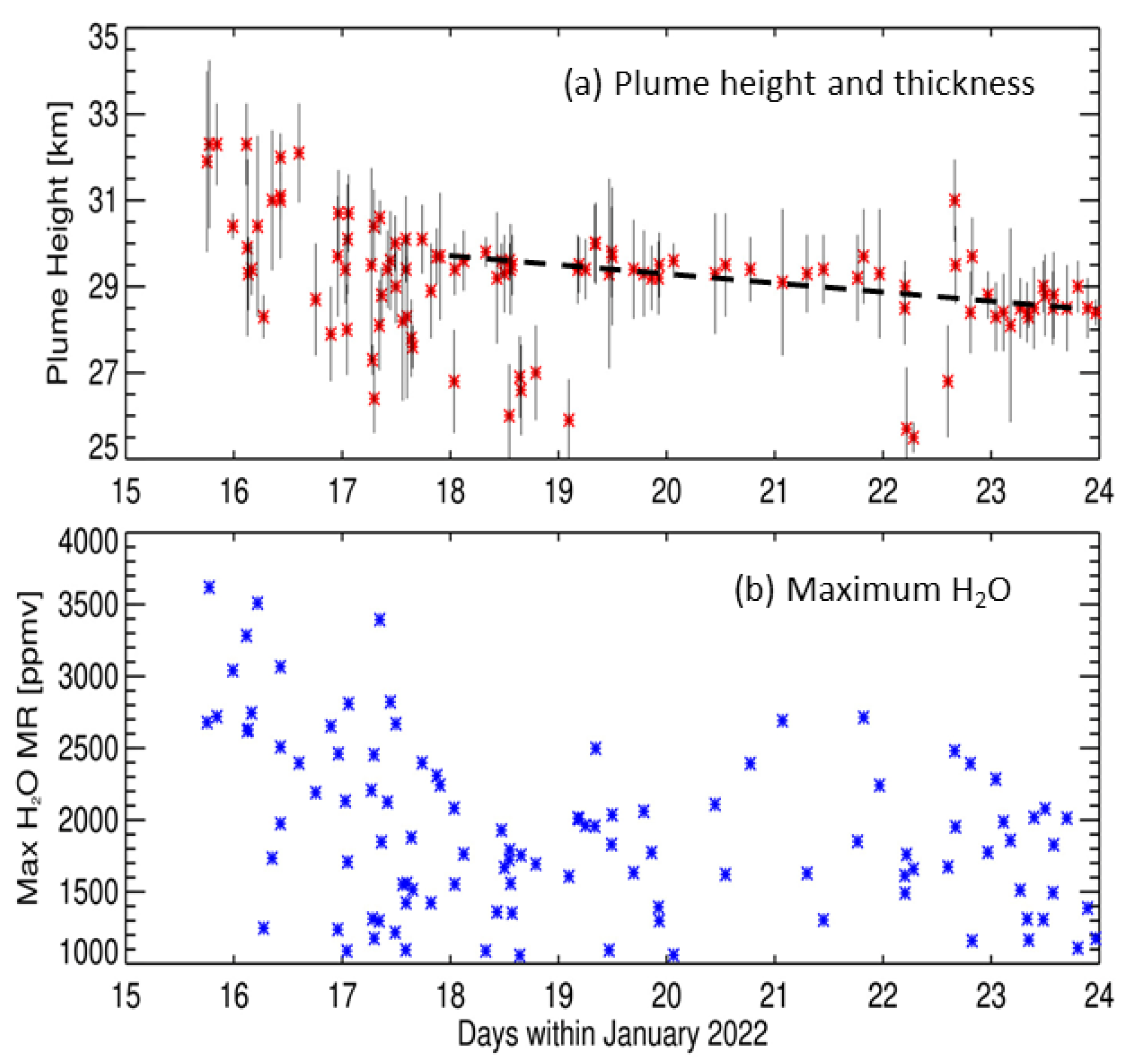
3.2. C2 HTHH Refractivity Observations and H₂O Retrievals

On the basis of the sensitivity calculations shown in Figure 1, our analyses search for positive N anomalies in C2 data that are larger than 3-sigma. Anomalies are calculated as percentage differences from the pre-volcanic background 1–14 January average. Figure 2 shows the C2 daily sampling density in the region of the HTHH eruption on 16–18 January (grey dots) along with the locations of local N anomalies with maximum values greater than 3-sigma over altitudes 25–35 km (colored symbols). The stratospheric plume from HTHH moved westward in the background stratospheric easterly winds (e.g., [1,2,3,4]), and the positive N anomalies >3-sigma in Figure 2 track the plume movement downstream from the 15 January eruption. The altitudes of the N anomaly maxima for these days generally range from 27 to 33 km. For comparison, Figure 2 includes corresponding maps of sulfur dioxide (SO₂) in the HTHH plume, as observed by satellite [16], showing westward movement and dispersion of the plume similar to that inferred from the C2 N anomalies.

Examples of the vertical profiles of N anomalies for the cases identified on 16 January (Figure 2a) are shown in Figure 3a. As selected, each of the profiles has a maximum N anomaly greater than 3%, with several local maxima greater than 6%, and the observed profiles peak over altitudes ~29–33 km. The vertical thickness of the N anomaly profiles is, on average, ~2 km.

Details of retrieving H₂O from C2 N measurements combined with ancillary temperature profiles from MLS are described in the Appendix A. Our results use the local retrieval based on Equation (1), incorporating C2 N observations with the nearest co-located MLS temperature profile (within 600 km and 6 h), and we use dry pressure (P_dry) from the C2 atmPrf files. We then solve Equation (1) for water vapor pressure e and convert to H₂O mixing ratio. As discussed in the Appendix A, this local retrieval will underestimate H₂O amounts compared with a more accurate non-local retrieval, but the underestimate is small (~10%) for narrow ~2 km thick H₂O layers, as is typical for HTHH. Our local H₂O retrievals also truncate at the bottom of the layer where the retrieved H₂O becomes negative, which results in a slight underestimation of the column amount. There are somewhat larger errors associated with multiple H₂O layers (Figure A2), and we simply omit a handful of profiles that exhibit multiple layers (mostly on 16 January, indicated by the orange curves in Figure 3a).

Figure 3b shows the derived H₂O profiles on 16 January, with maximum H₂O values of ~2500–3500 ppmv over altitudes ~29–33 km; the altitude maxima for H₂O approximately match the N anomaly maxima in Figure 3a. We note that these large H₂O amounts are below ice saturation with respect to the background stratospheric temperatures that increase with height (e.g., [1,2,3]). Figure 3c,d show derived H₂O profile results for the following days, showing a decrease in the maximum H₂O amounts to ~2000–3000 ppmv on 17 January and ~1800 ppmv on 18 January. The measured altitudes of the H₂O maxima also systematically decrease between 16–18 January.

While the HTHH plume was over Australia during 16–18 January, enhanced stratospheric H₂O was observed by operational radiosonde balloons [1,2,3]. H₂O profiles from these radiosonde data are shown in Figure 3 overlain on the RO retrievals. Note that the top of the radiosonde measurements is near 30 km (depending on the individual balloon flights), so that direct comparisons with RO calculations focus on altitudes below 30 km. In addition, the radiosonde profiles are not explicitly co-located with the C2 profiles but are intended to be representative of approximate H₂O magnitudes and altitudes in this region. The radiosonde-C2 comparisons in Figure 3b–d show reasonable agreement over ~26–30 km in terms of H₂O magnitude during these days, e.g., ~2000–3000 ppmv on 16 January. This agreement enhances confidence in the C2 H₂O retrievals, at least for the extreme maxima immediately following the eruption.

We also investigated several C2 measurements that intersected the HTHH plume early after the eruption on 15 January, and the associated N anomalies and retrieved H₂O amounts are shown in Figure 4. The results show large positive N anomalies (~10–25%) and extreme H₂O values (~4000–10,000 ppmv) over altitudes 30–40 km. However, these retrieved N profiles are mostly classified as ‘bad’, and the reason is not simply understood because there are numerous potential causes. While we are less confident in these retrievals, the vertical structure is reasonable, and the large H₂O values in Figure 4 are still below ice saturation and, thus, may represent actual geophysical structure, as noted in [3].

Analyses of the MLS temperatures show that there are not systematic temperature anomalies associated with the identified C2 N anomalies for the volcanic plume events (Figure 5), so that the stratospheric RO N anomalies arise primarily from enhanced H₂O. This statement is qualified by the lower vertical resolution of MLS temperatures (~4 km) compared with C2 retrievals (~1–2 km), in addition to imprecise co-locations of the MLS and C2 data. In contrast to the lack of temperature anomalies in the MLS data, temperatures derived from the C2 dry retrieval (atmPrf, neglecting the H₂O term in Equation (1)) show negative temperature anomalies up to −20 K (Figure 5). These are likely spurious results related to the large positive N anomalies when not accounting for H₂O effects. As a note, we have also examined co-located temperatures from ERA5 reanalysis [17] and find small systematic temperature anomalies for the co-locations, similar to the MLS results in Figure 5.

Regular westward propagation of the HTHH H₂O plume is detected by RO measurements over the first week after the eruption, as shown in Figure 6, selected for profiles with derived H₂O values > 1000 ppmv over 25–35 km. This behavior is in good agreement with the MLS H₂O retrievals shown in [4], which also demonstrated consistency with the background stratospheric easterly winds. Figure 6 also shows that while the enhanced H₂O values from HTHH clearly stand out, there are numerous additional events that are not coincident with the plume, and these likely represent noise in the C2 N profiles.

For the H₂O profiles identified in the HTHH plume (i.e., following the locus of westward propagating points in Figure 6), Figure 7 shows the corresponding derived plume height and thickness and the corresponding maximum H₂O values. Plume thickness is estimated as the altitudes over which H₂O exceeds 25% of the maximum value in each respective profile. Results show highest H₂O concentrations (up to ~3500 ppmv) and highest altitudes (~29–33 km) immediately after the eruption on 16 January. Maximum H₂O concentrations decrease, and the plume heights descend by ~2 km over the first two days, as seen in Figure 3. Maximum H₂O values are ~1000–2500 ppmv after 18 January, and some isolated larger H₂O maxima over 20–22 January could possibly be related to localized plume maxima identified in [6]. The general decreases in H₂O concentration and altitude are consistent with observations from radiosonde data [2,3] and MLS [4,7] following the eruption. The descent rate for the period 18–23 January in Figure 7a is approximately 200 m/day, which is consistent with values estimated from radiosondes over the first zonal circumnavigation of the plume [3]. We find less coherent variability in derived C2 H₂O amounts after ~24 January. Given the RO H₂O sensitivity inferred from Figure 1, together with observed C2 noise levels, we expect that C2 will lose sensitivity to stratospheric H₂O amounts below ~1000 ppmv (which corresponds to positive N anomalies ~2-sigma).

3.3. Mass of H₂O in the Early HTHH Plume

We calculate the mass of H₂O in the early HTHH plumes during 16–18 January by estimating the approximate area of the plumes and then integrating the derived H₂O profiles for all of the RO measurements within the plume areas. The estimated plume areas are shown in Figure 2, defined simply as latitude–longitude boxes containing enhanced N anomalies for each day. These boxes agree well with the approximate plume areas defined by maximum SO₂ amounts (Figure 2d–f), and the box on 18 January is selected with this agreement in mind. We calculate the vertically integrated H₂O (over 25–35 km) using all of the profiles within the boxes (not just the extreme N > 3-sigma profiles), and this accounts for heterogeneity of the plume structure. Multiplying the plume areas by the integrated H₂O profile amounts determines the H₂O mass within the plume volumes. Results of these calculations return values of 98 ± 11, 103 ± 14 and 102 ± 14 Tg (2-sigma uncertainties) on the three days of 16–18 January. Uncertainties in these estimates are derived by repeating the calculations numerous times but including random Gaussian-distributed ±1% variations to the individual N and T profiles, consistent with background noise in the C2 N measurements and uncertainties in the ancillary MLS temperatures. We note that the estimates for these three days are remarkably consistent (~100 Tg), given the evolving horizontal (Figure 2) and vertical (Figure 3) structure of the plumes over these days. Including the systematic underestimation of H₂O amounts by the local retrieval by ~10% provides an average value with uncertainties of 110 ± 14 Tg for the total mass, which equates to approximately 8% of the background mass of stratospheric water vapor. This value is consistent with the MLS v5 results [3] and somewhat less than those of MLS v4 [4]. Reference [3] estimated the H₂O mass from two individual RO measurements within the HTHH eruption umbrella on 15 January, giving a broad range of 70–150 Tg, which overlaps our results for 16–18 January.

4. Discussion

C2 data demonstrate that RO measurements are sensitive to the extremely large stratospheric H₂O perturbations during the first week following the HTHH eruption. This is tied to the large observed refractivity anomalies associated with the HTHH plume, combined with the low stratospheric background noise in the C2 measurements (with standard deviations of ~1% background values). Sensitivity calculations (Figure 1) suggest that stratospheric H₂O anomalies of ~1000–1500 ppmv or larger should be detectable in C2 refractivity measurements, equivalent to 2-sigma and 3-sigma noise levels, respectively. N anomalies for 1500 ppmv have similar magnitude to a localized −8 K temperature perturbation.

To separate H₂O and temperature effects on N, we used independent temperature observations from the MLS satellite. This has some limitations in terms of different vertical sensitivities and imperfect co-locations between C2 and MLS measurements but is a necessary step to separate H₂O and temperature influences on N. Comparison of MLS temperature anomalies vs. derived H₂O amount (Figure 5) suggests small systematic temperature anomalies for the H₂O plume during the first week after the eruption so that the C2 N anomalies are due primarily to H₂O influence. In contrast, C2 dry temperature retrievals (atmPrf) for the anomalous HTHH profiles analyzed here show large negative temperature anomalies (up to −20 K in Figure 5), which are believed to represent mainly the response of dry temperature to H₂O. We note that [2] identified small systematic temperature anomalies (~−2K) associated with enhanced H₂O in the radiosonde measurements after 29 January, possibly linked with radiative cooling from enhanced H₂O [1], but such patterns are not detected in MLS results (or ERA5 reanalysis) during the first week after the eruption. Larger systematic cold temperature anomalies (zonal means greater than 5 K) are observed in the months following HTHH, likely as a radiative and dynamical response to the anomalous H₂O and aerosols [18].

Our simple H₂O retrieval shows maximum values of ~2500–3500 ppmv during the first few days following the eruption, over altitudes ~29–33 km. The H₂O magnitudes from the C2 retrieval are in reasonable agreement with radiosonde measurements over Australia, which cover altitudes up to ~30 km. MLS H₂O retrievals show smaller maximum values (~200–400 ppmv) over these days, but the MLS vertical sensitivity represents broader vertical layers (~3–4 km thick) that can underestimate the peak concentration and overestimate the vertical extent for narrow layers. C2 measurements show a relatively rapid descent (~−1 km/day) of the plume over the first two days when H₂O concentrations are highest, and slower descent (~200 m/day) during the following week. Radiative cooling from the early H₂O enhancements [1] produces values of ~−10 K/day, which equate to ~−30 K/day in potential temperature or ~−1 km/day over altitudes near 30 km, which is approximately consistent with the C2 observations. Cooling and descent rates drop rapidly with H₂O dilution, as observed during the following week.

Combining the horizontal extent of the H₂O plumes observed by RO with the derived H₂O profiles provides a total mass of ~110 ± 14 Tg for the early plumes during 16–18 January. This estimate from RO data is in approximate agreement with results using MLS v5 retrievals of 119 ± 6 Tg [3], while MLS v4 data provide higher values of 137–146 ± 8 Tg [3,4,5]. Total HTHH H₂O mass estimates from radiosondes [2] provide values of 50–93 Tg but are limited by the vertical extent of the balloon measurements (~30 km). We note that while there are no observations of an increase in stratospheric H₂O after the Pinatubo eruption in 1991, reference [19] calculated 37.5 Tg injection of water vapor to the stratosphere in an idealized model. Combining the H₂O sensitivity of RO with C2 background noise levels suggests that the C2 measurements are less sensitive to H₂O amounts below ~1000 ppmv (equivalent to 2-sigma noise levels) so that C2 measurements are most useful for the large H₂O amounts in the first week after HTHH. Both radiosonde [2] and MLS data [3,4] show that the HTHH plume disperses over time, with H₂O values <100 ppmv several weeks after the eruption. These smaller values, while highly anomalous and long-lasting in the stratosphere, are not retrievable from RO measurements.