Combining an Eddy Detection Algorithm with In-Situ Measurements to Study North Brazil Current Rings
Abstract
1. Introduction
2. Materials and Methods
2.1. The EURECA-OA Cross-Calibrated Measurements
2.2. The Eddy Detection and Tracking Algorithm TOEddies
3. Results and Discussion
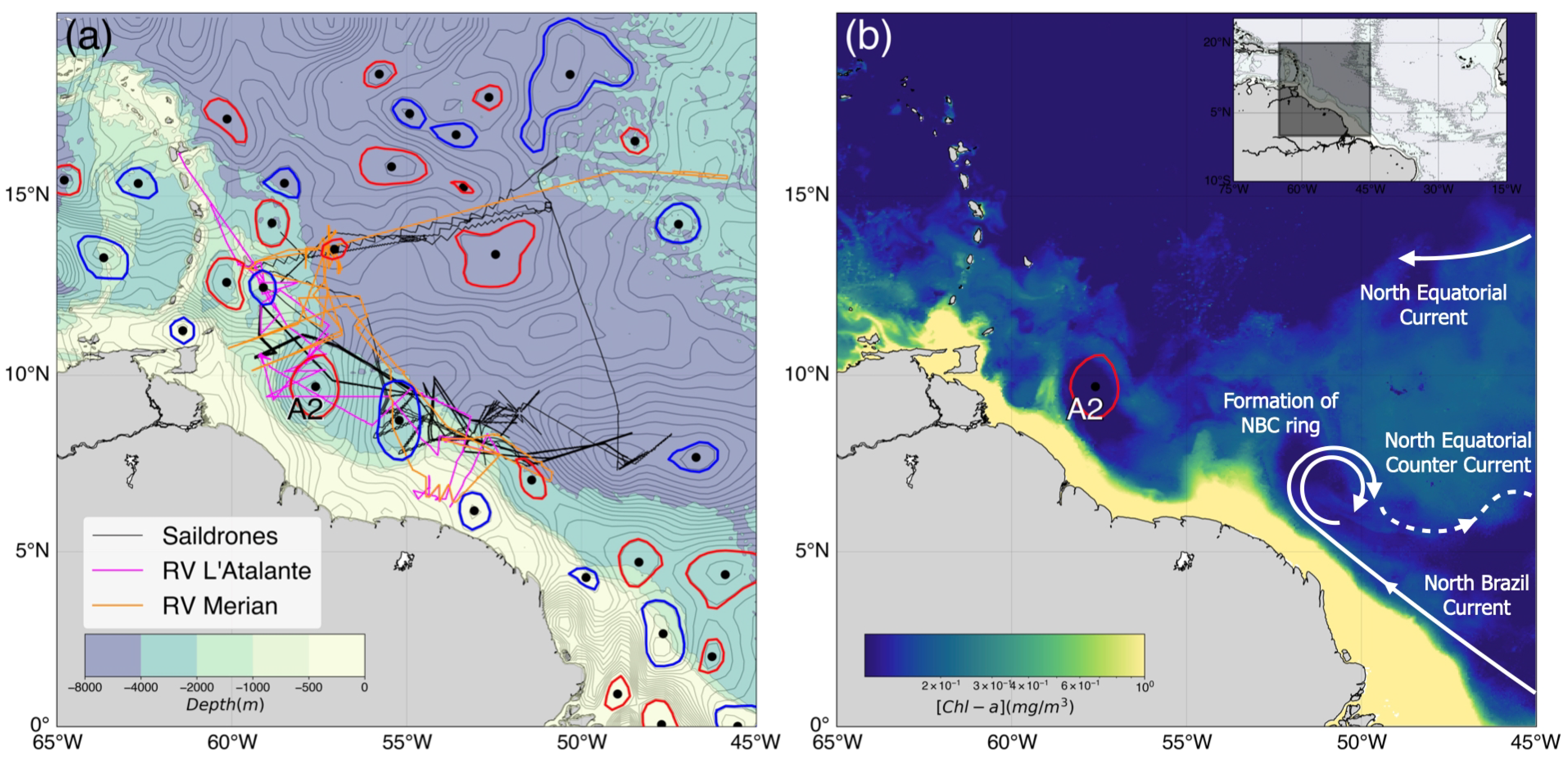
3.1. In-Situ and Satellite Observations of a North Brazil Current Ring
3.1.1. The A2 NBC Ring as Defined from Satellite Altimetry
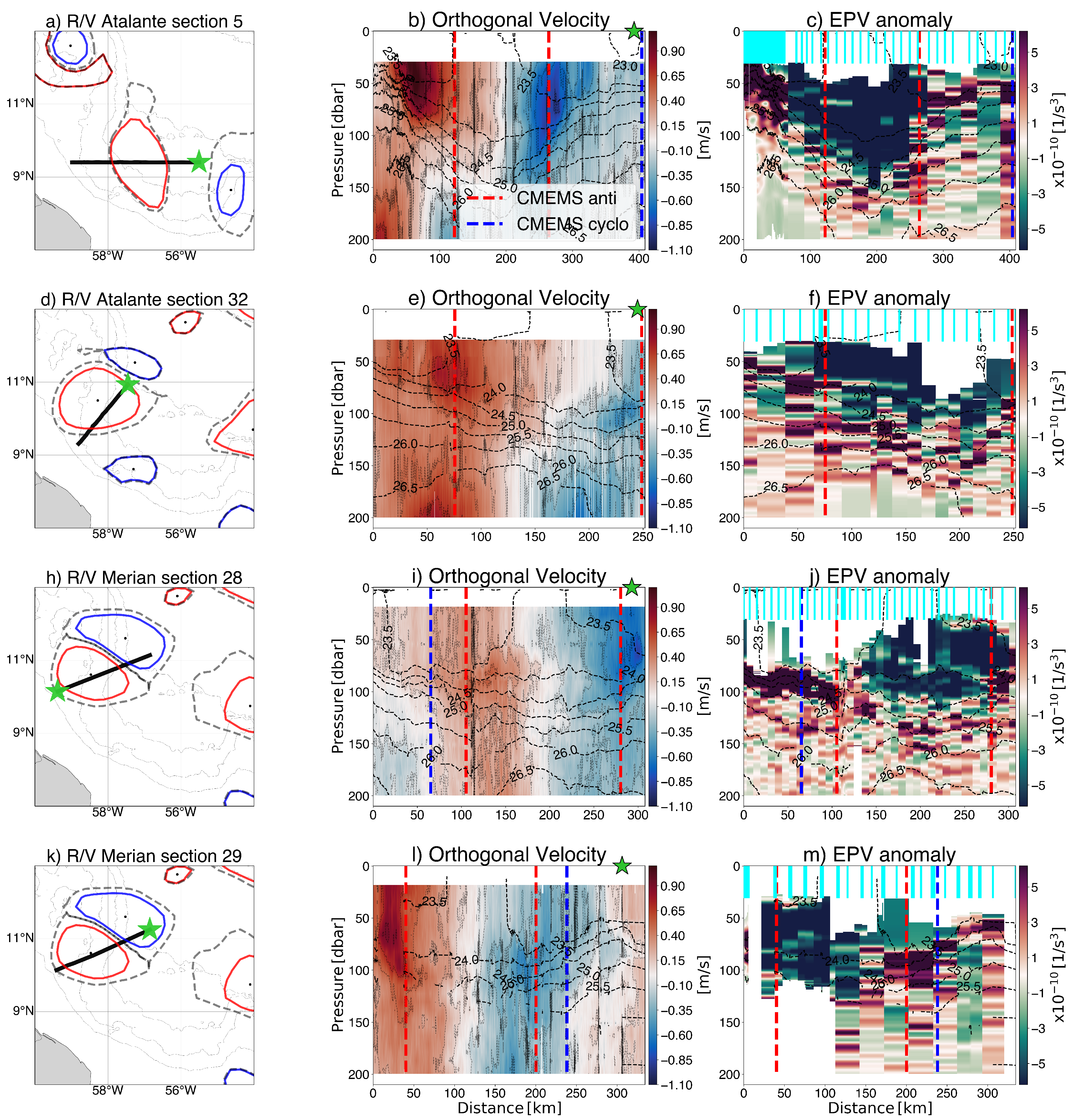
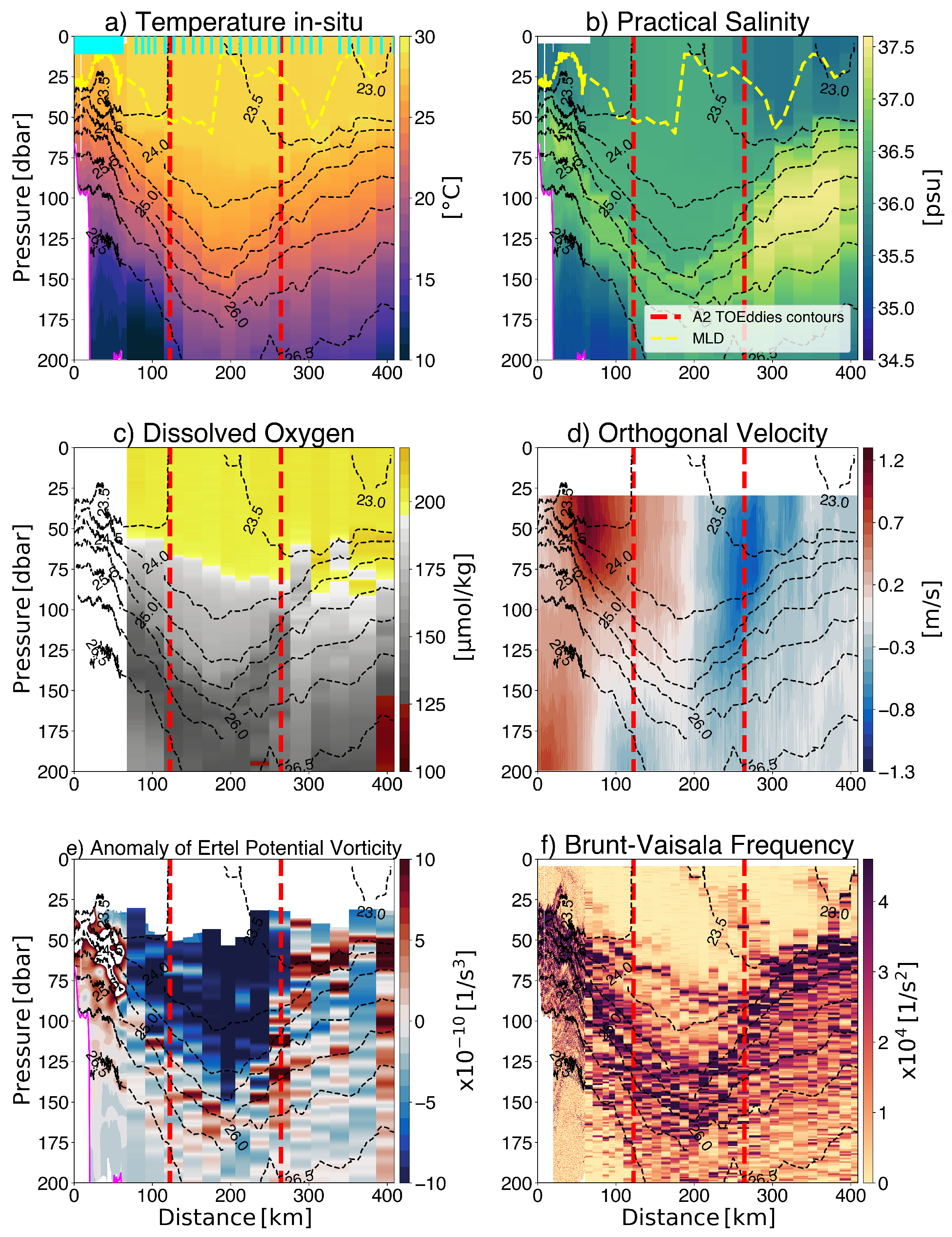
3.1.2. The A2 NBC Ring In-Situ Observed Characteristics
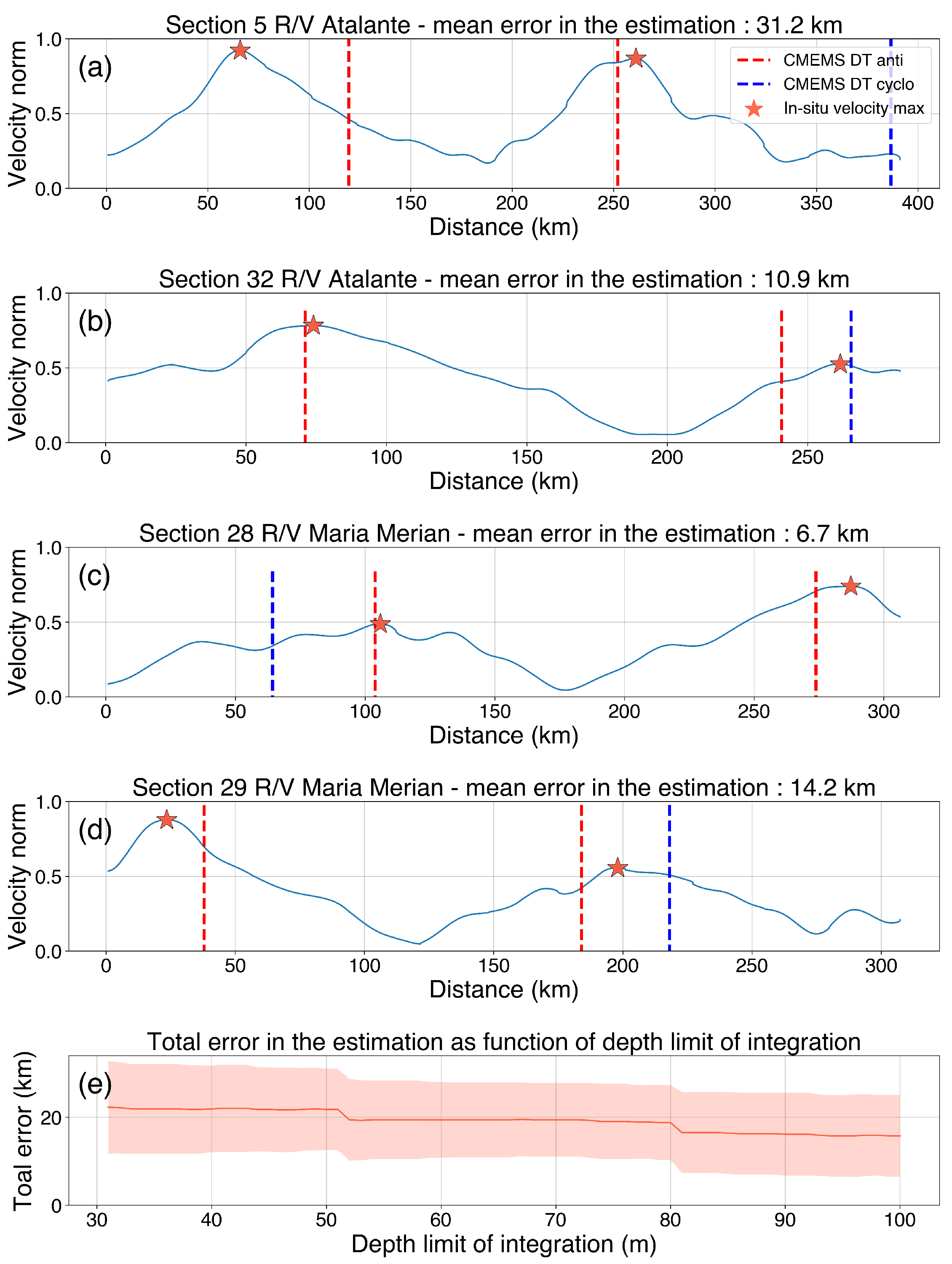
3.1.3. Computation of TOEddies Error in Estimating the Ring Center
3.1.4. Computation of TOEddies Error in Estimating the Contours of Maximum Velocity
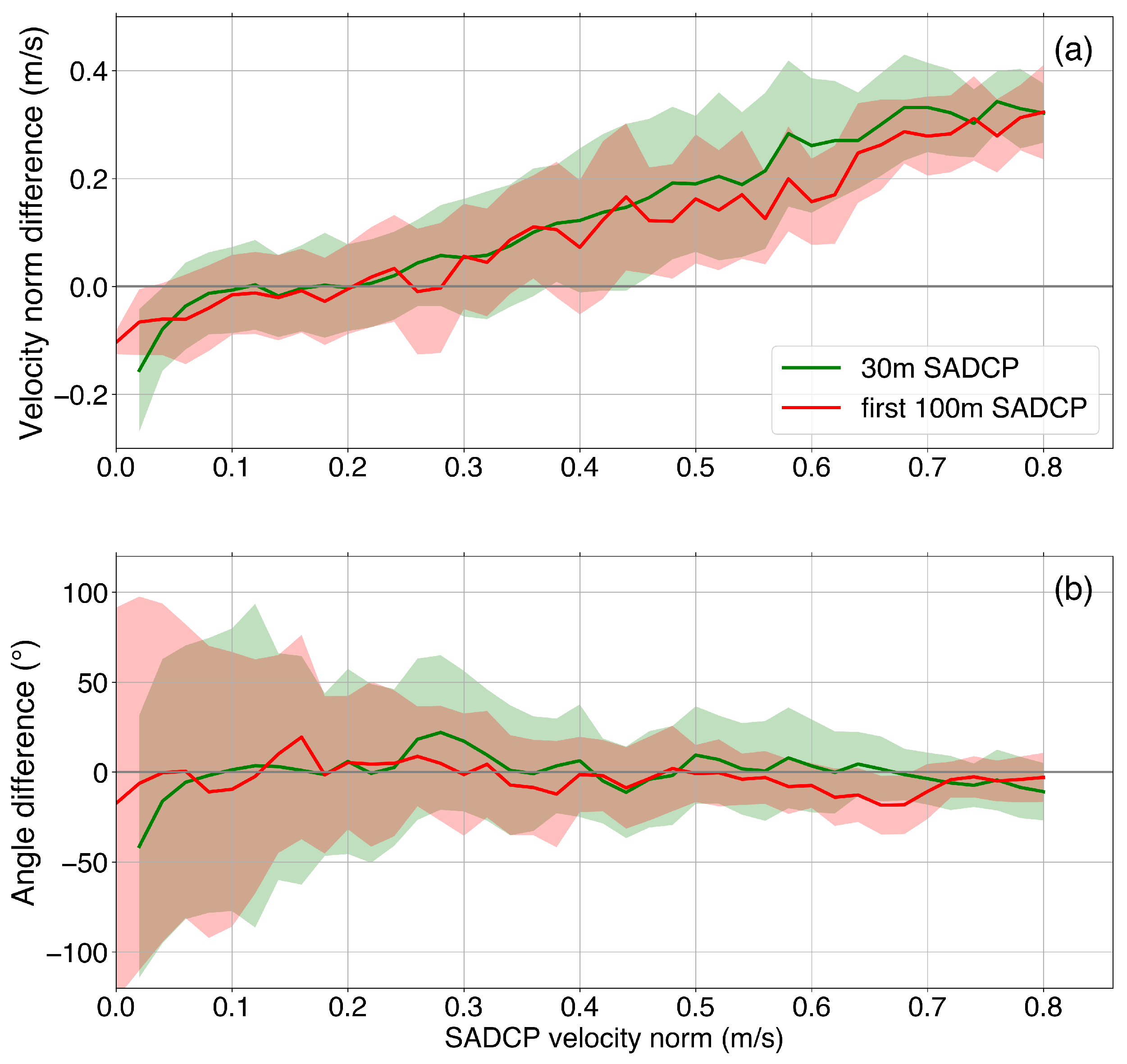
3.1.5. Comparison between In-Situ and Satellite-Derived Surface Geostrophic Velocities
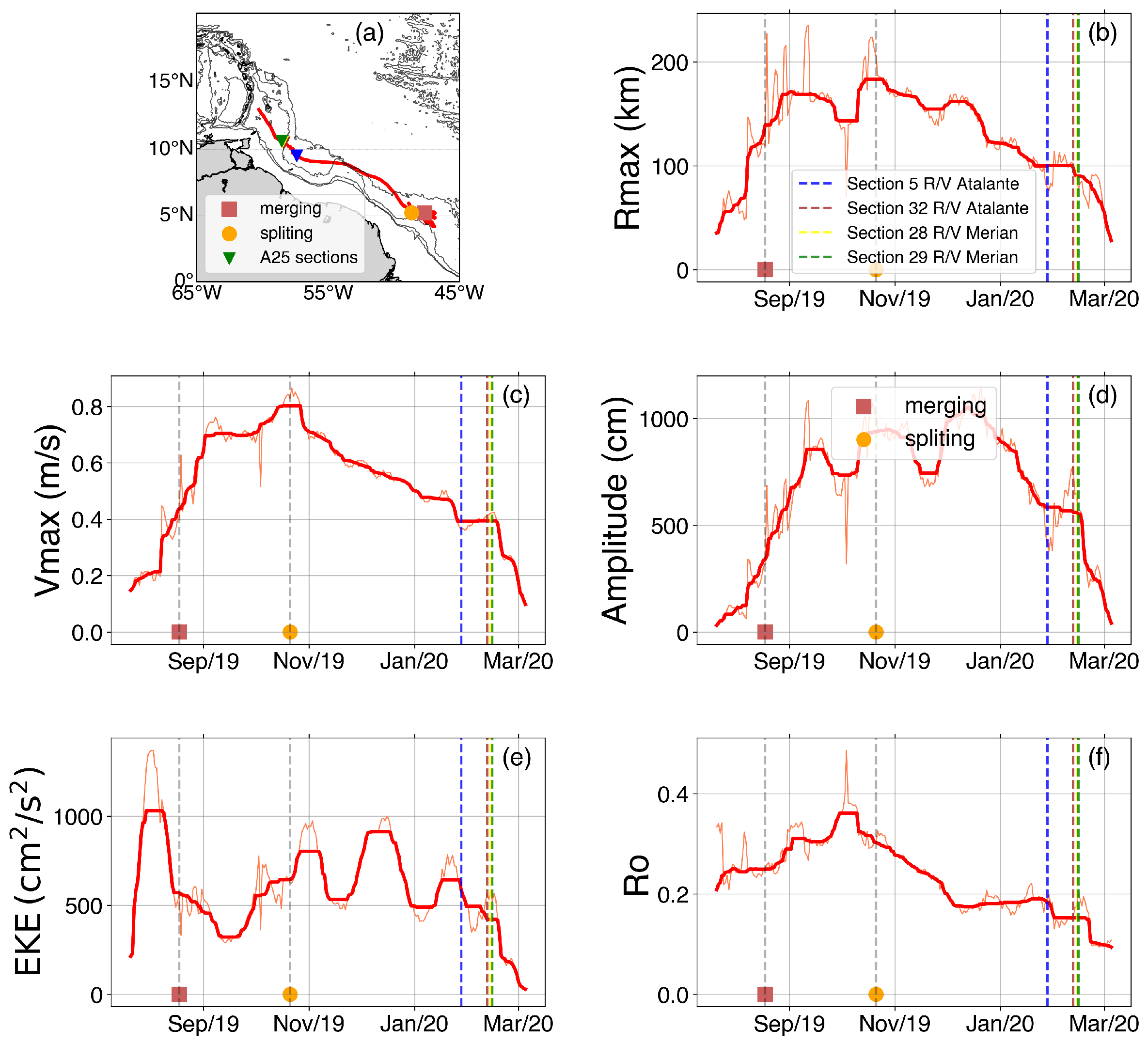
3.2. Spatio-Temporal Variability of NBC Rings Parameters as Derived from TOEddies
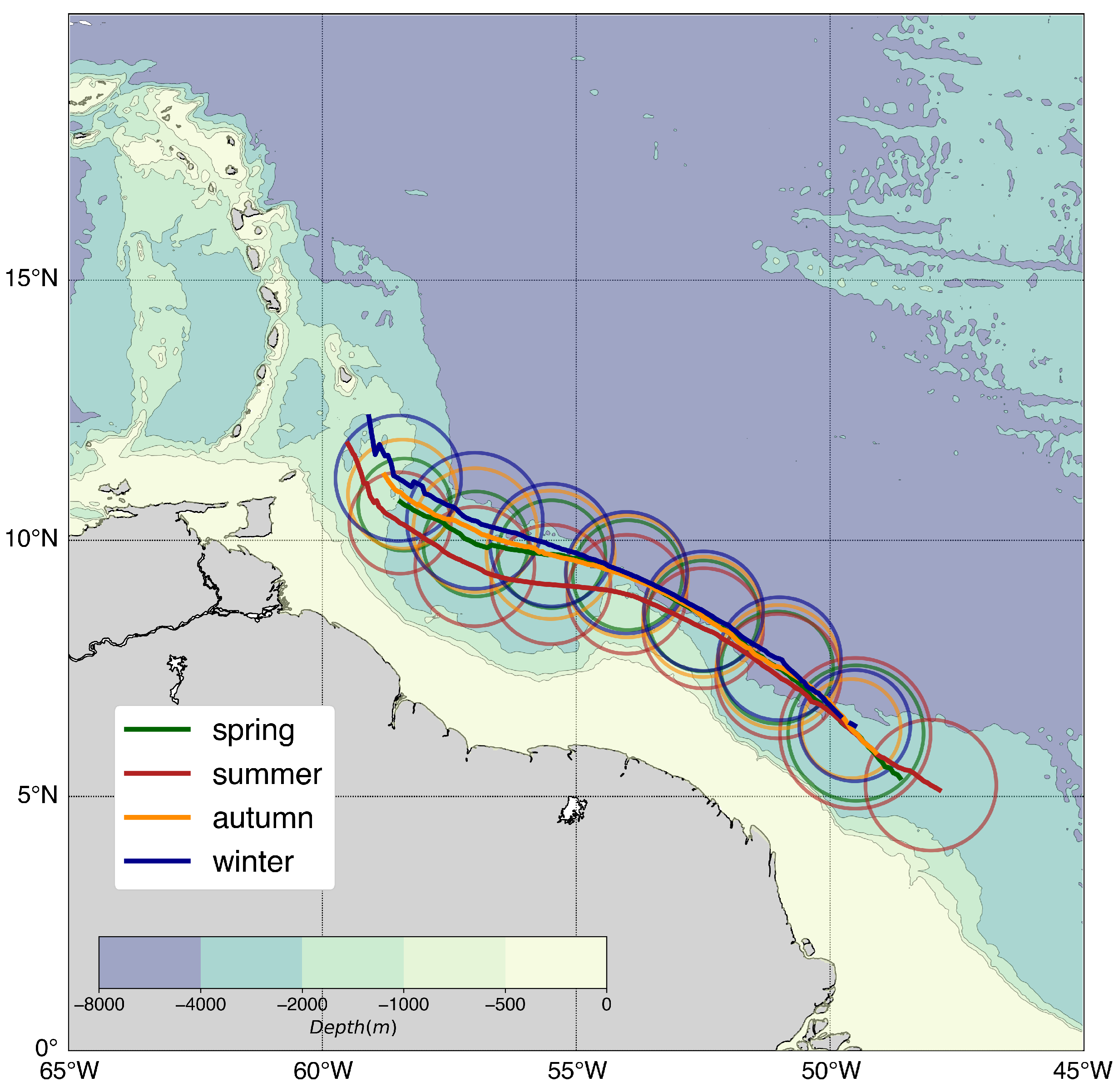
3.2.1. Variability in NBC Rings Generation
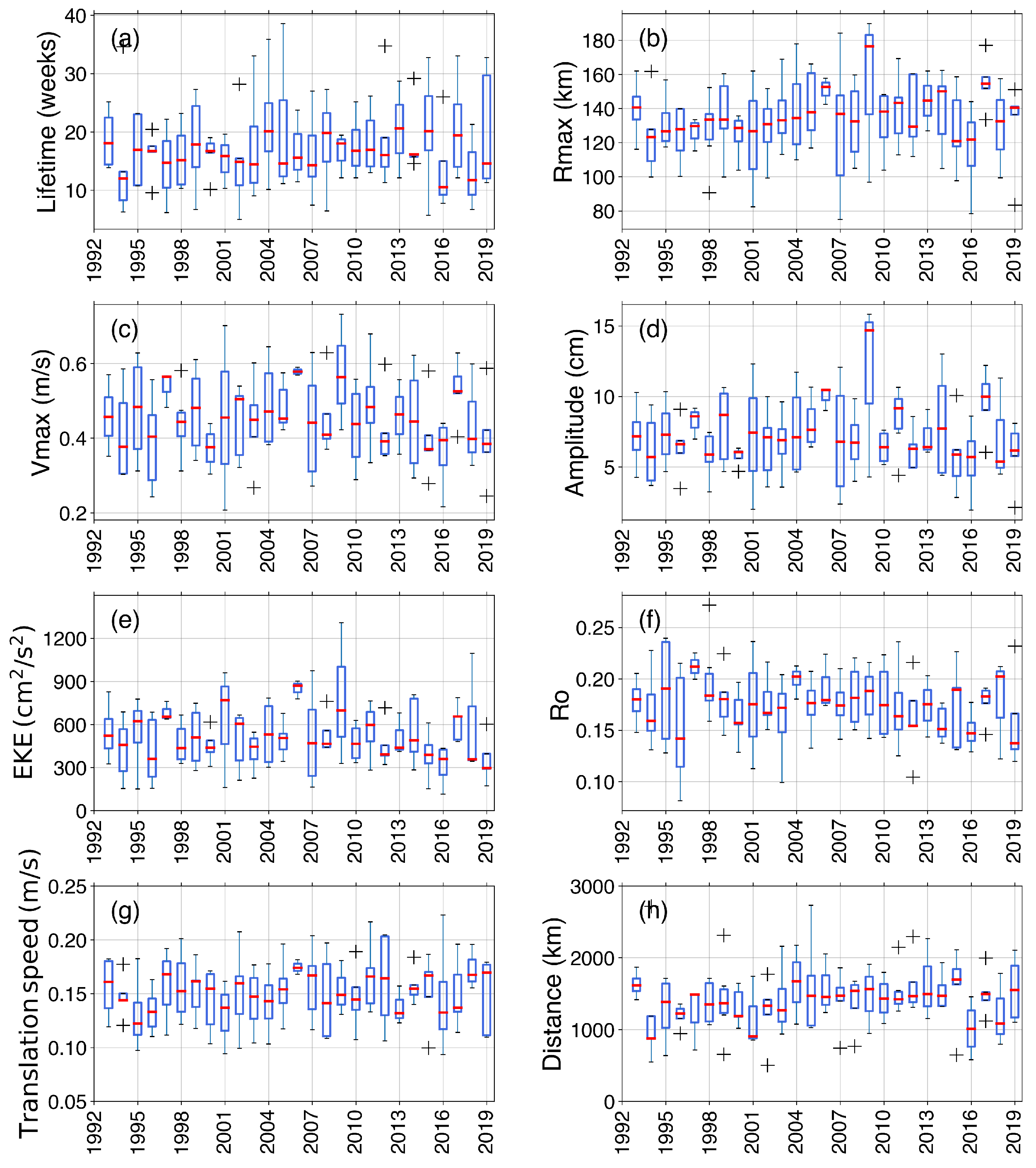
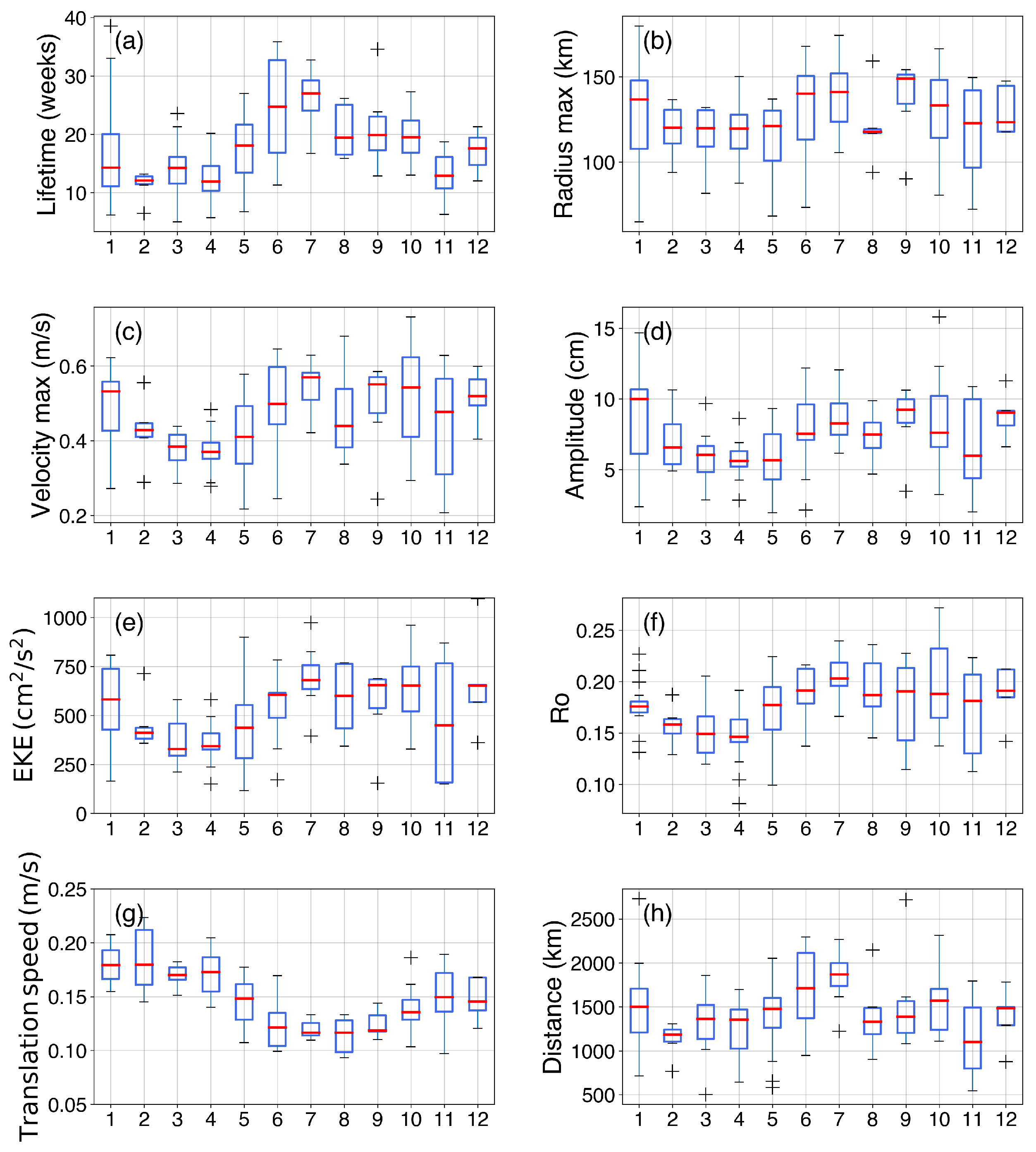
3.2.2. Statistics of Physical NBC Characteristics Derived from Satellite Altimetry
3.2.3. Inter-Annual and Intra-Annual Variability of Physical NBC Characteristics Derived from Satellite Altimetry
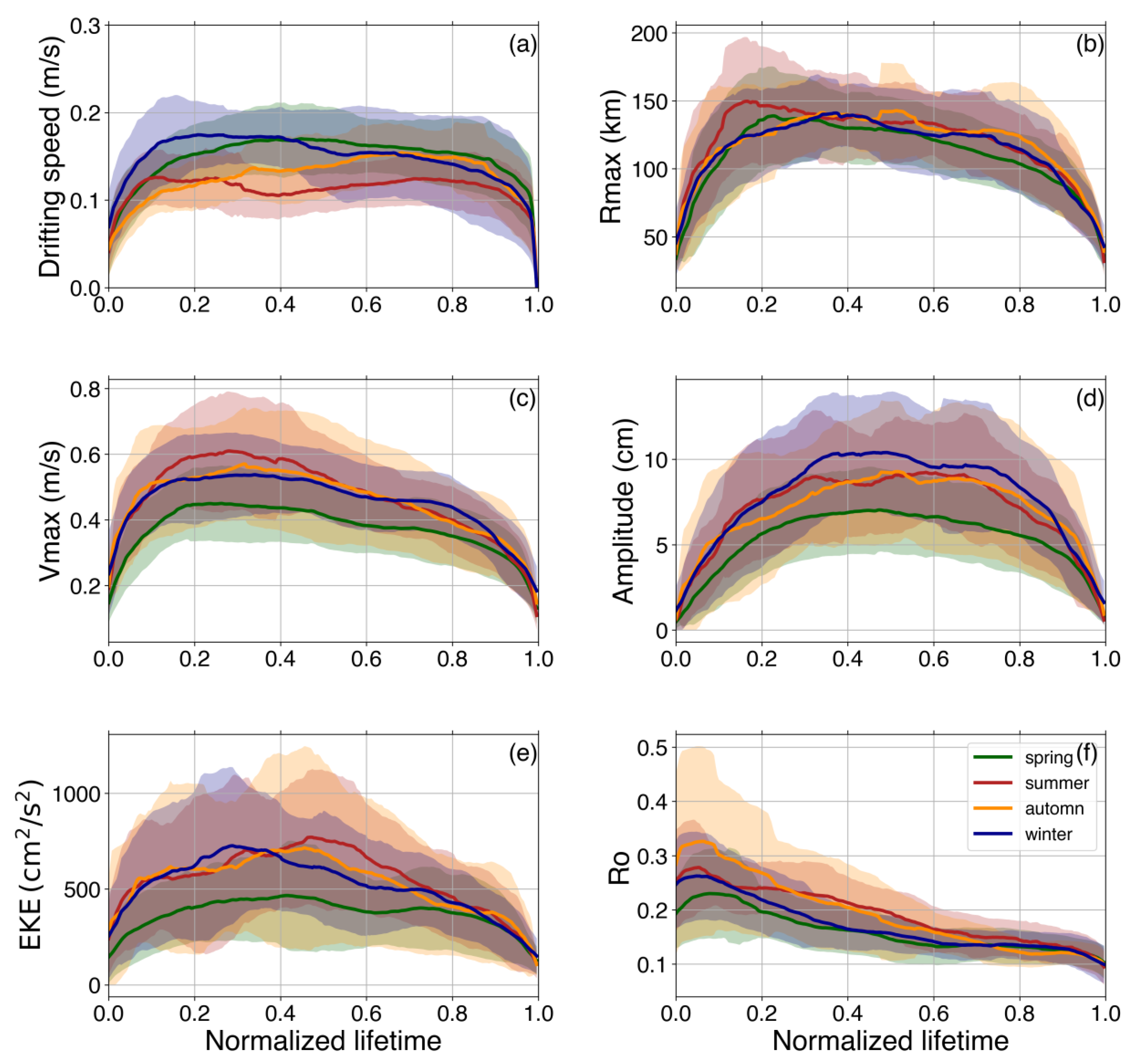
3.2.4. Normalized Ring Life Cycle
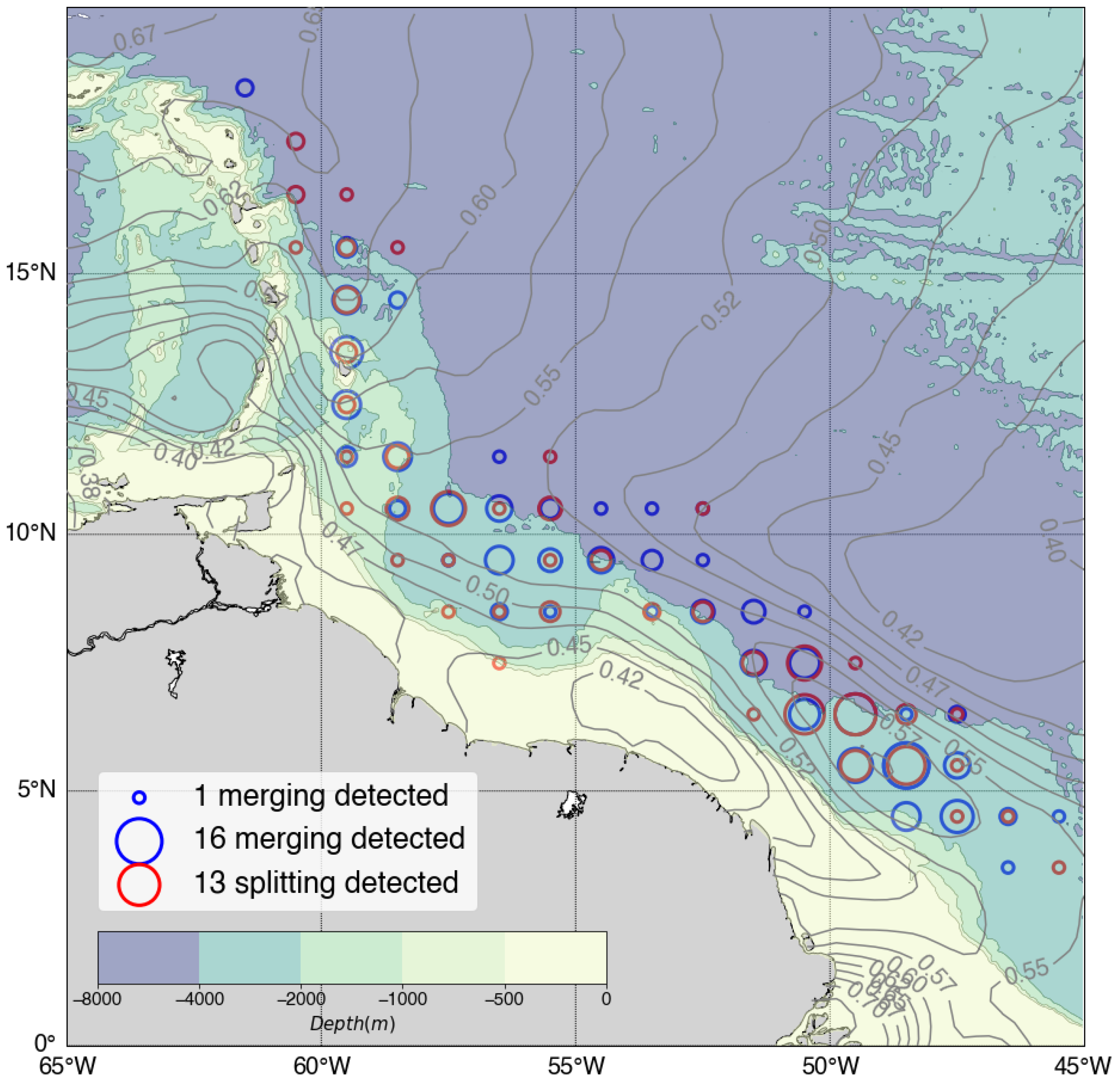
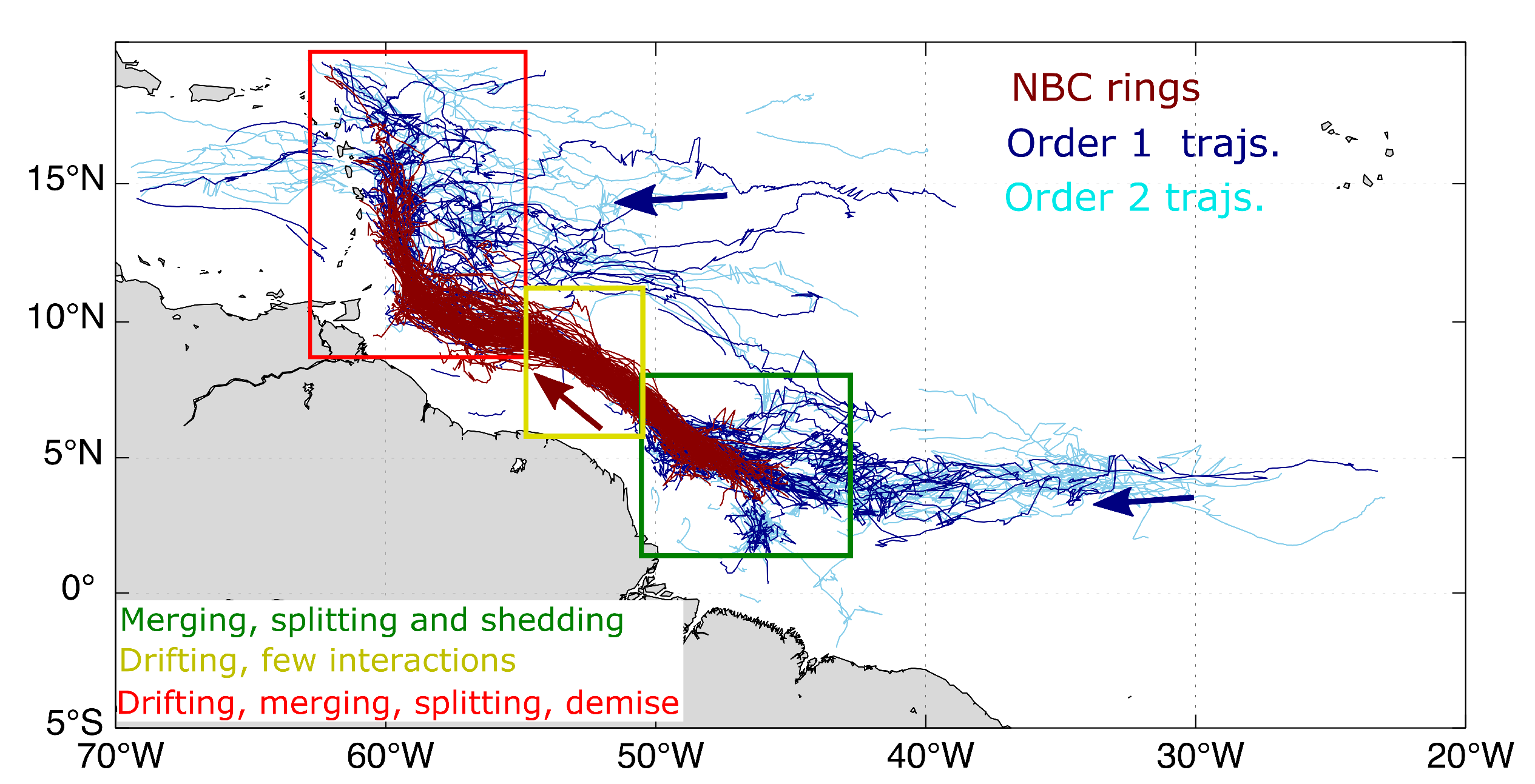
3.2.5. NBC Rings Merging and Splitting: Spatial Distribution and Impact
4. Summary and Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Stammer, D. Global characteristics of ocean variability estimated from regional TOPEX/POSEIDON altimeter measurements. J. Phys. Oceanogr. 1997, 27, 1743–1769. [Google Scholar] [CrossRef]
- Stammer, D.; Wunsch, C. Temporal changes in eddy energy of the oceans. Deep Sea Res. Part II Top. Stud. Oceanogr. 1999, 46, 77–108. [Google Scholar] [CrossRef]
- Chelton, D.B.; Schlax, M.G.; Samelson, R.M. Global observations of nonlinear mesoscale eddies. Prog. Oceanogr. 2011, 91, 167–216. [Google Scholar] [CrossRef]
- McWilliams, J.C. Submesoscale, coherent vortices in the ocean. Rev. Geophys. 1985, 23, 165–182. [Google Scholar] [CrossRef]
- Schott, F.A.; Fischer, J.; Stramma, L. Transports and pathways of the upper-layer circulation in the western tropical Atlantic. J. Phys. Oceanogr. 1998, 28, 1904–1928. [Google Scholar] [CrossRef]
- Johns, W.E.; Lee, T.; Beardsley, R.; Candela, J.; Limeburner, R.; Castro, B. Annual cycle and variability of the North Brazil Current. J. Phys. Oceanogr. 1998, 28, 103–128. [Google Scholar] [CrossRef]
- Johns, W.E.; Lee, T.N.; Schott, F.A.; Zantopp, R.J.; Evans, R.H. The North Brazil Current retroflection: Seasonal structure and eddy variability. J. Geophys. Res. Ocean. 1990, 95, 22103–22120. [Google Scholar] [CrossRef]
- Garzoli, S.L.; Ffield, A.; Yao, Q. North Brazil Current rings and the variability in the latitude of retroflection. In Elsevier Oceanography Series; Elsevier: Amsterdam, The Netherlands, 2003; Volume 68, pp. 357–373. [Google Scholar]
- Fratantoni, D.M.; Richardson, P.L. The evolution and demise of North Brazil Current rings. J. Phys. Oceanogr. 2006, 36, 1241–1264. [Google Scholar] [CrossRef]
- Fratantoni, D.M.; Glickson, D.A. North Brazil Current ring generation and evolution observed with SeaWiFS. J. Phys. Oceanogr. 2002, 32, 1058–1074. [Google Scholar] [CrossRef]
- Johns, W.E.; Zantopp, R.J.; Goni, G.J. Cross-gyre transport by North Brazil Current rings. In Elsevier Oceanography Series; Elsevier: Amsterdam, The Netherlands, 2003; Volume 68, pp. 411–441. [Google Scholar]
- Garraffo, Z.D.; Johns, W.E.; Chassignet, E.P.; Goni, G.J. North Brazil Current rings and transport of southern waters in a high resolution numerical simulation of the North Atlantic. In Elsevier Oceanography Series; Elsevier: Amsterdam, The Netherlands, 2003; Volume 68, pp. 375–409. [Google Scholar]
- Wilson, W.D.; Johns, W.E.; Garzoli, S.L. Velocity structure of North Brazil current rings. Geophys. Res. Lett. 2002, 29, 114-1–114-4. [Google Scholar] [CrossRef]
- Didden, N.; Schott, F. Eddies in the North Brazil Current retroflection region observed by Geosat altimetry. J. Geophys. Res. Ocean. 1993, 98, 20121–20131. [Google Scholar] [CrossRef]
- Jochumsen, K.; Rhein, M.; Hüttl-Kabus, S.; Böning, C.W. On the propagation and decay of North Brazil Current rings. J. Geophys. Res. Ocean. 2010, 115. [Google Scholar] [CrossRef]
- Andrade-Canto, F.; Beron-Vera, F. Do eddies connect the tropical Atlantic Ocean and the Gulf of Mexico? Geophys. Res. Lett. 2022, 49, e2022GL099637. [Google Scholar] [CrossRef]
- Huang, M.; Liang, X.; Zhu, Y.; Liu, Y.; Weisberg, R.H. Eddies connect the tropical Atlantic Ocean and the Gulf of Mexico. Geophys. Res. Lett. 2021, 48, e2020GL091277. [Google Scholar] [CrossRef]
- Reverdin, G.; Olivier, L.; Foltz, G.; Speich, S.; Karstensen, J.; Horstmann, J.; Zhang, D.; Laxenaire, R.; Carton, X.; Branger, H.; et al. Formation and evolution of a freshwater plume in the northwestern tropical Atlantic in February 2020. J. Geophys. Res. Ocean. 2021, 126, e2020JC016981. [Google Scholar] [CrossRef]
- Olivier, L.; Boutin, J.; Reverdin, G.; Lefèvre, N.; Landschützer, P.; Speich, S.; Karstensen, J.; Labaste, M.; Noisel, C.; Ritschel, M.; et al. Wintertime process study of the North Brazil Current rings reveals the region as a larger sink for CO2 than expected. Biogeosciences 2022, 19, 2969–2988. [Google Scholar] [CrossRef]
- Richardson, P.; Hufford, G.; Limeburner, R.; Brown, W. North Brazil current retroflection eddies. J. Geophys. Res. Ocean. 1994, 99, 5081–5093. [Google Scholar] [CrossRef]
- Fratantoni, D.M.; Johns, W.E.; Townsend, T.L. Rings of the North Brazil Current: Their structure and behavior inferred from observations and a numerical simulation. J. Geophys. Res. Ocean. 1995, 100, 10633–10654. [Google Scholar] [CrossRef]
- Goni, G.J.; Johns, W.E. A census of North Brazil Current rings observed from TOPEX/POSEIDON altimetry: 1992–1998. Geophys. Res. Lett. 2001, 28, 1–4. [Google Scholar] [CrossRef]
- Goni, G.J.; Johns, W.E. Synoptic study of warm rings in the North Brazil Current retroflection region using satellite altimetry. In Elsevier Oceanography Series; Elsevier: Amsterdam, The Netherlands, 2003; Volume 68, pp. 335–356. [Google Scholar]
- Mélice, J.L.; Arnault, S. Investigation of the Intra-Annual Variability of the North Equatorial Countercurrent/North Brazil Current Eddies and of the Instability Waves of the North Tropical Atlantic Ocean Using Satellite Altimetry and Empirical Mode Decomposition. J. Atmos. Ocean. Technol. 2017, 34, 2295–2310. [Google Scholar] [CrossRef]
- Aroucha, L.; Veleda, D.; Lopes, F.; Tyaquiçã, P.; Lefèvre, N.; Araujo, M. Intra-and Inter-Annual Variability of North Brazil Current Rings Using Angular Momentum Eddy Detection and Tracking Algorithm: Observations From 1993 to 2016. J. Geophys. Res. Ocean. 2020, 125, e2019JC015921. [Google Scholar] [CrossRef]
- Bueno, L.F.; Costa, V.S.; Mill, G.N.; Paiva, A.M. Volume and Heat Transports by North Brazil Current Rings. Front. Mar. Sci. 2022, 1053. [Google Scholar] [CrossRef]
- Legeckis, R.; Gordon, A.L. Satellite observations of the Brazil and Falkland currents—1975 1976 and 1978. Deep Sea Res. Part A Oceanogr. Res. Pap. 1982, 29, 375–401. [Google Scholar] [CrossRef]
- Sharma, N.; Anderson, S.P.; Brickley, P.; Nobre, C.; Cadwallader, M.L. Quantifying the seasonal and interannual variability of the formation and migration pattern of North Brazil Current Rings. In Proceedings of the OCEANS 2009, Biloxi, MS, USA, 26–29 October 2009; IEEE: Piscataway, NJ, USA, 2009; pp. 1–7. [Google Scholar]
- Le Vu, B.; Stegner, A.; Arsouze, T. Angular Momentum Eddy Detection and tracking Algorithm (AMEDA) and its application to coastal eddy formation. J. Atmos. Ocean. Technol. 2018, 35, 739–762. [Google Scholar] [CrossRef]
- Stevens, B.; Bony, S.; Farrell, D.; Ament, F.; Blyth, A.; Fairall, C.; Karstensen, J.; Quinn, P.K.; Speich, S.; Acquistapace, C.; et al. EUREC 4 a. Earth Syst. Sci. Data 2021, 13, 4067–4119. [Google Scholar] [CrossRef]
- Speich, S.; Embarked Science Team. EUREC4A-OA. Cruise Report. 19 January–19 February 2020. Vessel: L’ATALANTE 2021. Available online: https://archimer.ifremer.fr/doc/00689/80129/ (accessed on 1 January 2023).
- Karstensen, J.; Lavik, G.; Kopp, A.; Mehlmann, M.; Boeck, T.; Ribbe, J.; Guettler, J.; Nordsiek, F.; Philippi, M.; Bodenschatz, E.; et al. EUREC4A Campaign, Cruise No. MSM89, 17 January–20 February 2020, Bridgetown Barbados–Bridgetown Barbados, The Ocean Mesoscale Component in the EUREC4A++ Field Study, MARIA S. MERIAN-Berichte, 2020. Available online: https://oceanrep.geomar.de/id/eprint/56445/1/msm89_cruise_report.pdf (accessed on 1 January 2023).
- Quinn, P.K.; Thompson, E.J.; Coffman, D.J.; Baidar, S.; Bariteau, L.; Bates, T.S.; Bigorre, S.; Brewer, A.; De Boer, G.; De Szoeke, S.P.; et al. Measurements from the RV Ronald H. Brown and related platforms as part of the Atlantic Tradewind Ocean-Atmosphere Mesoscale Interaction Campaign (ATOMIC). Earth Syst. Sci. Data 2021, 13, 1759–1790. [Google Scholar] [CrossRef]
- Laxenaire, R.; Speich, S.; Blanke, B.; Chaigneau, A.; Pegliasco, C.; Stegner, A. Anticyclonic eddies connecting the western boundaries of Indian and Atlantic Oceans. J. Geophys. Res. Ocean. 2018, 123, 7651–7677. [Google Scholar] [CrossRef]
- Laxenaire, R.; Speich, S.; Stegner, A. Evolution of the thermohaline structure of one Agulhas ring reconstructed from satellite altimetry and Argo floats. J. Geophys. Res. Ocean. 2019, 124, 8969–9003. [Google Scholar] [CrossRef]
- Laxenaire, R.; Speich, S.; Stegner, A. Agulhas Ring Heat Content and Transport in the South Atlantic Estimated by Combining Satellite Altimetry and Argo Profiling Floats Data. J. Geophys. Res. Ocean. 2020, 125, e2019JC015511. [Google Scholar] [CrossRef]
- Taburet, G.; Sanchez-Roman, A.; Ballarotta, M.; Pujol, M.I.; Legeais, J.F.; Fournier, F.; Faugere, Y.; Dibarboure, G. DUACS DT2018: 25 years of reprocessed sea level altimetry products. Ocean Sci. 2019, 15, 1207–1224. [Google Scholar] [CrossRef]
- L’Hégaret, P.; Schütte, F.; Speich, S.; Reverdin, G.; Baranowski, D.B.; Czeschel, R.; Fischer, T.; Foltz, G.R.; Heywood, K.J.; Krahmann, G.; et al. Ocean cross-validated observations from the R/Vs L’Atalante, Maria S. Merian and Meteor and related platforms as part of the EUREC 4 A-OA/ATOMIC campaign. Earth Syst. Sci. Data Discuss. 2022, 1–39. [Google Scholar] [CrossRef]
- Müller, P. Ertel’s potential vorticity theorem in physical oceanography. Rev. Geophys. 1995, 33, 67–97. [Google Scholar] [CrossRef]
- Ballarotta, M.; Ubelmann, C.; Pujol, M.I.; Taburet, G.; Fournier, F.; Legeais, J.F.; Faugère, Y.; Delepoulle, A.; Chelton, D.; Dibarboure, G.; et al. On the resolutions of ocean altimetry maps. Ocean Sci. 2019, 15, 1091–1109. [Google Scholar] [CrossRef]
- Chaigneau, A.; Gizolme, A.; Grados, C. Mesoscale eddies off Peru in altimeter records: Identification algorithms and eddy spatio-temporal patterns. Prog. Oceanogr. 2008, 79, 106–119. [Google Scholar] [CrossRef]
- Chaigneau, A.; Eldin, G.; Dewitte, B. Eddy activity in the four major upwelling systems from satellite altimetry (1992–2007). Prog. Oceanogr. 2009, 83, 117–123. [Google Scholar] [CrossRef]
- Pegliasco, C.; Chaigneau, A.; Morrow, R. Main eddy vertical structures observed in the four major Eastern Boundary Upwelling Systems. J. Geophys. Res. Ocean. 2015, 120, 6008–6033. [Google Scholar] [CrossRef]
- Manta, G.; Speich, S.; Karstensen, J.; Hummels, R.; Kersalé, M.; Laxenaire, R.; Piola, A.; Chidichimo, M.; Sato, O.; Cotrim da Cunha, L.; et al. The South Atlantic Meridional Overturning Circulation and Mesoscale Eddies in the First GO-SHIP Section at 34.5°S. J. Geophys. Res. Ocean. 2021, 126, e2020JC016962. [Google Scholar] [CrossRef]
- Ioannou, A.; Speich, S.; Laxenaire, R. Mesoscale Eddy Connectivity in the Atlantic Ocean. In Proceedings of the AGU Fall Meeting Abstracts, Online, 1–17 December 2020; Volume 2020, p. OS017-06. [Google Scholar]
- Chen, Y.; Speich, S.; Laxenaire, R. Formation and Transport of the South Atlantic Subtropical Mode Water in Eddy-Permitting Observations. J. Geophys. Res. Ocean. 2022, 127, e2021JC017767. [Google Scholar] [CrossRef]
- Pegliasco, C.; Chaigneau, A.; Morrow, R.; Dumas, F. Detection and tracking of mesoscale eddies in the Mediterranean Sea: A comparison between the Sea Level Anomaly and the Absolute Dynamic Topography fields. Adv. Space Res. 2021, 68, 401–419. [Google Scholar] [CrossRef]
- Garzoli, S.L.; Ffield, A.; Johns, W.E.; Yao, Q. North Brazil Current retroflection and transports. J. Geophys. Res. Ocean. 2004, 109. [Google Scholar] [CrossRef]
- Guinehut, S.; Dhomps, A.L.; Larnicol, G.; Le Traon, P.Y. High resolution 3-D temperature and salinity fields derived from in situ and satellite observations. Ocean Sci. 2012, 8, 845–857. [Google Scholar] [CrossRef]
- Mulet, S.; Rio, M.H.; Mignot, A.; Guinehut, S.; Morrow, R. A new estimate of the global 3D geostrophic ocean circulation based on satellite data and in-situ measurements. Deep Sea Res. Part II Top. Stud. Oceanogr. 2012, 77, 70–81. [Google Scholar] [CrossRef]
- Jouanno, J.; Sheinbaum, J.; Barnier, B.; Molines, J.M.; Candela, J. Seasonal and interannual modulation of the eddy kinetic energy in the Caribbean Sea. J. Phys. Oceanogr. 2012, 42, 2041–2055. [Google Scholar] [CrossRef]
- Smith, W.H.; Sandwell, D.T. Global sea floor topography from satellite altimetry and ship depth soundings. Science 1997, 277, 1956–1962. [Google Scholar] [CrossRef]
- Flierl, G.R. Particle motions in large-amplitude wave fields. Geophys. Astrophys. Fluid Dyn. 1981, 18, 39–74. [Google Scholar] [CrossRef]
- De Marez, C.; Carton, X.; Morvan, M.; Reinaud, J.N. The interaction of two surface vortices near a topographic slope in a stratified ocean. Fluids 2017, 2, 57. [Google Scholar] [CrossRef]
- Morvan, M.; l’Hégaret, P.; Carton, X.; Gula, J.; Vic, C.; de Marez, C.; Sokolovskiy, M.; Koshel, K. The life cycle of submesoscale eddies generated by topographic interactions. Ocean Sci. 2019, 15, 1531–1543. [Google Scholar]
- Stramma, L.; Fischer, J.; Brandt, P.; Schott, F. Circulation, variability and near-equatorial meridional flow in the central tropical Atlantic. In Elsevier Oceanography Series; Elsevier: Amsterdam, The Netherlands, 2003; Volume 68, pp. 1–22. [Google Scholar]
- Coles, V.J.; Brooks, M.T.; Hopkins, J.; Stukel, M.R.; Yager, P.L.; Hood, R.R. The pathways and properties of the Amazon River Plume in the tropical North Atlantic Ocean. J. Geophys. Res. Ocean. 2013, 118, 6894–6913. [Google Scholar] [CrossRef]
- Stramma, L.; Rhein, M.; Brandt, P.; Dengler, M.; Böning, C.; Walter, M. Upper ocean circulation in the western tropical Atlantic in boreal fall 2000. Deep Sea Res. Part I Oceanogr. Res. Pap. 2005, 52, 221–240. [Google Scholar] [CrossRef]
- Doglioli, A.; Blanke, B.; Speich, S.; Lapeyre, G. Tracking coherent structures in a regional ocean model with wavelet analysis: Application to Cape Basin eddies. J. Geophys. Res. Ocean. 2007, 112. [Google Scholar] [CrossRef]
- Meunier, T.; Tenreiro, M.; Pallàs-Sanz, E.; Ochoa, J.; Ruiz-Angulo, A.; Portela, E.; Cusí, S.; Damien, P.; Carton, X. Intrathermocline eddies embedded within an anticyclonic vortex ring. Geophys. Res. Lett. 2018, 45, 7624–7633. [Google Scholar] [CrossRef]
- Meunier, T.; Pallás Sanz, E.; de Marez, C.; Pérez, J.; Tenreiro, M.; Ruiz Angulo, A.; Bower, A. The dynamical structure of a warm core ring as inferred from glider observations and along-track altimetry. Remote Sens. 2021, 13, 2456. [Google Scholar] [CrossRef]
- Garcia, H.E.; Weathers, K.W.; Paver, C.R.; Smolyar, I.; Boyer, T.P.; Locarnini, M.M.; Zweng, M.M.; Mishonov, A.V.; Baranova, O.K.; Seidov, D.; et al. World Ocean Atlas 2018, Volume 3: Dissolved Oxygen, Apparent Oxygen Utilization, and Dissolved Oxygen Saturation. Available online: https://archimer.ifremer.fr/doc/00651/76337/ (accessed on 1 January 2023).
- Locarnini, M.M.; Mishonov, A.V.; Baranova, O.K.; Boyer, T.P.; Zweng, M.M.; Garcia, H.E.; Seidov, D.; Weathers, K.; Paver, C.; Smolyar, I.; et al. World Ocean Atlas 2018, Volume 1: Temperature. Available online: https://archimer.ifremer.fr/doc/00651/76338/ (accessed on 1 January 2023).
- World Ocean Atlas 2018, Volume 2: Salinity. Available online: https://archimer.ifremer.fr/doc/00651/76339/ (accessed on 1 January 2023).
- Nencioli, F.; Kuwahara, V.S.; Dickey, T.D.; Rii, Y.M.; Bidigare, R.R. Physical dynamics and biological implications of a mesoscale eddy in the lee of Hawai’i: Cyclone Opal observations during E-Flux III. Deep Sea Res. Part II Top. Stud. Oceanogr. 2008, 55, 1252–1274. [Google Scholar] [CrossRef]
- Kersale, M.; Petrenko, A.; Doglioli, A.M.; Dekeyser, I.; Nencioli, F. Physical characteristics and dynamics of the coastal Latex09 Eddy derived from in situ data and numerical modeling. J. Geophys. Res. Ocean. 2013, 118, 399–409. [Google Scholar] [CrossRef]
- Boebel, O.; Barron, C. A comparison of in-situ float velocities with altimeter derived geostrophic velocities. Deep Sea Res. Part II Top. Stud. Oceanogr. 2003, 50, 119–139. [Google Scholar] [CrossRef]
- Ioannou, A.; Stegner, A.; Tuel, A.; LeVu, B.; Dumas, F.; Speich, S. Cyclostrophic corrections of AVISO/DUACS surface velocities and its application to mesoscale eddies in the Mediterranean Sea. J. Geophys. Res. Ocean. 2019, 124, 8913–8932. [Google Scholar] [CrossRef]
- Cao, Y.; Dong, C.; Stegner, A.; Bethel, B.J.; Li, C.; Dong, J.; Lü, H.; Yang, J. Global Sea Surface Cyclogeostrophic Currents Derived from Satellite Altimetry Data. J. Geophys. Res. Ocean. 2023, 128, e2022JC019357. [Google Scholar] [CrossRef]
- Nof, D.; Pichevin, T. The retroflection paradox. J. Phys. Oceanogr. 1996, 26, 2344–2358. [Google Scholar] [CrossRef]
- Castelão, G.; Johns, W. Sea surface structure of North Brazil Current rings derived from shipboard and moored acoustic Doppler current profiler observations. J. Geophys. Res. Ocean. 2011, 116. [Google Scholar] [CrossRef]
- Silva, A.; Bourlès, B.; Araujo, M. Circulation of the thermocline salinity maximum waters off the Northern Brazil as inferred from in situ measurements and numerical results. In Annales Geophysicae; Copernicus GmbH: Göttingen, Germany, 2009; Volume 27, pp. 1861–1873. [Google Scholar]
- Nof, D. On the β-induced movement of isolated baroclinic eddies. J. Phys. Oceanogr. 1981, 11, 1662–1672. [Google Scholar] [CrossRef]
- Aguedjou, H.; Dadou, I.; Chaigneau, A.; Morel, Y.; Alory, G. Eddies in the Tropical Atlantic Ocean and their seasonal variability. Geophys. Res. Lett. 2019, 46, 12156–12164. [Google Scholar] [CrossRef]
- Richardson, P.L.; Walsh, D. Mapping climatological seasonal variations of surface currents in the tropical Atlantic using ship drifts. J. Geophys. Res. Ocean. 1986, 91, 10537–10550. [Google Scholar] [CrossRef]
- Richardson, P.L.; Arnault, S.; Garzoli, S.; Bruce, J.G. Annual cycle of the Atlantic north equatorial countercurrent. Deep Sea Res. Part A Oceanogr. Res. Pap. 1992, 39, 997–1014. [Google Scholar] [CrossRef]
- Chen, G.; Han, G. Contrasting short-lived with long-lived mesoscale eddies in the global ocean. J. Geophys. Res. Ocean. 2019, 124, 3149–3167. [Google Scholar] [CrossRef]
- Cui, W.; Wang, W.; Zhang, J.; Yang, J. Multicore structures and the splitting and merging of eddies in global oceans from satellite altimeter data. Ocean Sci. 2019, 15, 413–430. [Google Scholar] [CrossRef]
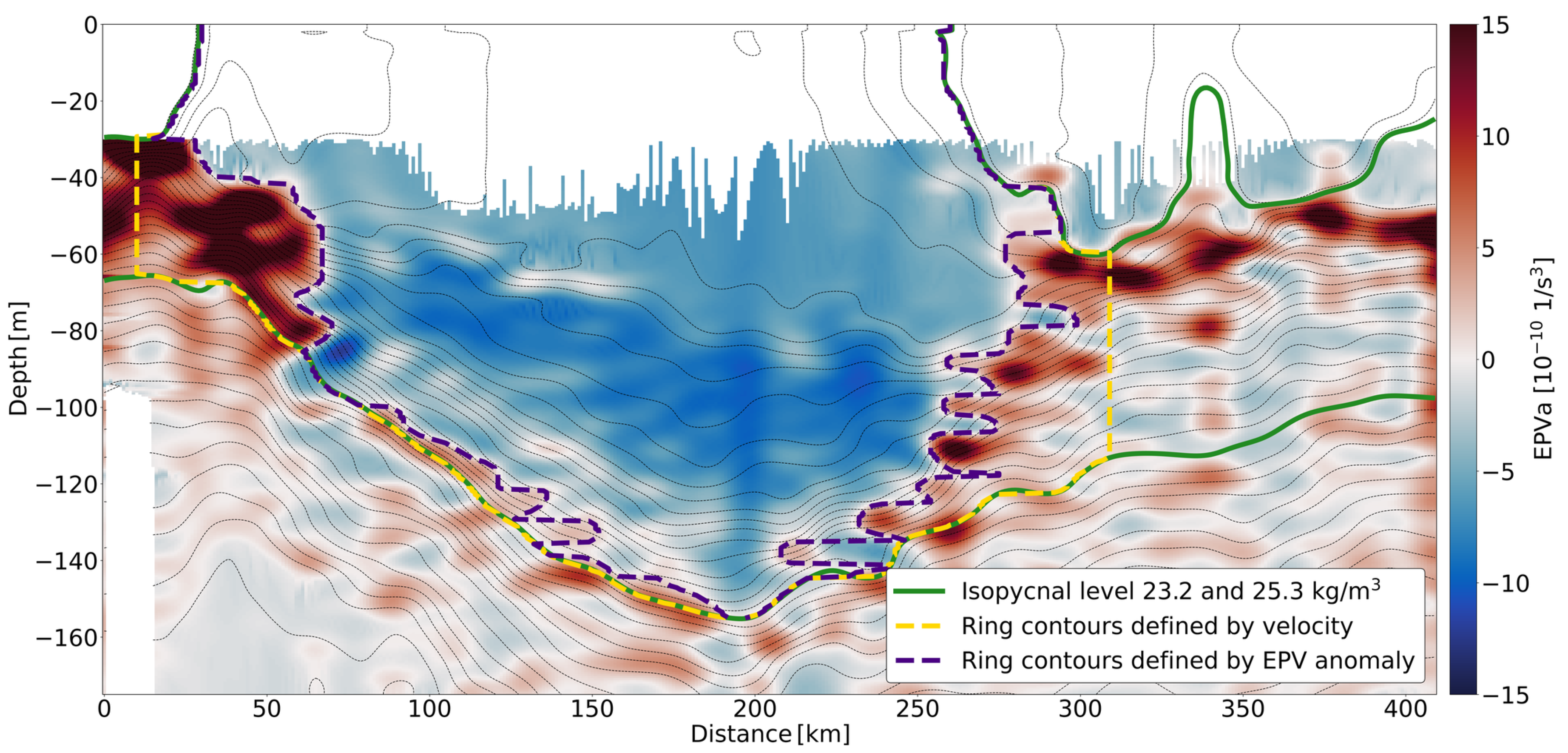



| EPVa Anomaly Method | Maximum Velocity Method | Cylinder Method | |
|---|---|---|---|
| Volume (10 m) | 4.51 | 6.27 | 10.9 |
| Transport (Sv) | 0.14 | 0.20 | 0.34 |
| Salt content (10 kg) | 167 | 233 | 407 |
| Salt content anom clim (10 kg) | 167 | 233 | 407 |
| Salt transport (Sv PSU kg/s) | 5.3 | 7.4 | 12.9 |
| Heat content (10 J) | 4.9 | 6.8 | 11.1 |
| Heat content anm clim (10 J) | 0.11 | 0.15 | 0.23 |
| Heat transport (PW) | 0.016 | 0.022 | 0.035 |
| Oxygen content (10 micro mol) | 88 | 121 | 202 |
| Oxygen content anom clim (10 micro mol) | −1.71 | −1.47 | −5.62 |
| Radius (km) | 126 | 150 | 150 |
| Vertical extension (m) | 155 | 155 | 155 |
| Norm Difference first 100 m | Norm Difference at 30 m | Direction Difference first 100 m | Direction Difference at 30 m | |
|---|---|---|---|---|
| Mean | 0.05 m/s | 0.09 m/s | −0.8° | 1.8° |
| Std | 0.14 m/s | 0.16 m/s | 49.6° | 48.8° |
| Min | −0.27 m/s | −0.33 m/s | −179° | −179° |
| Max | 0.48 m/s | 0.59 m/s | 179° | 179° |
| Ring Generation Rate per Year | Method | Mean Radius (km) | Mean Vmax (m/s) | |
|---|---|---|---|---|
| Johns et al. (1990) [7] | 3–4 (1987–1988) | Mooring | 200 | - |
| Didden and Schott (1993) [14] | 2 (1988–1989) | Altimetry | 130 | 0.4 |
| Richardson et al. (1994) [20] | 3–4 (1989–1992) | Drifter | 125 | 0.8 |
| Frantantoni et al. (1995) [21] | 3–5 (1987–1998) | Mooring | 125 | 0.42 |
| Goni and Johns (2001) [22] | 2–6 (1992–1998) | Altimetry | 97.5 | - |
| Frantantoni and Glickson (2002) [10] | 5 (1997–2000) | SeaWiFs | 100–150 | - |
| Johns et al. (2003) [11] | 8–9 (1998–2000:16) | R/V and mooring | 130 | 1 |
| Garzoli et al. (2003) [8] | 6–7 (1998–2000:11) | R/V | 195 | - |
| Frantantoni and Richardson (2006) [9] | 3–4 (1999–2001:10) | Drifter | 100-150 | 0.75 |
| Sharma et al. (2009) [28] | 5–6 (2001–2009:44) | Drifter | 180.8 | 0.7–2.0 |
| Jochumsen et al. (2010) [15] | 6–7 (1990–2004:102) | Model | 181.5 | 0.9–1 |
| castelao et al. (2011) [71] | 7 (1998–2000) | R/V and Mooring | 125 | 0.9 |
| Melice and Arnault (2017) [24] | 5 (1990–2004:102) | Altimetry | 125 | - |
| Aroucha et al. (2020) [25] | 5 (1993–2016:121) | Altimetry | 139.8 | 0.37 |
| Bueno et al. (2022) [26] | 5.3 (1993–2016:128) | Altimetry | 138 | 0.40 |
| Present study | 4.5 (1993–2020:122) | Altimetry | 123.9 | 0.45 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Subirade, C.; L’Hégaret, P.; Speich, S.; Laxenaire, R.; Karstensen, J.; Carton, X. Combining an Eddy Detection Algorithm with In-Situ Measurements to Study North Brazil Current Rings. Remote Sens. 2023, 15, 1897. https://doi.org/10.3390/rs15071897
Subirade C, L’Hégaret P, Speich S, Laxenaire R, Karstensen J, Carton X. Combining an Eddy Detection Algorithm with In-Situ Measurements to Study North Brazil Current Rings. Remote Sensing. 2023; 15(7):1897. https://doi.org/10.3390/rs15071897
Chicago/Turabian StyleSubirade, Corentin, Pierre L’Hégaret, Sabrina Speich, Rémi Laxenaire, Johannes Karstensen, and Xavier Carton. 2023. "Combining an Eddy Detection Algorithm with In-Situ Measurements to Study North Brazil Current Rings" Remote Sensing 15, no. 7: 1897. https://doi.org/10.3390/rs15071897
APA StyleSubirade, C., L’Hégaret, P., Speich, S., Laxenaire, R., Karstensen, J., & Carton, X. (2023). Combining an Eddy Detection Algorithm with In-Situ Measurements to Study North Brazil Current Rings. Remote Sensing, 15(7), 1897. https://doi.org/10.3390/rs15071897






