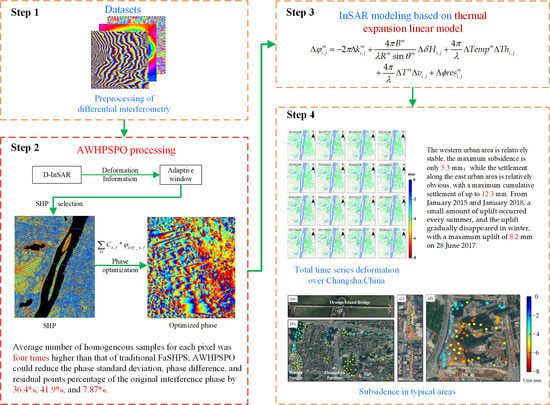
Slow Deformation Time-Series Monitoring for Urban Areas Based on the AWHPSPO Algorithm and TELM: A Case Study of Changsha, China
Abstract
1. Introduction
2. Methods
2.1. SHP Selection with Adaptive Window Considering the Deformation Information
2.2. Phase Optimization with Adaptive Window Considering the Deformation Information
2.3. Deformation Estimation Based on the Thermal Expansion Model
2.4. Processing Flow of the AWHPSPO Algorithm
3. Experiment
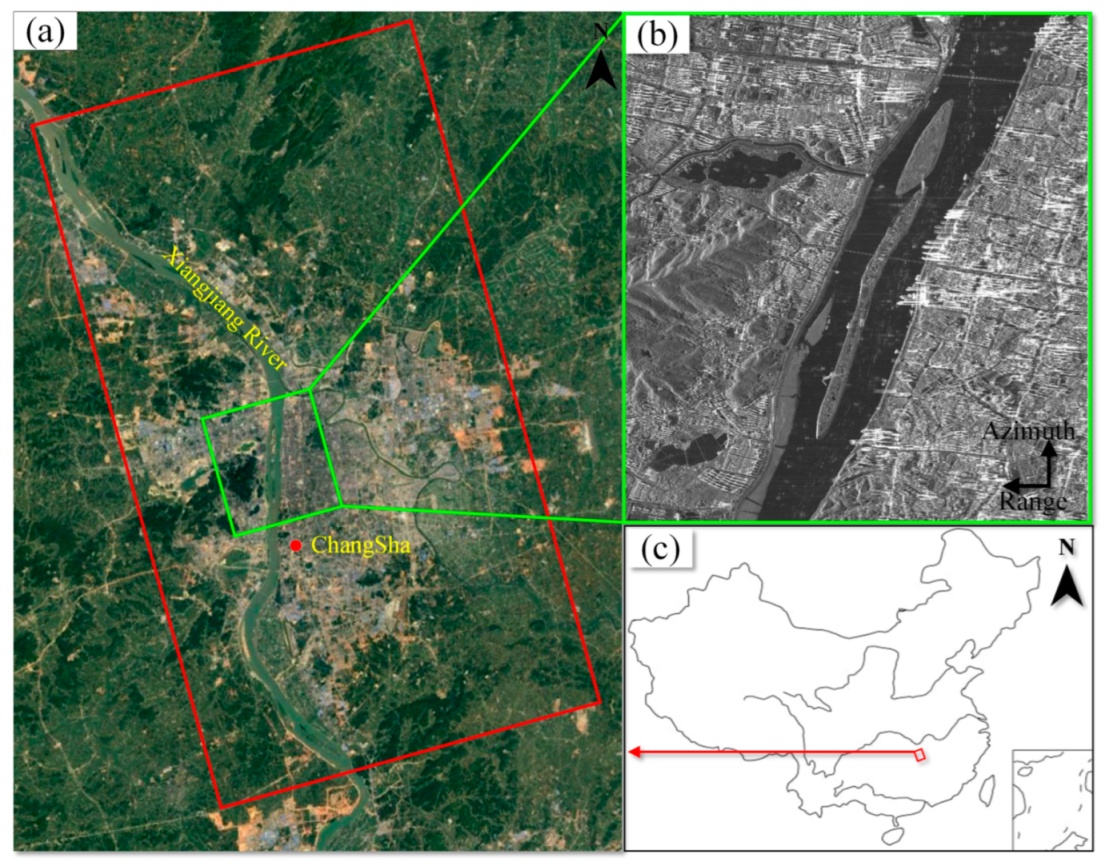
3.1. Background of the Study Area and Interferometry Preprocessing
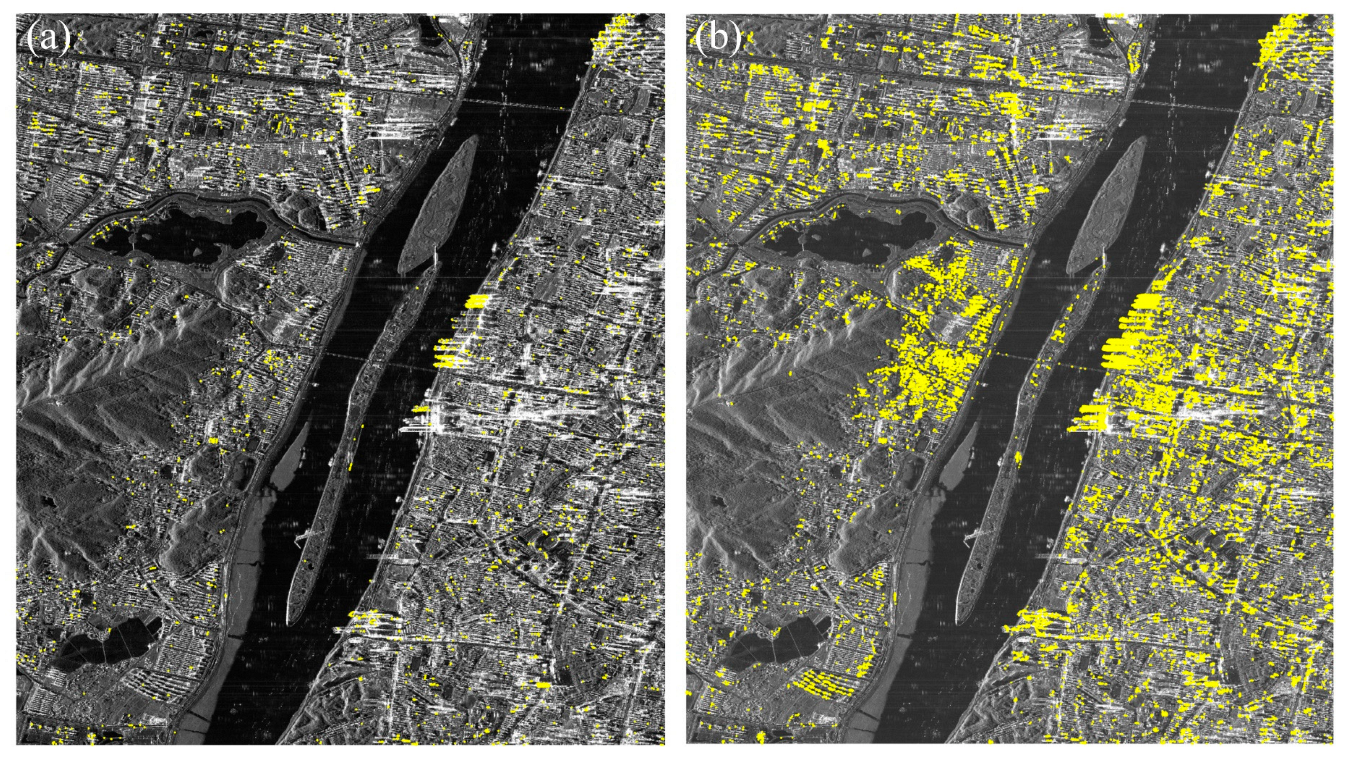
3.2. PS-DS SHP Selection
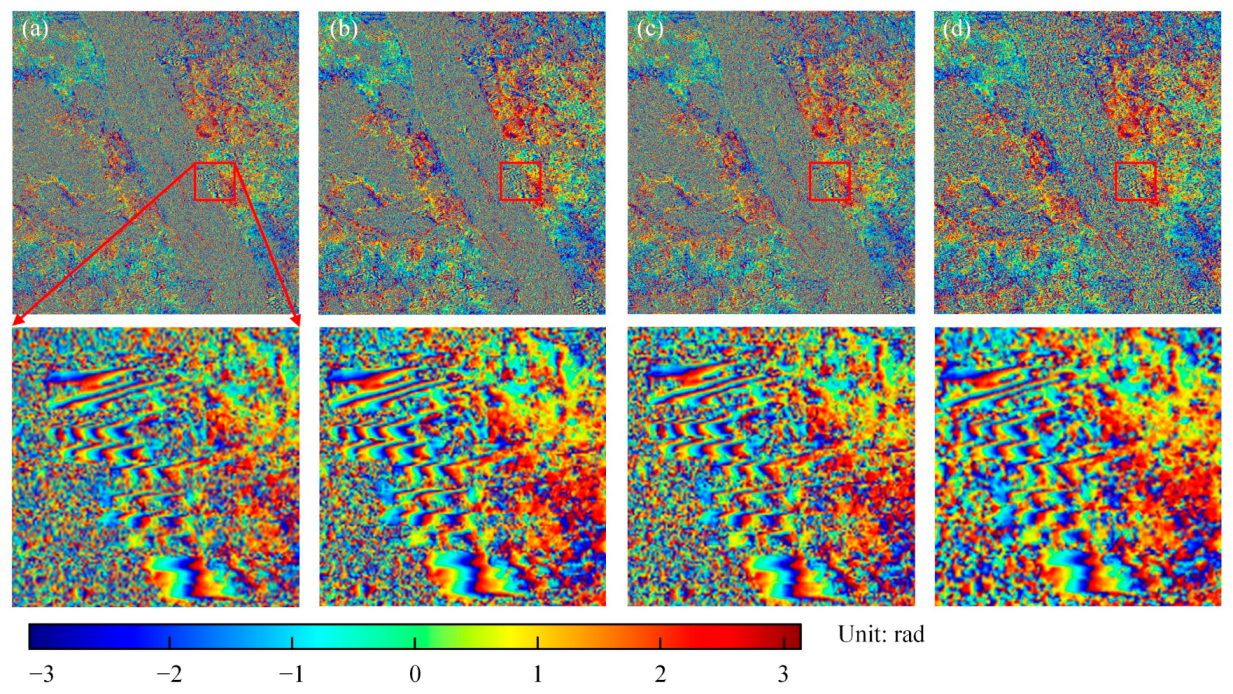
3.3. Phase Optimization
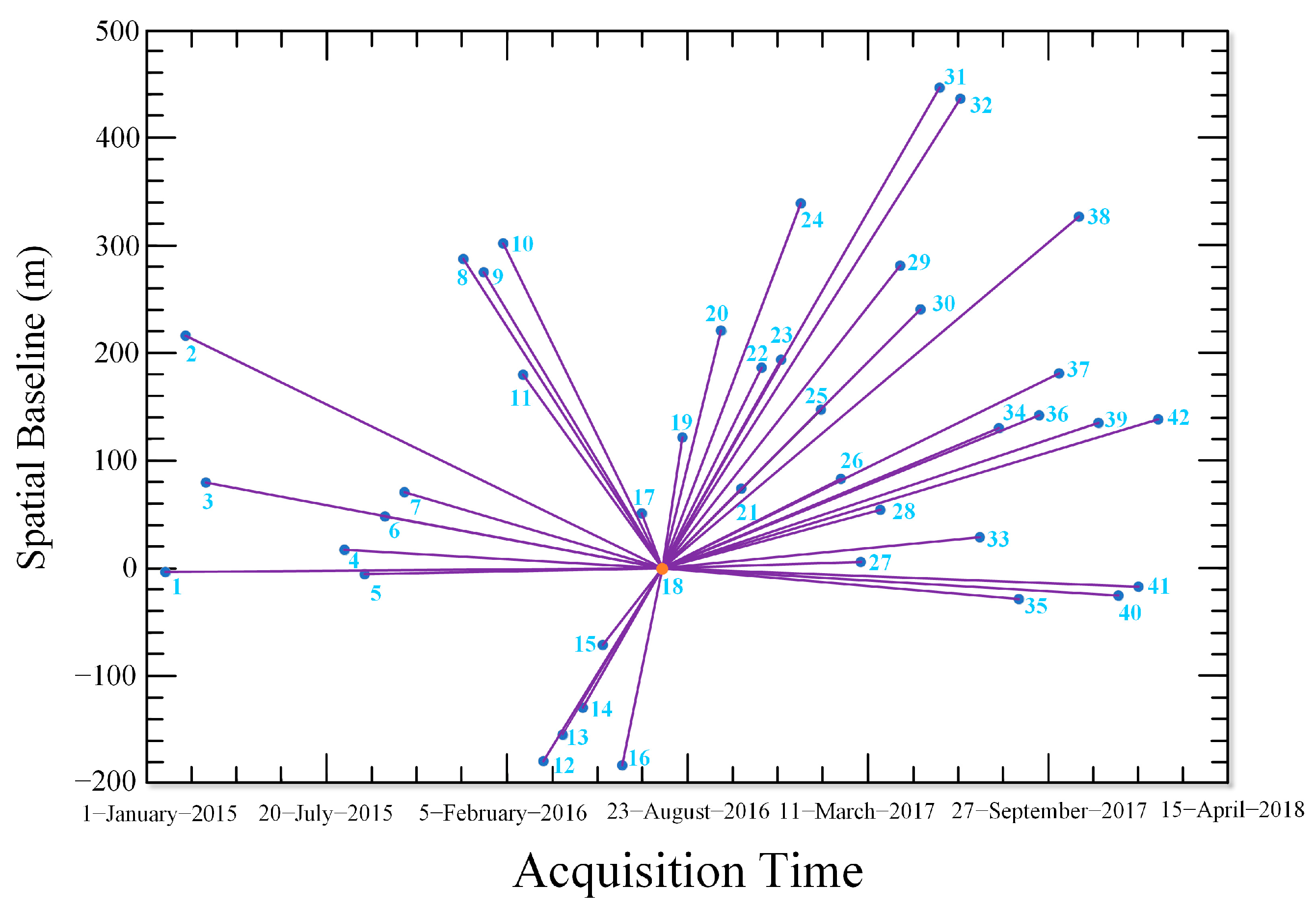
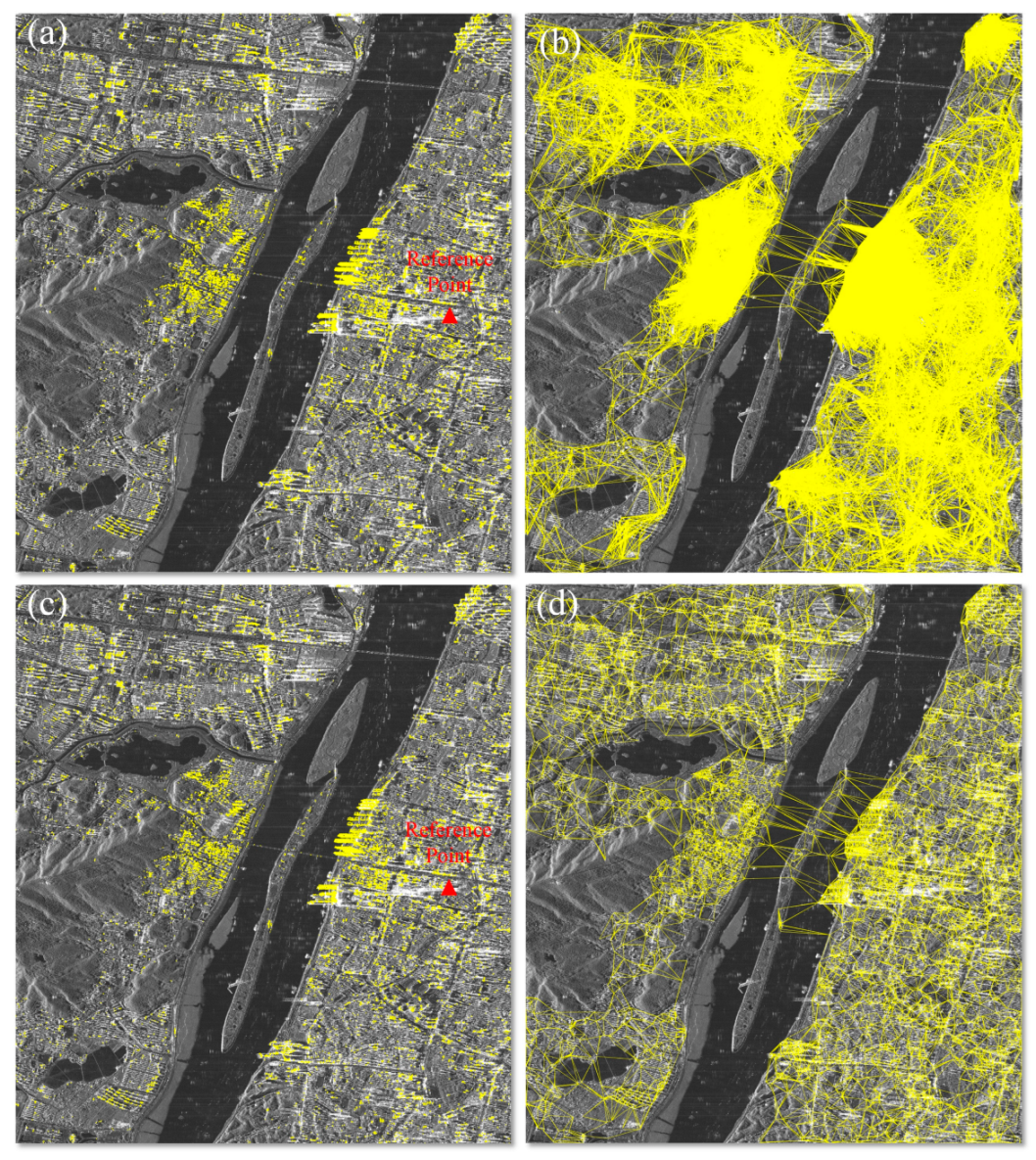
3.4. PS-DS Network Construction and Baseline Quality Assessment
4. Results
4.1. Model Parameter Estimation Results
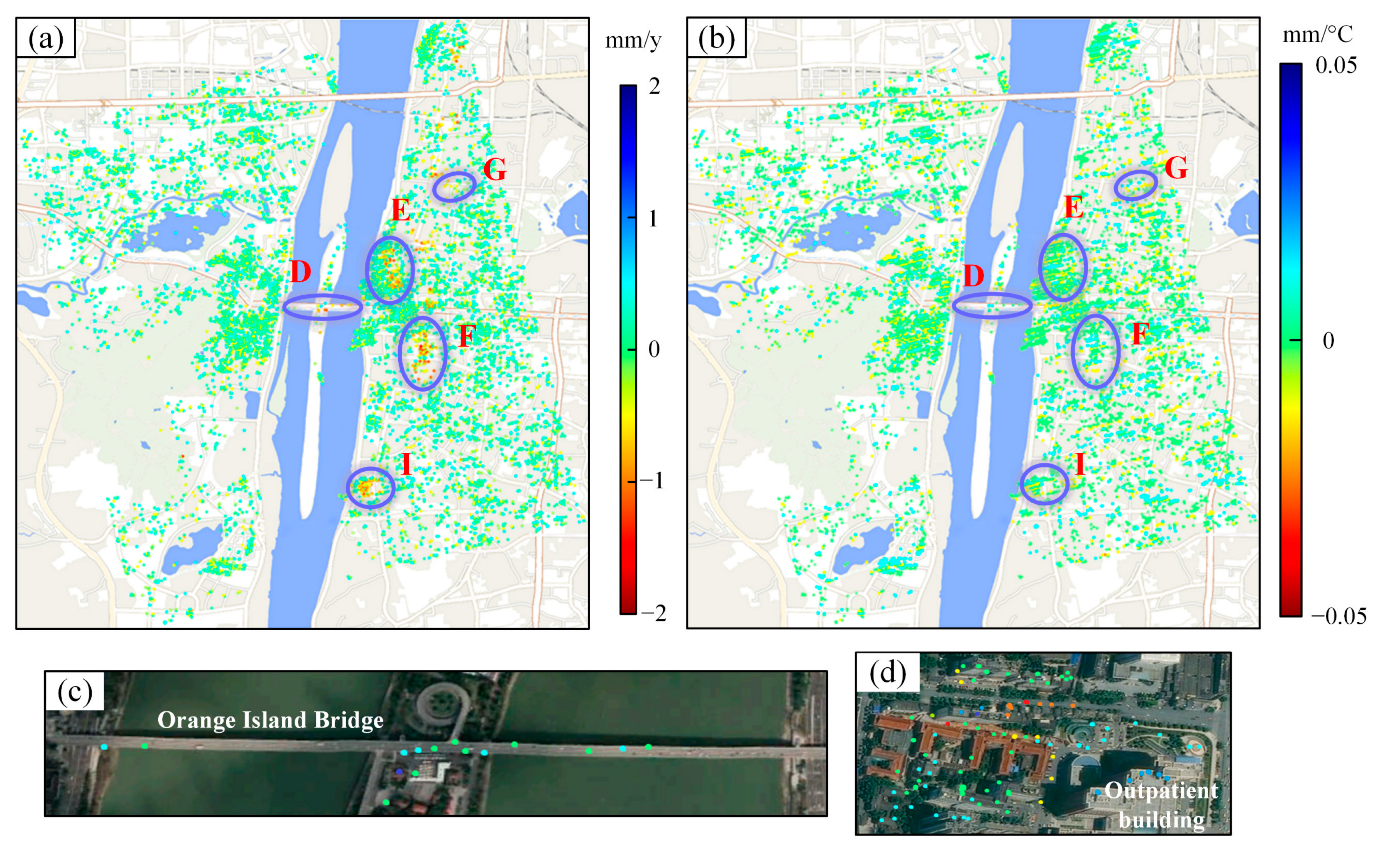
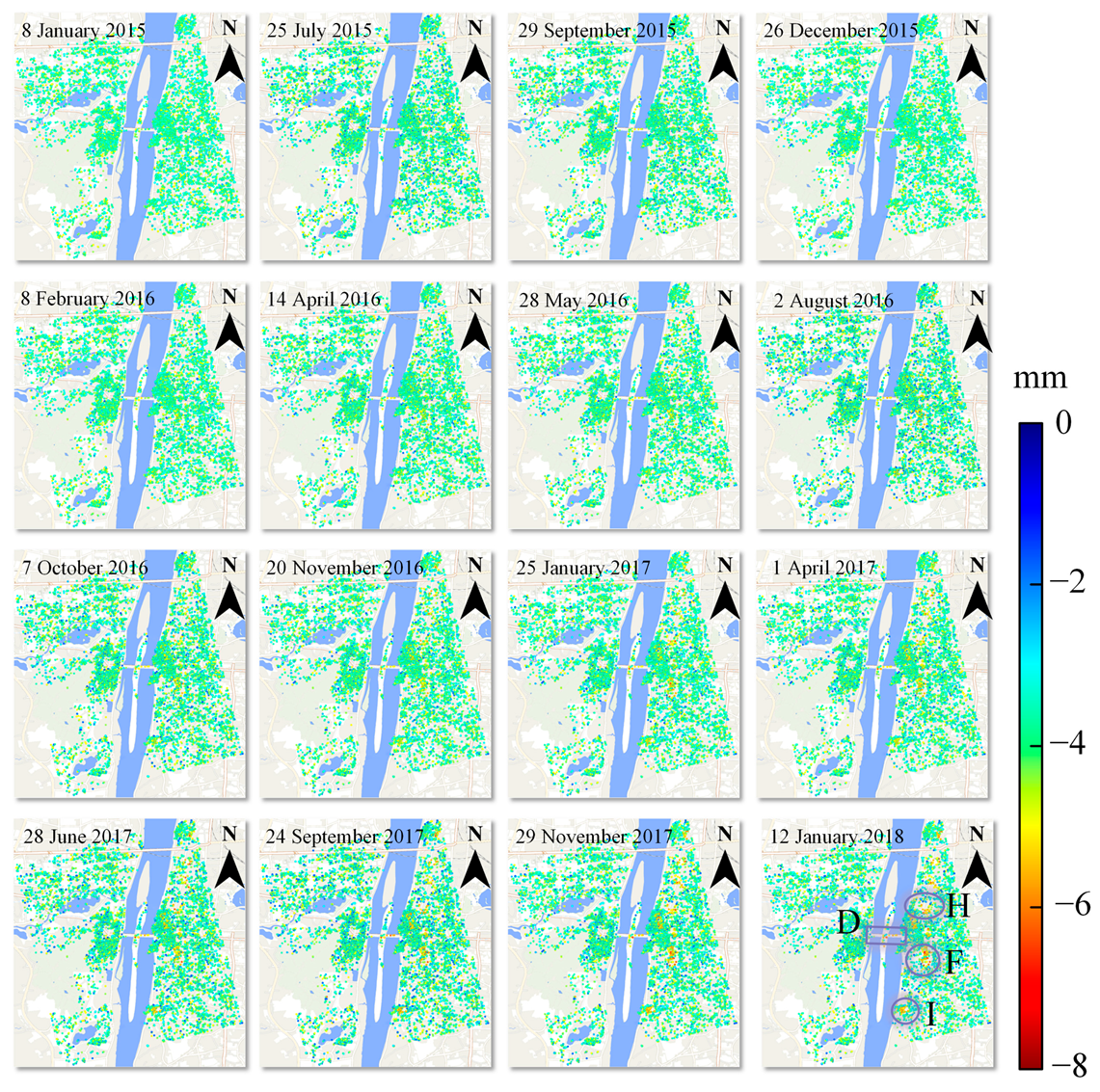
4.2. Time-Series Deformation Estimation Results
5. Analysis and Discussion
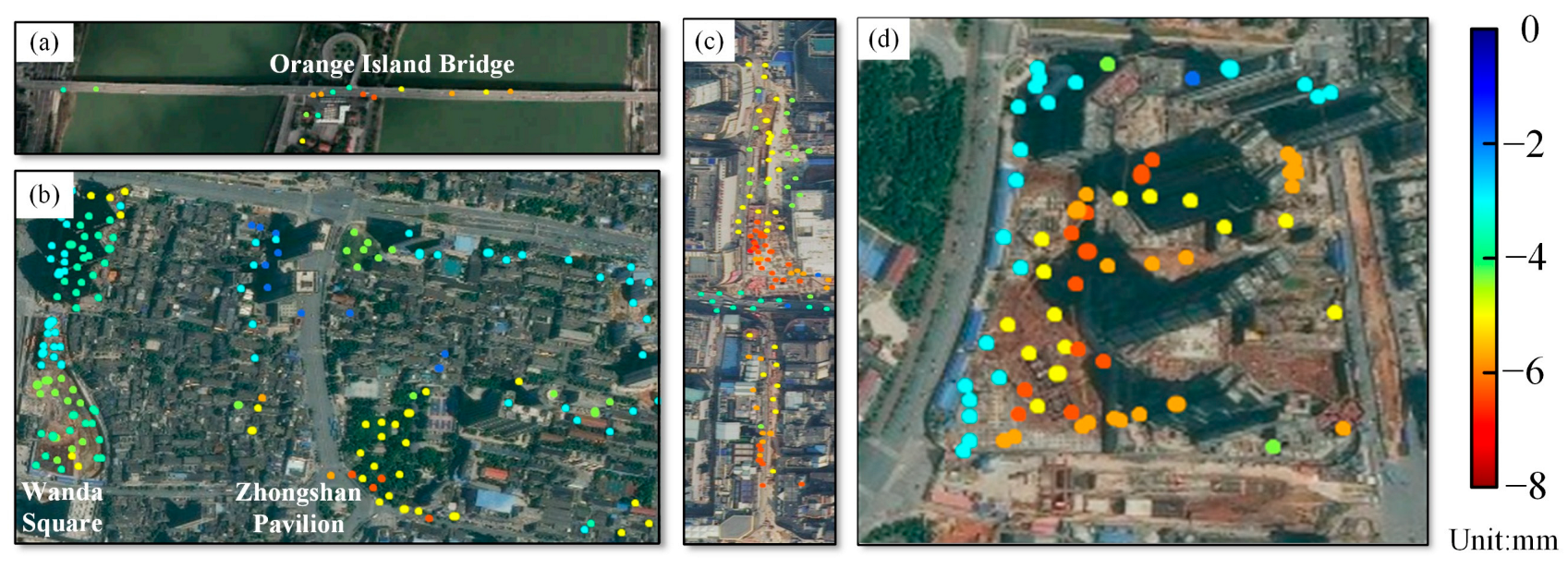
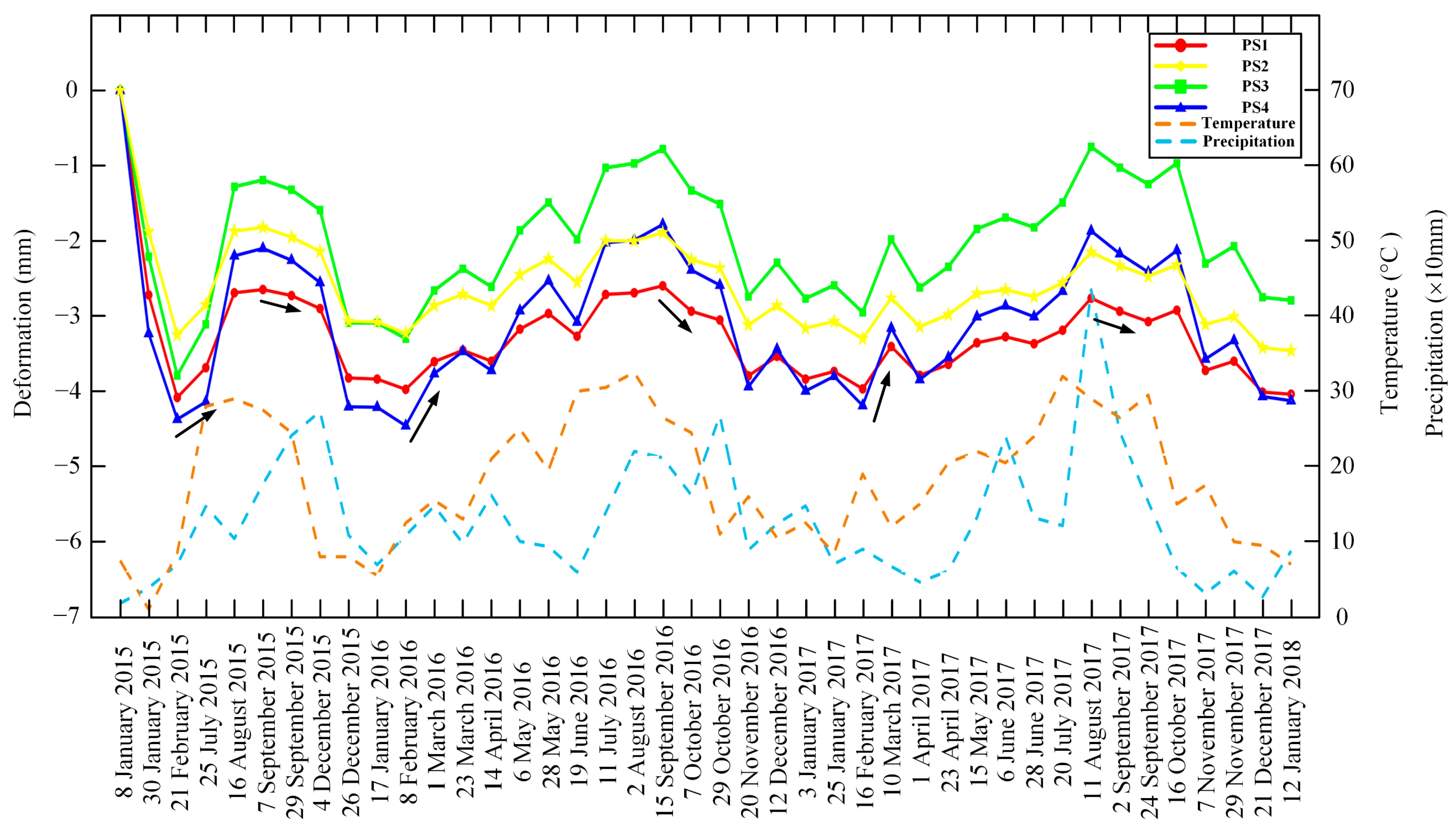
5.1. Analysis of Time-Series Deformation at Feature Points
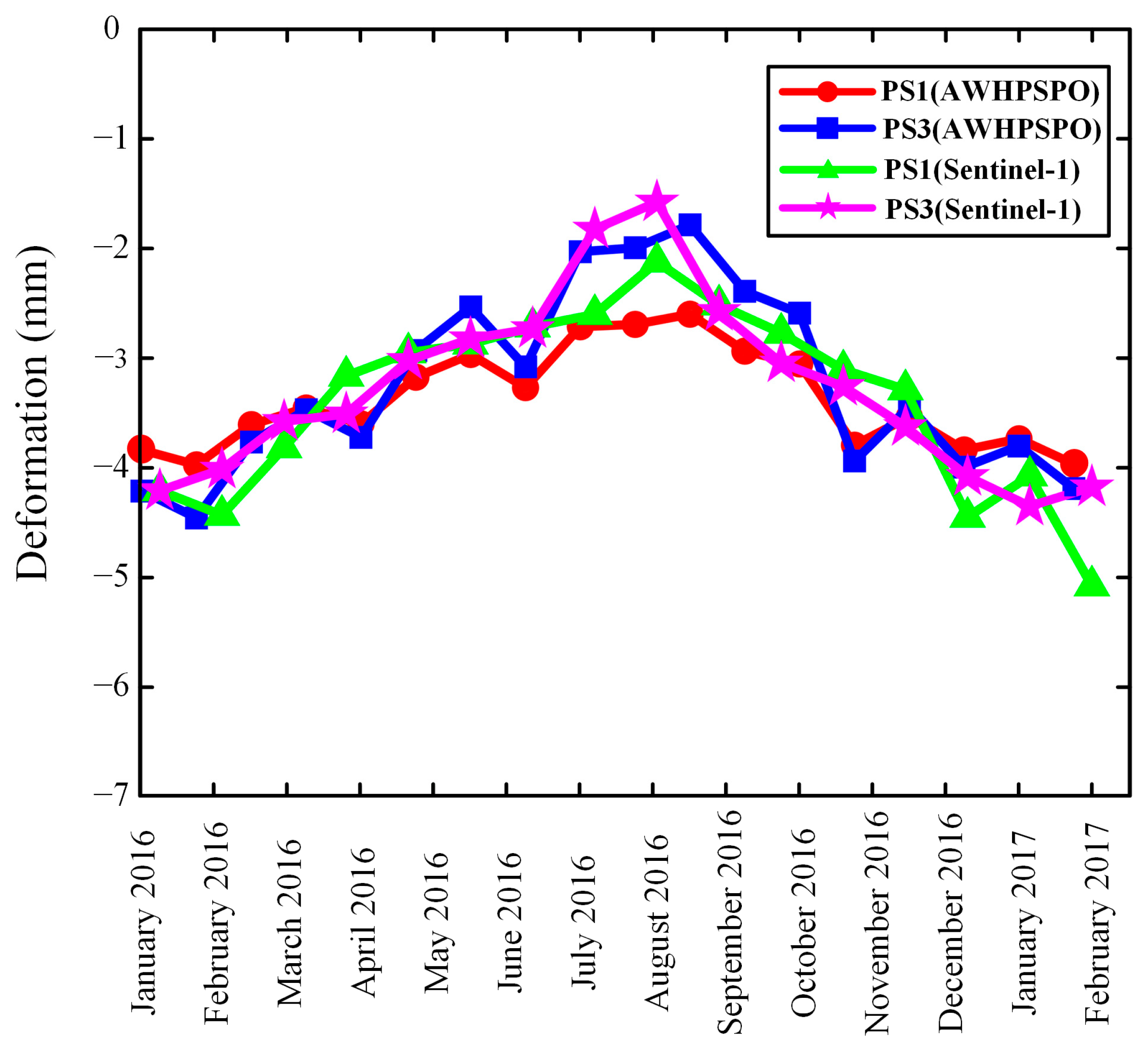
5.2. Accuracy Analysis
5.3. Discussion of AWHPSPO
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, X.; Zhu, C.; He, M.; Dong, M.; Zhang, G.; Zhang, F. Failure Mechanism and Long Short-Term Memory Neural Network Model for Landslide Risk Prediction. Remote Sens. 2021, 14, 166. [Google Scholar] [CrossRef]
- Song, S.; Zhao, M.; Zhu, C.; Wang, F.; Cao, C.; Li, H.; Ma, M. Identification of the Potential Critical Slip Surface for Fractured Rock Slope Using the Floyd Algorithm. Remote Sens. 2022, 14, 1284. [Google Scholar] [CrossRef]
- Hooper, A.; Segall, P.; Zebker, H. Persistent Scatterer Interferometric Synthetic Aperture Radar for Crustal Deformation Analysis, with Application to Volcán Alcedo, Galápagos. J. Geophys. Res. 2007, 112, B07407. [Google Scholar] [CrossRef]
- Zhang, S.; Si, J.; Niu, Y.; Zhu, W.; Fan, Q.; Hu, X.; Zhang, C.; An, P.; Ren, Z.; Li, Z. Surface Deformation of Expansive Soil at Ankang Airport, China, Revealed by InSAR Observations. Remote Sens. 2022, 14, 2217. [Google Scholar] [CrossRef]
- Crosetto, M.; Monserrat, O.; Cuevas-González, M.; Devanthéry, N.; Crippa, B. Persistent Scatterer Interferometry: A Review. ISPRS J. Photogramm. Remote Sens. 2016, 115, 78–89. [Google Scholar] [CrossRef]
- Shen, C.; Feng, Z.; Xie, C.; Fang, H.; Zhao, B.; Ou, W.; Zhu, Y.; Wang, K.; Li, H.; Bai, H.; et al. Refinement of Landslide Susceptibility Map Using Persistent Scatterer Interferometry in Areas of Intense Mining Activities in the Karst Region of Southwest China. Remote Sens. 2019, 11, 2821. [Google Scholar] [CrossRef]
- Ma, P.; Li, T.; Fang, C.; Lin, H. A Tentative Test for Measuring the Sub-Millimeter Settlement and Uplift of a High-Speed Railway Bridge Using COSMO-SkyMed Images. ISPRS J. Photogramm. Remote Sens. 2019, 155, 1–12. [Google Scholar] [CrossRef]
- Zhu, M.; Wan, X.; Fei, B.; Qiao, Z.; Ge, C.; Minati, F.; Vecchioli, F.; Li, J.; Costantini, M. Detection of Building and Infrastructure Instabilities by Automatic Spatiotemporal Analysis of Satellite SAR Interferometry Measurements. Remote Sens. 2018, 10, 1816. [Google Scholar] [CrossRef]
- Dittrich, J.; Hölbling, D.; Tiede, D.; Sæmundsson, Þ. Inferring 2D Local Surface-Deformation Velocities Based on PSI Analysis of Sentinel-1 Data: A Case Study of Öræfajökull, Iceland. Remote Sens. 2022, 14, 3166. [Google Scholar] [CrossRef]
- Li, G.; Ding, Z.; Li, M.; Hu, Z.; Jia, X.; Li, H.; Zeng, T. Bayesian Estimation of Land Deformation Combining Persistent and Distributed Scatterers. Remote Sens. 2022, 14, 3471. [Google Scholar] [CrossRef]
- Jiang, M.; Yong, B.; Tian, X.; Malhotra, R.; Hu, R.; Li, Z.; Yu, Z.; Zhang, X. The Potential of More Accurate InSAR Covariance Matrix Estimation for Land Cover Mapping. ISPRS J. Photogramm. Remote Sens. 2017, 126, 120–128. [Google Scholar] [CrossRef]
- Jänichen, J.; Schmullius, C.; Baade, J.; Last, K.; Bettzieche, V.; Dubois, C. Monitoring of Radial Deformations of a Gravity Dam Using Sentinel-1 Persistent Scatterer Interferometry. Remote Sens. 2022, 14, 1112. [Google Scholar] [CrossRef]
- Li, Y.; Zuo, X.; Zhu, D.; Wu, W.; Yang, X.; Guo, S.; Shi, C.; Huang, C.; Li, F.; Liu, X. Identification and Analysis of Landslides in the Ahai Reservoir Area of the Jinsha River Basin Using a Combination of DS-InSAR, Optical Images, and Field Surveys. Remote Sens. 2022, 14, 6274. [Google Scholar] [CrossRef]
- Liu, Y.; Ma, P.; Lin, H.; Wang, W.; Shi, G. Distributed Scatterer InSAR Reveals Surface Motion of the Ancient Chaoshan Residence Cluster in the Lianjiang Plain, China. Remote Sens. 2019, 11, 166. [Google Scholar] [CrossRef]
- Ferretti, A.; Fumagalli, A.; Novali, F.; Prati, C.; Rocca, F.; Rucci, A. A New Algorithm for Processing Interferometric Data-Stacks: SqueeSAR. IEEE Trans. Geosci. Remote Sens. 2011, 49, 3460–3470. [Google Scholar] [CrossRef]
- Fornaro, G.; Verde, S.; Reale, D.; Pauciullo, A. CAESAR: An Approach Based on Covariance Matrix Decomposition to Improve Multibaseline–Multitemporal Interferometric SAR Processing. IEEE Trans. Geosci. Remote Sens. 2015, 53, 2050–2065. [Google Scholar] [CrossRef]
- Jiang, M.; Guarnieri, A.M. Distributed Scatterer Interferometry with the Refinement of Spatiotemporal Coherence. IEEE Trans. Geosci. Remote Sens. 2020, 58, 3977–3987. [Google Scholar] [CrossRef]
- Shi, G.; Ma, P.; Lin, H.; Huang, B.; Zhang, B.; Liu, Y. Potential of Using Phase Correlation in Distributed Scatterer InSAR Applied to Built Scenarios. Remote Sens. 2020, 12, 686. [Google Scholar] [CrossRef]
- Even, M.; Schulz, K. InSAR Deformation Analysis with Distributed Scatterers: A Review Complemented by New Advances. Remote Sens. 2018, 10, 744. [Google Scholar] [CrossRef]
- Sun, Q.; Jiang, L.; Jiang, M.; Lin, H.; Ma, P.; Wang, H. Monitoring Coastal Reclamation Subsidence in Hong Kong with Distributed Scatterer Interferometry. Remote Sens. 2018, 10, 1738. [Google Scholar] [CrossRef]
- Li, G.; Zhao, C.; Wang, B.; Liu, X.; Chen, H. Land Subsidence Monitoring and Dynamic Prediction of Reclaimed Islands with Multi-Temporal InSAR Techniques in Xiamen and Zhangzhou Cities, China. Remote Sens. 2022, 14, 2930. [Google Scholar] [CrossRef]
- Samiei-Esfahany, S.; Martins, J.E.; van Leijen, F.; Hanssen, R.F. Phase Estimation for Distributed Scatterers in InSAR Stacks Using Integer Least Squares Estimation. IEEE Trans. Geosci. Remote Sens. 2016, 54, 5671–5687. [Google Scholar] [CrossRef]
- Jiang, M.; Miao, Z.; Gamba, P.; Yong, B. Application of Multitemporal InSAR Covariance and Information Fusion to Robust Road Extraction. IEEE Trans. Geosci. Remote Sens. 2017, 55, 3611–3622. [Google Scholar] [CrossRef]
- Jiang, M.; Ding, X.; Hanssen, R.F.; Malhotra, R.; Chang, L. Fast Statistically Homogeneous Pixel Selection for Covariance Matrix Estimation for Multitemporal InSAR. IEEE Trans. Geosci. Remote Sens. 2015, 53, 1213–1224. [Google Scholar] [CrossRef]
- Jiang, M.; Ding, X.; Tian, X.; Malhotra, R.; Kong, W. A Hybrid Method for Optimization of the Adaptive Goldstein Filter. ISPRS J. Photogramm. Remote Sens. 2014, 98, 29–43. [Google Scholar] [CrossRef]
- Jiang, M.; Ding, X.; Li, Z. Hybrid Approach for Unbiased Coherence Estimation for Multitemporal InSAR. IEEE Trans. Geosci. Remote Sens. 2014, 52, 2459–2473. [Google Scholar] [CrossRef]
- Dong, L.; Wang, C.; Tang, Y.; Zhang, H.; Xu, L. Improving CPT-InSAR Algorithm with Adaptive Coherent Distributed Pixels Selection. Remote Sens. 2021, 13, 4784. [Google Scholar] [CrossRef]
- Li, S.; Zhang, S.; Li, T.; Gao, Y.; Zhou, X.; Chen, Q.; Zhang, X.; Yang, C. An Adaptive Weighted Phase Optimization Algorithm Based on the Sigmoid Model for Distributed Scatterers. Remote Sens. 2021, 13, 3253. [Google Scholar] [CrossRef]
- Lin, K.-F.; Perissin, D. Identification of Statistically Homogeneous Pixels Based on One-Sample Test. Remote Sens. 2017, 9, 37. [Google Scholar] [CrossRef]
- Wang, Y.; Deng, Y.; Fei, W.; Wang, R.; Song, H.; Wang, J.; Li, N. Modified Statistically Homogeneous Pixels’ Selection with Multitemporal SAR Images. IEEE Geosci. Remote Sens. Lett. 2016, 13, 1930–1934. [Google Scholar] [CrossRef]
- Kampes, B.M.; Hanssen, R.F. Ambiguity Resolution for Permanent Scatterer Interferometry. IEEE Trans. Geosci. Remote Sens. 2004, 42, 2446–2453. [Google Scholar] [CrossRef]
- Teunissen, P.J.G. The Least-Squares Ambiguity Decorrelation Adjustment: A Method for Fast GPS Integer Ambiguity Estimation. J. Geod. 1995, 70, 65–82. [Google Scholar] [CrossRef]
- Jun, Z.; Lingjie, Z.; Xuemin, X.; Rui, Z.; Liang, B.; Tengfei, Z.; Haodan, B. Joint Estimation Method of Time-Series InSAR Deformation and Environmental Physical Parameters for Soft Clay Area over Dongting Lake. Available online: https://kns.cnki.net/kcms/detail/11.2089.P.20221019.1026.002.html (accessed on 11 December 2022). (In Chinese).
- Qiang, C.; Xiaoli, D.; Guoxiang, L.; Jyrching, H.U.; Linguo, Y. Baseline recognition and parameter estimation of persistent-scatterer network in radar interferometry. Chin. J. Geophys. 2009, 52, 2229–2236. (In Chinese) [Google Scholar] [CrossRef]
- Xuemin, X.; Xiaoli, D.; Jianjun, Z.; Changcheng, W.; Wei, D.; Yafu, Y.; Yongzhe, W. Detecting the regional linear subsidence based on CRInSAR and PSInSAR integration. Chin. J. Geophys. 2011, 54, 1193–1204. (In Chinese) [Google Scholar] [CrossRef]
- Zhu, L.; Xing, X.; Zhu, Y.; Peng, W.; Yuan, Z.; Xia, Q. An Advanced Time-Series InSAR Approach Based on Poisson Curve for Soft Clay Highway Deformation Monitoring. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2021, 14, 7682–7698. [Google Scholar] [CrossRef]
- Bao, L.; Xing, X.; Chen, L.; Yuan, Z.; Liu, B.; Xia, Q.; Peng, W. Time Series Deformation Monitoring over Large Infrastructures around Dongting Lake Using X-Band PSI with a Combined Thermal Expansion and Seasonal Model. J. Sens. 2021, 2021, 6664933. [Google Scholar] [CrossRef]
- Monserrat, O.; Crosetto, M.; Cuevas, M.; Crippa, B. The Thermal Expansion Component of Persistent Scatterer Interferometry Observations. IEEE Geosci. Remote Sens. Lett. 2011, 8, 864–868. [Google Scholar] [CrossRef]
- Jeong, J.-H.; Zollinger, D.G.; Lim, J.-S.; Park, J.-Y. Age and Moisture Effects on Thermal Expansion of Concrete Pavement Slabs. J. Mater. Civ. Eng. 2012, 24, 8–15. [Google Scholar] [CrossRef]
- Yu, Z.; Zhang, F.; Ma, X.; Yang, F.; Hu, D.; Zhou, H. Experimental Study on Thermal Expansion Behavior of Concrete under Three-Dimensional Stress. Adv. Civ. Eng. 2021, 2021, 5597918. [Google Scholar] [CrossRef]
- China Meteorological Administration. Available online: https://www.cma.gov.cn/en2014/ (accessed on 11 December 2022).
- Specification of Time Series InSAR Data Processing for Ground Deformation Monitoring. CH/T 6006-2018. Available online: https://std.samr.gov.cn/hb/search/stdHBDetailed?id=8B1827F241B9BB19E05397BE0A0AB44A (accessed on 11 December 2022).
- Xu, Y.; Li, J.; Wu, L.; Guo, L.; Xu, H. Monitoring ground subsidence in Changsha urban area between 2017–2020 based on SBAS-InSAR. Hydro. Survey Char. 2021, 41, 37–42. (In Chinese) [Google Scholar]

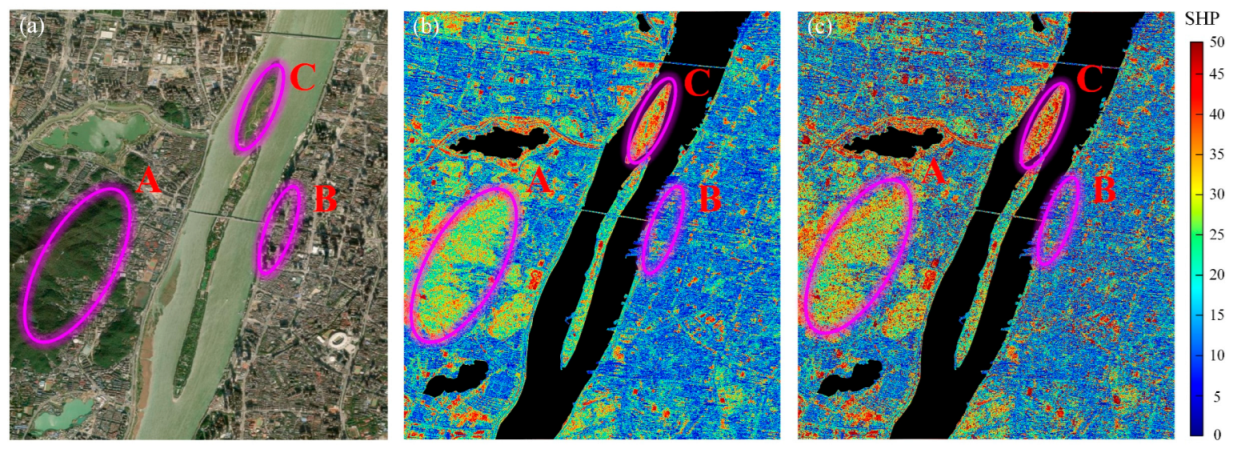


| Average SHP Number Interval for a Pixel | Total Pixel Number of an Image via FaSHPS | Total Pixel Number of an Image via AWHPSPO |
|---|---|---|
| 10 ≤ SHP < 20 * | 1.37 × 107 | 1.43 × 107 |
| 20 ≤ SHP < 30 | 9.45 × 106 | 1.08 × 107 |
| 30 ≤ SHP < 40 | 5.76 × 106 | 7.49 × 106 |
| 40 ≤ SHP < 225 | 3.31 × 106 | 5.01 × 106 |
| Average number of detected SHPs around a pixel | 13 | 17 |
| Indicator | Phase Standard Deviation | Sum of Phase Differences | Percentage of RPs | |||
|---|---|---|---|---|---|---|
| Method | Average Value/Rad | Improvement | Average Value/Rad | Improvement | ||
| Original Interferogram | 1.32 | - | 1.48 | - | 12.18% | |
| Goldstein | 1.11 | 15.9% | 1.22 | 17.6% | 7.94% | |
| Gaussian-weighted | 1.04 | 21.2% | 1.16 | 21.6% | 6.46% | |
| Adaptive Window | 0.84 | 36.4% | 0.86 | 41.9% | 4.31% | |
| Region | Accumulated Subsidence (mm) |
|---|---|
| Area D (Orange Island Bridge area) | −5.5 |
| Area F (area near International Finance Square) | −6.6 |
| Area H (area near Zhongshan Pavilion) | −5.7 |
| Area I (Poly International Plaza) | −5.8 |
| Statistical Items | PS1 | PS3 | ||||||
|---|---|---|---|---|---|---|---|---|
| SS (Sum of Squares) | df (Degrees of Freedom) | MS (Root Mean Square) | F-Statistic | SS (Sum of Squares) | df (Degrees of Freedom) | MS (Root Mean Square) | F-Statistic | |
| Between groups | 0.0001 | 1 | 0.00012 | 0.00026 | 0.0001 | 1 | 0.0001 | 0.00015 |
| Intergroup | 13.7784 | 34 | 0.45928 | - | 20.5425 | 34 | 0.68475 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xing, X.; Zhang, J.; Zhu, J.; Zhang, R.; Liu, B. Slow Deformation Time-Series Monitoring for Urban Areas Based on the AWHPSPO Algorithm and TELM: A Case Study of Changsha, China. Remote Sens. 2023, 15, 1492. https://doi.org/10.3390/rs15061492
Xing X, Zhang J, Zhu J, Zhang R, Liu B. Slow Deformation Time-Series Monitoring for Urban Areas Based on the AWHPSPO Algorithm and TELM: A Case Study of Changsha, China. Remote Sensing. 2023; 15(6):1492. https://doi.org/10.3390/rs15061492
Chicago/Turabian StyleXing, Xuemin, Jihang Zhang, Jun Zhu, Rui Zhang, and Bin Liu. 2023. "Slow Deformation Time-Series Monitoring for Urban Areas Based on the AWHPSPO Algorithm and TELM: A Case Study of Changsha, China" Remote Sensing 15, no. 6: 1492. https://doi.org/10.3390/rs15061492
APA StyleXing, X., Zhang, J., Zhu, J., Zhang, R., & Liu, B. (2023). Slow Deformation Time-Series Monitoring for Urban Areas Based on the AWHPSPO Algorithm and TELM: A Case Study of Changsha, China. Remote Sensing, 15(6), 1492. https://doi.org/10.3390/rs15061492