A Conical Model Approach for Invariant Points of Very Long Baseline Interferometry and Satellite Laser Ranging
Abstract
1. Introduction
2. Local Tie Strategy
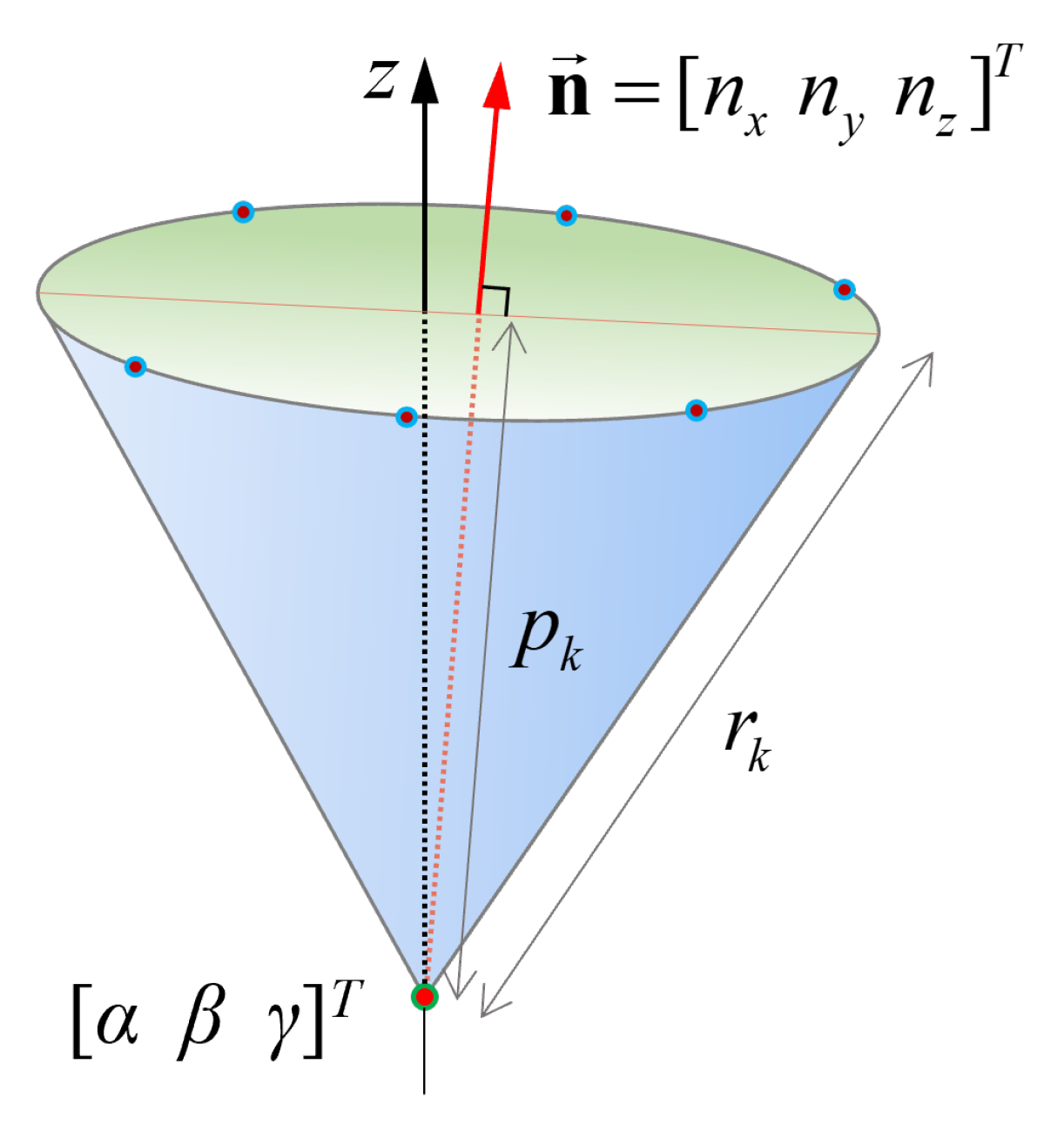
3. The Conical Model
3.1. Parameterization of the IVPs
3.2. Fixed Constraints
3.3. Antenna Offset and Tilting
4. Results and Discussion
4.1. Estimation of the IVP Parameters
4.2. Helmert Transformation to ITRF2014
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| VLBI | Very Long Baseline Interferometry |
| SLR | Satellite Laser Ranging |
| GNSS | Global Navigation Satellite System |
| IVP | InVariant Point |
Appendix A. Linearization of the Model
Appendix B. Antenna Offset and Tilting
Appendix C. The Complete Conical Model
References
- IERS. Available online: https://www.iers.org/IERS/EN/Science/Techniques/techniques.html (accessed on 26 November 2022).
- ITRF. International Terrestrial Reference Frame 2020. Available online: https://itrf.ign.fr/en/solutions/ITRF2020 (accessed on 26 November 2022).
- Altamimi, Z.; Rebischung, P.; Métivier, L.; Collilieux, X. ITRF2014: A new release of the international terrestrial reference frame modeling nonlinear station motions. J. Geophys. Res. Solid Earth 2016, 121, 6109–6131. [Google Scholar] [CrossRef]
- Métivier, L.; Altamimi, Z.; Rouby, H. Past and present ITRF solutions from geophysical perspectives. Adv. Space Res. 2020, 65, 2711–2722. [Google Scholar] [CrossRef]
- Johnston, G.; Riddell, A.; Hausler, G. The International GNSS Service. In Springer Handbook of Global Navigation Satellite Systems, 1st ed.; Teunissen, P.J.G., Montenbruck, O., Eds.; Springer International Publishing: Cham, Switzerland, 2017; pp. 967–982. [Google Scholar]
- Pearlman, M.R.; Noll, C.E.; Pavlis, E.C.; Lemoine, F.G.; Combrink, L.; Degnan, J.J.; Kirchner, G.; Schreiber, U. The ILRS: Approaching 20 years and planning for the future. J. Geod. 2019, 93, 2161–2180. [Google Scholar] [CrossRef]
- Nothnagel, A.; Artz, T.; Behrend, D.; Malkin, Z. International VLBI Service for Geodesy and Astrometry – Delivering high-quality products and embarking on observations of the next generation. J. Geod. 2019, 91, 711–721. [Google Scholar] [CrossRef]
- Willis, P.; Lemoine, F.G.; Moreaux, G.; Soudarin, L.; Ferrage, P.; Ries, J.; Otten, M.; Saunier, J.; Noll, C.; Biancale, R.; et al. The International DORIS Service (IDS), recent developments in preparation for ITRF2013. IAG Symp. Ser. 2016, 143, 631–639. [Google Scholar]
- IERS. Available online: https://itrf.ign.fr/en/network (accessed on 26 November 2022).
- Sarti, P.; Sillard, P.; Vittuari, L. Surveying co-located space-geodetic instruments for ITRF computation. J. Geod. 2004, 78, 210–222. [Google Scholar] [CrossRef]
- Dawson, J.; Sarti, P.; Johnston, G.M.; Vittuari, L. Indirect approach to invariant point determination for SLR and VLBI systems: An assessment. J. Geod. 2007, 81, 433–441. [Google Scholar] [CrossRef]
- Lösler, M. Reference point determination with a new mathematical model at the 20 m VLBI radio telescope in Wettzell. J. Appl. Geod. 2008, 2, 233–238. [Google Scholar] [CrossRef]
- Dawson, J.; Johnston, G.; Twilley, B. he Determination of Telescope and Antenna Invariant Point (IVP). IERS Technical Note No. 33. In Proceedings of the IERS Workshop on Site Co-Location, Matera, Italy, 23–24 October 2003. [Google Scholar]
- Leinen, S.; Becker, M.; Dow, J.; Feltens, J.; Sauermann, K. Geodetic determination of radio telescope antenna reference point and rotation axis parameters. J. Surv. Eng. 2007, 133, 41–51. [Google Scholar] [CrossRef]
- Ning, T.; Haas, R.; Elgered, G. Determination of the local tie vector between the VLBI and GNSS reference points at Onsala using GPS measurements. J. Geod. 2015, 89, 711–723. [Google Scholar] [CrossRef]
- Johnston, G.; Dawson, J.; Naebkhil, S. The 2003 Mount Stromlo Local Tie Survey; Record 2004/20; Geoscicence Australia: Canberra, ACT, Australia, 2004; 26p. [Google Scholar]
- Johnston, G.; Dawson, J. The 2002 Mount Pleasant (Hobart) Radio Telescope Local Tie Survey; Record 2004/21; Geoscicence Australia: Canberra, ACT, Australia, 2004; 21p. [Google Scholar]
- Woods, A.R. Tidbinbilla Local Tie Survey; Record 2007/XX; Geoscicence Australia: Canberra, ACT, Australia, 2007; 23p. [Google Scholar]
- Shen, Y.; You, X.; Wang, J.; Wu, B.; Chen, J.; Ma, X.; Gong, X. Mathematical model for computing precise local tie vectors for CMONOC co-located GNSS/VLBI/SLR stations. Stud Geophys. Geod. 2015, 6, 1–6. [Google Scholar] [CrossRef]
- Ma, X.; Yu, K.; Montillet, J.; He, X. One-step solution to local tie vector determination at co-located GNSS/VLBI sites. J. Geod. 2018, 62, 535–561. [Google Scholar] [CrossRef]
- Bae, T.S.; Hong, C.K. Sphero-conical modeling for the estimation of very long baseline interferometry invariant point. Sensors 2022, 22, 7937. [Google Scholar] [CrossRef] [PubMed]
- Bae, T.-S.; Hong, C.-K.; Lim, S.-H. Total least-squares (TLS) within Gauss-Helmert model: 3D planar fitting and Helmert transformation of geodetic reference frames. J. Korean Soc. Surv. Geod. Photogramm. Cartogr. 2022, 40, 315–324. [Google Scholar]
- Geometric Reference Systems in Geodesy. Available online: https://kb.osu.edu/bitstream/handle/1811/77986/Geom_Ref_Sys_Geodesy_2016.pdf?sequence=1&isAllowed=y (accessed on 16 July 2022).
- Nothnagel, A. Very Long Baseline Interferometry. In Handbuch der Geodäsie; Freeden, W., Rummel, R., Eds.; Springer Reference Naturwissenschaften Book Series; Springer Spektrum: Berlin/Heidelberg, Germany, 2019; pp. 1–58. [Google Scholar]
- Snow, K. Applications of Parameter Estimation and Hypothesis Testing to GPS Network Adjustments. Master’s Thesis, The Ohio State University, Columbus, OH, USA, 2002. [Google Scholar]
- Snow, K. Topics in Total Least-Squares Adjustment within the Errors-In-Variables Model: Singular Cofactor Matrices and Prior Information. Ph.D. Thesis, The Ohio State University, Columbus, OH, USA, 2012. [Google Scholar]
- Yoo, S.-M.; Jung, T.; Lee, S.-M.; Yoon, H.S.; Park, H.-E.; Chung, J.-K.; Wi, S.O.; Cho, J.; Byun, D.-Y. Determination of the invariant point of Korean VLBI network (KVN) radio telescopes: First results at KVN Ulsan and Tamna observatories. J. Korean Astron. Soc. 2018, 51, 143–153. [Google Scholar]
- Watson, G.A. Computing Helmert transformations. J. Comput. Appl. Math. 2006, 197, 387–394. [Google Scholar] [CrossRef]
- Ioannidou, S.; Pantazis, G. Helmert transformation problem. From Euler angles method to quaternion algebra. Int. J.-Geo-Inf. 2020, 9, 494. [Google Scholar] [CrossRef]
- Fang, X. Weighted total least squares: Necessary and sufficient conditions, fixed and random parameters. J. Geod. 2013, 87, 733–749. [Google Scholar] [CrossRef]
- Fang, X. Weighted total least-squares with constraints: A universal formula for geodetic symmetrical transformations. J. Geod. 2015, 89, 459–469. [Google Scholar] [CrossRef]
- Ghilani, C.D.; Wolf, P.R. Adjustment Computations: Spatial Data Analysis, 4th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2006. [Google Scholar]
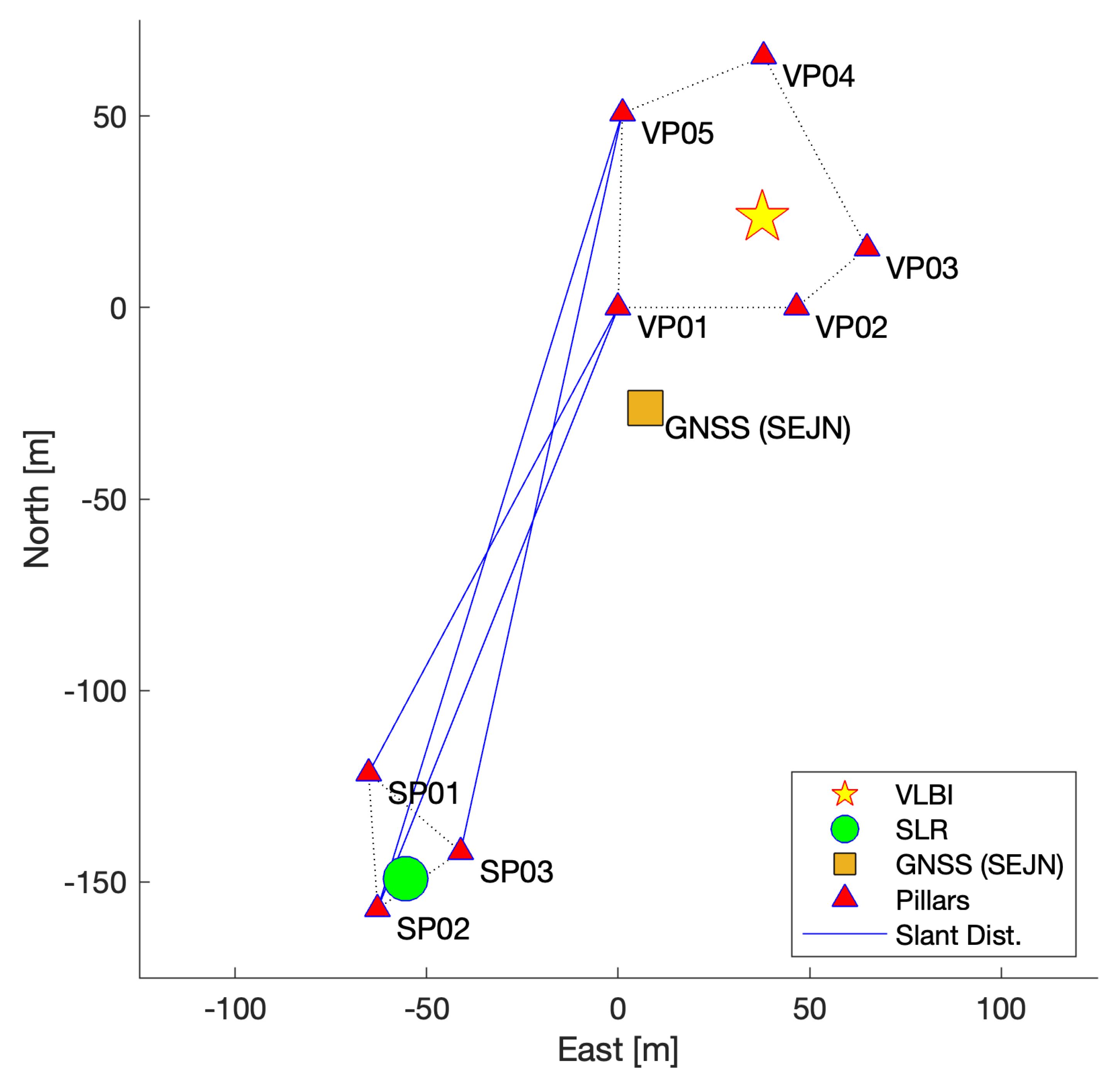



| Category | # Points | # Unknowns | ||
|---|---|---|---|---|
| IVP | VLBI | − | 3 | |
| − | 11 | |||
| − | 9 | |||
| h | − | 1 | ||
| − | 3 | |||
| − | 11 | |||
| − | 38 | |||
| SLR | − | 3 | ||
| − | 2 | |||
| − | 6 | |||
| h | − | 1 | ||
| − | 3 | |||
| − | 2 | |||
| − | 17 | |||
| Pillars | VLBI | 5 | 15 | |
| SLR | 3 | 9 | ||
| GNSS | 1 | 3 | ||
| 9 | 27 | |||
| Targets | VLBI | Horizontal | 208 | 624 |
| Vertical | 716 | 2148 | ||
| 924 | 2772 | |||
| SLR | Horizontal | 72 | 216 | |
| Vertical | 1201 | 3603 | ||
| 1273 | 3819 | |||
| Total | 6673 | |||
| Items | Conventional | Conical |
|---|---|---|
| IVP coordinates (): | ||
| Vertical coord. () | k | 1 |
| Horizontal coord. () | 2 | |
| Normal vector | 3 | |
| Distance to plane from IVP | n/a | k |
| Horizontal offset | n/a | 1 |
| Radius of circle | n/a | |
| Slant height of cone | n/a | |
| Total |
| Items | Values |
|---|---|
| Observation types: | |
| slant distance | 4089 |
| horizontal angle | 3406 |
| vertical angle | 4081 |
| height difference | 8 |
| Total unknown parameters (m) | 6673 |
| Observations (n) | 11,584 |
| Rank of A (q) | 6614 |
| Fix constraints (l) | 2479 |
| Datum deficiency () | 59 |
| Model redundancy () | 7449 |
| Convergence criterion () | 5.00 mm |
| Convergence criterion () | 0.50 mm |
| Number of iterations | 6 |
| Estimated variance component | 5.4186 |
| Norm of coordinate changes | 0.3093 |
| VLBI | SLR | |||||||
|---|---|---|---|---|---|---|---|---|
| Iteration# | ||||||||
| Initial | 37.6000 | 23.5000 | 194.0000 | −55.5000 | −149.0000 | 176.6000 | 0.0000 | n/a |
| 1 | 37.6301 | 23.4875 | 194.5947 | −55.4437 | −149.1018 | 176.4693 | 0.6208 | 5.5792 |
| 2 | 37.6303 | 23.4873 | 194.5953 | −55.4436 | −149.1019 | 176.4682 | 0.0012 | 5.3937 |
| 3 | 37.6303 | 23.4873 | 194.5953 | −55.4434 | −149.1019 | 176.4684 | 0.0002 | 5.4162 |
| 4 | 37.6303 | 23.4873 | 194.5953 | −55.4439 | −149.1019 | 176.4678 | 0.0008 | 5.4183 |
| 5 | 37.6303 | 23.4873 | 194.5953 | −55.4432 | −149.1018 | 176.4686 | 0.0011 | 5.4186 |
| 6 | 37.6303 | 23.4873 | 194.5953 | −55.4432 | −149.1020 | 176.4687 | 0.0002 | 5.4186 |
| Items | VLBI (m) | SLR (m) |
|---|---|---|
| IVP coordinates | ||
| 37.6303 ± 0.0004 | −55.4432 ± 0.0007 | |
| 23.4873 ± 0.0004 | −149.1020 ± 0.0008 | |
| 194.5953 ± 0.0007 | 176.4687 ± 0.0010 | |
| Slant heights of cones (H targets), | ||
| #01 | 5.3402 ± 0.0006 | 0.6207 ± 0.0006 |
| #02 | 5.3425 ± 0.0006 | 0.5959 ± 0.0006 |
| #03 | 5.3349 ± 0.0006 | n/a |
| #04 | 5.3344 ± 0.0006 | n/a |
| #05 | 4.2934 ± 0.0006 | n/a |
| #06 | 4.2950 ± 0.0006 | n/a |
| #07 | 4.2978 ± 0.0007 | n/a |
| #08 | 4.7499 ± 0.0011 | n/a |
| #09 | 4.7525 ± 0.0012 | n/a |
| #10 | 6.0082 ± 0.0009 | n/a |
| #11 | 6.0161 ± 0.0009 | n/a |
| Slant heights of cones (V targets), | ||
| #01 | 5.3350 ± 0.0005 | n/a |
| #02 | 5.3326 ± 0.0008 | n/a |
| #03 | 5.3421 ± 0.0006 | 0.6493 ± 0.0006 |
| #04 | 5.3461 ± 0.0008 | 0.6540 ± 0.0007 |
| #05 | 4.3049 ± 0.0008 | 0.8032 ± 0.0006 |
| #06 | 4.2936 ± 0.0004 | 0.7868 ± 0.0007 |
| #07 | 4.2904 ± 0.0005 | 0.6153 ± 0.0006 |
| #08 | n/a | 0.5936 ± 0.0006 |
| #12 | 3.9991 ± 0.0007 | n/a |
| #13 | 4.0063 ± 0.0008 | n/a |
| Horizontal offset at azimuth , h | 0.0120 ± 0.0008 | 0.0033 ± 0.0005 |
| Normal vector for horizontal planes | (no unit) | (no unit) |
| −0.0002 ± 0.0001 | 0.0005 ± 0.0009 | |
| 0.0006 ± 0.0001 | 0.0008 ± 0.0009 | |
| 1.0000 ± 0.0000 | 1.0000 ± 0.0000 | |
| Distances to planes from IVP, | ||
| #01 | 1.8312 ± 0.0009 | 0.2065 ± 0.0008 |
| #02 | 1.8287 ± 0.0009 | 0.2052 ± 0.0008 |
| #03 | 1.8211 ± 0.0008 | n/a |
| #04 | 1.8198 ± 0.0008 | n/a |
| #05 | 1.8326 ± 0.0008 | n/a |
| #06 | 1.8350 ± 0.0008 | n/a |
| #07 | 1.8327 ± 0.0009 | n/a |
| #08 | − 0.0033 ± 0.0013 | n/a |
| #09 | − 0.0034 ± 0.0013 | n/a |
| #10 | − 3.1290 ± 0.0011 | n/a |
| #11 | − 3.1361 ± 0.0011 | n/a |
| PID | x(m) | y(m) | z(m) | (mm) | (mm) | (mm) |
|---|---|---|---|---|---|---|
| IVP (VLBI) | 37.6303 | 23.4873 | 194.5953 | 0.4 | 0.4 | 0.7 |
| IVP (SLR) | −55.4432 | −149.1020 | 176.4687 | 0.7 | 0.8 | 1.0 |
| SEJN (GNSS) | 7.1613 | −26.3158 | 181.1962 | 2.6 | 3.4 | 1.2 |
| VP01 | −0.0019 | −0.0020 | 177.9101 | 0.4 | 0.5 | 0.4 |
| VP02 | 46.5320 | −0.0018 | 180.8347 | 0.4 | 0.5 | 0.4 |
| VP03 | 64.9506 | 15.4718 | 180.9519 | 0.4 | 0.5 | 0.4 |
| VP04 | 38.0174 | 65.5481 | 180.5847 | 0.5 | 0.5 | 0.4 |
| VP05 | 1.0543 | 50.7583 | 185.5924 | 0.4 | 0.5 | 0.4 |
| SP01 | −65.1534 | −121.5984 | 173.9717 | 0.7 | 0.8 | 0.8 |
| SP02 | −62.8026 | −156.9983 | 179.3956 | 0.7 | 0.8 | 0.8 |
| SP03 | −41.1289 | −142.0844 | 181.3180 | 0.6 | 0.8 | 0.8 |
| PH01_000_90 | 42.0240 | 25.9084 | 196.4260 | 1.7 | 2.8 | 0.8 |
| PH01_015_90 | 42.5024 | 24.6813 | 196.4269 | 1.0 | 2.9 | 0.8 |
| PH01_030_90 | 42.6450 | 23.3734 | 196.4277 | 0.7 | 2.9 | 0.8 |
| PH01_045_90 | 42.4428 | 22.0740 | 196.4284 | 1.0 | 2.7 | 0.8 |
| ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ | ⋮ |
| SV08_340_10 | −55.5786 | −149.6105 | 176.7379 | 4.5 | 2.5 | 4.2 |
| SV08_340_20 | −55.5759 | −149.6499 | 176.6459 | 4.5 | 1.9 | 4.5 |
| SV08_340_30 | −55.5741 | −149.6726 | 176.5488 | 4.5 | 1.5 | 4.7 |
| Parameter | Value | Sigma | |
|---|---|---|---|
| Translation (mm) | 1.45181 | 0.264 | |
| −11.99716 | 0.286 | ||
| −4.22572 | 0.274 | ||
| Rotation (arcsec) | −22.53129 | 0.782 | |
| 3.63510 | 2.245 | ||
| −3.83825 | 0.633 | ||
| Scale (ppm) | s | −119.39539 | 3.175 |
| Total unknown parameters (m) | 7 | ||
| No. observations (n) | 27 | ||
| Model redundancy () | 20 | ||
| Estimated variance component | 1.19570 | ||
| Local Frame | ITRF2014 | ||||||
|---|---|---|---|---|---|---|---|
| Station | (mm) | (mm) | (mm) | (mm) | (mm) | (mm) | |
| VLBI | VP01 | −0.012 | 0.006 | 0.005 | 0.016 | −0.003 | −0.007 |
| VP02 | −0.005 | 0.024 | 0.056 | 0.008 | −0.021 | −0.061 | |
| VP03 | −0.005 | 0.032 | 0.057 | 0.004 | −0.024 | −0.070 | |
| VP04 | 0.006 | 0.021 | 0.027 | −0.013 | −0.036 | −0.054 | |
| VP05 | −0.008 | 0.014 | −0.017 | 0.013 | −0.014 | 0.021 | |
| SLR | SP01 | −0.390 | 0.341 | 0.677 | 0.118 | −0.071 | −0.191 |
| SP02 | 0.040 | −0.908 | −0.710 | 0.017 | 0.234 | 0.201 | |
| SP03 | 0.175 | 1.132 | 0.417 | −0.107 | −0.301 | −0.132 | |
| GNSS | SEJN | 2.209 | −12.118 | −1.774 | −0.052 | 0.148 | 0.192 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bae, T.-S.; Hong, C.-K. A Conical Model Approach for Invariant Points of Very Long Baseline Interferometry and Satellite Laser Ranging. Remote Sens. 2023, 15, 806. https://doi.org/10.3390/rs15030806
Bae T-S, Hong C-K. A Conical Model Approach for Invariant Points of Very Long Baseline Interferometry and Satellite Laser Ranging. Remote Sensing. 2023; 15(3):806. https://doi.org/10.3390/rs15030806
Chicago/Turabian StyleBae, Tae-Suk, and Chang-Ki Hong. 2023. "A Conical Model Approach for Invariant Points of Very Long Baseline Interferometry and Satellite Laser Ranging" Remote Sensing 15, no. 3: 806. https://doi.org/10.3390/rs15030806
APA StyleBae, T.-S., & Hong, C.-K. (2023). A Conical Model Approach for Invariant Points of Very Long Baseline Interferometry and Satellite Laser Ranging. Remote Sensing, 15(3), 806. https://doi.org/10.3390/rs15030806







