A New Strategy for Extracting 3D Deformation of Mining Areas from a Single-Geometry Synthetic Aperture Radar Dataset
Abstract
:1. Introduction
2. Experimental Principle
2.1. Brief Review of the SIP-Based Method
2.2. Limitations of the SIP-Based Method
2.2.1. Directionality of Horizontal Displacement
2.2.2. Inclination of Coal Seam
2.2.3. The Stability of the RSIP/SIP-Based Method
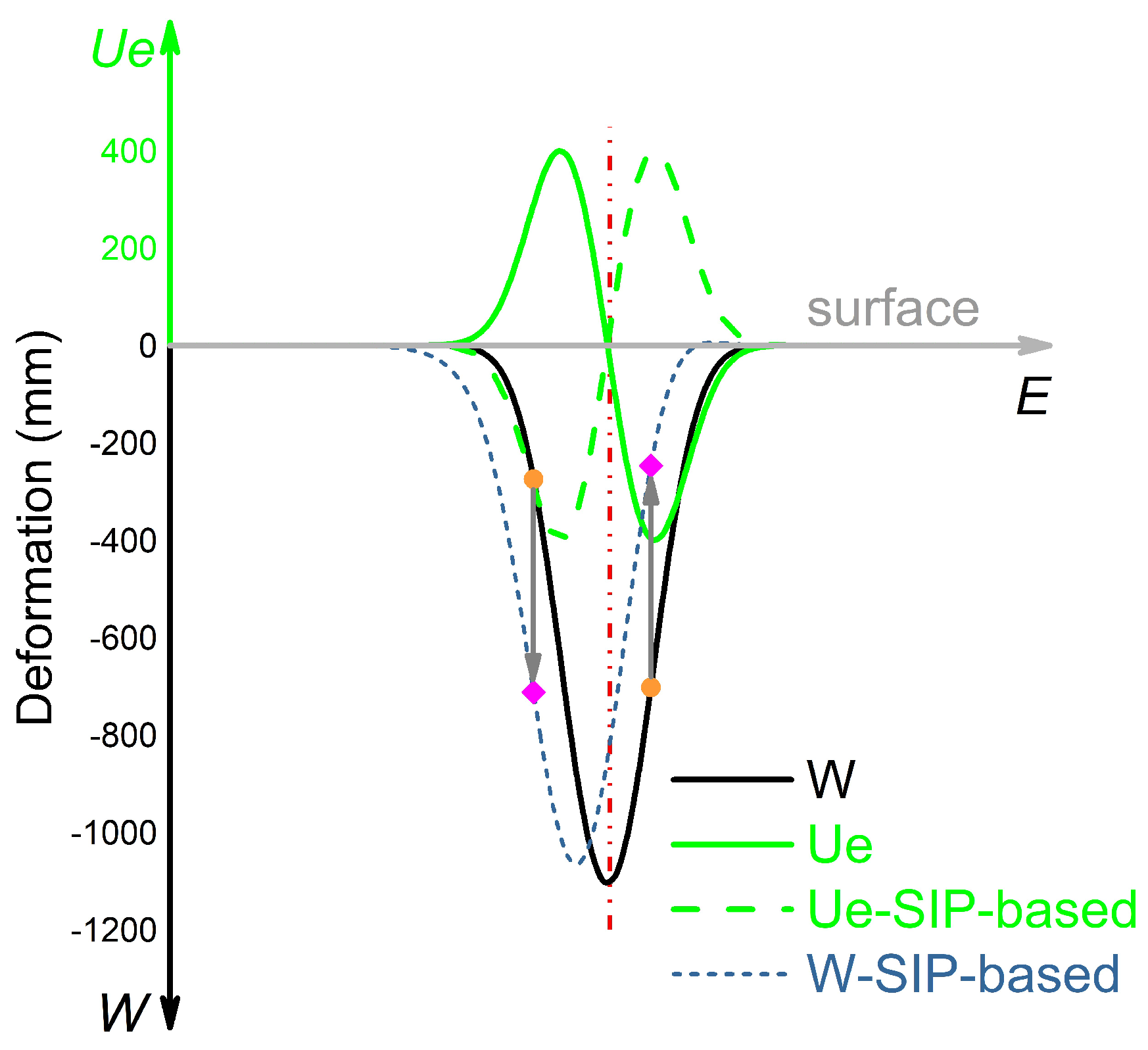
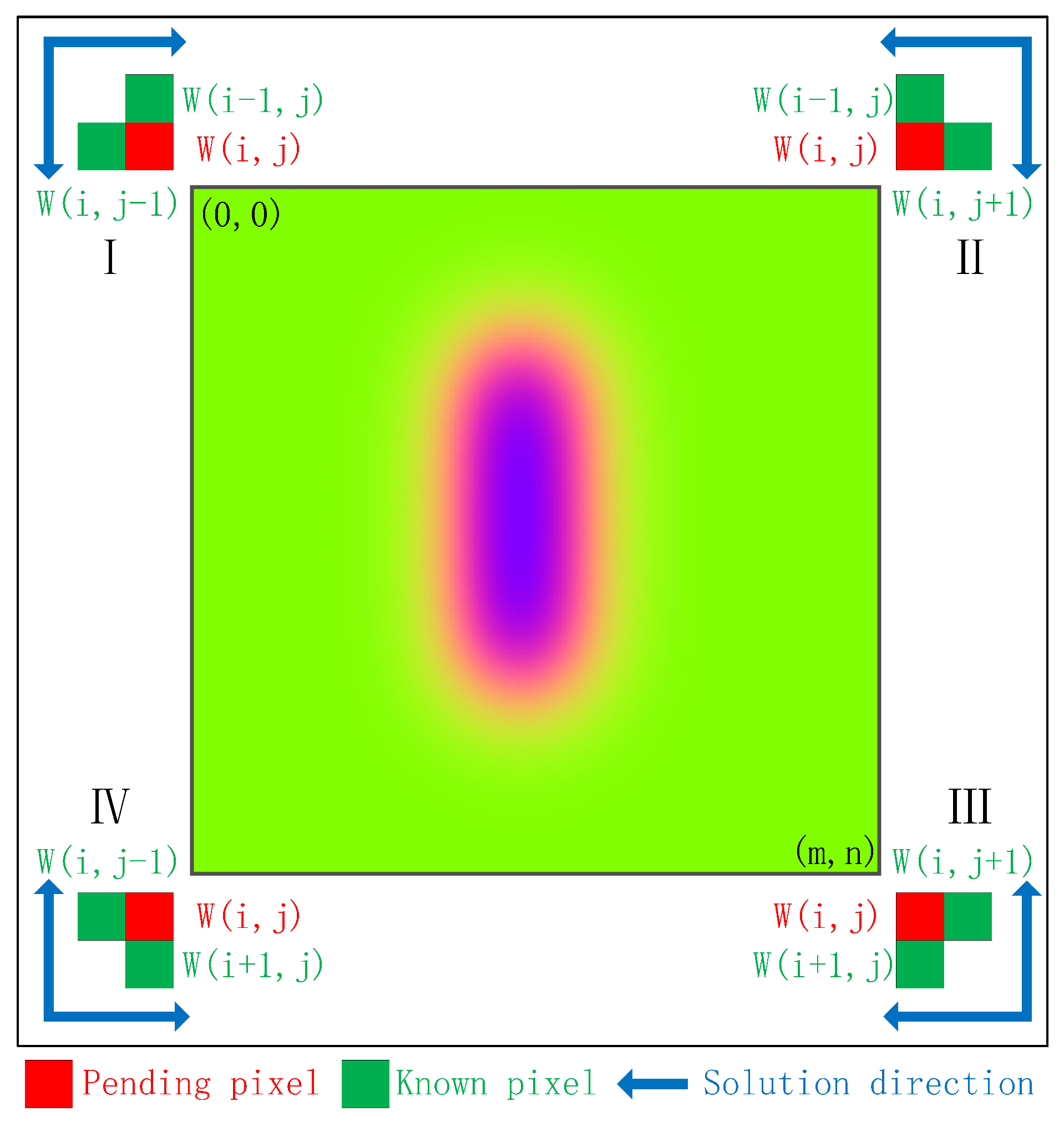
2.3. Solution Strategy of the RSIP-Based Method
3. Simulation and Real Data Tests
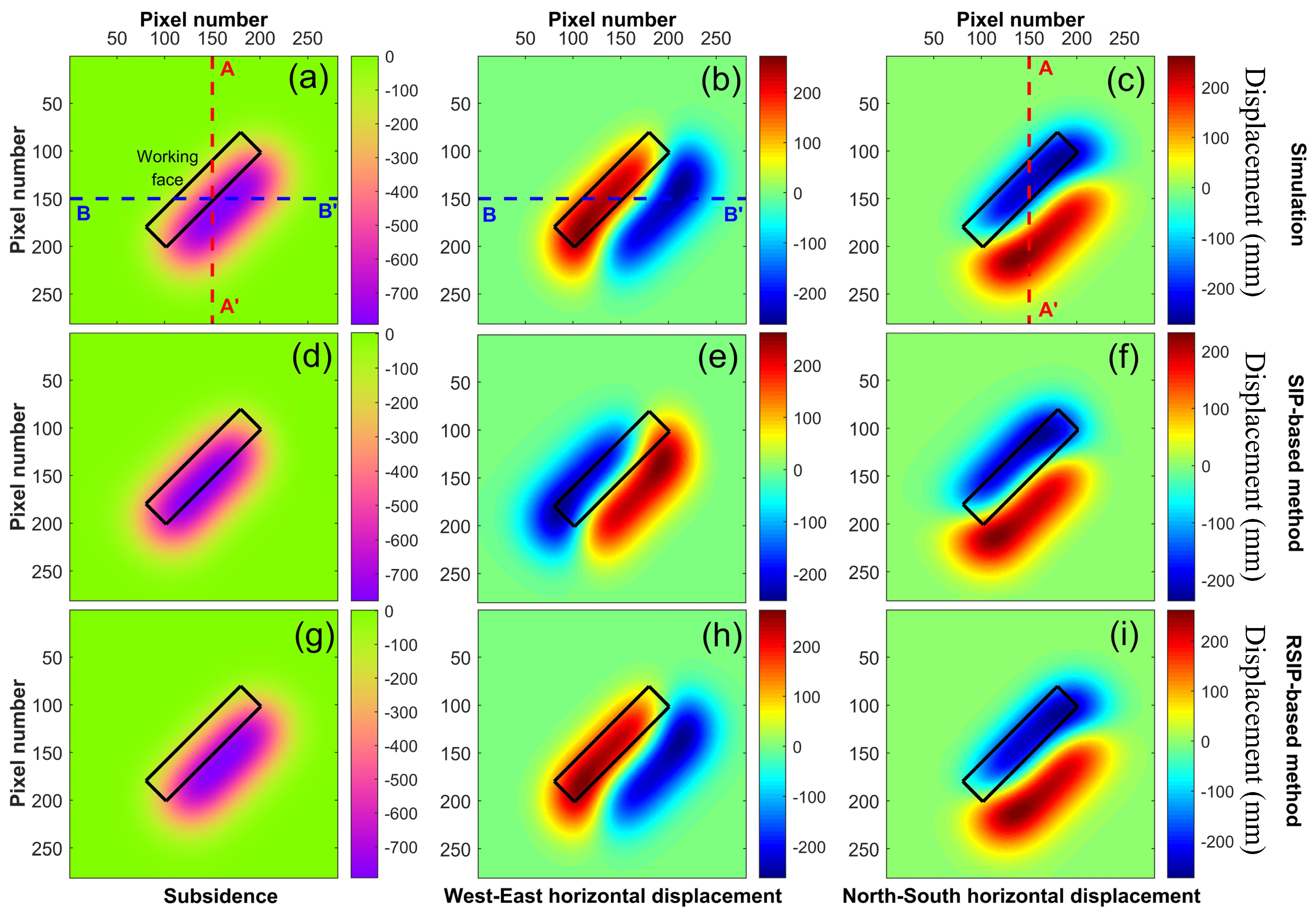
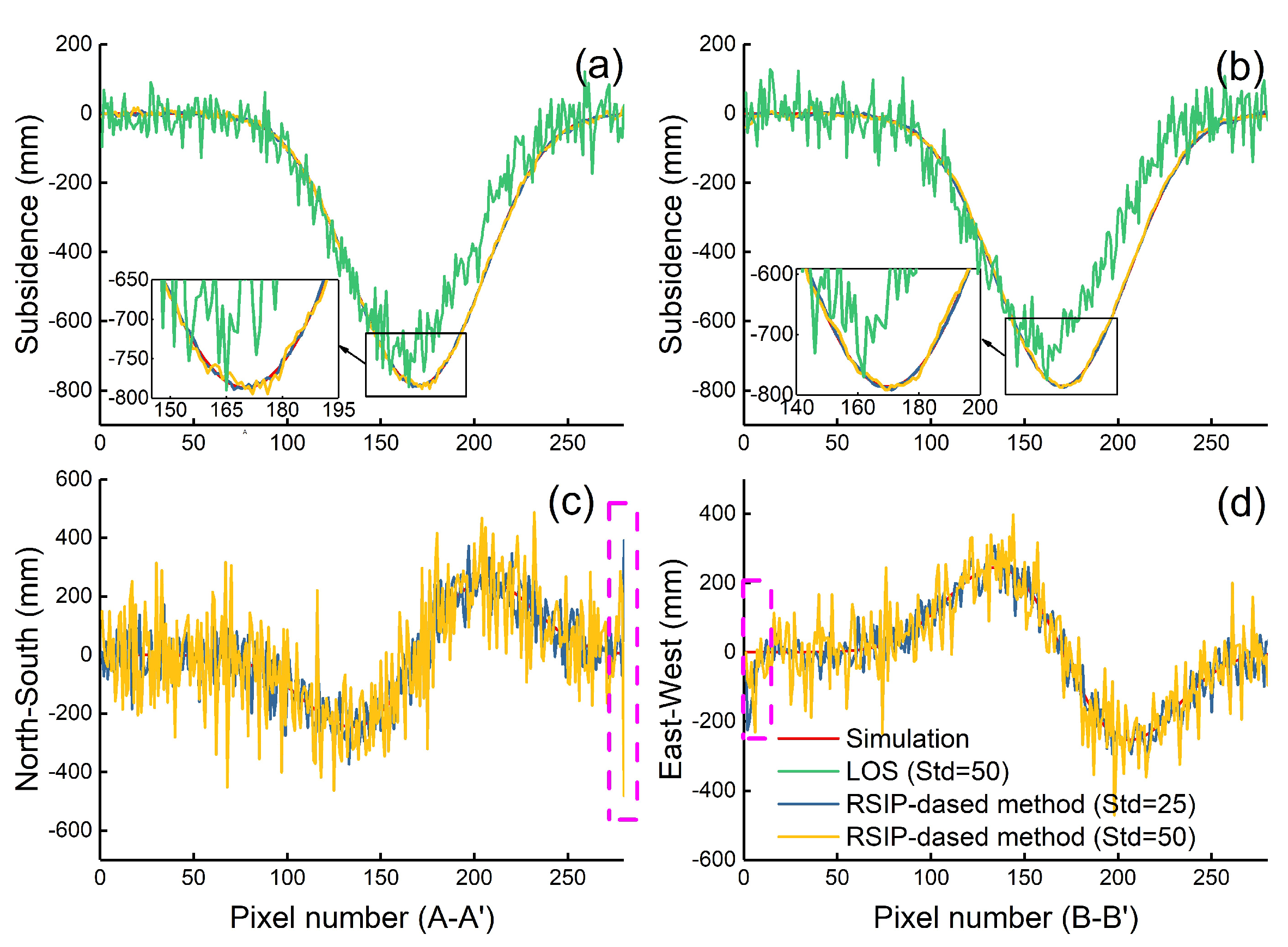
3.1. Simulation Experiment
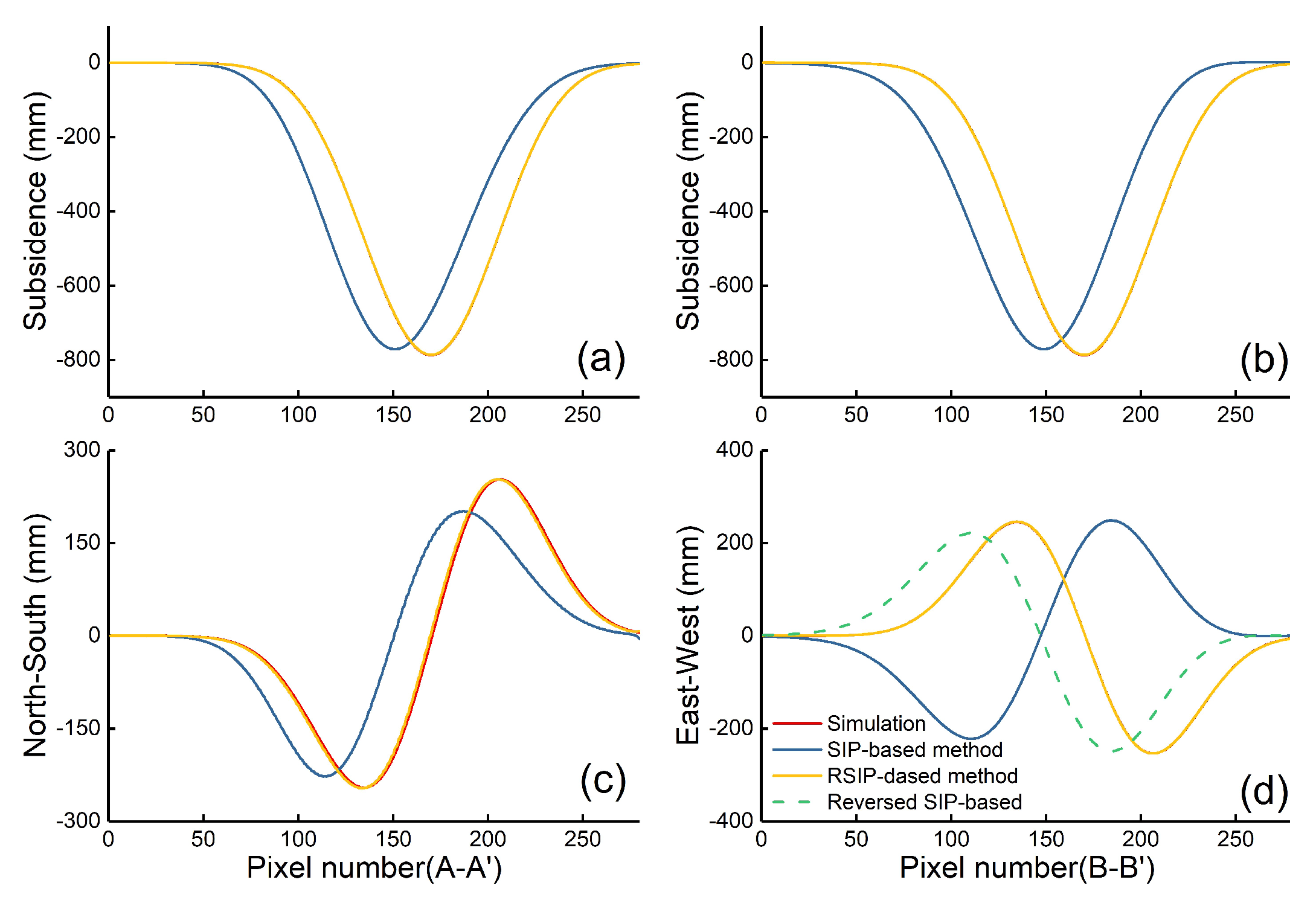
3.1.1. Simulation Experiment without Noise
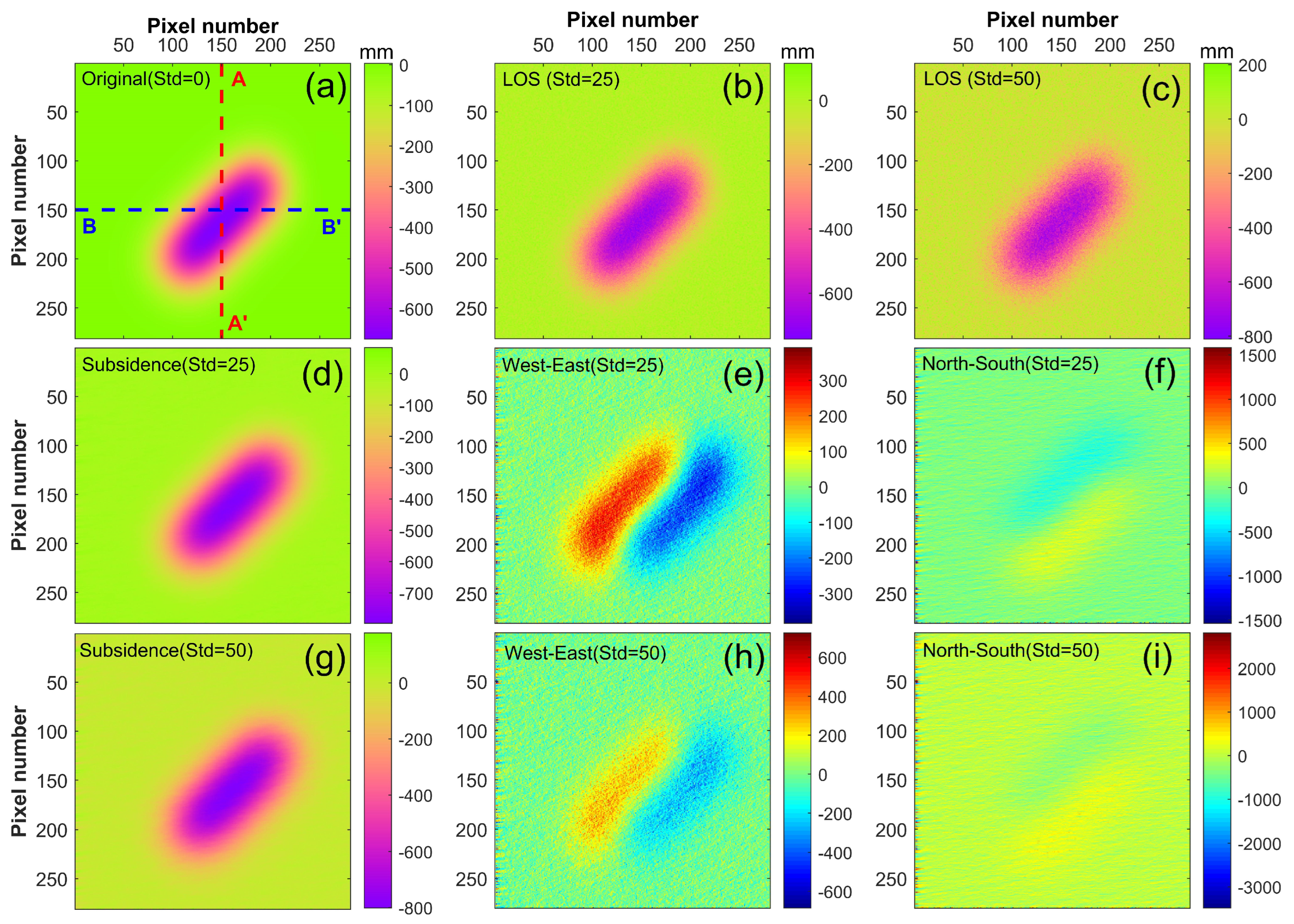
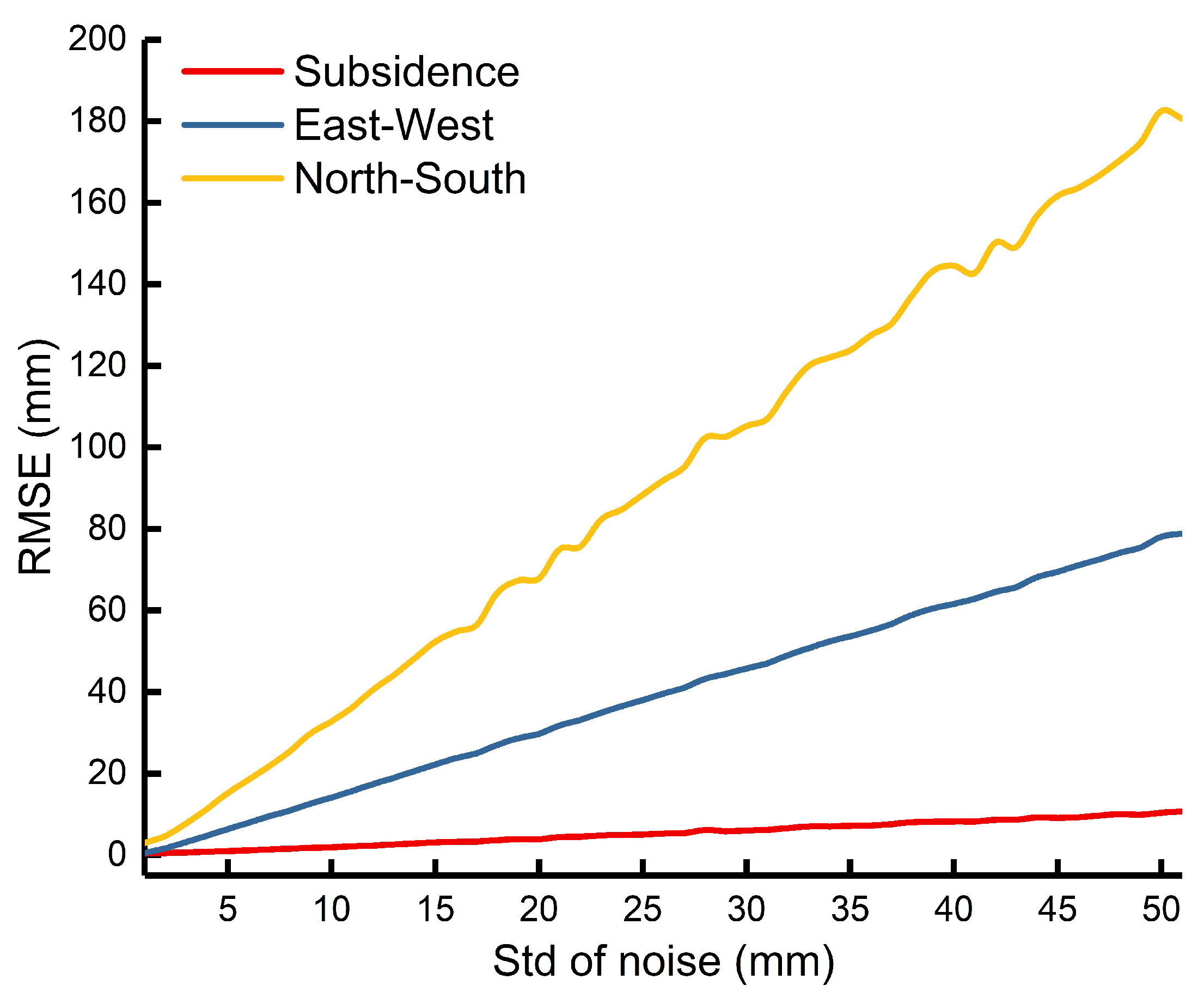
3.1.2. Simulation Experiment with Noise
3.2. Real Experiment
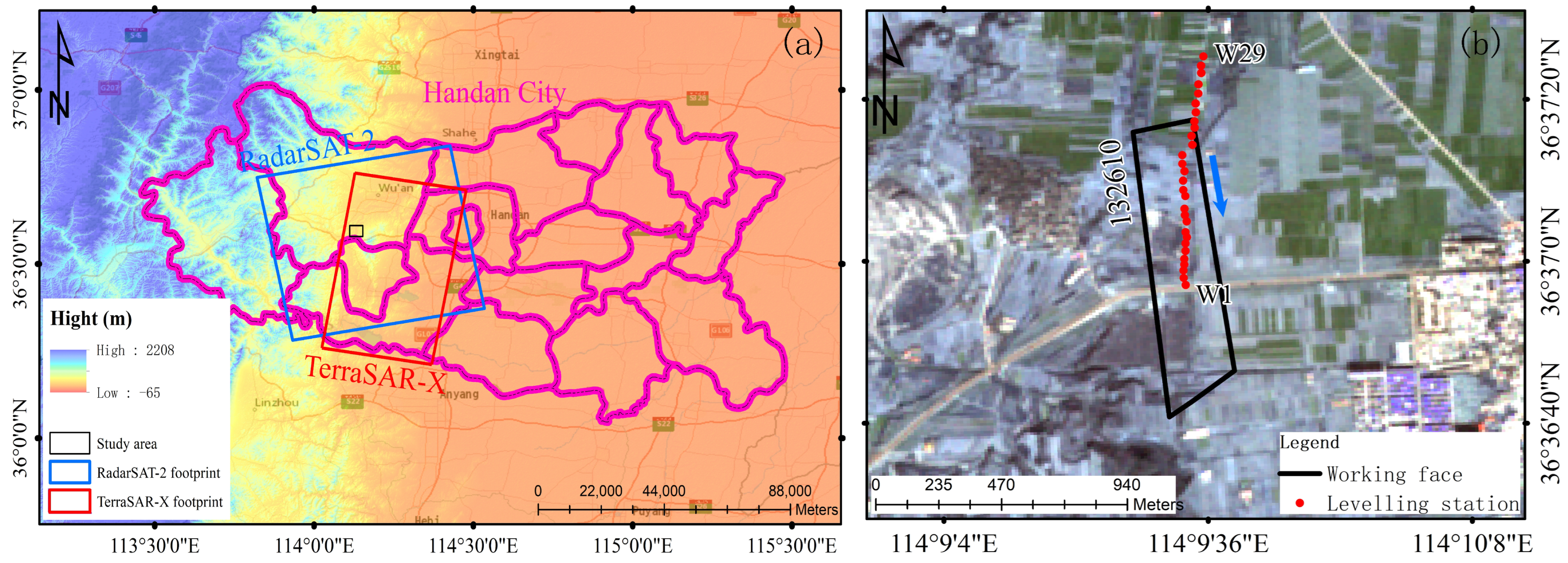
3.2.1. Study Area and Datasets
3.2.2. Data Processing
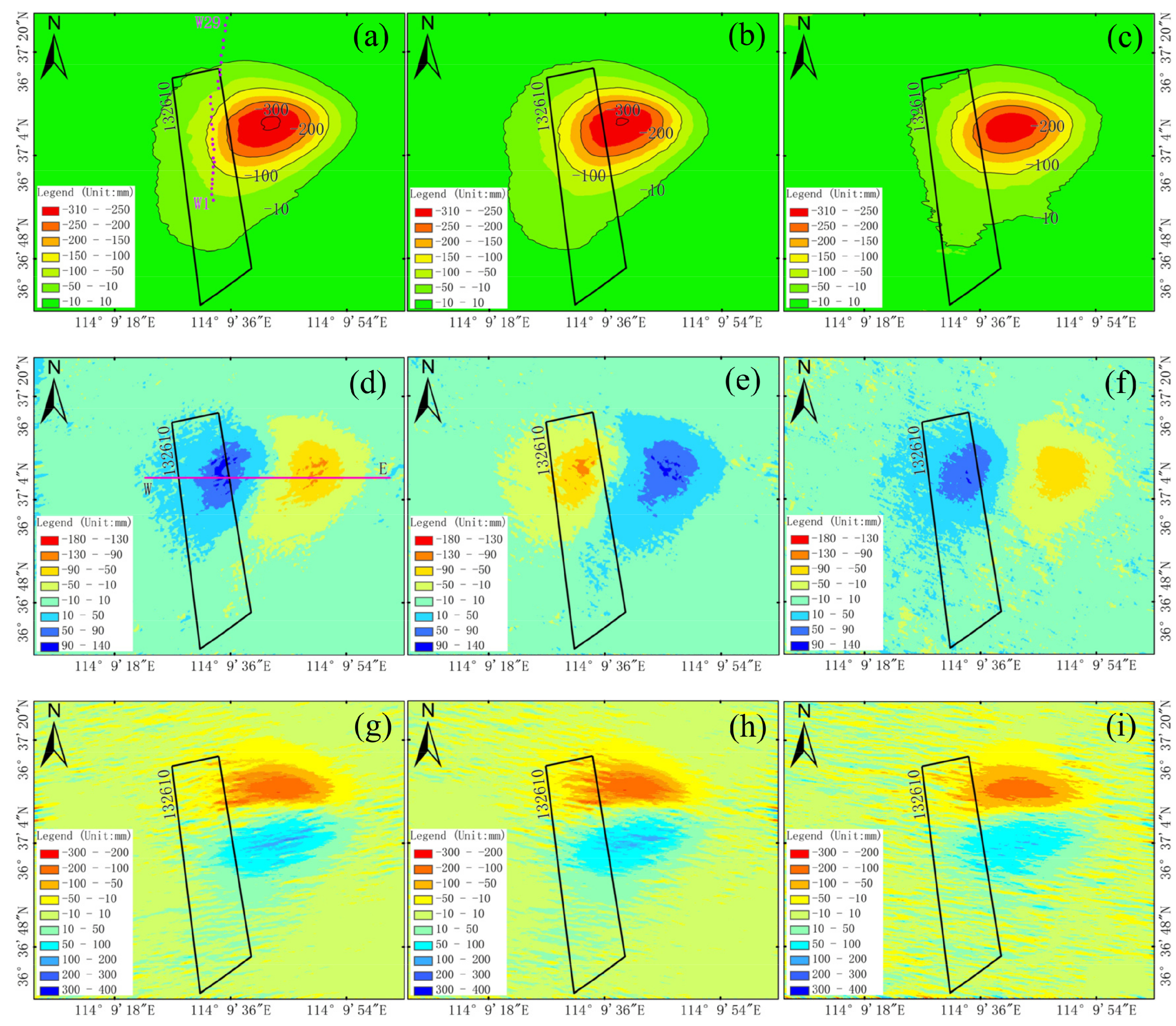
3.2.3. Estimating 3D Displacement Fields
3.2.4. Accuracy Evaluation
4. Discussion
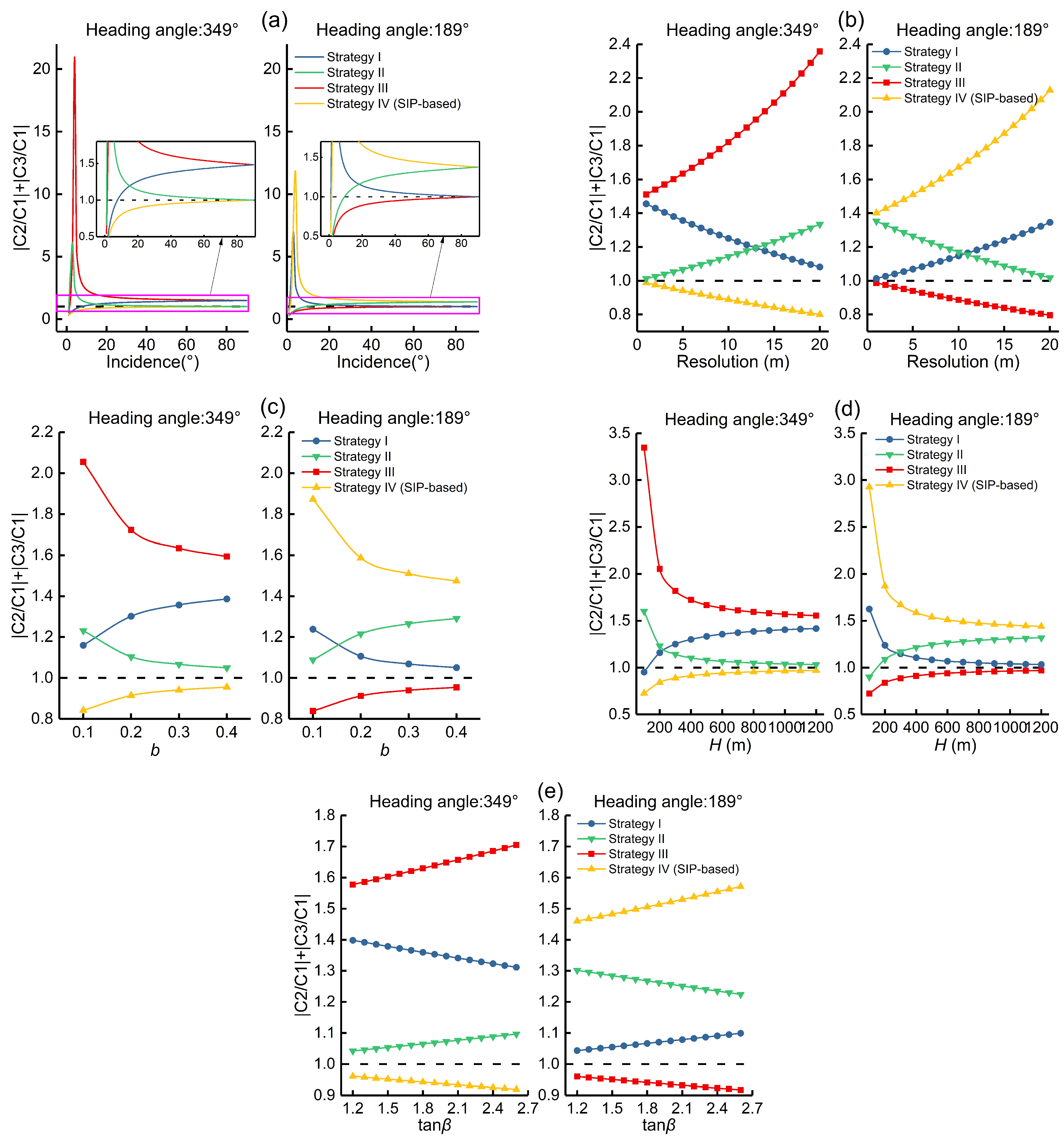
4.1. Applicable Condition of the Proposed Strategy
4.1.1. Relationship between and |C2/C1| + |C3/C1|
4.1.2. Relationship between , R, b, H, tan and |C2/C1| + |C3/C1|
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
Appendix C
Appendix D
| Satellite | Incidence/ | Heading/ | Interferometric Pair | B 1 | T 2 |
|---|---|---|---|---|---|
| TerraSAR-X | 41.07 | 189.70 | 20151219–20151230 | 9.96 | 11 |
| 20151230–20160110 | −228.42 | 11 | |||
| 20160110–20160121 | 93.39 | 11 | |||
| 20160121–20160201 | −49.45 | 11 | |||
| 20160201–20160212 | −124.28 | 11 | |||
| RadarSAT-2 | 35.51 | 349.14 | 20151224–20160117 | −43.31 | 24 |
| 20160117–20160305 | −37.78 | 48 |
| Satellite | Incidence/ | Heading/ | Interferometric Pair | B 1 | T 2 |
|---|---|---|---|---|---|
| TerraSAR-X | 41.07 | 189.70 | 20151219–20151230 | 9.96 | 11 |
| 20151219–20160110 | −218.48 | 22 | |||
| 20151219–20160121 | −125.10 | 33 | |||
| 20151219–20160201 | −174.53 | 44 | |||
| 20151230–20160110 | −228.42 | 11 | |||
| 20151230–20160121 | −135.04 | 22 | |||
| 20151230–20160201 | −184.49 | 33 | |||
| 20160110–20160121 | 93.39 | 11 | |||
| 20160110–20160201 | 43.93 | 22 | |||
| 20160121–20160201 | −49.45 | 11 | |||
| 20160201–20160212 | −124.28 | 11 | |||
| RadarSAT-2 | 35.51 | 349.14 | 20151224–20160117 | −43.31 | 24 |
| 20151224–20160305 | −81.09 | 72 | |||
| 20160117–20160305 | −37.78 | 48 | |||
| Sentinel-1A | 39.18 | 346.88 | 20151214–20151226 | 86.17 | 12 |
| 20151214–20160107 | 86.17 | 24 | |||
| 20151226–20160107 | 23.53 | 12 | |||
| 20160107–20160307 | −78.09 | 60 |
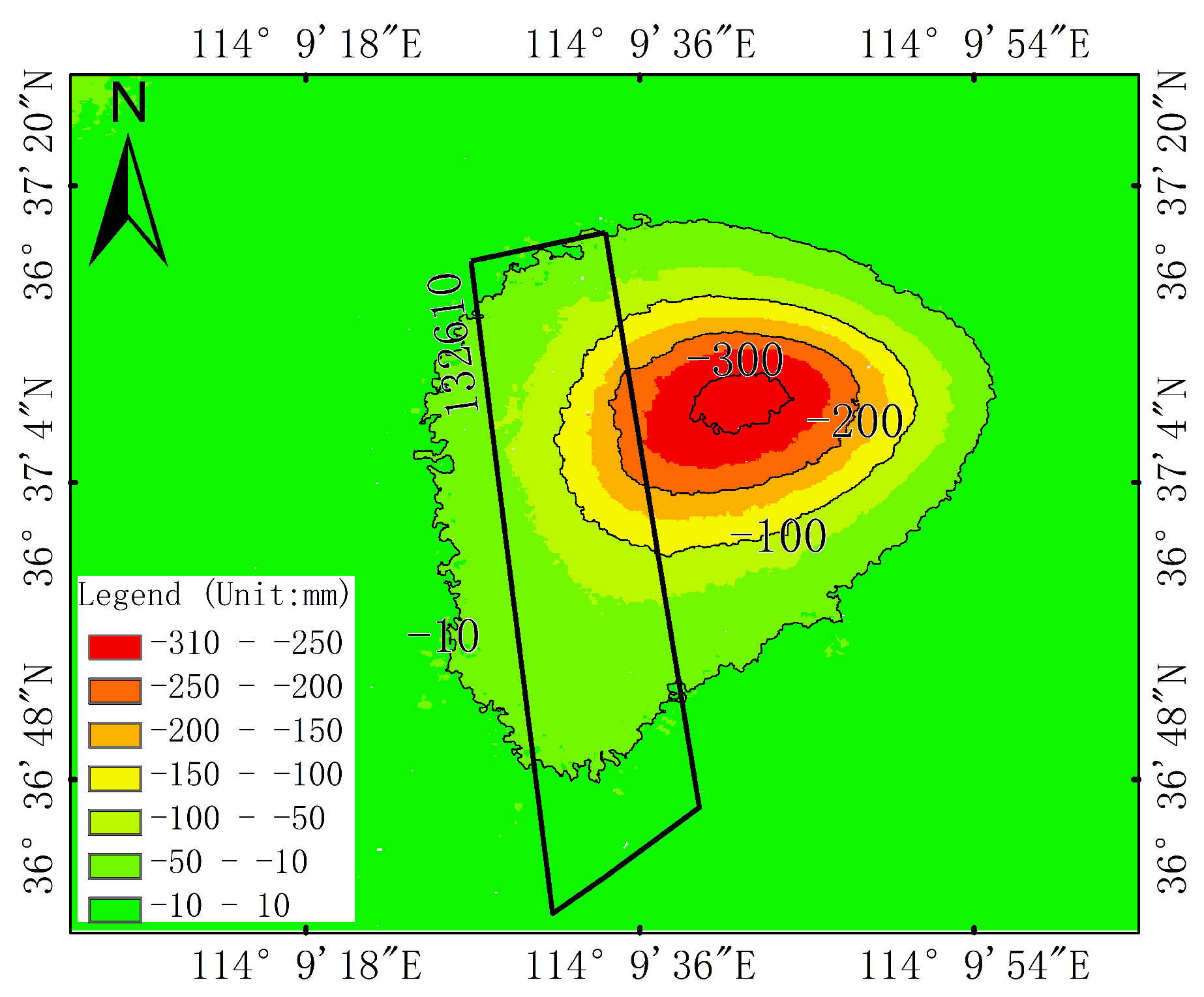
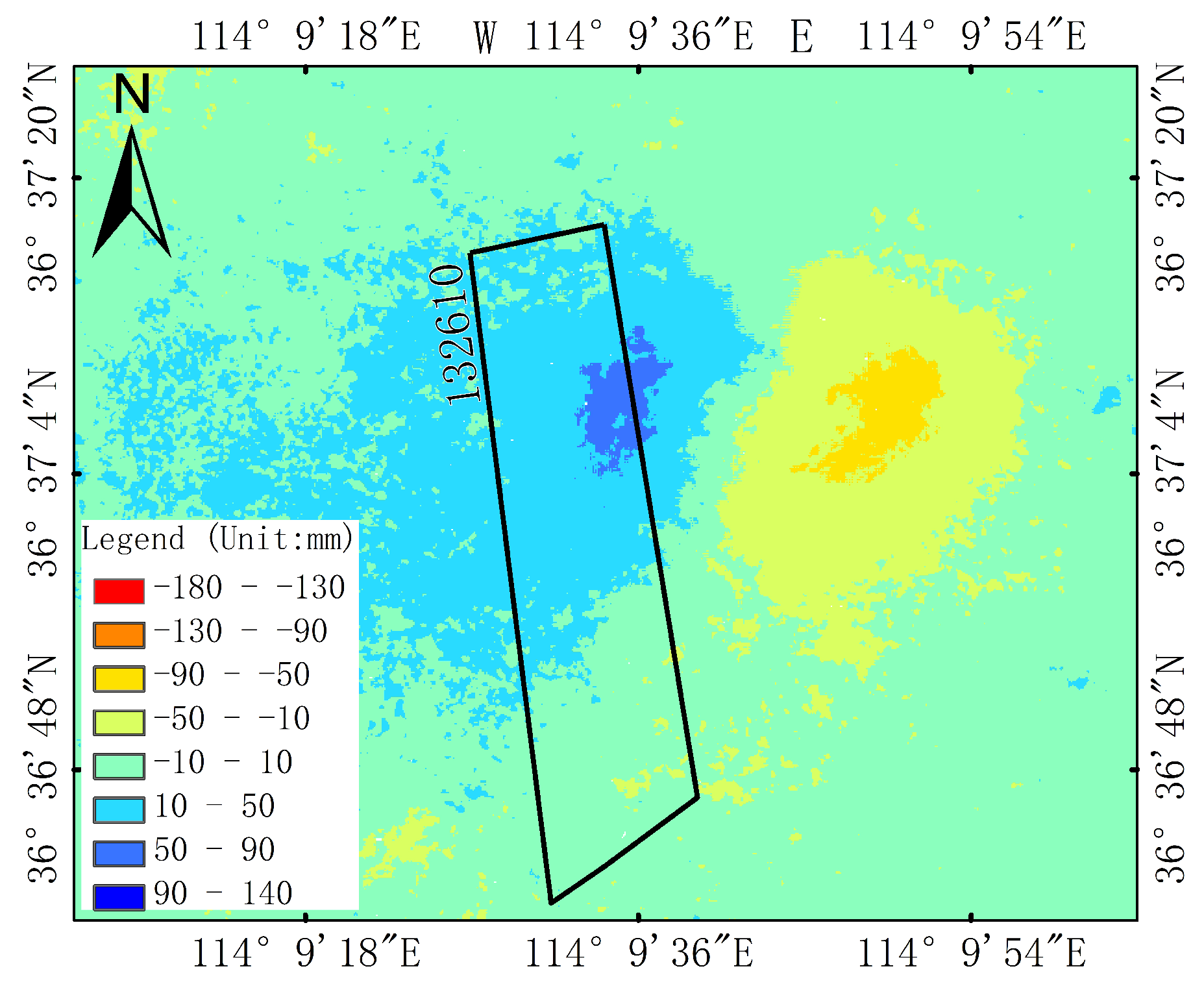
References
- He, G. Mining Subsidence; China University of Mining and Technology Press: Xuzhou, China, 1991. [Google Scholar]
- Milczarek, W. Application of a small baseline subset time series method with atmospheric correction in monitoring results of mining activity on ground surface and in detecting induced seismic events. Remote Sens. 2019, 11, 1008. [Google Scholar] [CrossRef]
- Syed, T.H.; Riyas, M.J.; Kuenzer, C. Remote sensing of coal fires in India: A review. Earth-Sci. Rev. 2018, 187, 338–355. [Google Scholar] [CrossRef]
- Guo, Q.; Guo, G.; Lv, X.; Zhang, W.; Lin, Y.; Qin, S. Strata movement and surface subsidence prediction model of dense solid backfilling mining. Environ. Earth Sci. 2016, 75, 1426. [Google Scholar] [CrossRef]
- Chen, B.; Li, Z.; Yu, C.; Fairbairn, D.; Kang, J.; Hu, J.; Liang, L. Three-dimensional time-varying large surface displacements in coal exploiting areas revealed through integration of SAR pixel offset measurements and mining subsidence model. Remote Sens. Environ. 2020, 240, 111663. [Google Scholar] [CrossRef]
- Zheng, M.; Deng, K.; Fan, H.; Du, S.; Zou, H. Monitoring and analysis of mining 3D time-series deformation based on multi-track SAR data. Int. J. Remote Sens. 2019, 40, 1409–1425. [Google Scholar] [CrossRef]
- Fan, H.; Lu, L.; Yao, Y. Method combining probability integration model and a small baseline subset for time series monitoring of mining subsidence. Remote Sens. 2018, 10, 1444. [Google Scholar] [CrossRef]
- Cuenca, M.C.; Hooper, A.J.; Hanssen, R.F. Surface deformation induced by water influx in the abandoned coal mines in Limburg, The Netherlands observed by satellite radar interferometry. J. Appl. Geophys. 2013, 88, 1–11. [Google Scholar] [CrossRef]
- Gama, F.F.; Paradella, W.R.; Mura, J.C.; de Oliveira, C.G. Advanced DINSAR analysis on dam stability monitoring: A case study in the Germano mining complex (Mariana, Brazil) with SBAS and PSI techniques. Remote Sens. Appl. Soc. Environ. 2019, 16, 100267. [Google Scholar] [CrossRef]
- Diao, X.; Wu, K.; Zhou, D.; Li, L. Integrating the probability integral method for subsidence prediction and differential synthetic aperture radar interferometry for monitoring mining subsidence in Fengfeng, China. J. Appl. Remote Sens. 2016, 10, 016028. [Google Scholar] [CrossRef]
- Yang, Z.F.; Li, Z.W.; Zhu, J.J.; Hu, J.; Wang, Y.J.; Chen, G.L. InSAR-based model parameter estimation of probability integral method and its application for predicting mining-induced horizontal and vertical displacements. IEEE Trans. Geosci. Remote Sens. 2016, 54, 4818–4832. [Google Scholar] [CrossRef]
- Ilieva, M.; Polanin, P.; Borkowski, A.; Gruchlik, P.; Smolak, K.; Kowalski, A.; Rohm, W. Mining deformation life cycle in the light of InSAR and deformation models. Remote Sens. 2019, 11, 745. [Google Scholar] [CrossRef]
- Yang, Z.; Li, Z.; Zhu, J.; Preusse, A.; Hu, J.; Feng, G.; Wang, Y.; Papst, M. An InSAR-based temporal probability integral method and its application for predicting mining-induced dynamic deformations and assessing progressive damage to surface buildings. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2018, 11, 472–484. [Google Scholar] [CrossRef]
- Diao, X.; Bai, Z.; Wu, K.; Zhou, D.; Li, Z. Assessment of mining-induced damage to structures using InSAR time series analysis: A case study of Jiulong Mine, China. Environ. Earth Sci. 2018, 77, 166. [Google Scholar] [CrossRef]
- Milczarek, W. Investigation of post inducted seismic deformation of the 2016 Mw4. 2 Tarnowek Poland mining tremor based on DInSAR and SBAS methods. Acta Geodyn. Geomater. 2019, 16, 183–193. [Google Scholar]
- Rudziński, Ł.; Mirek, K.; Mirek, J. Rapid ground deformation corresponding to a mining-induced seismic event followed by a massive collapse. Nat. Hazards 2019, 96, 461–471. [Google Scholar] [CrossRef]
- Yan, S.; Shi, K.; Li, Y.; Liu, J.; Zhao, H. Integration of satellite remote sensing data in underground coal fire detection: A case study of the Fukang region, Xinjiang, China. Front. Earth Sci. 2020, 14, 1–12. [Google Scholar] [CrossRef]
- Fan, H.; Deng, K.; Ju, C.; Zhu, C.; Xue, J. Land subsidence monitoring by D-InSAR technique. Min. Sci. Technol. 2011, 21, 869–872. [Google Scholar] [CrossRef]
- Yang, Z.; Li, Z.; Zhu, J.; Feng, G.; Wang, Q.; Hu, J.; Wang, C. Deriving time-series three-dimensional displacements of mining areas from a single-geometry InSAR dataset. J. Geod. 2018, 92, 529–544. [Google Scholar] [CrossRef]
- Ng, A.H.M.; Ge, L.; Zhang, K.; Chang, H.C.; Li, X.; Rizos, C.; Omura, M. Deformation mapping in three dimensions for underground mining using InSAR–Southern highland coalfield in New South Wales, Australia. Int. J. Remote Sens. 2011, 32, 7227–7256. [Google Scholar] [CrossRef]
- Zhu, C.; Zhang, J.; Deng, K.; Zhang, Y.H.; Fan, H.D.; Li, P.X. Three-dimensional displacement field of buildings detection from multi-source SAR imagery. J. China Univ. Min. Technol. 2014, 43, 701–706. [Google Scholar]
- Huang, J.; Bai, Y.; Lei, S.; Deng, K. Time-series SBAS pixel offset tracking method for monitoring three-dimensional deformation in a mining area. IEEE Access 2020, 8, 118787–118798. [Google Scholar] [CrossRef]
- Zheng, M.; Deng, K.; Fan, H.; Huang, J. Monitoring and analysis of mining 3D deformation by multi-platform SAR images with the probability integral method. Front. Earth Sci. 2019, 13, 169–179. [Google Scholar] [CrossRef]
- Yang, Z.; Li, Z.; Zhu, J.; Preusse, A.; Hu, J.; Feng, G.; Papst, M. Time-series 3-D mining-induced large displacement modeling and robust estimation from a single-geometry SAR amplitude data set. IEEE Trans. Geosci. Remote Sens. 2018, 56, 3600–3610. [Google Scholar] [CrossRef]
- Zhu, C.; Wang, Z.; Li, P.; Motagh, M.; Zhang, L.; Jiang, Z.; Long, S. Retrieval and Prediction of Three-Dimensional Displacements by Combining the DInSAR and Probability Integral Method in a Mining Area. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2020, 13, 1206–1217. [Google Scholar] [CrossRef]
- Tang, F.Q.; Dong, L.K.; Wang, Z.L.; Huang, J.C. A 3-D inversion model for InSAR detected displacements based on ground subsidence symmetry induced by horizontal coal mining. J. China Coal Soc. 2019, 1, 210–220. [Google Scholar]
- Yang, Z.; Li, Z.; Zhu, J.; Wang, Y.; Wu, L. Use of SAR/InSAR in Mining Deformation Monitoring, Parameter Inversion, and Forward Predictions: A Review. IEEE Geosci. Remote Sens. Mag. 2020, 8, 71–90. [Google Scholar] [CrossRef]
- Li, Z.W.; Yang, Z.F.; Zhu, J.J.; Hu, J.; Wang, Y.J.; Li, P.X.; Chen, G.L. Retrieving three-dimensional displacement fields of mining areas from a single InSAR pair. J. Geod. 2015, 89, 17–32. [Google Scholar] [CrossRef]
- Fan, H.; Wen, B.; Liu, J.; Liu, J.; Yin, J. An improved method of three-dimensional displacement field generation in mining areas with a single InSAR pair. Eur. J. Remote Sens. 2019, 52, 493–503. [Google Scholar] [CrossRef]
- Li, P.; T, Z. Mining influenced ground deformation state analysis and principal deformation calculation. J. Min. Saf. Eng. 2017, 34, 96–102. [Google Scholar]
- Wu, K.; Zhou, M. Mining Subsidence Prediction System; China University of Mining and Technology Press: Xuzhou, China, 1991. [Google Scholar]
- Werner, C.; Wegmüller, U.; Strozzi, T.; Wiesmann, A. Gamma SAR and Interferometric Processing Software. In Proceedings of the Ers-Envisat Symposium, Gothenburg, Sweden, 16–20 October 2000; Volume 1620. [Google Scholar]
- Chen, C.W.; Zebker, H.A. Network approaches to two-dimensional phase unwrapping: Intractability and two new algorithms. JOSA A 2000, 17, 401–414. [Google Scholar] [CrossRef]
- Hydrological Geological Team of Hebei Province Coal Geology Bureau. Report of Geology at Wannian Mine of Hebei Fengfeng Group Co., Ltd.; Hydrological Geological Team of Hebei Province Coal Geology Bureau: Handan, China, 2008. [Google Scholar]
- Samsonov, S.; d’Oreye, N. Multidimensional time-series analysis of ground deformation from multiple InSAR data sets applied to Virunga Volcanic Province. Geophys. J. Int. 2012, 191, 1095–1108. [Google Scholar]
- Samsonov, S.; d’Oreye, N.; Smets, B. Ground deformation associated with post-mining activity at the French–German border revealed by novel InSAR time series method. Int. J. Appl. Earth Obs. Geoinf. 2013, 23, 142–154. [Google Scholar] [CrossRef]
- Li, P. Study on Regularity and Prediction Method of Surface Subsidence Due to Deep Mining; China University of Mining and Technology: Xuzhou, China, 2012. [Google Scholar]
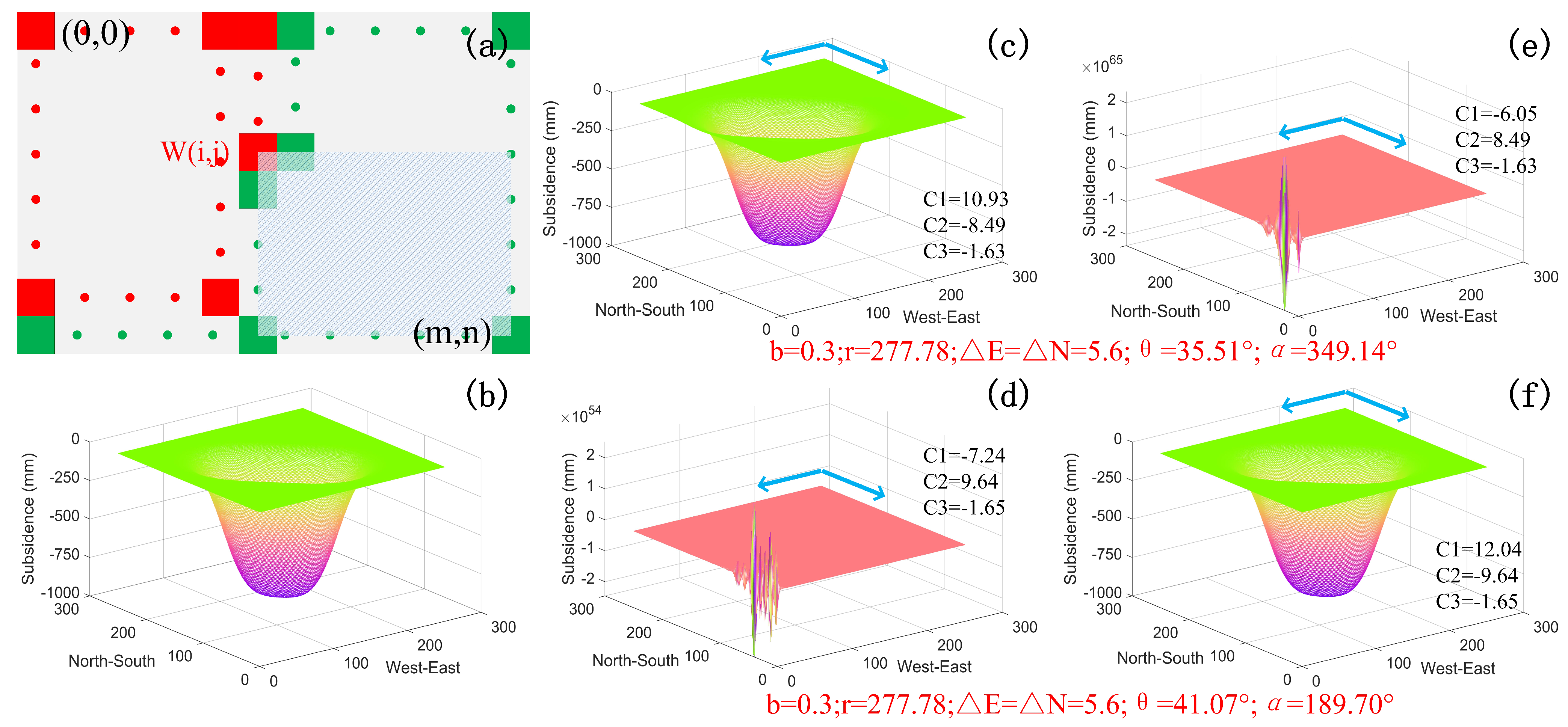




| Satellite Mission | Repeat Cycle | Wavelength/Band | Resolution (Azimuth × Range) | Incidence | Heading |
|---|---|---|---|---|---|
| TerraSAR-X | 11 days | 3.11 cm/X-band | 1.89 m × 1.36 m | 41.07 | 189.70 |
| RADARSAT-2 | 24 days | 5.55 cm/C-band | 2.90 m × 2.70 m | 35.51 | 349.14 |
| / | / | R/m | b | H/m | tan | |
|---|---|---|---|---|---|---|
| Range | 1–360 | 1–90 | 1–20 | 0.1–0.4 | 100–1200 | 1.2–2.6 |
| Fixed value | 189/349 | 35 | 5 | 0.3 | 600 | 1.85 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, R.; Viktorovich, Z.A.; Li, J.; Chen, C.; Zheng, M. A New Strategy for Extracting 3D Deformation of Mining Areas from a Single-Geometry Synthetic Aperture Radar Dataset. Remote Sens. 2023, 15, 5244. https://doi.org/10.3390/rs15215244
Zhao R, Viktorovich ZA, Li J, Chen C, Zheng M. A New Strategy for Extracting 3D Deformation of Mining Areas from a Single-Geometry Synthetic Aperture Radar Dataset. Remote Sensing. 2023; 15(21):5244. https://doi.org/10.3390/rs15215244
Chicago/Turabian StyleZhao, Ruonan, Zhabko Andrey Viktorovich, Junfeng Li, Chuang Chen, and Meinan Zheng. 2023. "A New Strategy for Extracting 3D Deformation of Mining Areas from a Single-Geometry Synthetic Aperture Radar Dataset" Remote Sensing 15, no. 21: 5244. https://doi.org/10.3390/rs15215244
APA StyleZhao, R., Viktorovich, Z. A., Li, J., Chen, C., & Zheng, M. (2023). A New Strategy for Extracting 3D Deformation of Mining Areas from a Single-Geometry Synthetic Aperture Radar Dataset. Remote Sensing, 15(21), 5244. https://doi.org/10.3390/rs15215244






