A Multi-Objective Geoacoustic Inversion of Modal-Dispersion and Waveform Envelope Data Based on Wasserstein Metric
Abstract
:1. Introduction
2. Individual Inversion Theories
2.1. DCs Inversion
2.1.1. Modal Propagation in a Single Receiver Context
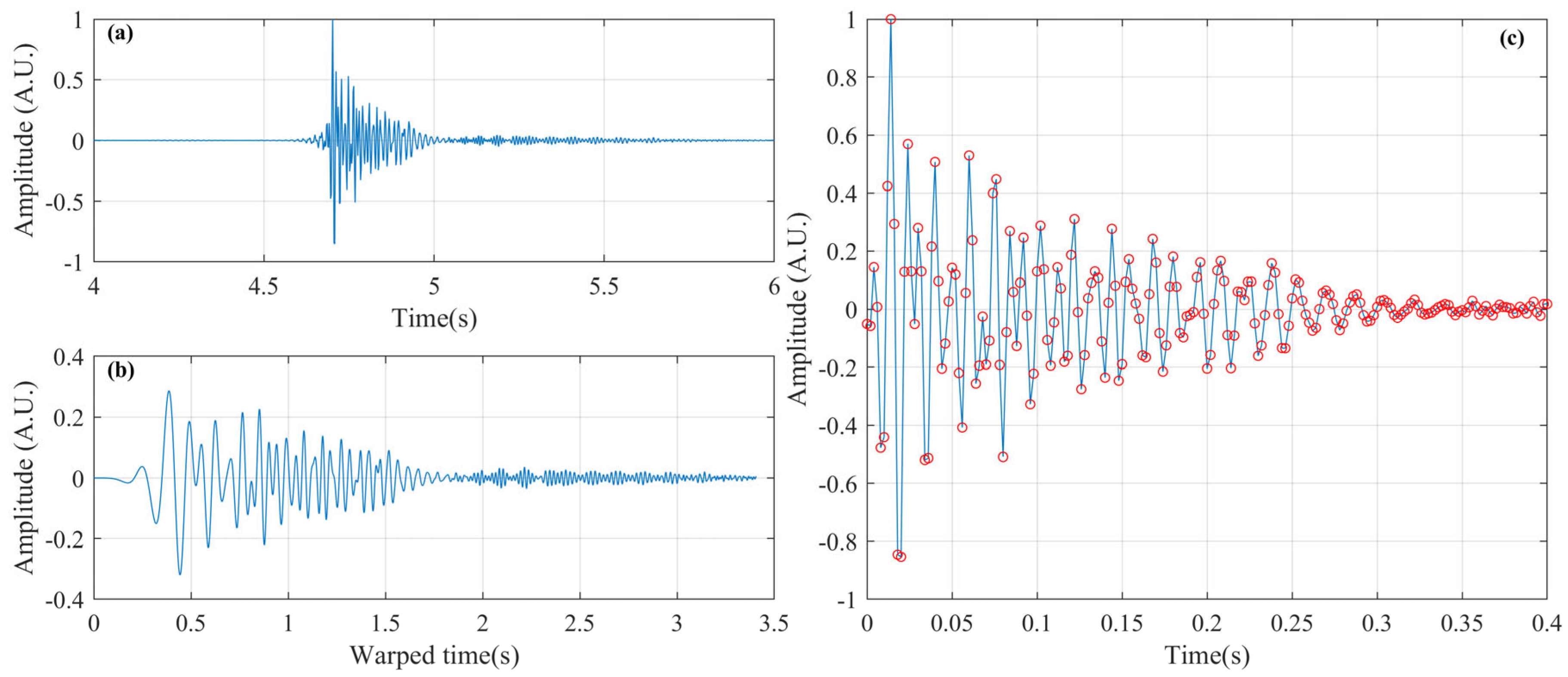
2.1.2. Estimation of the DCs Using Time-Warping
2.2. FWH Inversion
3. Inversion Methods
3.1. Bayesian Inversion Theory
3.2. Cost Function Based on L2 Norm and Wasserstein Metric
3.3. Multi-Objective Bayesian Optimization
4. Simulation Results
4.1. Environmental Setup and Simulated Data
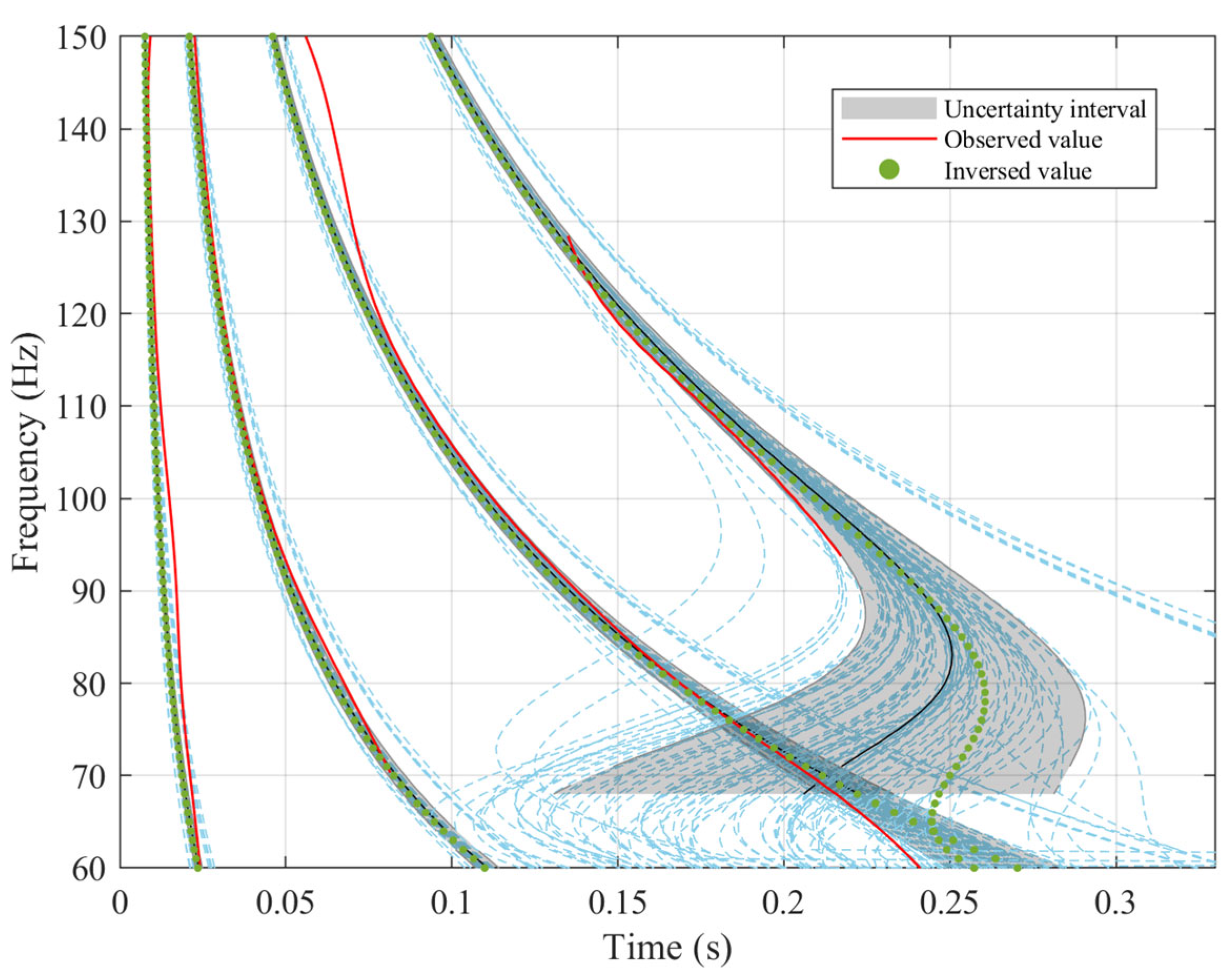
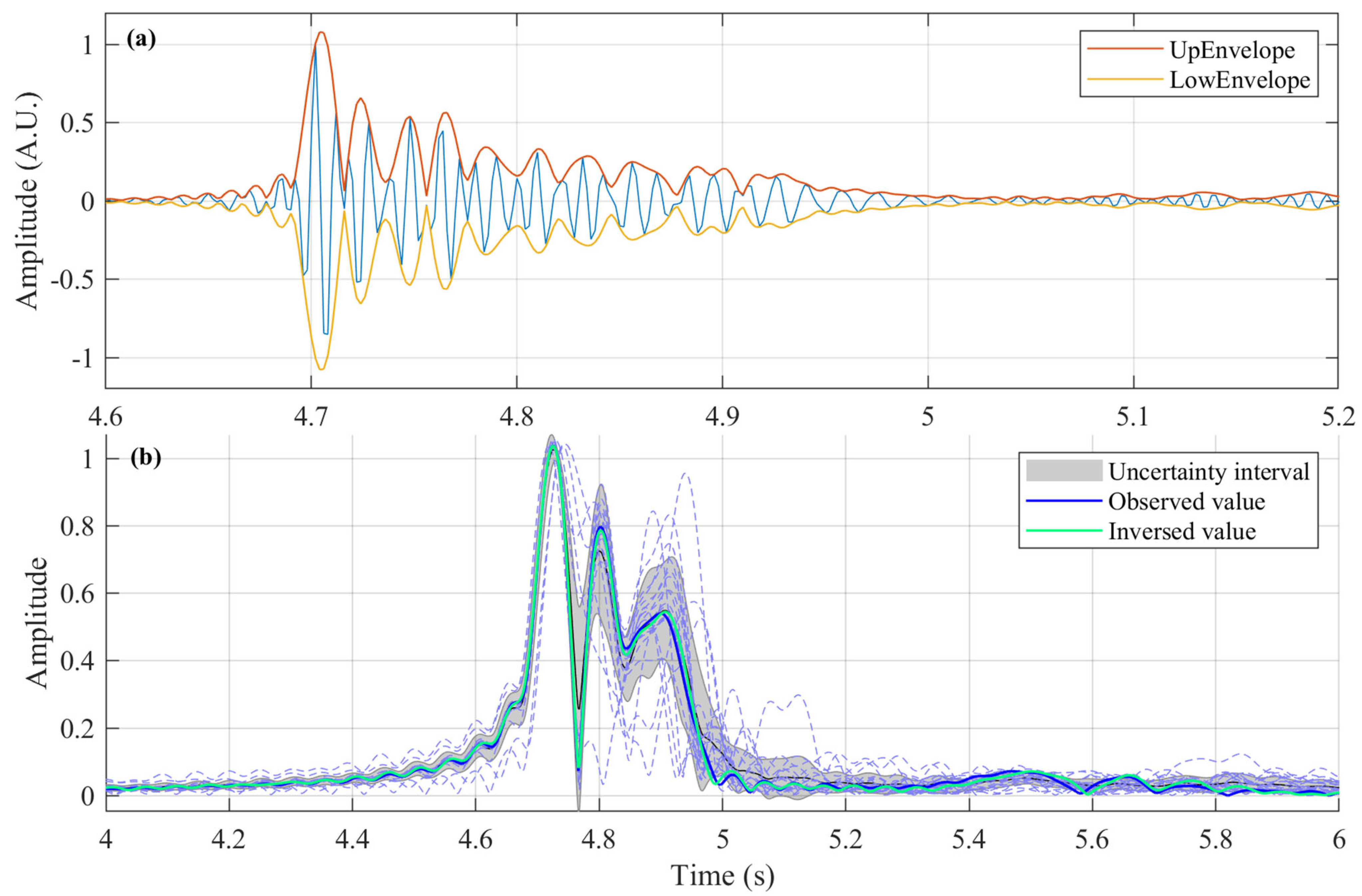
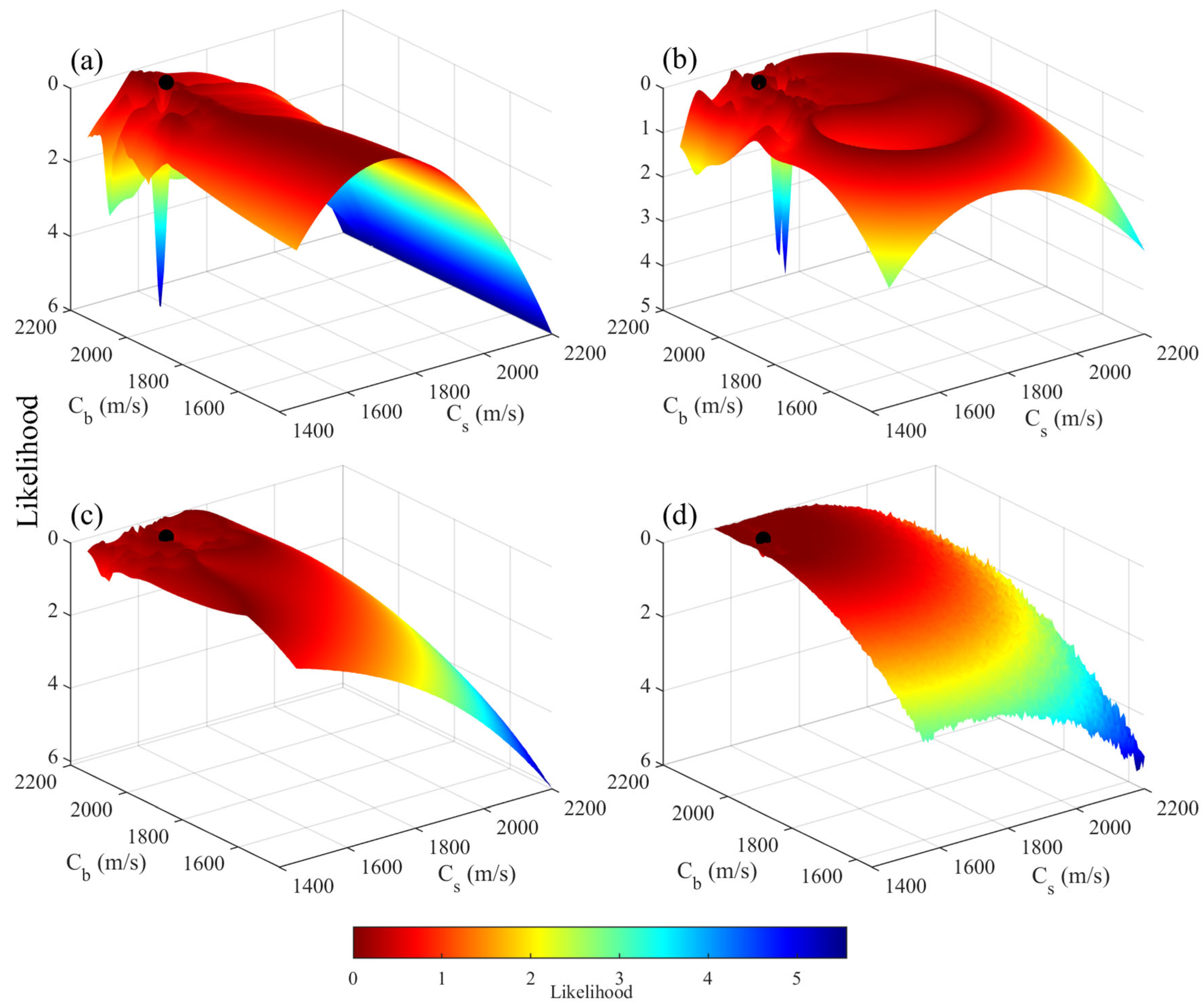
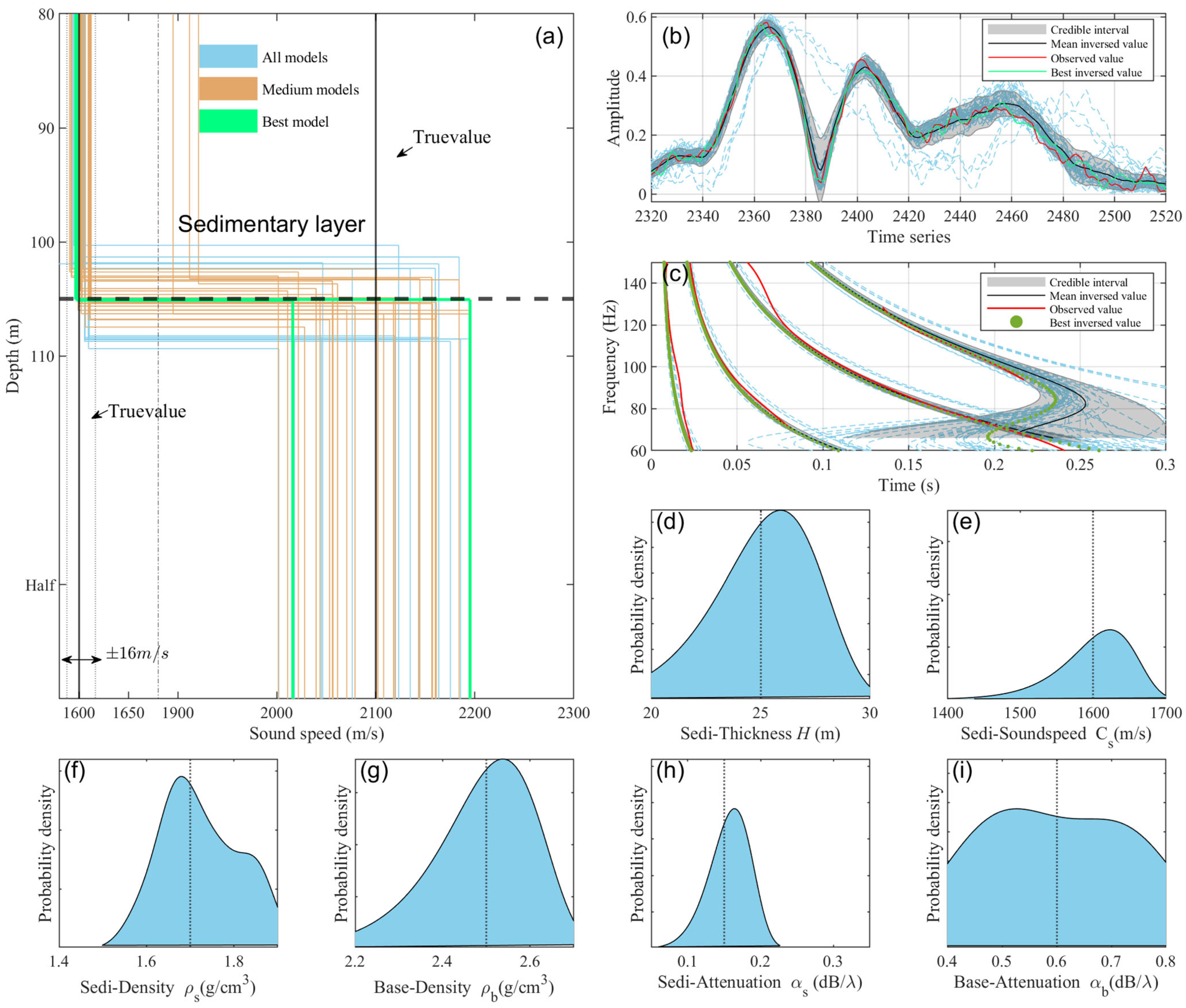
4.2. Comparison between Individual Inversions and Multi-Objective Inversions
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sun, M.; Jin, S. Multiparameter Elastic Full Waveform Inversion of Ocean Bottom Seismic Four-Component Data Based on a Modified Acoustic-Elastic Coupled Equation. Remote Sens. 2020, 12, 2816. [Google Scholar] [CrossRef]
- Zhou, J. Normal Mode Measurements and Remote Sensing of Sea-Bottom Sound Velocity and Attenuation in Shallow Water. J. Acoust. Soc. Am. 1985, 78, 1003–1009. [Google Scholar] [CrossRef]
- Oddo, P.; Falchetti, S.; Viola, S.; Pennucci, G.; Storto, A.; Borrione, I.; Giorli, G.; Cozzani, E.; Russo, A.; Tollefsen, C. Evaluation of Different Maritime Rapid Environmental Assessment Procedures with a Focus on Acoustic Performance. J. Acoust. Soc. Am. 2022, 152, 2962–2981. [Google Scholar] [CrossRef]
- Dosso, S.E. Environmental Uncertainty in Ocean Acoustic Source Localization. Inverse Probl. 2003, 19, 419–431. [Google Scholar] [CrossRef]
- Collins, M.D.; Turgut, A.; Buckingham, M.J.; Gerstoft, P.; Siderius, M. Selected Topics of the Past Thirty Years in Ocean Acoustics. J. Theor. Comp. Acout. 2022, 30, 2240001. [Google Scholar] [CrossRef]
- Chapman, N.R.; Shang, E.C. Review of Geoacoustic Inversion in Underwater Acoustics. J. Theor. Comp. Acout. 2021, 29, 2130004. [Google Scholar] [CrossRef]
- Wan, L.; Badiey, M.; Knobles, D.P. Geoacoustic Inversion Using Low Frequency Broadband Acoustic Measurements from L-Shaped Arrays in the Shallow Water 2006 Experiment. J. Acoust. Soc. Am. 2016, 140, 2358–2373. [Google Scholar] [CrossRef] [PubMed]
- Sazontov, A.G.; Malekhanov, A.I. Matched Field Signal Processing in Underwater Sound Channels (Review). Acoust. Phys. 2015, 61, 213–230. [Google Scholar] [CrossRef]
- Shi, J.; Dosso, S.E.; Sun, D.; Liu, Q. Geoacoustic Inversion of the Acoustic-Pressure Vertical Phase Gradient from a Single Vector Sensor. J. Acoust. Soc. Am. 2019, 146, 3159–3173. [Google Scholar] [CrossRef]
- Song, H.C.; Byun, G. An Overview of Array Invariant for Source-Range Estimation in Shallow Water. J. Acoust. Soc. Am. 2022, 151, 2336–2352. [Google Scholar] [CrossRef]
- Dettmer, J.; Dosso, S.E.; Holland, C.W. Full Wave-Field Reflection Coefficient Inversion. J. Acoust. Soc. Am. 2007, 122, 3327–3337. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Ma, Y.; Kan, G.; Liu, B.; Zhou, X.; Zhang, X. An Inversion Method for Geoacoustic Parameters in Shallow Water Based on Bottom Reflection Signals. Remote Sens. 2023, 15, 3237. [Google Scholar] [CrossRef]
- Potty, G.R.; Miller, J.H.; Lynch, J.F. Inversion for Sediment Geoacoustic Properties at the New England Bight. J. Acoust. Soc. Am. 2003, 114, 1874–1887. [Google Scholar] [CrossRef] [PubMed]
- Tan, T.W.; Godin, O.A.; Katsnelson, B.G.; Yarina, M. Passive Geoacoustic Inversion in the Mid-Atlantic Bight in the Presence of Strong Water Column Variability. J. Acoust. Soc. Am. 2020, 147, EL453–EL459. [Google Scholar] [CrossRef]
- Tan, T.W.; Godin, O.A. Passive Acoustic Characterization of Sub-Seasonal Sound Speed Variations in a Coastal Ocean. J. Acoust. Soc. Am. 2021, 150, 2717–2737. [Google Scholar] [CrossRef]
- Bonnel, J.; McNeese, A.R.; Wilson, P.S.; Dosso, S.E. Geoacoustic Inversion Using Simple Hand-Deployable Acoustic Systems. IEEE J. Ocean. Eng. 2023, 48, 592–603. [Google Scholar] [CrossRef]
- Guarino, A.L.; Smith, K.B.; Gemba, K.; Godin, O.A. Geoacoustic Inversion Using Waveform Matching as a Preliminary Step in Dispersion Curve Analysis to Assess Bottom Attenuation from a Single Vector Sensor. In Proceedings of the International Congress on Acoustics, Gyeongju, Republic of Korea, 24–28 October 2022; p. ABS-0288. [Google Scholar]
- Zhou, J.-X.; Zhang, X.-Z.; Knobles, D.P. Low-Frequency Geoacoustic Model for the Effective Properties of Sandy Seabottoms. J. Acoust. Soc. Am. 2009, 125, 2847–2866. [Google Scholar] [CrossRef]
- Bonnel, J.; Chapman, N.R. Geoacoustic Inversion in a Dispersive Waveguide Using Warping Operators. J. Acoust. Soc. Am. 2011, 130, EL101–EL107. [Google Scholar] [CrossRef]
- Bonnel, J.; Gervaise, C.; Nicolas, B.; Mars, J.I. Single-Receiver Geoacoustic Inversion Using Modal Reversal. J. Acoust. Soc. Am. 2012, 131, 119–128. [Google Scholar] [CrossRef]
- Bonnel, J.; Dosso, S.E.; Ross Chapman, N. Bayesian Geoacoustic Inversion of Single Hydrophone Light Bulb Data Using Warping Dispersion Analysis. J. Acoust. Soc. Am. 2013, 134, 120–130. [Google Scholar] [CrossRef]
- Guo, Q.; Zhang, H.; Tian, J.; Liang, L.; Shang, Z. A Nonlinear Multiparameter Prestack Seismic Inversion Method Based on Hybrid Optimization Approach. Arab. J. Geosci. 2018, 11, 48. [Google Scholar] [CrossRef]
- Zhao, X.; Huang, S. Atmospheric Duct Estimation Using Radar Sea Clutter Returns by the Adjoint Method with Regularization Technique. J. Atmos. Ocean. Technol. 2014, 31, 1250–1262. [Google Scholar] [CrossRef]
- Bonnel, J.; Thode, A.; Wright, D.; Chapman, R. Nonlinear Time-Warping Made Simple: A Step-by-Step Tutorial on Underwater Acoustic Modal Separation with a Single Hydrophone. J. Acoust. Soc. Am. 2020, 147, 1897–1926. [Google Scholar] [CrossRef]
- Rajan, S.D. Waveform Inversion for the Geoacoustic Parameters of the Ocean Bottom. J. Acoust. Soc. Am. 1992, 91, 3228–3241. [Google Scholar] [CrossRef]
- Feng, Y.; Schranner, F.S.; Winter, J.; Adams, N.A. A Multi-Objective Bayesian Optimization Environment for Systematic Design of Numerical Schemes for Compressible Flow. J. Comput. Phys. 2022, 468, 111477. [Google Scholar] [CrossRef]
- Jensen, F.B.; Kuperman, W.A.; Porter, M.B.; Schmidt, H. Normal Modes. In Computational Ocean Acoustics; Jensen, F.B., Kuperman, W.A., Porter, M.B., Schmidt, H., Eds.; Modern Acoustics and Signal Processing; Springer: New York, NY, USA, 2011; pp. 337–455. ISBN 978-1-4419-8678-8. [Google Scholar]
- Bonnel, J.; Le Touze, G.; Nicolas, B.; Mars, J.I. Physics-Based Time-Frequency Representations for Underwater Acoustics: Power Class Utilization with Waveguide-Invariant Approximation. IEEE Signal Process. Mag. 2013, 30, 120–129. [Google Scholar] [CrossRef]
- Bonnel, J.; Caporale, S.; Thode, A. Waveguide Mode Amplitude Estimation Using Warping and Phase Compensation. J. Acoust. Soc. Am. 2017, 141, 2243–2255. [Google Scholar] [CrossRef]
- Chapman, N.R. Chapter 9—Inverse Methods in Underwater Acoustics. In Applied Underwater Acoustics; Neighbors, T.H., Bradley, D., Eds.; Elsevier: Amsterdam, The Netherlands, 2017; pp. 553–585. ISBN 978-0-12-811240-3. [Google Scholar]
- Porter, M. The KRAKEN Normal Mode Program; Naval Research Laboratory: Washington, DC, USA, 2006. [Google Scholar]
- Westwood, E.K.; Tindle, C.T.; Chapman, N.R. A Normal Mode Model for Acousto-elastic Ocean Environments. J. Acoust. Soc. Am. 1996, 100, 3631–3645. [Google Scholar] [CrossRef]
- Zheng, G.; Zhu, H.; Wang, X.; Khan, S.; Li, N.; Xue, Y. Bayesian Inversion for Geoacoustic Parameters in Shallow Sea. Sensors 2020, 20, 2150. [Google Scholar] [CrossRef]
- Yang, Y.; Engquist, B.; Sun, J.; Hamfeldt, B.F. Application of Optimal Transport and the Quadratic Wasserstein Metric to Full-Waveform Inversion. Geophysics 2018, 83, R43–R62. [Google Scholar] [CrossRef]
- Engquist, B.; Froese, B.D. Application of the Wasserstein Metric to Seismic Signals. Commun. Math. Sci. 2014, 12, 979–988. [Google Scholar] [CrossRef]
- Bonnel, J.; Pecknold, S.P.; Hines, P.C.; Chapman, N.R. An Experimental Benchmark for Geoacoustic Inversion Methods. IEEE J. Ocean. Eng. 2021, 46, 261–282. [Google Scholar] [CrossRef]
- Holland, C.W.; Dosso, S.E. Hamilton’s Geoacoustic Model. J. Acoust. Soc. Am. 2022, 151, R1–R2. [Google Scholar] [CrossRef] [PubMed]
- Li, D.; Lamoureux, M.P.; Liao, W. Application of an Unbalanced Optimal Transport Distance and a Mixed L1/Wasserstein Distance to Full Waveform Inversion. Geophys. J. Int. 2022, 230, 1338–1357. [Google Scholar] [CrossRef]
- da Silva, S.L.E.F.; Karsou, A.; de Souza, A.; Capuzzo, F.; Costa, F.; Moreira, R.; Cetale, M. A Graph-Space Optimal Transport Objective Function Based on q-Statistics to Mitigate Cycle-Skipping Issues in FWI. Geophys. J. Int. 2022, 231, 1363–1385. [Google Scholar] [CrossRef]
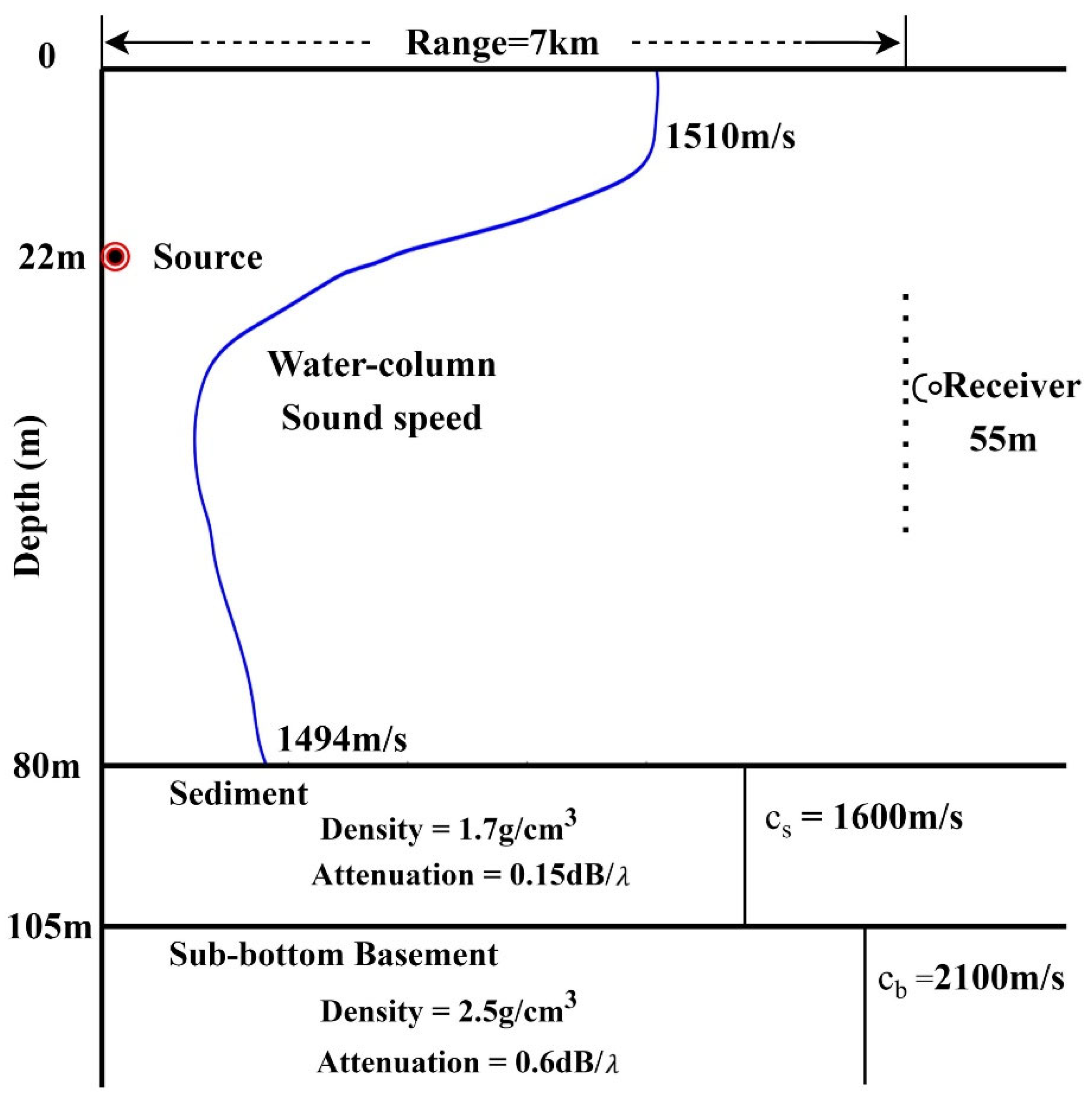


| Parameter | Units | True Values | Prior Bounds |
|---|---|---|---|
| Layer thickness 1 | m | 25.0 | [20, 30] |
| Sediment sound speed | 1600.0 | [1440, 2500] | |
| Sediment density | 1.7 | [1.3, 2.4] | |
| Sediment attenuation | 0.15 | [0.05, 0.5] | |
| Basement sound speed | 2100.0 | [1440, 2500] | |
| Basement density | 2.5 | [1.3, 2.4] | |
| Basement attenuation | 0.6 | [0.05, 0.7] | |
| Time shift | — | [−1.0, 2.0] |
| Parameter | NRMSE | |||||||
|---|---|---|---|---|---|---|---|---|
| L2-DCs | 24.5 (±2.9) | 1601.0 (±5.8) | 1.65 (±0.11) | 2260.0 (±119.7) | 2.58 (±0.16) | —— | —— | —— |
| L2-FWH | 29.7 (±4.2) | 1619.5 (±13.6) | 1.89 (±0.15) | 2091.0 (±99.4) | 2.45 (±0.13) | 0.220 (±0.025) | 0.405 (±0.158) | 7.35 × 10−5 |
| L2-MOBO | 26.3 (±2.4) | 1635.5 (±39.5) | 1.75 (±0.08) | 2077.5 (±122.0) | 2.55 (±0.12) | 0.160 (±0.025) | 0.628 (±0.125) | 7.32 × 10−5 |
| Wasserstein-MOBO | 27.1 (±4.3) | 1605.5 (±6.5) | 1.75 (±0.08) | 2136.0 (±70.0) | 2.54 (±0.11) | 0.170 (±0.015) | 0.604 (±0.125) | 5.67 × 10−5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, J.; Zhao, X.; Yang, P.; Fu, Y. A Multi-Objective Geoacoustic Inversion of Modal-Dispersion and Waveform Envelope Data Based on Wasserstein Metric. Remote Sens. 2023, 15, 4893. https://doi.org/10.3390/rs15194893
Ding J, Zhao X, Yang P, Fu Y. A Multi-Objective Geoacoustic Inversion of Modal-Dispersion and Waveform Envelope Data Based on Wasserstein Metric. Remote Sensing. 2023; 15(19):4893. https://doi.org/10.3390/rs15194893
Chicago/Turabian StyleDing, Jiaqi, Xiaofeng Zhao, Pinglv Yang, and Yapeng Fu. 2023. "A Multi-Objective Geoacoustic Inversion of Modal-Dispersion and Waveform Envelope Data Based on Wasserstein Metric" Remote Sensing 15, no. 19: 4893. https://doi.org/10.3390/rs15194893
APA StyleDing, J., Zhao, X., Yang, P., & Fu, Y. (2023). A Multi-Objective Geoacoustic Inversion of Modal-Dispersion and Waveform Envelope Data Based on Wasserstein Metric. Remote Sensing, 15(19), 4893. https://doi.org/10.3390/rs15194893







