The Doppler Characteristics of Sea Echoes Acquired by Motion Radar
Abstract
:1. Introduction
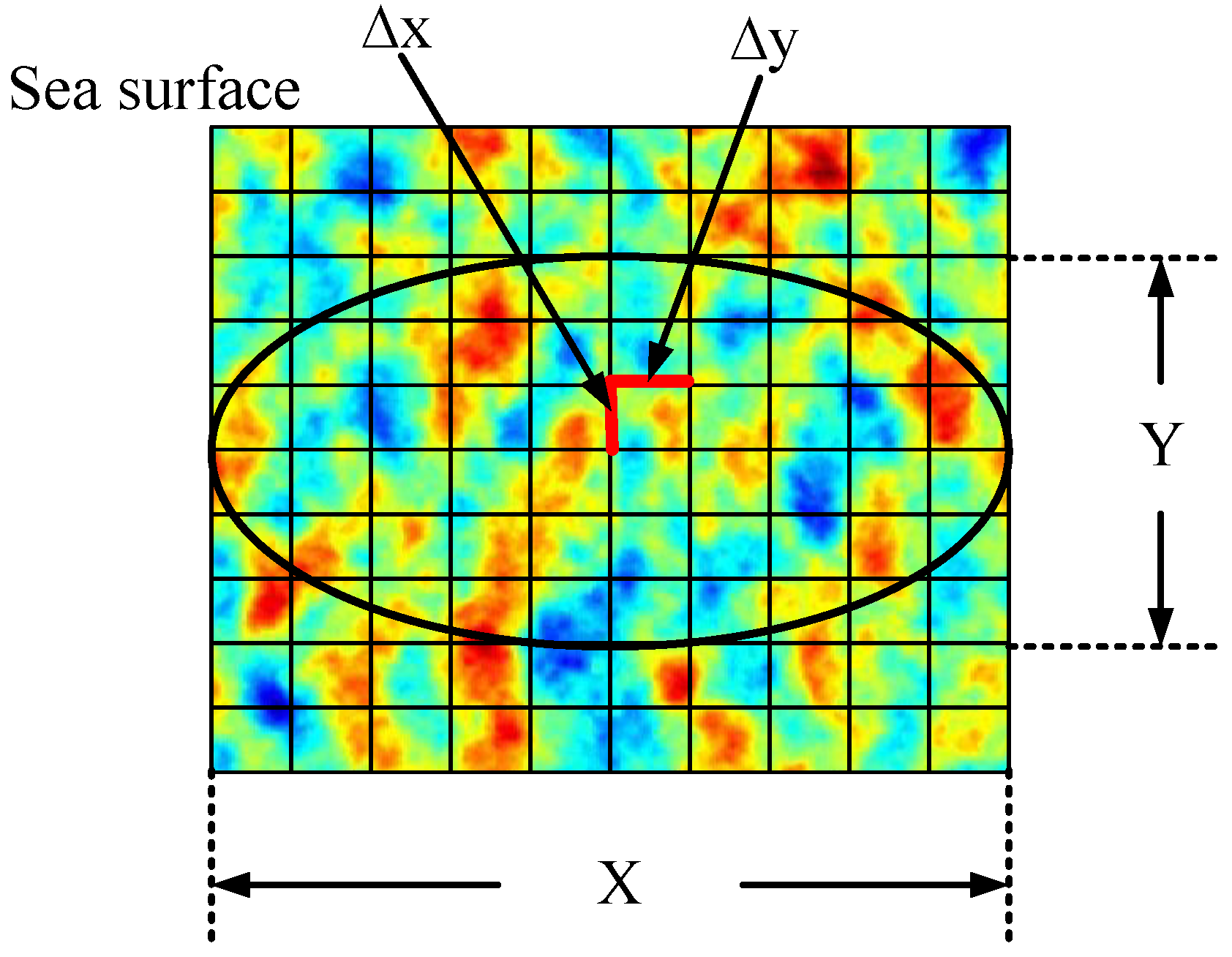
2. The Change in Radar Footprint with Platform Movement
3. The Incoherent Scattering Field Acquired by a Moving Radar
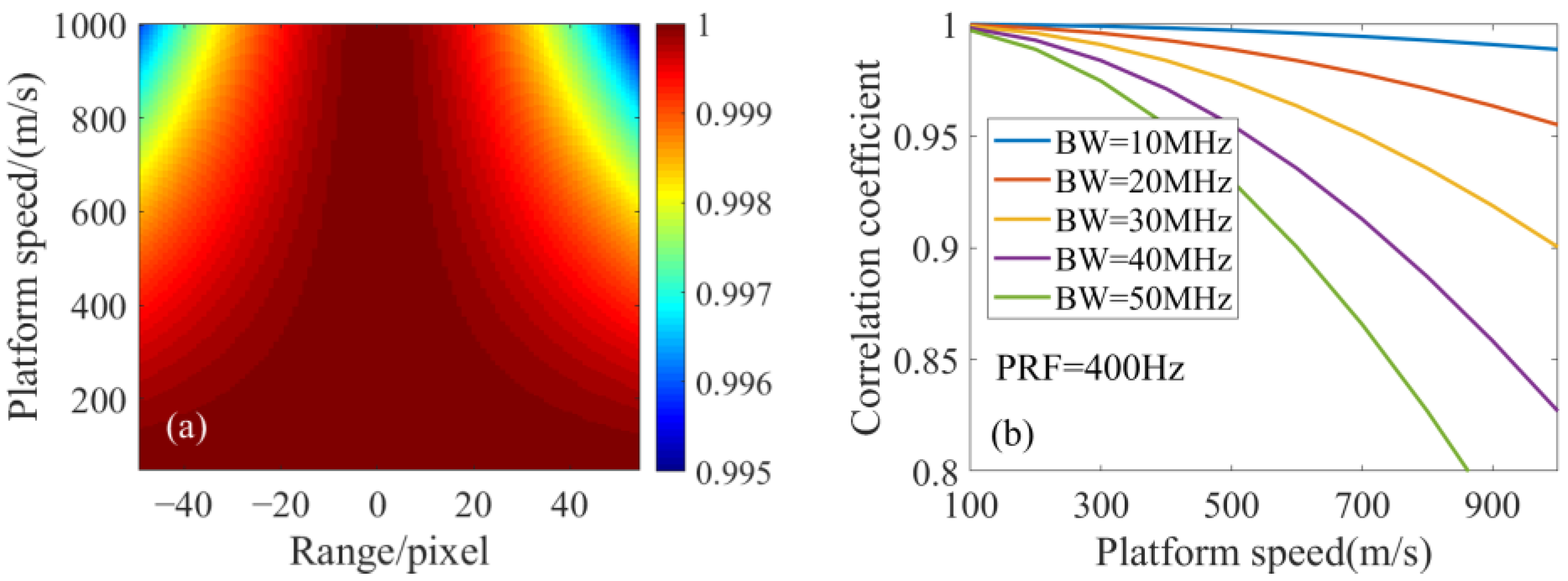
3.1. The Correlation Coefficient
3.2. The Correlation Coefficient
3.3. The Time Series of Scattering Field from Sea Surface
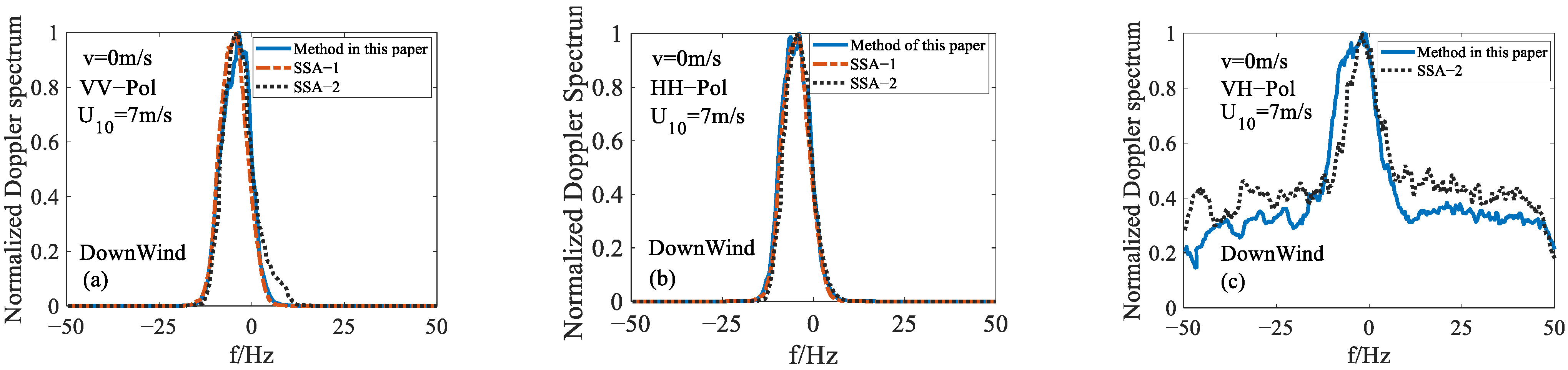
4. Validation of the Method
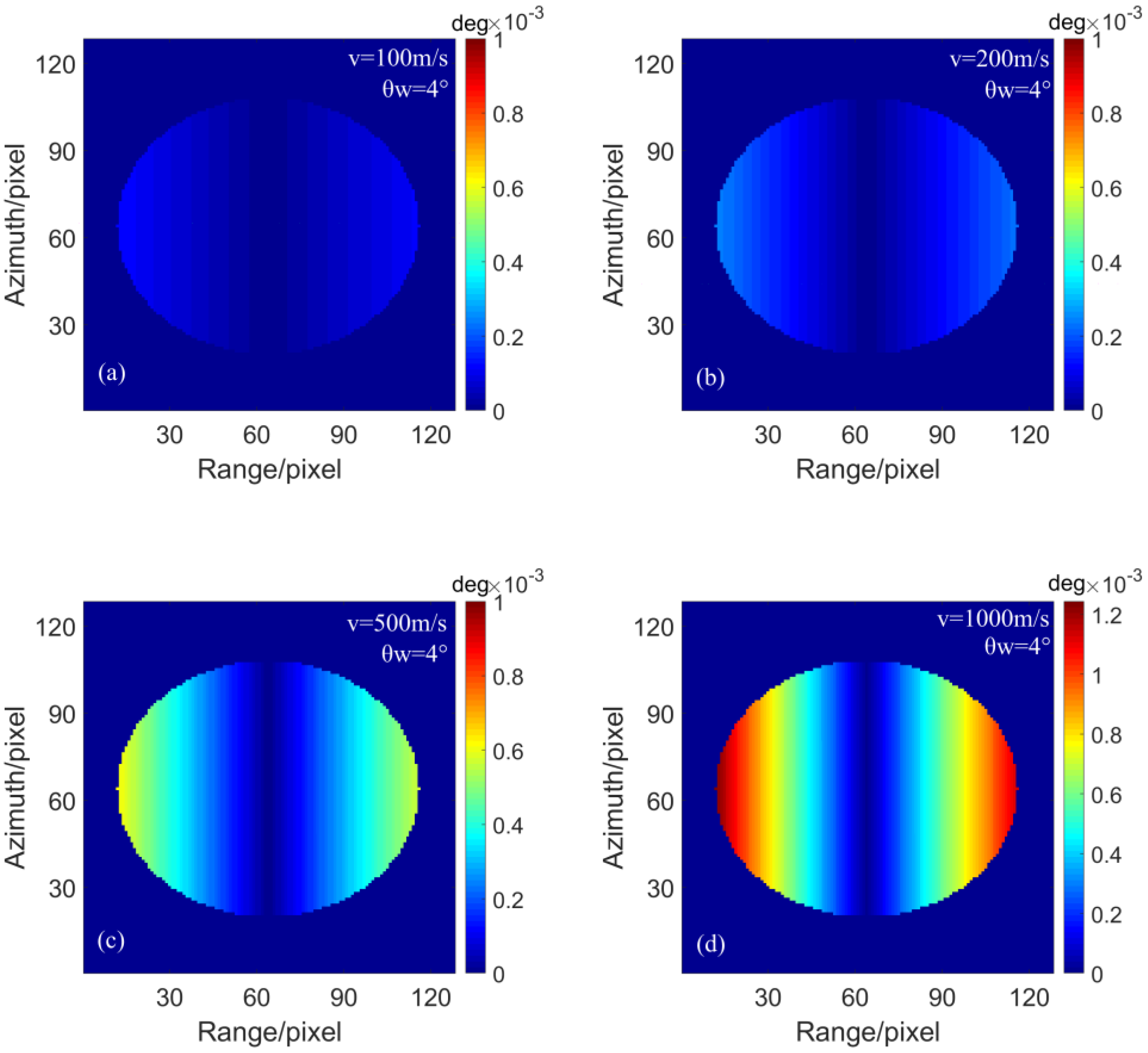
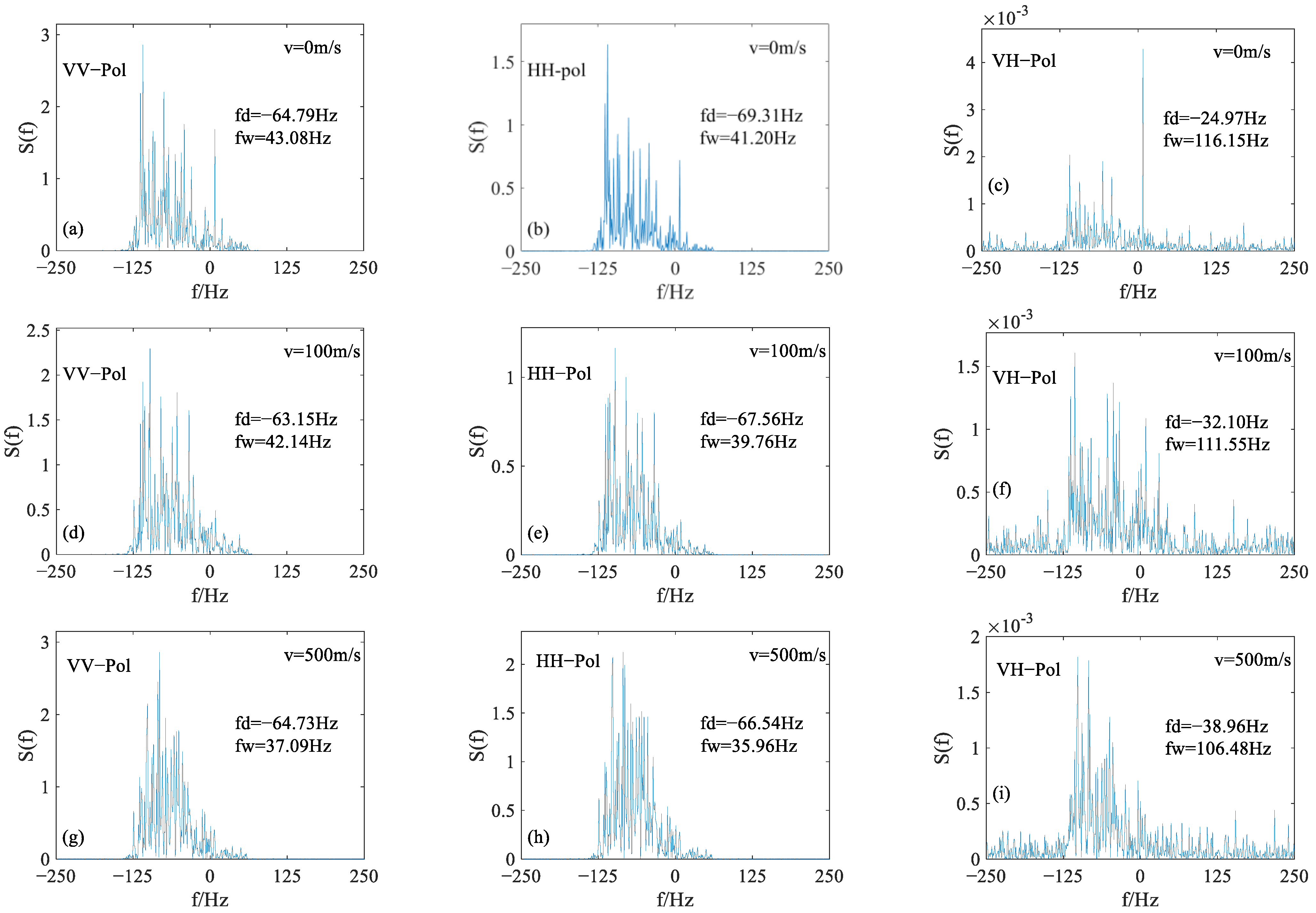
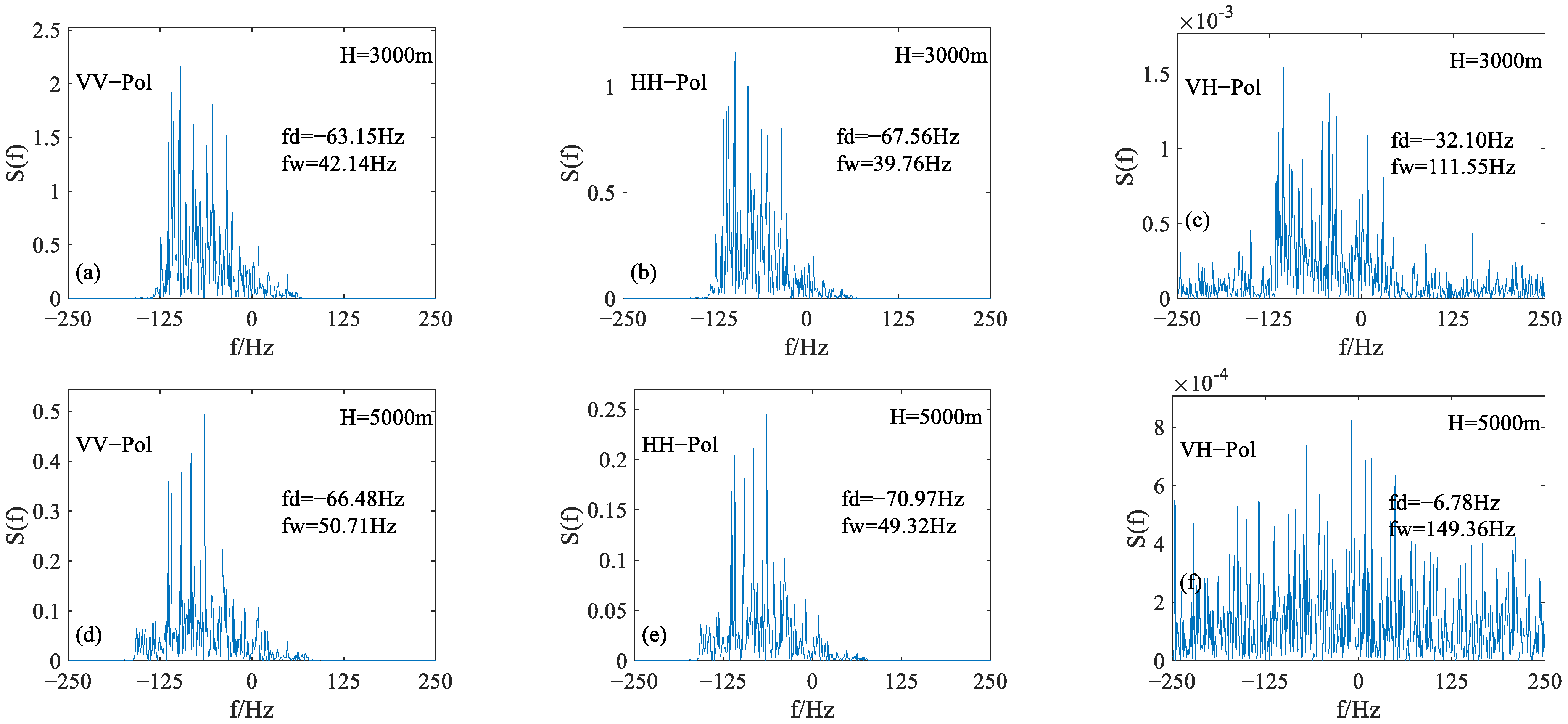
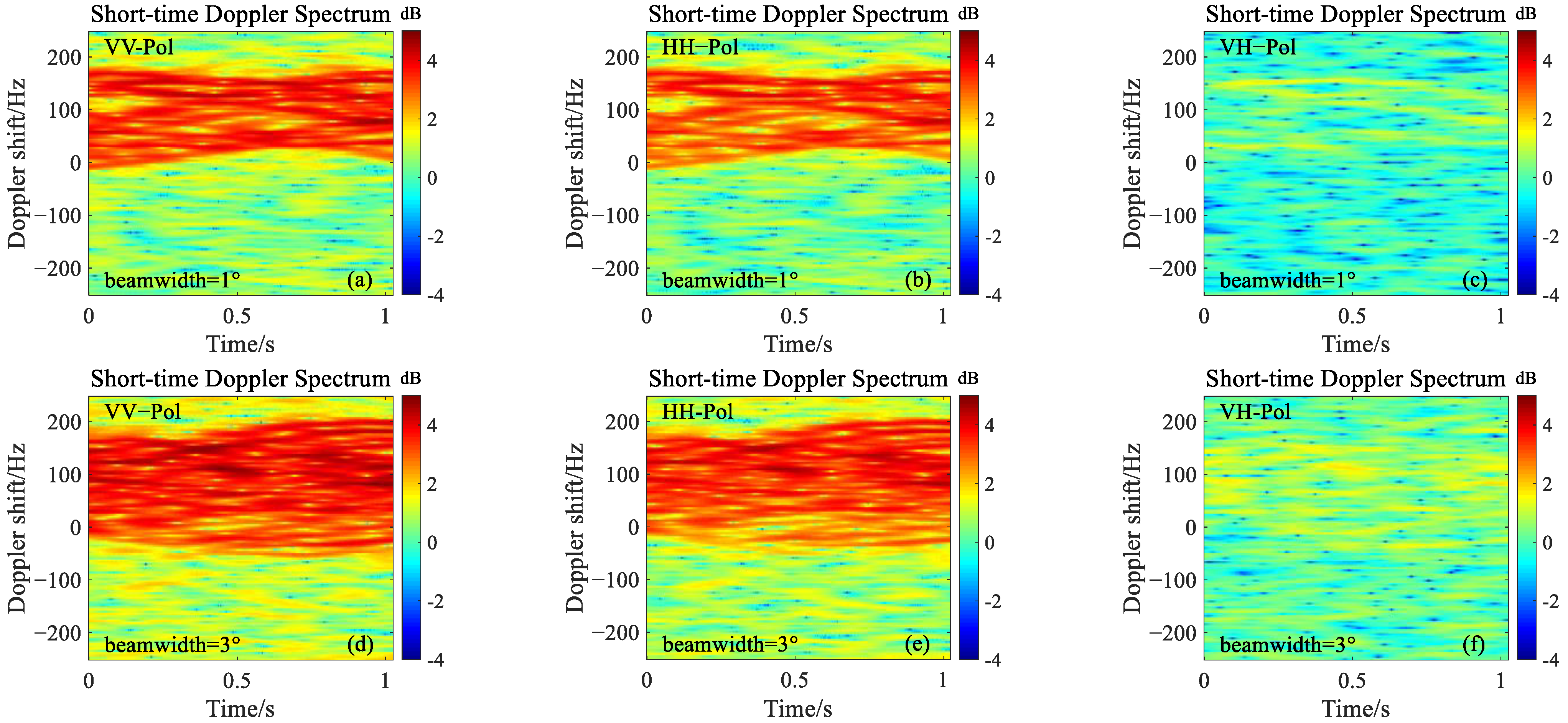
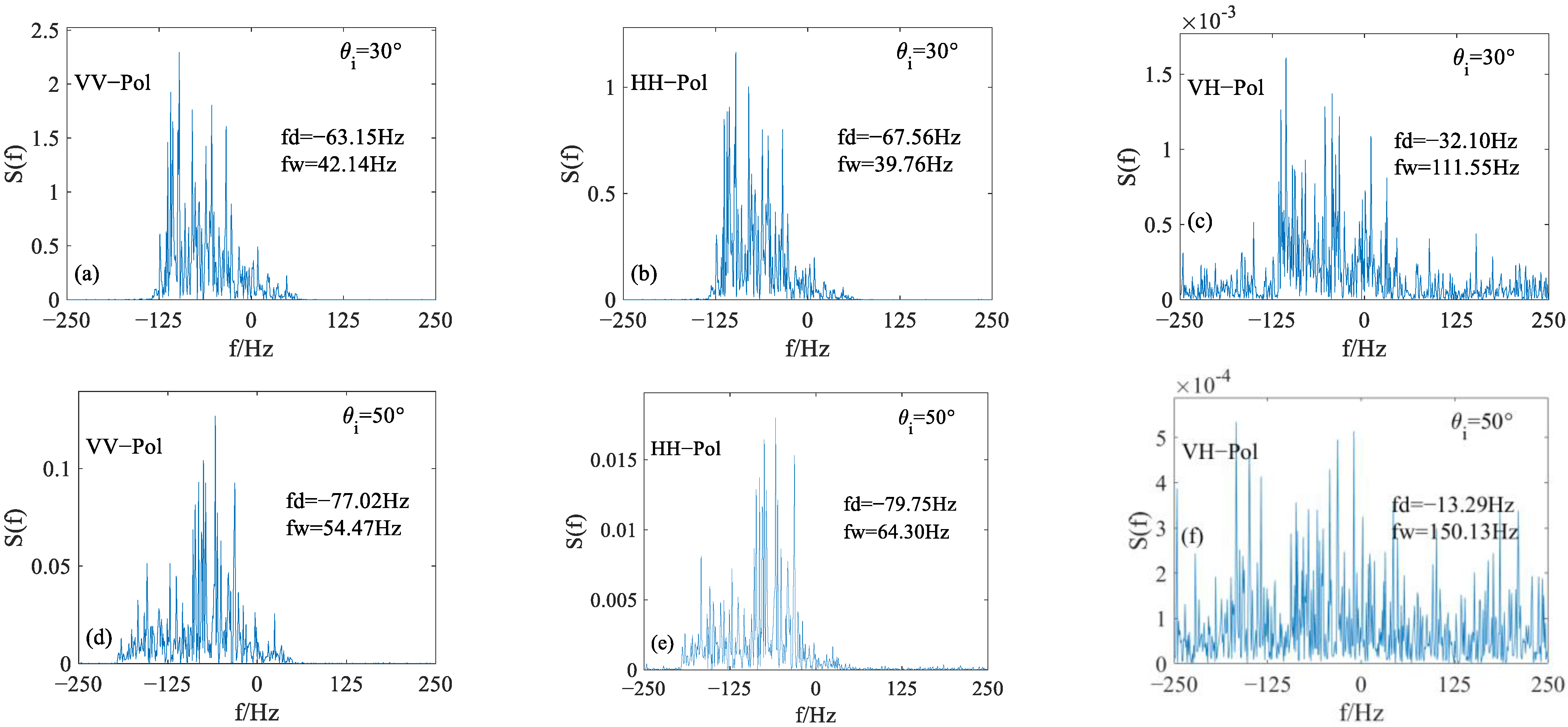
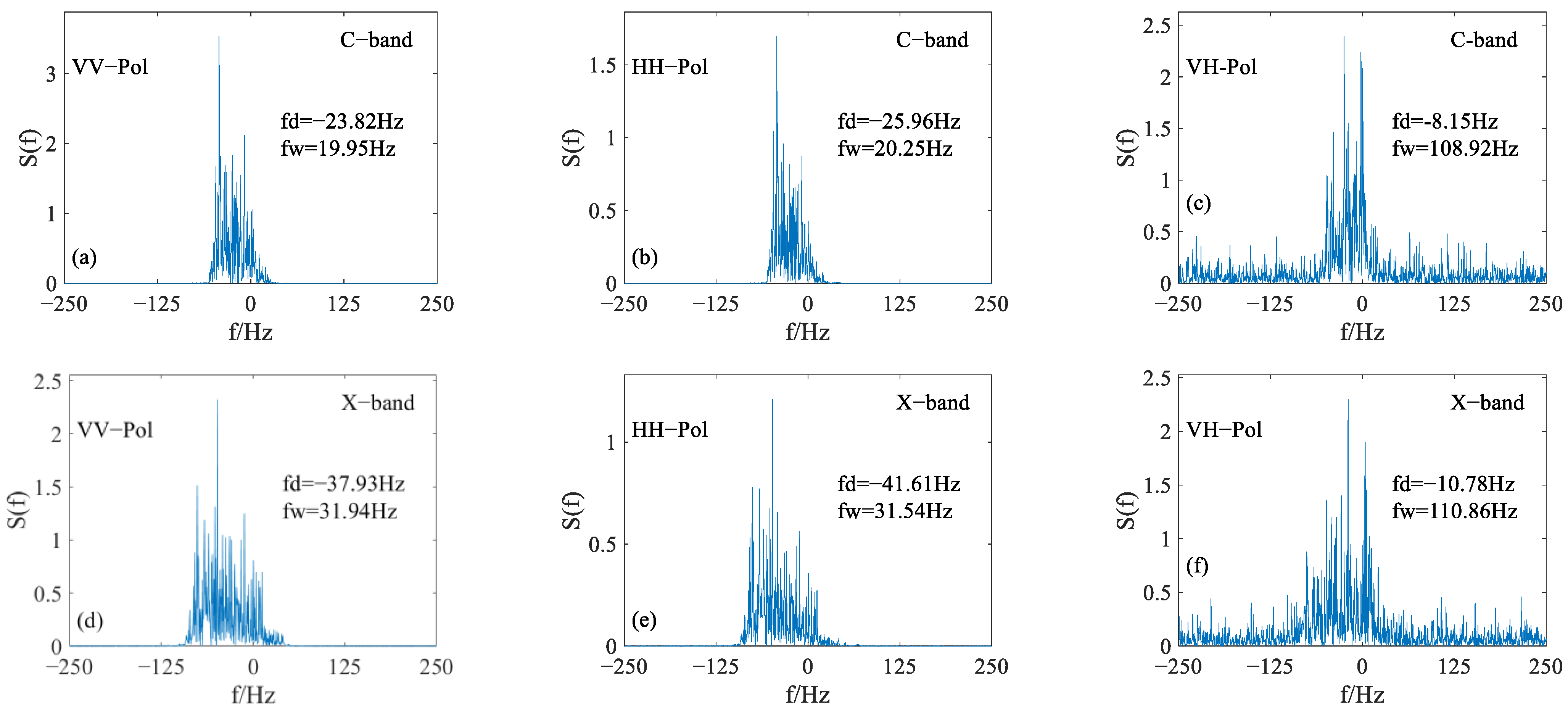
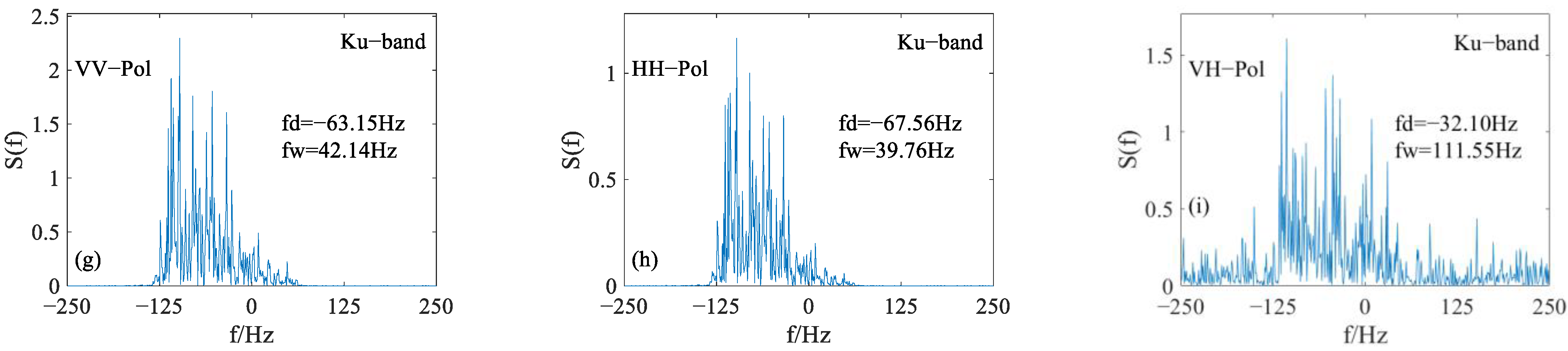
5. Analysis of Simulation Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chapron, B.; Collard, F.; Ardhuin, F. Direct measurements of ocean surface velocity from space: Interpretation and validation. J. Geophys. Res. Ocean. 2005, 110, 07008. [Google Scholar]
- Zamparelli, V.; De Santi, F.; De Carolis, G.; Fornaro, G. SAR Based Sea Surface Complex Wind Fields Estimation: An Analysis over the Northern Adriatic Sea. Remote Sens. 2023, 15, 2074. [Google Scholar]
- Mouche, A.A. On the Use of Doppler Shift for Sea Surface Wind Retrieval From SAR. IEEE Trans. Geosci. Remote Sens. 2012, 1, 2901–2909. [Google Scholar]
- Johnson, J.T.; Burkholder, R.J.; Toporkov, J.V.; Lyzenga, D.R.; Plant, W.J. A Numerical Study of the Retrieval of Sea Surface Height Profiles from Low Grazing Angle Radar Data. IEEE Trans. Geosci. Remote Sens. 2009, 47, 1641–1650. [Google Scholar]
- Hwang, P.A.; Sletten, M.A.; Toporkov, J.V. A note on Doppler processing of coherent radar backscatter from the water surface: With application to ocean surface wave measurements. J. Geophys. Res. 2010, 115, C03026. [Google Scholar]
- Chae, C.S.; Johnson, J.T. A Study of Sea Surface Range-Resolved Doppler Spectra Using Numerically Simulated Low-Grazing-Angle Backscatter Data. IEEE Trans. Geosci. Remote Sens. 2013, 51, 3452–3460. [Google Scholar]
- Sergievskaya, I.; Ermakov, S.; Plotnikov, L.; Kapustin, I.; Kupaev, A. On a Problem of Marine Current Velocity Estimation from Microwave Radar Data. Water 2023, 15, 1153. [Google Scholar]
- Crombie, D.D. Doppler Spectrum of Sea Echo at 13.56 Mc./s. Nature 1955, 175, 681–682. [Google Scholar]
- Barrick, D. First-order theory and analysis of MF/HF/VHF scatter from the sea. Antennas Propag. IEEE Trans. 1972, 20, 2–10. [Google Scholar]
- Zavorotny, V.U.; Voronovich, A.G. Two-scale model and ocean radar Doppler spectra at moderate- and low-grazing angles. Antennas Propag. IEEE Trans. 1998, 46, 84–92. [Google Scholar]
- Toporkov, J.V.; Brown, G.S. Numerical Simulations of Scattering from Time-Varying, Randomly Rough Surfaces. IEEE Trans. Geosci. Remote Sens. 2000, 38, 1616–1625. [Google Scholar]
- Toporkov, J.V.; Brown, G.S. Numerical study of the extended Kirchhoff approach and the lowest order small slope approximation for scattering from ocean-like surfaces: Doppler analysis. IEEE Trans. Antennas Propag. 2002, 50, 417–425. [Google Scholar]
- Walker, D. Experimentally motivated model for low grazing angle radar Doppler spectra of the sea surface. IEE Proc. Radar Sonar Navig. 2000, 147, 114–120. [Google Scholar]
- Wang, Y.; Zhang, Y. Investigation on Doppler Shift and Bandwidth of Backscattered Echoes from a Composite Sea Surface. IEEE Trans. Geosci. Remote Sens. 2011, 49, 1071–1081. [Google Scholar]
- Wang, Y. Doppler Spectra of Microwave Scattering Fields from Nonlinear Oceanic Surface at Moderate- and Low-Grazing Angles. IEEE Trans. Geosci. Remote Sens. 2012, 50, 1104–1116. [Google Scholar]
- Wang, Y.; Zhang, Y.M.; Guo, L.X. Microwave Doppler Spectra of Sea Echoes at High Incidence Angles: Influences of Large-Scale Waves. Prog. Electromagn. Res. B 2013, 48, 99–113. [Google Scholar]
- Yan-Min, Z.; Yun-Hua, W.; Chao-Fang, Z. Microwave Doppler spectra of sea return at small incidence angles: Specular point scattering contribution. Chin. Phys. B 2010, 19, 384–390. [Google Scholar]
- Zhang, Y.; Wang, Y.; Zheng, H.; Bai, Y. Doppler characteristics of electromagnetic echoes from sinusoidal water waves illuminated by plane wave/Gaussian beam. Waves Random Complex Media 2021, 1–16. [Google Scholar]
- Rozenberg, A.D.; Quigley, D.C. Laboratory study of polarized microwave scattering by surface waves at grazing incidence: The influence of long waves. IEEE Trans. Geosci. Remote Sens. 1996, 34, 1331–1342. [Google Scholar]
- Rozenberg, A.D.; Quigley, D.C.; Melville, W.K. Laboratory study of polarized scattering by surface waves at grazing incidence: I. Wind waves. IEEE Trans. Geosci. Remote Sens. 2002, 33, 1037–1046. [Google Scholar]
- Corretja, V.; Meslot, V.; Kemkemian, S.; Montigny, R. Statistical Analysis on Sea Clutter Doppler Properties in X-Band for Low Grazing Angles. In Proceedings of the 2017 IEEE Radar Conference (RadarConf17), Washington, DC, USA, 8–12 May 2017. [Google Scholar]
- Lee, P.H.Y.; Barter, J.D.; Beach, K.L.; Hindman, C.L.; Lake, B.M.; Rungaldier, H.; Shelton, J.C.; Williams, A.B.; Yee, R.; Yuen, H.C. X band microwave backscattering from ocean waves. J. Geophys. Res. Ocean. 1995, 100, 2591–2611. [Google Scholar]
- Rosenberg, L. Characterization of High Grazing Angle X-Band Sea-Clutter Doppler Spectra. IEEE Trans. Aerosp. Electron. Syst. 2013, 50, 406–417. [Google Scholar]
- Luo, X.; Liu, Z.; Lu, Z.; Fu, Q. Modeling and simulation of sea clutter for missile-borne radar. Proc. Appl. Opt. Photonics China 2011, 8191, 345–352. [Google Scholar]
- McDonald, M.; Cerutti-Maori, D. Multi-phase centre coherent radar sea clutter modelling and simulation. IET Radar Sonar Navig. 2017, 11, 1359–1366. [Google Scholar]
- Wentz, F.J.; Smith, D.K. A model function for the ocean-normalized radar cross section at 14 GHz derived from NSCAT observations. J. Geophys. Res. Ocean. 1999, 104, 11499–11514. [Google Scholar]
- Wentz, F.J.; Peteherych, S.; Thomas, L.A. A model function for ocean radar cross sections at 14.6 GHz. J. Geophys. Res. Ocean. 1984, 89, 3689–3704. [Google Scholar]
- Voronovich, A. Wave Scattering from Rough Surfaces. Rep. Prog. Phys. 1999, 17, 147–207. [Google Scholar]
- Valenzuela, G.R. Theories for the interaction of electromagnetic and oceanic waves—A review. Bound. Layer Meteorol. 1978, 13, 61–85. [Google Scholar]
- Valenzuela, G. Depolarization of EM waves by slightly rough surfaces. IEEE Trans. Antennas Propag. 1967, 15, 552–557. [Google Scholar]
- Wright, J. A new model for sea clutter. IEEE Trans. Antennas Propag. 1968, 16, 217–223. [Google Scholar]
- Plant, W.J. Bragg Scattering of Electromagnetic Waves from the Air/Sea Interface. In Surface Waves and Fluxes: Volume II—Remote Sensing; Geernaert, G.L., Plant, W.L., Eds.; Springer: Dordrecht, The Netherlands, 1990; pp. 41–108. [Google Scholar]
- Hwang, P.A.; Zhang, B.; Toporkov, J.V.; Perrie, W. Correction to “Comparison of composite Bragg theory and quad-polarization radar backscatter from RADARSAT-2: With applications to wave breaking and high wind retrieval”. J. Geophys. Res. 2010, 115, C11099. [Google Scholar]
- Wright, J. Backscattering from capillary waves with application to sea clutter. IEEE Trans. Antennas Propag. 1966, 14, 749–754. [Google Scholar]
- Plant, W.J. A stochastic, multiscale model of microwave backscatter from the ocean. J. Geophys. Res. Ocean. 2002, 107, 3–21. [Google Scholar]
- Hwang, P.A.; Plant, W.J. An analysis of the effects of swell and surface roughness spectra on microwave backscatter from the ocean. J. Geophys. Res. Ocean. 2010, 115, C04014. [Google Scholar]
- Kasilingam, D.P.; Shemdin, O.H. Theory for synthetic aperture radar imaging of the ocean surface: With application to the Tower Ocean Wave and Radar Dependence Experiment on focus, resolution, and wave height spectra. J. Geophys. Res. Ocean. 1988, 93, 13837–13848. [Google Scholar]
- Tsang, L.; Chan, C.H.; Pak, K.; Sangani, H. Monte-Carlo simulations of large-scale problems of random rough surface scattering and applications to grazing incidence with the BMIA/canonical grid method. Antennas Propag. IEEE Trans. 1995, 43, 851–859. [Google Scholar]
- Zebker, H.A.; Villasenor, J. Decorrelation in interferometric radar echoes. IEEE Trans. Geosci. Remote Sens. 1992, 30, 950–959. [Google Scholar]
- Bamler, R.; Hartl, P. Synthetic aperture radar interferometry. Inverse Probl. 1998, 14, R1. [Google Scholar]
- Elfouhaily, T.; Chapron, B.; Katsaros, K.; Vandemark, D. A unified directional spectrum for long and short wind-driven waves. J. Geophys. Res. Ocean. 1997, 102, 15781–15796. [Google Scholar]
- Hasselmann, K.; Hasselmann, S. On the nonlinear mapping of an ocean wave spectrum into a synthetic aperture radar image spectrum and its inversion. J. Geophys. Res. Ocean. 1991, 96, 10713–10729. [Google Scholar]
- Caponi, E.A.; Crawford, D.R.; Yuen, H.C.; Saffman, P.G. Modulation of radar backscatter from the ocean by a variable surface current. J. Geophys. Res. Ocean. 1988, 93, 12249–12263. [Google Scholar]
- Wang, Y.; Zhang, Y.; Li, H.; Chen, G. Doppler spectrum of microwave SAR signals from two-dimensional time-varying sea surface. J. Electromagn. Waves Appl. 2016, 30, 1265–1276. [Google Scholar]
- Apel, J.R. Principles of Ocean Physics; Academic Press: Cambridge, MA, USA, 1987. [Google Scholar]
- Boisot, O.; Nouguier, F.; Chapron, B.; Guérin, C.A. The GO4 Model in Near-Nadir Microwave Scattering from the Sea Surface. IEEE Trans. Geosci. Remote Sens. 2015, 53, 5889–5900. [Google Scholar]
- Liu, B.; He, Y. SAR Raw Data Simulation for Ocean Scenes Using Inverse Omega-K Algorithm. IEEE Trans. Geosci. Remote Sens. 2016, 54, 6151–6169. [Google Scholar]
- Soriano, G.; Saillard, M. Scattering of electromagnetic waves from two-dimensional rough surfaces with an impedance approximation. J. Opt. Soc. Am. A Opt. Image Sci. Vis. 2001, 18, 124–133. [Google Scholar] [PubMed]
- Berginc, G. Small-Slope Approximation Method: A Further Study of Vector Wave Scattering FROM Two-Dimensional Surfaces and Comparison with Experimental Data. Prog. Electromagn. Res. 2002, 27, 251–287. [Google Scholar]





| System Simulation Parameters | Values |
|---|---|
| Height | 1 km |
| Center frequency | 1.0 GHz |
| Beamwidth | 1° |
| Incident angle | 30° |
| Bandwidth | 10 MHz |
| Windspeed | 7 m/s |
| Wind direction | 0° (Downwind) |
| PRF | 100 Hz |
| Antenna gain | 40 dB |
| Method in This Paper | SSA | |
|---|---|---|
| Time consuming | 1878.93 s | 21,759.96 s |
| System Simulation Parameters | Values |
|---|---|
| Center frequency | 14.6 GHz |
| Bandwidth | 100 MHz |
| PRF | 500 Hz |
| Antenna gain | 40 dB |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, P.; Wang, Y.; Li, X.; Cui, J.; Zhang, Y.; Li, Q.; Zhang, Y. The Doppler Characteristics of Sea Echoes Acquired by Motion Radar. Remote Sens. 2023, 15, 4888. https://doi.org/10.3390/rs15194888
Du P, Wang Y, Li X, Cui J, Zhang Y, Li Q, Zhang Y. The Doppler Characteristics of Sea Echoes Acquired by Motion Radar. Remote Sensing. 2023; 15(19):4888. https://doi.org/10.3390/rs15194888
Chicago/Turabian StyleDu, Pengbo, Yunhua Wang, Xin Li, Jianbo Cui, Yanmin Zhang, Qian Li, and Yushi Zhang. 2023. "The Doppler Characteristics of Sea Echoes Acquired by Motion Radar" Remote Sensing 15, no. 19: 4888. https://doi.org/10.3390/rs15194888
APA StyleDu, P., Wang, Y., Li, X., Cui, J., Zhang, Y., Li, Q., & Zhang, Y. (2023). The Doppler Characteristics of Sea Echoes Acquired by Motion Radar. Remote Sensing, 15(19), 4888. https://doi.org/10.3390/rs15194888






