Simulation of Seismoelectric Waves Using Time-Domain Finite-Element Method in 2D PSVTM Mode
Abstract
1. Introduction
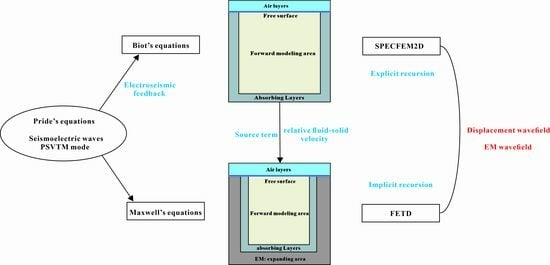
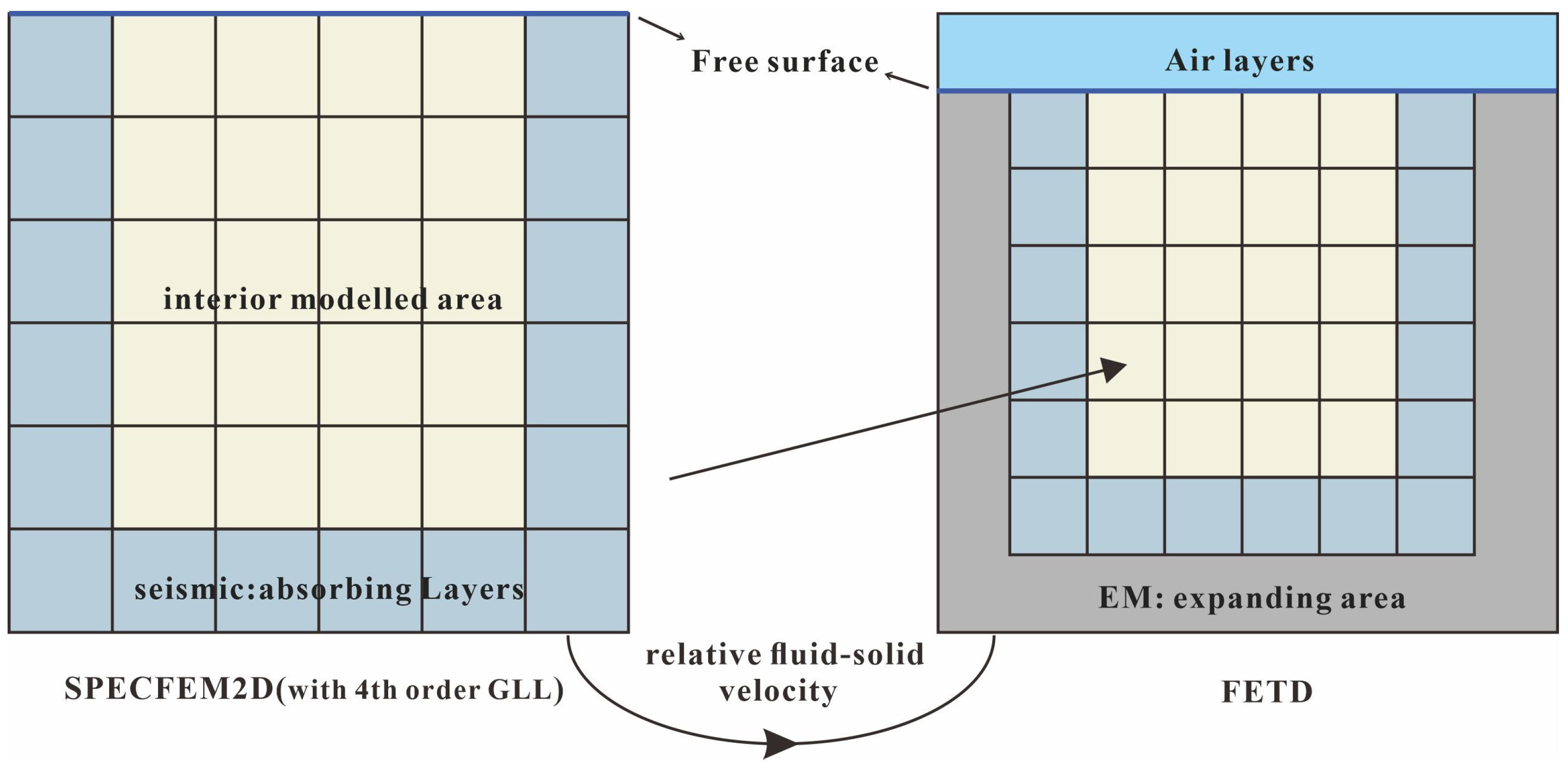
2. Methods
2.1. Governing Equations
2.2. FETD Scheme
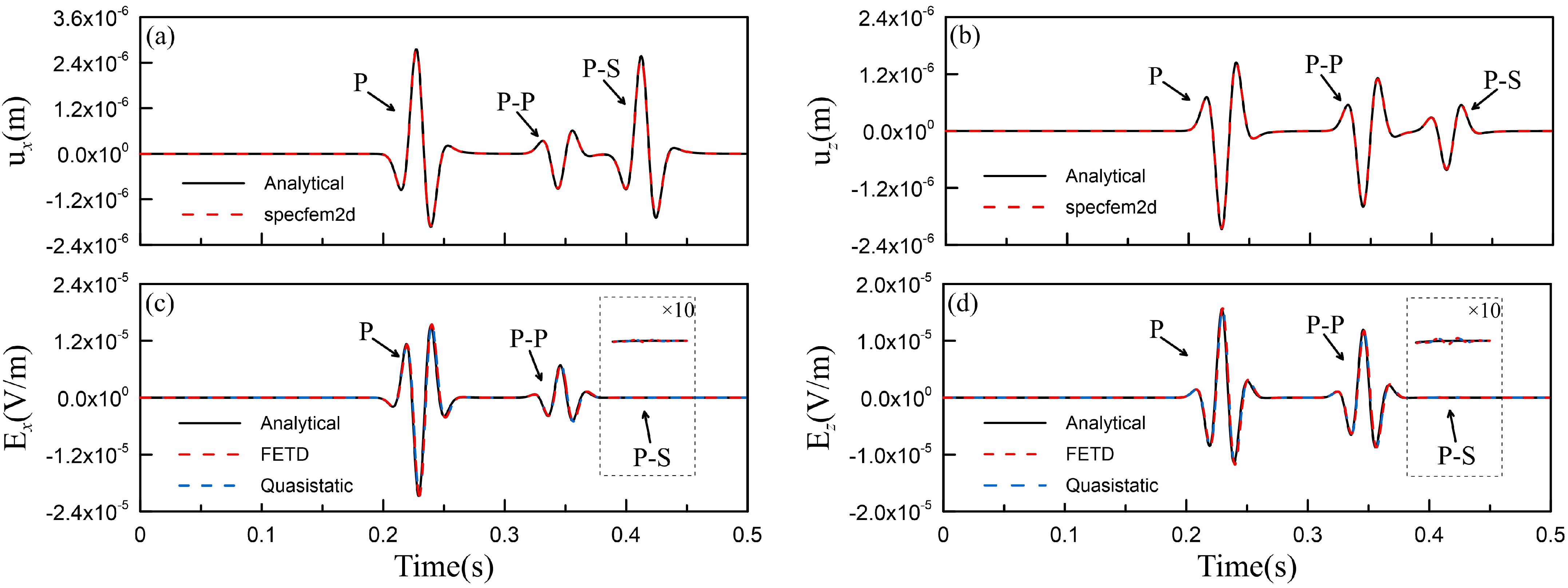
3. Accuracy Verification
3.1. A half-Space Model
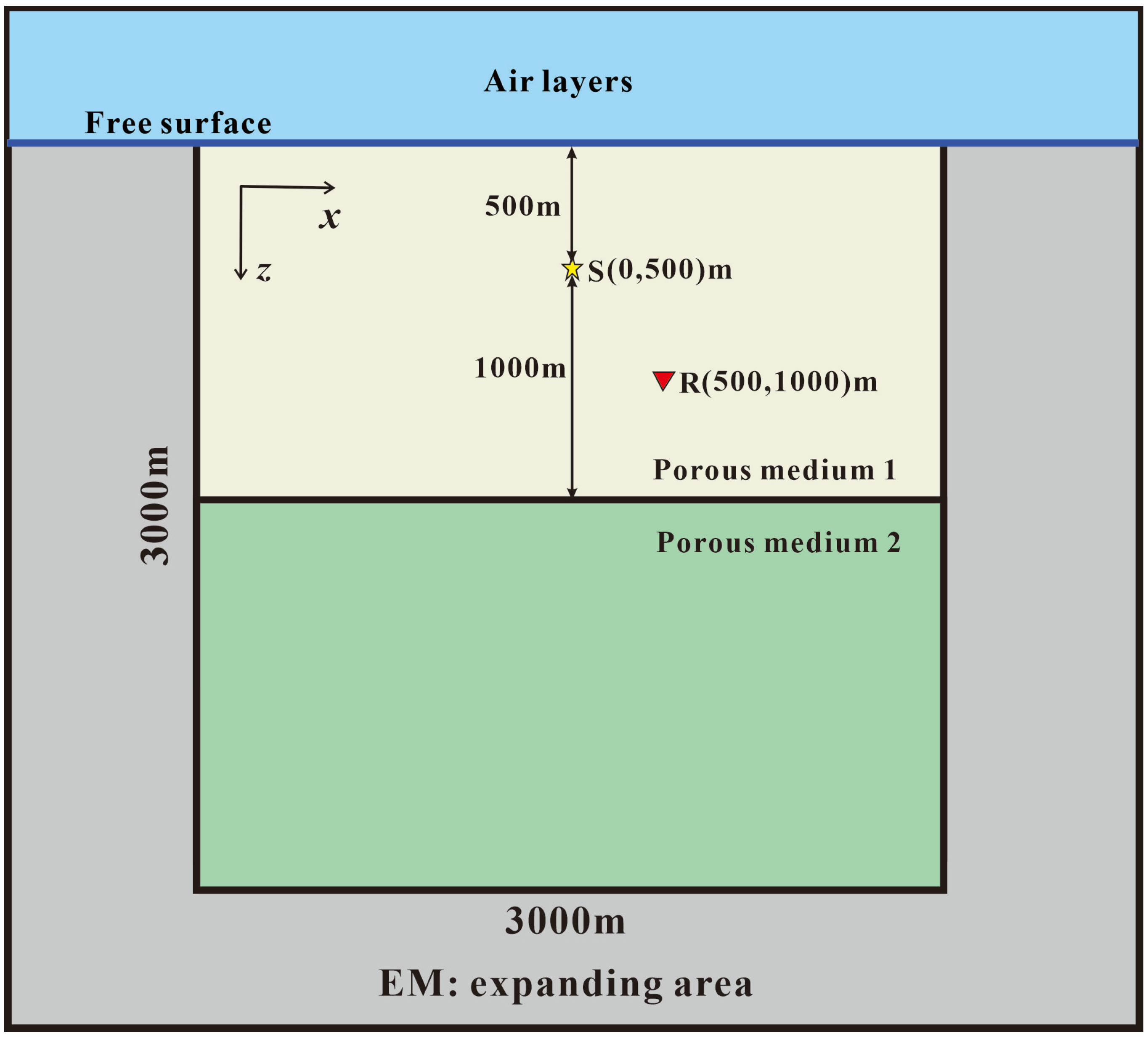
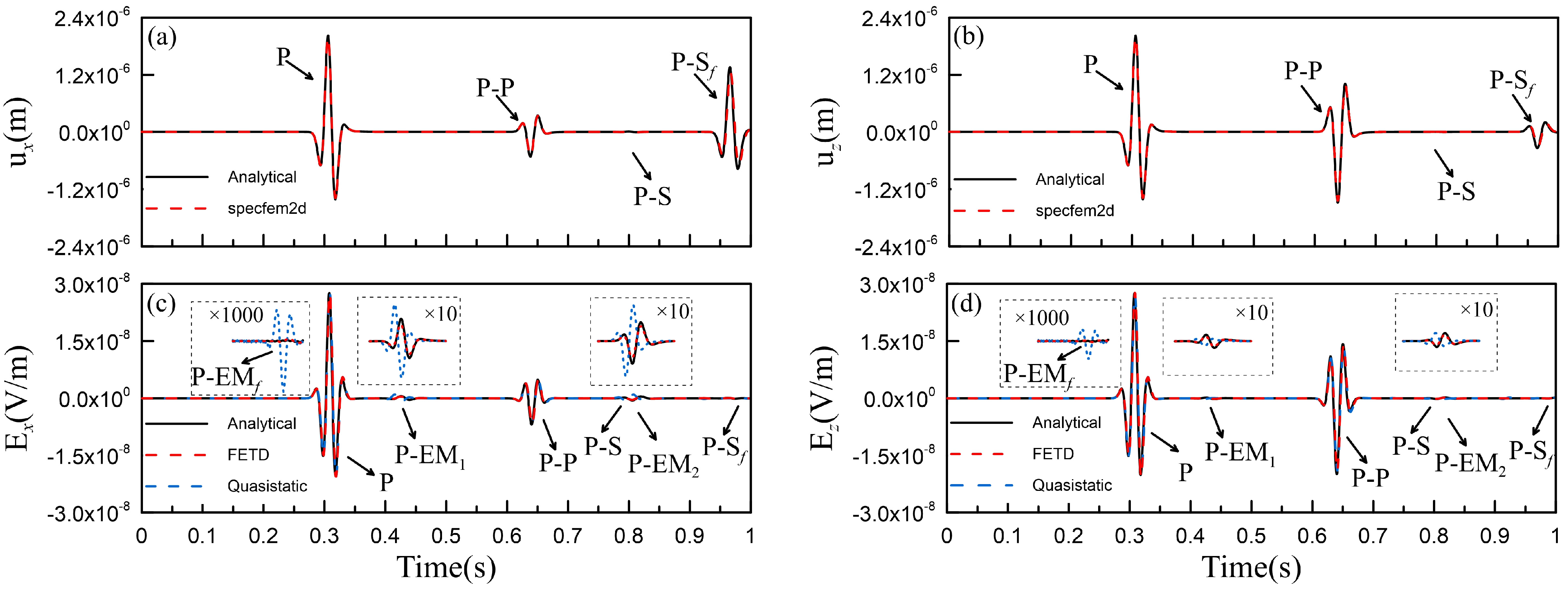
3.2. A Two-Layer Model
4. Numerical Results
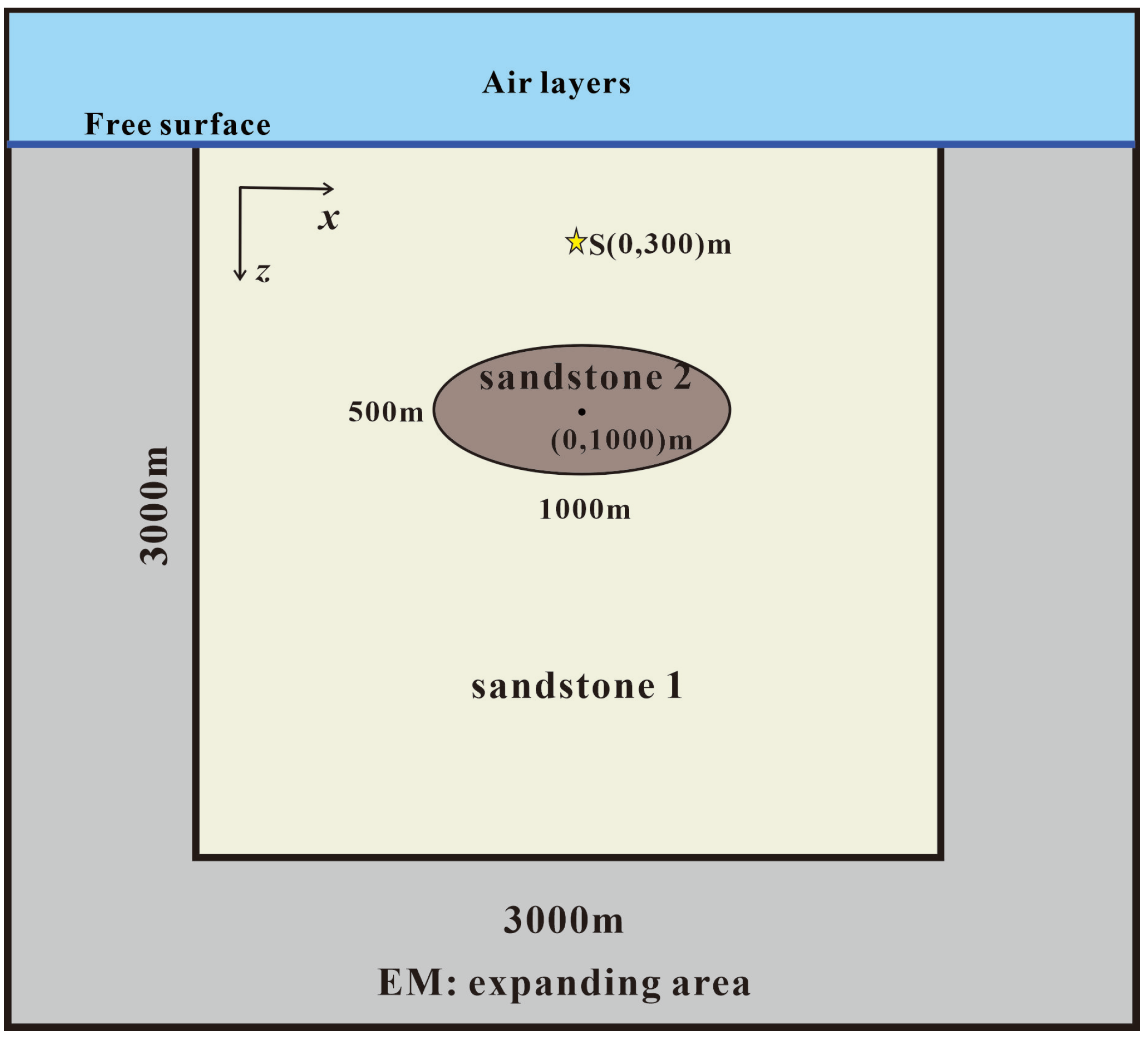
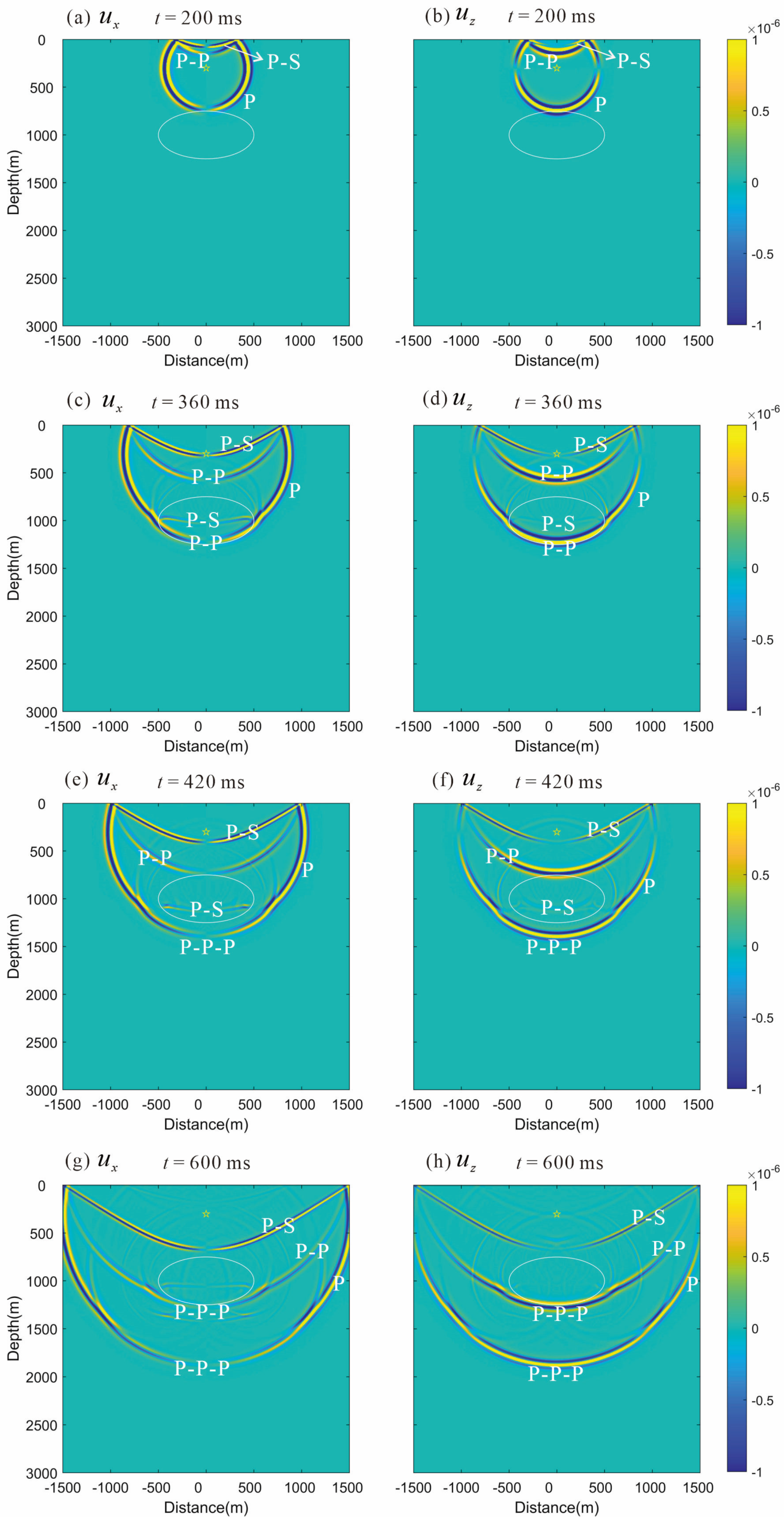
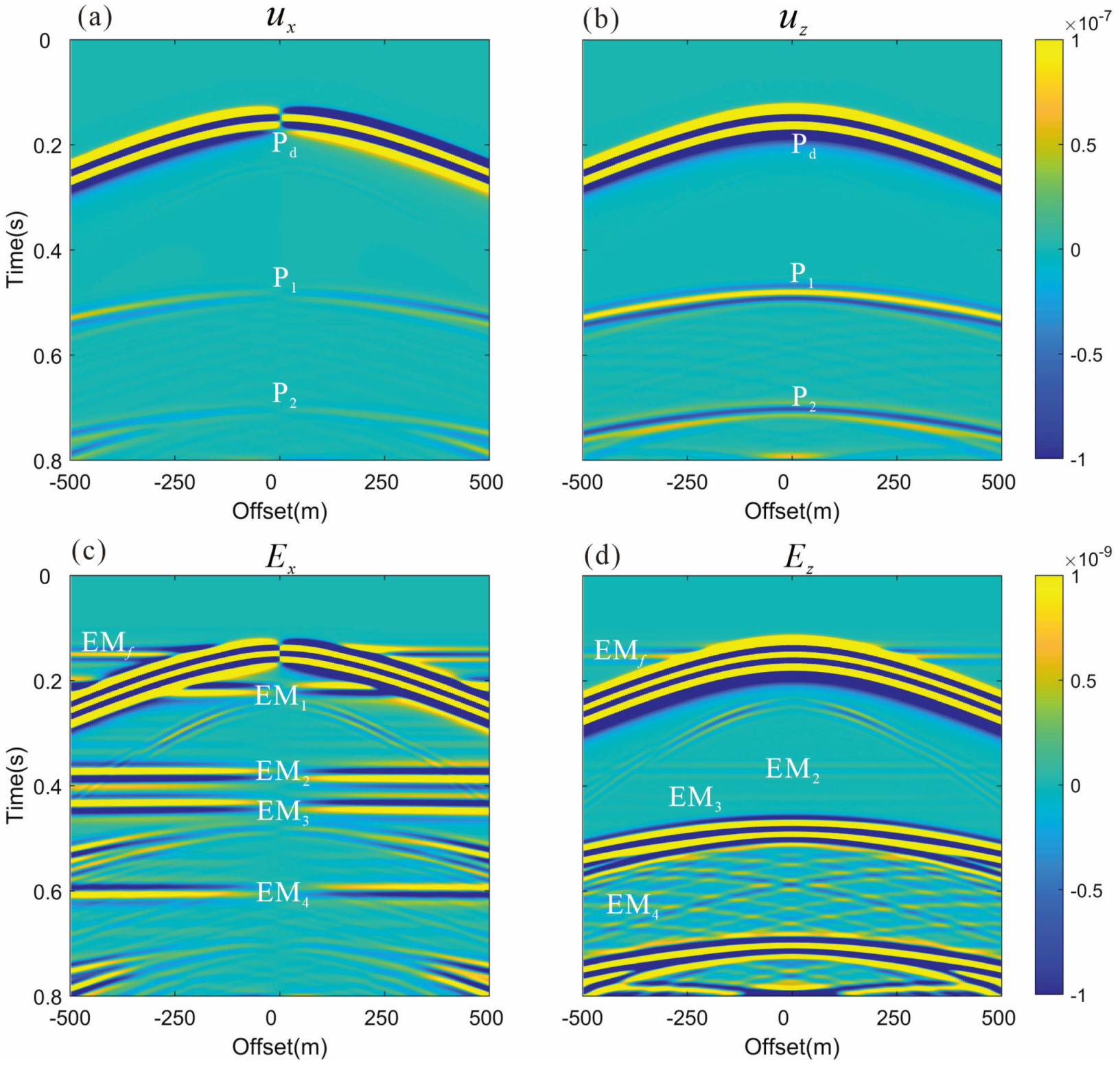
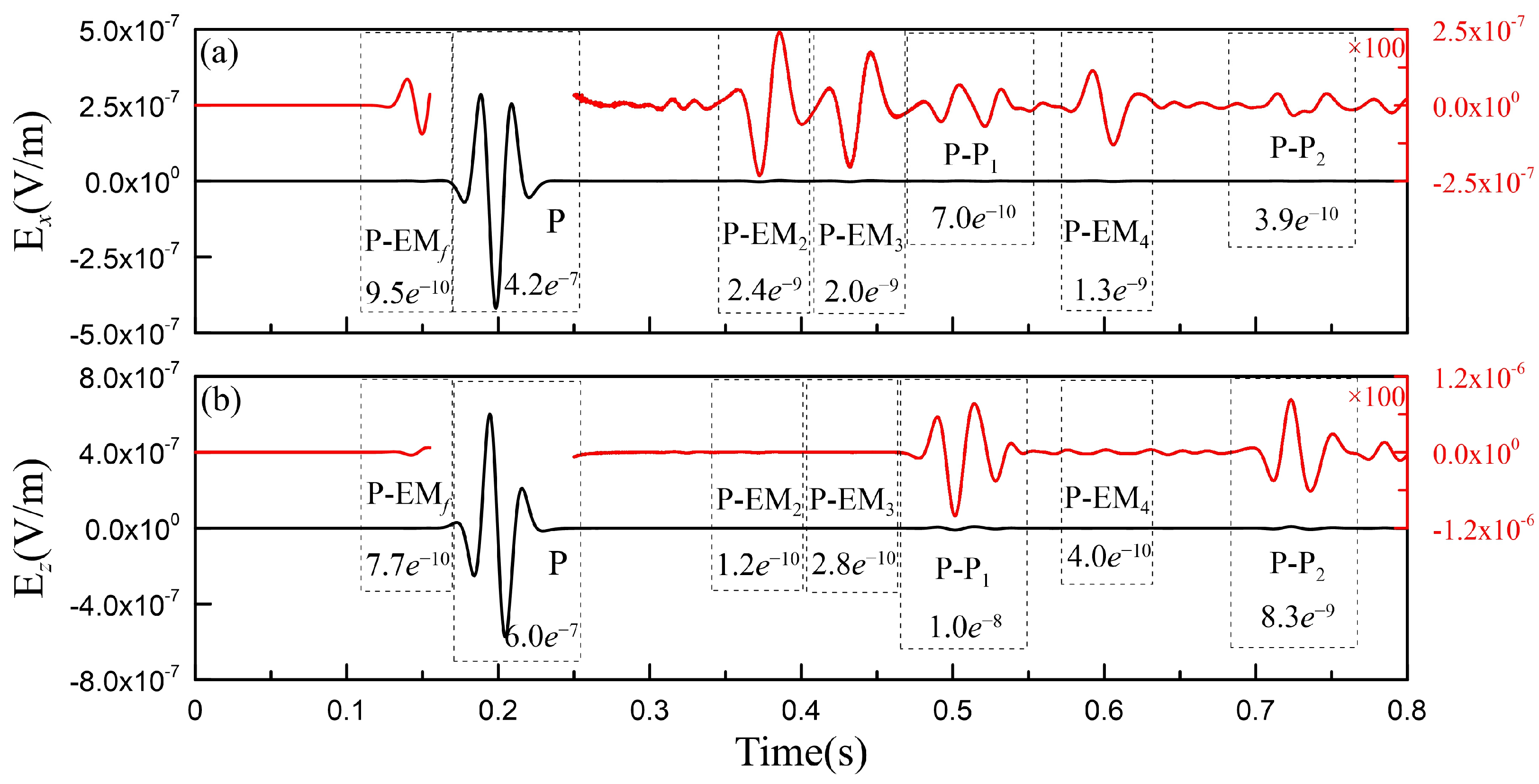
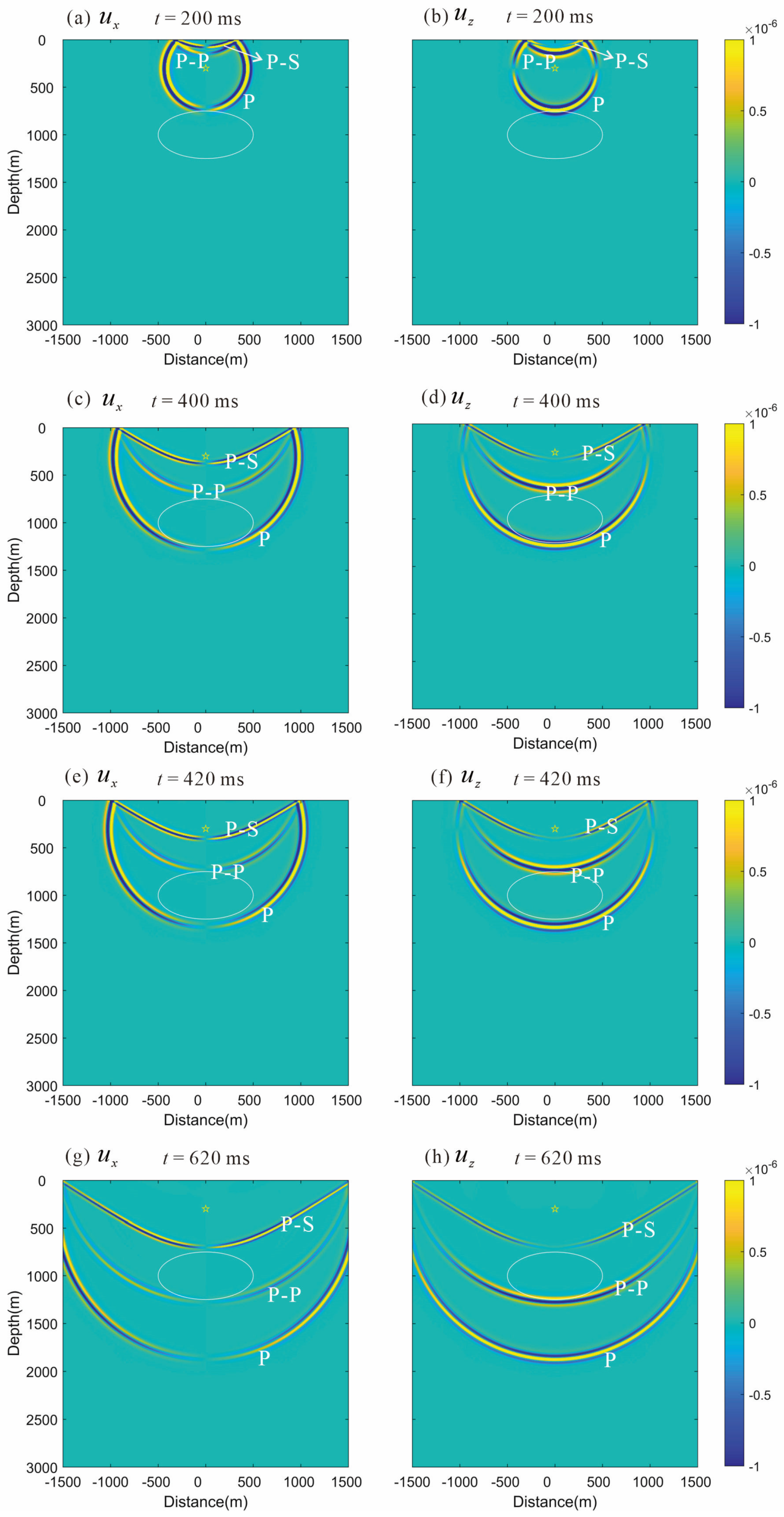
4.1. A Hydrocarbon Reservoir Model with Different Mechanical Properties
4.2. A Hydrocarbon Reservoir Model with the Same Mechanical Properties
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
References
- Pride, S. Governing equations for the coupled electromagnetics and acoustics of porous media. Phys. Rev. B Condens. Matter 1994, 50, 15678–15696. [Google Scholar] [CrossRef]
- Butler, K.E.; Russell, R.D.; Kepic, A.; Maxwell, M. Measurement of the seismoelectric response from a shallow boundary. Geophysics 1996, 61, 1769–1778. [Google Scholar] [CrossRef]
- Zhu, Z.; Haartsen, M.W.; Toksöz, M.N. Experimental studies of seismoelectric conversions in fluid-saturated porous media. J. Geophys. Res. Solid Earth 2000, 105, 28055–28064. [Google Scholar] [CrossRef]
- Gao, Y.; Hu, H. Seismoelectromagnetic waves radiated by a double couple source in a saturated porous medium. Geophys. J. Int. 2010, 181, 873–896. [Google Scholar] [CrossRef]
- Ren, H.; Huang, Q.; Chen, X. A new numerical technique for simulating the coupled seismic and electromagnetic waves in layered porous media. Earthq. Sci. 2010, 23, 167–176. [Google Scholar] [CrossRef]
- Grobbe, N.; Slob, E. Seismo-electromagnetic thin-bed responses: Natural signal enhancements? J. Geophys. Res. Solid Earth 2016, 121, 2460–2479. [Google Scholar] [CrossRef]
- Haartsen, M.W.; Pride, S.R. Electroseismic waves from point sources in layered media. J. Geophys. Res. Atmos. 1997, 102, 24745–24769. [Google Scholar] [CrossRef]
- Thompson, A.H.; Gist, G.A. Geophysical applications of electrokinetic conversion. Lead. Edge 1993, 12, 1169–1173. [Google Scholar] [CrossRef]
- Peng, R.; Di, B.; Glover, P.W.J.; Wei1, J.; Lorinczi, P.; Ding, P.; Liu, Z.; Zhang, Y.; Wu, M. The effect of rock permeability and porosity on seismoelectric conversion: Experiment and analytical modelling. Geophys. J. Int. 2019, 219, 328–345. [Google Scholar] [CrossRef]
- Wang, J.; Zhu, Z.; Gao, Y.; Morgan, F.D.; Hu, H. Measurements of the seismoelectric responses in a synthetic porous rock. Geophys. J. Int. 2020, 222, 436–448. [Google Scholar] [CrossRef]
- Pride, S.R.; Haartsen, M.W. Electroseismic wave properties. J. Acoust. Soc. Am. 1996, 100, 1301–1315. [Google Scholar] [CrossRef]
- Haines, S.S.; Pride, S.R.; Klemperer, S.; Biondi, B. Seismoelectric imaging of shallow targets. Geophysics 2007, 72, G9–G20. [Google Scholar] [CrossRef]
- Bordes, C.; Jouniaux, L.; Garambois, S.; Dietrich, M.; Pozzi, J.-P.; Gaffet, S. Evidence of the theoretically predicted seismo-magnetic conversion. Geophys. J. Int. 2008, 174, 489–504. [Google Scholar] [CrossRef]
- Schakel, M.D.; Smeulders, D.M.J.; Slob, E.; Heller, H.K.J. Seismoelectric interface response: Experimental results and forward model. Geophysics 2011, 76, N29–N36. [Google Scholar] [CrossRef]
- Ren, H.; Huang, Q.; Chen, X. Numerical simulation of seismo-electromagnetic fields associated with a fault in a porous medium. Geophys. J. Int. 2016, 206, 205–220. [Google Scholar] [CrossRef]
- Gao, Y.; Wang, M.; Hu, H.; Chen, X. Seismoelectric responses to an explosive source in a fluid above a fluid-saturated porous medium. J. Geophys. Res. Solid Earth 2017, 122, 7190–7218. [Google Scholar] [CrossRef]
- Gao, Y.; Wang, N.; Yao, C.; Guan, W.; Hu, H.; Wen, J.; Zhang, W.; Tong, P.; Yang, Q. Simulation of seismoelectric waves using finite-difference frequency-domain method: 2D SHTE mode. Geophys. J. Int. 2019, 216, 418–438. [Google Scholar] [CrossRef]
- Haines, S.S.; Pride, S.R. Seismoelectric numerical modeling on a grid. Geophysics 2006, 71, N57–N65. [Google Scholar] [CrossRef]
- Smeulders, D.D.; Grobbe, N.; Heller, H.; Schakel, M. Seismoelectric Conversion for the Detection of Porous Medium Interfaces between Wetting and Nonwetting Fluids. Vadose Zone J. 2014, 13, 1–7. [Google Scholar] [CrossRef]
- Ma, X.; Liu, Y.; Yin, C.; Zhang, B.; Ren, X. Estimation of fluid salinity using coseismic electric signal generated by an earthquake. Geophys. J. Int. 2022, 233, 127–144. [Google Scholar] [CrossRef]
- Peng, R.; Huang, X.; Liu, Z.; Li, H.; Di, B.; Wei, J. Numerical investigation on seismoelectric wave fields in porous media: Porosity and permeability. J. Geophys. Eng. 2023, 20, 1–11. [Google Scholar] [CrossRef]
- Grobbe, N.; Revil, A.; Zhu, Z.; Slob, E. Seismoelectric Exploration: Theory, Experiments, and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2020; Volume 252. [Google Scholar]
- Revil, A.; Linde, N. Chemico-electromechanical coupling in microporous media. J. Colloid Interface Sci. 2006, 302, 682–694. [Google Scholar] [CrossRef] [PubMed]
- Warden, S.; Garambois, S.; Jouniaux, L.; Brito, D.; Sailhac, P.; Bordes, C. Seismoelectric wave propagation numerical modelling in partially saturated materials. Geophys. J. Int. 2013, 194, 1498–1513. [Google Scholar] [CrossRef]
- Zyserman, F.; Monachesi, L.; Jouniaux, L. Dependence of shear wave seismoelectrics on soil textures: A numerical study in the vadose zone. Geophys. J. Int. 2016, 208, 918–935. [Google Scholar] [CrossRef]
- Jougnot, D. New approach to up-scale the frequency-dependent effective excess charge density for seismoelectric modeling. In Proceedings of the SEG Technical Program Expanded Abstracts; Society of Exploration Geophysicists: Houston, TX, USA, 2019; pp. 3608–3612. [Google Scholar] [CrossRef]
- Jougnot, D.; Solazzi, S.G. Predicting the frequency-dependent effective excess charge density: A new upscaling approach for seismoelectric modeling. Geophysics 2021, 86, WB19–WB28. [Google Scholar] [CrossRef]
- Biot, M.A. Theory of Propagation of Elastic Waves in a Fluid-Saturated Porous Solid. I. Low-Frequency Range. J. Acoust. Soc. Am. 1956, 28, 168–178. [Google Scholar] [CrossRef]
- Slob, E.; Mulder, M. Seismoelectromagnetic homogeneous space Green’s functions. Geophysics 2016, 81, F27–F40. [Google Scholar] [CrossRef]
- Hu, H.; Gao, Y. Electromagnetic field generated by a finite fault due to electrokinetic effect. J. Geophys. Res. 2011, 116, 7958. [Google Scholar] [CrossRef]
- Cheng, Q.; Gao, Y.; Zhou, G.; Chen, C.-H.; Wang, D.; Yao, C. Seismoelectric waves generated by a point source in horizontally stratified vertical transversely isotropic porous media. Geophysics 2023, 88, C53–C78. [Google Scholar] [CrossRef]
- Zyserman, F.I.; Gauzellino, P.M.; Santos, J.E. Finite element modeling of SHTE and PSVTM electroseismics. J. Appl. Geophys. 2010, 72, 79–91. [Google Scholar] [CrossRef]
- Zyserman, F.I.; Jouniaux, L.; Warden, S.; Garambois, S. Borehole seismoelectric logging using a shear-wave source: Possible application to CO2 disposal? Int. J. Greenh. Gas Control 2015, 33, 89–102. [Google Scholar] [CrossRef]
- Pain, C.C.; Saunders, J.H.; Worthington, M.H.; Singer, J.M.; Stuart-Bruges, W.; Mason, G.; Goddard, A. A mixed finite-element method for solving the poroelastic Biot equations with electrokinetic coupling. Geophys. J. Int. 2005, 160, 592–608. [Google Scholar] [CrossRef]
- Tohti, M.; Wang, Y.; Slob, E.; Zheng, Y.; Chang, X.; Yao, Y. Seismoelectric numerical simulation in 2D vertical transverse isotropic poroelastic medium. Geophys. Prospect. 2020, 68, 1927–1943. [Google Scholar] [CrossRef]
- Tohti, M.; Wang, Y.; Xiao, W.; Zhou, K. Numerical simulation of seismoelectric wavefields in 3D orthorhombic poroelastic medium. Chin. J. Geophys. 2022, 65, 4471–4484. [Google Scholar] [CrossRef]
- Ji, Y.; Han, L.; Huang, X.; Zhao, X.; Jensen, K.; Yu, Y. A high-order finite-difference scheme for time-domain modeling of time-varying seismoelectric waves. Geophysics 2022, 87, T135–T146. [Google Scholar] [CrossRef]
- Gao, Y.; Huang, F.; Hu, H. Comparison of full and quasi-static seismoelectric analytically based modeling. J. Geophys. Res. Solid Earth 2017, 122, 8066–8106. [Google Scholar] [CrossRef]
- Morency, C.; Tromp, J. Spectral-element simulations of wave propagation in porous media. Geophys. J. Int. 2008, 175, 301–345. [Google Scholar] [CrossRef]
- Thompson, A.H.; Hornbostel, S.; Burns, J.; Murray, T.; Raschke, R.; Wride, J.; McCammon, P.; Sumner, J.; Haake, G.; Bixby, M.; et al. Field tests of electroseismic hydrocarbon detection. Geophysics 2007, 72, N1–N9. [Google Scholar] [CrossRef]
- Clayton, R.; Engquist, B. Absorbing boundary conditions for acoustic and elastic wave equations. Bull. Seism. Soc. Am. 1977, 67, 1529–1540. [Google Scholar] [CrossRef]
- Barton, M.L.; Cendes, Z.J. New vector finite elements for three-dimensional magnetic field computation. J. Appl. Phys. 1987, 61, 3919–3921. [Google Scholar] [CrossRef]
- Biro, O.; Preis, K. On the use of the magnetic vector potential in the finite-element analysis of three-dimensional eddy currents. IEEE Trans. Magn. 1989, 25, 3145–3159. [Google Scholar] [CrossRef]
- Allaire, G.S.M.K. Numerical Linear Algebra; Springer: New York, NY, USA, 2008. [Google Scholar]
- Hughes, T.J.R. The Finite Element Method: Linear Static and Dynamic Finite Element Analysis; Courier Corporation: Englewood Cliffs, NJ, USA, 1987. [Google Scholar]
- De Basabe, J.D.; Sen, M.K. Stability of the high-order finite elements for acoustic or elastic wave propagation with high-order time stepping. Geophys. J. Int. 2010, 181, 577–590. [Google Scholar] [CrossRef]
- Amestoy, P.R.; Guermouche, A.; L’excellent, J.-Y.; Pralet, S. Hybrid scheduling for the parallel solution of linear systems. Parallel Comput. 2006, 32, 136–156. [Google Scholar] [CrossRef]
- Garambois, S.; Dietrich, M. Full waveform numerical simulations of seismoelectromagnetic wave conversions in fluid-saturated stratified porous media. J. Geophys. Res. Solid Earth 2002, 107, ESE 5-1–ESE 5-18. [Google Scholar] [CrossRef]
- Dzieran, L.; Thorwart, M.; Rabbel, W.; Ritter, O. Quantifying interface responses with seismoelectric spectral ratios. Geophys. J. Int. 2019, 217, 108–121. [Google Scholar] [CrossRef]
- Monachesi, L.B.; Zyserman, F.I.; Jouniaux, L. An analytical solution to assess the SH seismoelectric response of the vadose zone. Geophys. J. Int. 2018, 213, 1999–2019. [Google Scholar] [CrossRef]
- Wang, D.; Gao, Y.; Tong, P.; Wang, J.; Yao, C.; Wang, B. Electroseismic and seismoelectric responses at irregular interfaces: Possible application to reservoir exploration. J. Pet. Sci. Eng. 2021, 202, 108513. [Google Scholar] [CrossRef]
- Sheriff, R.E.; Geldart, L.P. Exploration Seismology; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Garambois, S.; Dietrich, M. Seismoelectric wave conversions in porous media: Field measurements and transfer function analysis. Geophysics 2001, 66, 1417–1430. [Google Scholar] [CrossRef]
- Crespy, A.; Revil, A.; Linde, N.; Byrdina, S.; Jardani, A.; Bolève, A.; Henry, P. Detection and localization of hydromechanical disturbances in a sandbox using the self-potential method. J. Geophys. Res. Atmos. 2008, 113. [Google Scholar] [CrossRef]
- Jardani, A.; Revil, A.; Slob, E.; Söllner, W. Stochastic joint inversion of 2D seismic and seismoelectric signals in linear poroelastic materials: A numerical investigation. Geophysics 2010, 75, N19–N31. [Google Scholar] [CrossRef]




| Symbol | Names |
|---|---|
| , , | Solid displacement |
| , , | Relative fluid–solid displacement |
| , , | Relative fluid–solid velocity |
| , , | Electric field |
| , | Magnetic field |
| , | Bulk stress |
| Shear stress | |
| Pore fluid pressure | |
| Solid bulk modulus | |
| Fluid bulk modulus | |
| Frame bulk modulus | |
| Shear modulus | |
| Bulk electric permittivity | |
| , | Relative permittivity of the solid grain and pore fluid |
| Vacuum permittivity | |
| Bulk density | |
| , | Solid grain and pore fluid density |
| Porosity | |
| Tortuosity | |
| Bulk magnetic permeability | |
| Pore fluid viscosity | |
| Bulk conductivity | |
| Permeability | |
| Electrokinetic coupling coefficient | |
| Pore fluid salinity |
| Symbol | Porous Medium 1 | Porous Medium 2 | Porous Medium 3 | Sandstone 1 | Sandstone 2 |
|---|---|---|---|---|---|
| 2650 | 2650 | 2400 | 2650 | 2400 | |
| 1000 | 1000 | 1000 | 1000 | 980 | |
| 0.1 | 0.1 | 0.2 | 0.2 | 0.35 | |
| 3 | 3 | 3 | 3 | 3 | |
| 12.2 | 12.2 | 21.252 | 12.2 | 21.252 | |
| 1.985 | 1.985 | 2.25 | 1.985 | 2.25 | |
| 9.6 | 9.6 | 7.199 | 9.6 | 7.199 | |
| 0.001 | 0.001 | 0.001 | 0.001 | 0.001 | |
| 5.1 | 5.1 | 5.92 | 5.1 | 5.92 | |
| 2628.87 | 2628.87 | 2998.17 | 2695.98 | 3047.1 | |
| 1434.92 | 1434.92 | 1673.63 | 1484.23 | 1765.05 | |
| 4 | 4 | 4 | 4 | 4 | |
| 80 | 80 | 80 | 80 | 80 | |
| 0.01 | 5 | 0.001 | 0.2 | 0.001 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Yin, C.; Liu, Y.; Wang, L.; Ma, X. Simulation of Seismoelectric Waves Using Time-Domain Finite-Element Method in 2D PSVTM Mode. Remote Sens. 2023, 15, 3321. https://doi.org/10.3390/rs15133321
Li J, Yin C, Liu Y, Wang L, Ma X. Simulation of Seismoelectric Waves Using Time-Domain Finite-Element Method in 2D PSVTM Mode. Remote Sensing. 2023; 15(13):3321. https://doi.org/10.3390/rs15133321
Chicago/Turabian StyleLi, Jun, Changchun Yin, Yunhe Liu, Luyuan Wang, and Xinpeng Ma. 2023. "Simulation of Seismoelectric Waves Using Time-Domain Finite-Element Method in 2D PSVTM Mode" Remote Sensing 15, no. 13: 3321. https://doi.org/10.3390/rs15133321
APA StyleLi, J., Yin, C., Liu, Y., Wang, L., & Ma, X. (2023). Simulation of Seismoelectric Waves Using Time-Domain Finite-Element Method in 2D PSVTM Mode. Remote Sensing, 15(13), 3321. https://doi.org/10.3390/rs15133321