LEO Satellite Clock Modeling and Its Benefits for LEO Kinematic POD
Abstract
1. Introduction
- ➢
- The clock stability might not be stable enough for a strong clock model. This problem is partially solved when clocks of better stability, e.g., Hydrogen-Maser (H-Maser) or optical clocks [2], can be used in LEO satellites in the future.
- ➢
- Complex once- and twice-per-revolution effects remain in the LEO clocks due to the low altitude of LEO satellites. These effects cannot be perfectly corrected with the extended formula of the relativistic effects for LEO satellites [17].
- ➢
2. Research Goal
3. Processing Methods
3.1. POD without Clock Modeling
3.2. POD with Clock Modeling
3.2.1. 2.5-State Model
3.2.2. Piece-Wise Linear Model
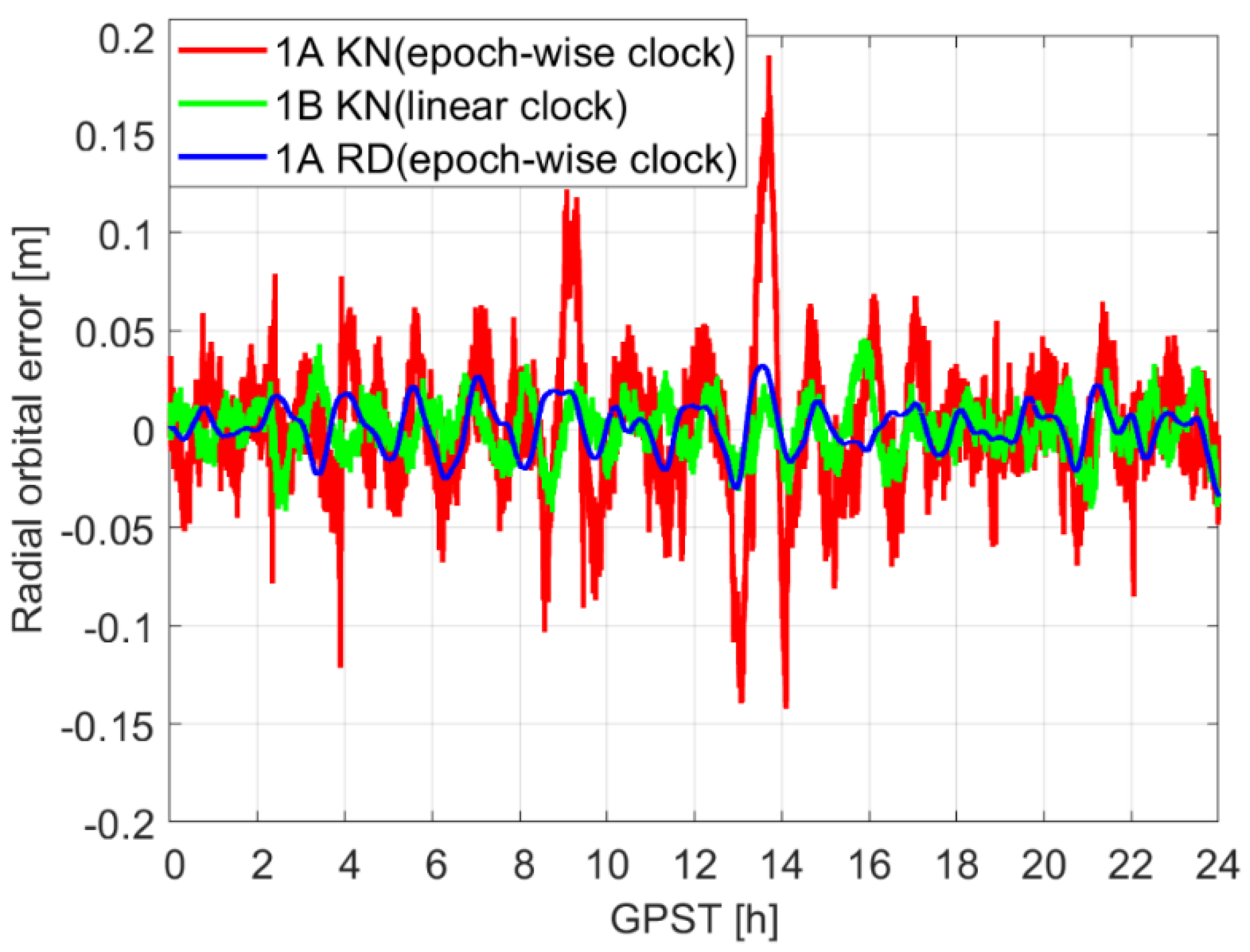
4. Test Results
4.1. Test Description
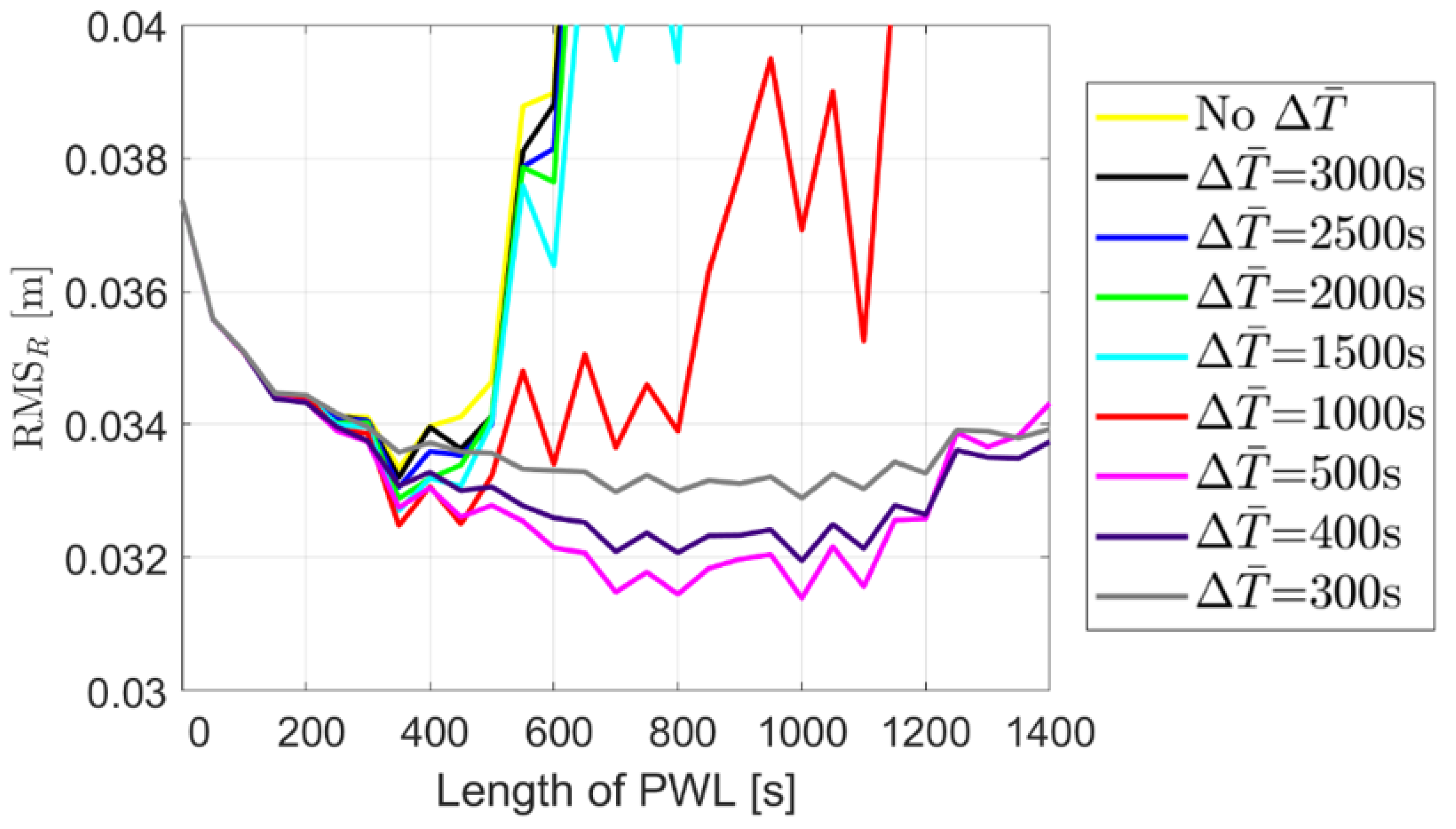
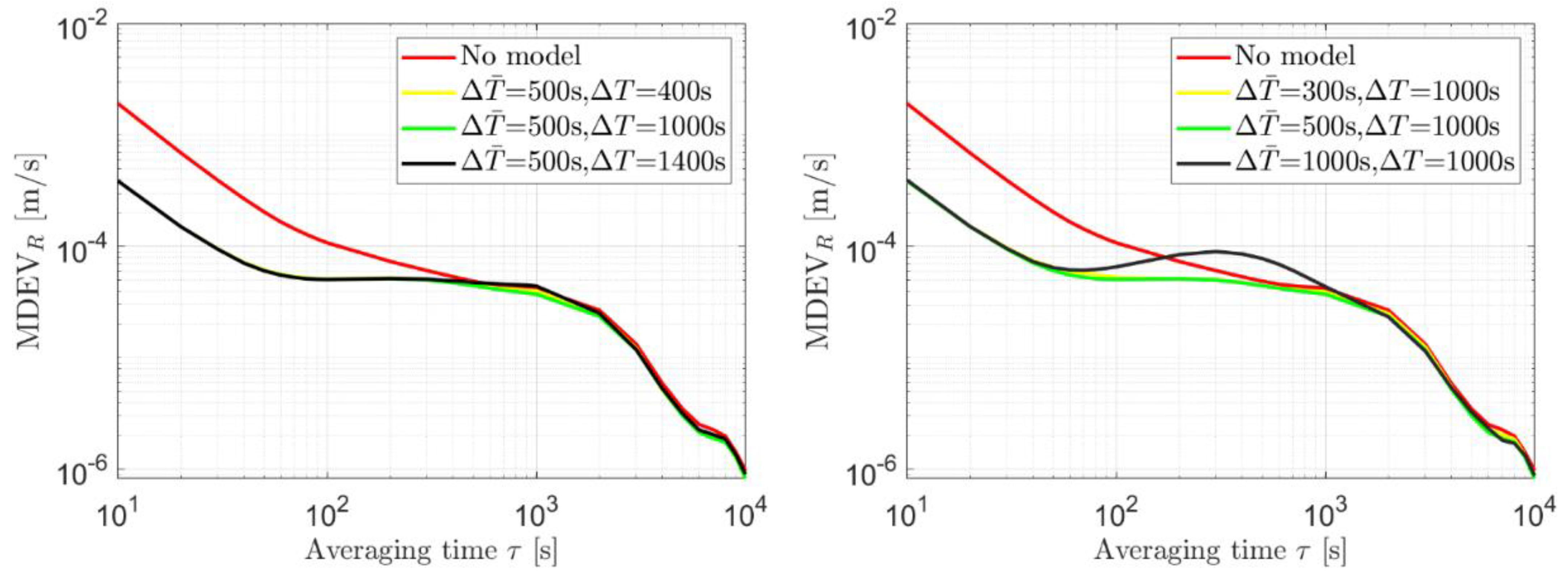
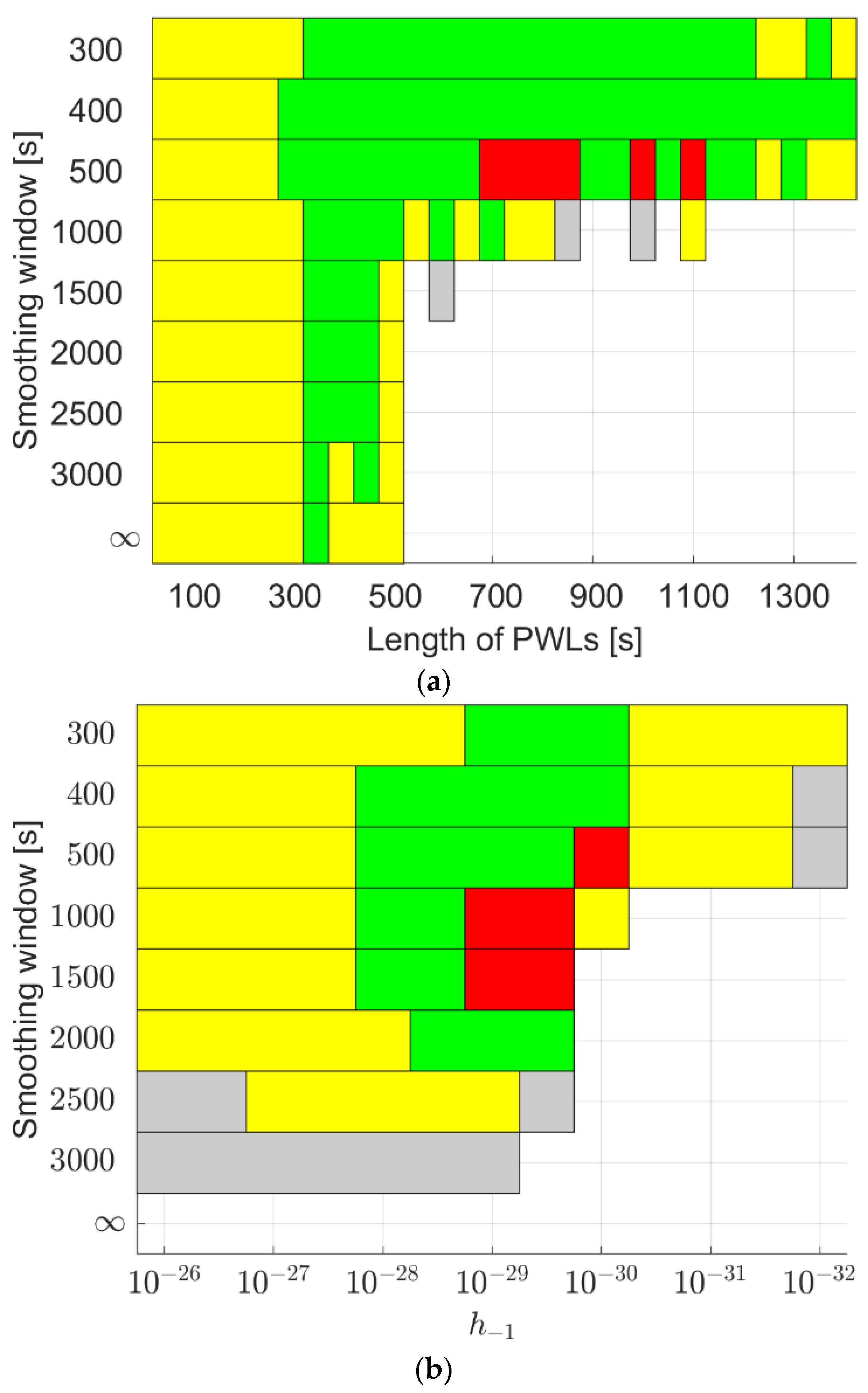
4.2. PWL Model
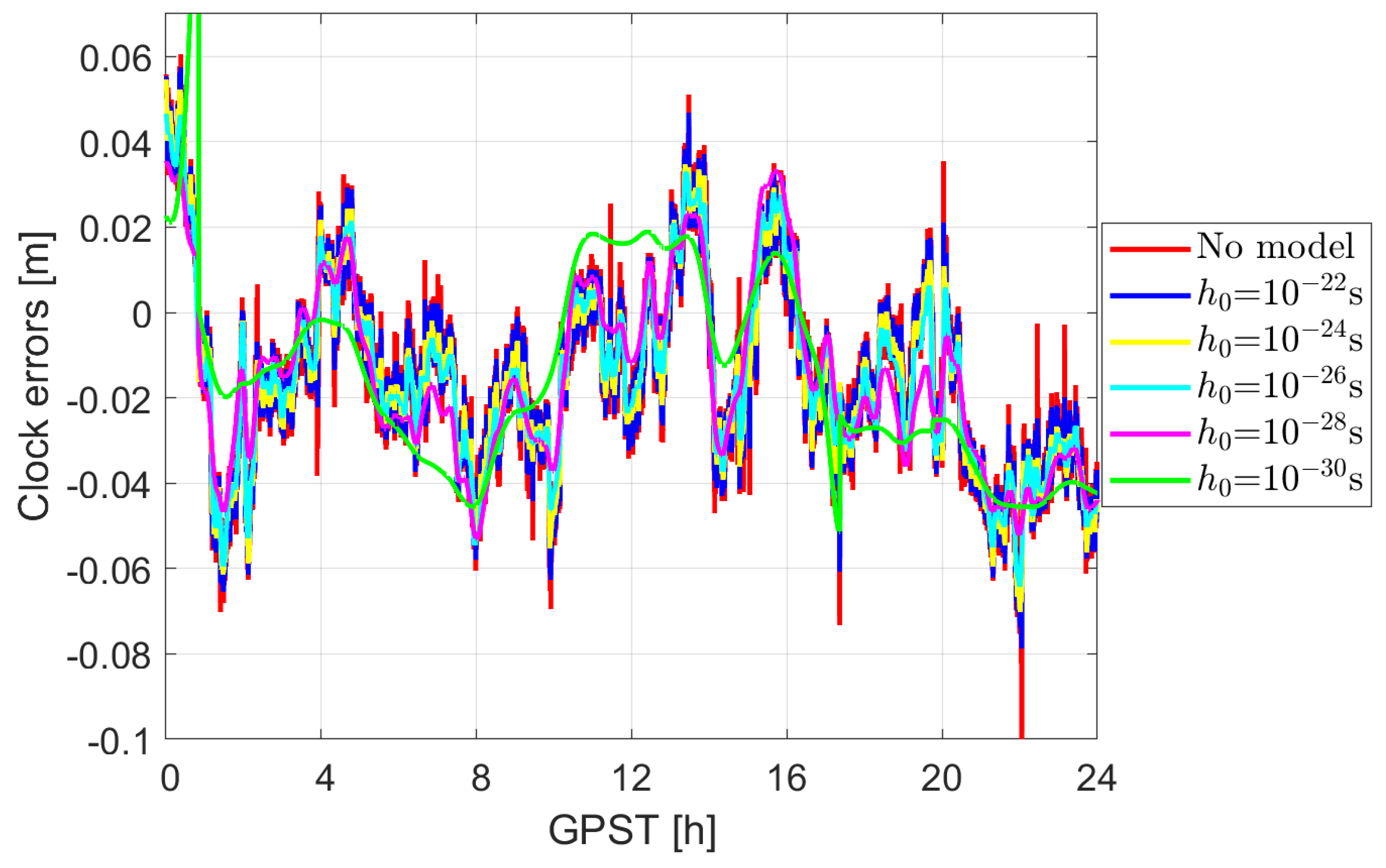
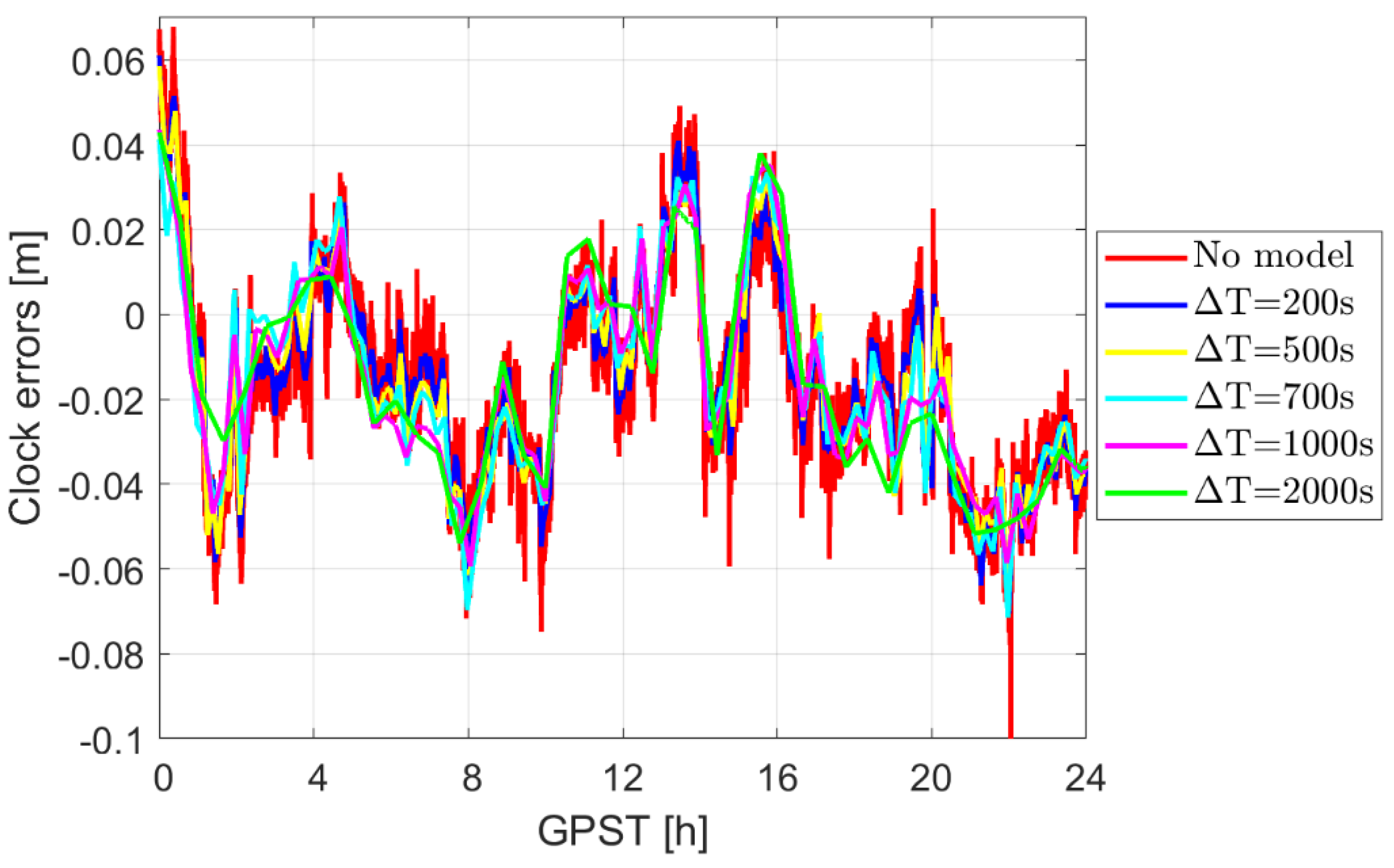
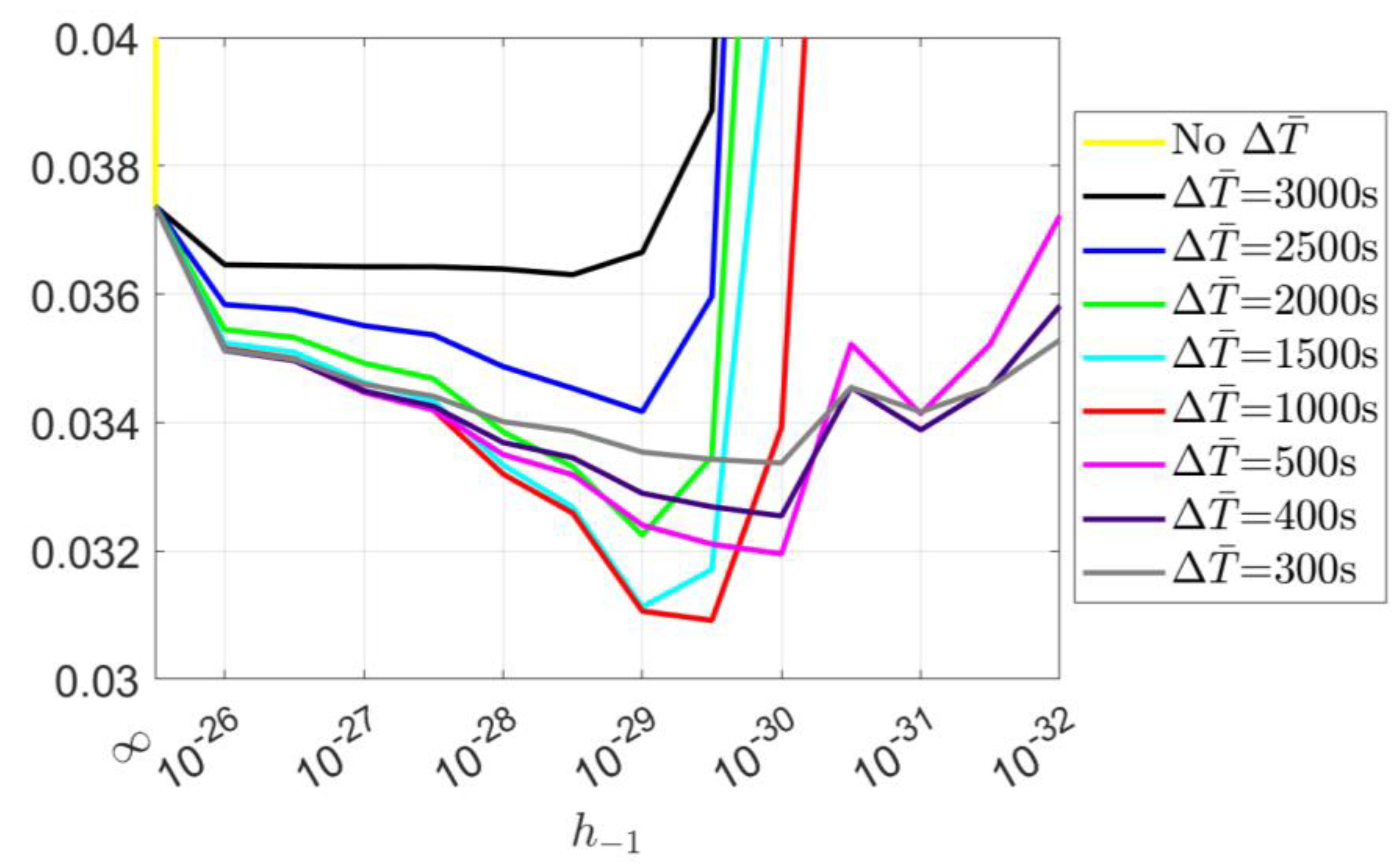
4.3. 2.5-State Model
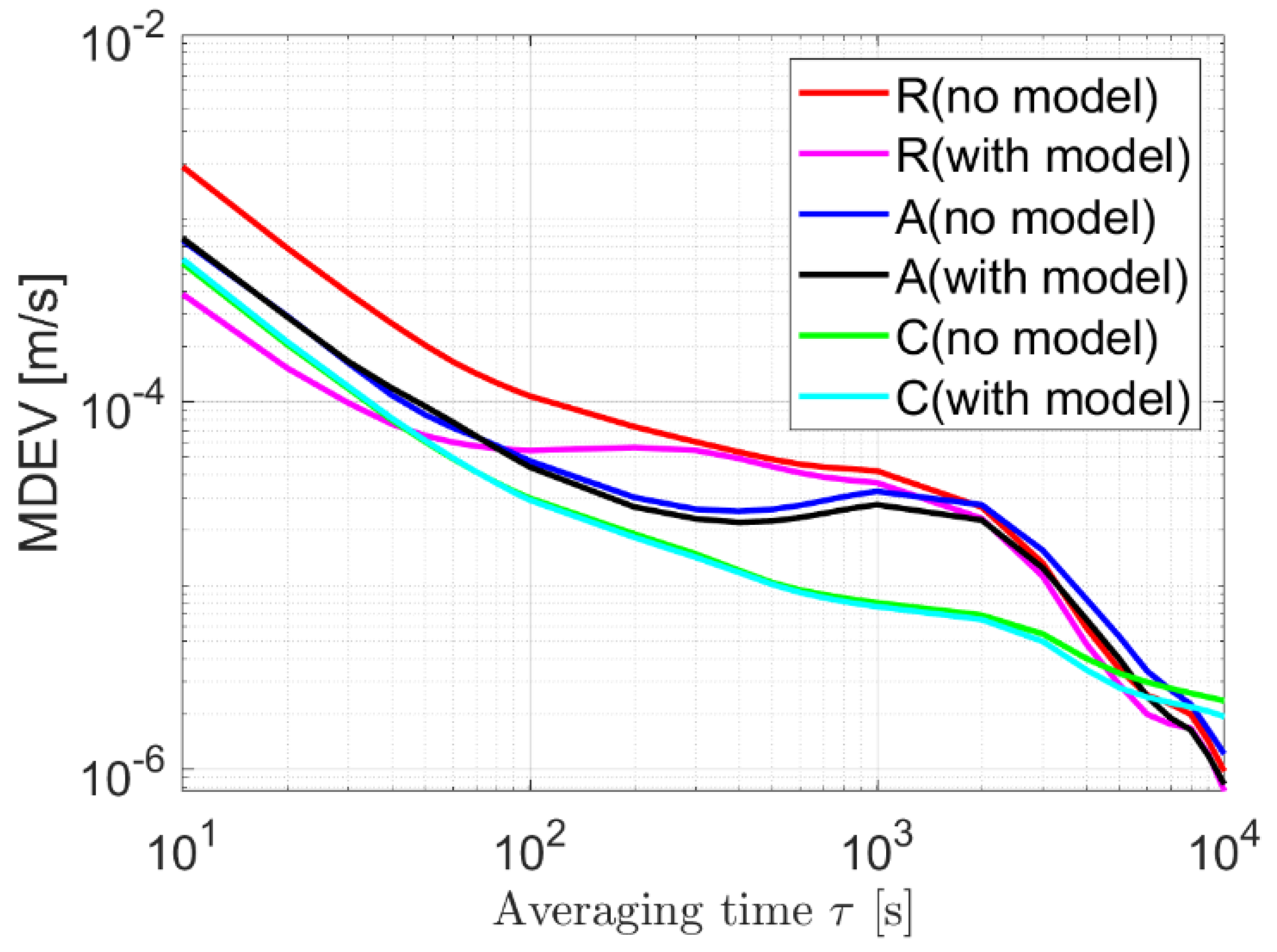
4.4. Summary for Improvement in the Radial Orbits
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Reid, T.G.; Neish, A.M.; Walter, T.; Enge, P.K. Broadband LEO constellations for navigation. Navig. J. Inst. Navig. 2018, 65, 205–220. [Google Scholar] [CrossRef]
- Michalak, G.; Glaser, S.; Neumayer, K.; König, R. Precise orbit and Earth parameter determination supported by LEO satellites, inter-satellite links and synchronized clocks of a future GNSS. Adv. Space Res. 2021, 68, 4753–4782. [Google Scholar] [CrossRef]
- Lawrence, D.; Cobb, H.S.; Gutt, G.; O’Connor, M.; Reid, T.G.; Walter, T. Navigation from LEO. 2017. Available online: https://www.gpsworld.com/innovation-navigation-from-leo/ (accessed on 14 June 2023).
- Li, K.; Zhou, X.; Wang, W.; Gao, Y.; Zhao, G.; Tao, E.; Xu, K. Centimeter-level orbit determination for TG02 spacelab using onboard GNSS data. Sensors 2018, 18, 2671. [Google Scholar] [CrossRef]
- Faragher, R.; Ziebart, M. OneWeb LEO PNT: Progress or Risky Gamble. Inside GNSS 2020, 28. Available online: https://insidegnss.com/oneweb-leo-pnt-progress-or-risky-gamble/ (accessed on 14 June 2023).
- Krawinkel, T.; Schön, S. Benefits of receiver clock modeling in code-based GNSS navigation. GPS Solut. 2016, 20, 687–701. [Google Scholar] [CrossRef]
- Yang, Y.; Yue, X.; Yuan, J.; Rizos, C. Enhancing the kinematic precise orbit determination of low earth orbiters using GPS receiver clock modelling. Adv. Space Res. 2014, 54, 1901–1912. [Google Scholar] [CrossRef]
- Montenbruck, O.; Gill, E.; Lutze, F. Satellite orbits: Models, methods, and applications. Appl. Mech. Rev. 2002, 55, B27–B28. Available online: https://link.springer.com/book/10.1007/978-3-642-58351-3 (accessed on 14 June 2023). [CrossRef]
- Guo, N.-N.; Zhou, X.-H.; Li, K.; Wu, B. Research on the impact factors of GRACE precise orbit determination by dynamic method. J. Appl. Geod. 2018, 12, 249–257. [Google Scholar] [CrossRef]
- Mao, X.; Arnold, D.; Girardin, V.; Villiger, A.; Jäggi, A. Dynamic GPS-based LEO orbit determination with 1 cm precision using the Bernese GNSS Software. Adv. Space Res. 2021, 67, 788–805. [Google Scholar] [CrossRef]
- Case, K.; Kruizinga, G.; Wu, S. GRACE Level 1B Data Product User Handbook; Jpl D-22027; 2010; Available online: https://earth.esa.int/eogateway/documents/20142/37627/GRACE-L1B-Handbook-v1.3.pdf (accessed on 14 June 2023).
- Kornfeld, R.P.; Arnold, B.W.; Gross, M.A.; Dahya, N.T.; Klipstein, W.M.; Gath, P.F.; Bettadpur, S. GRACE-FO: The gravity recovery and climate experiment follow-on mission. J. Spacecr. Rocket. 2019, 56, 931–951. [Google Scholar] [CrossRef]
- Fletcher, K. Sentinel-3: ESA’s Global Land and Ocean Mission for GMES Operational Services; ESA Communications, 2012; Available online: https://sentinel.esa.int/documents/247904/351187/S3_SP-1322_3.pdf (accessed on 14 June 2023).
- Zhou, X.; Jiang, W.; Chen, H.; Li, Z.; Liu, X. Improving the GRACE kinematic precise orbit determination through modified clock estimating. Sensors 2019, 19, 4347. [Google Scholar] [CrossRef] [PubMed]
- Weinbach, U.; Schön, S. Improved GRACE kinematic orbit determination using GPS receiver clock modeling. GPS Solut. 2013, 17, 511–520. [Google Scholar] [CrossRef]
- Allahvirdi-Zadeh, A.; Wang, K.; El-Mowafy, A. POD of small LEO satellites based on precise real-time MADOCA and SBAS-aided PPP corrections. GPS Solut. 2021, 25, 31. [Google Scholar] [CrossRef]
- Larson, K.M.; Ashby, N.; Hackman, C.; Bertiger, W. An assessment of relativistic effects for low Earth orbiters: The GRACE satellites. Metrologia 2007, 44, 484. Available online: https://iopscience.iop.org/article/10.1088/0026-1394/44/6/007 (accessed on 14 June 2023). [CrossRef]
- Wang, K.; El-Mowafy, A. LEO satellite clock analysis and prediction for positioning applications. Geo Spat. Inf. Sci. 2022, 25, 14–33. [Google Scholar] [CrossRef]
- Montenbruck, O.; Hackel, S.; Wermuth, M.; Zangerl, F. Sentinel-6A precise orbit determination using a combined GPS/Galileo receiver. J. Geod. 2021, 95, 109. [Google Scholar] [CrossRef]
- Ge, H.; Wu, T.; Li, B. Characteristics analysis and prediction of Low Earth Orbit (LEO) satellite clock corrections by using least-squares harmonic estimation. GPS Solut. 2022, 27, 38. [Google Scholar] [CrossRef]
- Wen, H.Y.; Kruizinga, G.; Paik, M.; Landerer, F.; Bertiger, W.; Sakumura, C.; Bandikova, T.; Mccullough, C. Gravity Recovery and Climate Experiment Follow-on (GRACE-FO) Level-1 Data Product User Handbook. JPL D-56935 (URS270772); 2019. Available online: https://podaac-tools.jpl.nasa.gov/drive/files/allData/gracefo/docs/GRACE-FO_L1_Handbook.pdf (accessed on 14 June 2023).
- Dach, R.; Schaer, S.; Meindl, M.; Bock, H.; Jäggi, A.; Lutz, S.; Meyer, U.; Ostini, L.; Prange, L.; Steinbach, A. Global Multi-GNSS Processing at CODE. J. Geod. 2009, 83, 353–365. [Google Scholar] [CrossRef]
- Ye, Z.; Li, H.; Wang, S. Characteristics analysis of the GNSS satellite clock. Adv. Space Res. 2021, 68, 3314–3326. [Google Scholar] [CrossRef]
- Kudrys, J.; Prochniewicz, D.; Zhang, F.; Jakubiak, M.; Maciuk, K. Identification of BDS Satellite Clock Periodic Signals Based on Lomb-Scargle Power Spectrum and Continuous Wavelet Transform. Energies 2021, 14, 7155. [Google Scholar] [CrossRef]
- GMV. Sentinels POD Product Handbook. Copernicus Sentinel-1, -2 and -3 Precise Orbit Determination Service (SENTINELSPOD). 2020. Available online: https://sentinel.esa.int/documents/247904/3372484/Sentinels-POD-Product-Handbook.pdf (accessed on 14 June 2023).
- Li, X.; Zhang, K.; Meng, X.; Zhang, Q.; Zhang, W.; Li, X.; Yuan, Y. LEO–BDS–GPS integrated precise orbit modeling using FengYun-3D, FengYun-3C onboard and ground observations. GPS Solut. 2020, 24, 48. [Google Scholar] [CrossRef]
- Wang, L.; Xu, B.; Fu, W.; Chen, R.; Li, T.; Han, Y.; Zhou, H. Centimeter-level precise orbit determination for the Luojia-1A satellite using BeiDou observations. Remote Sens. 2020, 12, 2063. [Google Scholar] [CrossRef]
- Yang, H.; Yang, X.; Zhang, Z.; Sun, B.; Qin, W. Evaluation of the Effect of High-order Ionopsheric Delay on GPS Precise Point Positioning Time Transfer. Remote Sens. 2020, 12, 2129. [Google Scholar] [CrossRef]
- Wang, K.; El-Mowafy, A.; Rizos, C. Integrity monitoring for precise orbit determination of LEO satellites. GPS Solut. 2021, 26, 32. [Google Scholar] [CrossRef]
- Dach, R.; Lutz, S.; Walser, P.; Fridez, P. Bernese GNSS Software Version 5.2; University of Bern, Bern Open Publishing: Bern, Switzerland, 2015. [Google Scholar] [CrossRef]
- Li, X.; Ma, F.; Li, X.; Lv, H.; Bian, L.; Jiang, Z.; Zhang, X. LEO constellation-augmented multi-GNSS for rapid PPP convergence. J. Geod. 2019, 93, 749–764. [Google Scholar] [CrossRef]
- Pavlis, N.; Holmes, S.; Kenyon, S.; Factor, J. An Earth Gravitational Model to Degree 2160: EGM2008. In Proceedings of the European Geosciences Union General Assembly, Vienna, Austria, 13–18 April 2008; Available online: http://earth-info.nga.mil/GandG/wgs84/gravitymod/egm2008/index.html (accessed on 14 June 2023).
- Standish, E. JPL Planetary and Lunar Ephemerides, DE405/LE405 (Memo IOM 312. F-98-048; Pasadena: JPL). 1998. Available online: http://ssd.jpl.nasa.gov/iau-comm4/de405iom/de405iom.pdf (accessed on 14 June 2023).
- Petit, G.; Luzum, B. IERS conventions. IERS Tech. Note 2010, 36, 2010. [Google Scholar]
- Lyard, F.; Lefevre, F.; Letellier, T.; Francis, O. Modelling the global ocean tides: Modern insights from FES2004. Ocean. Dyn. 2006, 56, 394–415. [Google Scholar] [CrossRef]
- Wang, K.; Rothacher, M. Stochastic modeling of high-stability ground clocks in GPS analysis. J. Geod. 2013, 87, 427–437. [Google Scholar] [CrossRef]
- Wang, K. Advanced Modeling and Algorithms for High-Precision GNSS Analysis; ETH: Zurich, Switzerland, 2016. [Google Scholar] [CrossRef]
- Van Dierendonck, A.J.; McGraw, J. Relationship between Allan variances and Kalman filter parameters. In Proceedings of the 16th Annual Precise Time and Time Interval Systems and Applications Meeting, Greenbelt, MD, USA, 27–29 November 1984; pp. 273–293. [Google Scholar]
- Humpherys, J.; West, J. Kalman filtering with Newton’s method [lecture notes]. IEEE Control Syst. Mag. 2010, 30, 101–106. [Google Scholar] [CrossRef]
- Odijk, D.; Zhang, B.; Khodabandeh, A.; Odolinski, R.; Teunissen, P.J. On the estimability of parameters in undifferenced, uncombined GNSS network and PPP-RTK user models by means of S-system theory. J. Geod. 2016, 90, 15–44. [Google Scholar] [CrossRef]
- Allan, D.W. Time and Frequency (Time-Domain) Characterization, Estimation, and Prediction of Precision Clocks and Oscillators. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 1987, 34, 647–654. [Google Scholar] [CrossRef] [PubMed]
- Kazmierski, K.; Sośnica, K.; Hadas, T. Quality assessment of multi-GNSS orbits and clocks for real-time precise point positioning. GPS Solut. 2018, 22, 11. [Google Scholar] [CrossRef]
- Riley, W.J.; Howe, D.A. Handbook of Frequency Stability Analysis; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2008.
- Wu, M.; Sun, B.; Wang, Y.; Zhang, Z.; Su, H.; Yang, X. Sub-nanosecond one-way real-time time service system based on UTC. GPS Solut. 2021, 25, 44. [Google Scholar] [CrossRef]






| Perturbation | Model |
|---|---|
| Gravitational attraction of the Earth (Earth’s non-sphericity and non-homogeneous mass distribution) | EGM2008 [32] |
| Gravitational attractions of other planets | JPL DE405 [33] |
| Solid Earth tides and pole tides | IERS Conventions 2010 [34] |
| Ocean tides | FES2004 [35] |
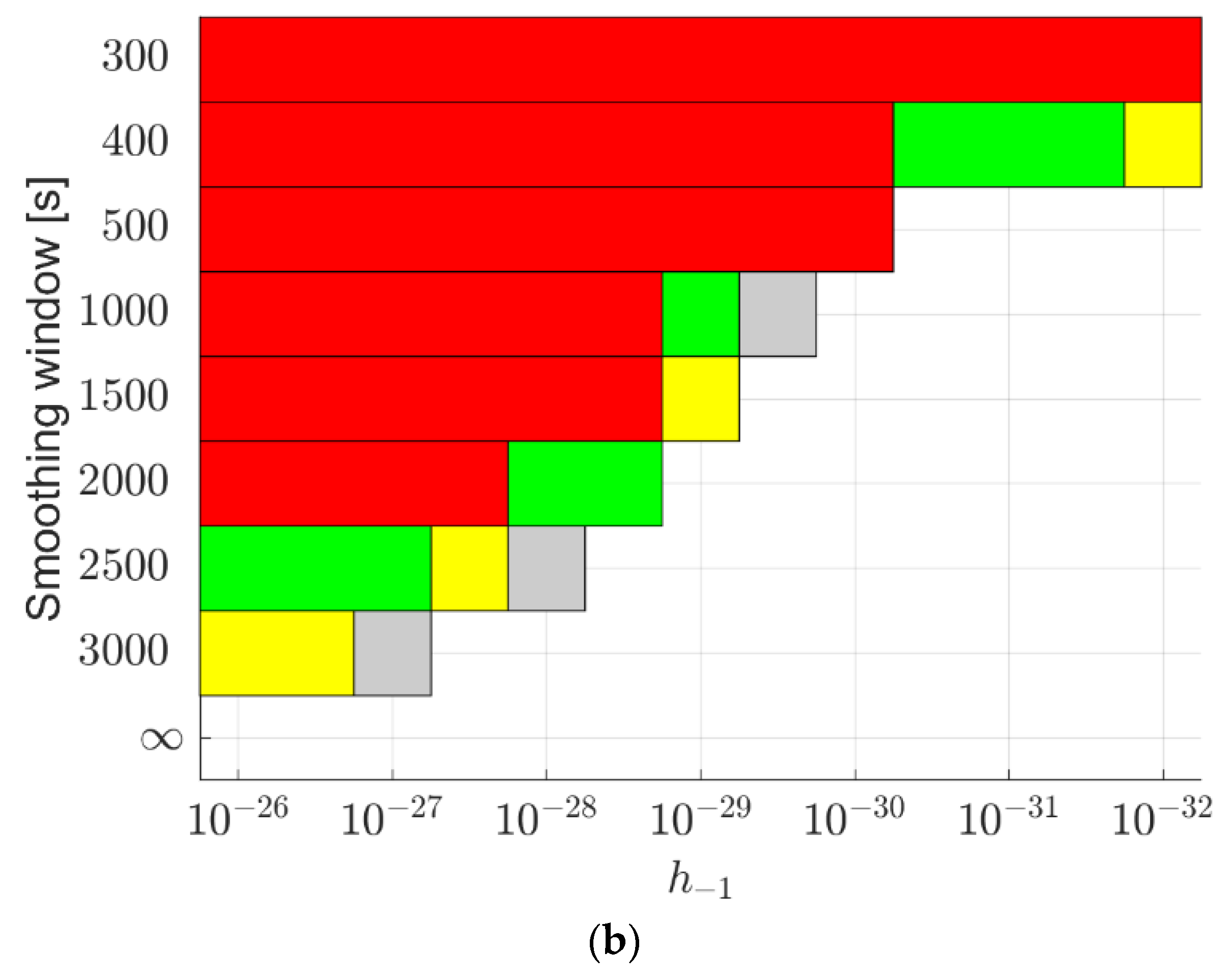
| Noise Type | Slope in ADEV | ||||
|---|---|---|---|---|---|
| WFN | −0.5 | 0 | |||
| FFN | 0 | ||||
| RWFN | 0.5 |
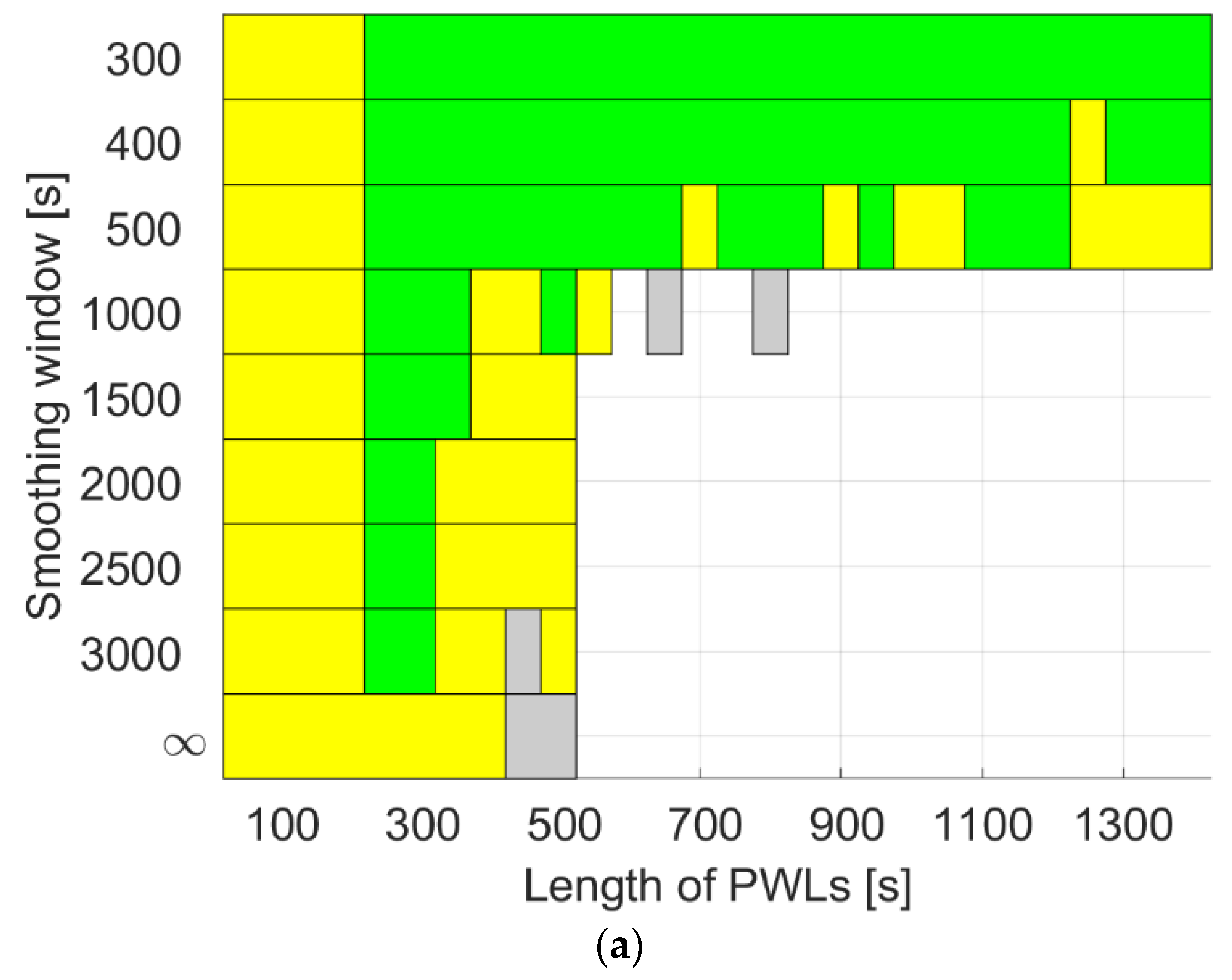
| Smoothing Window [s] | Orbital Improvement [%] at PWL Length of | ||||||
|---|---|---|---|---|---|---|---|
| 200 s | 400 s | 600 s | 800 s | 1000 s | 1200 s | 1400 s | |
| 300 | 8/4/3 | 10/6/7 | 11/7/9 | 12/8/7 | 12/7/6 | 11/7/7 | 9/6/5 |
| 500 | 8/4/3 | 12/9/11 | 14/11/16 | 16/13/13 | 16/13/8 | 13/12/10 | 8/10/8 |
| 1000 | 8/4/3 | 12/11/17 | 11/16/21 | 9/15/17 | 1/12/−3 | −20/7/−9 | −42/1/−3 |
| 2000 | 8/4/3 | 11/11/14 | −1/11/14 | −15/4/4 | −73/−28/−62 | −161/−58/−88 | −263/−112/−69 |
| 3000 | 8/4/3 | 9/10/14 | −4/9/9 | −27/3/6 | −117/−53/−74 | −264/−117/−142 | −467/−237/−112 |
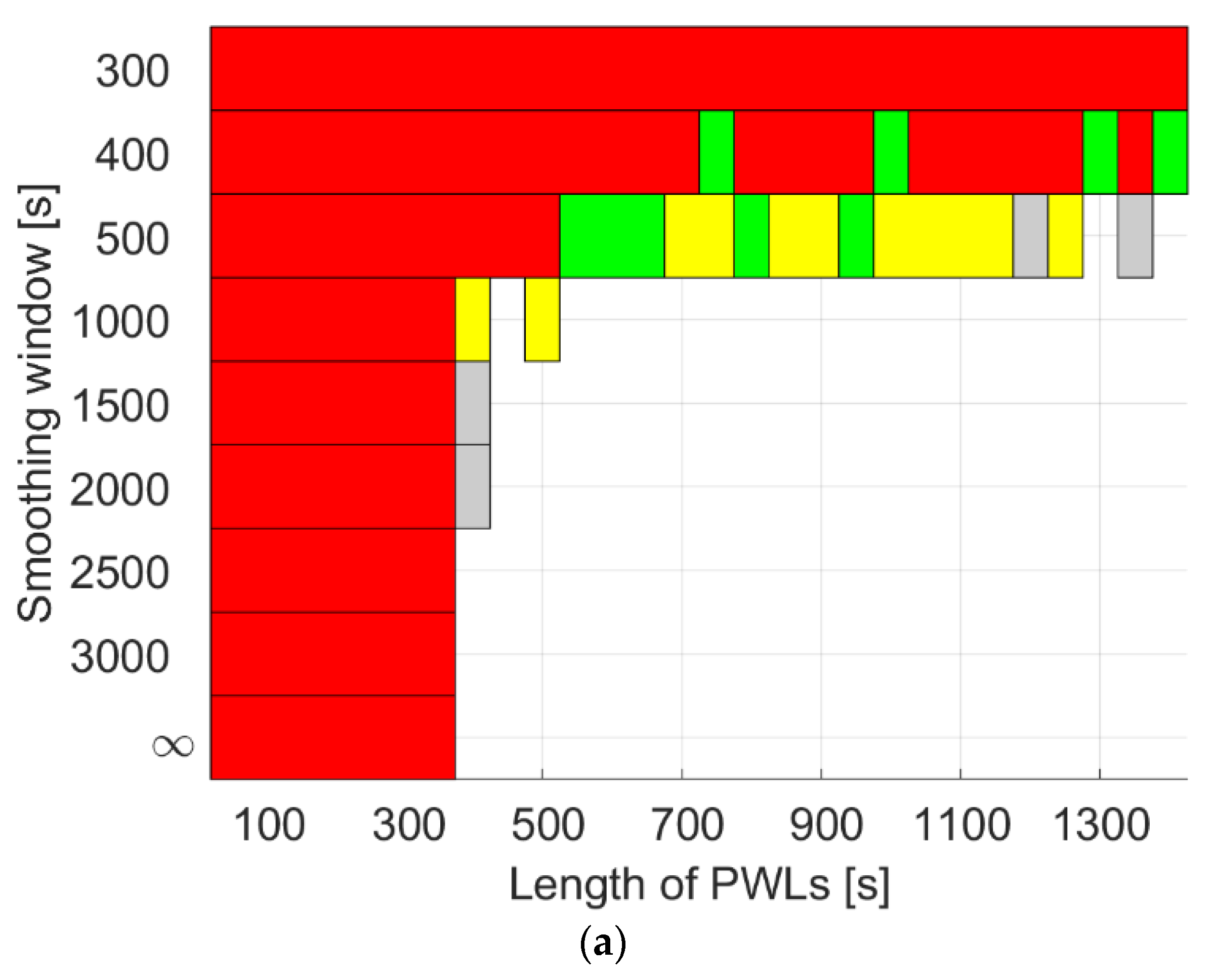
| Smoothing Window [s] | |||||||
|---|---|---|---|---|---|---|---|
| 300 | 6/2/2 | 7/3/2 | 9/5/4 | 10/6/6 | 11/7/6 | 9/5/6 | 6/1/6 |
| 500 | 6/2/2 | 8/4/3 | 10/6/5 | 13/10/9 | 15/12/11 | 9/8/10 | 0/0/10 |
| 1000 | 6/2/2 | 8/4/3 | 11/7/6 | 17/15/14 | 11/19/12 | −25/5/5 | −62/−32/−13 |
| 2000 | 5/2/2 | 7/4/3 | 9/7/6 | 14/15/11 | −36/8/−10 | −200/−94/−67 | −336/−265/−245 |
| 3000 | 5/2/2 | 3/3/2 | 3/5/5 | 2/13/10 | −76/−14/−16 | −377/−238/−166 | −648/−616/−622 |
| Smoothing Window [s] | RMS [cm] at PWL Length of | ||||||
|---|---|---|---|---|---|---|---|
| No Model | 300 s | 400 s | 600 s | 800 s | 1000 s | 1200 s | |
| 300 | 3.7 | 3.4 | 3.4 | 3.3 | 3.3 | 3.3 | 3.3 |
| 400 | 3.7 | 3.4 | 3.3 | 3.3 | 3.2 | 3.2 | 3.3 |
| 500 | 3.7 | 3.4 | 3.3 | 3.2 | 3.1 | 3.1 | 3.3 |
| Smoothing Window [s] | ||||||
|---|---|---|---|---|---|---|
| No Model | ||||||
| 300 | 3.7 | 3.4 | 3.4 | 3.4 | 3.3 | 3.3 |
| 400 | 3.7 | 3.4 | 3.3 | 3.3 | 3.3 | 3.3 |
| 500 | 3.7 | 3.4 | 3.3 | 3.2 | 3.2 | 3.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, K.; El-Mowafy, A.; Yang, X. LEO Satellite Clock Modeling and Its Benefits for LEO Kinematic POD. Remote Sens. 2023, 15, 3149. https://doi.org/10.3390/rs15123149
Wang K, El-Mowafy A, Yang X. LEO Satellite Clock Modeling and Its Benefits for LEO Kinematic POD. Remote Sensing. 2023; 15(12):3149. https://doi.org/10.3390/rs15123149
Chicago/Turabian StyleWang, Kan, Ahmed El-Mowafy, and Xuhai Yang. 2023. "LEO Satellite Clock Modeling and Its Benefits for LEO Kinematic POD" Remote Sensing 15, no. 12: 3149. https://doi.org/10.3390/rs15123149
APA StyleWang, K., El-Mowafy, A., & Yang, X. (2023). LEO Satellite Clock Modeling and Its Benefits for LEO Kinematic POD. Remote Sensing, 15(12), 3149. https://doi.org/10.3390/rs15123149







