Using Various Analysis Center Products to Assess the Time-Frequency Transfer Performance of GPS/Galileo/BDS PPPAR Methods
Abstract
1. Introduction
2. Materials and Methods
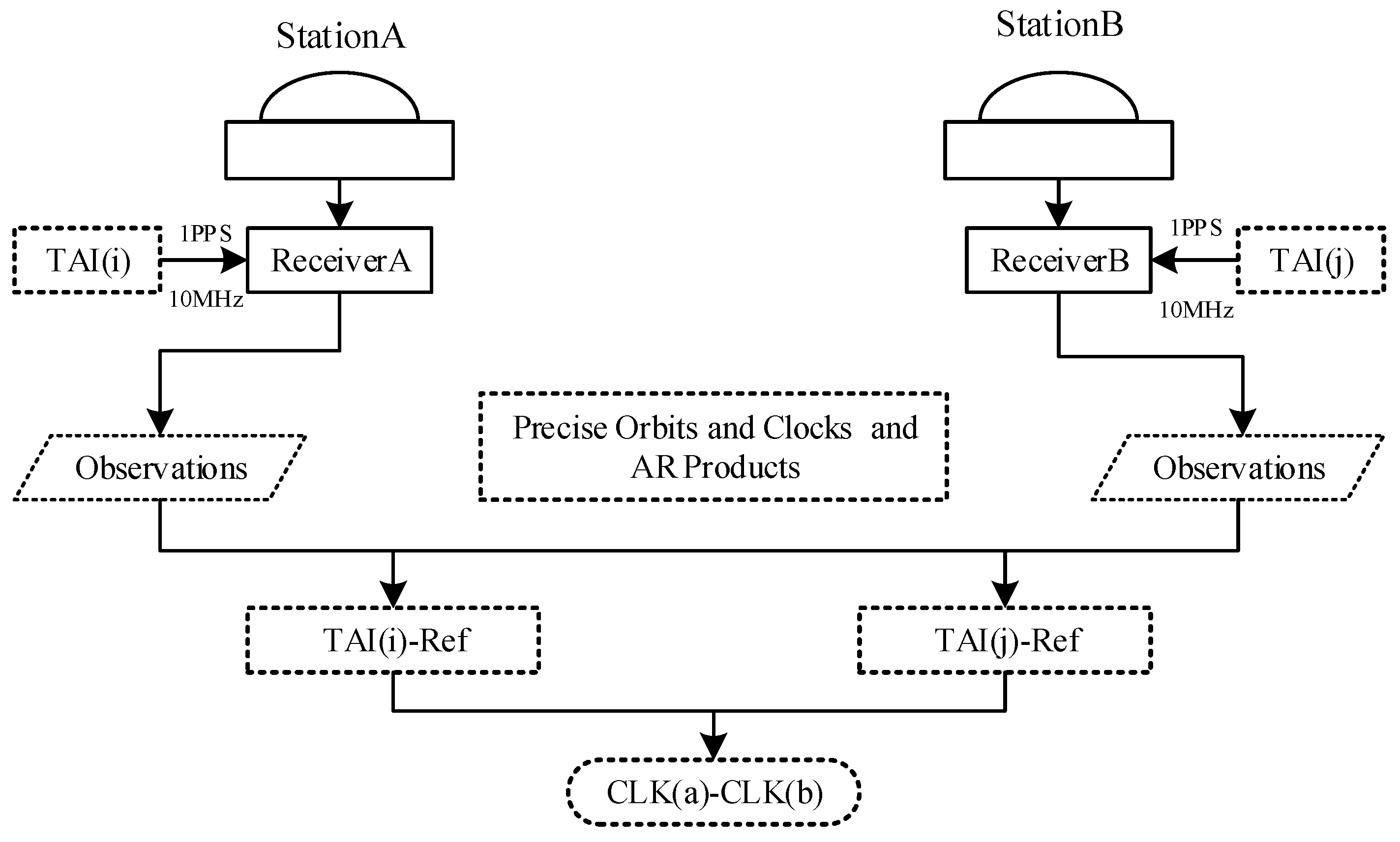
2.1. PPPAR Time Transfer
2.2. GNSS-PPPAR Model
3. Experimental Data and Processing Strategies
4. PPP Detailed Processing Strategy
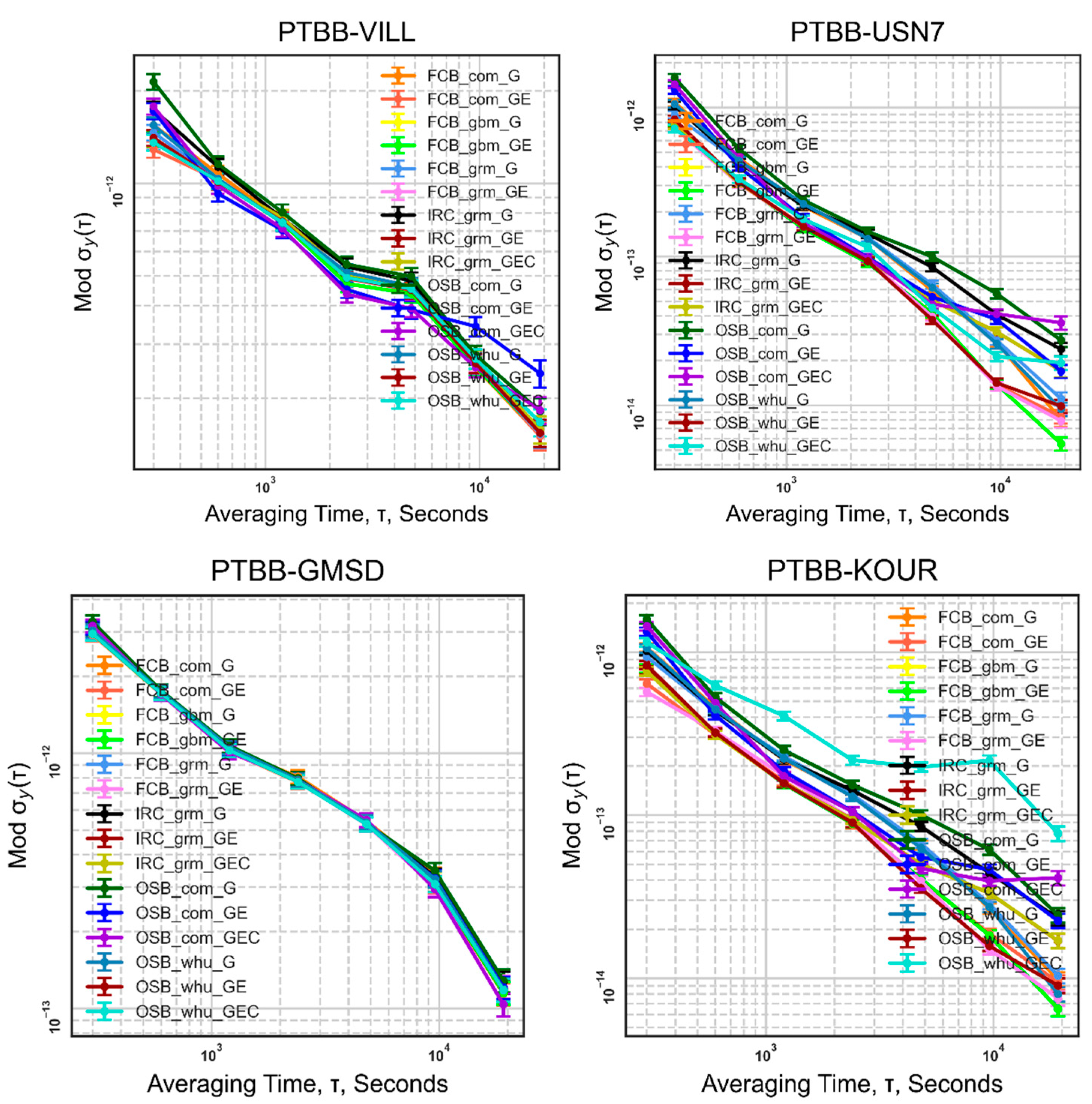
5. Validation and Analysis
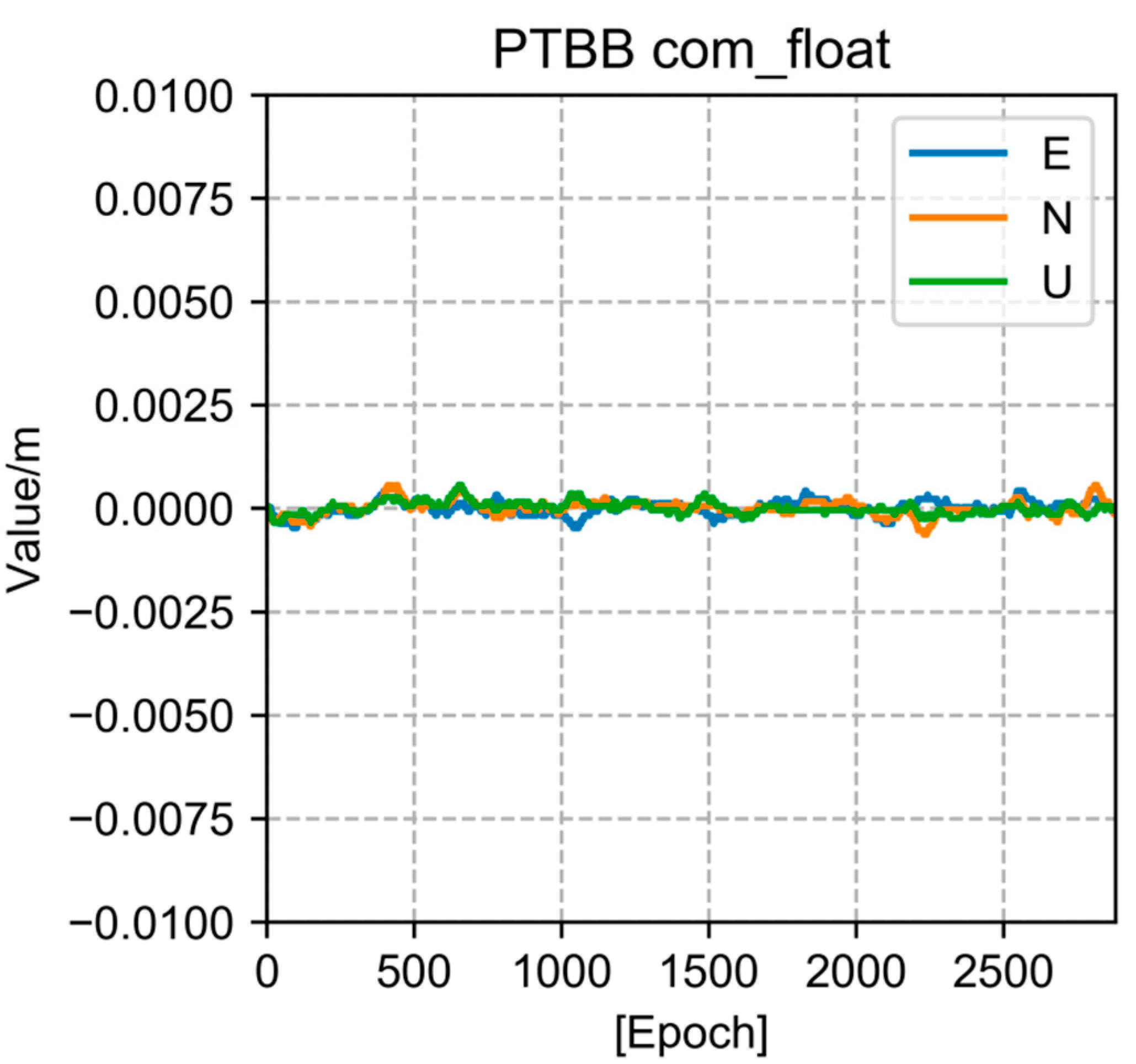
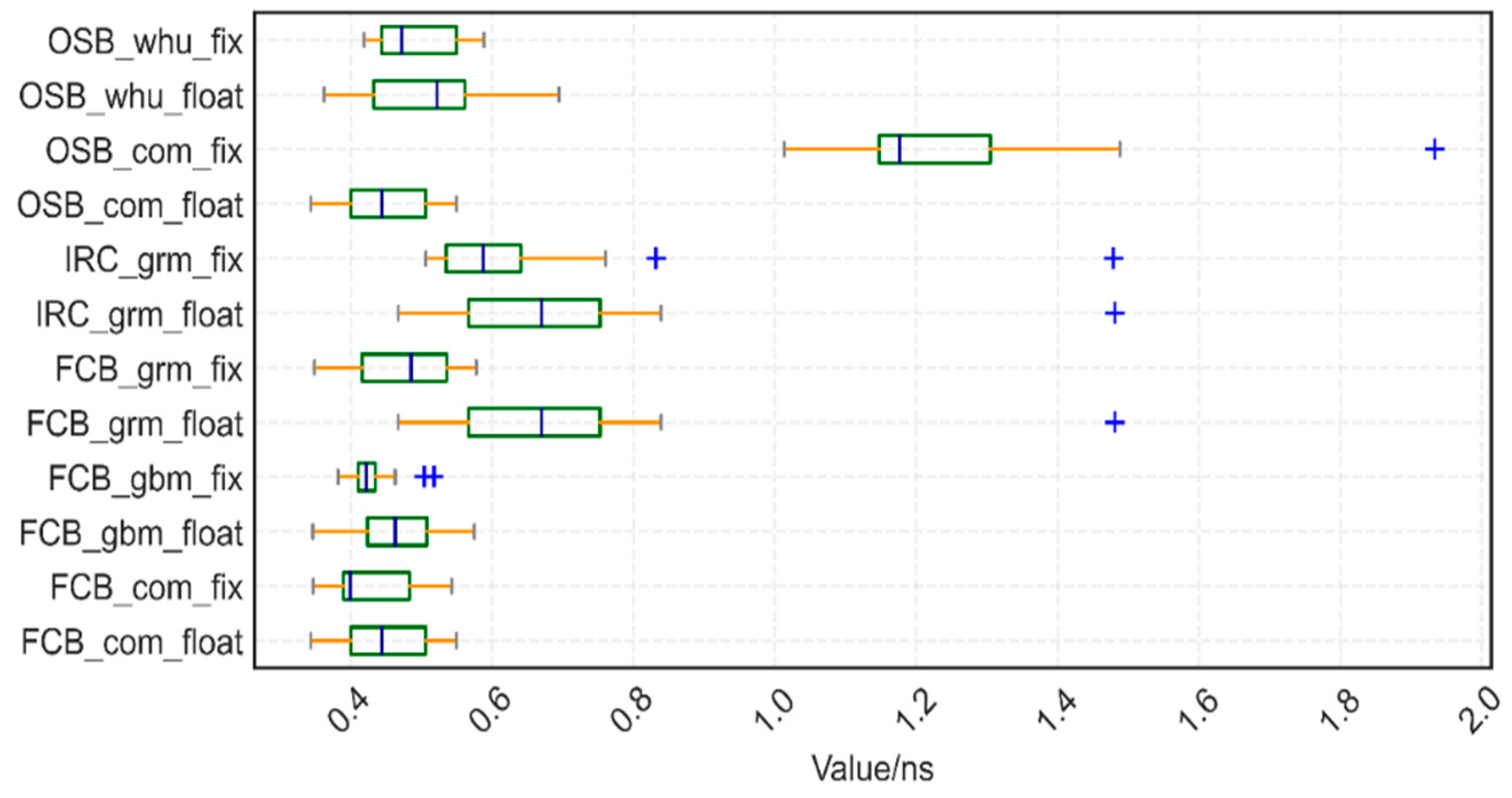
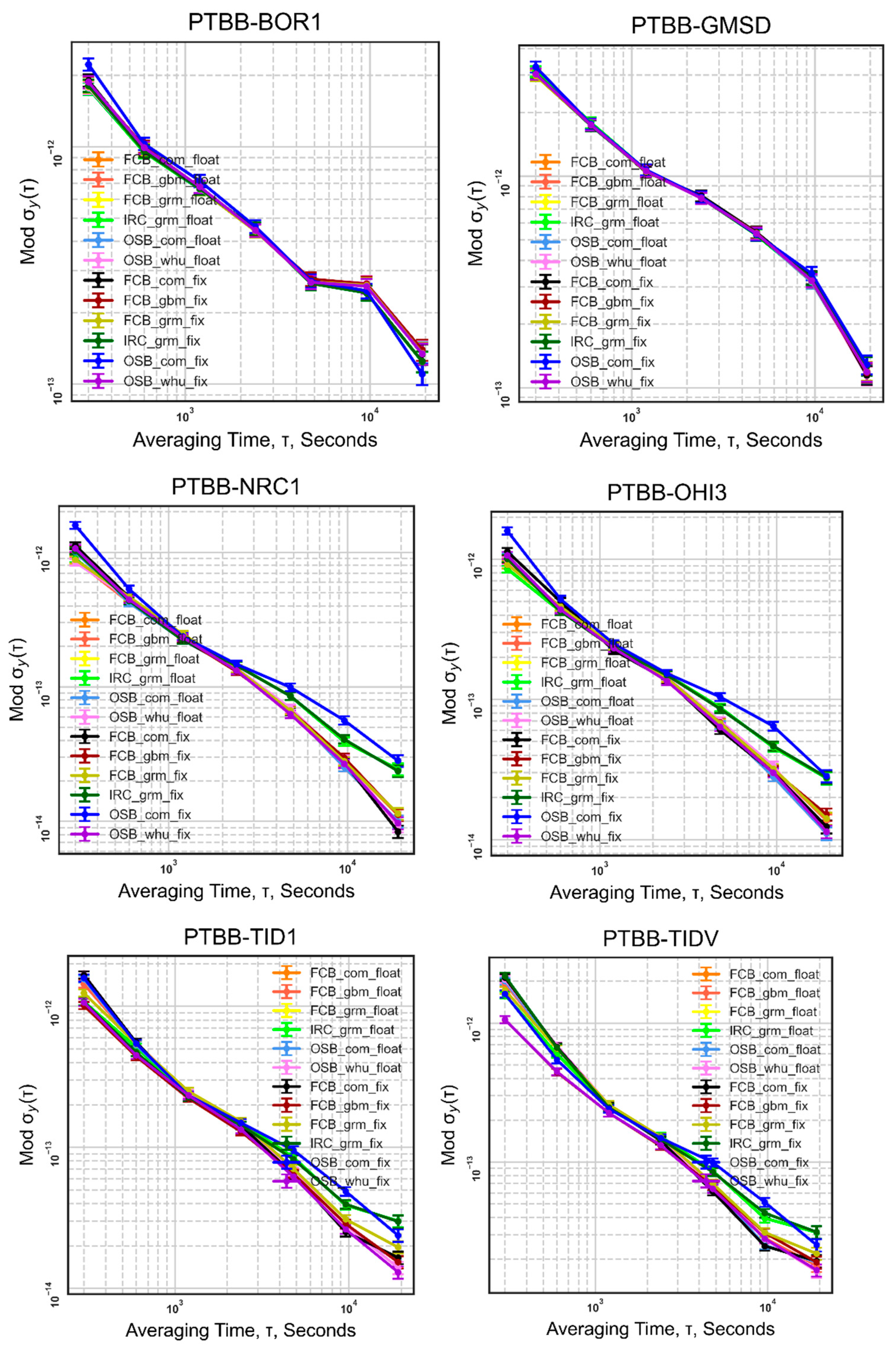
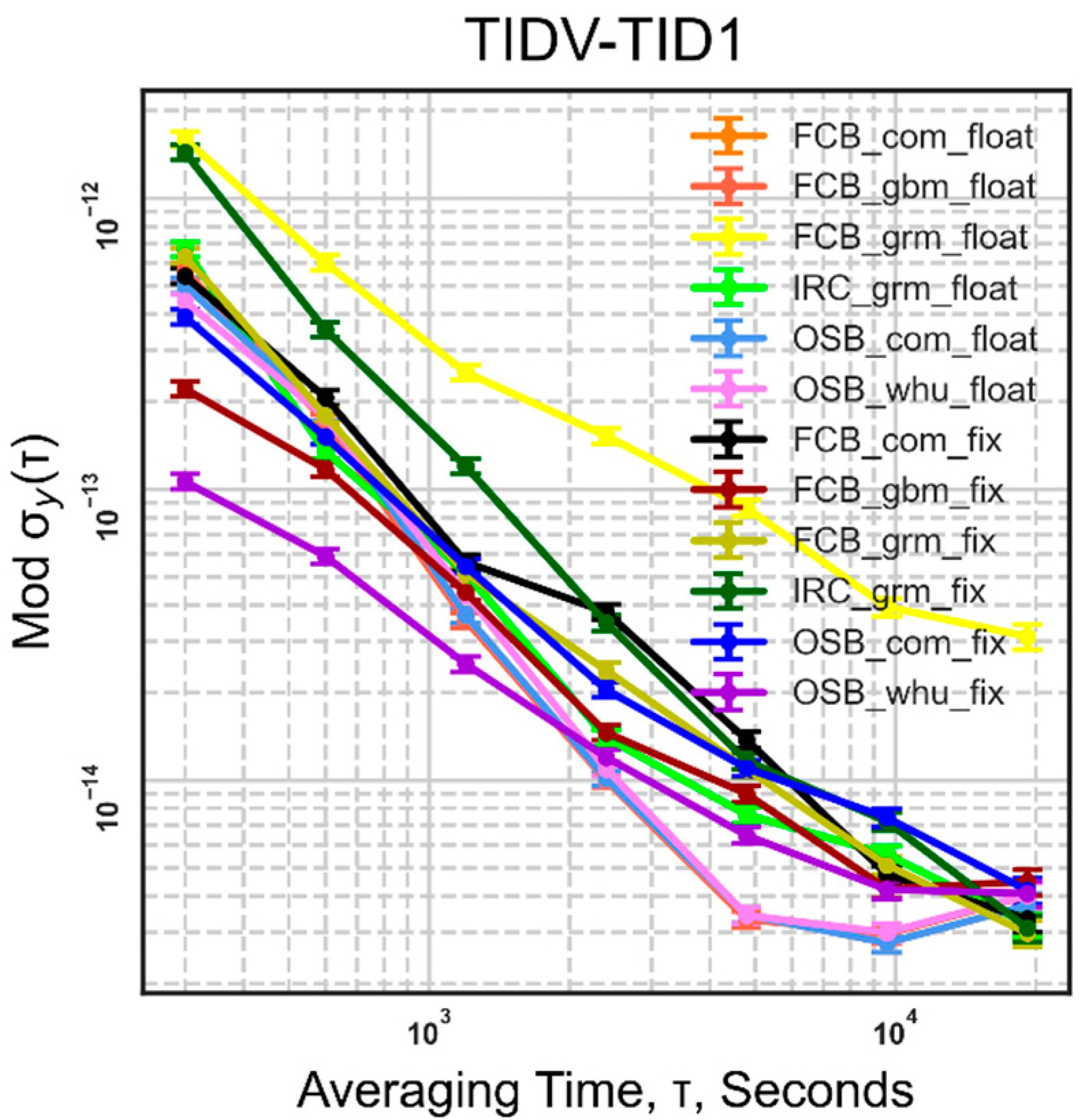
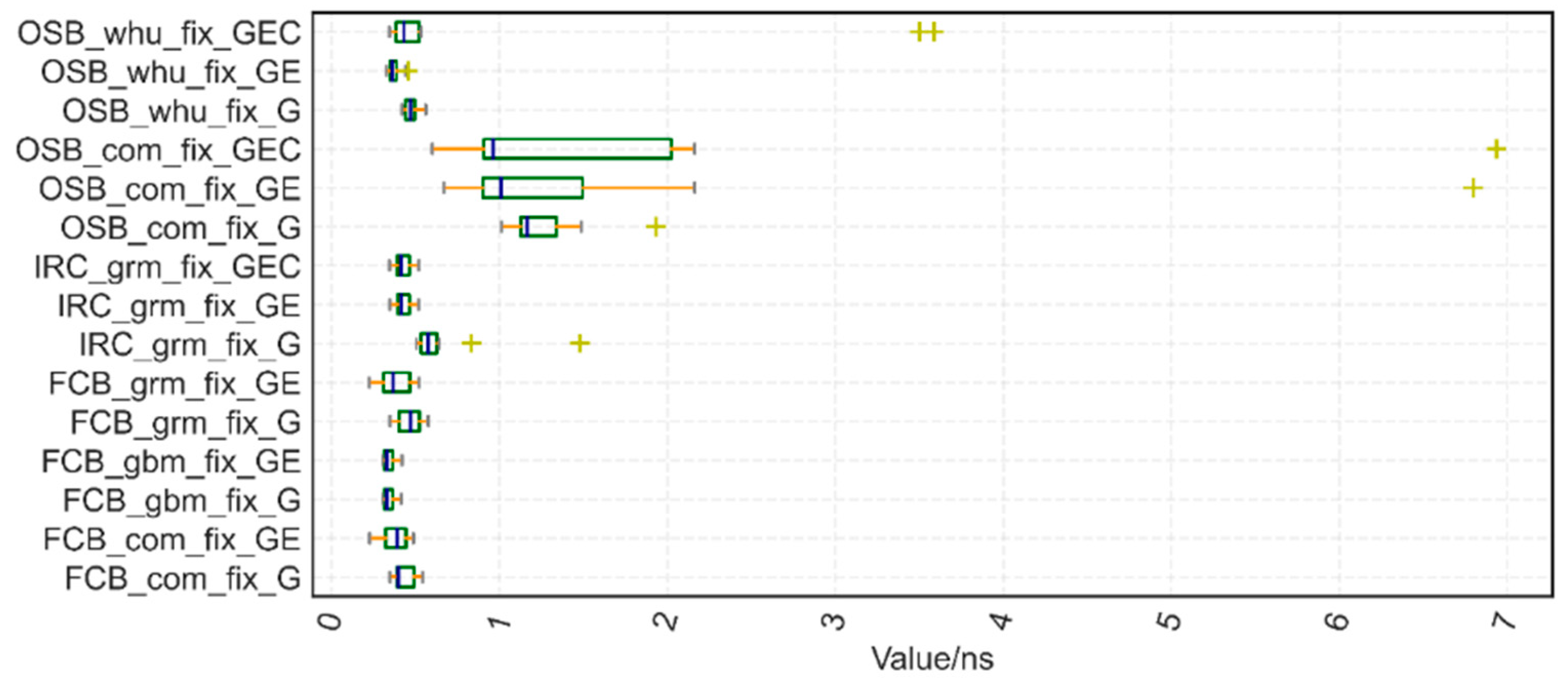
5.1. Test Case
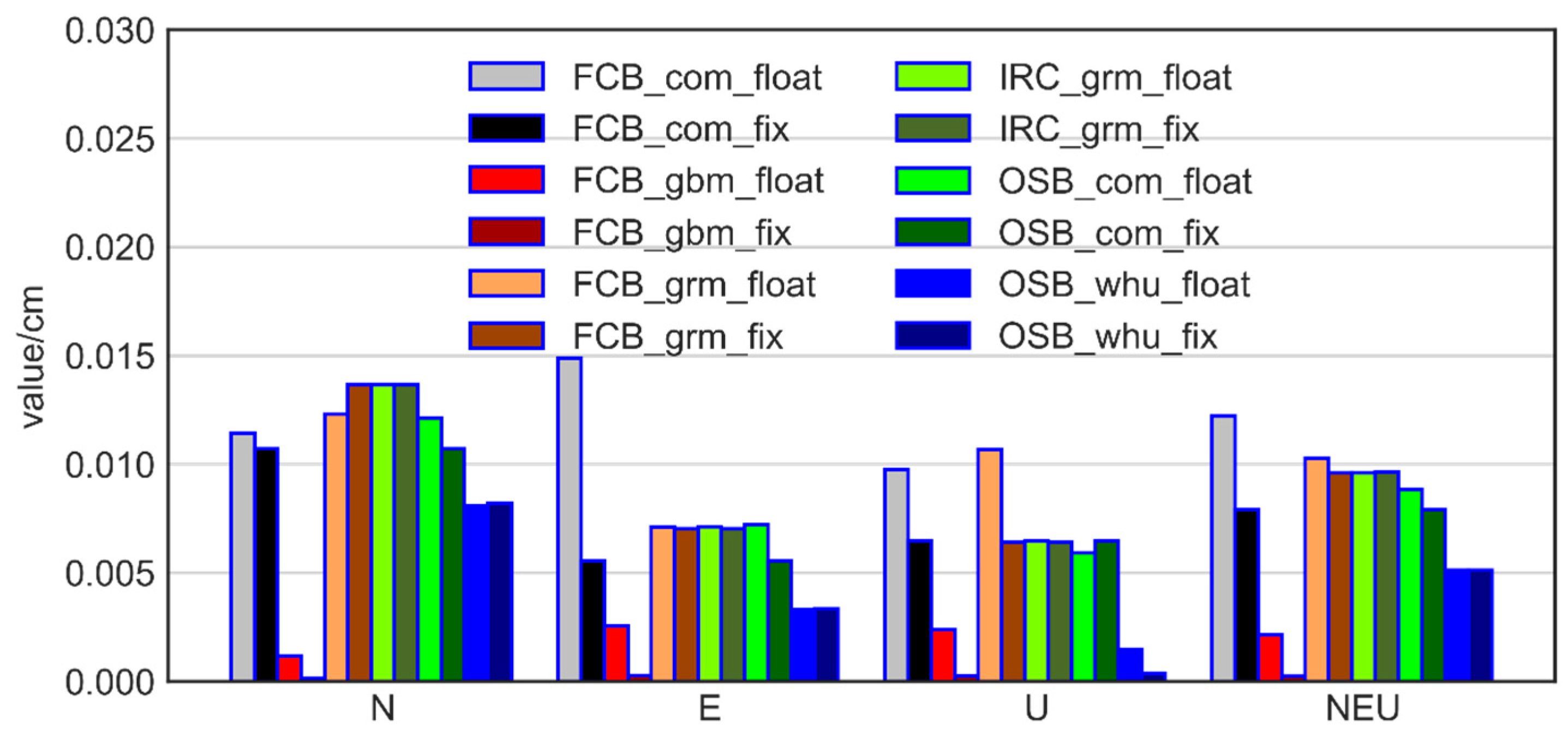
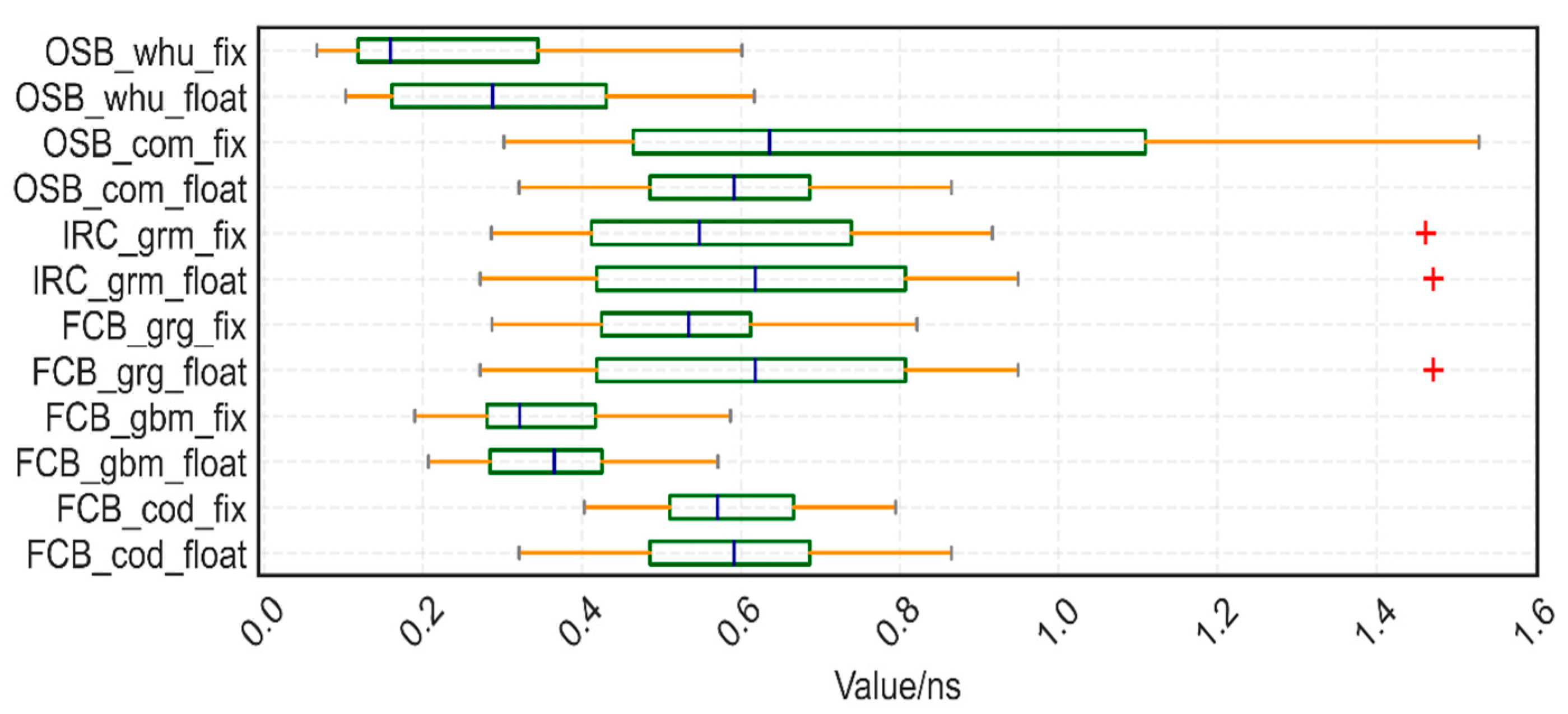
5.2. Muti-GNSS
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, H.; Yan, M.; Guang, W. Comparison of two-way and beidou common view time transfer between ntsc and beij station. Adv. Mater. Res. 2014, 1049–1050, 1997–2000. [Google Scholar] [CrossRef]
- Nawrocki, J.; Lewandowski, W.; Nogas, P.; Foks, A.; Lemanski, D. An experiment of GPS + GLONASS common-view time transfer using new multi-system receivers. In Proceedings of the Frequency and Time Forum (EFTF), 2006 20th European IEEE, Braunschweig, Germany, 27–30 March 2006. [Google Scholar]
- Allan, D.W.; Weiss, M.A. Accurate Time and Frequency Transfer During Common-View of a GPS Satellite. In Proceedings of the IEEE Symposium on Frequency Control, Vancouver, Canada, 29–31 August 2005. [Google Scholar]
- Harmegnies, A.; Defraigne, P.; Petit, G. Combining gps and glonass in all-in-view for time transfer. Metrologia 2013, 50, 277–287. [Google Scholar] [CrossRef]
- Petit, G.; Jiang, Z. Gps all in view time transfer for tai computation. Metrologia 2007, 45, 35. [Google Scholar] [CrossRef]
- Petit, G. The TAIPPP pilot experiment. In Proceedings of the IEEE International Frequency Control Symposium, 2009 Joint with the 22nd European Frequency and Time Forum, Besancon, France, 20–24 April 2009. [Google Scholar]
- Zhang, V.S.; Parker, T.E.; Jian, Y. Long-term uncertainty in time transfer using GPS and TWSTFT techniques. In Proceedings of the Frequency Control Symposium the European Frequency Time Forum, Denver, CO, USA, 12–16 April 2015. [Google Scholar]
- Ray, J.; Senior, K. Geodetic techniques for time and frequency comparisons using gps phase and code measurements. Metrologia 2005, 42, 215. [Google Scholar] [CrossRef]
- Petit, G.; Kanj, A.; Loyer, S.; Delporte, J.; Mercier, F.; Perosanz, F. 1 × 10−16 frequency transfer by gps ppp with integer ambiguity resolution. Metrologia 2015, 52, 301. [Google Scholar] [CrossRef]
- Zumberge, J.F.; Heflin, M.B.; Jefferson, D.C.; Watkins, M.M.; Webb, F.H. Precise point positioning for the efficient and robust analysis of GPS data from large networks. J. Geophys. Res. Solid Earth 1997, 102, 5005–5017. [Google Scholar] [CrossRef]
- Su, K.; Jin, S.; Ge, Y. Rapid displacement determination with a stand-alone multi-GNSS receiver: GPS, Beidou, GLONASS, and Galileo. GPS Solut. 2019, 23, 54. [Google Scholar] [CrossRef]
- Lu, C.; Li, X.; Nilsson, T.; Ning, T.; Heinkelmann, R.; Ge, M.; Glaser, S.; Schuh, H. Real-time retrieval of precipitable water vapor from gps and beidou observations. J. Geod. 2015, 89, 843–856. [Google Scholar] [CrossRef]
- Gao, Z.; Zhang, H.; Ge, M.; Niu, X.; Shen, W.; Wickert, J.; Schuh, H. Tightly coupled integration of multi-gnss ppp and mems inertial measurement unit data. Gps Solut. 2017, 21, 377–391. [Google Scholar] [CrossRef]
- Li, X.; Ge, M.; Guo, B.; Wickert, J.; Schuh, H. Temporal point positioning approach for real-time gnss seismology using a single receiver. Geophys. Res. Lett. 2013, 40, 5677–5682. [Google Scholar] [CrossRef]
- Laurichesse, D.; Mercier, F.; Berthias, J.P.; Broca, P.; Cerri, L. Integer ambiguity resolution on undifferenced gps phase measurements and its application to ppp and satellite precise orbit determination. Navigation 2009, 56, 135–149. [Google Scholar] [CrossRef]
- Ge, M.; Gendt, G.; Rothacher, M.; Shi, C.; Liu, J. Resolution of gps carrier-phase ambiguities in precise point positioning (ppp) with daily observations. J. Geod. 2008, 82, 389–399. [Google Scholar] [CrossRef]
- Collins, P.; Bisnath, S.; Lahaye, F.; Héroux, P. Undifferenced gps ambiguity resolution using the decoupled clock model and ambiguity datum fixing. Navigation 2010, 57, 123–135. [Google Scholar] [CrossRef]
- Shi, J.; Gao, Y. A comparison of three ppp integer ambiguity resolution methods. Gps Solut. 2014, 18, 519–528. [Google Scholar] [CrossRef]
- Geng, J.; Meng, R.; Dodson, R.H.; Teferle, R.N. Integer ambiguity resolution in precise point positioning: Method comparison. J. Geod. 2010, 84, 569–581. [Google Scholar] [CrossRef]
- Glaner, M.; Weber, R. Ppp with integer ambiguity resolution for gps and galileo using satellite products from different analysis centers. GPS Solut. 2021, 25, 102. [Google Scholar] [CrossRef]
- Chen, C.; Xiao, G.; Chang, G.; Xu, T.; Yang, L. Assessment of gps/galileo/bds precise point positioning with ambiguity resolution using products from different analysis centers. Remote Sens. 2021, 13, 3266. [Google Scholar] [CrossRef]
- Ouyang, M.; Li, J.; Li, W.; Ge, Y.; Dai, Z. Research on time and frequency transfer during ppp convergence with parameters correlation comparison. Measurement 2020, 173, 108597. [Google Scholar] [CrossRef]
- Lyu, D.; Zeng, F.; Ouyang, X.; Zhang, H. Real-time clock comparison and monitoring with multi-gnss precise point positioning: Gps, glonass and galileo sciencedirect. Adv. Space Res. 2020, 65, 560–571. [Google Scholar] [CrossRef]
- Zhao, L.; Ye, S.; Jia, S. Handling the satellite inter-frequency biases in triple-frequency observations. Adv. Space Res. 2017, 59, 2048–2057. [Google Scholar] [CrossRef]
- Liu, S.; Sun, F.; Zhang, L.; Li, W.; Zhu, X. Tight integration of ambiguity-fixed ppp and ins: Model description and initial results. GPS Solut. 2016, 20, 39–49. [Google Scholar] [CrossRef]
- Defraigne, P.; Aerts, W.; Pottiaux, E. Monitoring of utc(k)’s using ppp and igs real-time products. Gps Solut. 2015, 19, 165–172. [Google Scholar] [CrossRef]
- Sastamoinen, J. Atmospheric correction for troposphere and stratosphere in radio ranging of satellites, in the use of artifical satellites for geodesy. Geophys. Monogr. Ser. 1972, 15, 247–252. [Google Scholar]
- Kouba, J. Testing of global pressure/temperature (gpt) model and global mapping function (gmf) in gps analyses. J. Geod. 2009, 83, 199–208. [Google Scholar] [CrossRef]
- Petit, G.; Luzum, B. Iers Conventions IERS Technical Note; Bonifatius GmbH: Paderborn, Germany, 2010. [Google Scholar]
- Ge, Y.; Dai, P.; Qin, W.; Yang, X.; Zhou, F.; Wang, S.; Zhao, X. Performance of multi-gnss precise point positioning time and frequency transfer with clock modeling. Remote Sens. 2019, 11, 347. [Google Scholar] [CrossRef]



| Site | External Source | Receiver | Antenna | |
|---|---|---|---|---|
| 1 | BOR1 | EXTERNAL H-MASER | TRIMBLE NETR9 | TRM59800.00 |
| 2 | GMSD | EXTERNAL CESIUM | TRIMBLE NETR9 | TRM59800.00 |
| 3 | JFNG | INTERNAL | TRIMBLE ALLOY | TRM59800.00 |
| 4 | KOUR | EXTERNAL H-MASER | SEPT POLARX5 | SEPCHOKE_B3E6 |
| 5 | MGUE | EXTERNAL H-MASER | SEPT POLARX5TR | LEIAR25.R4 |
| 6 | MIZU | INTERNAL | SEPT ASTERX4 | SEPCHOKE_B3E47 |
| 7 | NRC1 | EXTERNAL H-MASER | JAVAD TRE_G3TH DELTA | SEPCHOKE_B3E37 |
| 8 | OHI3 | EXTERNAL H-MASER | LEICA GR50 | SEPCHOKE_B3E49 |
| 9 | REDU | EXTERNAL CESIUM | SEPT POLARX5 | SEPCHOKE_B3E102 |
| 10 | STR1 | EXTERNAL CESIUM | SEPT POLARX5 | SEPCHOKE_B3E151 |
| 11 | VILL | EXTERNAL CESIUM | SEPT POLARX5 | SEPCHOKE_B3E6 |
| 12 | YEL2 | EXTERNAL VCH-1008 MASER | SEPT POLARX5TR | LEIAR25.R4 |
| 13 | USN7 | EXTERNAL H-MASER | SEPT POLARX5TR | TPSCR.G5 |
| 14 | PTBB | EXTERNAL ACTIVE H-MASER | SEPT POLARX5TR | SEPCHOKE_B3E89 |
| 15 | TIDV | EXTERNAL H-MASER | SEPT POLARX5 | SEPCHOKE_B3E169 |
| 16 | TID1 | EXTERNAL H-MASER | SEPT POLARX5 | SEPCHOKE_B3E169 |
| Agency | Strategy | Constellation |
|---|---|---|
| CNES | IRC | GRE |
| WUM | FCB | GREB2J |
| CODE | OSBs | GREB2 |
| PRIDE | OSBs | GREB2B3 |
| Project | Strategy |
|---|---|
| Stations | 16 globally distributed MGEX stations |
| Period | January 2020, DOY 001 |
| Observations | GPS: L1, L2 and Galileo: E1, E5a BDS: B1I, B3I |
| Interval | 30 s |
| Weighting | sin elev2, cut-off angle 7° |
| Phase wind-up | Phase wind-up Corrected |
| Tropospheric delay (ZHD) | Global Pressure and Temperature (GPT) [27] model using the formulas of Saastamoinen |
| Tropospheric delay (ZWD) | Estimated as a continuous piecewise linear function (2 h parameter spacing), GMF mapping function [28] |
| Tidal displacements | IERS conventions 2010 [29] |
| Relativistic effect | Corrected |
| Sagnac | Corrected |
| Satellite antenna PCOs and PCVs | Fixed to the values from igs14.atx |
| Strategy | Extended Strategy |
|---|---|
| FCB | FCB-com |
| FCB-gbm | |
| FCB-grm | |
| IRC | IRC-grm |
| OSB | OSB-com |
| OSB-whu |
| Strategy | Extended Strategy | Extended Extended Strategy |
|---|---|---|
| FCB | FCB-com | FCB_com_fix_G |
| FCB_com_fix_GE | ||
| FCB-gbm | FCB_gbm_fix_G | |
| FCB_gbm_fix_GE | ||
| FCB-grg | FCB_grm_fix_G | |
| FCB_grm_fix_GE | ||
| IRC | IRC-grm | IRC_grm_fix_G |
| IRC_grm_fix_GE | ||
| IRC_grm_fix_GEC | ||
| OSB | OSB-com | OSB_com_fix_G |
| OSB_com_fix_GE | ||
| OSB_com_fix_GEC | ||
| OSB-whu | OSB_whu_fix_G | |
| OSB_whu_fix_GE | ||
| OSB_whu_fix_GEC |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ouyang, M.; Zhu, X.; Yi, R.; Lyu, D. Using Various Analysis Center Products to Assess the Time-Frequency Transfer Performance of GPS/Galileo/BDS PPPAR Methods. Remote Sens. 2023, 15, 92. https://doi.org/10.3390/rs15010092
Ouyang M, Zhu X, Yi R, Lyu D. Using Various Analysis Center Products to Assess the Time-Frequency Transfer Performance of GPS/Galileo/BDS PPPAR Methods. Remote Sensing. 2023; 15(1):92. https://doi.org/10.3390/rs15010092
Chicago/Turabian StyleOuyang, Mingjun, Xiangwei Zhu, Ruite Yi, and Daqian Lyu. 2023. "Using Various Analysis Center Products to Assess the Time-Frequency Transfer Performance of GPS/Galileo/BDS PPPAR Methods" Remote Sensing 15, no. 1: 92. https://doi.org/10.3390/rs15010092
APA StyleOuyang, M., Zhu, X., Yi, R., & Lyu, D. (2023). Using Various Analysis Center Products to Assess the Time-Frequency Transfer Performance of GPS/Galileo/BDS PPPAR Methods. Remote Sensing, 15(1), 92. https://doi.org/10.3390/rs15010092






