Moist Convection in the Giant Planet Atmospheres
Abstract
1. Introduction
2. Review of Observations
2.1. Jupiter
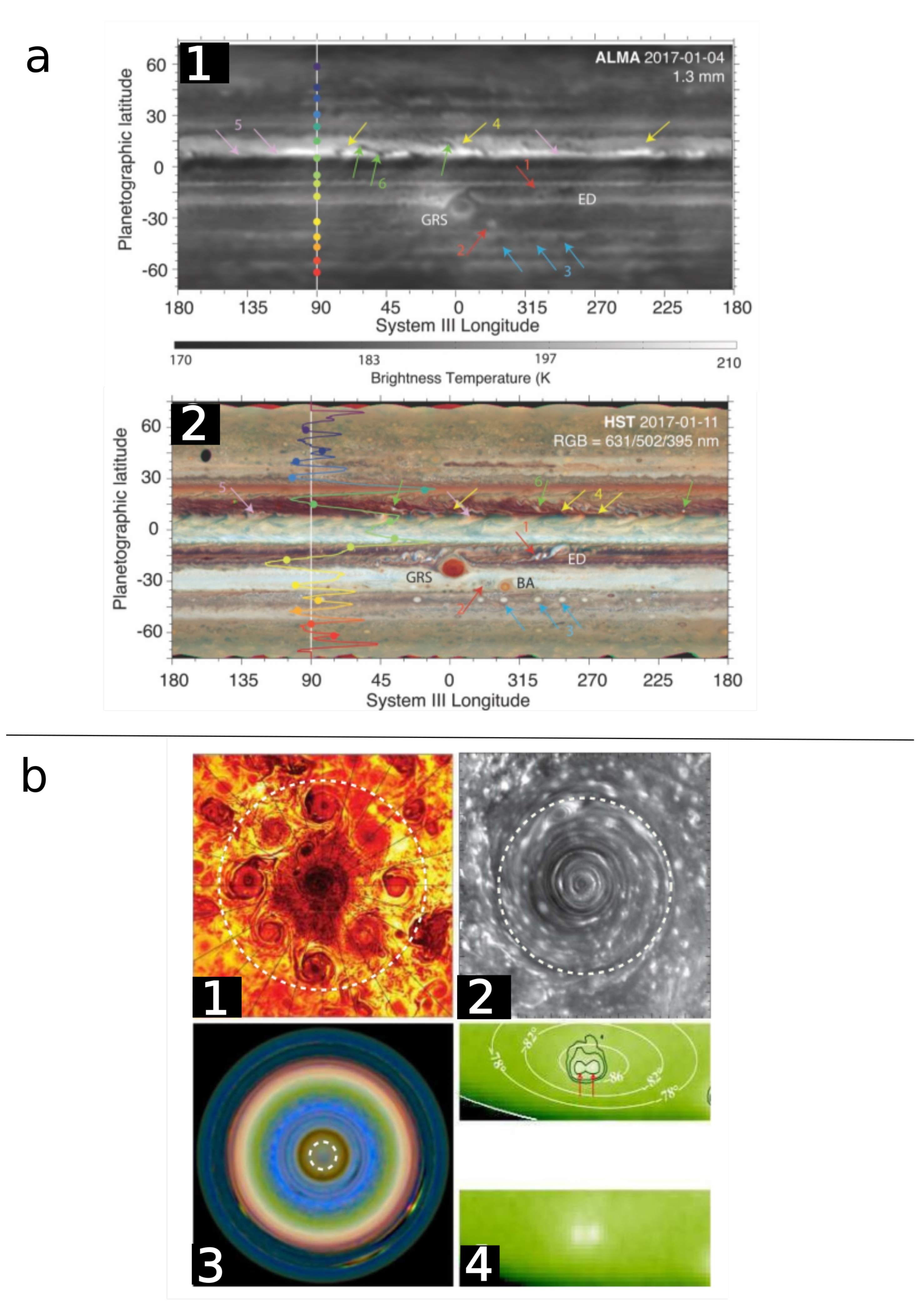
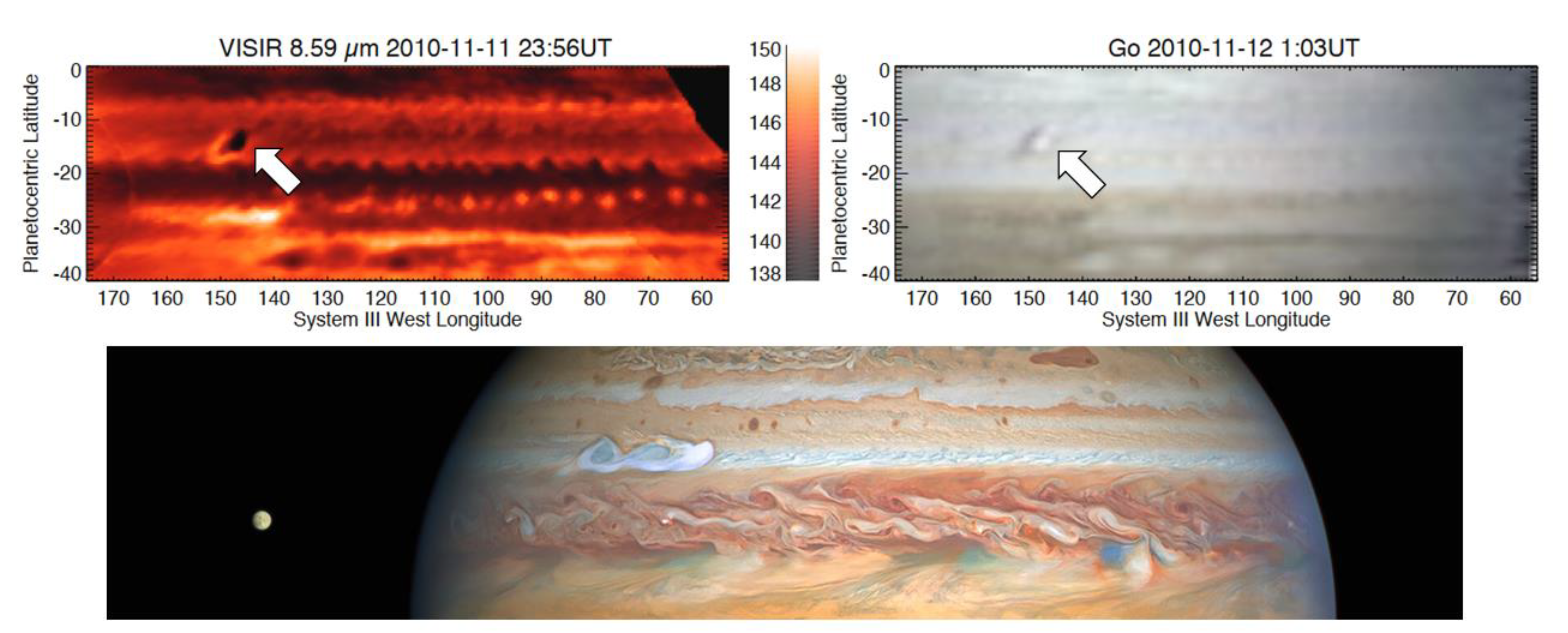
2.1.1. Zonal Disruption Events
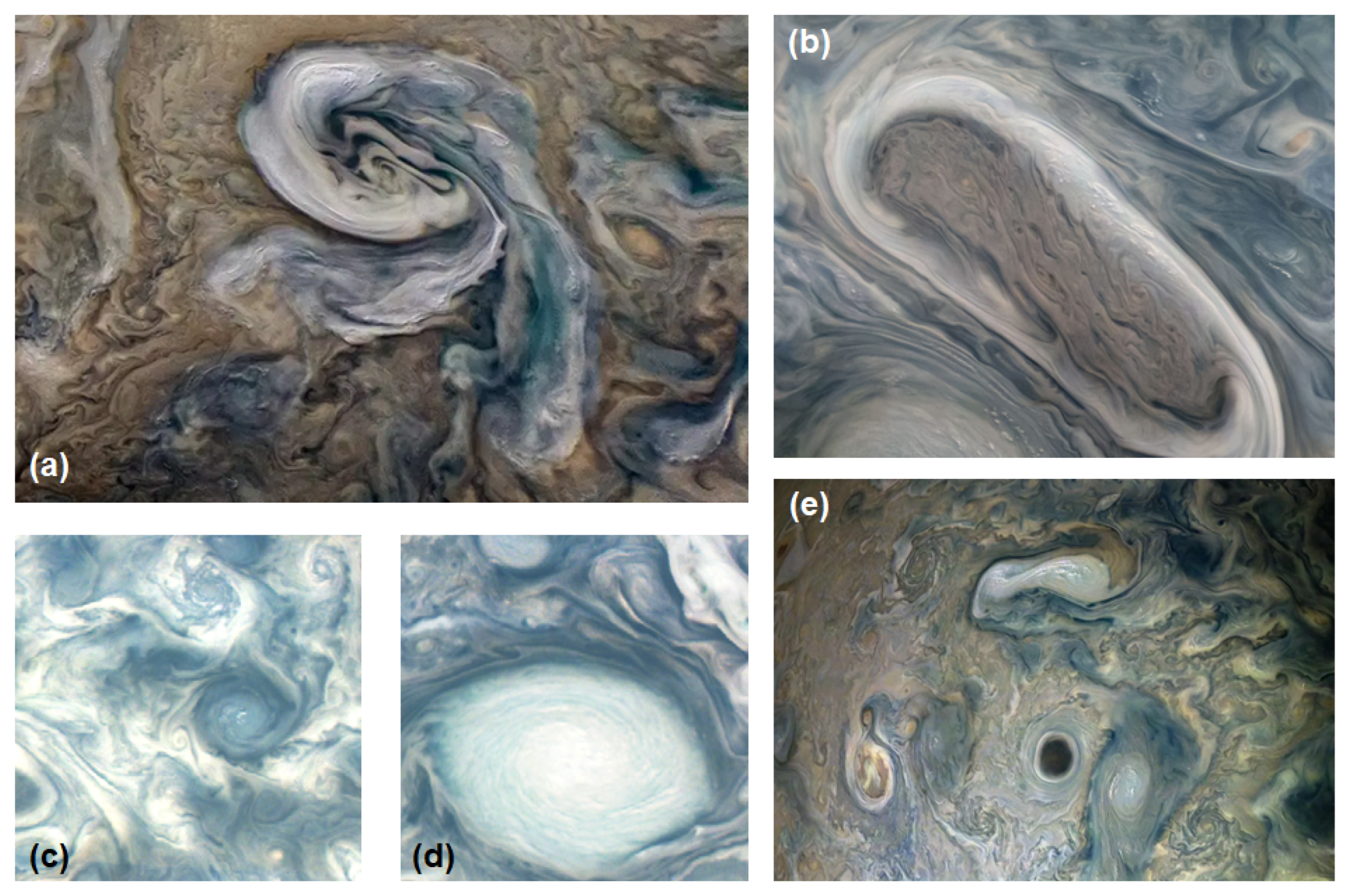
2.1.2. Cyclone Induced Convection
2.1.3. Pop-Up Clouds
2.2. Saturn
2.2.1. Local Convective Episodes
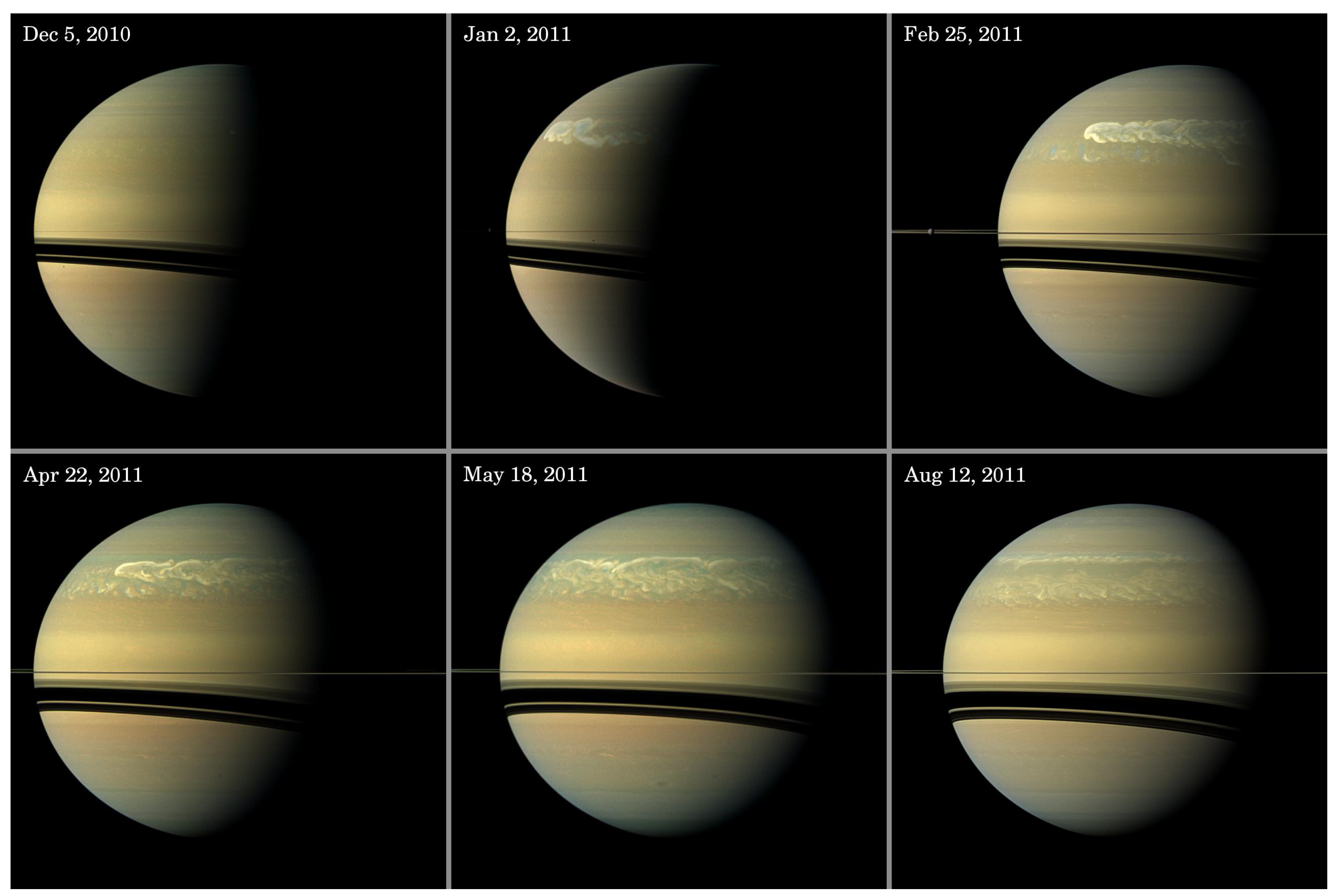
2.2.2. Great White Spots (GWSs)
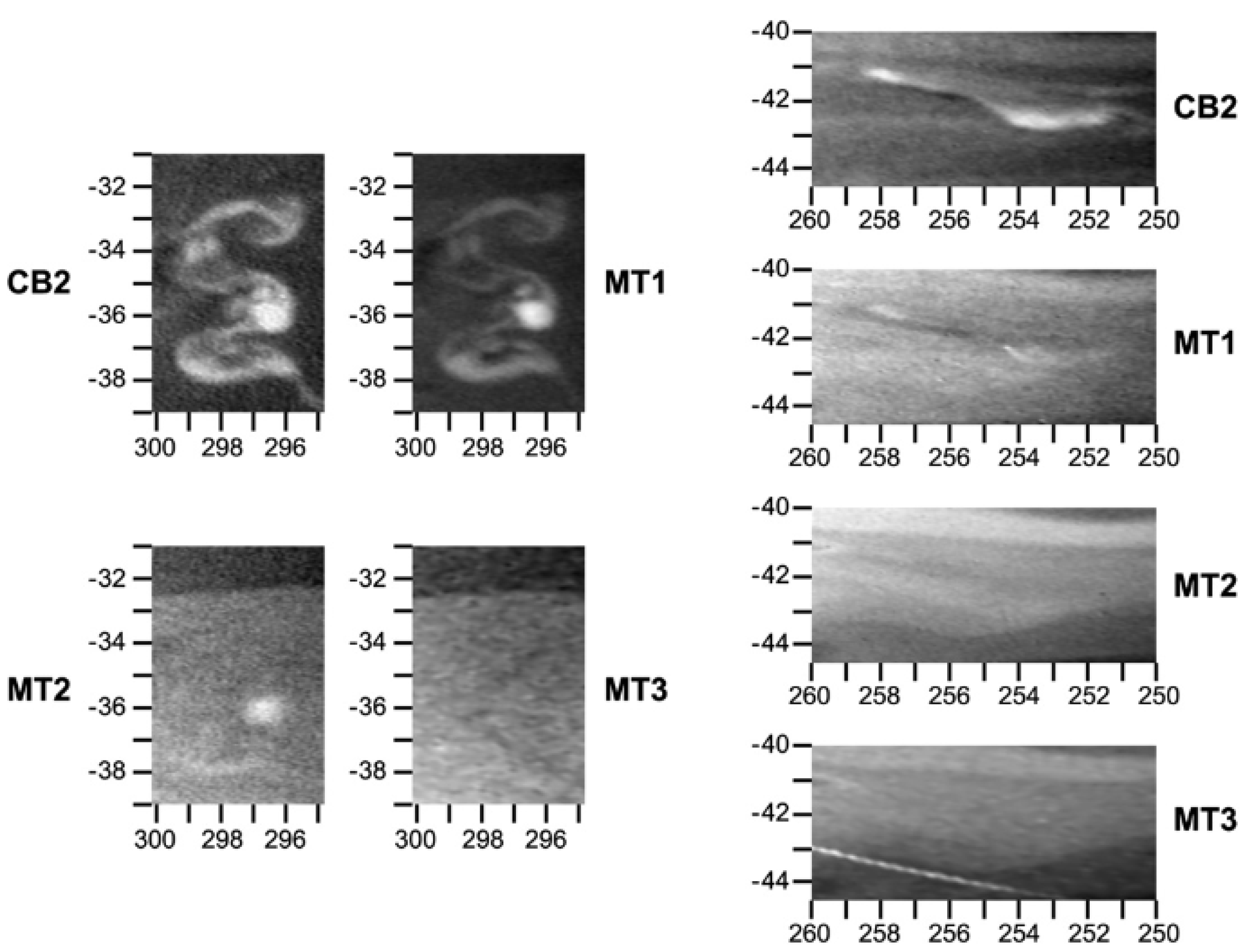
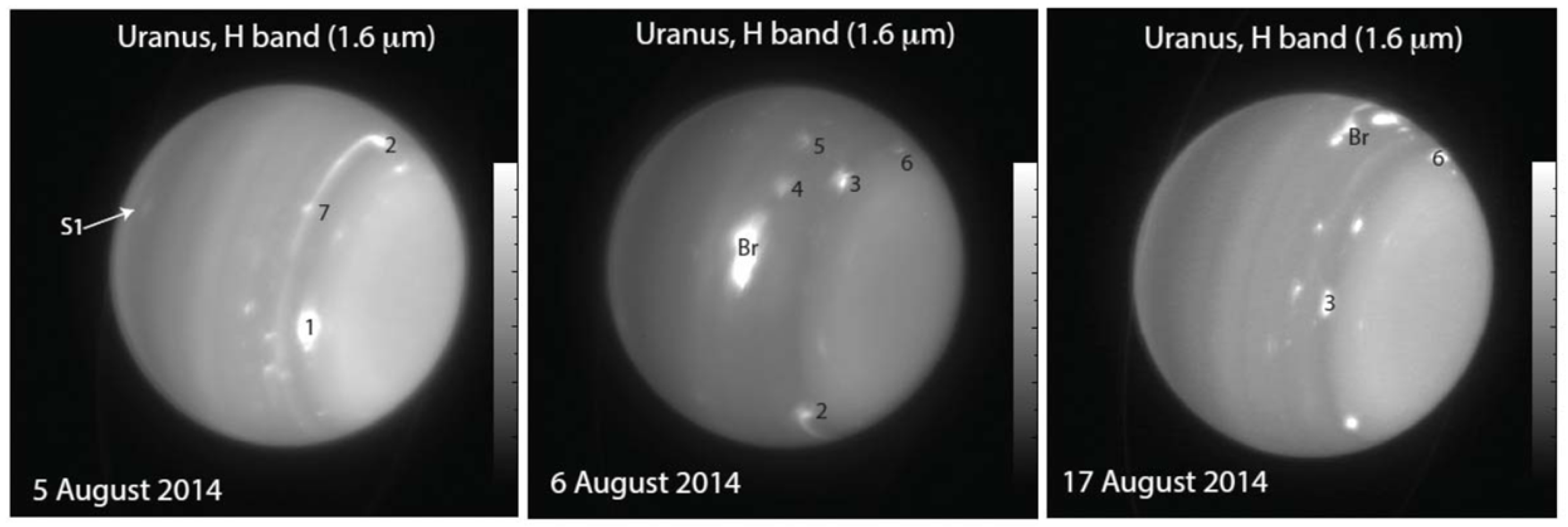
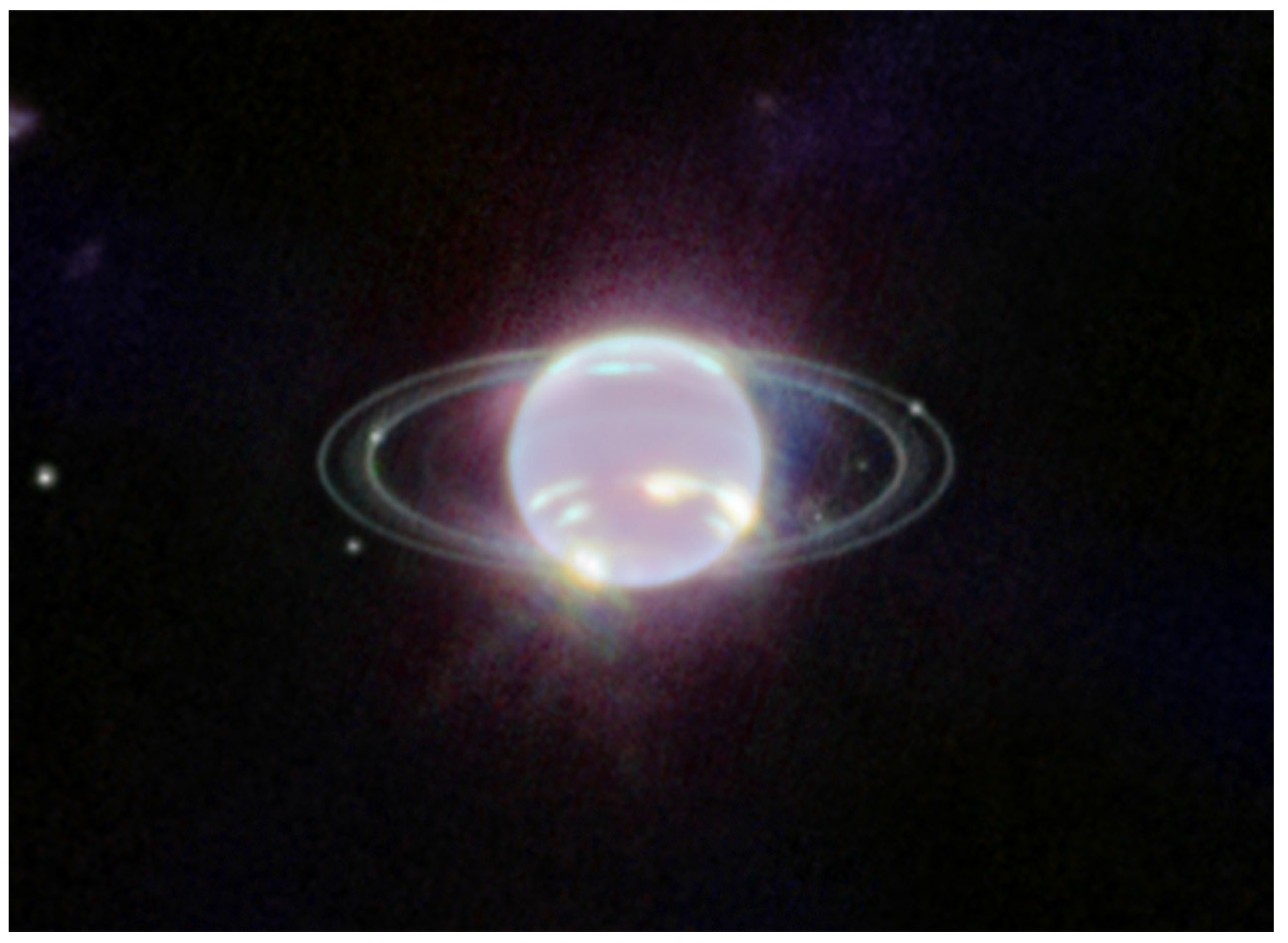
2.3. Uranus
2.4. Neptune
3. Dynamical and Microphysical Processes in Convective Storms
3.1. Cloud Microphysics: Lifetimes of Condensates in Planetary Atmospheres
- 1.
- Condensation/Deposition: Condensation is the process whereby moisture from the vapor surrounding the particle condenses directly on to the particle. For a super-saturated environment, the vapor will readily condense on the surface of the cloud particle, allowing for quick growth from the initial embryo. Given a constant ambient supersaturation, the rate of cloud size growth decreases with radius, thereby making this process very inefficient in generating large particle sizes. For Earth and even for Gas Giant atmospheres, condensation is primarily responsible for particle sizes up to m. For ice particles, the same process occurs, but the liquid phase is skipped, and vapor is directly deposited as ice on the particle.
- 2.
- Precipitation: Due to the small sizes of the particles, they quickly reach terminal velocity, and fall as they continue to grow. The terminal velocity of the particle depends on the shape and phase of the particle, and generally increases quickly with particle radius. Very small particles ( 1 m) fall very slowly, and thus clouds with such particles are generally treated as ‘non-precipitating’, with precipitation generally describing only larger particles ( 250 m) [109], whose terminal velocities reach on the order of cm/s to m/s. The shape of the particle (especially for ices), drastically affects the hydrodynamics of the particle, and larger particles deviate strongly from the Stokes regime [110]. Smaller particles (≲1 m) usually have high Knudsen number, and thus require the Cunningham correction [111]. Therefore, throughout the lifetime of a hydrometeor, the flow around the particle may pass through several different flow regimes. The typical terminal velocity profiles of cloud ice and snow particles in Solar System gas giants are shown in Figure 9. See Loftus and Wordsworth [112] for a detailed analysis of raindrops in planetary atmospheres and Guillot et al. [113, 114] for mixed-phase solid particles.
- 3.
- Collection (coagulation/aggregation and coalescence): Growth beyond the 1 m sizes occurs primarily through collisions and sticking between different particles. As particles grow, so too does their terminal velocity, and thus, larger particles will generally fall through a field of smaller sized particles. The sticking efficiency, usually denoted by , describes the probability of two particles of different sizes r and being able to constructively collide to form a larger particle. Sticking efficiency is usually a complicated function of the difference in velocities, the mechanical properties of species of the two particles (e.g., surface tension) as well as the nature of particles (ice/liquid). However, in general, sticking efficiency increases with the size of the particle, leading to a runaway growth in particle size, as long as there is sufficient cloud mass for a particle (now called a ‘hydrometeor’) to accrete. Coalescence is the process where smaller particles are absorbed into the larger particles due to the latter falling through a ‘field’ of smaller hydrometeors, while coagulation/aggregation refers to the process where nearly equally sized particles merge due to chance collisions from Brownian motion. Justifiably, coalescence dominates for larger particles, while coagulation is more efficient in the small radius regime.
3.2. Dynamics of a Convecting Parcel
3.3. Effects of Convection on Cloud Particle Growth
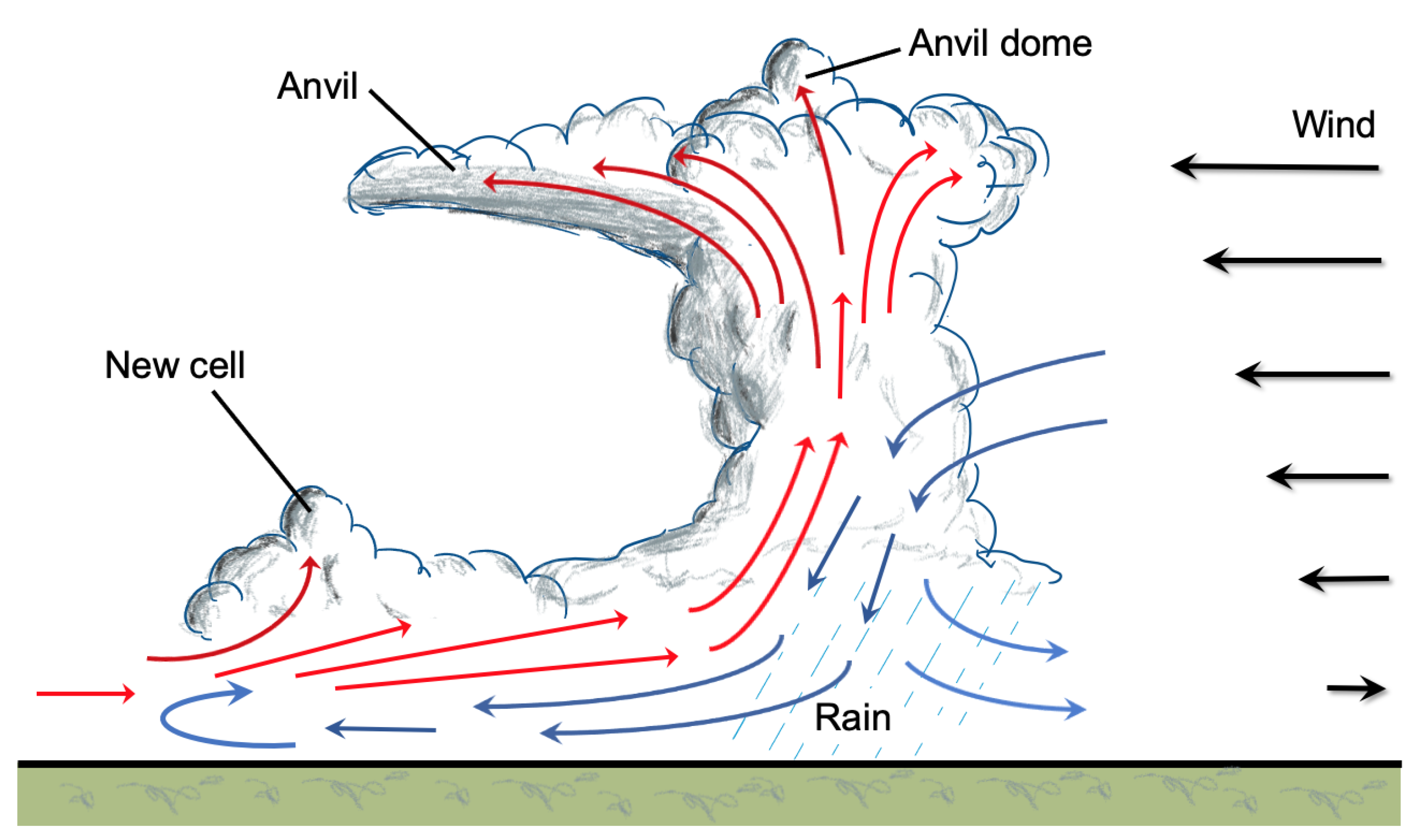
3.4. Types of Cumulus Convection
4. Convective Energy Cascade
4.1. Turbulence and the Inverse-Cascade: Jets
4.1.1. Turbulence: A Short Background for 3D, 2D, and Geostrophic Flows

4.1.2. Retrieved Kinetic Energy Power Spectra from Giant Planet Atmospheres
4.1.3. Retrieved Passive Tracer Power Spectra from Giant Planet Atmospheres
4.2. Numerical Modeling of Jet Dynamics on Giant Planets: Forcing and Organization
4.3. Turbulence and the Inverse-Cascade: Vortices
4.3.1. Large Vortices on the Giant Planets
4.3.2. Power Spectra in Giant Planet Large Vortices
4.3.3. Numerical Modeling of Polar Vortices on Giant Planets: Forcing and Organization
5. Summary and Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hooke, R. A spot on one of the belts of Jupiter. Philos. Trans. R. Soc. 1665, 1, 3. [Google Scholar]
- Hueso, R.; Legarreta, J.; Pérez-Hoyos, S.; Rojas, J.F.; Sánchez-Lavega, A.; Morgado, A. The international outer planets watch atmospheres node database of giant-planet images. Planet. Space Sci. 2010, 58, 1152–1159. [Google Scholar] [CrossRef]
- Hueso, R.; Juaristi, J.; Legarreta, J.; Sánchez-Lavega, A.; Rojas, J.F.; Erard, S.; Cecconi, B.; Le Sidaner, P. The Planetary Virtual Observatory and Laboratory (PVOL) and its integration into the Virtual European Solar and Planetary Access (VESPA). Planet. Space Sci. 2018, 150, 22–35. [Google Scholar] [CrossRef]
- Hansen, C.J.; Caplinger, M.A.; Ingersoll, A.; Ravine, M.A.; Jensen, E.; Bolton, S.; Orton, G. Junocam: Juno’s Outreach Camera. Space Sci. Rev. 2017, 213, 475–506. [Google Scholar] [CrossRef]
- Grassi, D.; Mura, A.; Sindoni, G.; Adriani, A.; Atreya, S.K.; Filacchione, G.; Fletcher, L.N.; Lunine, J.I.; Moriconi, M.L.; Noschese, R.; et al. On the clouds and ammonia in Jupiter’s upper troposphere from Juno JIRAM reflectivity observations. Mon. Not. R. Astron. Soc. 2021, 503, 4892–4907. [Google Scholar] [CrossRef]
- Bjoraker, G.L.; Wong, M.H.; de Pater, I.; Hewagama, T.; Ádámkovics, M. The Spatial Variation of Water Clouds, NH3, and H2O on Jupiter Using Keck Data at 5 Microns. Remote Sens. 2022, 14, 4567. [Google Scholar] [CrossRef]
- Wong, M.H.; Atreya, S.K.; Kuhn, W.R.; Romani, P.N.; Mihalka, K.M. Fresh clouds: A parameterized updraft method for calculating cloud densities in one-dimensional models. Icarus 2015, 245, 273–281. [Google Scholar] [CrossRef]
- Simon, A.A.; Wong, M.H.; Sromovsky, L.A.; Fletcher, L.N.; Fry, P.M. Giant Planet Atmospheres: Dynamics and Variability from UV to Near-IR Hubble and Adaptive Optics Imaging. Remote Sens. 2022, 14, 1518. [Google Scholar] [CrossRef]
- Cook, A.F., I; Duxbury, T.C.; Hunt, G.E. First results on Jovian lightning. Nature 1979, 280, 794. [Google Scholar] [CrossRef]
- Borucki, W.J.; Bar-Nun, A.; Scarf, F.L.; Cook, A.F.; Hunt, G.E. Lightning activity on Jupiter. Icarus 1982, 52, 492–502. [Google Scholar] [CrossRef]
- Magalhaes, J.A.; Borucki, W.J. Spatial distribution of visible lightning on Jupiter. Nature 1991, 349, 311–313. [Google Scholar] [CrossRef]
- Little, B.; Anger, C.D.; Ingersoll, A.P.; Vasavada, A.R.; Senske, D.A.; Breneman, H.H.; Borucki, W.J. Galileo Images of Lightning on Jupiter. Icarus 1999, 142, 306–323. [Google Scholar] [CrossRef]
- Porco, C.C.; West, R.A.; McEwen, A.; Del Genio, A.D.; Ingersoll, A.P.; Thomas, P.; Squyres, S.; Dones, L.; Murray, C.D.; Johnson, T.V.; et al. Cassini imaging of Jupiter’s atmosphere, satellites, and rings. Science 2003, 299, 1541–1547. [Google Scholar] [CrossRef]
- Ingersoll, A.P.; Gierasch, P.J.; Banfield, D.; Vasavada, A.R. Moist convection as an energy source for the large-scale motions in Jupiter’s atmosphere. Nature 2000, 403, 630–632. [Google Scholar] [CrossRef] [PubMed]
- Fletcher, L.N.; Kaspi, Y.; Guillot, T.; Showman, A.P. How Well Do We Understand the Belt/Zone Circulation of Giant Planet Atmospheres? Space Sci. Rev. 2020, 216, 1–33. [Google Scholar] [CrossRef] [PubMed]
- Vasavada, A.R.; Showman, A.P. Jovian atmospheric dynamics: An update after Galileo and Cassini. Rep. Prog. Phys. 2005, 68, 1935–1996. [Google Scholar] [CrossRef]
- Brown, S.; Janssen, M.; Adumitroaie, V.; Atreya, S.; Bolton, S.; Gulkis, S.; Ingersoll, A.; Levin, S.; Li, C.; Li, L.; et al. Prevalent lightning sferics at 600 megahertz near Jupiter’s poles. Nature 2018, 558, 87–90. [Google Scholar] [CrossRef]
- Sankar, R.; Palotai, C. A new convective parameterization applied to Jupiter: Implications for water abundance near the 24° N region. Icarus 2022, 380, 114973. [Google Scholar] [CrossRef]
- Orton, G.S.; Antunano, A.; Fletcher, L.N.; Sinclair, J.A.; Momary, T.W.; Fujiyoshi, T.; Yanamandra-Fisher, P.; Donnelly, P.T.; Greco, J.J.; Payne, A.V.; et al. Unexpected Long-Term Variability in Jupiter’s Tropospheric Temperatures. arXiv 2022, arXiv:2211.04398. [Google Scholar] [CrossRef]
- Sanchez-Lavega, A.; Quesada, J.A. Ground-based imaging of Jovian cloud morphologies and motions. II - The northern hemisphere from 1975 to 1985. Icarus 1988, 76, 533–557. [Google Scholar] [CrossRef]
- Sanchez-Lavega, A.; Laques, P.; Parker, D.C.; Viscardy, G. Midscale dynamical features observed during 1987 in the North Equatorial Belt of Jupiter. Icarus 1990, 87, 475–483. [Google Scholar] [CrossRef]
- Sánchez-Lavega, A.; Orton, G.S.; Hueso, R.; García-Melendo, E.; Pérez-Hoyos, S.; Simon-Miller, A.; Rojas, J.F.; Gómez, J.M.; Yanamandra-Fisher, P.; Fletcher, L.; et al. Depth of a strong jovian jet from a planetary-scale disturbance driven by storms. Nature 2008, 451, 437–440. [Google Scholar] [CrossRef] [PubMed]
- Sanchez-Lavega, A.; Rodrigo, R. Ground based observations of synoptic cloud systems in southern equatorial to temperate latitudes of Jupiter from 1975 to 1983. Astron. Astrophys. 1985, 148, 67–78. [Google Scholar]
- Lii, P.S.; Wong, M.H.; de Pater, I. Temporal variation of the tropospheric cloud and haze in the jovian equatorial zone. Icarus 2010, 209, 591–601. [Google Scholar] [CrossRef]
- Simon-Miller, A.A.; Chanover, N.J.; Orton, G.S.; Sussman, M.; Tsavaris, I.G.; Karkoschka, E. Jupiter’s White Oval turns red. Icarus 2006, 185, 558–562. [Google Scholar] [CrossRef]
- Sanchez-Lavega, A.; Gomez, J.M. The South Equatorial Belt of Jupiter, I: Its Life Cycle. Icarus 1996, 121, 1–17. [Google Scholar] [CrossRef]
- Fletcher, L.N.; Orton, G.S.; Rogers, J.H.; Simon-Miller, A.A.; de Pater, I.; Wong, M.H.; Mousis, O.; Irwin, P.G.J.; Jacquesson, M.; Yanamandra-Fisher, P.A. Jovian temperature and cloud variability during the 2009-2010 fade of the South Equatorial Belt. Icarus 2011, 213, 564–580. [Google Scholar] [CrossRef]
- Pérez-Hoyos, S.; Sanz-Requena, J.F.; Barrado-Izagirre, N.; Rojas, J.F.; Sánchez-Lavega, A.; IOPW Team. The 2009-2010 fade of Jupiter’s South Equatorial Belt: Vertical cloud structure models and zonal winds from visible imaging. Icarus 2012, 217, 256–271. [Google Scholar] [CrossRef]
- Fletcher, L.N.; Orton, G.S.; Rogers, J.H.; Giles, R.S.; Payne, A.V.; Irwin, P.G.; Vedovato, M. Moist convection and the 2010–2011 revival of Jupiter’s South Equatorial Belt. Icarus 2017, 286, 94–117. [Google Scholar] [CrossRef]
- Sanchez-Lavega, A.; Miyazaki, I.; Parker, D.; Laques, P.; Lecacheux, J. A disturbance in Jupiter’s high-speed North temperate jet during 1990. Icarus 1991, 94, 92–97. [Google Scholar] [CrossRef]
- Sánchez-Lavega, A.; Rogers, J.H.; Orton, G.S.; García-Melendo, E.; Legarreta, J.; Colas, F.; Dauvergne, J.L.; Hueso, R.; Rojas, J.F.; Pérez-Hoyos, S.; et al. A planetary-scale disturbance in the most intense Jovian atmospheric jet from JunoCam and ground-based observations. Geophys. Res. Lett. 2017, 44, 4679–4686. [Google Scholar] [CrossRef]
- Sankar, R.; Klare, C.; Palotai, C. The aftermath of convective events near Jupiter’s fastest prograde jet: Implications for clouds, dynamics and vertical wind shear. Icarus 2021, 368, 114589. [Google Scholar] [CrossRef]
- Antuñano, A.; del Río-Gaztelurrutia, T.; Sánchez-Lavega, A.; Rodríguez-Aseguinolaza, J. Cloud morphology and dynamics in Saturn’s northern polar region. Icarus 2018, 299, 117–132. [Google Scholar] [CrossRef]
- Hueso, R.; Iñurrigarro, P.; Sánchez-Lavega, A.; Foster, C.R.; Rogers, J.H.; Orton, G.S.; Hansen, C.; Eichstädt, G.; Ordonez-Etxeberria, I.; Rojas, J.F.; et al. Convective storms in closed cyclones in Jupiter’s South Temperate Belt: (I) observations. Icarus 2022, 380, 114994. [Google Scholar] [CrossRef]
- Iñurrigarro, P.; Hueso, R.; Legarreta, J.; Sánchez-Lavega, A.; Eichstädt, G.; Rogers, J.; Orton, G.; Hansen, C.; Pérez-Hoyos, S.; Rojas, J.; et al. Observations and numerical modelling of a convective disturbance in a large-scale cyclone in Jupiter’s South Temperate Belt. Icarus 2020, 336, 113475. [Google Scholar] [CrossRef]
- Orton, G.; Eichstaedt, G.; Guillot, T.; Rosenqvist, M.; Brueshaber, S.; Keaveney, C.; Hansen, C.; Wong, M.; Kelly, K.; Momary, T.; et al. Investigating Relative Cloud Heights in Jupiter Using Juno’s JunoCam Imager. AAS Div. Planet. Sci. 2022, 54, 306.06. [Google Scholar]
- Hansen, C.J.; Brueshaber, S.; Orton, G.; Momary, T.; Bolton, S.J. JunoCam Images of Castellanus Clouds on Jupiter. In Proceedings of the AGU Fall Meeting Abstracts, Washington, DC, USA, 9–13 December 2019; Volume 2019, p. P44A-05. [Google Scholar]
- Fletcher, L.; Oyafuso, F.; Orton, G.; Zhang, Z.; Brueshaber, S.; Wong, M.; Li, C.; Mura, A.; Grassi, D.; Melin, H.; et al. Juno Characterisation of Cyclonic “Folded Filamentary Regions” within Jupiter’s Polar Domains. In Proceedings of the 44th COSPAR Scientific Assembly, Athens, Greece, 16–24 July 2022. [Google Scholar]
- Atreya, S.K.; Hofstadter, M.H.; In, J.H.; Mousis, O.; Reh, K.; Wong, M.H. Deep Atmosphere Composition, Structure, Origin, and Exploration, with Particular Focus on Critical in situ Science at the Icy Giants. Space Sci. Rev. 2020, 216, 18. [Google Scholar] [CrossRef]
- Warwick, J.W.; Pearce, J.B.; Evans, D.R.; Carr, T.D.; Schauble, J.J.; Alexander, J.K.; Kaiser, M.L.; Desch, M.D.; Pedersen, M.; Lecacheux, A.; et al. Planetary Radio Astronomy Observations from Voyager 1 near Saturn. Science 1981, 212, 239–243. [Google Scholar] [CrossRef]
- Warwick, J.W.; Evans, D.R.; Romig, J.H.; Alexander, J.K.; Desch, M.D.; Kaiser, M.L.; Aubier, M.G.; Leblanc, Y.; Lecacheux, A.; Pedersen, B.M. Planetary Radio Astronomy Observations from Voyager 2 near Saturn. Science 1982, 215, 582–587. [Google Scholar] [CrossRef]
- Burns, J.A.; Showalter, M.R.; Cuzzi, J.N.; Durisen, R.H. Saturn’s electrostatic discharges: Could lightning be the cause? Icarus 1983, 54, 280–295. [Google Scholar] [CrossRef]
- Kaiser, M.L.; Connerney, J.E.P.; Desch, M.D. Atmospheric storm explanation of saturnian electrostatic discharges. Nature 1983, 303, 50–53. [Google Scholar] [CrossRef]
- Gurnett, D.A.; Kurth, W.S.; Hospodarsky, G.B.; Persoon, A.M.; Averkamp, T.F.; Cecconi, B.; Lecacheux, A.; Zarka, P.; Canu, P.; Cornilleau-Wehrlin, N.; et al. Radio and Plasma Wave Observations at Saturn from Cassini’s Approach and First Orbit. Science 2005, 307, 1255–1259. [Google Scholar] [CrossRef] [PubMed]
- Dyudina, U.A.; Ingersoll, A.P.; Ewald, S.P.; Porco, C.C.; Fischer, G.; Kurth, W.; Desch, M.; Del Genio, A.; Barbara, J.; Ferrier, J. Lightning storms on Saturn observed by Cassini ISS and RPWS during 2004-2006. Icarus 2007, 190, 545–555. [Google Scholar] [CrossRef]
- Fischer, G.; Kurth, W.S.; Dyudina, U.A.; Kaiser, M.L.; Zarka, P.; Lecacheux, A.; Ingersoll, A.P.; Gurnett, D.A. Analysis of a giant lightning storm on Saturn. Icarus 2007, 190, 528–544. [Google Scholar] [CrossRef]
- Porco, C.C.; Baker, E.; Barbara, J.; Beurle, K.; Brahic, A.; Burns, J.A.; Charnoz, S.; Cooper, N.; Dawson, D.D.; Del Genio, A.D.; et al. Cassini Imaging Science: Initial Results on Saturn’s Atmosphere. Science 2005, 307, 1243–1247. [Google Scholar] [CrossRef]
- Sromovsky, L.A.; Baines, K.H.; Fry, P.M. Models of bright storm clouds and related dark ovals in Saturn’s Storm Alley as constrained by 2008 Cassini/VIMS spectra. Icarus 2018, 302, 360–385. [Google Scholar] [CrossRef]
- Sánchez-Lavega, A.; García-Melendo, E.; Legarreta, J.; Hueso, R.; del Río-Gaztelurrutia, T.; Sanz-Requena, J.F.; Pérez-Hoyos, S.; Simon, A.A.; Wong, M.H.; Soria, M.; et al. A complex storm system in Saturn’s north polar atmosphere in 2018. Nat. Astron. 2020, 4, 180–187. [Google Scholar] [CrossRef]
- Gunnarson, J.L.; Sayanagi, K.M.; Fischer, G.; Barry, T.; Wesley, A.; Dyudina, U.A.; Ewald, S.P.; Ingersoll, A.P. Multiple convective storms within a single cyclone on Saturn. Icarus 2023, 389, 115228. [Google Scholar] [CrossRef]
- Fischer, G.; Delcroix, M.; Go, C.; Kurth, W.S.; Zarka, P.; Wesley, A.; Dyudina, U.A.; Ewald, S.P.; Porco, C.C.; Gurnett, D.A.; et al. A giant thunderstorm on Saturn. Nature 2011, 475, 75–77. [Google Scholar] [CrossRef]
- Sanchez-Lavega, A. Saturn’s Great White Spots. Chaos Interdiscip. J. Nonlinear Sci. 1994, 4, 341–353. [Google Scholar] [CrossRef]
- Westphal, J.A.; Baum, W.A.; Ingersoll, A.P.; Barnet, C.D.; de Jong, E.M.; Danielson, G.E.; Caldwell, J. Hubble Space Telescope observations of the 1990 equatorial disturbance on Saturn - Images, albedos, and limb darkening. Icarus 1992, 100, 485–498. [Google Scholar] [CrossRef]
- Hueso, R.; Sánchez-Lavega, A. A three-dimensional model of moist convection for the giant planets II: Saturn’s water and ammonia moist convective storms. Icarus 2004, 172, 255–271. [Google Scholar] [CrossRef]
- Sayanagi, K.M.; Showman, A.P. Effects of a large convective storm on Saturn’s equatorial jet. Icarus 2007, 187, 520–539. [Google Scholar] [CrossRef]
- Sayanagi, K.M.; Dyudina, U.A.; Ewald, S.P.; Fischer, G.; Ingersoll, A.P.; Kurth, W.S.; Muro, G.D.; Porco, C.C.; West, R.A. Dynamics of Saturn’s great storm of 2010-2011 from Cassini ISS and RPWS. Icarus 2013, 223, 460–478. [Google Scholar] [CrossRef]
- Sánchez-Lavega, A.; Fischer, G.; Fletcher, L.N.; Garcia-Melendo, E.; Hesman, B.; Perez-Hoyos, S.; Sayanagi, K.M.; Sromovsky, L.A. The Great Saturn Storm of 2010–2011. In Saturn in the 21st Century; Flasar, F.M., Baines, K.H., Eds.; Cambridge University Press: Cambridge, MA, USA, 2018. [Google Scholar]
- Sromovsky, L.A.; Baines, K.H.; Fry, P.M. Saturn’s Great Storm of 2010-2011: Evidence for ammonia and water ices from analysis of VIMS spectra. Icarus 2013, 226, 402–418. [Google Scholar] [CrossRef]
- Sayanagi, K.M.; Dyudina, U.A.; Ewald, S.P.; Muro, G.D.; Ingersoll, A.P. Cassini ISS observation of Saturn’s String of Pearls. Icarus 2014, 229, 170–180. [Google Scholar] [CrossRef]
- Conrath, B.J.; Hanel, R.A.; Samuelson, R.E. Thermal structure and heat balance of the outer planets. In Origin and Evolution of Planetary and Satellite Atmospheres; Atreya, S.K., Pollack, J.B., Matthews, M.S., Eds.; University of Arizona Press: Tucson, AZ, USA, 1989; pp. 513–538. [Google Scholar]
- Gierasch, P.J.; Conrath, B.J. Dynamics of the atmospheres of the outer planets: Post-Voyager measurement objectives. J. Geophys. Resaerch 1993, 98, 5459–5469. [Google Scholar] [CrossRef]
- Fouchet, T.; Greathouse, T.K.; Spiga, A.; Fletcher, L.N.; Guerlet, S.; Leconte, J.; Orton, G.S. Stratospheric aftermath of the 2010 Storm on Saturn as observed by the TEXES instrument. I. Temperature structure. Icarus 2016, 277, 196–214. [Google Scholar] [CrossRef]
- Li, L.; Trammell, H.J.; Knowles, B.; Flasar, F.M.; West, R.A.; Perez-Hoyos, S.; Pan, Y.; Hernandez, J.; Baines, K.H.; Gierasch, P.J.; et al. Saturn’s giant storm and global radiant energy. Geophys. Res. Lett. 2015, 42, 2144–2148. [Google Scholar] [CrossRef]
- Li, C.; Ingersoll, A.P. Moist convection in hydrogen atmospheres and the frequency of Saturn’s giant storms. Nat. Geosci. 2015, 8, 398–403. [Google Scholar] [CrossRef]
- Del Genio, A.D.; Barbara, J.M.; Ferrier, J.; Ingersoll, A.P.; West, R.A.; Vasavada, A.R.; Spitale, J.; Porco, C.C. Saturn eddy momentum fluxes and convection: First estimates from Cassini images. Icarus 2007, 189, 479–492. [Google Scholar] [CrossRef]
- Irwin, P.G.; Toledo, D.; Garland, R.; Teanby, N.A.; Fletcher, L.N.; Orton, G.A.; Bézard, B. Detection of hydrogen sulfide above the clouds in Uranus’s atmosphere. Nat. Astron. 2018, 2, 420–427. [Google Scholar] [CrossRef]
- Alexander, A.F.O. The Planet Uranus; A History of Observation, Theory, and Discovery; American Elsevier Pub. Co.: New York, NY, USA, 1965. [Google Scholar]
- Karkoschka, E. Uranus’ southern circulation revealed by Voyager 2: Unique characteristics. Icarus 2015, 250, 294–307. [Google Scholar] [CrossRef]
- Smith, B.A.; Soderblom, L.A.; Beebe, R.; Bliss, D.; Boyce, J.M.; Brahic, A.; Briggs, G.A.; Brown, R.H.; Collins, S.A.; Cook, A.F.; et al. Voyager 2 in the Uranian System: Imaging Science Results. Science 1986, 233, 43–64. [Google Scholar] [CrossRef] [PubMed]
- Zarka, P.; Pedersen, B.M. Radio detection of uranian lightning by Voyager 2. Nature 1986, 323, 605–608. [Google Scholar] [CrossRef]
- Weidenschilling, S.J.; Lewis, J.S. Atmospheric and cloud structures of the jovian planets. Icarus 1973, 20, 465–476. [Google Scholar] [CrossRef]
- Karkoschka, E. Clouds of High Contrast on Uranus. Science 1998, 280, 570. [Google Scholar] [CrossRef]
- Hammel, H.B.; Rages, K.; Lockwood, G.W.; Karkoschka, E.; de Pater, I. New Measurements of the Winds of Uranus. Icarus 2001, 153, 229–235. [Google Scholar] [CrossRef]
- Sromovsky, L.A.; Fry, P.M. Dynamics of cloud features on Uranus. Icarus 2005, 179, 459–484. [Google Scholar] [CrossRef]
- Sromovsky, L.A.; Fry, P.M.; Hammel, H.B.; Ahue, W.M.; de Pater, I.; Rages, K.A.; Showalter, M.R.; van Dam, M.A. Uranus at equinox: Cloud morphology and dynamics. Icarus 2009, 203, 265–286. [Google Scholar] [CrossRef][Green Version]
- De Pater, I.; Sromovsky, L.A.; Hammel, H.B.; Fry, P.M.; LeBeau, R.P.; Rages, K.; Showalter, M.; Matthews, K. Post-equinox observations of Uranus: Berg’s evolution, vertical structure, and track towards the equator. Icarus 2011, 215, 332–345. [Google Scholar] [CrossRef]
- Hammel, H.B.; de Pater, I.; Gibbard, S.G.; Lockwood, G.W.; Rages, K. New cloud activity on Uranus in 2004: First detection of a southern feature at 2.2 μm. Icarus 2005, 175, 284–288. [Google Scholar] [CrossRef]
- de Pater, I.; Sromovsky, L.A.; Fry, P.M.; Hammel, H.B.; Baranec, C.; Sayanagi, K.M. Record-breaking storm activity on Uranus in 2014. Icarus 2015, 252, 121–128. [Google Scholar] [CrossRef]
- Sromovsky, L.A.; Hammel, H.B.; de Pater, I.; Fry, P.M.; Rages, K.A.; Showalter, M.R.; Merline, W.J.; Tamblyn, P.; Neyman, C.; Margot, J.; et al. Episodic bright and dark spots on Uranus. Icarus 2012, 220, 6–22. [Google Scholar] [CrossRef]
- Karkoschka, E. Uranus’ Apparent Seasonal Variability in 25 HST Filters. Icarus 2001, 151, 84–92. [Google Scholar] [CrossRef]
- Hammel, H.B.; Pater, I.D.; Gibbard, S.; Lockwood, G.W.; Rages, K. Uranus in 2003: Zonal winds, banded structure, and discrete features. Icarus 2005, 175, 534–545. [Google Scholar] [CrossRef]
- de Pater, I.; Gibbard, S.G.; Macintosh, B.A.; Roe, H.G.; Gavel, D.T.; Max, C.E. Keck Adaptive Optics Images of Uranus and Its Rings. Icarus 2002, 160, 359–374. [Google Scholar] [CrossRef]
- de Pater, I.; Romani, P.N.; Atreya, S.K. Uranus deep atmosphere revealed. Icarus 1989, 82, 288–313. [Google Scholar] [CrossRef]
- Leconte, J.; Selsis, F.; Hersant, F.; Guillot, T. Condensation-inhibited convection in hydrogen-rich atmospheres. Stability against double-diffusive processes and thermal profiles for Jupiter, Saturn, Uranus, and Neptune. Astron. Astrophys. 2017, A98, 16. [Google Scholar]
- Friedson, A.J.; Gonzales, E.J. Inhibition of ordinary and diffusive convection in the water condensation zone of the ice giants and implications for their thermal evolution. Icarus 2017, 297, 160–178. [Google Scholar] [CrossRef]
- Li, C.; Ingersoll, A.P.; Oyafuso, F. Moist Adiabats with Multiple Condensing Species: A New Theory with Application to Giant-Planet Atmospheres. J. Atmos. Sci. 2018, 75, 1063–1072. [Google Scholar] [CrossRef]
- Lyot, B. L’aspect des planètes au Pic du Midi dans une lunette de 60 cm d’ouverture. L’Astronomie 1953, 67, 3. [Google Scholar]
- Dollfus, A. Visual and Photographic Studies of Planets at the Pic du Midi. In Planets and Satellites; Kuiper, G.P., Middlehurst, B.M., Eds.; The University of Chicago Press: Chicago, IL, USA, 1961; p. 534. [Google Scholar]
- Cruikshank, D.P. On the rotation period of Neptune. Astrophys. J. Lett. 1978, 220, L57–L59. [Google Scholar] [CrossRef]
- Slavsky, D.; Smith, H.J. The rotation period of Neptune. Astrophys. J. Lett. 1978, 226, L49–L52. [Google Scholar] [CrossRef]
- Belton, M.J.S.; Wallace, L.; Hayes, S.H.; Price, M.J. Neptune’s rotation period: A correction and a speculation on the difference between photometric and spectroscopic results. Icarus 1980, 42, 71–78. [Google Scholar] [CrossRef]
- Smith, B.A.; Reitsema, H.J.; Larson, S.M. Discrete Cloud Features on Neptune. Bull. Am. Astron. Soc. 1979, 11, 570. [Google Scholar]
- Smith, B.A. Ground-Based Observations of Uranus and Neptune Using CCD Instruments. Astron. Vestn. 1985, 19, 42–47. [Google Scholar]
- Hammel, H.B.; Buie, M.W. An atmospheric rotation period of Neptune determined from methane-band imaging. Icarus 1987, 72, 62–68. [Google Scholar] [CrossRef]
- Hammel, H.B. The Atmosphere of Neptune Studied with CCD Imaging at Methane-Band and Continuum Wavelengths. Publ. Astron. Soc. Pac. 1988, 100, 1586. [Google Scholar] [CrossRef]
- Hammel, H.B. Discrete cloud structure on Neptune. Icarus 1989, 80, 14–22. [Google Scholar] [CrossRef]
- Hammel, H.B. Neptune Cloud Structure at Visible Wavelengths. Science 1989, 244, 1165–1167. [Google Scholar] [CrossRef]
- Smith, B.A.; Soderblom, L.A.; Banfield, D.; Barnet, C.; Basilevksy, A.T.; Bollinger, K.; Boyce, J.M.; Brahic, A.; Briggs, G.A.; Brown, R.H.; et al. Voyager 2 at Neptune: Imaging Science Results. Science 1989, 246, 1422–1449. [Google Scholar] [CrossRef] [PubMed]
- Hammel, H.B.; Beebe, R.F.; de Jong, E.M.; Hansen, C.J.; Howell, C.D.; Ingersoll, A.P.; Johnson, T.V.; Limaye, S.S.; Magalhaes, J.A.; Pollack, J.B.; et al. Neptune’s Wind Speeds Obtained by Tracking Clouds in Voyager Images. Science 1989, 245, 1367–1369. [Google Scholar] [CrossRef] [PubMed]
- Sromovsky, L.A.; Limaye, S.S.; Fry, P.M. Dynamics of Neptune’s Major Cloud Features. Icarus 1993, 105, 110–141. [Google Scholar] [CrossRef]
- Gurnett, D.A.; Kurth, K.; Cairns, I.; Granroth, L. Whistlers in Neptune’s Magnetosphere: Evidence of Atmospheric Lightning. J. Geophys. Res. 1990, 95, 20967–20976. [Google Scholar] [CrossRef]
- Wong, M.H.; Tollefson, J.; Hsu, A.I.; Pater, I.D.; Simon, A.A.; Hueso, R.; Sánchez-Lavega, A.; Sromovsky, L.; Fry, P.; Luszcz-Cook, S.; et al. A New Dark Vortex on Neptune. Astron. J. 2018, 155, 117. [Google Scholar] [CrossRef]
- Molter, E.; de Pater, I.; Luszcz-Cook, S.; Hueso, R.; Tollefson, J.; Alvarez, C.; Sánchez-Lavega, A.; Wong, M.H.; Hsu, A.I.; Sromovsky, L.A.; et al. Analysis of Neptune’s 2017 bright equatorial storm. Icarus 2019, 321, 324–345. [Google Scholar] [CrossRef]
- Karkoschka, E. Neptune’s cloud and haze variations 1994-2008 from 500 HST-WFPC2 images. Icarus 2011, 215, 759–773. [Google Scholar] [CrossRef]
- Irwin, P.G.; Fletcher, L.N.; Tice, D.; Owen, S.J.; Orton, G.S.; Teanby, N.A.; Davis, G.R. Time variability of Neptune’s horizontal and vertical cloud structure revealed by VLT/SINFONI and Gemini/NIFS from 2009 to 2013. Icarus 2016, 271, 418–437. [Google Scholar] [CrossRef]
- Hueso, R.; Pater, I.D.; Simon, A.; Sánchez-lavega, A.; Delcroix, M.; Wong, M.H.; Tollefson, J.W.; Baranec, C.; Kleer, K.D.; Luszcz-Cook, S.H.; et al. Neptune long-lived atmospheric features in 2013–2015 from small ( 28-cm ) to large ( 10-m ) telescopes. Icarus 2017, 295, 89–109. [Google Scholar] [CrossRef]
- Roman, M.T.; Fletcher, L.N.; Orton, G.S.; Greathouse, T.K.; Moses, J.I.; Rowe-Gurney, N.; Irwin, P.G.J.; Antuñano, A.; Sinclair, J.; Kasaba, Y.; et al. Subseasonal Variation in Neptune’s Mid-infrared Emission. Planet. Sci. J. 2022, 3, 78. [Google Scholar] [CrossRef]
- NASA. New Webb Image Captures Clearest View of Neptune’s Rings in Decades. Available online: https://www.nasa.gov/feature/goddard/2022/new-webb-image-captures-clearest-view-of-neptune-s-rings-in-decades (accessed on 2 November 2022).
- Hong, S.Y.; Dudhia, J.; Chen, S.H. A revised approach to ice microphysical processes for the bulk parameterization of clouds and precipitation. Mon. Weather. Rev. 2004, 132, 103. [Google Scholar] [CrossRef]
- Pruppacher, H.; Klett, J. Hydrodynamics of single cloud and precipitation particles. In Microphysics of Clouds and Precipitation; Springer: Dordrecht, The Netherlands, 2010; pp. 361–446. [Google Scholar] [CrossRef]
- Cunningham, E. On the velocity of steady fall of spherical particles through fluid medium. Proc. R. Soc. Lond. Ser. A 1910, 83, 357–365. [Google Scholar] [CrossRef]
- Loftus, K.; Wordsworth, R.D. The Physics of Falling Raindrops in Diverse Planetary Atmospheres. J. Geophys. Res. Planets 2021, 126, e2020JE006653. [Google Scholar] [CrossRef]
- Guillot, T.; Stevenson, D.J.; Atreya, S.K.; Bolton, S.J.; Becker, H.N. Storms and the depletion of ammonia in Jupiter: I. Microphysics of “Mushballs”. J. Geophys. Res. (Planets) 2020, 125, e06403. [Google Scholar] [CrossRef]
- Guillot, T.; Li, C.; Bolton, S.J.; Brown, S.T.; Ingersoll, A.P.; Janssen, M.A.; Levin, S.M.; Lunine, J.I.; Orton, G.S.; Steffes, P.G.; et al. Storms and the depletion of ammonia in Jupiter: II. Explaining the Juno observations. J. Geophys. Res. (Planets) 2020, 125, e06404. [Google Scholar] [CrossRef]
- Rossow, W.B. Cloud Microphysics: Analysis of the Clouds of Earth, Venus, Mars, and Jupiter. Icarus 1978, 36, 1–50. [Google Scholar] [CrossRef]
- Carlson, B.E.; Rossow, W.B.; Orton, G.S. Cloud microphysics of the giant planets. J. Atmos. Sci. 1988, 45, 2066–2081. [Google Scholar] [CrossRef]
- Ackerman, A.S.; Marley, M.S. Precipitating Condensation Clouds in Substellar Atmospheres. Astrophys. J. 2001, 556, 872–884. [Google Scholar] [CrossRef]
- Ohno, K.; Okuzumi, S. Microphysical Modeling of Mineral Clouds in GJ1214 b and GJ436 b: Predicting Upper Limits on the Cloud-top Height. Astrophys. J. 2018, 859, 34. [Google Scholar] [CrossRef]
- Barth, E. PlanetCARMA: A New Framework for Studying the Microphysics of Planetary Atmospheres. Atmosphere 2020, 11, 1064. [Google Scholar] [CrossRef]
- Emanuel, K.A. Atmospheric Convection; Oxford University Press: New York, NY, USA, 1994. [Google Scholar] [CrossRef]
- Bluestein, H.B. Ordinary-cell convective storms. In Severe Convective Storms and Tornadoes: Observations and Dynamics; Springer: Berlin/Heidelberg, Germany, 2013; pp. 95–164. [Google Scholar] [CrossRef]
- Raymond, D.J.; Raga, G.B.; Bretherton, C.S.; Molinari, J.; López-Carrillo, C.; Željka, F. Convective Forcing in the Intertropical Convergence Zone of the Eastern Pacific. J. Atmos. Sci. 2003, 60, 2064–2082. [Google Scholar] [CrossRef]
- Weisman, M.L.; Klemp, J.B. Characteristics of Isolated Convective Storms. In Mesoscale Meteorology and Forecasting; Ray, P.S., Ed.; American Meteorological Society: Boston, MA, USA, 1986; pp. 331–358. [Google Scholar] [CrossRef]
- Guillot, T. Condensation of methane, ammonia, and water and the inhibition of convection in Giant Planets. Science 1995, 269, 1697–1699. [Google Scholar] [CrossRef] [PubMed]
- Gierasch, P.J.; Ingersoll, A.P.; Banfield, D.; Ewald, S.P.; Helfenstein, P.; Simon-Miller, A.; Vasavada, A.; Breneman, H.H.; Sensko, D.A. Observation of moist convection in Jupiter’s atmosphere. Nature 2000, 403, 628–630. [Google Scholar] [CrossRef] [PubMed]
- Palotai, C.; Dowling, T.E. Addition of water and ammonia cloud microphysics to the EPIC model. Icarus 2008, 194, 303–326. [Google Scholar] [CrossRef]
- Ingersoll, A.P.; Atreya, S.; Bolton, S.J.; Brueshaber, S.; Fletcher, L.N.; Levin, S.M.; Li, C.; Li, L.; Lunine, J.I.; Orton, G.S.; et al. Jupiter’s Overturning Circulation: Breaking Waves Take the Place of Solid Boundaries. Geophys. Res. Lett. 2021, 48, e95756. [Google Scholar] [CrossRef]
- Jensen, E.J.; Toon, O.B.; Selkirk, H.B.; Spinhirne, J.D.; Schoeberl, M.R. On the formation and persistence of subvisible cirrus clouds near the tropical tropopause. J. Geophys. Res. Atmos. 1996, 101, 21361–21375. [Google Scholar] [CrossRef]
- Rosenfeld, D.; Lohmann, U.; Raga, G.B.; O’Dowd, C.D.; Kulmala, M.; Fuzzi, S.; Reissell, A.; Andreae, M.O. Flood or Drought: How Do Aerosols Affect Precipitation? Science 2008, 321, 1309–1313. [Google Scholar] [CrossRef]
- WMO. International Cloud Atlas Volume II, rev. ed.; Secretariat of the World Meteorological Organization Geneva: Geneva, Switzerland, 1987. [Google Scholar]
- Maddox, R.A. Mesoscale convective complexes (USA). Bull. Am. Meteorol. Soc. 1980, 61, 1374–1387. [Google Scholar] [CrossRef]
- Houze, R.A. Chapter 9—Mesoscale Convective Systems. In Cloud Dynamics; Houze, R.A., Ed.; Academic Press: Cambridge, MA, USA, 2014; Volume 104, pp. 237–286. [Google Scholar] [CrossRef]
- Sugiyama, K.; Nakajima, K.; Odaka, M.; Kuramoto, K.; Hayashi, Y.Y. Numerical simulations of Jupiter’s moist convection layer: Structure and dynamics in statistically steady states. Icarus 2014, 229, 71–91. [Google Scholar] [CrossRef]
- Sugiyama, K.; Nakajima, K.; Odaka, M.; Ishiwatari, M.; Kuramoto, K.; Morikawa, Y.; Nishizawa, S.; Takahashi, Y.O.; Hayashi, Y.Y. Intermittent cumulonimbus activity breaking the three-layer cloud structure of Jupiter. Geophys. Res. Lett. 2011, 38, 2–6. [Google Scholar] [CrossRef]
- Hueso, R.; Sánchez-Lavega, A. A three dimensional model of moist convection for the Giant Planets: The Jupiter case. Icarus 2001, 151, 257–274. [Google Scholar] [CrossRef]
- Fletcher, L.N.; de Pater, I.; Orton, G.S.; Hofstadter, M.D.; Irwin, P.G.J.; Roman, M.T.; Toledo, D. Ice Giant Circulation Patterns: Implications for Atmospheric Probes. Space Sci. Rev. 2020, 216, 21. [Google Scholar] [CrossRef]
- Vallis, G.K. Atmospheric and Oceanic Fluid Dynamics; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Hueso, R.; Sánchez-Lavega, A.; Guillot, T. A model for large-scale convective storms in Jupiter. J. Geophys. Res. Planets 2002, 107, 5. [Google Scholar] [CrossRef]
- Young, R.M.; Read, P.L. Forward and inverse kinetic energy cascades in Jupiter’s turbulent weather layer. Nat. Phys. 2017, 13, 1135–1140. [Google Scholar] [CrossRef]
- Rhines, P.B. Waves and turbulence on a beta-plane. J. Fluid Mech. 1975, 69, 417–443. [Google Scholar] [CrossRef]
- Cho, J.Y.; Polvani, L.M. The emergence of jets and vortices in freely evolving, shallow-water turbulence on a sphere. Phys. Fluids 1996, 8, 1531–1552. [Google Scholar] [CrossRef]
- Okuno, A.; Masuda, A. Effect of horizontal divergence on the geostrophic turbulence on a beta-plane: Suppression of the Rhines effect. Phys. Fluids 2003, 15, 56–65. [Google Scholar] [CrossRef]
- Arbic, B.K.; Müller, M.; Richman, J.G.; Shriver, J.F.; Morten, A.J.; Scott, R.B.; Sérazin, G.; Penduff, T. Geostrophic Turbulence in the Frequency-Wavenumber Domain: Eddy-Driven Low-Frequency Variability. J. Phys. Oceanogr. 2014, 44, 2050–2069. [Google Scholar] [CrossRef]
- Richardson, L.F. Weather prediction by numerical process. By Lewis F. Richardson. Cambridge (University Press), 1922. 4°. Pp. xii + 236. 30s.net. Q. J. R. Meteorol. Soc. 1922, 48, 282–284. [Google Scholar] [CrossRef]
- Merilees, P.E.; Warn, H. On energy and enstrophy exchanges in two-dimensional non-divergent flow. J. Fluid Mech. 1975, 69, 625–630. [Google Scholar] [CrossRef]
- Tung, K.K.; Orlando, W.W. The k-3 and k-5/3 Energy Spectrum of Atmospheric Turbulence: Quasigeostrophic Two-Level Model Simulation. J. Atmos. Sci. 2003, 60, 824–835. [Google Scholar] [CrossRef]
- Charney, J.G. Geostrophic Turbulence. J. Atmos. Sci. 1971, 28, 1087–1095. [Google Scholar] [CrossRef]
- Lindborg, E. Can the atmospheric kinetic energy spectrum be explained by two-dimensional turbulence? J. Fluid Mech. 1999, 388, 259–288. [Google Scholar] [CrossRef]
- Blumen, W. Uniform Potential Vorticity Flow: Part I. Theory of Wave Interactions and Two-Dimensional Turbulence. J. Atmos. Sci. 1978, 35, 774–783. [Google Scholar] [CrossRef]
- Tulloch, R.; Smith, K.S. From the Cover: A theory for the atmospheric energy spectrum: Depth-limited temperature anomalies at the tropopause. Proc. Natl. Acad. Sci. USA 2006, 103, 14690–14694. [Google Scholar] [CrossRef]
- Lapeyre, G. Surface Quasi-Geostrophy. Fluidika 2017, 2, 7. [Google Scholar] [CrossRef]
- Vallis, G.K.; Maltrud, M.E. Generation of Mean Flows and Jets on a Beta Plane and over Topography. J. Phys. Oceanogr. 1993, 23, 1346–1362. [Google Scholar] [CrossRef]
- Huang, H.P.; Galperin, B.; Sukoriansky, S. Anisotropic spectra in two-dimensional turbulence on the surface of a rotating sphere. Phys. Fluids 2001, 13, 225–240. [Google Scholar] [CrossRef]
- Sukoriansky, S.; Dikovskaya, N.; Galperin, B. On the arrest of inverse energy cascade and the rhines scale. J. Atmos. Sci. 2006, 64, 3312–3327. [Google Scholar] [CrossRef]
- Showman, A.P. Numerical simulations of forced shallow-water turbulence: Effects of moist convection on the large-scale circulation of Jupiter and Saturn. J. Atmos. Sci. 2007, 64, 3132. [Google Scholar] [CrossRef]
- Read, P.L.; Yamazaki, Y.H.; Lewis, S.R.; Williams, P.D.; Wordsworth, R.; Miki-Yamazaki, K.; Sommeria, J.; Didelle, H. Dynamics of convectively driven banded jets in the laboratory. J. Atmos. Sci. 2007, 64, 4031–4052. [Google Scholar] [CrossRef]
- Read, P.L.; Jacoby, T.N.L.; Rogberg, P.H.T.; Wordsworth, R.D.; Yamazaki, Y.H.; Miki-Yamazaki, K.; Young, R.M.B.; Sommeria, J.; Didelle, H.; Viboud, S. An experimental study of multiple zonal jet formation in rotating, thermally driven convective flows on a topographic beta-plane. Phys. Fluids 2015, 27, 085111. [Google Scholar] [CrossRef]
- Read, P.L.; Young, R.M.B.; Kennedy, D. The turbulent dynamics of Jupiter’s and Saturn’s weather layers: Order out of chaos? Geosci. Lett. 2020, 7, 10. [Google Scholar] [CrossRef]
- Liu, J.; Schneider, T. Formation Mechanisms of Jets And Superrotation on Giant Planets; American Astronomical Society: Washington, DC, USA, 2009; Volume 41. [Google Scholar]
- Cosentino, R.G.; Butler, B.; Sault, B.; Morales-Juberías, R.; Simon, A.; de Pater, I. Atmospheric waves and dynamics beneath Jupiter’s clouds from radio wavelength observations. Icarus 2017, 292, 168–181. [Google Scholar] [CrossRef][Green Version]
- Galperin, B.; Sukoriansky, S.; Dikovskaya, N.; Read, P.L.; Yamazaki, Y.H.; Wordsworth, R. Anisotropic turbulence and zonal jets in rotating flows with a β-effect. Nonlinear Process. Geophys. 2006, 13, 83–98. [Google Scholar] [CrossRef]
- Brethouwer, G.; Lindborg, E. Passive scalars in stratified turbulence. Geophys. Res. Lett. 2008, 35, L06809. [Google Scholar] [CrossRef]
- Cosentino, R.G.; Simon, A.; Morales-Juberías, R. Jupiter’s Turbulent Power Spectra From Hubble Space Telescope. J. Geophys. Res. Planets 2019, 124, 1204–1225. [Google Scholar] [CrossRef]
- Mitchell, J. The Nature of Large-Scale Turbulence in the Jovian Atmosphere. Ph.D. Thesis, Jet Propulsion Laboratory, California Institute of Technology, Pasadena, CA, USA, 1982. [Google Scholar]
- Mitchell, J.L.; Maxworthy, T. Large-scale turbulence in the Jovian atmosphere. In Turbulence and Chaotic Phenomena in Fluids; North-Holland: Amsterdam, The Netherlands, 1984; pp. 543–547. [Google Scholar]
- Galperin, B.; Sukoriansky, S.; Huang, H.P. Universal n-5 spectrum of zonal flows on giant planets. Phys. Fluids 2001, 13, 1545–1548. [Google Scholar] [CrossRef]
- Salyk, C.; Ingersoll, A.P.; Lorre, J.; Vasavada, A.; Del Genio, A.D. Interaction between eddies and mean flow in Jupiter’s atmosphere: Analysis of Cassini imaging data. Icarus 2006, 185, 430–442. [Google Scholar] [CrossRef]
- Beebe, R.F.; Ingersoll, A.P.; Hunt, G.E.; Mitchell, J.L.; Muller, J.P. Measurements of wind vectors, eddy momentum transports, and energy conversions in Jupiter’s atmosphere from Voyager 1 images. Geophys. Res. Lett. 1980, 7, 1–4. [Google Scholar] [CrossRef]
- Choi, D.S.; Showman, A.P. Power spectral analysis of Jupiter’s clouds and kinetic energy from Cassini. Icarus 2011, 216, 597–609. [Google Scholar] [CrossRef]
- Harrington, J.; Dowling, T.E.; Baron, R.L. Jupiter’s tropospheric thermal emission: II. Power spectrum analysis and wave search. Icarus 1996, 124, 32–44. [Google Scholar] [CrossRef]
- Barrado-Izagirre, N.; Pérez-Hoyos, S.; Sánchez-Lavega, A. Brightness power spectral distribution and waves in Jupiter’s upper cloud and hazes. Icarus 2009, 202, 181. [Google Scholar] [CrossRef]
- Cosentino, R.G.; Morales-Juberías, R.; Greathouse, T.; Orton, G.; Johnson, P.; Fletcher, L.N.; Simon, A. New Observations and Modeling of Jupiter’s Quasi-Quadrennial Oscillation. J. Geophys. Res. Planets 2017, 122, 2719–2744. [Google Scholar] [CrossRef]
- Galperin, B.; Read, P. Zonal Jets: Phenomenology, Genesis, and Physics; Cambridge University Press: Cambridge, MA, USA, 2019. [Google Scholar] [CrossRef]
- Jacobson, M.Z. Fundamentals of Atmospheric Modeling, 2nd ed.; Cambridge University Press: Cambridge, MA, USA, 2005. [Google Scholar] [CrossRef]
- Durran, D.R. Numerical Methods for Fluid Dynamics—With Applications to Geophysics; Springer: New York, NY, USA, 2010. [Google Scholar] [CrossRef]
- Warner, T.T. Numerical Weather and Climate Prediction; Cambridge University Press: Cambridge, MA, USA, 2010. [Google Scholar] [CrossRef]
- Lauritzen, P.; Jablonowski, C.; Taylor, M.; Nair, R. Numerical Techniques for Global Atmospheric Models; Lecture Notes in Computational Science and Engineering; Springer: Berlin, Germany, 2011. [Google Scholar]
- DeCaria, A.; Van Knowe, G. A First Course in Atmospheric Numerical Modeling; Sundog Publishing: Madison, WI, USA, 2014. [Google Scholar]
- Young, R.M.B.; Read, P.L.; Wang, Y. Simulating Jupiter’s weather layer. Part I: Jet spin-up in a dry atmosphere. Icarus 2019, 326, 225–252. [Google Scholar] [CrossRef]
- Young, R.M.B.; Read, P.L.; Wang, Y. Simulating Jupiter’s weather layer. Part II: Passive ammonia and water cycles. Icarus 2019, 326, 253–268. [Google Scholar] [CrossRef]
- Spiga, A.; Guerlet, S.; Millour, E.; Indurain, M.; Meurdesoif, Y.; Cabanes, S.; Dubos, T.; Leconte, J.; Boissinot, A.; Lebonnois, S.; et al. Global climate modeling of Saturn’s atmosphere. Part II: Multi-annual high-resolution dynamical simulations. Icarus 2020, 335, 113377. [Google Scholar] [CrossRef]
- Lian, Y.; Richardson, M.I. Unstructured Grid Dynamical Modeling of Planetary Atmospheres using planetMPAS: The Influence of the Rigid Lid, Computational Efficiency, and Examples of Martian and Jovian Application. arXiv 2022, arXiv:2210.17430. [Google Scholar]
- Yoden, S.; Yamada, M. A Numerical Experiment on Two-Dimensional Decaying Turbulence on a Rotating Sphere. J. Atmos. Sci. 1993, 50, 631–644. [Google Scholar] [CrossRef]
- Kitamura, Y.; Matsuda, Y. Numerical experiments of two-level decaying turbulence on a rotating sphere. Fluid Dyn. Res. 2004, 34, 33–57. [Google Scholar] [CrossRef]
- Dritschel, D.G.; McIntyre, M.E. Multiple jets as PV staircases: The phillips effect and the resilience of eddy-transport barriers. J. Atmos. Sci. 2008, 65, 855–874. [Google Scholar] [CrossRef]
- Sayanagi, K.M.; Showman, A.P.; Dowling, T.E. The Emergence of Multiple Robust Zonal Jets from Freely Evolving, Three-Dimensional Stratified Geostrophic Turbulence with Applications to Jupiter. J. Atmos. Sci. 2008, 65, 3947–3962. [Google Scholar] [CrossRef]
- Theiss, J. Equatorward energy cascade, critical latitude, and the predominance of cyclonic vortices in geostraphic turbulence. J. Phys. Oceanogr. 2004, 34, 1663–1678. [Google Scholar] [CrossRef]
- Li, L.; Ingersoll, A.P.; Huang, X. Interaction of moist convection with zonal jets on Jupiter and Saturn. Icarus 2006, 180, 113–123. [Google Scholar] [CrossRef]
- Scott, R.K.; Polvani, L.M. Forced-dissipative shallow-water turbulence on the sphere and the atmospheric circulation of the giant planets. J. Atmos. Sci. 2007, 64, 3158–3176. [Google Scholar] [CrossRef]
- Cosentino, R.G.; Simon, A.; Morales-Juberias, R.; Sayanagi, K.M. Observations and Numerical Modeling of the Jovian Ribbon. Astrophys. J. Lett. 2015, 810, L10. [Google Scholar] [CrossRef]
- Liu, J.; Schneider, T. Mechanisms of Jet Formation on the Giant Planets. J. Atmos. Sci. 2010, 67, 3652–3672. [Google Scholar] [CrossRef]
- Liu, J.; Schneider, T. Convective Generation of Equatorial Superrotation in Planetary Atmospheres. J. Atmos. Sci. 2011, 68, 2742–2756. [Google Scholar] [CrossRef]
- Lian, Y.; Showman, A.P. Generation of equatorial jets by large-scale latent heating on the giant planets. Icarus 2010, 207, 373–393. [Google Scholar] [CrossRef][Green Version]
- Lian, Y.; Showman, A.P. Deep jets on gas-giant planets. Icarus 2008, 194, 597–615. [Google Scholar] [CrossRef]
- Mac Low, M.M.; Ingersoll, A.P. Merging of vortices in the atmosphere of Jupiter: An analysis of Voyager images. Icarus 1986, 65, 353–369. [Google Scholar] [CrossRef]
- Rogers, J.H. The Giant Planet Jupiter; Cambridge University Press: New York, NY, USA, 1995. [Google Scholar] [CrossRef]
- Janssen, M.A.; Oswald, J.E.; Brown, S.T.; Gulkis, S.; Levin, S.M.; Bolton, S.J.; Allison, M.D.; Atreya, S.K.; Gautier, D.; Ingersoll, A.P.; et al. MWR: Microwave Radiometer for the Juno Mission to Jupiter. Space Sci. Rev. 2017, 213, 139–185. [Google Scholar] [CrossRef]
- de Pater, I.; Sault, R.J.; Moeckel, C.; Moullet, A.; Wong, M.H.; Goullaud, C.; DeBoer, D.; Butler, B.J.; Bjoraker, G.; Ádámkovics, M.; et al. First ALMA Millimeter-wavelength Maps of Jupiter, with a Multiwavelength Study of Convection. Astron. J. 2019, 158, 139. [Google Scholar] [CrossRef]
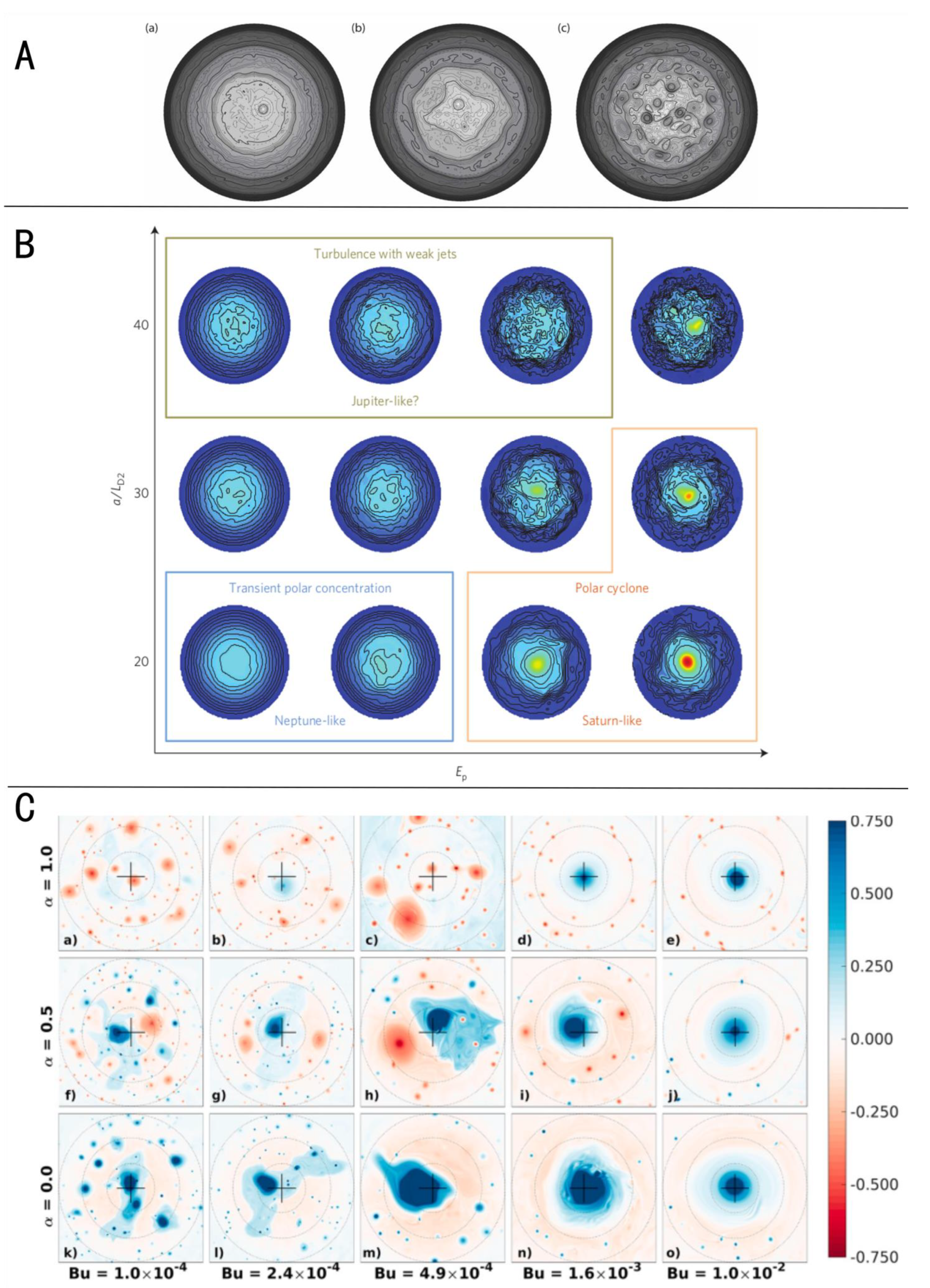
- Brueshaber, S.R.; Sayanagi, K.M.; Dowling, T.E. Dynamical regimes of giant planet polar vortices. Icarus 2019, 323, 46–61. [Google Scholar] [CrossRef]
- Moses, J.I.; Armstrong, E.S.; Fletcher, L.N.; Friedson, A.J.; Irwin, P.G.; Sinclair, J.A.; Hesman, B.E. Evolution of stratospheric chemistry in the Saturn storm beacon region. Icarus 2015, 261, 149–168. [Google Scholar] [CrossRef] [PubMed]
- Sromovsky, L.A.; Fry, P.M. The source of widespread 3-μm absorption in Jupiter’s clouds: Constraints from 2000 Cassini VIMS observations. Icarus 2010, 210, 230–257. [Google Scholar] [CrossRef]
- Smith, K.S. A local model for planetary atmospheres forced by small-scale convection. J. Atmos. Sci. 2004, 61, 1420–1433. [Google Scholar] [CrossRef]
- Smith, L.M.; Waleffe, F. Transfer of energy to two-dimensional large scales in forced, rotating three-dimensional turbulence. Phys. Fluids 1999, 11, 1608–1622. [Google Scholar] [CrossRef]
- Orlanski, I. A rational subdivision of scales for atmospheric processes. Bull. Am. Meteorol. Soc. 1975, 56, 527–530. [Google Scholar]
- Nastrom, G.D.; Gage, K.S. A Climatology of Atmospheric Wavenumber Spectra of Wind and Temperature Observed by Commercial Aircraft. J. Atmos. Sci. 1985, 42, 950–960. [Google Scholar] [CrossRef]
- Rubio, A.; Julien, K.; Knobloch, E.; Weiss, J. Upscale Energy Transfer in Three-Dimensional Rapidly Rotating Turbulent Convection. Phys. Rev. Lett. 2014, 112, 144501. [Google Scholar] [CrossRef] [PubMed]
- Adriani, A.; Bracco, A.; Grassi, D.; Moriconi, M.L.; Mura, A.; Orton, G.; Altieri, F.; Ingersoll, A.; Atreya, S.K.; Lunine, J.I.; et al. Two-Year Observations of the Jupiter Polar Regions by JIRAM on Board Juno. J. Geophys. Res. Planets 2020, 125, e2019JE006098. [Google Scholar] [CrossRef]
- Moriconi, M.L.; Migliorini, A.; Altieri, F.; Adriani, A.; Mura, A.; Orton, G.; Lunine, J.I.; Grassi, D.; Atreya, S.K.; Ingersoll, A.P.; et al. Turbulence Power Spectra in Regions Surrounding Jupiter’s South Polar Cyclones From Juno/JIRAM. J. Geophys. Res. Planets 2020, 125, e2019JE006096. [Google Scholar] [CrossRef]
- Ingersoll, A.P.; Ewald, S.P.; Tosi, F.; Adriani, A.; Mura, A.; Grassi, D.; Plainaki, C.; Sindoni, G.; Li, C.; Siegelman, L.; et al. Vorticity and divergence at scales down to 200 km within and around the polar cyclones of Jupiter. Nat. Astron. 2022, 6, 1280–1286. [Google Scholar] [CrossRef]
- Siegelman, L.; Klein, P.; Ingersoll, A.P.; Ewald, S.P.; Young, W.R.; Bracco, A.; Mura, A.; Adriani, A.; Grassi, D.; Plainaki, C.; et al. Moist convection drives an upscale energy transfer at Jovian high latitudes. Nat. Phys. 2022, 18, 357–361. [Google Scholar] [CrossRef]
- Adriani, A.; Filacchione, G.; Di Iorio, T.; Turrini, D.; Noschese, R.; Cicchetti, A.; Grassi, D.; Mura, A.; Sindoni, G.; Zambelli, M.; et al. JIRAM, the Jovian Infrared Auroral Mapper. Space Sci. Rev. 2017, 213, 393–446. [Google Scholar] [CrossRef]
- Juckes, M. Quasigeostrophic Dynamics of the Tropopause. J. Atmos. Sci. 1994, 51, 2756–2768. [Google Scholar] [CrossRef]
- Adem, J. A Series Solution for the Barotropic Vorticity Equation and its Application in the Study of Atmospheric Vortices. Tellus 1956, 8, 364–372. [Google Scholar] [CrossRef]
- Smith, R.B. A hurricane beta-drift law. J. Atmos. Sci. 1993, 50, 3213–3215. [Google Scholar] [CrossRef]
- Fiorino, M.; Elsberry, R.L. Some aspects of vortex structure related to tropical cyclone motion. J. Atmos. Sci. 1989, 46, 975–990. [Google Scholar] [CrossRef]
- Richardson, P.L.; Price, J.F.; Walsh, D.; Armi, L.; Schröder, M. Tracking Three Meddies with SOFAR Floats. J. Phys. Oceanogr. 1989, 19, 371–383. [Google Scholar] [CrossRef]
- Lebeau, R.P.; Dowling, T.E. EPIC Simulations of Time-Dependent, Three-Dimensional Vortices with Application to Neptune’s Great Dark Spot. Icarus 1998, 265, 239–265. [Google Scholar] [CrossRef]
- Dowling, T.E.; Fischer, A.S.; Gierasch, P.J.; Harrington, J.; Lebeau, R.P.; Santori, C.M. The Explicit Planetary Isentropic-Coordinate (EPIC) Atmospheric Model. Icarus 1998, 132, 221–238. [Google Scholar] [CrossRef]
- Scott, R.K. Polar accumulation of cyclonic vorticity. Geophys. Astrophys. Fluid Dyn. 2011, 105, 409–420. [Google Scholar] [CrossRef]
- O’Neill, M.E.; Emanuel, K.A.; Flierl, G.R. Polar vortex formation in giant-planet atmospheres due to moist convection. Nat. Geosci. 2015, 8, 523–526. [Google Scholar] [CrossRef]
- O’Neill, M.E.; Emanuel, K.A.; Flierl, G.R. Weak Jets and strong cyclones: Shallow-water modeling of giant planet polar caps. J. Atmos. Sci. 2016, 73, 1841–1855. [Google Scholar] [CrossRef]
- Hogg, N.; Stommel, H. The heton, an elementary interaction between discrete baroclinic geostrophic vortices, and its implications concerning eddy heat-flow. Proc. R. Soc. Lond. A Math. Phys. Sci. 1985, 397, 1–20. [Google Scholar] [CrossRef]
- Montgomery, M.T.; Nicholls, M.E.; Cram, T.A.; Saunders, A.B. A vortical hot tower route to tropical cyclogenesis. J. Atmos. Sci. 2006, 63, 355–386. [Google Scholar] [CrossRef]
- Brueshaber, S.R.; Sayanagi, K.M. Effects of forcing scale and intensity on the emergence and maintenance of polar vortices on Saturn and Ice Giants. Icarus 2021, 361, 114386. [Google Scholar] [CrossRef]
- O’Neill, M.E.; Kaspi, Y. Slantwise convection on fluid planets. Geophys. Res. Lett. 2016, 43, 10611–10620. [Google Scholar] [CrossRef]
- Fine, K.S.; Cass, A.C.; Flynn, W.G.; Driscoll, C.F. Relaxation of 2D Turbulence to Vortex Crystals. Phys. Rev. Lett. 1995, 75, 3277–3280. [Google Scholar] [CrossRef] [PubMed]
- Cai, T.; Chan, K.L.; Mayr, H.G. Deep, Closely Packed, Long-lived Cyclones on Jupiter’s Poles. Planet. Sci. J. 2021, 2, 81. [Google Scholar] [CrossRef]
- Siegelman, L.; Ingersoll, A.; Young, W. Polar vortex crystals: Emergence and structure. Proc. Natl. Acad. Sci. USA 2022, 119, e2120486119. [Google Scholar] [CrossRef] [PubMed]
- Gavriel, N.; Kaspi, Y. The number and location of Jupiter’s circumpolar cyclones explained by vorticity dynamics. Nat. Geosci. 2021, 14, 559–563. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Palotai, C.; Brueshaber, S.; Sankar, R.; Sayanagi, K. Moist Convection in the Giant Planet Atmospheres. Remote Sens. 2023, 15, 219. https://doi.org/10.3390/rs15010219
Palotai C, Brueshaber S, Sankar R, Sayanagi K. Moist Convection in the Giant Planet Atmospheres. Remote Sensing. 2023; 15(1):219. https://doi.org/10.3390/rs15010219
Chicago/Turabian StylePalotai, Csaba, Shawn Brueshaber, Ramanakumar Sankar, and Kunio Sayanagi. 2023. "Moist Convection in the Giant Planet Atmospheres" Remote Sensing 15, no. 1: 219. https://doi.org/10.3390/rs15010219
APA StylePalotai, C., Brueshaber, S., Sankar, R., & Sayanagi, K. (2023). Moist Convection in the Giant Planet Atmospheres. Remote Sensing, 15(1), 219. https://doi.org/10.3390/rs15010219




