A New Approach for Improving GNSS Geodetic Position by Reducing Residual Tropospheric Error (RTE) Based on Surface Meteorological Data
Abstract
1. Introduction and Background
2. Methodology
- The presented methodology implies the creation of the most mutual and frequent alignment of positional and meteorological parameters, and thus the choice of used regional GNSS stations is conditioned (the availability of regional meteorological records is within a 10-min frequency).
- In accordance with the initial spatial limitation, the selection of available GNSS positional data measurements was limited to the GPS and GLONASS, and there was no possibility of using records from other GNSS systems. As a full-fledged part of the GNSS, GLONASS data were used due to their relative underrepresentation in similar research.
- The main goal of the research was to determine a possible statistically significant correlation between realistic surface meteorological parameters and the geodetic accuracy of GNSS position deviations. Therefore, the model was developed based on positional and meteorological data with a geographical resolution of 3.7° × 2.9° (geographical grid) which declared as of regional character.
- The format of the available GNSS data limited the possibilities of their processing. Therefore, single-frequency (L1) positioning and the Klobuchar model for ionospheric delay were used. Input clock parameters and ephemerides are contained in the navigation message (including the broadcast ephemerides and clock parameters). Processing of solid tides and multipath corrections was not accessible.
- Other more accurate positioning techniques, such as PPP (Precise Point Positioning) or RTK (Real-time Kinematics), were not supported.
2.1. Time Frame of the Study
2.2. Meteorological Data Collection
2.3. Geographic GNSS Data Collection
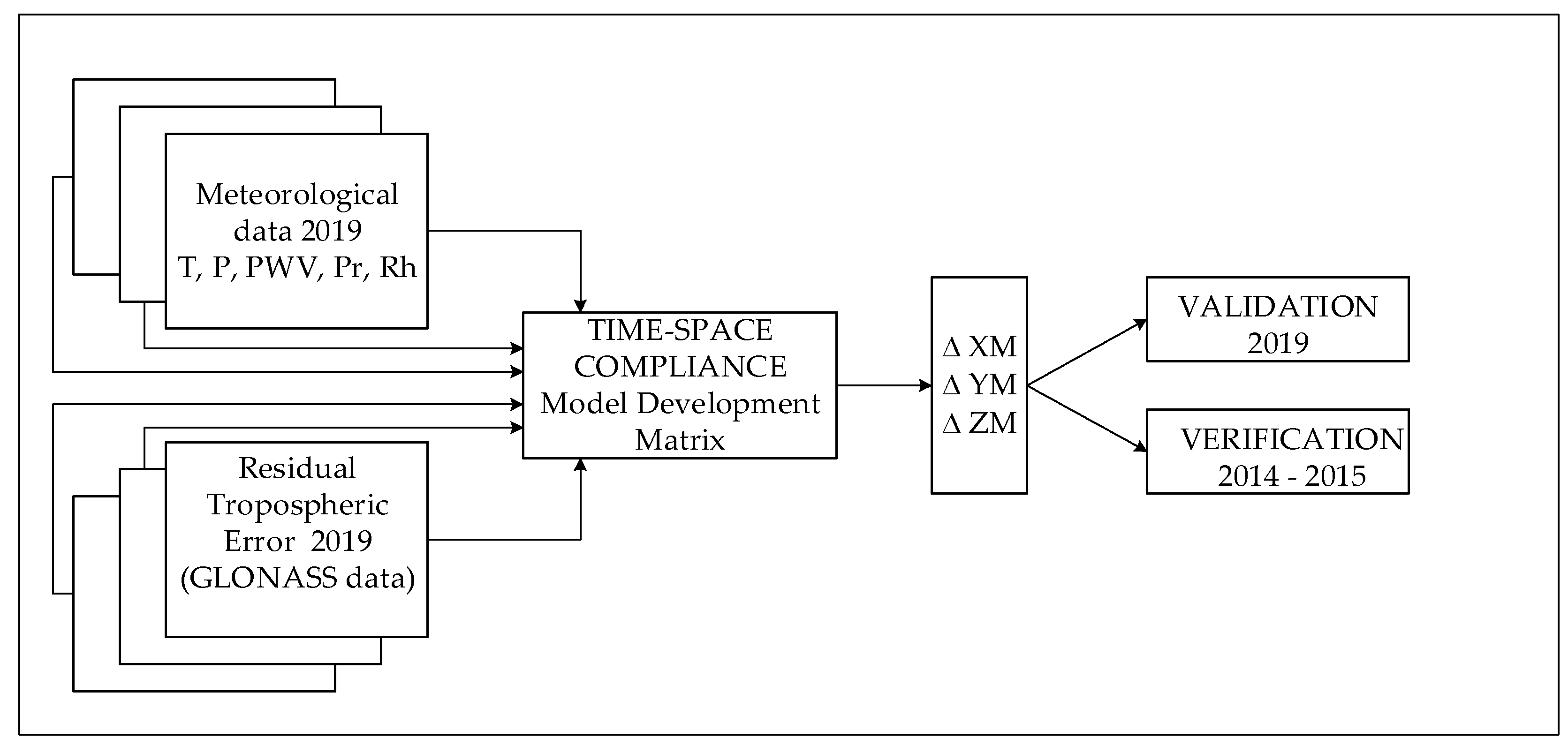
2.4. Model Development
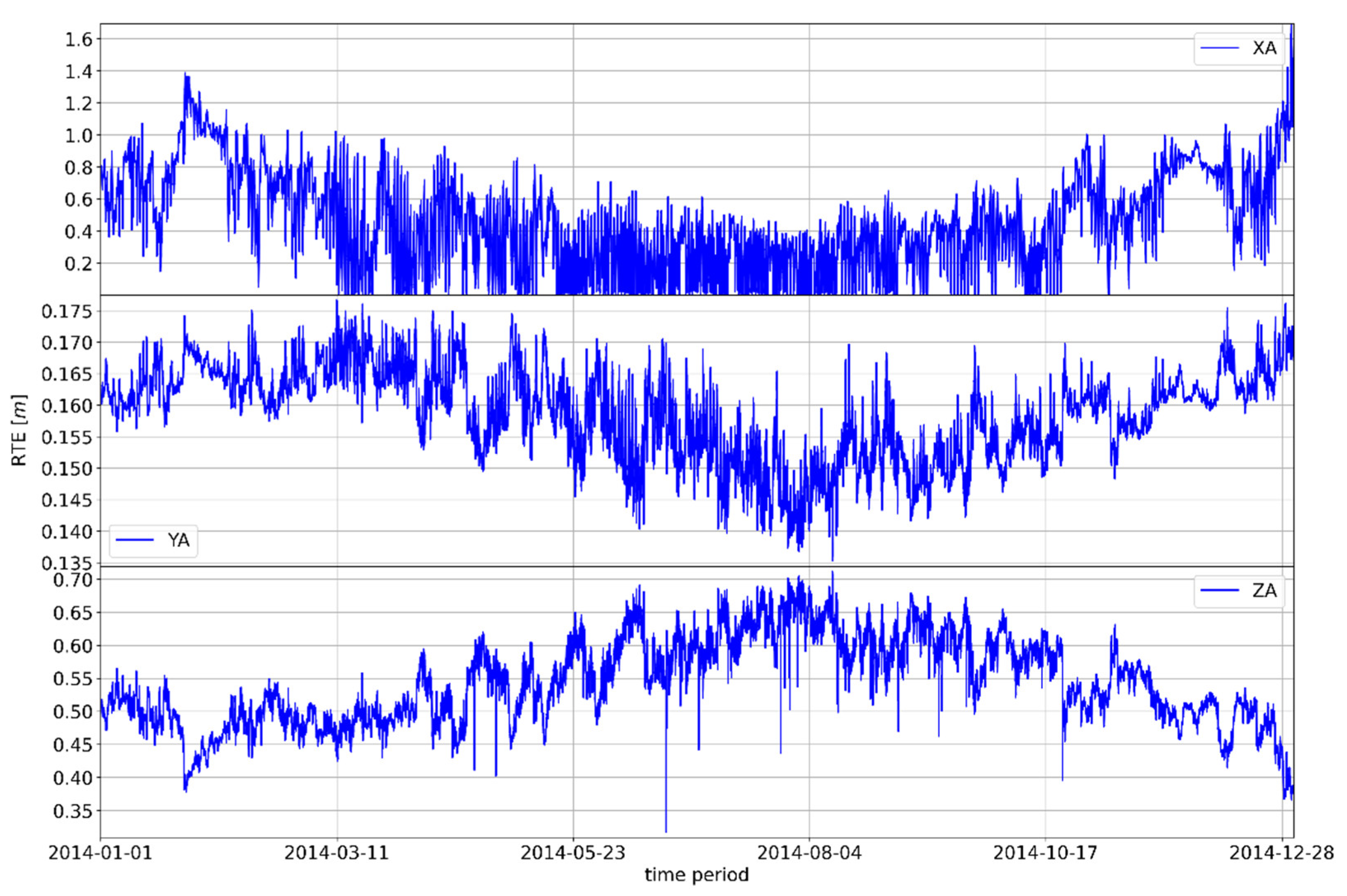
2.4.1. Determination of the Size of the Tropospheric Error
2.4.2. Statistical Analysis and Model Development
2.4.3. Validation of the Proposed Model
3. Results and Findings
3.1. Verification for Čakovec Location
3.2. Verification for Zadar Location
3.3. Verification for Dubrovnik Location
4. Discussion
4.1. Model Suitability for Application within the GPS
4.2. Periodic Effect of the Proposed Model on the Positioning Accuracy
4.3. Observations on the Specifics of the Conducted Research
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DHMZ | Croatian Meteorological and Hydrological Service |
| ECEF | Earth Centered, Earth Fixed |
| EKF | Extended Kalman Filter |
| EUREF | Regional Reference Frame Sub-Commission for Europe |
| GLONASS | Global Navigation Satellite System |
| GNSS | Global Navigation Satellite System |
| GPS | Global Positioning System |
| NMF | (New) Mapping Functions |
| NWM | Numerical Weather Models |
| PPP | Precise Point Positioning |
| PWV | Precipitable Water Vapor |
| RINEX | Receiver Independent Exchange |
| RMSE | Root Mean Square Error |
| RTE | Residual Tropospheric Error |
| RTK | Real-time Kinematics |
| SBAS | Satellite-based Augmentation Systems |
| STD | Standard Deviation |
| ZHD | Zenith Hydrostatic Delay |
| ZTD | Zenith Tropospheric Delay |
| ZWD | Zenith Wet Delay |
Appendix A



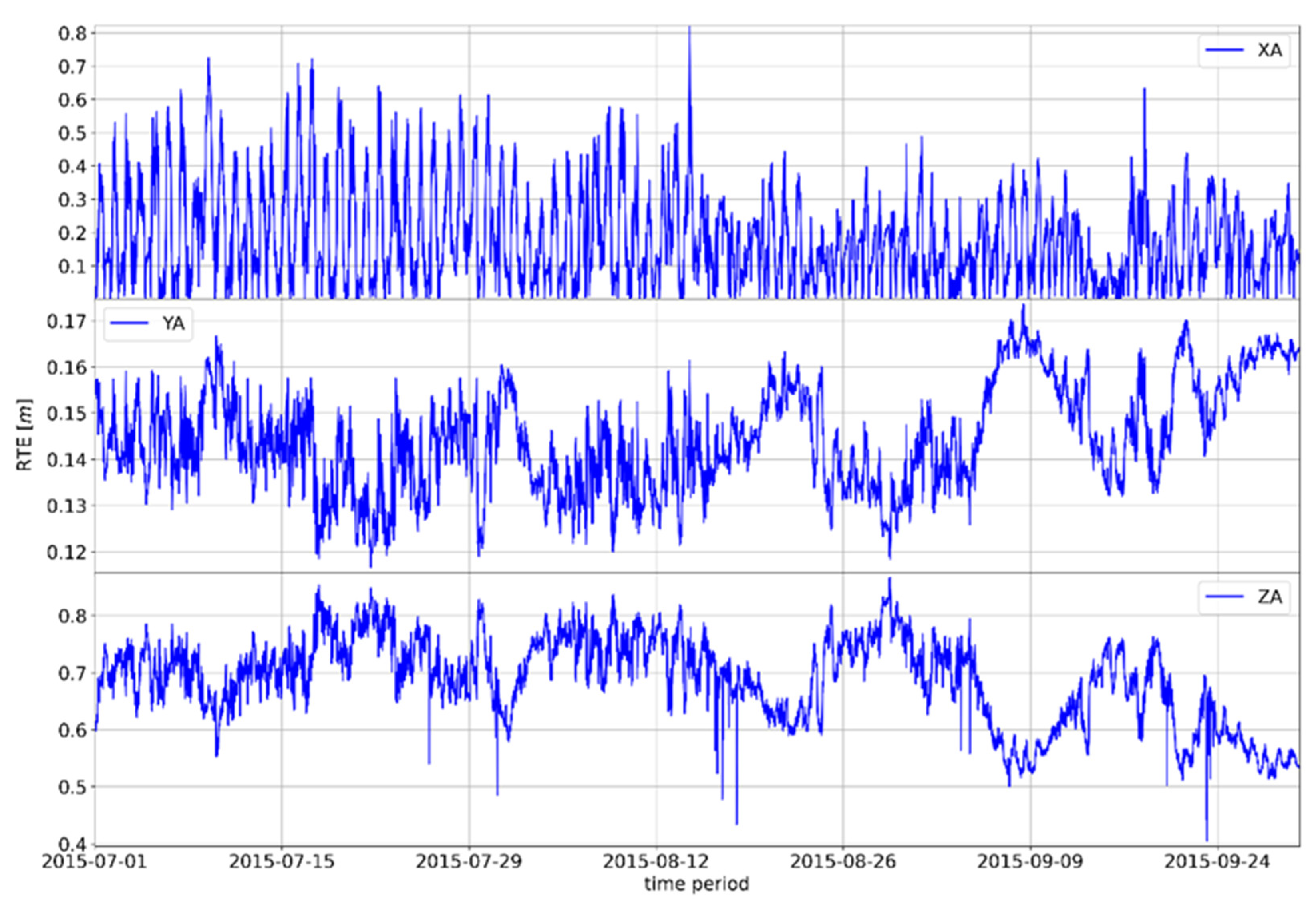
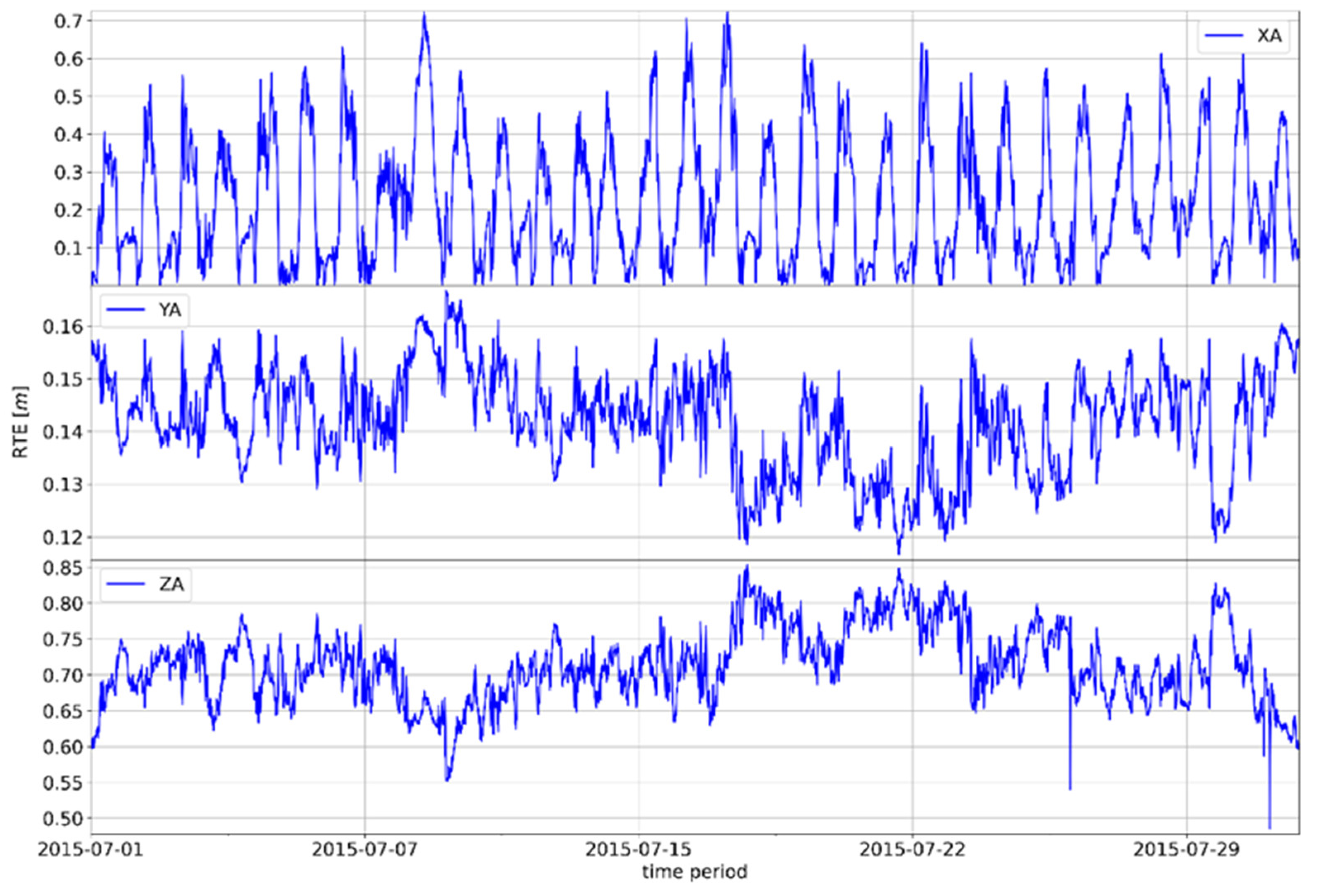
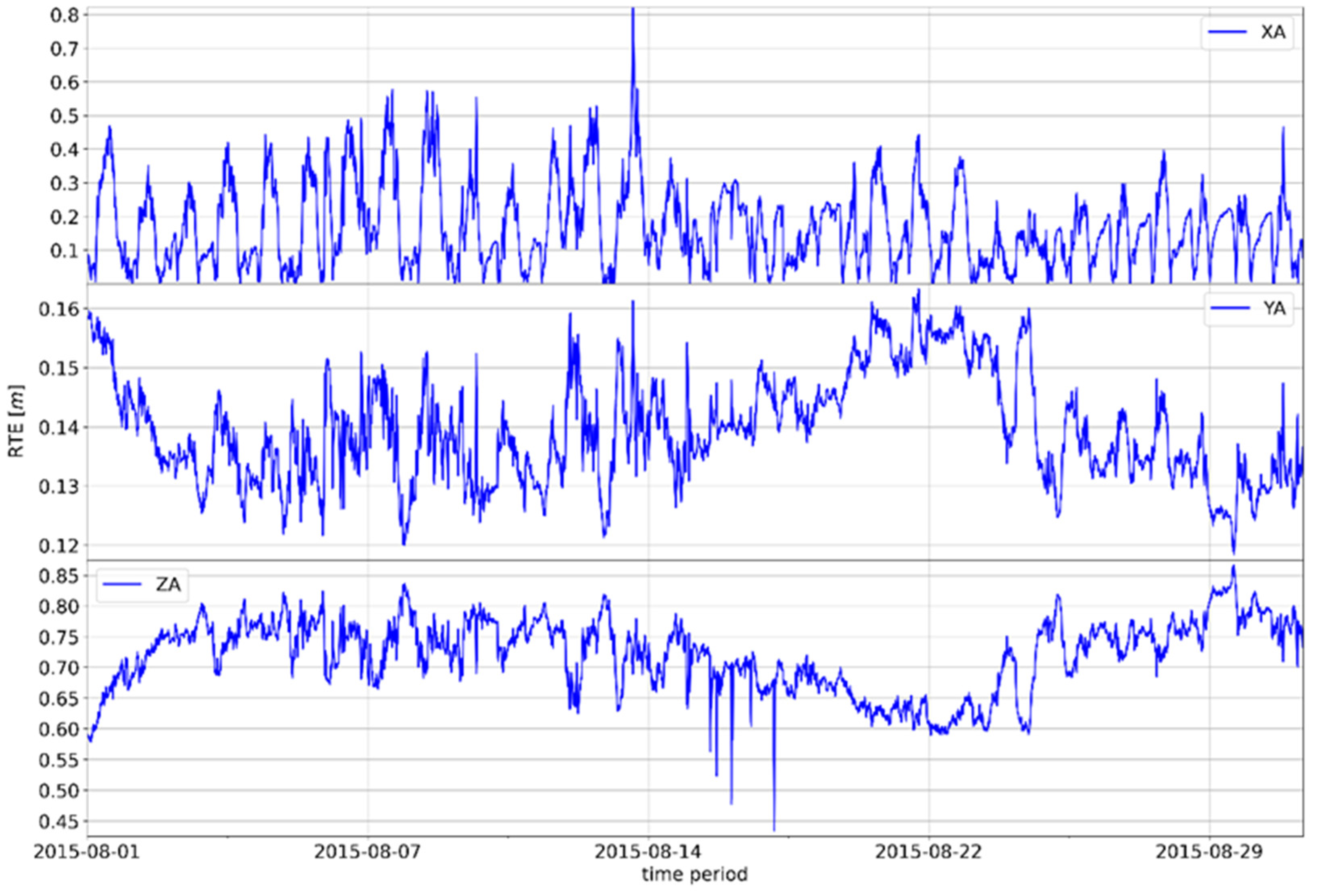
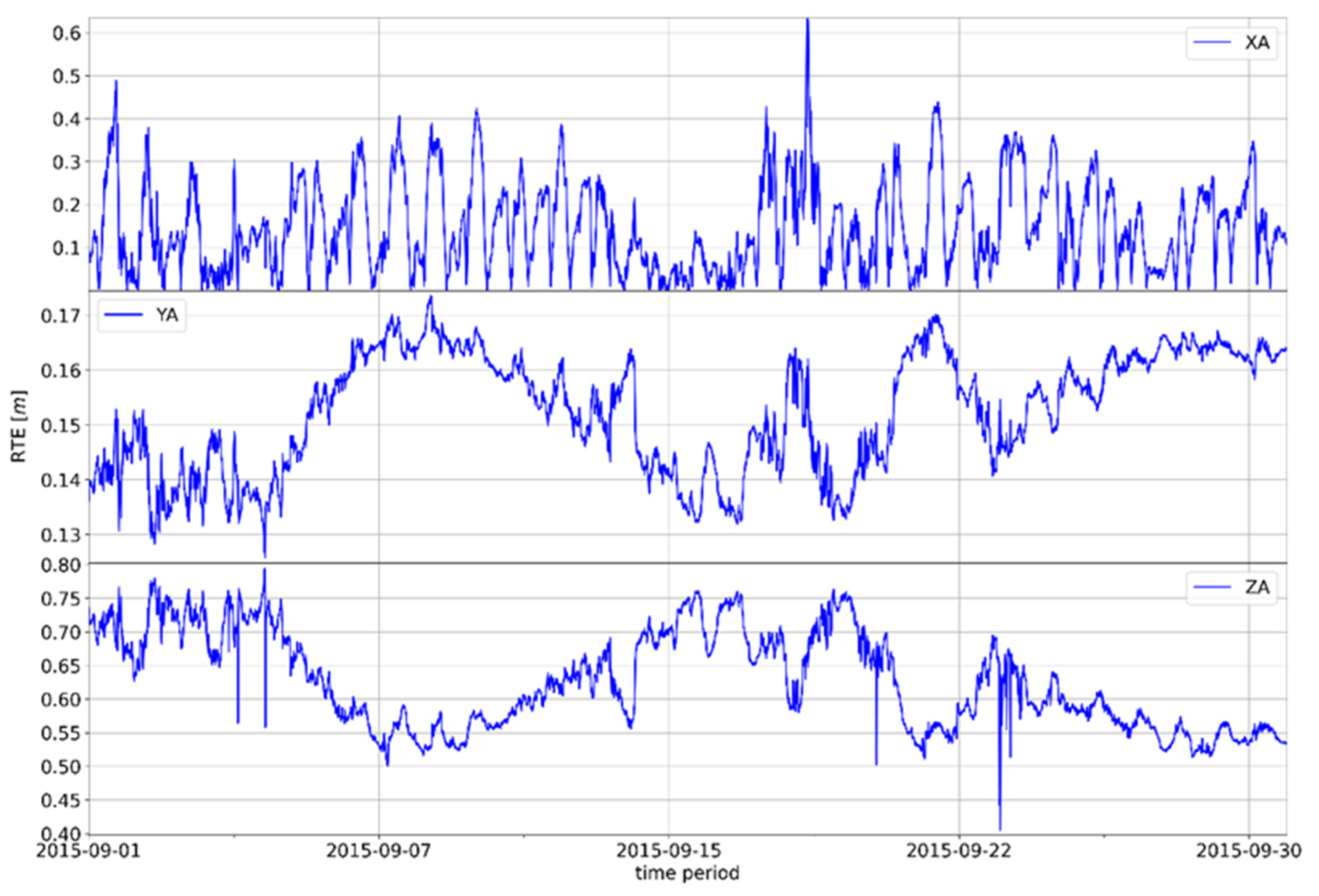
References
- Ansari, K.; Jamjareegulgarn, P. Effect of Weighted PDOP on Performance of Linear Kalman Filter for RTK Drone Data. IEEE Geosci. Remote Sens. Lett. 2022, 19, 3513504. [Google Scholar] [CrossRef]
- Kaplan, E.D.; Hegarty, C.J. Understanding GPS: Principles and Application, 2nd ed.; Artech House: Boston, MA, USA, 2006. [Google Scholar]
- Brčić, D.; Ćelić, J.; Valčić, S. Reconstruction of Geomagnetic Event as Observed in Northern Adriatic Region and Its Correlation with GPS Single-frequency Positioning Deviations. Int. J. Mar. Navig. Saf. Sea Transp. 2020, 14, 349–357. [Google Scholar] [CrossRef]
- Subirana, J.S.; Zornoza, J.M.J.; Hernández-Pajares, M. GNSS DATA PROCESSING Volume I: Fundamentals and Algorithms Acknowledgements; ESA Communications ESTE: Leiden, The Netherlands, 2013. [Google Scholar]
- Elektronika, Uređaji. Širenje Valova. In Tehnička Enciklopedija; LZMIK: Zagreb, Croatia, 1998; pp. 623–627. [Google Scholar]
- Ustinov, U.M.; Afanasev, V.V.; Pripotnyuk, A.V.; Marinich, A.N. Судoвые Радиoлoкациoнные Системы, 3rd ed.; Веленара: Sankt-Petersburg, Russia, 2009. [Google Scholar]
- Jin, S.P.; Cardellach, E.; Xie, F. GNSS Atmospheric and Multipath Delays. In GNSS Remote Sensing; Springer: Dordrecht, The Netherlands, 2014; Volume 19, pp. 17–30. [Google Scholar]
- El-Arini, M.B. Tropospheric Effects on GNSS Bertram Arbesser-Rastburg. In Proceedings of the Atmosphere and its Effect on GNSS Systems, Santiago, Chile, 14–16 April 2018; pp. 146–165. [Google Scholar]
- Dodo, S.Y.; Ojigi, J.D.; Tsebeje, L.M. Determination of the best-fit Tropospheric Delay Model on the Nigerian Permanent GNSS Network. J. Geosci. Geomat. 2015, 3, 88–95. [Google Scholar]
- Katsougiannopoulos, S.; Pikridas, C.; Rossikopoulos, D.; Ifadis, I.M.; Fotiou, A. Tropospheric Refraction Estimation Using Various Models, Radiosonde Measurements and Permanent GPS Data. In Proceedings of the XXIII FIG Congress, Munich, Germany, 8–13 October 2006. [Google Scholar]
- Hopfield, H.S. Two-quartic tropospheric refractivity profile for correcting satellite data. J. Geophys. Res. 1969, 74, 4487–4499. [Google Scholar] [CrossRef]
- Saastamoinen, J. Contributions to the theory of atmospheric refraction. Bull. Géodésique 1972, 105, 279–298. [Google Scholar] [CrossRef]
- Pershin, D.Y. Comparative analysis of tropospheric delay models in precise point positioning in satellite navigation systems GLONASS/GPS. Вестник Нoвoсибирскoгo Гoсударственнoгo Университета. Серия: Инфoрмациoнные Технoлoгии 2009, 7, 84–91. [Google Scholar]
- Marini, J.W. Correction of Satellite Tracking Data for an Arbitrary Tropospheric Profile. Radio Sci. 1972, 7, 223–231. [Google Scholar] [CrossRef]
- Davis, J.L.; Herring, T.A.; Shapiro, I.I.; Rogers, A.E.E. Geodesy by radio interferometry: Effects of atmospheric modeling errors on estimates of baseline length. Radio Sci. 1985, 20, 1593–1607. [Google Scholar] [CrossRef]
- Ifadis, I.M. The excess propagation path of radio waves: Study of the influence of the atmospheric parameters on its elevation dependence. Surv. Rev. 2013, 31, 289–298. [Google Scholar] [CrossRef]
- Askne, J.; Nordius, H. Estimation of tropospheric delay for microwaves from surface weather data. Radio Sci. 1987, 22, 379–386. [Google Scholar] [CrossRef]
- Shrestha, S.M. Investigations into the Estimation of Tropospheric Delay; University of Calgary: Calgary, AB, Canada, 2003. [Google Scholar]
- Kleijer, F. Troposphere Modeling and Filtering for Precise GPS Leveling; Delft University of Technology: Delft, The Netherlands, 2004. [Google Scholar]
- Xia, P.; Xia, J.; Ye, S.; Xu, C. A new method for estimating tropospheric zenith wet-component delay of gnss signals from surface meteorology data. Remote Sens. 2020, 12, 3497. [Google Scholar] [CrossRef]
- Dousa, J.; Elias, M. An improved model for calculating tropospheric wet delay. Geophys. Res. Lett. 2014, 41, 4389–4397. [Google Scholar] [CrossRef]
- Parameswaran, K.; Saha, K.; Raju, S.C. Development of a regional tropospheric delay model for GPS-based navigation with emphasis to the Indian Region. Radio Sci. 2008, 43, 1–10. [Google Scholar] [CrossRef]
- Parameswaran, K.; Raju, C.S.; Saha, K. Region-specific Tropospheric Delay Model for the Indian Subcontinent. In Proceedings of the ICG-Meeting, Bangalore, India, 5–7 September 2007. [Google Scholar]
- Isioye, O.A.; Combrinck, L.; Botai, J. Performance Evaluation of Blind Tropospheric Delay correction Models over Africa. S. Afr. J. Geomat. 2015, 4, 502. [Google Scholar] [CrossRef][Green Version]
- Hu, Y.; Yao, Y. A new method for vertical stratification of zenith tropospheric delay. Adv. Sp. Res. 2019, 63, 2857–2866. [Google Scholar] [CrossRef]
- Yang, F.; Guo, J.; Zhang, C.; Li, Y.; Li, J. A regional zenith tropospheric delay (Ztd) model based on Gpt3 and Ann. Remote Sens. 2021, 13, 838. [Google Scholar] [CrossRef]
- Morel, L.; Pottiaux, E.; Durand, F.; Fund, F.; Boniface, K.; Junior, P.S.d.; van Baelen, J. Validity and behaviour of tropospheric gradients estimated by GPS in Corsica. Adv. Sp. Res. 2015, 55, 135–149. [Google Scholar] [CrossRef]
- Deng, Z.; Bender, M.; Zus, F.; Ge, M.; Dick, G.; Ramatschi, M.; Wickert, J.; Löhnert, U.; Schön, S. Validation of tropospheric slant path delays derived from single and dual frequency GPS receivers. Radio Sci. 2011, 46, 6007. [Google Scholar] [CrossRef]
- Mohammed, A.N.; Abdelfatah, M.A.; Mousa, A.E.K.; El-Fiky, G.S. Regional Egypt tropospheric mapping function model. Alex. Eng. J. 2018, 57, 4157–4165. [Google Scholar] [CrossRef]
- Younes, S.A.M.; Afify, H.A. Accuracy improvement of tropospheric delay correction models in space geodetic data. Case study: Egypt. Geod. Cartogr. 2014, 40, 148–155. [Google Scholar] [CrossRef][Green Version]
- Yang, Y.F.; Chen, X.P.; Yao, M.H.; Zhou, C.L.; Liao, C.M. Research on zenith tropospheric delay modeling of regional cors network. Int. Arch. Photogramm. Remote Sens. Spat. Inf. Sci. 2020, XLII-3/W10, 1197–1200. [Google Scholar] [CrossRef]
- Dodo, J.D.; Ekeanyanwu, U.O.; Ono, M.N. Evaluation of Five Tropospheric Delay Models on Global Navigation Satellite System Measurements in Southern Nigeria. J. Geosci. Geomat. 2019, 7, 201–211. [Google Scholar] [CrossRef]
- Li, F.; Zhang, Q.; Zhang, S.; Lei, J.; Li, W. Evaluation of Spatio-Temporal Characteristics of Different Zenith Tropospheric Delay Models in Antarctica. Radio Sci. 2020, 55, e2019RS006909. [Google Scholar] [CrossRef]
- Krueger, E.; Schueler, T.; Arbesser-rastburg, B. The Standard Tropospheric Correction Model for the European Satellite Navi−Gation System Galileo. In Proceedings of the General As-sembly URSI, New Delhi, India, 2005; Available online: https://www.researchgate.net/profile/Bertram_Arbesser−Rastburg/publication/252717445_THE_STANDARD_TROPOSPHER-IC_CORRECTION_MODEL_FOR_THE_EUROPEAN_SATELLITE_NAVIGATION_SYSTEM_GALILEO/links/00b4952c318413b26d000000/THE−STANDARD−TROPOSPHERIC−CORRECTION−MODEL−FOR−THE−EUROPEAN−SATELLITE−NAVIGATION−SYSTEM−GALILEO.pdf (accessed on 30 November 2021).
- Snajdrova, K.; Boehm, J.; Willis, P.; Haas, R.; Schuh, H. Multi-technique comparison of tropospheric zenith delays derived during the CONT02 campaign. J. Geod. 2006, 79, 613. [Google Scholar] [CrossRef]
- Ajayi, G. Physics of the Tropospheric Radiopropagation; International Centre for Theoretical Physic: Trieste, Italy, 1989. [Google Scholar]
- Grabner, M.; Kvicera, V. Atmospheric Refraction and Propagation in Lower Troposphere. In Electromagnetic Waves; Prentice Hall: Hoboken, NJ, USA, 2011. [Google Scholar] [CrossRef]
- Coleman, C.J. A direction-sensitive model of atmospheric noise and its application to the analysis of HF receiving antennas. Radio Sci. 2002, 37, 3-1–3-10. [Google Scholar] [CrossRef]
- Hatanaka, Y. A Compression Format and Tools for GNSS Observation Data. Bull. Geogr. Surv. Inst. 2008, 55, 21–30. [Google Scholar]
- DHMZ—Državni Hidrometeorološki Zavod. Available online: http://meteo.hr/index.php (accessed on 28 November 2021).
- Volarić, B.; Lisac, I. Klimatska podjela Hrvatske prema značajkama godišnjeg hoda temperature zraka. Acta Geogr. Croat. 1984, 19, 3–11. [Google Scholar]
- Tadić, M.P.; Čapka, G.M.; Zaninović, K.; Cindrić, K. Drought Vulnerability in Croatia. Agric. Conspec. Sci. 2014, 79, 31–38. [Google Scholar]
- EUREF Permanent GNSS Network. Available online: http://www.epncb.oma.be/index.php (accessed on 16 August 2021).
- RTKLIB: Documents. Available online: http://www.rtklib.com/rtklib_document.htm (accessed on 10 November 2021).
- Astudillo, M.J.; Lau, L.; Tang, Y.T.; Moore, T. Analysing the Zenith Tropospheric Delay Estimates in On-line Precise Point Positioning (PPP) Services and PPP Software Packages. Sensors 2018, 18, 580. [Google Scholar] [CrossRef]
- RTKLIB: An Open Source Program Package for GNSS Positioning. Available online: http://www.rtklib.com (accessed on 29 November 2021).
- Niell, A.E. Global mapping functions for the atmosphere delay at radio wavelengths. J. Geophys. Res. Solid Earth 1996, 101, 3227–3246. [Google Scholar] [CrossRef]
- Niell, A.E. Improved atmospheric mapping functions for VLBI and GPS. Earth Planets Space 2000, 52, 699–702. [Google Scholar] [CrossRef]
- Niell, A.E. Preliminary evaluation of atmospheric mapping functions based on numerical weather models. Phys. Chem. Earth Part A Solid Earth Geod. 2001, 26, 475–480. [Google Scholar] [CrossRef]
- ICD GLONASS CDMA. Инфoрмациoннo-Аналитический Центр Кoнтрoля ГЛОНАСС и GPS. Available online: https://www.glonass-iac.ru/ (accessed on 16 August 2021).
- Plischke, M.; Bergersen, B. Equilibrium Statistical Physics, 3rd ed.; World Scientific Publishing Co. Pte. Ltd.: Singapore, 2006. [Google Scholar]
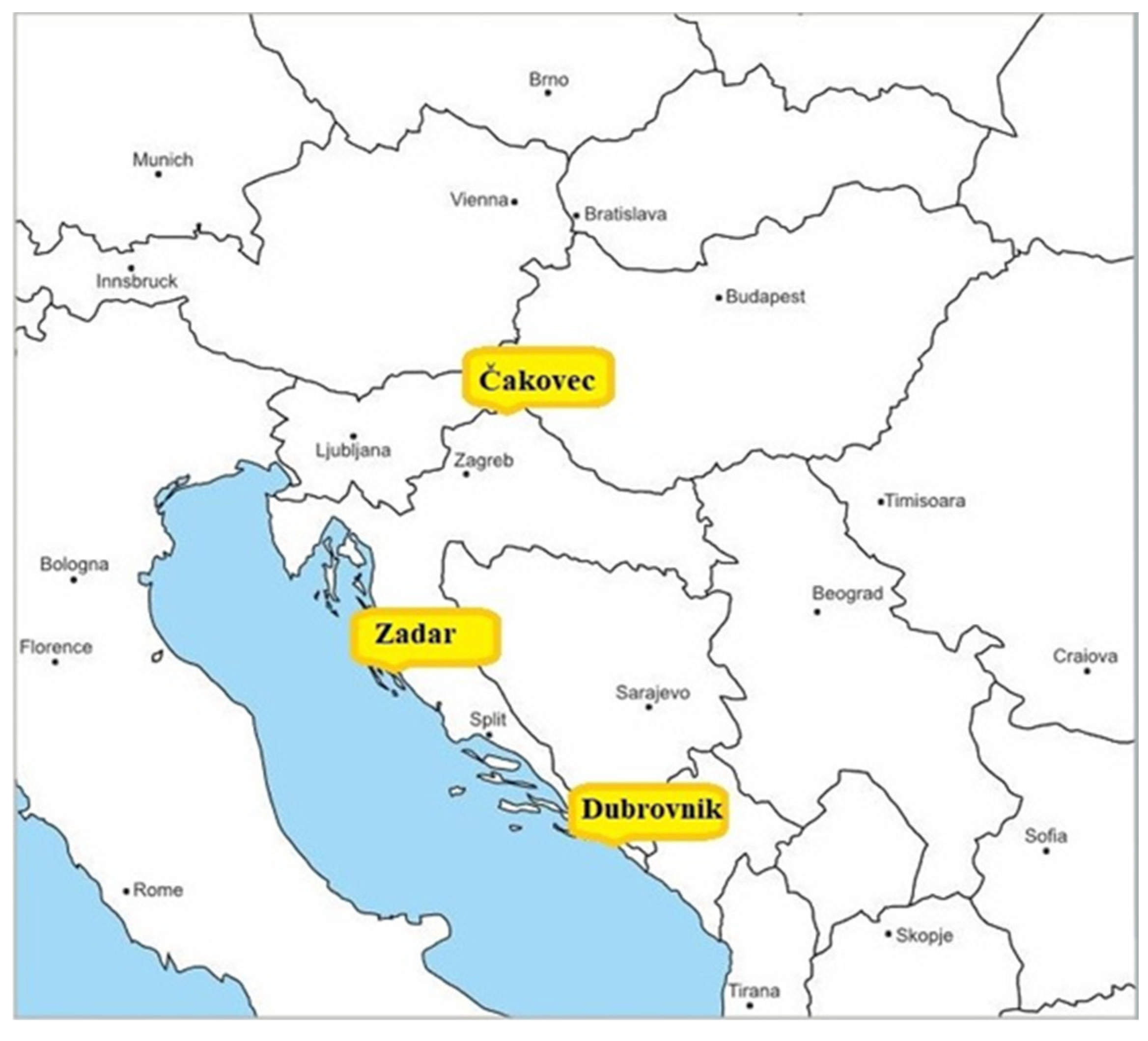


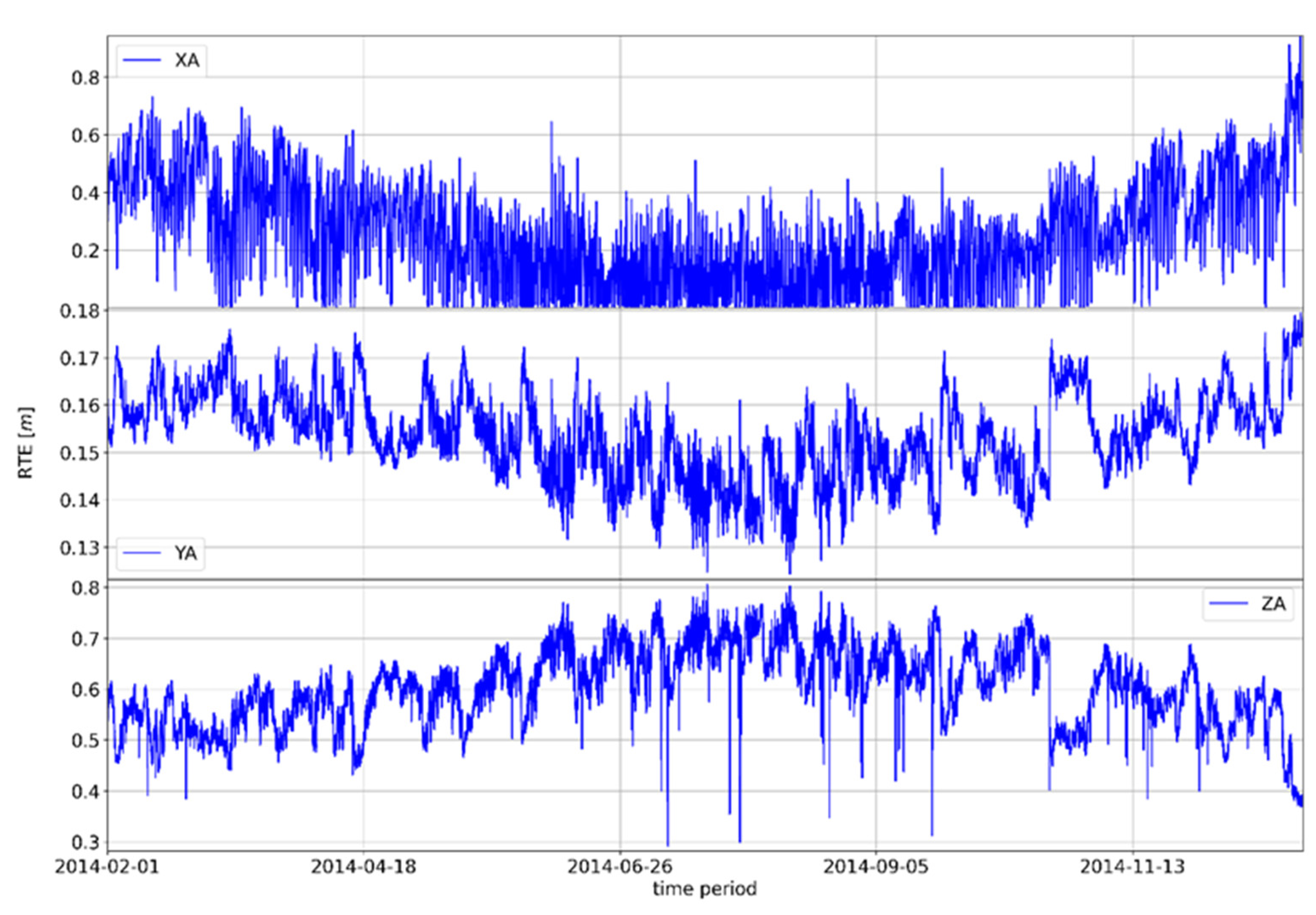
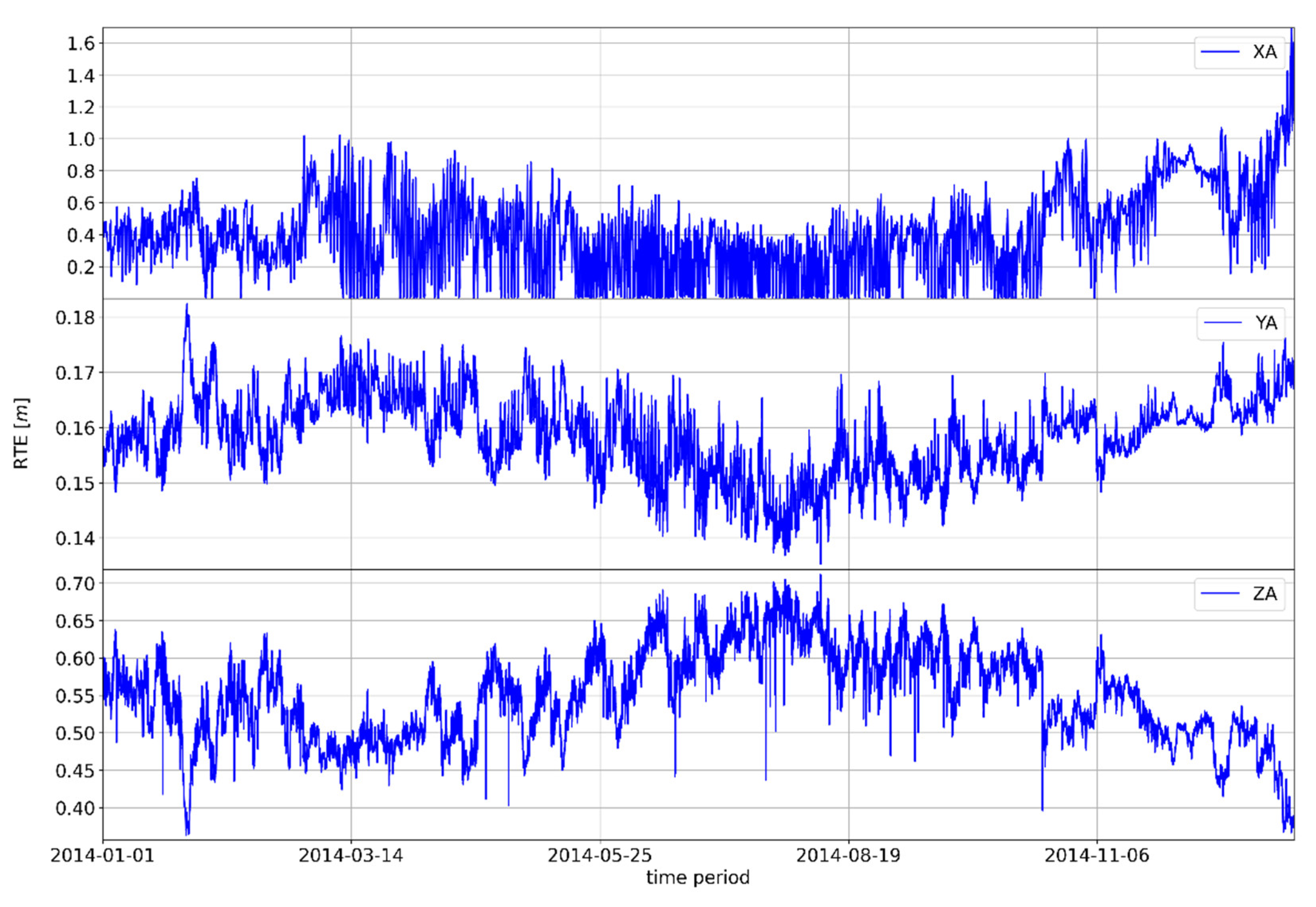
| Station Log | City | Latitude φ (° N) | Longitude λ (° E) | Elevation h (m) | ECEF (ETRS 89) Coordinates 1 (m) | ||
|---|---|---|---|---|---|---|---|
| x | y | z | |||||
| CAKO00 HRV | Čakovec | 46.387 | 16.439 | 222.1 | 4,227,250.7 | 1,247,280.6 | 4,595,193.3 |
| DUB200 HRV | Dubrovnik | 42.650 | 18.110 | 457.9 | 4,465,932.8 | 1,460,581.6 | 4,299,308.5 |
| ZADA00 HRV | Zadar | 44.113 | 15.227 | 64.3 | 4,425,737.1 | 1,204,734.5 | 4,417,173.4 |
| Multiple Correlation | Multiple Determination | Adjusted Determination Coefficient | p-Value | |
|---|---|---|---|---|
| X axis | 0.115 | 0.013 | 0.013 | 0.00037 |
| Y axis | 0.034 | 0.001 | 0.001 | 0.00273 |
| Z axis | 0.092 | 0.008 | 0.008 | 0.00019 |
| City | Year | Range | x | y | z |
|---|---|---|---|---|---|
| Čakovec | 2014 | Max | 1.69 | −0.13 | −0.31 |
| Min | −0.64 | −0.17 | −0.71 | ||
| 2015 | Max | 1.61 | −0.12 | −0.35 | |
| Min | −0.85 | −0.18 | −0.75 |
| Saastamoinen Model | Proposed Model | |||||
|---|---|---|---|---|---|---|
| 2014 | x | y | z | x | y | z |
| Max | 27.731 | 15.087 | 26.671 | 27.110 | 15.257 | 27.168 |
| Min | −21.003 | −17.843 | −20.919 | −21.186 | −17.690 | −20.328 |
| RMSE | 3.666 | 2.246 | 4.676 | 3.565 | 2.245 | 4.518 |
| STD | 3.593 | 2.244 | 4.400 | 3.553 | 2.244 | 4.396 |
| Median | 0.753 | −0.113 | −1.517 | 0.355 | 0.045 | -0.969 |
| 2015 | x | y | z | x | y | z |
| Max | 23.429 | 12.394 | 20.610 | 22.653 | 12.549 | 21.036 |
| Min | −15.533 | −15.782 | −23.515 | −15.842 | −15.621 | −22.958 |
| RMSE | 3.681 | 2.355 | 4.111 | 3.539 | 2.362 | 4.036 |
| STD | 3.464 | 2.355 | 4.027 | 3.436 | 2.355 | 4.025 |
| Median | 1.318 | −0.024 | −0.815 | 0.925 | 0.136 | −0.280 |
| City | Year | Range | x | y | z |
|---|---|---|---|---|---|
| Zadar | 2014 | Max | −0.67 | −0.17 | −0.80 |
| Min | 0.90 | −0.12 | −0.29 | ||
| 2015 | Max | −0.85 | −0.18 | −0.86 | |
| Min | 0.88 | −0.11 | −0.28 |
| Saastamoinen Model | Proposed Model | |||||
|---|---|---|---|---|---|---|
| 2014 | x | y | z | x | y | z |
| Max | 24.007 | 14.924 | 43.551 | 23.987 | 15.070 | 43.995 |
| Min | −68.814 | −28.262 | −69.978 | −69.145 | −28.093 | −69.437 |
| RMSE | 4.266 | 2.565 | 5.235 | 4.233 | 2.556 | 5.008 |
| STD | 4.257 | 2.554 | 4.737 | 4.232 | 2.554 | 4.737 |
| Median | 0.284 | −0.279 | −2.129 | 0.076 | −0.124 | −1.520 |
| 2015 | x | y | z | x | y | z |
| Max | 31.536 | 16.820 | 25.550 | 31.410 | 16.971 | 25.978 |
| Min | −50.777 | −20.134 | −37.990 | −50.937 | −19.988 | −37.365 |
| RMSE | 4.312 | 2.505 | 4.147 | 4.233 | 2.521 | 4.133 |
| STD | 3.836 | 2.498 | 4.130 | 3.809 | 2.498 | 4.127 |
| Median | 2.051 | 0.105 | −0.372 | 1.859 | 0.260 | 0.219 |
| City | Year | Range | x | y | z |
|---|---|---|---|---|---|
| Dubrovnik | 2014 | Max | 1.69 | −0.13 | −0.36 |
| Min | −0.65 | −0.18 | −0.71 | ||
| 2015 | Max | 0.80 | −0.11 | −0.29 | |
| Min | −0.96 | −0.18 | −0.82 |
| Saastamoinen Model | Proposed Model | |||||
|---|---|---|---|---|---|---|
| 2014 | x | y | z | x | y | z |
| Max | 56.471 | 43.660 | 66.975 | 56.238 | 43.820 | 67.519 |
| Min | −40.470 | −34.888 | −49.833 | −41.077 | −34.733 | −49.264 |
| RMSE | 4.519 | 2.736 | 5.457 | 4.532 | 2.711 | 5.218 |
| STD | 4.514 | 2.687 | 4.796 | 4.497 | 2.687 | 4.798 |
| Median | −0.176 | −0.455 | −2.424 | −0.542 | −0.297 | 1.867 |
| 2015 | x | y | z | x | y | z |
| Max | 47.847 | 13.006 | 24.051 | 47.525 | 13.184 | 24.494 |
| Min | −28.606 | −21.630 | −21.456 | −29.167 | −21.458 | −20.922 |
| RMSE | 4.313 | 2.602 | 4.337 | 4.291 | 2.598 | 4.226 |
| STD | 4.180 | 2.598 | 4.185 | 4.175 | 2.598 | 4.186 |
| Median | 1.134 | −0.120 | 1.096 | 1.047 | 0.038 | −0.538 |
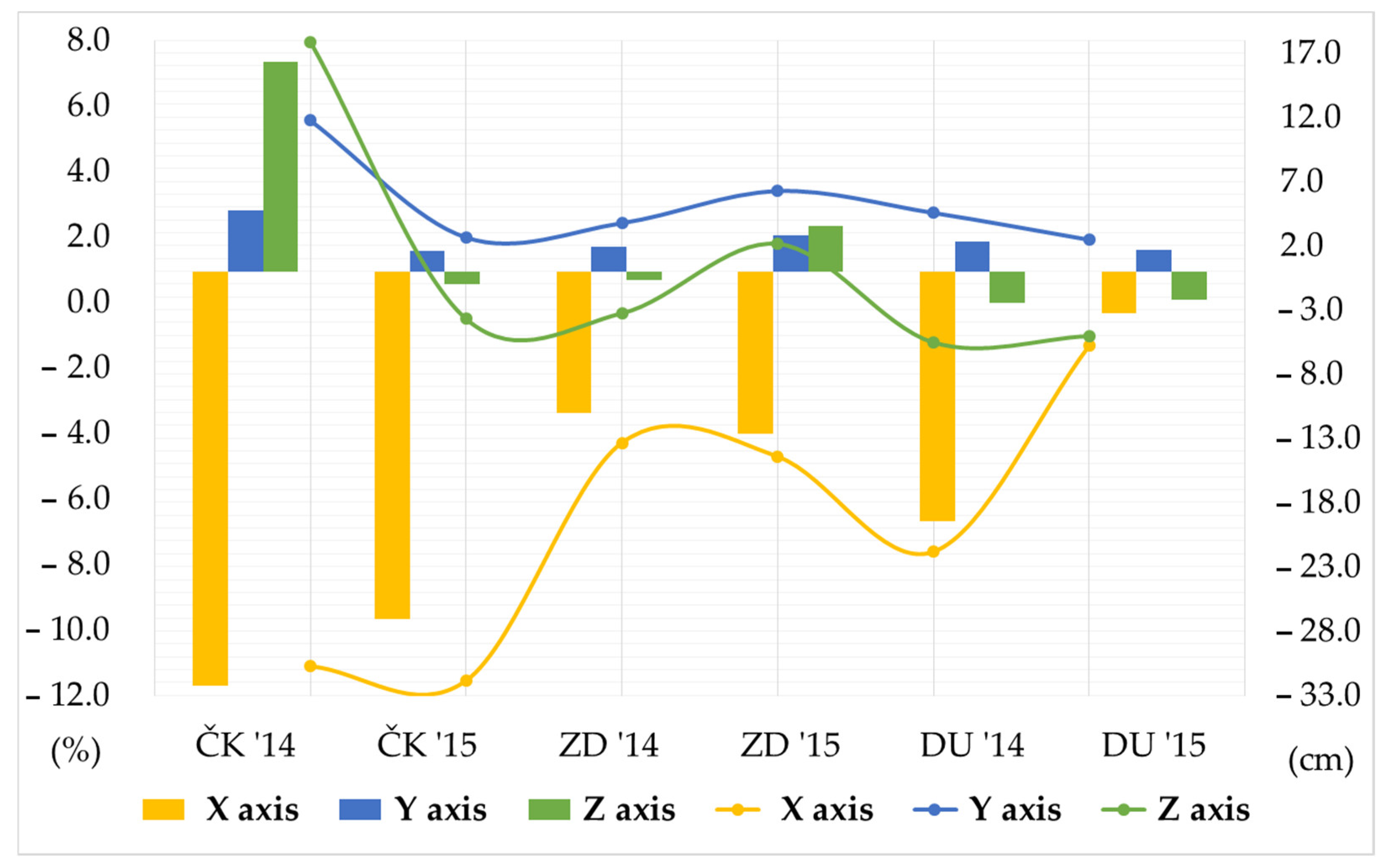
| City & Year | RMSE & STD Tendency | x | y | z | |||
|---|---|---|---|---|---|---|---|
| [%] | [cm] | [%] | [cm] | [%] | [cm] | ||
| Čakovec, 2014 | RMSE | −2.739 | −10.043 | −0.042 | −0.094 | −3.389 | −15.851 |
| STD | −1.118 | −4.020 | 0.014 | 0.033 | −0.075 | −0.331 | |
| Čakovec, 2015 | RMSE | −3.875 | −14.267 | 0.322 | 0.758 | −1.840 | −7.569 |
| STD | −0.827 | −2.867 | 0.007 | 0.016 | −0.047 | −0.192 | |
| Zadar, 2014 | RMSE | −0.781 | −3.335 | −0.374 | −0.962 | −4.338 | −22.717 |
| STD | −0.572 | −2.438 | 0.012 | 0.031 | 0.001 | 0.006 | |
| Zadar, 2015 | RMSE | −2.600 | −11.214 | 0.659 | 1.652 | −0.355 | −1.475 |
| STD | −0.725 | −2.783 | 0.013 | 0.033 | −0.055 | −0.229 | |
| Dubrovnik, 2014 | RMSE | 0.300 | 1.356 | −0.920 | −2.518 | −4.374 | −23.873 |
| STD | −0.395 | −1.783 | 0.001 | 0.004 | 0.026 | 0.127 | |
| Dubrovnik, 2015 | RMSE | −0.517 | −2.232 | −0.132 | −0.343 | −2.572 | −11.156 |
| STD | −0.119 | −0.500 | 0.014 | 0.036 | 0.0346 | 0.144 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bakota, M.; Kos, S.; Mrak, Z.; Brčić, D. A New Approach for Improving GNSS Geodetic Position by Reducing Residual Tropospheric Error (RTE) Based on Surface Meteorological Data. Remote Sens. 2023, 15, 162. https://doi.org/10.3390/rs15010162
Bakota M, Kos S, Mrak Z, Brčić D. A New Approach for Improving GNSS Geodetic Position by Reducing Residual Tropospheric Error (RTE) Based on Surface Meteorological Data. Remote Sensing. 2023; 15(1):162. https://doi.org/10.3390/rs15010162
Chicago/Turabian StyleBakota, Mario, Serdjo Kos, Zoran Mrak, and David Brčić. 2023. "A New Approach for Improving GNSS Geodetic Position by Reducing Residual Tropospheric Error (RTE) Based on Surface Meteorological Data" Remote Sensing 15, no. 1: 162. https://doi.org/10.3390/rs15010162
APA StyleBakota, M., Kos, S., Mrak, Z., & Brčić, D. (2023). A New Approach for Improving GNSS Geodetic Position by Reducing Residual Tropospheric Error (RTE) Based on Surface Meteorological Data. Remote Sensing, 15(1), 162. https://doi.org/10.3390/rs15010162








