Assessment of Different Complementary-Relationship-Based Models for Estimating Actual Terrestrial Evapotranspiration in the Frozen Ground Regions of the Qinghai-Tibet Plateau
Abstract
:1. Introduction
2. Materials and Methods
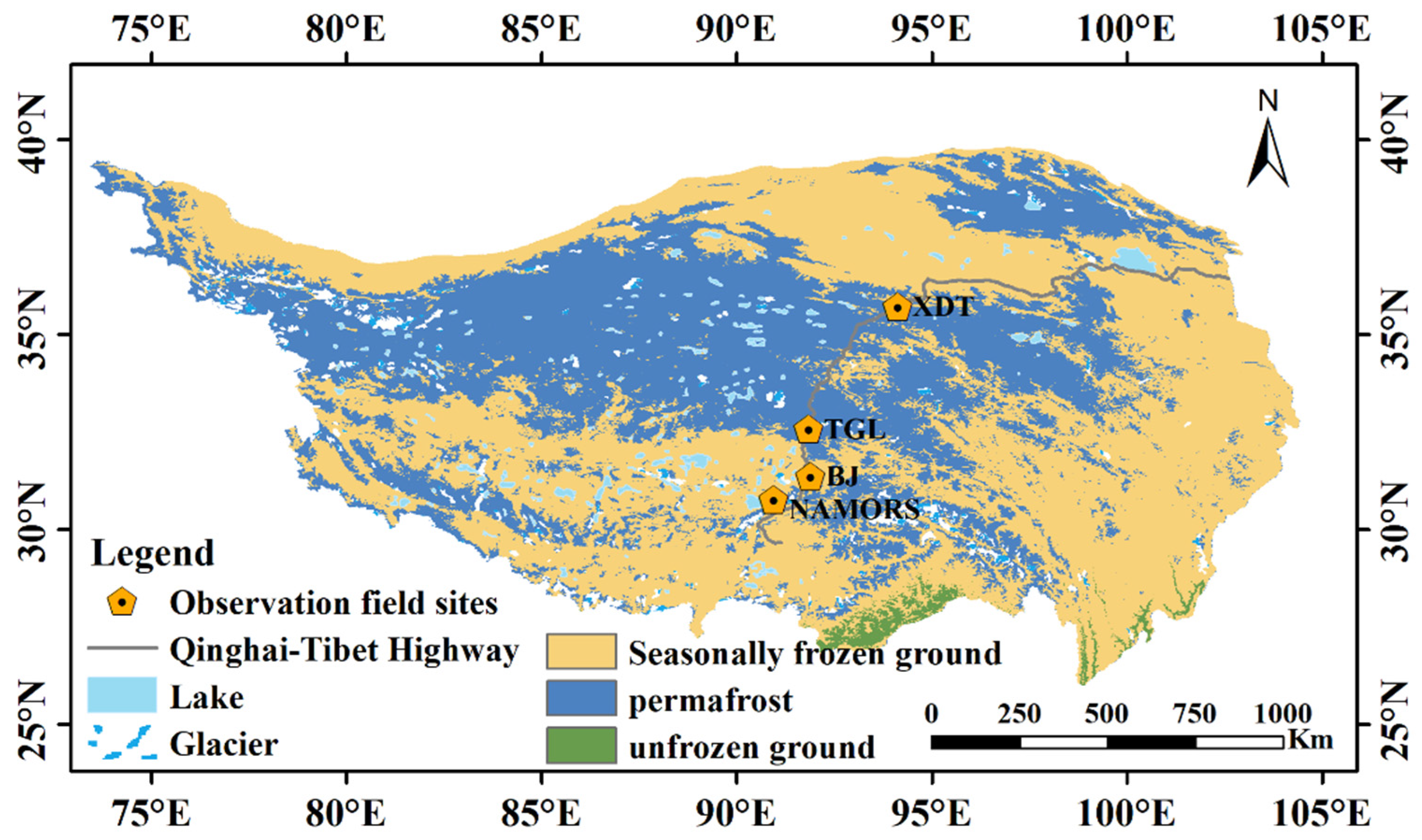
2.1. Site Description
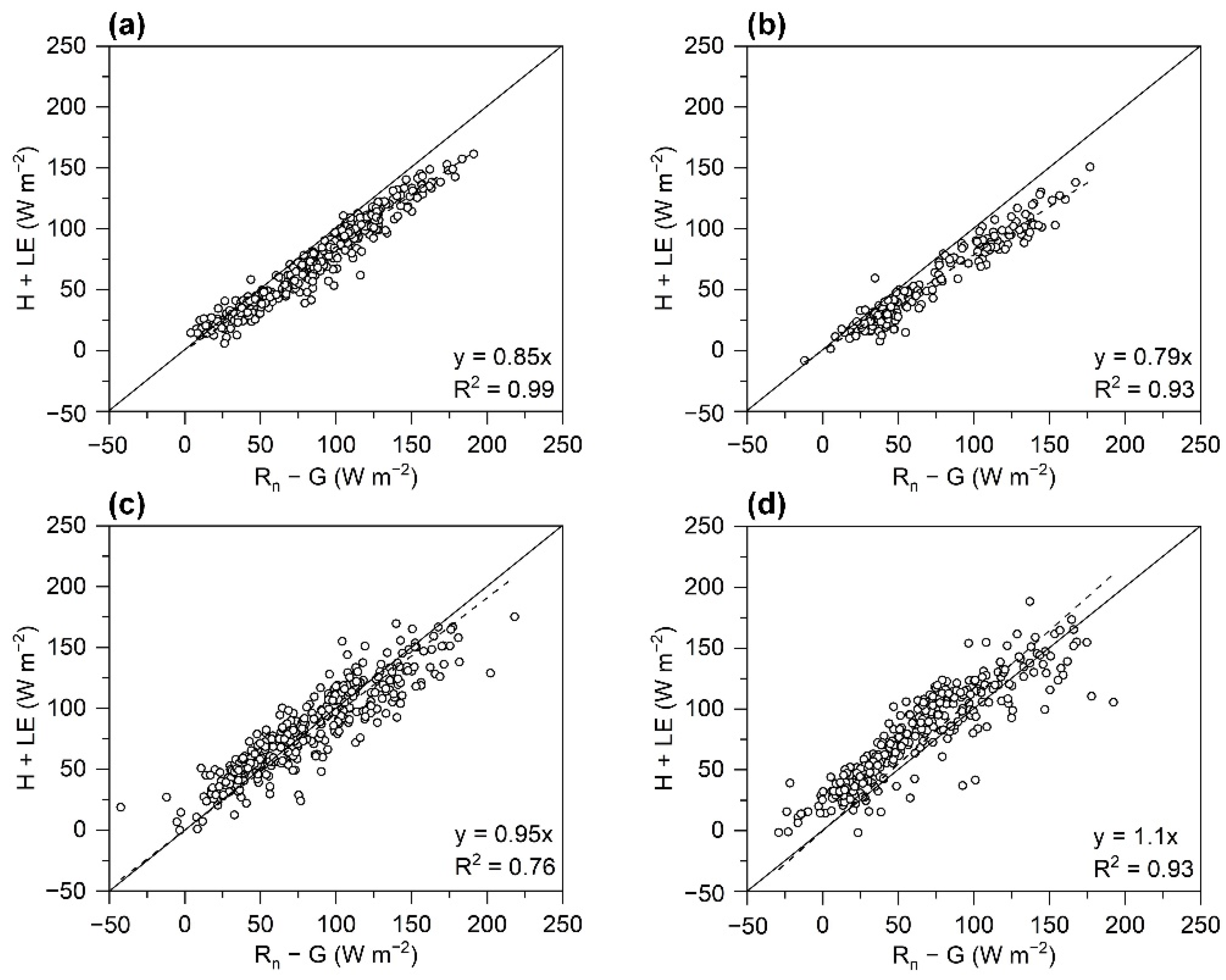
2.2. In Situ Measurement Data and Data Processing
2.3. Complementary Relationship (CR) Approach
2.3.1. Modified AA Model
2.3.2. Polynomial Generalized Complementary Function
2.3.3. Calibration-Free CR Function
2.3.4. Rescaled Complementary Function
2.3.5. Sigmoid Generalized Complementary Function
2.4. Model Parameter Calibration Methods
2.5. Model Evaluation Criteria
3. Results
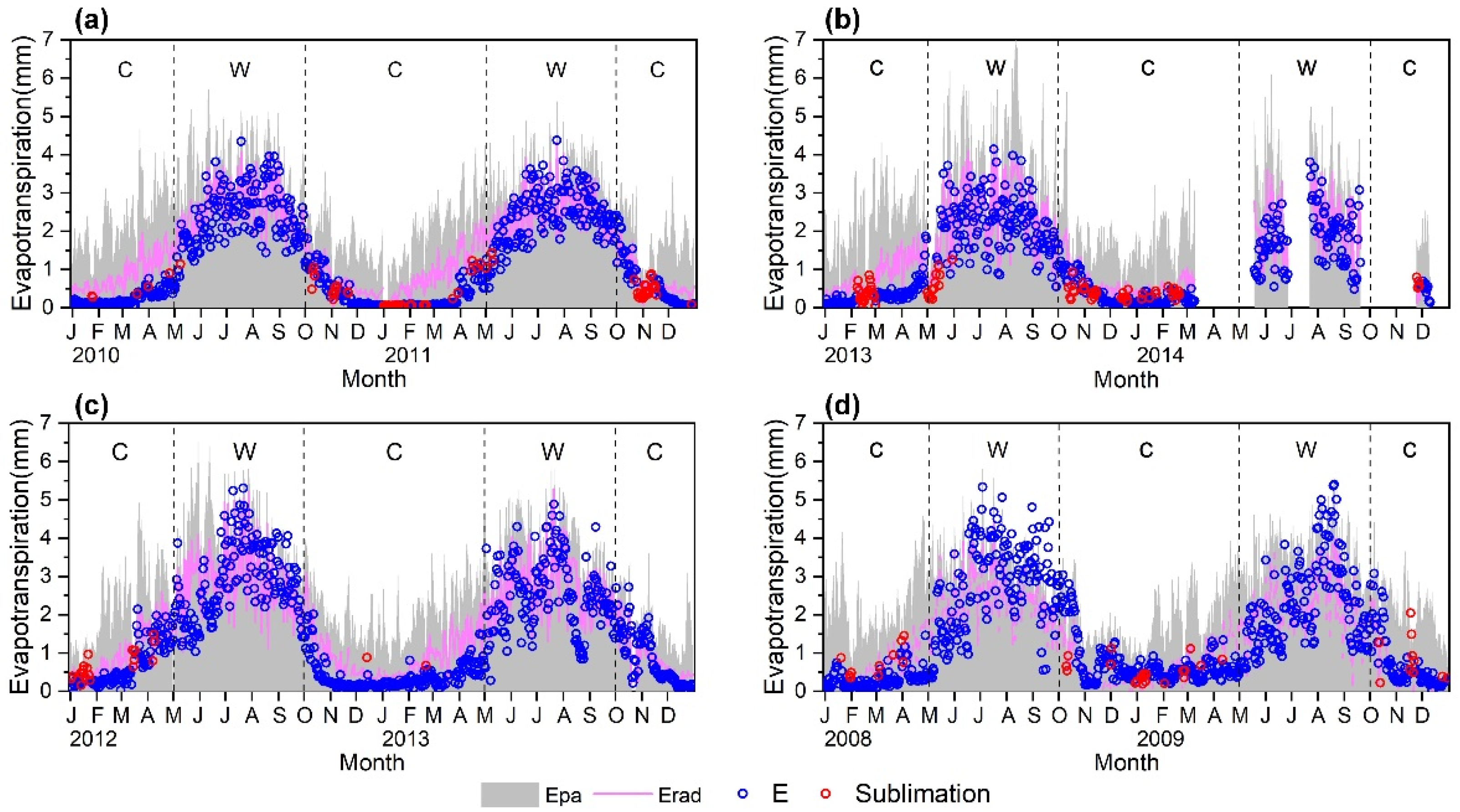
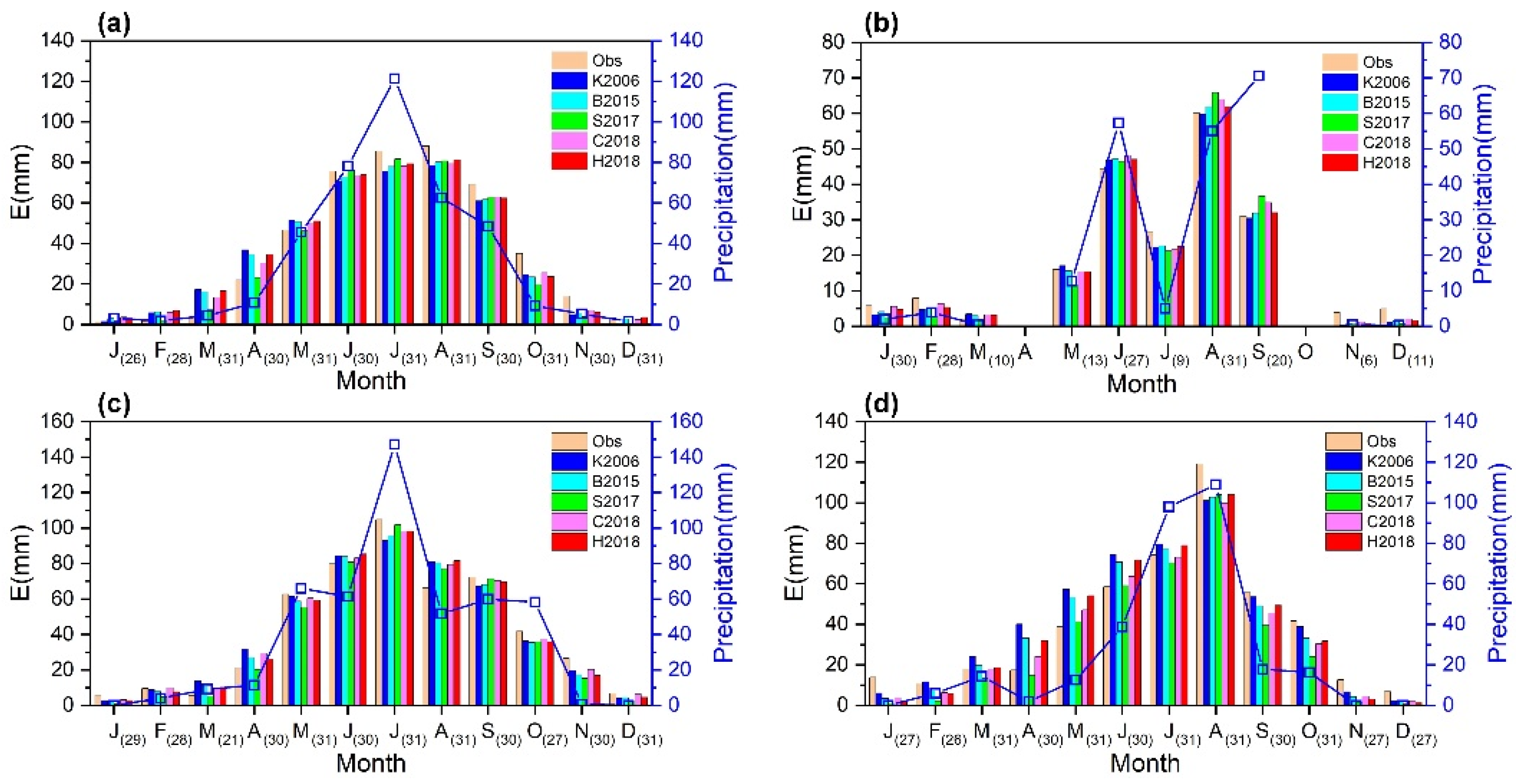
3.1. Variations in ET Rates at Four Observation Sites
3.2. Evaluating Model Performance
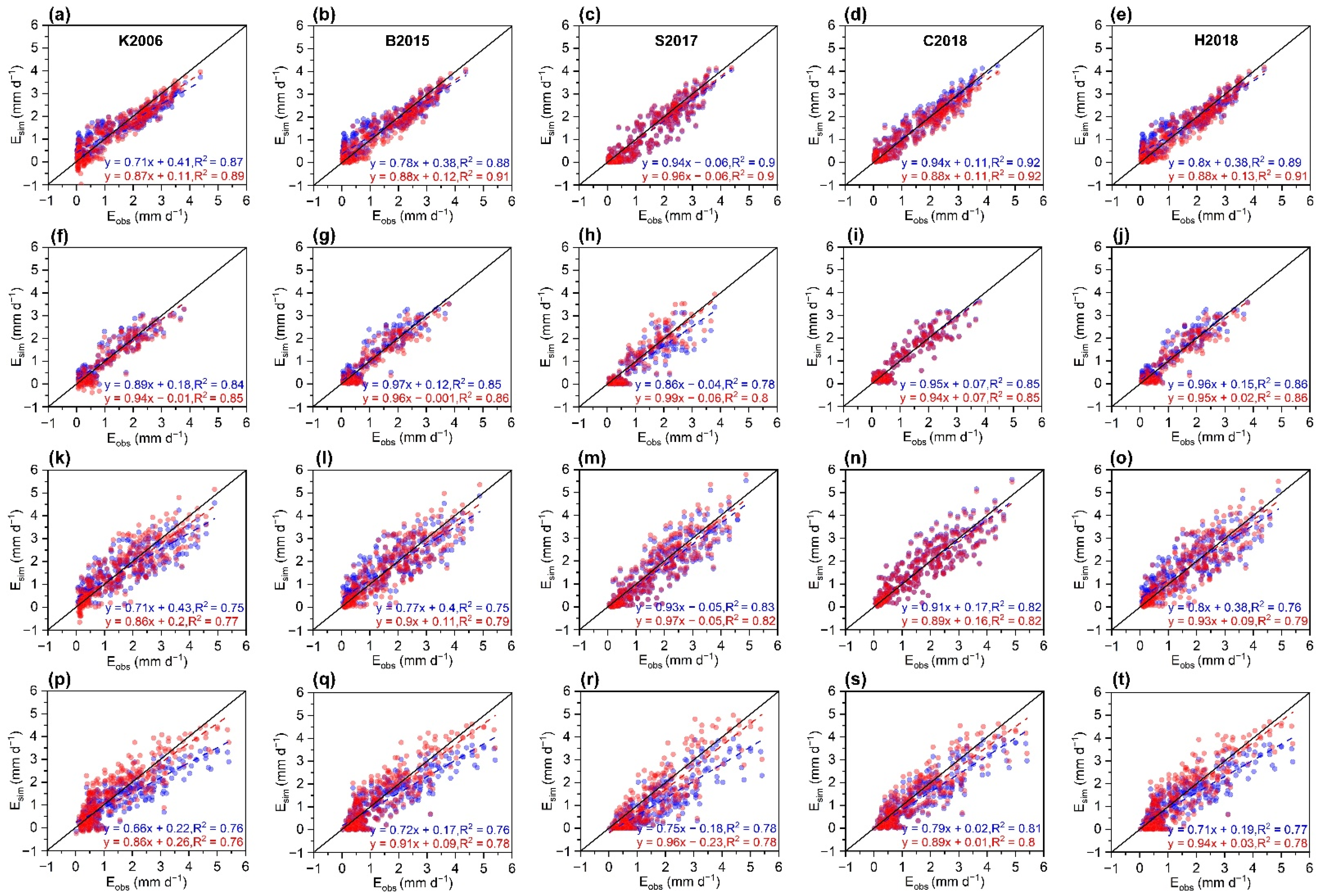
3.2.1. Model Performance with Default and Calibrated Parameter Values on a Daily Timescale
3.2.2. Performance of Different CR-Based Functions against Relationships among Three Evapotranspiration Variables
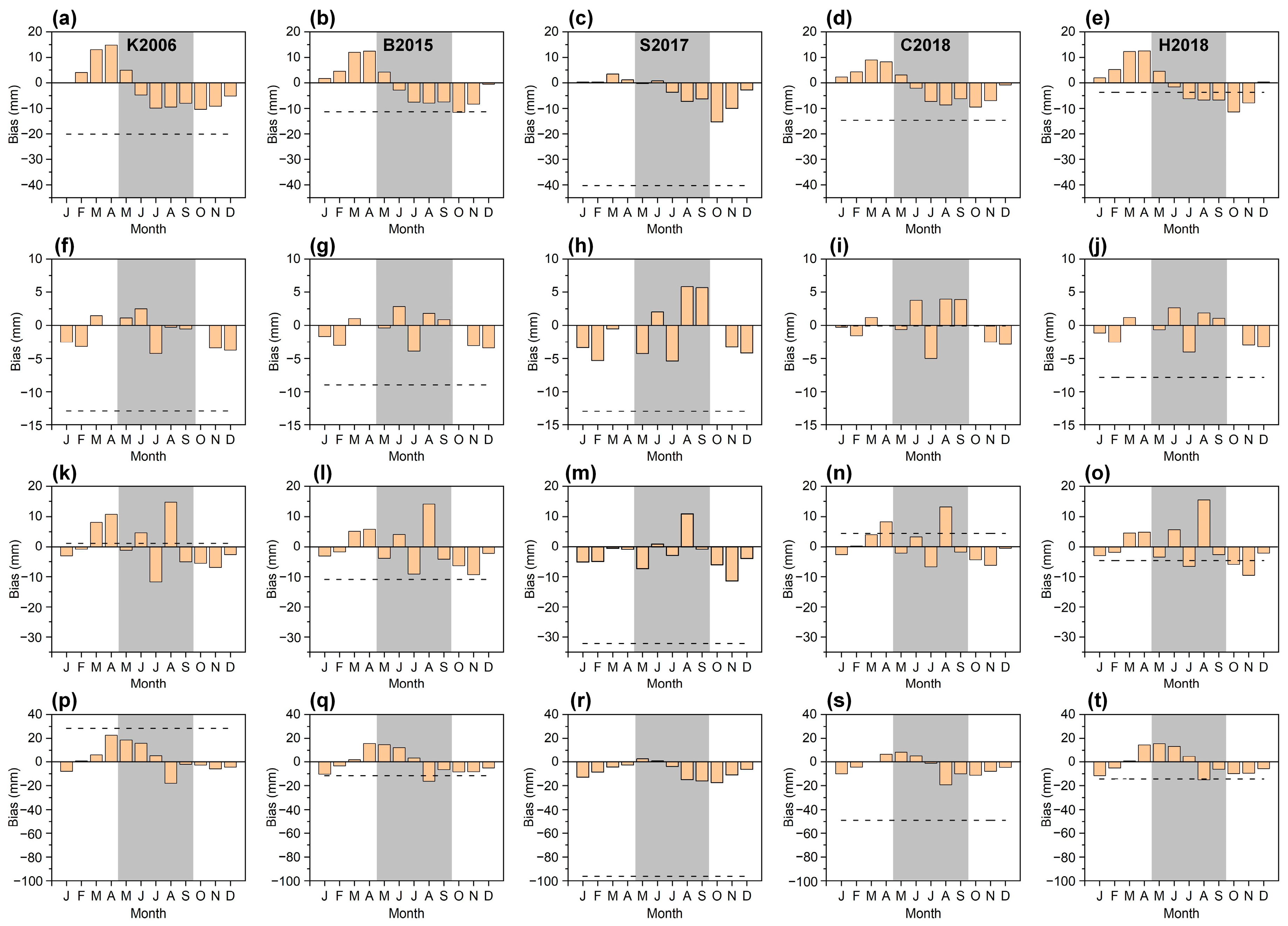
3.2.3. Model Performance with Calibrated Parameter Values on a Monthly Timescale
4. Discussion
4.1. Uncertainty of Actual Evapotranspiration Estimation by the CR Approach
4.1.1. Influence of Parameter Values on Actual Evapotranspiration Estimation
4.1.2. Sensitivity Analysis of CR-Based Models to Parameter Values
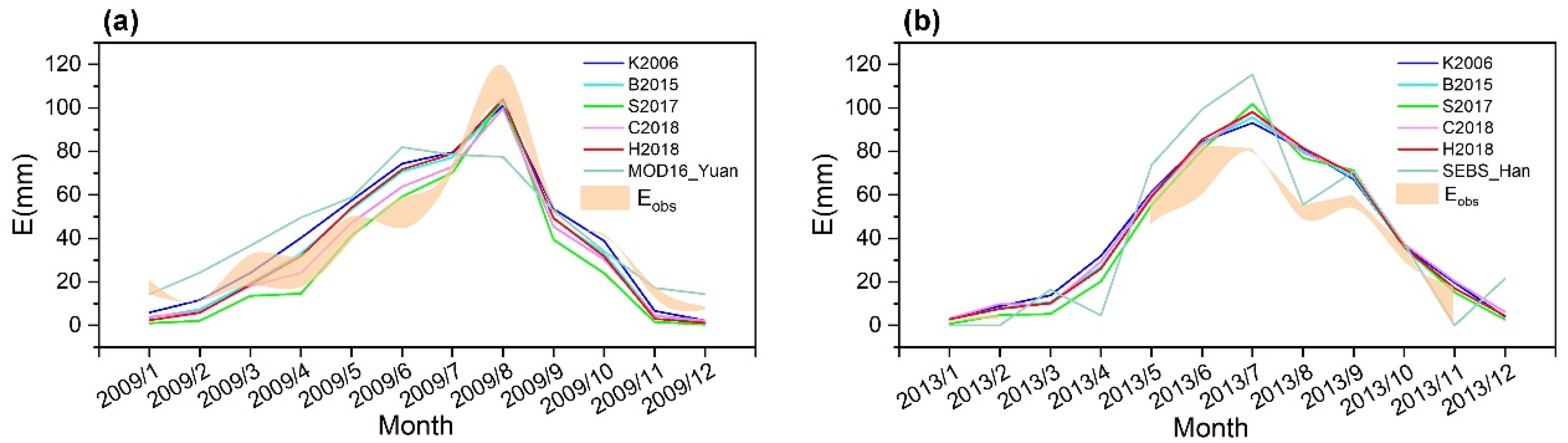
4.2. Comparison with Previous Studies on the QTP at a Single Point Scale
4.3. Perspectives from the Present CR-Based Model Evaluations
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, K.; Dickinson, R.E. A review of global terrestrial evapotranspiration: Observation, modeling, climatology, and climatic variability. Rev. Geophys. 2012, 50, RG2005. [Google Scholar] [CrossRef]
- Jung, M.; Reichstein, M.; Ciais, P.; Seneviratne, S.I.; Sheffield, J.; Goulden, M.L.; Bonan, G.; Cescatti, A.; Chen, J.; Jeu, R.; et al. Recent decline in the global land evapotranspiration trend due to limited moisture supply. Nature 2010, 467, 951–954. [Google Scholar] [CrossRef]
- Fisher, J.B.; Melton, F.; Middleton, E.; Hain, C.; Anderson, M.; Allen, R.; McCabe, M.F.; Hook, S.; Baldocchi, D.; Townsend, P.A.; et al. The future of evapotranspiration: Global requirements for ecosystem functioning, carbon and climate feedbacks, agricultural management, and water resources. Water Resour. Res. 2017, 53, 2618–2626. [Google Scholar] [CrossRef]
- Anderson, M.C.; Allen, R.G.; Morse, A.; Kustas, W.P. Use of Landsat thermal imagery in monitoring evapotranspiration and managing water resources. Remote Sens. Environ. 2012, 122, 50–65. [Google Scholar] [CrossRef]
- Xiang, K.; Li, Y.; Horton, R.; Feng, H. Similarity and difference of potential evapotranspiration and reference crop evapotranspiration—A review. Agric. Water Manag. 2020, 232, 106043. [Google Scholar] [CrossRef]
- Allen, R.G.; Pereira, L.S.; Howell, T.A.; Jensen, M.E. Evapotranspiration information reporting: I. Factors governing measurement accuracy. Agric. Water Manag. 2011, 98, 899–920. [Google Scholar] [CrossRef] [Green Version]
- McMahon, T.A.; Peel, M.C.; Lowe, L.; Srikanthan, R.; McVicar, T.R. Estimating actual, potential, reference crop and pan evaporation using standard meteorological data: A pragmatic synthesis. Hydrol. Earth Syst. Sci. 2013, 17, 1331–1363. [Google Scholar] [CrossRef] [Green Version]
- Salvucci, G.D.; Gentine, P. Emergent relation between surface vapor conductance and relative humidity profiles yields evaporation rates from weather data. Proc. Natl. Acad. Sci. USA 2013, 110, 6287–6291. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fisher, J.B.; Lee, B.; Purdy, A.J.; Halverson, G.H.; Dohlen, M.B.; Cawse-Nicholson, K.; Wang, A.; Anderson, R.G.; Aragon, B.; Arain, M.A.; et al. ECOSTRESS: NASA’s Next Generation Mission to Measure Evapotranspiration From the International Space Station. Water Resour. Res. 2020, 56, e2019WR026058. [Google Scholar] [CrossRef]
- Mueller, B.; Hirschi, M.; Jimenez, C.; Ciais, P.; Dirmeyer, P.A.; Dolman, A.J.; Fisher, J.B.; Jung, M.; Ludwig, F.; Maignan, F.; et al. Benchmark products for land evapotranspiration: LandFlux-EVAL multi-data set synthesis. Hydrol. Earth Syst. Sci. 2013, 17, 3707–3720. [Google Scholar] [CrossRef] [Green Version]
- Zhang, K.; Kimball, J.S.; Running, S.W. A review of remote sensing based actual evapotranspiration estimation. Wires. Water. 2016, 3, 834–853. [Google Scholar] [CrossRef]
- Jung, M.; Koirala, S.; Weber, U.; Ichii, K.; Gans, F.; Camps-Valls, G.; Papale, D.; Schwalm, C.; Tramontana, G.; Reichstein, M. The FLUXCOM ensemble of global land-atmosphere energy fluxes. Sci. Data 2019, 6, 74. [Google Scholar] [CrossRef] [Green Version]
- Fisher, J.B.; Tu, K.P.; Baldocchi, D.D. Global estimates of the land–atmosphere water flux based on monthly AVHRR and ISLSCP-II data, validated at 16 FLUXNET sites. Remote Sens. Environ. 2008, 112, 901–919. [Google Scholar] [CrossRef]
- Vinukollu, R.K.; Wood, E.F.; Ferguson, C.R.; Fisher, J.B. Global estimates of evapotranspiration for climate studies using multi-sensor remote sensing data: Evaluation of three process-based approaches. Remote Sens. Environ. 2011, 115, 801–823. [Google Scholar] [CrossRef]
- Wang, K.; Ma, N.; Zhang, Y.; Qiang, Y.; Guo, Y. Evapotranspiration and energy partitioning of a typical alpine wetland in the central Tibetan Plateau. Atmos. Res. 2022, 267, 105931. [Google Scholar] [CrossRef]
- Pan, S.; Pan, N.; Tian, H.; Friedlingstein, P.; Sitch, S.; Shi, H.; Arora, V.K.; Haverd, V.; Jain, A.K.; Kato, E.; et al. Evaluation of global terrestrial evapotranspiration using state-of-the-art approaches in remote sensing, machine learning and land surface modeling. Hydrol. Earth Syst. Sci. 2020, 24, 1485–1509. [Google Scholar] [CrossRef] [Green Version]
- Penman, H.L. Natural evaporation from open water, bare soil and grass. Proc. R. Soc. Lond. Ser. A—Math. Phys. Sci. 1948, 193, 120–145. [Google Scholar] [CrossRef] [Green Version]
- Monteith, J.L. Evaporation and Environment. 19th Symposia of the Society for Experimental Biology; University Press: Cambridge, UK, 1965; Volume 19, pp. 205–234. [Google Scholar]
- Ramírez, J.A.; Hobbins, M.T.; Brown, T.C. Observational evidence of the complementary relationship in regional evaporation lends strong support for Bouchet’s hypothesis. Geophys. Res. Lett. 2005, 32, L15401. [Google Scholar] [CrossRef] [Green Version]
- Han, S.; Tian, F.; Hu, H. Positive or negative correlation between actual and potential evaporation? Evaluating using a nonlinear complementary relationship model. Water Resour. Res. 2014, 50, 1322–1336. [Google Scholar] [CrossRef]
- Bouchet, R.J. Evapotranspiration reelle, evapotranspiration potentielle, et production agricole. Ann. Agron. 1963, 14, 743–824. [Google Scholar]
- Ma, N.; Zhang, Y.; Xu, C.Y.; Szilagyi, J. Modeling actual evapotranspiration with routine meteorological variables in the data-scarce region of the Tibetan Plateau: Comparisons and implications. J. Geophys. Res. Biogeosci. 2015, 120, 1638–1657. [Google Scholar] [CrossRef] [Green Version]
- Ma, N.; Szilagyi, J.; Zhang, Y.; Liu, W. Complementary-Relationship-Based Modeling of Terrestrial Evapotranspiration Across China During 1982–2012: Validations and Spatiotemporal Analyses. J. Geophys. Res. Atmos. 2019, 124, 4326–4351. [Google Scholar] [CrossRef]
- Han, S.; Tian, F. A review of the complementary principle of evaporation: From the original linear relationship to generalized nonlinear functions. Hydrol. Earth Syst. Sci. 2020, 24, 2269–2285. [Google Scholar] [CrossRef]
- Brutsaert, W.; Stricker, H. An advection-aridity approach to estimate actual regional evapotranspiration. Water Resour. Res. 1979, 15, 443–450. [Google Scholar] [CrossRef]
- Morton, F.I. Operational estimates of areal evapotranspiration and their significance to the science and practice of hydrology. J. Hydrol. 1983, 66, 1–76. [Google Scholar] [CrossRef]
- Granger, R.J.; Gray, D.M. Evaporation from natural nonsaturated surfaces. J. Hydrol. 1989, 111, 21–29. [Google Scholar] [CrossRef]
- Kahler, D.M.; Brutsaert, W. Complementary relationship between daily evaporation in the environment and pan evaporation. Water Resour. Res. 2006, 42, W05413. [Google Scholar] [CrossRef]
- Szilagyi, J. On the inherent asymmetric nature of the complementary relationship of evaporation. Geophys. Res. Lett. 2007, 34, L02405. [Google Scholar] [CrossRef] [Green Version]
- Han, S.; Hu, H.; Yang, D. A complementary relationship evaporation model referring to the Granger model and the advection-aridity. Hydrol. Process. 2011, 25, 2094–2101. [Google Scholar] [CrossRef]
- Han, S.; Hu, H.; Tian, F. A nonlinear function approach for the normalized complementary relationship evaporation model. Hydrol. Process. 2012, 26, 3973–3981. [Google Scholar] [CrossRef]
- Brutsaert, W. A generalized complementary principle with physical constraints for land-surface evaporation. Water Resour. Res. 2015, 51, 8087–8093. [Google Scholar] [CrossRef] [Green Version]
- Crago, R.; Szilagyi, J.; Qualls, R.; Huntington, J. Rescaling the complementary relationship for land surface evaporation. Water Resour. Res. 2016, 52, 8461–8471. [Google Scholar] [CrossRef]
- Crago, R.D.; Qualls, R.J. Evaluation of the Generalized and Rescaled Complementary Evaporation Relationships. Water Resour. Res. 2018, 54, 8086–8102. [Google Scholar] [CrossRef]
- Szilagyi, J.; Crago, R.; Qualls, R. A calibration-free formulation of the complementary relationship of evaporation for continental-scale hydrology. J. Geophys. Res. Atmos. 2017, 122, 264–278. [Google Scholar] [CrossRef]
- Han, S.; Tian, F. Derivation of a Sigmoid Generalized Complementary Function for Evaporation With Physical Constraints. Water Resour. Res. 2018, 54, 5050–5068. [Google Scholar] [CrossRef]
- Gao, B.; Xu, X. Derivation of an exponential complementary function with physical constraints for land surface evaporation estimation. J. Hydrol. 2021, 593, 125623. [Google Scholar] [CrossRef]
- Crago, R.; Crowley, R. Complementary relationships for near-instantaneous evaporation. J. Hydrol. 2005, 300, 199–211. [Google Scholar] [CrossRef]
- Huntington, J.L.; Szilagyi, J.; Tyler, S.W.; Pohll, G.M. Evaluating the complementary relationship for estimating evapotranspiration from arid shrublands. Water Resour. Res. 2011, 47, W05533. [Google Scholar] [CrossRef] [Green Version]
- Xu, X.; Li, X.; Wang, X.; He, C.; Tian, W.; Tian, J.; Yang, L. Estimating daily evapotranspiration in the agricultural-pastoral ecotone in Northwest China: A comparative analysis of the Complementary Relationship, WRF-CLM4.0, and WRF-Noah methods. Sci. Total Environ. 2020, 729, 138635. [Google Scholar] [CrossRef]
- Zhou, H.; Han, S.; Liu, W. Evaluation of two generalized complementary functions for annual evaporation estimation on the Loess Plateau, China. J. Hydrol. 2020, 587, 124980. [Google Scholar] [CrossRef]
- Wang, L.; Han, S.; Tian, F. At which timescale does the complementary principle perform best in evaporation estimation? Hydrol. Earth Syst. Sci. 2021, 25, 375–386. [Google Scholar] [CrossRef]
- Qiu, J. China: The third pole. Nature 2008, 454, 393–396. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Duan, A.M.; Wu, G.X.; Liu, Y.M.; Ma, Y.M.; Zhao, P. Weather and climate effects of the Tibetan Plateau. Adv. Atmos. Sci. 2012, 29, 978–992. [Google Scholar] [CrossRef]
- Wu, G.; Duan, A.; Liu, Y.; Mao, J.; Ren, R.; Bao, Q.; He, B.; Liu, B.; Hu, W. Tibetan Plateau climate dynamics: Recent research progress and outlook. Natl. Sci. Rev. 2015, 2, 100–116. [Google Scholar] [CrossRef] [Green Version]
- Zou, D.; Zhao, L.; Sheng, Y.; Chen, J.; Hu, G.; Wu, T.; Wu, J.; Xie, C.; Wu, X.; Pang, Q.; et al. A new map of permafrost distribution on the tibetan plateau. Cryosphere 2017, 11, 2527–2542. [Google Scholar] [CrossRef] [Green Version]
- Cheng, G.; Wu, T. Responses of permafrost to climate change and their environmental significance, Qinghai-Tibet Plateau. J. Geophys. Res. Earth. 2007, 112, F02S03. [Google Scholar] [CrossRef] [Green Version]
- Zhao, L.; Zou, D.; Hu, G.; Du, E.; Pang, Q.; Xiao, Y.; Li, R.; Sheng, Y.; Wu, X.; Sun, Z.; et al. Changing climate and the permafrost environment on the Qinghai–Tibet (Xizang) plateau. Permafrost. Periglac. 2020, 31, 396–405. [Google Scholar] [CrossRef]
- Ma, Y.; Kang, S.; Zhu, L.; Xu, B.; Tian, L.; Yao, T. ROOF OF THE WORLD: Tibetan Observation and Research Platform. Bull. Am. Meteorol. Soc. 2008, 89, 1487–1492. [Google Scholar] [CrossRef] [Green Version]
- Ma, Y.; Ma, W.; Zhong, L.; Hu, Z.; Li, M.; Zhu, Z.; Han, C.; Wang, B.; Liu, X. Monitoring and Modeling the Tibetan Plateau’s climate system and its impact on East Asia. Sci. Rep. 2017, 7, 44574. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, G.; Lin, S.; Hu, Z.; Lu, Y.; Sun, X.; Huang, K. Improving Actual Evapotranspiration Estimation Integrating Energy Consumption for Ice Phase Change Across the Tibetan Plateau. J. Geophys. Res. Atmos. 2020, 125, e2019JD031799. [Google Scholar] [CrossRef]
- Zhao, L.; Zou, D.; Hu, G.; Wu, T.; Du, E.; Liu, G.; Xiao, Y.; Li, R.; Pang, Q.; Qiao, Y.; et al. A synthesis dataset of permafrost thermal state for the Qinghai–Tibet (Xizang) Plateau, China. Earth Syst. Sci. Data 2021, 13, 4207–4218. [Google Scholar] [CrossRef]
- Ma, Y.; Hu, Z.; Xie, Z.; Ma, W.; Wang, B.; Chen, X.; Li, M.; Zhong, L.; Sun, F.; Gu, L.; et al. A long-term (2005–2016) dataset of hourly integrated land-atmosphere interaction observations on the Tibetan Plateau. Earth Syst. Sci. Data 2020, 12, 2937–2957. [Google Scholar] [CrossRef]
- Liu, S.M.; Xu, Z.W.; Wang, W.Z.; Jia, Z.Z.; Zhu, M.J.; Bai, J.; Wang, J.M. A comparison of eddy-covariance and large aperture scintillometer measurements with respect to the energy balance closure problem. Hydrol. Earth Syst. Sci. 2011, 15, 1291–1306. [Google Scholar] [CrossRef] [Green Version]
- You, Q.; Xue, X.; Peng, F.; Dong, S.; Gao, Y. Surface water and heat exchange comparison between alpine meadow and bare land in a permafrost region of the Tibetan Plateau. Agric. For. Meteorol. 2017, 232, 48–65. [Google Scholar] [CrossRef]
- Gu, L.; Yao, J.; Hu, Z.; Zhao, L. Comparison of the surface energy budget between regions of seasonally frozen ground and permafrost on the Tibetan Plateau. Atmos. Res. 2015, 153, 553–564. [Google Scholar] [CrossRef]
- Yang, K.; Wang, J. A temperature prediction-correction method for estimating surface soil heat flux from soil temperature and moisture data. Sci. China. Ser. D Earth Sci. 2008, 51, 721–729. [Google Scholar] [CrossRef]
- Yao, J.; Zhao, L.; Gu, L.; Qiao, Y.; Jiao, K. The surface energy budget in the permafrost region of the Tibetan Plateau. Atmos. Res. 2011, 102, 394–407. [Google Scholar] [CrossRef]
- Ma, N.; Zhang, Y.; Guo, Y.; Gao, H.; Zhang, H.; Wang, Y. Environmental and biophysical controls on the evapotranspiration over the highest alpine steppe. J. Hydrol. 2015, 529, 980–992. [Google Scholar] [CrossRef]
- Parlange, M.B.; Katul, G.G. An Advection-Aridity evaporation model. Water Resour. Res. 1992, 28, 127–132. [Google Scholar] [CrossRef]
- Szilagyi, J.; Jozsa, J. New findings about the complementary relationship-based evaporation estimation methods. J. Hydrol. 2008, 354, 171–186. [Google Scholar] [CrossRef] [Green Version]
- Szilagyi, J. Temperature corrections in the Priestley–Taylor equation of evaporation. J. Hydrol. 2014, 519, 455–464. [Google Scholar] [CrossRef]
- Dai, L.; Fu, R.; Guo, X.; Ke, X.; Du, Y.; Zhang, F.; Li, Y.; Qian, D.; Zhou, H.; Cao, G. Evaluation of actual evapotranspiration measured by large-scale weighing lysimeters in a humid alpine meadow, northeastern Qinghai-Tibetan Plateau. Hydrol. Process. 2021, 35, e14051. [Google Scholar] [CrossRef]
- Wang, L.; Tian, F.; Han, S.; Wei, Z. Determinants of the Asymmetric Parameter in the Generalized Complementary Principle of Evaporation. Water Resour. Res. 2020, 56, e2019WR026570. [Google Scholar] [CrossRef]
- Han, S.; Tian, F. Research Progress of the Generalized Nonlinear Complementary Relationships of Evaporation. Adv. Earth Sci. 2021, 36, 849–861, (In Chinese with English Abstract). [Google Scholar]
- Gan, G.; Liu, Y.; Chen, D.; Zheng, C. Investigation of a non-linear complementary relationship model for monthly evapotranspiration estimation at global flux sites. J. Hydrometeorol. 2021, 22, 2645–2658. [Google Scholar] [CrossRef]
- Szilagyi, J.; Crago, R.D. Comment on “Derivation of a Sigmoid Generalized Complementary Function for Evaporation With Physical Constraints” by S. Han and F. Tian. Water Resour. Res. 2019, 55, 868–869. [Google Scholar] [CrossRef]
- Crago, R.D.; Szilagyi, J.; Qualls, R. Comment on: “A review of the complementary principle of evaporation: From the original linear relationship to generalized nonlinear functions” by Han and Tian (2020). Hydrol. Earth Syst. Sci. 2021, 25, 63–68. [Google Scholar] [CrossRef]
- Ma, N.; Zhang, Y.; Szilagyi, J.; Guo, Y.; Zhai, J.; Gao, H. Evaluating the complementary relationship of evapotranspiration in the alpine steppe of the Tibetan Plateau. Water Resour. Res. 2015, 51, 1069–1083. [Google Scholar] [CrossRef] [Green Version]
- Brutsaert, W.; Cheng, L.; Zhang, L. Spatial Distribution of Global Landscape Evaporation in the Early Twenty-First Century by Means of a Generalized Complementary Approach. J. Hydrometeorol. 2020, 21, 287–298. [Google Scholar] [CrossRef]
- Yuan, L.; Ma, Y.; Chen, X.; Wang, Y.; Li, Z. An Enhanced MOD16 Evapotranspiration Model for the Tibetan Plateau During the Unfrozen Season. J. Geophys. Res. Atmos. 2021, 126, e2020JD032787. [Google Scholar] [CrossRef]
- Han, C.; Ma, Y.; Wang, B.; Zhong, L.; Ma, W.; Chen, X.; Su, Z. Long-term variations in actual evapotranspiration over the Tibetan Plateau. Earth Syst. Sci. Data 2021, 13, 3513–3524. [Google Scholar] [CrossRef]
- Zhang, L.; Brutsaert, W. Blending the Evaporation Precipitation Ratio With the Complementary Principle Function for the Prediction of Evaporation. Water Resour. Res. 2021, 57, e2021WR029729. [Google Scholar] [CrossRef]
- Zhou, H.; Li, Z.; Liu, W. Connotation analysis of parameters in the generalized nonlinear advection aridity model. Agric. For. Meteorol. 2021, 301, 108343. [Google Scholar] [CrossRef]
- Szilagyi, J.; Crago, R.; Ma, N. Dynamic Scaling of the Generalized Complementary Relationship Improves Long-term Tendency Estimates in Land Evaporation. Adv. Atmos. Sci. 2020, 37, 975–986. [Google Scholar] [CrossRef]
- Ma, N.; Szilagyi, J. The CR of Evaporation: A Calibration-Free Diagnostic and Benchmarking Tool for Large-Scale Terrestrial Evapotranspiration Modeling. Water Resour. Res. 2019, 55, 7246–7274. [Google Scholar] [CrossRef] [Green Version]


| Sites | Variables | First Year | Second Year | ||||
|---|---|---|---|---|---|---|---|
| Warm Season | Cold Season | Annual | Warm Season | Cold Season | Annual | ||
| TGL | Rainfall | 339.5 | 54.3 | 393.8 | 355.9 | 36 | 391.9 |
| (N1 = 365; | E plus Sublimation | 362.1 | 70.9 | 433 | 364.7 | 81.6 | 446.3 |
| N2 = 360) | Epa | 537.5 | 409.7 | 947.2 | 496 | 391.1 | 887.1 |
| XDT | Rainfall | 299.3 | 65.1 | 364.4 | 200.6 | 7 | 207.6 |
| (N1 = 363; | E plus Sublimation | 312.4 | 82.9 | 395.3 | 178 | 24.4 | 202.4 |
| N2 = 185) | Epa | 515.9 | 410.1 | 926 | 309.7 | 141.9 | 451.6 |
| BJ | Rainfall a | - | - | - | 386 | 83.2 | 469.2 |
| (N1 = 363; | E plus Sublimation | 432.9 | 118.9 | 551.8 | 385.5 | 117.3 | 502.8 |
| N2 = 350) | Epa | 580.8 | 421.5 | 1002.3 | 549.8 | 363.4 | 913.2 |
| NAMORS | Rainfall b | 494.7 | 54.2 | 548.9 | 327 | 47.3 | 374.3 |
| (N1 = 337; | E plus Sublimation | 413.6 | 128.9 | 542.5 | 346 | 121.2 | 467.2 |
| N2 = 352) | Epa | 493.1 | 336.1 | 829.2 | 531.6 | 411.4 | 943 |
| Sites | Model | αe | b | c | RMSE (mm d−1) | MAE (mm d−1) | MBE (mm d−1) | NSE | R2 | Average (mm d−1) |
|---|---|---|---|---|---|---|---|---|---|---|
| TGL | K2006 | 0.88 (1.01) | 16.67 (2.41) | - | 0.457 (0.381) | 0.375 (0.31) | 0.053 (−0.056) | 0.84 (0.889) | 0.872 (0.891) | 1.24 |
| B2015 | 0.92 (1.03) | - | −1.35 (2.22) | 0.428 (0.347) | 0.338 (0.269) | 0.109 (−0.032) | 0.86 (0.908) | 0.88 (0.91) | ||
| S2017 | 1.12 (1.13) | - | - | 0.394 (0.388) | 0.279 (0.275) | −0.136 (−0.112) | 0.881 (0.885) | 0.897 (0.899) | ||
| C2018 | 1.12 (1.08) | - | - | 0.321 (0.324) | 0.244 (0.245) | 0.039 (−0.041) | 0.921 (0.919) | 0.924 (0.924) | ||
| H2018 | 0.97 (1.07) | 5.56 (1.44) | - | 0.415 (0.343) | 0.328 (0.266) | 0.128 (−0.01) | 0.868 (0.91) | 0.889 (0.91) | ||
| XDT | K2006 | 0.88 (0.94) | 16.67 (4.1) | - | 0.372 (0.375) | 0.282 (0.281) | 0.052 (−0.07) | 0.839 (0.837) | 0.845 (0.852) | 1.09 |
| B2015 | 0.92 (1.01) | - | −1.35 (1.48) | 0.388 (0.361) | 0.287 (0.268) | 0.093 (−0.049) | 0.826 (0.849) | 0.852 (0.861) | ||
| S2017 | 1.12 (1.18) | - | - | 0.486 (0.467) | 0.354 (0.341) | −0.198 (−0.07) | 0.725 (0.746) | 0.778 (0.799) | ||
| C2018 | 1.12 (1.11) | - | - | 0.37 (0.366) | 0.277 (0.274) | 0.017 (−3.4 × 10−4) | 0.841 (0.844) | 0.852 (0.852) | ||
| H2018 | 0.97 (1.04) | 5.56 (1.8) | - | 0.381 (0.36) | 0.289 (0.269) | 0.105 (−0.042) | 0.831 (0.849) | 0.856 (0.859) | ||
| BJ | K2006 | 0.88 (1.03) | 16.67 (2.63) | - | 0.609 (0.59) | 0.463 (0.439) | 0.001 (0.003) | 0.746 (0.762) | 0.748 (0.773) | 1.44 |
| B2015 | 0.92 (1.05) | - | −1.35 (2.55) | 0.604 (0.578) | 0.453 (0.42) | 0.074 (−0.031) | 0.75 (0.772) | 0.755 (0.789) | ||
| S2017 | 1.12 (1.14) | - | - | 0.544 (0.553) | 0.405 (0.41) | −0.156 (−0.092) | 0.798 (0.791) | 0.826 (0.824) | ||
| C2018 | 1.12 (1.11) | - | - | 0.531 (0.523) | 0.377 (0.371) | 0.038 (0.013) | 0.808 (0.813) | 0.819 (0.821) | ||
| H2018 | 0.97 (1.11) | 5.56 (1.17) | - | 0.596 (0.584) | 0.45 (0.421) | 0.092 (−0.013) | 0.757 (0.767) | 0.764 (0.792) | ||
| NAMORS | K2006 | 0.88 (1.13) | 16.67 (10.07) | - | 0.637 (0.601) | 0.458 (0.464) | −0.231 (0.08) | 0.704 (0.737) | 0.755 (0.757) | 1.33 |
| B2015 | 0.92 (1.17) | - | −1.35 (0.76) | 0.607 (0.585) | 0.448 (0.455) | −0.204 (−0.033) | 0.731 (0.751) | 0.764 (0.774) | ||
| S2017 | 1.12 (1.25) | - | - | 0.756 (0.653) | 0.573 (0.516) | −0.516 (−0.273) | 0.583 (0.689) | 0.78 (0.785) | ||
| C2018 | 1.12 (1.2) | - | - | 0.579 (0.549) | 0.422 (0.413) | −0.264 (−0.139) | 0.756 (0.78) | 0.806 (0.803) | ||
| H2018 | 0.97 (1.28) | 5.56 (1.72) | - | 0.601 (0.6) | 0.431 (0.475) | −0.197 (−0.041) | 0.737 (0.738) | 0.769 (0.776) |
| Site | Model | Jan | Feb | Mar | Apr | May | Jun | Jul | Aug | Sep | Oct | Nov | Dec | Warm Season | Cold Season | Year |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| TGL | K2006 | 0.06 | 4 | 13.07 | 14.84 | 4.91 | −4.75 | −9.91 | −9.49 | −8.03 | −10.39 | −9.12 | −5.21 | −27.27 | 7.25 | −20.02 |
| B2015 | 1.65 | 4.49 | 12.01 | 12.54 | 4.18 | −2.86 | −7.55 | −7.9 | −7.44 | −11.54 | −8.36 | −0.59 | −21.57 | 10.2 | −11.37 | |
| S2017 | 0.22 | 0.2 | 3.35 | 1.15 | −0.32 | 0.69 | −3.77 | −7.25 | −6.33 | −15.3 | −10.06 | −2.86 | −16.98 | −23.3 | −40.28 | |
| C2018 | 2.23 | 4.28 | 9.02 | 8.33 | 3.09 | −2.08 | −7.31 | −8.68 | −6.21 | −9.51 | −7 | −0.8 | −21.19 | 6.55 | −14.64 | |
| H2018 | 1.94 | 5.12 | 12.38 | 12.61 | 4.51 | −1.67 | −6.21 | −6.76 | −6.72 | −11.41 | −7.85 | 0.3 | −16.85 | 13.09 | −3.76 | |
| XDT | K2006 | −2.54 | −3.19 | 1.47 | - | 1.12 | 2.48 | −4.26 | −0.27 | −0.52 | - | −3.41 | −3.77 | −1.45 | −11.44 | −12.89 |
| B2015 | −1.68 | −3.06 | 1.02 | - | −0.37 | 2.82 | −3.92 | 1.81 | 0.88 | - | −3.07 | −3.42 | 1.22 | −10.21 | −8.99 | |
| S2017 | −3.4 | −5.35 | −0.52 | - | −4.28 | 2.02 | −5.42 | 5.8 | 5.67 | - | −3.29 | −4.2 | 3.79 | −16.76 | −12.97 | |
| C2018 | −0.28 | −1.55 | 1.19 | - | −0.61 | 3.76 | −5.01 | 3.93 | 3.9 | - | −2.52 | −2.87 | 5.97 | −6.03 | −0.06 | |
| H2018 | −1.15 | −2.54 | 1.17 | - | −0.65 | 2.66 | −4.04 | 1.87 | 1.05 | - | −2.99 | −3.23 | 0.89 | −8.74 | −7.85 | |
| BJ | K2006 | −3.07 | −0.8 | 8.06 | 10.73 | −1.25 | 4.63 | −11.72 | 14.75 | −5.06 | −5.56 | −6.92 | −2.66 | 1.35 | −0.22 | 1.13 |
| B2015 | −3.13 | −1.77 | 5.08 | 5.79 | −3.9 | 4.11 | −9.11 | 14.12 | −4.22 | −6.35 | −9.28 | −2.25 | 1 | −11.91 | −10.91 | |
| S2017 | −5.14 | −4.91 | −0.5 | −0.89 | −7.31 | 0.89 | −2.93 | 10.88 | −0.86 | −6.06 | −11.33 | −3.98 | 0.67 | −32.81 | −32.14 | |
| C2018 | −2.66 | 0.25 | 4.02 | 8.24 | −2.22 | 3.27 | −6.72 | 13.17 | −1.86 | −4.37 | −6.23 | −0.48 | 5.64 | −1.23 | 4.41 | |
| H2018 | −2.99 | −1.92 | 4.54 | 4.86 | −3.48 | 5.65 | −6.55 | 15.5 | −2.68 | −5.9 | −9.49 | −2.21 | 8.44 | −13.11 | −4.67 | |
| NAMORS | K2006 | −7.92 | 0.73 | 6.17 | 22.71 | 18.67 | 15.86 | 5.41 | −18 | −2.06 | −2.72 | −5.96 | −4.59 | 19.88 | 8.42 | 28.3 |
| B2015 | −10.5 | −3.52 | 1.7 | 15.62 | 14.63 | 12.23 | 3.11 | −16.32 | −6.57 | −8.49 | −8.37 | −5.12 | 7.08 | −18.68 | −11.6 | |
| S2017 | −13.01 | −8.75 | −4.44 | −2.77 | 2.54 | 0.67 | −3.92 | −15.14 | −16.21 | −17.58 | −11.14 | −6.4 | −32.06 | −64.09 | −96.15 | |
| C2018 | −10.07 | −4.58 | 0.08 | 6.63 | 8.45 | 5.21 | −1.21 | −19.3 | −10.15 | −11.23 | −8.03 | −4.66 | −17 | −31.86 | −48.86 | |
| H2018 | −11.56 | −5.13 | 0.77 | 14.39 | 15.45 | 13.3 | 4.75 | −15.05 | −6.28 | −9.85 | −9.5 | −5.7 | 12.17 | −26.58 | −14.41 |
| Site | Model | Calibrated Period by Warm Season | Calibrated Period by Whole Year | ||||||
|---|---|---|---|---|---|---|---|---|---|
| αe | b | c | NSE | αe | b | c | NSE | ||
| TGL | K2006 | 1.02 | 2.49 | - | 0.759 | 1.01 | 2.41 | - | 0.73 |
| B2015 | 1 | - | 0.8 | 0.764 | 1.03 | - | 2.22 | 0.752 | |
| S2017 | 1.12 | - | - | 0.559 | 1.13 | - | - | 0.581 | |
| C2018 | 1.08 | - | - | 0.731 | 1.08 | - | - | 0.731 | |
| H2018 | 1.01 | 2.58 | - | 0.764 | 1.07 | 1.44 | - | 0.762 | |
| XDT | K2006 | 1 | 2.93 | - | 0.657 | 0.94 | 4.1 | - | 0.669 |
| B2015 | 0.98 | - | 0.38 | 0.656 | 1.01 | - | 1.48 | 0.657 | |
| S2017 | 1.18 | - | - | 0.376 | 1.18 | - | - | 0.376 | |
| C2018 | 1.12 | - | - | 0.607 | 1.11 | - | - | 0.617 | |
| H2018 | 0.99 | 3.03 | - | 0.657 | 1.04 | 1.8 | - | 0.65 | |
| BJ | K2006 | 1.11 | 1.13 | - | 0.323 | 1.03 | 2.63 | - | 0.357 |
| B2015 | 1.1 | - | 4.66 | 0.294 | 1.05 | - | 2.55 | 0.361 | |
| S2017 | 1.14 | - | - | 0.429 | 1.14 | - | - | 0.429 | |
| C2018 | 1.11 | - | - | 0.466 | 1.11 | - | - | 0.466 | |
| H2018 | 1.17 | 0.75 | - | 0.27 | 1.11 | 1.17 | - | 0.341 | |
| NAMORS | K2006 | 1.18 | 3.81 | - | 0.577 | 1.13 | 10.07 | - | 0.58 |
| B2015 | 1.19 | - | 1.01 | 0.577 | 1.17 | - | 0.76 | 0.588 | |
| S2017 | 1.25 | - | - | 0.492 | 1.25 | - | - | 0.492 | |
| C2018 | 1.2 | - | - | 0.622 | 1.2 | - | - | 0.622 | |
| H2018 | 1.35 | 1.03 | - | 0.525 | 1.28 | 1.72 | - | 0.573 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shang, C.; Wu, T.; Ma, N.; Wang, J.; Li, X.; Zhu, X.; Wang, T.; Hu, G.; Li, R.; Yang, S.; et al. Assessment of Different Complementary-Relationship-Based Models for Estimating Actual Terrestrial Evapotranspiration in the Frozen Ground Regions of the Qinghai-Tibet Plateau. Remote Sens. 2022, 14, 2047. https://doi.org/10.3390/rs14092047
Shang C, Wu T, Ma N, Wang J, Li X, Zhu X, Wang T, Hu G, Li R, Yang S, et al. Assessment of Different Complementary-Relationship-Based Models for Estimating Actual Terrestrial Evapotranspiration in the Frozen Ground Regions of the Qinghai-Tibet Plateau. Remote Sensing. 2022; 14(9):2047. https://doi.org/10.3390/rs14092047
Chicago/Turabian StyleShang, Chengpeng, Tonghua Wu, Ning Ma, Jiemin Wang, Xiangfei Li, Xiaofan Zhu, Tianye Wang, Guojie Hu, Ren Li, Sizhong Yang, and et al. 2022. "Assessment of Different Complementary-Relationship-Based Models for Estimating Actual Terrestrial Evapotranspiration in the Frozen Ground Regions of the Qinghai-Tibet Plateau" Remote Sensing 14, no. 9: 2047. https://doi.org/10.3390/rs14092047
APA StyleShang, C., Wu, T., Ma, N., Wang, J., Li, X., Zhu, X., Wang, T., Hu, G., Li, R., Yang, S., Chen, J., Yao, J., & Yang, C. (2022). Assessment of Different Complementary-Relationship-Based Models for Estimating Actual Terrestrial Evapotranspiration in the Frozen Ground Regions of the Qinghai-Tibet Plateau. Remote Sensing, 14(9), 2047. https://doi.org/10.3390/rs14092047