Range-Ambiguous Clutter Suppression via FDA MIMO Planar Array Radar with Compressed Sensing

Abstract

Range-ambiguous clutter is an inevitable issue for airborne forward-looking array radars, especially with the high pulse repetition frequency (PRF). In this paper, a method to suppress the range-ambiguous clutter is proposed in an FDA-MIMO radar with a forward-looking planar array. Compressed sensing FDA technology is used to suppress the range-ambiguous clutter and the forward-looking non-uniformity short-range clutter of radar. Specifically, first, the range ambiguous clutter in different regions is separated by the characteristics of the planar array radar elevation dimension and FDA radar range coupling. Meanwhile, regarding the issue of the FDA radar main lobe moving between coherent pulses, a main lobe correction (MLC) algorithm proposes a solution for the issue, where the FDA radar cannot coherently accumulate signals in the case of non-full angle illumination. Finally, compressed sensing technology and elevation dimension filtering are utilized to suppress the range ambiguous clutter at the receiver, with the approach alleviating the range dependence of clutter in the observation region. A small number of clutter snapshots can obtain an approximately ideal clutter covariance matrix through compressed sensing sparse recovery. The method not only reduces the number of training samples, but also overcomes the problem of clutter non-uniformity in the forward-looking array. Therefore, the clutter suppression problems faced by the high repetition frequency airborne radar forward-looking array structure are solved. At the analysis stage, a comparison among the conventional MIMO and FDA methods is carried on by analyzing the improvement factor (IF) curves. Numerical results verify the effectiveness of the proposed method in range-ambiguous clutter suppression.

1. Introduction

When an airborne radar detects ground targets in the downward-looking mode, the strong ground clutter power will submerge the target and it is difficult to detect the moving target. Therefore, the clutter suppression of airborne radar is the core issue in radar target detection. Space-time adaptive processing (STAP) technology has advantages and shows considerable development in solving airborne radar clutter suppression; it has been used in a variety of early warning radars. It requires training samples that meet the conditions of independent and identical distribution (IID), the number of which is larger than or equal to twice the radar degrees of freedom [1,2]. However, according to actual requirements, the radar array is not necessarily consistent with the flight direction of the carrier platform. There is a particular angle between the flight direction and the radar array plane. When the included angle is 90 degrees, the radar changes from a side view array to a forward-looking array configuration. For example, most fire control radars are forward-looking array structures. Under this array structure, the relationship between Doppler and angular frequencies, corresponding to clutter at different ranges, varies with the change in range. Therefore, it is a challenge for training samples obtained by radar to be independent and identically distributed. It is difficult to obtain the covariance matrix for estimating the desired range cell. Furthermore, for high-speed moving platforms, radars generally choose high PRF to avoid Doppler overlap, but high PRF means that the range-ambiguous number increases within the same main lobe coverage range [3]. Short-range clutter and long-range clutter will pollute more echo power detection areas. Clutter range dependence and range ambiguous clutter are the two problems faced by forward-looking array radar clutter suppression. Here, we aim to address the issue that the relationship between clutter Doppler and angular frequencies of the forward-looking array configuration changes with the range. In order to ensure that the STAP method can obtain a sufficient number of independent and identically distributed training samples, a series of methods to reduce the clutter range dependence have been developed to compensate for the non-uniformity of echoes between different range cells, such as the Doppler compensation method [4], the registration-based compensation method [5], and the joint space-time interpolation method [6]. These methods basically compensate the echo signal in the Doppler domain or angle Doppler two-dimensional domain to align the echo of the training sample with the echo of the cell under test to be detected, which can effectively compensate the clutter range dependence for one single clutter area. However, when there are exit clutters from multiple range ambiguous regions at the same time, the clutter range dependence in different regions is different. The short-range clutter Doppler frequency changes rapidly with the range and the long-range clutter Doppler frequency changes slowly with range. The range dependence of short-range clutter is more serious than that of long-range clutter [7]. When these range compensation methods compensate short-range clutter, the long-range clutter will be overcompensated, and the clutter extending will be aggravated. Therefore, range dependence compensation methods of single clutter area cannot take into account that of multiple range ambiguous clutter areas at the same time.

To solve the problem, some studies have proposed using three-dimensional STAP processing of elevation array elements for clutter suppression [8,9,10], but the algorithm complexity is high and the increased degree of freedom also involves more requirements for the number of STAP IID training samples. In order to solve the range-ambiguous of the clutter, a direct data domain method (D3) is proposed in the article [11], which divides single snapshot data of a large dimension into multiple data of small dimensions by sliding a window on single snapshot data, so as to obtain enough training samples that meet the IID condition. This method sacrifices radar aperture, which will result in performance loss for subsequent signal parameter estimation and clutter suppression.

A new radar system, frequency diverse array (FDA) radar, is proposed in articles [12,13,14] by Paul Antonik and his team. By introducing a linear frequency increment between the emitting array elements that is much smaller than the carrier frequency, the radar produces a time-varying transmission beampattern, and the target echo has a range coupled phase term. The articles [15,16,17,18,19] study the flexible beam of FDA radar and its transmitting pattern. Multiple-input multiple-output (MIMO) radar separates different transmission channels by transmitting mutually orthogonal waveforms. At the same time, the degree of freedom of the transmission dimension is obtained. The combination of FDA and MIMO technology at the receiving end can separate the transmitted signals of FDA with different carrier frequencies from one another [20,21,22,23]. In article [24], it is proposed to use an FDA radar range dependent beampattern to suppress range-ambiguous clutter in order to improve the performance of the forward-looking array radar STAP method. The article [25] uses the FDA radar range coupling characteristics to separate the clutter of different range ambiguous regions in the emission domain to achieve forward-looking array clutter suppression, but the effect of clutter separation is affected by the range ambiguous clutter number and frequency increment parameters.

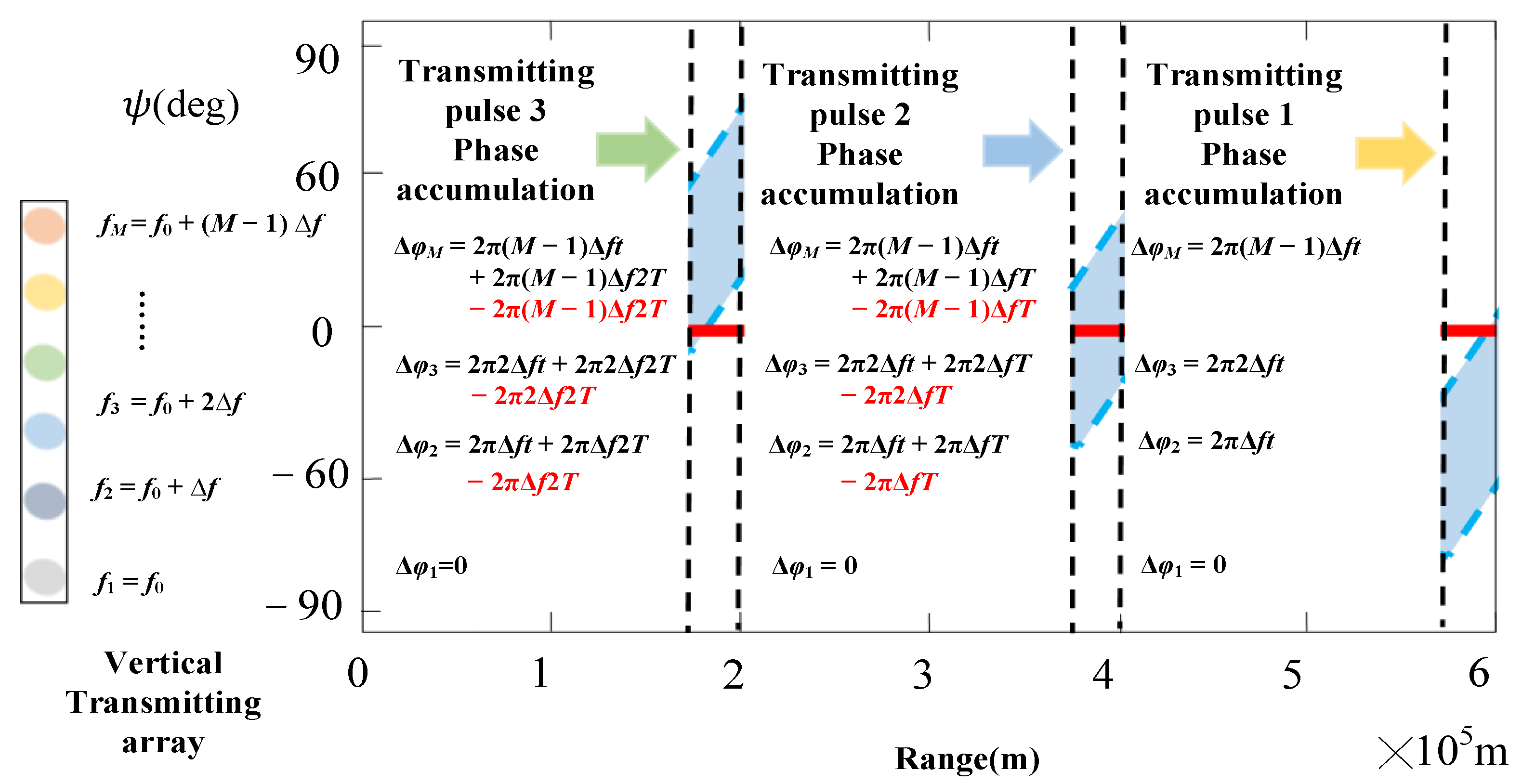
However, most studies have little analysis on the influence of the variation of the FDA radar transmitting beam gain between different pulses and the main lobe illumination angle on the target signal-to-noise ratio (SNR) under the pulse system. Generally, FDA radar uses the S-shaped main lobe at the transmitting end for full angle irradiation. All angle areas have the same high gain irradiation, resulting in strong clutter or jamming of the undesired angle area entering the receiving end through the receiving beampattern side lobe. Using full angle irradiation within the pulse width means loss of radar power and a low signal-to-noise ratio. Therefore, we study FDA radar in non-full angle irradiation mode. FDA radar can provide an extra degree of freedom in the range dimension which gives it an advantage in handling problems, in comparison with conventional radars. However, it also has a problem in the transmitting beampattern. Under the pulse system of non-full angle irradiation, the main lobe of the FDA radar transmitting beampattern within the pulse width has range coupling characteristics, hence exhibiting an S-shaped beampattern [26]. The main lobe of FDA beampattern with range coupling will illuminate different angle regions between coherent pulses. In conventional pulse framework radar, carrier frequency f₀ will form phase accumulation within pulse repetition period T. for the k-th pulse, the accumulation is 2πf₀(k−1)T. Therefore, the product of f₀ and pulse period T, in pulse radar, is set as an integer, which is called the system initial phase locking condition, so as to ensure that different pulse main lobes illuminate the same angle. For FDA radar, which has Δf frequency deviation between emitting array elements, after a pulse, the phase difference of adjacent array elements φ = 2πΔfT. Therefore, the initial phases of different pulse accumulations are different. The main lobe of the radar pattern irradiates different angle zones in different coherent pulses; this is the main lobe moving problem of FDA radar. The coherent accumulation of the same target cannot be carried out within the coherent processing interval (CPI) and the subsequent signal processing, such as clutter cancellation.

In addition, STAP technology cannot obtain training samples that meet the IID requirements for the configuration of forward-looking array radar. Compressed sensing [27] technology can make use of the sparsity of data in different transformation dimensions, reducing the number of radar samples, or alleviating the requirement of STAP for more than twice the number of radar training sample degrees of freedom. Article [28] demonstrates that the radar clutter power spectrum is sparse. In the spatial Doppler two-dimensional power spectrum, clutter only occupies a few positions in the spectrum, and is therefore sparse. A small amount of signal snapshots is used to recover clutter and targets. Article [29], combining compressed sensing with an STAP algorithm, uses sparse recovery to obtain the training samples that meet the requirements of STAP, and then constructs the covariance matrix through the training samples to calculate the optimal filtering weight. Article [30] studies the sparse recovery STAP method. It determines the support set of sparse recovery clutter through a variety of norm calculations, then recovers the clutter data according to the sparse dictionary, constructs the clutter power covariance matrix, and then suppresses the clutter.

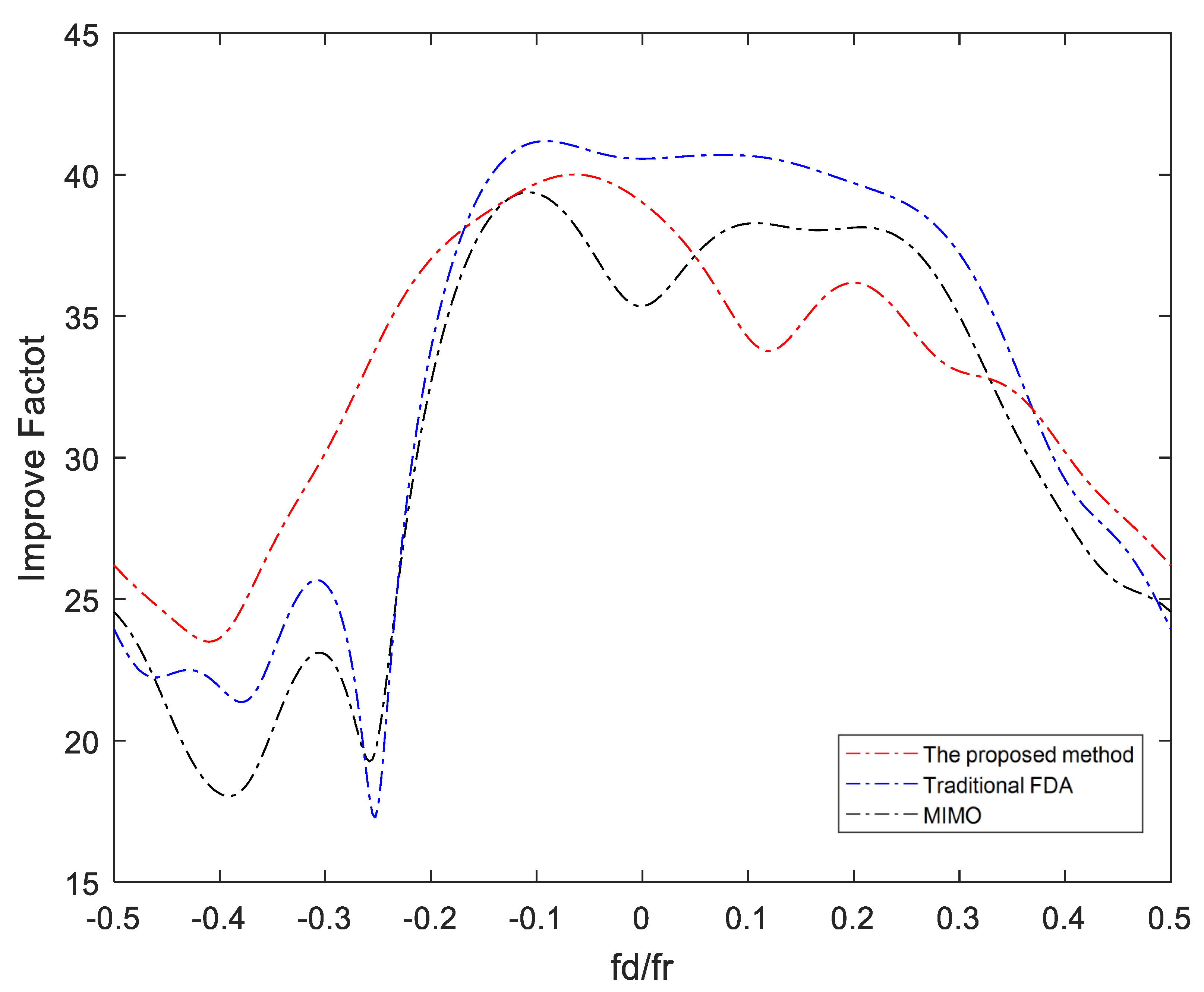
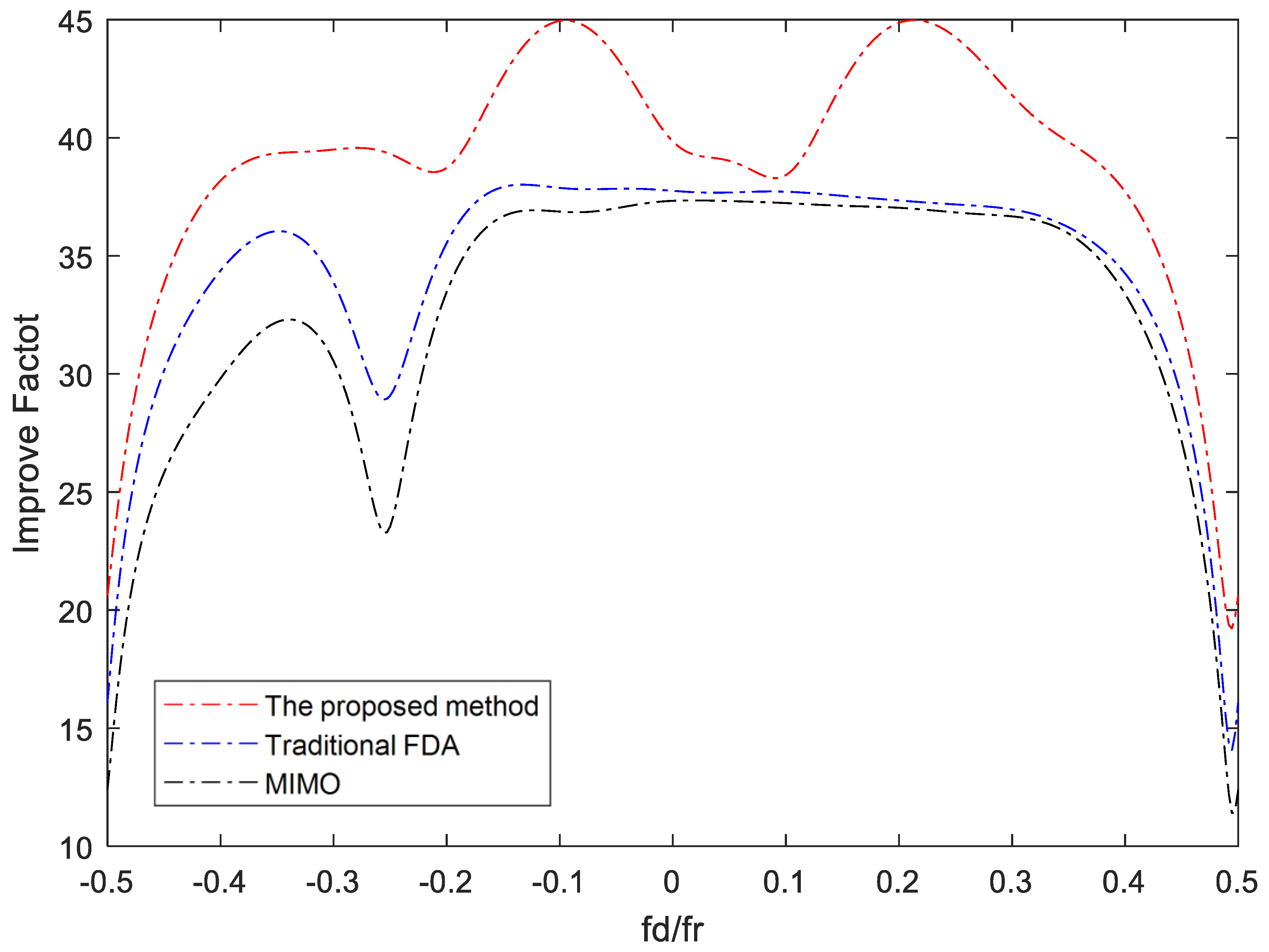
Regarding the clutter ambiguous issue of airborne forward-looking array radar, this paper proposes an approach for resolving clutter ambiguous via FDA-MIMO with main lobe rectification and compressed sensing. This method is implemented under the framework of planar array radar. By transmitting FDA waveform in elevation dimension and adding a main lobe moving rectification vector to the transmitted signal, the radar can adjust the irradiation angle area of the main lobe between coherent pulses while using the freedom of the FDA range dimension, so that the desired target angle position can be irradiated by different pulse main lobes. After receiving the signal, the signals of different channels are mixed and separated through an MIMO orthogonal waveform, and then through signal compensation. Because the clutter of different areas has different ranges, FDA range coupling characteristics can separate them. The range coupled clutter is filtered out through the elevation dimension of the planar array. The filtered clutter snapshot is range dependent. It is difficult to obtain samples that meet the IID conditions by STAP method. Furthermore, it is observed that the clutter range gate has sparse characteristics in the angle Doppler two-dimensional power spectrum. Therefore, the power spectrum dictionary required for compressed sensing is constructed by discretizing the whole two-dimensional power spectrum. Then, by selecting a small number of snapshots around the range gate to be measured for sparse processing, the clutter support set approximate to a single range gate can be obtained, followed by recovery ofs the clutter covariance matrix according to the dictionary and support set, so as to overcome the issue of power spectrum clutter spread due to non-uniform clutter snapshot data.

Finally, the problems of range-ambiguous clutter and clutter range dependence of forward-looking array radar are solved. Compared with the performances of conventional planar array MIMO radar and FDA radar, simulation results prove the effectiveness of the proposed method. The innovations of this method are summarized as follows:

(1)

In view of the range dependence of the forward-looking array radar clutter, this paper uses compressed sensing technology to divide the dictionary grid and construct a sparse dictionary according to the characteristics of the forward-looking radar clutter steering vector in the spatial Doppler two-dimensional power spectrum structure. Combined with the FDA technology to resist the range ambiguous clutter, this paper overcomes the problem that the conventional forward-looking array multiple range ambiguous clutters lack sparsity. The clutter is transformed into a sparsely recoverable target. A small number of echo snapshots are received near the detection range gate through compressed sensing to reconstruct the clutter covariance matrix, which is not approximately affected by the range dependence. It solves the contradiction that the conventional STAP requires both training samples to meet the IID condition and the selection of enough samples. Especially when the forward-looking clutter range dependence of training samples is serious, this method improves the performance of radar clutter spreading and clutter suppression and the moving target detection ability of forward-looking array radar.

(2)

Aiming to address the issue of main lobe moving in the transmitting pattern of FDA MIMO pulse radar, the main lobe rectification method of equivalent transmitting pattern is proposed. During the same coherent processing interval, the initial phase of an FDA radar pulse is compensated and restored at the transmitting and receiving ends, respectively. Although the coherent processing pulse irradiates different angle areas, they are all able to contain the desired target angle, so that the coherent processing pulses can irradiate the same target, improving the signal-to-noise ratio and completing the coherent accumulation. On the basis of this, the subsequent signal processing of pulse framework FDA radar is carried out.

(3)

Addressing the clutter ambiguous issue of airborne forward-looking array radar, clutters from different ranges have different power spectrum distributed characteristics because of the phase term of range coupling by combining FDA radar and the elevation dimension freedom of planar array. Different from the conventional ambiguous clutter, this method makes the target signal and clutter of the desired region maintain high gain, while the range-ambiguous clutter of the undesired region has a wide area low gain distribution, which is suppressed. Combined with clutter range dependent compensation technology, radar target parameter searching is realized.

2. MLC-FDA Signal Model

2.1. Transmitting of MLC-FDA Radar

In this paper, we will discuss the range-ambiguous clutter suppression method of airborne forward-looking planar array radar. The geometry of FDA radar is shown in Figure 1a, which is in the right-hand coordinate system. The radar pulse-repetition interval is T = 1/f_PRF, where f_PRF is the pulse repetition frequency. The number of coherent processing pulses is K. The speed of the radar platform is V, which is parallel to the y-axis. The planar array is perpendicular to the speed direction, and the height of the platform is H. The azimuth, elevation and spatial cone angle corresponding to the clutter block are expressed as φ, θ, ψ, respectively. Consequently, we have $\cos \psi =\cos \theta ·\cos \phi$. The planar array is M × N-dimensional array element distribution, in which the carrier frequencies transmitted by each row are the same, and the transmission waveforms of different rows keep orthogonality to each other, as shown in Figure 1b. The carrier frequencies emitted by the first to M-th array elements in the same column are shown in the following equation:

f_m = f₀ + (m−1)∆f m = 1,2,…, M,

(1)

where ∆f is the frequency increment between adjacent rows. It must keep much smaller than the carrier frequency f₀. It should be noted that the large value size of ∆f will have the effect of decoherence of the target reflection coefficient [31,32].

The corresponding normalized Doppler frequency, normalized elevation vertical spatial frequency and azimuth horizontal spatial frequency are, respectively, expressed as:

$$\{\begin{array}{l}{f}_d{}_m=\frac{2v}{c{f}_{PRF}}\cos \psi f_m\\ f_v{}_m=\frac{d}{c}\sin \phi f_m\\ f_h{}_m=\frac{d}{c}\cos \phi \sin \theta f_m.\end{array}$$

(2)

The signal transmitted by the array element in row m can be expressed as:

$$s_m=\mathrm{rect}(\frac{t}{T_p}){\Phi }_m(t)e^{j2\pi f_{m}t},$$

(3)

where ${\Phi }_m(t)$ represents the orthogonal waveform corresponding to row m-th. Here, discussion is based on an ideal orthogonal waveform premise. Therefore, the conditions satisfied are:

$$\{\begin{array}{l}{\displaystyle \underset{T_p}{\int }{\Phi }_p(t){\Phi }_q^{*}(t-\tau )dt=0,q\ne p}\\ {\displaystyle \underset{T_p}{\int }{\Phi }_p(t){\Phi }_q^{*}(t-\tau )dt=1,q=p,}\end{array}$$

(4)

where $\tau$ represents time delay. $\forall q,p=1,2,\dots ,M$. The conventional pulse system FDA radar pattern is characterized by S-shaped moving within the pulse width. When the pulse width is not large enough, the high gain irradiated zone of its main lobe is limited and the full angle coverage of [−90, 90] cannot be realized. Due to the frequency increment between array elements, the initial phases accumulated by each array element are also different after a pulse period, T. As a result, because each pulse has different initial phases, the high gain irradiated angular region of the main lobe within the pulse width is also different, i.e., the main lobe moving problem of FDA radar. Therefore, it is impossible to irradiate the target at the same angle with high gain within the coherent processing interval, let alone carry out signal processing such as coherent accumulation or clutter cancellation for the same target. The radar cannot complete its normal operation. Figure 2 is the schematic diagram of FDA radar range (time domain)-angle two-dimensional transmitting pattern. The red 0 degree is the desired target position. In the figure, three pulses transmitted by the radar are drawn. The gray box represents the pulse width, the main lobe distribution of FDA radar pattern in the box is represented by a blue dotted line, and the initial phase of the array element of the three pulses is also marked. In the figure, ∆f = 1/(2T). We note that the range of the main lobe irradiation angle of the first pulse pattern is [−30, 0], while each array element of the second pulse accumulates the initial phase of 2π∆fT(m−1), compared with the first pulse. M represents the array element serial number. The zone of the main lobe irradiation angle of the second pulse becomes [−60, −90] and [70, 90]. The accumulated phase of the third pulse accumulation is 2π∆f2T(m−1). As a result of ∆f = 1/(2T) in the figure, the initial phase of the third pulse accumulation is an integral multiple of 2π. Therefore, the third pulse and the first pulse have the same main lobe irradiation zone.

In this paper, the phase weighting between pulses of transmitting array elements is used to adjust the beampattern main lobe position, so as to have a solution of main lobe moving of FDA radar. When transmitting the signal, each array element adds the main lobe moving correction weight, corresponding to pulses. Thus, the issue that the main lobe of coherent pulses cannot irradiate the target due to the accumulation of ∆f phase is adjusted, as shown in Figure 3, and the angle areas of the main lobe of the corrected FDA pulse pattern can irradiate the same target. In the coherent accumulation time, the radar can achieve high gain illumination of the same target. The red correction phase part in the figure shows the effect of the main lobe correction weight after the array element is weighted. In the weighted intra pulse pattern, the first pulse main lobe is irradiated in the area of [−30, 0], the second pulse is irradiated in the area of [−20, 20], and the third pulse main lobe is irradiated in the area of [−5, 38]. Three pulse main lobes all cover the desired target position, 0 degree.

The main lobe rectification weighting vector added to each array element is constructed as follows when transmitting signals:

$$\begin{array}{l}{\overline{\mathit{g}}}_C\\ =\left[\begin{array}{cccc}{g}_{11}& g_{12}& \dots & g_{1K}\\ g_{21}& g_{22}& \dots & g_{2K}\\ ⋮& ⋮& \ddots & ⋮\\ g_{M1}& g_{M2}& \cdots & g_{MK}\end{array}\right]\\ =\left[\begin{array}{ccc}{e}^{-j2\pi (1-1)\Delta f(1-1+z_0)/f_{PRF}}& \cdots & e^{-j2\pi (1-1)\Delta f(K-1+z_{K-1})/f_{PRF}}\\ ⋮& e^{-j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}}& ⋮\\ e^{-j2\pi (M-1)\Delta f(1-1+z_0)/f_{PRF}}& \cdots & e^{-j2\pi (M-1)\Delta f(K-1+z_{K-1})/f_{PRF}}\end{array}\right],\end{array}$$

(5)

where $\otimes$ represents the Kronecker product, ${\overline{\mathit{g}}}_C\in {ℂ}^{MK\times 1}$, ${\overline{\mathit{g}}}_C(mk)=e^{-j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}}$ and z_k−1 are the compensation coefficient corresponding to the k-th pulse, which determine the main lobe movement of the k-th pulse relative to that of the first pulse. Here, we take z_k−1 = (k−1)x/180, with x the offset coefficient to reflect the angle difference of the main lobe illumination area of the adjacent pulse beampattern (since the FDA pattern is S-shaped, there is a case where individual pulse positions z_k−1 need to be adjusted nonlinearly). Substitute the above equation into Equation (3), and the signal of the k-th pulse transmitted by the m-th array element reaching the clutter block can be written as:

$$\begin{array}{c}{\tilde{s}}_{mk}(t)\\ =\epsilon \mathrm{rect}[(t-{\tau }_r/2)/T_p]{\Phi }_m(t-{\tau }_r/2)\\ ·\exp \{j2\pi f_m(t-{\tau }_r/2-{\tau }_{sm})+j2\pi {\tilde{f}}_{dmt}t-j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}\}+{\tilde{n}}_c,\end{array}$$

(6)

where $\epsilon$ is the product of target scattering coefficient and signal propagation gain. ${\tau }_r=2R/c$, being a signal reaching the clutter block, the range delay term only produces one-way delay ${\tau }_r/2$; ${\tau }_{sm}=(m-1)d\sin \phi /c=(m-1)f_{hm}/f_m$ is the phase delay term generated by the spatial position of the array element. c represents the speed of light and ${\tilde{n}}_c$ is the Gaussian white noise in the propagation process.$\cos \psi =\cos \theta ·\cos \phi$, ${\tilde{f}}_{dm}=2v\cos \psi f_m/c$ represents the Doppler frequency, corresponding to f_m generated by platform motion. In Equation (6), it is only affected by the velocity of the transmitting array, so we have ${\tilde{f}}_{dmt}=v\cos \psi f_m/c$. Since the radar transmission is a narrow-band signal, there are ${\Phi }_m(t-{\tau }_t-{\tau }_{sm})\approx {\Phi }_m(t-{\tau }_t)$, $\mathrm{rect}[(t-{\tau }_r/2-{\tau }_{sm})/T_p]\approx \mathrm{rect}[(t-{\tau }_r/2)/T_p]$. The last term $-j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}$ in the above equation is the compensation term substituted into the transmitted signal Equation (3) by main lobe correction weighting, which corresponds to the red correction phase part in Figure 3.

2.2. Receiving of MLC-FDA Radar

After being reflected by the clutter block, the k-th pulse echo signal received by the n-th array element can be written as:

$$\begin{array}{c}{s}_{nk}(t)\\ =\epsilon {\displaystyle \sum _{m=1}^M{\tilde{s}}_{mk}(t)}+{\tilde{n}}_c\\ =\epsilon {\displaystyle \sum _{m=1}^M\{\mathrm{rect}[(t-{\tau }_r)/T_p]}{\Phi }_m(t-{\tau }_r)\\ \begin{array}{r}·\exp (j2\pi f_m(t-{\tau }_r-{\tau }_{sm}-{\tau }_{sn})+j2\pi {\tilde{f}}_{dm}t\\ -j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF})\end{array}\}+{\tilde{n}}_c,\end{array}$$

(7)

where ${\tau }_{sn}=\sin \theta \cos \phi (n-1)d/c=(n-1)f_v/f_m$. It can be seen from the above equation that the n-th receiving array element receives the superimposed signal ${\displaystyle \sum _{m=1}^M{\tilde{s}}_{mk}(t)}$ of M-channel transmitting array elements. After the superimposed signals enter the receiver, it is necessary to separate the signals of each channel, and then carry out subsequent signal processing. The processing process is shown in Figure 4. After the received signal reaches each receiving array element, it is presented as s_n. Each channel is separated into M channels through matched filtering and down conversion. Therefore, the received signal is changed from N channels to MN channels. After adding a total of K coherent pulses, the received data is MNK dimension.

The signal of the k-th pulse, emitted by the m-th one and received by the n-th element, is presented as:

$$\begin{array}{l}{s}_{mnk}\\ =\epsilon \exp \left\{\begin{array}{l}j2\pi f_m\left(-\frac{R-\sin \phi (m-1)d}{c}-\frac{R-\cos \phi \sin \theta (n-1)d}{c}\right)\\ +j2\pi (k-1){\tilde{f}}_{dm}/f_{PRF}-j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}\end{array}\right\}+{\tilde{n}}_c\\ =\epsilon \exp \left\{\begin{array}{l}-j2\pi [(m-1)\Delta f+f_0]\frac{2R}{c}\\ +j2\pi [(m-1)\Delta f+f_0]\left(\frac{\sin \phi (m-1)d}{c}+\frac{\cos \phi \sin \theta (n-1)d}{c}\right)\\ +j2\pi [\Delta f_{dm}+f_{d0}](k-1)-j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}\end{array}\right\}+{\tilde{n}}_c\\ =\mu \epsilon \exp \left\{\begin{array}{l}-j2\pi \frac{2R}{c}(m-1)\Delta f\\ +j2\pi [(m-1)f_v+(m-1)\Delta f_{vm}+(n-1)f_h+(n-1)\Delta f_{hm}]\\ +j2\pi (k-1)[\Delta f_{dm}+f_{d0}]-j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}\end{array}\right\}+{\tilde{n}}_c,\end{array}$$

(8)

where

$$\{\begin{array}{l}\mu =\exp \left[-j2\pi f_0\frac{2R}{c}\right]\\ f_d{}_0=\frac{2v}{c{f}_{PRF}}\cos \psi f_0\\ \Delta f_d{}_m=\frac{2v}{c{f}_{PRF}}\cos \psi (m-1)\Delta f\\ f_v=\frac{d}{c}\sin \phi f_0\\ \Delta f_{vm}=\frac{d}{c}\sin \phi (m-1)\Delta f\\ f_h=\frac{d}{c}\cos \phi \sin \theta f_0\\ \Delta f_{hnm}=\frac{d}{c}\cos \phi \sin \theta (m-1)\Delta f.\end{array}$$

(9)

Since Equation (1) ∆f << f₀, we have:

$$\{\begin{array}{l}\frac{\Delta f_d{}_m}{f_d{}_0}=\frac{(m-1)\Delta f}{f_0}\le \frac{(M-1)\Delta f}{f_0}\ll 1\\ \frac{\Delta f_v{}_m}{f_v}\le \frac{(M-1)\Delta f}{f_0}\ll 1\\ \frac{\Delta f_h{}_{nm}}{f_h}\le \frac{(M-1)\Delta f}{f_0}\ll 1.\end{array}$$

(10)

The phase term containing ∆f in the FDA expression can be simplified to:

$$\begin{array}{l}{s}_{mnk}\\ =\mu \epsilon \exp \left\{\begin{array}{l}-j2\pi \frac{2R}{c}(m-1)\Delta f+j2\pi [\Delta f_{dm}+f_{d0}](k-1)\\ +j2\pi [(m-1)f_v+(m-1)\Delta f_{vm}+(n-1)f_h+(n-1)\Delta f_{hnm}]\\ -j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}\end{array}\right\}+{\tilde{n}}_c\\ \approx \mu \epsilon \exp \left\{\begin{array}{l}-j2\pi \frac{2R}{c}(m-1)\Delta f+j2\pi (k-1)f_{d0}\\ +j2\pi [(m-1)f_v+(n-1)f_h]\\ -j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}\end{array}\right\}+{\tilde{n}}_c.\end{array}$$

(11)

The received and processed data is MNK*1-dimensional data, which can be written in the form of a steering vector, as:

$$\begin{array}{cc}\hfill S& =[\begin{array}{cccccc}{s}_{111},& s_{112},& \dots ,& s_{mnk},& \dots ,& s_{MNK}\end{array}]\hfill \\ & =\eta \epsilon g_C\odot c_d(f_{d0})\otimes \left\{b_h(f_h)\otimes a_{cv}(f_{cv})\right\}+{\tilde{n}}_c\hfill \\ & =\eta \epsilon g_C\odot c_d(f_{d0})\otimes \left\{b_h(f_h)\otimes [a_R(f_R)\odot a_v(f_v)]\right\}+{\tilde{n}}_c,\hfill \end{array}$$

(12)

where $c_d(f_{d0})\in {ℂ}^{K\times 1}$, $b_h(f_h)\in {ℂ}^{N\times 1}$, $a_{cv}(f_{cv})\in {ℂ}^{M\times 1}$ are Doppler, horizontal reception and composite vertical emitting steering vectors, respectively; where the composite vertical emitting steering vector is composed of FDA range coupling steering vector and emitting angle steering vector, respectively, denoted as $a_R(f_R)\in {ℂ}^{M\times 1}$, $a_v(f_v)\in {ℂ}^{M\times 1}$. Their specific forms are given below:

$$\{\begin{array}{l}{c}_d(f_{d0})={\left[1,\exp \left\{j2\pi f_{d0}\right\},\dots ,\exp \left\{j2\pi (K-1)f_{d0}\right\}\right]}^T\\ b_h(f_h)={\left[1,\exp \left\{j2\pi f_h\right\},\dots ,\exp \left\{j2\pi (N-1)f_h\right\}\right]}^T\\ a_{cv}(f_{cv})=a_R(f_R)\odot a_v(f_v)\\ ={\left[1,\exp \left\{-j2\pi f_R\right\},\dots ,\exp \left\{-j2\pi (M-1)f_R\right\}\right]}^T\\ \odot {\left[1,\exp \left\{j2\pi f_v\right\},\dots ,\exp \left\{j2\pi (M-1)f_v\right\}\right]}^T\end{array}$$

(13)

where $f_R=-\Delta f\frac{2R}{c}$. The main lobe rectification compensation weight vector is written as:

$$\begin{array}{ll}\hfill g_C& =\exp \left\{h_{K1}\otimes P_{N1}\otimes q(-\Delta f/f_{PRF})\right\}\hfill \\ & =\exp \left\{\begin{array}{l}{\left[0+z_0,1+z_1,\dots ,K-1+z_{K-1}\right]}^T\otimes P_{N1}\\ \otimes {\left[0,-j2\pi \Delta f/f_{PRF},\dots ,-j2\pi (M-1)\Delta f/f_{PRF}\right]}^T\end{array}\right\},\hfill \end{array}$$

(14)

where $h_{K1}={\left[0+z_0,1+z_1,\dots ,K-1+z_{K-1}\right]}^T$, $q(-\Delta f/f_{PRF})={\left[0,-j2\pi \Delta f/f_{PRF},\dots ,-j2\pi (M-1)\Delta f/f_{PRF}\right]}^T$, $P_{N1}$ is an N × 1-dimensional all-one matrix.

According to Equation (12), when there are U times of range ambiguous areas and N_C clutter blocks in the same illumination range, the total echoes received by the receiver can be written as:

$$\begin{array}{l}y\\ ={\displaystyle \sum _{p=0}^U{\displaystyle \sum _{q=1}^{N_c}{S}_{pq}+{\tilde{n}}_c}}\\ ={\displaystyle \sum _{p=0}^U{\displaystyle \sum _{q=1}^{N_c}{\eta }_p{\epsilon }_{pq}{g}_C\odot c_d(f_{d0pq})\otimes \left\{b_h(f_{hpq})\otimes a_{cv}(f_{cvpq})\right\}}}+{\tilde{n}}_c\\ ={\displaystyle \sum _{p=0}^U{\displaystyle \sum _{q=1}^{N_c}{\eta }_p{\epsilon }_{pq}{g}_C\odot c_d(f_{d0pq})\otimes \left\{b_h(f_{hpq})\otimes [a_R(f_{Rp})\odot a_v(f_{vpq})]\right\}}+{\tilde{n}}_c}.\end{array}$$

(15)

3. MLC-FDA Range-Ambiguous Clutter Suppression Method Based on Compressed Sensing

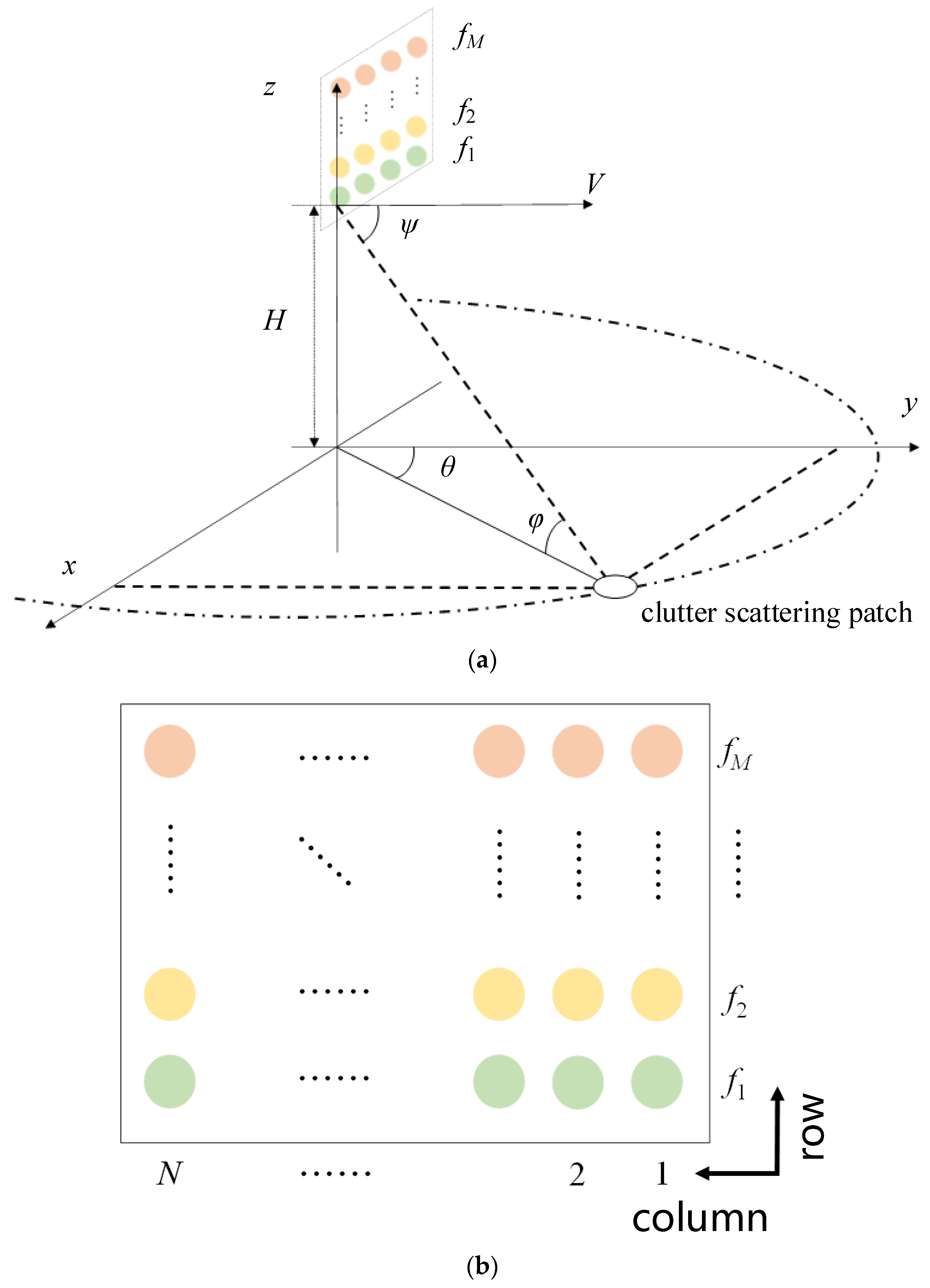
According to Equation (13), the spatial angular frequency of composite vertical transmitting is expressed as $f_{cv}=f_R+f_v=-\Delta f\frac{2R}{c}+\frac{d}{c}\sin \phi f_0$. For the desired target located at R₀, according to [25,33], and especially Equation (15) of Article [25], we construct the range compensation steering vector:

$$\begin{array}{l}{e}_R(f_{R_0})=p_{K1}\otimes p_{N1}\\ \otimes {\left[1,\exp \left\{j2\pi \frac{2R_0}{c}\Delta f\right\},\dots ,\exp \left\{j2\pi \frac{2R_0}{c}(M-1)\Delta f\right\}\right]}^T,\end{array}$$

(16)

where $f_{R_0}=\Delta f2R_0/c$, $p_{K1}\in K\times 1$, $p_{N1}\in N\times 1$ are all one-column vectors. After substituting the above equation into Equation (15) for compensation, the composite vertical transmitting spatial angular frequencies of the unambiguous region and the 1st ambiguous region are expressed as:

$$\{\begin{array}{l}{f}_{cv0}(R_0)=f_R+f_v+f_{R0}\\ =-\Delta f\frac{2R_0}{c}+\frac{d}{c}\sin \phi f_0+\Delta f\frac{2R_0}{c}=\frac{d}{c}\sin \phi f_0\\ f_{cv1}(R_1)=f_R+f_v+f_{R0}=-\Delta f\frac{2R_1}{c}+\frac{d}{c}\sin \phi f_0+\Delta f\frac{2R_0}{c}\\ =-\Delta f\frac{2(R_1-R_0)}{c}+\frac{d}{c}\sin \phi f_0=-\Delta f\frac{2R_u}{c}+\frac{d}{c}\sin \phi f_0\end{array}$$

(17)

where R₀ and R₁ represent the oblique ranges of the unambiguous range region and the 1st range-ambiguous region, respectively. R₁ = R₀ + R_u. The maximum unambiguous range can be expressed as R_u = c/(2f_PRF).

Figure 5 shows the situation where the signals of different range-ambiguous clutter are superimposed into the radar receiver. Because the reflected clutter blocks differ by a maximum unambiguous range R_u, and the difference between pulses is a pulse period T = 1/f_PRF, taking the range unambiguous region and the 1st range-ambiguous region as an example, the superimposed pulses differ by one pulse. For the k-th transmitting pulse, the sequence number of the received pulse corresponding to the range unambiguous range area is k unchanged. Here we take coherent pulse number K = 5 as an example. The sequence number corresponding to the superimposed pulse in the 1st range ambiguous area is k₁ = ${\mathrm{mod}}_5|(k-1)+4|+1$. When k = 1, 2, 3, 4, 5, the echo sequence numbers received from the 1st range ambiguous area are k₁ = 5, 1, 2, 3, 4, respectively.

Since we compensate the main lobe movement of the FDA pattern during transmission, we need to eliminate the effect of main lobe rectification compensation on the signal when receiving the signal. Therefore, we carry out corresponding main lobe correction reduction compensation for the receiving signal, and the reduction compensation vector is constructed as follows:

$$\begin{array}{ll}\hfill g_{RC}& =\exp \left\{h_{K1}\otimes P_{N1}\otimes q(\Delta f/f_{PRF})\right\}\hfill \\ & =\exp \left\{\begin{array}{l}{\left[0+z_0,1+z_1,\dots ,K-1+z_{K-1}\right]}^T\otimes P_{N1}\\ \otimes {\left[0,j2\pi \Delta f/f_{PRF},\dots ,j2\pi \Delta f(M-1)/f_{PRF}\right]}^T\end{array}\right\}.\hfill \end{array}$$

(18)

This is substituted into Equation (15) (taking the signal of the k-th pulse, emitted by the m-th one and received by the n-th element as an example). The process of twice compensation is shown as follows. The echo signal in the range unambiguous region is written as follows:

$$\begin{array}{l}{s}_{mnk}(R_0)\\ =\eta \epsilon \exp \left\{\begin{array}{l}-j2\pi \frac{2(R_0-R_0)}{c}(m-1)\Delta f\\ +j2\pi f_0\left(\frac{(m-1)d\sin \phi }{c}+\frac{(n-1)d\cos \phi \sin \theta }{c}\right)\\ +j2\pi (k-1)f_{d0}-j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}\\ +j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}\end{array}\right\}+{\tilde{n}}_c\\ =\eta \epsilon \exp \left\{j2\pi f_0\left(\frac{(m-1)d\sin \phi }{c}+\frac{(n-1)d\cos \phi \sin \theta }{c}\right)+j2\pi f_{d0}(k-1)\right\}+{\tilde{n}}_c.\end{array}$$

(19)

The echo from the 1st range-ambiguous region can be expressed as:

$$\begin{array}{l}{s}_{mnk}(R_1)\\ =\eta \epsilon \exp \left\{\begin{array}{l}-j2\pi \frac{2(R_1-R_0)}{c}(m-1)\Delta f+j2\pi (k-1)f_{d0}\\ +j2\pi f_0\left(\frac{(m-1)d\sin \phi }{c}+\frac{(n-1)d\cos \phi \sin \theta }{c}\right)\\ -j2\pi (m-1)\Delta f[{\mathrm{mod}}_5|(k-1)+4|+z_{{\mathrm{mod}}_5|(k-1)+4|}]/f_{PRF}\\ +j2\pi (m-1)\Delta f(k-1+z_{k-1})/f_{PRF}\end{array}\right\}+{\tilde{n}}_c\\ =\eta \epsilon \exp \left\{\begin{array}{l}-j2\pi \frac{2R_u}{c}(m-1)\Delta f+j2\pi (k-1)f_{d0}\\ +j2\pi f_0\left(\frac{(m-1)d\sin \phi }{c}+\frac{(n-1)d\cos \phi \sin \theta }{c}\right)\\ -j2\pi (m-1)\Delta f\left[{\mathrm{mod}}_5|(k-1)+4|-(k-1)+\Delta z\right]/f_{PRF}\end{array}\right\}+{\tilde{n}}_c,\end{array}$$

(20)

where $\Delta z=z_{{\mathrm{mod}}_5|k-1+4|}-z_{k-1}$. After two compensations, because of $R_u=c/(2f_{PRF})$, the 1st ambiguous region echo of Equation (20) can be further written as:

$$\begin{array}{l}{s}_{mnk}(R_1)\\ =\eta \epsilon \exp \left\{\begin{array}{l}-j2\pi \frac{2R_u}{c}(m-1)\Delta f+j2\pi (k-1)f_{d0}\\ +j2\pi f_0\left(\frac{(m-1)\sin \theta d}{c}+\frac{(n-1)\sin \theta \cos \phi d}{c}\right)\\ -j2\pi (m-1)\Delta f\left[{\mathrm{mod}}_5|(k-1)+4|-(k-1)+\Delta z\right]/f_{PRF}\end{array}\right\}+{\tilde{n}}_c\\ =\eta \epsilon \exp \left\{\begin{array}{l}-j2\pi (m-1)\Delta f/f_{PRF}+j2\pi (k-1)f_{d0}\\ +j2\pi f_0\left(\frac{(m-1)\sin \phi d}{c}+\frac{(n-1)\sin \theta \cos \phi d}{c}\right)\\ -j2\pi (m-1)\Delta f\left[{\mathrm{mod}}_5|(k-1)+4|-(k-1)+\Delta z\right]/f_{PRF}\end{array}\right\}+{\tilde{n}}_c\\ =\eta \epsilon \exp \left\{\begin{array}{l}j2\pi f_0\left(\frac{(m-1)\sin \phi d}{c}+\frac{(n-1)\sin \theta \cos \phi d}{c}\right)+j2\pi f_{d0}(k-1)\\ -j2\pi (m-1)\Delta f\left[{\mathrm{mod}}_5|(k-1)+4|-(k-1)+1+\Delta z\right]/f_{PRF}\end{array}\right\}+{\tilde{n}}_c\\ =\eta \epsilon \exp \left\{\begin{array}{l}j2\pi f_0\left(\frac{(m-1)\sin \phi d}{c}+\frac{(n-1)\sin \theta \cos \phi d}{c}\right)+j2\pi f_{d0}(k-1)\\ -j2\pi (m-1)\Delta f\beta (k)/f_{PRF}\end{array}\right\}+{\tilde{n}}_c.\end{array}$$

(21)

$\beta ={\mathrm{mod}}_5|(k-1)+4|-(k-1)+1+\Delta z={\mathrm{mod}}_5|k+3|-k+2+\Delta z$. According to the above equation, through twice compensation, the signal from the unambiguous region is the same as MIMO radar signal form, and its FDA range coupling phase term and main lobe correction phase term are compensated and eliminated. It can be denoted as:

$$s_{mnk}(R_0)=\eta \epsilon \exp \left\{\begin{array}{l}j2\pi f_0\left(\frac{(m-1)d\sin \phi }{c}+\frac{(n-1)d\cos \phi \sin \theta }{c}\right)\\ +j2\pi (k-1)f_{d0}\end{array}\right\}+{\tilde{n}}_c.$$

(22)

The signal form of the 1st ambiguous clutter region can be expressed as:

$$s_{mnk}(R_1)=\eta \epsilon \exp \left\{\begin{array}{l}j2\pi f_0\left(\frac{(m-1)\sin \phi d}{c}+\frac{(n-1)\sin \theta \cos \phi d}{c}\right)\\ +j2\pi f_{d0}(k-1)-j2\pi (m-1)\Delta f\beta (k)/f_{PRF}\end{array}\right\}+{\tilde{n}}_c.$$

(23)

From Equations (22) and (23), we can see that the spatial angular frequency of composite vertical transmitting of the range unambiguous area is $f_{cv0}=d/c\sin \phi f_0$. The spatial angular frequency of composite vertical transmitting of the 1st range ambiguous area is $f_{cv1}=-\Delta f\beta (k)/f_{PRF}+d/c\sin \phi f_0$. Compared with the range unambiguous region, the composite vertical transmission spatial angular frequency of the range ambiguous region has one more term, $-\Delta f\beta /f_{PRF}$, and the $\beta$ corresponding to different range ambiguous regions is different. Meanwhile, for the same range ambiguous region, when the value of k is different, the corresponding value of $\beta$ is also different. The coherent pulse number is denoted as K. Taking K = 5 with the offset coefficient x = 3 as an example, when k equals 1, 2, 3, 4, and 5, respectively, the $\beta (k)$ values are 76/15, −1/60, −1/60, −1/60, and −1/60. Therefore, during power spectrum scanning, for the same ambiguous area, the spatial angular frequencies of the composite vertical transmitting are different. The clutter power spectrum is low gain with dispersed distribution, and a high gain clutter ridge can be difficult to form.

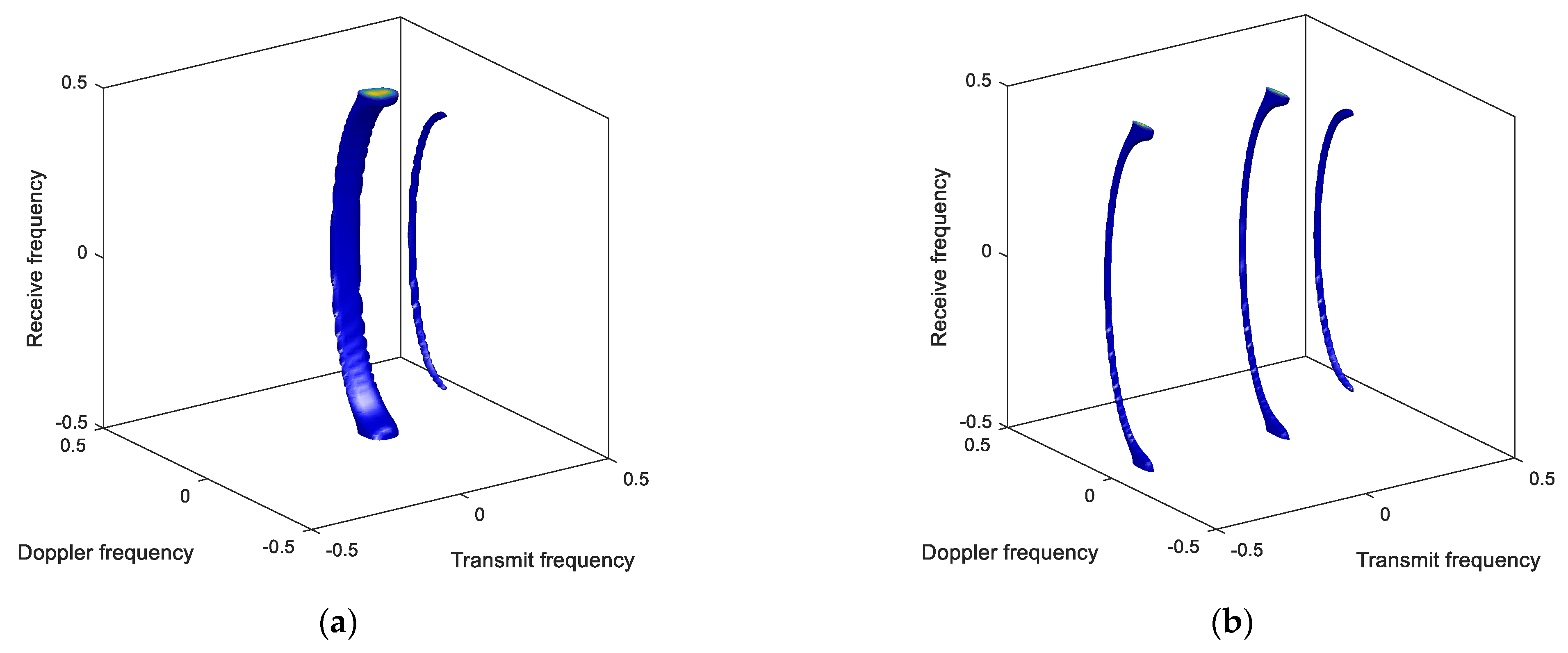
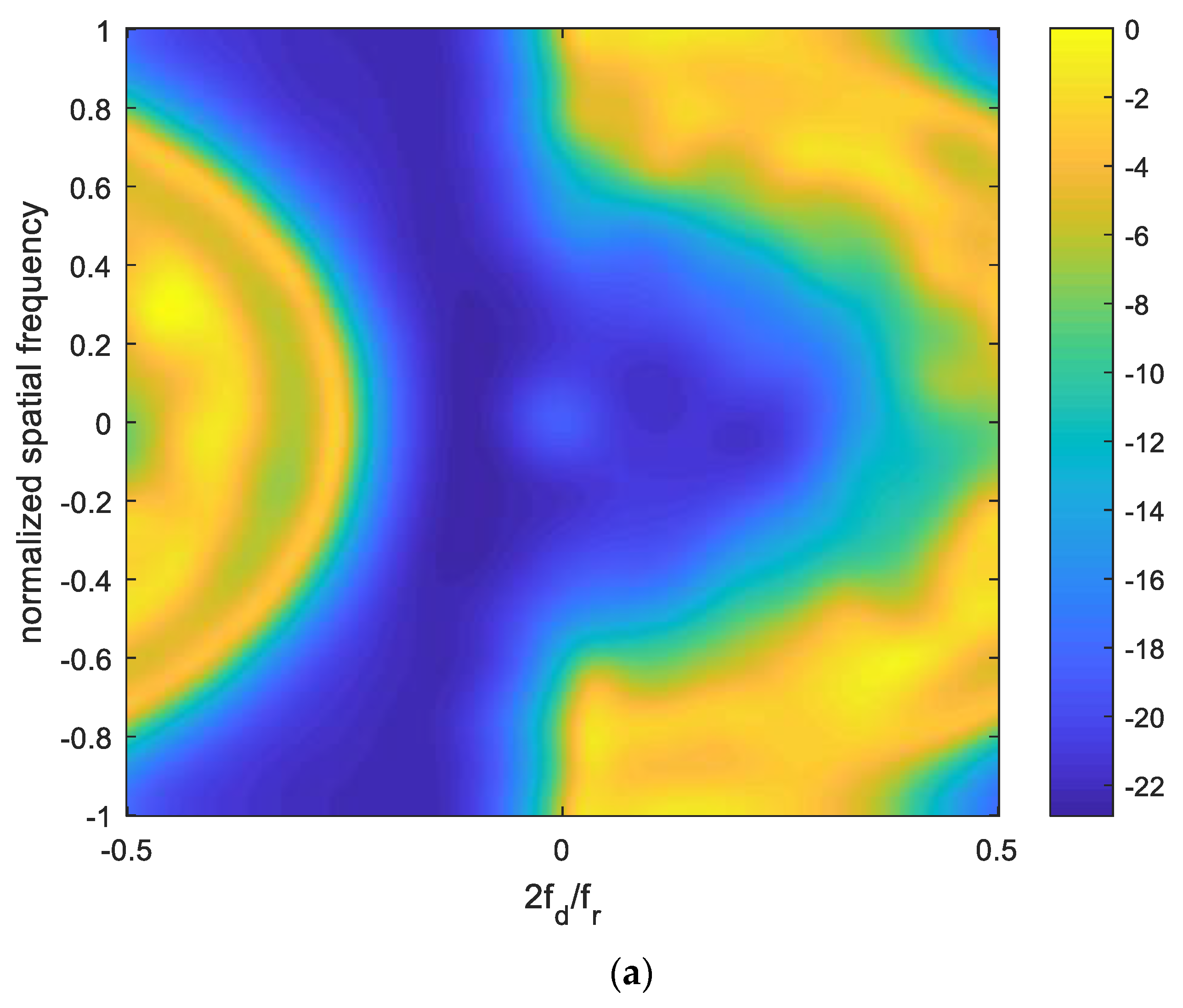
During the spectrum scanning, the clutter of the range unambiguous region is the same as the conventional MIMO radar signal form. Under the geometric framework of the forward-looking array, it presents a positive elliptical distribution in the elevation angle, horizontal angle and Doppler three-dimensional power spectrum. Since the radar illumination angle zone is [0, 180], the clutter presents a semi-elliptical shape. As shown in Figure 6, there is a three-dimensional clutter spectrum distribution of clutter in different areas under a single range cell. Figure 6a represents MIMO radar. The blue represents the clutter power spectrum distribution of the unambiguous area. It corresponds to a large elevation angle, with the clutter distribution characteristics leading to a small ellipse radius. The corresponding elevation angles of echoes from range ambiguous areas with different ranges are different. And the change in elevation angle decreases with increase in range. Therefore, the difference in corresponding elevation angles of clutter from range ambiguous areas is small. Their clutter distribution overlaps each other. Different orange semi-ellipses in the figure represent the clutter distribution of the 1st and the 2nd range-ambiguous regions. 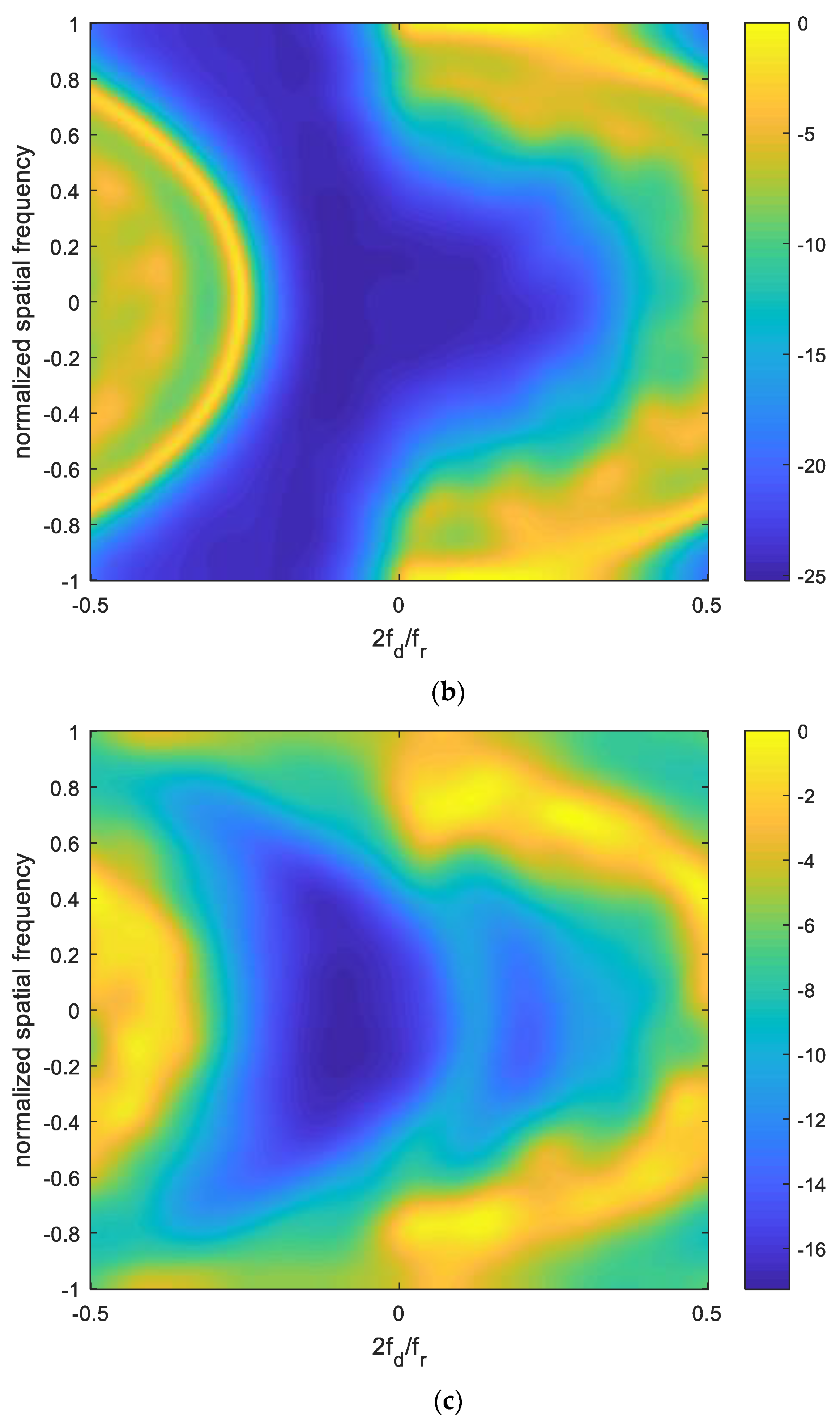Figure 6b shows the distribution characteristics of range ambiguous clutter of conventional FDA radar. Because of the range coupled phase terms of elevation spatial frequency, clutter corresponding to different ranges are separated from one another. 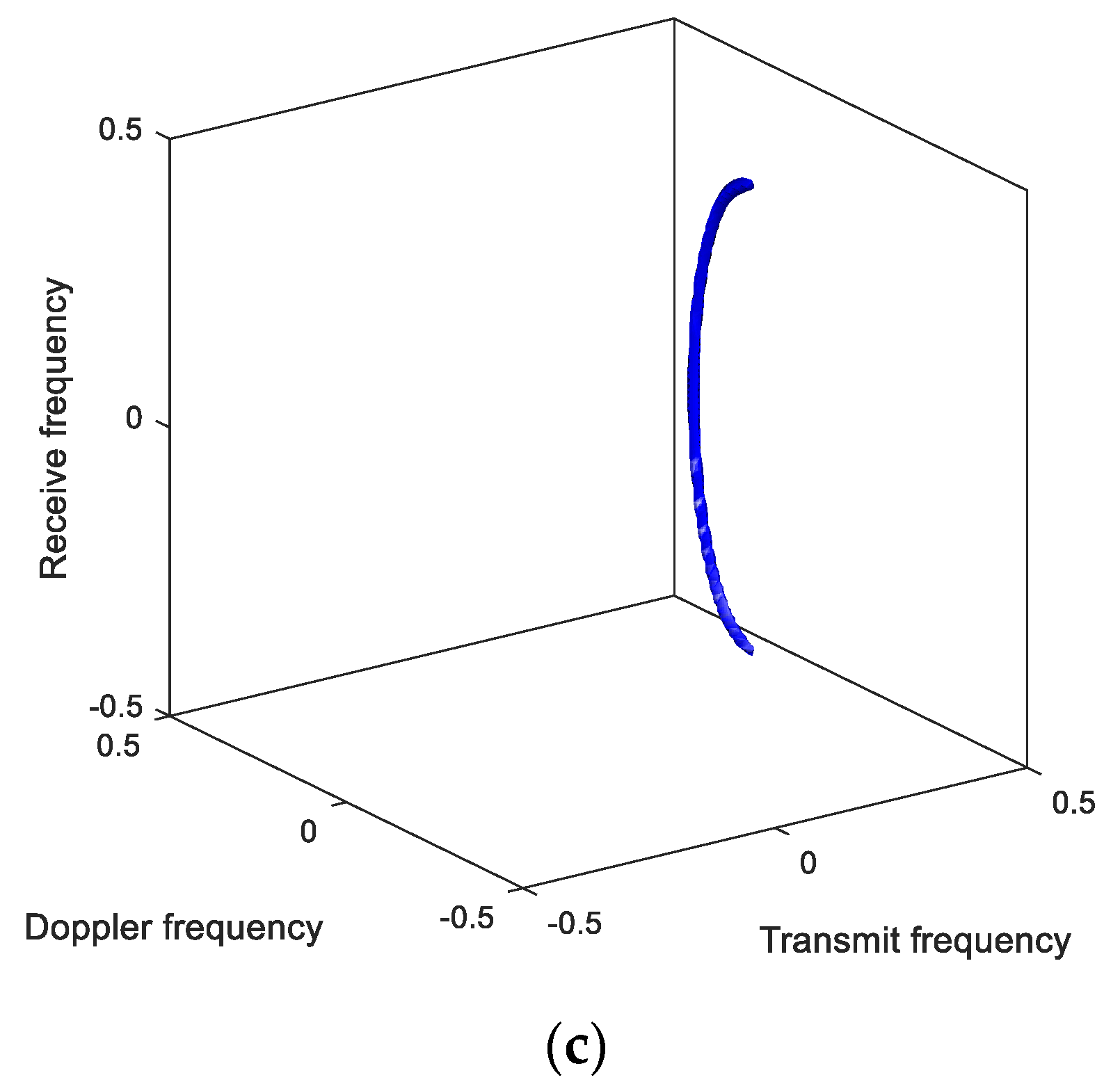Figure 6c shows the range-ambiguous clutter of MLC-FDA radar. Through twice compensation, the phase term of the clutter unambiguous region is the same as that of conventional MIMO and presents a semi-elliptical distribution. Due to the existence of the $-\Delta f\beta /f_{PRF}$ term in the spatial angular frequency of composite vertical transmission of the ambiguous region, the clutter energy of the range ambiguous region is challening to concentrate to form a high gain clutter ridge during power spectrum scanning. Instead, it presents a low gain distribution in the whole three-dimensional space.

Substituting the two compensation vectors $g_{RC}$, $e_R$ into Equation (15), it can be denoted as:

$$\begin{array}{l}y\\ ={\displaystyle \sum _{p=0}^U{\displaystyle \sum _{q=1}^{N_c}{g}_{RC}\odot e_R(f_{R0})\odot S_{pq}+{\tilde{n}}_c}}\\ ={\displaystyle \sum _{p=0}^U{\displaystyle \sum _{q=1}^{N_c}{\eta }_p{\epsilon }_{pq}{g}_{RC}\odot e_R(f_{R0})\odot g_C\odot c_d(f_{d0pq})\otimes \left\{b_h(f_{hpq})\otimes a_{cv}(f_{cvpq})\right\}}}+{\tilde{n}}_c\\ ={\displaystyle \sum _{p=0}^U{\displaystyle \sum _{q=1}^{N_c}{\eta }_p{\epsilon }_{pq}{c}_d(f_{d0pq})\otimes \left\{b_h(f_{hpq})\otimes [a_R(f_{Rp}+f_{R0}+f_{g_C}+f_{g_{RC}})\odot a_v(f_{vpq})]\right\}}+{\tilde{n}}_c}\\ ={\displaystyle \sum _{p=0}^U{\displaystyle \sum _{q=1}^{N_c}{\eta }_p{\epsilon }_{pq}{c}_d(f_{d0pq})\otimes \left\{b_h(f_{hpq})\otimes [a_R(f_R(p\frac{R_u}{c})+f_{g_C}+f_{g_{RC}})\odot a_v(f_{vpq})]\right\}}+{\tilde{n}}_c}\\ ={\displaystyle \sum _{p=0}^U{\displaystyle \sum _{q=1}^{N_c}{\eta }_p{\epsilon }_{pq}{c}_d(f_{d0pq})\otimes \left\{b_h(f_{hpq})\otimes \left\{a[f({\beta }_p/f_{PRF})]\odot a_v(f_{vpq})\right\}\right\}}+{\tilde{n}}_c}.\end{array}$$

(24)

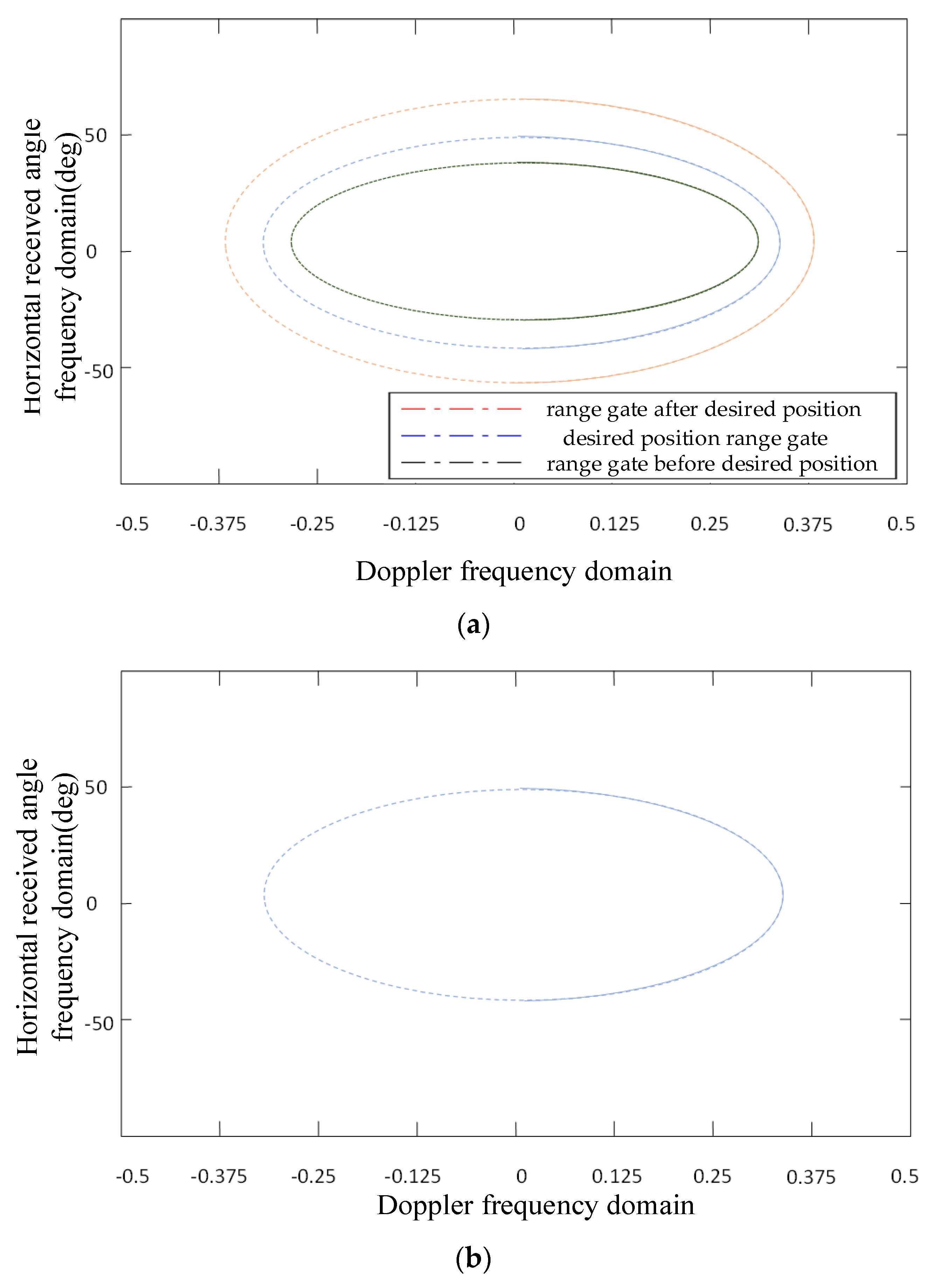
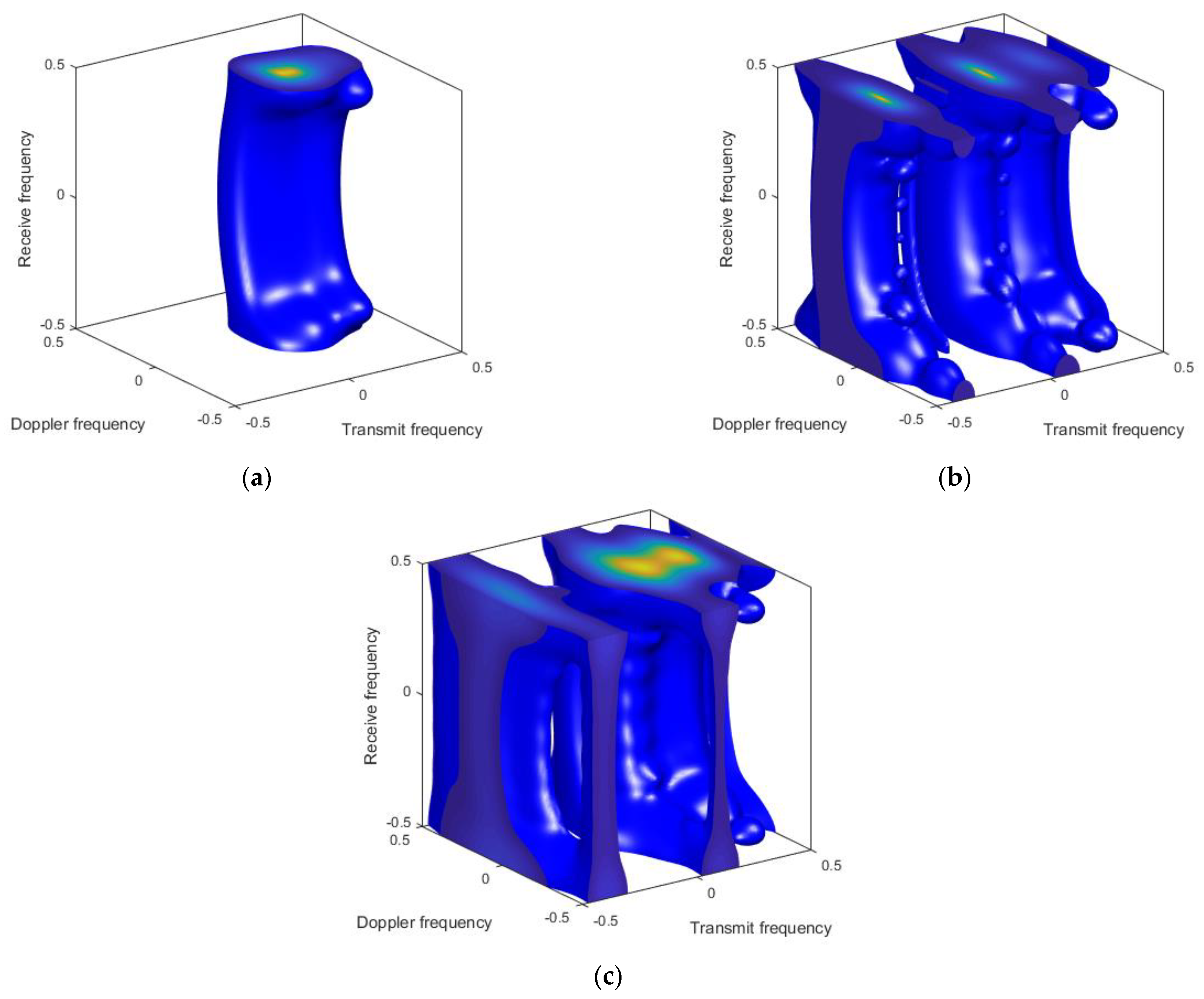
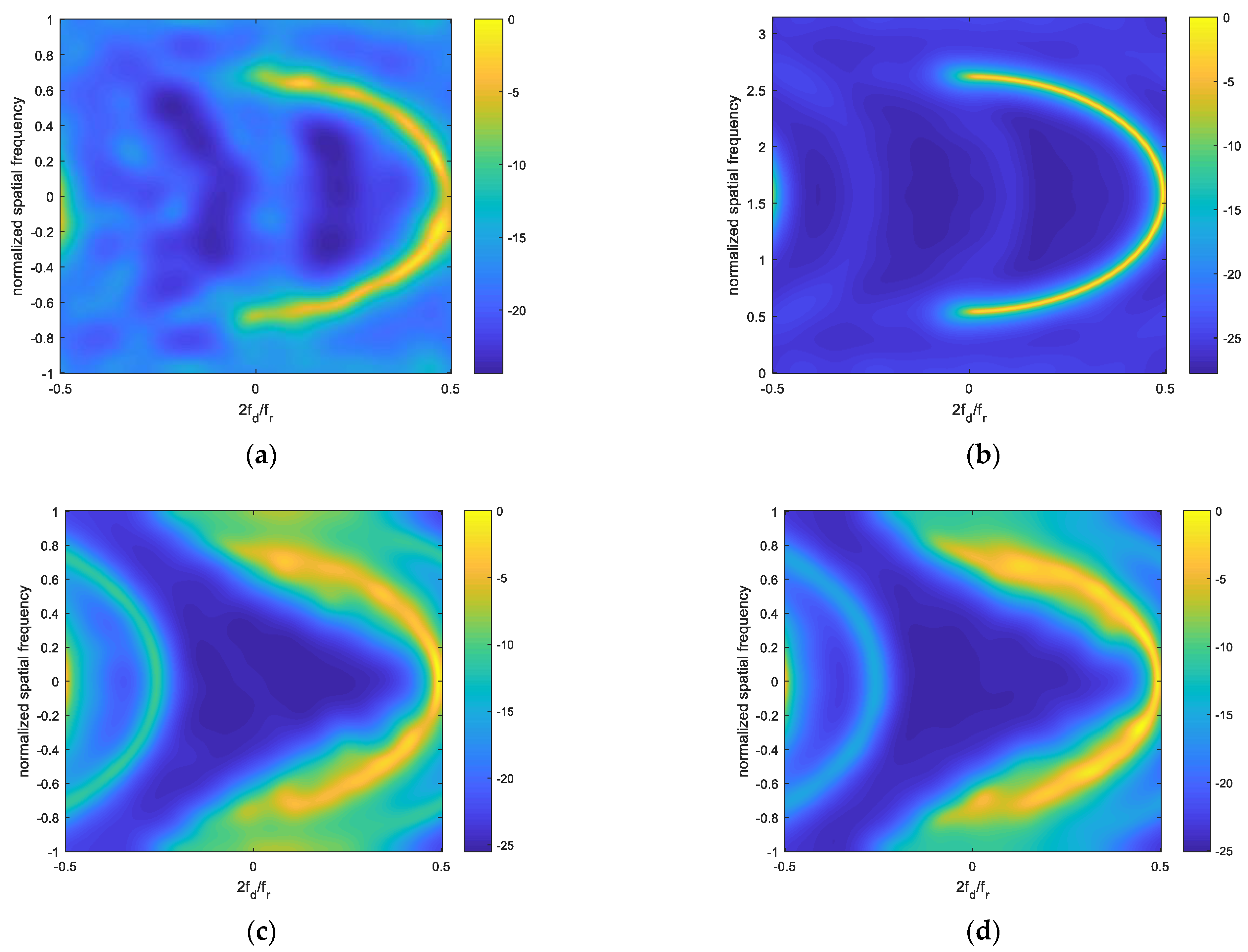
Subsequently, after elevation dimension filtering, the clutter distribution in the horizontal receive angle Doppler two-dimensional domain of the signal after two compensations is shown in Figure 7.

Due to the forward-looking array configuration, the clutter after elevation dimension filtering still has serious range dependence. We use the compressed sensing method and a small number of snapshots data around the desired range gate to recover the clutter covariance matrix of the desired position. Firstly, we construct the horizontal reception angle and Doppler two-dimensional domain dictionary Ω. N_s is the horizontal reception spatial dispersion coefficient and N_d is the Doppler dispersion coefficient. The horizontal reception angle and Doppler frequencies are $f_h\in [-0.5,0.5]$, $f_d\in [-0.5,0.5]$, respectively. A complete clutter spectrum dictionary is constructed as:

$$\Omega =[s(f_h{{}_{,}}_1,f_d{{}_{,}}_1),s(f_h{{}_{,}}_1,f_d{{}_{,}}_2),\dots ,s(f_h{{}_{,}}_{\mathrm{Ns}},f_d{{}_{,}}_{\mathrm{Nd}})],$$

(25)

and the dictionary vector can be written as:

$$\begin{array}{l}s(f_{h,n_s},f_{d,n_d})\\ =c_d(f_{d,n_d})\otimes b_h(f_{h,n_s})\\ ={\left[1,\exp \left\{j2\pi f_{d,n_d}\right\},\dots ,\exp \left\{j2\pi (K-1)f_{d,n_d}\right\}\right]}^T\\ \otimes {\left[1,\exp \left\{j2\pi f_{h,n_s}\right\},\dots ,\exp \left\{j2\pi (N-1)f_{h,n_s}\right\}\right]}^T,\end{array}$$

(26)

where $\{\begin{array}{l}{f}_{h,n_s}=-0.5+n_s\ast (1/N_s)\\ f_{d,n_d}=-0.5+n_d\ast (1/N_d)\end{array}$, $n_s\in [0,N_s]$, $n_d\in [0,N_d]$. The two-dimensional power spectrum is meshed by sparse dictionary Ω. For L range gate echo data:

$$y=\Omega \gamma y\in {ℂ}^{NK\times L},\gamma \in {ℂ}^{N_s{N}_d\times L}.$$

(27)

According to the reconstruction process of sparse recovery, we have:

$$\gamma =\underset{\gamma }{\arg \min }{\Vert y-\Omega \gamma \Vert }_2^{2}subjectto{\Vert \gamma \Vert }_0\le \mu ,$$

(28)

where $\mu$ is the sparsity constraint parameter. Here, we take $\mu$ as one third of the freedom degree NK of the planar array. We use the orthogonal matching pursuit (OMP) method for sparse recovery. The calculation process will not be repeated; CVX toolbox or an iterative algorithm can be used. The algorithm can be referred to in the relevant literature. It is worth noting that we set two iteration termination conditions in the calculation process; one is that ${\Vert y-\Omega \gamma \Vert }_2^2$ is less than or equal to the noise power, and the other is that the number of iterations is greater than or equal to the sparsity constraint parameter $\mu$. The OMP algorithm will terminate the iteration if any condition is met. After obtaining the sparse coefficient matrix $\gamma$, the clutter covariance matrix $\widehat{R}=\Omega \gamma \ast {\left(\Omega \gamma \right)}^H$ of compressed sensing estimation is recovered, and the clutter adaptive weight is calculated according to the clutter covariance matrix. The method performance is discussed in the next section.

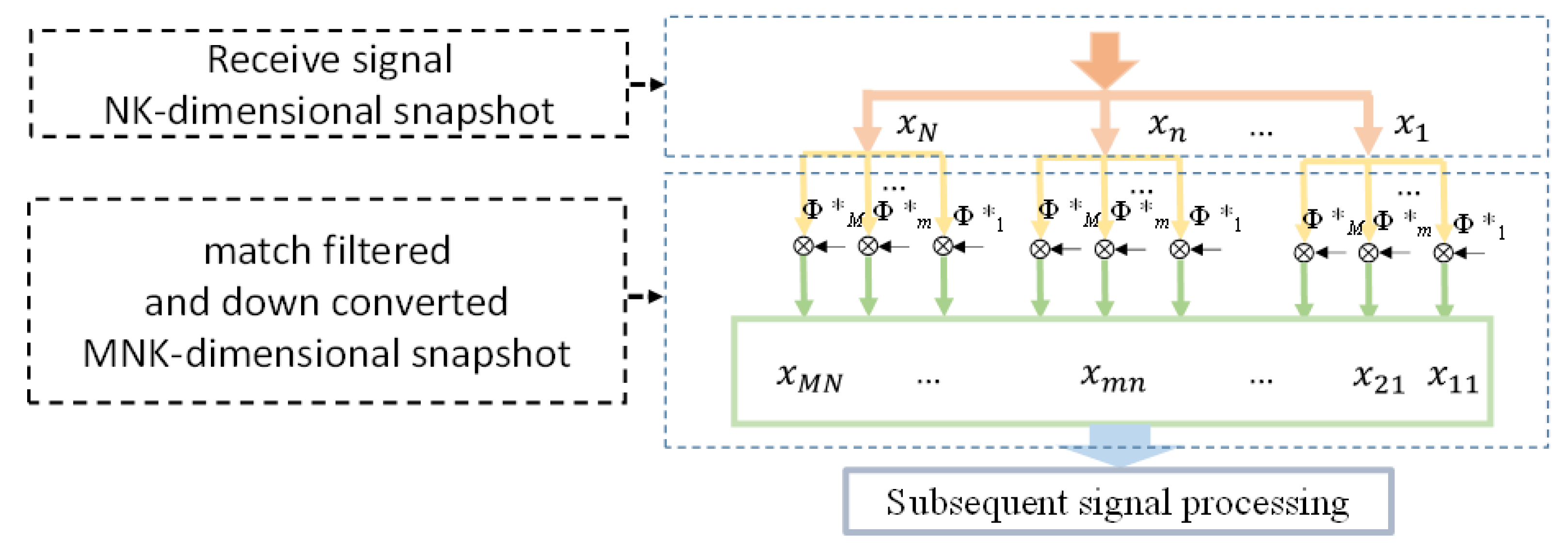
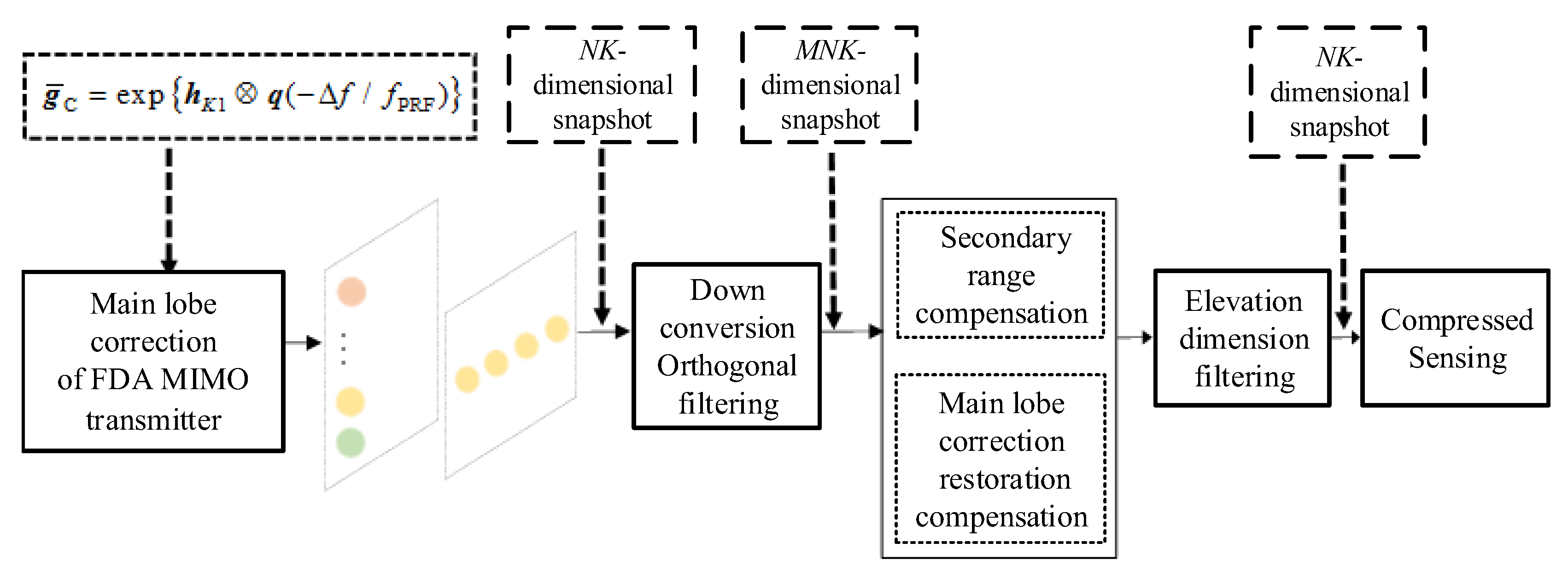
Figure 8 shows the entire compressed sensing main lobe rectification FDA radar signal processing flow. The main lobe rectification vector ${\overline{g}}_C\in MK\times 1$ is weighted at the transmitting end to correct the transmitting main lobe. The K coherent pulses received by the N receiving array elements are converted into MNK-dimensional signal data through orthogonal filtering and down-conversion. The secondary range compensation and main lobe rectification restoration compensations are carried out. The echo of the range unambiguous region after compensation is consistent with the conventional MIMO signal form. The transmission spatial angular frequency of the signal from the range-ambiguous region contains the range coupling phase term $-\Delta f\beta /f_{PRF}$, and can therefore be different from the signal of the unambiguous region in the power spectrum. At the same time, the separation of the ambiguous clutter is completed. Then signal is converted into NK dimension data through elevation dimension filtering. Then, the data is processed by compressed sensing covariance matrix sparse recovery, adaptive filtering and other subsequent signal processing.

4. Simulation Results

In this section, the algorithms above will be simulated and discussed. According to the signal processing sequence, there are three parts. The simulation mainly discusses the correction of the pattern main lobe after transmission, the three-dimensional power spectrum distribution of clutter after twice compensation, and the final clutter suppression by compressed sensing sparse recovery after elevation dimension filtering. The radar system parameters are listed in

Table 1. Simulation Parameters List.

Parameter	Values
Horizontal array element number M	8
Vertical array element number N	9
Inter-element spacing	0.1 m
Reference carrier frequency f0	1.5 GHz
Frequency increment	5000 Hz
CNR (Clutter noise ratio)	30 dB
Radar flight height	6 km
Radar system speed	300 m/s
Maximum unambiguous range	75 km
Coherent pulse number K	9
Pulse repetition frequency	10,000 Hz
Offset coefficient x	3
Target angle	0°
Target range	9 km
Horizontal receiving space dispersion coefficient Ns	90
Doppler dispersion coefficient Nd	90

, unless otherwise specified.

4.1. MLC FDA Radar Main Lobe Correction Emission Pattern

The main lobe irradiation angle areas of three pulse FDA emission patterns are shown in Figure 9. It is assumed that the target is at 0 degree, the pulse width set as 4.76 × 10⁵ s, and the pulse period is T= 6.67 × 10⁴ s, ∆f = 1/(2T). Because FDA radar contains the phase term of range coupling, the irradiation angle zone of the main lobe within the pulse width changes with the range, showing an S-shaped moving. It can be observed from pulses in the figure that the irradiation position of the main lobe tilts in the direction of the angle decreasing with the change in range (time). Three continuous pulses are shown in Figure 9a. In the conventional FDA pattern, the main lobe irradiation region moves around between pulses. From the observation of Figure 9a, we can see that the 1st pulse main lobe irradiation region is [−30°, 0°]. Due to the existence of ∆f, each array element accumulates different initial phases when transmitting the 2nd pulse after one pulse period. Therefore, the main lobe irradiation angle is affected by the initial phase, and the irradiation zone of the main lobe changes to [−68°, −90°] and [26°, 90°]. At the 3rd pulse, because of ∆f = 1/(2T), the initial phase of each array element Δf undergoing 2T accumulation is an integer multiple of 2π, so the 3rd pulse main lobe irradiation zone is the same as that of the 1st pulse. From the Figure 9a, we can see that the angle of the main lobe irradiated from different pulses is not the same. The conventional FDA cannot maintain the position of the main lobe beampattern. Therefore, during pulse coherent accumulation processing, the desired target echo signal received by the radar cannot maintain high gain, so it is impossible to carry on subsequent signal processing. To overcome these problems, we use the main lobe correction weight. The elements are weighted between coherent pulses in Figure 9b, so as to suppress the influence of the initial phase accumulation generated by ∆f on the main lobe irradiation angle. Therefore, the main lobe with different beam directions within pulse width can irradiate the same angle of the target, so that the target echo signal has high gain of the main lobe. As shown in Figure 9b, the 0° position of the desired target is within the irradiation areas of the main lobe of the different pulses. According to Equation (5), the 1st pulse period initial phase compensation is 0, so the irradiation position of its main lobe is the same as that in Figure 9a. The m-th array element compensation amount for the 2nd pulse period is $2\pi (m-1)\Delta f(1+z_1)/f_{PRF}$. After main lobe correction compensation, the 2nd pulse main lobe area of the beampattern changes from [−34°, 4°] of the 1st pulse, to [−16.5°, 11.5°]. The 3rd pulse compensation is $2\pi (m-1)\Delta f(2+z_2)/f_{PRF}$. After compensation, the main lobe irradiation area is [−1°, 38°]. The target position 0° has been covered in the irradiation areas of coherent pulse main lobes, so as to ensure that the radar can process the signals at the same angle. Figure 9c shows the situation after compensation with coherent pulse number K = 5. Similar to the above, it will not be discussed in detail here.

4.2. Three-Dimensional Distribution Structure of Received Clutter Signal Power Spectrum

Figure 10 and Figure 11 show the power spectrum distribution of the conventional MIMO, FDA radars and the proposed approach in the horizontal, vertical spatial angular frequencies and Doppler three-dimensional space before the elevation dimension filtering. In order to illustrate the clutter ridge structure, the simulation in the figures shows the clutter power spectrum distribution of a single range cell of three range ambiguous regions. The main slant ranges of the range ambiguous areas are 9 km, 84 km, 159 km, respectively, corresponding to the range unambiguous region, the 1st range ambiguous region, and the 2nd ambiguous region. The spatial power spectra with power greater than or equal to −10 dB and −30 dB are selected for explanation. Observing the three groups of data in Figure 10, we can see that the vertical spatial angular frequency corresponding to the unambiguous region in Figure 10a is f_cv0 = 0.3. The corresponding frequencies of the clutters corresponding to the 1st and 2nd range ambiguous regions are f_cv1 = 0.1222 and f_cv2 = 0.0778, respectively. Since the difference is small, it can be seen from Figure 10a that they overlap each other in spectrum. Figure 10b shows the power spectrum of conventional FDA radar after full angle irradiation or solving the issue of the main lobe moving. Clutter of different regions are separated from each other, and the separation degree is affected by radar parameter ∆f. The elevation dimension filter and the positions of the separated clutter influence the suppression result of range ambiguous clutter. The proposed method, clutter power spectrum, is shown in Figure 10c. As described in the above section, after twice compensation, the spatial angular frequency of unambiguous signal echo composite vertical transmission $f_{cv}=d/c\sin \phi f_0$ is the same as that of conventional MIMO. Therefore, the clutter ridge distribution is consistent with that of conventional MIMO. The corresponding vertical spatial angular frequency is f_cv0 = 0.3. For range ambiguous areas, the spatial angular frequency of the composite vertical transmission is $f_{cv}=-\Delta f\beta /f_{PRF}+d/c\sin \phi f_0$. Due to the phase term of the range region and pulse number coupling, the clutter echo in the range ambiguous region difficultly forms a clutter ridge with high gain during power spectrum scanning, but is distributed in a wide area with low gain in three-dimensional space. Therefore, the three-dimensional clutter power spectrum is not displayed. Therefore, we distinguish the clutter in the range unambiguous region from the clutter in the range ambiguous region. By observing the −30 dB three-dimensional power spectrum, it can be seen that the display zones of the conventional MIMO radar and FDA radar clutter power spectrum in Figure 11a,b become larger. While in Figure 11c, the clutter in the ambiguous region of the proposed method is irregularly distributed in three-dimensional space. Compared with the first two methods, the clutter energy is dispersed in the whole three-dimensional space and the gain is low.

The above mainly discusses the proposed method’s spatial distribution characteristics of clutter in range unambiguous and ambiguous areas after twice compensation, and compares it with the clutter structure of conventional MIMO radar and FDA radar.

4.3. Power Spectrum Clutter Suppression Effect and Analysis

As shown in Figure 8, after twice compensation and elevation dimension filtering, the MKN*1-dimensional echo data becomes KN*1-dimensional. Through different elevation dimension filter design, the radar has different suppression effects on clutter at different angles, which will not be discussed in depth here. We use the ordinary target beamforming results as the elevation dimension filter. The structure of the clutter spectrum under a single range cell is shown above. Here, we use all range cells to illustrate the final suppression effect.

Figure 12 illustrates two-dimensional clutter spectrum scanning in the horizontal space domain and Doppler domain of conventional MIMO, FDA and the proposed approach. The range dependence of forward-looking array clutter is serious. Different clutter range cell echoes spreading in the power spectrum pollute a large target detection area, and the performance of radar parameter estimation decreases sharply. As shown in Figure 12a, the clutter of MIMO radar is seriously spread. The range ambiguous clutter makes the radar observation zone almost entirely occupied by the range dependent clutter. In Figure 12b, FDA radar improves the pollution of the clutter power spectrum, compared with MIMO, by separating the clutter of different range-ambiguous regions. Figure 12c shows the proposed approach. Due to the ambiguous clutter phase term coupled with ambiguous multiplicity and pulse number, the clutter has a low gain distribution in a wide area of three-dimensional space. In addition to the elevation dimension filtering, the clutter energy of the range ambiguous regions is further filtered. Therefore, in the two-dimensional power spectrum in the horizontal spatial domain and Doppler domain, the clutter power is the least affected by range-ambiguous clutter.

Figure 13 gives us the improvement factor (IF) curves of three approaches at 0° position. The black, blue and red represent the MIMO, FDA and the proposed approach, respectively. Compared with MIMO and FDA radars, it can be seen from Figure 13 that the most serious clutter spreading phenomenon of the power spectrum is MIMO radar, followed by FDA radar. The clutter ridge distribution of the two methods is relatively wide on the Doppler dimension power spectrum. The clutter spreading image of this method is the lightest and the clutter ridge is the narrowest. Meanwhile, the main lobe region of the coherent pulse beampattern can be maintained. The performance of radar target detection is superior.

Then we use the compressed sensing method to restore the unambiguous range dependent clutter covariance matrix. Figure 14a,b show the reception space and Doppler domains’ two-dimensional power spectrum scanning figures, corresponding to the compressed sensing restored clutter covariance matrix of 10 snapshot data and the ideal clutter covariance matrix. Compared with Figure 12c, it can be seen that, after compressed sensing restoration, the clutter spreading phenomenon is greatly improved. The clutter covariance matrix after compressed sensing is very close to the ideal covariance matrix of the desired range gate in Figure 14b, and this method only needs 10 training samples. It not only solves the problem of few training samples meeting the IID condition, but also obtains the range independent covariance matrix. It reduces the serious range dependence of radar clutter and discards the influence of clutter in range ambiguous areas of target detection. The radar surveillance area will no longer face the pollution of a large number of clutter signals. This method improves the range ambiguous clutter overlap and clutter range dependence of high-speed moving radar in the forward-looking planar array system, and improves the radar target detection performance.

Subsequently, through comparison with the DW (Doppler warping) method, we illustrate the advantages of this method on forward-looking array range dependence suppression. The DW method compensates the sample data of different range cells by estimating the Doppler dimensional frequency differences between the fixed angle expected range cell and other training samples. After elevation dimension filtering, the conventional MIMO and FDA methods use the DW method to suppress clutter spreading, and a comparison is made with the method in this paper. The compensated power spectra are shown in Figure 14c,d. Figure 14c shows that of conventional MIMO radar. Compared with Figure 12a, the influence of clutter range dependence in the range unambiguous area on power spectrum spreading is significantly reduced. The clutter of the range ambiguous area still has an impact on the radar power spectrum after elevation dimension filtering. In Figure 14c, the area with f_d/f_r = −0.2 can be observed. The clutter from the 1st range-ambiguous region generates −10 to −12 dB clutter power here, polluting the radar detectable area. Figure 14d shows the clutter power spectrum corresponding to FDA radar after compensation. In addition, in the f_d/f_r = −0.2 zone, the distribution of clutter ridges in the 1st ambiguous region can be seen, and the power is between [−12, −16] dB. Compared with conventional MIMO radar, the influence of clutter power gain is reduced. Due to the existence of range-ambiguous clutter in the two methods, the available zone of parameter estimation of the radar power spectrum decreases. The range compensation method is affected by range ambiguous clutter. And the improvement effect after compensation is limited. Comparing with Figure 12, we notice that the clutter power spectrum spreading caused by the clutter range-dependent problem of the three radar systems has been improved. From the improved clutter power spectra, we can more clearly observe the influence of clutter in the ambiguous area on the clutter power spectra. The parameter estimation performance of the radar power spectrum for the MIMO and FDA methods is reduced because of range ambiguous clutter. The range compensation method is affected by the range-ambiguous clutter. At the same time, the clutter range dependence leads to widening of the clutter ridge, and the improvement effect after compensation is limited. Therefore, the proposed method has the best performance.

Figure 15 is the performance evaluation curve of the improvement factor (IF) of radar clutter in the Doppler domain with a horizontal receiving angle of 0 degree. The black is the IF curve of MIMO radar. It can be seen from the curve that there are two notches at −0.5 and −0.25. We can see that they are caused by range unambiguous region clutter and the 1st range ambiguous region clutter, respectively. In these two areas and their middle area of Doppler domain, the radar performance has decreased significantly. The blue is the IF curve of the conventional FDA radar. There are two notches at −0.5 and −0.25, the same as that of the MIMO radar, remaining the clutter echo of the range unambiguous and the 1st range ambiguous areas. As a result, similar to the MIMO radar curve, the radar performance degrades significantly at and around the ambiguous clutter notches. In the case of range unambiguous clutter, there is only one notch in the corresponding IF curve. DW technology can approximately align the spectrum peak in the Doppler frequency domain, so as to further improve clutter suppression performance. Conventional radar can effectively suppress the clutter ridge spreading and maintain good target detection performance. However, in the presence of range ambiguous clutter, the performance of these kinds of methods will decline significantly. Range ambiguous clutter and unambiguous clutter have different clutter spreading degrees in the Doppler domain. The compensation methods cannot compensate multiple range ambiguous clutter at the same time. Therefore, clutter pollution still exists in the radar monitoring area. The method in this paper, shown by the red line in Figure 15, eliminates the influence of ambiguous clutter on the radar power spectrum because of the different clutter distribution characteristics of different regions. Therefore, there is only one clutter at −0.5, and the radar performance is no longer affected by range ambiguous clutter. Because it is not affected by clutter in other areas, the power spectrum has a sparse characteristic. The clutter in the unambiguous range area is heavily dependent on range. The compressed sensing method restores the clutter covariance matrix with a small amount of snapshot data, so that the unambiguous clutter spreading phenomenon is the lightest, and there is no conflict between clutter compensation in different range regions, so as to improve the performance of target detection.

5. Conclusions

This paper introduces a range ambiguous clutter suppression method for forward-looking planar array FDA-MIMO radar. The FDA-MIMO radar through weighting the main lobe rectification phase at the transmitting end makes the main lobe illumination zones of coherent pulses cover the same target angle, so that the radar can coherently accumulate for a target at the same range and angle. It improves the signal-to-noise ratio. Subsequently, signal compensations are carried out at the receiving end. Because of the range coupling characteristics of FDA echoes, clutters of different range-ambiguous regions have different composite transmission spatial frequencies after compensation. The signal of the observed desired region is the same as the conventional MIMO signal, while the range-ambiguous echoes of non-observed regions present a low gain discrete distribution in the power spectrum. Therefore, the ambiguous clutter can be eliminated. Because it is not affected by clutter in other areas, the power spectrum has a sparse characteristic. The approximate ideal covariance matrix can be recovered by using only a few clutter snapshots through compressed sensing. At the same time, the problems of insufficient training samples and non-uniformity of clutter data in the conventional STAP method are solved. The detection performance of the conventional STAP method is improved. The simulation results show the effectiveness of this method compared with conventional methods. It is important to emphasize that this approach is applicable to general array geometries.