Influence of Range-Dependent Sound Speed Profile on Position of Convergence Zones
Abstract
:1. Introduction
2. Methods
2.1. Position of Convergence Zones
2.2. Case of Linearly Varying Sound Speed
2.3. Case of Ellipsoidal Gaussian Eddy
3. Simulations
3.1. Case of Linearly Varying Sound Speed
3.2. Case of Ellipsoidal Gaussian Eddy
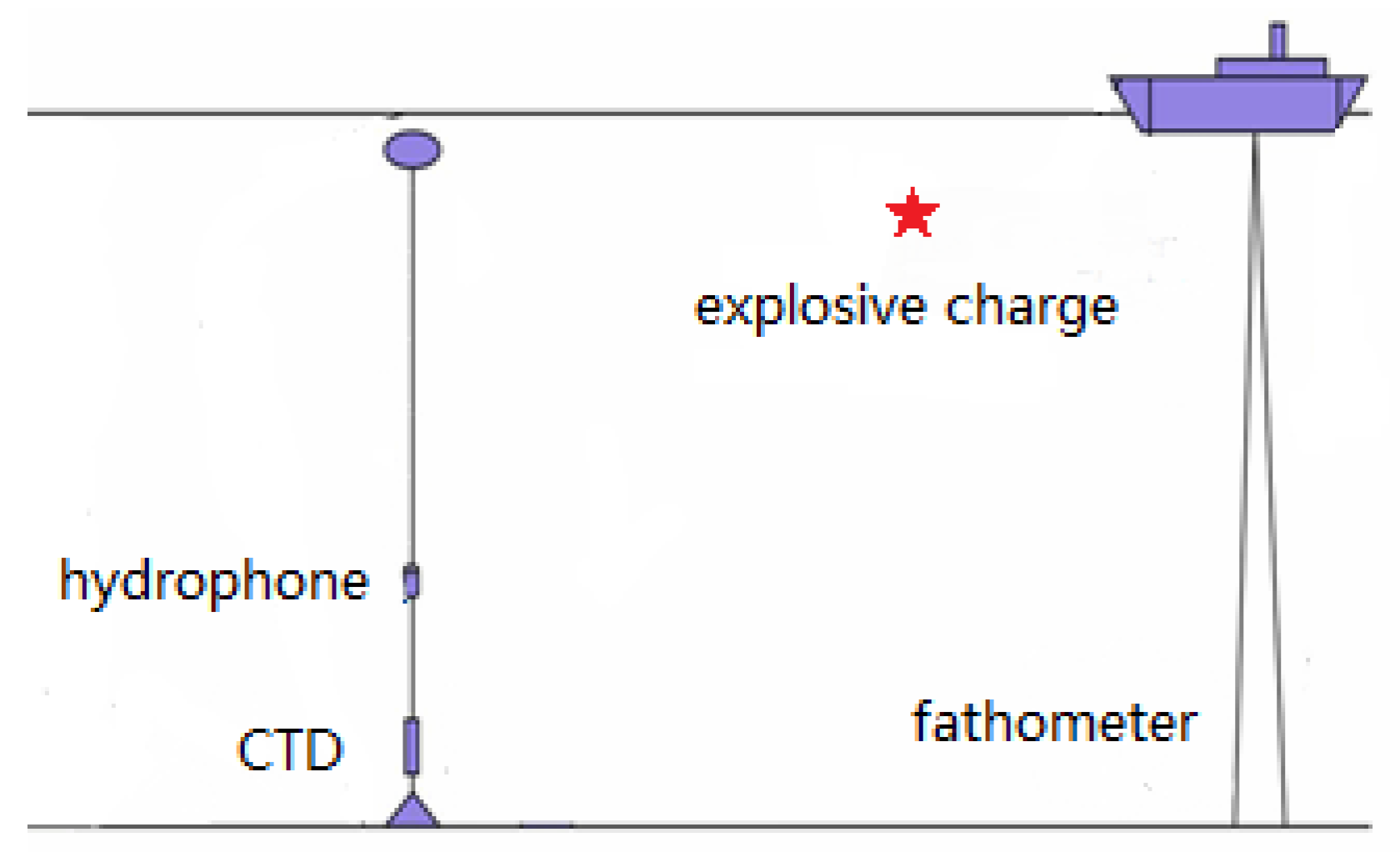
4. Experiments
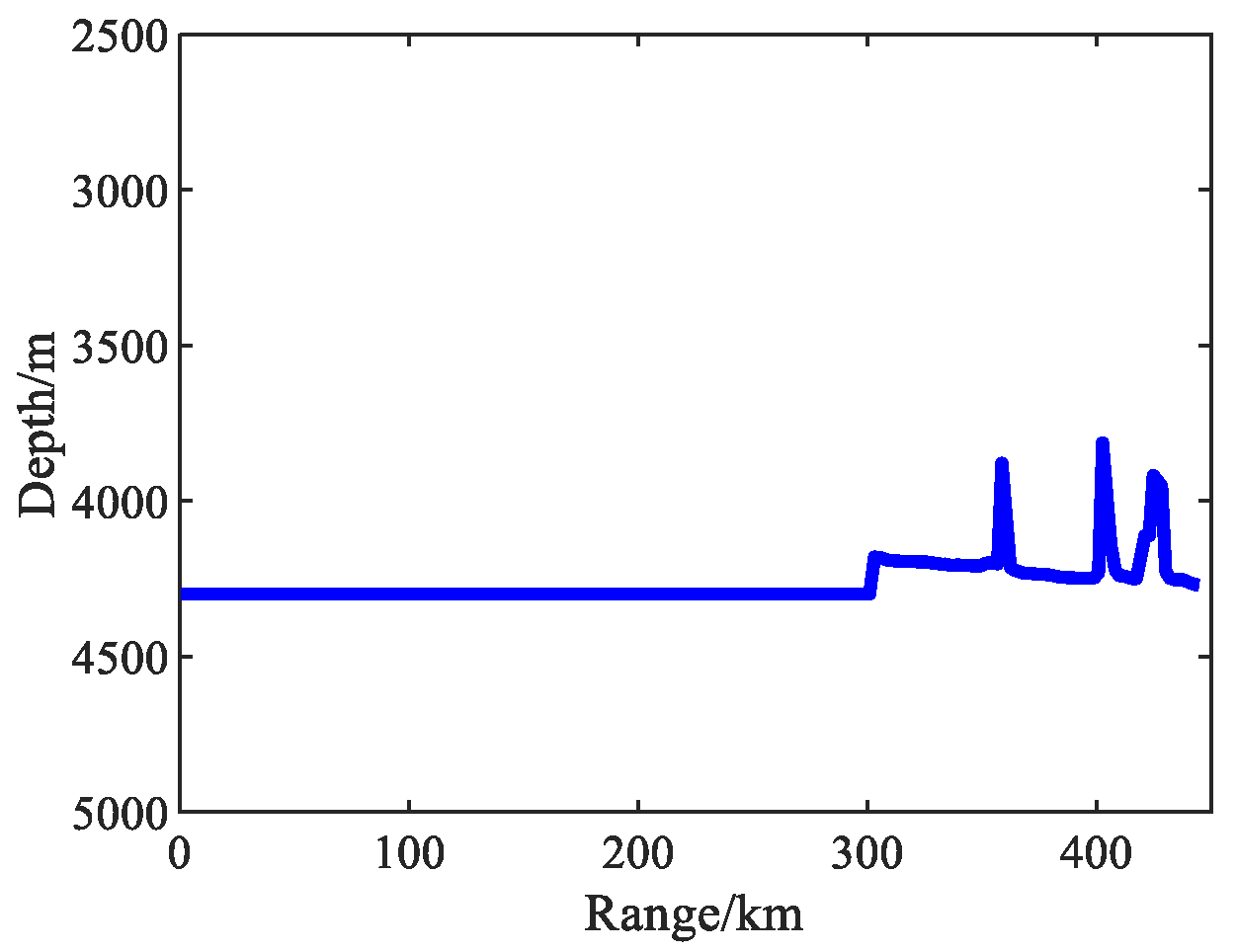
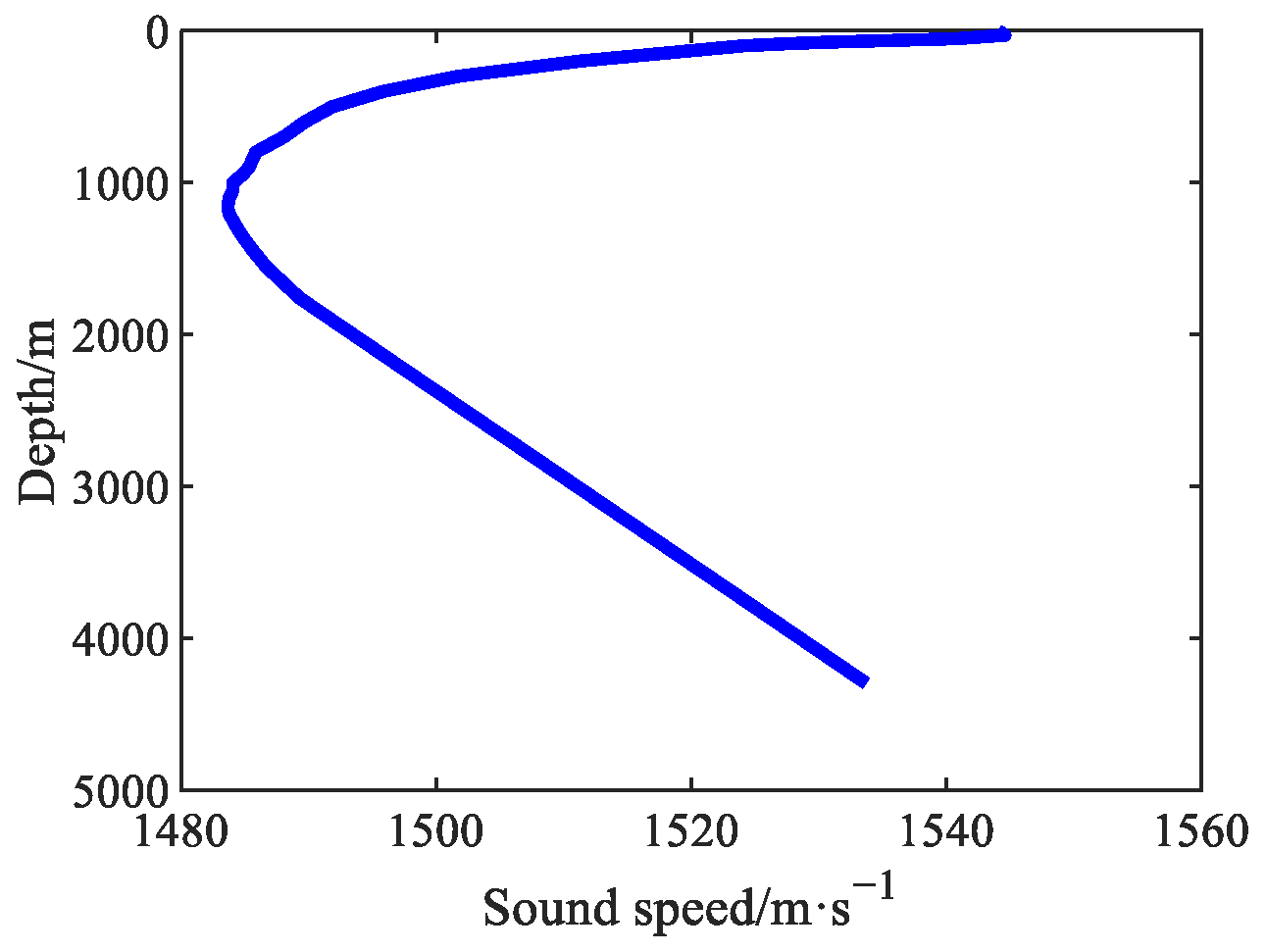
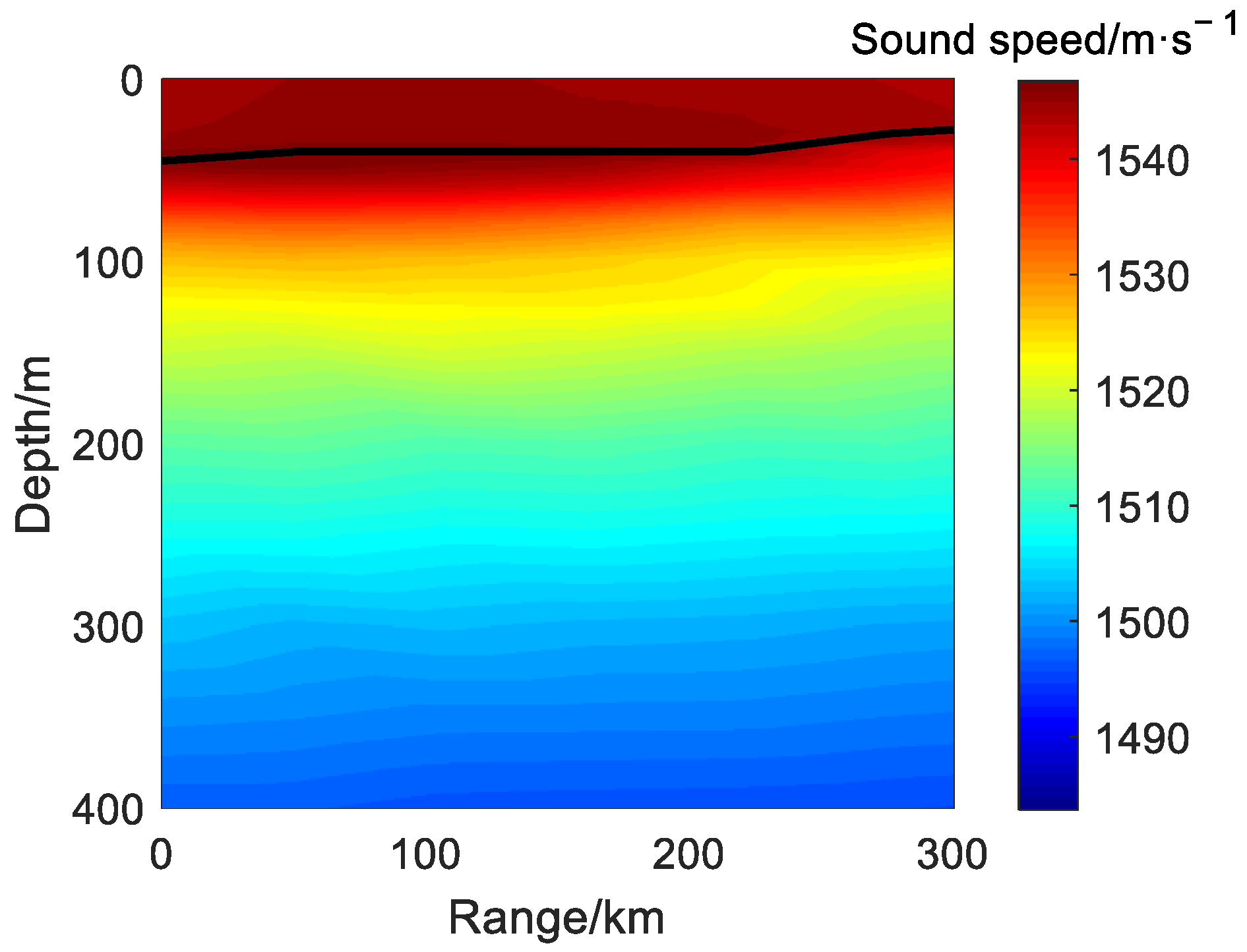
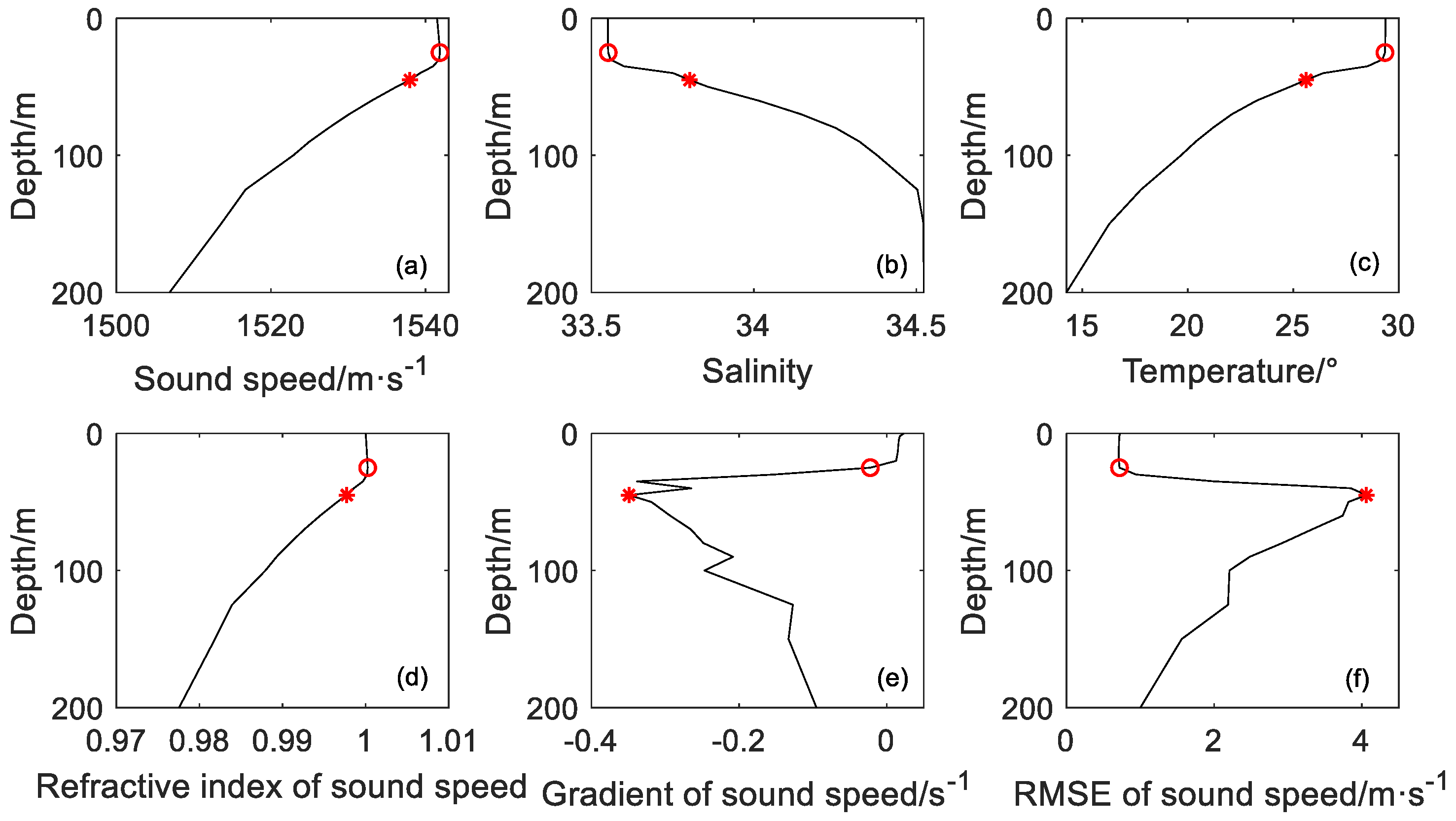
4.1. Experimental Setup and Data Processing
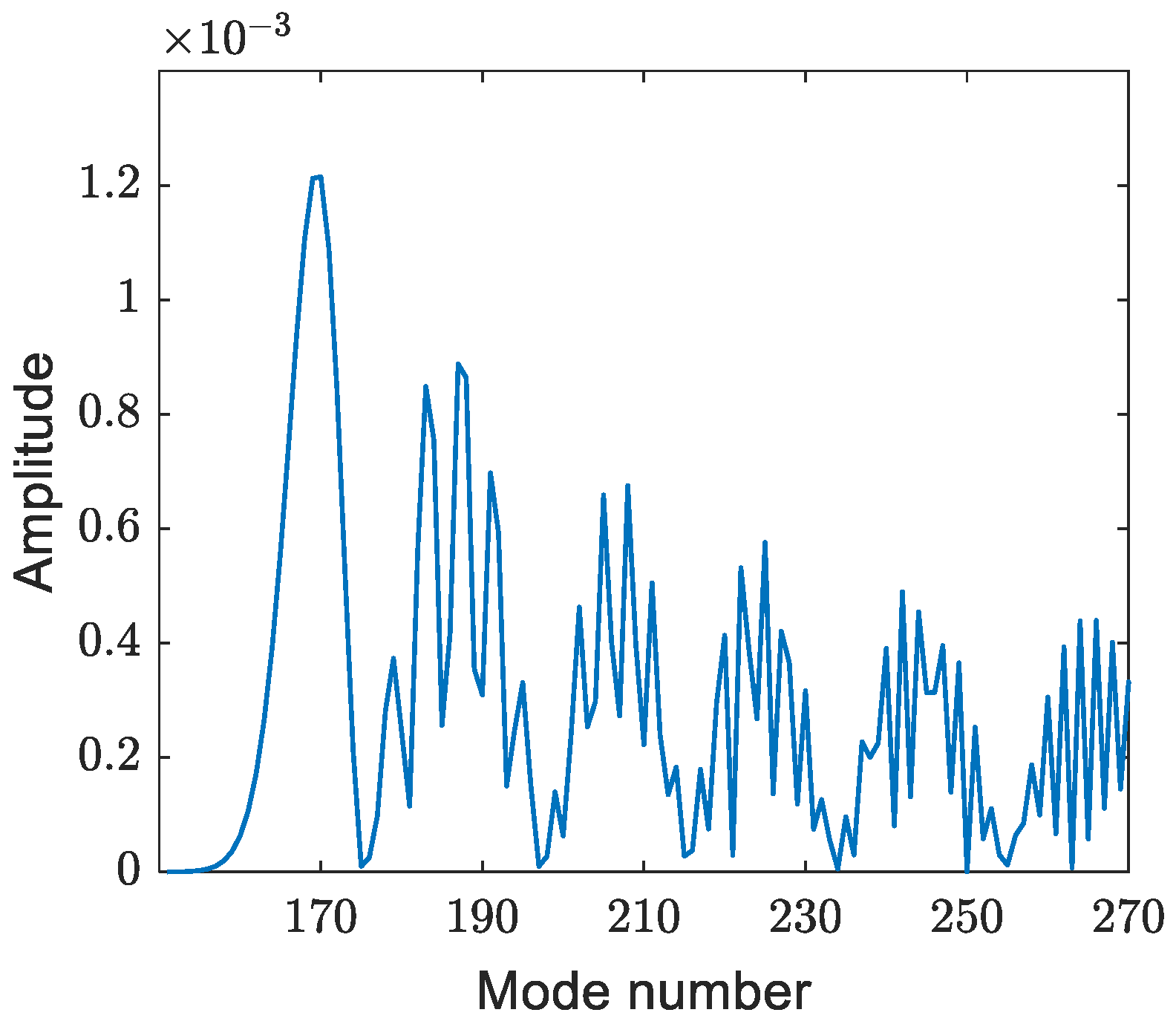
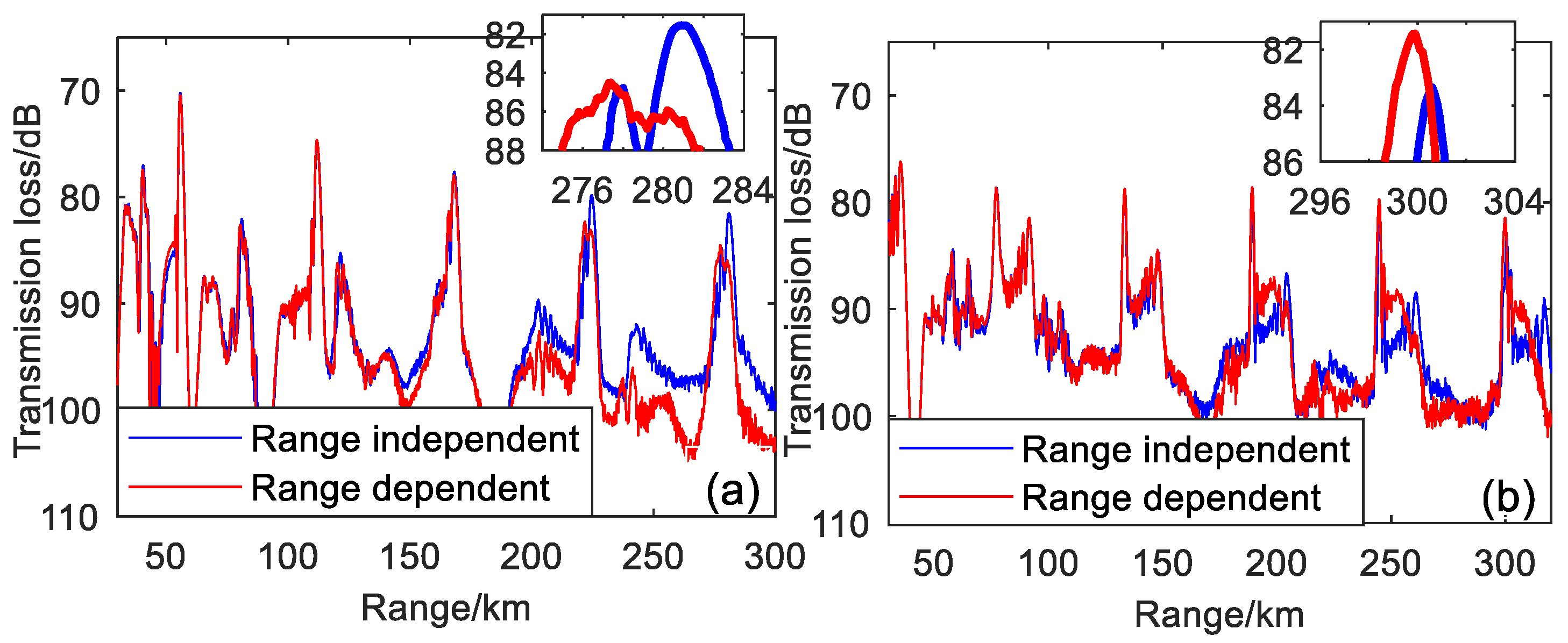
4.2. Experimental Results and Analysis
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Urick, R.J. Principle of Underwater Sound; McGraw-Hill: New York, NY, USA, 1983; pp. 79–162. [Google Scholar]
- Woezel, J.L.; Ewing, M.; Pekeris, C.L. Explosion Sounds in Shallow Water. Geol. Soc. Am. Mem. 1948, 27, 1–62. [Google Scholar] [CrossRef]
- Brekhovskikh, L.M. Propagation of acoustic and infrared waves in natural waveguides over long distances. Sov. Phys. Usp. 1960, 3, 159–166. [Google Scholar] [CrossRef]
- Urick, R.J. Caustics and Convergence Zones in Deep-Water Sound Transmission. J. Acoust. Soc. Am. 1965, 38, 348–358. [Google Scholar] [CrossRef]
- Schulkin, M. Surface-Coupled Losses in Surface Sound Channels. J. Acoust. Soc. Am. 1968, 44, 1152–1154. [Google Scholar] [CrossRef]
- Bongiovanni, K.P.; Siegmann, W.L.; Ko, D.S. Convergence zone feature dependence on ocean temperature structure. J. Acoust. Soc. Am. 1996, 100, 3033–3041. [Google Scholar] [CrossRef]
- Zhang, R.H. Normal mode sound field in shallow sea surface channel. Acta Phys. Sin. 1975, 24, 200–209. [Google Scholar] [CrossRef]
- Wang, L.; Wang, K.P. Varieties of Sound Propagating in Convergence Zone Caused by Sound Spring Layer. Mar. Geod. Cartogr. 2014, 34, 40–42. [Google Scholar] [CrossRef]
- Chen, C.; Yan, F.G.; Jin, T.; Zhou, Z.Q. Investigating acoustic propagation in the Sonic Duct related with subtropical mode water in Northwestern Pacific Ocean. Appl. Acoust. 2020, 169, 107478. [Google Scholar] [CrossRef]
- Yang, F.; Wang, H.; Gao, W.D.; Meng, X.S. Zoning of sound speed profile types and characteristics of convergence zone in the deep North Atlantic Ocean. Mar. Forecast. 2021, 38, 103–110. [Google Scholar] [CrossRef]
- Bi, S.Z.; Peng, Z.H. Effect of earth curvature on long range sound propagation. Acta Phys. Sin. 2021, 70, 114303. [Google Scholar] [CrossRef]
- Henrick, R.F.; Siegmann, W.L. General analysis of ocean eddy effects for sound transmission applications. J. Acoust. Soc. Am. 1977, 62, 860–870. [Google Scholar] [CrossRef]
- Rudnick, D.L.; Munk, W. Scattering from the mixed layer base into the sound shadow. J. Acoust. Soc. Am. 2006, 120, 2580–2594. [Google Scholar] [CrossRef]
- Colosi, J.A.; Rudnick, D.L. Observations of upper ocean sound-speed structures in the North Pacific and their effects on long-range acoustic propagation at low and mid-frequencies. J. Acoust. Soc. Am. 2020, 148, 2040–2060. [Google Scholar] [CrossRef] [PubMed]
- Li, J.X.; Zhang, R. Ocean mesoscale eddy modeling and its application in studying the effect on underwater acoustic propagation. Mar. Sci. Bull. 2011, 30, 37–46. [Google Scholar] [CrossRef]
- Colosi, J.A.; William, Z.L. Sensitivity of mixed layer duct propagation to deterministic ocean features. J. Acoust. Soc. Am. 2021, 149, 1969–1978. [Google Scholar] [CrossRef]
- White, A.W.; Henyey, F.S. Internal tides and deep diel fades in acoustic intensity. J. Acoust. Soc. Am. 2016, 140, 3952–3962. [Google Scholar] [CrossRef]
- Zhang, L.; Liu, D. Deep-sea acoustic field effect under mesoscale eddy conditions. Mar. Sci. 2020, 44, 66–73. [Google Scholar] [CrossRef]
- Piao, S.C.; Li, Z.Y. Lower turning point convergence zone in deep water with an incomplete channel. Acta Phys. Sin. 2021, 70, 024301. [Google Scholar] [CrossRef]
- Wu, S.L.; Li, Z.L.; Qin, J.X.; Wang, M.Y. Influence of tropical dipole in the East Indian Ocean on acoustic convergence region. Acta Phys. Sin. 2022, 71, 134301. [Google Scholar] [CrossRef]
- Chandrayadula, T.K.; Periyasamy, S.; Colosi, J.A. Observations of low-frequency, long-range acoustic propagation in the Philippine Sea and comparisons with mode transport theory. J. Acoust. Soc. Am. 2020, 147, 877–897. [Google Scholar] [CrossRef]
- Tindle, C.T.; Guthrie, K.M. Rays as interfering modes in underwater acoustics. J. Sound Vib. 1974, 34, 291–295. [Google Scholar] [CrossRef]
- Beilis, A. Convergence zone positions via ray mode theory. J. Acoust. Soc. Am. 1983, 74, 171–180. [Google Scholar] [CrossRef]
- Shaji, C.; Wang, C.; Halliwell, G.R. Simulation of tropical Pacific and Atlantic Oceans using a HYbrid Coordinate Ocean Model. Ocean Model. 2005, 9, 253–282. [Google Scholar] [CrossRef]

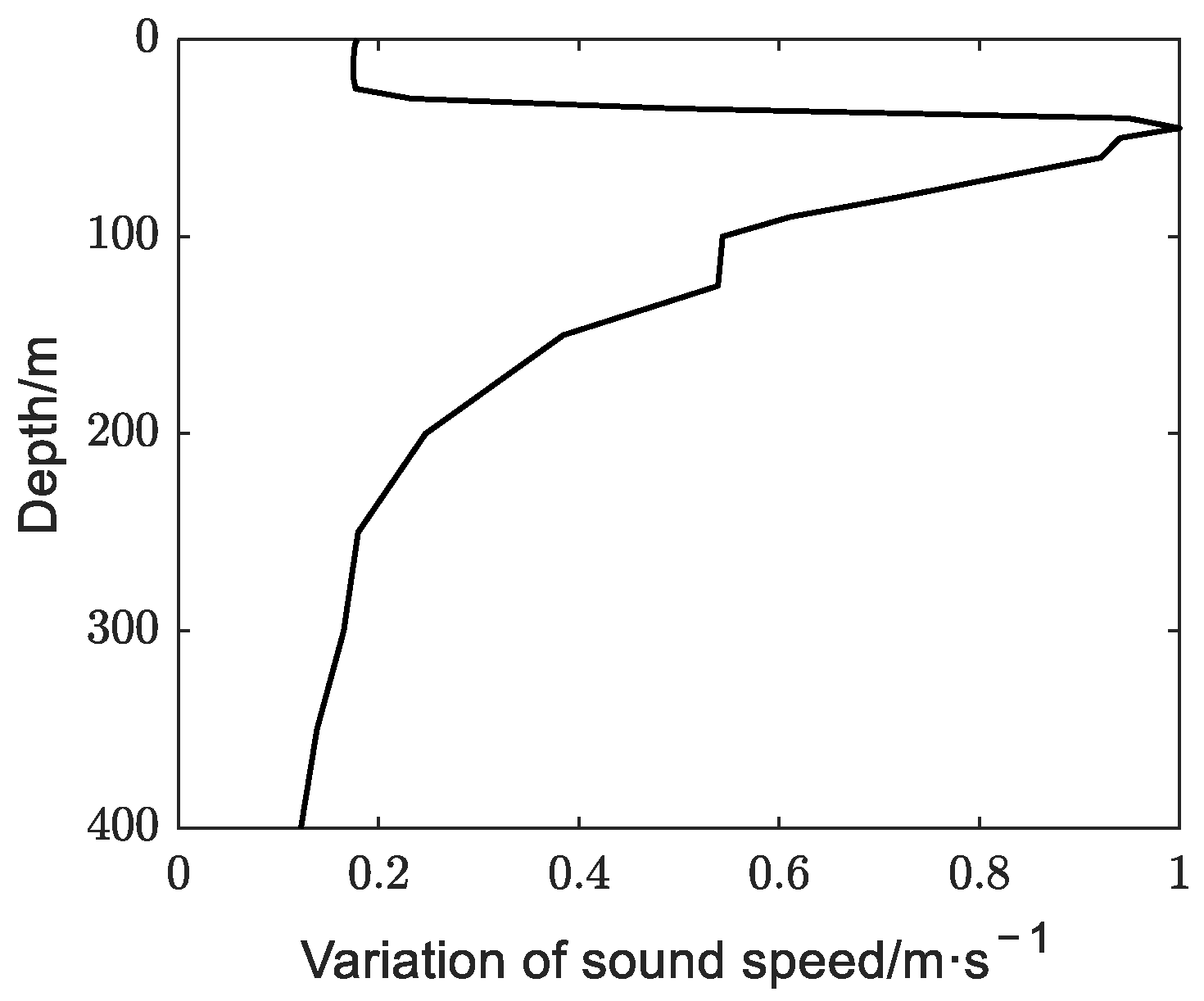
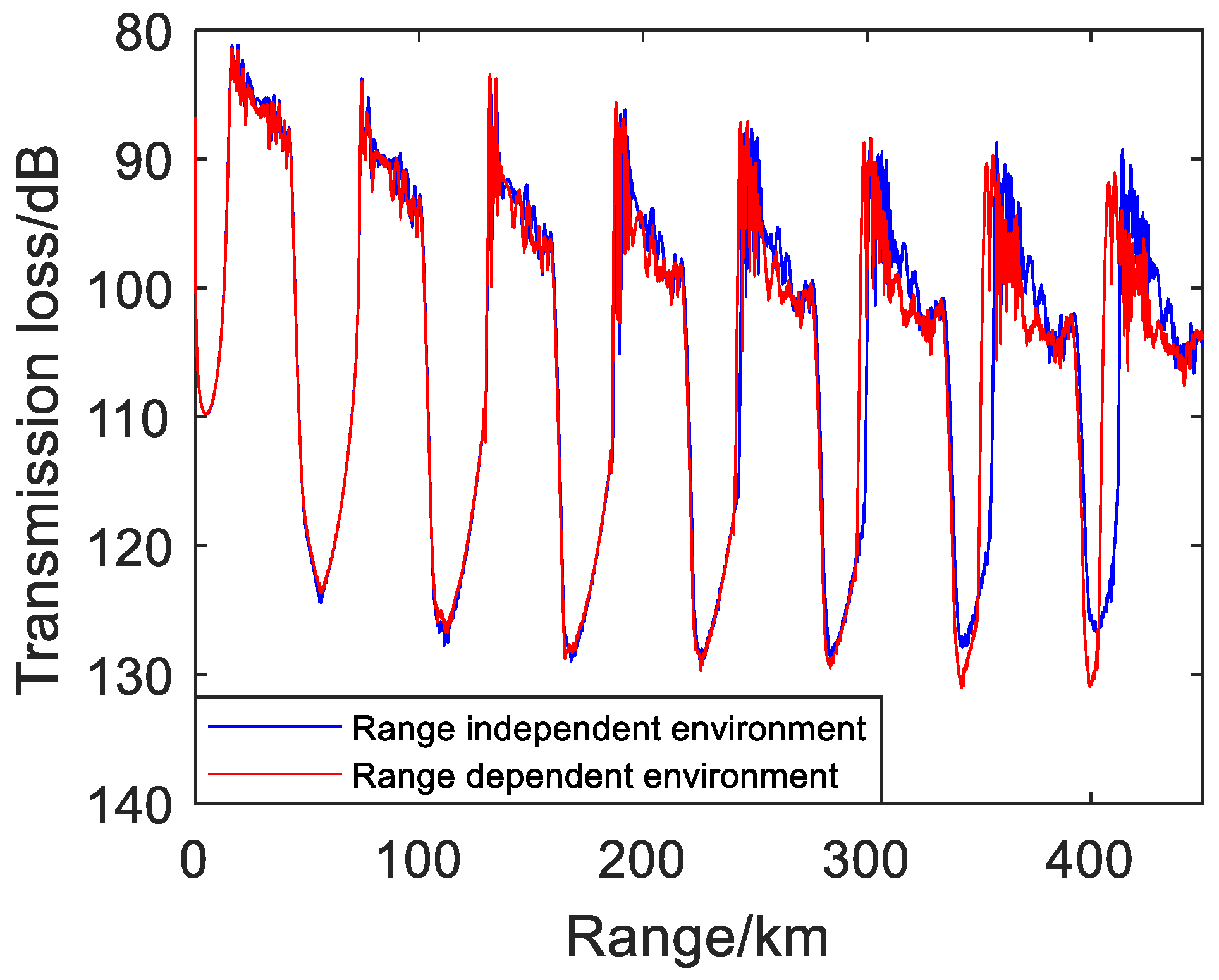

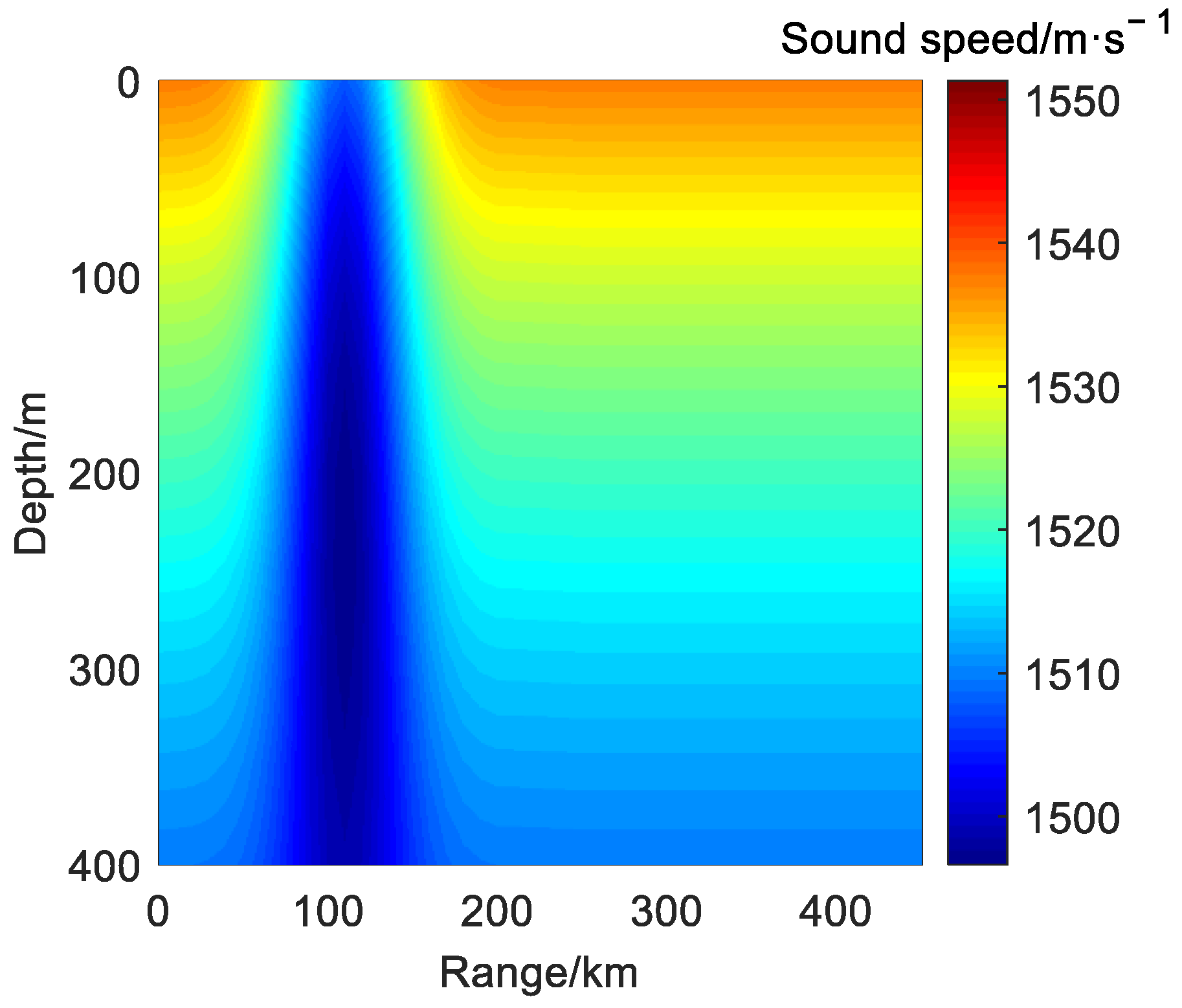

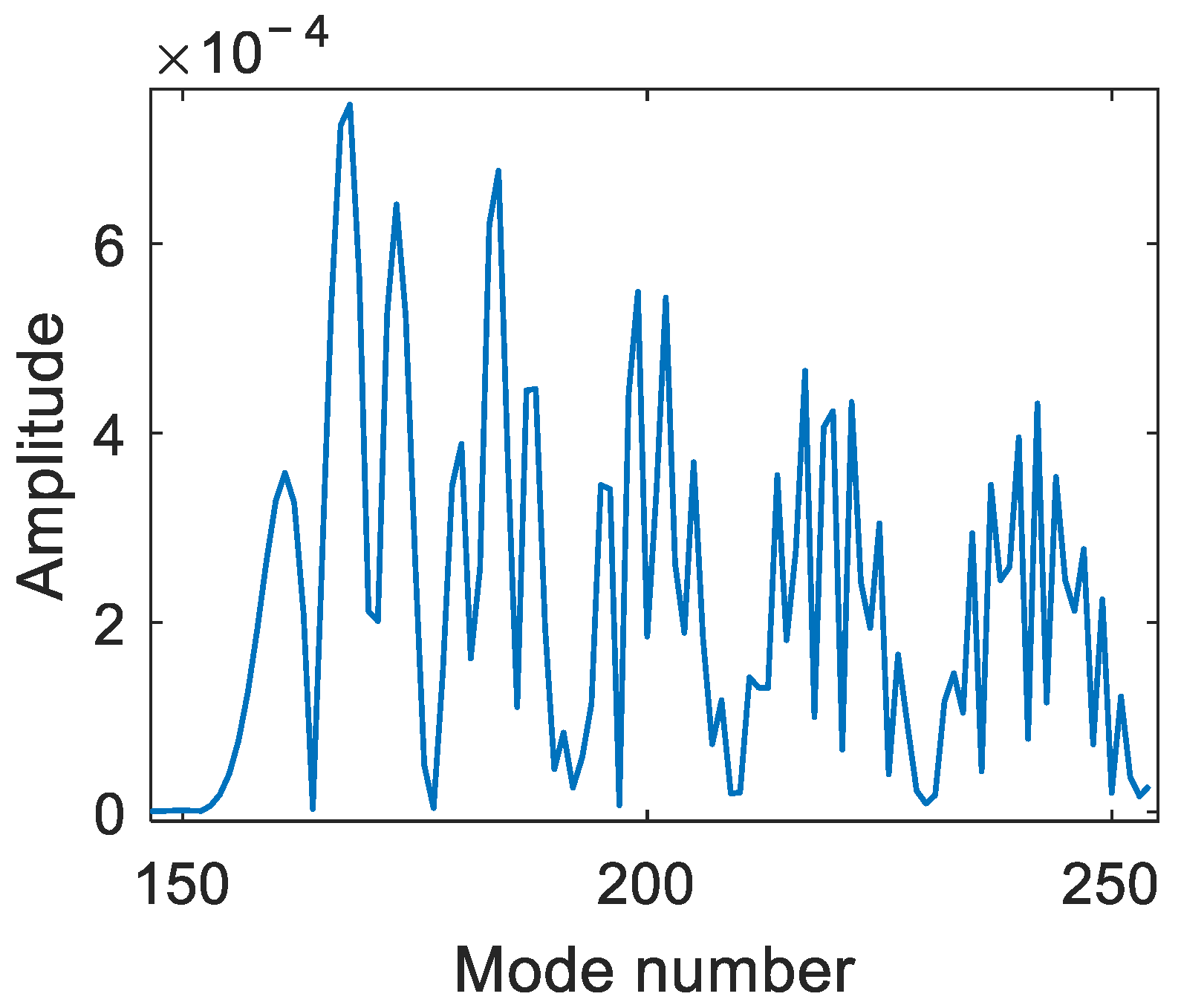


| Number of Convergence Zones, m | /km | /km | /km |
|---|---|---|---|
| 1 | 76.6 | 76.6 | 76.6 |
| 2 | 134.5 | 134.4 | 134.4 |
| 3 | 192.0 | 191.1 | 190.0 |
| 4 | 248.5 | 246.6 | 245.6 |
| 5 | 305.0 | 301.9 | 301.3 |
| 6 | 361.5 | 356.3 | 356.8 |
| 7 | 417.9 | 411.1 | 412.4 |
| m | /km | /km | /km |
|---|---|---|---|
| 1 | 76.6 | 76.4 | 76.4 |
| 2 | 134.5 | 131.4 | 131.1 |
| 3 | 192.0 | 187.2 | 186.7 |
| 4 | 248.5 | 243.4 | 242.5 |
| 5 | 305.0 | 299.7 | 298.3 |
| 6 | 361.5 | 355.9 | 354.1 |
| 7 | 417.9 | 412.0 | 409.9 |
| m | /km | /km | /km | /km |
|---|---|---|---|---|
| 1 | 72.7 | 72.6 | 73.6 | 72.6 |
| 2 | 124.9 | 124.4 | 122.1 | 124.3 |
| 3 | 175.2 | 174.3 | 173.7 | 173.8 |
| 4 | 226.4 | 224.9 | 225.2 | 224.3 |
| 5 | 277.6 | 275.8 | 275.6 | 274.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Piao, S.; Zhang, M.; Gong, L. Influence of Range-Dependent Sound Speed Profile on Position of Convergence Zones. Remote Sens. 2022, 14, 6314. https://doi.org/10.3390/rs14246314
Li Z, Piao S, Zhang M, Gong L. Influence of Range-Dependent Sound Speed Profile on Position of Convergence Zones. Remote Sensing. 2022; 14(24):6314. https://doi.org/10.3390/rs14246314
Chicago/Turabian StyleLi, Ziyang, Shengchun Piao, Minghui Zhang, and Lijia Gong. 2022. "Influence of Range-Dependent Sound Speed Profile on Position of Convergence Zones" Remote Sensing 14, no. 24: 6314. https://doi.org/10.3390/rs14246314
APA StyleLi, Z., Piao, S., Zhang, M., & Gong, L. (2022). Influence of Range-Dependent Sound Speed Profile on Position of Convergence Zones. Remote Sensing, 14(24), 6314. https://doi.org/10.3390/rs14246314






