1. Introduction
Synthetic aperture radar (SAR) is an active microwave sensor that is able to obtain a high-resolution image of a scene, regardless of the weather and daylight [
1,
2]. Moreover, modern SAR usually has the operation mode of ground moving target indication (GMTI). SAR-GMTI systems can image the illustrated area and indicate ground moving targets in the SAR image simultaneously, and have played an important role in applications such as traffic monitoring and military reconnaissance [
3,
4,
5,
6,
7].
With the development of satellite technology, GMTI with spaceborne SAR has attracted a lot of attentions in recent years [
8,
9,
10]. Low-Earth-orbit (LEO) spaceborne SAR-GMTI has been widely studied. However, since the altitude of the orbit is relatively low, LEO SARs suffer the problems of long revisit time and limited coverage, which severely limits their applications in GMTI. To address this issue, many attentions have been paid to SARs with higher orbital altitude [
11,
12,
13,
14], such as medium-Earth-orbit (MEO) and geosynchronous-orbit (GEO) SARs. Compared with GEO SAR, MEO SAR has shorter synthetic aperture time, lower transmission cost and lower system complexity, and thus is more suitable for GMTI applications.
Many studies on MEO SAR have been conducted in recent years [
15,
16,
17,
18,
19,
20]. The observation performance of MEO SAR and the signal characteristics of static targets had been studied in [
15]. In [
16], the author studied the orbit design, coverage, and revisit time of MEO SAR, and concluded that MEO SAR had great value due to its advantages of wide coverage and short revisit time. In [
17,
18,
19], the imaging of the stationary scene was studied, and several imaging algorithms that could address the 2-D spatial of the signal and the curvature of the radar track were proposed. However, studies on MEO SAR-GMTI are still rare.
The focusing of ground moving targets can improve the signal-to-clutter-noise ratio (SCNR), thus improving the GMTI performance of the system [
21,
22]. Particularly for MEO SAR, the high orbital altitude makes it suffer the problems of low signal-to-noise ratio (SNR) and limited computation resource. Therefore, it is essential to propose an imaging method with high computational efficiency for MEO SAR-GMTI systems to improve their performance. However, the existing imaging methods of MEO SAR [
17,
18,
19] are designed only for the stationary scene and thus may not be suitable for SAR-GMTI systems. For example, [
19] proposed an imaging algorithm based on a novel coordinate system. Since the imaging coordinate used in this algorithm depends on the Doppler parameters of the stationary scene, it cannot accurately correct the range cell migrations (RCMs) of moving targets.
In this paper, we propose an efficient imaging method suitable for the MEO SAR-GMTI system. This method is designed with the consideration of both the stationary scene and ground moving targets. It utilizes the fact that, in a 2-D frequency domain, the couplings between the range and azimuth of static targets and the moving targets of no Doppler ambiguity are approximately identical, and thus the RCMs of these targets can be corrected simultaneously via a phase multiplication. The proposed imaging method is very efficient because it needs only four Fourier transforms (FTs) and twice phase multiplications. Thus, the proposed imaging method is very suitable for MEO SAR, which suffers the problem of limited energy and computation resources.
The analysis and understanding of moving targets’ signal characteristics in the image domain are very important for the development of the GMTI method [
23], e.g., the clutter suppression and motion parameter estimation methods. Therefore, one other objective of this paper is to reveal moving targets’ signal characteristics in the SAR image obtained by the proposed imaging method. Specifically, in the paper, the analytical expressions for the azimuth and range displacements and the along-track interferometric (ATI) phase of the target are derived, and their variation with the target’s parameters are investigated.
Note that the MEO SAR-GMTI system in this paper is assumed to have a relatively low resolution. The considerations are as follows: (1) A relatively low resolution can improve the SNR and reduce the data volume. This is important for MEO SAR, which suffers limited energy and computation resources and relatively high system complexity because of the increased orbital altitude; (2) For GMTI, a relatively low resolution can reduce the probability of Doppler ambiguity of moving targets.
The remainder of this paper is organized as follows. In
Section 2, the target’s range equation model and 2-D spectrum for MEO multichannel SAR are derived. The proposed method is introduced in
Section 3. The signal characteristics of the target in SAR image are analyzed in
Section 4. In
Section 5, experimental results are presented to verify the work of this paper. In
Section 6, some discussions are presented. Finally, the paper is concluded in
Section 7.
3. Proposed Imaging Method
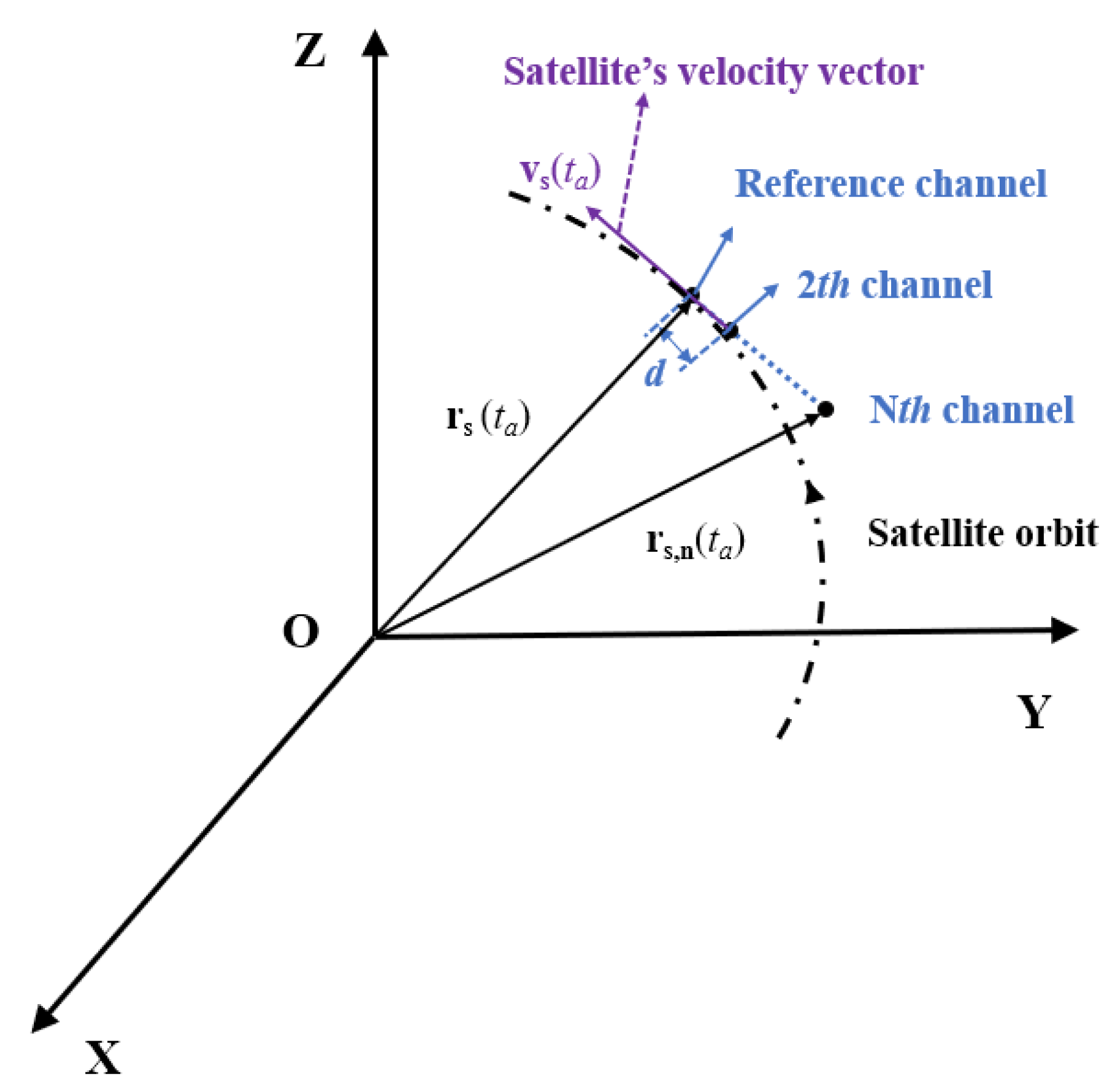
In this section, an efficient imaging method is proposed. The secondary range compression (SRC) and RCM correction (RCMC) are accomplished efficiently in the 2-D frequency domain via phase multiplications. In addition, the azimuth compression is implemented in the range Doppler domain. The main steps of this imaging method are introduced below. Note that the signal of the reference channel (i.e., n = 1) is taken as an example to describe the proposed method in this section.
3.1. Secondary Range Compression
It can be seen from (23) that there are still quadratic and cubic terms of range frequency after range compression. If these terms are not compensated, defocus will occur in the range direction. Therefore, the operation of SRC is implemented, and the filter is constructed as follows
with
where
l10,
l20 and
l30 define the coefficients of the linear, quadratic, and cubic terms of range history of the static target located at the center of the illuminated scene.
Comparing (25) with (28) and (26) with (29), respectively, one can see that there is a residual phase error after SRC by (27), and it can be expressed as
This phase error is very small (about 10
−10) and much less than π/4, and thus it can be neglected. Therefore, the signal after SRC is given by
3.2. Range Cell Migration Correction
In order to improve the efficiency, a phase multiplication in 2-D frequency domain is used to accomplish RCMC. Based on (31), the RCM of target can be corrected by compensating the term containing
fr. Therefore, the RCMC filter can be constructed as follows
By multiplying (32) into (31) and then performing inverse FT (IFT), one can obtain the range-Doppler domain signal as follows:
From the range envelope of (33), one can see that there is still a residual RCM, which can be expressed as
Considering –
PRF/2 <
fa <
PRF/2, the maximum possible value of the residual RCM is given by
For the targets moving with such a radial velocity that there is no Doppler ambiguity (i.e.,
M = 0),
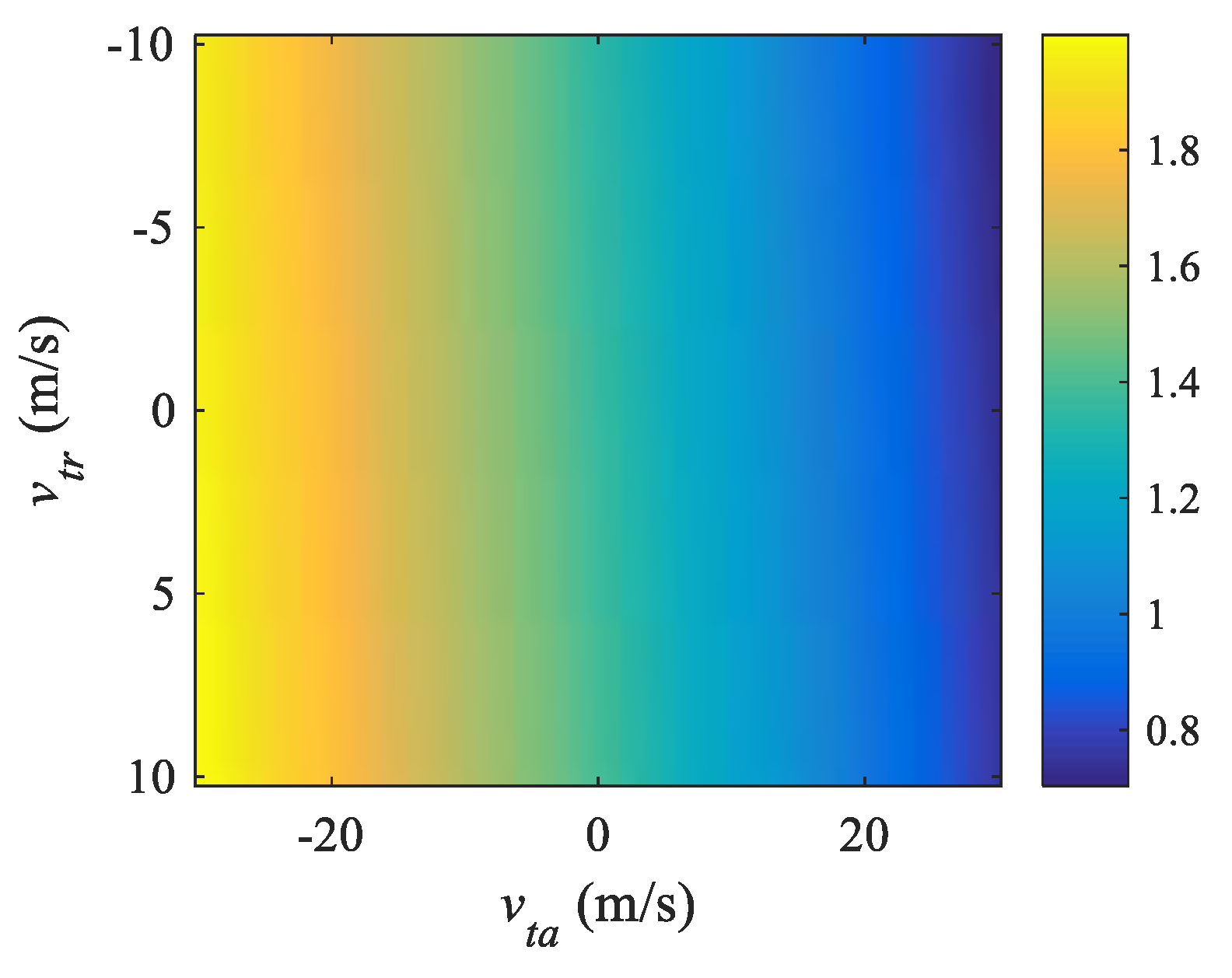
will be smaller than half of a range resolution cell, and thus the residual RCM can be neglected. To validate this statement, numerical simulations are conducted. The MEO SAR parameters for simulations are presented in
Table 1. The result is illustrated in
Figure 3, which shows the
of the targets with all possible velocities (note that, when there is no Doppler ambiguity,
vtr will be less than about 10 m/s). As can be seen from
Figure 3, the maximum value of
is about 1.8 m. Therefore,
will not exceed half the range resolution cell in the cases that the range resolution is not high.
The RCMC filter shown in (32) is constructed without any parameter of the target. Therefore, this filter can simultaneously correct the RCMs of static scenes and the multiple moving targets of no Doppler ambiguity. Note that after the RCM is corrected there will be no defocusing in the range direction after azimuth compression.
3.3. Azimuth Compression
To finely focus the static scene, we perform the azimuth compression in the range Doppler domain. Based on (33), the azimuth compression filter is constructed as follows
The target signal after azimuth compression is given by (the negligible residual RCM is omitted)
The focused SAR image can be obtained via applying an azimuth IFT to (37). From (37) it can be seen that, for static targets, they can be finely focused. For moving targets, since there are residual azimuth modulations, they will suffer from azimuth defocusing. It should be noted that, since the RCMs of the moving targets without Doppler ambiguity have been corrected, the range defocusing will not occur after azimuth compression. Therefore, the SCNR of the target can be improved, which will improve the GMTI performance of the system.
4. Property Analysis of Moving Target in the Complex Image Domain
Analyzing and understanding the moving targets’ characteristics in the SAR image is of great significance for moving target indication. However, it is difficult to derive the analytical expression of the target signal in complex image domain, because there is residual azimuth modulation after azimuth compression. In this section, some properties of the target signal in the image obtained by the proposed imaging method, e.g., the target’s range displacement, azimuth displacement, and ATI phase, are quantitatively analyzed.
4.1. Range Displacement
The target’s location in range is displaced from
R0, which can be seen from the range envelope of (37). Thus, the target will suffer range displacement after focusing. Based on (37), the range displacement can be calculated as
Since the terms containing
l3 are smaller than a range cell, they can be neglected. Therefore,
rshift can be simplified as
where
fb defines the target’s baseband Doppler center frequency, and it can be expressed as
.
In order to reveal the relationship between the range displacement and the target’s parameters, the expressions of
l2 and
fb are substituted into (39), and
rshift can be rewritten as
Note that, since is far smaller than , it is ignored.
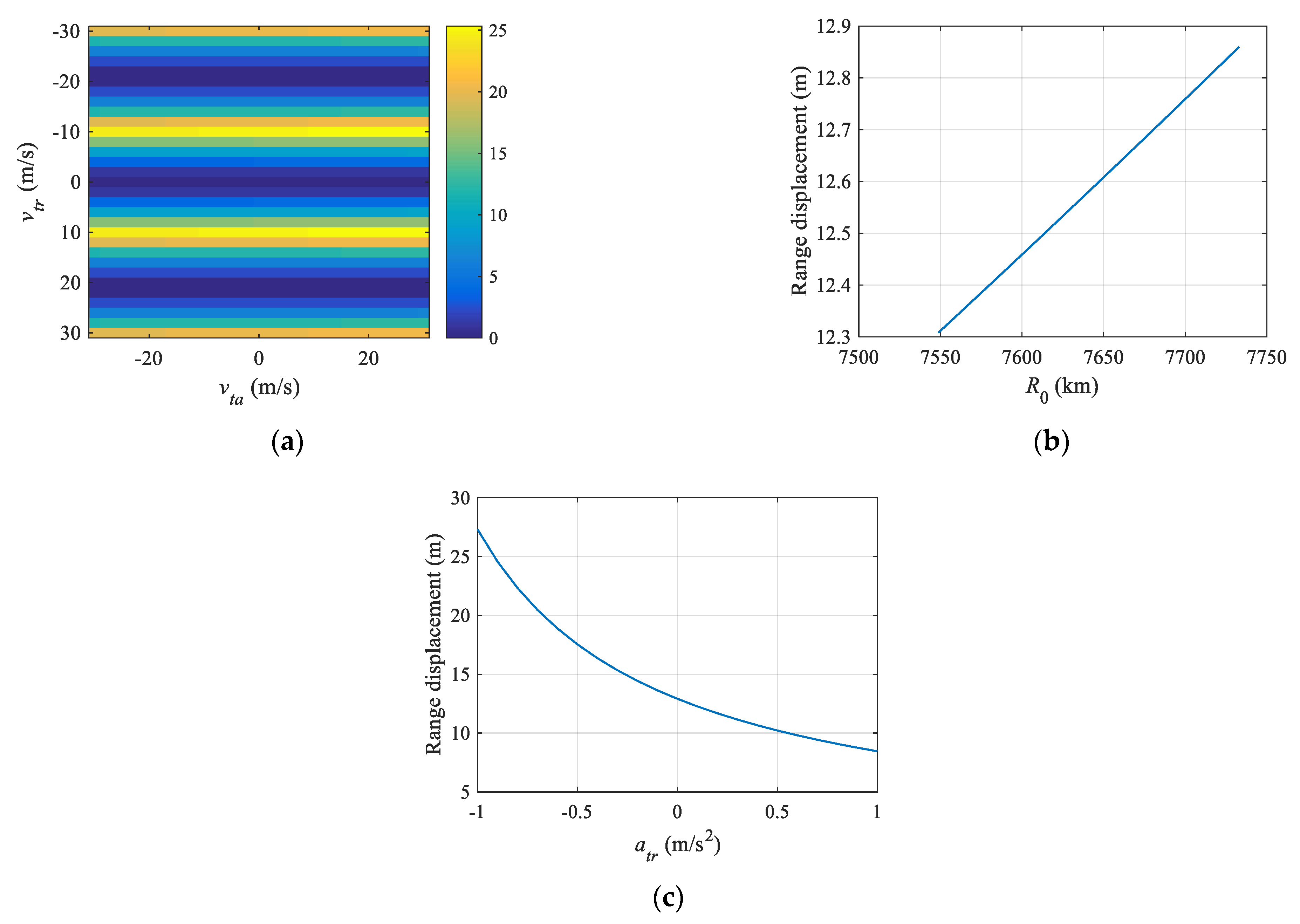
From (39) one can see that the target’s range displacement is caused by its radial velocity, i.e., the range displacement will be equal to zero when the radial velocity is zero. Moreover, it is interesting that the range displacement is dependent on its baseband Doppler center frequency rather than the absolute Doppler center frequency. This property indicates that the range displacement is independent of Doppler ambiguity and will not be monotonically increasing with the target’s radial velocity. In addition, from (40) one can see that the range displacement is also related to the target’s radial acceleration, along-track velocity, and distance. However, it is very difficult to find out the variation of the range displacement with these parameters directly from (40). To address this issue, numerical simulations are conducted, with the results being presented in
Figure 4.
Figure 4a illustrates the variation of the range displacement with the velocities of the target. It can be seen that the range displacement varies with
vtr periodically and gets the maximum value when the baseband Doppler center frequency equals to
PRF/2. In addition, one can see that the range displacement is almost independent of
vta.
Figure 4b,c show the variation of the range displacement with the distance and radial acceleration of the target, respectively. It can be seen that the range displacement increases with
R0 approximately linearly, and is inversely proportional to
atr.
4.2. Azimuth Displacement
Besides the range displacement, the motion of the target will also lead to an azimuth offset in SAR image. According to (37) and the properties of FT, the target’s azimuth displacement can be expressed as
where
vg is the velocity of the beam sweeping along the surface of earth.
Substituting the expressions of
l1,
l2 and
l3 into (41),
ashift can be rewritten as
Note that since the value of is much smaller than , it can be ignored.
From (42), one can see that the azimuth displacement is also caused by the target’s radial velocity and is dependent on the baseband Doppler center frequency rather than the absolute Doppler center frequency. Moreover, the azimuth displacement is also dependent on the target’s along-track velocity, accelerations, and distance. In order to reveal the variation of the range displacement with these parameters, numerical experiments are carried out, with the results presented in
Figure 5.
From
Figure 5a it can be seen that the azimuth displacement varies with
vtr periodically and gets the maximum value when the baseband Doppler center frequency equals to
PRF/2. In addition, one can see that the range displacement is almost independent of
vta. The variation of the azimuth displacement with the target’s distance and accelerations are shown in
Figure 5b,c. It can be seen that the range displacement increases with
R0 approximately linearly. From
Figure 5c one can see that the azimuth displacement is inversely proportional to
atr and almost independent of
ata.
4.3. Along-Track Interferometry Phase
ATI phase is a very important parameter in the SAR-GMTI field. It can be utilized to estimate the radial velocity of the target. In addition, moving targets can be detected according to the interference phase diagram.
Based on (37), the signal of the second channel after registration is:
According to (37) and (43), the interference phase between channel 1 and channel 2 can be expressed as
where * represents a conjugate operation on the signal.
Since the items containing
d2α2,
d3α3 and
l3 are much smaller than other items, they can be ignored. Therefore,
can be simplified to
In order to find out the variation of ATI phase with the target’s parameters, the expressions of
α and
l2 are substituted into (45). Then, the ATI phase can be rewritten as:
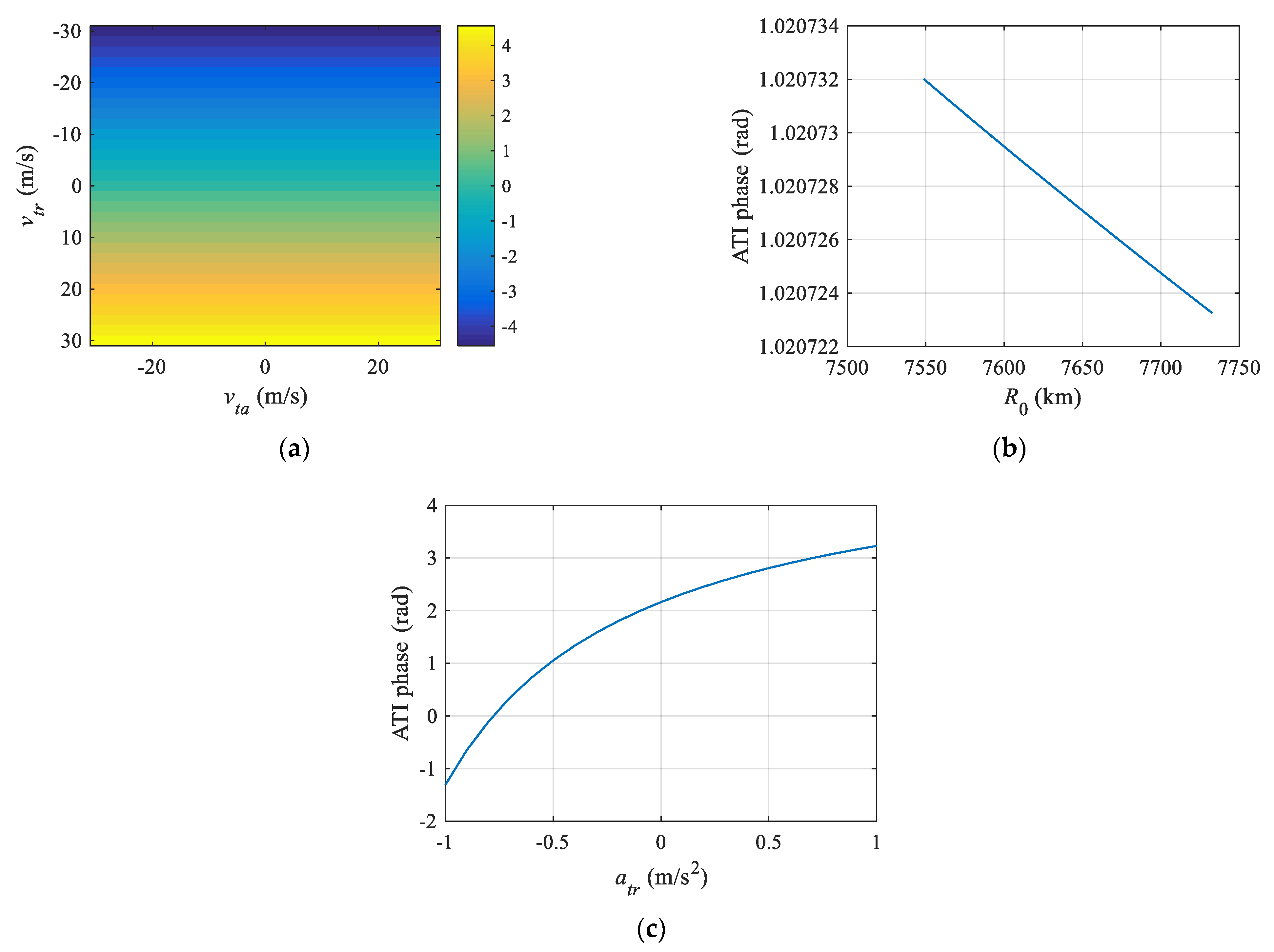
It can be seen from (46) that the ATI phase is also caused by the target’s radial velocity and is only dependent on the radial velocity when there is no Doppler ambiguity. For the cases where Doppler ambiguity is happening, in addition to the radial velocity the ATI phase depends also on the along-track velocity, radial acceleration and distance. To find out the variation of the ATI phase with these parameters, numerical experiments are carried out, with the results being presented in
Figure 6.
Figure 6a shows the relationship between the ATI phase and velocities. One can find out that the ATI phase is proportional to
vtr, while the variation of the ATI phase with
vta is very slight. In addition, the variation of ATI phase with the target’s distance and radial acceleration are shown in
Figure 6b,c. One can see that the ATI phase is inversely proportional to
R0, and that the ATI phase is proportional to
atr when there is Doppler ambiguity.
5. Experimental Results
In this section, numerical experiments are conducted to validate the proposed imaging method and the analysis presented in
Section 4. The MEO SAR parameters used for simulation are given in
Table 1. Five targets are simulated, including four moving targets and one stationary target. The parameters of these targets are given in
Table 2.
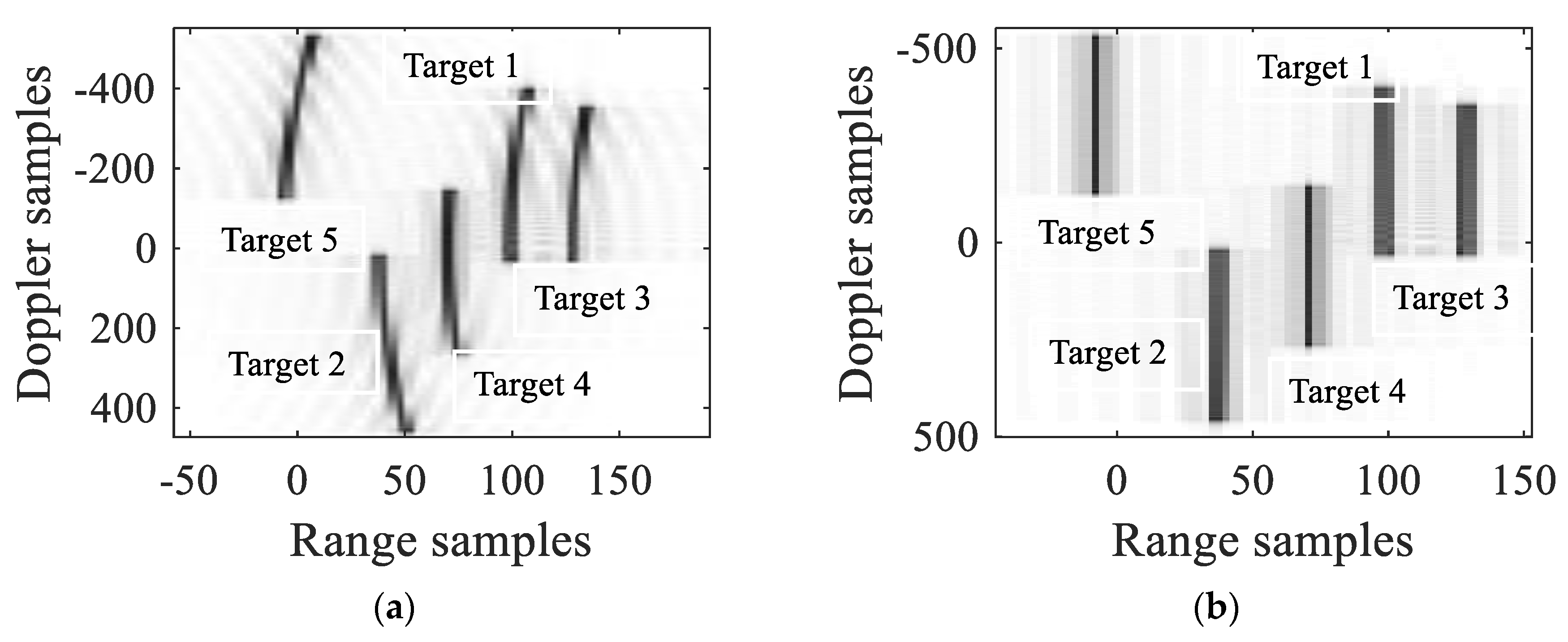
The results of RCMC are presented in
Figure 7. Comparing
Figure 7a with
Figure 7b, it is evident that the five targets’ RCMs are corrected at the same time. In addition, the residual RCMs of five targets are also calculated by (34), which are 0.42 m, 0.85 m, 0.53 m, 0 m, and 0.16 m. They are so much smaller than a range cell that can be ignored.
The azimuth compression results are given in
Figure 8, from which it can be found out that the targets are focused simultaneously. Furthermore, it can be seen that the static target (target 4) is finely focused. In addition, the four moving targets are also well focused in range direction with defocusing only in the azimuth direction.
In order to verify the target’s signal characteristic analysis presented in
Section 4, the range and azimuth displacements and the ATI phases of the four moving targets are measured, with the results shown in
Table 3. Note that the measured displacements are calculated based on target’s position in the SAR image, and thus they can only be the integer multiples of the size of a pixel. From
Table 3 it can be seen that the measured values are very close to the theoretical ones. The differences between the theoretical range displacements and the measured ones are all less than half of the range length of a pixel (i.e., 3.75 m), which indicates the measured values being exactly consistent with the theoretical ones. The differences between the theoretical azimuth displacements and measured ones are slightly larger. This could be caused by the residual cubic azimuth modulation (see Equation (37)) and azimuth defocusing.
7. Conclusions
This paper has proposed an efficient imaging method that can finely focus static scenes and coarsely focus the moving targets of no Doppler ambiguity at the same time. Based on the fact that, in the 2-D frequency domain, the couplings between the range and azimuth of static targets and the moving targets without Doppler ambiguity are approximately identical, the proposed method efficiently corrects the RCMs of these targets via a phase multiplication simultaneously. In addition, moving targets’ characteristics, including the range and azimuth displacements and ATI phases, in the SAR image focused by the proposed method have been analyzed. Furthermore, the dependence of these characteristics on the parameters of moving targets have been revealed. Experiments have been conducted to verify the proposed imaging method and theoretical analyses.
After moving targets have been efficiently and coarsely focused by the proposed method, their SCNRs will be improved. Therefore, the proposed method is very suitable for MEO SAR, which suffers from limited energy and computation resources due to the high orbital altitude. Moreover, the analysis of the target’s displacements and ATI phase in the SAR image will benefit the design of the GMTI method for MEO multichannel SAR.