Comparison between Different TomoSAR Imaging Models for Airborne Platform Flying at Low Altitude
Abstract
1. Introduction
- In a low-altitude airborne case, should the reflectivity function of the illuminated scene corresponding to a pixel in a 2-D SAR image still refer to elevation in the third dimension?
- The purpose and consequence of making the approximation to obtain the Fourier transform.
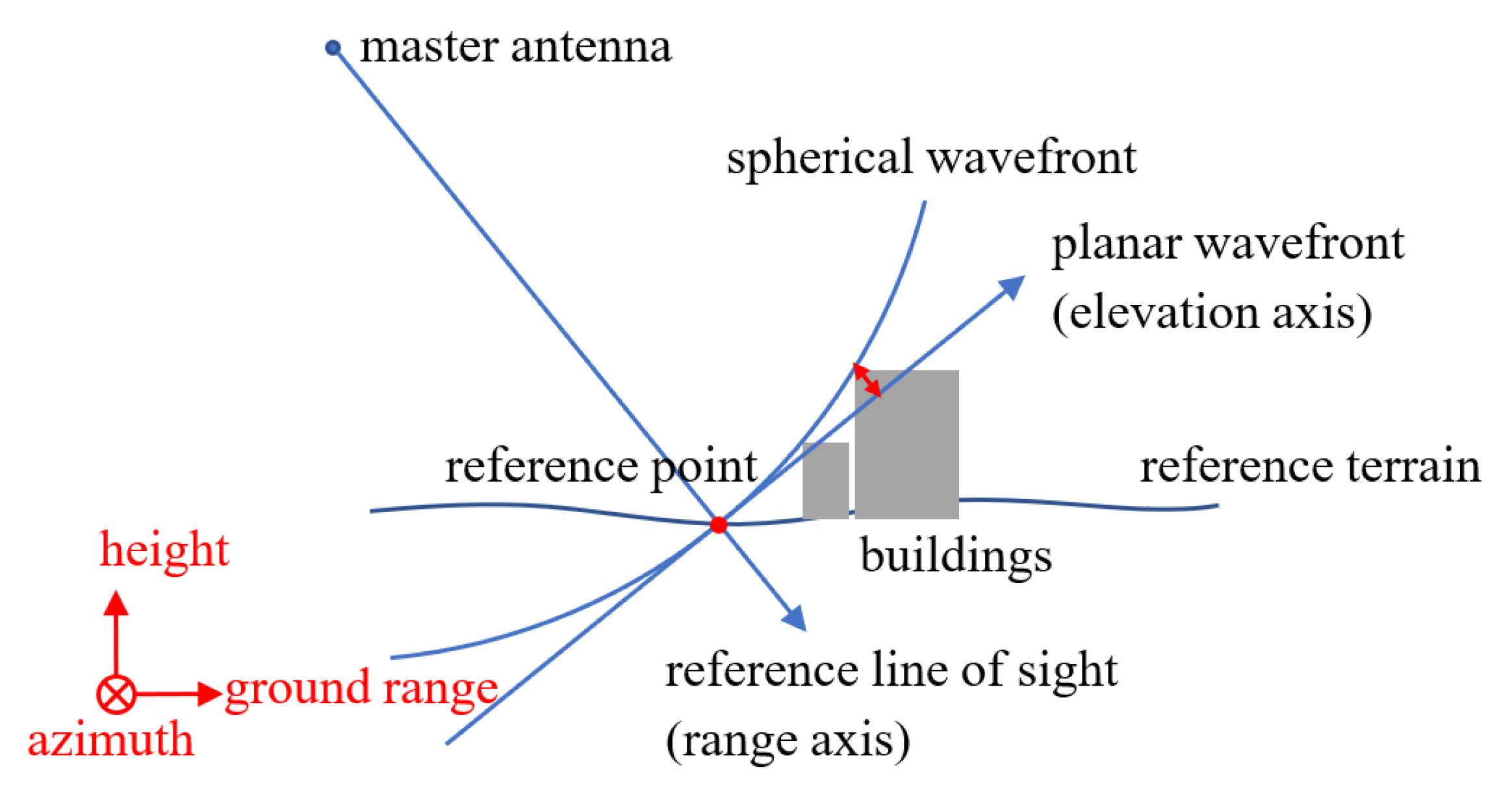
2. TomoSAR Imaging Models
- The distribution of scatterers. There are two kinds of distributions. One is a straight-line distribution and the other is an arc-line distribution;
- The formulas of the range between antennas and scatterers and the expression of the value of the 2-D SAR image. Regarding the former, for different scatterer distributions, there are different range formulas, and these formulas will lead to different approximate forms. As for the latter, the expression is formed in the radar frame. For the straight-line distribution, the radar frame is in rectangular coordinate system. For the arc-line distribution, the radar frame is in polar coordinate system;
- The geodetic coordinate transformation method. Because the expression of the value of the 2-D pixel is formed in the range-azimuth plane, the inversion results should be transformed into the geodetic coordinate system to be more intuitive.
2.1. Planar Wavefront Models
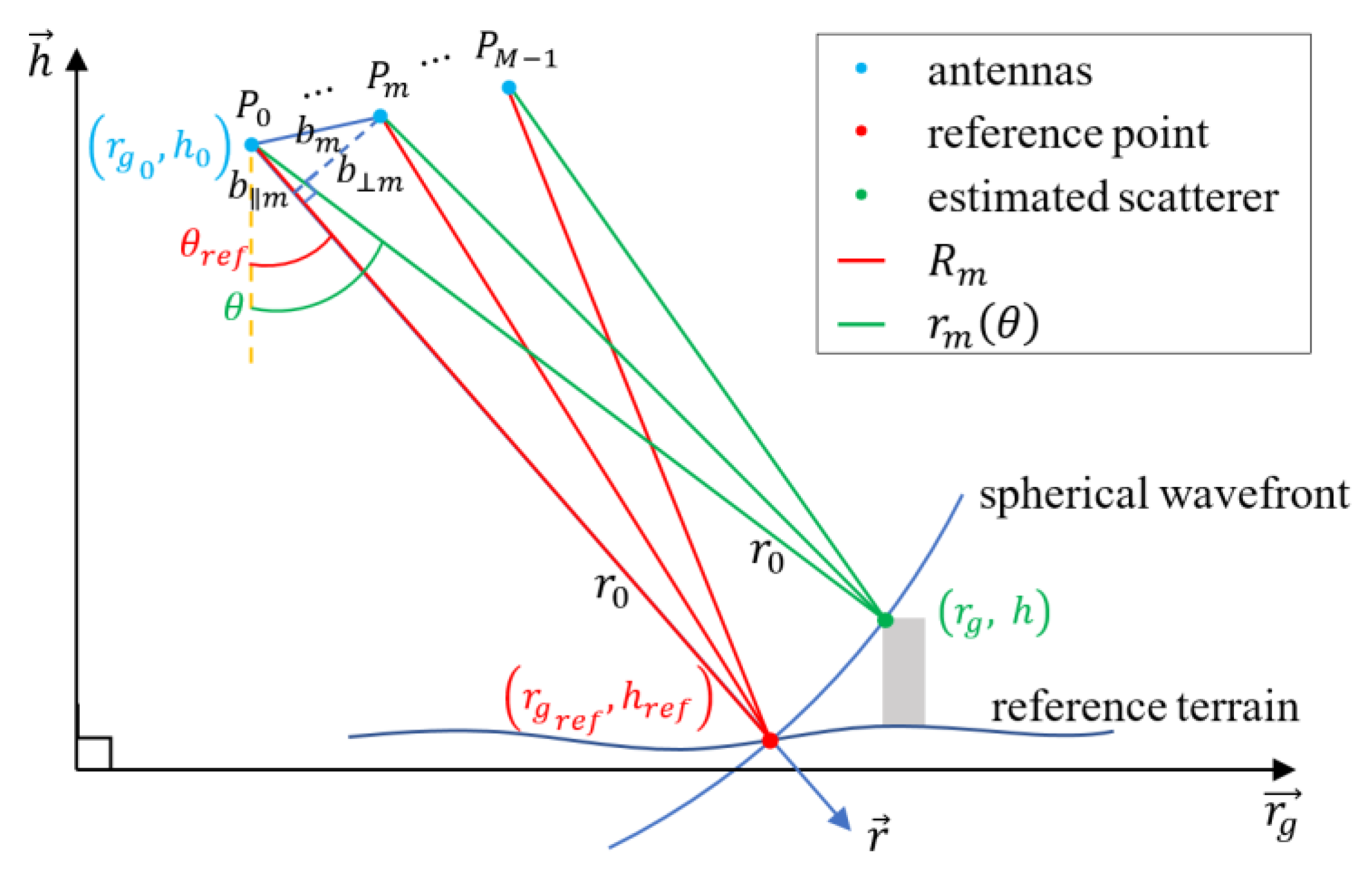
2.2. Spherical Wavefront Models
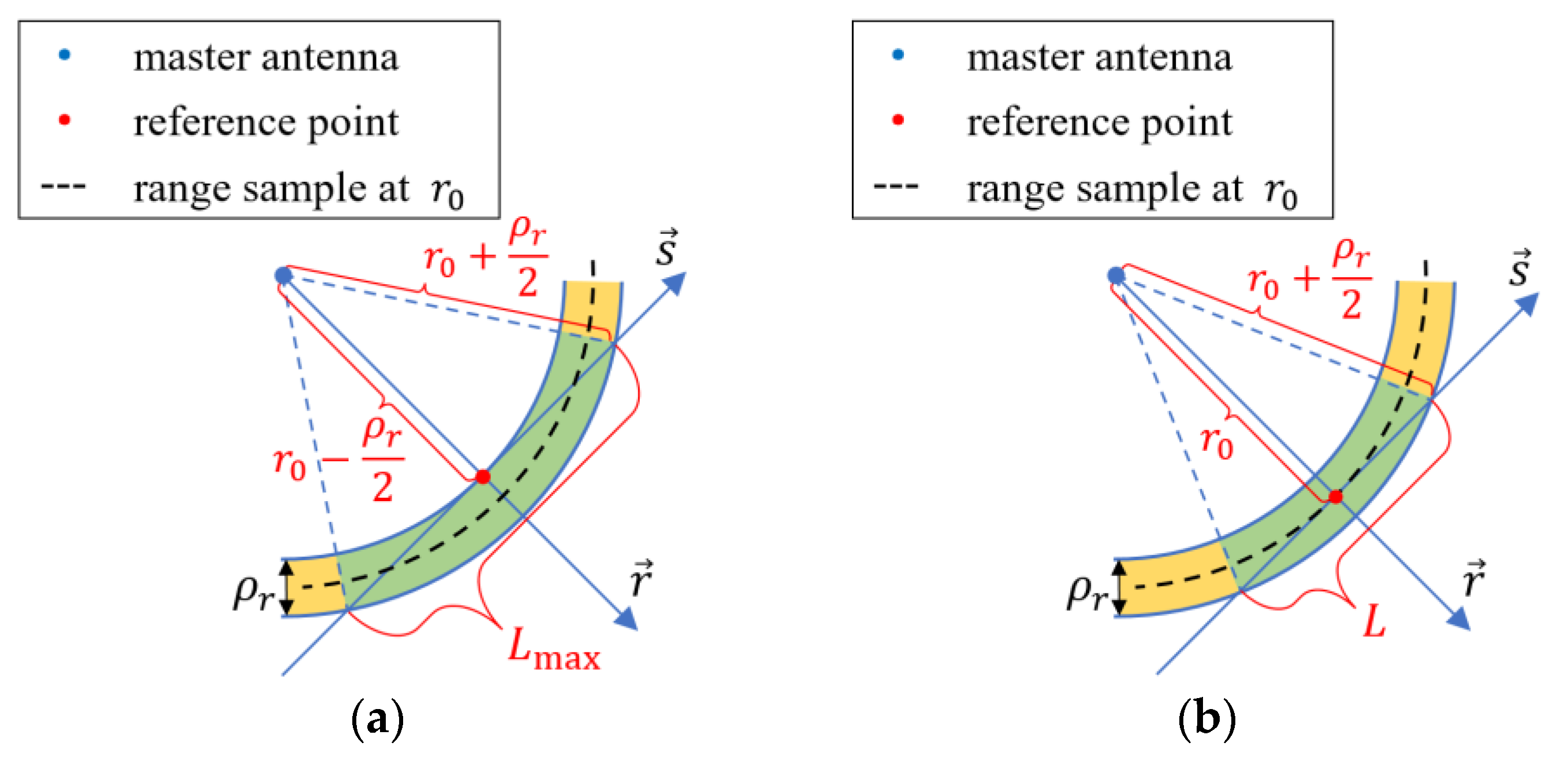
2.3. Discretization of Models
2.4. Transformation between Models
3. Experiments and Results
3.1. Experiments on Simulated Data
3.1.1. Simulated Dataset
3.1.2. Evaluation Metrics
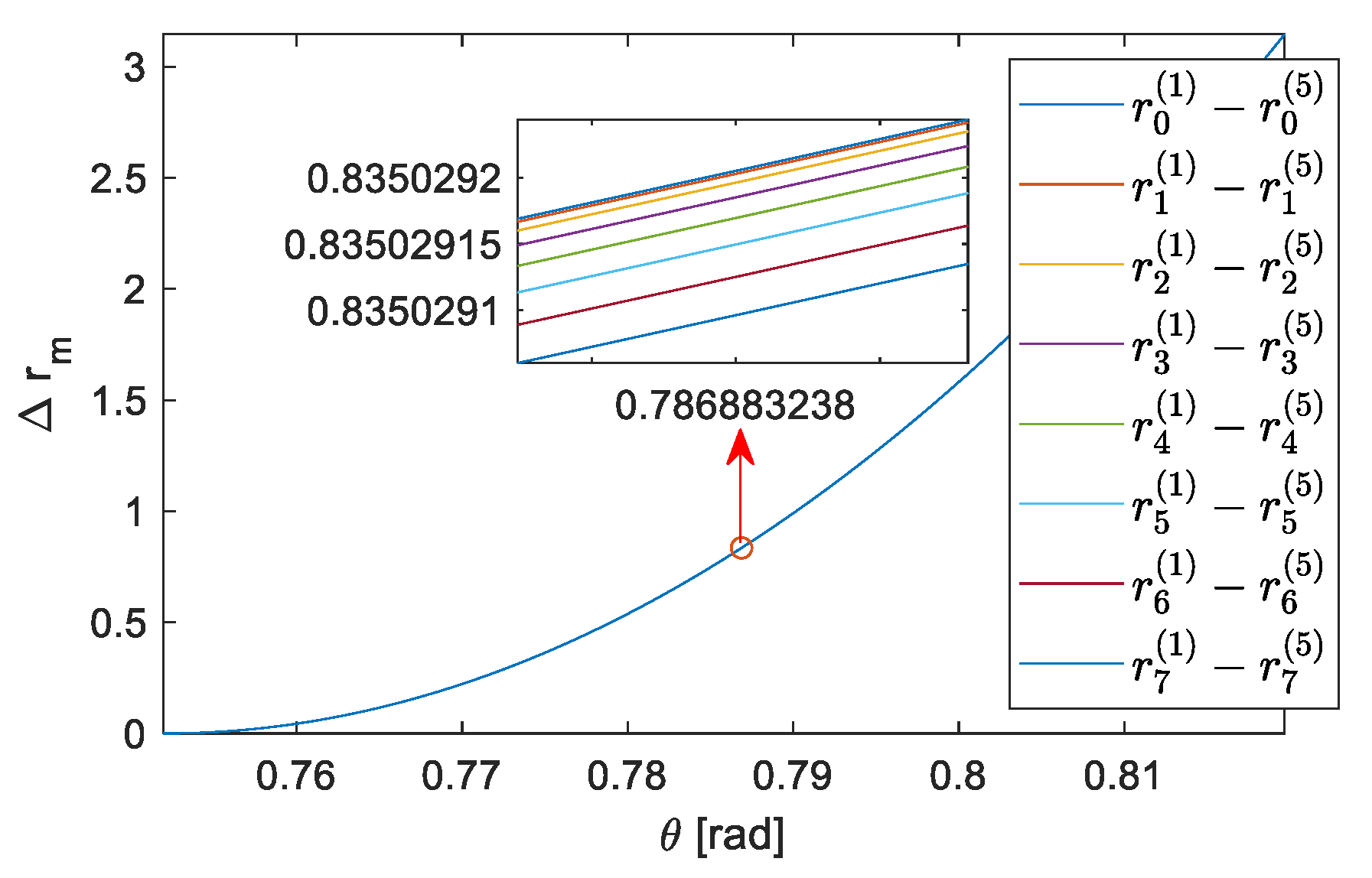
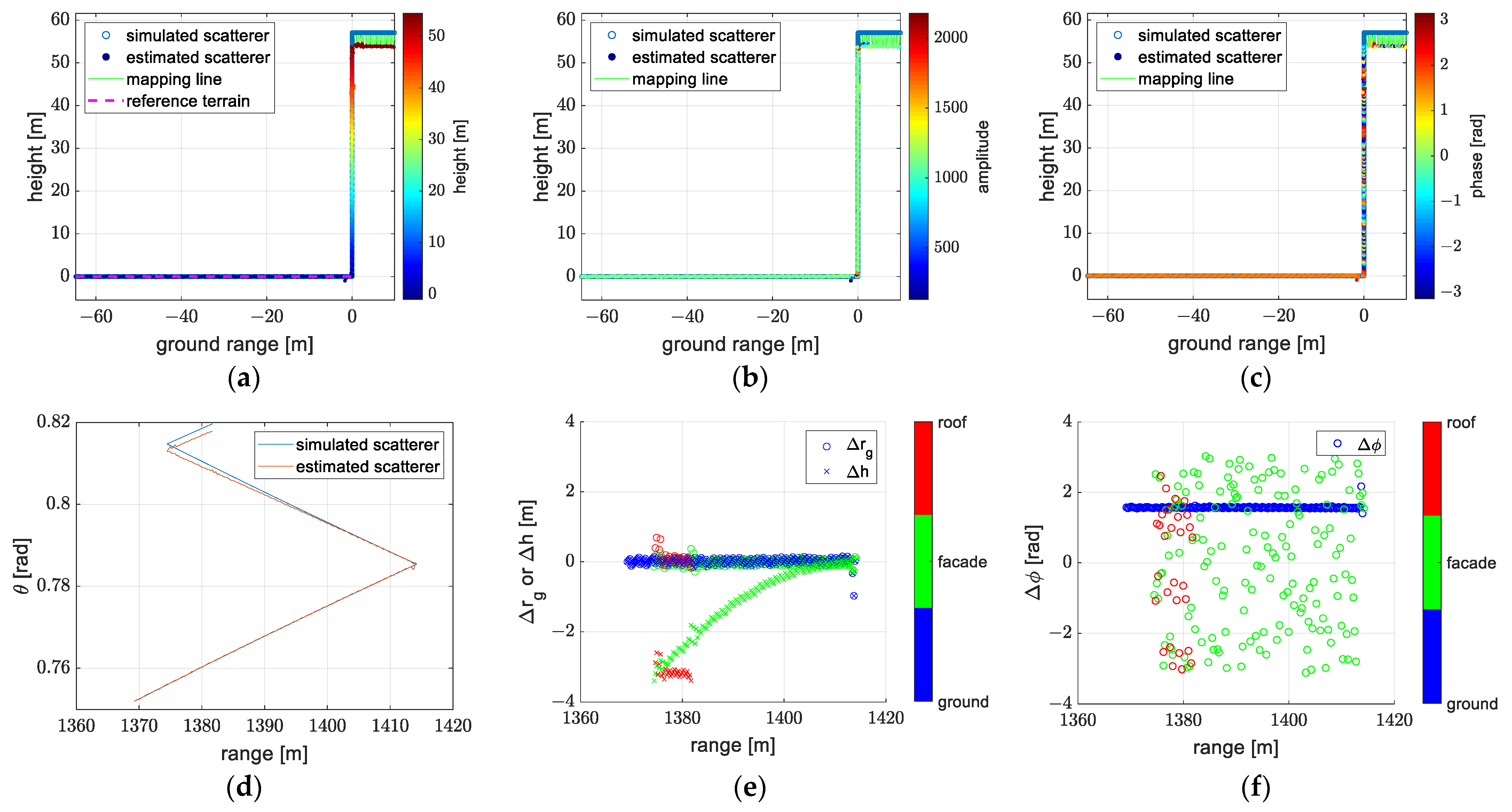
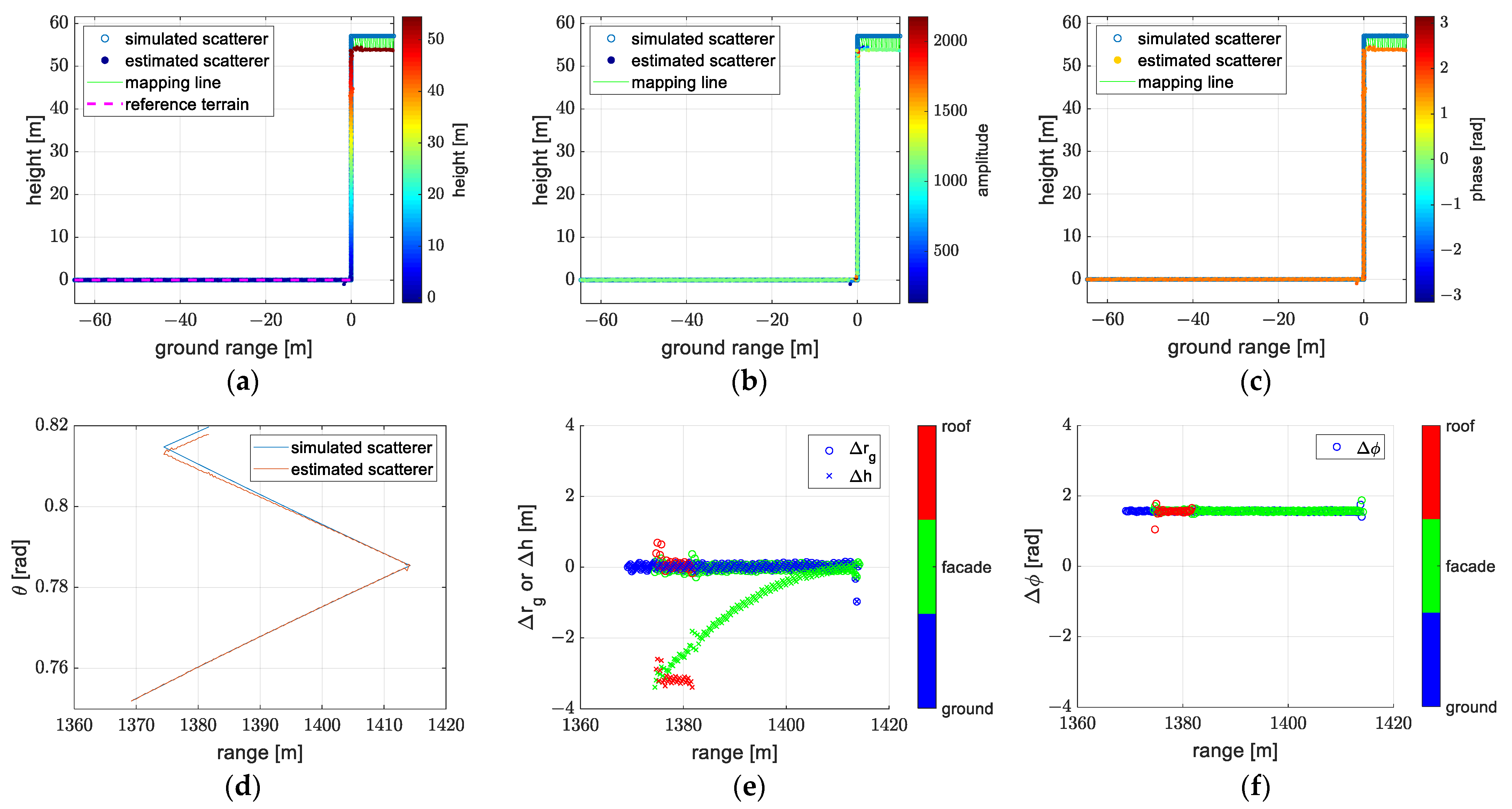
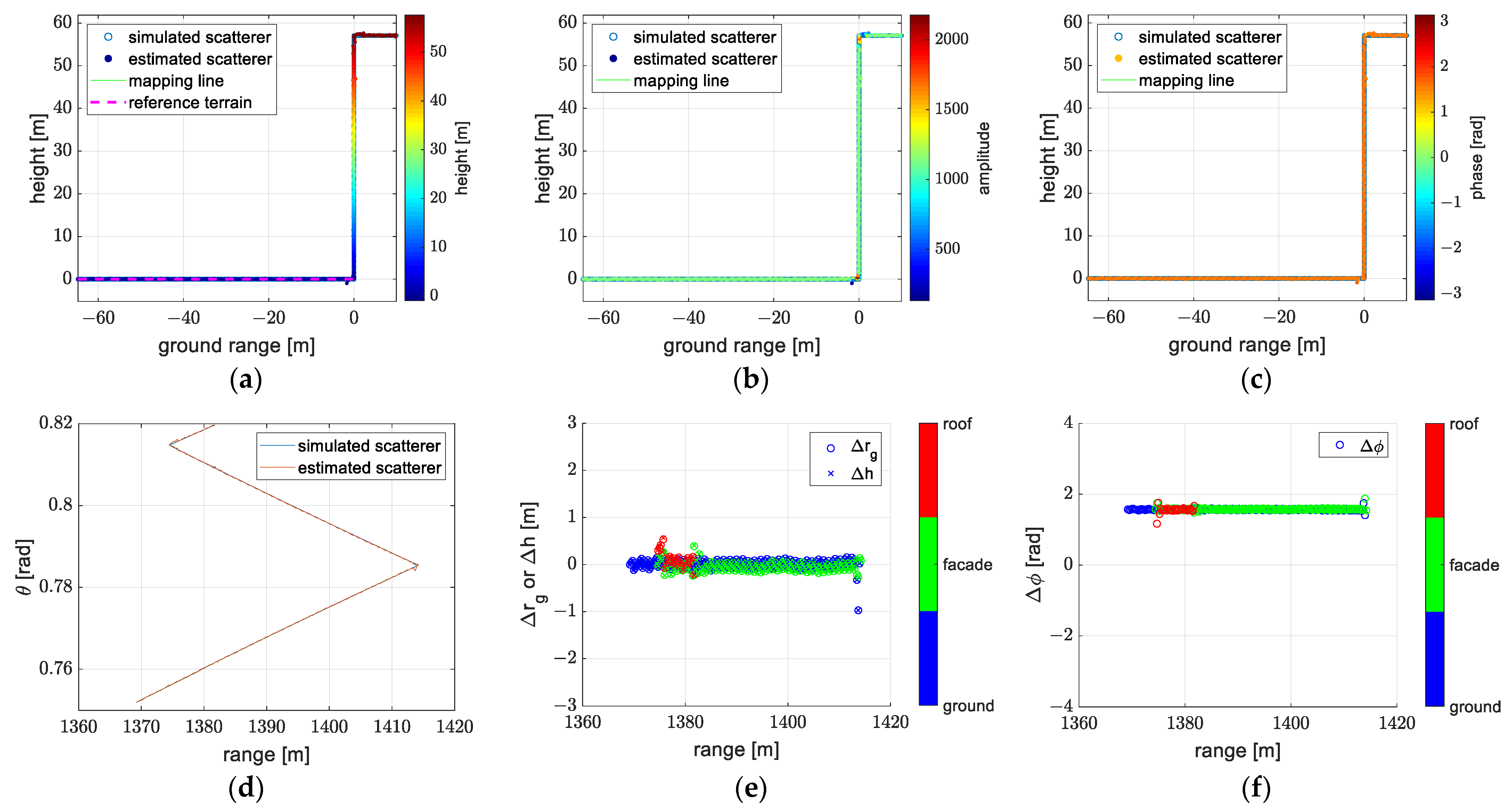
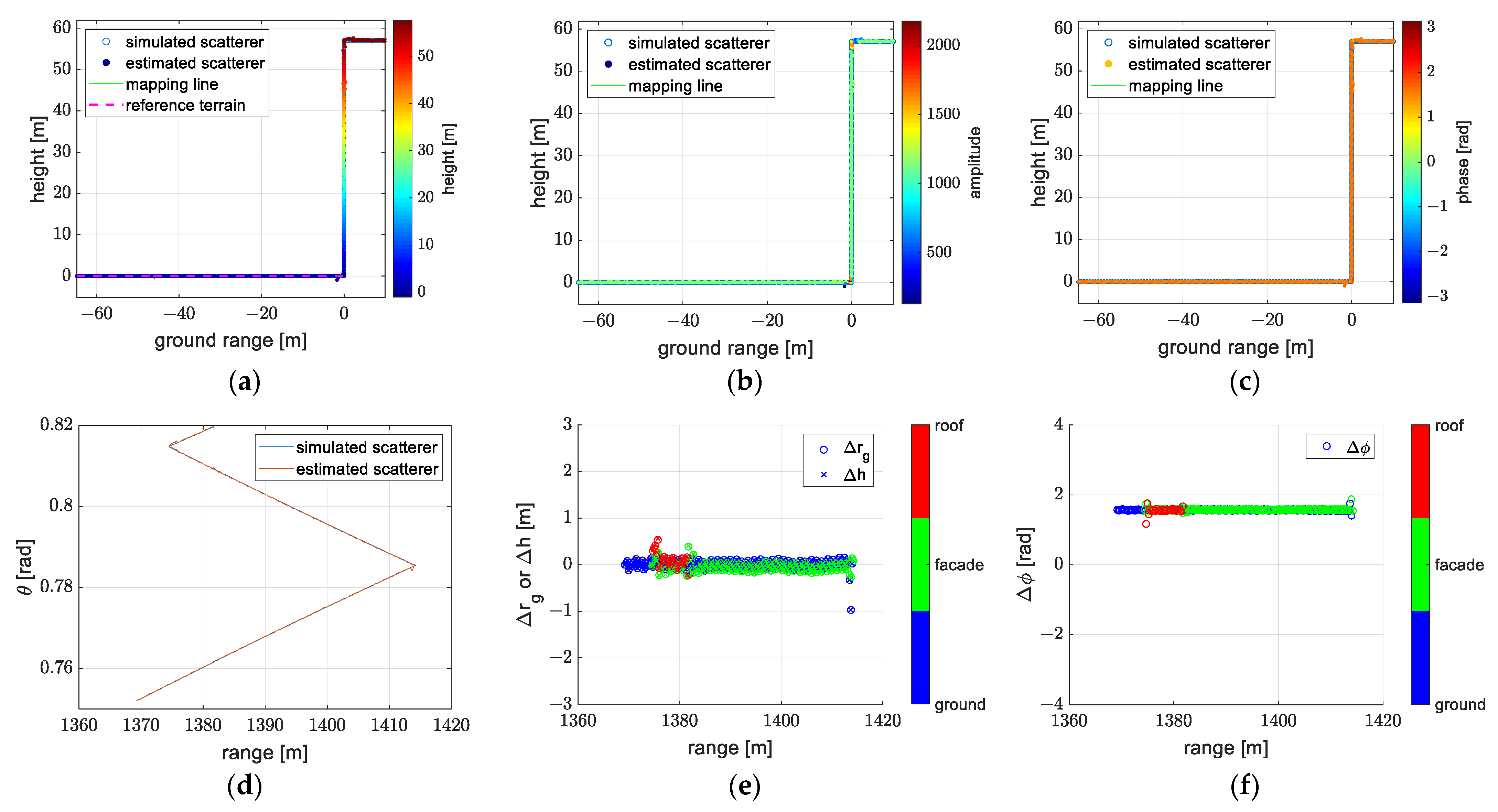
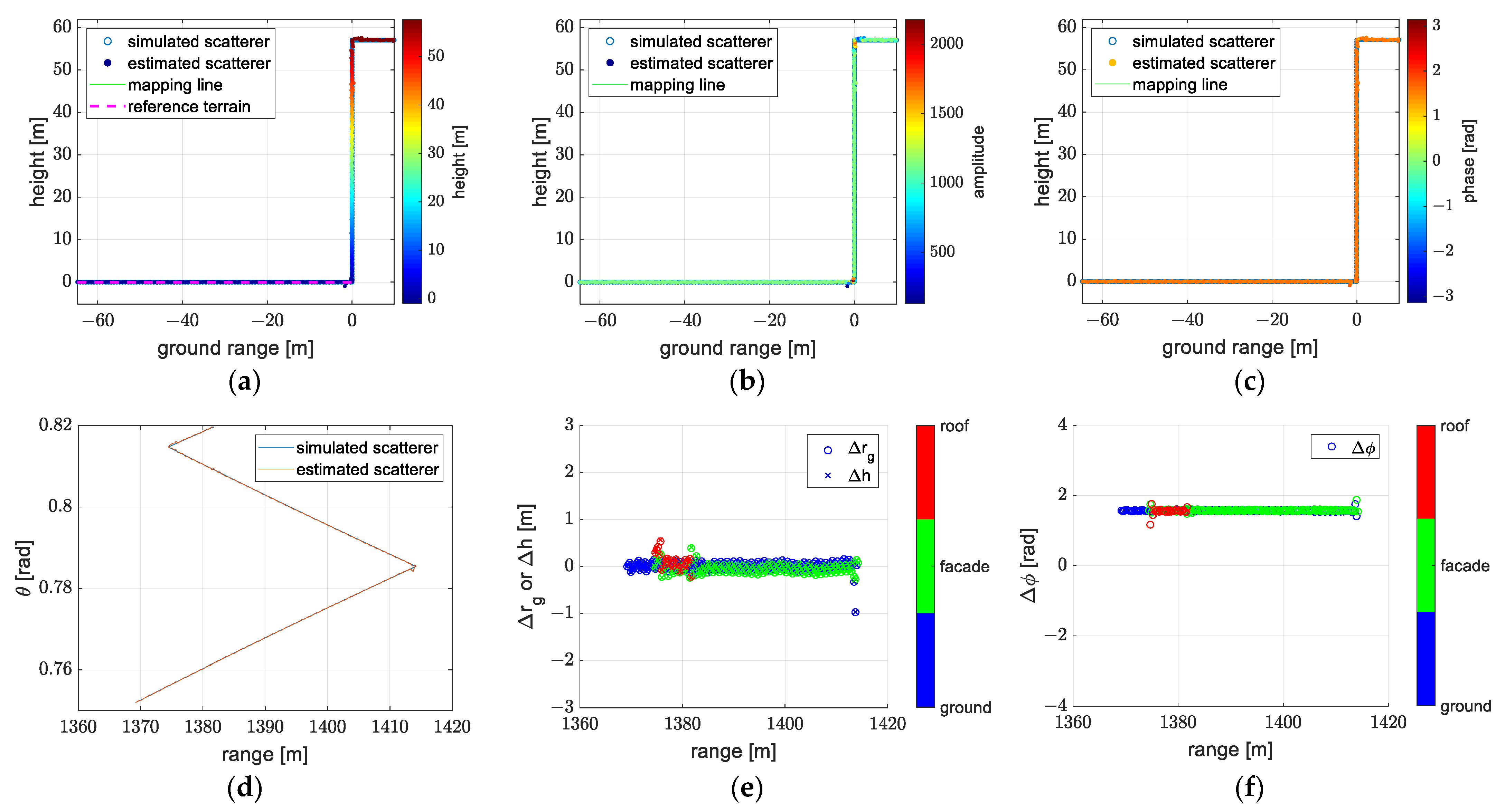
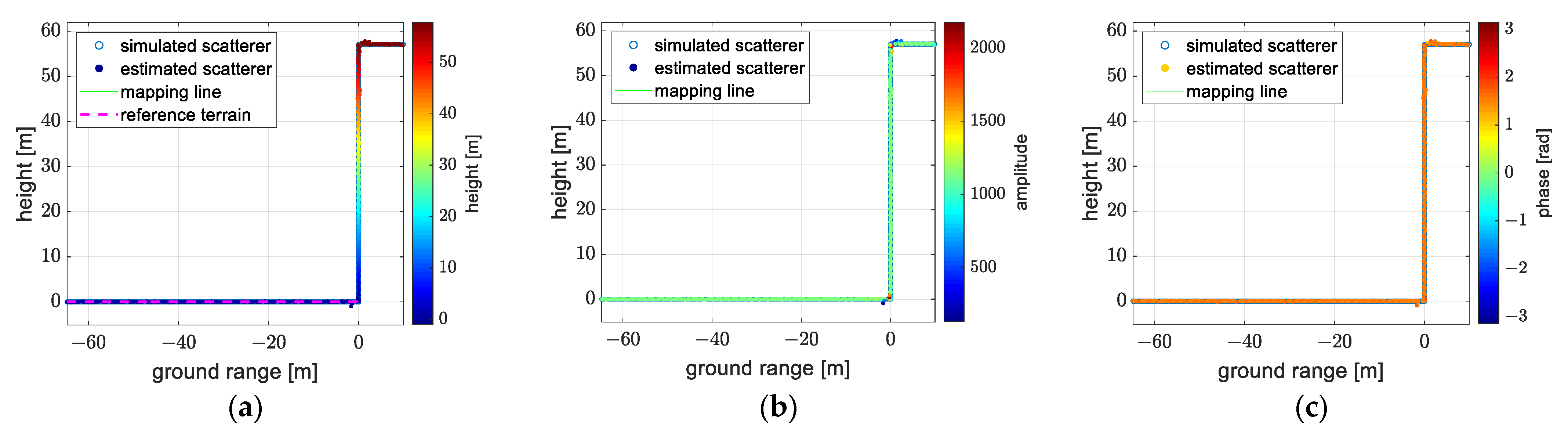
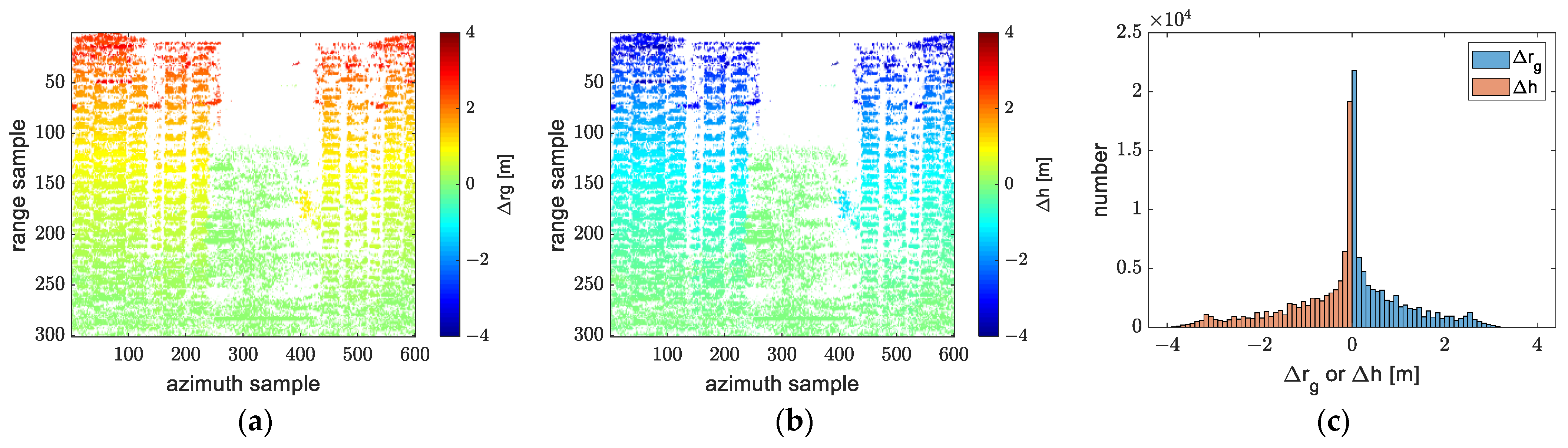
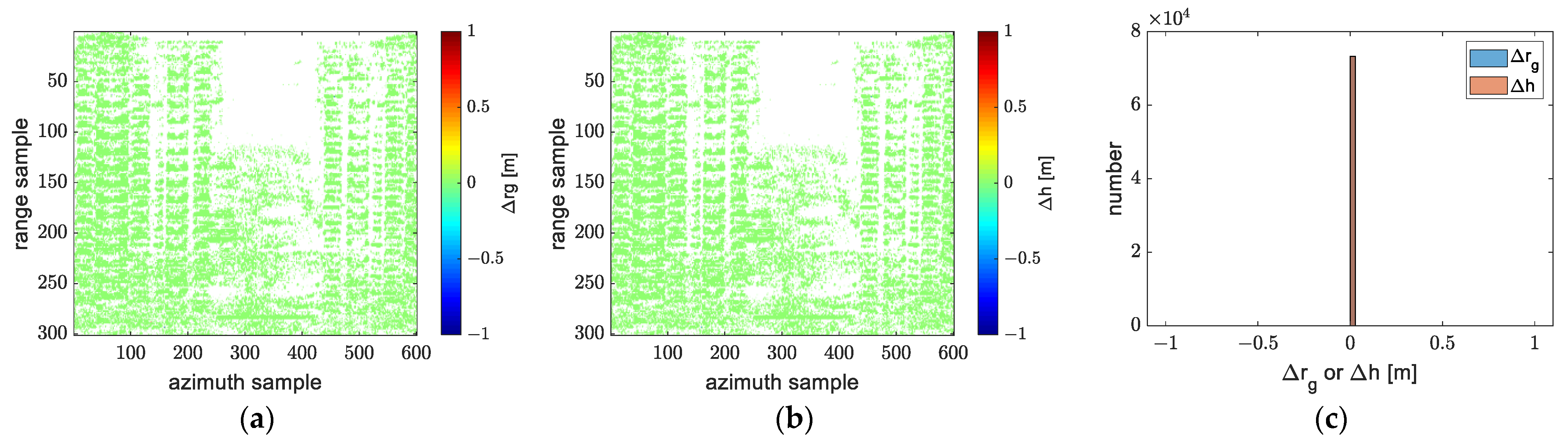
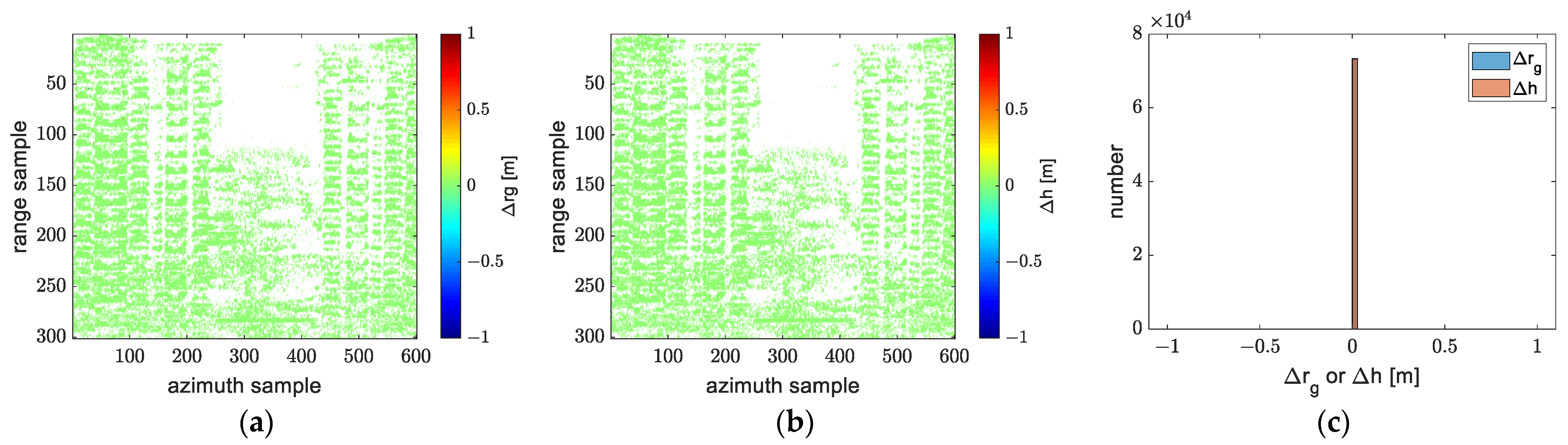
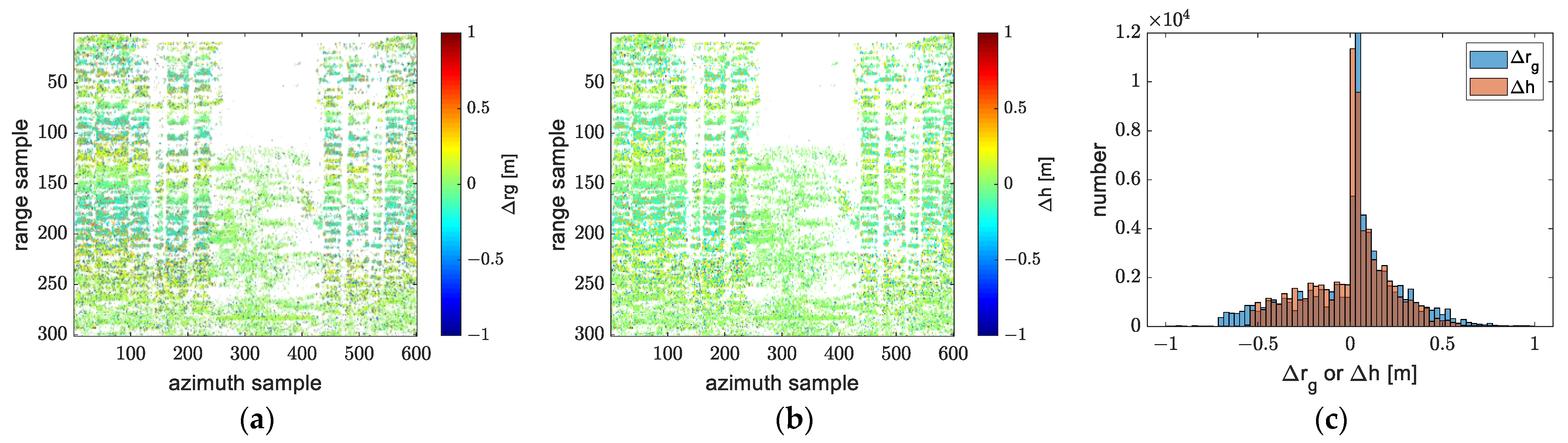
3.1.3. Results of Experiment 1
3.1.4. Results of Experiment 2
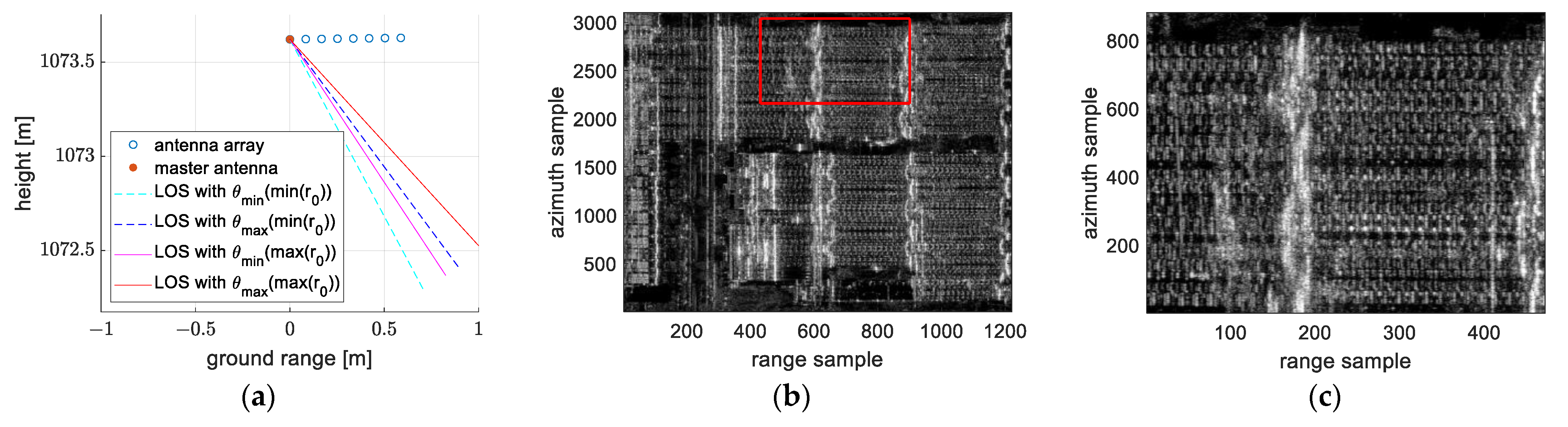
3.2. Experiments on Measured Data
3.2.1. Measured Dataset
3.2.2. Evaluation Methods
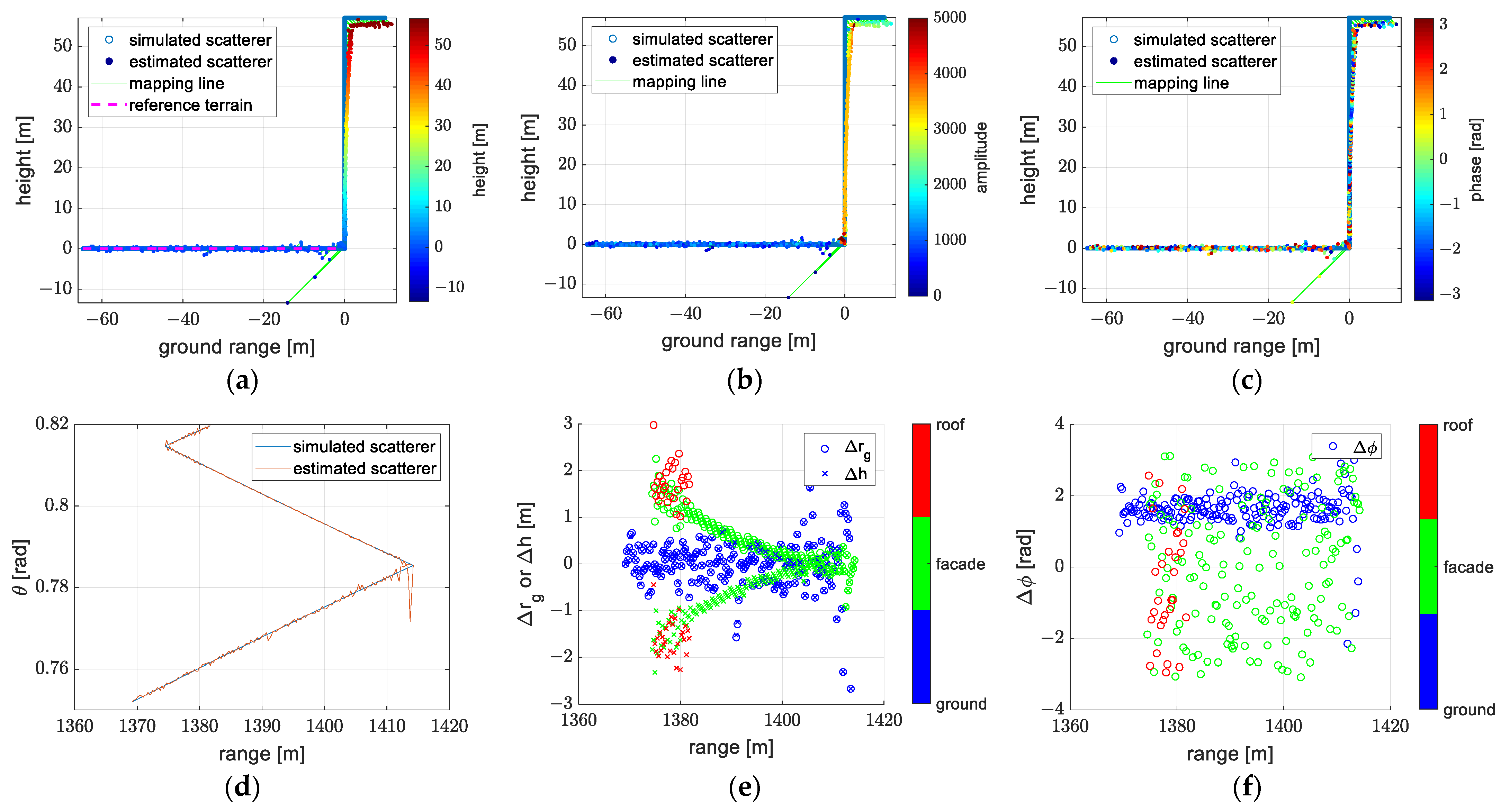
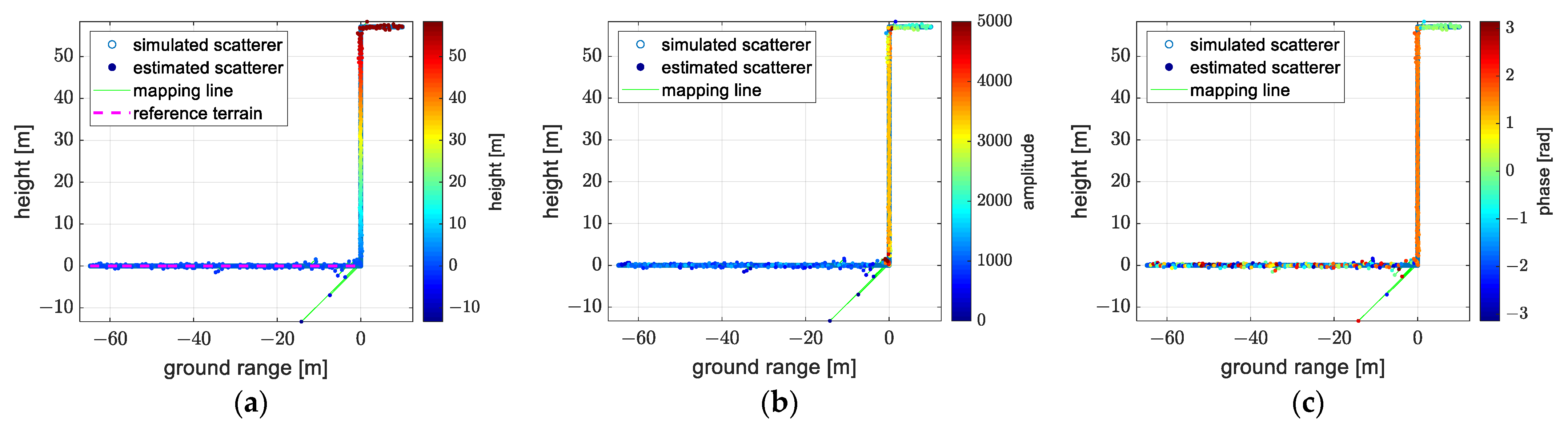
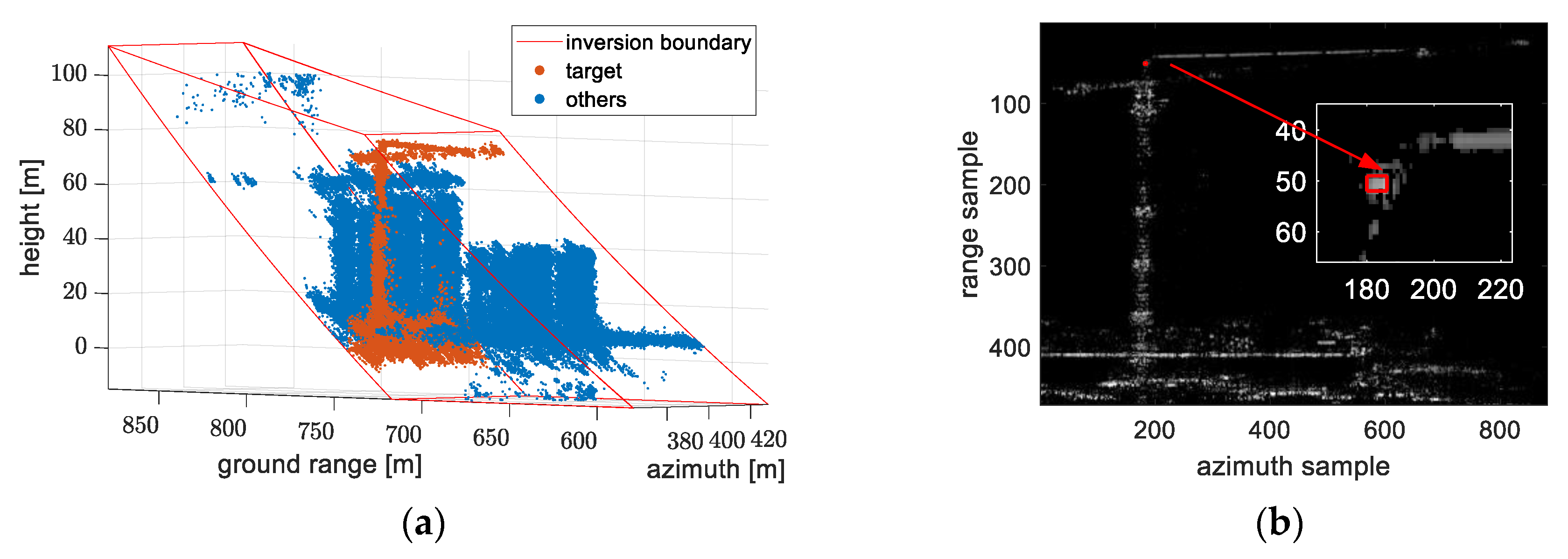
3.2.3. Results of Experiment 1
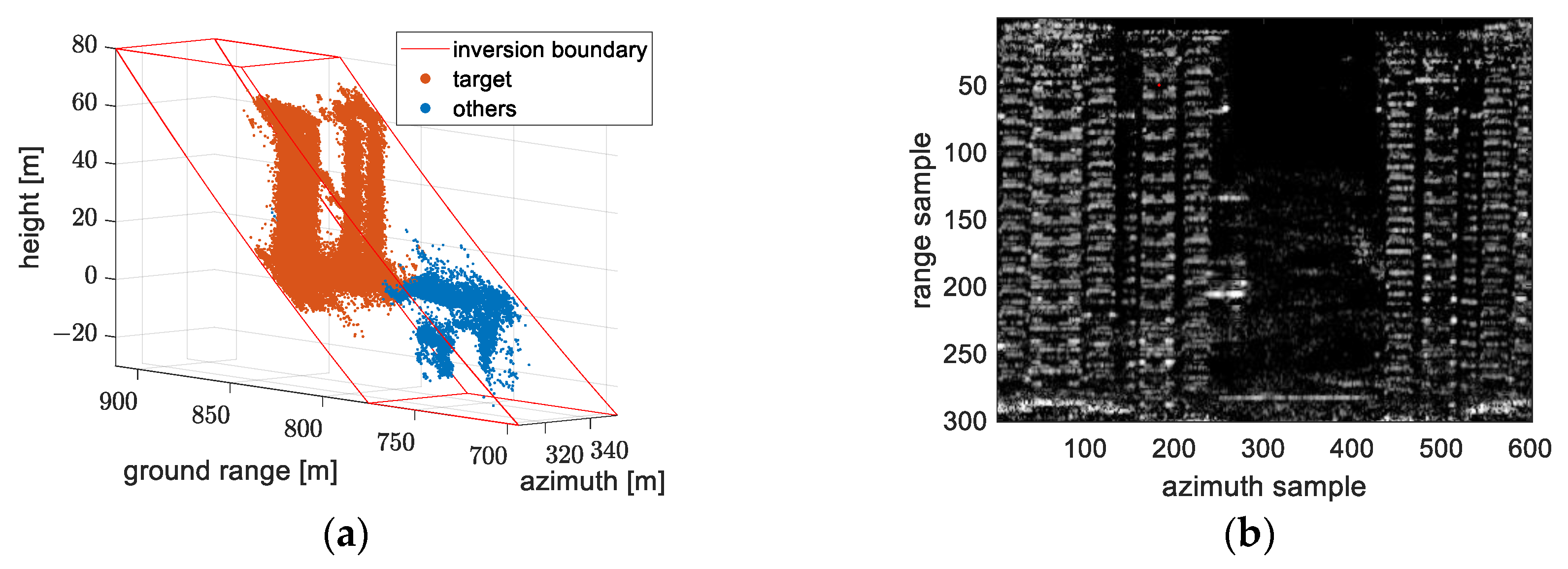
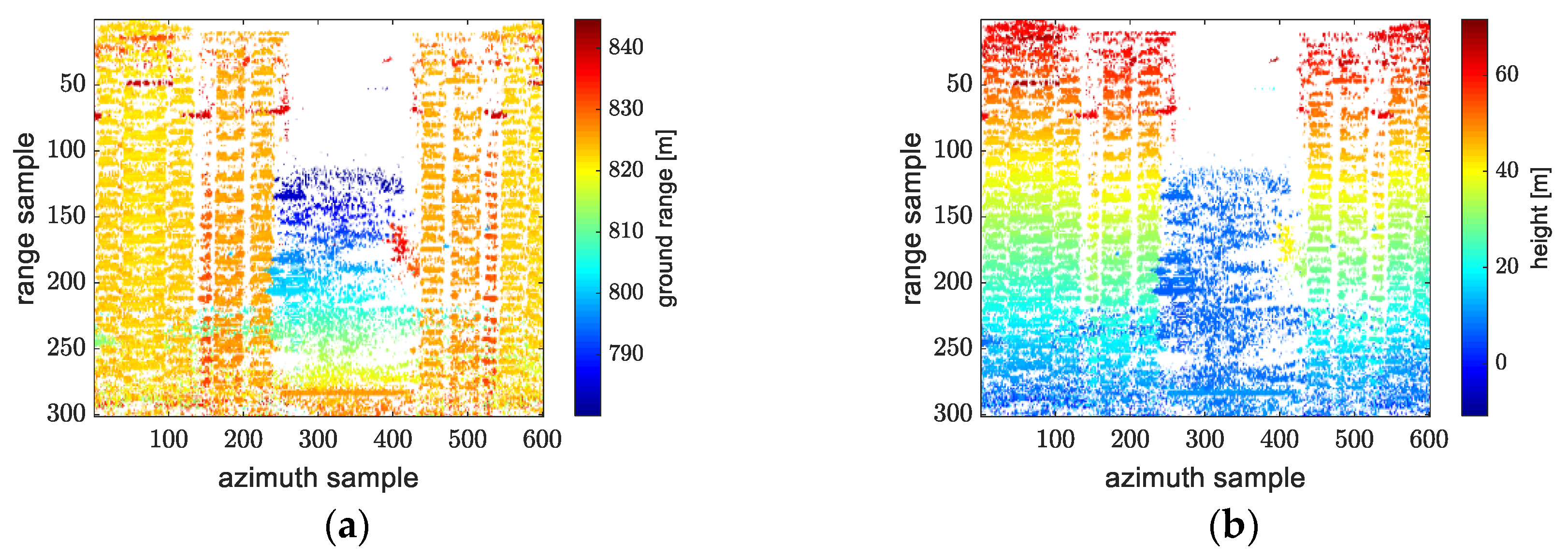
3.2.4. Results of Experiment 2
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Moreira, A.; Prats-Iraola, P.; Younis, M.; Krieger, G.; Hajnsek, I.; Papathanassiou, K.P. A Tutorial on Synthetic Aperture Radar. IEEE Geosci. Remote Sens. Mag. 2013, 1, 6–43. [Google Scholar] [CrossRef]
- Massonnet, D.; Rabaute, T. Radar interferometry: Limits and potential. IEEE Trans. Geosci. Remote Sens. 1993, 31, 455–464. [Google Scholar] [CrossRef]
- Ferretti, A.; Prati, C.; Rocca, F. Permanent scatterers in SAR interferometry. IEEE Trans. Geosci. Remote Sens. 2001, 39, 8–20. [Google Scholar] [CrossRef]
- Pasquali, P.; Prati, C.; Rocca, F.; Seymour, H. A 3-D SAR experiment with EMSL data. In Proceedings of the 1995 International Geoscience and Remote Sensing Symposium (IGARSS 95), Florence, Italy, 10–14 July 1995; pp. 784–786. [Google Scholar]
- Knaell, K.K. Radar tomography for the generation of three-dimensional images. IEE Proc. -Radar. Sonar Navig. 1995, 142, 54–60. [Google Scholar] [CrossRef]
- Reale, D.; Pauciullo, A.; Fornaro, G.; Maio, A.D. A scatterers detection scheme in SAR Tomography for reconstruction and monitoring of individual buildings. In Proceedings of the 2011 Joint Urban Remote Sensing Event, 11–13 April 2011; pp. 249–252. [Google Scholar]
- Wu, X.; Jezek, K.C.; Rodriguez, E.; Gogineni, S.; Rodriguez-Morales, F.; Freeman, A. Ice Sheet Bed Mapping with Airborne SAR Tomography. IEEE Trans. Geosci. Remote Sens. 2011, 49, 3791–3802. [Google Scholar] [CrossRef]
- Quegan, S.; Le Toan, T.; Chave, J.; Dall, J.; Exbrayat, J.-F.; Minh, D.H.T.; Lomas, M.; D’Alessandro, M.M.; Paillou, P.; Papathanassiou, K.; et al. The European Space Agency BIOMASS mission: Measuring forest above-ground biomass from space. Remote Sens. Environ. 2019, 227, 44–60. [Google Scholar] [CrossRef]
- Nannini, M.; Scheiber, R.; Horn, R.; Moreira, A. First 3-D Reconstructions of Targets Hidden Beneath Foliage by Means of Polarimetric SAR Tomography. IEEE Geosci. Remote Sens. Lett. 2012, 9, 60–64. [Google Scholar] [CrossRef]
- Reigber, A.; Moreira, A. First demonstration of airborne SAR tomography using multibaseline L-band data. IEEE Trans. Geosci. Remote Sens. 2000, 38, 2142–2152. [Google Scholar] [CrossRef]
- Yu, Y.; d’Alessandro, M.M.; Tebaldini, S.; Liao, M. Signal Processing Options for High Resolution SAR Tomography of Natural Scenarios. Remote Sens. 2020, 12, 1638. [Google Scholar] [CrossRef]
- Ren, Y.; Xiao, A.; Hu, F.; Xu, F.; Qiu, X.; Ding, C.; Jin, Y.-Q. Coprime Sensing for Airborne Array Interferometric SAR Tomography. IEEE Trans. Geosci. Remote Sens. 2022, 60, 1–15. [Google Scholar] [CrossRef]
- Rambour, C.; Denis, L.; Tupin, F.; Oriot, H.M. Introducing Spatial Regularization in SAR Tomography Reconstruction. IEEE Trans. Geosci. Remote Sens. 2019, 57, 8600–8617. [Google Scholar] [CrossRef]
- Zhu, X.X.; Bamler, R. Very High Resolution Spaceborne SAR Tomography in Urban Environment. IEEE Trans. Geosci. Remote Sens. 2010, 48, 4296–4308. [Google Scholar] [CrossRef]
- Fornaro, G.; Lombardini, F.; Serafino, F. Three-dimensional multipass SAR focusing: Experiments with long-term spaceborne data. IEEE Trans. Geosci. Remote Sens. 2005, 43, 702–714. [Google Scholar] [CrossRef]
- Rambour, C.; Budillon, A.; Johnsy, A.C.; Denis, L.; Tupin, F.; Schirinzi, G. From Interferometric to Tomographic SAR: A Review of Synthetic Aperture Radar Tomography-Processing Techniques for Scatterer Unmixing in Urban Areas. IEEE Geosci. Remote Sens. Mag. 2020, 8, 6–29. [Google Scholar] [CrossRef]
- Ding, C.; Qiu, X.; Xu, F.; Liang, X.; Jiao, Z.; Zhang, F. Synthetic Aperture Radar Three-dimensional Imaging—From TomoSAR and Array InSAR to Microwave Vision. J. Radars 2019, 8, 693–709. [Google Scholar]
- Fornaro, G.; Serafino, F.; Soldovieri, F. Three-dimensional focusing with multipass SAR data. IEEE Trans. Geosci. Remote Sens. 2003, 41, 507–517. [Google Scholar] [CrossRef]
- Zhu, X.X.; Bamler, R. Tomographic SAR Inversion by L1-Norm Regularization—The Compressive Sensing Approach. IEEE Trans. Geosci. Remote Sens. 2010, 48, 3839–3846. [Google Scholar] [CrossRef]


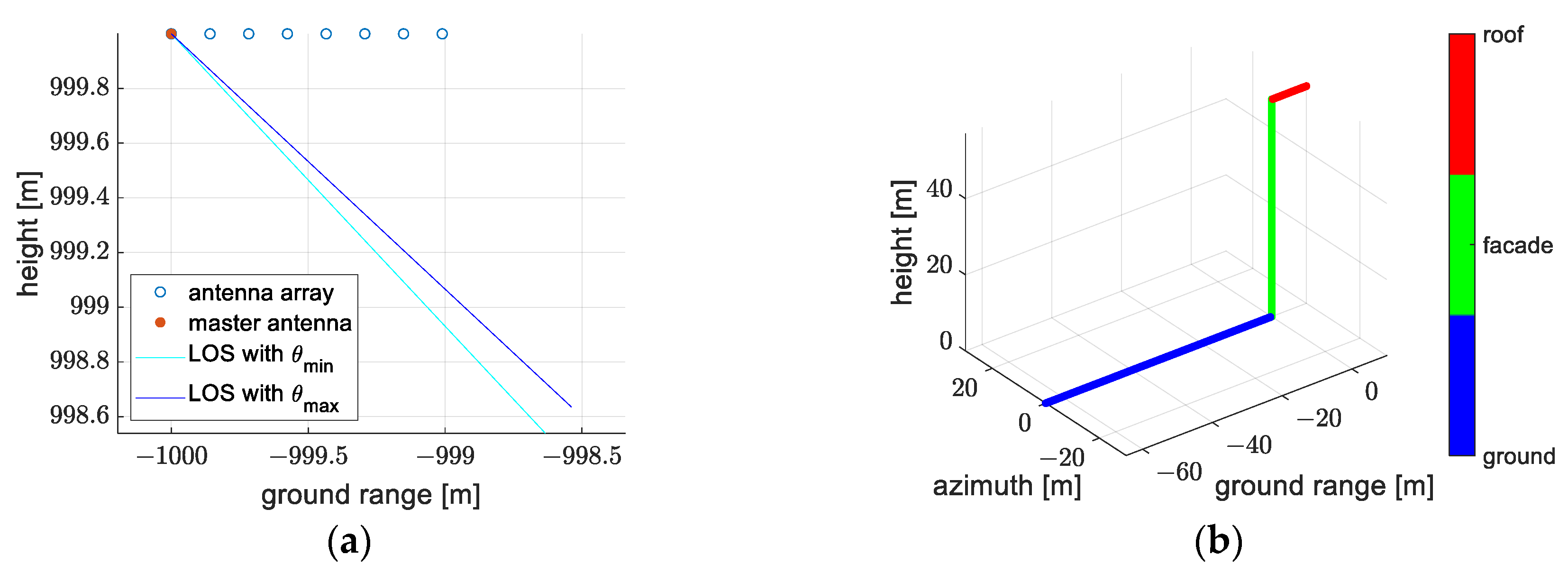

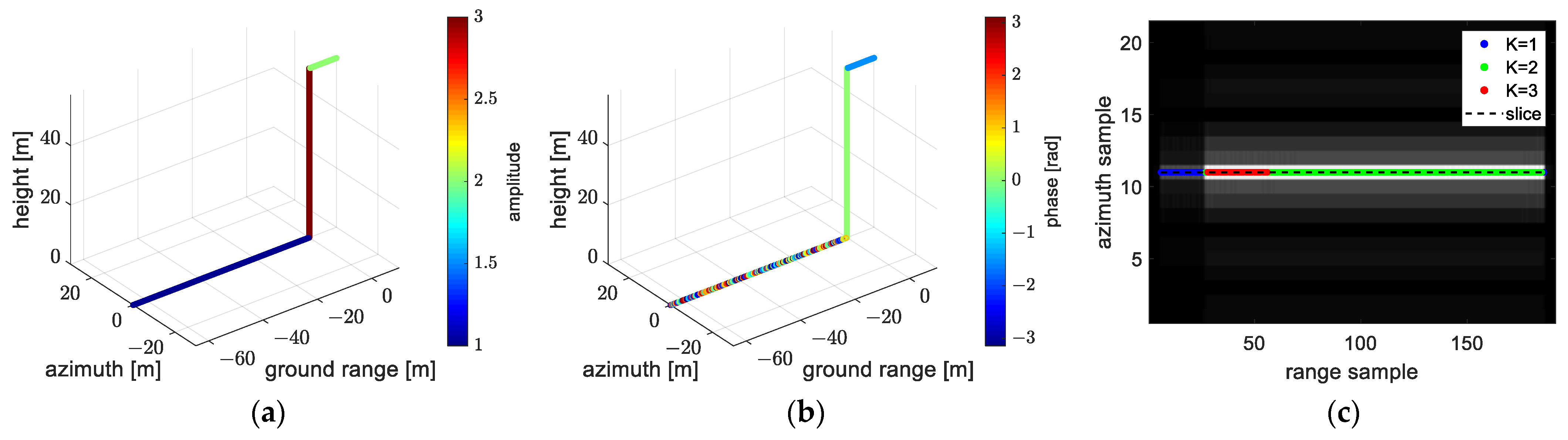
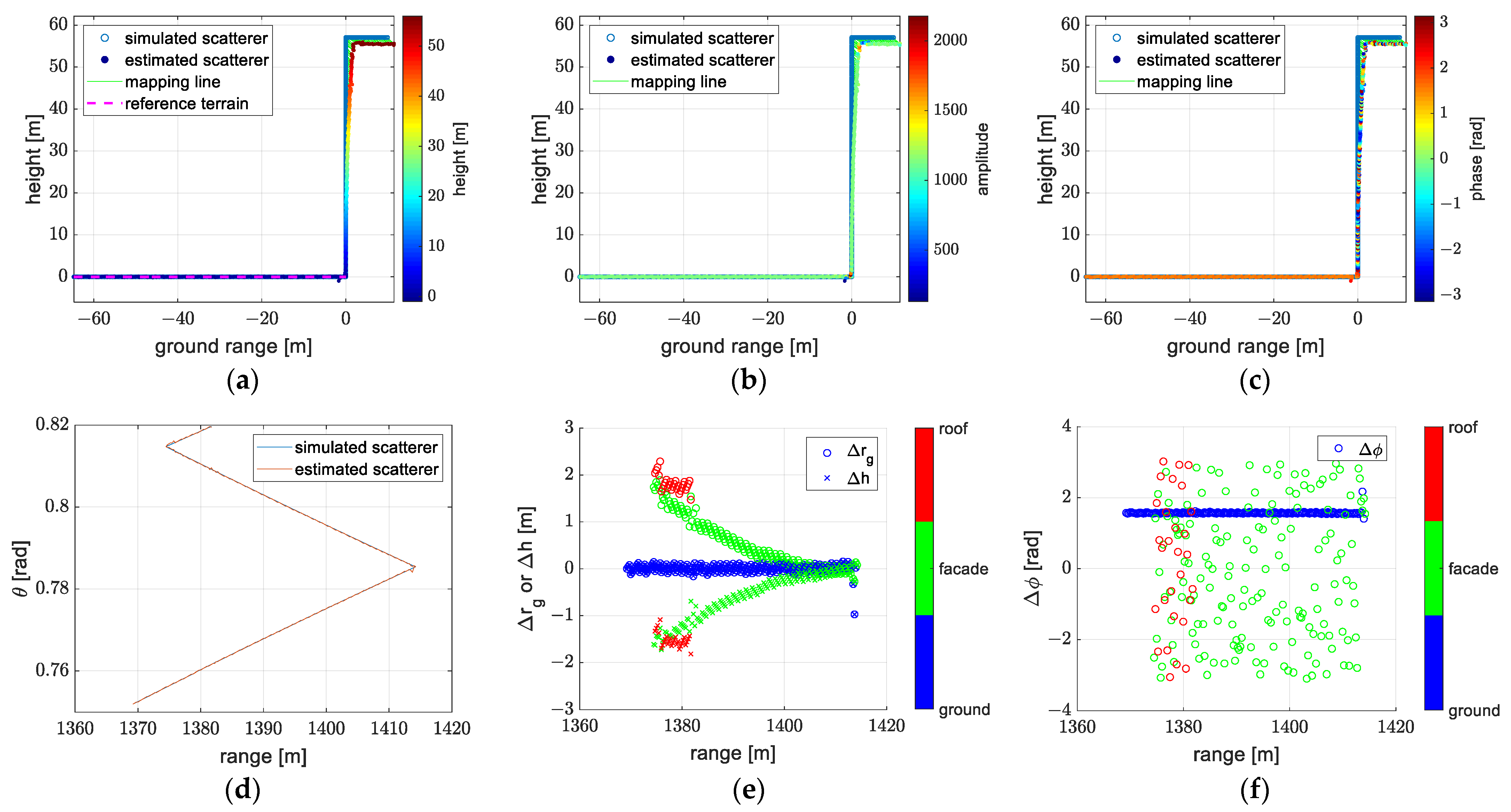
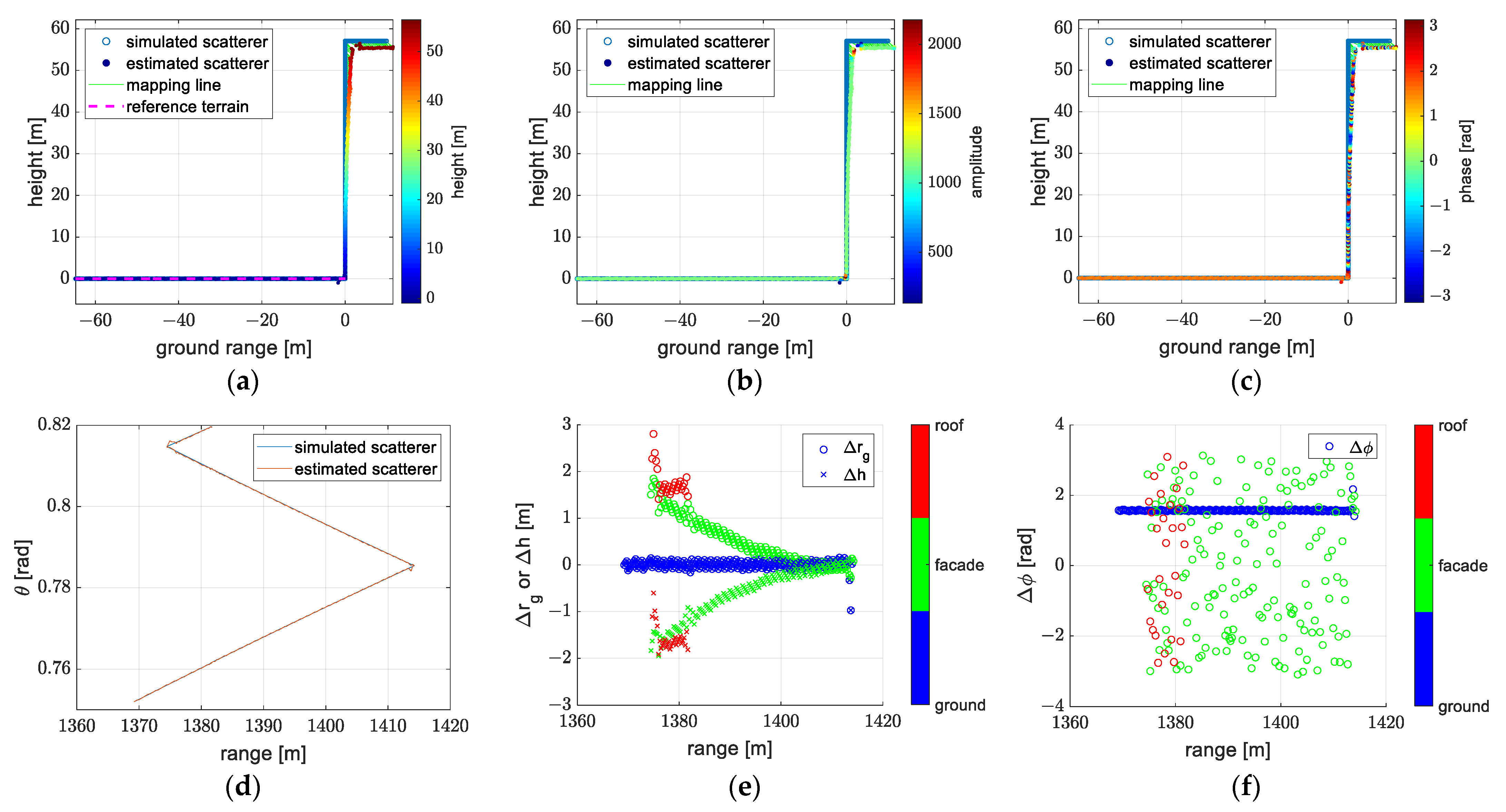






| Geometric | Pixel Formula | Range Formula | Geodetic Transform | |
|---|---|---|---|---|
| model 1 | planar wavefront | (2) | (3) | (13) |
| model 2 | planar wavefront | (2) | (10) | (13) |
| model 3 | planar wavefront | (2) | (11) | (13) |
| model 4 | planar wavefront | (2) | (12) | (13) |
| model 5 | spherical wavefront | (17) | (18) | (22) |
| model 6 | spherical wavefront | (17) | (21) | (22) |
| Parameter | Symbol | Value |
|---|---|---|
| Wavelength | 0.02 m | |
| Range resolution | 0.25 m | |
| Azimuth resolution | 0.2083 m | |
| Flight height | 1000 m | |
| Channel number | 8 | |
| Baseline length | 0 m, 0.141 m, 0.283 m, 0.424 m, 0.566 m, 0.707 m, 0.848 m, 0.990 m | |
| Baseline incline angle | 0°, 0°, 0°, 0°, 0°, 0°, 0°, 0° | |
| Range | 1369.2 m~1414.2 m | |
| Minimum off-nadir angle | 43.0846° | |
| Maximum off-nadir angle | 46.9648° |
| Ground Range (m) | Height (m) | Phase (rad) | Amplitude | ||||||
|---|---|---|---|---|---|---|---|---|---|
| ME | RMSE | ME | RMSE | ||||||
| Model 1 | Roof | 1.815 | 1.822 | −1.517 | 1.524 | 0.214 | 1.884 | 1055.6 | 184.8 |
| Façade | 0.523 | 0.750 | −0.580 | 0.754 | −0.211 | 1.843 | 1157.1 | 127.0 | |
| Ground | 0 | 0.103 | 0 | 0.102 | 1.571 | 0.049 | 1130.4 | 91.7 | |
| Model 4 | Roof | 0.104 | 0.218 | −3.139 | 3.144 | 1.550 | 0.108 | 1051.6 | 211.8 |
| Façade | −0.020 | 0.094 | −1.099 | 1.472 | 1.572 | 0.034 | 1157.6 | 136.0 | |
| Ground | 0.007 | 0.104 | 0.007 | 0.103 | 1.565 | 0.024 | 1129.9 | 91.6 | |
| Model 5 | Roof | 0.093 | 0.181 | 0.098 | 0.193 | 1.553 | 0.090 | 1055.8 | 184.5 |
| Façade | −0.040 | 0.100 | −0.041 | 0.103 | 1.576 | 0.033 | 1157.1 | 126.9 | |
| Ground | 0 | 0.104 | 0 | 0.102 | 1.566 | 0.024 | 1130.4 | 91.7 | |
| Model 6 | Roof | 0.093 | 0.181 | 0.098 | 0.193 | 1.553 | 0.090 | 1056.3 | 184.0 |
| Façade | −0.040 | 0.100 | −0.041 | 0.103 | 1.576 | 0.034 | 1157.0 | 126.7 | |
| Ground | 0 | 0.104 | 0 | 0.102 | 1.566 | 0.024 | 1130.4 | 91.7 | |
| Transformed Model 1 | Roof | 0.093 | 0.181 | 0.098 | 0.193 | 1.553 | 0.090 | 1055.6 | 184.8 |
| Façade | −0.040 | 0.100 | −0.041 | 0.103 | 1.576 | 0.033 | 1157.1 | 127.0 | |
| Ground | 0 | 0.104 | 0 | 0.102 | 1.566 | 0.024 | 1130.4 | 91.7 | |
| Transformed Model 4 | Roof | 0.104 | 0.218 | 0.110 | 0.232 | 1.550 | 0.108 | 1051.6 | 211.8 |
| Façade | −0.020 | 0.094 | −0.020 | 0.097 | 1.572 | 0.034 | 1157.6 | 136.0 | |
| Ground | 0.007 | 0.104 | 0.007 | 0.102 | 1.565 | 0.024 | 1129.9 | 91.6 | |
| Ground Range (m) | Height (m) | Phase (rad) | Amplitude | ||||||
|---|---|---|---|---|---|---|---|---|---|
| ME | RMSE | ME | RMSE | ||||||
| Model 1 | Roof | 1.742 | 1.787 | −1.586 | 1.630 | −0.427 | 1.624 | 2562.6 | 1551.0 |
| Façade | 0.528 | 0.752 | −0.576 | 0.780 | 0.032 | 1.782 | 3629.1 | 1510.6 | |
| Ground | −0.122 | 1.238 | −0.122 | 1.233 | 1.607 | 0.522 | 1137.4 | 1236.5 | |
| Transformed Model 1 | Roof | 0.024 | 0.374 | 0.025 | 0.399 | 1.518 | 0.327 | 2562.6 | 1551.0 |
| Façade | −0.036 | 0.178 | −0.037 | 0.183 | 1.574 | 0.063 | 3629.1 | 1510.6 | |
| Ground | −0.123 | 1.244 | 0.121 | 1.227 | 1.591 | 0.327 | 1137.4 | 1236.5 | |
| Parameter | Symbol | Value |
|---|---|---|
| Wavelength | 0.021 m | |
| Range resolution | 0.1499 m | |
| Azimuth resolution | 0.0734 m | |
| Flight height | 1073.621 m | |
| Channel number | 8 | |
| Baseline length | 0 m, 0.084 m, 0.170 m, 0.252 m, 0.336 m, 0.420 m, 0.504 m, 0.588 m | |
| Baseline incline angle | 0°, 0.726°, 0.725°, 0.747°, 0.742°, 0.738°, 0.747°, 0.734° |
| Parameter | Symbol | Value |
|---|---|---|
| Range | 1233.196 m~1303.647 m | |
| Minimum off-nadir angle at different ranges | 28.022°~33.378° | |
| Maximum off-nadir angle at different ranges | 36.448°~42.392° |
| Parameter | Symbol | Value |
|---|---|---|
| Range | 1303.647 m~1348.616 m | |
| Minimum off-nadir angle at different ranges | 32.160°~35.081° | |
| Maximum off-nadir angle at different ranges | 40.342°~42.542° |
| Ground Range (m) | Height (m) | |||
|---|---|---|---|---|
| Model 1 | 742.968 | 0.876 | 70.247 | 0.516 |
| Model 4 | 738.474 | 0.784 | 67.645 | 0.488 |
| Model 5 | 738.269 | 0.806 | 76.593 | 0.607 |
| Model 6 | 738.297 | 0.817 | 76.613 | 0.612 |
| Transformed model 1 | 738.269 | 0.806 | 76.593 | 0.607 |
| Transformed model 4 | 738.358 | 0.782 | 76.659 | 0.625 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, Q.; Jiao, Z.; Qiu, X.; Wang, B.; Ding, C. Comparison between Different TomoSAR Imaging Models for Airborne Platform Flying at Low Altitude. Remote Sens. 2022, 14, 5452. https://doi.org/10.3390/rs14215452
Yan Q, Jiao Z, Qiu X, Wang B, Ding C. Comparison between Different TomoSAR Imaging Models for Airborne Platform Flying at Low Altitude. Remote Sensing. 2022; 14(21):5452. https://doi.org/10.3390/rs14215452
Chicago/Turabian StyleYan, Qiancheng, Zekun Jiao, Xiaolan Qiu, Bingnan Wang, and Chibiao Ding. 2022. "Comparison between Different TomoSAR Imaging Models for Airborne Platform Flying at Low Altitude" Remote Sensing 14, no. 21: 5452. https://doi.org/10.3390/rs14215452
APA StyleYan, Q., Jiao, Z., Qiu, X., Wang, B., & Ding, C. (2022). Comparison between Different TomoSAR Imaging Models for Airborne Platform Flying at Low Altitude. Remote Sensing, 14(21), 5452. https://doi.org/10.3390/rs14215452







