Efficacious GPR Implementations of Z-Transform-Based Hybrid LOD-FDTD with Subgridding Scheme: Theoretical Formalism and Numerical Study
Abstract
1. Introduction
2. Materials and Methods
2.1. LOD-FDTD Method Based on the Z-Transform Technique
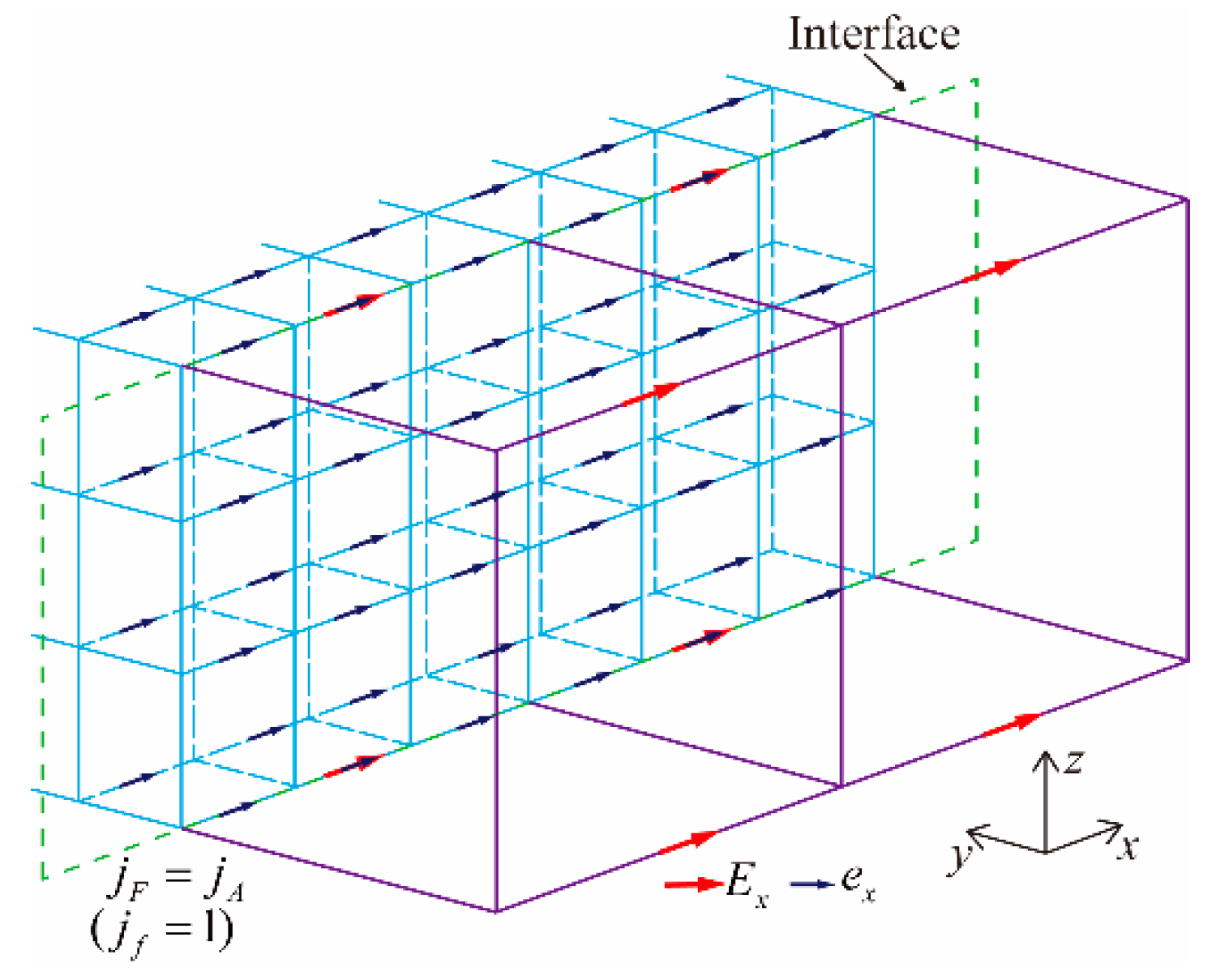
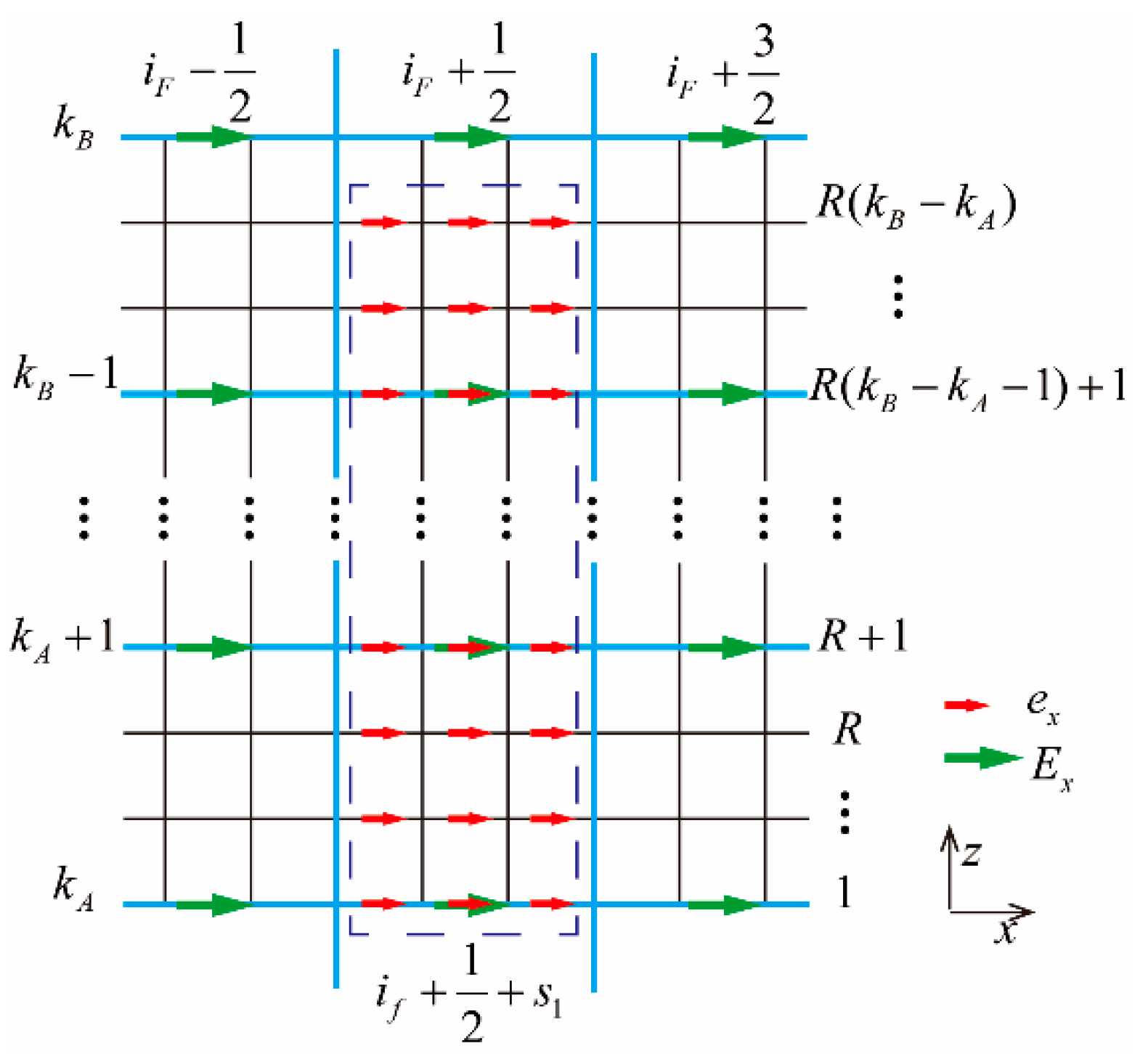
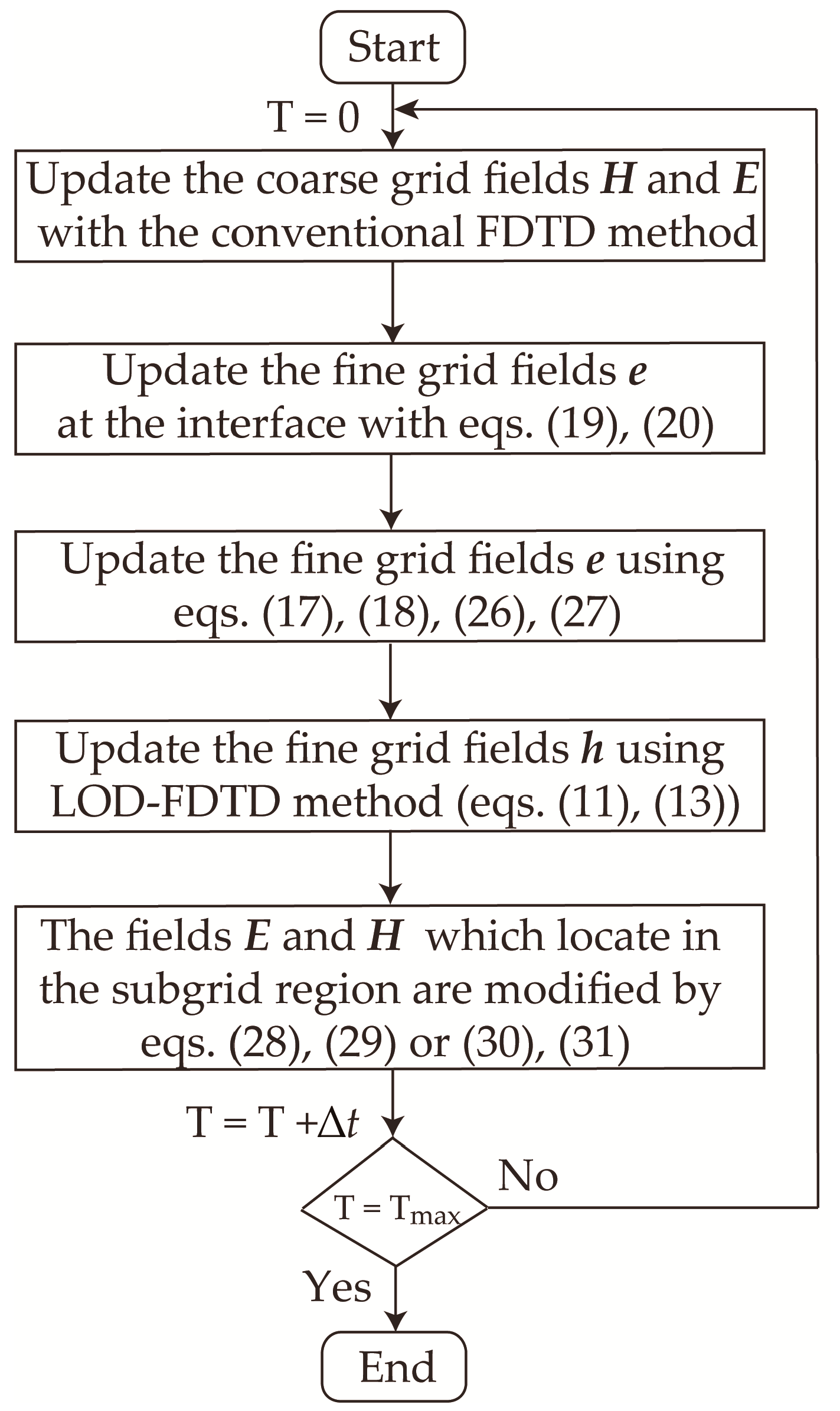
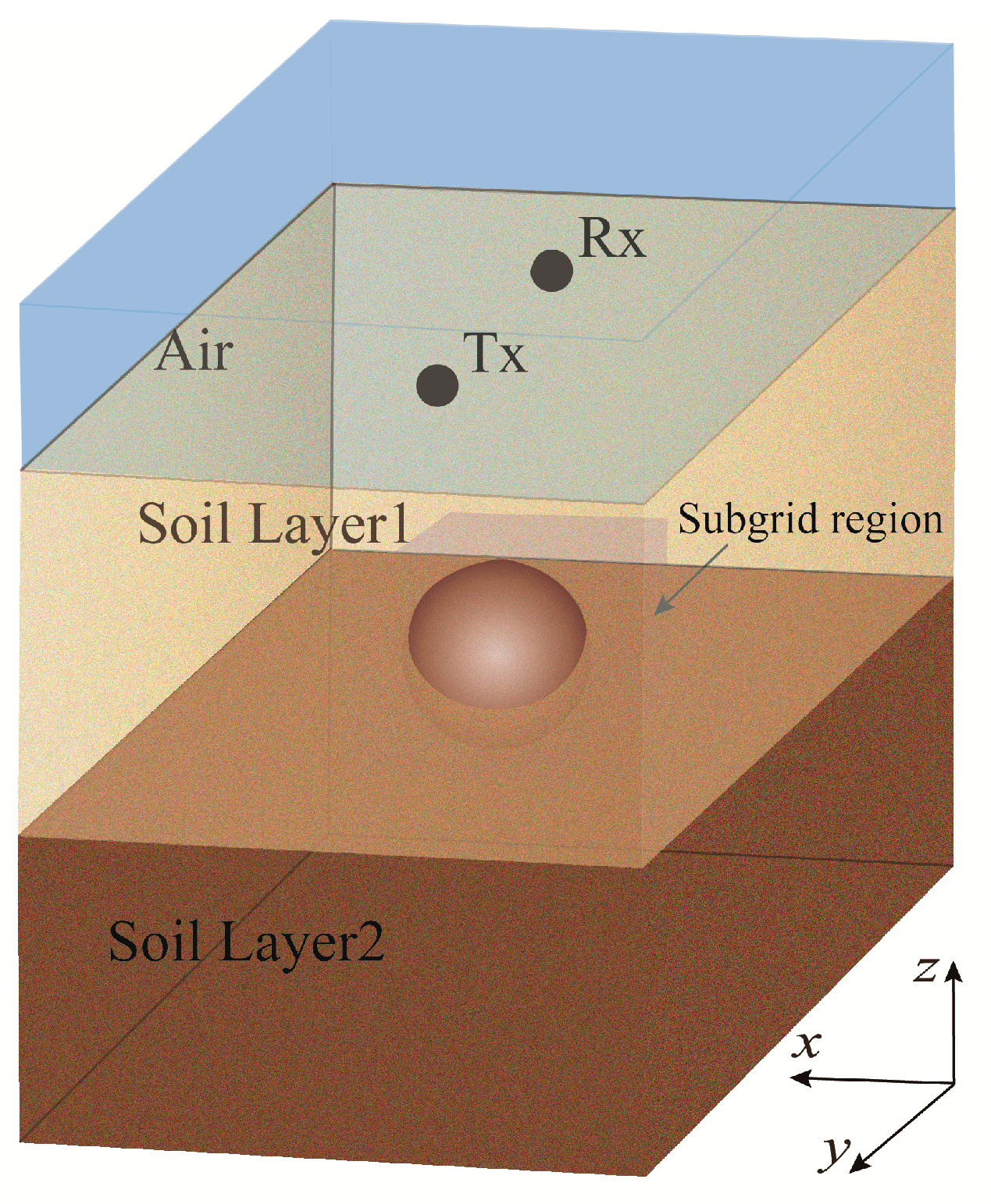
2.2. Hybrid LOD-FDTD with the Subgridding Scheme
3. Results and Discussion
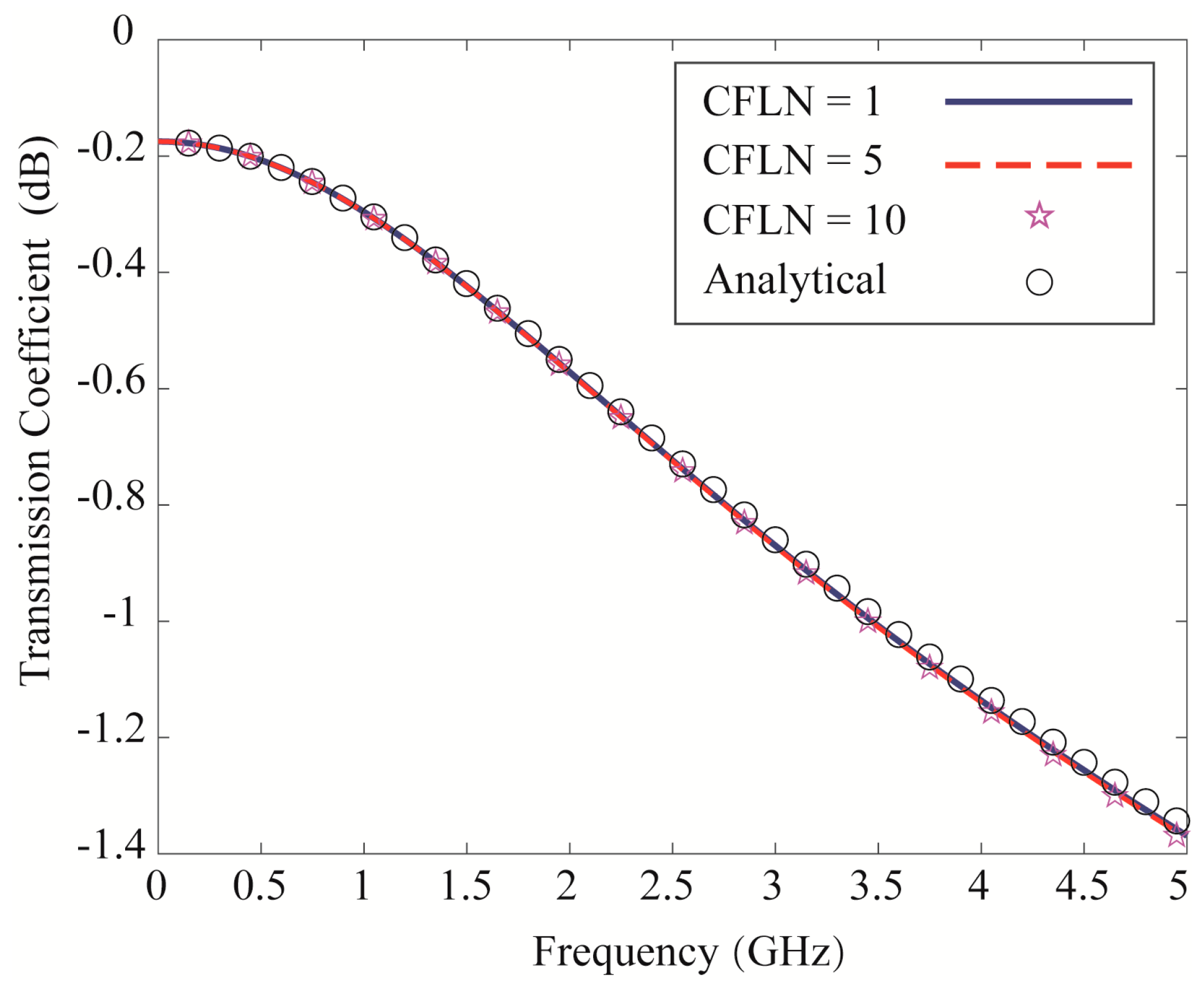
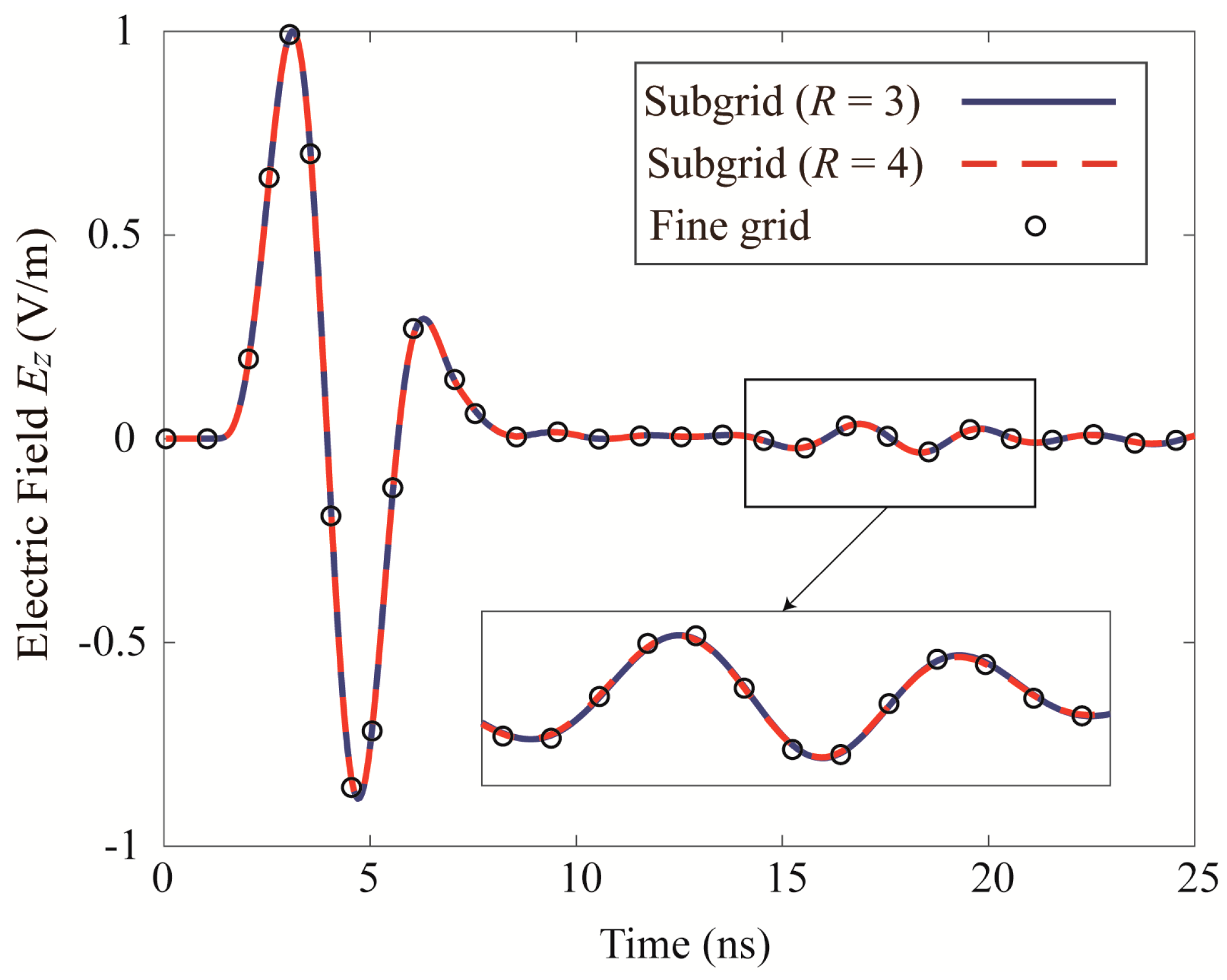
3.1. Rectangular Waveguide
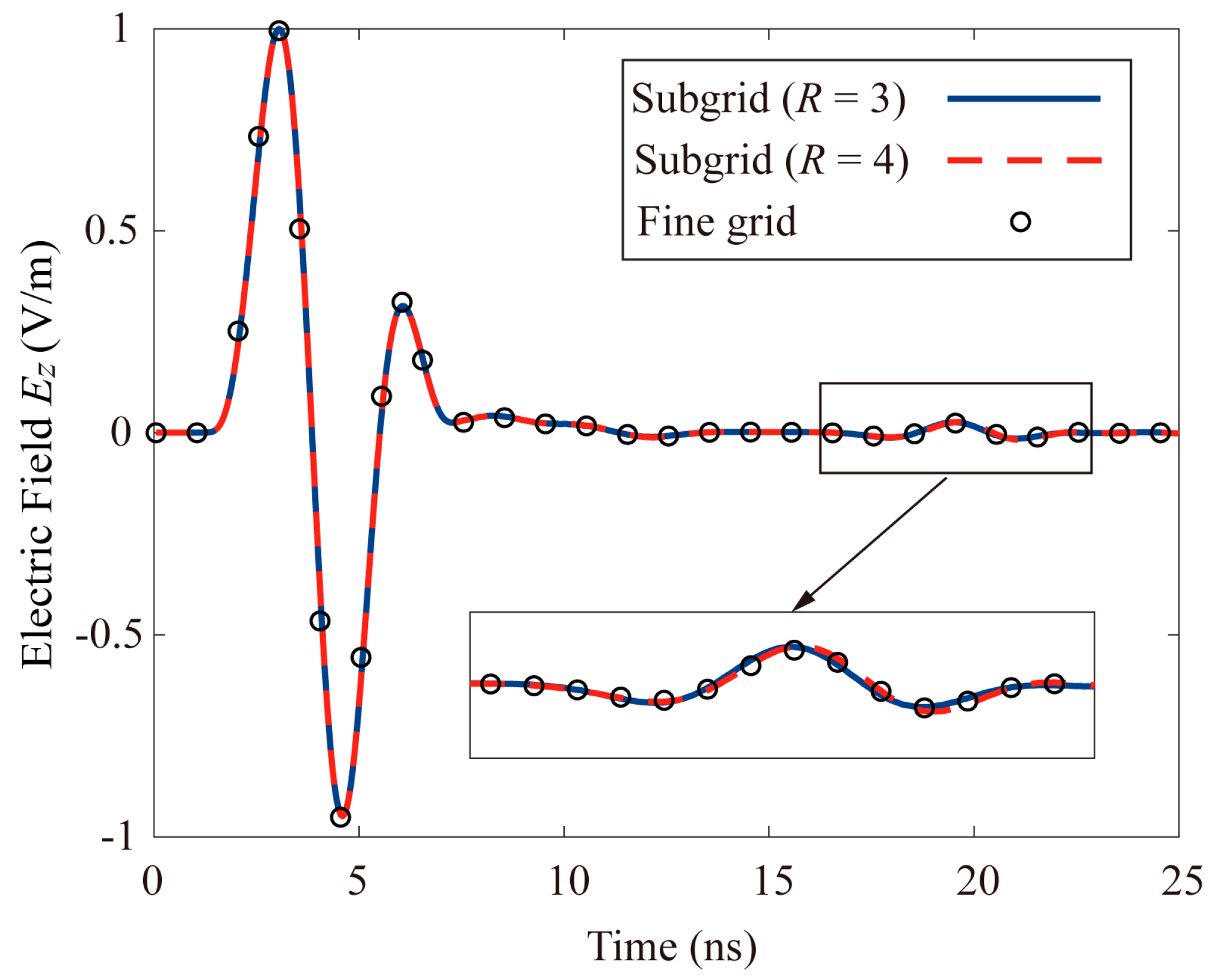
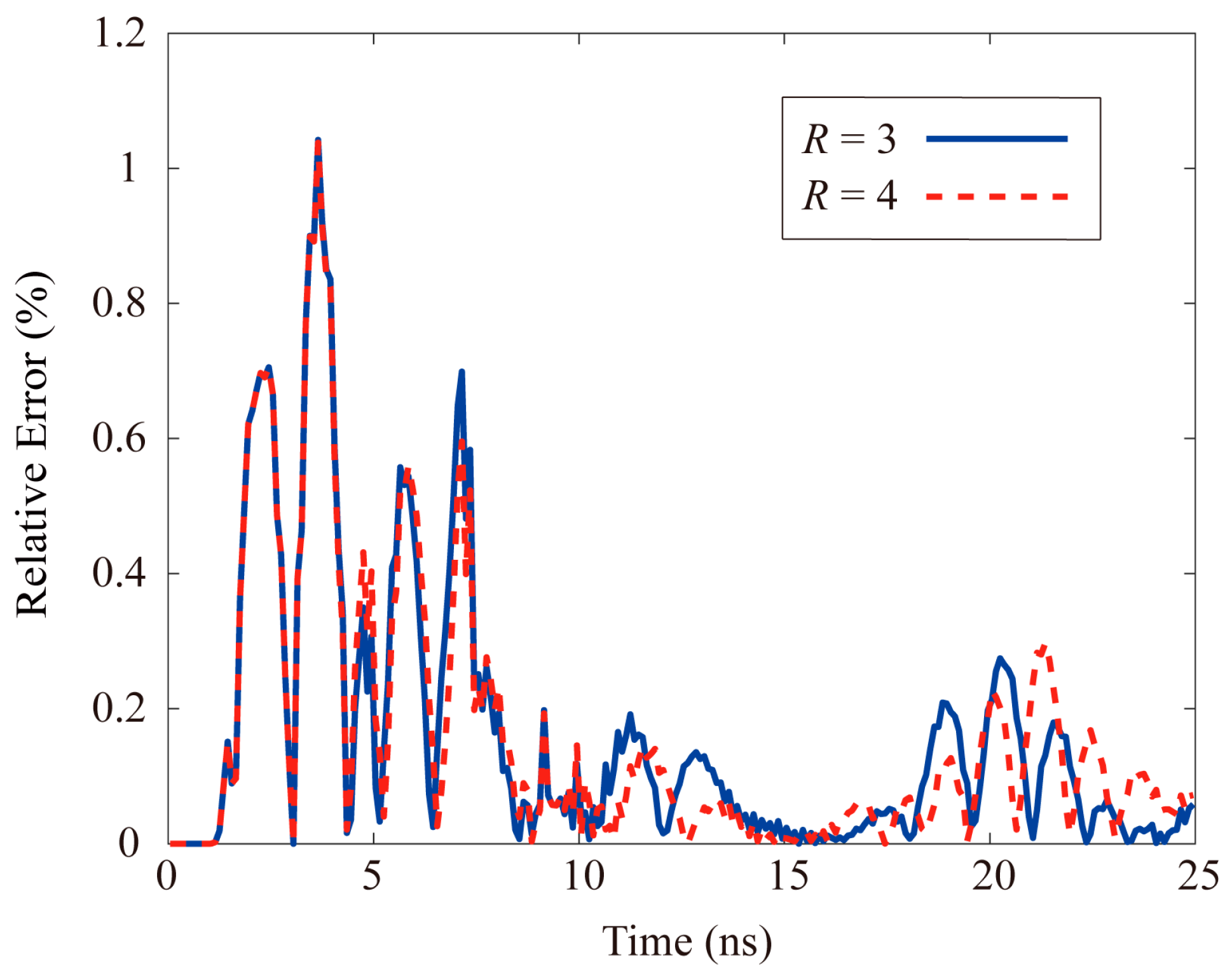
3.2. Dielectric Sphere
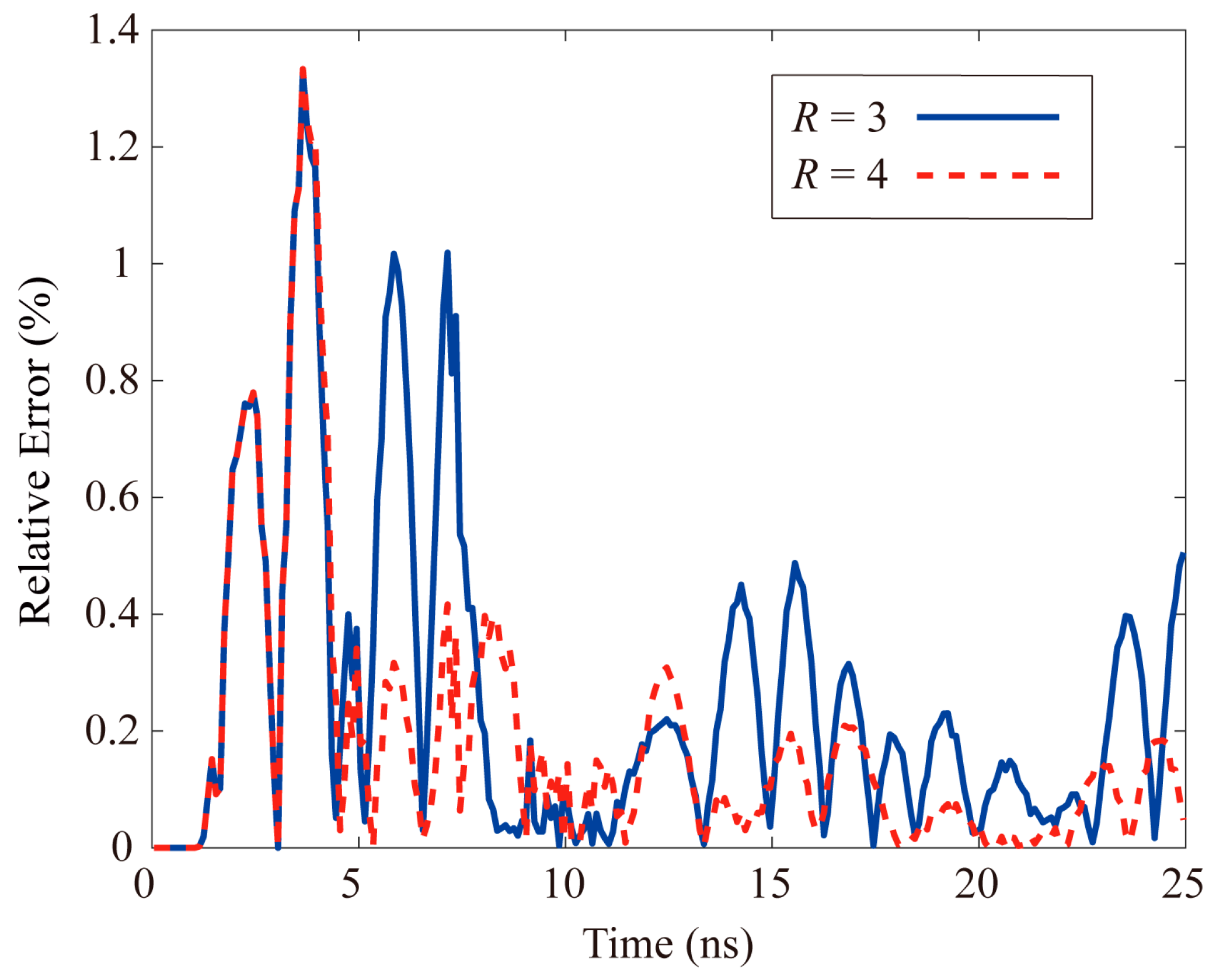
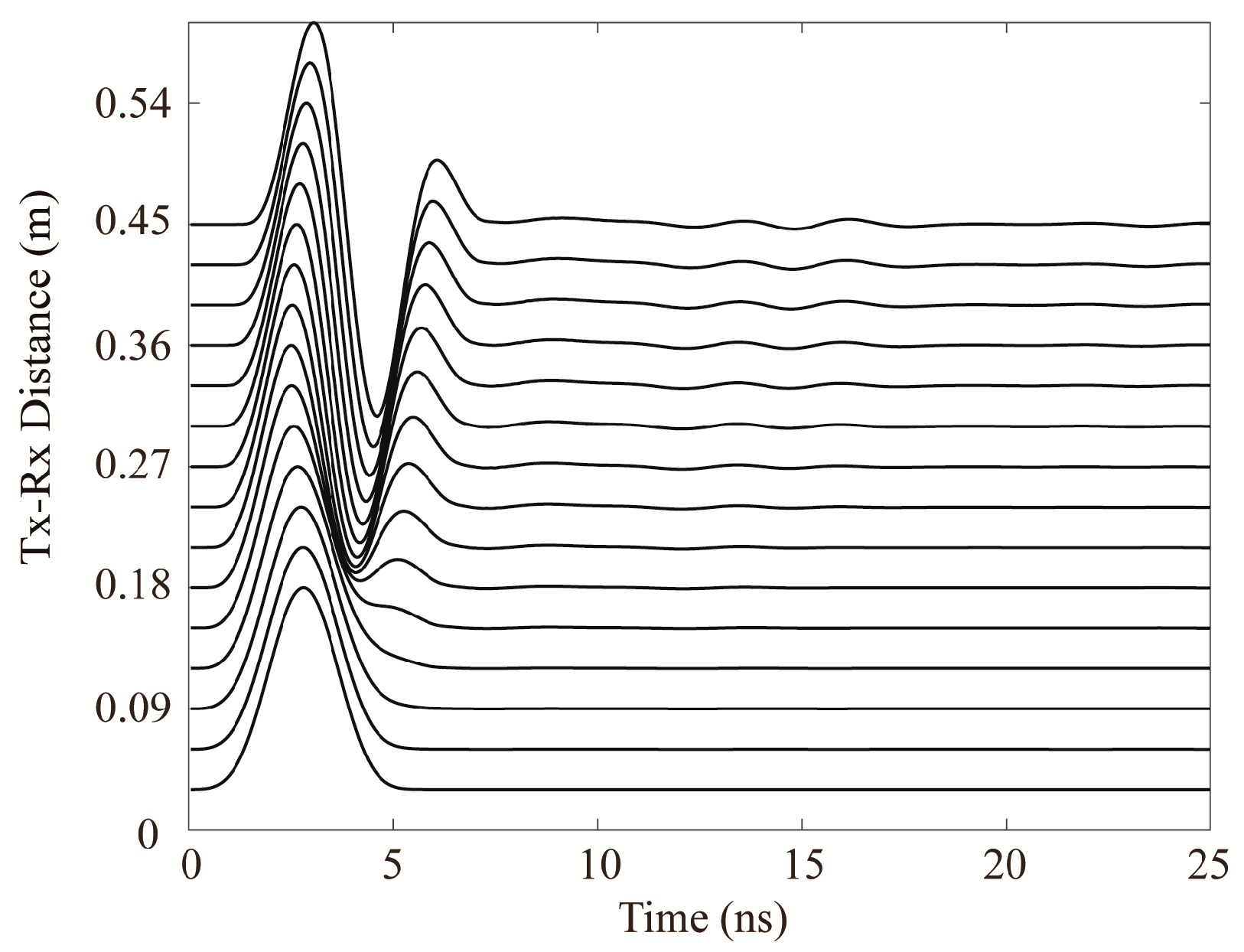
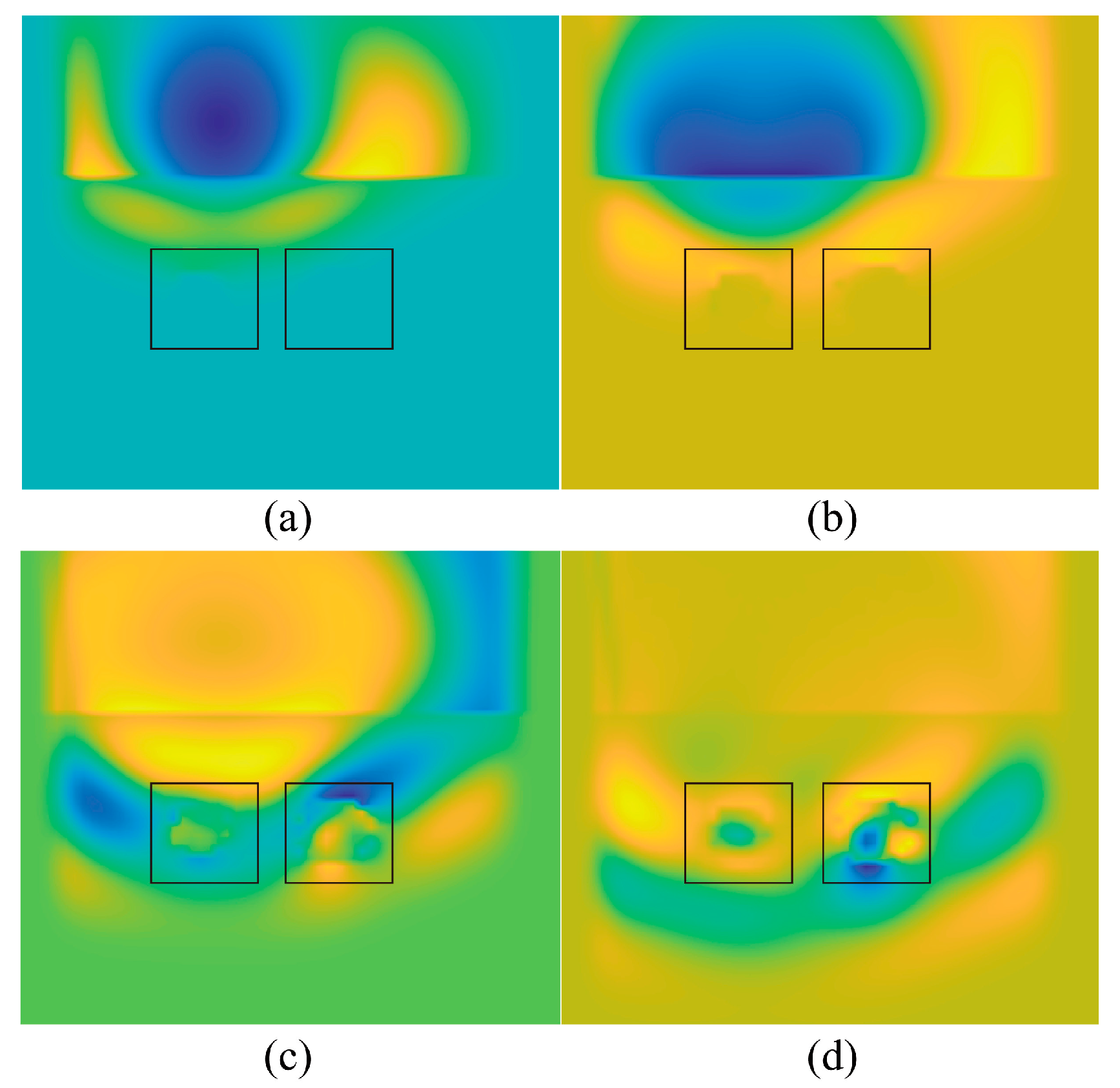
3.3. Multi-Target Detection with Dielectric Cylinder and Dielectric Sphere
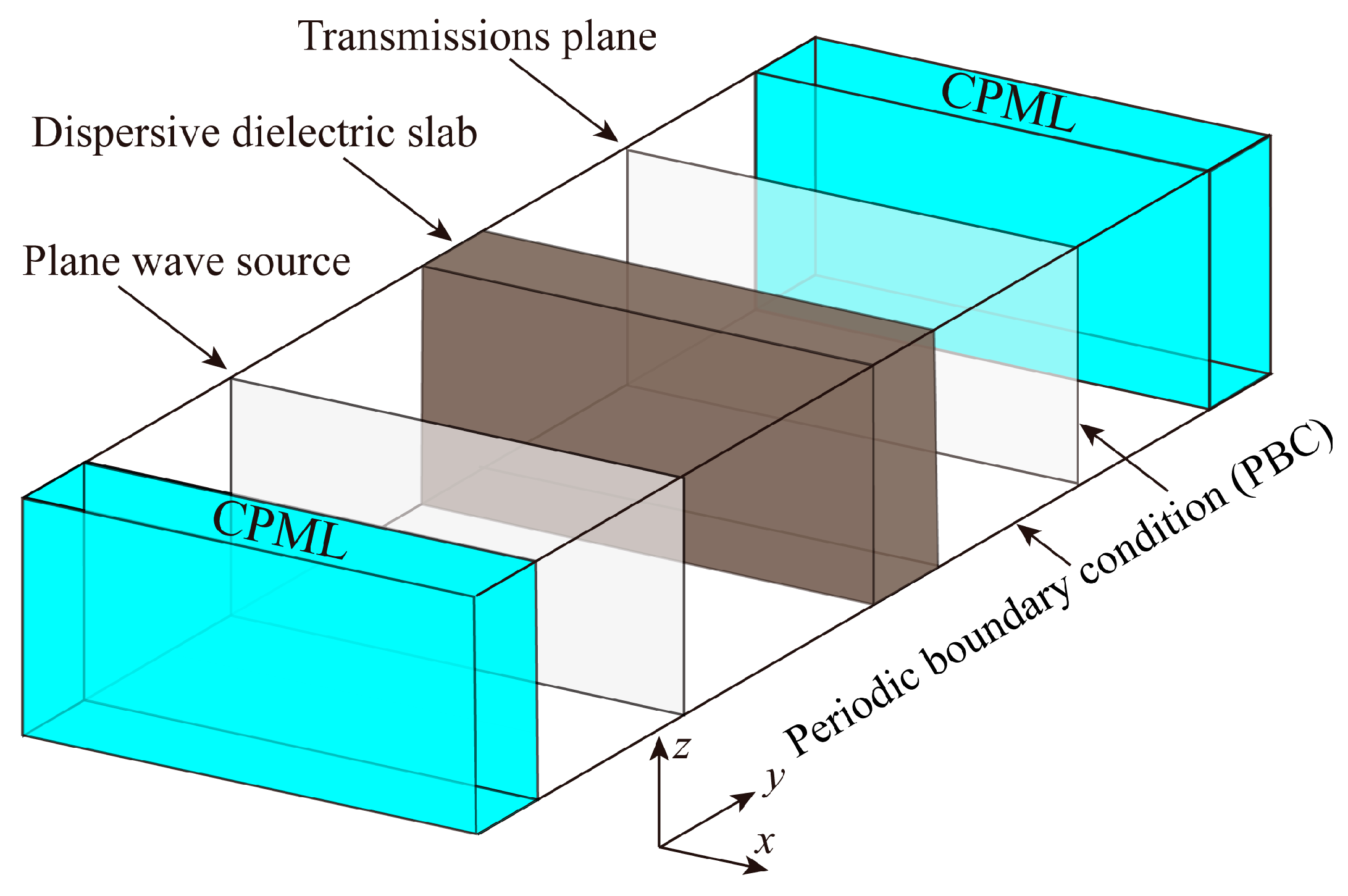
3.4. Dispersive Sphere
4. Conclusions and Future Prospects
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jonard, F.; André, F.; Pinel, N.; Warren, C.; Vereecken, H.; Lambot, S. Modeling of multilayered media Green’s functions with rough interfaces. IEEE Trans. Geosci. Remote Sens. 2019, 57, 7671–7681. [Google Scholar] [CrossRef]
- Harry, M. Ground Penetrating Radar Theory and Applications; Elsevier: Amsterdam, The Netherlands, 2008. [Google Scholar]
- Jaufer, R.M.; Ihamouten, A.; Goyat, Y.; Todkar, S.S.; Guilbert, D.; Assaf, A.; Derobert, X. A preliminary numerical study to compare the physical method and machine learning methods applied to GPR data for underground utility network characterization. Remote Sens. 2022, 14, 1047. [Google Scholar] [CrossRef]
- Shangguan, P.; Al-Qadi, I.L. Calibration of FDTD simulation of GPR signal for asphalt pavement compaction monitoring. IEEE Trans. Geosci. Remote Sens. 2014, 53, 1538–1548. [Google Scholar] [CrossRef]
- Giannakis, I.; Giannopoulos, A.; Warren, C. A machine learning-based fast-forward solver for ground penetrating radar with application to full-waveform inversion. IEEE Trans. Geosci. Remote Sens. 2019, 57, 4417–4426. [Google Scholar] [CrossRef]
- Stadler, S.; Igel, J. Developing realistic FDTD GPR antenna surrogates by means of particle swarm optimization. IEEE Trans. Antennas Propag. 2022, 70, 4259–4272. [Google Scholar] [CrossRef]
- Van der Kruk, J.; Diamanti, N.; Giannopoulos, A.; Vereecken, H. Inversion of dispersive GPR pulse propagation in waveguides with heterogeneities and rough and dipping interfaces. J. Appl. Geophys. 2012, 81, 88–96. [Google Scholar] [CrossRef]
- Koyan, P.; Tronicke, J. 3D modeling of ground-penetrating radar data across a realistic sedimentary model. Comput. Geosci. 2020, 137, 104422. [Google Scholar] [CrossRef]
- Liu, L.; Li, Z.; Arcone, S.; Fu, L.; Qiang, H. Radar wave scattering loss in a densely packed discrete random medium: Numerical modeling of a box-of-boulders experiment in the Mie regime. J. Appl. Geophys. 2013, 99, 68–75. [Google Scholar] [CrossRef]
- Meles, G.A.; Greenhalgh, S.A.; Green, A.G.; Maurer, H.; van der Kruk, J. GPR full-waveform sensitivity and resolution analysis using an FDTD adjoint method. IEEE Trans. Geosci. Remote Sens. 2011, 50, 1881–1896. [Google Scholar] [CrossRef]
- Loewer, M.; Igel, J.; Wagner, N. Spectral decomposition of soil electrical and dielectric losses and prediction of in situ GPR performance. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2015, 9, 212–220. [Google Scholar] [CrossRef]
- Giannakis, I.; Giannopoulos, A.; Warren, C. A realistic FDTD numerical modeling framework of ground penetrating radar for landmine detection. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2015, 9, 37–51. [Google Scholar] [CrossRef]
- Yang, M.; Fang, H.; Wang, F.; Jia, H.; Lei, J.; Zhang, D. The three dimension first-order symplectic partitioned Runge-Kutta scheme simulation for GPR wave propagation in pavement structure. IEEE Access 2019, 7, 151705–151712. [Google Scholar] [CrossRef]
- Liao, D.H.; Dogaru, T. Full-wave characterization of rough terrain surface scattering for forward-looking radar applications. IEEE Trans. Antennas Propag. 2012, 60, 3853–3866. [Google Scholar] [CrossRef]
- Wu, P.; Xie, Y.; Jiang, H.; Natsuki, T. Modeling of bandpass GPR problem by HIE procedure with enhanced absorption. IEEE Geosci. Remote Sens. Lett. 2021, 19, 1–5. [Google Scholar] [CrossRef]
- Natsui, M.; Ametani, A.; Mahseredjian, J.; Motoyama, H. Earth current and GPR distributions due to lightning and effect of a distribution line. IEEE Trans. Electromagn. Compat. 2019, 62, 2119–2127. [Google Scholar] [CrossRef]
- Yee, K.S. Numerical solution of initial boundary value problems involving Maxwell’s equations in isotropic media. IEEE Trans. Antennas Propag. 1966, 14, 302–307. [Google Scholar]
- Taflove, A.; Hagness, S.C.; Piket-May, M. Computational electromagnetics: The finite-difference time-domain method. Electr. Eng. Handb. 2005, 3, 629–670. [Google Scholar]
- Bekmambetova, F.; Triverio, P. A dissipation theory for potentials-based FDTD for lossless Inhomogeneous media. IEEE Antennas Wirel. Propag. Lett. 2021, 21, 486–490. [Google Scholar] [CrossRef]
- Al-Jabr, A.A.; Alsunaidi, M.A.; Ng, T.; Ooi, B.S. A simple FDTD algorithm for simulating EM-wave propagation in general dispersive anisotropic material. IEEE Trans. Antennas Propag. 2012, 61, 1321–1326. [Google Scholar] [CrossRef]
- Luebbers, R.; Hunsberger, F.P.; Kunz, K.; Standler, R.B.; Schneider, M. A frequency-dependent finite-difference time-domain formulation for dispersive materials. IEEE Trans. Electromagn. Compat. 1990, 32, 222–227. [Google Scholar] [CrossRef]
- Joseph, R.M.; Hagness, S.C.; Taflove, A. Direct time integration of Maxwell’s equations in linear dispersive media with absorption for scattering and propagation of femtosecond electromagnetic pulses. Opt. Lett. 1991, 16, 1412–1414. [Google Scholar] [CrossRef]
- Warren, C.; Giannopoulos, A.; Giannopoulos, I. gprMax: Open source software to simulate electromagnetic wave propagation for Ground Penetrating Radar. Comput. Phys. Commun. 2016, 209, 163–170. [Google Scholar] [CrossRef]
- Lin, C.; Wang, X.; Li, Y.; Zhang, F.; Xu, Z.; Du, Y. Forward modelling and GPR imaging in leakage detection and grouting evaluation in tunnel lining. KSCE J. Civ. Eng. 2020, 24, 278–294. [Google Scholar] [CrossRef]
- Shaari, A.; Ahmad, R.S.; Chew, T.H. Effects of antenna-target polarization and target-medium dielectric contrast on GPR signal from non-metal pipes using FDTD simulation. NDT E Int. 2010, 43, 403–408. [Google Scholar] [CrossRef]
- Xie, G.; Huang, Z.; Fang, M.; Wei, E.I. Simulating Maxwell–Schrödinger equations by high-order symplectic FDTD algorithm. IEEE J. Multiscale Multiphysics Comput. Tech. 2019, 4, 143–151. [Google Scholar] [CrossRef]
- Sha, W.; Huang, Z.; Wu, X.; Chen, M. Application of the symplectic finite-difference time-domain scheme to electromagnetic simulation. J. Comput. Phys. 2007, 225, 33–50. [Google Scholar] [CrossRef]
- Xie, G.; Huang, Z.; Fang, M.; Wu, X. A unified 3-D ADI-FDTD algorithm with one-step leapfrog approach for modeling frequency-dependent dispersive media. Int. J. Numer. Model. Electron. Netw. Devices Fields 2020, 33, e2666. [Google Scholar] [CrossRef]
- Chen, J.; Li, J.; Liu, Q.H. Analyzing graphene-based absorber by using the WCS-FDTD method. IEEE Trans. Microw. Theory Tech. 2017, 65, 3689–3696. [Google Scholar] [CrossRef]
- Yan, J.; Jiao, D. An unsymmetric FDTD subgridding algorithm with unconditional stability. IEEE Trans. Antennas Propag. 2018, 66, 4137–4150. [Google Scholar] [CrossRef]
- Kuo, C.W.; Kuo, C.M. Finite-difference time-domain analysis of the shielding effectiveness of metallic enclosures with apertures using a novel subgridding algorithm. IEEE Trans. Electromagn. Compat. 2016, 58, 1595–1601. [Google Scholar] [CrossRef]
- Ye, Z.; Liao, C.; Xiong, X.; Zhang, M. A novel FDTD subgridding method with improved separated temporal and spatial subgridding interfaces. IEEE Antennas Wirel. Propag. Lett. 2016, 16, 1011–1015. [Google Scholar] [CrossRef]
- Kulas, L.; Mrozowski, M. Low-reflection subgridding. IEEE Trans. Microw. Theory Tech. 2005, 53, 1587–1592. [Google Scholar] [CrossRef]
- Ye, Z.; Liao, X.; Zhang, J. A novel three-dimensional FDTD subgridding method for the coupling analysis of shielded cavity excited by ambient wave. IEEE Trans. Electromagn. Compat. 2019, 62, 2441–2449. [Google Scholar] [CrossRef]
- Xiao, K.; Pommerenke, D.J.; Drewniak, J.L. A three-dimensional FDTD subgridding algorithm with separated temporal and spatial interfaces and related stability analysis. IEEE Trans. Antennas Propag. 2007, 55, 1981–1990. [Google Scholar] [CrossRef]
- Mai, H.X.; Chen, J.; Zhang, A. A hybrid algorithm based on FDTD and HIE-FDTD methods for simulating shielding enclosure. IEEE Trans. Electromagn. Compat. 2017, 60, 1393–1399. [Google Scholar] [CrossRef]
- Wang, B.Z.; Wang, Y.; Yu, W.; Mittra, R. A hybrid 2-D ADI-FDTD subgridding scheme for modeling on-chip interconnects. IEEE Trans. Adv. Packag. 2001, 24, 528–533. [Google Scholar] [CrossRef]
- Ahmed, I.; Chen, Z. A hybrid ADI-FDTD subgridding scheme for efficient electromagnetic computation. Int. J. Numer. Model. Electron. Netw. Devices Fields 2004, 17, 237–249. [Google Scholar] [CrossRef]
- Wei, X.; Shao, W.; Wang, X.H. Hybrid sub-gridded time-domain method for ground penetrating radar simulations including dispersive materials. IEEE Access 2018, 6, 15777–15786. [Google Scholar] [CrossRef]
- Wei, X.K.; Zhang, X.; Diamanti, N.; Shao, W.; Sarris, C.D. Subgridded FDTD modeling of ground penetrating radar scenarios beyond the courant stability limit. IEEE Trans. Geosci. Remote Sens. 2017, 55, 7189–7198. [Google Scholar] [CrossRef]
- Shibayama, J.; Nomura, A.; Ando, R.; Tamauchi, J.; Nakano, H. A frequency-dependent LOD-FDTD method and its application to the analyses of plasmonic waveguide devices. IEEE J. Quantum Electron. 2009, 46, 40–49. [Google Scholar] [CrossRef]
- Tan, E. Unconditionally stable LOD–FDTD method for 3-D Maxwell’s equations. IEEE Microw. Wirel. Compon. Lett. 2007, 17, 85–87. [Google Scholar] [CrossRef]
- Liang, T.; Shao, W.; Wei, X.; Liang, M.S. Hybrid sub-gridding ADE–FDTD method of modeling periodic metallic nanoparticle arrays. Chin. Phys. B 2018, 27, 100204. [Google Scholar] [CrossRef]
- Prokopidis, K.; Zografopoulos, D. Modeling plasmonic structures using LOD-FDTD methods with accurate dispersion models of metals at optical wavelengths. J. Lightwave Technol. 2016, 35, 193–200. [Google Scholar] [CrossRef]
- Sullivan, D. Z-transform theory and the FDTD method. IEEE Trans. Antennas Propag. 1996, 44, 28–34. [Google Scholar] [CrossRef]
- Jiang, H.; Cui, T.; Wu, L.; Bo, X.C.; Wu, H.T. Efficient implementations of SC-PML for arbitrary media using DSP techniques. IEEE Trans. Electromagn. Compat. 2018, 61, 962–965. [Google Scholar] [CrossRef]
- Tekbas, K.; Costen, F.; Bérenger, J.P.; Himeno, R.; Yokota, H. Subcell modeling of frequency-dependent thin layers in the FDTD method. IEEE Trans. Antennas Propag. 2017, 65, 278–286. [Google Scholar] [CrossRef]



| Method | Memory Cost (MB) | Execution Time (s) |
|---|---|---|
| Fine-grid FDTD | 798.2 | 829.5 |
| subgrid method (R = 3) | 142.4 | 62.0 |
| subgrid method (R = 4) | 240.4 | 88.6 |
| Method | Memory Cost (MB) | Execution Time (s) |
|---|---|---|
| Fine-grid FDTD | 1866.6 | 1182.4 |
| subgrid method (R = 3) | 188.8 | 75.4 |
| subgrid method (R = 4) | 390.0 | 129.9 |
| Method | Memory Cost (MB) | Execution Time (s) |
|---|---|---|
| Fine-grid FDTD | 610.5 | 798.2 |
| subgrid method (R = 3) | 59.2 | 142.2 |
| subgrid method (R = 4) | 86.7 | 240.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, G.; Song, Z.; Hou, G.; Fang, M.; Feng, N.; Huang, Z. Efficacious GPR Implementations of Z-Transform-Based Hybrid LOD-FDTD with Subgridding Scheme: Theoretical Formalism and Numerical Study. Remote Sens. 2022, 14, 5393. https://doi.org/10.3390/rs14215393
Xie G, Song Z, Hou G, Fang M, Feng N, Huang Z. Efficacious GPR Implementations of Z-Transform-Based Hybrid LOD-FDTD with Subgridding Scheme: Theoretical Formalism and Numerical Study. Remote Sensing. 2022; 14(21):5393. https://doi.org/10.3390/rs14215393
Chicago/Turabian StyleXie, Guoda, Ziheng Song, Guilin Hou, Ming Fang, Naixing Feng, and Zhixiang Huang. 2022. "Efficacious GPR Implementations of Z-Transform-Based Hybrid LOD-FDTD with Subgridding Scheme: Theoretical Formalism and Numerical Study" Remote Sensing 14, no. 21: 5393. https://doi.org/10.3390/rs14215393
APA StyleXie, G., Song, Z., Hou, G., Fang, M., Feng, N., & Huang, Z. (2022). Efficacious GPR Implementations of Z-Transform-Based Hybrid LOD-FDTD with Subgridding Scheme: Theoretical Formalism and Numerical Study. Remote Sensing, 14(21), 5393. https://doi.org/10.3390/rs14215393










