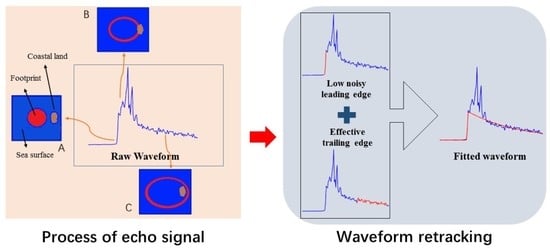
Coastal Waveform Retracking for HY-2B Altimeter Data by Determining the Effective Trailing Edge and the Low Noise Leading Edge
Abstract
:1. Introduction
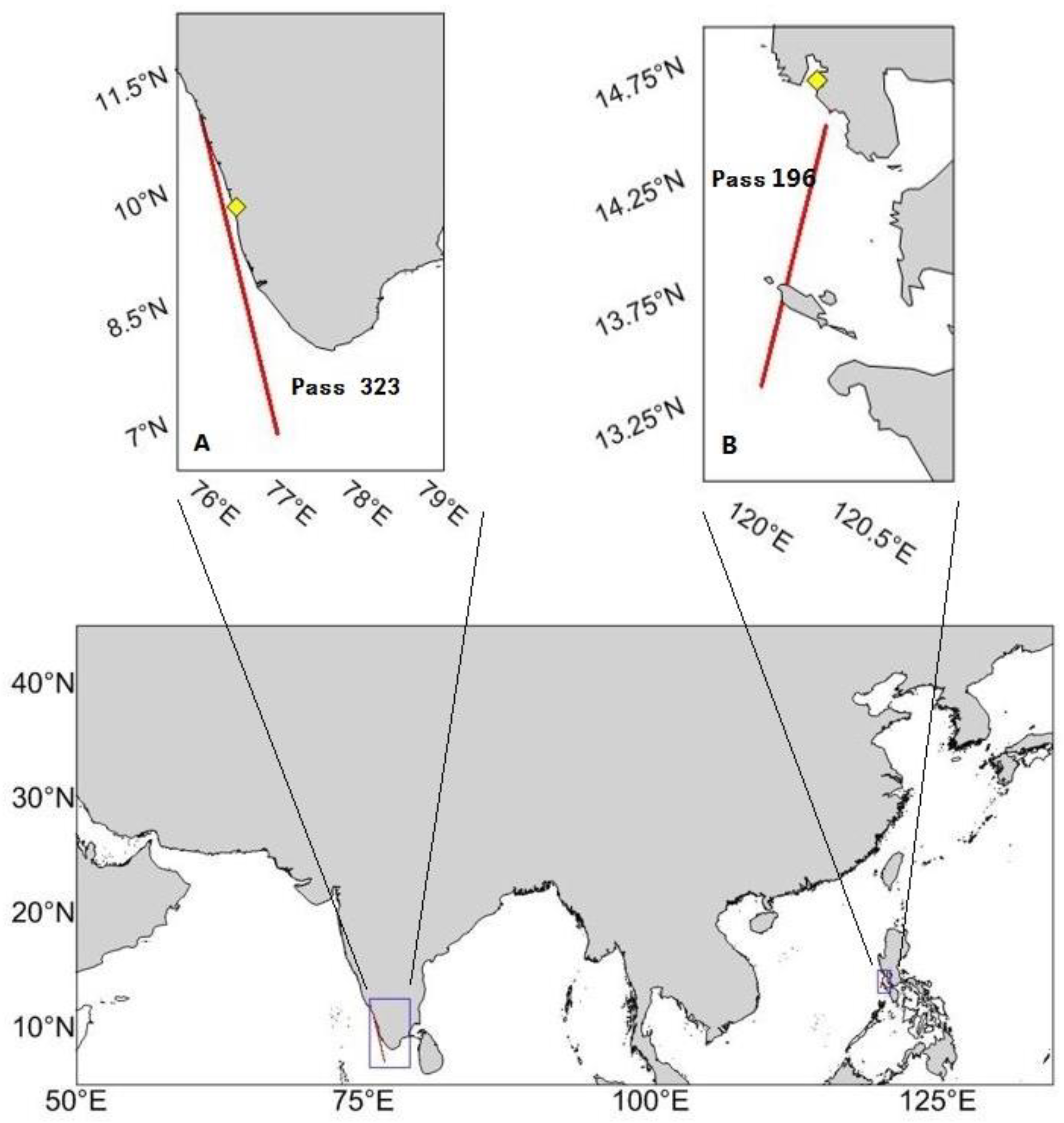
2. Study Areas and Data
3. Method
3.1. The Retracking Functional Form
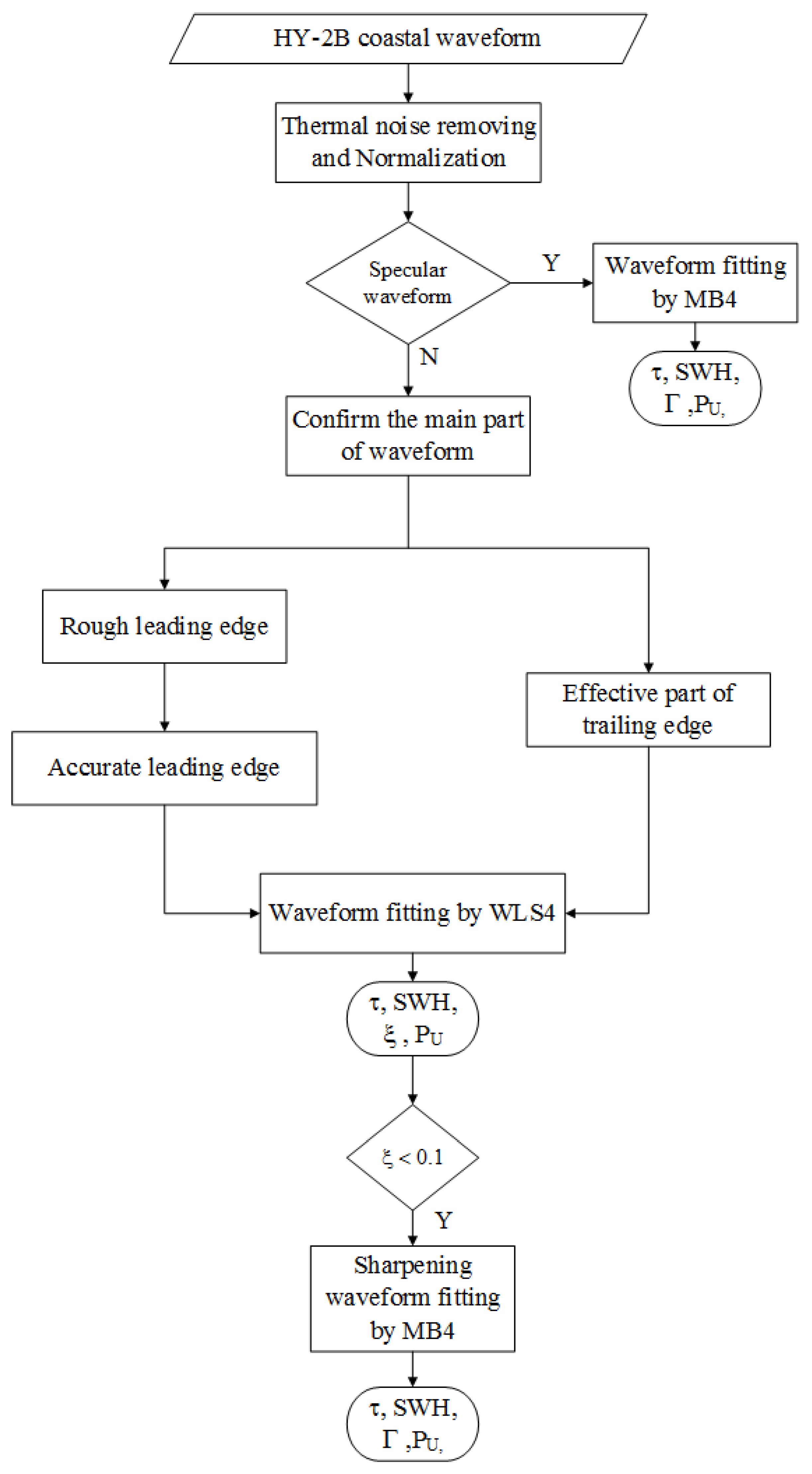
3.2. Waveform Retracking Method
3.2.1. Thermal Noise Removal and Normalization
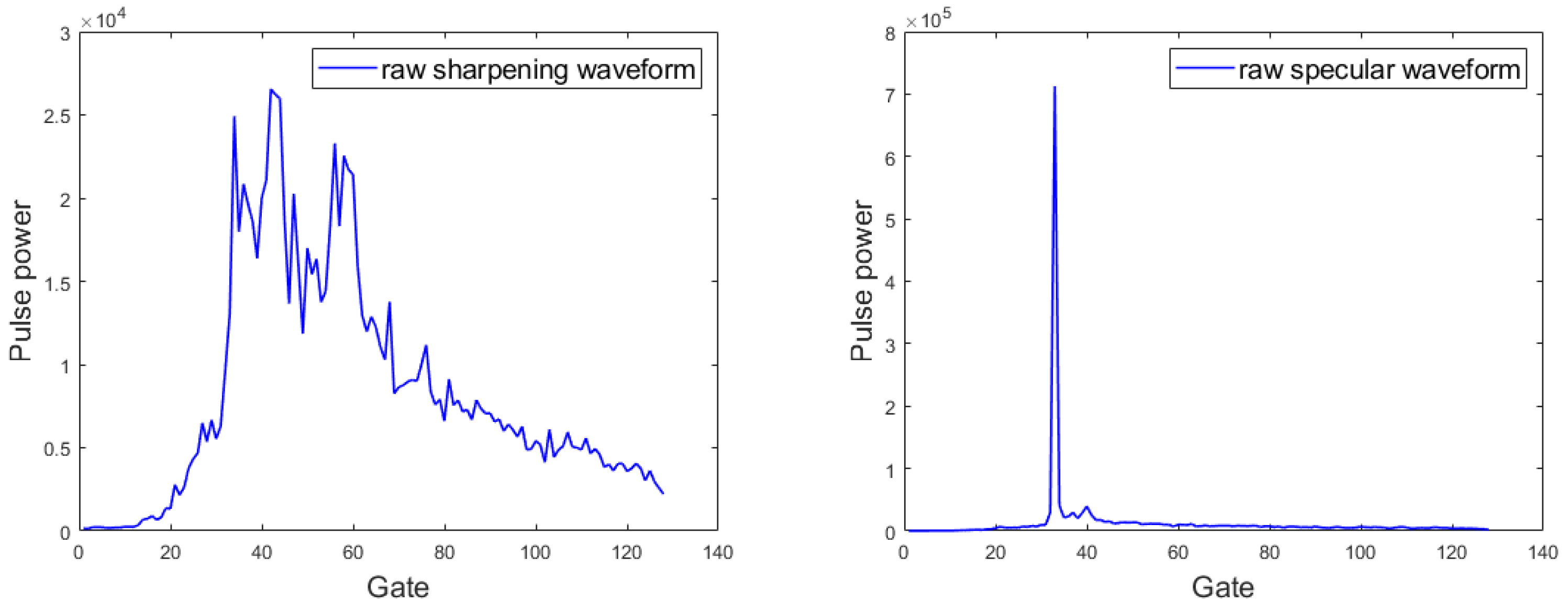
3.2.2. Specular Echo Processing
3.2.3. Non-Specular Echo Processing
- Confirming the main part of waveform
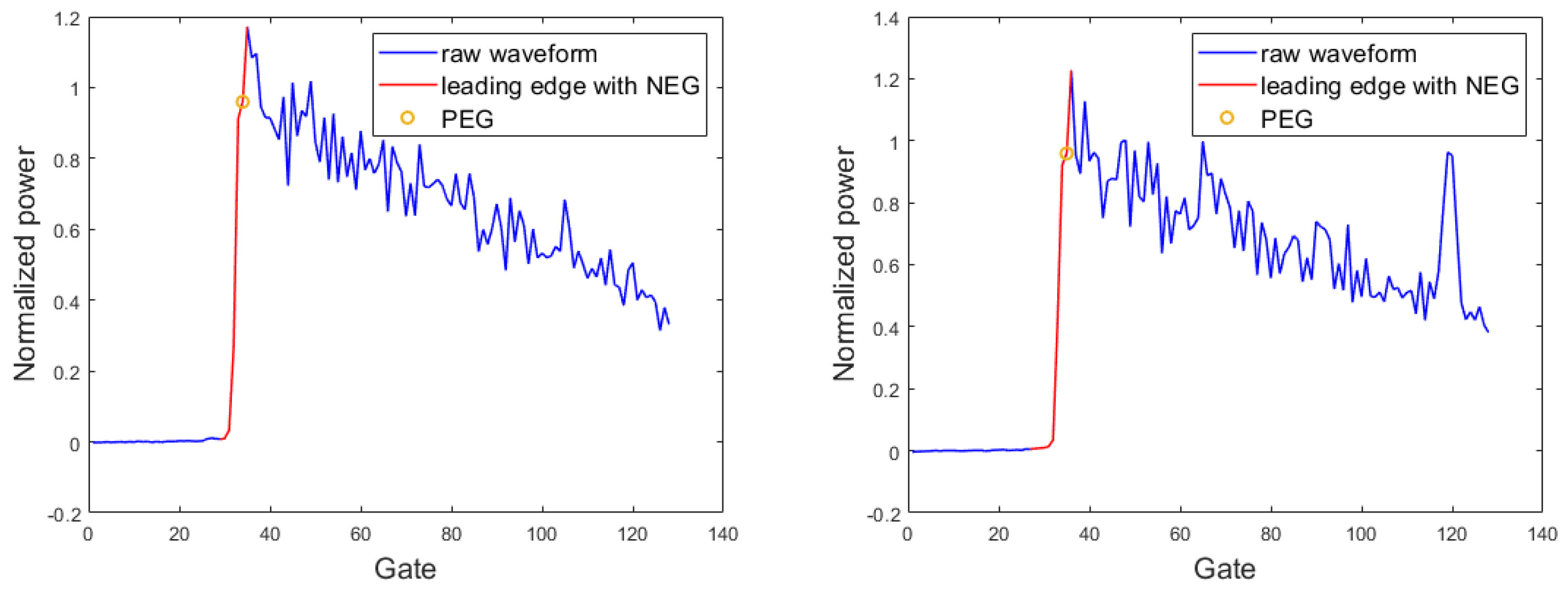
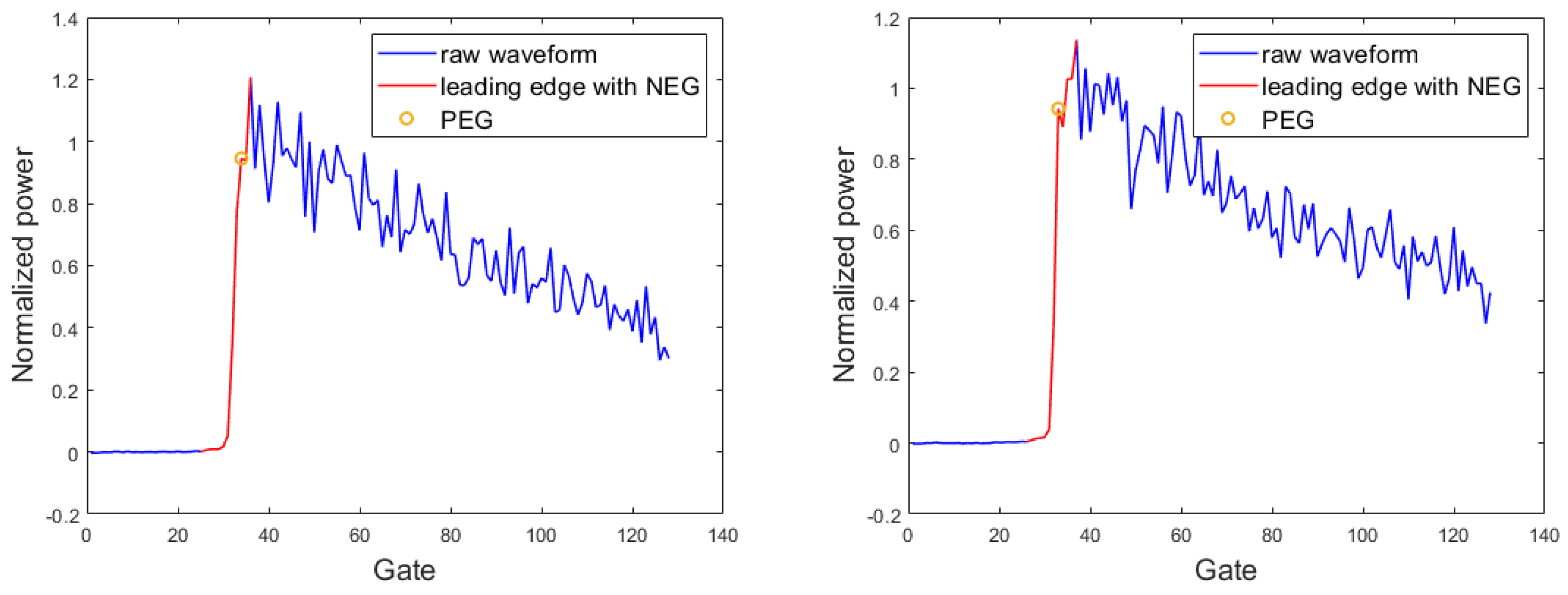
- Leading edge processing
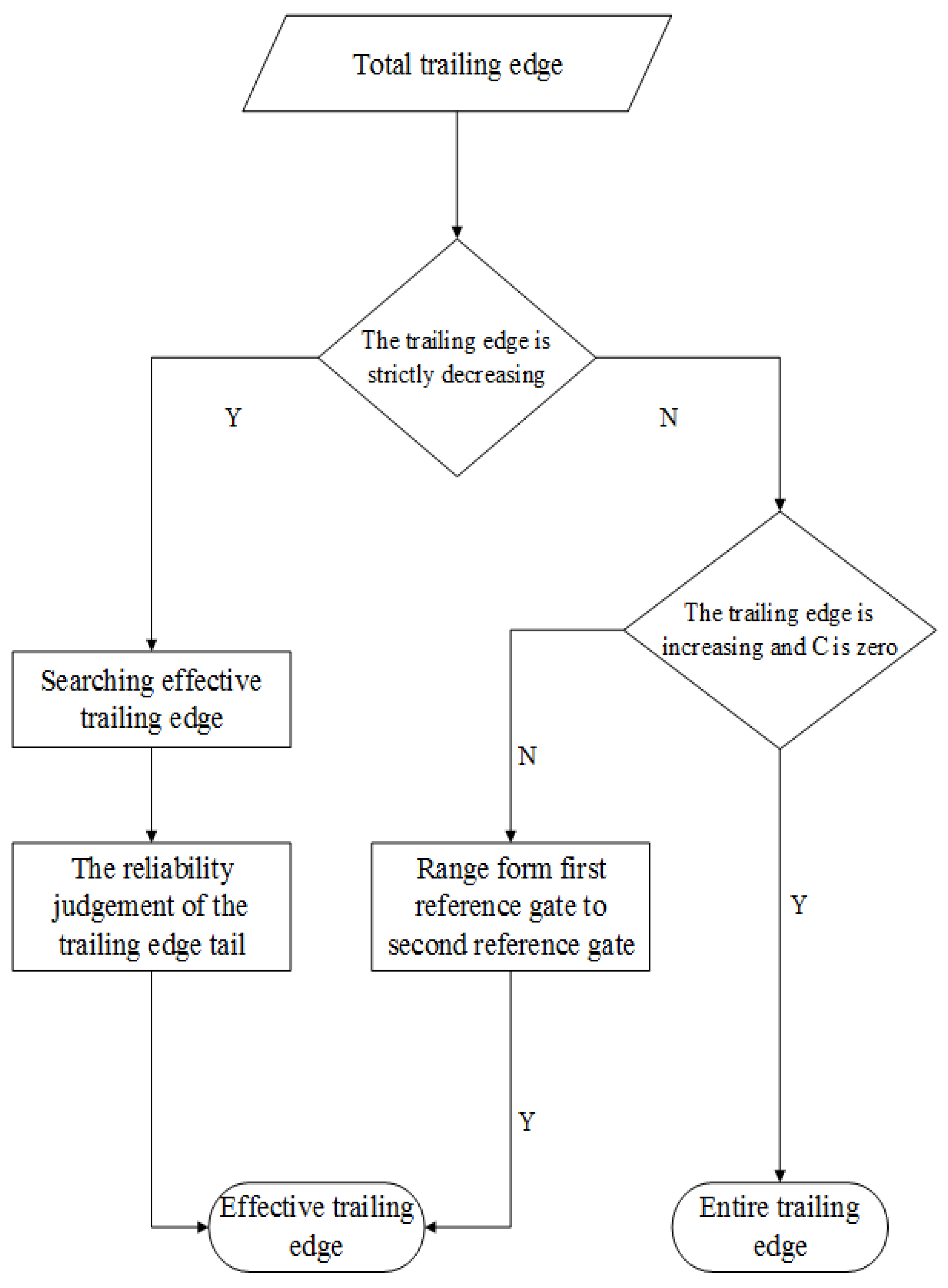
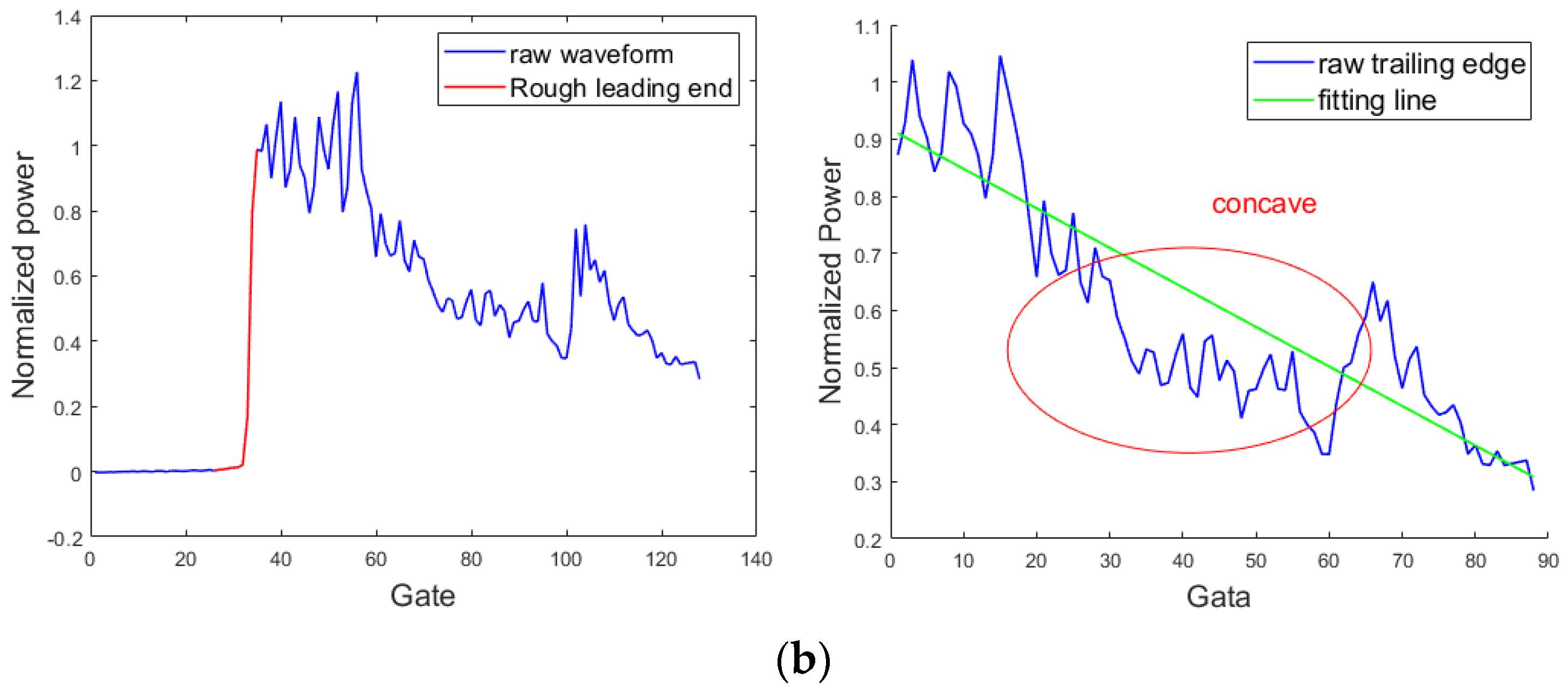
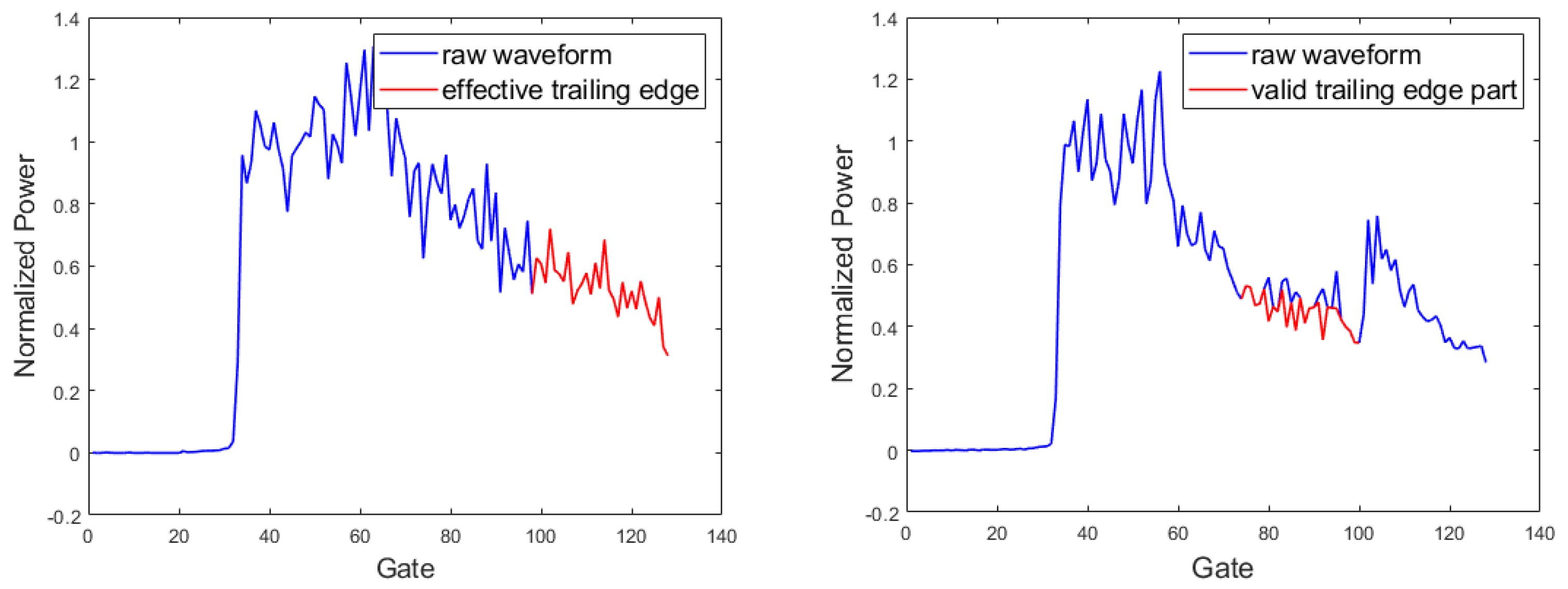
- The processing of trailing edge
- Weighting and fitting
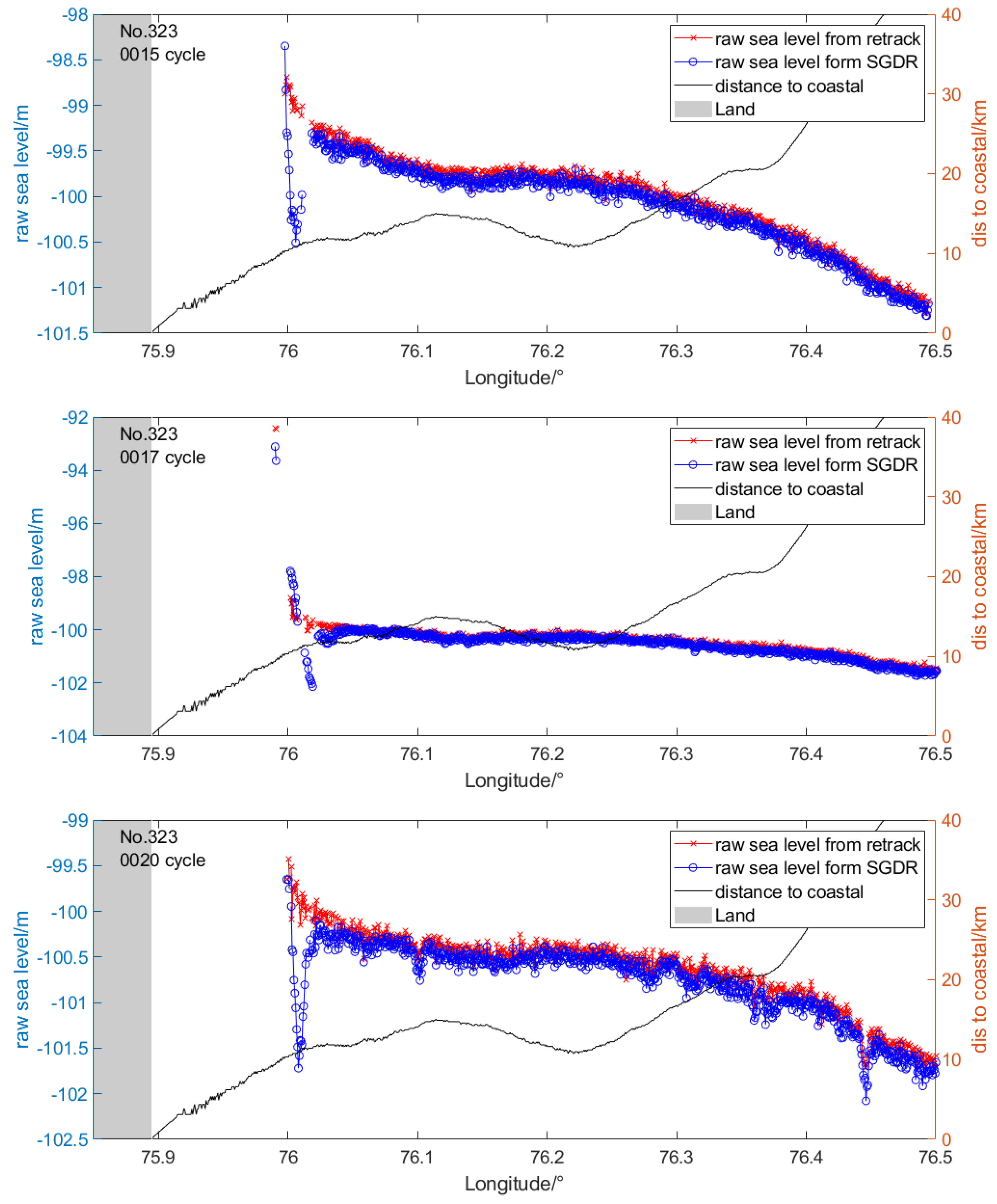
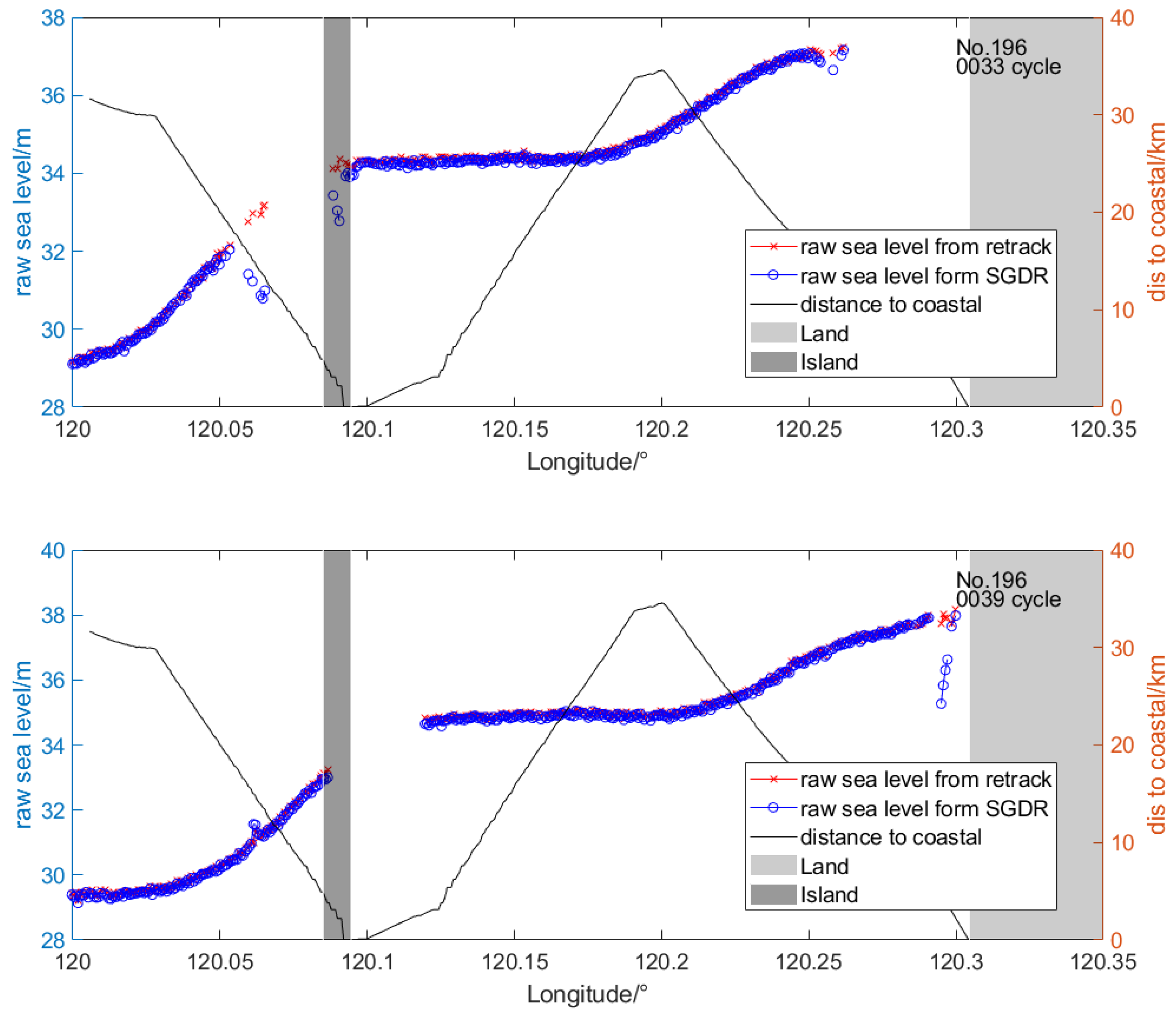
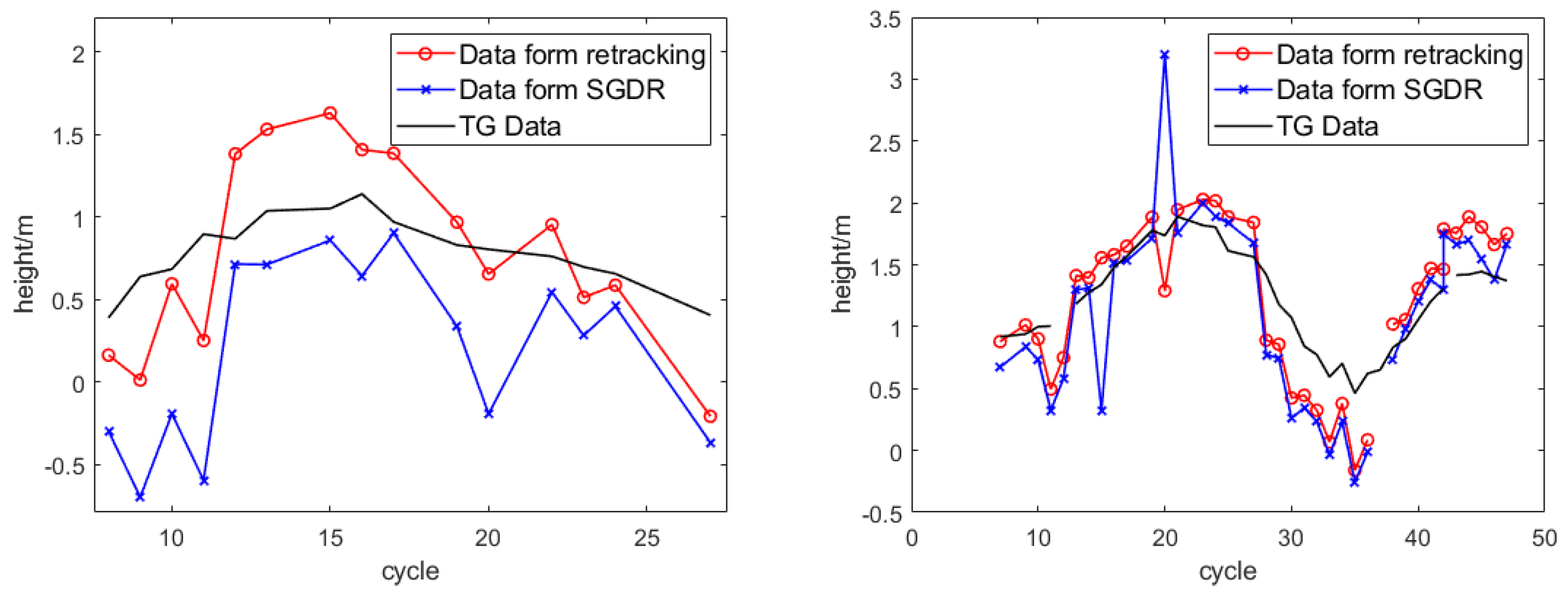
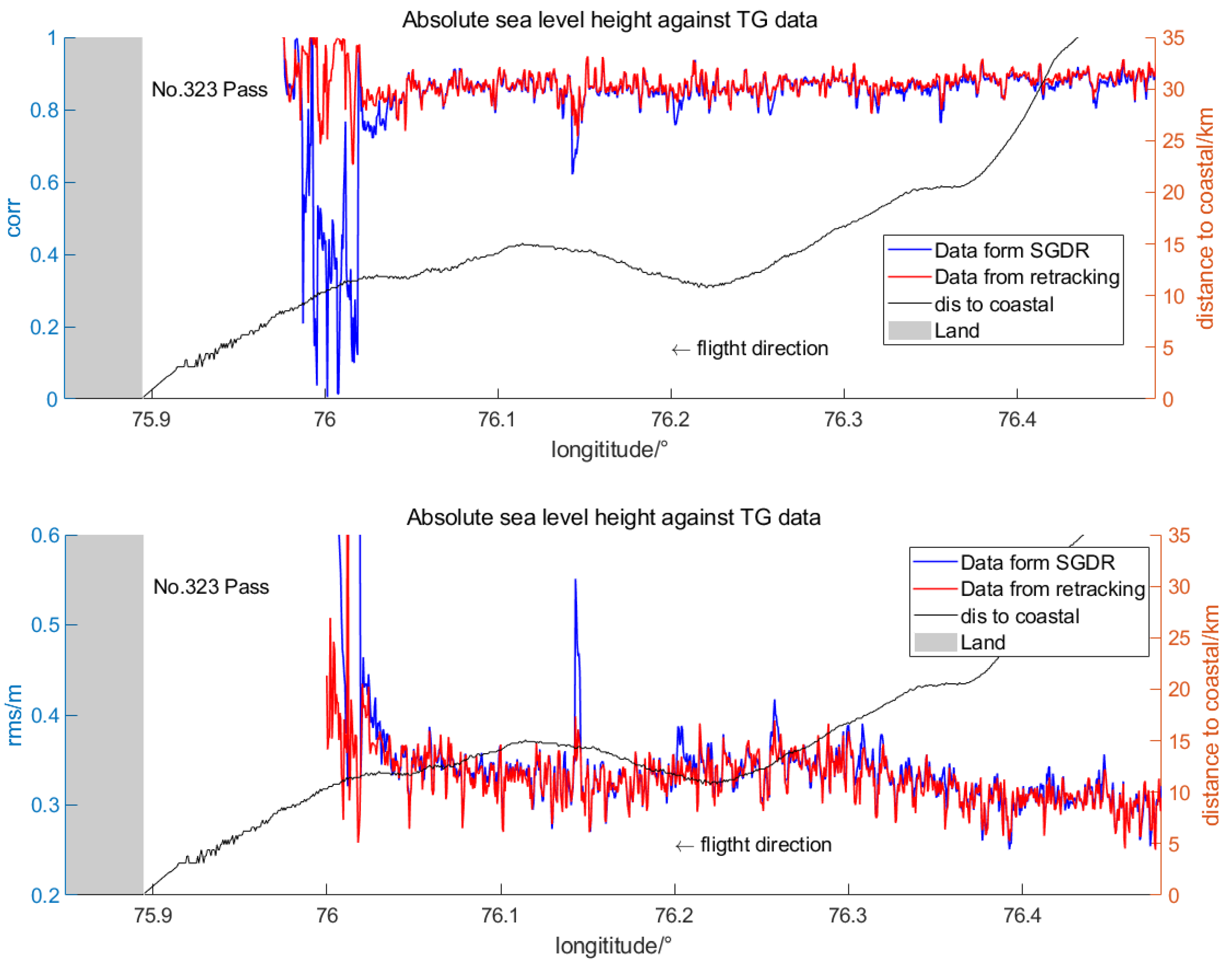
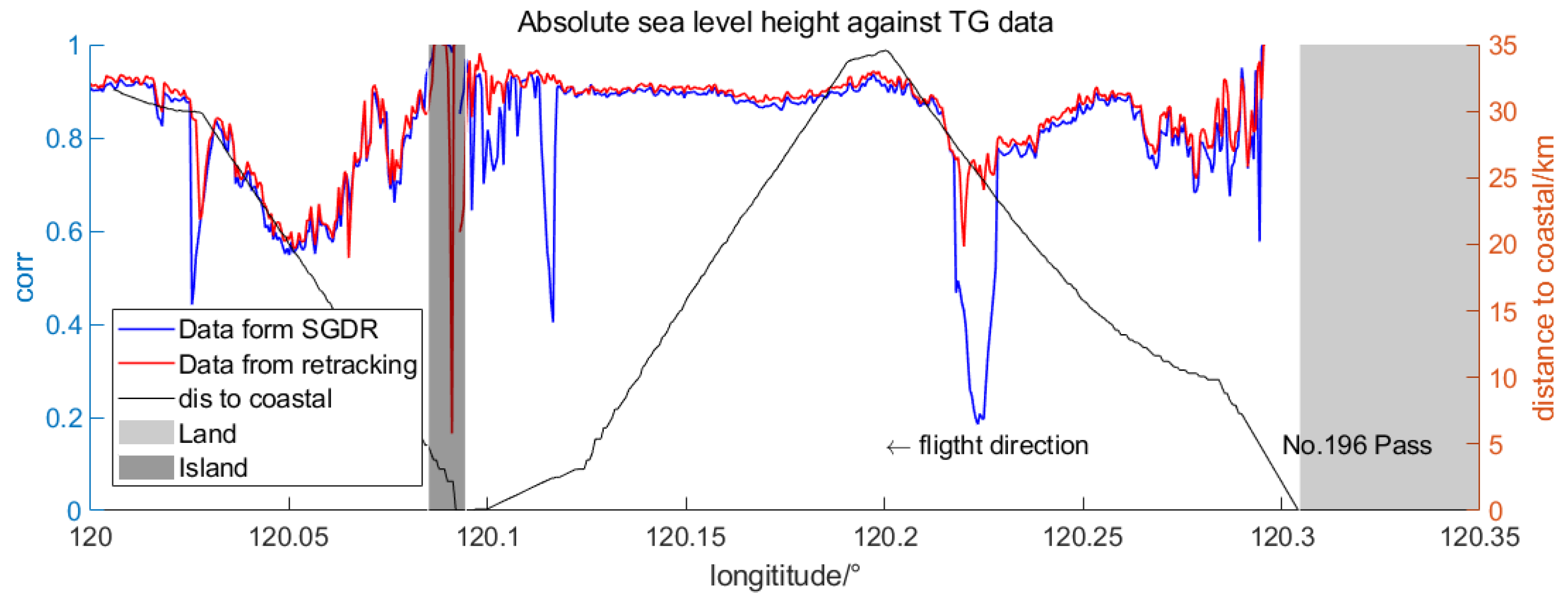
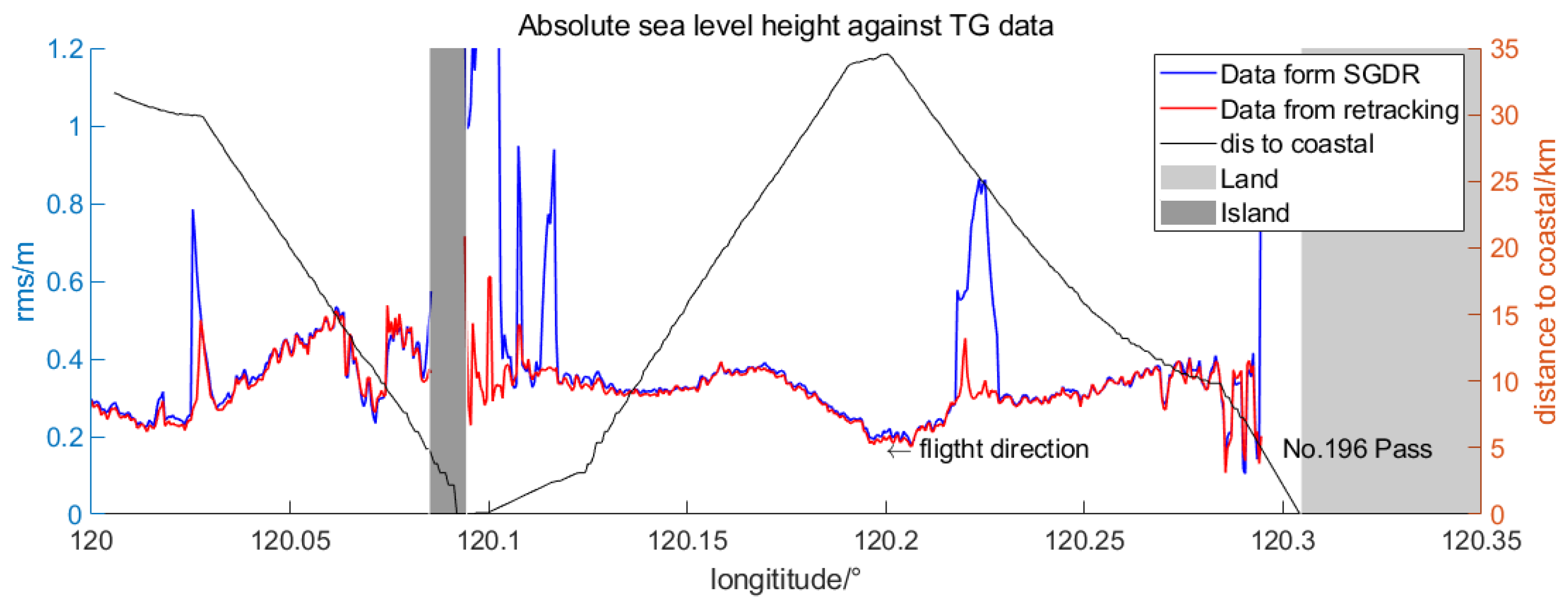
4. Waveform Retracking Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Brown, G. The average impulse response of a rough surface and its applications. IEEE Trans. Antennas Propag. 1997, 25, 67–74. [Google Scholar] [CrossRef]
- Hayne, G.S. Radar altimeter mean return waveforms from near-normal-incidence ocean surface scattering. IEEE Trans. Antennas Propag. 1980, 28, 687–692. [Google Scholar] [CrossRef] [Green Version]
- Martin, T.V.; Zwally, H.J.; Brenner, A.C. Analysis and retracking of continental ice sheet radar altimeter waveforms. J. Geophys. Res. Ocean. 1983, 88, 1608–1616. [Google Scholar] [CrossRef]
- Wingham, D.J.; Raplet, C.G.; Griffiths, H. New techniques in satellite tracking system. In Proceedings of the IGARSS’86 Symposium, Zurich, Switzerland, 8 September 1986; pp. 1339–1344. [Google Scholar]
- Davis, C.H. A robust threshold retracking algorithm for measuring ice-sheet surface elevation change from satellite radar altimeters. IEEE Trans. Geosci. Remote Sens. 1997, 35, 974–979. [Google Scholar]
- Hwang, C.; Guo, J.; Deng, X.; Hsu, H.Y.; Liu, Y. Coastal gravity anomalies from retracked GEOSAT/GM altimetry: Improvement, limitation and the role of airborne gravity data. J. Geod. 2006, 80, 204–216. [Google Scholar] [CrossRef]
- Sandwell, D.T.; Smith, W. Retracking RES-1 altimeter waveforms for optimal gravity field recovery. Geophys. J. R. Astron. Soc. 2005, 163, 79–89. [Google Scholar] [CrossRef] [Green Version]
- Quartly, G.D.; Smith, W.H.F.; Passaro, M. Removing Intra-1-Hz Covariant Error to Improve Altimetric Profiles of σ0 and Sea Surface Height. IEEE Trans. Geosci. Remote Sens. 2019, 57, 3741–3752. [Google Scholar] [CrossRef]
- Passaro, M.; Cipollini, P.; Vignudelli, S.; Quartly, G.D.; Snaith, H.M. ALES: A multi-mission adaptive subwaveform retracker for coastal and open ocean altimetry. Remote Sens. Environ. 2014, 145, 173–189. [Google Scholar] [CrossRef] [Green Version]
- Passaro, M.; Fenoglio-Marc, L.; Cipollini, P. Validation of significant wave height from improved satellite altimetry in the german bight. IEEE Trans. Geosci. Remote Sens. 2015, 53, 2046–2156. [Google Scholar] [CrossRef]
- Birol, F.; Léger, F.; Passaro, M.; Cazenave, A.; Benveniste, J. The X-TRACK/ALES multi-mission processing system: New advances in altimetry towards the coast. Adv. Space Res. 2021, 67, 2398–2415. [Google Scholar] [CrossRef]
- Dettmering, D.; Müller, F.L.; Oelsmann, J.; Passaro, M.; Seitz, F. North SEAL: A new dataset of sea level changes in the North Sea from satellite altimetry. Earth Syst. Sci. Data 2021, 13, 3733–3753. [Google Scholar] [CrossRef]
- Arabsahebi, R.; Voosoghi, B.; Tourian, M.J. The Inflection-Point Retracking Algorithm: Improved Jason-2 Sea Surface Heights in the Strait of Hormuz. Mar. Geod. 2018, 41, 331–352. [Google Scholar] [CrossRef]
- Roscher, R.; Uebbing, B.; Kusche, J. STAR: Spatio-temporal altimeter waveform retracking using sparse representation and conditional random fields. Remote Sens. Environ. 2017, 201, 148–164. [Google Scholar] [CrossRef] [Green Version]
- Schlembach, F.; Passaro, M.; Quartly, G.D.; Kurekin, A.; Donlon, C. Round Robin Assessment of Radar Altimeter Low Resolution Mode and Delay-Doppler Retracking Algorithms for Significant Wave Height. Remote Sens. 2020, 12, 1254. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.; Ichikawa, K.; Wei, D. Coastal Waveform Retracking in the Slick-Rich Sulawesi Sea of Indonesia Based on Variable Footprint Size with Homogeneous Sea Surface Roughness. Remote Sens. 2019, 11, 1274. [Google Scholar] [CrossRef] [Green Version]
- Quartly, G.D. Determination of Oceanic Rain Rate and Rain Cell Structure from Altimeter Waveform Data. J. Atmos. Ocean. Technol. 1998, 15, 1361–1378. [Google Scholar] [CrossRef]
- Quartly, G.D. Hyperbolic Retracker: Removing Bright Target Artefacts from Altimetric Waveform Data. In ESA SP-686, Living Planet Symposium; ESA Publication: Bergen, Norway, 2010; SP-686. [Google Scholar]
- Tournadre, J. Signature of lighthouses, ships, and small islands in altimeter waveforms. J. Atmos. Ocean. Technol. 2007, 24, 1143–1149. [Google Scholar] [CrossRef]
- Wang, X.; Ichikawa, K. Coastal Waveform Retracking for Jason-2 Altimeter Data Based on Along-Track Echograms around the Tsushima Islands in Japan. Remote Sens. 2017, 9, 762. [Google Scholar] [CrossRef] [Green Version]
- Peng, F.; Deng, X. A new retracking technique for Brown peaky altimetric waveforms. Mar. Geod. 2017, 41, 99–125. [Google Scholar] [CrossRef]
- Peng, F.; Deng, X. Validation of Sentinel-3A SAR mode sea level anomalies around the Australian coastal region. Remote Sens. Environ. 2020, 237, 111548. [Google Scholar] [CrossRef]
- Deng, X.; Featherstone, W.E. A coastal retracking system for satellite radar altimeter waveforms: Application to ERS-2 around Australia. J. Geophys. Res. Ocean. 2006, 111, 95. [Google Scholar] [CrossRef]
- Yang, L.E.; Lin, M.; Liu, Q.; Pan, D. A coastal altimetry retracking strategy based on waveform classification and sub-waveform extraction. Int. J. Remote Sens. 2012, 33, 7806–7819. [Google Scholar] [CrossRef]
- Nurul, I.; Xiaoli, D.; Ami, M.D.; Nurul, I. CAWRES: A Waveform Retracking Fuzzy Expert System for Optimizing Coastal Sea Levels from Jason-1 and Jason-2 Satellite Altimetry Data. Remote Sens. 2017, 9, 603. [Google Scholar]
- Peng, F.; Deng, X.; Cheng, X. Quantifying the precision of retracked Jason-2 sea level data in the 0–5 km Australian coastal zone. Remote Sens. Environ. 2021, 263, 112539. [Google Scholar] [CrossRef]
- Jia, Y.; Yang, J.; Lin, M.; Zhang, Y.; Fan, C. Global Assessments of the HY-2B Measurements and Cross-Calibrations with Jason-3. Remote Sens. 2020, 12, 2470. [Google Scholar] [CrossRef]
- Amarouche, L.; Thibaut, P.; Zanife, O.Z.; Dumont, J.P.; Vincent, P.; Steunou, N. Improving the Jason-1 ground retracking to better account for attitude effects. Mar. Geod. 2004, 27, 171–197. [Google Scholar] [CrossRef]
- Poisson, J.C.; Ouartly, G.D.; Kurekin, A.A.; Thibaut, P.; Hoang, D.; Nencioli, F. Development of an ENVISAT altimetry processor providing sea level continuity between open ocean and Arctic leads. IEEE Trans. Geosci. Remote Sens. 2018, 56, 5299–5319. [Google Scholar] [CrossRef]
- Halimi, A.; Mailhes, C.; Tourneret, J.Y.; Thibaut, P.; Boy, F. Parameter estimation for peaky altimetric waveforms. IEEE Trans. Geosci. Remote Sens. 2013, 51, 1568–1577. [Google Scholar] [CrossRef]
- Peacock, N.R.; Laxon, S.W. Sea surface height determination in the Arctic ocean from ERS altimetry. J. Geophys. Res. Ocean. 2004, 109, 120. [Google Scholar] [CrossRef]
- Chelton, D.B.; Walsh, E.J.; Macarthur, J.L. Pulse compression and sea level tracking in satellite altimetry. J. Atmos. Ocean. Technol. 1989, 6, 407–438. [Google Scholar] [CrossRef]
- Cipollini, P.; Calafat, F.M.; Jevrejeva, S.; Melet, A.; Prandi, P. Monitoring Sea Level in the Coastal Zone with Satellite Altimetry and Tide Gauges. Surv. Geophys. 2017, 38, 33–57. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Passaro, M.; Nadzir, Z.A.; Quartly, G.D. Improving the precision of sea level data from satellite altimetry with high-frequency and regional sea state bias corrections. Remote Sens. Environ. 2018, 218, 245–254. [Google Scholar] [CrossRef]
- Peng, F.; Deng, X. Improving precision of high-rate altimeter sea level anomalies by removing the sea state bias and intra-1-Hz covariant error. Remote Sens. Environ. 2020, 251, 112081. [Google Scholar] [CrossRef]
- Fenoglio-Marc, L.; Fehlau, M.; Ferri, L.; Becker, M.; Bao, Y.; Vignudelli, S. Coastal sea surface heights from improved altimeter data in the Mediterranean Sea. Gravity Geoid Earth Obs. 2010, 135, 253–261. [Google Scholar]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hong, Z.; Yang, J.; Liu, S.; Jia, Y.; Fan, C.; Cui, W. Coastal Waveform Retracking for HY-2B Altimeter Data by Determining the Effective Trailing Edge and the Low Noise Leading Edge. Remote Sens. 2022, 14, 5026. https://doi.org/10.3390/rs14195026
Hong Z, Yang J, Liu S, Jia Y, Fan C, Cui W. Coastal Waveform Retracking for HY-2B Altimeter Data by Determining the Effective Trailing Edge and the Low Noise Leading Edge. Remote Sensing. 2022; 14(19):5026. https://doi.org/10.3390/rs14195026
Chicago/Turabian StyleHong, Zhiheng, Jungang Yang, Shanwei Liu, Yongjun Jia, Chenqing Fan, and Wei Cui. 2022. "Coastal Waveform Retracking for HY-2B Altimeter Data by Determining the Effective Trailing Edge and the Low Noise Leading Edge" Remote Sensing 14, no. 19: 5026. https://doi.org/10.3390/rs14195026
APA StyleHong, Z., Yang, J., Liu, S., Jia, Y., Fan, C., & Cui, W. (2022). Coastal Waveform Retracking for HY-2B Altimeter Data by Determining the Effective Trailing Edge and the Low Noise Leading Edge. Remote Sensing, 14(19), 5026. https://doi.org/10.3390/rs14195026