Adaptive DDK Filter for GRACE Time-Variable Gravity Field with a Novel Anisotropic Filtering Strength Metric
Abstract
1. Introduction
2. Materials and Methods
2.1. Regularization Strategies
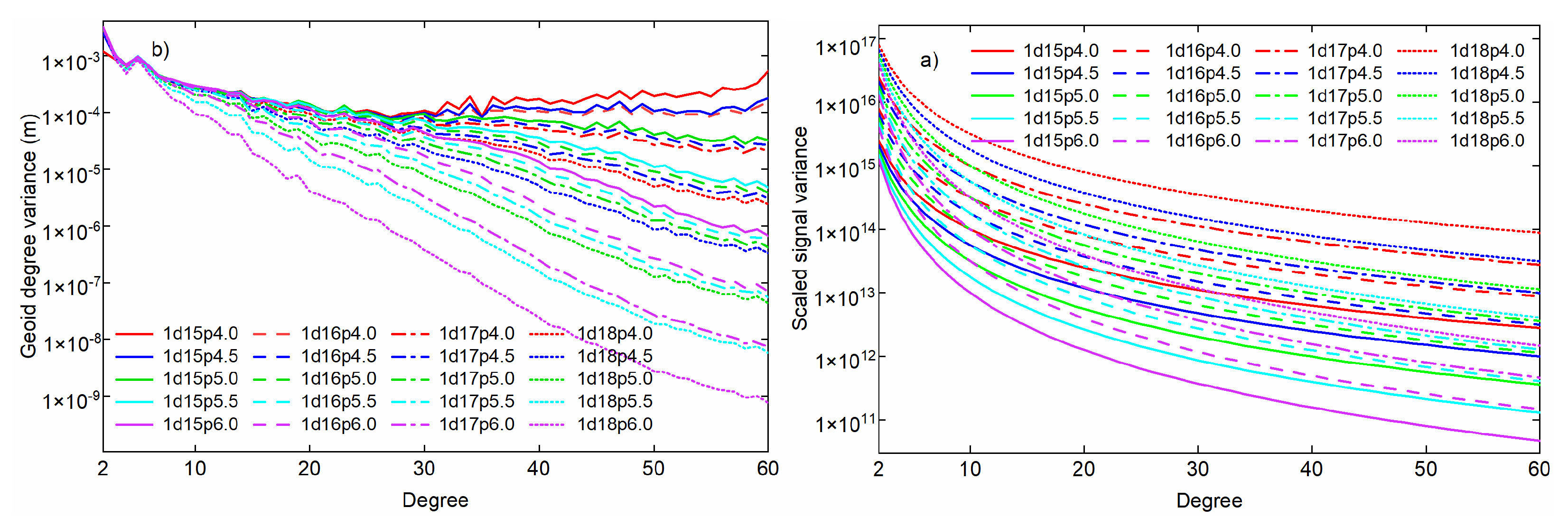
2.2. Variance and Covariance Analysis
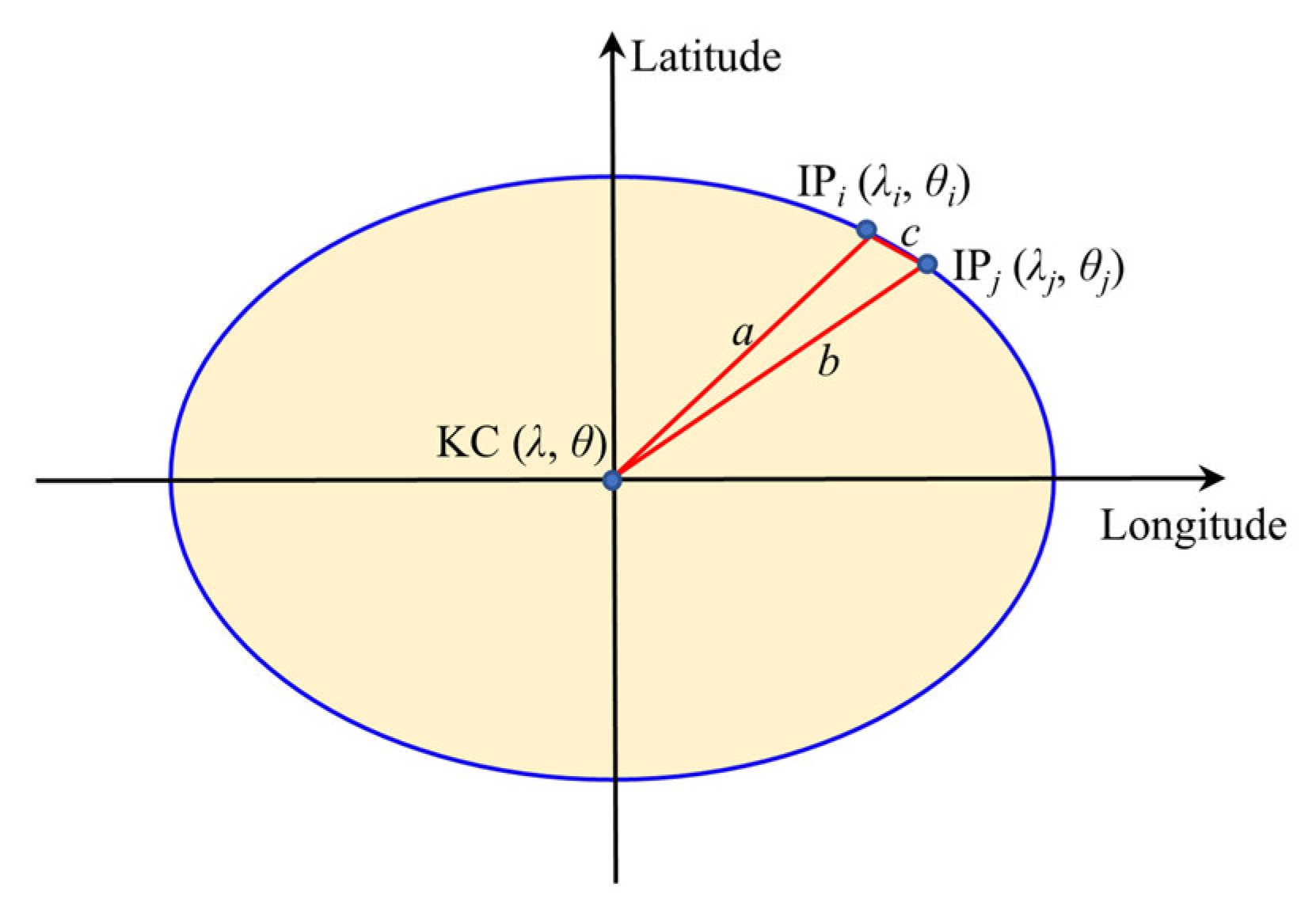
2.3. A Novel Filtering Strength Metric: Half-Weight Polygon Area of the Smoothing Kernel
3. Results
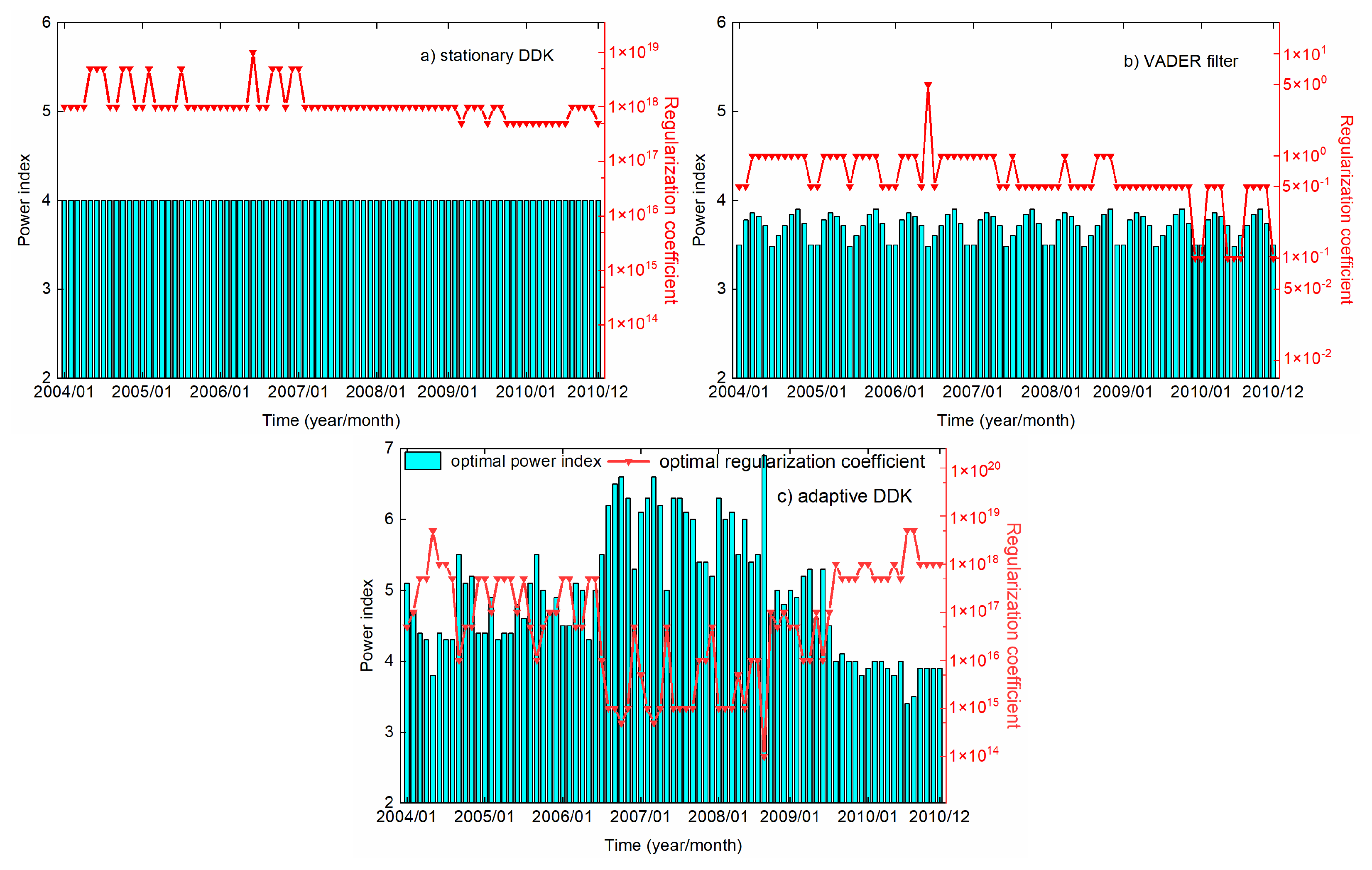
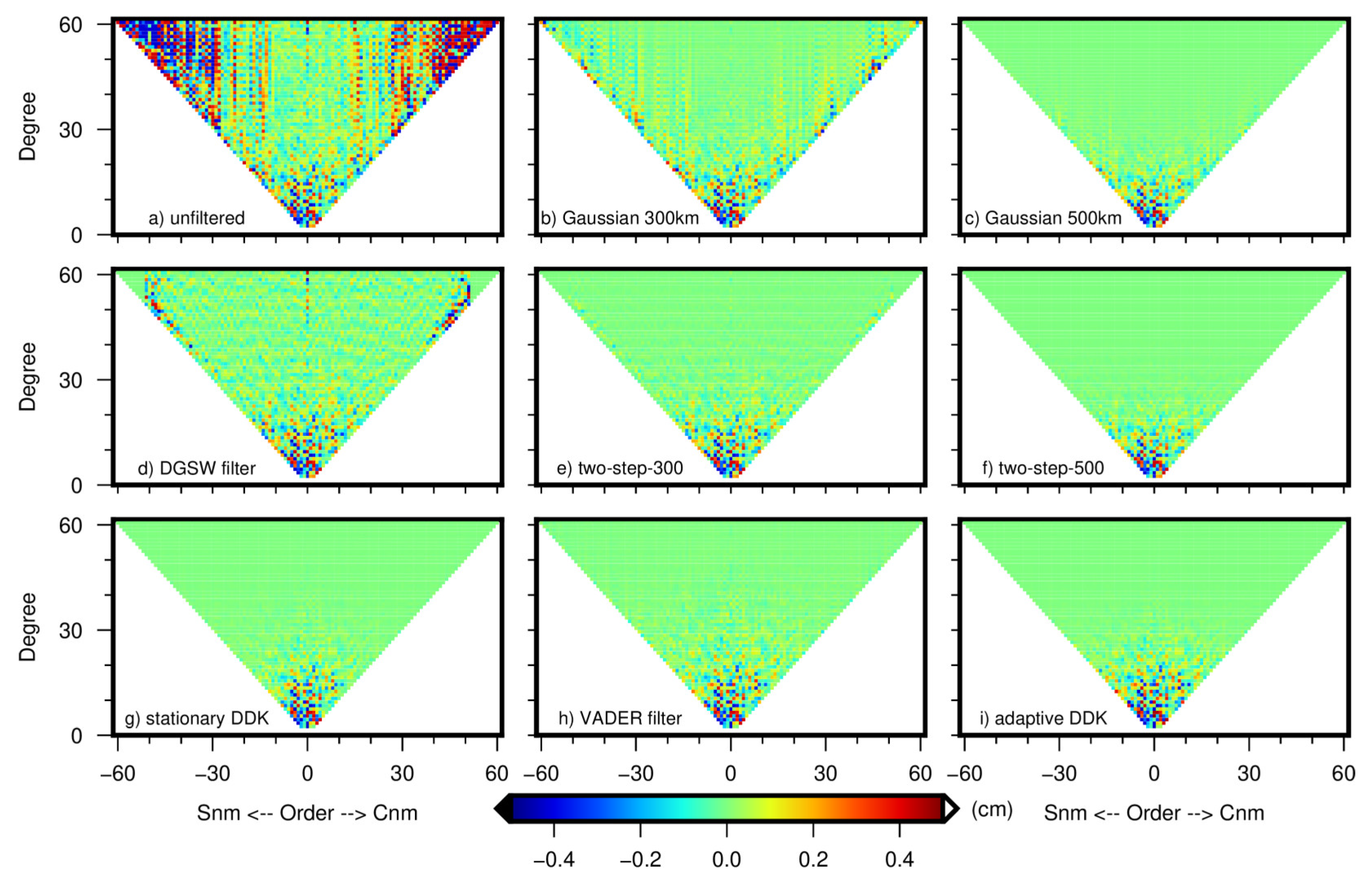
3.1. Analysis of Properties of Adaptive DDK Filter
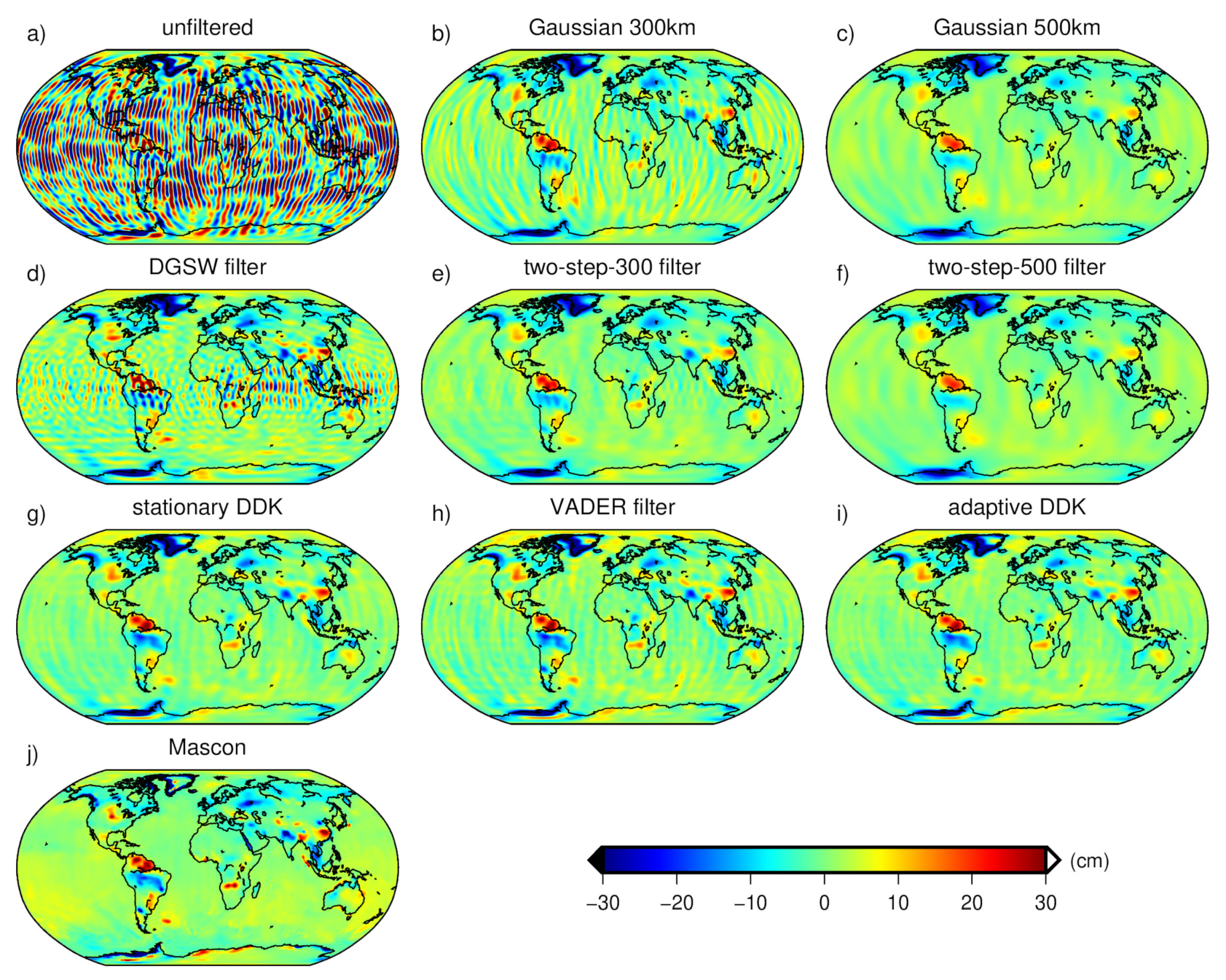
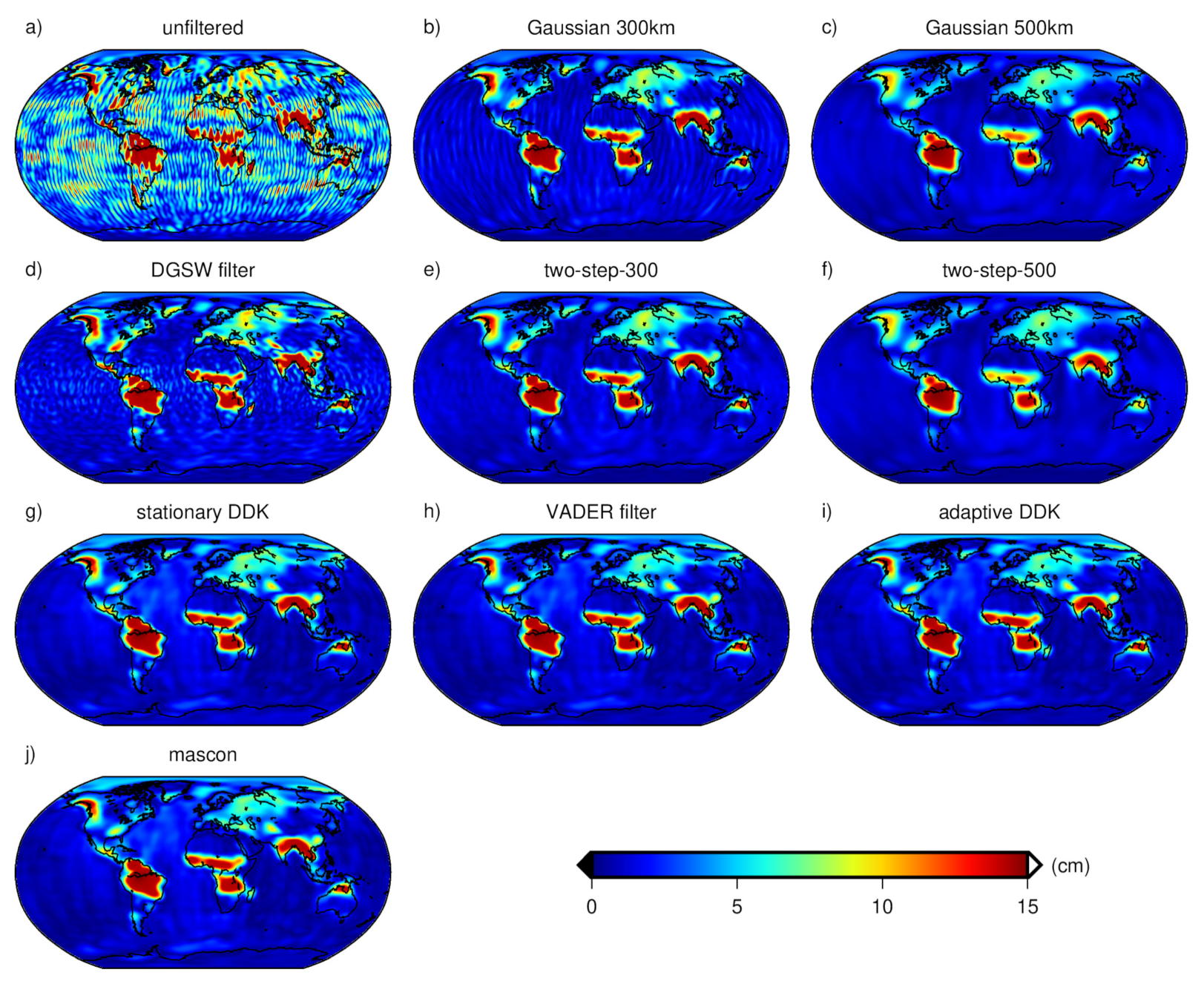
3.2. Evaluation of Filtered Mass Anomaly
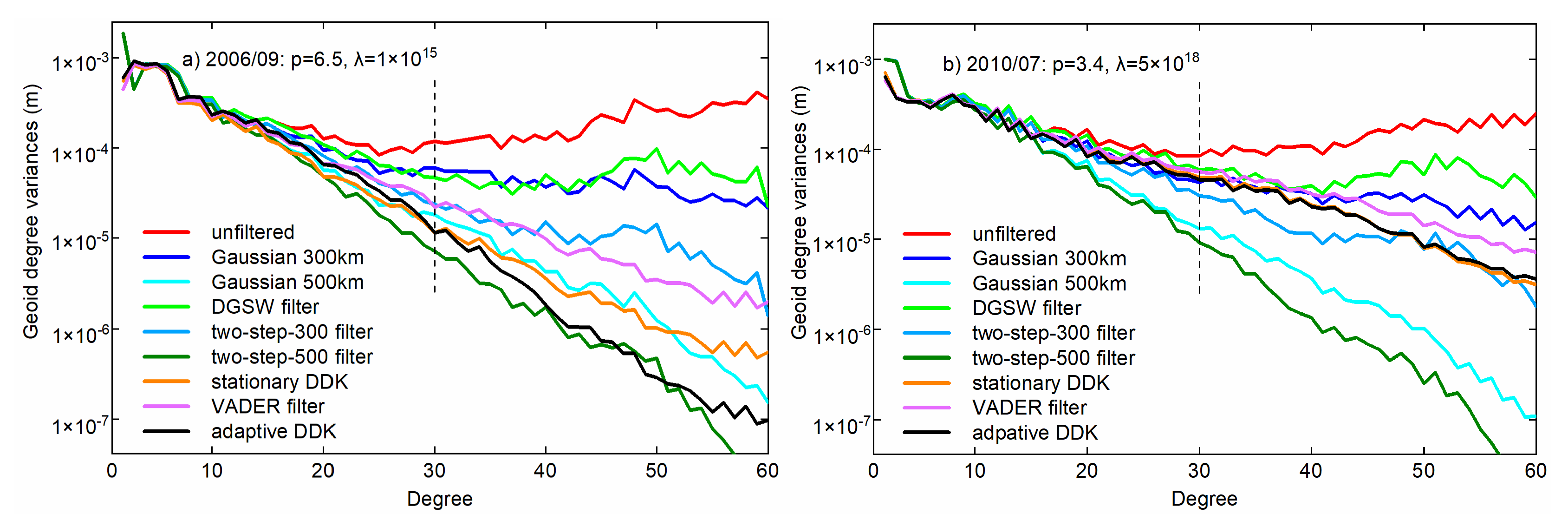
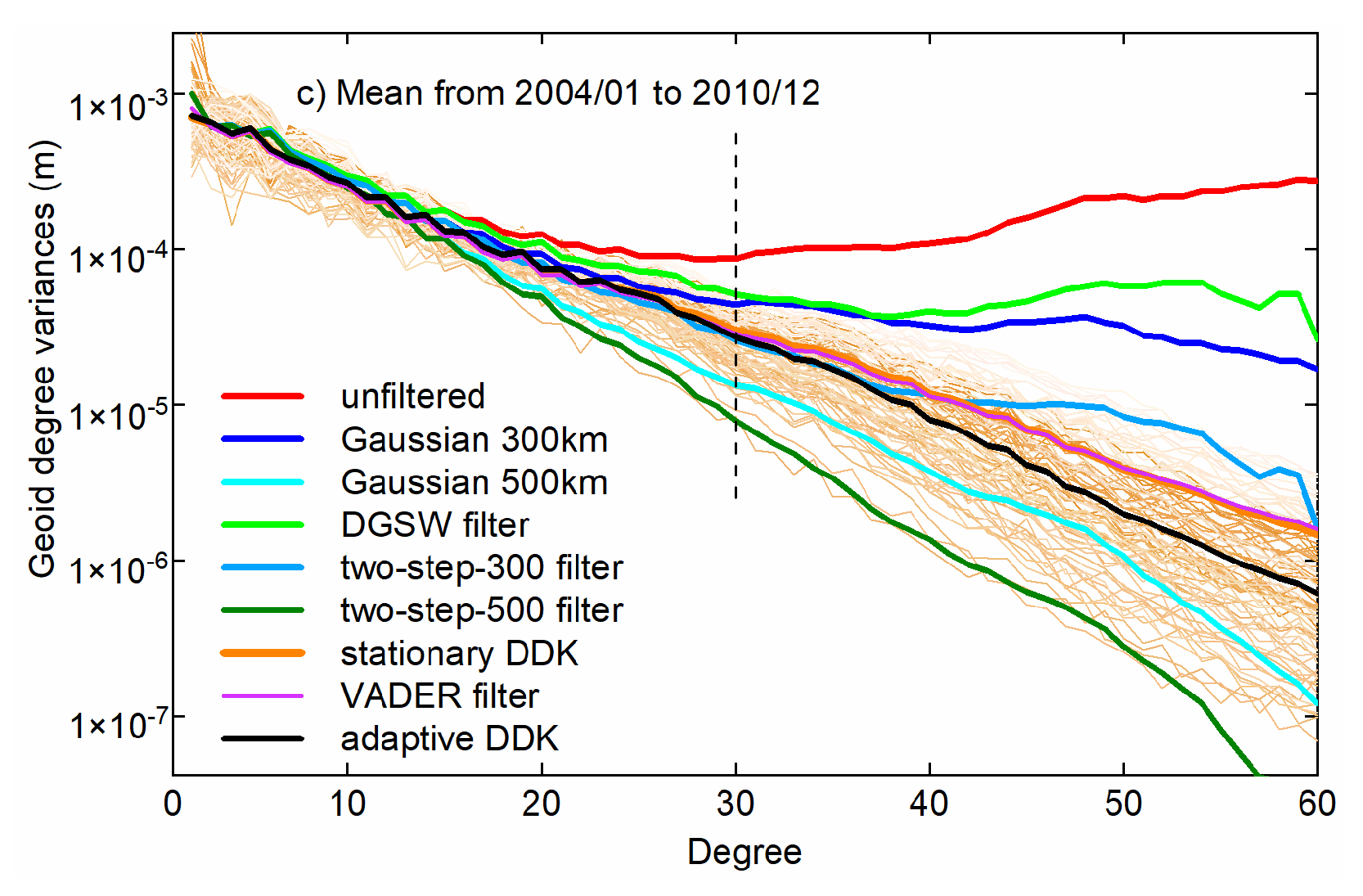
3.3. Analysis of Signal and Noise Level
4. Discussion
5. Conclusions
- (1)
- Both the regularization coefficient and the power index can be used to adjust the signal variances. Although the increase of the regularization coefficient and the power index results in a stronger smoothing strength, the mechanisms of the two parameters are different. The regularization coefficient controls the signal variances of all degrees, while the power index regulates the signal variances between different degrees. In other words, the former is global regulation, whereas the latter is local regulation. By tuning the two parameters according to the GCV criterion, the adaptability to the data and the optimality of the filtering results can be significantly enhanced;
- (2)
- Compared with the equivalent smoothing radius in [11], the proposed half-weight kernel polygon area is proved to have a more significant distinguishing ability, especially when the filtering strength is too weak and close, or the filtering strength is too strong;
- (3)
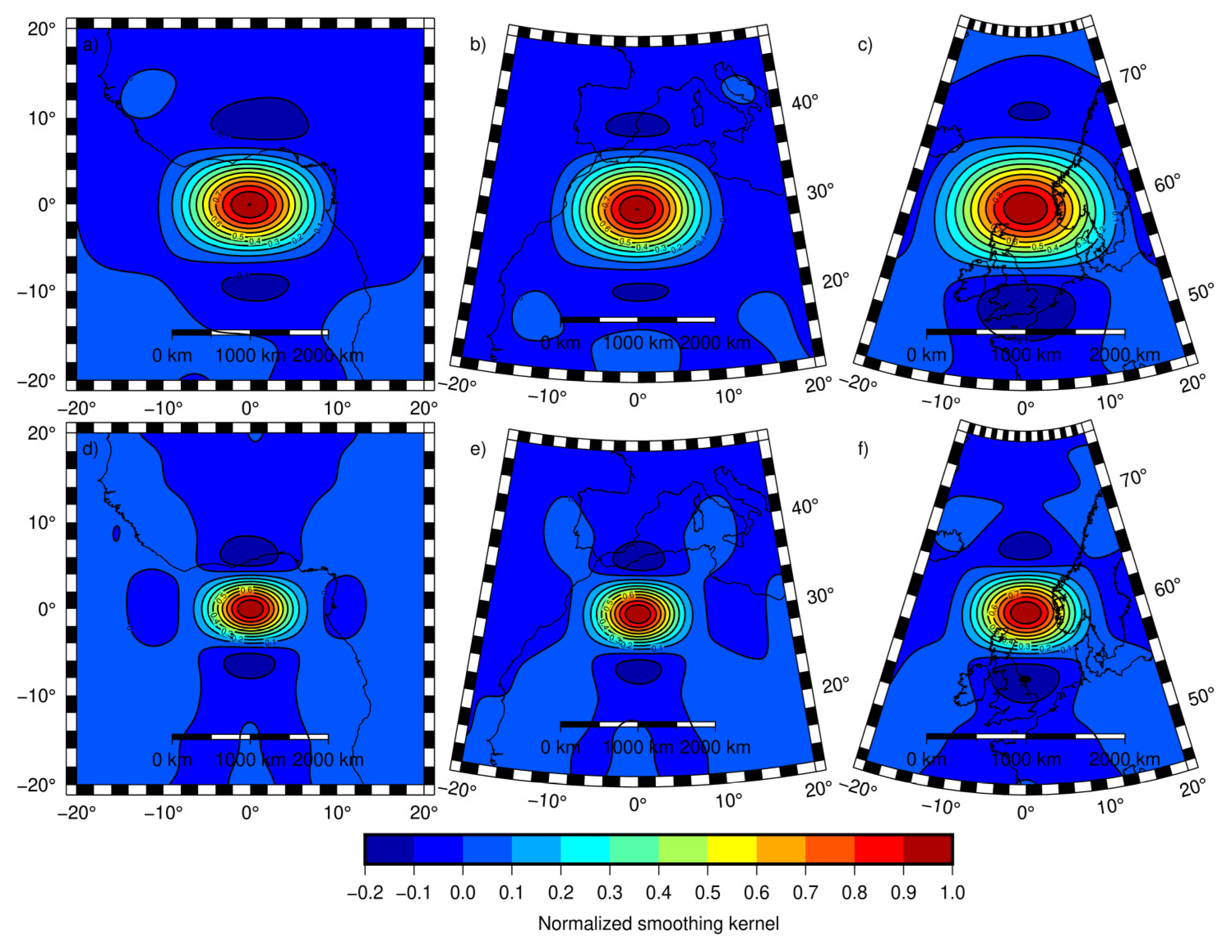
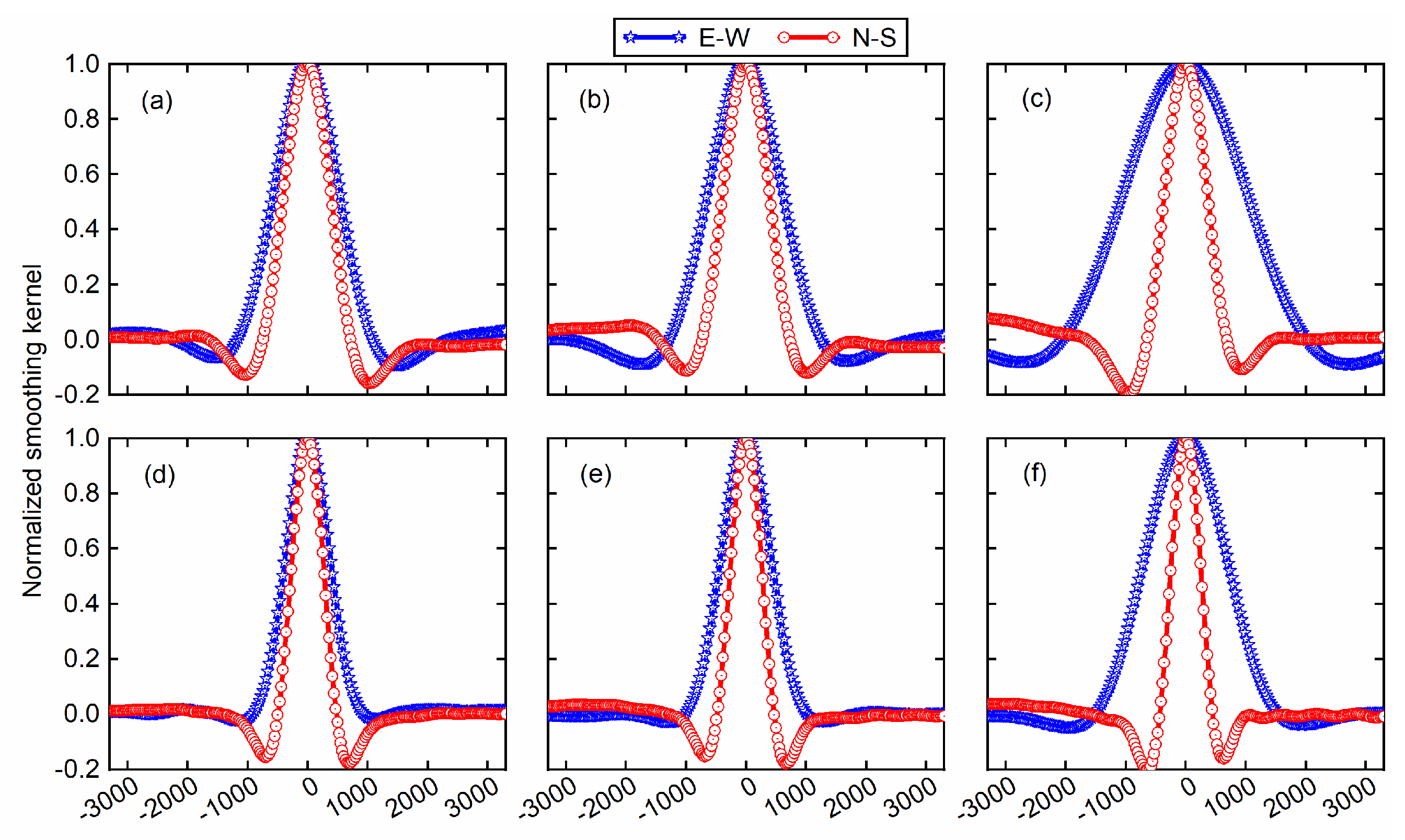
- The need for filtering is mainly split up in an along- and cross-track direction, based on orbit inclination, orbit height, and ground track spacing. The authors think that filtering with only four cardinal directions taken into account is a simplification in itself, as shown in Figure 5a and Figure 6a. Are the stripes strictly distributed north–south? The answer is, not necessarily. The causes include but are not limited to: (1) the de-aliasing error caused by the incorrectness of the models of tidal and atmospheric ocean non-tidal variation [30]; (2) the shortcomings of instrument accuracy and existing GRACE data processing methods [9,13]. This raises the necessity that the filter can be designed to take into account directions other than the cardinal ones. The anisotropic DDK filter is exactly such a filter that takes into account all directions including the cardinal directions. As shown in Figure 14, the filter kernel is not distributed strictly according to north-south and west-east directions, and the filter kernel can be approximately rectangular/elliptical, or wide/narrow. This indicates: (1) in the conventional approach, the algebraic averages of half-weight smoothing radii in four main directions (east, west, north, and south) are not accurate enough to measure the filtering strength; (2) the proposed HWPA, taking all directions into account, can reasonably measure the filtering strength, regardless of the shape and scope of the kernel. In the future, the research will be focused on optimizing the azimuth-anisotropic HWPA to make it more reasonable to measure the smoothing strength of the location-inhomogeneous filter.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yi, S.; Sneeuw, N. A novel spatial filter to reduce north–south striping noise in GRACE spherical harmonic coefficients. J. Geod. 2022, 96, 1–17. [Google Scholar] [CrossRef]
- Guo, J.Y.; Li, W.D.; Chang, X.T.; Zhu, G.B.; Liu, X.; Guo, B. Terrestrial water storage changes over Xinjiang extracted by combining Gaussian filter and multichannel singular spectrum analysis from GRACE. Geophys. J. Int. 2018, 213, 397–407. [Google Scholar] [CrossRef]
- Xiang, L.; Wang, H.; Steffen, H.; Qiao, B.; Feng, W.; Jia, L.; Gao, P. Determination of Weak Terrestrial Water Storage Changes from GRACE in the Interior of the Tibetan Plateau. Remote Sens. 2022, 14, 544. [Google Scholar] [CrossRef]
- Swenson, S.; Wahr, J. Post-processing removal of correlated errors in GRACE data. Geophys. Res. Lett. 2006, 33, 8. [Google Scholar] [CrossRef]
- Duan, X.J.; Guo, J.Y.; Shum, C.K.; van der Wal, W. On the postprocessing removal of correlated errors in GRACE temporal gravity field solutions. J. Geod. 2009, 83, 1095–1106. [Google Scholar] [CrossRef]
- Belda, S.; Garcia-Garcia, D.; Ferrandiz, J.M. On the decorrelation filtering of RL05 GRACE data for global applications. Geophy. J. Int. 2015, 200, 173–184. [Google Scholar] [CrossRef][Green Version]
- Jekeli, C. Alternative Methods to Smooth the Earth’s Gravity Field; Ohio State University: Columbus, OH, USA, 1981. [Google Scholar]
- Wahr, J.; Molenaar, M.; Bryan, F. Time variability of the Earth’s gravity field: Hydrological and oceanic effects and their possible detection using GRACE. J. Geophy. Res. Solid Earth 1998, 103, 30205–30229. [Google Scholar] [CrossRef]
- Kusche, J. Approximate decorrelation and non-isotropic smoothing of time-variable GRACE-type gravity field models. J. Geod. 2007, 81, 733–749. [Google Scholar] [CrossRef]
- Chang, G.; Qian, N.; Chen, C.; Gao, J. Precise instantaneous velocimetry and accelerometry with a stand-alone GNSS receiver based on sparse kernel learning. Measurement 2020, 159, 107803. [Google Scholar] [CrossRef]
- Kusche, J.; Schmidt, R.; Petrovic, S.; Rietbroek, R. Decorrelated GRACE time-variable gravity solutions by GFZ, and their validation using a hydrological model. J. Geod. 2009, 83, 903–913. [Google Scholar] [CrossRef]
- Horvath, A.; Murböck, M.; Pail, R.; Horwath, M. Decorrelation of GRACE time variable gravity field solutions using full covariance information. Geosciences 2018, 8, 323. [Google Scholar] [CrossRef]
- Klees, R.; Revtova, E.; Gunter, B.; Ditmar, P.; Oudman, E.; Winsemius, H.; Savenije, H. The design of an optimal filter for monthly GRACE gravity models. Geophy. J. Int. 2008, 175, 417–432. [Google Scholar] [CrossRef]
- Kaula, W.M. The investigation of the gravitational fields of the moon and planets with artificial satellites. Advan. Space Sci. Technol. 1963, 5, 210–230. [Google Scholar]
- Xu, P.; Fukuda, Y.; Liu, Y. Multiple parameter regularization: Numerical solutions and applications to the determination of geopotential from precise satellite orbits. J. Geod. 2006, 80, 17–27. [Google Scholar] [CrossRef]
- Xu, P.; Rummel, R. Generalized ridge regression with applications in determination of potential fields. Manuscr. Geod. 1994, 20, 8–20. [Google Scholar]
- Golub, G.H.; Heath, M.; Technometrics, G.W.J. Generalized cross-validation as a method for choosing a good ridge parameter. Technometrics 1979, 21, 215–223. [Google Scholar] [CrossRef]
- Qian, N.; Chang, G.; Gao, J.; Pan, C.; Yang, L.; Li, F.; Yu, H.; Bu, J. Vehicle’s Instantaneous Velocity Reconstruction by Combining GNSS Doppler and Carrier Phase Measurements Through Tikhonov Regularized Kernel Learning. IEEE Trans. Veh. Technol. 2021, 70, 4190–4202. [Google Scholar] [CrossRef]
- Qian, N.; Chang, G.; Ditmar, P.; Gao, J.; Wei, Z. Sparse DDK: A Data-Driven Decorrelation Filter for GRACE Level-2 Products. Remote Sens. 2022, 14, 2810. [Google Scholar] [CrossRef]
- Ditmar, P. Conversion of time-varying Stokes coefficients into mass anomalies at the Earth’s surface considering the Earth’s oblateness. J. Geod. 2018, 92, 1401–1412. [Google Scholar] [CrossRef]
- Pu, L.; Fan, D.; You, W.; Yang, X.; Nigatu, Z.M.; Jiang, Z. Extracting terrestrial water storage signals from GRACE solutions in the Amazon Basin using an iterative filtering approach. Remote Sens. Lett. 2022, 13, 14–23. [Google Scholar] [CrossRef]
- Koch, K.R.; Kusche, J. Regularization of geopotential determination from satellite data by variance components. J. Geod. 2002, 76, 259–268. [Google Scholar] [CrossRef]
- Kusche, J. A Monte-Carlo technique for weight estimation in satellite geodesy. J. Geod. 2003, 76, 641–652. [Google Scholar] [CrossRef]
- Qian, N.; Chang, G. Optimal filtering for state space model with time-integral measurements. Measurement 2021, 176, 109209. [Google Scholar] [CrossRef]
- Kleinherenbrink, M.; Riva, R.; Sun, Y. Sub-basin-scale sea level budgets from satellite altimetry, Argo floats and satellite gravimetry: A case study in the North Atlantic Ocean. Ocean. Sci. 2016, 12, 1179–1203. [Google Scholar] [CrossRef]
- Zhang, X.; Li, J.; Dong, Q.; Wang, Z.; Zhang, H.; Liu, X. Bridging the gap between GRACE and GRACE-FO using a hydrological model. Sci. Total Environ. 2022, 822, 153659. [Google Scholar] [CrossRef] [PubMed]
- Luthcke, S.B.; Rowlands, D.D.; Lemoine, F.G.; Klosko, S.M.; Chinn, D.; McCarthy, J.J. Monthly spherical harmonic gravity field solutions determined from GRACE inter-satellite range-rate data alone. Geophys. Res. Lett. 2006, 33, 2402. [Google Scholar] [CrossRef]
- Chen, Q.; Shen, Y.; Chen, W.; Zhang, X.; Hsu, H. An improved GRACE monthly gravity field solution by modeling the non-conservative acceleration and attitude observation errors. J. Geod. 2016, 90, 503–523. [Google Scholar] [CrossRef]
- Chen, J.L.; Wilson, C.R.; Blankenship, D.; Tapley, B.D. Accelerated Antarctic ice loss from satellite gravity measurements. Nat. Geosci. 2009, 2, 859–862. [Google Scholar] [CrossRef]
- Kurtenbach, E.; Mayer-Guerr, T.; Eicker, A. Deriving daily snapshots of the Earth’s gravity field from GRACE L1B data using Kalman filtering. Geophys. Res. Lett. 2009, 36, 17102. [Google Scholar] [CrossRef]
- Feng, W. GRAMAT: A comprehensive Matlab toolbox for estimating global mass variations from GRACE satellite data. Earth Sci. Inform. 2019, 12, 389–404. [Google Scholar] [CrossRef]
- Wessel, P.; Smith, W.H.; Scharroo, R.; Luis, J.; Wobbe, F. Generic mapping tools: Improved version released. Eos Trans. Am. Geophys. Union 2013, 94, 409–410. [Google Scholar] [CrossRef]





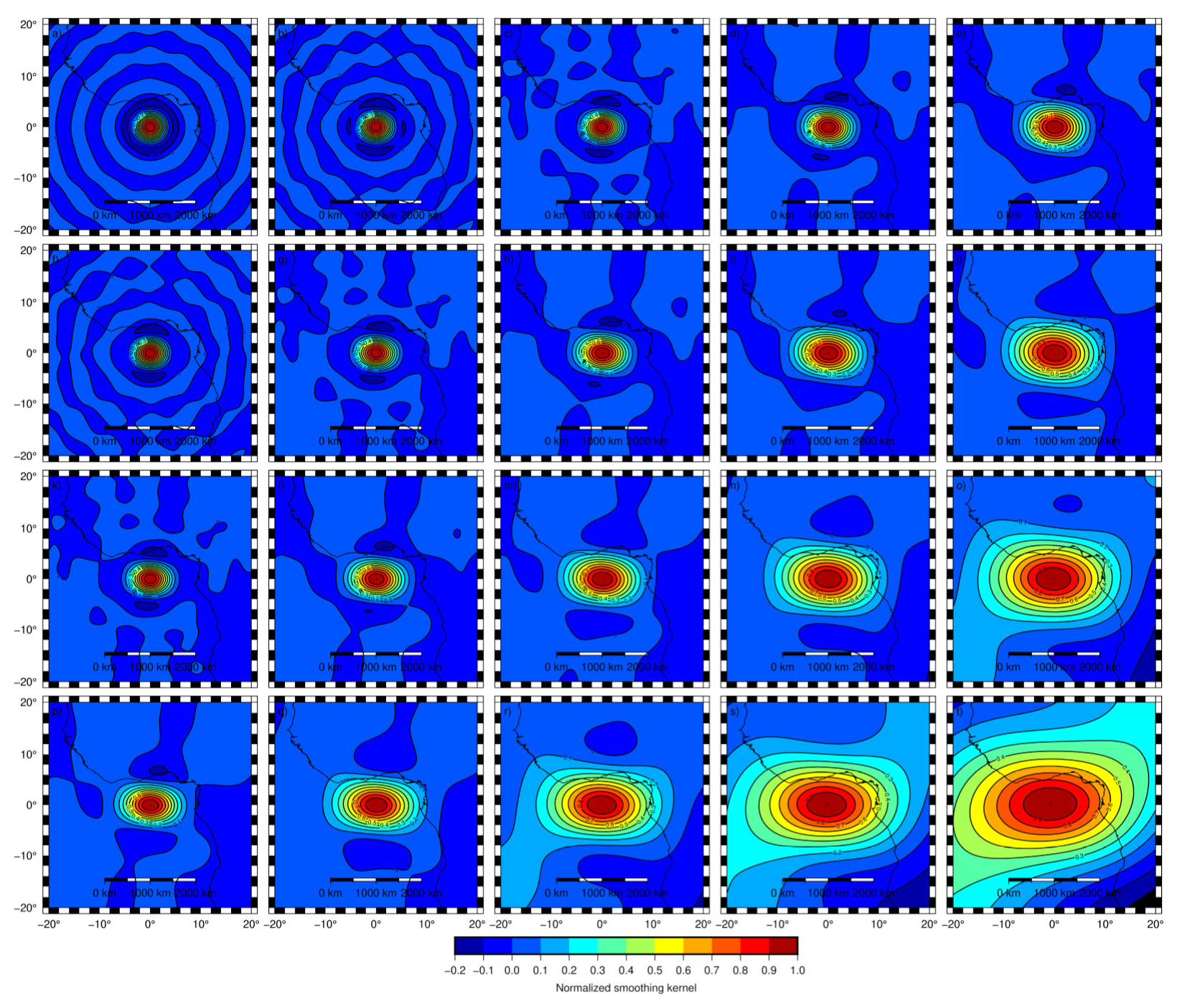

| DDK | HWPA (0°, 0°) | HWPA (0°, 30°) | HWPA (0°, 60°) | Average HWPA | ESR |
|---|---|---|---|---|---|
| DDK1 | 1613 | 7990 | 22,853 | 10,819 | 536 |
| DDK2 | 808 | 2657 | 6244 | 3236 | 347 |
| DDK3 | 414 | 916 | 2281 | 1204 | 242 |
| DDK4 | 362 | 855 | 1811 | 1009 | 219 |
| DDK5 | 255 | 513 | 1035 | 601 | 183 |
| DDK6 | 245 | 414 | 854 | 504 | 172 |
| DDK7 | 190 | 286 | 739 | 405 | 149 |
| DDK8 | 194 | 246 | 700 | 380 | 149 |
| Filtered Solutions | September 2006 | July 2010 | ||||
|---|---|---|---|---|---|---|
| Max | Min | RMS | Max | Min | RMS | |
| Gaussian 300 | 36.8 | −50.0 | 5.8 | 32.2 | −69.5 | 6.4 |
| Gaussian 500 | 24.5 | −33.9 | 4.1 | 21.9 | −39.7 | 5.0 |
| DGSW filter | 66.9 | −66.0 | 6.8 | 64.7 | −89.6 | 8.1 |
| two-step-300 | 28.3 | −40.9 | 4.7 | 30.8 | −60.2 | 5.9 |
| two-step-500 | 23.1 | −31.0 | 4.0 | 21.3 | −38.8 | 4.9 |
| stationary DDK | 25.2 | −33.7 | 4.2 | 32.9 | −100.2 | 6.9 |
| VADER filter | 30.8 | −40.2 | 4.4 | 26.0 | −105.2 | 6.6 |
| adaptive DDK | 31.0 | −40.0 | 4.8 | 30.6 | −98.4 | 6.7 |
| Mascon | 143.6 | −137.0 | 6.3 | 165.8 | −345.3 | 11.8 |
| Filtered Solutions | Congo | Ganges | Rhein | Hai |
|---|---|---|---|---|
| Gaussian 300 km | 46.4 | 28.1 | 7.4 | 11.0 |
| Gaussian 500 km | 51.4 | 39.3 | 6.9 | 12.1 |
| DGSW filter | 46.4 | 26.7 | 5.9 | 9.0 |
| two-step-300 | 46.5 | 31.5 | 5.9 | 9.6 |
| two-step-500 | 52.4 | 42.7 | 6.9 | 12.2 |
| Stationary DDK | 49.1 | 26.8 | 5.5 | 9.5 |
| VADER filter | 63.1 | 28.6 | 5.5 | 9.7 |
| adaptive DDK | 46.0 | 26.7 | 5.3 | 9.4 |
| Filter | Indicator | Congo | Ganges | Rhein | Hai |
|---|---|---|---|---|---|
| Gaussian 300 km | AAMP | 12.1 | 12.8 | 5.1 | 1.9 |
| RSTD | 5.2 | 5.3 | 3.7 | 4.0 | |
| Gaussian 500 km | AAMP | 10.2 | 11.4 | 4.1 | 1.0 |
| RSTD | 3.1 | 3.1 | 1.8 | 1.9 | |
| DGSW filter | AAMP | 13.7 | 13.8 | 5.2 | 3.7 |
| RSTD | 9.1 | 6.3 | 3.2 | 3.8 | |
| two-step-300 | AAMP | 12.0 | 12.5 | 4.8 | 2.1 |
| RSTD | 4.0 | 3.7 | 2.2 | 2.7 | |
| two-step-500 | AAMP | 10.1 | 11.1 | 4.1 | 1.2 |
| RSTD | 3.1 | 2.9 | 2.0 | 1.6 | |
| Stationary DDK | AAMP | 12.5 | 13.0 | 4.2 | 1.5 |
| RSTD | 3.7 | 4.1 | 2.4 | 2.2 | |
| VADER filter | AAMP | 12.3 | 12.7 | 4.1 | 1.5 |
| RSTD | 3.8 | 4.0 | 2.4 | 2.2 | |
| adaptive DDK | AAMP | 12.9 | 13.4 | 4.3 | 1.6 |
| RSTD | 3.8 | 4.1 | 2.4 | 2.2 | |
| mascon | AAMP | 13.1 | 13.4 | 6.8 | 1.8 |
| RSTD | 3.5 | 6.1 | 2.2 | 3.3 |
| Indicator | Reg.\Pow. | 4.0 | 4.5 | 5.0 | 5.5 | 6.0 |
|---|---|---|---|---|---|---|
| ESR | 1 × 1015 | 149 | 149 | 175 | 219 | 281 |
| 1 × 1016 | 149 | 183 | 236 | 300 | 393 | |
| 1 × 1017 | 190 | 249 | 334 | 453 | 570 | |
| 1 × 1018 | 272 | 393 | 570 | 570 | 570 | |
| HWPA | 1 × 1015 | 364 | 369 | 603 | 920 | 1998 |
| 1 × 1016 | 442 | 573 | 1122 | 2467 | 5332 | |
| 1 × 1017 | 656 | 1367 | 3199 | 7163 | 16,824 | |
| 1 × 1018 | 1500 | 3714 | 9555 | 24,232 | 56,903 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qian, N.; Chang, G.; Gao, J.; Shen, W.; Yan, Z. Adaptive DDK Filter for GRACE Time-Variable Gravity Field with a Novel Anisotropic Filtering Strength Metric. Remote Sens. 2022, 14, 3114. https://doi.org/10.3390/rs14133114
Qian N, Chang G, Gao J, Shen W, Yan Z. Adaptive DDK Filter for GRACE Time-Variable Gravity Field with a Novel Anisotropic Filtering Strength Metric. Remote Sensing. 2022; 14(13):3114. https://doi.org/10.3390/rs14133114
Chicago/Turabian StyleQian, Nijia, Guobin Chang, Jingxiang Gao, Wenbin Shen, and Zhengwen Yan. 2022. "Adaptive DDK Filter for GRACE Time-Variable Gravity Field with a Novel Anisotropic Filtering Strength Metric" Remote Sensing 14, no. 13: 3114. https://doi.org/10.3390/rs14133114
APA StyleQian, N., Chang, G., Gao, J., Shen, W., & Yan, Z. (2022). Adaptive DDK Filter for GRACE Time-Variable Gravity Field with a Novel Anisotropic Filtering Strength Metric. Remote Sensing, 14(13), 3114. https://doi.org/10.3390/rs14133114







