Modeling and Analysis of RFI Impacts on Imaging between Geosynchronous SAR and Low Earth Orbit SAR
Abstract
:1. Introduction
2. Accurate Modeling of SINR in the Presence of RFI
2.1. RFI Impact Quantitative Analysis Model
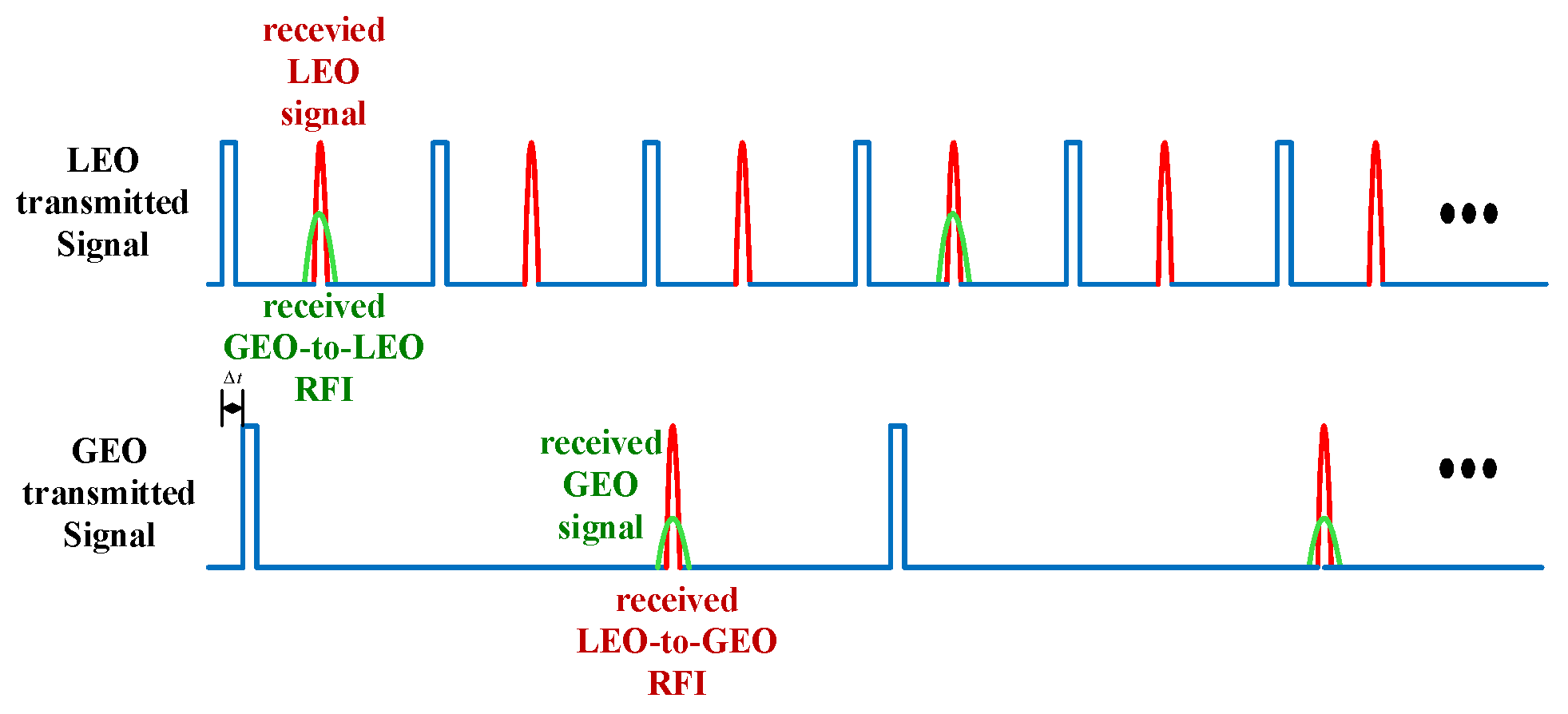
2.2. Times Series of GEO SARs and LEO SARs
2.3. Gains in Mismatched Filtering
2.4. RFI Power Affecting the Pixel after SAR Processing
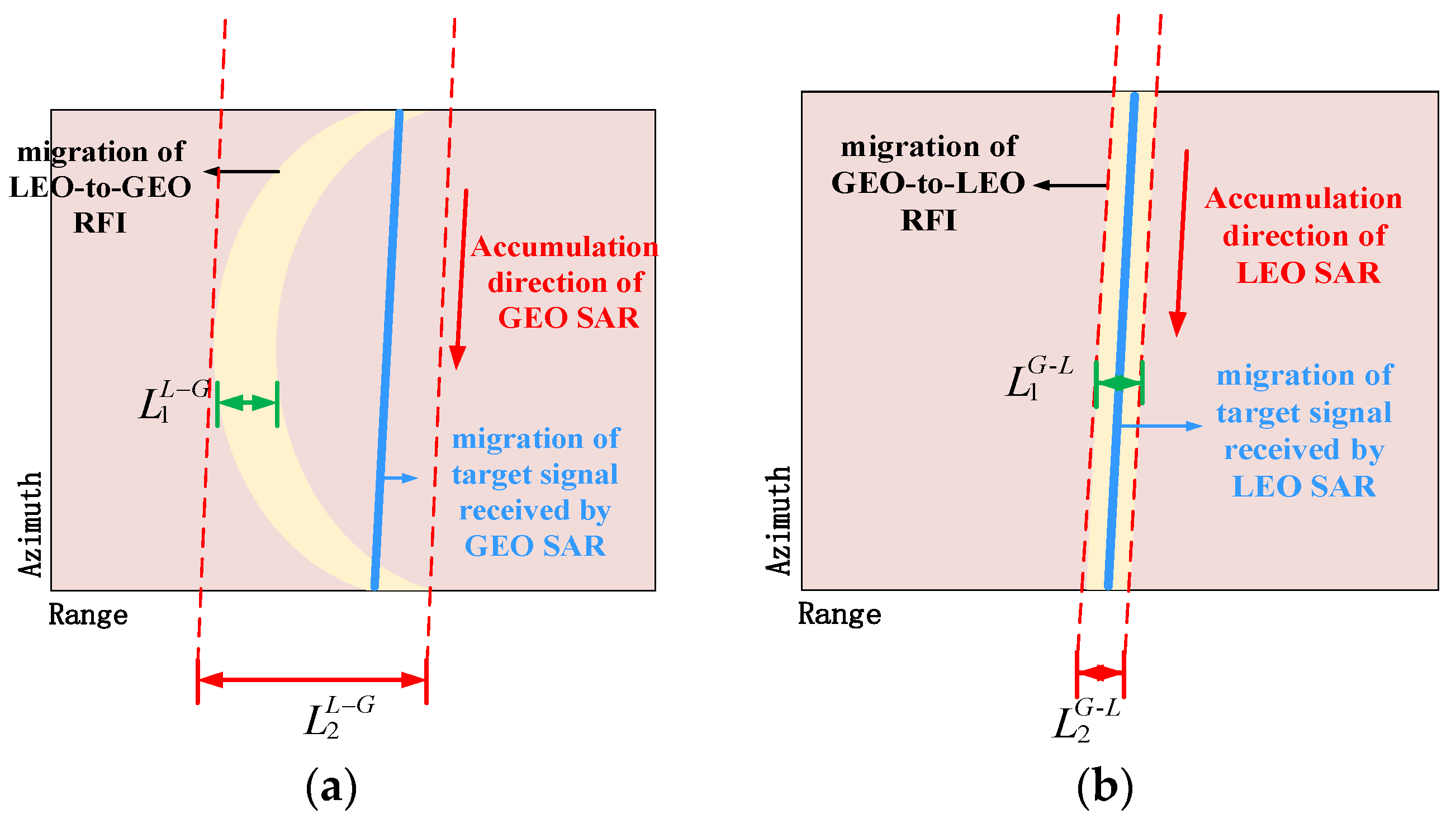
2.4.1. GEO-TO-LEO RFI
2.4.2. LEO-TO-GEO RFI
2.4.3. Discussion on GEO-TO-LEO RFI and LEO-TO-GEO RFI
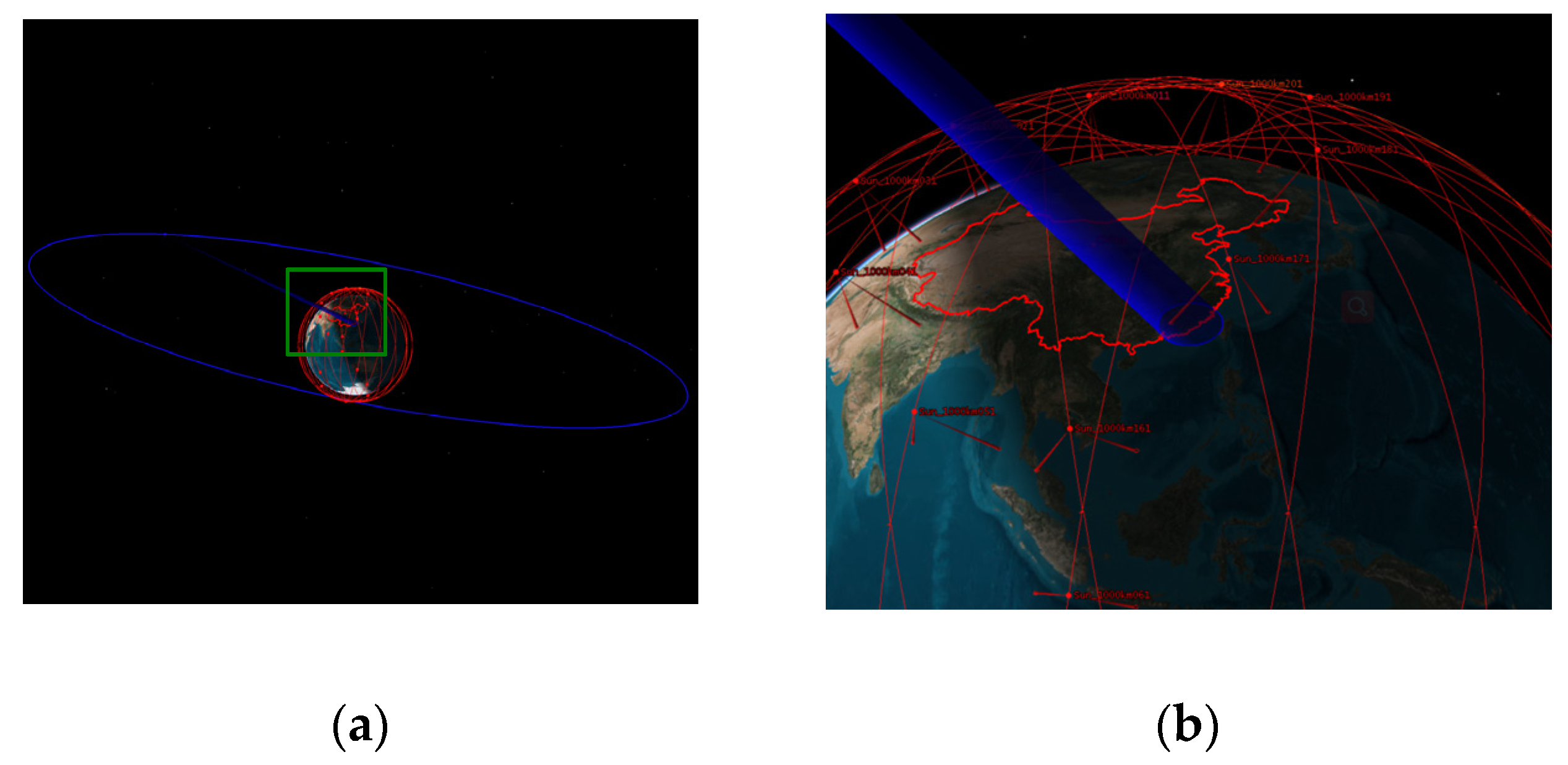
3. Numerical Verification
3.1. Verification of the SINR Expressions in the Presence of RFI
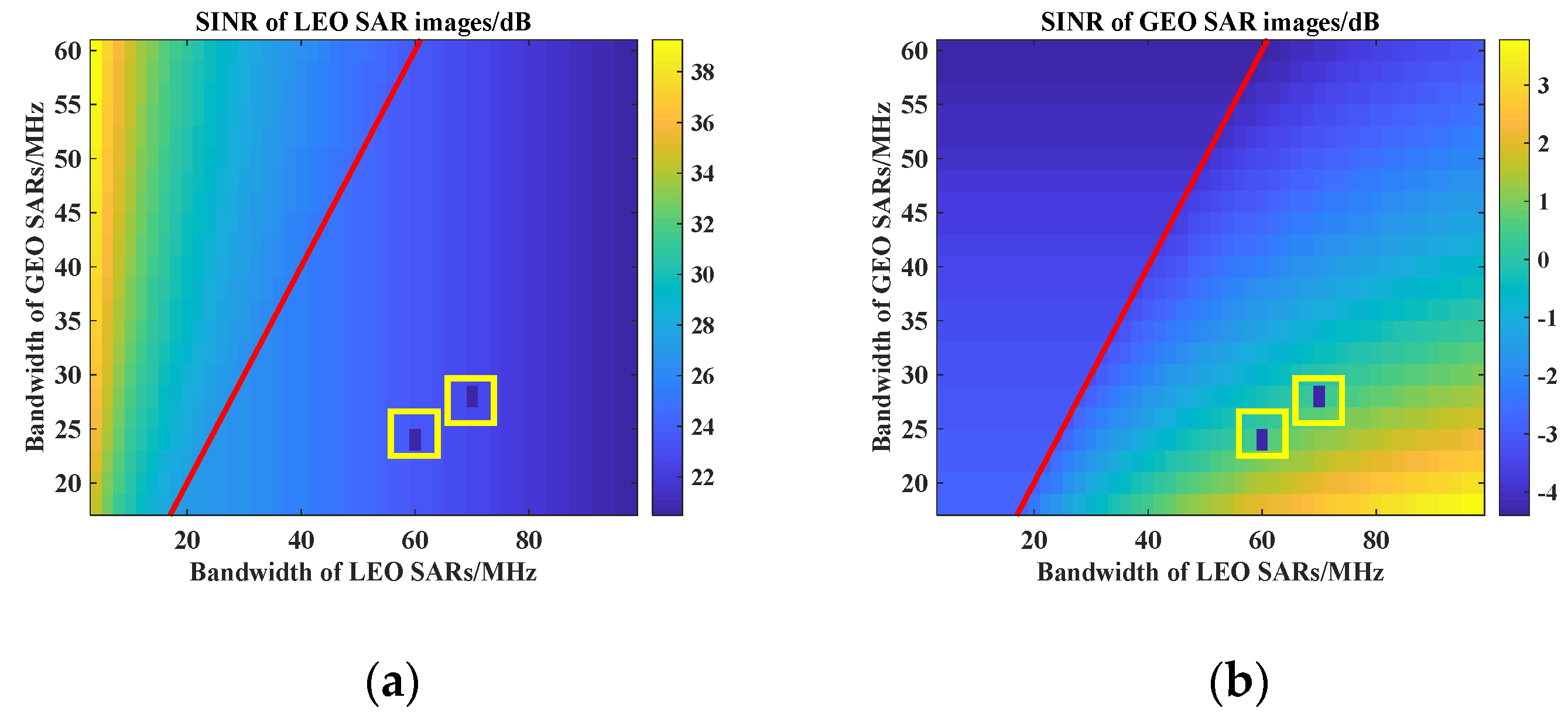
3.2. SINR in Different System Parameters of LEO SARs and GEO SARs
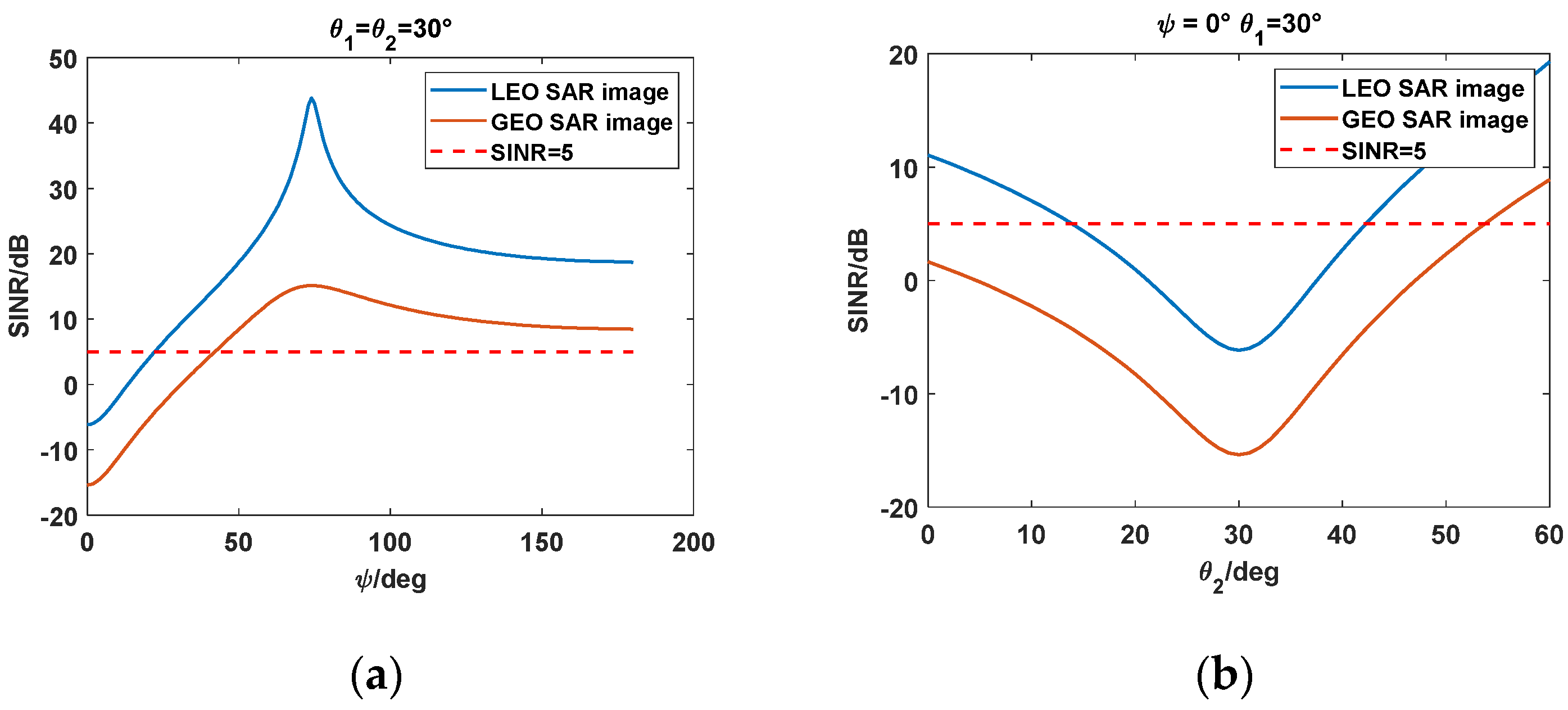
3.3. SINR under Different Bistatic Configurations
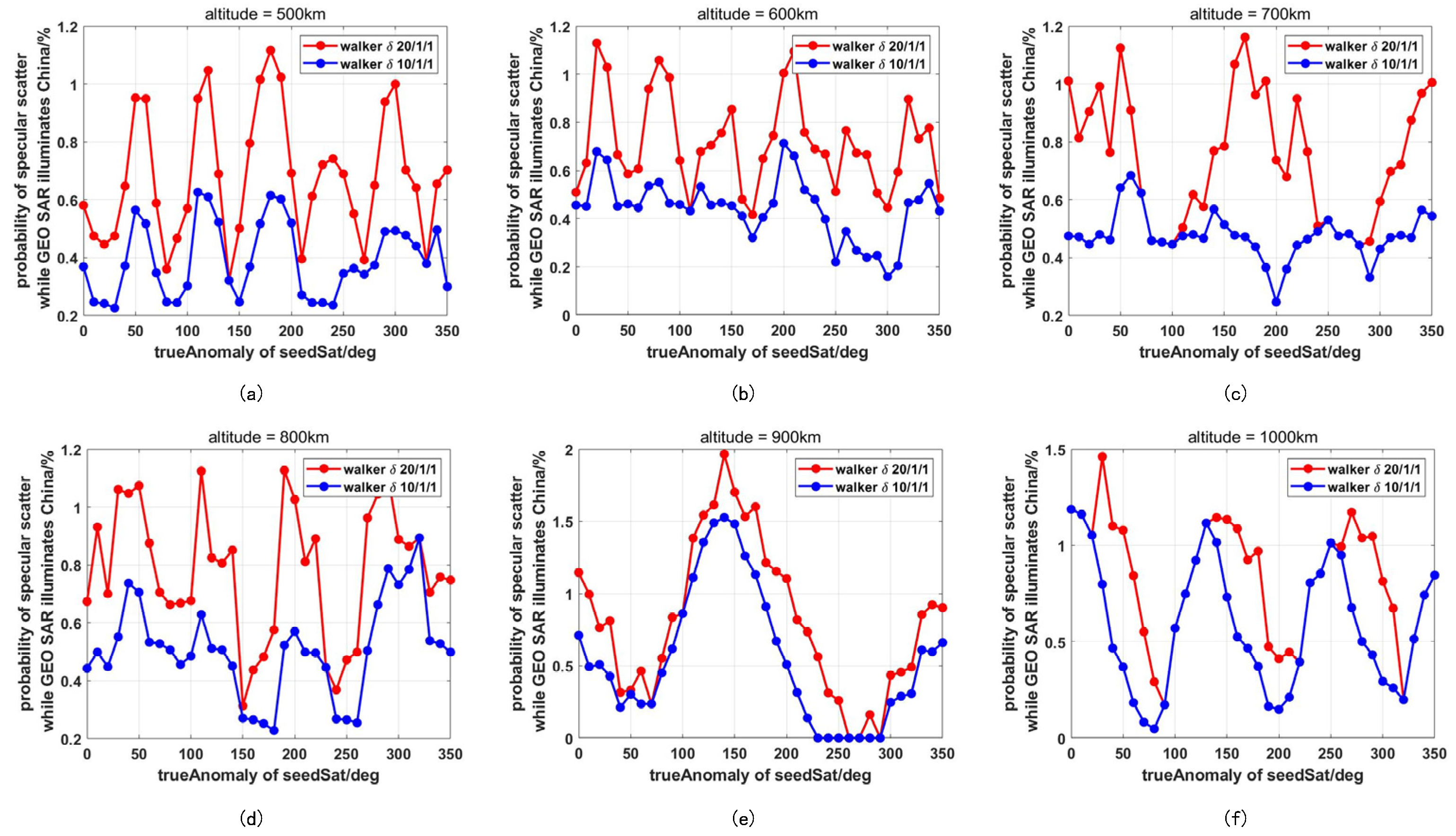
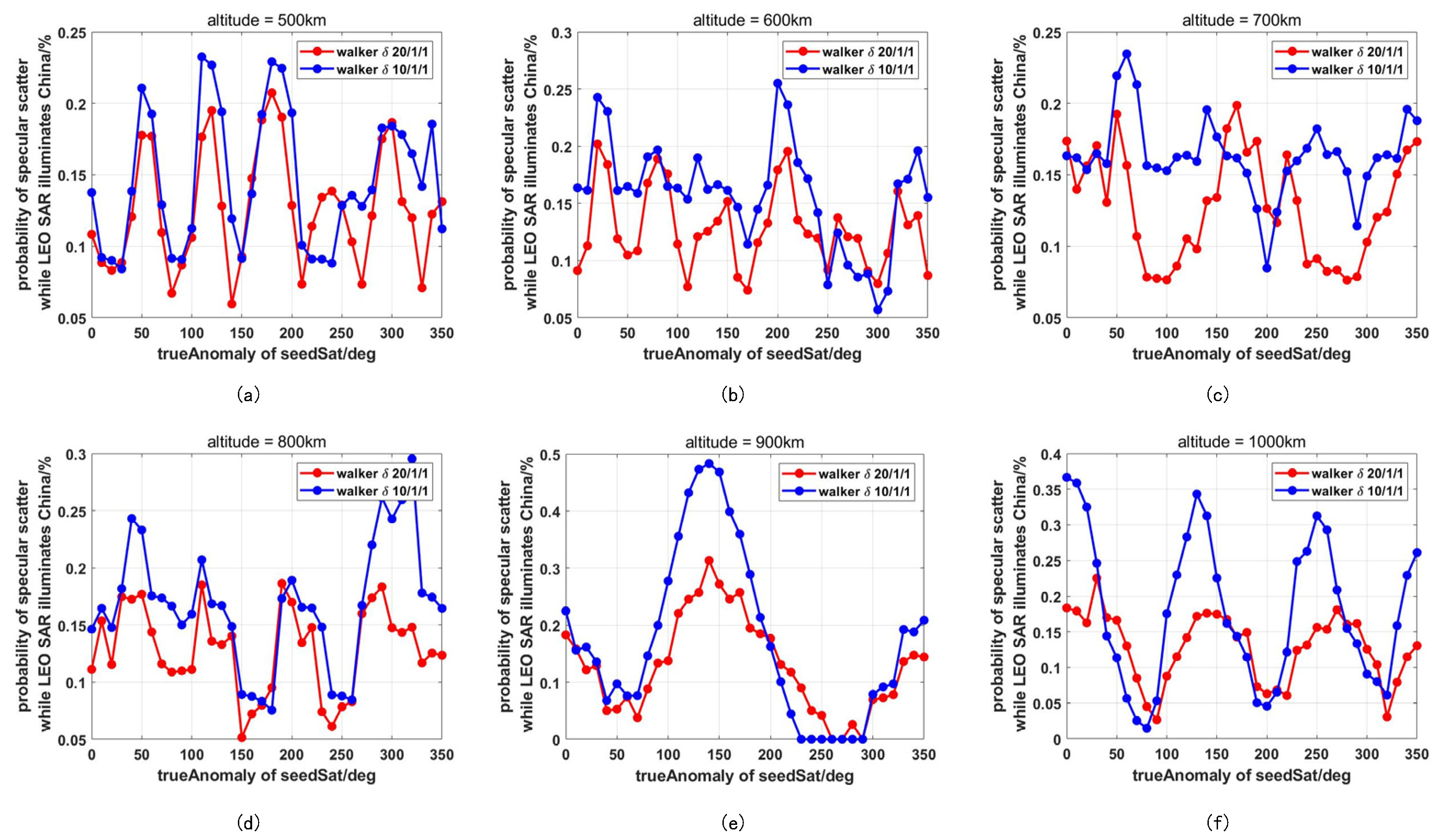
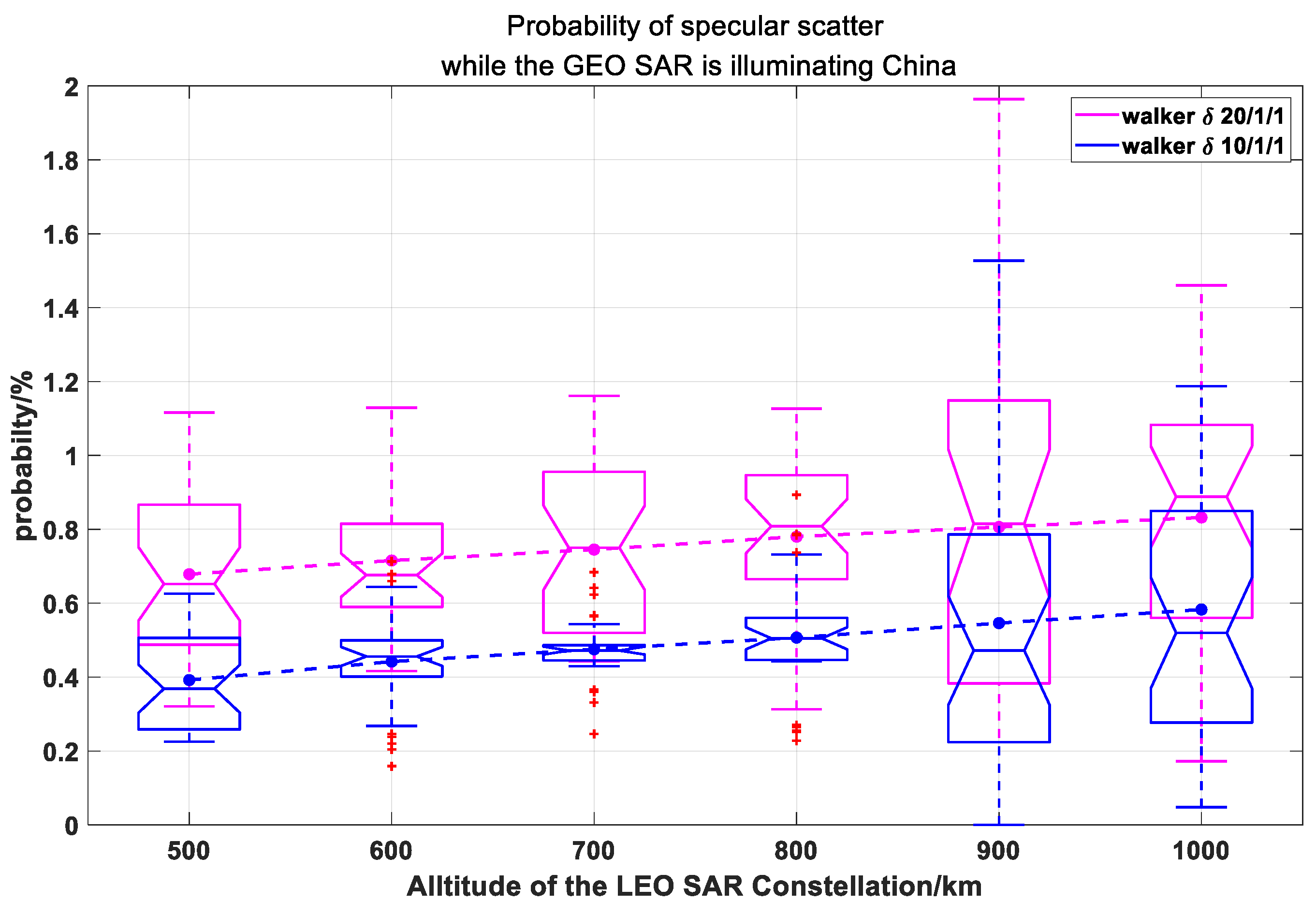
4. Discussion on Probability of Specular Scattering Case
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
Appendix B
References
- Moreira, A.; Prats-Iraola, P.; Younis, M.; Krieger, G.; Hajnsek, I. A tutorial on synthetic aperture radar. Geosci. Remote Sens. Mag. IEEE 2013, 1, 6–43. [Google Scholar] [CrossRef] [Green Version]
- Reigber, A.; Scheiber, R.; Jager, M.; Prats-Iraola, P.; Hajnsek, I.; Jagdhuber, T.; Papathanassiou, K.P.; Nannini, M.; Aguilera, E.; Baumgartner, S. Very-High-Resolution Airborne Synthetic Aperture Radar Imaging: Signal Processing and Applications. Proc. IEEE 2013, 101, 759–783. [Google Scholar] [CrossRef] [Green Version]
- Kussul, N.; Shelestov, A.; Skakun, S. Flood Monitoring from SAR Data. In Use of Satellite and In-Situ Data to Improve Sustainability; Springer: Dordrecht, The Netherlands, 2011. [Google Scholar]
- Raucoules, D.; Colesanti, C.; Carnec, C. Use of SAR interferometry for detecting and assessing ground subsidence. C. R.—Geosci. 2007, 339, 289–302. [Google Scholar] [CrossRef]
- Meyer, F.J.; Nicoll, J.B.; Doulgeris, A.P. Correction and Characterization of Radio Frequency Interference Signatures in L-Band Synthetic Aperture Radar Data. IEEE Trans. Geosci. Remote Sens. 2013, 51, 4961–4972. [Google Scholar] [CrossRef] [Green Version]
- Chen, D.; Yang, J.; Wu, J.; Hao, T.; Ming, H. Spectrum occupancy analysis based on radio monitoring network. In Proceedings of the 1st IEEE International Conference on Communications in China (ICCC), Beijing, China, 15–17 August 2012. [Google Scholar]
- Islam, M.H.; Koh, C.L.; Oh, S.W.; Qing, X.; Toh, W. Spectrum Survey in Singapore: Occupancy Measurements and Analyses. In Proceedings of the 3rd International Conference on Cognitive Radio Oriented Wireless Networks and Communications (CrownCom), Singapore, 15–17 May 2008. [Google Scholar]
- Küçük, İ.; Üler, İ.; Öz, Ş.; Onay, S.; Özdemir, A.; Gülşen, M.; Sarıkaya, M.; DağTekin, N.; Özeren, F. Site selection for a radio astronomy observatory in Turkey: Atmospherical, meteorological, and radio frequency analyses. Exp. Astron. 2012, 33, 1–26. [Google Scholar] [CrossRef]
- Koutsoudis, T.; Lovas, L.A. rf interference suppression in ultrawideband radar receivers. Proc. SPIE—Int. Soc. Opt. Eng. 1995, 2487, 107–118. [Google Scholar]
- Nguyen, M.T.; Long, B.L. Adjacent channel interference cancellation for robust spectrum sharing in satellite communications systems. In Proceedings of the 2017 IEEE 28th Annual International Symposium on Personal, Indoor, and Mobile Radio Communications (PIMRC), Montreal, QC, Canada, 8–13 October 2017. [Google Scholar]
- Shimada, M. L-band radio interferences observed by the JERS-1 SAR and its global distribution. In Proceedings of the 2005 IEEE International Geoscience and Remote Sensing Symposium, Seoul, Korea, 29 July 2005. [Google Scholar]
- Rosen, P.A.; Hensley, S.; Le, C. Observations and mitigation of RFI in ALOS PALSAR SAR data: Implications for the DESDynI mission. In Proceedings of the Radar Conference (RADAR ‘08), Rome, Italy, 26–30 May 2008. [Google Scholar]
- Meyer, F.J.; Nicoll, J.B.; Doulgeris, A.P. Characterization and extent of randomly-changing radio frequency interference in ALOS PALSAR data. In Proceedings of the 2011 IEEE International Geoscience and Remote Sensing Symposium, Vancouver, BC, Canada, 24–29 July 2011. [Google Scholar]
- Sentinel-1A-Annual-Performance-Report-2015. Available online: https://sentinel.esa.int/documents/247904/1814124/Sentinel-1A-Annual-Performance-Report-2015.pdf/1285c804-d83e-48e9-a934-4db30208f28a?t=1461670723000 (accessed on 8 April 2020).
- Saini, O.; Bhardwaj, A.; Chatterjee, R.S. Radio Frequency Interference Pattern Detection from Sentinel-1 SAR Data Using U-NET-Like Convolutional Neural Network. In Proceedings of the MOL2NET 2020 International Conference on Multidisciplinary Sciences, 6th Edition Session USINEWS-04: US-IN-EU Worldwide Science Workshop Series, Duluth, MN, USA, 21–23 June 2020. [Google Scholar]
- Nabil, H.; Jie, C.; Kamel, H.; Hui, K. Bidirectional notch filter for suppressing pulse modulated radio-frequency-interference in SAR data. In Proceedings of the IEEE Geoscience and Remote Sensing Symposium, Quebec City, QC, Canada, 13–18 July 2014. [Google Scholar]
- Van Leijen, F.; Rommen, B.; Davidson, M.; Hanssen, R. The impact of ground-based uncorrelated radio frequency interference (RFI) sources on satellite radar interferometric ground motion analysis. In Proceedings of the 2015 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Milan, Italy, 26–31 July 2015; pp. 4093–4096. [Google Scholar]
- Yang, L.; Zheng, H.; Feng, J.; Li, N.; Chen, J. Detection and suppression of narrow band RFI for synthetic aperture radar imaging. Chin. J. Aeronaut. 2015, 28, 1189–1198. [Google Scholar] [CrossRef] [Green Version]
- Tao, M.; Su, J.; Huang, Y.; Wang, L. Mitigation of Radio Frequency Interference in Synthetic Aperture Radar Data: Current Status and Future Trends. Remote Sens. 2019, 11, 2438. [Google Scholar] [CrossRef] [Green Version]
- Shao, P.; Lu, X.; Huang, P.; Xu, W.; Dong, Y. Impact Analysis of Radio Frequency Interference on SAR Image Ship Detection Based on Deep Learning. In Proceedings of the 2020 IEEE International Geoscience and Remote Sensing Symposium (IGARSS), Waikoloa, HI, USA, 26 September–2 October 2020. [Google Scholar]
- Reigber, A.; Ferro-Famil, L. Interference Suppression in Synthesized SAR Images. IEEE Geosci. Remote Sens. Lett. 2005, 2, 45–49. [Google Scholar] [CrossRef]
- Ding, B.; Mao, S.X.; Xing, D.L. Analysis of the effect of radio frequency interference on interferometric phase. In Proceedings of the 2010 IEEE International Geoscience and Remote Sensing Symposium, Honolulu, HI, USA, 25–30 July 2010. [Google Scholar]
- Reigber, A.; Ulbricht, A. P-band repeat-pass interferometry with the DLR experimental SAR (ESAR): First results. In Proceedings of the 1998 IEEE International Geoscience and Remote Sensing Symposium, Seattle, WA, USA, 6–10 July 1998. [Google Scholar]
- Guarnieri, A.M.; Rocca, F.; Ibars, A.B. Impact of atmospheric water vapor on the design of a Ku band geosynchronous SAR system. In Proceedings of the 2009 IEEE International Geoscience and Remote Sensing Symposium, Cape Town, South Africa, 12–17 July 2009; Volume 2, pp. II-945–II-948. [Google Scholar]
- Zhou, F.; Wu, R.; Xing, M.; Bao, Z. Eigensubspace-Based Filtering with Application in Narrow-Band Interference Suppression for SAR. IEEE Geosci. Remote Sens. Lett. 2007, 4, 75–79. [Google Scholar] [CrossRef]
- Andrea, M.G.; Davide, G.; Andrea, R. Identification of C-Band Radio Frequency Interferences from Sentinel-1 Data. Remote Sens. 2017, 9, 1183. [Google Scholar] [CrossRef] [Green Version]
- Tomiyasu, K. Synthetic aperture radar in geosynchronous orbit. In Proceedings of the Antennas & Propagation Society International Symposium, Washington, DC, USA, 15–19 March 1978. [Google Scholar]
- Hobbs, S.; Mitchell, C.; Forte, B.; Holley, R.; Snapir, B.; Whittaker, P. System design for geosynchronous synthetic aperture radar missions. IEEE Trans. Geosci. Remote Sens. 2014, 52, 7750–7763. [Google Scholar] [CrossRef] [Green Version]
- Rodon, J.R.; Broquetas, A.; Guarnieri, A.M.; Rocca, F. Geosynchronous SAR focusing with atmospheric phase screen retrieval and compensation. IEEE Trans. Geosci. Remote Sens. 2013, 51, 4397–4404. [Google Scholar] [CrossRef]
- Cheng, H.; Zca, C.; Yl, D.; Xda, E.; Sh, F. Research Progress on Geosynchronous Synthetic Aperture Radar. Fundam. Res. 2021, 1, 346–363. [Google Scholar]
- Chen, Z.; Hu, C.; Dong, X.; Li, Y.; Hobbs, S. Coherence-Based Geosynchronous SAR Tomography Employing Formation Flying: System Design and Performance Analysis. IEEE Trans. Geosci. Remote Sens. 2021, 59, 1–15. [Google Scholar] [CrossRef]
- Li, Y.; Monti Guarnieri, A.; Hu, C.; Rocca, F. Performance and requirements of GEO SAR systems in the presence of radio frequency interferences. Remote Sens. 2018, 10, 82. [Google Scholar] [CrossRef] [Green Version]
- Leanza, A.; Manzoni, M.; Monti-Guarnieri, A.; di Clemente, M. LEO to GEO-SAR Interferences: Modelling and Performance Evaluation. Remote Sens. 2019, 11, 1720. [Google Scholar] [CrossRef] [Green Version]
- Sui, Y.; Dong, X.; Yin, P.; Hu, C.; Chen, Z.; Li, Y. Modeling and Analysis of Radio Frequency Interference Impacts from Geosynchronous SAR on Low Earth Orbit SAR. In Proceedings of the 2021 IEEE International Geoscience and Remote Sensing Symposium IGARSS, Brussels, Belgium, 12 October 2021; pp. 1666–1669. [Google Scholar]
- Tao, M. Research on Radio Frequency Interference Mitigration and Land Cover Classification for PolSAR. Ph.D. Thesis, Xidian University, Xi’an, China, 2016. [Google Scholar]
- Skolnik, M.I. Radar Handbook; McGraw-Hill Book Co.: New York, NY, USA, 1990. [Google Scholar]
- Curlander, J.C.; Mcdonough, R.N. Synthetic Aperture Radar: Systems and Signal Processing; Wiley: Hoboken, NJ, USA, 1991. [Google Scholar]
- Nezlin, D.V.; Kostylev, V.; Blyakhman, A.B.; Ryndyk, A.G.; Myakinkov, A. Bistatic Radar: Principles and Practice; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Cumming, I.G.; Wong, F.H. Digital Signal Processing of Synthetic Aperture Radar Data: Algorithms and Implementation; Artech House: Norwood, MA, USA, 2004. [Google Scholar]
- Kankaku, Y.; Suzuki, S.; Osawa, Y. ALOS-2 mission and development status. In Proceedings of the 2013 IEEE International Geoscience and Remote Sensing Symposium—IGARSS, Melbourne, Australia, 21–26 July 2013; pp. 2396–2399. [Google Scholar]
- Wu, T.D.; Chen, K.S.; Shi, J.; Lee, H.W.; Fung, A.K. A Study of an AIEM Model for Bistatic Scattering from Randomly Rough Surfaces. IEEE Trans. Geosci. Remote Sens. 2008, 46, 2584–2598. [Google Scholar]





| Index | Operation |
|---|---|
| Step 1 | |
| Step 2 | and length N |
| Step 3 | |
| Step 4 | represents average. |
| Parameter | LEO SAR | GEO SAR |
|---|---|---|
| peak transmitted power/w | 610 | 5000 |
| Antenna gain/dB | 38.2 | 50 |
| Slant range/km | 740 | 36,500 |
| Wavelength/m | 0.229 | 0.229 |
| Bandwidth/MHz | 28 | 15 |
| Pulse width /us | 50 | 20 |
| PRF/Hz | 1500 | 100 |
| Satellite velocity/m/s | 7629 | 855 |
| Incident angle/° | 30 | 30 |
| Azimuth beamwidth/° | 0.3/1.47 | -- |
| Integration time/s | 0.5 | 25/50/220 |
| Sampling rate/MHz | 40 | 30 |
| backscattering coefficient | 0.2 | |
| Bistatic scattering coefficient | 0.2 | |
| Pixel interval/m | 20 | |
| Target | RFI | |
|---|---|---|
| Power before BP algorithm/dBw | −162.3863 | −141.6115 |
| Evaluated gains in BP algorithm/dB | 123.5218 | 56.3548 |
| Evaluated power after BP algorithm/dB | −38.8646 | −85.2567 |
| Calculated power after BP algorithm/dBw | −38.8606 | −85.4212 |
| Error/% | 0.1 | 3.7 |
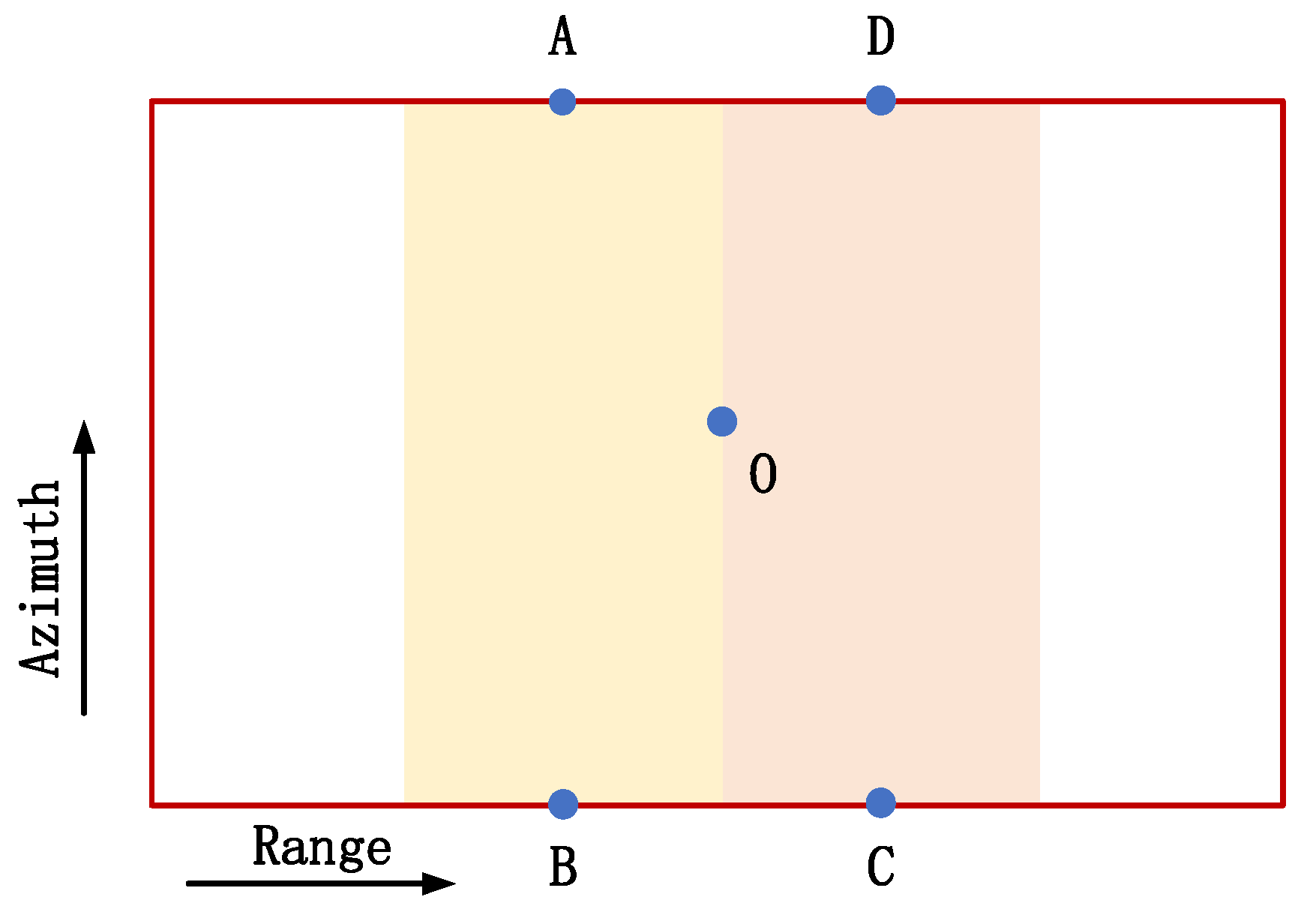
| Point | Integration Time/s | Calculated Results/km | Evaluated Results/km | Range Drift/m |
|---|---|---|---|---|
| A | 25 | 6.20 | 6.25 | 179 |
| 50 | 25.00 | 24.45 | 679 | |
| D | 25 | 6.28 | 6.25 | 170 |
| 50 | 25.5 | 24.4 | 668 |
| Parameter | Integration Time 25 s | Integration Time 50 s |
|---|---|---|
| /km | 6.25 | 24.4 |
| /km | 2.04 | 2.04 |
| 3.07 | 12 | |
| /dBw | −84.75 | −87.54 |
| /dBw | −79.63 | −76.66 |
| 3.26 | 12.28 |
| Point | Evaluated Results/dBw | Calculated Results/dBw | Error/% |
|---|---|---|---|
| GEO-to-LEO RFI | −42.2548 | −42.3657 | 2.4 |
| LEO-to-GEO RFI | −42.5571 | −42.3284 | 5.2 |
| Case | in Figure 1 |
|---|---|
| Specular scattering | |
| Non-specular scattering | |
| GEO SAR | LEO SAR Constellation | ||
|---|---|---|---|
| Orbit altitude/km | 35,793 | Orbit type | sun-synchronous |
| Inclination/° | 16 | Orbit altitude/km | 500–1000 |
| Eccentricity | 0 | Constellation type | Walker |
| True anomaly/° | 0 | Patten | 20/1/1; 10/1/1 |
| Argument of Perigee/° | 0 | Satellite total number | 20; 10 |
| Lon.Ascn.Node/° | 88 | True anomaly/° | 0–360 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dong, X.; Sui, Y.; Li, Y.; Chen, Z.; Hu, C. Modeling and Analysis of RFI Impacts on Imaging between Geosynchronous SAR and Low Earth Orbit SAR. Remote Sens. 2022, 14, 3048. https://doi.org/10.3390/rs14133048
Dong X, Sui Y, Li Y, Chen Z, Hu C. Modeling and Analysis of RFI Impacts on Imaging between Geosynchronous SAR and Low Earth Orbit SAR. Remote Sensing. 2022; 14(13):3048. https://doi.org/10.3390/rs14133048
Chicago/Turabian StyleDong, Xichao, Yi Sui, Yuanhao Li, Zhiyang Chen, and Cheng Hu. 2022. "Modeling and Analysis of RFI Impacts on Imaging between Geosynchronous SAR and Low Earth Orbit SAR" Remote Sensing 14, no. 13: 3048. https://doi.org/10.3390/rs14133048
APA StyleDong, X., Sui, Y., Li, Y., Chen, Z., & Hu, C. (2022). Modeling and Analysis of RFI Impacts on Imaging between Geosynchronous SAR and Low Earth Orbit SAR. Remote Sensing, 14(13), 3048. https://doi.org/10.3390/rs14133048










