Potential Applications of CE-2 Microwave Radiometer Data in Understanding Basaltic Volcanism in Heavily Ejecta-Contaminated Mare Frigoris
Abstract
:1. Introduction
2. Data Processing
2.1. CE-2 MRM Data Processing
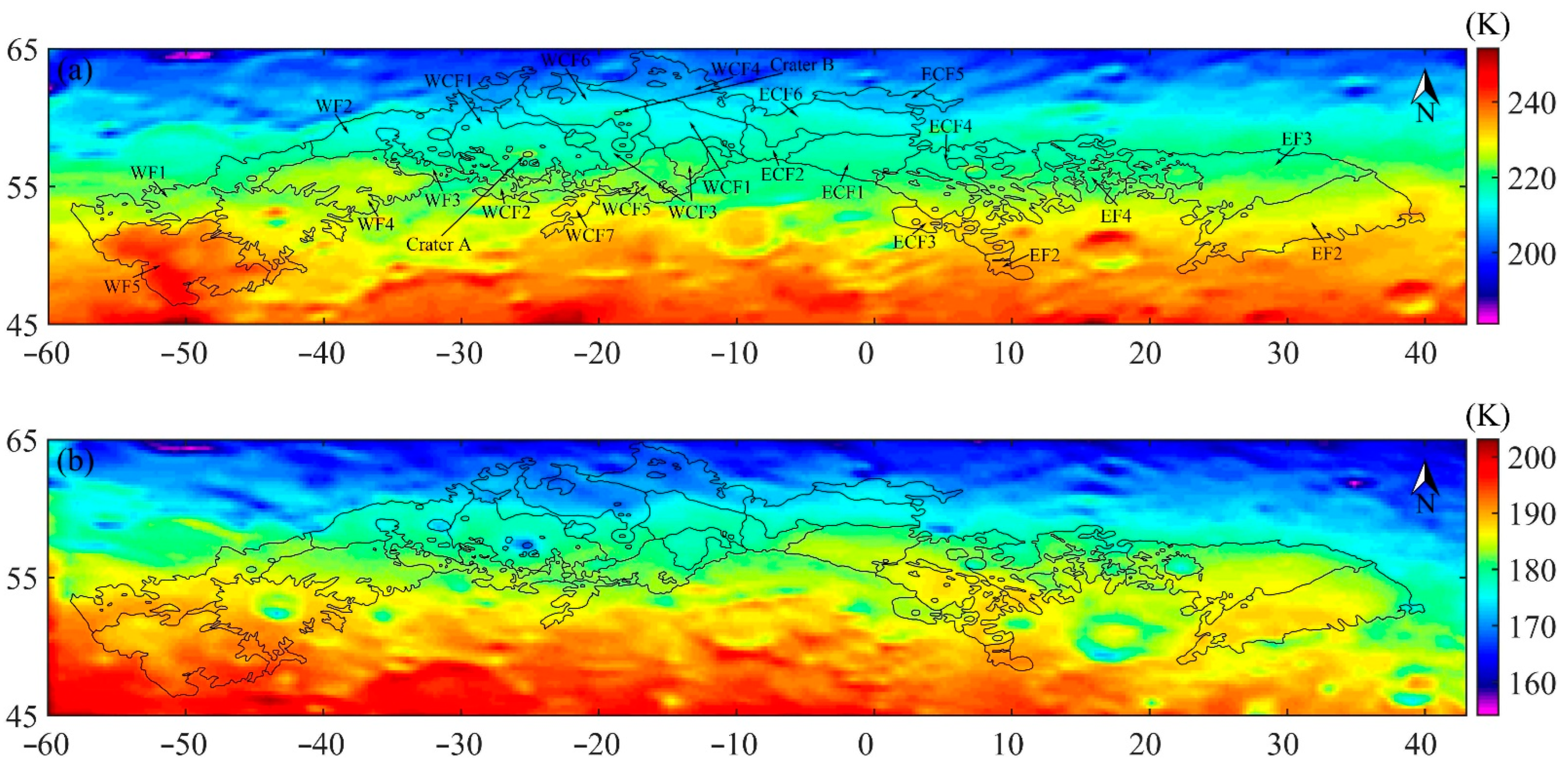
2.1.1. TB Maps Generation
2.1.2. nTB and TBD Maps Generation
2.2. FeO and TiO2 Abundance (FTA)
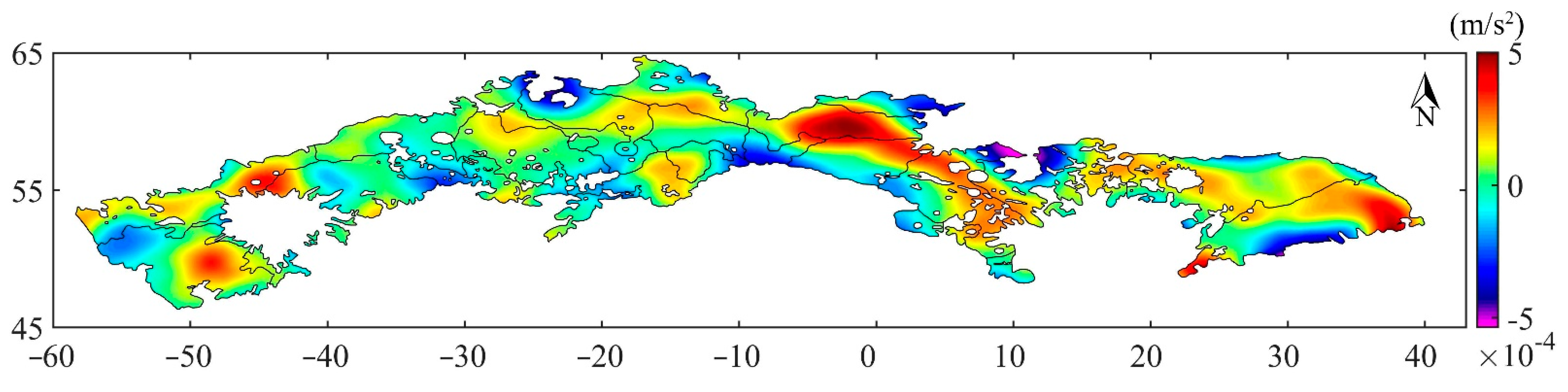
2.3. Bouguer Gravity Anomaly
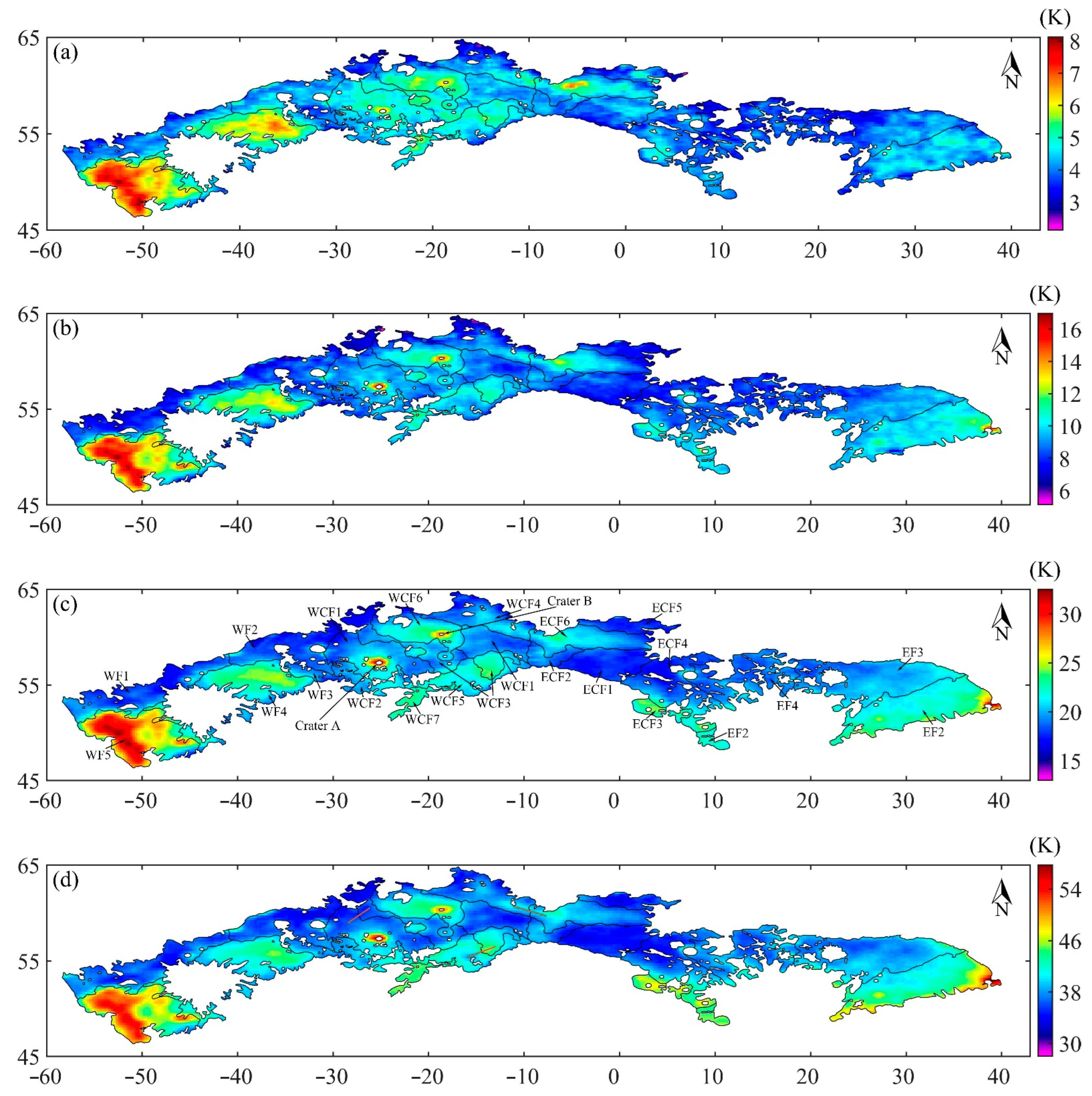
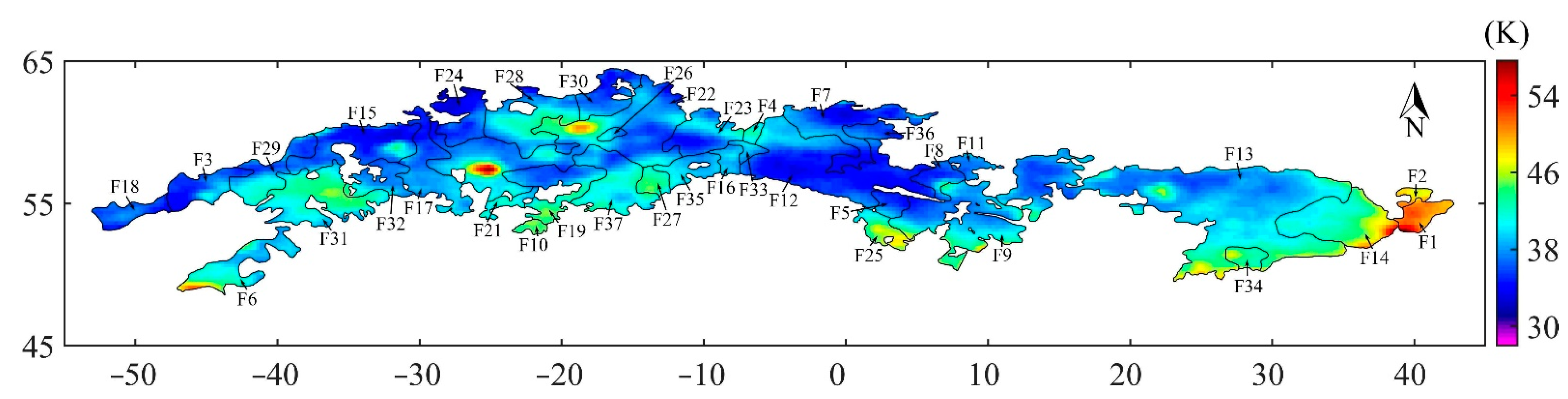
3. Results
3.1. Assessing the Basaltic Results
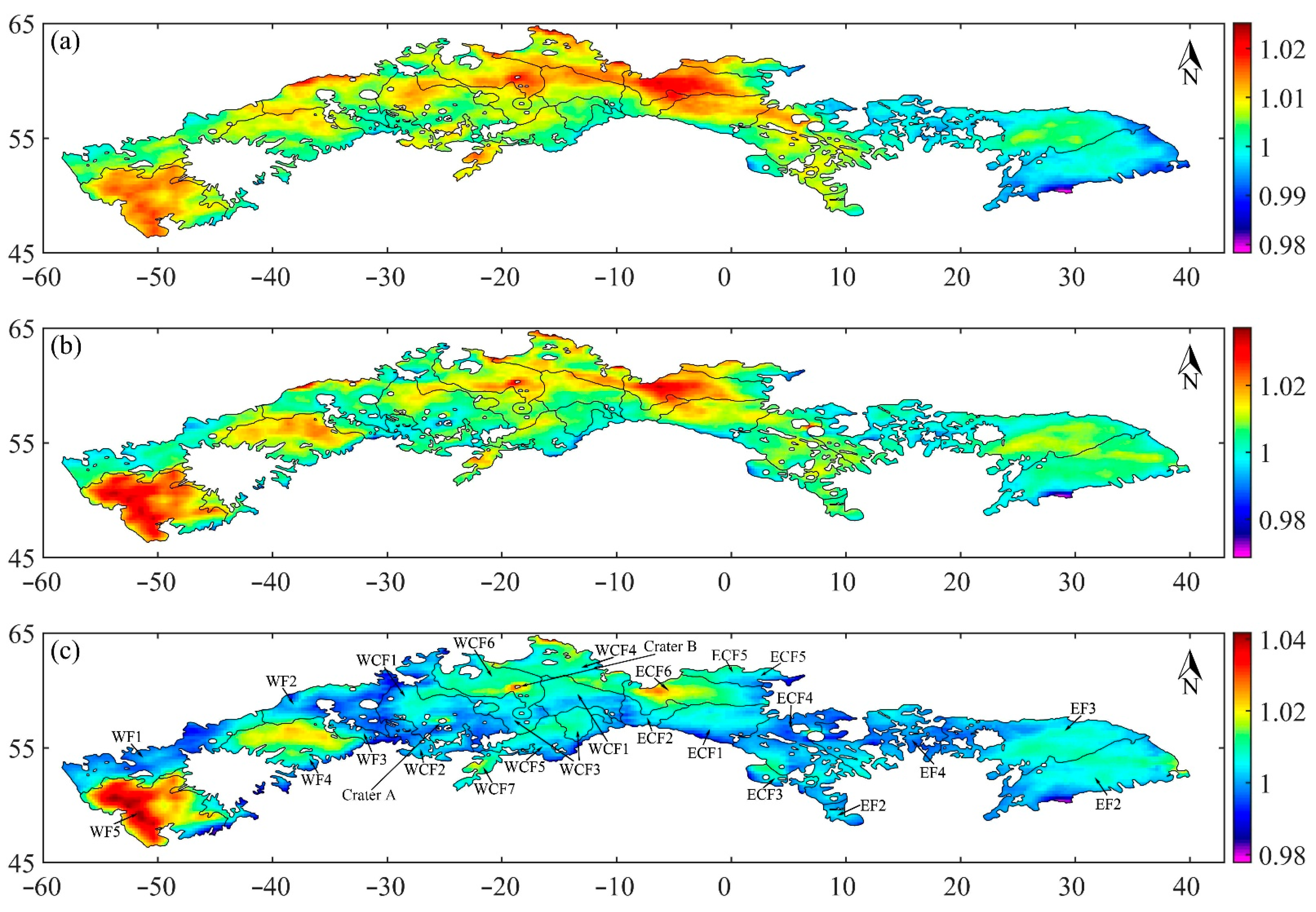
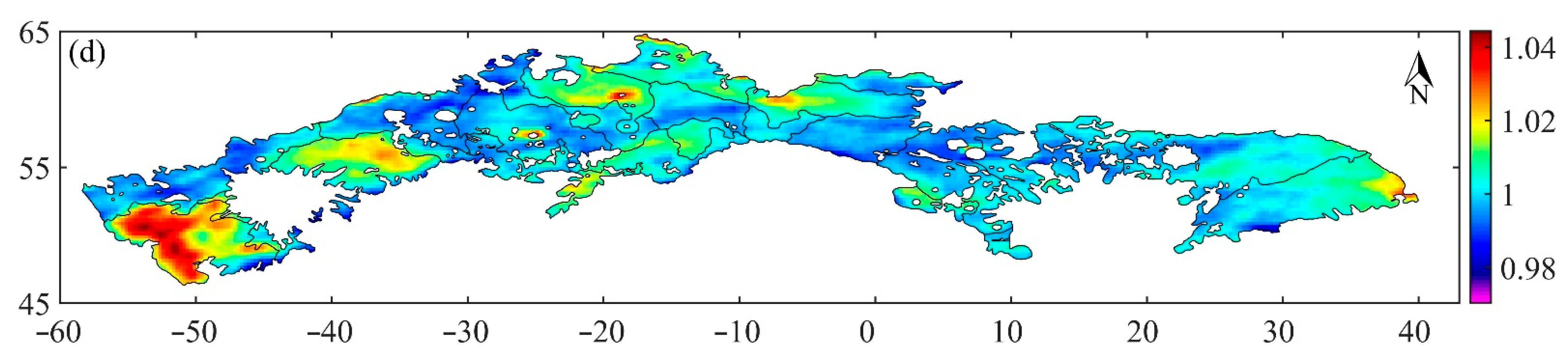
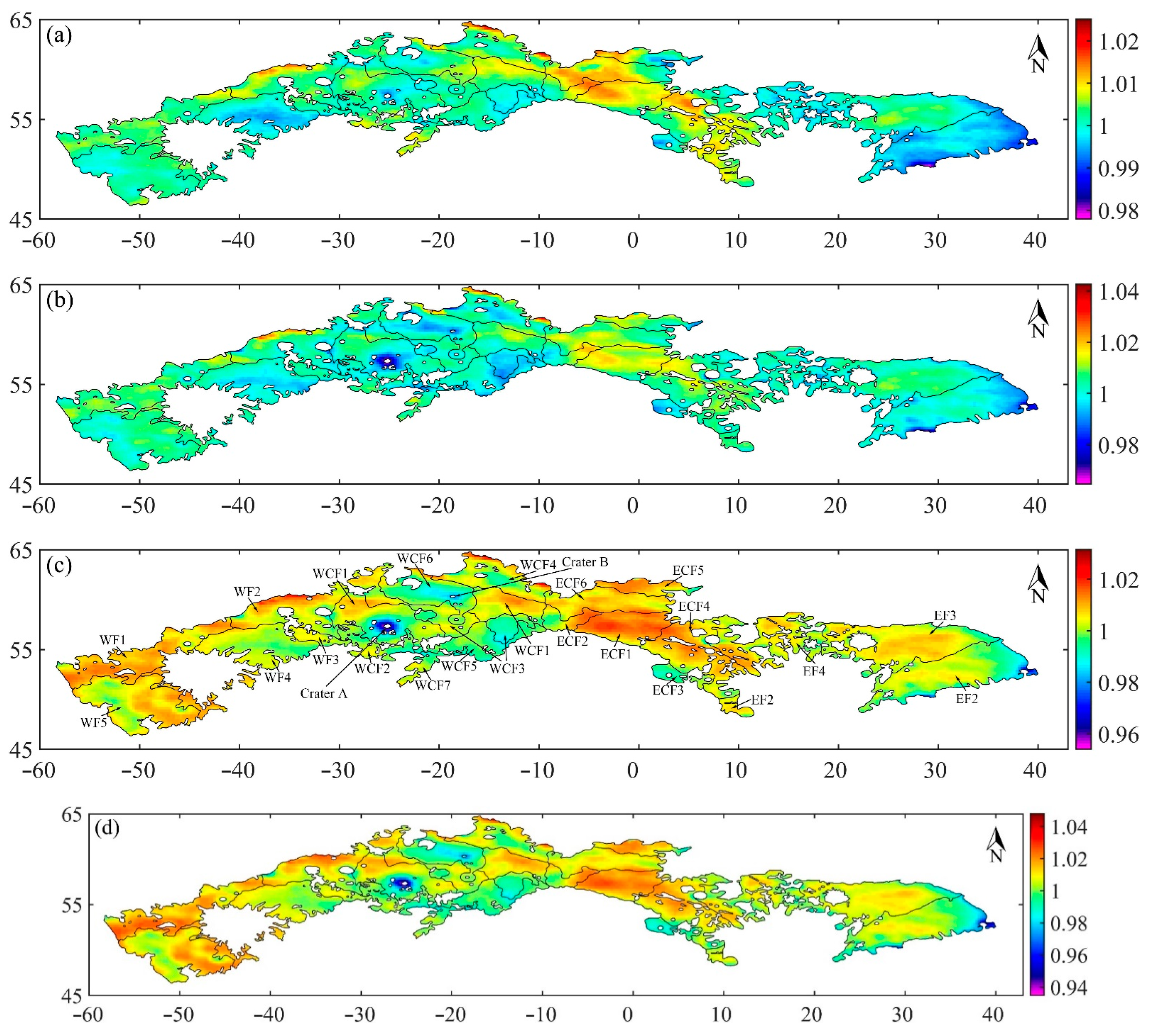
3.2. nTB and TBD Performances of Basaltic units
3.2.1. Western Frigoris
3.2.2. West-Central Frigoris
3.2.3. East-Central Frigoris
3.2.4. Eastern Frigoris
3.3. Redrawing Geologic Sketch of Basaltic Units and Remaining Problems
- (1)
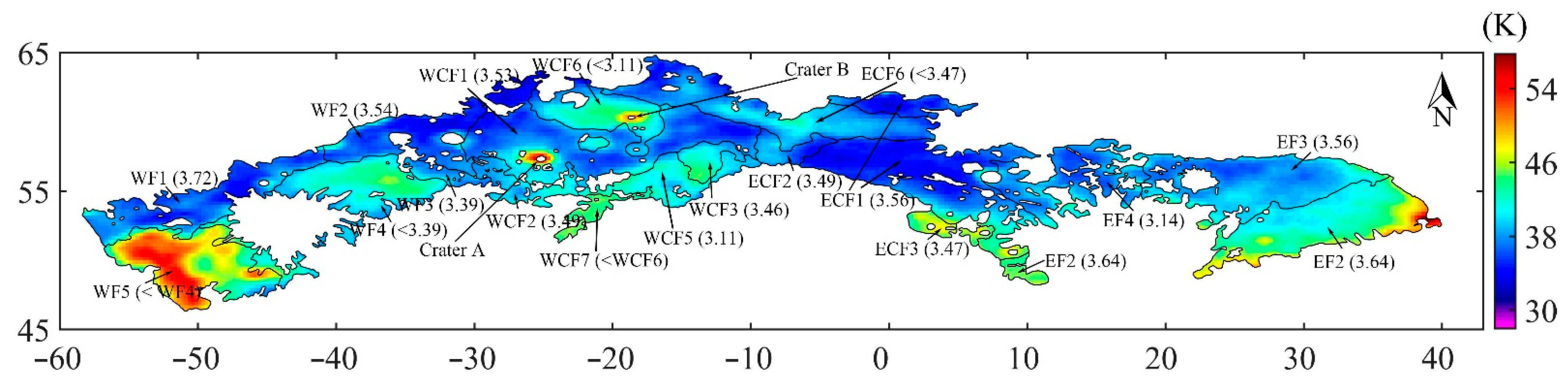
- The spatial distributions of the nTB and TBD values are consistent with the boundaries of the basaltic units in the WF, WCF, and ECF quadrants mapped by Kramer et al. [2], as shown in Figure 4, Figure 5 and Figure 6. This comparison hints at the fact that the MRM data have the potential capability to probe the pristine basalt under ejecta, such as that reflected by the SCREP craters.
- (2)
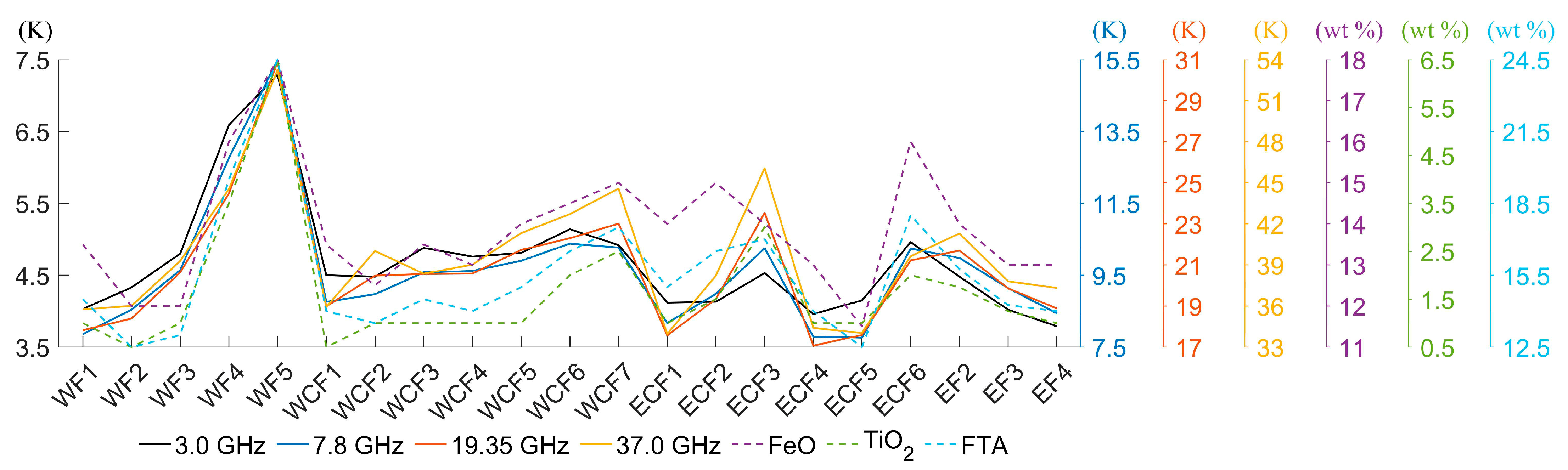
- In most of the basaltic units mapped by Kramer et al. [2], the TBD results have a strong relationship with the FTA. Whether this relationship is valid or not should be verified for the whole of Mare Frigoris.
- (3)
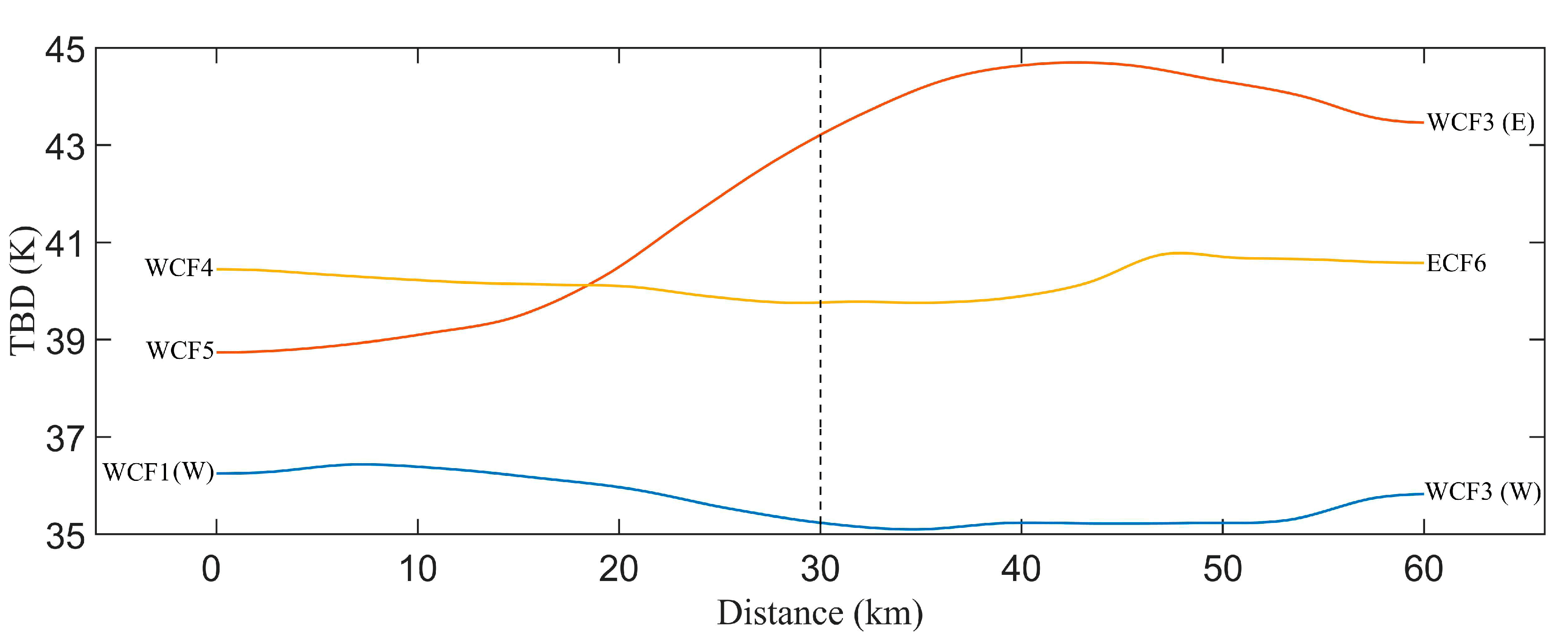
- In most quadrants, the TBD results have a good agreement with the basaltic units. However, there were abnormal 3.0-GHz nTB results for the ECF1, ECF2, ECF5, and ECF6 units, which are relatively high both at noon and at night. The penetration depth of a 3.0-GHz microwave is about 1 m to 2 m [15], and the substrate temperature at this depth is slightly influenced by the surface temperature [30]. The causes of the TB anomaly, which will be discussed further in Section 4.2.
4. Discussion
4.1. New Views of Basaltic Volcanism in Mare Frigoris
4.1.1. Representation of TB to Basaltic Units
4.1.2. New Views of Basaltic Volcanism
4.2. TB Anomalies in Mare Frigoris
4.2.1. Cold Microwave Anomaly
4.2.2. Warm Microwave Anomaly
5. Conclusions
- (1)
- The nTB and TBD results agree with the basaltic units based on the small crater rim and ejecta probing (SCREP) methodology. This indicates that MRM data are highly related to the (FeO + TiO2) abundance of pristine basalts and are less influenced by ejecta contamination when compared to SCREP-based FeO and TiO2 abundances.
- (2)
- Based on the nTB and TBD results, the SCREP-based (FeO + TiO2) abundance, and the superposition relationship of the stratum, it was found that, in most quadrants of Mare Frigoris, the (FeO + TiO2) abundances of basaltic units were higher at younger ages.
- (3)
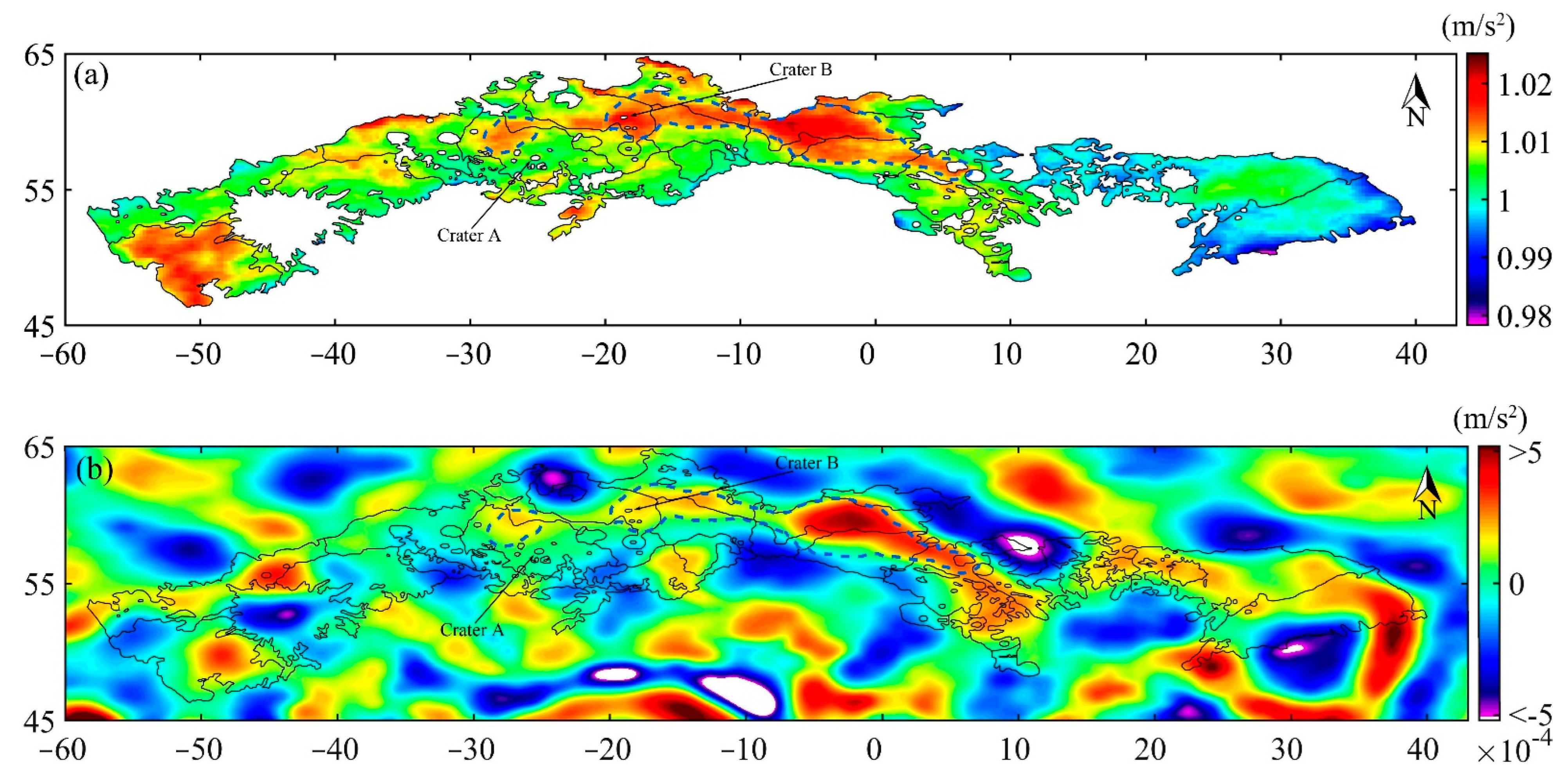
- A comparison of the rock abundance map and the nTB and TBD results for Craters A and B indicated that, at least in Mare Frigoris, the (FeO + TiO2) abundances of surface deposits remains the main influencing factor behind the TB.
- (4)
- The warm microwave anomaly was revealed in the case of the ECF1, ECF6, WCF4, and WCF6 units, and showed a similar distribution to the Bouguer gravity anomaly derived from GRAIL data. This provides useful information in the context of determining the cause of the warm microwave anomaly.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Whitford-Stark, J.L. The volcanotectonic evolution of Mare Frigoris. In Proceedings of the Lunar and Planetary Science Conference, Houston, TX, USA, 12–16 March 1990; pp. 175–185. [Google Scholar]
- Kramer, G.; Jaiswal, B.; Hawke, B.; Öhman, T.; Giguere, T.; Johnson, K. The basalts of mare Frigoris. J. Geophys. Res. Planets 2015, 120, 1646–1670. [Google Scholar] [CrossRef] [Green Version]
- Williams, N.R.; Bell, J.F., III; Watters, T.R.; Banks, M.E.; Daud, K.; French, R.A. Evidence for recent and ancient faulting at Mare Frigoris and implications for lunar tectonic evolution. Icarus 2019, 326, 151–161. [Google Scholar] [CrossRef]
- Giguere, T.; Hawke, B.R.; Gaddis, L.; Gustafson, J.; Lawrence, S.; Stopar, J.; Mattson, S.; Robinson, M. Geologic studies of volcanic constructs in eastern Mare Frigoris. In Proceedings of the Lunar and Planetary Science Conference, The Woodlands, TX, USA, 17–21 March 2014; p. 1318. [Google Scholar]
- Albin, E.; Harris, R. Volcanism Within Mare Frigoris: Further Evidence for Recent Eruptions on the Moon. In Proceedings of the Lunar and Planetary Science Conference, The Woodlands, TX, USA, 16–20 March 2015; p. 2633. [Google Scholar]
- Andrews-Hanna, J.C.; Besserer, J.; Head, J.W., III; Howett, C.J.; Kiefer, W.S.; Lucey, P.J.; McGovern, P.J.; Melosh, H.J.; Neumann, G.A.; Phillips, R.J. Structure and evolution of the lunar Procellarum region as revealed by GRAIL gravity data. Nature 2014, 514, 68–71. [Google Scholar] [CrossRef]
- Boyce, J.M. Ages of flow units in the lunar nearside maria based on Lunar Orbiter IV photographs. In Proceedings of the Lunar and Planetary Science Conference, Houston, TX, USA, 15–19 March 1976; pp. 2717–2728. [Google Scholar]
- Wilhelms, D.E.; John, F.; Trask, N.J. The Geologic History of the Moon; USGS: Reston, VA, USA, 1987; pp. 2330–7102. [Google Scholar]
- Staid, M.I.; Pieters, C.M. Integrated spectral analysis of mare soils and craters: Applications to eastern nearside basalts. Icarus 2000, 145, 122–139. [Google Scholar] [CrossRef]
- Hiesinger, H.; Head, J., III; Wolf, U.; Jaumann, R.; Neukum, G. Ages and stratigraphy of lunar mare basalts in Mare Frigoris and other nearside maria based on crater size-frequency distribution measurements. J. Geophys. Res. Planets 2010, 115, E03003. [Google Scholar] [CrossRef] [Green Version]
- Morota, T.; Haruyama, J.; Ohtake, M.; Matsunaga, T.; Honda, C.; Yokota, Y.; Kimura, J.; Ogawa, Y.; Hirata, N.; Demura, H. Timing and characteristics of the latest mare eruption on the Moon. Earth Planet. Sci. Lett. 2011, 302, 255–266. [Google Scholar] [CrossRef]
- Kramer, G.Y. Characterizing bedrock lithologies using small crater rims and ejecta probing (SCREP). Adv. Space Res. 2010, 45, 1257–1267. [Google Scholar] [CrossRef]
- Kramer, G.Y.; Combe, J.P.; Harnett, E.M.; Hawke, B.R.; Noble, S.K.; Blewett, D.T.; McCord, T.B.; Giguere, T.A. Characterization of lunar swirls at Mare Ingenii: A model for space weathering at magnetic anomalies. J. Geophys. Res. Planets 2011, 116, E04008. [Google Scholar] [CrossRef] [Green Version]
- Weider, S.Z.; Crawford, I.A.; Joy, K.H. Individual lava flow thicknesses in Oceanus Procellarum and Mare Serenitatis determined from Clementine multispectral data. Icarus 2010, 209, 323–336. [Google Scholar] [CrossRef] [Green Version]
- Campbell, B.A.; Carter, L.M.; Hawke, B.R.; Campbell, D.B.; Ghent, R.R. Volcanic and impact deposits of the Moon’s Aristarchus Plateau: A new view from Earth-based radar images. Geology 2008, 36, 135–138. [Google Scholar] [CrossRef]
- Fang, T.; Fa, W. High frequency thermal emission from the lunar surface and near surface temperature of the Moon from Chang’E-2 microwave radiometer. Icarus 2014, 232, 34–53. [Google Scholar] [CrossRef]
- Meng, Z.; Hu, S.; Wang, T.; Li, C.; Cai, Z.; Ping, J. Passive microwave probing mare basalts in mare imbrium using CE-2 CELMS data. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2018, 11, 3097–3104. [Google Scholar] [CrossRef]
- Jin, Y.; Yan, F.; Liang, Z. Simulation of brightness temperature from the lunar surface using multi-channels microwave radiometers. Chin. J. Radio Sci. 2003, 18, 477–486. [Google Scholar]
- Hu, G.; Chan, K.L.; Zheng, Y.; Tsang, K.T.; Xu, A. Comparison and evaluation of the Chang’E microwave radiometer data based on theoretical computation of brightness temperatures at the Apollo 15 and 17 sites. Icarus 2017, 294, 72–80. [Google Scholar] [CrossRef]
- Mustard, J.F.; Head, J.W. Buried stratigraphic relationships along the southwestern shores of Oceanus Procellarum: Implications for early lunar volcanism. J. Geophys. Res. Planets 1996, 101, 18913–18925. [Google Scholar] [CrossRef]
- Xie, M.; Xiao, Z.; Zhang, X.; Xu, A. The provenance of regolith at the Chang’e-5 candidate landing region. J. Geophys. Res. Planets 2020, 125, e2019JE006112. [Google Scholar] [CrossRef]
- Zheng, Y.; Tsang, K.; Chan, K.; Zou, Y.; Zhang, F.; Ouyang, Z. First microwave map of the Moon with Chang’E-1 data: The role of local time in global imaging. Icarus 2012, 219, 194–210. [Google Scholar] [CrossRef]
- Zheng, Y.; Chan, K.L.; Tsang, K.T.; Zhu, Y.; Hu, G.P.; Blewett, D.T.; Neish, C. Analysis of Chang’E-2 brightness temperature data and production of high spatial resolution microwave maps of the Moon. Icarus 2019, 319, 627–644. [Google Scholar] [CrossRef]
- Fa, W.; Jin, Y. A primary analysis of microwave brightness temperature of lunar surface from Chang-E 1 multi-channel radiometer observation and inversion of regolith layer thickness. Icarus 2010, 207, 605–615. [Google Scholar] [CrossRef]
- Cai, Z.; Lan, T. Lunar brightness temperature model based on the microwave radiometer data of Chang’E-2. IEEE Trans. Geosci. Remote Sens. 2017, 55, 5944–5955. [Google Scholar] [CrossRef]
- Chan, K.L.; Tsang, K.T.; Kong, B.; Zheng, Y. Lunar regolith thermal behavior revealed by Chang’E-1 microwave brightness temperature data. Earth Planet. Sci. Lett. 2010, 295, 287–291. [Google Scholar] [CrossRef]
- Meng, Z.; Chen, S.; Zheng, Y.; Cheng, W.; Zhu, Y.; Cai, Z.; Zhang, Y.; Cao, W.; Hou, L. Mare Deposits Identification and Feature Analysis in Mare Australe Based on CE-2 CELMS Data. J. Geophys. Res. Planets 2020, 125, e2019JE006330. [Google Scholar] [CrossRef]
- Meng, Z.; Chen, S.; Wang, Y.; Wang, T.; Cai, Z.; Zhang, Y.; Zheng, Y.; Hu, S. Reevaluating Mare Moscoviense and its vicinity using Chang’E-2 microwave sounder data. Remote Sens. 2020, 12, 535. [Google Scholar] [CrossRef] [Green Version]
- Hu, G.; Chan, K.L.; Zheng, Y.; Xu, A. A rock model for the cold and hot spots in the Chang’E microwave brightness temperature map. IEEE Trans. Geosci. Remote Sens. 2018, 56, 5471–5480. [Google Scholar] [CrossRef]
- Heiken, G.H.; Vaniman, D.T.; French, B.M. Lunar Sourcebook, a User’s Guide to the Moon; Cambridge University Press: New York, NY, USA, 1991. [Google Scholar]
- Whitford-Stark, J.L.; Fryer, R. Origin of Mare Frigoris. Icarus 1975, 26, 231–242. [Google Scholar] [CrossRef]
- Hiesinger, H.; Head, J., III; Wolf, U.; Jaumann, R.; Neukum, G. Ages and stratigraphy of mare basalts in oceanus procellarum, mare nubium, mare cognitum, and mare insularum. J. Geophys. Res. Planets 2003, 108, 5065. [Google Scholar] [CrossRef] [Green Version]
- Lucey, P.G.; Blewett, D.T.; Jolliff, B.L. Lunar iron and titanium abundance algorithms based on final processing of Clementine ultraviolet-visible images. J. Geophys. Res. Planets 2000, 105, 20297–20305. [Google Scholar] [CrossRef]
- Meng, Z.; Yang, G.; Ping, J.; Cai, Z.; Gusev, A.; Osei, E.M. Influence of (FeO+ TiO2) abundance on the microwave thermal emissions of lunar regolith. Sci. China Earth Sci. 2016, 59, 1498–1507. [Google Scholar] [CrossRef]
- Meng, Z.; Lei, J.; Qian, Y.; Xiao, L.; Head, J.W.; Chen, S.; Cheng, W.; Shi, J.; Ping, J.; Kang, Z. Thermophysical Features of the Rümker Region in Northern Oceanus Procellarum: Insights from CE-2 CELMS Data. Remote Sens. 2020, 12, 3272. [Google Scholar] [CrossRef]
- Campbell, B.A.; Hawke, B.; Campbell, D.B. Surface morphology of domes in the Marius Hills and Mons Rümker regions of the Moon from Earth-based radar data. J. Geophys. Res. Planets 2009, 114, E01001. [Google Scholar] [CrossRef] [Green Version]
- Lee Rodgers, J.; Nicewander, W.A. Thirteen ways to look at the correlation coefficient. Am. Stat. 1988, 42, 59–66. [Google Scholar] [CrossRef]
- Zhou, H.; Deng, Z.; Xia, Y.; Fu, M. A new sampling method in particle filter based on Pearson correlation coefficient. Neurocomputing 2016, 216, 208–215. [Google Scholar] [CrossRef]
- Meng, Z.; Lei, J.; Xiao, Z.; Cao, W.; Cai, Z.; Cheng, W.; Feng, X.; Ping, J. Re-Evaluating Influence of Rocks on Microwave Thermal Emission of Lunar Regolith Using CE-2 MRM Data. IEEE Trans. Geosci. Remote Sens. 2022, 60, 1–12. [Google Scholar] [CrossRef]
- Bugiolacchi, R.; Hu, G.P. The Moon in the Microwave: Shedding New Light on the Lunar Farside. J. Geophys. Res. Planets 2022, 127, e2021JE007039. [Google Scholar] [CrossRef]
- Bandfield, J.L.; Ghent, R.R.; Vasavada, A.R.; Paige, D.A.; Lawrence, S.J.; Robinson, M.S. Lunar surface rock abundance and regolith fines temperatures derived from LRO Diviner Radiometer data. J. Geophys. Res. Planets 2011, 116, E00H02. [Google Scholar] [CrossRef]
- Keihm, S.J. Interpretation of the lunar microwave brightness temperature spectrum: Feasibility of orbital heat flow mapping. Icarus 1984, 60, 568–589. [Google Scholar] [CrossRef]






| Units | TBD (K) | FeO (wt %) | TiO2 (wt %) | FTA (wt %) | Age (Ga) | |||
|---|---|---|---|---|---|---|---|---|
| 3.0 GHz | 7.8 GHz | 19.35 GHz | 37 GHz | |||||
| WF1 | 4.0 | 7.9 | 17.8 | 35.8 | 13–14 | 1 | 14–15 | 3.72 |
| WF2 | 4.3 | 8.5 | 18.4 | 36.0 | 12 | <1 | 12–13 | 3.54 |
| WF3 | 4.2 | 8.8 | 19.5 | 38.0 | 12 | 1 | 13 | 3.39 |
| WF4 | 6.6 | 12.8 | 24.5 | 44.5 | 16 | 3–4 | 19–20 | <3.39 |
| WF5 | 7.3 | 15.5 | 30.9 | 53.2 | 18 | 6–7 | 24–25 | <WF4 |
| Units | TBD (K) | FeO (wt %) | TiO2 (wt %) | FTA (wt %) | Age (Ga) | |||
|---|---|---|---|---|---|---|---|---|
| 3.0 GHz | 7.8 GHz | 19.35 GHz | 37 GHz | |||||
| WCF1 | 4.5 | 8.8 | 19.0 | 35.9 | 13–14 | <1 | 13–15 | 3.53 |
| WCF1 (W) | 4.7 | 9.1 | 19.1 | 36.3 | 13–14 | <1 | 13–15 | 3.53 |
| WCF1 (E) | 4.2 | 8.3 | 18.8 | 35.4 | 13–14 | <1 | 13–15 | 3.53 |
| WCF2 | 4.5 | 9.0 | 20.5 | 40.0 | 12–13 | 1 | 13–14 | 3.49 |
| WCF3 | 4.9 | 9.6 | 20.6 | 38.4 | 13–14 | 1 | 14–15 | 3.46 |
| WCF3 (W) | 4.9 | 9.1 | 19.5 | 36.4 | 13–14 | 1 | 14–15 | 3.46 |
| WCF3 (E) | 4.9 | 10.4 | 22.7 | 42.2 | 13–14 | 1 | 14–15 | 3.46 |
| WCF4 | 4.8 | 9.6 | 20.6 | 39.0 | 13 | 1 | 14 | 3.43 |
| WCF5 | 4.8 | 9.9 | 21.7 | 41.3 | 14 | 1 | 15 | 3.11 |
| WCF6 | 5.1 | 10.4 | 22.3 | 42.7 | 14–15 | 2 | 16–17 | <3.11 |
| WCF7 | 4.9 | 10.3 | 23.0 | 44.6 | 15 | 2–3 | 17–18 | <WCF6 |
| Units | TBD (K) | FeO (wt %) | TiO2 (wt %) | FTA (wt %) | Age (Ga) | |||
|---|---|---|---|---|---|---|---|---|
| 3.0 GHz | 7.8 GHz | 19.35 GHz | 37 GHz | |||||
| ECF1 | 4.1 | 8.2 | 17.6 | 34 | 14 | 1 | 15 | 3.56 |
| ECF2 | 4.1 | 9 | 19.3 | 38.2 | 15 | 1.5 | 16.5 | 3.54 |
| ECF3 | 4.5 | 10.2 | 23.5 | 46.1 | 14 | 3 | 17 | 3.47 |
| ECF4 | 4 | 7.8 | 17.1 | 34.4 | 13 | 1 | 14 | 3.56 |
| ECF5 | 4.1 | 7.8 | 17.6 | 34 | 11–12 | 1 | 12–13 | 3.56 |
| ECF6 | 5 | 10.2 | 21.2 | 39.6 | 16 | 2 | 18 | <3.47 |
| Units | TBD (K) | FeO (wt %) | TiO2 (wt %) | FTA (wt %) | Age (Ga) | |||
|---|---|---|---|---|---|---|---|---|
| 3.0 GHz | 7.8 GHz | 19.35 GHz | 37 GHz | |||||
| EF2 | 4.5 | 10 | 21.7 | 41.3 | 14 | 1.5–2 | 15.5–16 | 3.64 |
| EF3 | 4 | 9.1 | 19.9 | 37.8 | 13 | 1–1.5 | 14–14.5 | 3.56 |
| EF4 | 3.8 | 8.1 | 18.5 | 36.2 | 13 | 1 | 14 | 3.14 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lei, J.; Meng, Z.; Wang, Y.; Huang, S.; Ping, J.; Cai, Z.; Zhang, Y. Potential Applications of CE-2 Microwave Radiometer Data in Understanding Basaltic Volcanism in Heavily Ejecta-Contaminated Mare Frigoris. Remote Sens. 2022, 14, 2725. https://doi.org/10.3390/rs14112725
Lei J, Meng Z, Wang Y, Huang S, Ping J, Cai Z, Zhang Y. Potential Applications of CE-2 Microwave Radiometer Data in Understanding Basaltic Volcanism in Heavily Ejecta-Contaminated Mare Frigoris. Remote Sensing. 2022; 14(11):2725. https://doi.org/10.3390/rs14112725
Chicago/Turabian StyleLei, Jietao, Zhiguo Meng, Yongzhi Wang, Shaopeng Huang, Jinsong Ping, Zhanchuan Cai, and Yuanzhi Zhang. 2022. "Potential Applications of CE-2 Microwave Radiometer Data in Understanding Basaltic Volcanism in Heavily Ejecta-Contaminated Mare Frigoris" Remote Sensing 14, no. 11: 2725. https://doi.org/10.3390/rs14112725
APA StyleLei, J., Meng, Z., Wang, Y., Huang, S., Ping, J., Cai, Z., & Zhang, Y. (2022). Potential Applications of CE-2 Microwave Radiometer Data in Understanding Basaltic Volcanism in Heavily Ejecta-Contaminated Mare Frigoris. Remote Sensing, 14(11), 2725. https://doi.org/10.3390/rs14112725









