Galileo Time Transfer with Five-Frequency Uncombined PPP: A Posteriori Weighting, Inter-Frequency Bias, Precise Products and Multi-Frequency Contribution
Abstract
:1. Introduction
2. Methods
2.1. Galileo Five-Frequency Uncombined PPP Model for Time Transfer
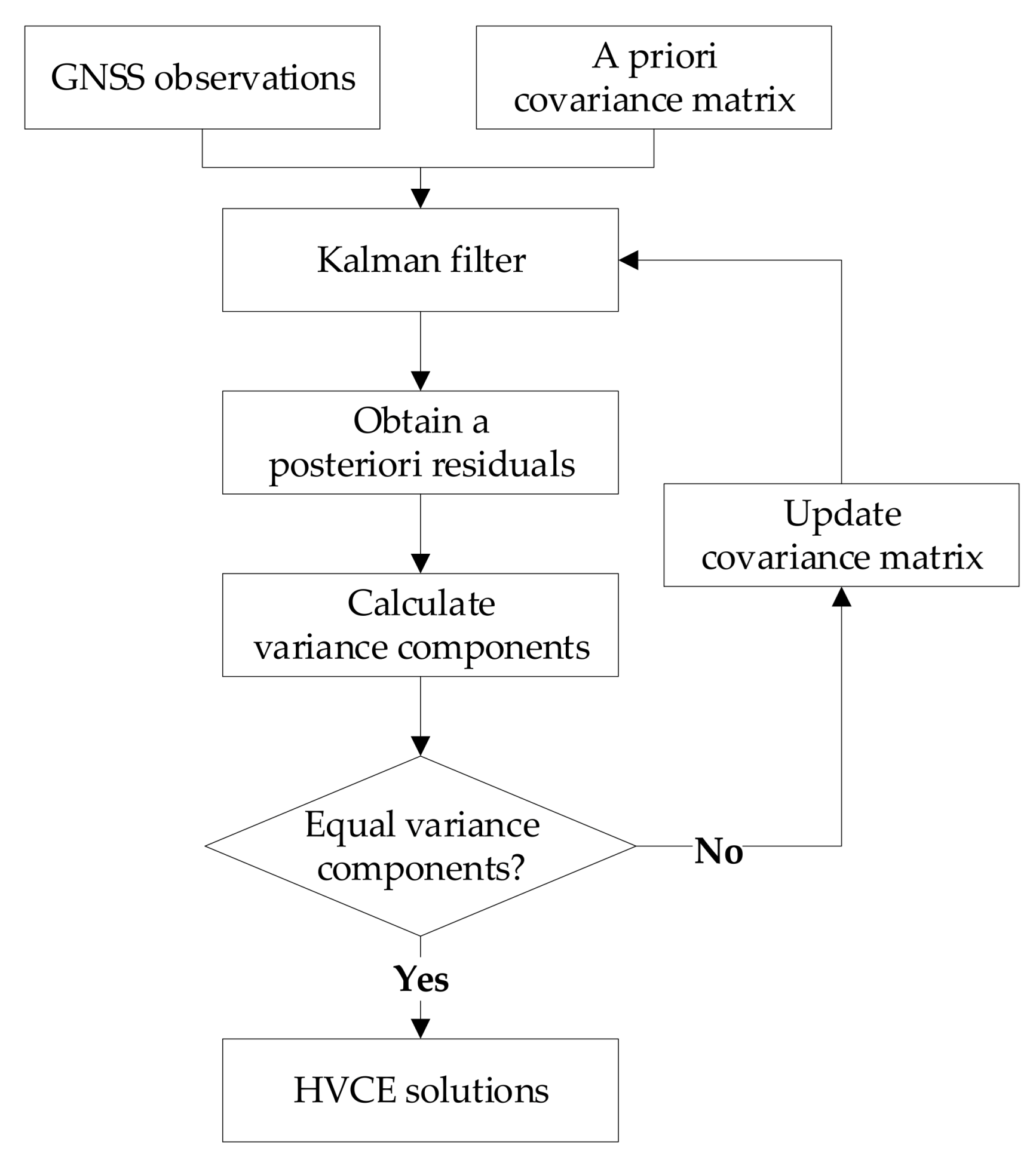
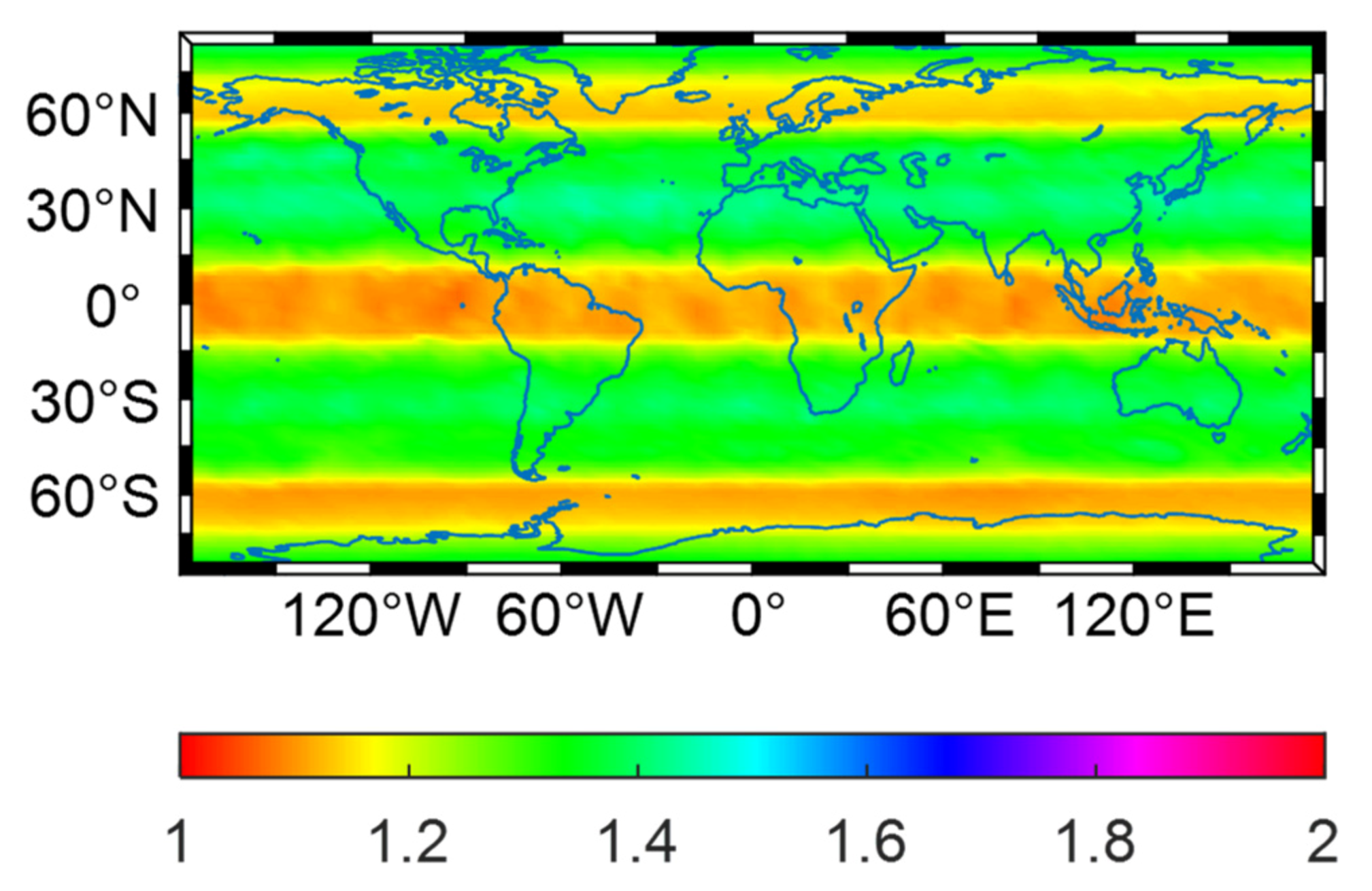
2.2. Helmert Variance Component Estimation Method for Kalman Filter
3. Results
3.1. Datasets and Processing Strategies
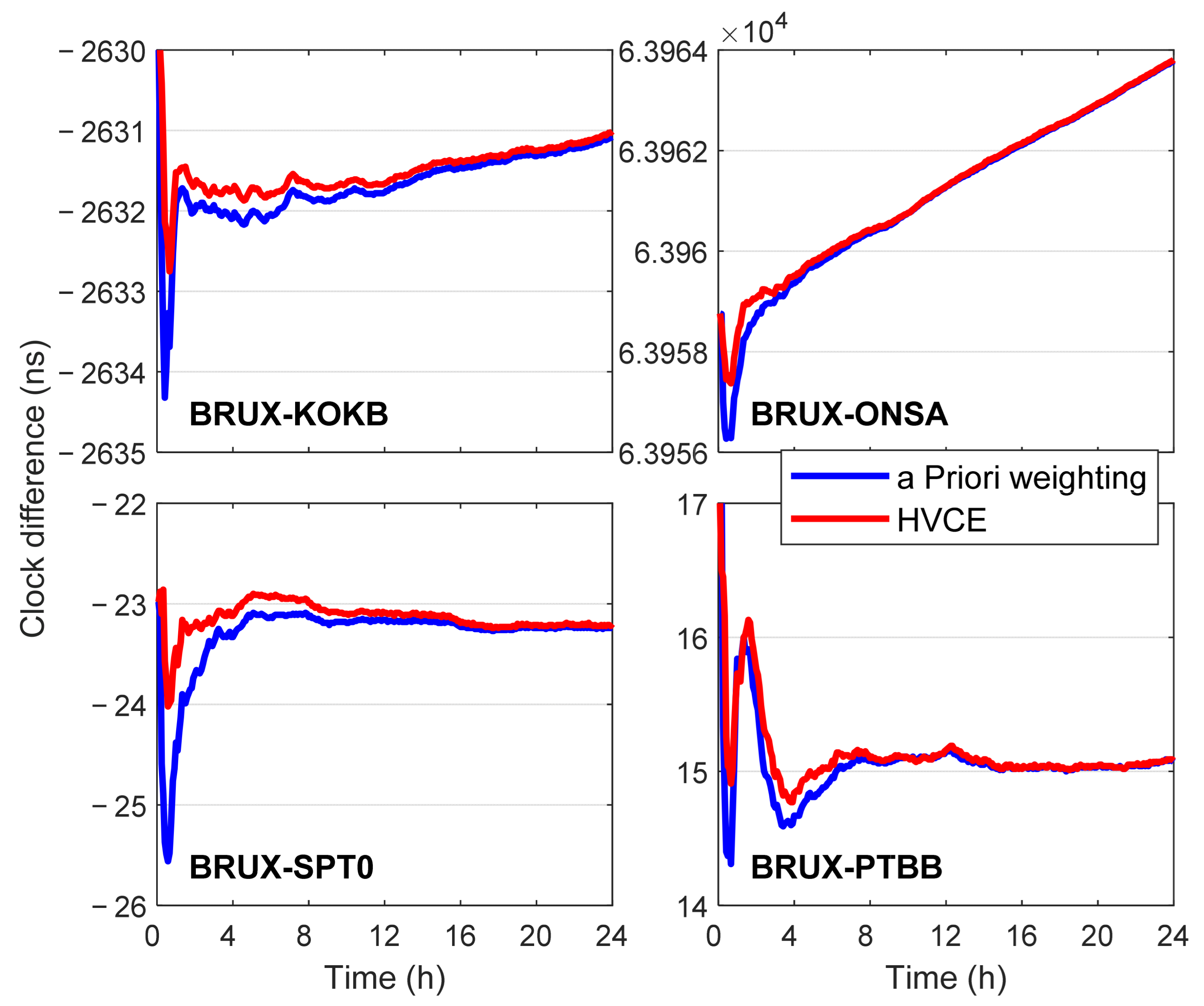
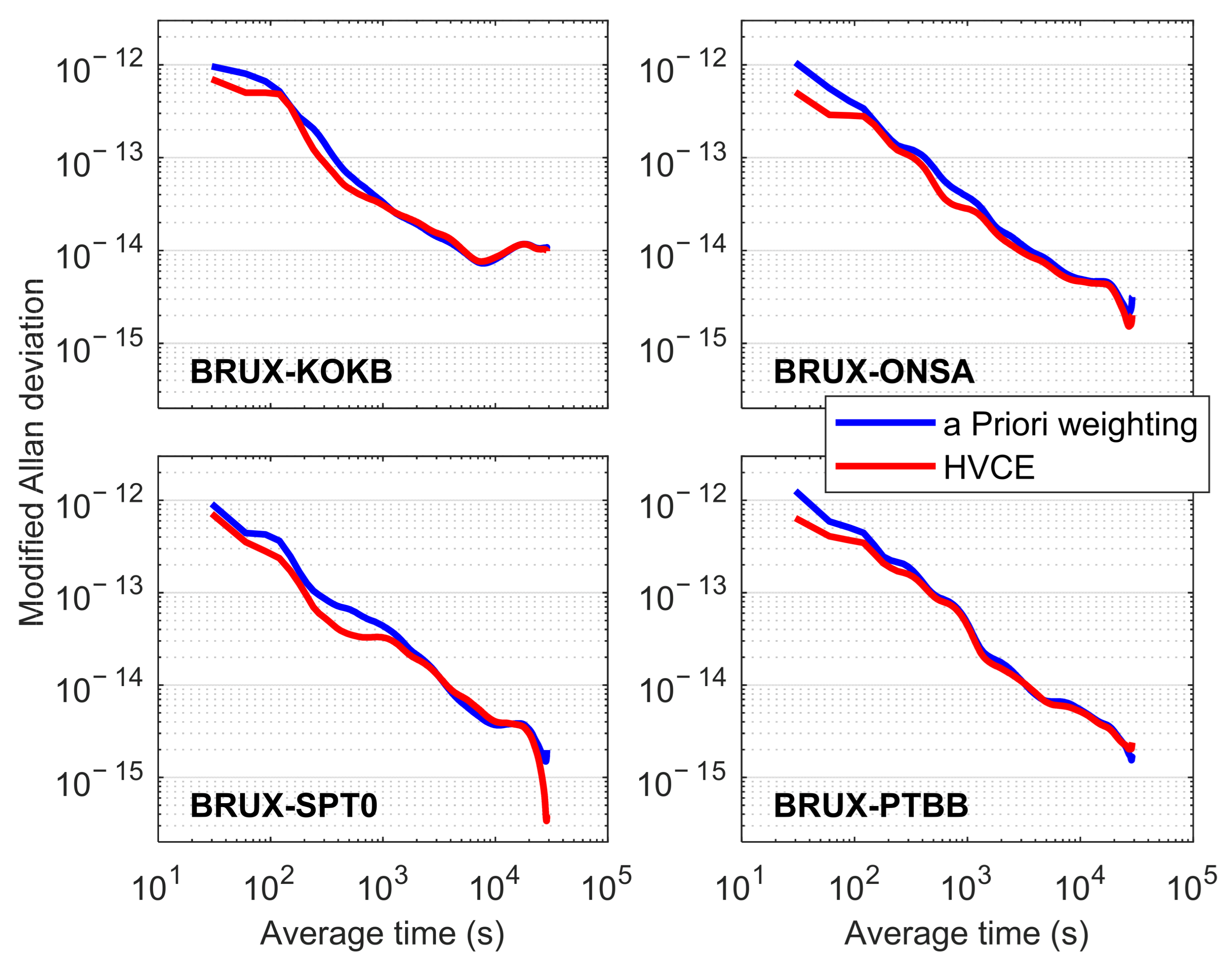
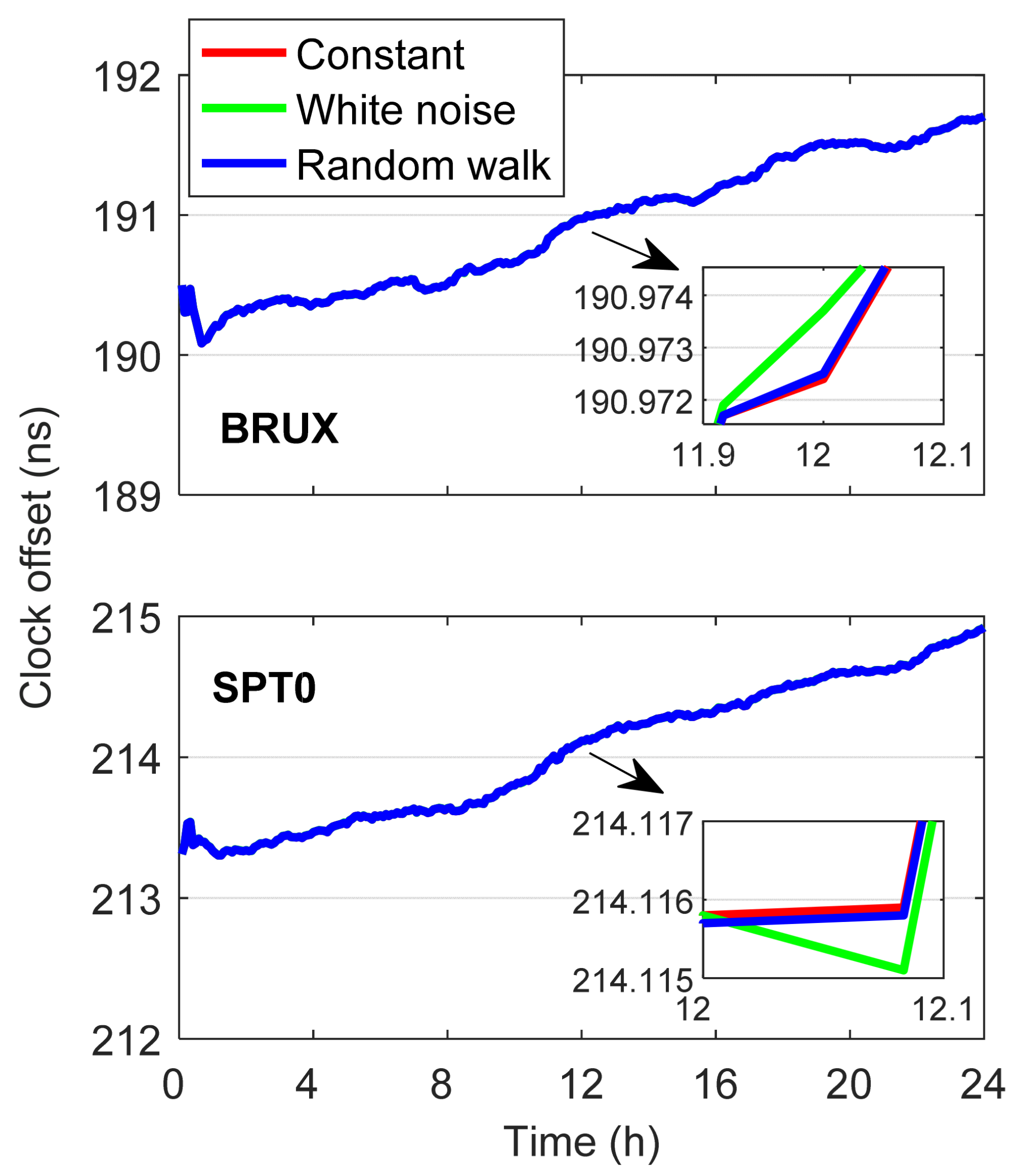
3.2. Time Transfer Results with HVCE Method
3.3. Time Transfer Results with Different IFB Dynamic Models
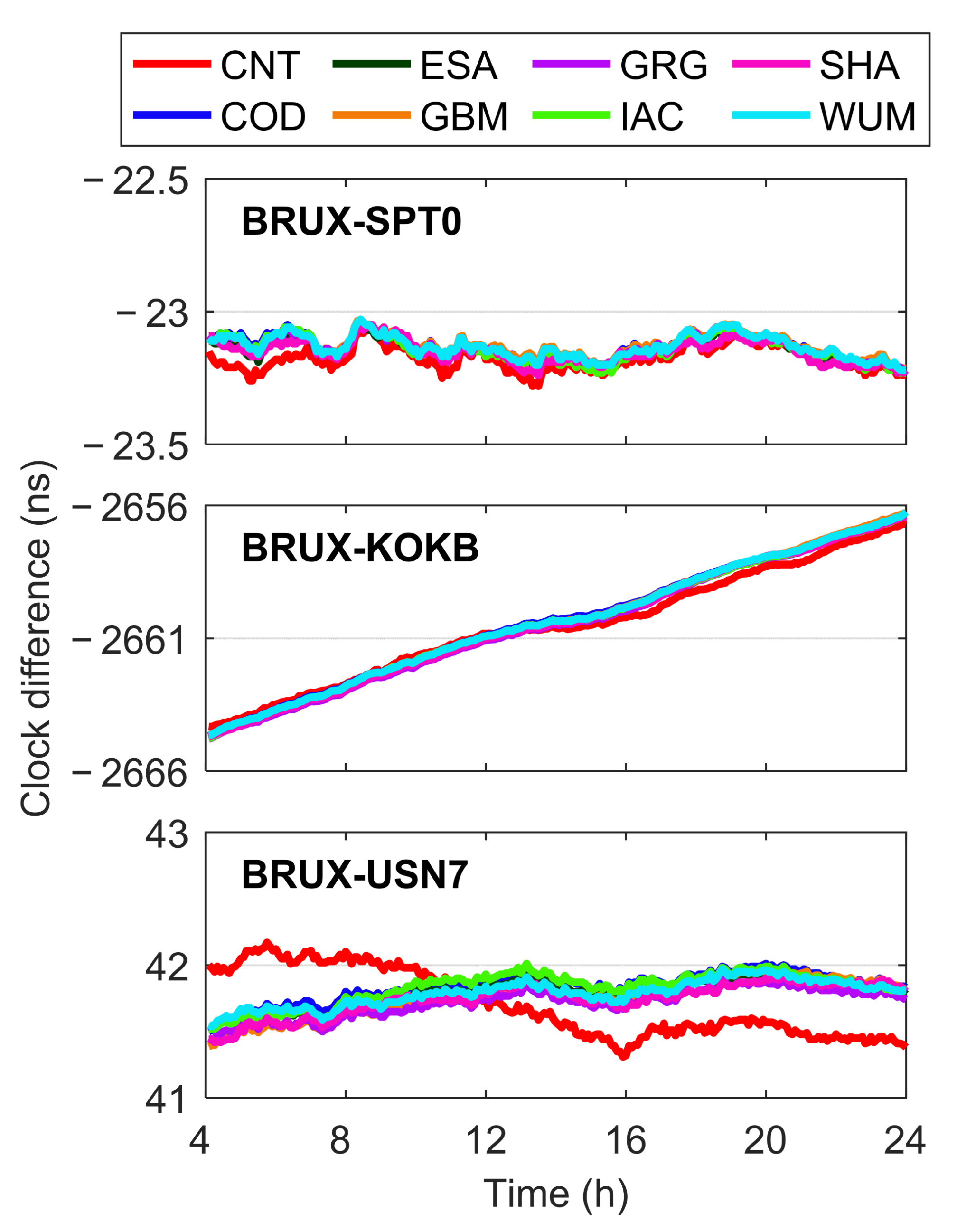
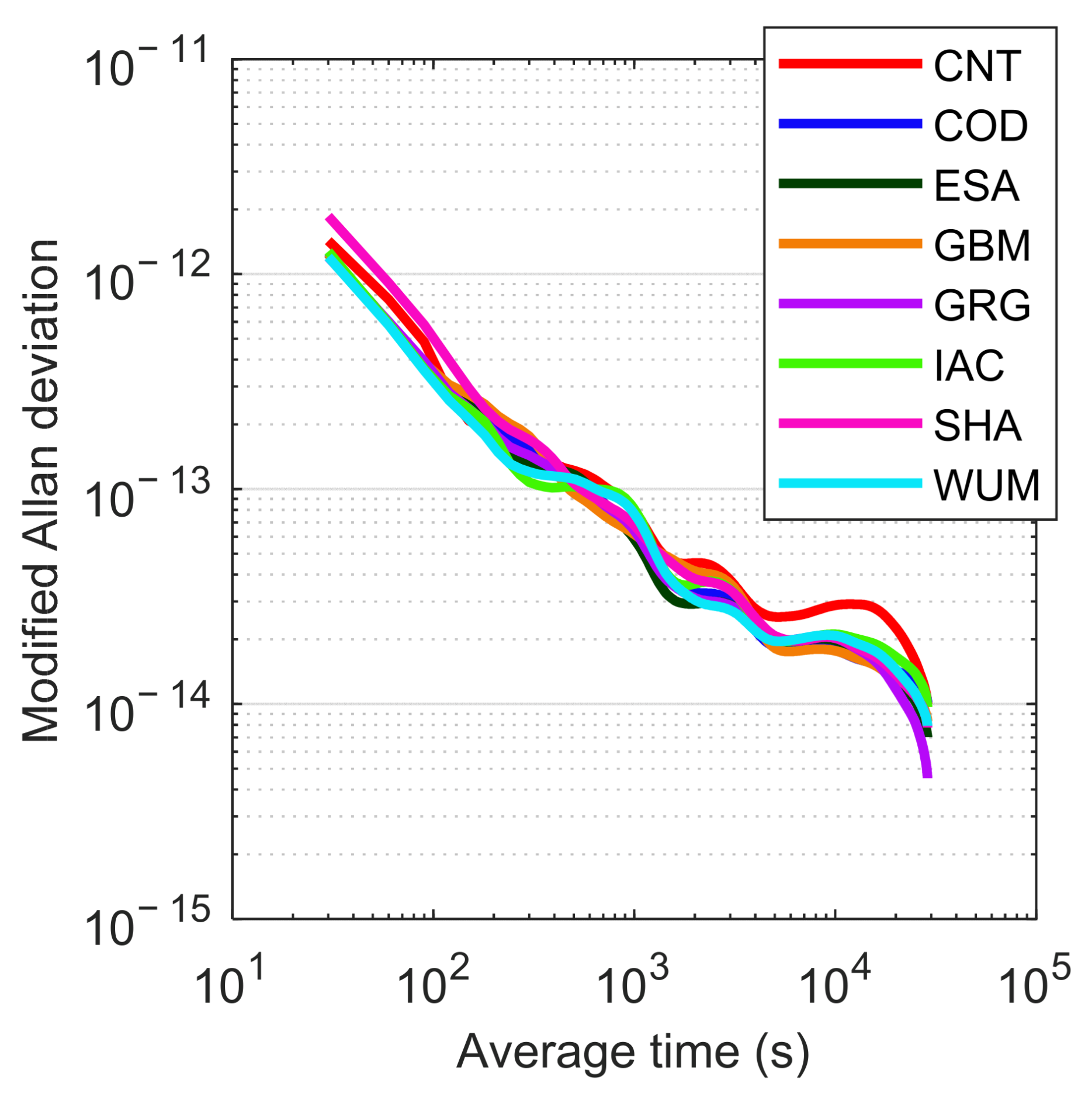
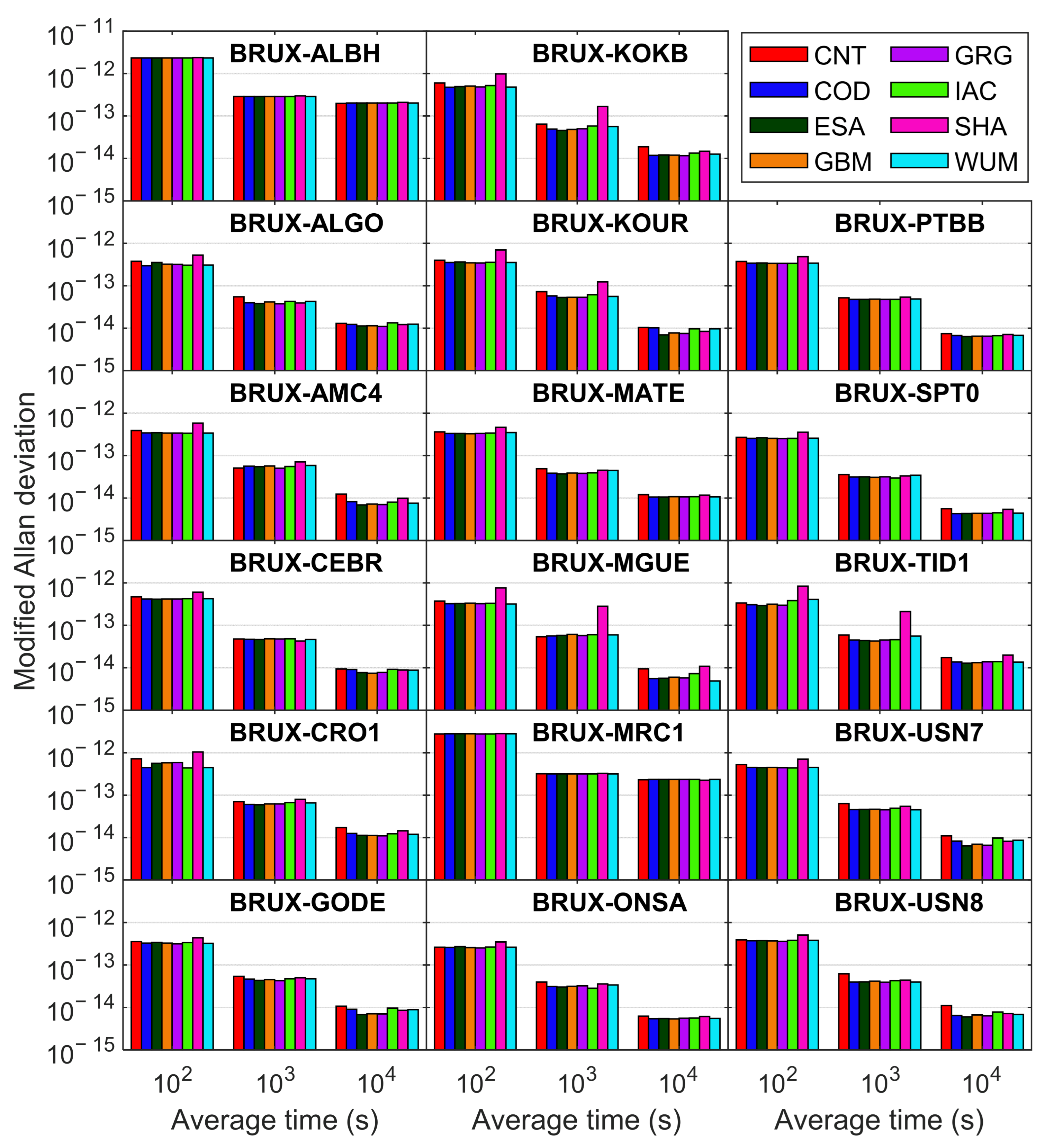
3.4. Time Transfer Results with Precise Products from Different Analysis Centers
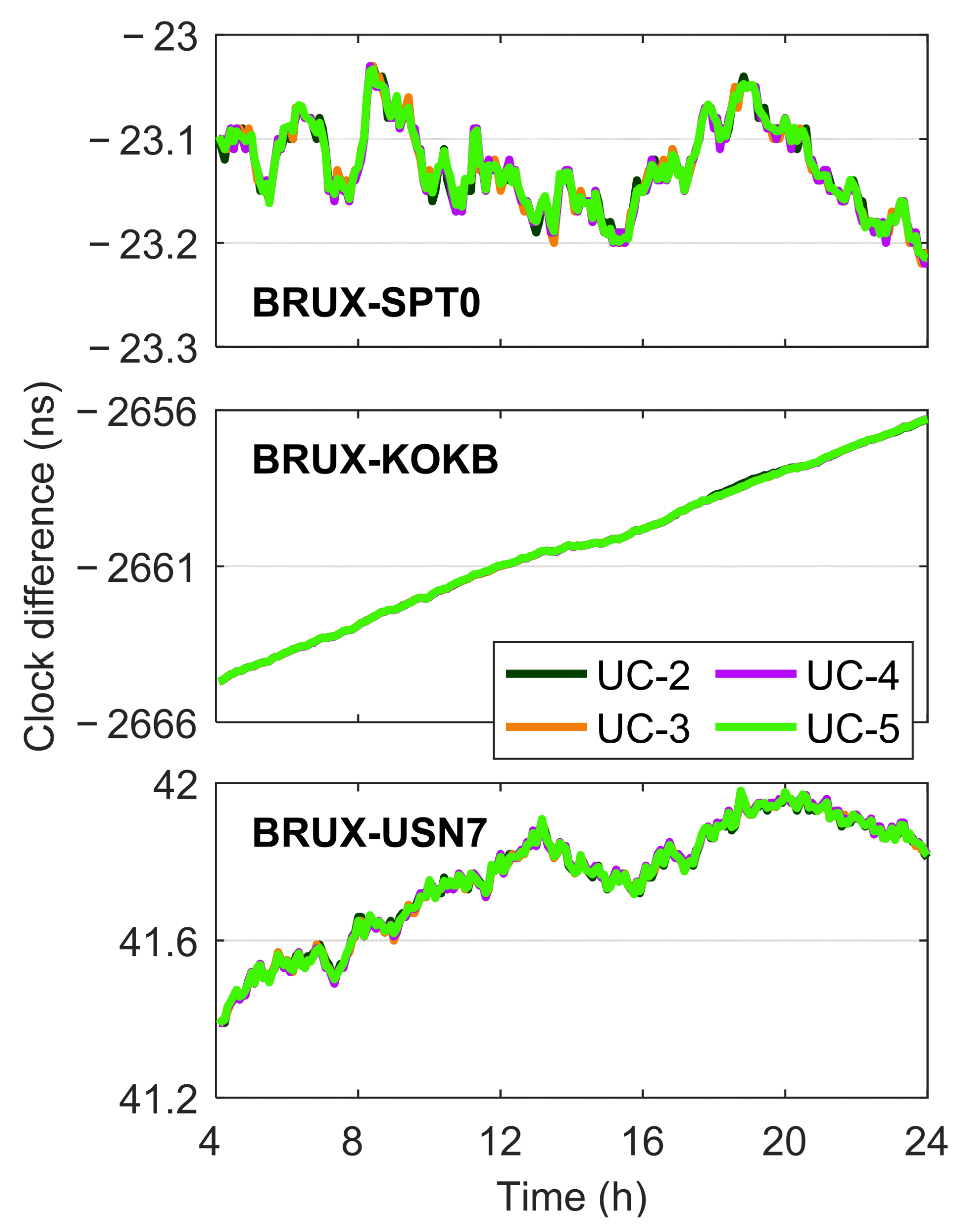
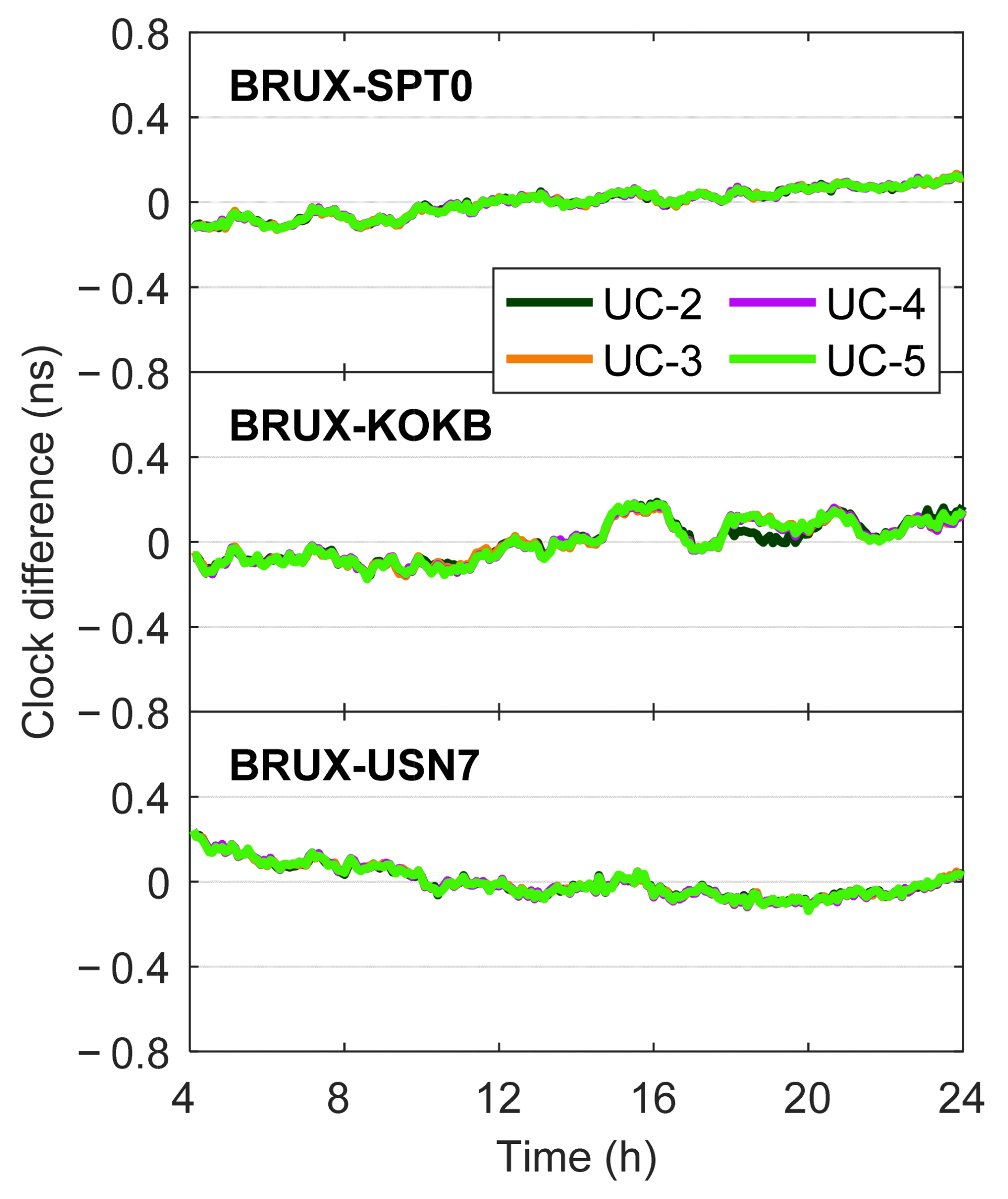
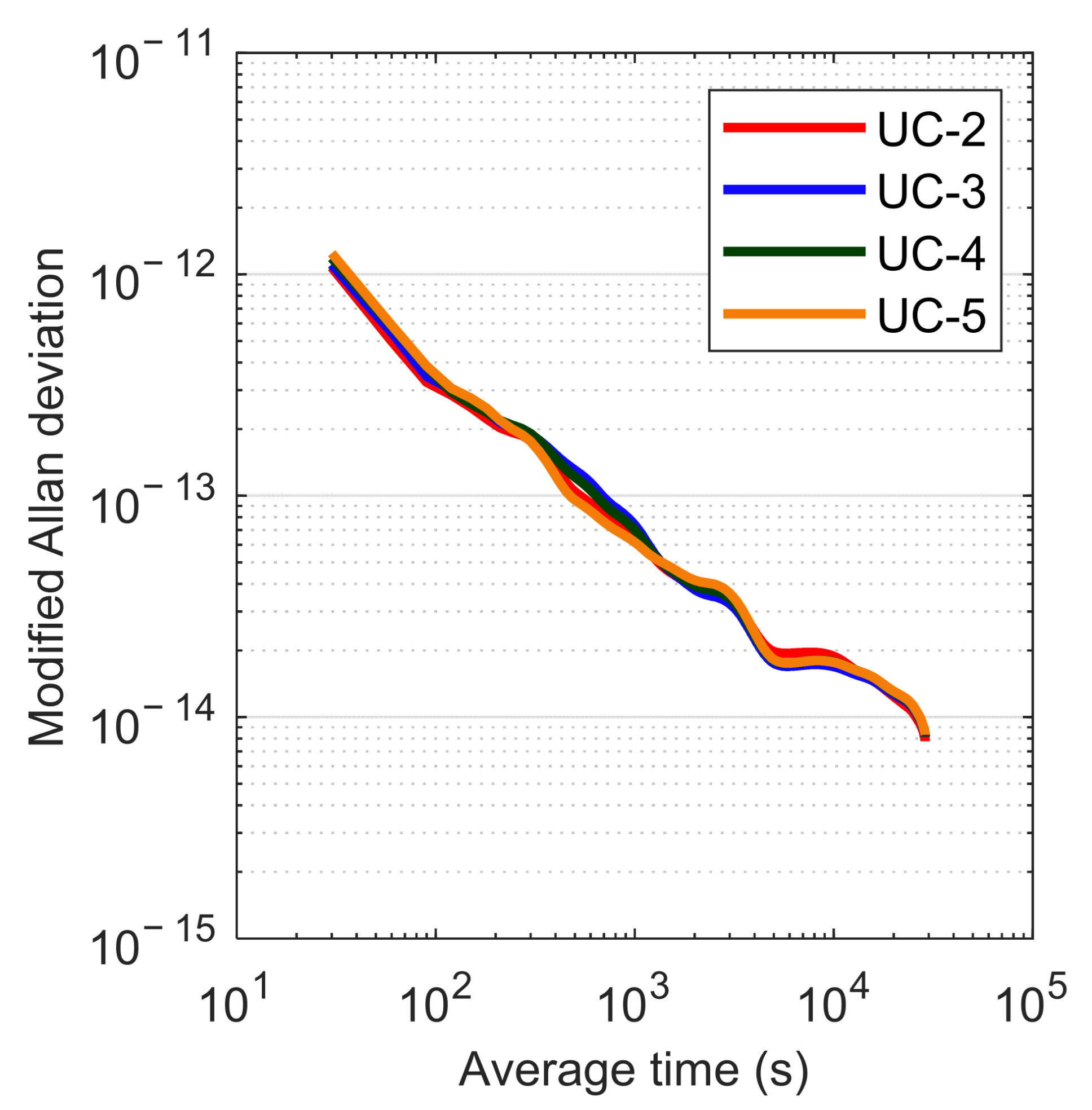
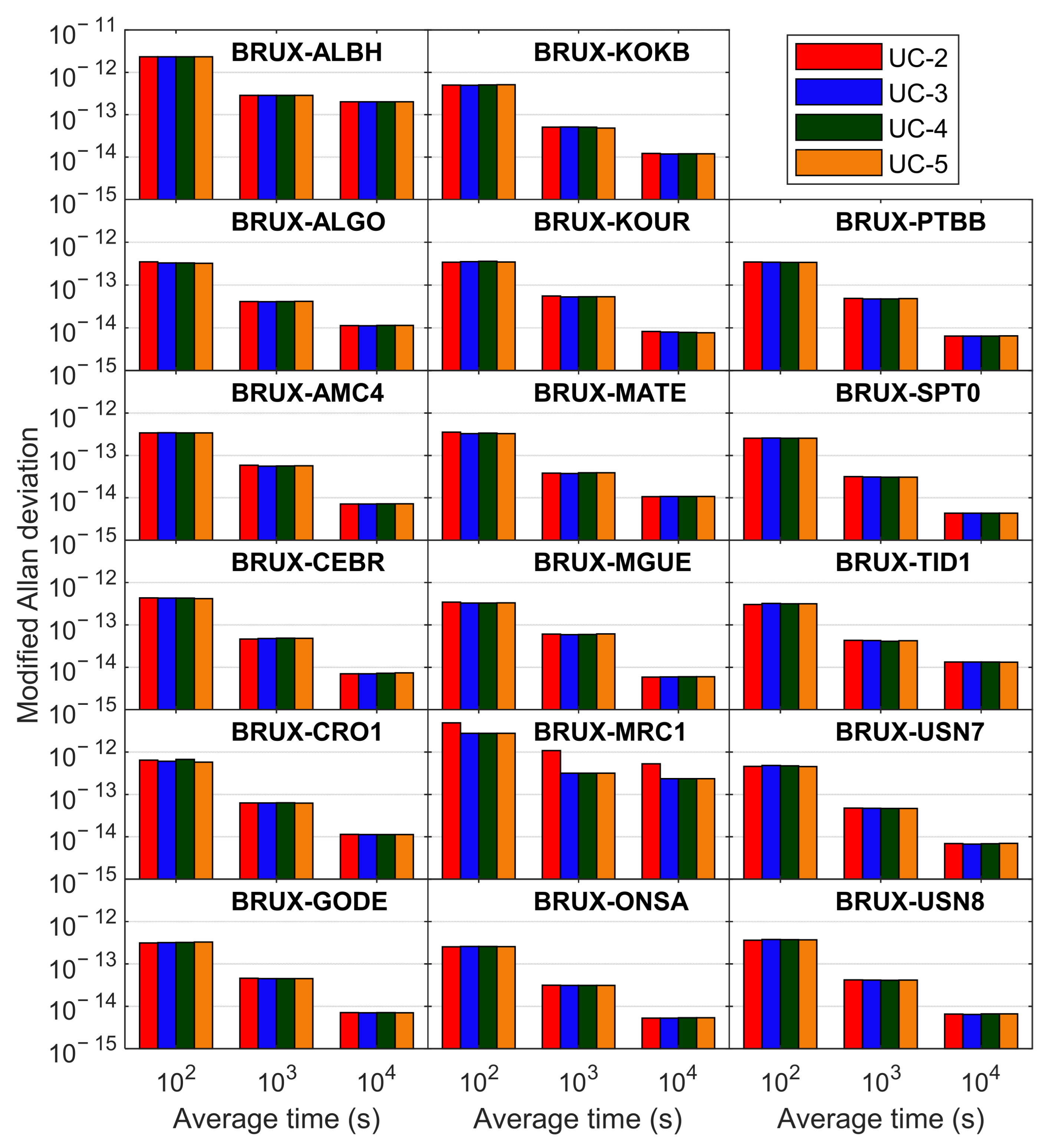
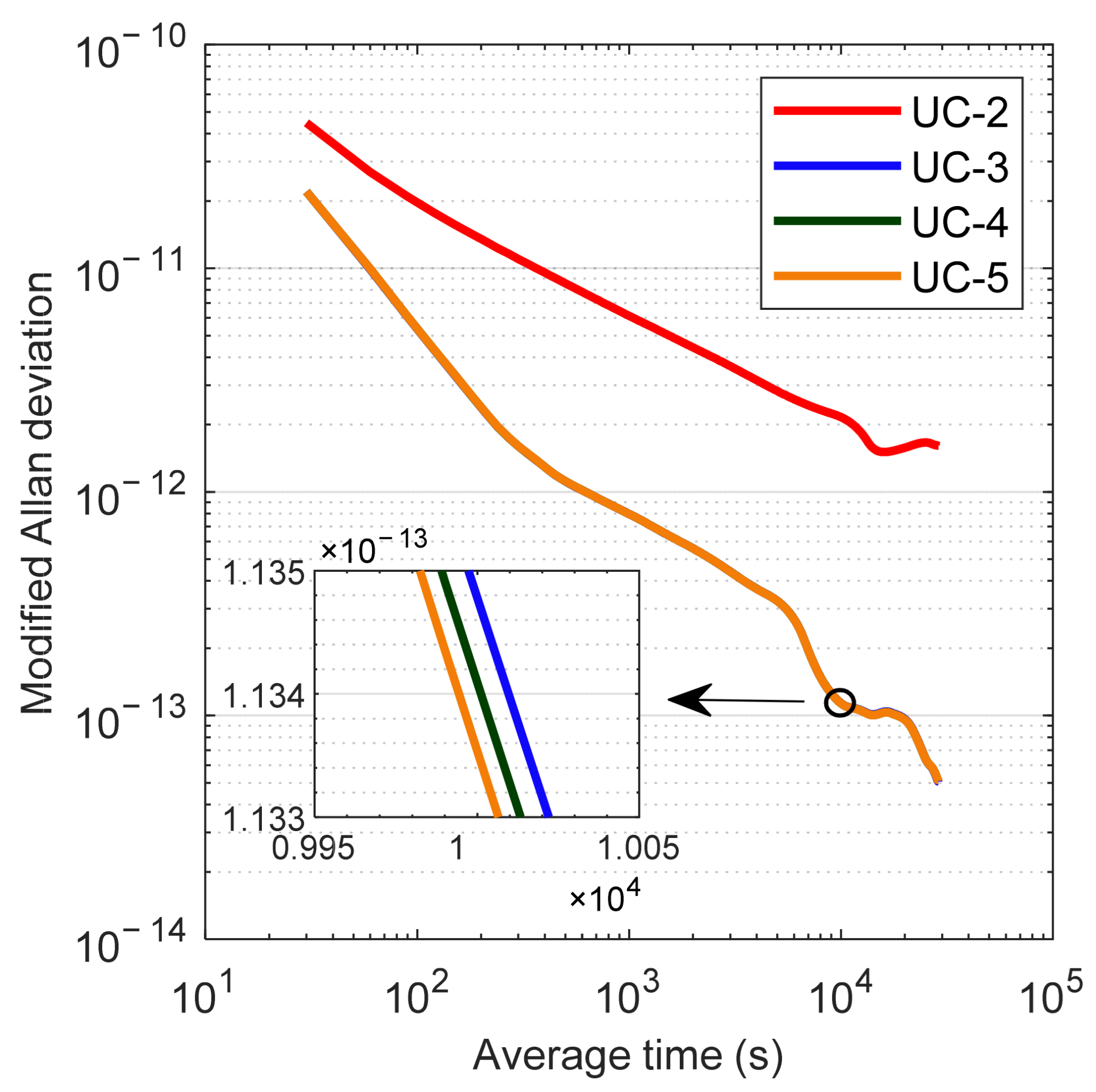
3.5. Time Transfer Results with Galileo Dual-, Triple-, Four- and Five-Frequency UC PPP
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ouyang, M.; Li, J.; Li, W.; Ge, Y.; Zhu, X.; Chen, Z.; Dai, Z. Research on Time and Frequency Transfer during PPP Convergence with Parameters Correlation Comparison. Measurement 2021, 173, 108597. [Google Scholar] [CrossRef]
- Jiang, Z.; Petit, G. Combination of TWSTFT and GNSS for Accurate UTC Time Transfer. Metrologia 2009, 46, 305–314. [Google Scholar] [CrossRef]
- Defraigne, P.; Aerts, W.; Pottiaux, E. Monitoring of UTC(k)’s Using PPP and IGS Real-Time Products. GPS Solut. 2015, 19, 165–172. [Google Scholar] [CrossRef]
- Petit, G.; Jiang, Z. Precise Point Positioning for TAI Computation. Int. J. Navig. Obs. 2008, 2008, 562878. [Google Scholar] [CrossRef]
- Petit, G. Sub-10–16 Accuracy GNSS Frequency Transfer with IPPP. GPS Solut. 2021, 25, 22. [Google Scholar] [CrossRef]
- Petit, G.; Kanj, A.; Loyer, S.; Delporte, J.; Mercier, F.; Perosanz, F. 1 × 10−16 Frequency Transfer by GPS PPP with Integer Ambiguity Resolution. Metrologia 2015, 52, 301–309. [Google Scholar] [CrossRef]
- Ge, Y.; Qin, W.; Cao, X.; Zhou, F.; Wang, S.; Yang, X. Consideration of GLONASS Inter-Frequency Code Biases in Precise Point Positioning (PPP) International Time Transfer. Appl. Sci. 2018, 8, 1254. [Google Scholar] [CrossRef] [Green Version]
- Xu, W.; Shen, W.; Cai, C.; Li, L.; Wang, L.; Ning, A.; Shen, Z. Comparison and Evaluation of Carrier Phase PPP and Single Difference Time Transfer with Multi-GNSS Ambiguity Resolution. GPS Solut. 2022, 26, 58. [Google Scholar] [CrossRef]
- Zhang, P.; Tu, R.; Zhang, R.; Gao, Y.; Cai, H. Combining GPS, BeiDou, and Galileo Satellite Systems for Time and Frequency Transfer Based on Carrier Phase Observations. Remote Sens. 2018, 10, 324. [Google Scholar] [CrossRef] [Green Version]
- Ge, Y.; Zhou, F.; Dai, P.; Qin, W.; Wang, S.; Yang, X. Precise Point Positioning Time Transfer with Multi-GNSS Single-Frequency Observations. Measurement 2019, 146, 628–642. [Google Scholar] [CrossRef]
- Ge, Y.; Dai, P.; Qin, W.; Yang, X.; Zhou, F.; Wang, S.; Zhao, X. Performance of Multi-GNSS Precise Point Positioning Time and Frequency Transfer with Clock Modeling. Remote Sens. 2019, 11, 347. [Google Scholar] [CrossRef] [Green Version]
- Lyu, D.; Zeng, F.; Ouyang, X. Time Transfer Algorithm Using Multi-GNSS PPP with Ambiguity Resolution. Chin. Astron. Astrophys. 2020, 44, 371–382. [Google Scholar]
- Tu, R.; Zhang, P.; Zhang, R.; Liu, J.; Lu, X. Modeling and Performance Analysis of Precise Time Transfer Based on BDS Triple-Frequency Un-Combined Observations. J. Geod. 2019, 93, 837–847. [Google Scholar] [CrossRef]
- Su, K.; Jin, S. Triple-Frequency Carrier Phase Precise Time and Frequency Transfer Models for BDS-3. GPS Solut. 2019, 23, 86. [Google Scholar] [CrossRef]
- Zhang, P.; Tu, R.; Gao, Y.; Zhang, R.; Han, J. Performance of Galileo Precise Time and Frequency Transfer Models Using Quad-Frequency Carrier Phase Observations. GPS Solut. 2020, 24, 40. [Google Scholar] [CrossRef]
- Ge, Y.; Cao, X.; Shen, F.; Yang, X.; Wang, S. BDS-3/Galileo Time and Frequency Transfer with Quad-Frequency Precise Point Positioning. Remote Sens. 2021, 13, 2704. [Google Scholar] [CrossRef]
- Prochniewicz, D.; Grzymala, M. Analysis of the Impact of Multipath on Galileo System Measurements. Remote Sens. 2021, 13, 2295. [Google Scholar] [CrossRef]
- Li, X.; Li, X.; Liu, G.; Xie, W.; Guo, F.; Yuan, Y.; Zhang, K.; Feng, G. The Phase and Code Biases of Galileo and BDS-3 BOC Signals: Effect on Ambiguity Resolution and Precise Positioning. J. Geod. 2020, 94, 9. [Google Scholar] [CrossRef]
- Steigenberger, P.; Montenbruck, O. Galileo Status: Orbits, Clocks, and Positioning. GPS Solut. 2017, 21, 319–331. [Google Scholar] [CrossRef]
- Wang, N.; Yuan, Y.; Li, Z.; Montenbruck, O.; Tan, B. Determination of Differential Code Biases with Multi-GNSS Observations. J. Geod. 2016, 90, 209–228. [Google Scholar] [CrossRef]
- Satirapod, C.; Luansang, M. Comparing Stochastic Models Used in GPS Precise Point Positioning Technique. Surv. Rev. 2008, 40, 188–194. [Google Scholar] [CrossRef]
- Gao, Z.; Shen, W.; Zhang, H.; Ge, M.; Niu, X. Application of Helmert Variance Component Based Adaptive Kalman Filter in Multi-GNSS PPP/INS Tightly Coupled Integration. Remote Sens. 2016, 8, 553. [Google Scholar] [CrossRef]
- Li, M.; Nie, W.; Xu, T.; Rovira-Garcia, A.; Fang, Z.; Xu, G. Helmert Variance Component Estimation for Multi-GNSS Relative Positioning. Sensors 2020, 20, 669. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yang, Y.; Xu, T.; Song, L. Robust Estimation of Variance Components with Application in Global Positioning System Network Adjustment. J. Surv. Eng. 2005, 131, 107–112. [Google Scholar] [CrossRef]
- European Global Navigation Satellite Systems Agency. The Current Constellation of the Galileo. Available online: https://www.gsc-europa.eu/system-service-status/constellation-information (accessed on 20 April 2022).
- Zhang, P.; Tu, R.; Gao, Y.; Zhang, R.; Liu, N. Improving the Performance of Multi-GNSS Time and Frequency Transfer Using Robust Helmert Variance Component Estimation. Sensors 2018, 18, 2878. [Google Scholar] [CrossRef] [PubMed] [Green Version]





| Technology | Basic Idea | Accuracy | Advantage | Disadvantage |
|---|---|---|---|---|
| CV | Utilizing observations of enough common-view satellites from two stations, and cancelling out or weakening many errors through single-difference between stations | 1–10 ns | Low cost and simple data processing | The time transfer accuracy degrades with the increasing distance between the two stations, and is also limited by the precision of code observations |
| AV | Measuring the time differences between two stations with the code observations from all observed satellites as well as the precise satellite orbit and clock offset products | Better than CV method | Low cost and free of the negative effects of the distance between stations | The implementation needs to rely on the precise satellite orbit and clock offset products, and the time transfer accuracy is limited by the precision of code observations |
| TWSTFT | Exchanging time signals between two ground stations through a geostationary satellite, and cancelling out many delay variations of the transmitted signals due to the reciprocity of the propagation path | Higher than AV method | High accuracy, and high long-term frequency stability | This method needs the support of communication satellites and the employed equipment must have the ability to communicate with satellites, which limits its applications |
| CP | Employing the precise carrier phase observations as well as the precise satellite orbit and clock offset products to conduct the time transfer through the precise point positioning technology | A sub-nanosecond level | Low cost, free of the negative effects of the distance between stations, high accuracy, and high short-term frequency stability | The implementation needs to rely on the precise satellite orbit and clock offset products |
| Institution | ID | Orbit | Clock |
|---|---|---|---|
| CODE | COD0MGXFIN | 5 min | 30 s |
| GFZ | GBM0MGXRAP | 5 min | 30 s |
| WHU | WUM0MGXFIN | 15 min | 30 s |
| SHAO | SHA0MGXRAP | 5 min | 5 min |
| IAC | IAC0MGXFIN | 5 min | 30 s |
| ESA | ESA0MGNFIN | 5 min | 30 s |
| CNES | GRG0MGXFIN | 5 min | 30 s |
| CNT | 5 min | 5 s |
| Average Time (s) | Modified Allan Deviation | |||||||
|---|---|---|---|---|---|---|---|---|
| BRUX-KOKB | BRUX-ONSA | BRUX-SPT0 | BRUX-PTBB | |||||
| A Priori | HVCE | A Priori | HVCE | A Priori | HVCE | A Priori | HVCE | |
| 30 | 9.650 × 10−13 | 7.006 × 10−13 | 1.061 × 10−12 | 5.090 × 10−13 | 9.162 × 10−13 | 7.169 × 10−13 | 1.263 × 10−12 | 6.434 × 10−13 |
| 60 | 8.007 × 10−13 | 5.006 × 10−13 | 5.588 × 10−13 | 2.888 × 10−13 | 4.422 × 10−13 | 3.540 × 10−13 | 5.899 × 10−13 | 4.074 × 10−13 |
| 120 | 5.182 × 10−13 | 4.828 × 10−13 | 3.386 × 10−13 | 2.789 × 10−13 | 3.640 × 10−13 | 2.356 × 10−13 | 4.455 × 10−13 | 3.470 × 10−13 |
| 240 | 2.050 × 10−13 | 1.233 × 10−13 | 1.353 × 10−13 | 1.202 × 10−13 | 1.048 × 10−13 | 6.975 × 10−14 | 2.137 × 10−13 | 1.712 × 10−13 |
| 480 | 6.794 × 10−14 | 4.809 × 10−14 | 8.353 × 10−14 | 5.872 × 10−14 | 6.722 × 10−14 | 3.617 × 10−14 | 1.008 × 10−13 | 9.569 × 10−14 |
| 960 | 3.444 × 10−14 | 3.174 × 10−14 | 3.901 × 10−14 | 2.849 × 10−14 | 4.491 × 10−14 | 3.296 × 10−14 | 5.091 × 10−14 | 4.818 × 10−14 |
| 1920 | 1.969 × 10−14 | 2.080 × 10−14 | 1.677 × 10−14 | 1.419 × 10−14 | 2.201 × 10−14 | 1.957 × 10−14 | 1.796 × 10−14 | 1.550 × 10−14 |
| 3840 | 1.239 × 10−14 | 1.369 × 10−14 | 9.514 × 10−15 | 8.338 × 10−15 | 9.108 × 10−15 | 9.473 × 10−15 | 8.254 × 10−15 | 8.604 × 10−15 |
| 7680 | 7.280 × 10−15 | 7.627 × 10−15 | 5.543 × 10−15 | 5.028 × 10−15 | 4.300 × 10−15 | 5.054 × 10−15 | 6.399 × 10−15 | 5.832 × 10−15 |
| 15,360 | 1.110 × 10−14 | 1.114 × 10−14 | 4.651 × 10−15 | 4.398 × 10−15 | 3.835 × 10−15 | 3.738 × 10−15 | 3.901 × 10−15 | 3.716 × 10−15 |
| 28,800 | 1.079 × 10−14 | 9.842 × 10−15 | 3.168 × 10−15 | 2.016 × 10−15 | 1.978 × 10−15 | 3.568 × 10−16 | 1.730 × 10−15 | 2.396 × 10−15 |
| Products | STD (ns) | |||
|---|---|---|---|---|
| BRUX-SPT0 | BRUX-KOKB | BRUX-USN7 | Mean | |
| CNT | 0.054 | 0.388 | 0.317 | 0.253 |
| COD | 0.071 | 0.136 | 0.056 | 0.088 |
| ESA | 0.066 | 0.126 | 0.046 | 0.079 |
| GBM | 0.066 | 0.095 | 0.076 | 0.079 |
| GRG | 0.069 | 0.103 | 0.045 | 0.072 |
| IAC | 0.074 | 0.154 | 0.070 | 0.099 |
| SHA | 0.070 | 0.114 | 0.054 | 0.079 |
| WUM | 0.068 | 0.139 | 0.041 | 0.083 |
| Products | Mean Modified Allan Deviation | ||
|---|---|---|---|
| 90 s | 990 s | 9990 s | |
| CNT | 6.648 × 10−13 | 8.460 × 10−14 | 3.541 × 10−14 |
| COD | 6.125 × 10−13 | 7.630 × 10−14 | 3.367 × 10−14 |
| ESA | 6.257 × 10−13 | 7.529 × 10−14 | 3.283 × 10−14 |
| GBM | 6.235 × 10−13 | 7.661 × 10−14 | 3.301 × 10−14 |
| GRG | 6.185 × 10−13 | 7.562 × 10−14 | 3.296 × 10−14 |
| IAC | 6.212 × 10−13 | 7.814 × 10−14 | 3.406 × 10−14 |
| SHA | 8.539 × 10−13 | 1.152 × 10−13 | 3.459 × 10−14 |
| WUM | 6.207 × 10−13 | 7.881 × 10−14 | 3.355 × 10−14 |
| Strategy | STD (ns) | ||
|---|---|---|---|
| BRUX-SPT0 | BRUX-KOKB | BRUX-USN7 | |
| UC-2 | 0.066 | 0.090 | 0.075 |
| UC-3 | 0.067 | 0.092 | 0.077 |
| UC-4 | 0.067 | 0.094 | 0.077 |
| UC-5 | 0.066 | 0.095 | 0.076 |
| Strategy | Mean Modified Allan Deviation | ||
|---|---|---|---|
| 90 s | 990 s | 9990 s | |
| UC-2 | 4.955 × 10−13 | 6.198 × 10−14 | 2.034 × 10−14 |
| UC-3 | 4.944 × 10−13 | 6.132 × 10−14 | 2.026 × 10−14 |
| UC-4 | 4.983 × 10−13 | 6.149 × 10−14 | 2.032 × 10−14 |
| UC-5 | 4.885 × 10−13 | 6.155 × 10−14 | 2.033 × 10−14 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Z.; Pan, L. Galileo Time Transfer with Five-Frequency Uncombined PPP: A Posteriori Weighting, Inter-Frequency Bias, Precise Products and Multi-Frequency Contribution. Remote Sens. 2022, 14, 2538. https://doi.org/10.3390/rs14112538
Zhang Z, Pan L. Galileo Time Transfer with Five-Frequency Uncombined PPP: A Posteriori Weighting, Inter-Frequency Bias, Precise Products and Multi-Frequency Contribution. Remote Sensing. 2022; 14(11):2538. https://doi.org/10.3390/rs14112538
Chicago/Turabian StyleZhang, Zhehao, and Lin Pan. 2022. "Galileo Time Transfer with Five-Frequency Uncombined PPP: A Posteriori Weighting, Inter-Frequency Bias, Precise Products and Multi-Frequency Contribution" Remote Sensing 14, no. 11: 2538. https://doi.org/10.3390/rs14112538
APA StyleZhang, Z., & Pan, L. (2022). Galileo Time Transfer with Five-Frequency Uncombined PPP: A Posteriori Weighting, Inter-Frequency Bias, Precise Products and Multi-Frequency Contribution. Remote Sensing, 14(11), 2538. https://doi.org/10.3390/rs14112538







