High-Accuracy Real-Time Kinematic Positioning with Multiple Rover Receivers Sharing Common Clock
Abstract
1. Introduction
2. Methodology
2.1. IRB-Float Model
2.2. IRB-Fixed Model
3. Redundancy and Solvability Analysis
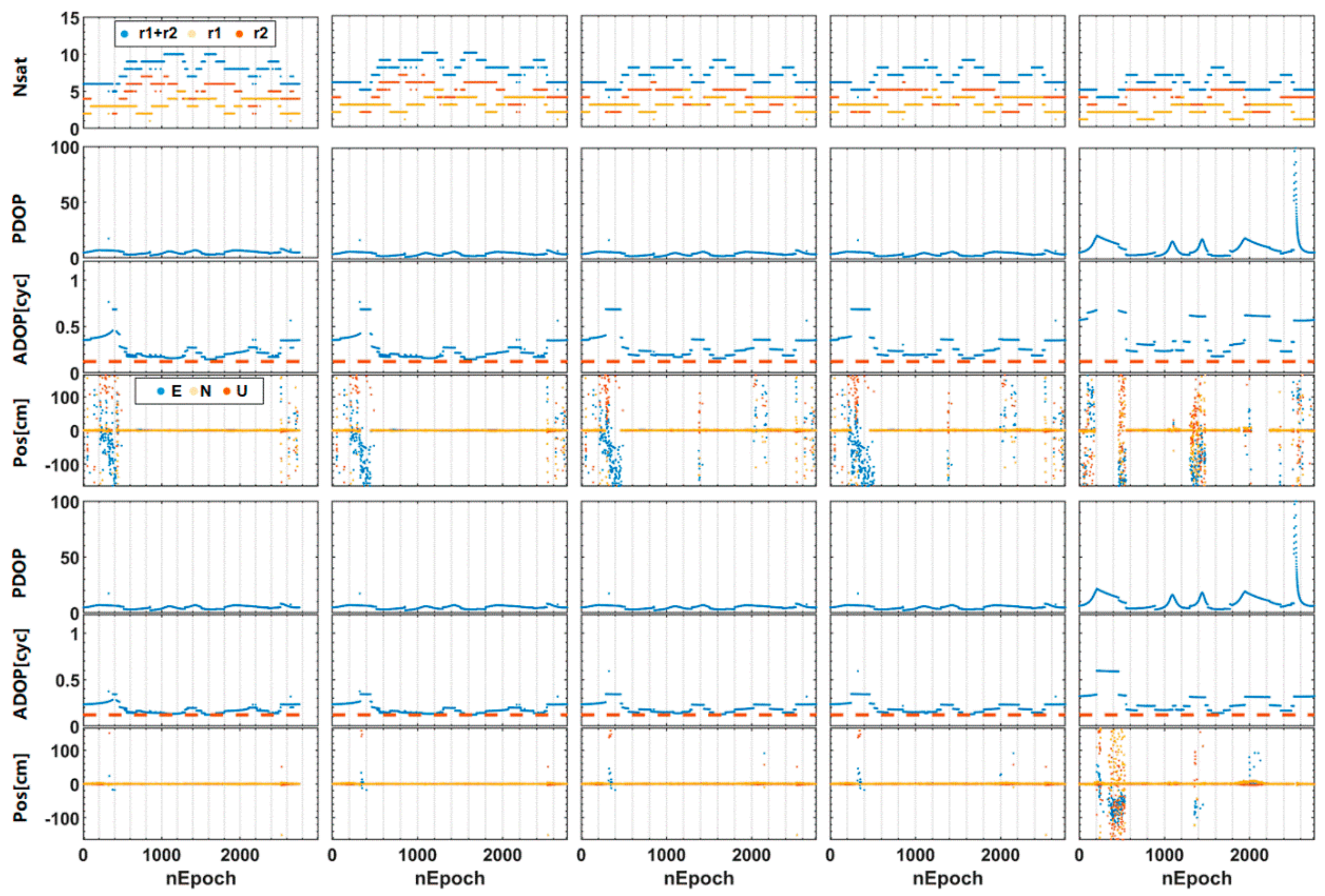
4. Experimental Analysis and Discussion
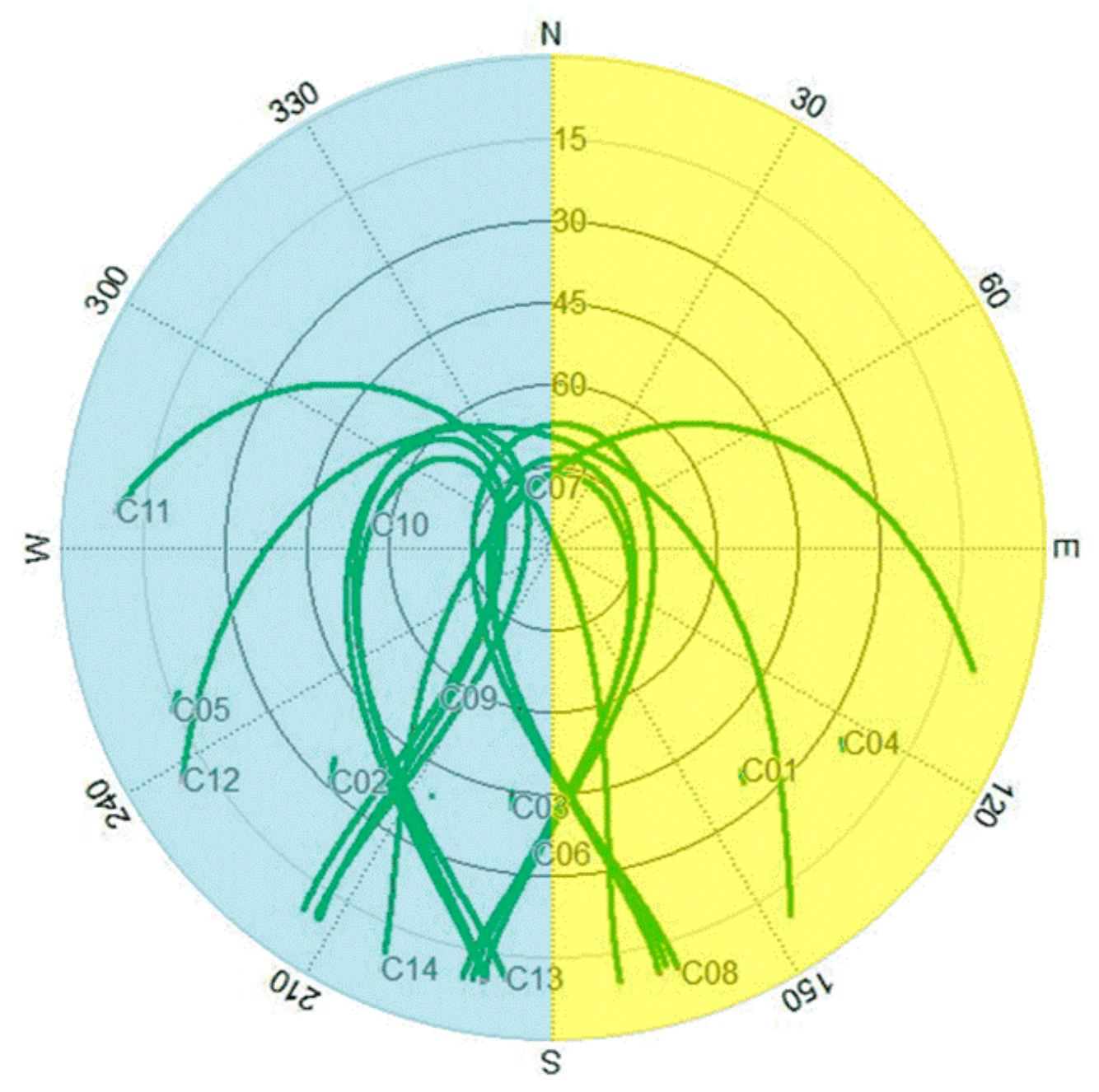
4.1. Receivers Setup
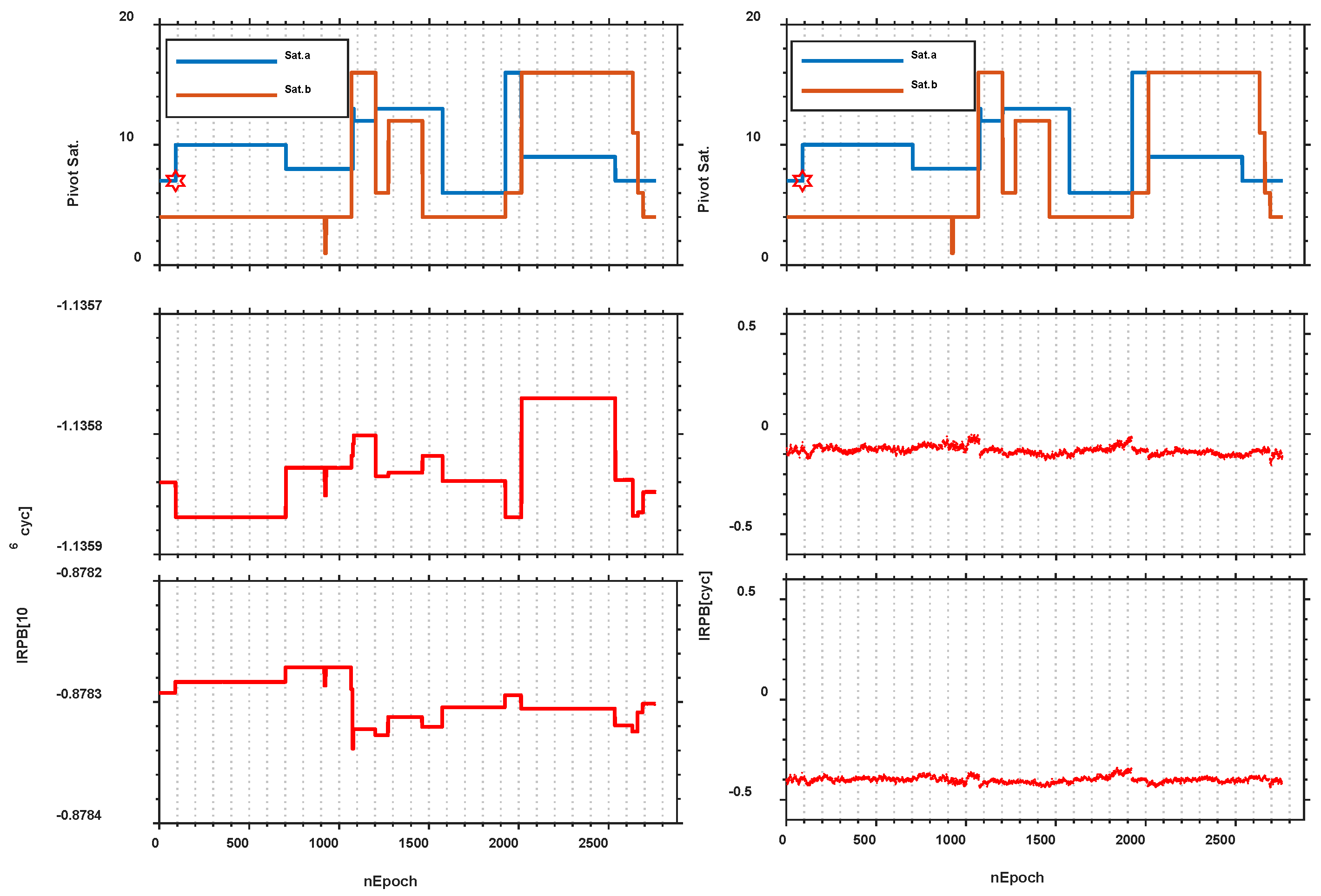
4.2. IRPB Estimation Results
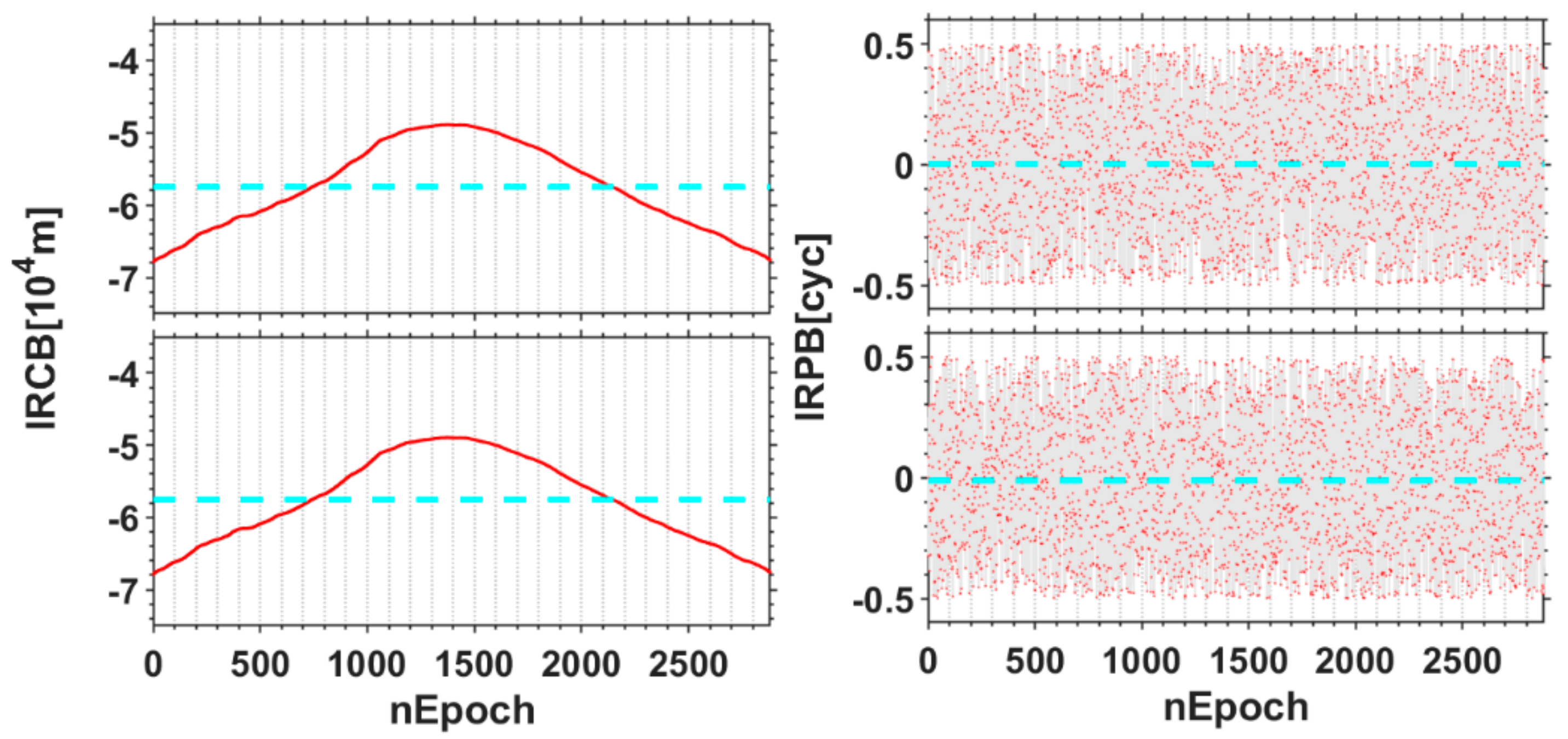
4.3. IRB Analysis under the Non-common Clock Mode
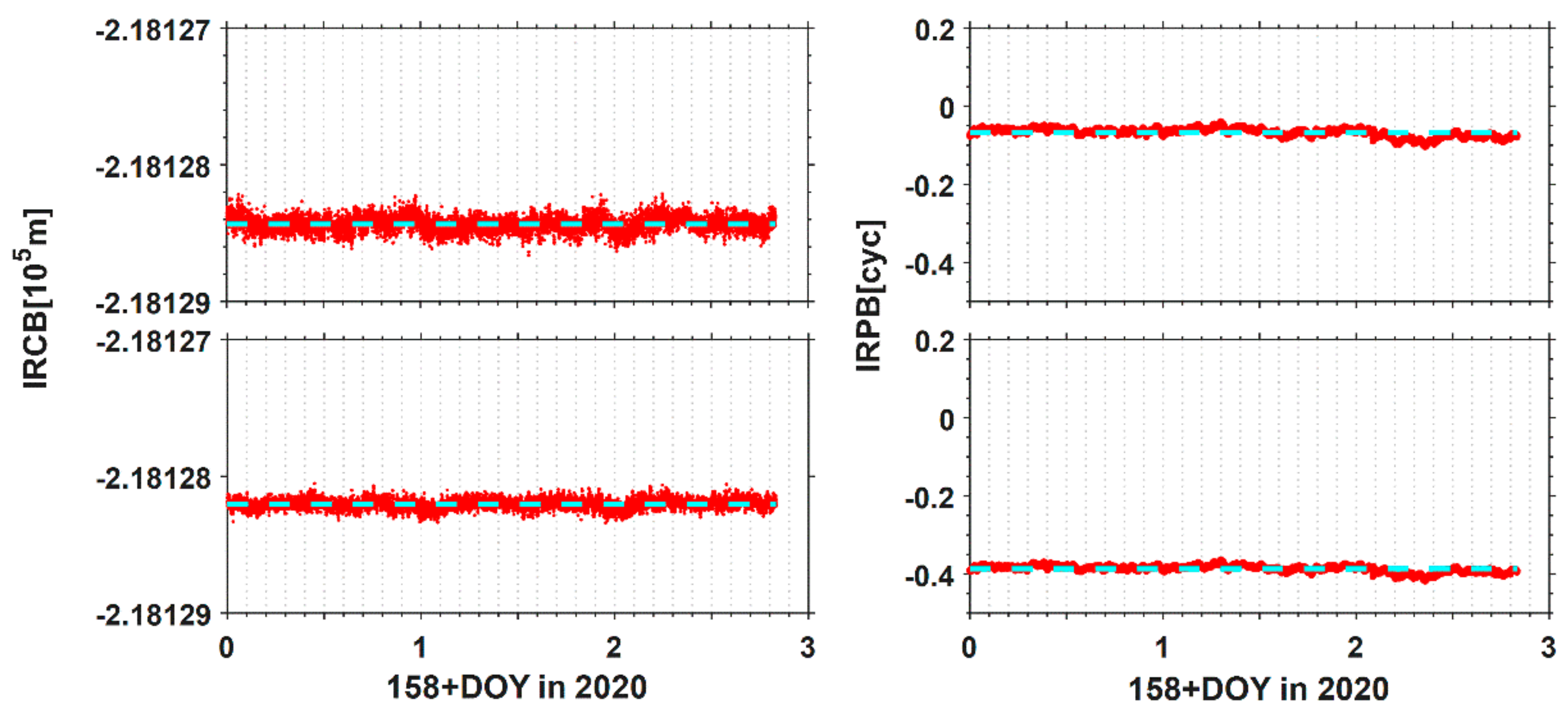
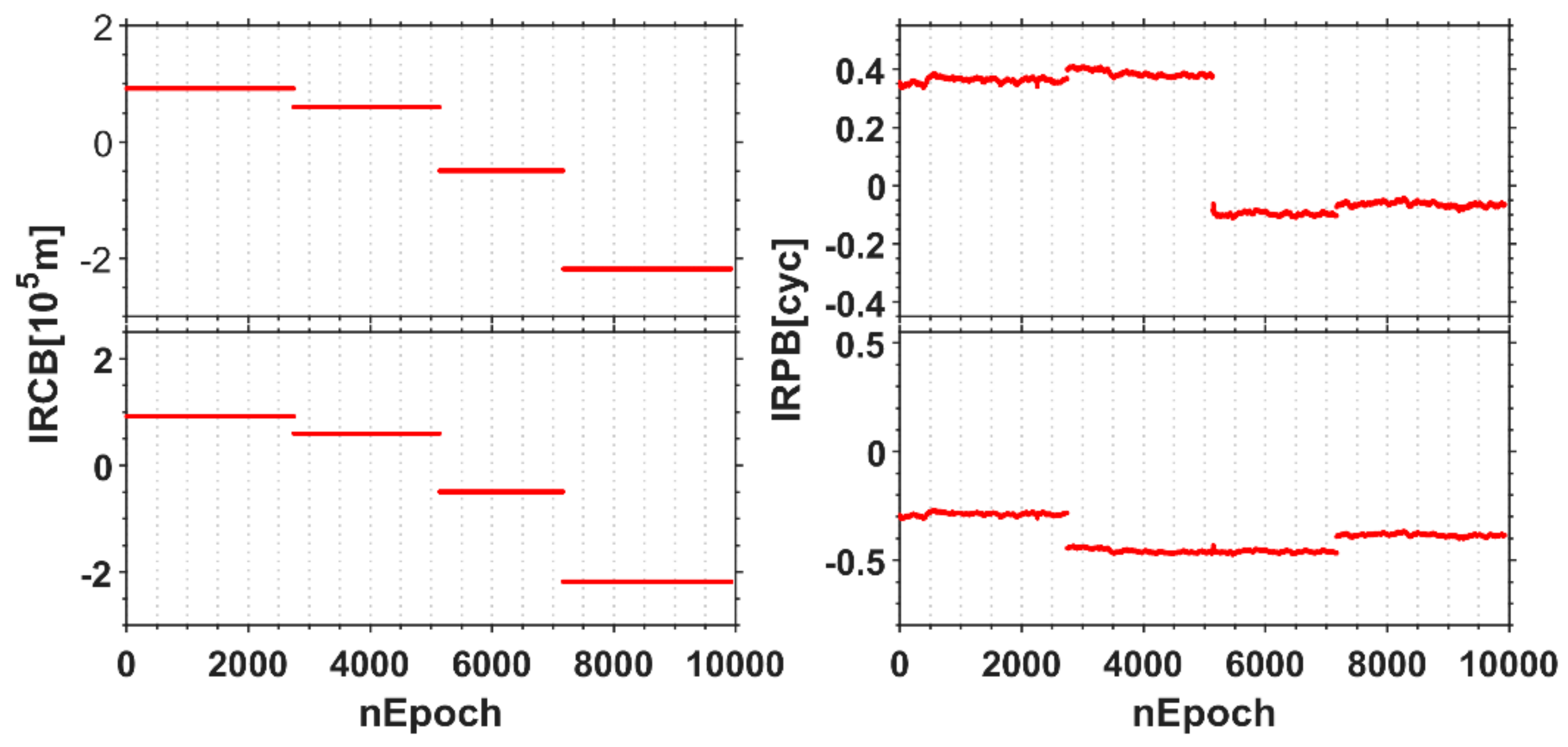
4.4. Characterization of IRB under the Common Clock Mode
4.5. Performance of the IRB-Fixed Model
5. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, B.; Teunissen, P.J.G. Array-Aided CORS Network Ambiguity Resolution; Springer: Berlin/Heidelberg, Germany, 2014; pp. 599–605. [Google Scholar]
- Li, B.; Teunissen, P.J.G. Real-Time Kinematic positioning using fused data from multiple GNSS antennas. In Proceedings of the 2012 15th International Conference on Information Fusion, Singapore, 9–12 July 2012; pp. 933–938. [Google Scholar]
- Teunissen, P.J.G. The least-squares ambiguity decorrelation adjustment: A method for fast GPS integer ambiguity estimation. J. Geod. 1995, 70, 65–82. [Google Scholar] [CrossRef]
- Li, L.; Jia, C.; Zhao, L.; Yang, F.; Li, Z. Integrity monitoring-based ambiguity validation for triple-carrier ambiguity resolution. Gps Solut. 2017, 21, 797–810. [Google Scholar] [CrossRef]
- Borio, D.; Gioia, C. Galileo: The Added Value for Integrity in Harsh Environments. Sensors 2016, 16, 111. [Google Scholar] [CrossRef]
- Bitter, M.; Feuerle, T.; von Wulfen, B.; Steen, M.; Hecker, P. Testbed for Dual-Constellation GBAS Concepts. In Proceedings of the 2010 IEEE-Ion Position Location and Navigation Symposium Plans, Indian Wells, CA, USA, 4–6 May 2010; pp. 474–481. [Google Scholar]
- Giorgi, G.; Teunissen, P.J.G.; Verhagen, S.; Buist, P.J. Instantaneous Ambiguity Resolution in Global-Navigation-Satellite-System-Based Attitude Determination Applications: A Multivariate Constrained Approach. J. Guid. Control Dyn. 2012, 35, 51–67. [Google Scholar] [CrossRef]
- Paziewski, J. Precise GNSS single epoch positioning with multiple receiver configuration for medium-length baselines: Methodology and performance analysis. Meas. Sci. Technol. 2015, 26, 035002. [Google Scholar] [CrossRef]
- Li, H.; Gao, S.; Li, L.; Jia, C.; Zhao, L. Real Time Precise Relative Positioning with Moving Multiple Reference Receivers. Sensors 2018, 18, 2109. [Google Scholar] [CrossRef] [PubMed]
- Teunissen, P.J.G. A-PPP: Array-Aided Precise Point Positioning with Global Navigation Satellite Systems. IEEE Trans. Signal Process. 2012, 60, 2870–2881. [Google Scholar] [CrossRef]
- Li, W.; Nadarajah, N.; Teunissen, P.J.G.; Khodabandeh, A.; Chai, Y. Array-Aided Single-Frequency State-Space RTK with Combined GPS, Galileo, IRNSS, and QZSS L5/E5a Observations. J. Surv. Eng. 2017, 143, 04017006. [Google Scholar] [CrossRef]
- Chen, Y.; Ding, X.; Huang, D.; Zhu, J. A multi-antenna GPS system for local area deformation monitoring. Earth Planets Space 2000, 52, 873–876. [Google Scholar] [CrossRef]
- Buist, P.J.; Teunissen, P.J.G.; Giorgi, G.; Verhagen, S. Multivariate bootstrapped relative positioning of spacecraft using GPS L1/Galileo E1 signals. Adv. Space Res. 2011, 47, 770–785. [Google Scholar] [CrossRef]
- Li, N.; Zhao, L.; Li, L.; Jia, C. Integrity monitoring of high-accuracy GNSS-based attitude determination. Gps Solut. 2018, 22, 120. [Google Scholar] [CrossRef]
- Khodabandeh, A.; Teunissen, P.J.G. Array-based satellite phase bias sensing: Theory and GPS/BeiDou/QZSS results. Meas. Sci. Technol. 2014, 25, 095801. [Google Scholar] [CrossRef]
- Khodabandeh, A.; Teunissen, P.J.G. Array-Aided Multifrequency GNSS Ionospheric Sensing: Estimability and Precision Analysis. IEEE Trans. Geosci. Remote Sens. 2016, 54, 5895–5913. [Google Scholar] [CrossRef]
- Gioia, C.; Borio, D. Android positioning: From stand-alone to cooperative approaches. Appl. Geomat. 2020. [Google Scholar] [CrossRef]
- Kube, F.; Bischof, C.; Alpers, P.; Wallat, C.; Schön, S. A virtual receiver concept and its application to curved aircraft-landing procedures and advanced LEO positioning. Gps Solut. 2018, 22, 41. [Google Scholar] [CrossRef]
- Khanafseh, S.; Kempny, B.; Pervan, B. New Applications of Measurement Redundancy in High Performance Relative Navigation Systems for Aviation. In Proceedings of the 19th International Technical Meeting of the Satellite Division of the Institute of Navigation, Fort Worth, TX, USA, 26–29 September 2006; pp. 3024–3034. [Google Scholar]
- Khanafseh, S.M.; Pervan, B. Autonomous Airborne Refueling of Unmanned Air Vehicles Using the Global Positioning System. J. Aircr. 2007, 44, 1670–1682. [Google Scholar] [CrossRef]
- Wang, Z.; Chen, W.; Dong, D.; Wang, M.; Cai, M.; Yu, C.; Zheng, Z.; Liu, M. Multipath mitigation based on trend surface analysis applied to dual-antenna receiver with common clock. GPS Solut. 2019, 23, 104. [Google Scholar] [CrossRef]
- Keong, J.; Lachapelle, G. Heading and Pitch Determination Using GPS/GLONASS. GPS Solut. 2000, 3, 26–36. [Google Scholar] [CrossRef]
- Chen, W.; Yu, C.; Dong, D.; Cai, M.; Zhou, F.; Wang, Z.; Zhang, L.; Zheng, Z. Formal Uncertainty and Dispersion of Single and Double Difference Models for GNSS-Based Attitude Determination. Sensors 2017, 17, 408. [Google Scholar] [CrossRef] [PubMed]
- Odijk, D.; Teunissen, P.J.G. Characterization of between-receiver GPS-Galileo inter-system biases and their effect on mixed ambiguity resolution. GPS Solut. 2013, 17, 521–533. [Google Scholar] [CrossRef]
- Paziewski, J.; Wielgosz, P. Accounting for Galileo–GPS inter-system biases in precise satellite positioning. J. Geod. 2015, 89, 81–93. [Google Scholar] [CrossRef]
- Zhang, B.; Teunissen, P.J.G. Characterization of multi-GNSS between-receiver differential code biases using zero and short baselines. Sci. Bull. 2015, 60, 1840–1849. [Google Scholar] [CrossRef]
- Jia, C.; Zhao, L.; Li, L.; Lu, R. BDS triple-frequency tightly coupled short-baseline RTK method by calibrating the between-receiver inter-frequency biases. Sci. Sin. Terrae 2020. [Google Scholar] [CrossRef]
- Zhang, B.; Liu, T.; Yuan, Y. GPS receiver phase biases estimable in PPP-RTK networks: Dynamic characterization and impact analysis. J. Geod. 2018, 92, 659–674. [Google Scholar] [CrossRef]
- Eueler, H.-J.; Goad, C.C. On optimal filtering of GPS dual frequency observations without using orbit information. Bull. Géodésique 1991, 65, 130–143. [Google Scholar] [CrossRef]
- Li, L.; Li, Z.; Yuan, H.; Wang, L.; Hou, Y. Integrity monitoring-based ratio test for GNSS integer ambiguity validation. Gps Solut. 2016, 20, 573–585. [Google Scholar] [CrossRef]
- Li, L.; Shi, H.; Jia, C.; Cheng, J.; Li, H.; Zhao, L. Position-domain integrity risk-based ambiguity validation for the integer bootstrap estimator. GPS Solut 2018, 22, 39. [Google Scholar] [CrossRef]
- Verhagen, S.; Teunissen, P.J.G. Ambiguity resolution performance with GPS and BeiDou for LEO formation flying. Adv. Space Res. 2014, 54, 830–839. [Google Scholar] [CrossRef]
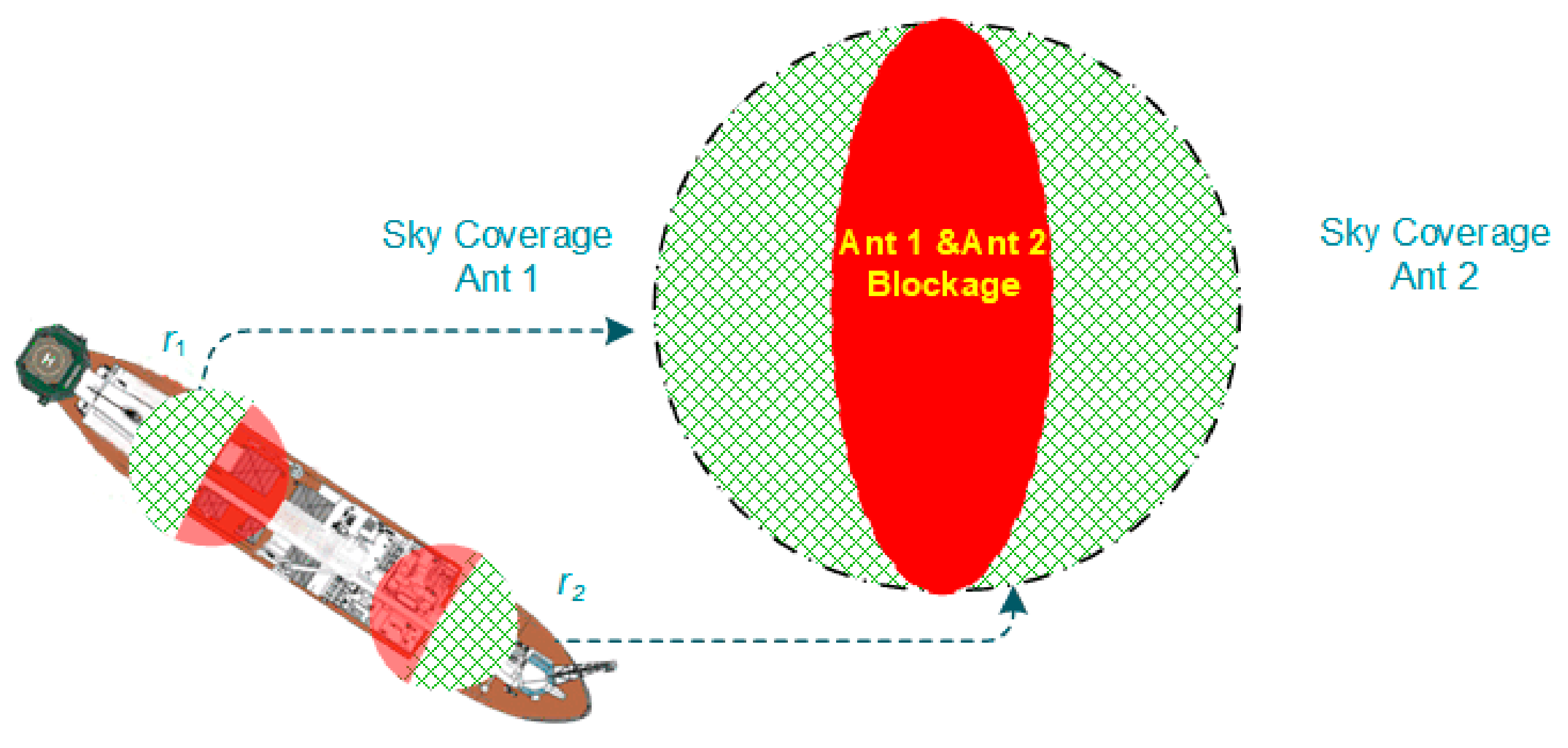
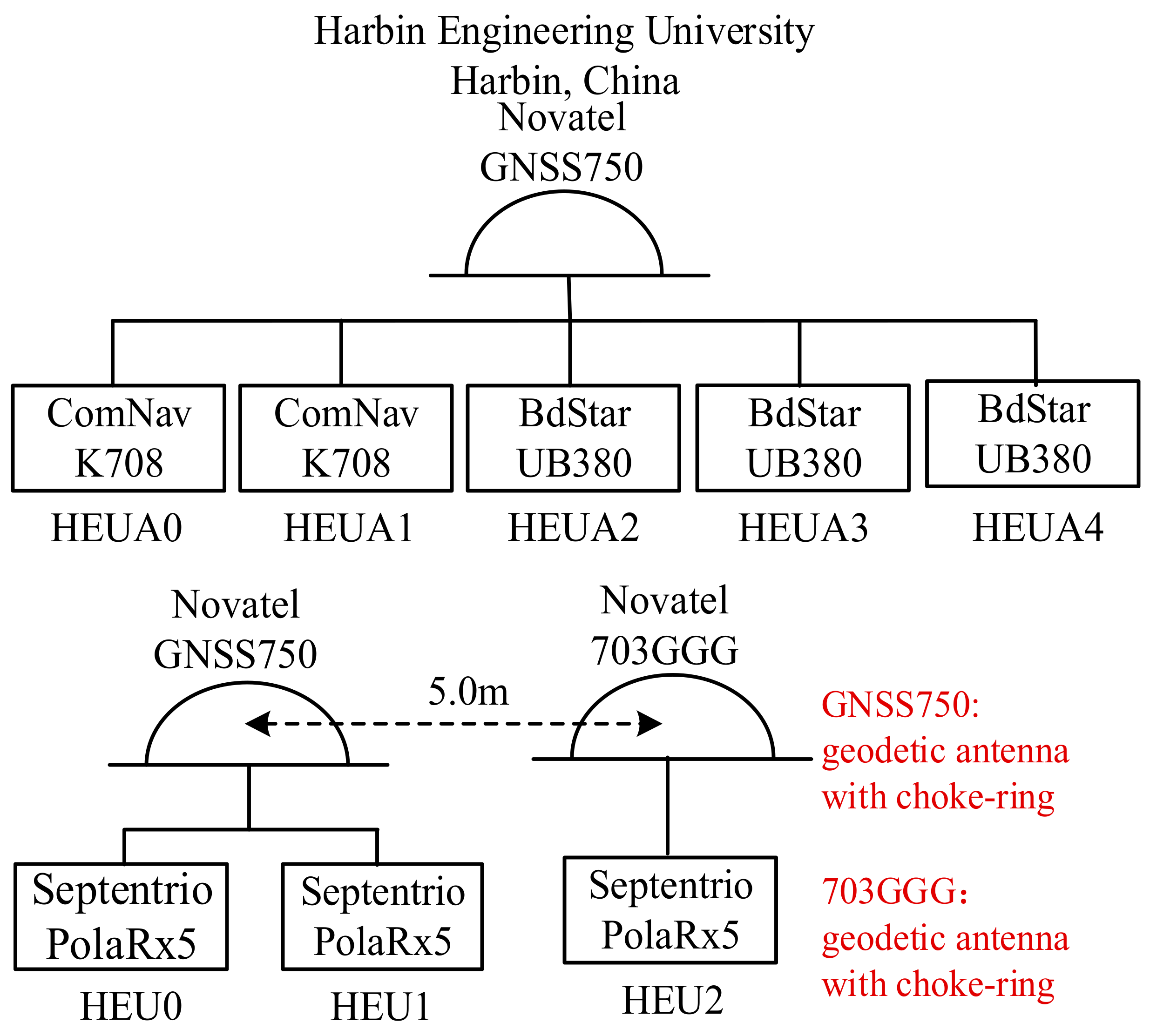

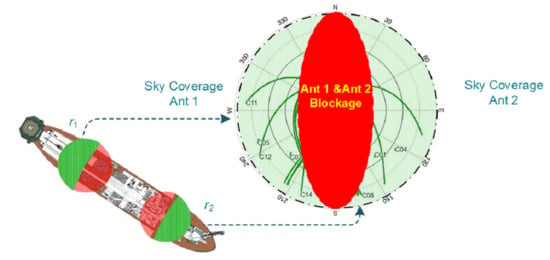
| Model | NC-RTK Model | IRB-Float Model | IRB-Fixed Model |
|---|---|---|---|
| # of observations | 2f(n − k − 1) | 2f(n − 1) | 2f(n − 1) |
| # of parameters | f(n − k − 1) + 3 | f(n + k − 1) + 3 | f(n − 1) + 3 |
| Redundancy | f(n − k − 1) − 3 | f(n − k − 1) − 3 | f(n − 1) − 3 |
| No. | Reference | Rover | Signals | Interval(s) | Distance(m) | Observation Span |
|---|---|---|---|---|---|---|
| 1 | HEUA2 | HEUA0 | B1 B2 | 30 | 0 | DOY147, 2019 |
| HEUA1 | B1 B2 | 30 | 0 | |||
| 2 | HEU2 | HEU0 | B1 B2 | 30 | 5.0 | DOY153–156, 158–161, 2020 |
| HEU1 | B1 B2 | 30 | 5.0 | |||
| 3 | HEUA0 | HEUA2 | B1 B2 | 30 | 0 | DOY147, 2019 |
| HEUA3 | B1 B2 | 30 | 0 | |||
| 4 | HEUA4 | HEUA2 | B1 B2 | 30 | 0 | DOY148, 2019 |
| HEUA3 | B1 B2 | 30 | 0 |
| Baseline | Fre | IRCB | IRPB | ||
| Mean (m) | STD (m) | Mean (Cycle) | STD (Cycle) | ||
| {HEUA0,HEUA1}-HEUA2 | B1 | −0.8725 | 0.3399 | 0.0045 | 0.30 |
| B2 | −0.8320 | 0.3249 | 0.0050 | 0.30 | |
| {HEUA2,HEUA3}-HEUA0 | B1 | 65,902.9 | 139,203.6 | 0.0067 | 0.29 |
| B2 | 65,902.5 | 139,203.7 | 0.0010 | 0.29 | |
| {HEUA2,HEUA3}-HEUA4 | B1 | −57,534.6 | 5843.1 | 0.0128 | 0.29 |
| B2 | −57,535.1 | 5843.2 | 0.0036 | 0.29 | |
| Type | B1 | B2 | ||
|---|---|---|---|---|
| Mean | STD | Mean | STD | |
| IRCB (m) | −218,128.42 | 0.28 | −218,128.20 | 0.22 |
| IRPB (cycle) | −0.081 | 0.021 | −0.40 | 0.015 |
| α (deg) | θ (deg) | NC-RTK (cm) | C-RTK (cm) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| σE,RMS | σN,RMS | σU,RMS | Ps | σE,RMS | σN,RMS | σU,RMS | Ps | ||
| (180,180) | 10 | 0.35 | 0.34 | 0.82 | 100% | 0.19 | 0.28 | 0.68 | 100% |
| 15 | 0.35 | 0.34 | 0.76 | 100% | 0.19 | 0.27 | 0.63 | 100% | |
| 20 | 0.31 | 2.40 | 2.51 | 99.96% | 0.24 | 0.33 | 0.92 | 100% | |
| 25 | 0.32 | 2.41 | 2.52 | 99.96% | 0.25 | 0.34 | 0.95 | 100% | |
| 30 | 8.97 | 10.09 | 25.01 | 98.2% | 0.35 | 0.44 | 1.28 | 100% | |
| (170,190) | 10 | 17.27 | 33.91 | 69.47 | 97.57% | 0.98 | 2.41 | 4.50 | 99.96% |
| 15 | 19.82 | 41.65 | 83.66 | 97.03% | 0.59 | 5.88 | 11.75 | 99.86% | |
| 20 | 21.93 | 45.97 | 92.03 | 95.62% | 0.13 | 9.3 | 18.49 | 99.67% | |
| 25 | 28.90 | 61.88 | 122.84 | 93.33% | 2.20 | 10.18 | 20.26 | 99.57% | |
| 30 | 29.52 | 34.72 | 83.48 | 82.5% | 14.48 | 31.78 | 51.81 | 95% | |
| (160,200) | 10 | 25.93 | 56.59 | 104.86 | 93.44% | 0.68 | 5.12 | 9.69 | 99.86% |
| 15 | 32.58 | 70.51 | 129.95 | 93.04% | 0.84 | 8.84 | 18.63 | 99.67% | |
| 20 | 37.84 | 78.22 | 143.88 | 90.29% | 2.20 | 10.23 | 20.46 | 99.52% | |
| 25 | 41.76 | 84.42 | 158.05 | 88.77% | 2.32 | 11.37 | 22.71 | 99.45% | |
| 30 | 51.61 | 56 | 114.76 | 72.83% | 16.69 | 34.64 | 61.61 | 92.32% | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, L.; Jiang, J.; Li, L.; Jia, C.; Cheng, J. High-Accuracy Real-Time Kinematic Positioning with Multiple Rover Receivers Sharing Common Clock. Remote Sens. 2021, 13, 823. https://doi.org/10.3390/rs13040823
Zhao L, Jiang J, Li L, Jia C, Cheng J. High-Accuracy Real-Time Kinematic Positioning with Multiple Rover Receivers Sharing Common Clock. Remote Sensing. 2021; 13(4):823. https://doi.org/10.3390/rs13040823
Chicago/Turabian StyleZhao, Lin, Jiachang Jiang, Liang Li, Chun Jia, and Jianhua Cheng. 2021. "High-Accuracy Real-Time Kinematic Positioning with Multiple Rover Receivers Sharing Common Clock" Remote Sensing 13, no. 4: 823. https://doi.org/10.3390/rs13040823
APA StyleZhao, L., Jiang, J., Li, L., Jia, C., & Cheng, J. (2021). High-Accuracy Real-Time Kinematic Positioning with Multiple Rover Receivers Sharing Common Clock. Remote Sensing, 13(4), 823. https://doi.org/10.3390/rs13040823