Ionospheric Turbulence and the Equatorial Plasma Density Irregularities: Scaling Features and RODI
Abstract
1. Introduction
2. Data
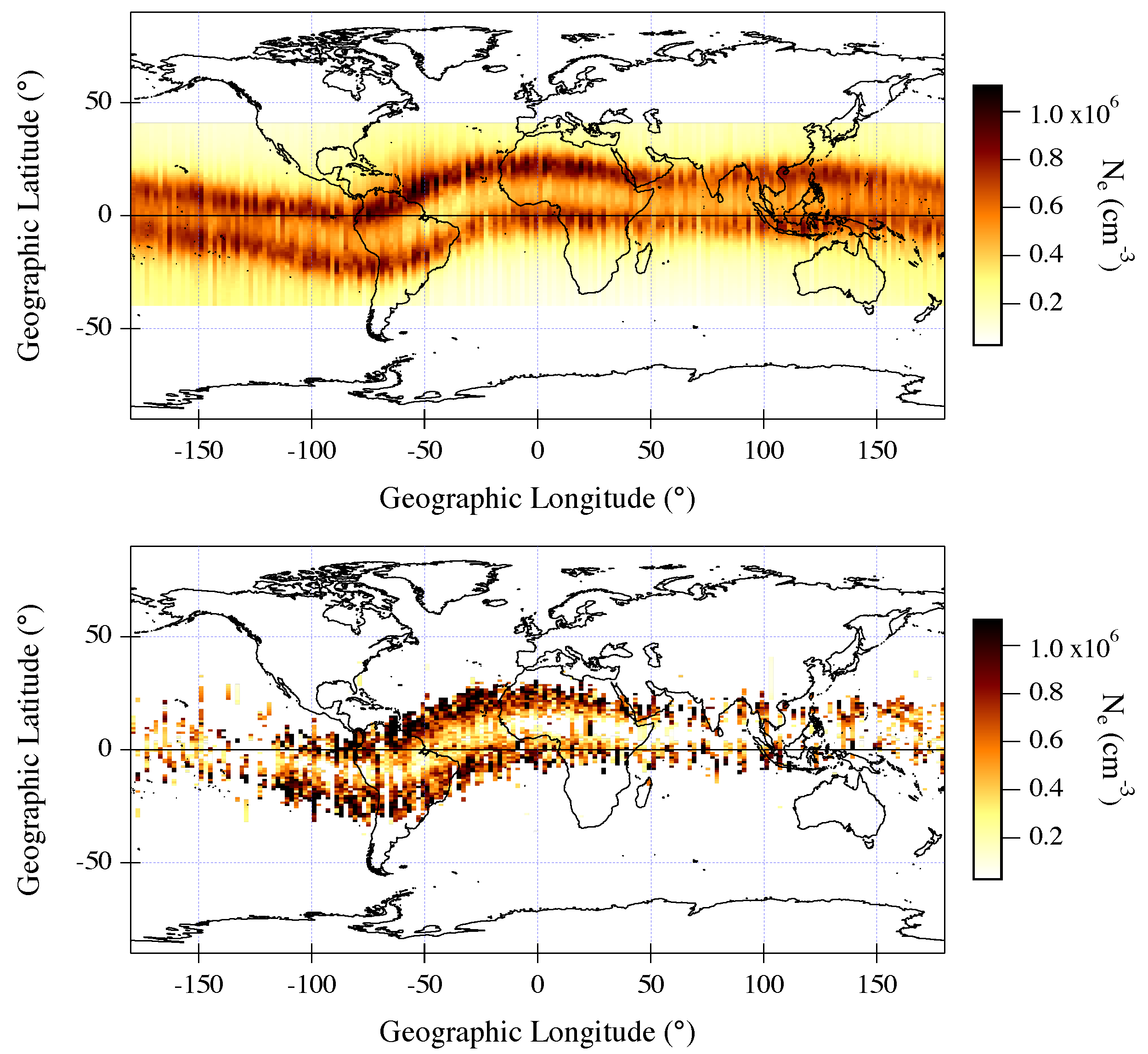
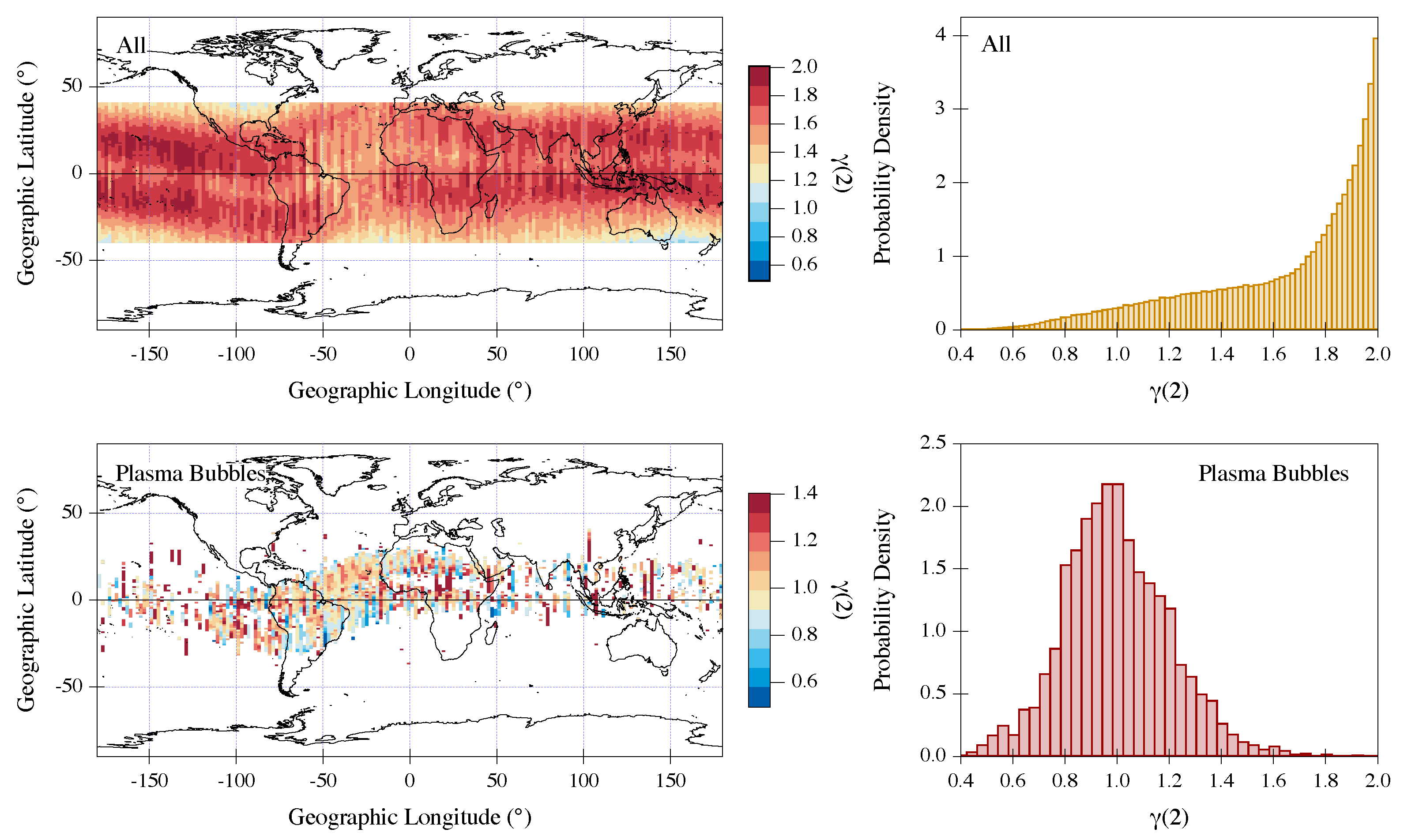
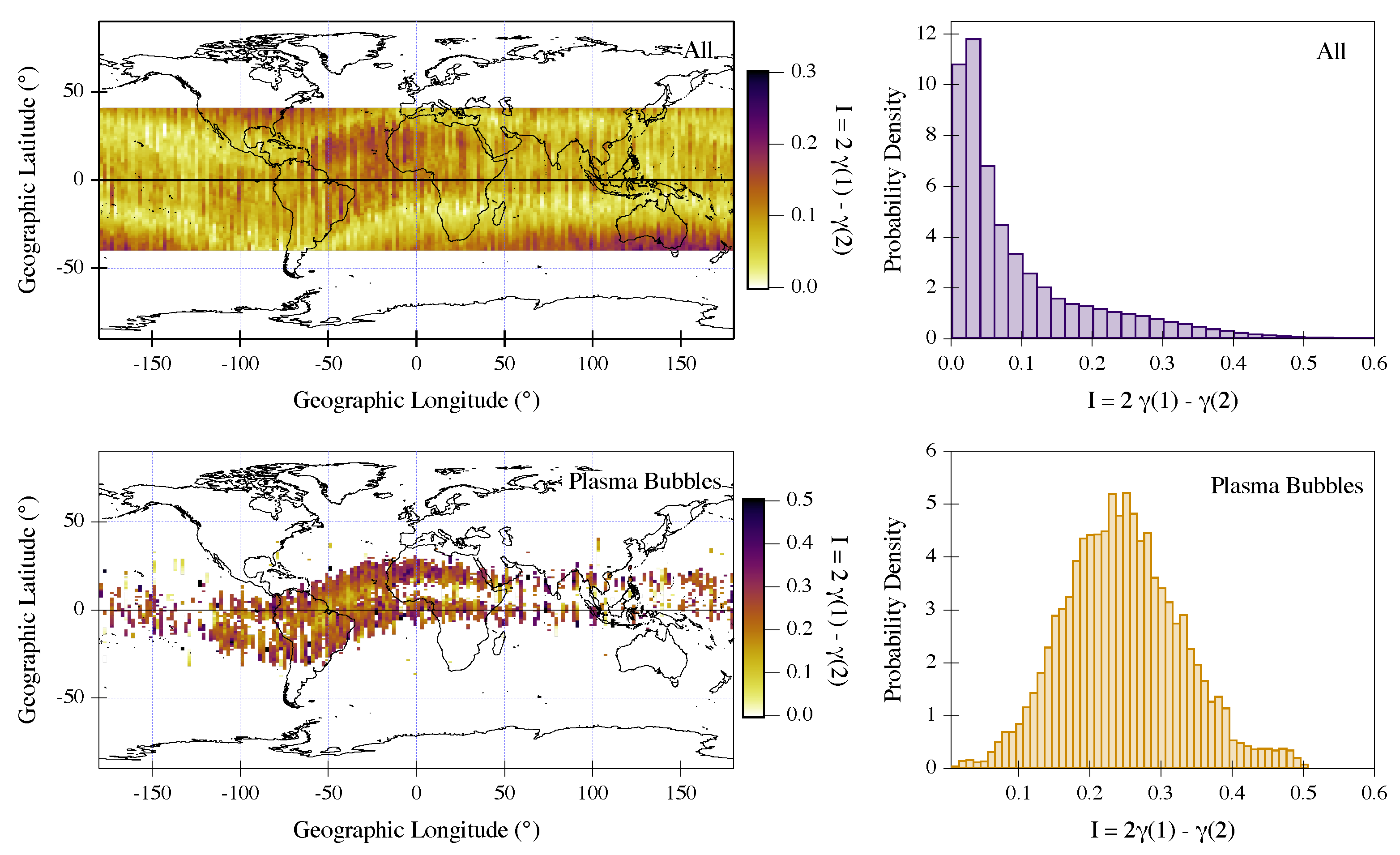
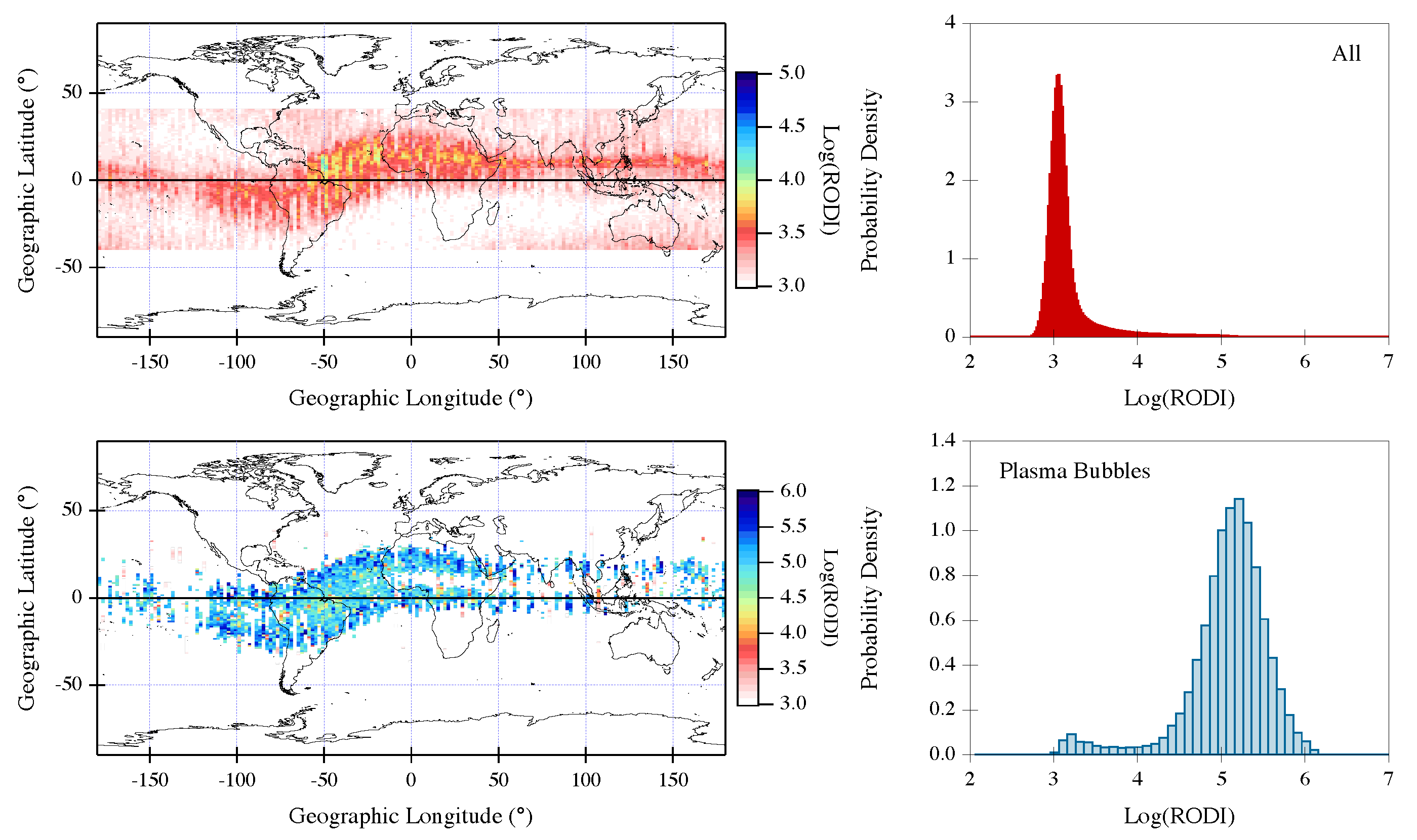
3. Method and Analysis Results
4. Discussion
5. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ESA | European Space Agency |
| BF | Bubble Flag |
| GNSS | Global Navigation Satellite System |
| GPS | Global Positioning System |
| IBI | Swarm Level-2 Ionospheric Bubble Index |
| PSD | Power Spectral density |
| RODI | Rate Of change of electron Density Index |
References
- Kil, H.; Heelis, R.A. Global distribution of density irregularities in the equatorial ionosphere. J. Geophys. Res. 1998, 103, 407–418. [Google Scholar] [CrossRef]
- Woodman, R.F.; La Hoz, C. Radar observations of F region equatorial irregularities. J. Geophys. Res. 1976, 81, 5447–5466. [Google Scholar] [CrossRef]
- Kelley, M. The Earth’s Ionosphere: Plasma Physics and Electrodynamics; International Geophysics, Elsevier Science: Amsterdam, The Netherlands, 2009. [Google Scholar]
- Schunk, R.W.; Nagy, A.F. Ionospheres: Physics, Plasma Physics, and Chemistry; Cambridge Atmospheric and Space Science Series; Cambridge University Press: Cambridge, UK, 2009. [Google Scholar]
- Cherniak, I.; Zakharenkova, I.; Sokolovsky, S. Multi-Instrumental Observation of Storm-Induced Ionospheric Plasma Bubbles at Equatorial and Middle Latitudes. J. Geophys. Res. Space Phys. 2019, 124, 1491–1508. [Google Scholar] [CrossRef]
- Lühr, H.; Xiong, C.; Park, J.; Rauberg, J. Systematic study of intermediate-scale structures of equatorial plasma irregularities in the ionosphere based on CHAMP observations. Front. Phys. 2014, 2, 15. [Google Scholar] [CrossRef]
- Hysell, D.L.; Seyler, C.E. A renormalization group approach to estimation of anomalous diffusion in the unstable equatorial F region. J. Geophys. Res. 1998, 103, 26731–26738. [Google Scholar] [CrossRef]
- Tsunoda, R.T.; Livingston, R.C.; McClure, J.P.; Hanson, W.B. Equatorial plasma bubbles: Vertically elongated wedges from the bottomside F layer. J. Geophys. Res. 1982, 87, 9171–9180. [Google Scholar] [CrossRef]
- Kil, H.; Heelis, R.A.; Paxton, L.J.; Oh, S.J. Formation of a plasma depletion shell in the equatorial ionosphere. J. Geophys. Res. Space Phys. 2009, 114, A11302. [Google Scholar] [CrossRef]
- Tsunoda, R.T. Control of the seasonal and longitudinal occurrence of equatorial scintillations by the longitudinal gradient in integrated E region pedersen conductivity. J. Geophys. Res. 1985, 90, 447–456. [Google Scholar] [CrossRef]
- Prikryl, P.; Jayachandran, P.T.; Mushini, S.C.; Chadwick, R. Climatology of GPS phase scintillation and HF radar backscatter for the high-latitude ionosphere under solar minimum conditions. Ann. Geophys. 2011, 29, 377–392. [Google Scholar] [CrossRef]
- Jin, Y.; Moen, J.I.; Miloch, W.J. GPS scintillation effects associated with polar cap patches and substorm auroral activity: Direct comparison. J. Space Weather. Space Clim. 2014, 4, A23. [Google Scholar] [CrossRef]
- Jin, Y.; Moen, J.I.; Miloch, W.J. On the collocation of the cusp aurora and the GPS phase scintillation: A statistical study. J. Geophys. Res. Space Phys. 2015, 120, 9176–9191. [Google Scholar] [CrossRef]
- Kintner, P.M.; Seyler, C.E. The status of observations and theory of high latitude ionospheric and magnetospheric plasma turbulence. Space Sci. Rev. 1985, 41, 91–129. [Google Scholar] [CrossRef]
- Hysell, D.L.; Shume, E.B. Electrostatic plasma turbulence in the topside equatorial F region ionosphere. J. Geophys. Res. Space Phys. 2002, 107, 1269. [Google Scholar] [CrossRef]
- Hassam, A.B.; Hall, W.; Huba, J.D.; Keskinen, M.J. Spectral characteristics of interchange turbulence. J. Geophys. Res. 1986, 91, 13513–13522. [Google Scholar] [CrossRef]
- Zargham, S.; Seyler, C.E. Collisional and inertial dynamics of the ionospheric interchange instability. J. Geophys. Res. 1989, 94, 9009–9027. [Google Scholar] [CrossRef]
- Kraichnan, R.H. Inertial Ranges in Two-Dimensional Turbulence. Phys. Fluids 1967, 10, 1417–1423. [Google Scholar] [CrossRef]
- Kraichnan, R.H.; Montgomery, D. REVIEW ARTICLE: Two-dimensional turbulence. Rep. Prog. Phys. 1980, 43, 547–619. [Google Scholar] [CrossRef]
- Yokoyama, T.; Shinagawa, H.; Jin, H. Nonlinear growth, bifurcation, and pinching of equatorial plasma bubble simulated by three-dimensional high-resolution bubble model. J. Geophys. Res. Space Phys. 2014, 119, 10474–10482. [Google Scholar] [CrossRef]
- Yokoyama, T. A review on the numerical simulation of equatorial plasma bubbles toward scintillation evaluation and forecasting. Prog. Earth Planet. Sci. 2017, 4, 37. [Google Scholar] [CrossRef]
- Kelley, M.C. The Earth’s Ionosphere; Elsevier: Amsterdam, The Netherlands, 1989. [Google Scholar]
- Materassi, M.; Forte, B.; Coster, A.J.; Skone, S. (Eds.) The Dynamical Ionosphere; Elsevier: Amsterdam, The Netherlands, 2020. [Google Scholar]
- Tsunoda, R.T. High-latitude F-region irregularities: A review and synthesis. Rev. Geophys. 1988, 26, 719–760. [Google Scholar] [CrossRef]
- Sahr, J.D.; Fejer, B.G. Auroral electrojet plasma irregularity theory and experiment: A critical review of present understanding and future directions. J. Geophys. Res. 1996, 101, 26893–26910. [Google Scholar] [CrossRef]
- Hysell, D.L. An overview and synthesis of plasma irregularities in equatorial spread /F. J. Atmos. Sol. Terr. Phys. 2000, 62, 1037–1056. [Google Scholar] [CrossRef]
- Dyson, P.L.; McClure, J.P.; Hanson, W.B. In situ measurements of the spectral characteristics of F region ionospheric irregularities. J. Geophys. Res. 1974, 79, 1497. [Google Scholar] [CrossRef]
- Livingston, R.C.; Rino, C.L.; McClure, J.P.; Hanson, W.B. Spectral characteristics of medium-scale equatorial F region irregularities. J. Geophys. Res. 1981, 86, 2421–2428. [Google Scholar] [CrossRef]
- Cerisier, J.C.; Berthelier, J.J.; Beghin, C. Unstable density gradients in the high-latitude ionosphere. Radio Sci. 1985, 20, 755–761. [Google Scholar] [CrossRef]
- Hobara, Y.; Lefeuvre, F.; Parrot, M.; Molchanov, O.A. Low-latitude ionospheric turbulence observed by Aureol-3 satellite. Ann. Geophys. 2005, 23, 1259–1270. [Google Scholar] [CrossRef][Green Version]
- Xiong, C.; Stolle, C.; Lühr, H.; Park, J.; Fejer, B.G.; Kervalishvili, G.N. Scale analysis of equatorial plasma irregularities derived from Swarm constellation. Earth Planets Space 2016, 68, 121. [Google Scholar] [CrossRef]
- Xiong, C.; Stolle, C.; Park, J. Climatology of GPS signal loss observed by Swarm satellites. Ann. Geophys. 2018, 36, 679–693. [Google Scholar] [CrossRef]
- Friis-Christensen, E.; Lühr, H.; Knudsen, D.; Haagmans, R. Swarm An Earth Observation Mission investigating Geospace. Adv. Space Res. 2008, 41, 210–216. [Google Scholar] [CrossRef]
- Stolle, C.; Lühr, H.; Rother, M.; Balasis, G. Magnetic signatures of equatorial spread F as observed by the CHAMP satellite. J. Geophys. Res. Space Phys. 2006, 111, A02304. [Google Scholar] [CrossRef]
- Xiong, C.; Lühr, H.; Ma, S.Y.; Stolle, C.; Fejer, B.G. Features of highly structured equatorial plasma irregularities deduced from CHAMP observations. Ann. Geophys. 2012, 30, 1259–1269. [Google Scholar] [CrossRef]
- Basu, S.; Basu, S. Equatorial scintillations: Advances since ISEA-6. J. Atmos. Terr. Phys. 1985, 47, 753–768. [Google Scholar] [CrossRef]
- Kelley, M.C.; Makela, J.J.; Ledvina, B.M.; Kintner, P.M. Observations of equatorial spread-F from Haleakala, Hawaii. Geophys. Res. Lett. 2002, 29, 2003. [Google Scholar] [CrossRef]
- Sahai, Y.; Fagundes, P.; Abalde, J.; Pimenta, A.; Bittencourt, J.; Otsuka, Y.; Rios, V. Generation of large-scale equatorial F-region plasma depletions during low range spread-F season. Ann. Geophys. 2004, 22, 15–23. [Google Scholar] [CrossRef][Green Version]
- Hysell, D.L.; Burcham, J.D. JULIA radar studies of equatorial spread F. J. Geophys. Res. Space Phys. 1998, 103, 29155–29168. [Google Scholar] [CrossRef]
- Huang, C.Y.; Burke, W.J.; Machuzak, J.S.; Gentile, L.C.; Sultan, P.J. Equatorial plasma bubbles observed by DMSP satellites during a full solar cycle: Toward a global climatology. J. Geophys. Res. Space Phys. 2002, 107, 1434. [Google Scholar] [CrossRef]
- Burke, W.J.; Gentile, L.C.; Huang, C.Y.; Valladares, C.E.; Su, S.Y. Longitudinal variability of equatorial plasma bubbles observed by DMSP and ROCSAT-1. J. Geophys. Res. Space Phys. 2004, 109, A12301. [Google Scholar] [CrossRef]
- Burke, W.; Huang, C.; Gentile, L.; Bauer, L. Seasonal-longitudinal variability of equatorial plasma bubbles. Ann. Geophys. 2004, 22, 3089–3098. [Google Scholar] [CrossRef]
- Su, S.Y.; Liu, C.H.; Ho, H.H.; Chao, C.K. Distribution characteristics of topside ionospheric density irregularities: Equatorial versus midlatitude regions. J. Geophys. Res. Space Phys. 2006, 111, A06305. [Google Scholar] [CrossRef]
- Gentile, L.C.; Burke, W.J.; Rich, F.J. A climatology of equatorial plasma bubbles from DMSP 1989-2004. Radio Sci. 2006, 41. [Google Scholar] [CrossRef]
- Gentile, L.C.; Burke, W.J.; Rich, F.J. A global climatology for equatorial plasma bubbles in the topside ionosphere. Ann. Geophys. 2006, 24, 163–172. [Google Scholar] [CrossRef]
- Nishioka, M.; Saito, A.; Tsugawa, T. Occurrence characteristics of plasma bubble derived from global ground-based GPS receiver networks. J. Geophys. Res. Space Phys. 2008, 113. [Google Scholar] [CrossRef]
- Park, J.; Noja, M.; Stolle, C.; Lühr, H. The Ionospheric Bubble Index deduced from magnetic field and plasma observations onboard Swarm. Earth Planets Space 2013, 65, 1333–1344. [Google Scholar] [CrossRef]
- Park, J.; Min, K.W.; Kim, V.P.; Kil, H.; Lee, J.J.; Kim, H.J.; Lee, E.; Lee, D.Y. Global distribution of equatorial plasma bubbles in the premidnight sector during solar maximum as observed by KOMPSAT-1 and Defense Meteorological Satellite Program F15. J. Geophys. Res. Space Phys. 2005, 110, A07308. [Google Scholar] [CrossRef]
- Whalen, J.A. Dependence of equatorial bubbles and bottomside spread F on season, magnetic activity, and E × B drift velocity during solar maximum. J. Geophys. Res. Space Phys. 2002, 107, 1024. [Google Scholar] [CrossRef]
- De Michelis, P.; Consolini, G.; Tozzi, R. Magnetic field fluctuation features at Swarm’s altitude: A fractal approach. Geophys. Res. Lett. 2015, 42, 3100–3105. [Google Scholar] [CrossRef]
- Frisch, U. Turbulence: The Legacy of A.N. Kolmogorov; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Consolini, G.; De Michelis, P.; Alberti, T.; Giannattasio, F.; Coco, I.; Tozzi, R.; Chang, T.T.S. On the Multifractal Features of Low-Frequency Magnetic Field Fluctuations in the Field-Aligned Current Ionospheric Polar Regions: Swarm Observations. J. Geophys. Res. Space Phys. 2020, 125, e27429. [Google Scholar] [CrossRef]
- Davis, A.; Marshak, A.; Wiscombe, W.; Cahalan, R. Multifractal characterizations of nonstationarity and intermittency in geophysical fields: Observed, retrieved, or simulated. J. Geophys. Res. Space Phys. 1994, 99, 8055–8072. [Google Scholar] [CrossRef]
- Basu, S.; MacKenzie, E.; Basu, S.; Coley, W.R.; Sharber, J.R.; Hoegy, W.R. Plasma structuring by the gradient drift instability at high latitudes and comparison with velocity shear driven processes. J. Geophys. Res. Space Phys. 1990, 95, 7799–7818. [Google Scholar] [CrossRef]
- Kraichnan, R.H. On Kolmogorov’s inertial-range theories. J. Fluid Mech. 1974, 62, 305–330. [Google Scholar] [CrossRef]
- Monin, A.S.; Yaglom, A.M. Statistical Fluid Mechanics: Mechanics of Turbulence; MIT Press: Cambridge, MA, USA, 1975. [Google Scholar]
- Rose, H.A.; Sulem, P.L. Fully developed turbulence and statistical mechanics. J. Phys. 1978, 39, 1938–1943. [Google Scholar] [CrossRef]
- De Michelis, P.; Pignalberi, A.; Consolini, G.; Coco, I.; Tozzi, R.; Pezzopane, M.; Giannattasio, F.; Balasis, G. On the 2015 St. Patrick’s Storm Turbulent State of the Ionosphere: Hints From the Swarm Mission. J. Geophys. Res. Space Phys. 2020, 125, e27934. [Google Scholar] [CrossRef]
- Pignalberi, A. TITIPy: A python tool for the calculation and mapping of topside ionosphere turbulence indices. Comput. Geosci. 2021, 104675. [Google Scholar] [CrossRef]
- Jin, Y.; Spicher, A.; Xiong, C.; Clausen, L.B.N.; Kervalishvili, G.; Stolle, C.; Miloch, W.J. Ionospheric Plasma Irregularities Characterized by the Swarm Satellites: Statistics at High Latitudes. J. Geophys. Res. Space Phys. 2019, 124, 1262–1282. [Google Scholar] [CrossRef]
- Piersanti, M.; De Michelis, P.; Del Moro, D.; Tozzi, R.; Pezzopane, M.; Consolini, G.; Marcucci, M.F.; Laurenza, M.; Di Matteo, S.; Pignalberi, A.; et al. From the Sun to Earth: Effects of the 25 August 2018 geomagnetic storm. Ann. Geophys. 2020, 38, 703–724. [Google Scholar] [CrossRef]
- Chang, T.S. An Introduction to Space Plasma Complexity; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
- Wu, C.C.; Chang, T. Dynamical evolution of coherent structures in intermittent two-dimensional MHD turbulence. IEEE Trans. Plasma Sci. 2000, 28, 1938–1943. [Google Scholar] [CrossRef]
- Chang, T.; Tam, S.W.Y.; Wu, C.C. Complexity induced anisotropic bimodal intermittent turbulence in space plasmas. Phys. Plasmas 2004, 11, 1287–1299. [Google Scholar] [CrossRef]
- Pécseli, H.L. Spectral properties of electrostatic drift wave turbulence in the laboratory and the ionosphere. Ann. Geophys. 2015, 33, 875–900. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Michelis, P.; Consolini, G.; Tozzi, R.; Pignalberi, A.; Pezzopane, M.; Coco, I.; Giannattasio, F.; Marcucci, M.F. Ionospheric Turbulence and the Equatorial Plasma Density Irregularities: Scaling Features and RODI. Remote Sens. 2021, 13, 759. https://doi.org/10.3390/rs13040759
De Michelis P, Consolini G, Tozzi R, Pignalberi A, Pezzopane M, Coco I, Giannattasio F, Marcucci MF. Ionospheric Turbulence and the Equatorial Plasma Density Irregularities: Scaling Features and RODI. Remote Sensing. 2021; 13(4):759. https://doi.org/10.3390/rs13040759
Chicago/Turabian StyleDe Michelis, Paola, Giuseppe Consolini, Roberta Tozzi, Alessio Pignalberi, Michael Pezzopane, Igino Coco, Fabio Giannattasio, and Maria Federica Marcucci. 2021. "Ionospheric Turbulence and the Equatorial Plasma Density Irregularities: Scaling Features and RODI" Remote Sensing 13, no. 4: 759. https://doi.org/10.3390/rs13040759
APA StyleDe Michelis, P., Consolini, G., Tozzi, R., Pignalberi, A., Pezzopane, M., Coco, I., Giannattasio, F., & Marcucci, M. F. (2021). Ionospheric Turbulence and the Equatorial Plasma Density Irregularities: Scaling Features and RODI. Remote Sensing, 13(4), 759. https://doi.org/10.3390/rs13040759








