LiDAR Observations of Multi-Modal Swash Probability Distributions on a Dissipative Beach
Abstract
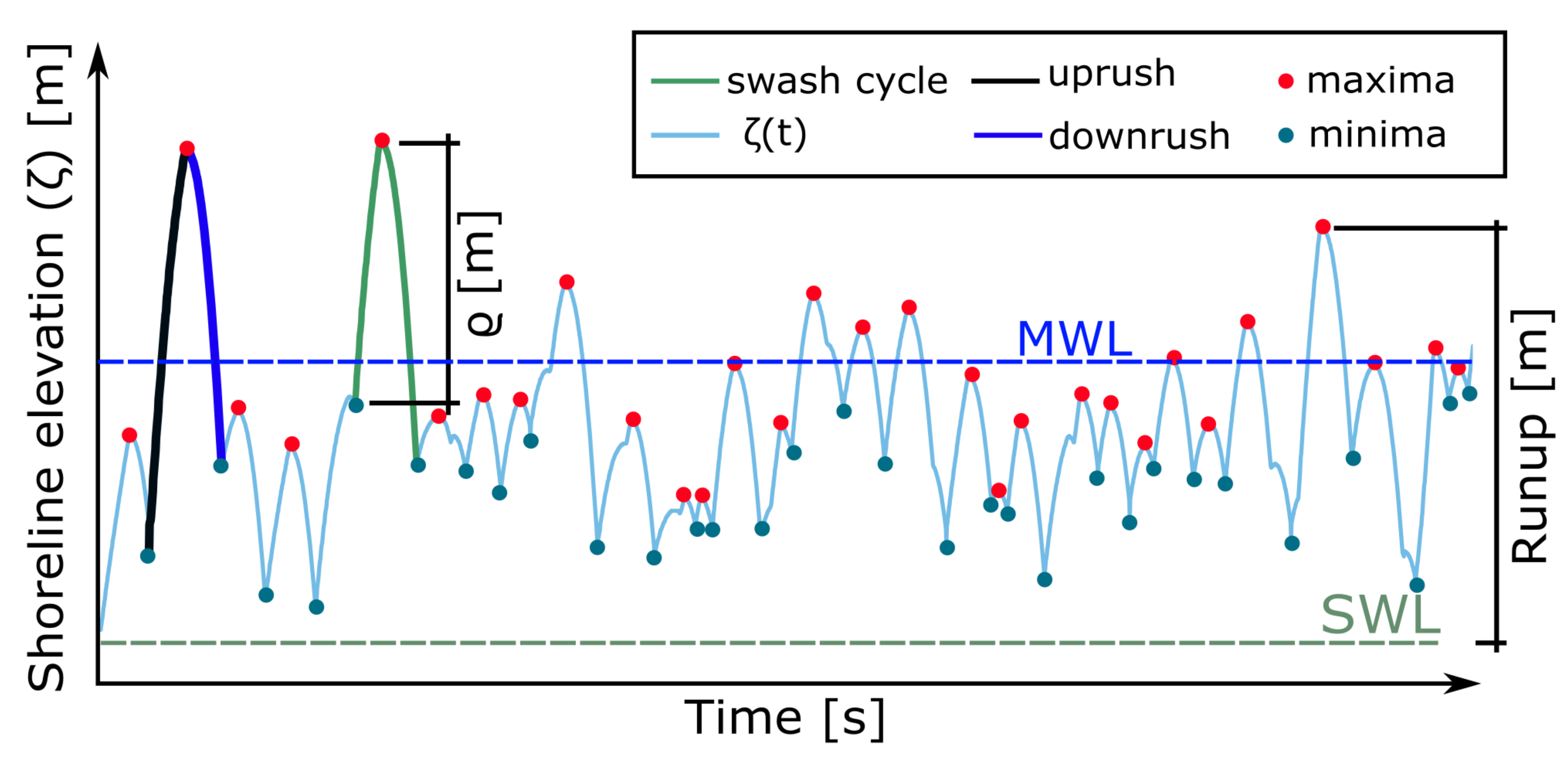
1. Introduction
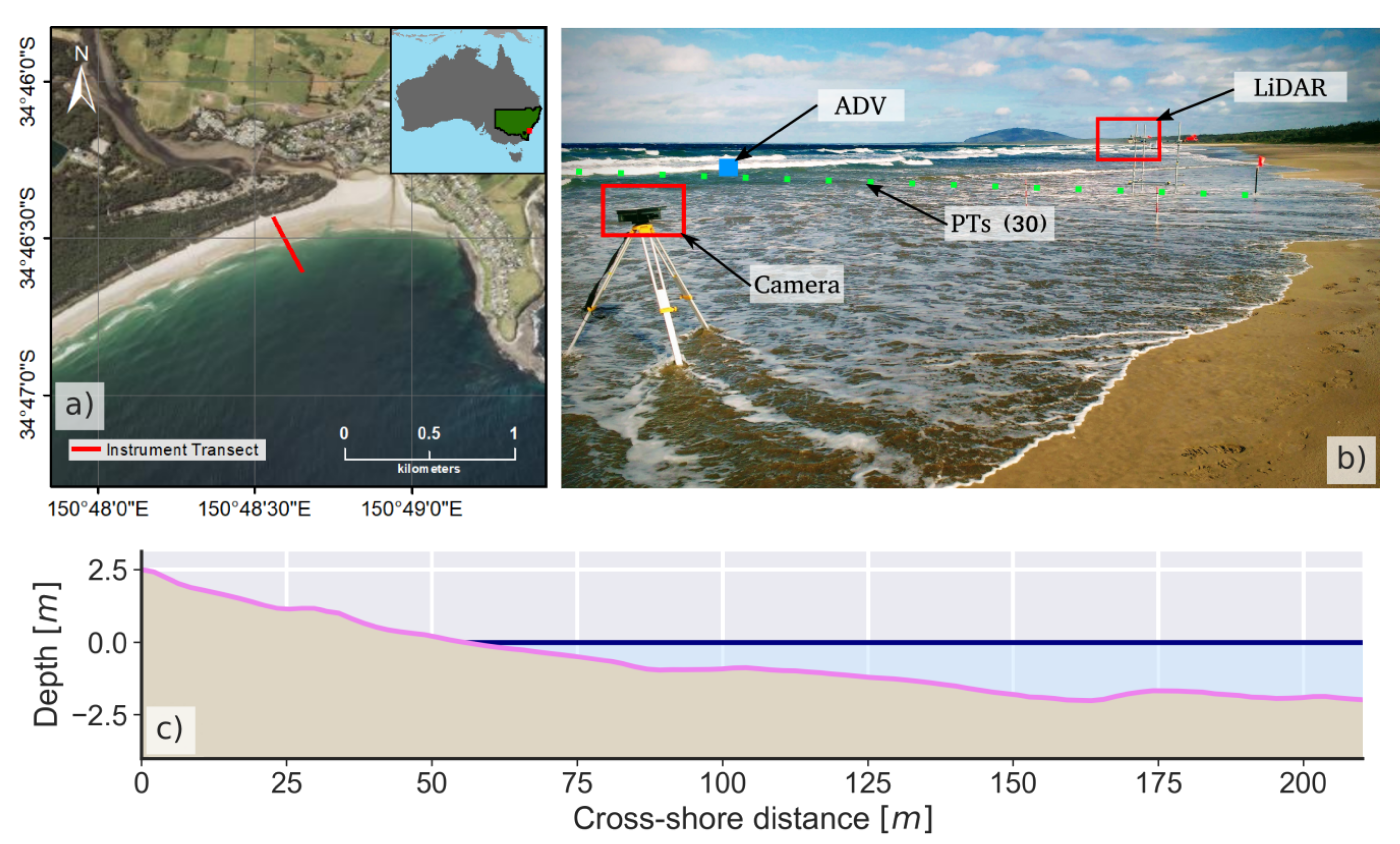
2. Materials and Methods
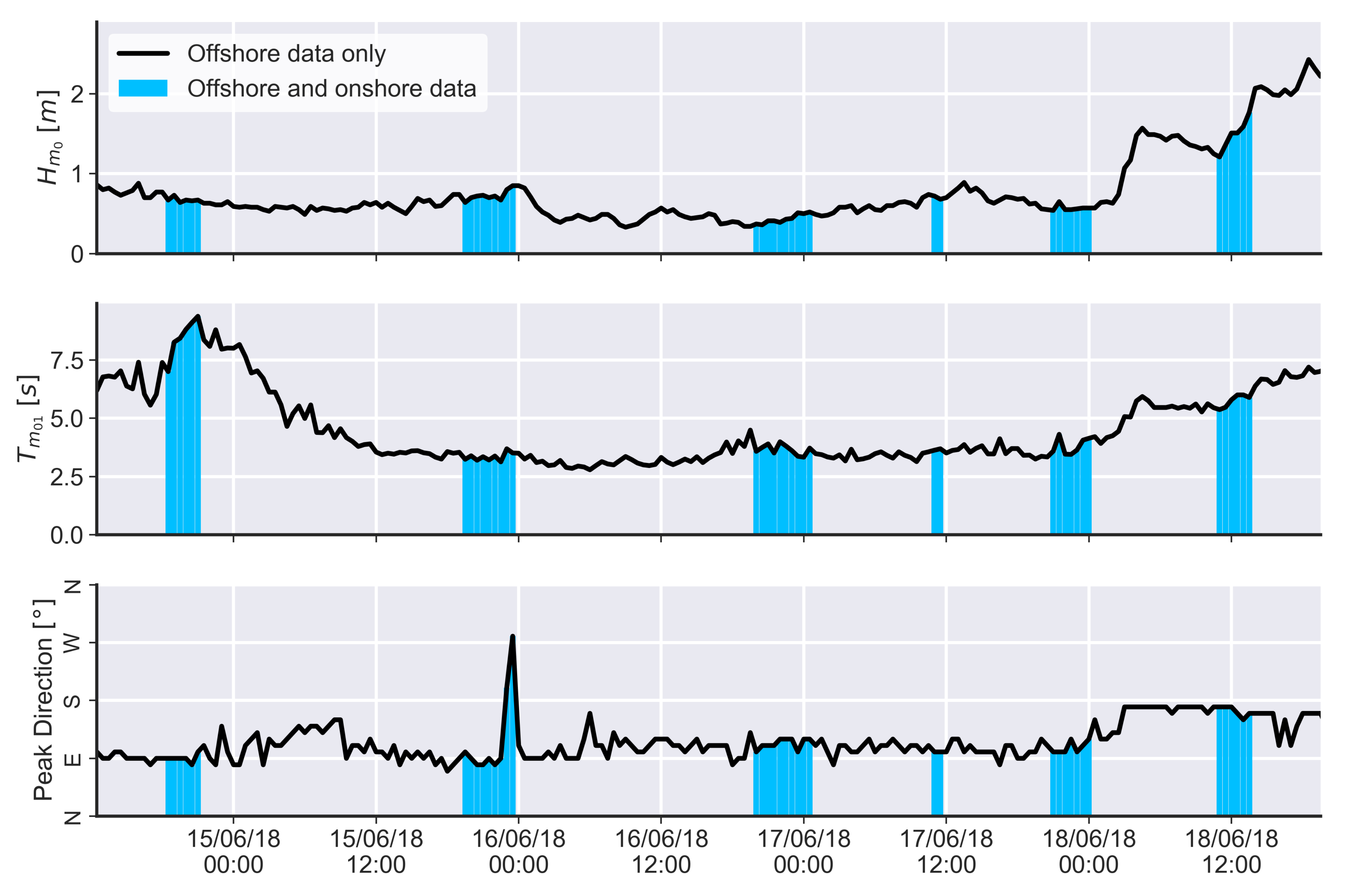
2.1. Data Collection
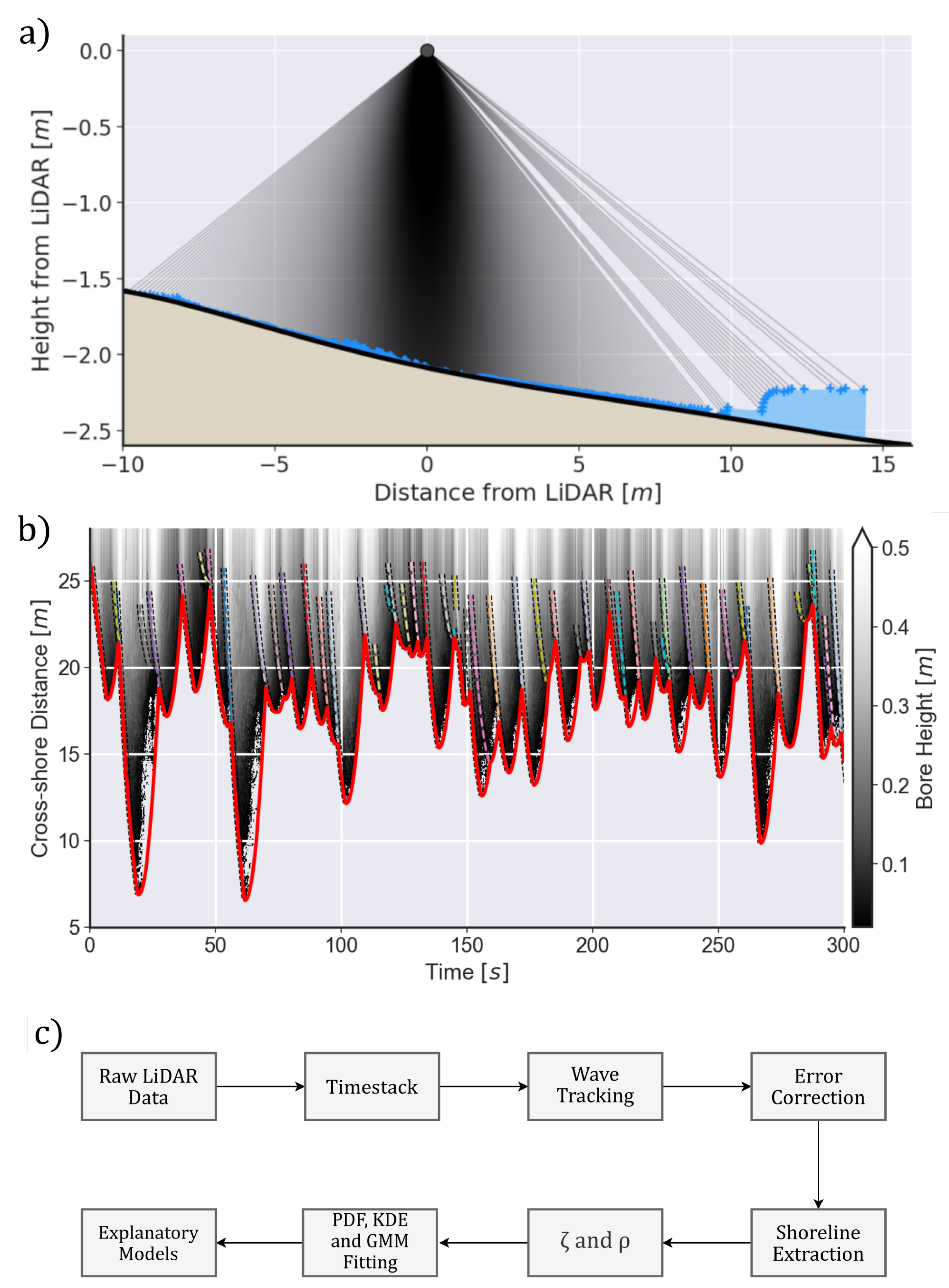
2.2. Data Processing
3. Results
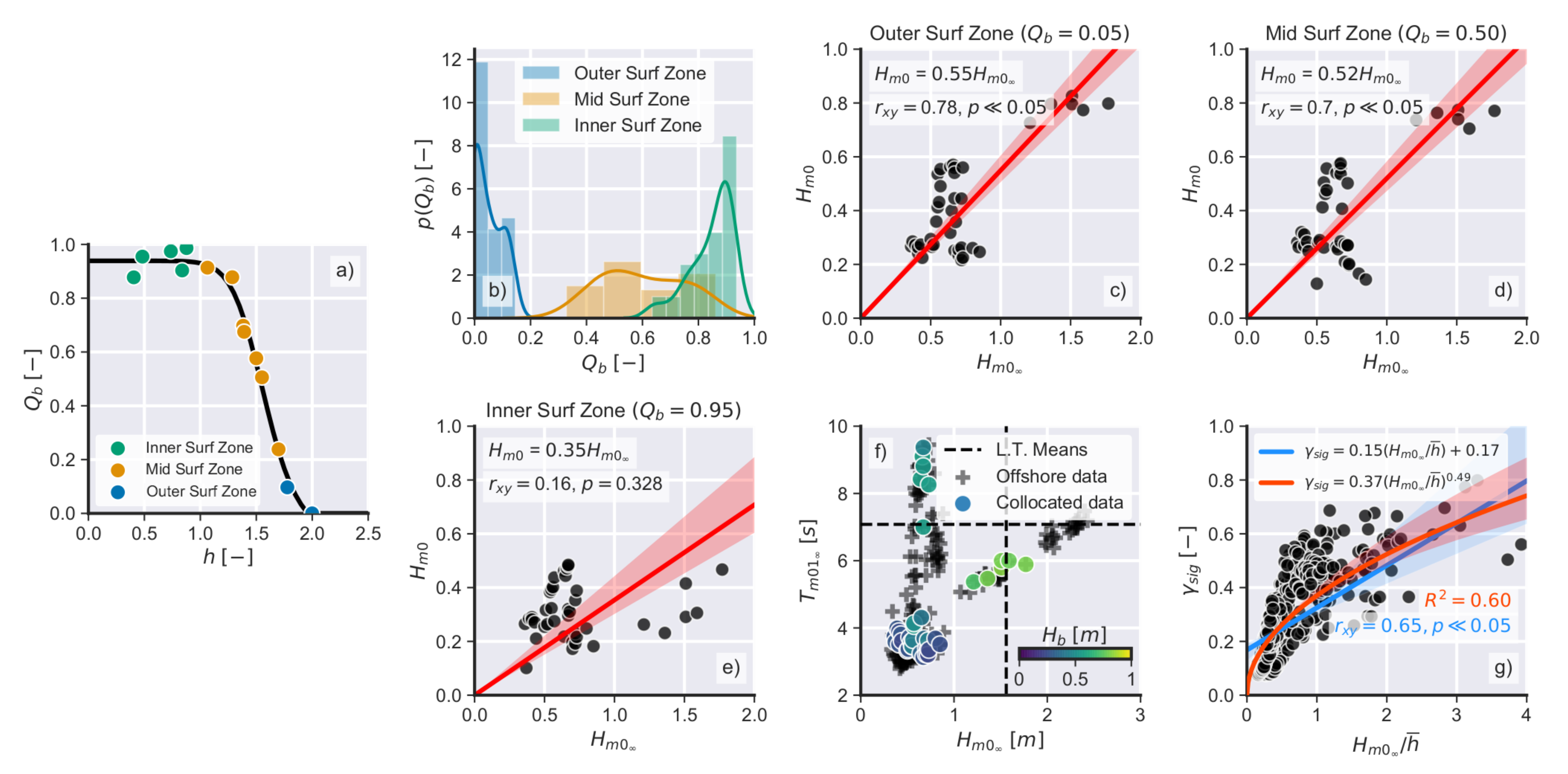
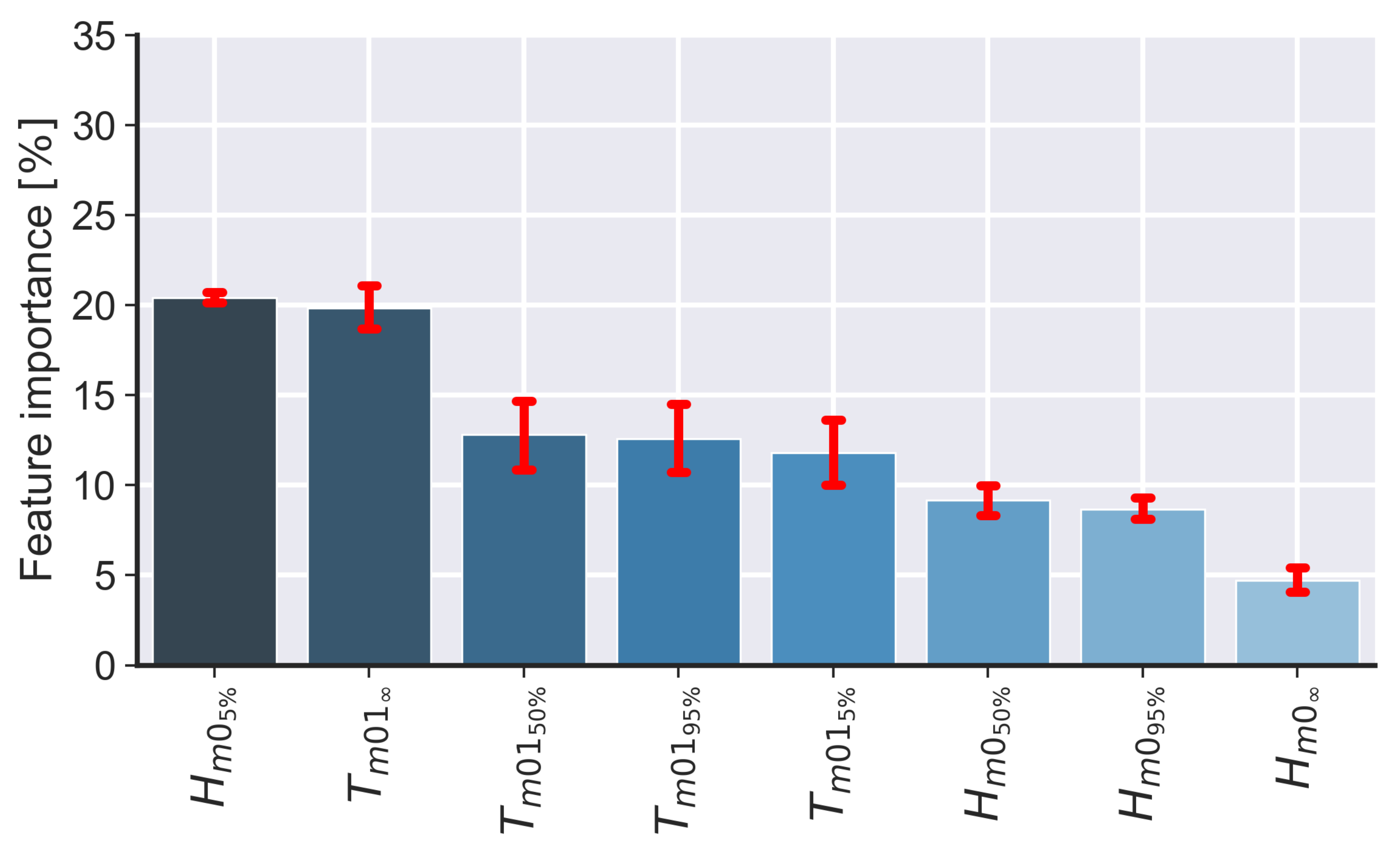
3.1. Surf Zone Dynamics
3.2. Shoreline Height Timeseries PDFs
3.3. Trough-To-Peak Swash Height PDFs
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Komar, P.D. Beach Processes and Sedimentation; Prentice-Hall: Upper Saddle River, NJ, USA, 1976. [Google Scholar]
- Masselink, G.; Puleo, J.A. Swash-zone morphodynamics. Cont. Shelf Res. 2006, 26, 661–680. [Google Scholar] [CrossRef]
- Atkinson, A.L.; Power, H.E.; Moura, T.; Hammond, T.; Callaghan, D.P.; Baldock, T.E. Assessment of runup predictions by empirical models on non-truncated beaches on the south-east Australian coast. Coast. Eng. 2017, 119, 15–31. [Google Scholar] [CrossRef]
- Power, H.E.; Gharabaghi, B.; Bonakdari, H.; Robertson, B.; Atkinson, A.L.; Baldock, T.E. Prediction of wave runup on beaches using Gene-Expression Programming and empirical relationships. Coast. Eng. 2019, 144, 47–61. [Google Scholar] [CrossRef]
- Hughes, M.G.; Moseley, A.S.; Baldock, T.E. Probability distributions for wave runup on beaches. Coast. Eng. 2010, 57, 575–584. [Google Scholar] [CrossRef]
- Holland, K.T.; Holman, R.A. The Statistical Distribution of Swash Maxima on Natural Beache. J. Geophys. Res. 1993, 98, 271–278. [Google Scholar] [CrossRef]
- Cartwright, D.E.; Longuet-Higgins, M.S. The Statistical Distribution of the Maxima of a Random Function. Proc. R. Soc. A Math. Phys. Eng. Sci. 1956, 283, 212–232. [Google Scholar]
- Goda, Y. Reanalysis of Regular and Random Breaking Wave Statistics. Coast. Eng. J. 2010, 52, 71–106. [Google Scholar] [CrossRef]
- Longuet-Higgins, M.S. On the Statistical Distribution of the Heights of Sea Waves. JMR 1952, 11, 245–266. [Google Scholar]
- Guza, R.T.; Thornton, E.B. Swash Oscillations on a Natural Beach. J. Geophys. Res. 1982, 87, 483–491. [Google Scholar] [CrossRef]
- Wright, L.D.; Guza, R.T.; Short, A.D. Dynamics of a high-energy dissipative surf zone. Mar. Geol. 1982, 45, 41–62. [Google Scholar] [CrossRef]
- Wright, L.D.; Short, A.D. Morphodynamic variability of surf zones and beaches: A synthesis. Mar. Geol. 1984, 56, 93–118. [Google Scholar] [CrossRef]
- Stringari, C.E.; Harris, D.L.; Power, H.E. A Novel Machine Learning Algorithm for Tracking Remotely Sensed Waves in the Surf Zone. Coast. Eng. 2019, 147, 149–158. [Google Scholar] [CrossRef]
- Stringari, C.E.; Power, H.E. The Fraction of Broken Waves in Natural Surf Zones. J. Geophys. Res. Ocean. 2019, 124, 1–27. [Google Scholar] [CrossRef]
- Power, H.E.; Kinsela, M.A.; Stringari, C.E.; Kendall, M.J.; Morris, B.D.; Hanslow, D.J. Automated sensing of wave inundation across a rocky shore platform using a low-cost camera system. Remote Sens. 2018, 10, 11. [Google Scholar] [CrossRef]
- Vousdoukas, M.I.; Kirupakaramoorthy, T.; Oumeraci, H.; de la Torre, M.; Wübbold, F.; Wagner, B.; Schimmels, S. The role of combined laser scanning and video techniques in monitoring wave-by-wave swash zone processes. Coast. Eng. 2014, 83, 150–165. [Google Scholar] [CrossRef]
- Aagaard, T.; Holm, J. Digitization of Wave Run-up Using Video Records. J. Coast. Res. 1989, 5, 547–551. [Google Scholar]
- Sobel, I. An Isotropic 3 × 3 Image Gradient Operator. Comput. Sci. 1968. [Google Scholar] [CrossRef]
- Ester, M.; Kriegel, H.P.; Sander, J.; Xu, X. Density-Based Clustering Methods. Compr. Chemom. 1996, 2, 635–654. [Google Scholar] [CrossRef]
- Fiedler, J.W.; Brodie, K.L.; McNinch, J.E.; Guza, R.T. Observations of runup and energy flux on a low-slope beach with high-energy, long-period ocean swell. Geophys. Res. Lett. 2015, 42, 9933–9941. [Google Scholar] [CrossRef]
- Bergsma, E.W.J.; Blenkinsopp, C.E.; MArtins, K.; Almar, R.; de Almeida, L.P.M. Bore collapse and wave run-up on a sandy beach. Cont. Shelf Res. 2019, 174, 132–139. [Google Scholar] [CrossRef]
- Roelse, A.; Granger, H.W.; Graham, J.W. Technical Report 12: The Adjustment of the Australian Levelling Survey 1970–1971; Division of National Mapping: Canberra, Australia, 1975. [Google Scholar]
- Power, H.E.; Hughes, M.G.; Aagaard, T.; Baldock, T.E. Nearshore wave height variation in unsaturated surf. J. Geophys. Res. Ocean. 2010, 115, 1–15. [Google Scholar] [CrossRef]
- Svendsen, I.A.; Madsen, P.A.; Hansen, J.B. Wave Characteristics in the Surf Zone. In Proceedings of the 16th International Conference on Coastal Engineering, Hamburg, Germany, 27 August–3 September 1978; pp. 520–539. [Google Scholar]
- Iribarren, C.R.; Nogales, C.M. Protection des Ports. In Proceedings of the XVIIth International Naval Congress, Lisbon, Portugal, 19–22 September 1949. [Google Scholar]
- Power, H.E.; Holman, R.A.; Baldock, T.E. Swash zone boundary conditions derived from optical remote sensing of swash zone flow patterns. J. Geophys. Res. Ocean. 2011, 116, 1–13. [Google Scholar] [CrossRef]
- Freedman, D.; Diaconis, P. On the histogram as a density estimator:L 2 theory. Z. Wahrscheinlichkeitstheorie Verwandte Geb. 1981, 57, 453–476. [Google Scholar] [CrossRef]
- New South Wales Department of Planning Industry, and Environment. Ocean Wave Data Collection Program. Available online: https://www.mhl.nsw.gov.au/Station-PTKMOW (accessed on 11 December 2020).
- Shapiro, S.S.; Wilk, M.B. An Analysis of Variance Test for Normality (Complete Samples). Biometrika 1965, 52, 311–319. [Google Scholar] [CrossRef]
- Kullback, S.; Leibler, R.A. On infromation and sufficiency. Ann. Math. Statist. 1951, 37, 688–697. [Google Scholar] [CrossRef]
- Jones, E.; Oliphant, T.; Peterson, P. SciPy: Open Source Scientific Tools for Python. 2001. Available online: https://www.scipy.org/ (accessed on 25 January 2021).
- Akaike, H. A New Look at the Statistical Model Identification. IEEE Trans. Autom. Control 1974, 19, 716–723. [Google Scholar] [CrossRef]
- Hogben, D.; Pinkham, R.S.; Wilk, M.B. The Moments of the Non-Central t-Distribution. Biometrika 1961, 48, 465–468. [Google Scholar] [CrossRef]
- Hastie, T.; Tibshirani, R.; Friedman, J. The Elements of Statistical Learning; Springer Series in Statistics; Springer: New York, NY, USA, 2001. [Google Scholar]
- Stringari, C.E.; Power, H.E. Quantifying Bore-bore Capture on Natural Beaches. J. Geophys. Res. Ocean. 2020, 125, 1–16. [Google Scholar] [CrossRef]
- Hughes, M.G.; Aagaard, T.; Baldock, T.E.; Power, H.E. Spectral signatures for swash on reflective, intermediate and dissipative beaches. Mar. Geol. 2014, 355, 88–97. [Google Scholar] [CrossRef]
- Hughes, M.G.; Baldock, T.E.; Aagaard, T. Swash saturation: An assessment of available models. Ocean Dyn. 2018, 68, 911–922. [Google Scholar] [CrossRef]
- Stringari, C.E. Data-Driven Investigations of Broken Wave Behaviour in the Surf And Swash Zones. Ph.D. Thesis, University of Newcastle, Callaghan, Australia, 2020. Available online: http://hdl.handle.net/1959.13/1411217 (accessed on 25 January 2021).
- Chen, T.; Guestrin, C. XGBoost: Reliable Large-scale Tree Boosting System. In Proceedings of the Conference on Knowledge Discovery and Data Mining, San Francisco, CA, USA, 13–17 August 2016; pp. 1–6. [Google Scholar]
- Friedman, J. Greedy Function Approximation: A Gradient Boosting Machine. Ann. Stat. 2001, 29, 1189–1232. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stringari, C.E.; Power, H.E. LiDAR Observations of Multi-Modal Swash Probability Distributions on a Dissipative Beach. Remote Sens. 2021, 13, 462. https://doi.org/10.3390/rs13030462
Stringari CE, Power HE. LiDAR Observations of Multi-Modal Swash Probability Distributions on a Dissipative Beach. Remote Sensing. 2021; 13(3):462. https://doi.org/10.3390/rs13030462
Chicago/Turabian StyleStringari, Caio Eadi, and Hannah E. Power. 2021. "LiDAR Observations of Multi-Modal Swash Probability Distributions on a Dissipative Beach" Remote Sensing 13, no. 3: 462. https://doi.org/10.3390/rs13030462
APA StyleStringari, C. E., & Power, H. E. (2021). LiDAR Observations of Multi-Modal Swash Probability Distributions on a Dissipative Beach. Remote Sensing, 13(3), 462. https://doi.org/10.3390/rs13030462






