A New Mapping Function for Spaceborne TEC Conversion Based on the Plasmaspheric Scale Height
Abstract
:1. Introduction
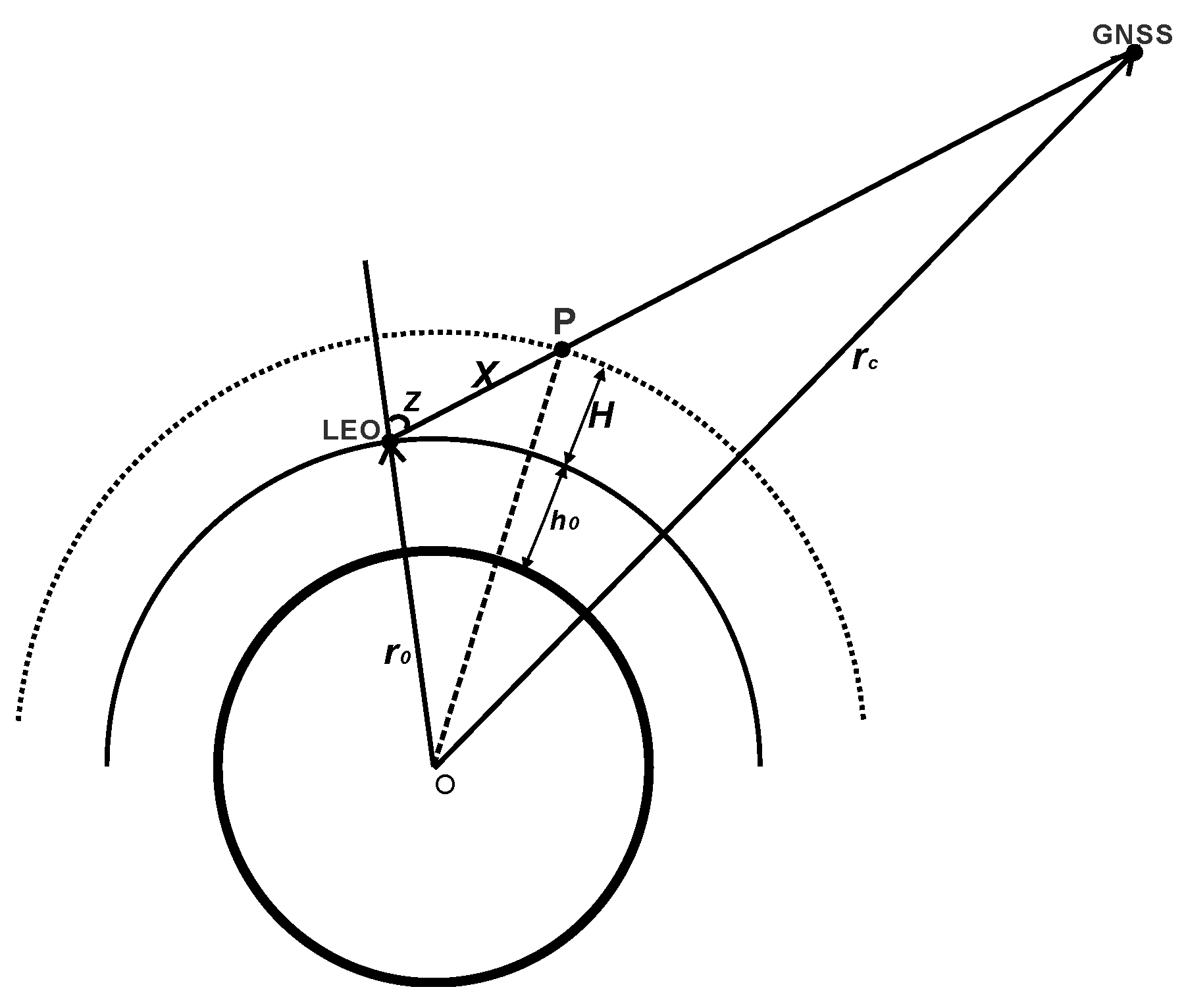
2. Materials and Methods
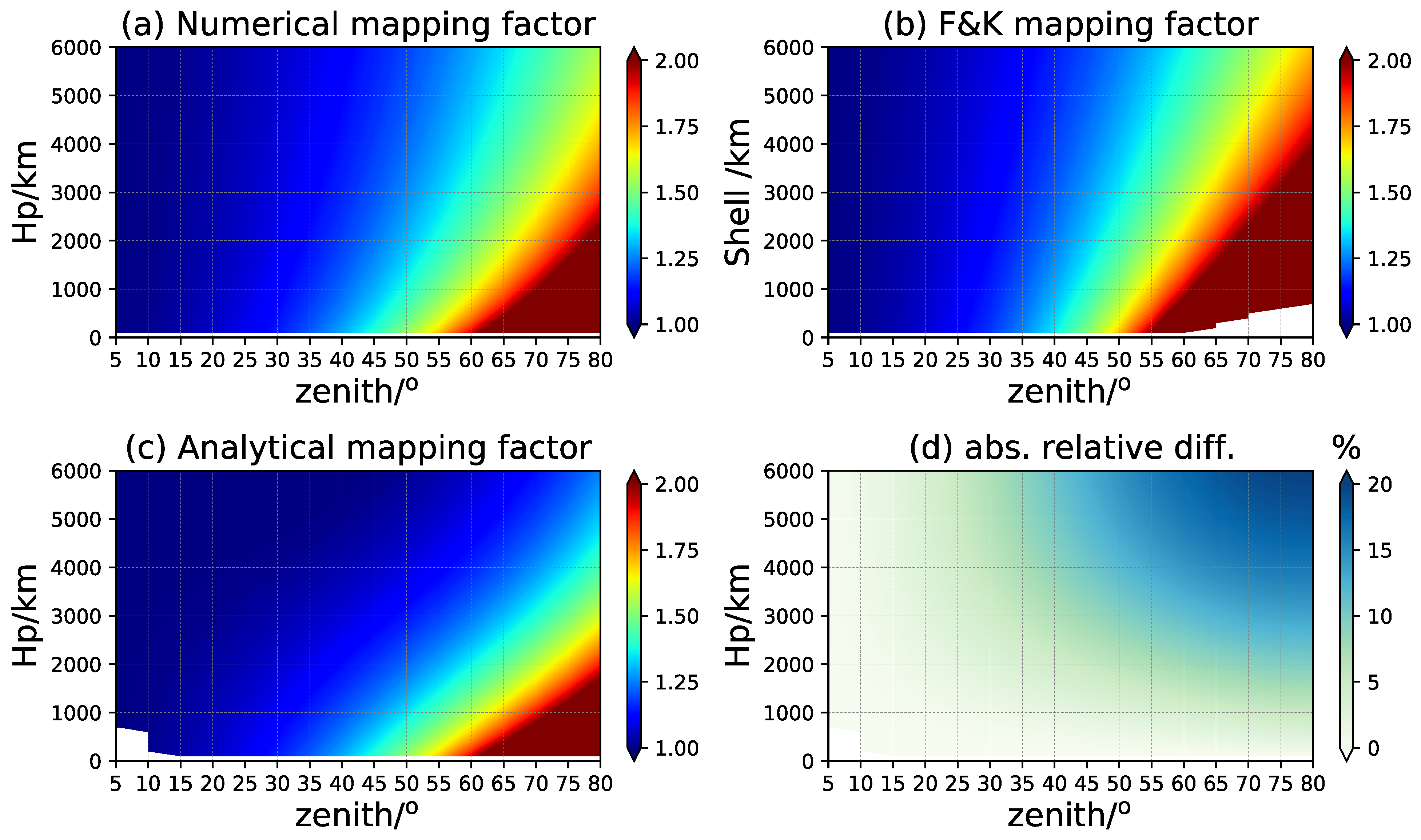
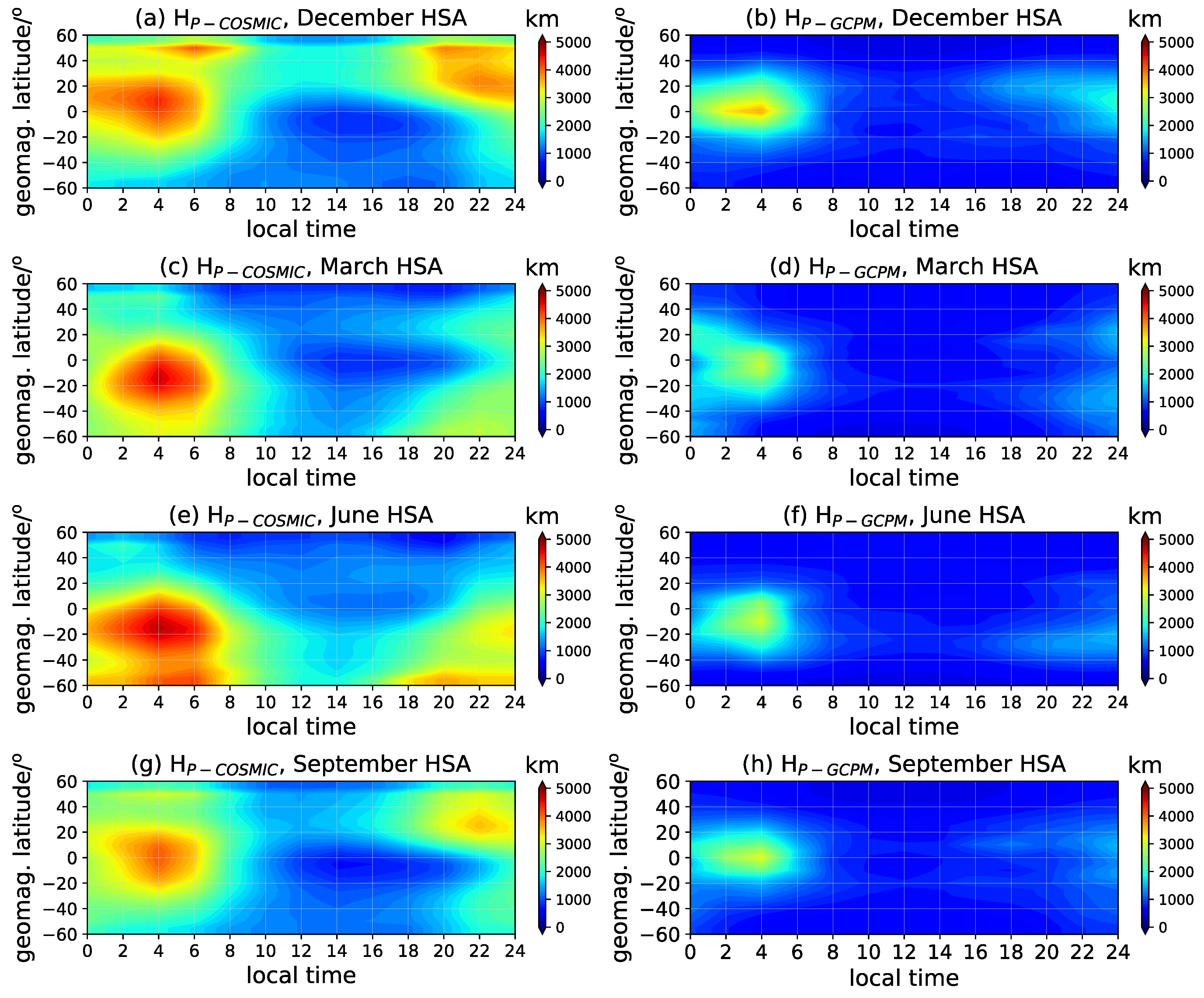
3. Results
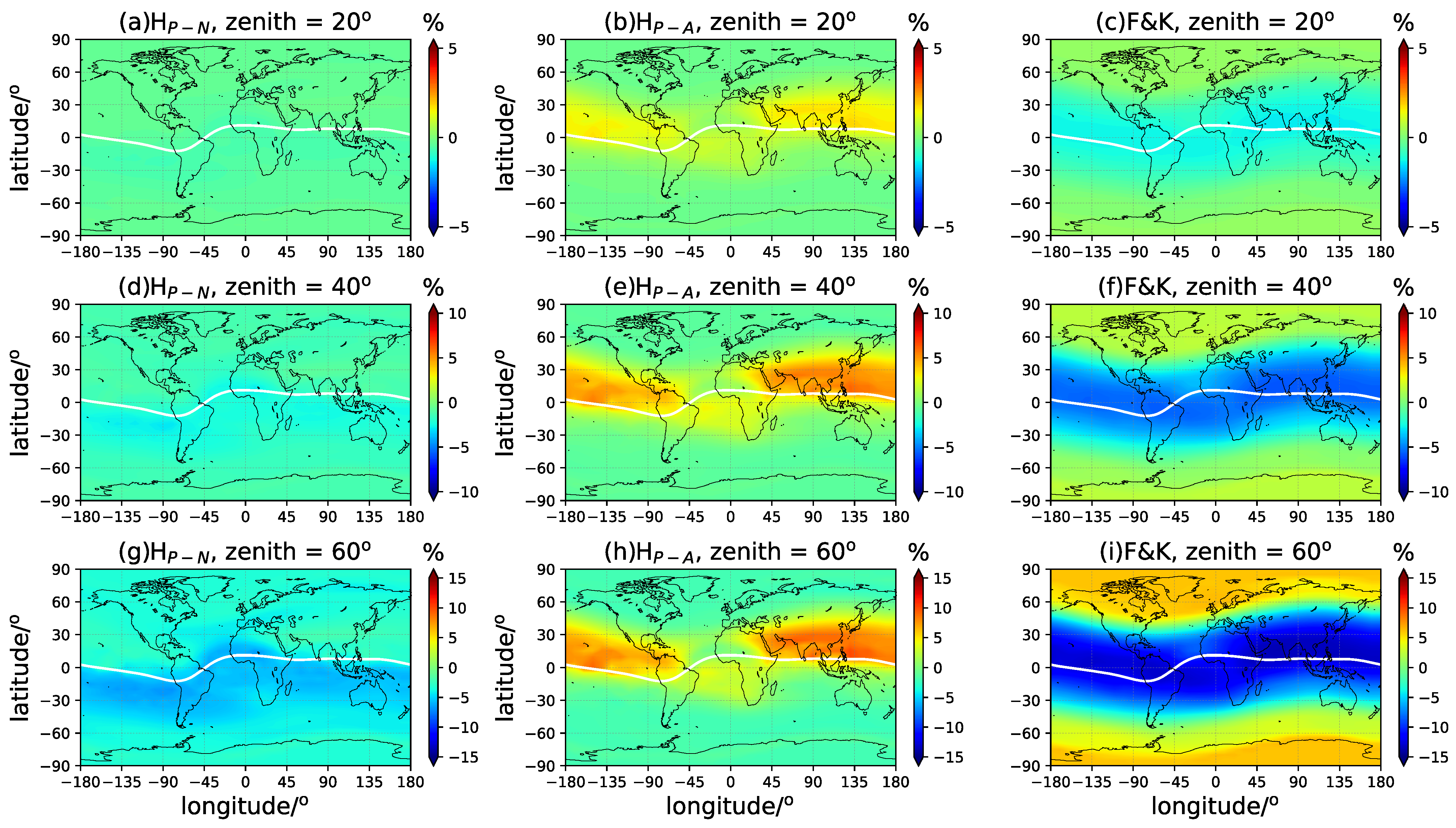
4. Discussion
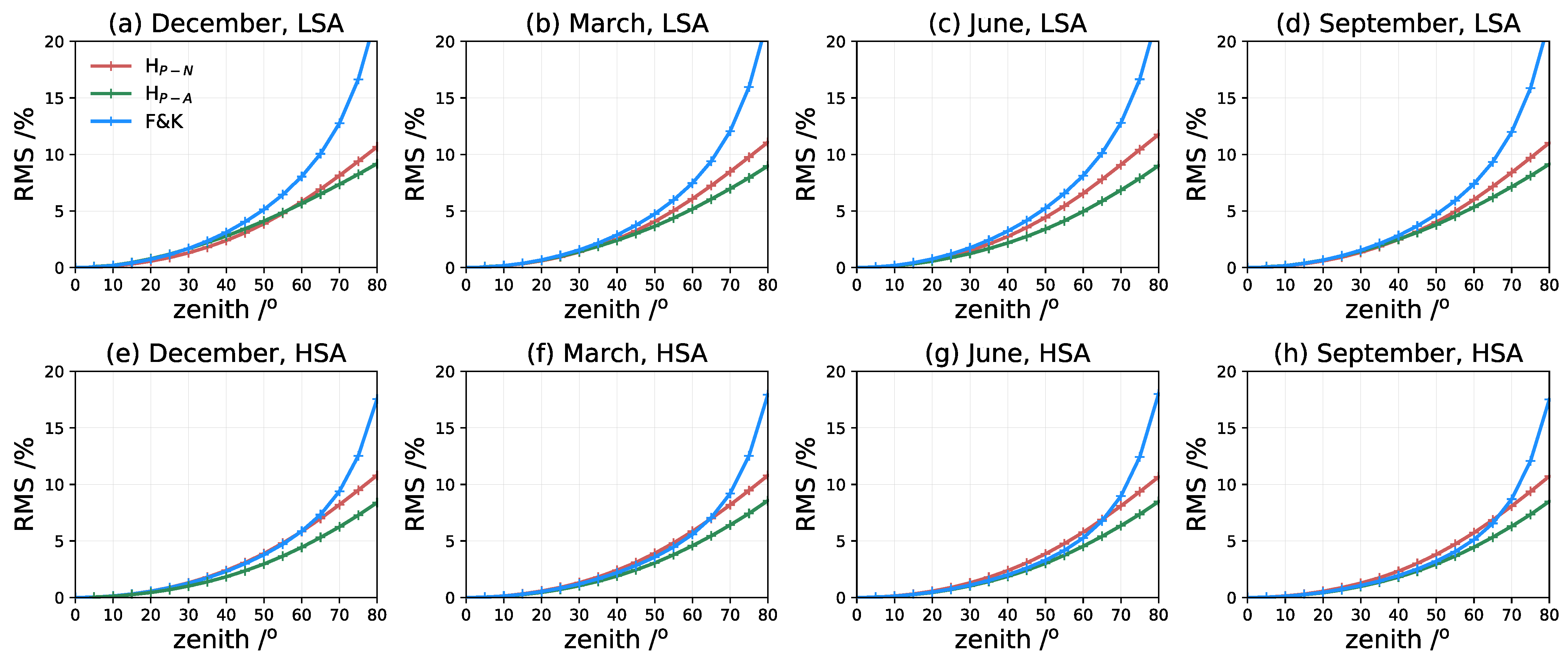
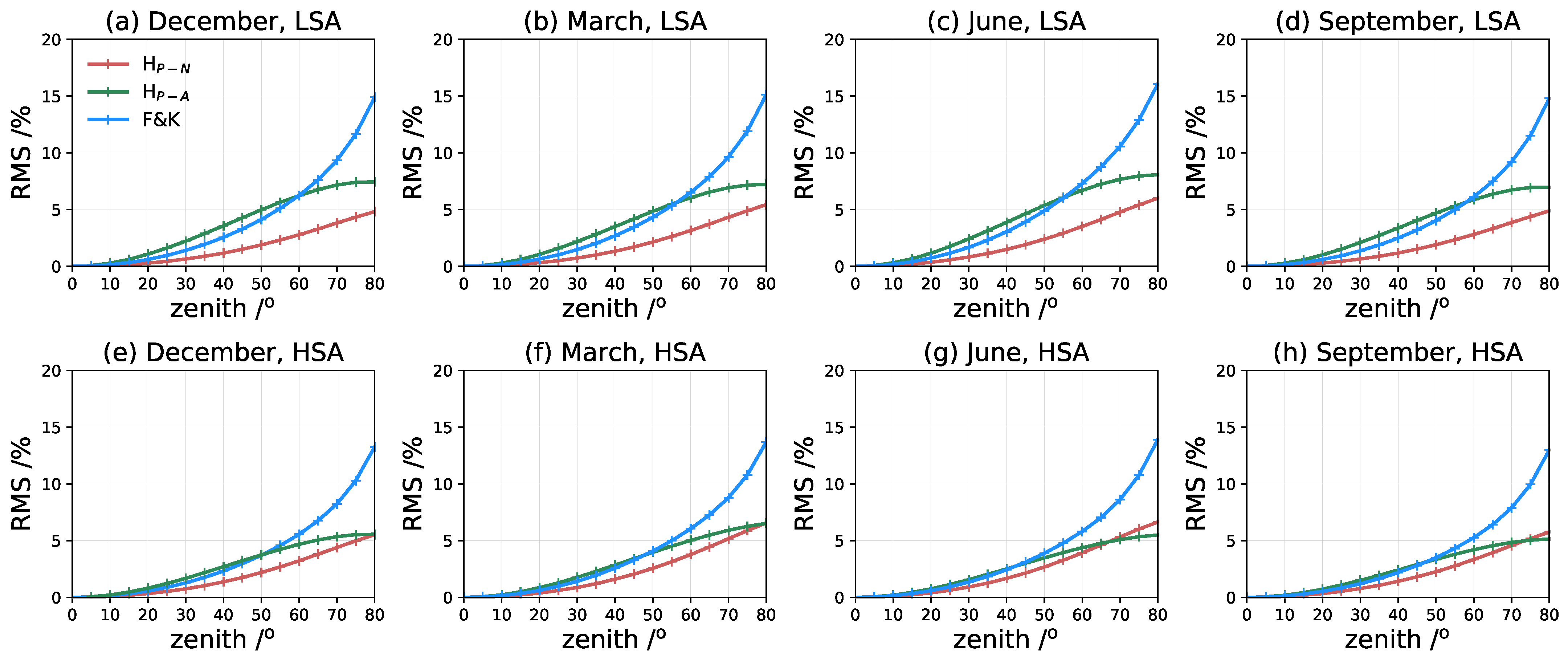
4.1. Assessment by Global Statistics
4.2. Global Variations of Mapping Errors
4.3. Influence of LEO Orbit Altitudes
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviation List
| TEC | total electron content |
| STEC | slant TEC |
| VTEC | vertical TEC |
| LEO | low Earth orbit |
| GCPM | global core plasma model |
| GNSS | global navigation satellite system |
| COSMIC | Constellation Observing System for Meteorology, Ionosphere, and Climate |
| DCB | differential code bias |
| MF | mapping function |
| TLM | thin layer model |
| IEH | ionospheric effective height |
| IRI | International Reference Ionosphere |
| MSA | medium solar activity |
| LSA | low solar activity |
| HAS | high solar activity |
| CDAAC | COSMIC data analysis and archive center |
| SBAS | space-based augmentation system |
| ISIS | International Satellites for Ionospheric Studies |
| RMS | root mean square |
Appendix A
References
- Schreiner, W.; Rocken, C.; Sokolovskiy, S.; Syndergaard, S.; Hunt, D. Estimates of the precision of GPS radio occultations from the COSMIC/FORMOSAT-3 mission. Geophys. Res. Lett. 2007, 34, 1–5. [Google Scholar] [CrossRef] [Green Version]
- Yue, X.; Schreiner, W.S.; Kuo, Y.H.; Hunt, D.C.; Wang, W.; Solomon, S.C.; Burns, A.G.; Bilitza, D.; Liu, J.Y.; Wan, W.; et al. Global 3-D ionospheric electron density reanalysis based on multisource data assimilation. J. Geophys. Res. Space Phys. 2012, 117, 1–17. [Google Scholar] [CrossRef] [Green Version]
- Lin, J.; Yue, X.; Zhao, S. Estimation and analysis of GPS satellite DCB based on LEO observations. GPS Solut. 2016, 20, 251–258. [Google Scholar] [CrossRef]
- Wu, M.J.; Guo, P.; Fu, N.F.; Hu, X.G.; Hong, Z.J. Improvement of the IRI Model Using F2 Layer Parameters Derived From GPS/COSMIC Radio Occultation Observations. J. Geophys. Res. Space Phys. 2018, 123, 9815–9835. [Google Scholar] [CrossRef]
- Fu, N.; Guo, P.; Wu, M.; Huang, Y.; Hu, X.; Hong, Z. The two-parts step-by-step ionospheric assimilation based on ground-based/spaceborne observations and its verification. Remote Sens. 2019, 11, 1172. [Google Scholar] [CrossRef] [Green Version]
- Mannucci, A.J.; Wilson, B.D.; Yuan, D.N.; Ho, C.H.; Lindqwister, U.J.; Runge, T.F. A global mapping technique for GPS-derived ionospheric total electron content measurements. Radio Sci. 1998, 33, 565–582. [Google Scholar] [CrossRef]
- Syndergaard, S. A new algorithm for retrieving GPS radio occultation total electron content. Geophys. Res. Lett. 2002, 29, 55-1–55-4. [Google Scholar] [CrossRef] [Green Version]
- Yue, X.; Schreiner, W.S.; Hunt, D.C.; Rocken, C.; Kuo, Y.H. Quantitative evaluation of the low Earth orbit satellite based slant total electron content determination. Space Weather 2011, 9. [Google Scholar] [CrossRef] [Green Version]
- Yuan, L.; Jin, S.; Hoque, M. Estimation of LEO-GPS receiver differential code bias based on inequality constrained least square and multi-layer mapping function. GPS Solut. 2020, 24, 57. [Google Scholar] [CrossRef]
- Noja, M.; Stolle, C.; Park, J.; Lühr, H. Long-term analysis of ionospheric polar patches based on CHAMP TEC data. Radio Sci. 2013, 48, 289–301. [Google Scholar] [CrossRef]
- Yuan, L.; Hoque, M.; Jin, S. A new method to estimate GPS satellite and receiver differential code biases using a network of LEO satellites. GPS Solut. 2021, 25, 71. [Google Scholar] [CrossRef]
- Klobuchar, J.A. Ionospheric Time-Delay Algorithm for Single-Frequency GPS Users. IEEE Trans. Aerosp. Electron. Syst. 1987, AES-23, 325–331. [Google Scholar] [CrossRef]
- Foelsche, U.; Kirchengast, G. A simple “geometric” mapping function for the hydrostatic delay at radio frequencies and assessment of its performance. Geophys. Res. Lett. 2002, 29, 1473. [Google Scholar] [CrossRef] [Green Version]
- Schaer, S. Mapping and predicting the Earth’s ionosphere using the Global Positioning System. Ph.D. TThesis, University of Bern, Bern, Switzerland, 1999. [Google Scholar]
- Hoque, M.M.; Jakowski, N. Mitigation of ionospheric mapping function error. In Proceedings of the 26th International Technical Meeting of the Satellite Division of the Institute of Navigation, ION GNSS+ 2013, Nashville, Tennessee, 16–20 September 2013; Volume 3, pp. 1848–1855. [Google Scholar]
- Xiang, Y.; Gao, Y. An enhanced mapping function with ionospheric varying height. Remote Sens. 2019, 11, 1497. [Google Scholar] [CrossRef] [Green Version]
- Lyu, H.; Hernández-Pajares, M.; Nohutcu, M.; García-Rigo, A.; Zhang, H.; Liu, J. The Barcelona ionospheric mapping function (BIMF) and its application to northern mid-latitudes. GPS Solut. 2018, 22, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Su, K.; Jin, S.; Jiang, J.; Hoque, M.; Yuan, L. Ionospheric VTEC and satellite DCB estimated from single-frequency BDS observations with multi-layer mapping function. GPS Solut. 2021, 25, 67. [Google Scholar] [CrossRef]
- Zhong, J.; Lei, J.; Dou, X.; Yue, X. Assessment of vertical TEC mapping functions for space-based GNSS observations. GPS Solut. 2016, 20, 353–362. [Google Scholar] [CrossRef]
- Huang, Z.; Yuan, H. Analysis and improvement of ionospheric thin shell model used in SBAS for China region. Adv. Space Res. 2013, 51, 2035–2042. [Google Scholar] [CrossRef]
- Bilitza, D.; Altadill, D.; Truhlik, V.; Shubin, V.; Galkin, I.; Reinisch, B.; Huang, X. International Reference Ionosphere 2016: From ionospheric climate to real-time weather predictions. Space Weather 2017, 15, 418–429. [Google Scholar] [CrossRef]
- Gulyaeva, T.L.; Nava, B.; Stanislawska, I. Modeling Center-of-Mass of the Ionosphere From the Slab-Thickness. Radio Sci. 2021, 56, 1–17. [Google Scholar] [CrossRef]
- Pedatella, N.M.; Zakharenkova, I.; Braun, J.J.; Cherniak, I.; Hunt, D.; Schreiner, W.S.; Straus, P.R.; Valant-Weiss, B.L.; Vanhove, T.; Weiss, J.; et al. Processing and Validation of FORMOSAT-7/COSMIC-2 GPS Total Electron Content Observations. Radio Sci. 2021, 56, 1–15. [Google Scholar] [CrossRef]
- Hernàndez-Pajares, M.; Juan, J.M.; Sanz, J.; Garcia-Fernàndez, M. Towards a more realistic ionospheric mapping function. In Proceedings of the XXVIII URSI General Assembly, Delhi, India, 23–29 October 2005. [Google Scholar]
- Pignalberi, A.; Pezzopane, M.; Nava, B.; Coïsson, P. On the link between the topside ionospheric effective scale height and the plasma ambipolar diffusion, theory and preliminary results. Sci. Rep. 2020, 10, 17541. [Google Scholar] [CrossRef] [PubMed]
- Wu, M.; Xu, X.; Li, F.; Guo, P.; Fu, N. Plasmaspheric scale height modeling based on COSMIC radio occultation data. J. Atmos. Solar-Terr. Phys. 2021, 217, 105555. [Google Scholar] [CrossRef]
- Liu, L.; Le, H.; Wan, W.; Sulzer, M.P.; Lei, J.; Zhang, M.L. An analysis of the scale heights in the lower topside ionosphere based on the Arecibo incoherent scatter radar measurements. J. Geophys. Res. Space Phys. 2007, 112. [Google Scholar] [CrossRef] [Green Version]
- Liu, L.; Wan, W.; Zhang, M.L.; Ning, B.; Zhang, S.R.; Holt, J.M. Variations of topside ionospheric scale heights over Millstone Hill during the 30-day incoherent scatter radar experiment. Ann. Geophys. 2007, 25, 2019–2027. [Google Scholar] [CrossRef] [Green Version]
- Luan, X.; Liu, L.; Wan, W.; Lei, J.; Zhang, S.R.; Holt, J.M.; Sulzer, M.P. A study of the shape of topside electron density profile derived from incoherent scatter radar measurements over Arecibo and Millstone Hill. Radio Sci. 2006, 41, 1–11. [Google Scholar] [CrossRef]
- Stankov, S.M.; Jakowski, N.; Heise, S.; Muhtarov, P.; Kutiev, I.; Warnant, R. A new method for reconstruction of the vertical electron density distribution in the upper ionosphere and plasmasphere. J. Geophys. Res. Space Phys. 2003, 108. [Google Scholar] [CrossRef]
- Hoque, M.M.; Jakowski, N.; Berdermann, J. A new approach for mitigating ionospheric mapping function errors. In Proceedings of the 27th International Technical Meeting of the Satellite Division of the Institute of Navigation, Tampa, FL, USA, 8–12 September 2014. [Google Scholar]
- Gallagher, D.L.; Craven, P.D.; Comfort, R.H. Global core plasma model. J. Geophys. Res. Space Phys. 2000, 105, 18819–18833. [Google Scholar] [CrossRef]
- Fonda, C.; Coïsson, P.; Nava, B.; Radicella, S.M. Comparison of analytical functions used to describe topside electron density profiles with satellite data. Ann. Geophys. 2009, 48. [Google Scholar] [CrossRef]
- Pignalberi, A.; Pezzopane, M.; Rizzi, R. Modeling the Lower Part of the Topside Ionospheric Vertical Electron Density Profile Over the European Region by Means of Swarm Satellites Data and IRI UP Method. Space Weather 2018, 16, 304–320. [Google Scholar] [CrossRef]
- Stankov, T.; Verhulst, S.M. Evaluation of ionospheric profilers using topside sounding data. Radio Sci. 2014, 49, 181–195. [Google Scholar] [CrossRef]
- Kutiev, I.; Marinov, P. Topside sounder model of scale height and transition height characteristics of the ionosphere. Adv. Space Res. 2007, 39, 759–766. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, M.; Guo, P.; Zhou, W.; Xue, J.; Han, X.; Meng, Y.; Hu, X. A New Mapping Function for Spaceborne TEC Conversion Based on the Plasmaspheric Scale Height. Remote Sens. 2021, 13, 4758. https://doi.org/10.3390/rs13234758
Wu M, Guo P, Zhou W, Xue J, Han X, Meng Y, Hu X. A New Mapping Function for Spaceborne TEC Conversion Based on the Plasmaspheric Scale Height. Remote Sensing. 2021; 13(23):4758. https://doi.org/10.3390/rs13234758
Chicago/Turabian StyleWu, Mengjie, Peng Guo, Wei Zhou, Junchen Xue, Xingyuan Han, Yansong Meng, and Xiaogong Hu. 2021. "A New Mapping Function for Spaceborne TEC Conversion Based on the Plasmaspheric Scale Height" Remote Sensing 13, no. 23: 4758. https://doi.org/10.3390/rs13234758
APA StyleWu, M., Guo, P., Zhou, W., Xue, J., Han, X., Meng, Y., & Hu, X. (2021). A New Mapping Function for Spaceborne TEC Conversion Based on the Plasmaspheric Scale Height. Remote Sensing, 13(23), 4758. https://doi.org/10.3390/rs13234758




