Electromagnetic Scattering Model for Far Wakes of Ship with Wind Waves on Sea Surface
Abstract
1. Introduction
2. Method
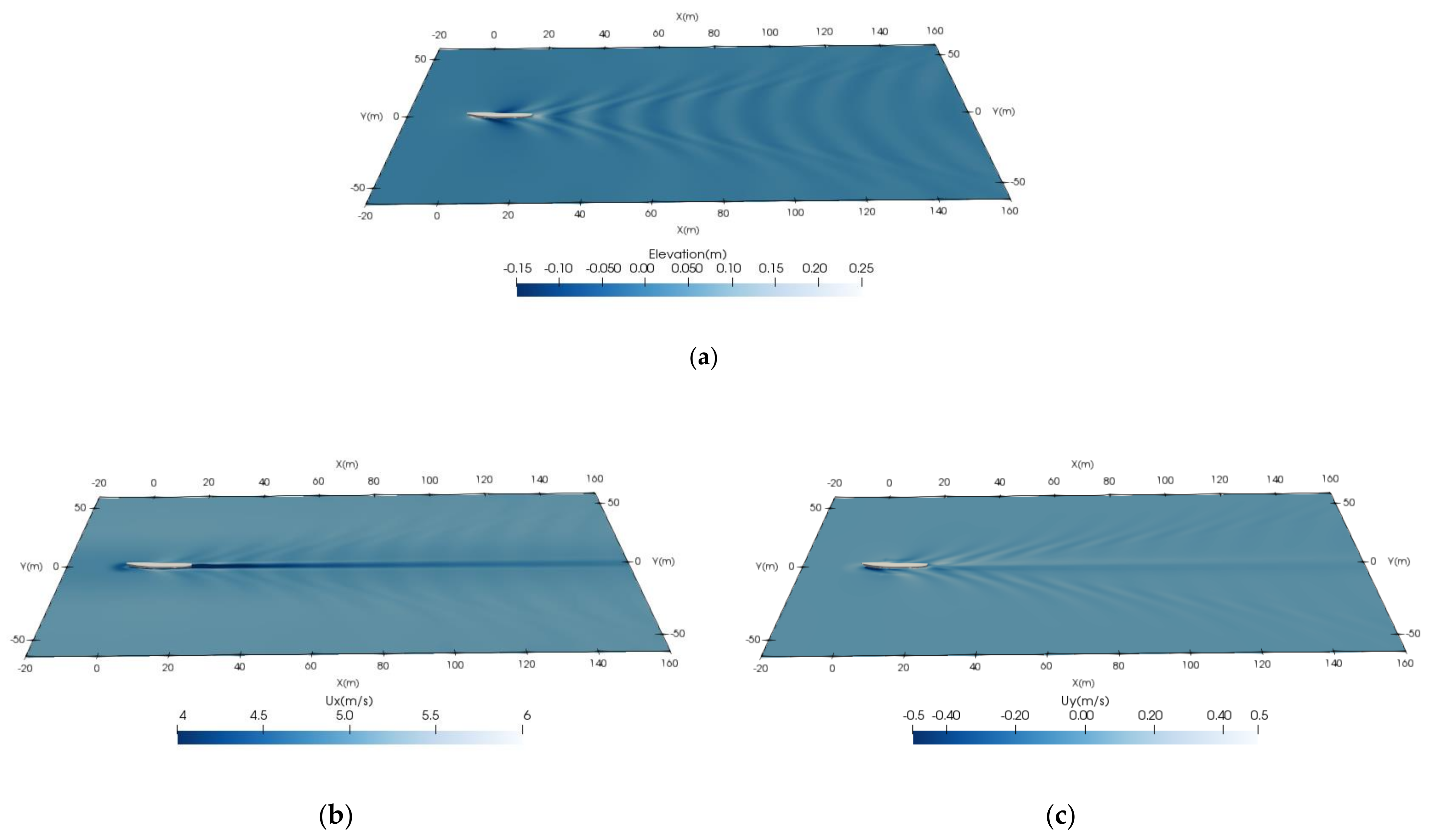
2.1. Simulation of the Ship Wake Model
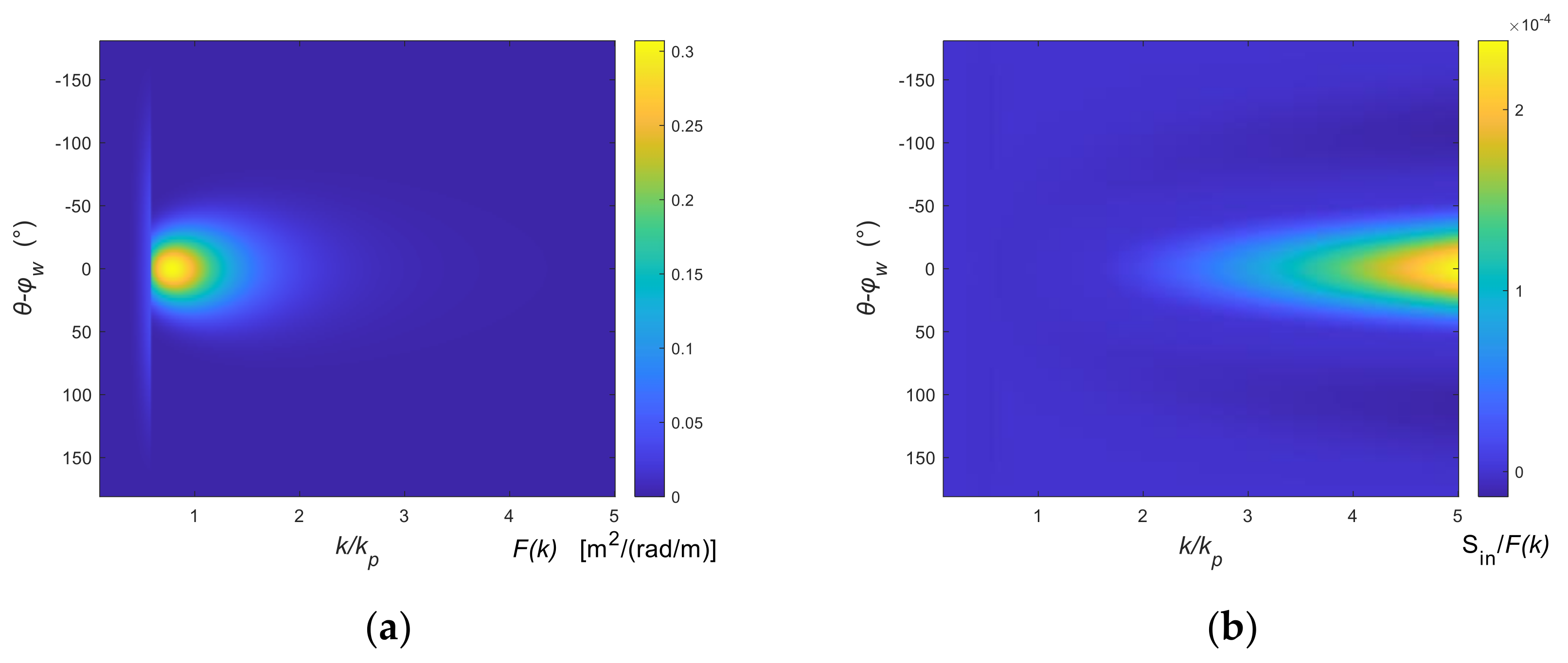
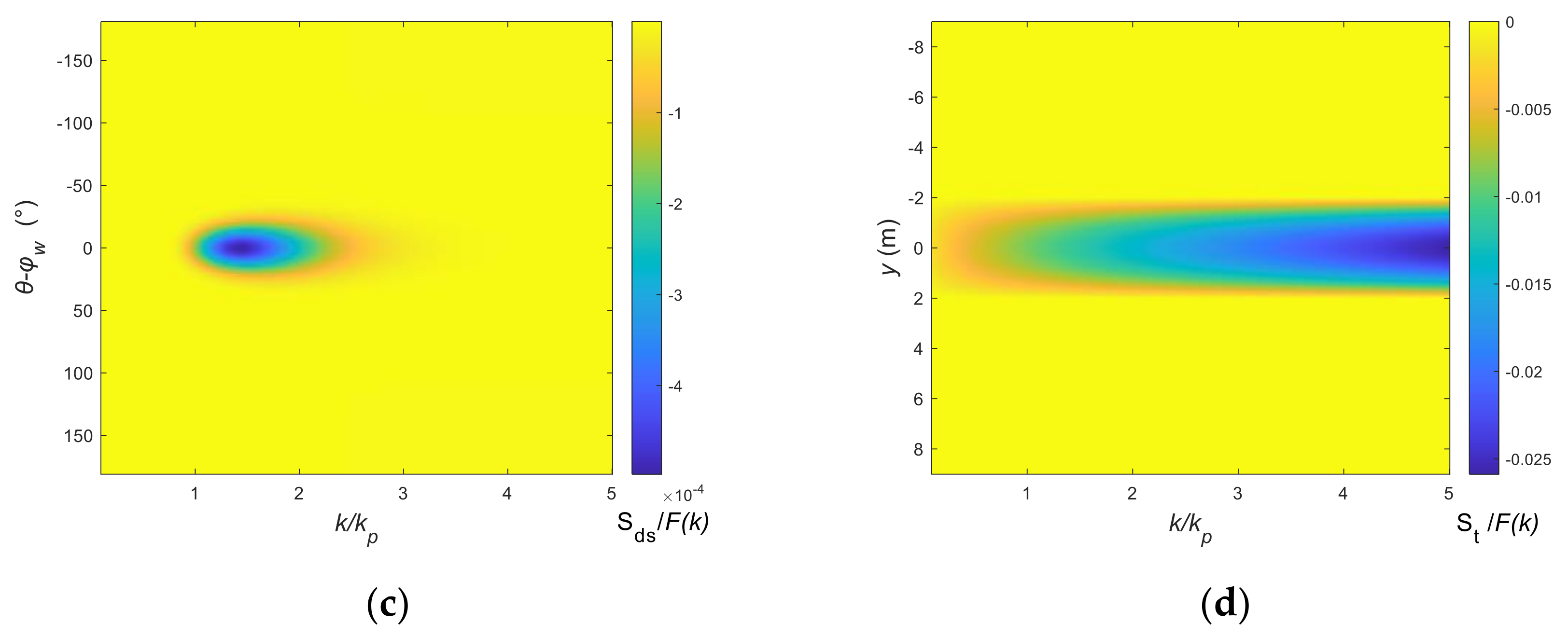
2.2. Modulation Spectrum Model of the Mixed Water Waves
2.3. Modulation Facet Scattering Model
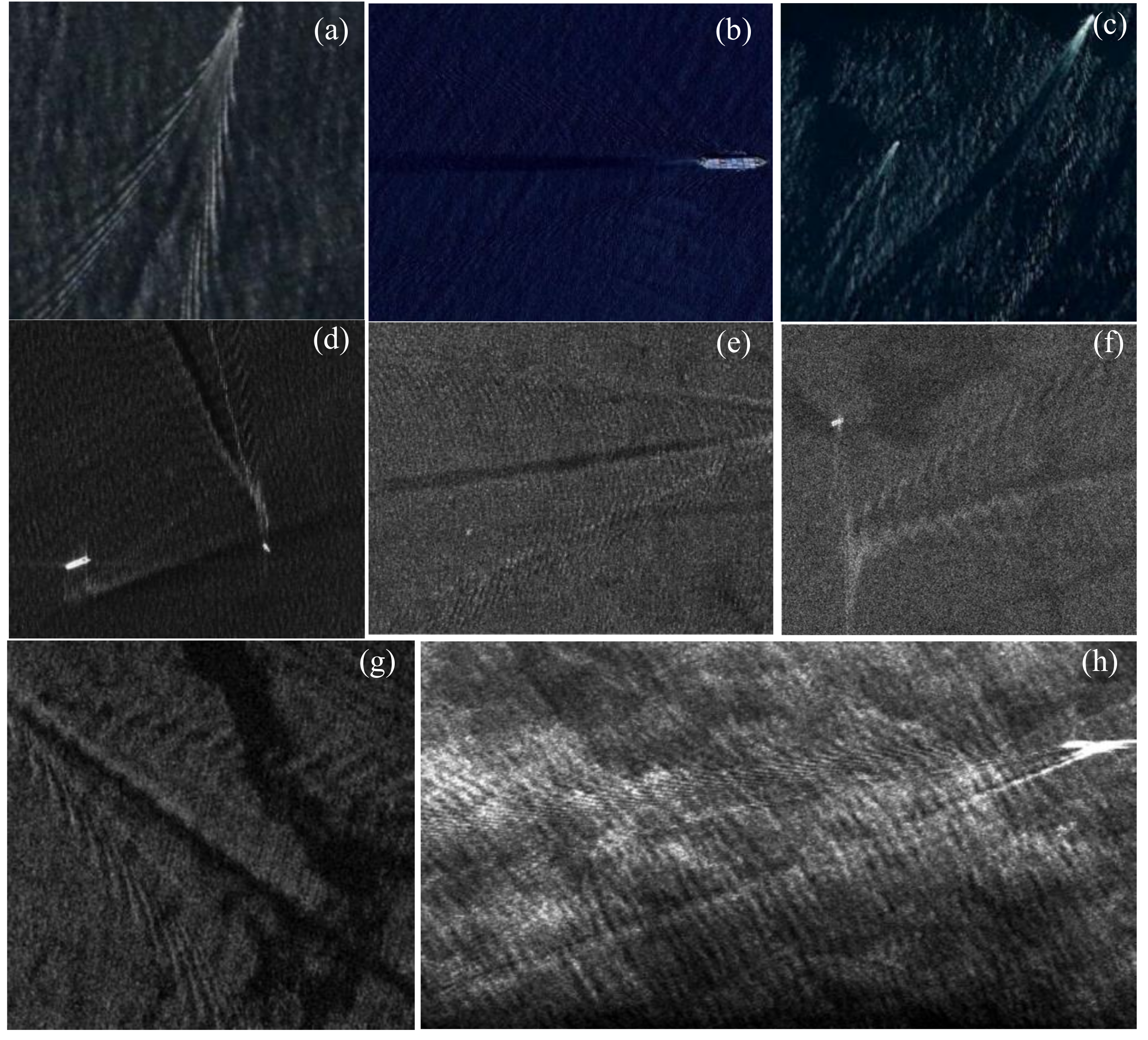
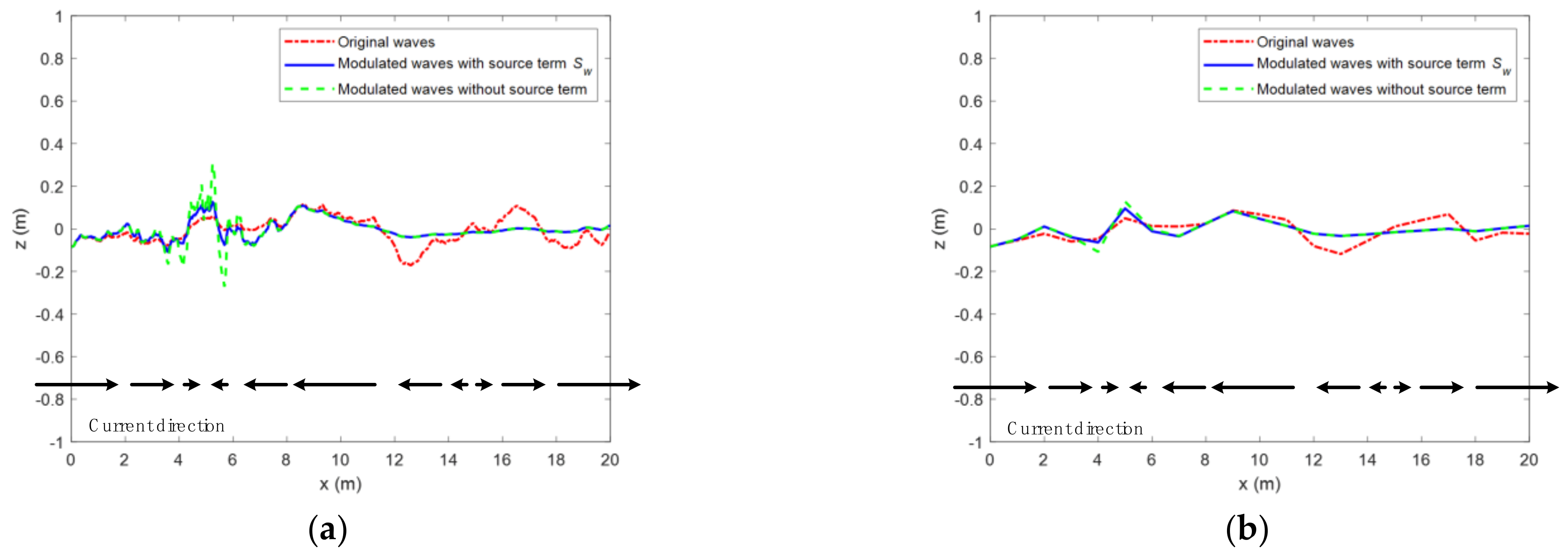
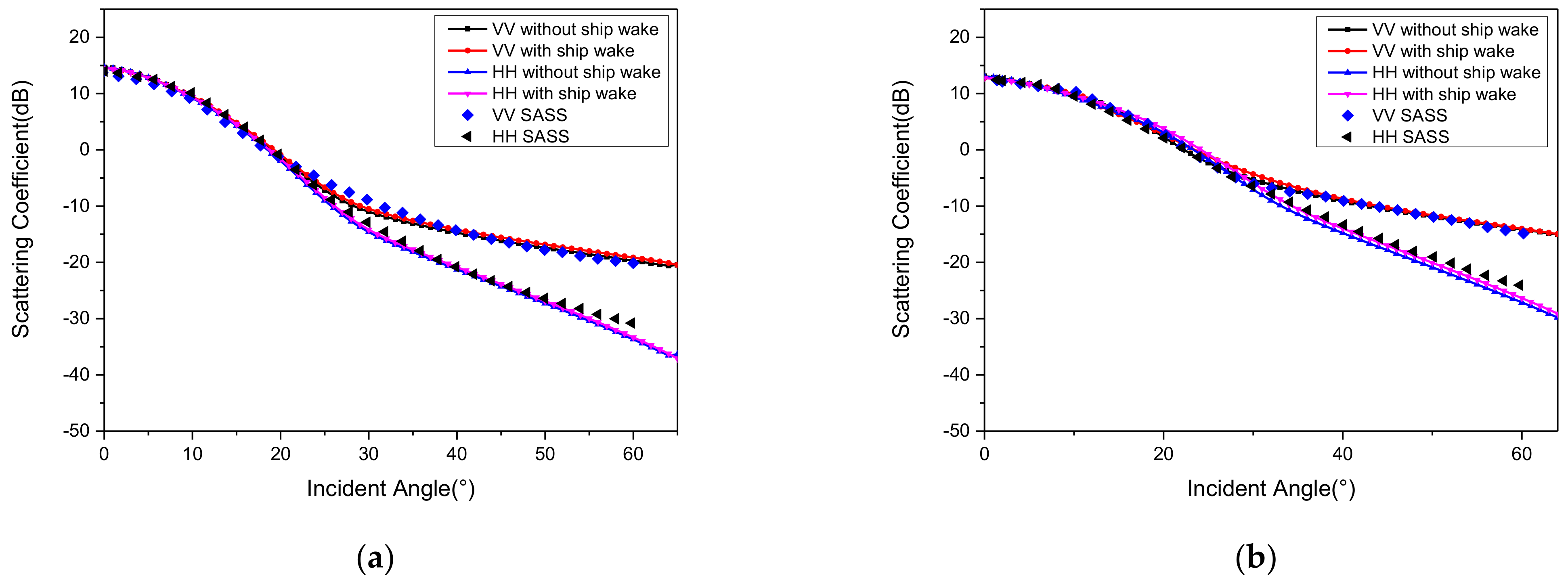
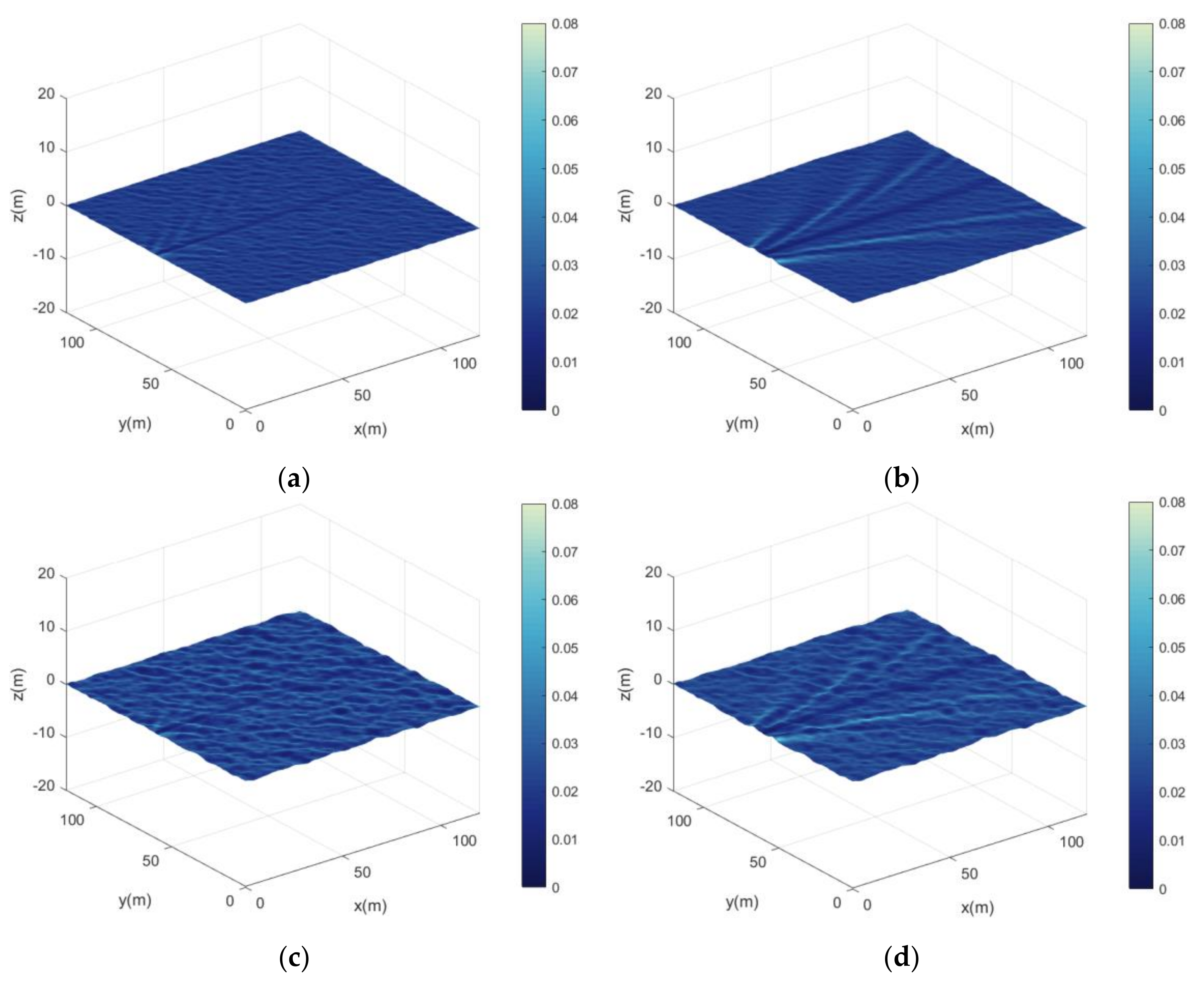
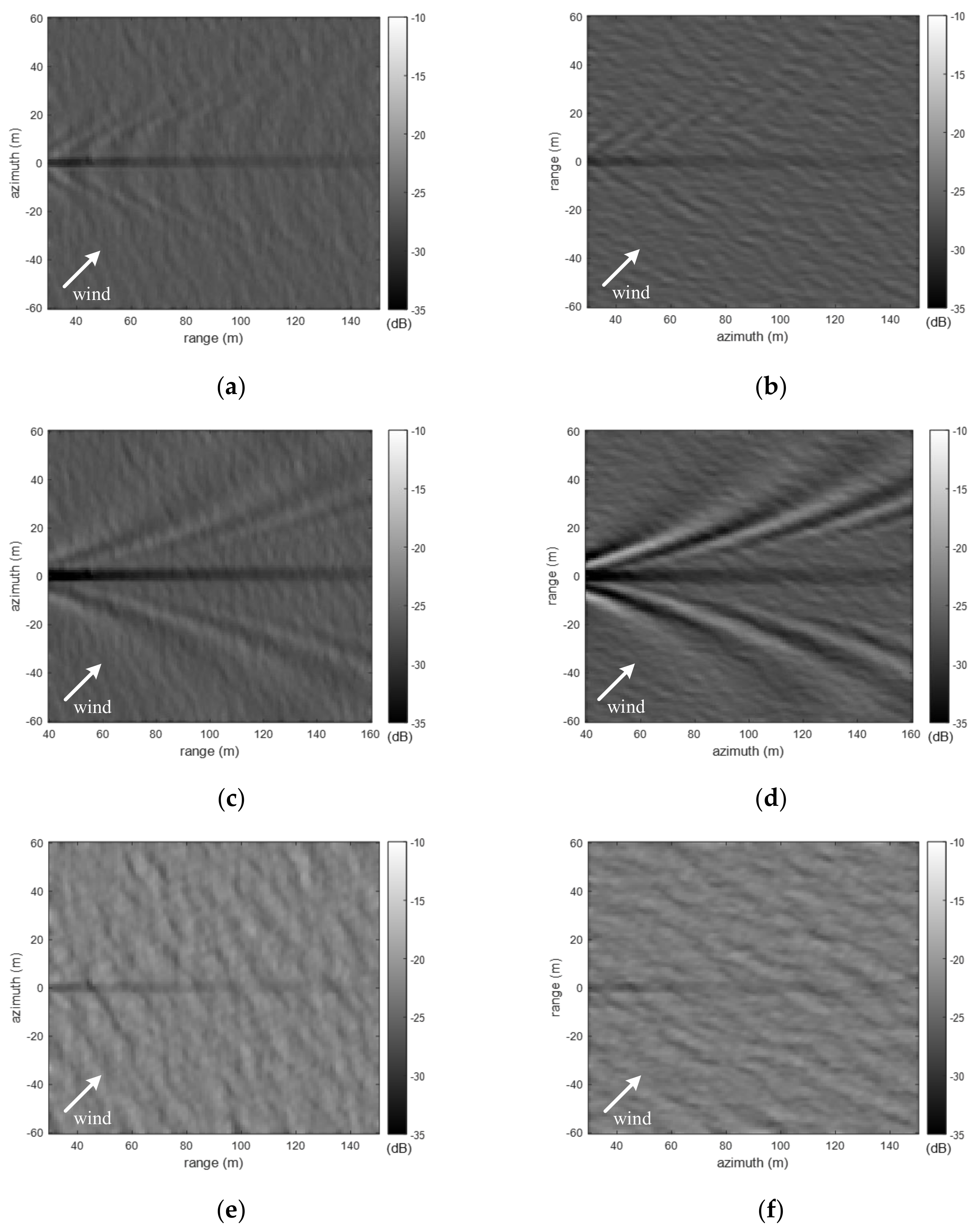
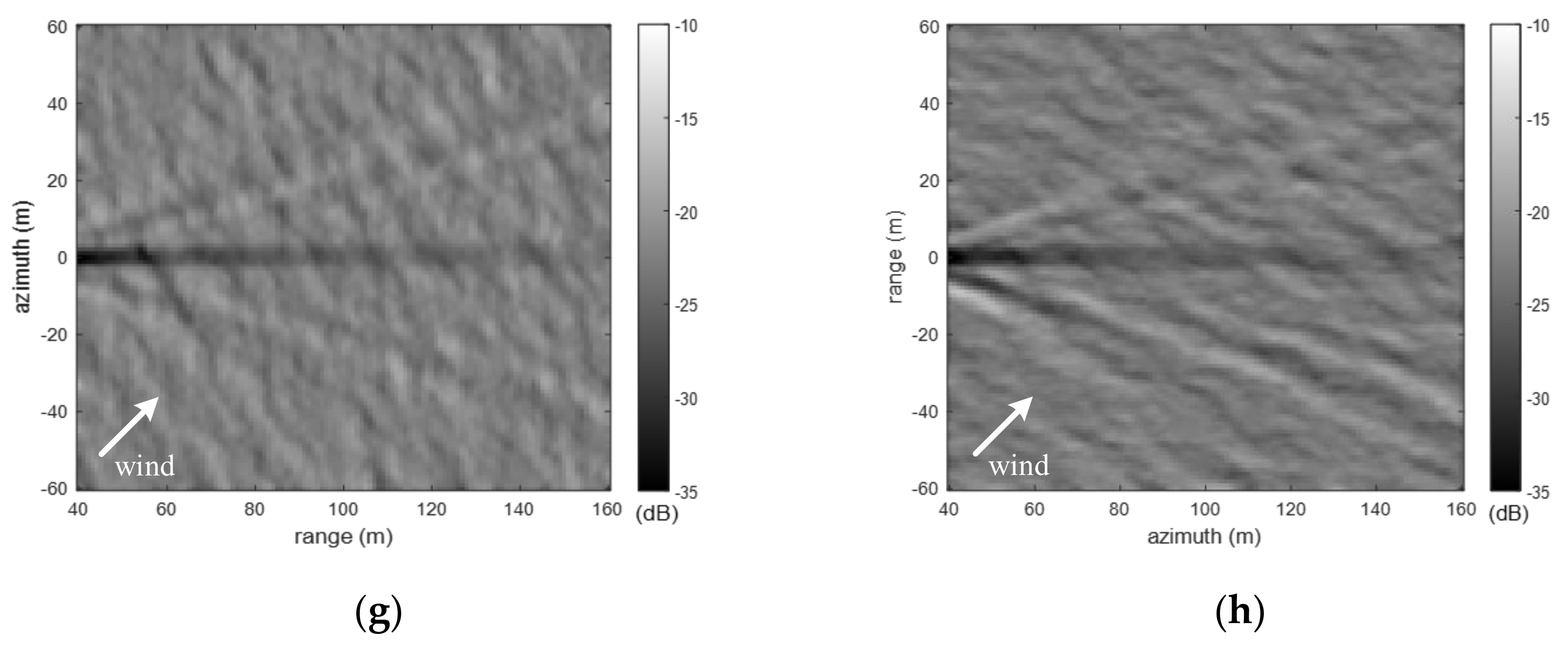
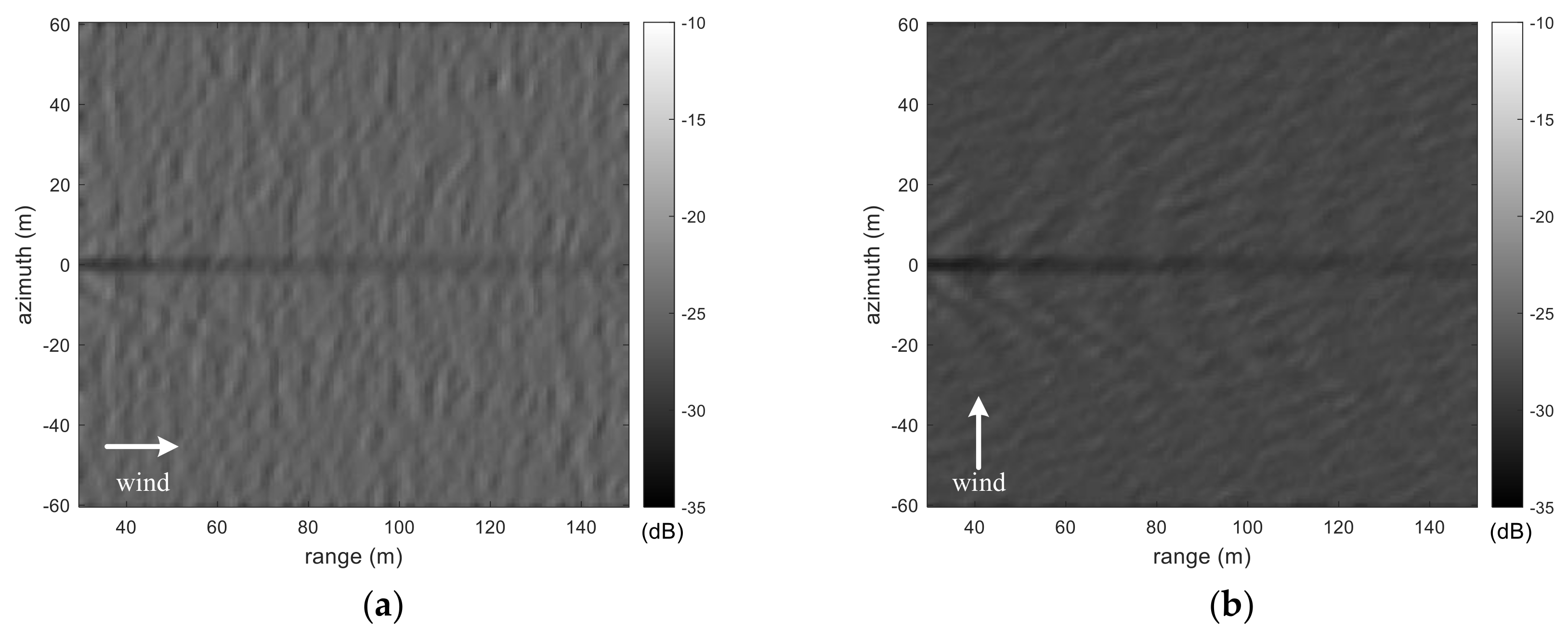
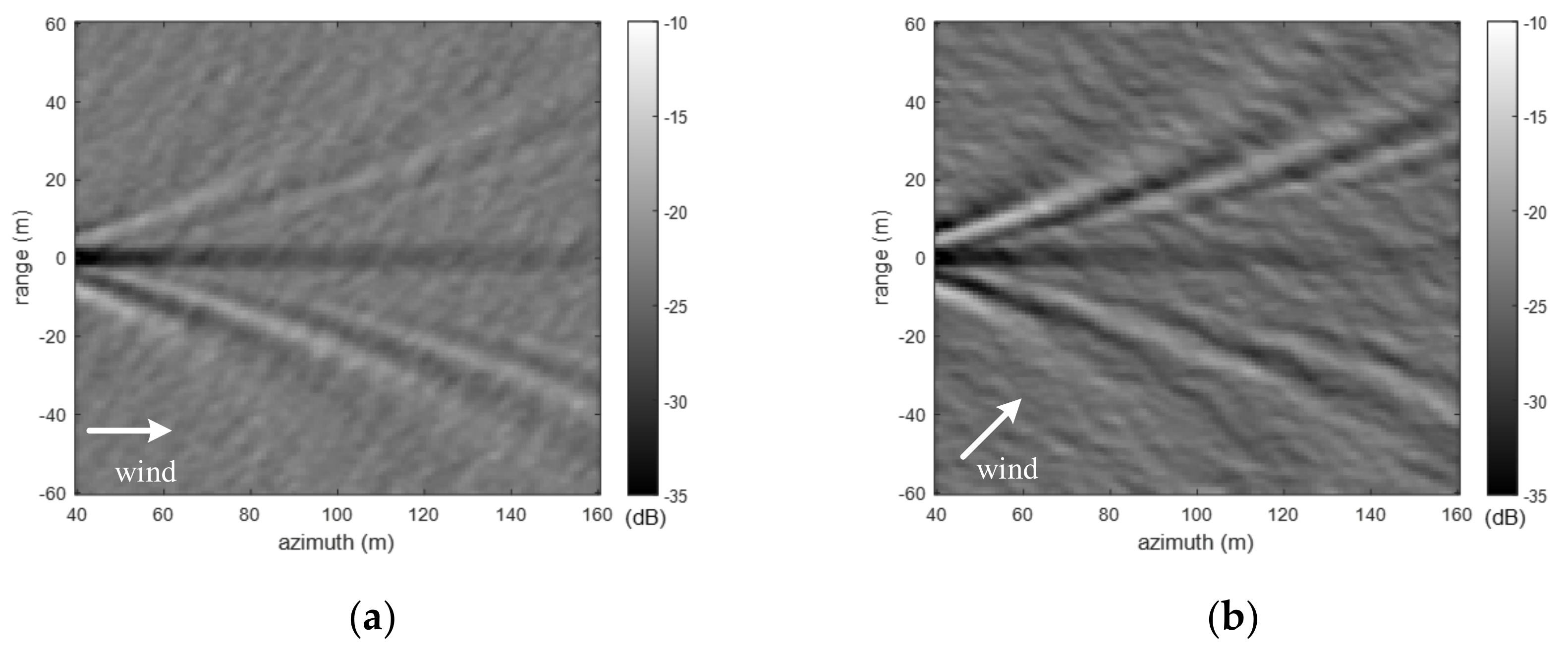
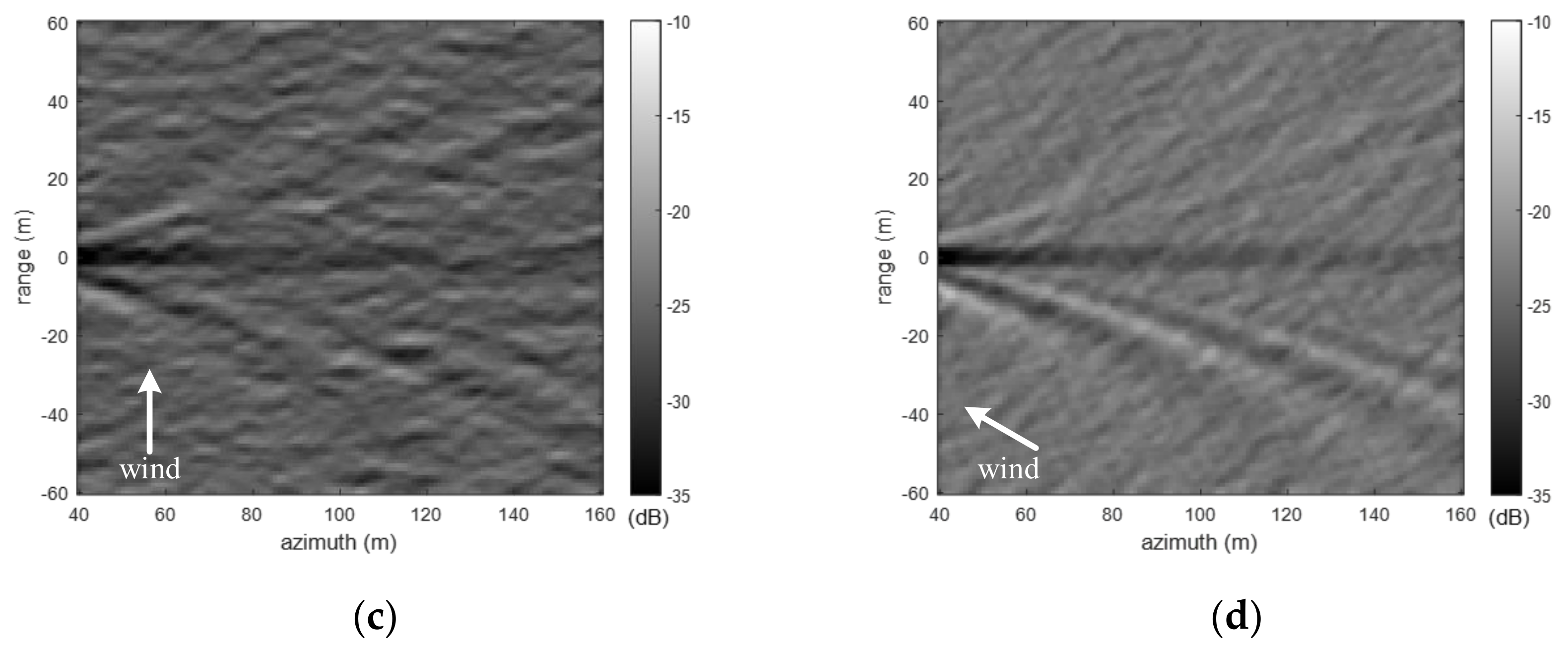
3. Simulation Results and Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Thomson, W. On ship waves. Proc. Inst. Mech. Eng. 1887, 38, 409–463. [Google Scholar] [CrossRef]
- Noblesse, F.; He, J.; Zhu, Y.; Hong, L.; Zhang, C.; Zhu, R.; Yang, C. Why can ship wakes appear narrower than Kelvin’s angle? Eur. J. Mech.-B/Fluids 2014, 46, 164–171. [Google Scholar] [CrossRef]
- Darmon, A.; Benzaquen, M.; Raphaël, E. Kelvin wake pattern at large Froude numbers. J. Fluid Mech. 2014, 738, 10. [Google Scholar] [CrossRef]
- Reed, A.M.; Milgram, J.H. Ship wakes and their radar images. Annu. Rev. Fluid Mech. 2002, 34, 469–502. [Google Scholar] [CrossRef]
- Hogan, G.; Chapman, R.; Watson, G.; Thompson, D. Observations of ship-generated internal waves in SAR images from Loch Linnhe, Scotland, and comparison with theory and in situ internal wave measurements. IEEE Trans. Geosci. Remote Sens. 1996, 34, 532–542. [Google Scholar] [CrossRef]
- Soomere, T. Nonlinear components of ship wake waves. Appl. Mech. Rev. 2007, 60, 120–138. [Google Scholar] [CrossRef]
- Shomina, O.; Kapustin, I.; Ermakov, S. Damping of gravity–capillary waves on the surface of turbulent fluid. Exp. Fluids 2020, 61, 1–12. [Google Scholar] [CrossRef]
- Google Earth. Available online: http://earth.google.com (accessed on 30 September 2021).
- HH Stripmap Mode TerraSAR-X Image of the Straight of Gibraltar. Available online: http://www.intelligenceairbusds.com/en/23-sample-imagery.php# (accessed on 30 September 2021).
- Graziano, M.D.; D’Errico, M.; Rufino, G. Ship heading and velocity analysis by wake detection in SAR images. Acta Astronaut. 2016, 128, 72–82. [Google Scholar] [CrossRef]
- Oumansou, K.; Wang, Y.; Saillard, J. Multi-frequency SAR observation of a ship wake. IEE Proc. Radar Sonar Navig. 1996, 143, 275–280. [Google Scholar] [CrossRef]
- Hennings, I.; Romeiser, R.; Alpers, W.; Viola, A.P. Radar imaging of Kelvin arms of ship wakes. Int. J. Remote Sens. 1999, 20, 2519–2543. [Google Scholar] [CrossRef]
- Shemer, L.; Kagan, L.; Zilman, G. Simulation of ship wakes image by an along-track interferometric SAR. Int. J. Remote Sens. 1996, 17, 3577–3597. [Google Scholar] [CrossRef]
- Arnold-Bos, A.; Khenchaf, A.; Martin, A. Bistatic radar imaging of the marine environment—Part II: Simulation and results analysis. IEEE Trans. Geosci. Remote Sens. 2007, 45, 3384–3396. [Google Scholar] [CrossRef]
- Zilman, G.; Zapolski, A.; Marom, M. On detectability of a ship’s Kelvin wake in simulated SAR images of rough sea surface. IEEE Trans. Geosci. Remote Sens. 2014, 53, 609–618. [Google Scholar] [CrossRef]
- Peltzer, R.D.; Griffin, O.M.; Barger, W.D.; Kaiser, J.A.C. High resolution measurements of surface-active film redistribution in ship wakes. J. Geophys. Res. 1992, 97, 5231–5252. [Google Scholar] [CrossRef]
- Marmorino, G.O.; Trump, C.L. Preliminary Side-Scan ADCP Measurements across a Ship’s Wake. J. Atmos. Ocean. Technol. 1996, 13, 507–513. [Google Scholar] [CrossRef][Green Version]
- Ermakov, S.A.; Kapustin, I.A. Experimental study of turbulent-wake expansion from a surface ship. Izv. Atmos. Ocean. Phys. 2010, 46, 524–529. [Google Scholar] [CrossRef]
- George, S.G.; Tatnall, A.R. Measurement of turbulence in the oceanic mixed layer using Synthetic Aperture Radar (SAR). Ocean Sci. Discuss. 2012, 9, 2851–2883. [Google Scholar]
- Soloviev, A.; Gilman, M.; Young, K.; Brusch, S.; Lehner, S. Sonar measurements in ship wakes simultaneous with TerraSAR-X overpasses. IEEE Trans. Geosci. Remote Sens. 2009, 48, 841–851. [Google Scholar] [CrossRef]
- Fujimura, A.; Soloviev, A.; Kudryavtsev, V. Numerical simulation of the wind-stress effect on SAR imagery of far wakes of ships. IEEE Geosci. Remote Sens. Lett. 2010, 7, 646–649. [Google Scholar] [CrossRef]
- Fujimura, A.; Soloviev, A.; Rhee, S.H.; Romeiser, R. Coupled Model Simulation of Wind Stress Effect on Far Wakes of Ships in SAR Images. IEEE Trans. Geosci. Remote. Sens. 2016, 54, 2543–2551. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, M.; Chen, J. Investigation on the Electromagnetic Scattering from the Accurate 3-D Breaking Ship Waves Generated by CFD Simulation. IEEE Trans. Geosci. Remote Sens. 2019, 57, 2689–2699. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, M.; Chen, J.; Cai, Z. Application of facet scattering model in SAR imaging of sea surface waves with Kelvin wake. Prog. Electromagn. Res. 2016, 67, 107–120. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, M.; Wang, J. Synthetic aperture radar image simulation of the internal waves excited by a submerged object in a stratified ocean. Waves Random Complex Media 2020, 30, 177–191. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, M.; Wang, L. Coupled model simulation of the internal wave wakes induced by a submerged body in SAR imaging. Waves Random Complex Media 2020, 1–18. [Google Scholar] [CrossRef]
- Group, T.W. The WAM model—A third generation ocean wave prediction model. J. Phys. Oceanogr. 1988, 18, 1775–1810. [Google Scholar] [CrossRef]
- Tolman, H.L. The WAVEWATCH III Development Group (WW3DG), (2019): User Manual and System Documentation of WAVEWATCH III R Version 6.07, Tech. Note 333; NOAA/NWS/NCEP/MMAB: College Park, MD, USA, 2019; pp. 120–160. [Google Scholar]
- Chen, H.; Zhang, M.; Zhao, Y. An efficient slope-deterministic facet model for SAR imagery simulation of marine scene. IEEE Trans. Antennas Propag. 2010, 58, 3751–3756. [Google Scholar] [CrossRef]
- Menter, F.R. Review of the shear-stress transport turbulence model experience from an industrial perspective. Int. J. Comput. Fluid Dyn. 2009, 23, 305–316. [Google Scholar] [CrossRef]
- Milgram, J.H.; Skop, R.A.; Peltzer, R.D.; Griffin, O.M. Modeling short sea wave energy distributions in the far wakes of ships. J. Geophys. Res. Space Phys. 1993, 98, 7115–7124. [Google Scholar] [CrossRef]
- Elfouhaily, T.; Chapron, B.; Katsaros, K.; VanDeMark, D. A unified directional spectrum for long and short wind-driven waves. J. Geophys. Res. Space Phys. 1997, 102, 15781–15796. [Google Scholar] [CrossRef]
- Li, J. Upstream nonoscillatory advection schemes. Mon. Weather Rev. 2008, 136, 4709–4729. [Google Scholar] [CrossRef]
- Chen, P.; Zheng, G.; Hauser, D.; Xu, F. Quasi-Gaussian probability density function of sea wave slopes from near nadir Ku-band radar observations. Remote. Sens. Environ. 2018, 217, 86–100. [Google Scholar] [CrossRef]
- Voronovich, A.G.; Zavorotny, V.U. Theoretical model for scattering of radar signals in Ku- and C-bands from a rough sea surface with breaking waves. Waves Random Media 2001, 11, 247–269. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Zhang, M.; Liu, J. Electromagnetic Scattering Model for Far Wakes of Ship with Wind Waves on Sea Surface. Remote Sens. 2021, 13, 4417. https://doi.org/10.3390/rs13214417
Wang L, Zhang M, Liu J. Electromagnetic Scattering Model for Far Wakes of Ship with Wind Waves on Sea Surface. Remote Sensing. 2021; 13(21):4417. https://doi.org/10.3390/rs13214417
Chicago/Turabian StyleWang, Letian, Min Zhang, and Jiong Liu. 2021. "Electromagnetic Scattering Model for Far Wakes of Ship with Wind Waves on Sea Surface" Remote Sensing 13, no. 21: 4417. https://doi.org/10.3390/rs13214417
APA StyleWang, L., Zhang, M., & Liu, J. (2021). Electromagnetic Scattering Model for Far Wakes of Ship with Wind Waves on Sea Surface. Remote Sensing, 13(21), 4417. https://doi.org/10.3390/rs13214417






