Tensor-Based Reduced-Dimension MUSIC Method for Parameter Estimation in Monostatic FDA-MIMO Radar
Abstract
:1. Introduction
2. Basic Knowledge of Tensor and Signal Model Based on Tensor
2.1. Basic Knowledge of Tensor
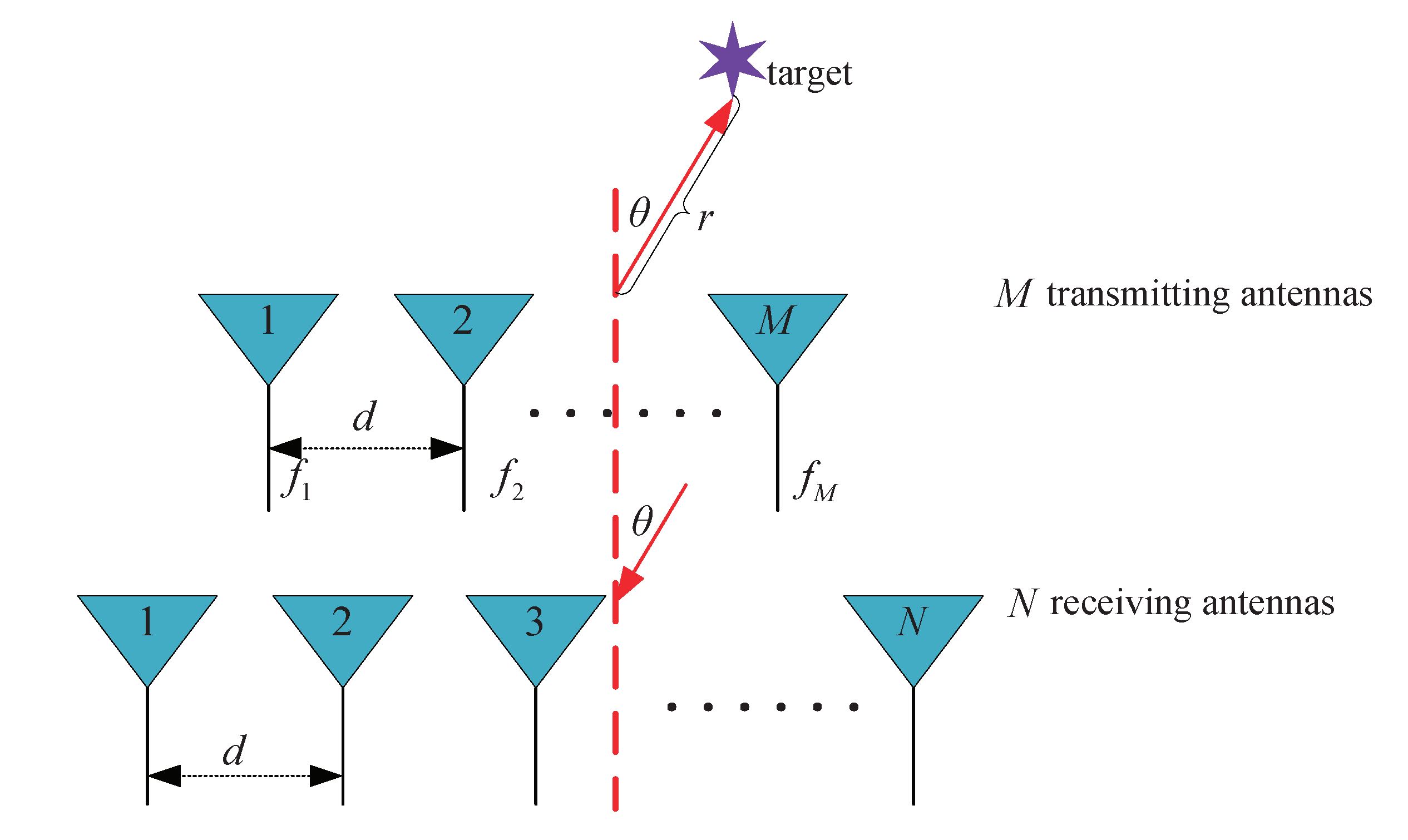
2.2. Signal Model Based on Tensor
3. Doa and Range Estimation VIA Tensor for FDA-Mimo Radar
3.1. Signal Subspace Estimation VIA HOSVD
3.2. DOA Estimation VIA Tensor-Based Reduced-Dimension Music
3.3. Range Estimation
4. Performance Analysis of the Proposed Method
4.1. Computation Complexity
- (1) The HOSVD computation complexity of is in Equation (11);
- (2) The signal subspace estimation needs in Equation (15);
- (3) In Equation (21), the dimensionality reduction of the two-dimensional search requires ;
- (4) The spectrum peak search of DOA estimation in Equation (27) is , where represents the search times within the search DOA, and stands for factorial;
- (5) Computing the range requires ;
4.2. Cramr-Rao Bound
5. Numerical Simulations
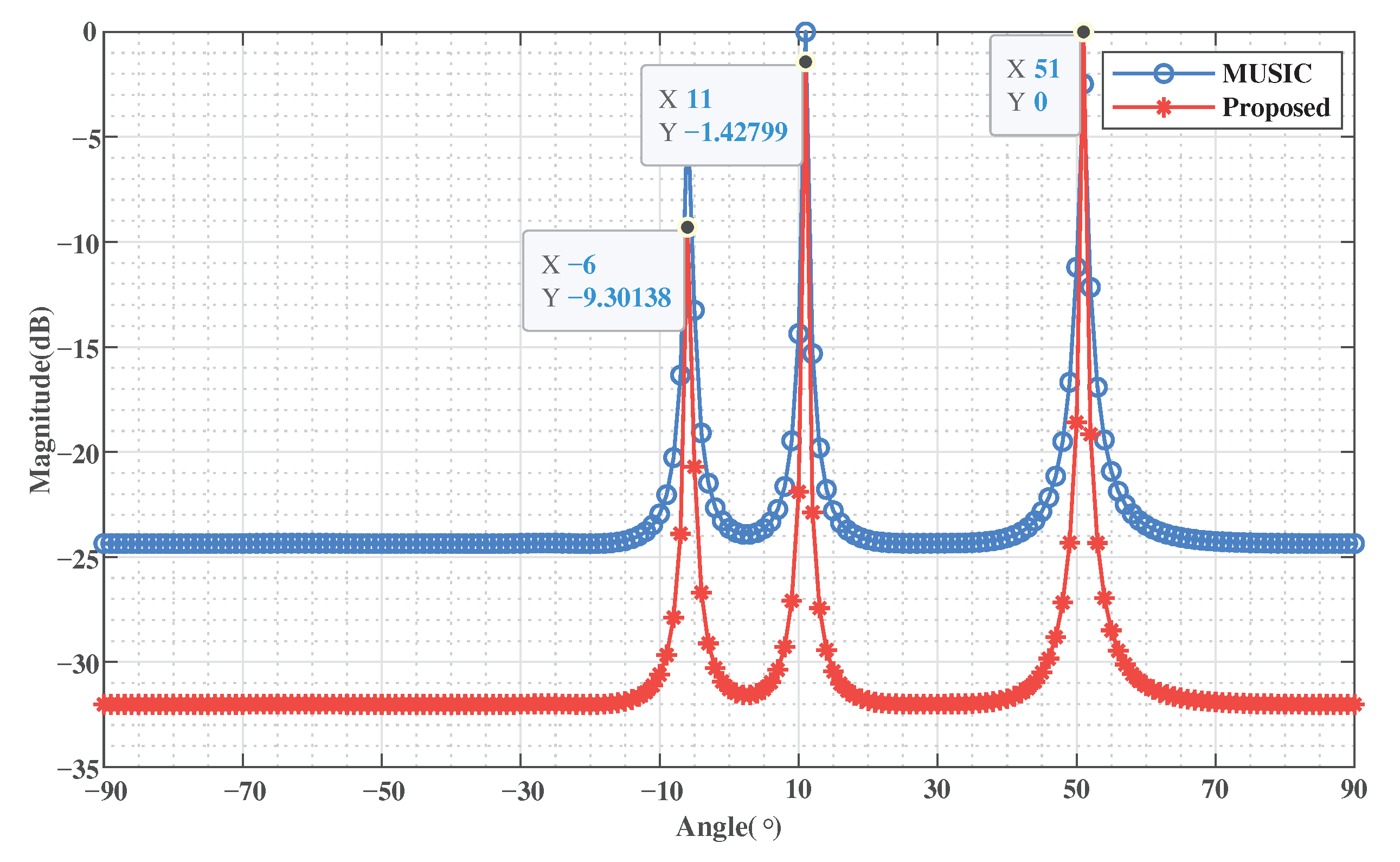
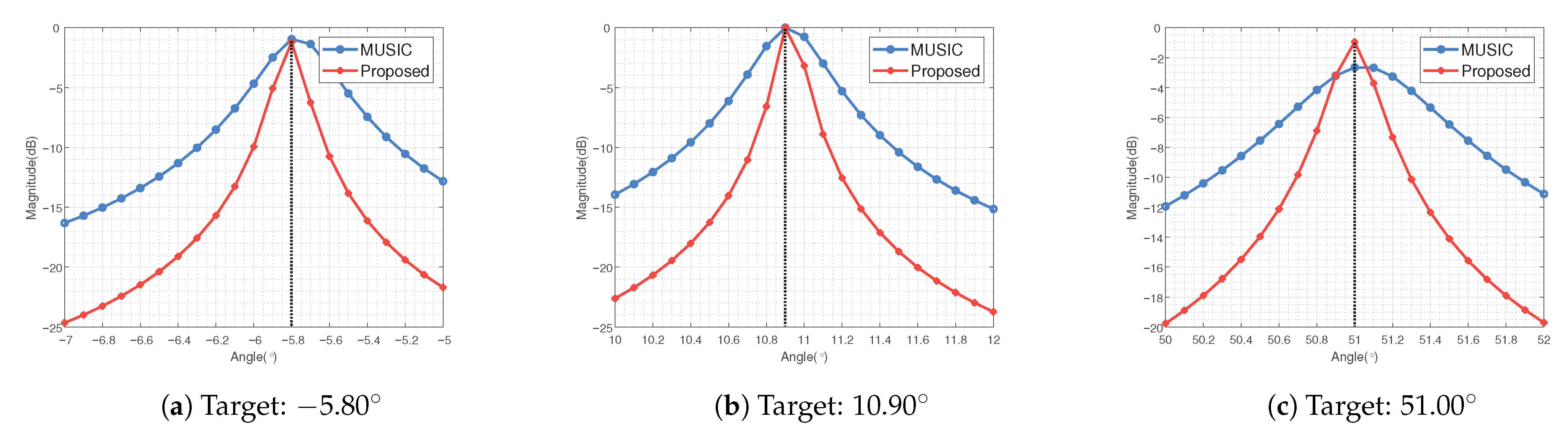
5.1. Spectrum Peak Search for DOA Estimation
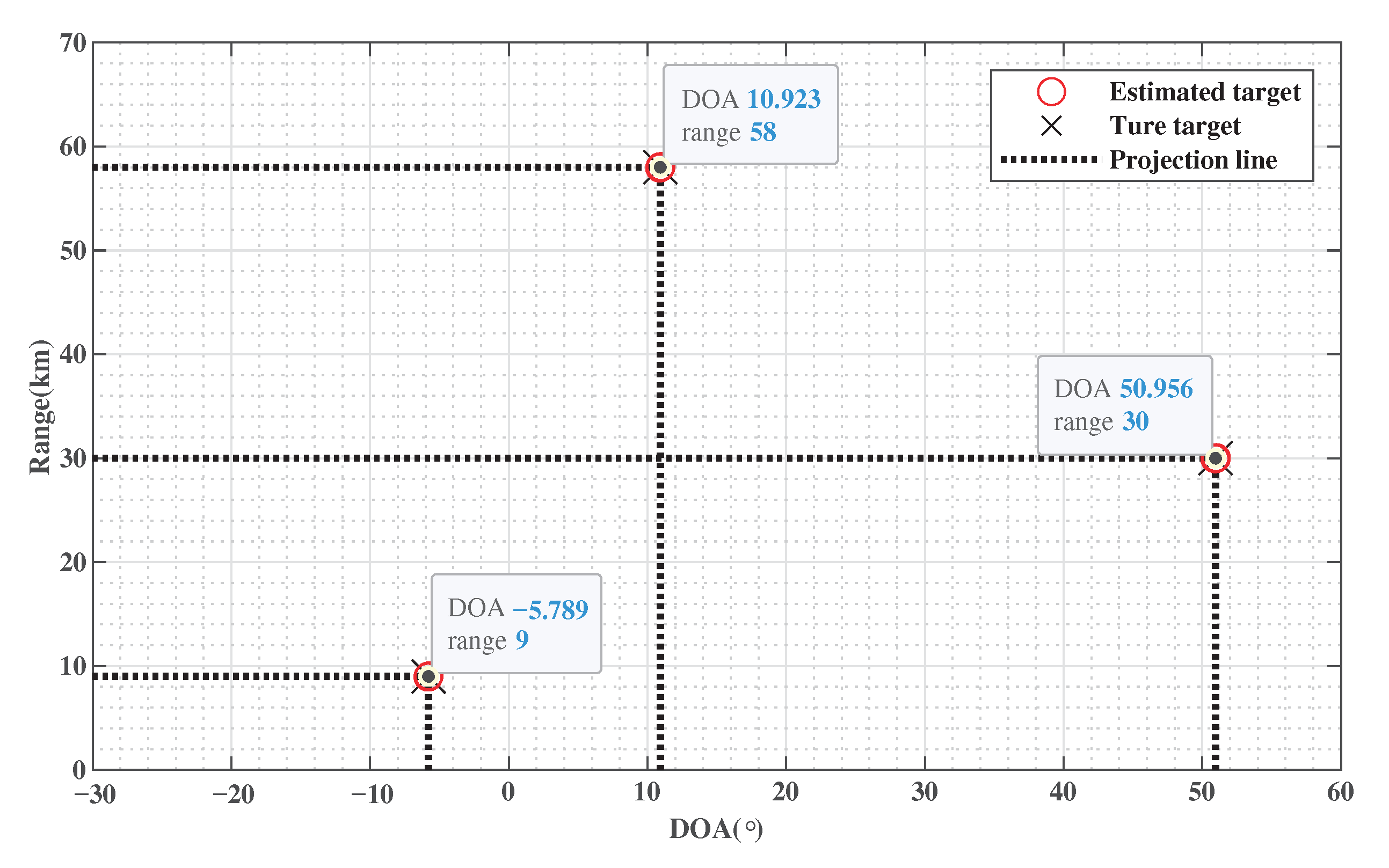
5.2. 2D Point Cloud of the Target Landing Point
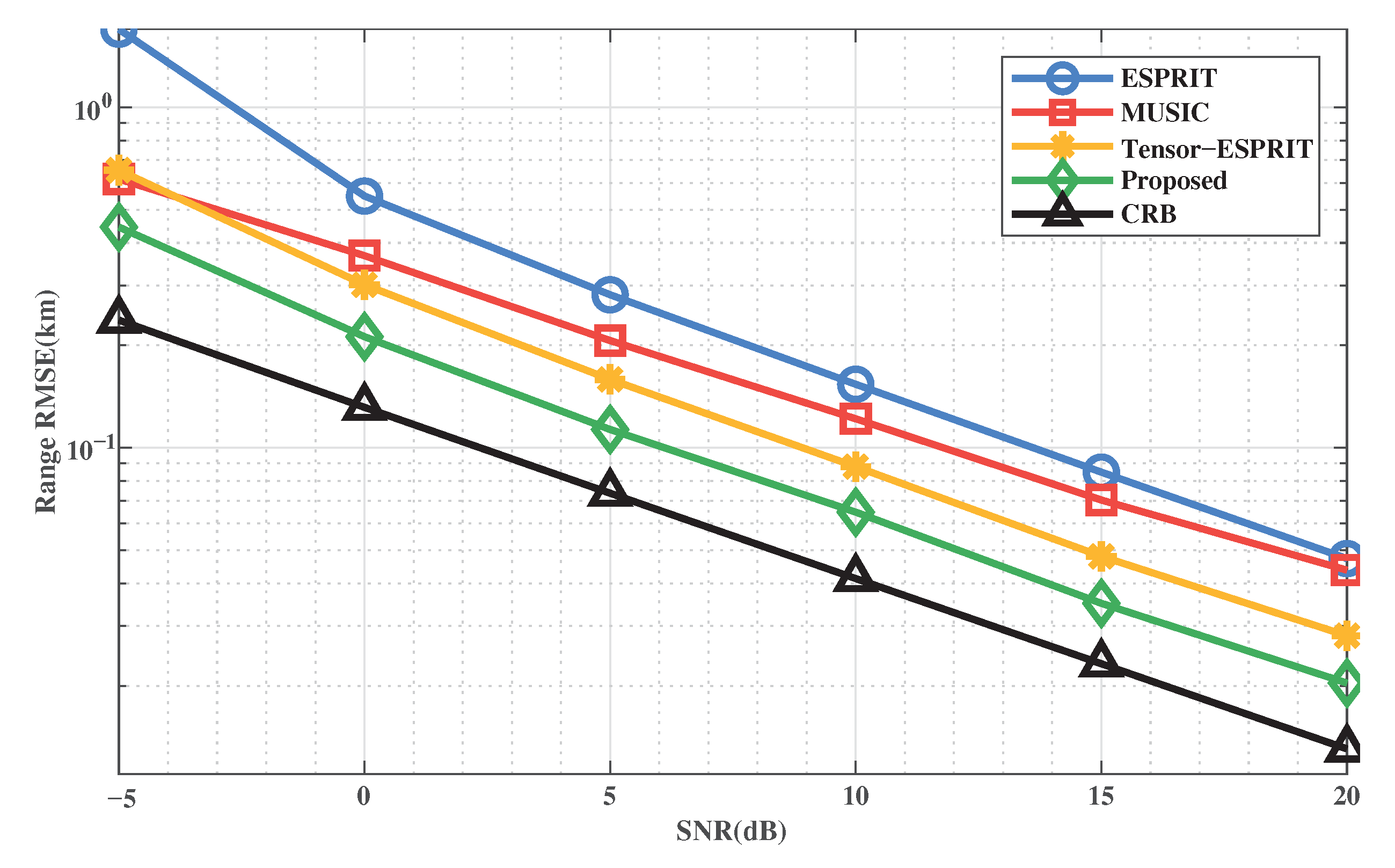
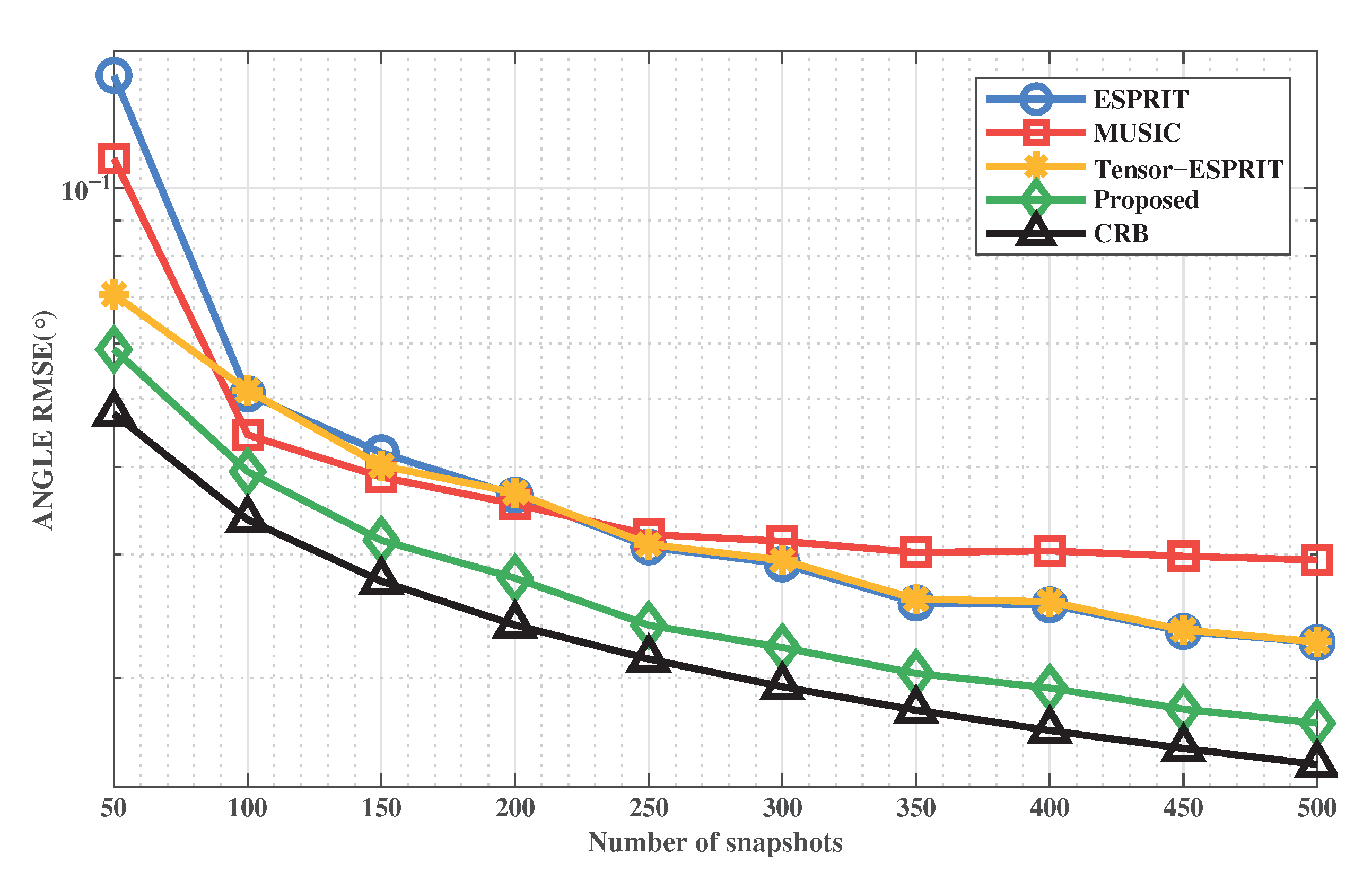
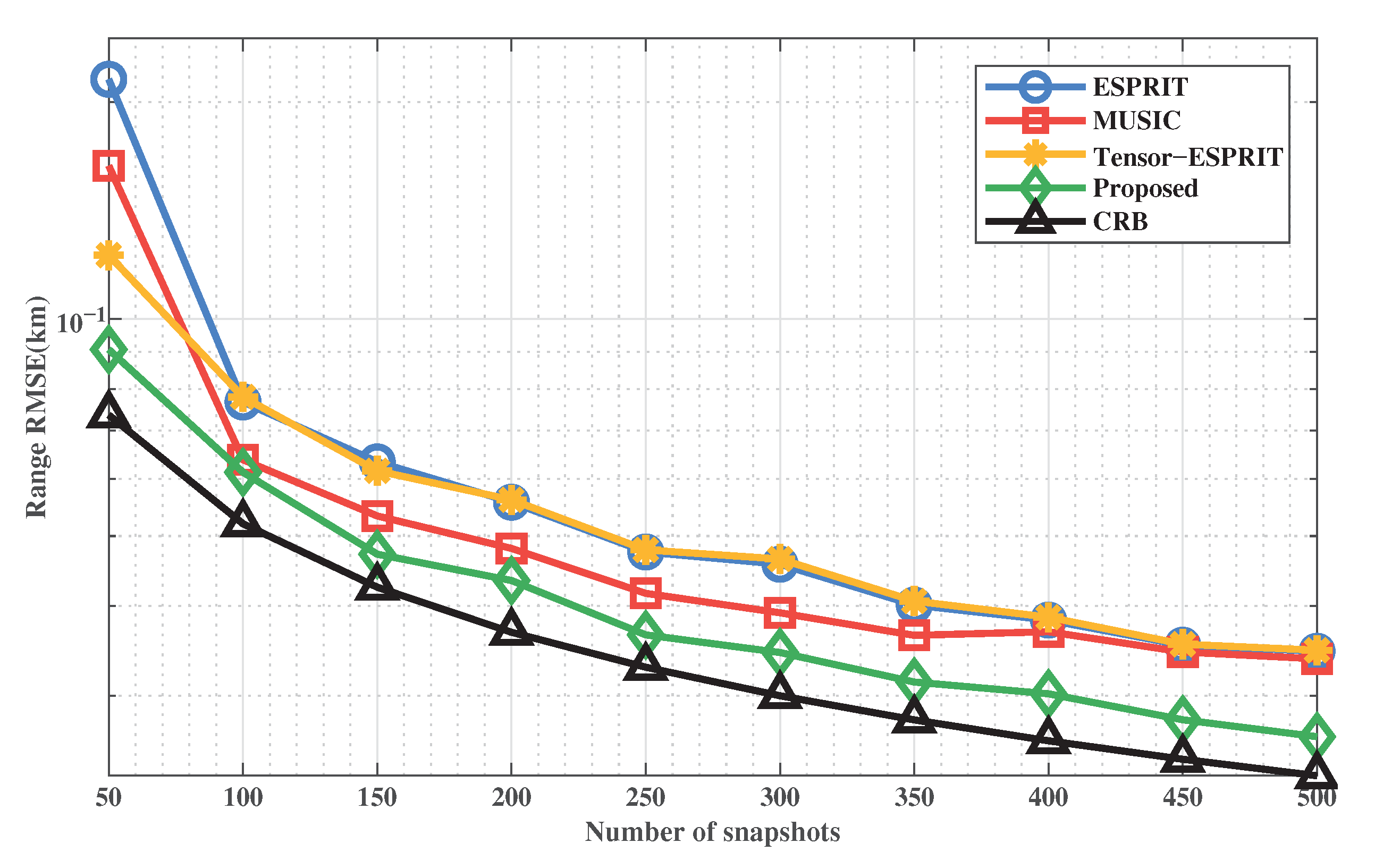
5.3. RMSE Performance
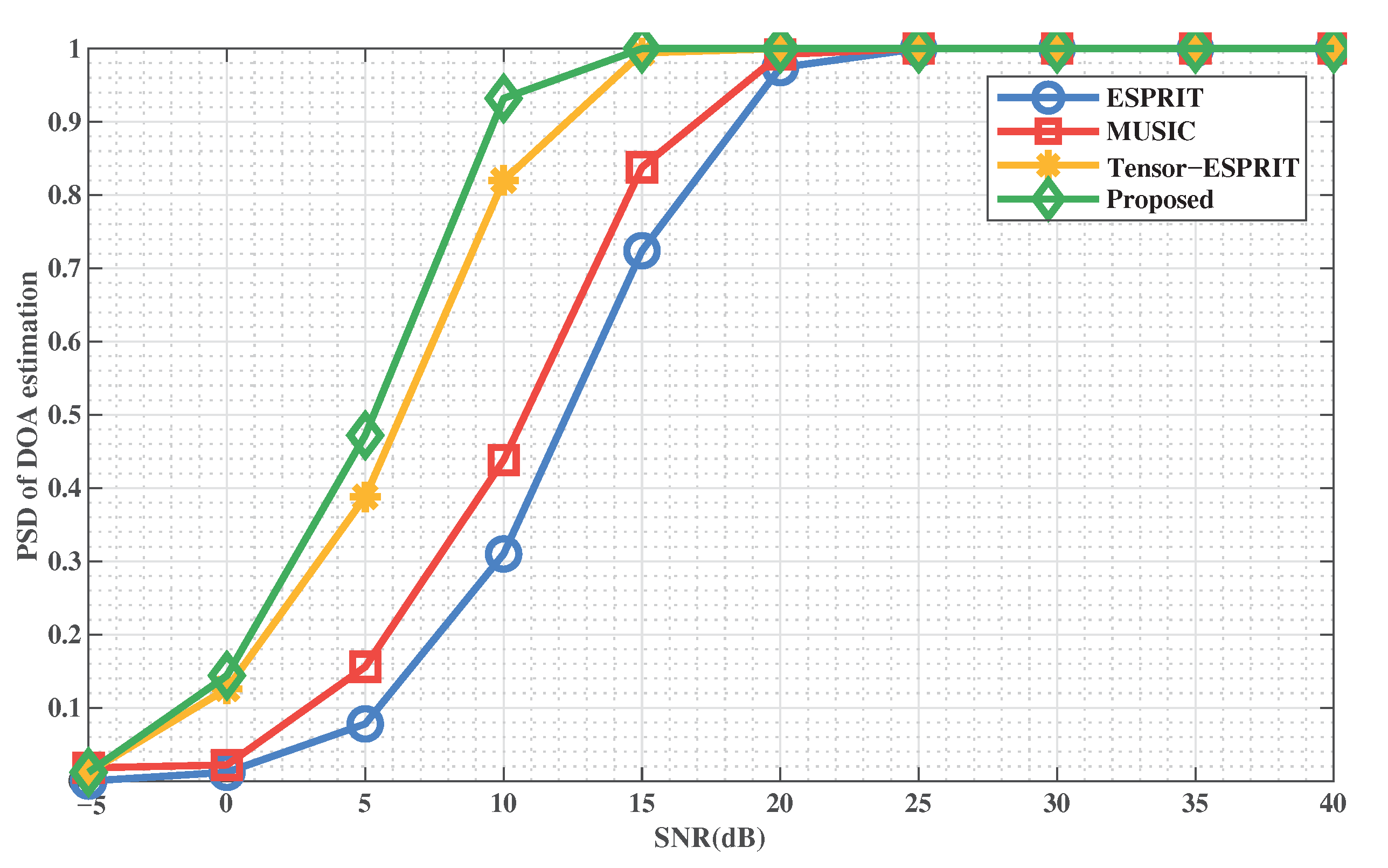
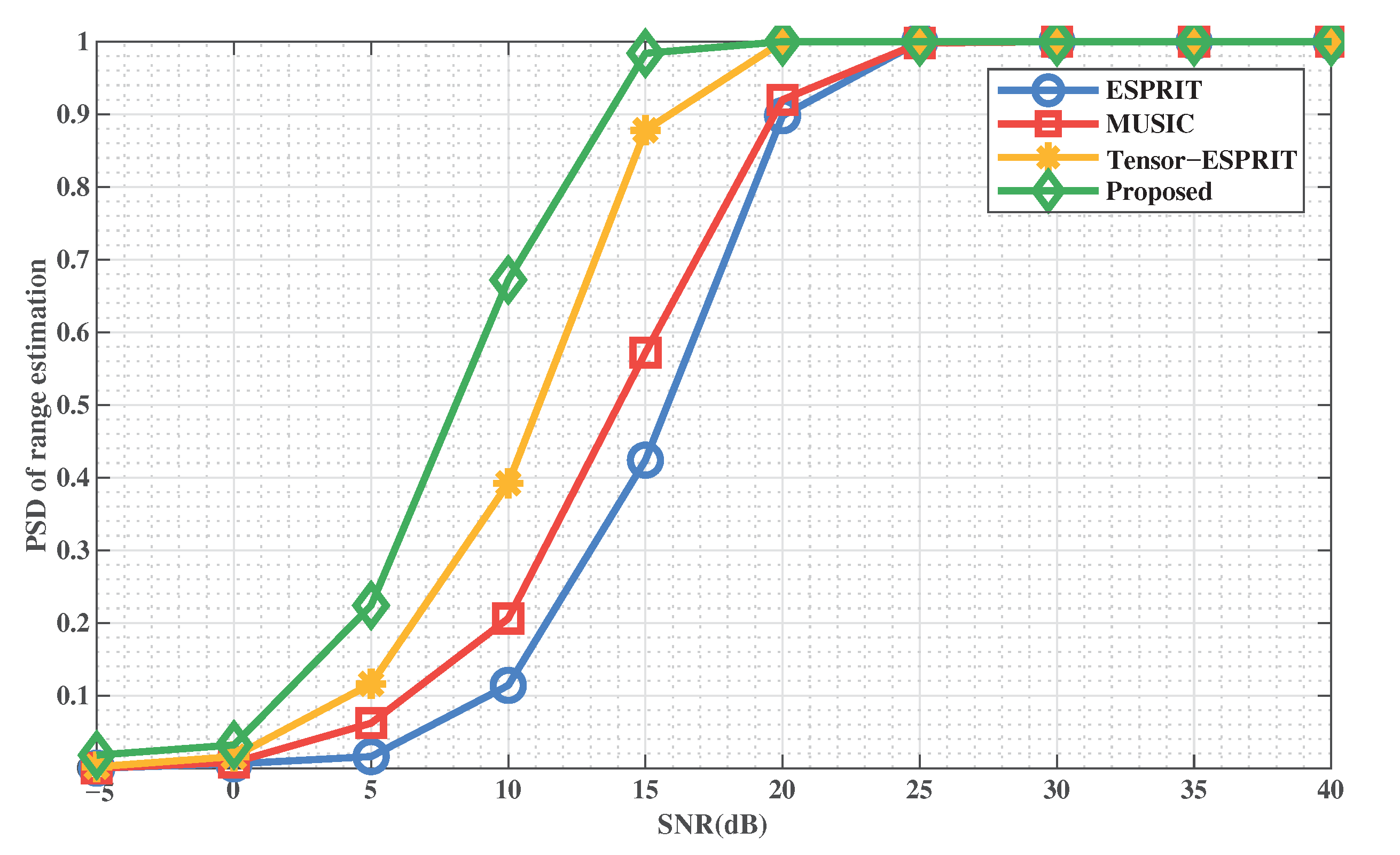
5.4. Probability of Successful Detection
5.5. The Simulation Time Versus Trial Number
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Krim, H.; Viberg, M. Two decades of array signal processing research: The parametric approach. IEEE Signal Process. Mag. 1996, 13, 67–94. [Google Scholar] [CrossRef]
- Fishler, E.; Haimovich, A.; Blum, R.; Cimini, R.; Chizhik, D.; Valenzuela, R. Performance of MIMO radar systems: Advantages of angular diversity. In Proceedings of the Thirty-Eighth Asilomar Conference on Signals, Systems and Computers, Pacific Grove, CA, USA, 7–10 November 2004; Volume 1, pp. 305–309. [Google Scholar]
- Amadori, P.V.; Masouros, C. Low RF-complexity millimeter-wave beamspace-MIMO systems by beam selection. IEEE Trans. Commun. 2015, 63, 2212–2223. [Google Scholar] [CrossRef]
- Wang, H.; Wan, L.; Dong, M.; Ota, K.; Wang, X. Assistant vehicle localization based on three collaborative base stations via SBL-based robust DOA estimation. IEEE Internet Things J. 2019, 6, 5766–5777. [Google Scholar] [CrossRef]
- Wang, X.; Huang, M.; Wan, L. Joint 2D-DOD and 2D-DOA estimation for coprime EMVS–MIMO radar. Circ. Syst. Signal Process. 2021, 40, 1–17. [Google Scholar] [CrossRef]
- Wang, W.; So, H.C.; Farina, A. An overview on time/frequency modulated array processing. IEEE J. Sel. Top. Signal Process. 2016, 11, 228–246. [Google Scholar] [CrossRef]
- Jiao, Z.; Ding, C.; Liang, X.; Chen, L.; Zhang, F. Sparse Bayesian learning based three-dimensional imaging algorithm for off-grid air targets in MIMO radar array. Remote Sens. 2018, 10, 369. [Google Scholar] [CrossRef] [Green Version]
- Xiong, J.; Wang, W.; Gao, K. FDA-MIMO radar range–angle estimation: CRLB, MSE, and resolution analysis. IEEE Trans. Aerosp. Electron. Syst. 2017, 54, 284–294. [Google Scholar] [CrossRef]
- Zhang, L.; Wen, F. A Novel MIMO Radar Orthogonal Waveform Design Algorithm Based on Intelligent Ions Motion. Remote Sens. 2021, 13, 1968. [Google Scholar] [CrossRef]
- Fishler, E.; Haimovich, A.; Blum, R.; Chizhik, D.; Cimini, L.; Valenzuela, R. MIMO radar: An idea whose time has come. In Proceedings of the 2004 IEEE Radar Conference (IEEE Cat. No. 04CH37509), Philadelphia, PA, USA, 29–29 April 2004; pp. 71–78. [Google Scholar]
- Wan, L.; Sun, L.; Liu, K.; Wang, X.; Lin, Q.; Zhu, T. Autonomous vehicle source enumeration exploiting non-cooperative UAV in software defined internet of vehicles. IEEE Trans. Intell. Transp. Syst. 2020, 22, 3603–3615. [Google Scholar] [CrossRef]
- Wang, X.; Yang, L.T.; Meng, D.; Dong, M.; Ota, K.; Wang, H. Multi-UAV cooperative localization for marine targets based on weighted subspace fitting in SAGIN environment. IEEE Internet Things J. 2021, in press. [Google Scholar]
- Fishler, E.; Haimovich, A.; Blum, R.S.; Cimini, L.J.; Chizhik, D.; Valenzuela, R.A. Spatial diversity in radars—Models and detection performance. IEEE Trans. Signal Process. 2006, 54, 823–838. [Google Scholar] [CrossRef]
- Li, J.; Stoica, P.; Xu, L.; Roberts, W. On parameter identifiability of MIMO radar. IEEE Signal Process. Lett. 2007, 14, 968–971. [Google Scholar]
- Hu, Z.; Wang, W.; Dong, F. MIMO Radar Accurate Imaging and Motion Estimation for 3-D Maneuvering Ship Target. IEEE Trans. Instrum. Meas. 2021, 70, 1–12. [Google Scholar]
- Wan, L.; Liu, K.; Liang, Y.C.; Zhu, T. DOA and polarization estimation for non-circular signals in 3-D millimeter wave polarized massive MIMO systems. IEEE Trans. Wirel. Commun. 2021, 20, 3152–3167. [Google Scholar] [CrossRef]
- Antonik, P.; Wicks, M.C.; Griffiths, H.D.; Baker, C.J. Frequency diverse array radars. In Proceedings of the 2006 IEEE Conference on Radar, Verona, NY, USA, 24–27 April 2006; p. 3. [Google Scholar]
- Wang, W.Q. Frequency diverse array antenna: New opportunities. IEEE Antennas Propag. Mag. 2015, 57, 145–152. [Google Scholar] [CrossRef]
- Antonik, P.; Wicks, M.C.; Griffiths, H.D.; Baker, C.J. Range-dependent beamforming using element level waveform diversity. In Proceedings of the 2006 International Waveform Diversity & Design Conference, Lihue, HI, USA, 22–27 January 2006; pp. 1–6. [Google Scholar]
- Smith, C.M.; Feder, H.J.S.; Leonard, J.J. Multiple target tracking with navigation uncertainty. In Proceedings of the 37th IEEE Conference on Decision and Control (Cat. No. 98CH36171), Tampa, FL, USA, 18 December 1998; Volume 1, pp. 760–761. [Google Scholar]
- Wan, L.; Sun, Y.; Sun, L.; Ning, Z.; Rodrigues, J.J. Deep learning based autonomous vehicle super resolution DOA estimation for safety driving. IEEE Trans. Intell. Transp. Syst. 2020, 22, 4301–4315. [Google Scholar] [CrossRef]
- Secmen, M.; Demir, S.; Hizal, A.; Eker, T. Frequency diverse array antenna with periodic time modulated pattern in range and angle. In Proceedings of the 2007 IEEE Radar Conference, Waltham, MA, USA, 17–20 April 2007; pp. 427–430. [Google Scholar]
- Wang, W.; Shao, H. Range-angle localization of targets by a double-pulse frequency diverse array radar. IEEE J. Sel. Top. Signal Process. 2013, 8, 106–114. [Google Scholar] [CrossRef]
- Wang, W. Subarray-based frequency diverse array radar for target range-angle estimation. IEEE Trans. Aerosp. Electron. Syst. 2014, 50, 3057–3067. [Google Scholar] [CrossRef]
- Cui, C.; Xu, J.; Gui, R.; Wang, W.Q.; Wu, W. Search-free DOD, DOA and range estimation for bistatic FDA-MIMO radar. IEEE Access 2018, 6, 15431–15445. [Google Scholar] [CrossRef]
- Zhang, J.J.; Papandreou-Suppappola, A. MIMO radar with frequency diversity. In Proceedings of the 2009 International Waveform Diversity and Design Conference, Kissimmee, FL, USA, 8–13 February 2009; pp. 208–212. [Google Scholar]
- Gao, K.; Shao, H.; Chen, H.; Cai, J.; Wang, W.Q. Impact of frequency increment errors on frequency diverse array MIMO in adaptive beamforming and target localization. Digit. Signal Process. 2015, 44, 58–67. [Google Scholar] [CrossRef]
- Xu, T.; Yang, Y.; Huang, M.; Wang, H.; Wu, D.; Qu, Y. Tensor-Based Angle and Range Estimation Method in Monostatic FDA-MIMO Radar. Math. Probl. Eng. 2020, 2020, 1–8. [Google Scholar] [CrossRef]
- Wang, W.Q.; So, H.C. Transmit subaperturing for range and angle estimation in frequency diverse array radar. IEEE Trans. Signal Process. 2014, 62, 2000–2011. [Google Scholar] [CrossRef]
- Xu, T.; Wang, X.; Su, T.; Wan, L.; Sun, L. Vehicle Location in Edge Computing Enabling IoTs Based on Bistatic FDA-MIMO Radar. IEEE Access 2021, 9, 46398–46408. [Google Scholar] [CrossRef]
- Xu, J.; Liao, G.; Zhu, S.; Huang, L.; So, H.C. Joint range and angle estimation using MIMO radar with frequency diverse array. IEEE Trans. Signal Process. 2015, 63, 3396–3410. [Google Scholar] [CrossRef]
- Li, B.; Bai, W.; Zheng, G. Successive ESPRIT algorithm for joint DOA-range-polarization estimation with polarization sensitive FDA-MIMO radar. IEEE Access 2018, 6, 36376–36382. [Google Scholar] [CrossRef]
- Liu, F.; Wang, X.; Huang, M.; Wan, L.; Wang, H.; Zhang, B. A Novel Unitary ESPRIT Algorithm for Monostatic FDA-MIMO Radar. Sensors 2020, 20, 827. [Google Scholar] [CrossRef] [Green Version]
- Liu, F.; Wang, X.; Huang, M.; Wan, L. Joint angle and range estimation for bistatic FDA-MIMO radar via real-valued subspace decomposition. Signal Process. 2021, 185, 108065. [Google Scholar] [CrossRef]
- van Belzen, F.; Weiland, S.; de Graaf, J. Singular value decompositions and low rank approximations of multi-linear functionals. In Proceedings of the 2007 46th IEEE Conference on Decision and Control, New Orleans, LA, USA, 12–14 December 2007; pp. 3751–3756. [Google Scholar]
- Wang, Q.; Wang, H.; Dou, T.; Hou, C. Block Sparse Representation based DOA Estimation for FDA with Unknown Mutual Coupling. In Proceedings of the 2018 IEEE Asia-Pacific Conference on Antennas and Propagation (APCAP), Auckland, New Zealand, 5–8 August 2018; pp. 536–538. [Google Scholar]
- Liu, Q.; Wang, X.; Huang, M.; Lan, X.; Sun, L. DOA and Range Estimation for FDA-MIMO Radar with Sparse Bayesian Learning. Remote Sens. 2021, 13, 2553. [Google Scholar] [CrossRef]
- Nion, D.; Sidiropoulos, N.D. Tensor algebra and multidimensional harmonic retrieval in signal processing for MIMO radar. IEEE Trans. Signal Process. 2010, 58, 5693–5705. [Google Scholar] [CrossRef]
- Sidiropoulos, N.D.; De Lathauwer, L.; Fu, X.; Huang, K.; Papalexakis, E.E.; Faloutsos, C. Tensor decomposition for signal processing and machine learning. IEEE Trans. Signal Process. 2017, 65, 3551–3582. [Google Scholar] [CrossRef]
- Zhang, X.; Xu, L.; Xu, L.; Xu, D. Direction of departure (DOD) and direction of arrival (DOA) estimation in MIMO radar with reduced-dimension MUSIC. IEEE Commun. Lett. 2010, 14, 1161–1163. [Google Scholar] [CrossRef]
- Kolda, T.G.; Bader, B.W. Tensor decompositions and applications. SIAM Rev. 2009, 51, 455–500. [Google Scholar] [CrossRef]
- Itskov, M. Tensor Algebra and Tensor Analysis for Engineers; Springer: New York, NY, USA, 2007. [Google Scholar]
- Zhang, X. Matrix Analysis and Applications; Tsinghua University Press: Beijing, China, 2004. [Google Scholar]
- Tucker, L.R. Some mathematical notes on three-mode factor analysis. Psychometrika 1966, 31, 279–311. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Wang, W.; Liu, J.; Liu, Q.; Wang, B. Tensor-based real-valued subspace approach for angle estimation in bistatic MIMO radar with unknown mutual coupling. Signal Process. 2015, 116, 152–158. [Google Scholar] [CrossRef]
- Liu, X.; Liao, G. Direction finding and mutual coupling estimation for bistatic MIMO radar. Signal Process. 2012, 92, 517–522. [Google Scholar] [CrossRef]


| Notation | Definition |
|---|---|
| (bold Euler script letter) | tensor |
| (bold capital letter) | matrix |
| (bold lowercase letter) | vector |
| ∘ | Hadamard product |
| ⊗ | Kronecker product |
| ⊙ | Khatri-Rao product |
| identity matrix | |
| zero matrix | |
| conjugate of matrix | |
| transpose of matrix | |
| conjugation-transpose of matrix | |
| diagonalization of matrix | |
| extract phase | |
| matrix set |
| Method | Computation Complexity |
|---|---|
| Proposed | |
| ESPRIT | |
| MUSIC | |
| Tensor-ESPRIT |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, T.; Wang, X.; Huang, M.; Lan, X.; Sun, L. Tensor-Based Reduced-Dimension MUSIC Method for Parameter Estimation in Monostatic FDA-MIMO Radar. Remote Sens. 2021, 13, 3772. https://doi.org/10.3390/rs13183772
Xu T, Wang X, Huang M, Lan X, Sun L. Tensor-Based Reduced-Dimension MUSIC Method for Parameter Estimation in Monostatic FDA-MIMO Radar. Remote Sensing. 2021; 13(18):3772. https://doi.org/10.3390/rs13183772
Chicago/Turabian StyleXu, Tengxian, Xianpeng Wang, Mengxing Huang, Xiang Lan, and Lu Sun. 2021. "Tensor-Based Reduced-Dimension MUSIC Method for Parameter Estimation in Monostatic FDA-MIMO Radar" Remote Sensing 13, no. 18: 3772. https://doi.org/10.3390/rs13183772
APA StyleXu, T., Wang, X., Huang, M., Lan, X., & Sun, L. (2021). Tensor-Based Reduced-Dimension MUSIC Method for Parameter Estimation in Monostatic FDA-MIMO Radar. Remote Sensing, 13(18), 3772. https://doi.org/10.3390/rs13183772







